(source)
(source)
|
Sir George Biddell Airy
(27 Jul 1801 - 2 Jan 1892)
English astronomer and mathematician who was the seventh Astronomer Royal. He made a mathematical study of the rainbow, researched optical interference fringes. Airy computed the density of the Earth by swinging a pendulum at a deep mine. He determined the mass of Jupiter, its rotational period and orbits of comets.
|
LECTURE ON THE
PENDULUM-EXPERIMENTS
AT HARTON PIT,
DELIVERED IN THE CENTRAL HALL, SOUTH SHIELDS,
OCTOBER 24, 1854.
By GEORGE BIDDELL AIRY, Esq.,
ASTRONOMER ROYAL.
TOGETHER WITH
A LETTER CONTAINING THE RESULTS OF THE EXPERIMENTS.
INTRODUCTION.
[p.iii] THE Astronomer Royal, at the request of a deputation of gentlemen of South Shields, wrote in extenso, from memory, the following Lecture which he had delivered, orally, upon the 24th October, in the Central Hall of that place. The abstruse subject was so simplified, and the steps of the observations were so lucidly explained, that a crowded audience, assembled from various towns of the district, carried from the Hall a clear understanding of the process and object; and were inspired by one common wish that the Lecturer might have his labours, his energies, and genius, crowned with complete success.
South Shields and the coal districts of the North have reason to congratulate themselves that, amid the bustle of their commercial and shipping operations, and the gigantic developments of their mining wonders, Science, which has ever been the handmaid of their many successes, has, in this instance, peculiarly graced them with its presence.
To turn so simple an instrument as the pendulum into the means of ascertaining a chief property of those heavenly bodies, far and near, moved and acted on by the same mysterious influences as our Earth, is indeed an exercise of human thought that allies man to the Great Source of Intelligence.
The experiments with this instrument, at Harton Pit, by the Astronomer Royal, were of a different nature from those made by Kater, Sabine, Foster, and others. The former were intended to discover the exact number of vibrations of the invariable pendulum upon the surface, and at the lowest ascertainable depth below it. The latter to ascertain the actual length of the seconds pendulum, or the number of vibrations at different places of the Earth’s surface. One to discover the absolute density of the Earth; the other its form, and the relative position of its parts.
[p.iv] Since the time of the discovery, by Galileo, that the vibrations of the pendulum are nearly equal in time whatever the extent of the arc,— its application to clocks in 1649 by his son, Vincent Galileo, at Venice, and its successful development by Christian Huyghens, this little instrument, based on a great law of the Universe, has been growing in importance and value, till now, in its employment by the Astronomer Royal in the South Shields experiments, it has achieved new and unexpected results.
The careful perusal of the Lecture will shew the reader how, in the hands of Science and indomitable energy, results the most gigantic and absorbing may be wrought out by skilful combinations of acknowledged data and the simplest means.
Harton Pit, the site of the pendulum experiments detailed in the Lecture, is within two miles of South Shields, in a S.S.W. direction. It is the property of Messrs. Blackett, Anderson, Wood, & Philipson, whose liberal conduct in this matter has been so justly praised by Professor Airy. Commerce and Trade honor themselves, when, in their exciting career, they stop and hold forth a frank and generous hand to that which elevates and ennobles our race.
This Pit is 1260 feet deep, and has been connected in its workings below with those of St. Hilda Pit, placed within the precincts of the town of South Shields. The subterraneous passages of these mines extend to upwards of 80 miles; some of them more than three miles in a direct line. The shaft at Harton Pit is descended and ascended by cages and tubs, conducted by slides or guides,—a sort of vertical railway,—by which the descent or ascent is regularly made in about one minute. As the Pit was to be descended and ascended many times a day by the Astronomical Observers, this arrangement afforded facilities for observation and for the conveyance of the instruments, which were important elements of the experiment.
During the entire three weeks that the observations continued, all went on smoothly and well; but on the day succeeding their termination an accident occurred in the shaft that, previously, would have interrupted their advance and continuity, so necessary to their success. From the first to the last, however, nothing in this great experiment has occurred to mar the progress and exactitude of the operations. It is now most gratifying to be able to point to the letter of the Astronomer Royal, at the conclusion of the Lecture, for their results.
The public cannot but rejoice at this fresh achievement of Science, by [p.v] which the important question of the real density of the Earth has been at length solved: through which the distant revolving Worlds, in their beautiful and regulated flights, are to be weighed,—and so another clear advance obtained in the progress of knowledge of the Great Works of the Creator.
All that now remains to be done is to ascertain, through the various deposits, the average weight of the shell, 1260 feet thick, between the bottom of Harton Pit and the surface of the Earth, and a few miles of surface-levels. Men of science, in all parts of the world, who know its value, will receive the intimation of the Astronomer Royal, contained in his letter, with the highest satisfaction.
South Shields, January 1st, 1855.
LECTURE.
[p.1] THE Astronomer Royal,—after adverting to the liberality “with which his application for permission to use the Harton Colliery, as a locality for his pendulum-observations, had been received by Mr. Anderson and the other proprietors of the colliery, and acknowledging the cordial attention with which every request had been almost anticipated by the proprietors, by the superintendent of the mine, Mr. Arkley, and by every person attached to the mine,—proceeded to make three remarks preliminary to the main subject of the lecture. The first was, that the experiments are in no ordinary degree connected with the county of Durham; not only because they were made within the county, but also because three of the six gentlemen who co-operated in the actual conduct of the experiments are, or have been, in some way connected with the Durham Observatory. The second remark was, that at the present time it is impossible to say whether the experiments are or are not successful. The looseness of a single screw, which could scarcely be detected by the sight or touch, would be sufficient to spoil all. The observations, however, have been so framed as to check themselves; and when the extensive calculations which are necessary to lead to a result shall be finished, it will be possible to pronounce positively on the value of the [p.2] experiments: in the mean time, the examination of the instruments gives every hope of good success. The third remark was, that even if the operation were perfectly successful, it would lead in the first instance to no very striking result. The audience would in vain expect from it, for instance, such speculations as those in “The Plurality of Worlds:” yet he trusted it would appear, in the course of the lecture, that the conclusions which the experiments are intended to furnish are such as serve for the foundation to speculations similar to those to which he had alluded, and, in fact, that they are indispensable for the establishment of those higher theories. He might consider that he stood in the same relation to the philosopher who employed himself in the more elevated parts of science, in which the quarryman stands to the architect; and if it should be found that he had cut out a sound corner-stone for the Temple of Science, he should be well satisfied with his success.
Proceeding now to the main subject of the lecture,—the ultimate purpose of the experiments is to give the means of weighing, by the use of a pound weight,’ not only the Earth, but also the Sun, Jupiter, and all the principal bodies of the Solar System. It is quite evident that this can be no simple operation; it requires the connexion of a long series of discoveries in theory and in observation, made by the efforts of mighty intellects in different ages, and hanging together like the links of a chain; one of the links, however, which is absolutely essential to the completeness of the chain, consisting in experiments equivalent to those now to be described. It is convenient to explain—(I.) The relation between the masses of the different bodies of the solar system and the mass of the earth. (II.) The nature of the methods which have been heretofore used for finding the mass of the earth, and the nature of the method now proposed for that [p.3] purpose. (III.) The details of the apparatus used, and of the method of using it.
(I.) It is impossible in the course of one or of several lectures, even if expressly devoted to the purpose, to explain the methods by which the dimensions of the orbits of the planets and satellites of the solar system are ascertained. It must be taken for granted that all are known with considerable accuracy, and some with extreme exactness. Nor is it possible in any short time to explain the wonderful series of reasonings upon which the theory of gravitation is established, although its rules, as required in reference to the present experiments, can be easily stated. Consider, then, the curved motion of a planet in its orbit. The fact that a planet has been moving in a curve up to a certain moment does not at all account for its moving in a curve after that moment. One of the laws of motion (which were established successively by Galileo and Newton) is, that, however the motion of a body may have been acquired, it will, when in motion, continue to move with uniform velocity in a straight line, till it is disturbed by some external force. A familiar instance in which the line of subsequent motion is evidently independent of the curve of previous motion, is that of the motion of a stone thrown from a sling: although the stone has been whirled round and round in a circle, it has no tendency when set free to continue to move in that circle, but it goes off in the straight line whose direction is that of the portion of the circle in which it happens to be when it is set free. In like manner, a planet may be conceived at any moment to be free to move on in a straight line whose direction is the same as the direction of the curve in which it is moving at that particular moment; and, if nothing disturbed it, it would move on in a straight line. And what then makes it move in a curve? It is the attraction of the [p.4] central body. In the instance of a planet moving round the sun, the central body is the sun, and the force which compels the planet to abandon the straight line, in which it would otherwise move, is the attraction of the sun. In the instance of a satellite moving round a planet, the force which disturbs the satellite from the straight line is the attraction of the planet. Now if, from the known dimensions of the orbit and the known periodic time, we compute how far the planet or satellite would have moved in any short time, (say an hour;) and if then, by the rules of geometry, we compute the distance between the point of the curve which the planet reaches in an hour, and the corresponding point in the straight line which it would have reached in an hour if no disturbing force had acted on it; then it is clear that the distance of the place where the planet is from the place where it would have been is the effect of the disturbing force, and is therefore a proportionate measure of that force. And by computing the lengths of these distances, in different orbits, we can find the proportions, for instance, of the force with which the earth draws the moon, the force with which Jupiter draws one of his satellites, the force with which the sun draws the earth,—and so on.
But when we have made this calculation, the thing which we have found is, the proportion of the forces exerted by the attracting bodies at the distances at which the attracted bodies happen to be in the circumstances for which the calculation is made. For instance, we have thus found the proportion of the sun’s attraction on the earth, (the earth being at a great distance from the sun,) to Jupiter’s attraction on his first satellite, (the satellite being at a small distance from Jupiter.) But we want to find what would be the proportion of attraction if the distances were equal. For this purpose we must avail ourselves of that part of the [p.5] law of gravitation which, asserts that the force of attraction to any attracting body is inversely as the square of the distance of the body which is attracted. Thus, if Jupiter’s first satellite were removed to double its present distance from Jupiter, the attraction of Jupiter upon it would be only one-fourth of Jupiter’s present attraction upon it:—if the satellite were removed to ten times its present distance, Jupiter’s attraction upon it would be only one-hundredth part of Jupiter’s present attraction. By use of this rule, having found the attraction of Jupiter upon his satellite in its present state, we can at once find what would be the attraction of Jupiter upon the satellite, if the satellite were as far from Jupiter as the earth is from the sun; and, comparing this with the sun’s attraction upon the earth, we find the proportion of the attraction of the sun upon a foreign body to the attraction of Jupiter upon a foreign body when the circumstances of distance arc the same.
But these are attractions, not masses. What we want, however, in the present inquiry, is the proportion of masses. For this we must refer to another part of the law of gravitation; namely, that attractions (as measured by the space through which attracted bodies are pulled from the straight line in which they would otherwise move) do not depend in any degree upon the masses of the attracted bodies, but are exactly proportional to the masses of the attracting bodies. This has been the subject of most careful experimental investigation by Newton and Bessel. It is a point in which the attraction of gravitation differs strikingly from other less important or less universal attractions, as, for instance, that of magnetism. The proportion of the magnetic attractions of two magnets is not the same as the proportion of their masses. Availing ourselves then of this principle as applies to gravitation, and having found the proportion of the [p.6] attraction of the sun on a foreign body to the attraction of Jupiter on a foreign body at the same distance, we say at once that we have found the proportion of the mass of the sun to the mass of Jupiter.
In this way we may find the proportions of the masses of all the bodies which have planets or satellites revolving about them; and we can at once refer these to the mass of the earth, either by applying computations to the motion of our moon similar to those which have been applied to Jupiter’s satellite, or by considering how far a stone falls in a short time—that is, how far the earth attracts a stone in a short time when the place of attraction is very near the earth’s surface. The results obtained by the two methods are exactly the same. For those planets which have no satellites, other methods must be used which it is impossible now to explain. It is sufficient to state that in those cases as well as the others, the character of the results at which we arrive is the same: in all cases we find the proportion of the mass of the sun or planet to the mass of the earth. And therefore, in order to find the real mass of the sun or planet, it is necessary for us to find the real mass of the earth.
(II.) Our actual acquaintance with the material of the earth is limited to a very small thickness of its external coat. And so great has been the obscurity with regard to its internal constitution, that a philosopher of some eminence in the present century actually conceived that the earth must be a hollow globe with a thin crust or shell, its inside being filled with light. It seems a much more rational idea to imagine that, as the inner parts are pressed by the weight of the outer, the inside is more dense than the rocks near the surface. To remove this doubt has been the object of some important experiments.
[p.7] The first of these “was the celebrated Schehallien experiment, undertaken in the last century at the expense of George the Third, by Dr. Maskelyne, then Astronomer Royal. The immediate object of this experiment was, to find the position to which a plumb-line would be drawn by the attraction of the mountain. It is easily seen that the knowledge of the angle through which the plumb-line is drawn would give information as to the quantity of the mountain’s attraction. If the attraction of the mountain sideways were just as great as the attraction of the earth downwards, the plumb-line would be pulled into a position making half a right angle with its ordinary position; and, therefore, if we found the plumb-line pulled aside through half a right angle, we should infer that the mountain’s attraction at that place was equal to the earth’s attraction. If it were less inclined, we should infer that the mountain’s attraction was less, according to rules easily derived from the theories of mechanics. It is now necessary to explain how the difference of position of the plumb-line at two places can be found. The instrument most convenient for this purpose is the zenith sector. A telescope has two pivots near to its object glass, and upon these it can swing like a pendulum. Near to its eye-end there is fixed upon it an arc (a part of a circle) divided into degrees, &c. The telescope is usually pointed almost perpendicularly upwards, so that if a plumb-line is hung over the end of one of the upper pivots, it passes over some part of the arc. Suppose, now, that this telescope, at whatever station it may be used, is always pointed to the same star, when that star is passing the meridian. It is quite certain, then, that the telescope is always pointed in one definite direction, and therefore if, on so using the instrument at two places, the plumb-line passes over different parts of the graduated arc, we can find exactly [p.8] how much the positions of the plumb-line differ at the two places. Now the mountain Schehallien is a steep ridge running nearly east and west. Two stations were selected on the north and south slopes, very nearly in the same meridian; and the zenith sector was used at those two stations to find the difference of positions of the plumb-line, in the manner just described. But how does this show the angle through which the attraction of the mountain disturbed the plumb-line? It is thus. A most careful survey was carried over the mountain, by which the distance between the two stations was found in feet. Now we know the dimensions of the earth so well that, having the distance between the two stations given in feet, we can infer with great accuracy how much the two plumb-lines at those two points would be inclined if there were no disturbing mountain between them. The scale is, (with considerable exactness) one second of inclination of the plumb-line for every 100 feet of distance. In this manner it was found from the survey that the inclination of the two plumb-lines, if there had been no mountain between them, would have been 43 seconds. But it was found from the observations of a star with the zenith sector that the inclination of the plumb-lines really was 54½ seconds: the difference, 11½ seconds, was the effect of the mountain’s attraction on the plumb-lines. The next thing was, to measure most carefully every part of the mountain, and to compute, on the law of gravitation, what would be its attraction (as compared to the earth’s), and what inclination of the plumb-line it would produce, supposing its density to be the same as the earth’s general density. This computed inclination was 20¾ seconds. Consequently the earth’s general density is greater than the mountain’s density in the proportion of 20¾ to 11½. And, as the mountain’s density was found to be about 2¾ times [p.9] that of water, it followed that the earth’s general density is something less than five times that of water.
This must be regarded as a most interesting and noble experiment. The Astronomer Royal, however, stated that, having himself examined the mountain, he distrusted the accuracy of the inference. The mountain is nearly surrounded by other mountains, of which one (Ben Lawers) is much higher than itself; and the geology of the country is complicated. Though the result of 11½ seconds’ disturbance is certain, yet the computation of the mountain’s attraction is by no means certain.
The second experiment was that known as the Cavendish experiment. It was first proposed by the Rev. J. Mitchell, of Cambridge; and it was tried successively by Mr. Cavendish, Mr. Reich (of Freyburg,) and more lately by Mr. F. Baily. A horizontal rod, carrying two small balls, is suspended at its middle, in some cases by a single wire, in others by two wires. Two large leaden balls are carried by a turning frame, in such a manner that they can either be brought near to the sides of the small balls or can be moved far away. When the leaden balls are brought near, they attract the small balls; and when the angle of disturbance of the small balls is observed (by telescopes,) then, by a complicated calculation, the actual proportion of the leaden balls’ attraction to the earth’s attraction can be found from it. By another calculation, we can find “what would have been the proportion of the balls’ attraction to the earth’s attraction, if, instead of being made of lead, they had been as dense as the earth generally. The proportion of these numbers informs us at once what is the density of the earth generally. It was found to be more than 5½ times as dense as water. The only failing in this experiment is the difficulty of guarding against disturbance. It was necessary to inclose [p.10] the small balls in a double or treble box,—and an imperceptible current of air in that box made the experiment useless. If a halfpenny, slightly heated, was laid on the box, it produced currents of air, which gave to the small balls a greater motion than the attraction of the great balls would produce. It was difficult to avoid suspecting that the temperature of the great balls might sometimes produce a part of the effect which was ascribed to their attraction.
This, the Astronomer Royal stated, was the position of the question (except that Reich and Baily had not then repeated Cavendish’s experiment,) when, in 1826, he proposed to his friend Mr. Whewell (now Dr. Whewell, Master of Trinity College, Cambridge,) to join him in trying experiments with pendulums, for ascertaining the variation of gravity in descending to the bottom of a deep mine. Before alluding further to the particular experiments, it will be advantageous to point out, first, what is the relation between the movements of a pendulum and the force of gravity; secondly, how the observation of the variation of gravity in a deep mine may be made subservient to a determination of the earth’s density.
When a pendulum is drawn away sideways from its vertical position, so that its length is inclined to the horizon, the force which makes it return to its vertical position is the force of gravity, or the attraction of the earth pulling the bob downwards. But when the bob is thus brought to the vertical position it is not then in a state of rest; it is moving with considerable velocity, which carries it to the other side of the vertical position, with its length inclined the opposite way to the horizon; and then the pull of the earth’s attraction first retards it, after a time brings it to momentary rest, and subsequently pulls it back again to pass the vertical position the opposite way,—and so on. When [p.11] there is no cause of resistance, the motion goes on for an indefinitely long time. Pendulums with the delicate mounting shortly to be described, even if vibrating in the air, would vibrate in an observable arc for six or eight hours; but if they were vibrating in a place from which the air had been extracted, their vibrations would be perfectly observable at the end of twenty-four hours. Now, suppose that we have a string fastened to the bob of the pendulum, and that when the pendulum is in the inclined position we pull the bob downwards by the string. It is obvious that the pendulum will reach the vertical position more quickly than if it had not been pulled. Suppose the pull continued after it has passed the vertical position; the pendulum will then be retarded and brought to a momentary state of rest more quickly than if it had not been pulled; and the motion in the opposite direction will be restored more quickly than if it had not been pulled. But the pull of which we have spoken is exactly the same thing as an increase of the force of gravity. Thus it appears that an increase of the force of gravity causes every vibration of the pendulum to be completed in a shorter time than if gravity were not increased. And, conversely, if we find that from descending a mine, or any other cause, the vibrations of a pendulum are accelerated, we can at once infer that the force of gravity is greater there. If the number of vibrations in a day were doubled there, it would show that gravity is there four times as great; and, as a general rule, the proportion of the increase of gravity is as the square of the number of vibrations.
Now, to show how this can be used to determine the earth’s density;—conceive the earth to be spherical; and conceive a spherical surface whose centre coincides with the earth’s centre, to pass through the lower station at the bottom of the mine, dividing the earth into two parts, [p.12] namely, an inner sphere, and an outer shell or rind whose thickness is every where equal to the depth of the mine. (It is not meant that this represents exactly the state of things, because the earth’s surface is irregular; but it serves to give the first notions of the principle of calculation.) Then these two results of mathematical investigation must be taken for granted. First, that when an attracted body (for instance, the bob of a pendulum) is outside of a sphere or a spherical shell, the attraction of the matter of that sphere or spherical shell upon the attracted body is exactly the same as if all the matter were collected at the centre; and, for different distances of the attracted body, the attraction is inversely proportional to the square of its distance from the centre. Secondly, that if an attracted body is inside of a spherical shell, the attractions of the different parts of that spherical shell exactly neutralize each other, and on the whole it produces no effect at all. Now then, to fix our ideas, suppose that the semidiameter of the inner sphere is 4000 miles, and suppose the depth of the mine to be a quarter of a mile, so that the semidiameter of the outer sphere is 4000¼ miles. At the bottom of the mine, the attraction of the shell (as has been mentioned) is nothing, and the gravity there is produced only by the inner sphere. At the top of the mine, which is more distant from the centre by 1/16000 part of the whole, the attraction of the inner sphere (by the rule of inverse proportion to the square of the distance) would be lessened by 1/8000 part of the whole, very nearly. But suppose that, upon trying our pendulums, we find that the attraction is lessened at the top, but only by 1/24000 part of the whole. How is the difference between these two, or the 1/12000 part of the whole, to be accounted for? Why, it is produced by the attraction of the shell. And thus we find that the attraction of the shell (which is the [p.13] same as if all the matter of the shell were collected at the centre) is 1/12000 part of the attraction of the inner sphere, and, therefore, the quantity of matter in the shell is 1/12000 part, or 4/48000 part, of the matter in the sphere. But the bulk, or solid content of the shell, is 3/16000 part, or 9/48000 part, of the solid content of the sphere. Therefore, the density of matter in the shell is to the density of matter in the sphere as 4 to 9. And now comes in the application of the pound weight. We can take specimens of all the various beds through which the shaft of the mine passes, and can weigh them, and thus we can find the density of matter in the shell. And, therefore, we can at once find, from the proportion just obtained, what is the density of the matter in the sphere. The numbers which are used above are imaginary, but probably they are not very far from the truth.
The preceding reasoning shews that the observations proposed do give the means of attaining the desired result. But every observation is liable to error; and it is desirable to ascertain what influence the inevitable errors will produce on the result. Now, it has been stated by other observers that, by contrivances hereafter to be described, the number of vibrations made by a pendulum in 24 hours may be determined with no greater error than 1/10 of a vibration; and the Astronomer Royal is inclined to think that, if the instruments are not injured, an accuracy even greater than this may be obtained. This error corresponds to an error of 1/432000 in the determination of the force of gravity. The error which this would produce in the determination of the earth’s density is only 1/36 of the whole; an error below those to which the two preceding methods may be considered liable. There was, therefore, good reason for undertaking the experiment in this new form.
[p.14] Accordingly in 1826 Mr. Whewell and Mr. Airy made a series of experiments in the Dolcoath mine, near Camborne in Cornwall, which probably was, at that time, the most favorable of all the English mines for such an experiment. The labour of the experiment was excessively great, (principally because the ascent and descent are effected entirely by ladders); but the most serious annoyance was the difficulty of comparing the upper and lower clocks, (the necessity for which will be explained hereafter) by carrying chronometers attached to a man’s body. At length, when certain progress had been made, and instruments were to be raised to the surface, the packing (by some unknown means) took fire, and partly by burning, and partly by falling, the essential instruments were destroyed. And thus the labour of 1826 was entirely lost.
In 1828, the same persons, with the assistance of several friends, again undertook the experiment, in the same place. Several difficulties were now overcome; but the pendulums themselves changed their forms. Means were devised for obviating the effects of this change, when there occurred in the mine a “fall,” or slip of the solid rock, affecting the ground through a large portion of a square mile. The machinery of the pumps was stopped, and the party were finally drowned out of their lower station. The results, as far as any were obtained, were considered so unsatisfactory as to be unworthy of publication.
It will readily be seen, from these instances, that in Science, not less than in War or Commerce, pertinacity of purpose is one essential element of success. The intention to follow up the proposed scheme of observations was never laid aside; and three circumstances contributed to direct the Astronomer Royal’s attention to the matter more distinctly in late years. The first was, that one of the elements of [p.15] reduction (that depending on the weight of the atmosphere) was made, by Colonel Sabine’s experiments, more accurate than it formerly was. The second, that the depth of the coal mines was increased; and several circumstances in the coal mines (the facility of access, the regularity of the geological arrangement of strata, our intimate knowledge of the strata, &c.) render them preferable to any other if sufficient depth can be obtained. The third, that the introduction into the Royal Observatory of a galvanic system of observation had given to the Astronomer Royal and his assistants a practical command of the methods of galvanic communication for the comparison of clocks, which would remove one of the most annoying difficulties. These considerations induced the Astronomer Royal in the past summer to visit the coal-field of North Durham. The welcome which he received from Mr. Anderson, and the desire to meet all his wishes shewn by the able viewer of the mine, Mr. Arkley, satisfied him that now, if ever, there was a good prospect of carrying out the work successfully. With Mr. Arkley’s assistance, two stations were selected, the upper one a part of a stable near the Mine Office, the lower one exactly under the upper station, but about 1260 feet below it. In the upper station, the soft ground was removed to a considerable depth, and the space was filled with square stones grouted together, and finally paved with good flags, to give a solid bearing to the apparatus; the walls and roof were doubled, and the space between them filled with non-conducting materials, to prevent rapid changes of temperature; and gas was laid on to a gas stove, to give the observers some command of the temperature. In the lower station, the floor of solid rock was cut level and paved; brick walls were erected, and a ceiling put over them; and a most comfortable suite of three rooms was made.
[p.16] III. Before describing the apparatus, the principle of the method used for counting the vibrations of a detached pendulum may be explained. Our object is, to ascertain how many vibrations per day will be made by a pendulum which is absolutely unaffected by any force except that of gravity. A clock pendulum will not suffice for this purpose; the train of wheels in the clock presses on the clock pendulum with a small force, which effectually disturbs its vibrations from their natural state: the pendulum for our experiments must be absolutely untouched. But how can its vibrations be counted, especially as it will continue to vibrate only for a few hours? It is done thus. The free pendulum, upon its own stand, is placed in front of a clock. On the bob of the clock pendulum is fixed a small inclined disk covered with gold leaf, which is illuminated by a lamp. A small telescope is fixed at the distance of eight or nine feet, for viewing the pendulums. The free pendulum has a tail projecting below its bob, expressly for the purpose of hanging in front of the disk of the clock pendulum. When the two pendulums are hanging in their vertical positions, and the observer looks through the telescope, the tail of the free pendulum covers the disk of the clock pendulum. Now, while the free pendulum is at rest, if the clock pendulum were made to vibrate, its disk would be seen on both sides of the pendulum tail. A pair of cheeks is placed between the two pendulums, having an opening between them which admits of adjustment. While the free pendulum is still at rest, the cheeks are moved towards the centre, till the vibrating disk cannot be seen past the edges of the pendulum tail; and then the apparatus is ready for use. For, suppose the pendulums to be both vibrating: if they do not pass the vertical positions at the same time, the pendulum tail does not cover the opening between the adjustable cheeks at the instant when [p.17]the disk is passing, and the disk is seen by the observer. But if they do pass the vertical positions at the same time, the pendulum tail covers the opening exactly when the disk is passing, and the disk cannot be seen at all. This marks what is called a Coincidence. Suppose now that each vibration of the free pendulum occupies a rather longer time than a vibration of the clock pendulum. Perhaps when they are started they do not pass the vertical position at the same time: but the clock pendulum gains a little at every swing, and at length they will pass the vertical position at the same time, or there will be a Coincidence, (observed, as is mentioned above, by the disappearance of the disk.) Then the clock pendulum will advance on the free pendulum, and more and more of the disk will be seen. This will continue till the clock pendulum has gained exactly two swings, or one double swing (backwards and forwards), upon the free pendulum, and then there will be another Coincidence, to be observed in the same way. If then we observe these two Coincidences, and note the interval between them as shewn by the clock face, and the corresponding number of swings of the clock pendulum, we know that the number of swings of the free pendulum in the same time is exactly that number of swings of the clock pendulum diminished by 2. And thus we can find the proportion of the number of swings of the free pendulum to the number of swings of the clock pendulum. And the clock counts the swings of its own pendulum during a day, or during any other time that we have occasion to use; and therefore, by the proportion, the number of swings of the free pendulum in the same time can be found. The peculiar advantages of this method are: first, that the counting is done entirely by the clock; secondly, that though the observation is very coarse, the result is exceedingly accurate. Suppose that the interval [p.18] of Coincidence is about 400 seconds of time; this implies that in 400s the free pendulum loses two vibrations, and therefore in every vibration it loses 1/200 part of a vibration. And therefore, if an error of 1s is committed in observing the time of coincidence, the error really committed with regard to the pendulum-movement is only 1/200 of a vibration.
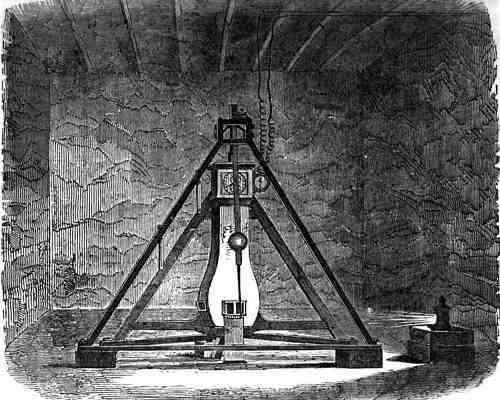
This method being understood, the nature of the apparatus will be easily seen. The stand which carries the free pendulum is a kind of iron tripod, made of very strong bars, and so shaped that the clock, mounted on its proper stand, can be placed under the iron stand, and at a short distance behind the free pendulum. The pendulum is a brass bar with brass bob; and near its upper end is fixed a prism, of the hardest steel that can be procured, with one edge very carefully ground to a somewhat obtuse angle. This is technically called a “knife-edge.” The knife-edge passes through the bar and projects on both sides. (There is exactly the same arrangement in any ordinary good scale-beam.) On the top of the iron stand there are mounted two level plates of agate; the knife-edges rock upon these, (as in a bullion-balance,) and the pendulum hangs between them. These are the essential parts of the apparatus.
There are, however, some minor parts, which are by no means unimportant. There is a graduated arc to measure the extent of the pendulum’s swing. The time of vibration of a pendulum is nearly, but not exactly, the same in a large arc as in a small one: but when we know the length of the arc, we can compute the correction that is to be applied in order to find what would have been the number of vibrations if the arc had been exceedingly small. There are two thermometers suspended near to the pendulum, to inform us what is its temperature. The length of a pendulum changes with different degrees of heat, (in fact, a single [p.19] degree of the ordinary Fahrenheit’s thermometer produces a change of half a vibration per day in a brass pendulum,) and it is necessary to apply the proper correction in order to find what would have been the number of vibrations if the pendulum had been at a certain degree of heat, say 50° of the thermometer. A barometer is near the pendulum; it gives information on the density of the air, which is required for the following reason. Every one knows that a piece of brass feels lighter in water than out of water, being partly supported by the “buoyancy.” Now air produces its “buoyancy,” in a degree corresponding to its density; and, the pull of gravity upon the pendulum being thus diminished, its vibrations are not performed so quickly as they would otherwise have been. A correction is therefore necessary, depending on the density of the air as shown by the barometer, to increase the number of vibrations to what they would have been if made in a place from which the air is exhausted. Another correction, depending also on the density of the air, is necessary for this reason. A pendulum is encumbered in its motion by a certain quantity of air sticking to it, which does not add to the force of gravity on it, but which does add to the mass of matter that has to be put in motion at every swing, and which therefore makes the vibrations slower than they would otherwise be: the correction for this cause depends also on the density as shewn by the barometer.—The whole of this apparatus is inclosed in a room, which the observer does not enter while the vibrations are going on. The viewing telescope is fixed in a hole in the wall.
It will be remarked that the first result of the observation of Coincidences is to give the proportion of the free-pendulum swings under the actual circumstances to the clock-pendulum swings under the actual circumstances: but the [p.20] effect of applying all the corrections is, to give the proportion of free-pendulum swings under the imaginary circumstances of “very small arc,” “standard temperature,” “vibrations in a vacuum,” to the clock-pendulum swings under the actual circumstances.
Such an apparatus was used at the upper station, and a similar apparatus at the lower station, at the same time. Corrections computed on similar principles are to be applied to the observations at both stations; and thus we can find the proportion of the vibrations of the upper free-pendulum under the imaginary circumstances to the vibrations of the upper clock-pendulum under the actual circumstances; and the proportion of the vibrations of the lower free-pendulum under the imaginary circumstances to the vibrations of the lower clock-pendulum under the actual circumstances,—at the same time.
But we want to find the proportion of the vibrations of the upper free-pendnlum under the imaginary circumstances to the vibrations of the lower free-pendulum under the imaginary circumstances.
It is evident that what is above stated is not sufficient for this purpose. We want one thing more, namely, the proportion of the vibrations of the upper clock-pendulum to the vibrations of the lower clock-pendulum. This will be found if at the beginning of any long period (as a day or a portion of a day) we can find what is the hour, minute, second, and fraction of a second on one clock, and what is the hour, minute, &c, on the other clock, at one and the same instant; and if also, at the end of the same long period, we can in like manner find the readings of the clocks corresponding to one and the same instant. For then we have the same absolute extent of time measured by means of the numbers of the vibrations of the pendulums of the two clocks.
[p.21] These corresponding times on the two clocks are found by simultaneous galvanic signals. In the upper station there was a battery of 24 cells; the current passed through two springs in an auxiliary time-piece, which were forced into contact by pins on one of the time-piece wheels, at every 15 swings of its pendulum. The wire from the spring passed through the coils of a common telegraph needle which was fixed to the side of the upper clock; thence it was led to the mine shaft, and down the shaft, (being partially supported at every 100 feet on pegs fixed in the brattice,) and to the lower station, where it passed through the coils of a similar telegraph needle fixed to the side of the lower clock; and then it was returned up the shaft to the other pole of the battery. Thus at every fifteenth swing of the time-piece pendulum there were simultaneous starts of the upper needle and of the lower needle; and the upper and lower observers noted the times shewn by their respective clocks when these signals occurred. To each telegraph needle was attached a small apparatus, by the use of which either of the observers could at pleasure interrupt the circuit, and thereby stop the signals at both stations. A very simple code was established which enabled each observer, using this apparatus, to ascertain that the other observer was attending to the signals; and which also enabled them to identify the observations of signals at one station corresponding to definite observations at the other station.
This, then, supplied the means of comparing the clocks; and we had now the means of ascertaining the proportion of the number of vibrations of the upper pendulum (which we will call A) under the imaginary circumstances, to the number of vibrations of the lower pendulum (which we will call B) under the imaginary circumstances. Still we have not obtained the result which we require. In [p.22] order to determine the variation of gravity we must find the proportion of the number of vibrations of A above to the number of vibrations of A below in the same time. As we cannot have A above and A below at the same time, we are compelled to have recourse to an indirect process. The method which has been adopted in these experiments is the following. In the manner described above, the proportion of the number of vibrations of A above to those of B below was found. When this operation was completed, the pendulums were interchanged; A was carried to the lower station and B was brought to the upper station; and the proportion of the number of vibrations of B above to those of A below was found. Now if there was any difference between the two pendulums, so that (for instance) under similar circumstances the number of vibrations of B would be greater than those of A; then, in the first part of the operation, we should get too many vibrations at the lower station, and in the second part we should get too many at the upper station, or (which amounts to the same thing) too few at the lower station; and the mean between the two results is the true proportion of the vibrations of A above to A below, or of B above to B below, freed from all effects of the difference between the two pendulums.
The actual operation was thus conducted. With A above and B below, twenty-six series of vibrations were observed at each station, each series occupying four hours; so that at each station there were observations going on incessantly for 104 hours, day and night. During this time two observers were constantly occupied, one above and one below; each observer took a turn of twelve hours. The galvanic signals were observed before the beginning and after the end, and also at the end of every four-hour series. Then the [p.23] pendulums were interchanged; and with the pendulums were changed the agate planes upon which they vibrate, and the thermometers which shew their temperature. It had been proposed to interchange also the iron stands; but upon considering the extreme firmness of their construction it was judged that nothing would be gained by this change, and that there would be risk of disturbance to the solidity of their connexion,—and this idea was given up. These observations were made for 104 hours with B above and A below. The pendulums were again interchanged, and were observed for 60 hours with A above and B below. They were interchanged a third time, and were observed for 60 hours with B above and A below; and this completed the operation. The object of the third and fourth parts of the work was to ascertain whether either or both of the pendulums had changed after the first part; and until the results of the third and fourth parts have been computed and compared with those of the first and second, it will be impossible to say whether the condition of the pendulums has been so invariable as to make the results quite trustworthy.
The observations of every kind, the reversions of the pendulums, &c, have been made under the immediate superintendence of Mr. Edwin Dunkin, one of the Assistants of the Royal Observatory, Greenwich. The other observers were, Mr. Ellis, (of the Royal Observatory,) Mr. Pogson, (of the Oxford Observatory,) Mr. Rümker, (of the Durham Observatory,) Mr. Criswick, (of the Cambridge Observatory,) and Mr. Simmonds, (of the Red Hill Observatory.) A finer set of observers was never collected; and, should the experiment be successful, the success must be ascribed to the unwearied and well-directed exertions of these gentlemen. Under the vigilant care of Mr. Dunkin, and the [p.24] zealous efforts of all the members of the party, the operations of every kind went on with the greatest regularity and accuracy; and perhaps it is not too much to say that no work of observation, requiring the co-operation of numerous observers, has ever been effected in a more systematic manner. At all events the Astronomer Royal considers that the result of these experiments will decide on the applicability of the pendulum to this purpose; if it fails now, there is no hope that it can ever be used successfully again.
The Astronomer Royal expressed his gratification that he had been able, in thus meeting so large an assembly of the principal inhabitants of South Shields and its neighbourhood, to offer a slight acknowledgment of the courtesy which himself, and all the members of his party, had received from the residents of the country; but in particular to express his feeling of the cordial assistance which the experiment had received from every person connected with the Harton Colliery.
[p.25] SINCE the delivery of this Lecture, the following letter has been forwarded to South Shields.
“Royal Observatory, Greenwich, December 2nd, 1854.
“My dear Sir,—It will be, I am sure, a matter of satisfaction to you to know that the result of the computations of the pendulum-vibrations gives the highest confidence in the certainty of the results to be deduced from them. The comparison of the rates of the pendulums before and after their interchanges, shews that there is no evidence of their having undergone any mechanical change whatever, and almost positive evidence against their having undergone any change, amounting, in its effect on their vibrations, to 1/20 part of a vibration in a day.
“The immediate result of the computations is this. Supposing that a clock was adjusted to go true time at the top of the mine, it would gain 2¼ seconds per day at the bottom. Or it may be stated thus: that gravity is greater at the bottom of the mine than at the top by 1/19190 part.
“To go a little further into the interpretation. If there had been no coal-measures or rocks of any kind between the top and the bottom, but merely an imaginary stand to support the pendulums, the gravity at the top would have been less than at the bottom by 1/8400 part nearly. But it is less by only 1/19200 part. And what is the cause of the difference? It is the attraction of the shell of matter whose thickness is included between the top and the bottom of the mine. The attraction of that shell, therefore, is the difference between the two numbers which I have given, or is 1/14900 part of gravity nearly.
[p.26] “But if that shell had been as dense as the earth generally, its attraction would have been 1/5600 part of gravity nearly. Therefore the earth generally is more dense than the coal measures, in the proportion of 149 to 56 nearly.
“You will remark that all these numbers are rough, and that to make their results available, some small corrections are required, (to which I have not alluded) and some knowledge of the density of the different beds, &c, which I do not possess at present.
“I am, my dear Sir,
“Yours very truly,
“G. B. Airy.
“James Mather, Esq., South Shields.”
[p.vi] The excellent photograph of the surface works of Harton Pit, and the well-executed diagram of the instruments employed in the Astronomer Royal’s experiments, have been supplied by the liberality of the Editor of the Illustrated London News, who, from the first, has taken a deep interest in the operations.
- Science Quotes by Sir George Biddell Airy.
- 27 Jul - short biography, births, deaths and events on date of Airy's birth.