Mathematics Quotes (1408 quotes)
Math Quotes, Maths Quotes, Mathematical Quotes, Mathematick Quotes
Math Quotes, Maths Quotes, Mathematical Quotes, Mathematick Quotes
… how the real proof should run. The main thing is the content, not the mathematics. With mathematics one can prove anything.
Commenting on a mistake in some work from Planck, for which “the result is correct but the proof is faulty.” As quoted in Denis Brian, Einstein—A Life (1996), 76.
… just as the astronomer, the physicist, the geologist, or other student of objective science looks about in the world of sense, so, not metaphorically speaking but literally, the mind of the mathematician goes forth in the universe of logic in quest of the things that are there; exploring the heights and depths for facts—ideas, classes, relationships, implications, and the rest; observing the minute and elusive with the powerful microscope of his Infinitesimal Analysis; observing the elusive and vast with the limitless telescope of his Calculus of the Infinite; making guesses regarding the order and internal harmony of the data observed and collocated; testing the hypotheses, not merely by the complete induction peculiar to mathematics, but, like his colleagues of the outer world, resorting also to experimental tests and incomplete induction; frequently finding it necessary, in view of unforeseen disclosures, to abandon one hopeful hypothesis or to transform it by retrenchment or by enlargement:—thus, in his own domain, matching, point for point, the processes, methods and experience familiar to the devotee of natural science.
In Lectures on Science, Philosophy and Art (1908), 26
… the reasoning process [employed in mathematics] is not different from that of any other branch of knowledge, … but there is required, and in a great degree, that attention of mind which is in some part necessary for the acquisition of all knowledge, and in this branch is indispensably necessary. This must be given in its fullest intensity; … the other elements especially characteristic of a mathematical mind are quickness in perceiving logical sequence, love of order, methodical arrangement and harmony, distinctness of conception.
In Treatise on Infinitesimal Calculus (1868), Vol. 8, 6.
… the three positive characteristics that distinguish mathematical knowledge from other knowledge … may be briefly expressed as follows: first, mathematical knowledge bears more distinctly the imprint of truth on all its results than any other kind of knowledge; secondly, it is always a sure preliminary step to the attainment of other correct knowledge; thirdly, it has no need of other knowledge.
In Mathematical Essays and Recreations (1898), 35.
… what is physical is subject to the laws of mathematics, and what is spiritual to the laws of God, and the laws of mathematics are but the expression of the thoughts of God.
In 'The Uses of Mathesis', Bibliotheca Sacra, 32, 523.
…indeed what reason may not go to Schoole to the wisdome of Bees, Aunts, and Spiders? what wise hand teacheth them to doe what reason cannot teach us? Ruder heads stand amazed at those prodigious pieces of nature, Whales, Elephants, Dromidaries and Camels; these I confesse, are the Colossus and Majestick pieces of her hand; but in these narrow Engines there is more curious Mathematicks, and the civilitie of these little Citizens more neatly sets forth the wisedome of their Maker.
In Religio Medici and Other Writings (1909), 17.
…nature seems very conversant with the rules of pure mathematics, as our own mathematicians have formulated them in their studies, out of their own inner consciousness and without drawing to any appreciable extent on their experience of the outer world.
In The Mysterious Universe (1930), 113.
...the source of all great mathematics is the special case, the concrete example. It is frequent in mathematics that every instance of a concept of seemingly generality is, in essence, the same as a small and concrete special case.
I Want to be a Mathematician: an Automathography in Three Parts (1985), 324.
‘I was reading an article about “Mathematics”. Perfectly pure mathematics. My own knowledge of mathematics stops at “twelve times twelve,” but I enjoyed that article immensely. I didn’t understand a word of it; but facts, or what a man believes to be facts, are always delightful. That mathematical fellow believed in his facts. So do I. Get your facts first, and’—the voice dies away to an almost inaudible drone—’then you can distort ‘em as much as you please.’
In 'An Interview with Mark Twain', in Rudyard Kipling, From Sea to Sea (1899), Vol. 2, 180.
“Can you do Addition?” the White Queen said. “What's one and one and one and one and one and one and one and one and one and one?”
“I don't know,” said Alice. “I lost count.”
“She can’t do Addition,” the Red Queen interrupted.
“I don't know,” said Alice. “I lost count.”
“She can’t do Addition,” the Red Queen interrupted.
Through the Looking Glass and What Alice Found There (1871, 1897), 189.
“Every moment dies a man,/ Every moment one is born”:
I need hardly point out to you that this calculation would tend to keep the sum total of the world's population in a state of perpetual equipoise whereas it is a well-known fact that the said sum total is constantly on the increase. I would therefore take the liberty of suggesting that in the next edition of your excellent poem the erroneous calculation to which I refer should be corrected as follows:
'Every moment dies a man / And one and a sixteenth is born.” I may add that the exact figures are 1.167, but something must, of course, be conceded to the laws of metre.
I need hardly point out to you that this calculation would tend to keep the sum total of the world's population in a state of perpetual equipoise whereas it is a well-known fact that the said sum total is constantly on the increase. I would therefore take the liberty of suggesting that in the next edition of your excellent poem the erroneous calculation to which I refer should be corrected as follows:
'Every moment dies a man / And one and a sixteenth is born.” I may add that the exact figures are 1.167, but something must, of course, be conceded to the laws of metre.
Unpublished letter to Tennyson in response to his Vision of Sin (1842). Quoted in Philip and Emily Morrison, Charles Babbage and his Calculating Engines: Selected Writings by Charles Babbage and Others (1961), xxiii.
“I think you’re begging the question,” said Haydock, “and I can see looming ahead one of those terrible exercises in probability where six men have white hats and six men have black hats and you have to work it out by mathematics how likely it is that the hats will get mixed up and in what proportion. If you start thinking about things like that, you would go round the bend. Let me assure you of that!”
In The Mirror Crack’d (1962), 190.
“Obvious” is the most dangerous word in mathematics.
In The Queen of the Sciences (1938), 14.
[1155] Mechanics are the Paradise of mathematical science, because here we come to the fruits of mathematics.
Notebook E (1513), folio 8 back. In the original Italian: “La meccanica è il paradiso delle sciētie matematiche, perchè cō quella si viene al frutto matematico.” English and Italian in Jean Paul Richter (trans.), 'Philosophical Maxims: Of Mechanics', The Literary Works of Leonardo da Vinci (1883), Vol. 1, Part 2, 289, Aphorism 1155. [Note: da Vinci wrote ē=en, ō=on] Also translated as “Mechanics is the paradise of the mathematical sciences, because by means of it one comes to the fruits of mathematics,” in Edward McCurdy, The Notebooks of Leonardo Da Vinci (1939, 1958), Vol. 1, 613.
[1157] The man who blames the supreme certainty of mathematics feeds on confusion, and can never silence the contradictions of sophistical sciences which lead to an eternal quackery.
W. An. III. 241 a. From the original Italian: “Chi biasima la soma certezza della matematica, si pasce di confusione mai porrà silentio alle contraditioni delle soffistiche sciētie, colle quali s’inpara vno eterno gridore.” English and Italian in Jean Paul Richter (trans.), 'Philosophical Maxims: Of Mechanics', The Literary Works of Leonardo da Vinci (1883), Vol. 1, Part 2, 289, Aphorism 1157. [Note: Da Vinci writes ē=en.] Also translated beginning, “Those who condemn…”. Also seen translated as “Whoever despises the high wisdom of mathematics nourishes himself on delusion and will never still the sophistic sciences whose only product is an eternal uproar,” in Nicholas J. Rose Mathematical Maxims and Minims (1988).
[1158] There is no certainty in sciences where one of the mathematical sciences cannot be applied, or which are not in relation with these mathematics.
Notebook G (c.1515), sheet 95 back. In the original Italian: “Nessuna certezza delle sciētie è, dove no si può applicare vna delle sciētie matematiche e che non son vnight con esse matematiche.” English and Italian in Jean Paul Richter (trans.), 'Philosophical Maxims: Of Mechanics', The Literary Works of Leonardo da Vinci (1883), Vol. 1, Part 2, 289, Aphorism 1158. [Note: da Vinci wrote ē=en; v=u] The following, found on the web, without citation, seems to be a paraphrase: “No human investigation can be called real science if it cannot be demonstrated mathematically.”
[Adams] supposed that, except musicians, everyone thought Beethoven a bore, as every one except mathematicians thought mathematics a bore.
The Education of Henry Brooks Adams: An Autobiography (1918), 80.
[All phenomena] are equally susceptible of being calculated, and all that is necessary, to reduce the whole of nature to laws similar to those which Newton discovered with the aid of the calculus, is to have a sufficient number of observations and a mathematics that is complex enough.
Unpublished Manuscript. Quoted In Frank Edward Manuel and Fritzie Prigohzy Manuel, Utopian Thought in the Western World (1979, 2009), 493.
[An appealing problem is] a combination of being fairly concrete—so one can understand concretely examples—and also connecting with a lot of other ideas. For example, you see the analysis in a minimal surface equation, but then you also realize it has connections with other geometric questions that are not just analysis. I am definitely very attracted to the idea that there are a lot of different facets in mathematics and seeing the connections.
From Allyn Jackson, 'Interview with Karen Uhlenbeck', part of Celebratio Mathematica on the celebratio.org website.
[At high school in Cape Town] my interests outside my academic work were debating, tennis, and to a lesser extent, acting. I became intensely interested in astronomy and devoured the popular works of astronomers such as Sir Arthur Eddington and Sir James Jeans, from which I learnt that a knowledge of mathematics and physics was essential to the pursuit of astronomy. This increased my fondness for those subjects.
'Autobiography of Allan M. Cormack,' Les Prix Nobel/Nobel Lectures 1979, editted by Wilhelm Odelberg.
[Before the time of Benjamin Peirce it never occurred to anyone that mathematical research] was one of the things for which a mathematical department existed. Today it is a commonplace in all the leading universities. Peirce stood alone—a mountain peak whose absolute height might be hard to measure, but which towered above all the surrounding country.
In 'The Story of Mathematics at Harvard', Harvard Alumni Bulletin (3 Jan 1924), 26, 376. Cited by R. C. Archibald in 'Benjamin Peirce: V. Biographical Sketch', The American Mathematical Monthly (Jan 1925), 32, No. 1, 10.
[Cantor’s set theory is] The finest product of mathematical genius and one of the supreme achievements of purely intellectual human activity.
As quoted in Constance Reid, Hilbert (1970), 176.
[Comte] may truly be said to have created the philosophy of higher mathematics.
In System of Logic (1846), 369.
[Euclid's Elements] has been for nearly twenty-two centuries the encouragement and guide of that scientific thought which is one thing with the progress of man from a worse to a better state. The encouragement; for it contained a body of knowledge that was really known and could be relied on, and that moreover was growing in extent and application. For even at the time this book was written—shortly after the foundation of the Alexandrian Museum—Mathematics was no longer the merely ideal science of the Platonic school, but had started on her career of conquest over the whole world of Phenomena. The guide; for the aim of every scientific student of every subject was to bring his knowledge of that subject into a form as perfect as that which geometry had attained. Far up on the great mountain of Truth, which all the sciences hope to scale, the foremost of that sacred sisterhood was seen, beckoning for the rest to follow her. And hence she was called, in the dialect of the Pythagoreans, ‘the purifier of the reasonable soul.’
From a lecture delivered at the Royal Institution (Mar 1873), collected postumously in W.K. Clifford, edited by Leslie Stephen and Frederick Pollock, Lectures and Essays, (1879), Vol. 1, 296.
[Experimental Physicist] Phys. I know that it is often a help to represent pressure and volume as height and width on paper; and so geometry may have applications to the theory of gases. But is it not going rather far to say that geometry can deal directly with these things and is not necessarily concerned with lengths in space?
[Mathematician] Math. No. Geometry is nowadays largely analytical, so that in form as well as in effect, it deals with variables of an unknown nature. …It is literally true that I do not want to know the significance of the variables x, y, z, t that I am discussing. …
Phys. Yours is a strange subject. You told us at the beginning that you are not concerned as to whether your propositions are true, and now you tell us you do not even care to know what you are talking about.
Math. That is an excellent description of Pure Mathematics, which has already been given by an eminent mathematician [Bertrand Russell].
[Mathematician] Math. No. Geometry is nowadays largely analytical, so that in form as well as in effect, it deals with variables of an unknown nature. …It is literally true that I do not want to know the significance of the variables x, y, z, t that I am discussing. …
Phys. Yours is a strange subject. You told us at the beginning that you are not concerned as to whether your propositions are true, and now you tell us you do not even care to know what you are talking about.
Math. That is an excellent description of Pure Mathematics, which has already been given by an eminent mathematician [Bertrand Russell].
In Space, Time and Gravitation: An Outline of the General Relativity Theory (1920, 1921), 14.
[I can] scarcely write upon mathematics or mathematicians. Oh for words to express my abomination of the science.
Lamenting mathematics whilst an undergraduate at Cambridge, 1818.
Lamenting mathematics whilst an undergraduate at Cambridge, 1818.
Quoted in John Gascoigne, Cambridge in the Age of Enlightenment (1989), 272.
[I was advised] to read Jordan's 'Cours d'analyse'; and I shall never forget the astonishment with which I read that remarkable work, the first inspiration for so many mathematicians of my generation, and learnt for the first time as I read it what mathematics really meant.
In A Mathematician’s Apology (1940, reprint with Foreward by C.P. Snow 1992), 23.
[I]f in other sciences we should arrive at certainty without doubt and truth without error, it behooves us to place the foundations of knowledge in mathematics, in so far as disposed through it we are able to reach certainty in other sciences and truth by the exclusion of error. (c.1267)
Translation by Robert Burke, Opus Majus of Roger Bacon (1928), vol 1, 124. In Fred R. Shapiro, The Yale Book of Quotations (2006), 39.
[In junior high school] I liked math—that was my favorite subject—and I was very interested in astronomy and in physical science.
Interview conducted on Scholastic website (20 Nov 1998).
[In mathematics] There are two kinds of mistakes. There are fatal mistakes that destroy a theory, but there are also contingent ones, which are useful in testing the stability of a theory.
In 'Ten Lessons I Wish I Had Been Taught', Indiscrete Thoughts (2008), 202.
[In mathematics] we behold the conscious logical activity of the human mind in its purest and most perfect form. Here we learn to realize the laborious nature of the process, the great care with which it must proceed, the accuracy which is necessary to determine the exact extent of the general propositions arrived at, the difficulty of forming and comprehending abstract concepts; but here we learn also to place confidence in the certainty, scope and fruitfulness of such intellectual activity.
In Ueber das Verhältnis der Naturwissenschaften zur Gesammtheit der Wissenschaft, Vorträge und Reden (1896), Bd. 1, 176. Also seen translated as “In mathematics we see the conscious logical activity of our mind in its purest and most perfect form; here is made manifest to us all the labor and the great care with which it progresses, the precision which is necessary to determine exactly the source of the established general theorems, and the difficulty with which we form and comprehend abstract conceptions; but we also learn here to have confidence in the certainty, breadth, and fruitfulness of such intellectual labor”, in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 20. From the original German, “Hier sehen wir die bewusste logische Thätigkeit unseres Geistes in ihrer reinsten und vollendetsten Form; wir können hier die ganze Mühe derselben kennen lernen, die grosse Vorsicht, mit der sie vorschreiten muss, die Genauigkeit, welche nöthig ist, um den Umfang der gewonnenen allgemeinen Sätze genau zu bestimmen, die Schwierigkeit, abstracte Begriffe zu bilden und zu verstehen; aber ebenso auch Vertrauen fassen lernen in die Sicherheit, Tragweite und Fruchtbarkeit solcher Gedankenarbeit.”
[In the Royal Society, there] has been, a constant Resolution, to reject all the amplifications, digressions, and swellings of style: to return back to the primitive purity, and shortness, when men deliver'd so many things, almost in an equal number of words. They have exacted from all their members, a close, naked, natural way of speaking; positive expressions; clear senses; a native easiness: bringing all things as near the Mathematical plainness, as they can: and preferring the language of Artizans, Countrymen, and Merchants, before that, of Wits, or Scholars.
The History of the Royal Society (1667), 113.
[Karen] Uhlenbeck’s research has led to revolutionary advances at the intersection of mathematics and physics. Her pioneering insights have applications across a range of fascinating subjects, from string theory, which may help explain the nature of reality, to the geometry of space-time.
In news release, 'Mathematics’ Highest Prize Awarded to UT Austin’s Karen Uhlenbeck', UT News (19 Mar 2019) on website of University of Texas at Austin.
[Kepler] had to realize clearly that logical-mathematical theoretizing, no matter how lucid, could not guarantee truth by itself; that the most beautiful logical theory means nothing in natural science without comparison with the exactest experience. Without this philosophic attitude, his work would not have been possible.
From Introduction that Einstein wrote for Carola Baumgardt and Jamie Callan, Johannes Kepler Life and Letters (1953), 13.
[Mathematics is] the study of ideal constructions (often applicable to real problems), and the discovery thereby of relations between the parts of these constructions, before unknown.
In 'Mathematics', Century Dictionary.
[Mathematics is] the study of the measurement, properties, and relationships of quantities and sets, using numbers and symbols.
Definition of Mathematics in William morris (ed.), American Heritage Dictionary (2000).
[Mathematics] has for its object the indirect measurement of magnitudes, and it proposes to determine magnitudes by each other, according to the precise relations which exist between them.
In The Positive Philosophy of Auguste Comte, translated by Harriet Martineau, (1896), Vol. 1, 40.
[Mathematics] is an independent world
Created out of pure intelligence.
Created out of pure intelligence.
In The Prelude, Book 6, lines 186-187. [Preceding lines refer to “laws of Nature” and “a treatise of geometry.” Wordsworth did not use the word “mathematics”, which is added parenthetically to give context to the quote.]
[Mathematics] is security. Certainty. Truth. Beauty. Insight. Structure. Architecture. I see mathematics, the part of human knowledge that I call mathematics, as one thing—one great, glorious thing. Whether it is differential topology, or functional analysis, or homological algebra, it is all one thing. … They are intimately interconnected, they are all facets of the same thing. That interconnection, that architecture, is secure truth and is beauty. That’s what mathematics is to me.
From interview with Donald J. Albers. In John H. Ewing and Frederick W. Gehring, Paul Halmos Celebrating 50 Years of Mathematics (1991), 13.
[Mathematics] is that [subject] which knows nothing of observation, nothing of experiment, nothing of induction, nothing of causation.
In 'The Scientific Aspects of Positivism', Fortnightly Review (1898) in Lay Sermons, Addresses and Reviews, (1872), 169.
[P]olitical and social and scientific values … should be correlated in some relation of movement that could be expressed in mathematics, nor did one care in the least that all the world said it could not be done, or that one knew not enough mathematics even to figure a formula beyond the schoolboy s=(1/2)gt2. If Kepler and Newton could take liberties with the sun and moon, an obscure person ... could take liberties with Congress, and venture to multiply its attraction into the square of its time. He had only to find a value, even infinitesimal, for its attraction.
The Education of Henry Adams: An Autobiography? (1918), 376.
[P]ure mathematics is on the whole distinctly more useful than applied. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics.
In A Mathematician’s Apology (1940, reprint with Foreward by C.P. Snow 1992), 134.
[Pure mathematics is] good to give chills in the spine to a certain number of people, me included. I don’t know what else it is good for, and I don’t care. But … like von Neumann said, one never knows whether someone is going to find another use for it.
In The Beauty of Doing Mathematics: Three Public Dialogues (1985), 49.
[Referring to Fourier’s mathematical theory of the conduction of heat] … Fourier's great mathematical poem…
In W. Thomson and P. G. Tait, Treatise on Natural Philosophy. Reprinted as Principles of Mechanics and Dynamics (2000), 470.
[Regarding mathematics,] there are now few studies more generally recognized, for good reasons or bad, as profitable and praiseworthy. This may be true; indeed it is probable, since the sensational triumphs of Einstein, that stellar astronomy and atomic physics are the only sciences which stand higher in popular estimation.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 63-64.
[Student:} I only use my math book on special equations.
From movie Rock 'n' Roll High School (1979). Writers, Richard Whitley, Russ Dvonch and Joseph McBride. In Larry Langman and Paul Gold, Comedy Quotes from the Movies (2001), 359.
[The error in the teaching of mathematics is that] mathematics is expected either to be immediately attractive to students on its own merits or to be accepted by students solely on the basis of the teacher’s assurance that it will be helpful in later life. [And yet,] mathematlcs is the key to understanding and mastering our physical, social and biological worlds.
In editorial in Focus, a Journal of the Mathematical Association of America (1986), quoted in obituary by Eric Pace, New York Times (11 Jun 1992).
[The famous attack of Sir William Hamilton on the tendency of mathematical studies] affords the most express evidence of those fatal lacunae in the circle of his knowledge, which unfitted him for taking a comprehensive or even an accurate view of the processes of the human mind in the establishment of truth. If there is any pre-requisite which all must see to be indispensable in one who attempts to give laws to the human intellect, it is a thorough acquaintance with the modes by which human intellect has proceeded, in the case where, by universal acknowledgment, grounded on subsequent direct verification, it has succeeded in ascertaining the greatest number of important and recondite truths. This requisite Sir W. Hamilton had not, in any tolerable degree, fulfilled. Even of pure mathematics he apparently knew little but the rudiments. Of mathematics as applied to investigating the laws of physical nature; of the mode in which the properties of number, extension, and figure, are made instrumental to the ascertainment of truths other than arithmetical or geometrical—it is too much to say that he had even a superficial knowledge: there is not a line in his works which shows him to have had any knowledge at all.
In Examination of Sir William Hamilton's Philosophy (1878), 607.
[The infinitely small] neither have nor can have theory; it is a dangerous instrument in the hands of beginners [ ... ] anticipating, for my part, the judgement of posterity, I would dare predict that this method will be accused one day, and rightly, of having retarded the progress of the mathematical sciences.
Annales des Mathematiques Pures et Appliquées (1814-5), 5, 148.
[The] humanization of mathematical teaching, the bringing of the matter and the spirit of mathematics to bear not merely upon certain fragmentary faculties of the mind, but upon the whole mind, that this is the greatest desideratum is. I assume, beyond dispute.
Address (28 Mar 1912), Michigan School Masters' Club, Ann Arbor, 'The Humanization of the Teaching of Mathematics. Printed in Science (26 Apr 1912). Collected in The Human Worth of Rigorous Thinking: Essays and Addresses (1916), 62-63.
[There is] one distinctly human thing - the story. There can be as good science about a turnip as about a man. ... [Or philosophy, or theology] ...There can be, without any question at all, as good higher mathematics about a turnip as about a man. But I do not think, though I speak in a manner somewhat tentative, that there could be as good a novel written about a turnip as a man.
In 'A Much Repeated Repetition', Daily News (26 Mar 1904). Collected in G. K. Chesterton and Dale Ahlquist (ed.), In Defense of Sanity: The Best Essays of G.K. Chesterton (2011), 84.
[There is] some mathematical quality in Nature, a quality which the casual observer of Nature would not suspect, but which nevertheless plays an important role in Nature’s scheme.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 122.
[There was] in some of the intellectual leaders a great aspiration to demonstrate that the universe ran like a piece of clock-work, but this was was itself initially a religious
aspiration. It was felt that there would be something defective in Creation itself—something not quite worthy of God—unless the whole system of the universe could be shown to be interlocking, so that it carried the pattern of reasonableness and orderliness. Kepler, inaugurating the scientist’s quest for a mechanistic universe in the seventeenth century, is significant here—his mysticism, his music of the spheres, his rational deity demand a system which has the beauty of a piece of mathematics.
In The Origins of Modern Science (1950), 105.
[There] are still to be found text-books of the old sort, teaching Mathematics under the guise of Physics, presenting nothing but the dry husks of the latter.
A paper read at the Association for the Improvement of Geometrical Teaching (19 Jan 1889), 'The Vices of our Scientific Education', in Nature (6 Jun 1889), 40, 128.
[Urbain Jean Joseph] Le Verrier—without leaving his study, without even looking at the sky—had found the unknown planet [Neptune] solely by mathematical calculation, and, as it were, touched it with the tip of his pen!
In Camille Flammarion, Astronomy (1914), 171.
[W]hen Galileo discovered he could use the tools of mathematics and mechanics to understand the motion of celestial bodies, he felt, in the words of one imminent researcher, that he had learned the language in which God recreated the universe. Today we are learning the language in which God created life. We are gaining ever more awe for the complexity, the beauty, the wonder of God's most devine and sacred gift.
From White House Announcement of the Completion of the First Survey of the Entire Human Genome Project, broadcast on the day of the publication of the first draft of the human genome. Quoted in transcript on the National Archives, Clinton White House web site, 'Text of Remarks on the Completion of the First Survey of the Entire Human Genome Project' (26 Jun 2000).
[We] can easily distinguish what relates to Mathematics in any question from that which belongs to the other sciences. But as I considered the matter carefully it gradually came to light that all those matters only were referred to Mathematics in which order and measurements are investigated, and that it makes no difference whether it be in numbers, figures, stars, sounds or any other object that the question of measurement arises. I saw consequently that there must be some general science to explain that element as a whole which gives rise to problems about order and measurement, restricted as these are to no special subject matter. This, I perceived was called “Universal Mathematics,” not a far-fetched asignation, but one of long standing which has passed into current use, because in this science is contained everything on account of which the others are called parts of Mathematics.
Rules for the Direction of the Mind (written 1628). As translated by Elizabeth Sanderson Haldane and George Robert Thomson Ross in The Philosophical Works of Descartes (1911, 1931), 13.
Apud me omnia fiunt Mathematicè in Natura.
In my opinion, everything happens in nature in a mathematical way.
In my opinion, everything happens in nature in a mathematical way.
In letter (11 Mar 1640) to Père Marin Mersenne. English version inspired by a translation of the original Latin in German, “Nach meiner Ansicht geschieht alles in der Natur auf mathematische Art,” in René Descartes and Artur Buchenau (trans., ed.), René Descartes' Philosophische Werke (1905), 246. The Latin is often seen misquoted as “omnia apud me mathematica fiunt.” See context in longer quote that begins, “I have no doubt….” on the René Descartes Quotes page of this website.
Ces détails scientifiques qui effarouchent les fabricans d’un certain âge, ne seront qu’un
jeu pour leurs enfans, quand ils auront apprit dans leurs collèges un peu plus de mathématiques et un peu moins de Latin; un peu plus de Chimie, et un peu moins de Grec!
The scientific details which now terrify the adult manufacturer will be mere trifles to his children when they shall be taught at school, a little more Mathematics and a little less Latin, a little more Chemistry, and a little less Greek.
The scientific details which now terrify the adult manufacturer will be mere trifles to his children when they shall be taught at school, a little more Mathematics and a little less Latin, a little more Chemistry, and a little less Greek.
As quoted in 'Sketches From Life of Some Eminent Foreign Scientific Lecturers: Dumas', Magazine of Popular Science, and Journal of the Useful Arts (1836). Vol. 1, 177.
Das ist nicht Mathematik, das ist Theologie!
This is not mathematics; this is theology.
[Remark about David Hilbert's first proof of his finite basis theorem.]
This is not mathematics; this is theology.
[Remark about David Hilbert's first proof of his finite basis theorem.]
Attributed. It does not seem to appear in Gordan’s written work. According to Colin McClarty, in 'Theology and its Discontents: the Origin of the Myth of Modern Mathematics' (2008), “The quote first appeared a quarter of a century after the event, as an unexplained side comment in a eulogy to Gordan by his long-time colleague Max Noether. Noether was a reliable witness, but he says little about what Gordan meant.” See Noether's obituary of Gordan in Mathematische Annalen (1914), 75, 18. It is still debated if the quote is pejorative, complimentary or merely a joke.
Das Leben der Gotter ist Mathematik.
Mathematics is the Life of the Gods.
Mathematics is the Life of the Gods.
Attributed.
Every teacher certainly should know something of non-euclidean geometry. Thus, it forms one of the few parts of mathematics which, at least in scattered catch-words, is talked about in wide circles, so that any teacher may be asked about it at any moment. … Imagine a teacher of physics who is unable to say anything about Röntgen rays, or about radium. A teacher of mathematics who could give no answer to questions about non-euclidean geometry would not make a better impression.
On the other hand, I should like to advise emphatically against bringing non-euclidean into regular school instruction (i.e., beyond occasional suggestions, upon inquiry by interested pupils), as enthusiasts are always recommending. Let us be satisfied if the preceding advice is followed and if the pupils learn to really understand euclidean geometry. After all, it is in order for the teacher to know a little more than the average pupil.
On the other hand, I should like to advise emphatically against bringing non-euclidean into regular school instruction (i.e., beyond occasional suggestions, upon inquiry by interested pupils), as enthusiasts are always recommending. Let us be satisfied if the preceding advice is followed and if the pupils learn to really understand euclidean geometry. After all, it is in order for the teacher to know a little more than the average pupil.
In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 72.
Je me rends parfaitement compte du desagreable effet que produit sur la majorite de l'humanité, tout ce qui se rapporte, même au plus faible dègré, á des calculs ou raisonnements mathematiques.
I am well aware of the disagreeable effect produced on the majority of humanity, by whatever relates, even at the slightest degree to calculations or mathematical reasonings.
I am well aware of the disagreeable effect produced on the majority of humanity, by whatever relates, even at the slightest degree to calculations or mathematical reasonings.
From 'French Reply to Baron Czyllak' concerning the game at Monte Carlo, Monte Carlo Facts and Fallacies (1904), 290, originally published in L'Écho de la Mediterranée as a response to an earlier open letter by the Baron in the same magazine. Maxim defended his prior mathematical calculations about gambling games. At the end of his paper giving a cautionary mathematical analysis of 'The Gambler's Ruin', < a href="http://todayinsci.com/C/Coolidge_Julian/CoolidgeJulian-Quotations.htm">Julian Coolidge referenced this quotation, saying “it gives the best explanation which I have seen for the fact that the people continue to gamble.”
La chaleur pénètre, comme la gravité, toutes les substances de l’univers, ses rayons occupent toutes les parties de l’espace. Le but de notre ouvrage est d’exposer les lois mathématiques que suit cet élément. Cette théorie formera désormais une des branches les plus importantes de la physique générale.
Heat, like gravity, penetrates every substance of the universe, its rays occupy all parts of space. The object of our work is to set forth the mathematical laws which this element obeys. The theory of heat will hereafter form one of the most important branches of general physics.
Heat, like gravity, penetrates every substance of the universe, its rays occupy all parts of space. The object of our work is to set forth the mathematical laws which this element obeys. The theory of heat will hereafter form one of the most important branches of general physics.
From 'Discours Préliminaire' to Théorie Analytique de la Chaleur (1822), i, translated by Alexander Freeman in The Analytical Theory of Heat (1878), 1.
Les mathématique sont un triple. Elles doivent fournir un instrument pour l'étude de la nature. Mais ce n'est pas tout: elles ont un but philosophique et, j'ose le dire, un but esthétique.
Mathematics has a threefold purpose. It must provide an instrument for the study of nature. But this is not all: it has a philosophical purpose, and, I daresay, an aesthetic purpose.
Mathematics has a threefold purpose. It must provide an instrument for the study of nature. But this is not all: it has a philosophical purpose, and, I daresay, an aesthetic purpose.
La valeur de la science. In Anton Bovier, Statistical Mechanics of Disordered Systems (2006), 161.
Longtemps les objets dont s'occupent les mathématiciens étaient our la pluspart mal définis; on croyait les connaître, parce qu'on se les représentatit avec le sens ou l'imagination; mais on n'en avait qu'une image grossière et non une idée précise sure laquelle le raisonment pût avoir prise.
For a long time the objects that mathematicians dealt with were mostly ill-defined; one believed one knew them, but one represented them with the senses and imagination; but one had but a rough picture and not a precise idea on which reasoning could take hold.
For a long time the objects that mathematicians dealt with were mostly ill-defined; one believed one knew them, but one represented them with the senses and imagination; but one had but a rough picture and not a precise idea on which reasoning could take hold.
La valeur de la science. In Anton Bovier, Statistical Mechanics of Disordered Systems (2006), 97.
Mathematical Knowledge adds a manly Vigour to the Mind, frees it from Prejudice, Credulity, and Superstition.
In An Essay On the Usefulness of Mathematical Learning, (1701), 7.
Mathematical truth has validity independent of place, personality, or human authority. Mathematical relations are not established, nor can they be abrogated, by edict. The multiplication table is international and permanent, not a matter of convention nor of relying upon authority of state or church. The value of π is not amenable to human caprice. The finding of a mathematical theorem may have been a highly romantic episode in the personal life of the discoverer, but it cannot be expected of itself to reveal the race, sex, or temperament of this discoverer. With modern means of widespread communication even mathematical notation tends to be international despite all nationalistic tendencies in the use of words or of type.
In 'Light Thrown on the Nature of Mathematics by Certain Aspects of Its Development', Mathematics in General Education (1940), 256. This is the Report of the Committee on the Function of Mathematics in General Education of the Commission on Secondary School Curriculum, which was established by the Executive Board of the Progressive Education Association in 1932.
Natura non facit saltum or, Nature does not make leaps… If you assume continuity, you can open the well-stocked mathematical toolkit of continuous functions and differential equations, the saws and hammers of engineering and physics for the past two centuries (and the foreseeable future).
From Benoit B. Mandelbrot and Richard Hudson, The (Mis)Behaviour of Markets: A Fractal View of Risk, Ruin and Reward (2004,2010), 85-86.
Neumann, to a physicist seeking help with a difficult problem: Simple. This can be solved by using the method of characteristics.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Attributed, as related by Dr. Felix Smith (Head of Molecular Physics, Stanford Research Institute) to author Gary Zukav, who quoted it in The Dancing Wu Li Masters: An Overview of the New Physics (1979, 2001), 208, footnote. The physicist (a friend of Dr. Smith) worked at Los Alamos after WW II. It should be noted that although the author uses quotation marks around the spoken remarks, that they represent the author's memory of Dr. Smith's recollection, who heard it from the physicist. Therefore the fourth-hand wording is very likely not verbatim. Webmaster finds Zukav's book seems to be the only source for this quote.
Simplicibus itaque verbis gaudet Mathematica Veritas, cum etiam per se simplex sit Veritatis oratio. (So Mathematical Truth prefers simple words since the language of Truth is itself simple.)
Epistolarum astronomicarum liber primus (1596)
The Annotated Alice, of course, does tie in with math, because Lewis Carroll was, as you know, a professional mathematician. So it wasn’t really too far afield from recreational math, because the two books are filled with all kinds of mathematical jokes. I was lucky there in that I really didn’t have anything new to say in The Annotated Alice because I just looked over the literature and pulled together everything in the form of footnotes. But it was a lucky idea because that’s been the best seller of all my books.
In Anthony Barcellos, 'A Conversation with Martin Gardner', The Two-Year College Mathematics Journal (Sep 1979), 10, No. 4, 241.
Ultima se tangunt. How expressive, how nicely characterizing withal is mathematics! As the musician recognizes Mozart, Beethoven, Schubert in the first chords, so the mathematician would distinguish his Cauchy, Gauss, Jacobi, Helmholtz in a few pages.
In Ceremonial Speech (15 Nov 1887) celebrating the 301st anniversary of the Karl-Franzens-University Graz. Published as Gustav Robert Kirchhoff: Festrede zur Feier des 301. Gründungstages der Karl-Franzens-Universität zu Graz (1888), 29, as translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 186-187. From the original German, “Ultima se tangunt. Und wie ausdrucksfähig, wie fein charakterisirend ist dabei die Mathematik. Wie der Musiker bei den ersten Tacten Mozart, Beethoven, Schubert erkennt, so würde der Mathematiker nach wenig Seiten, seinen Cauchy, Gauss, Jacobi, Helmholtz unterscheiden.” [The Latin words translate as “the final touch”. —Webmaster]
~~[Attributed, authorship undocumented]~~ Mathematical demonstrations are a logic of as much or more use, than that commonly learned at schools, serving to a just formation of the mind, enlarging its capacity, and strengthening it so as to render the same capable of exact reasoning, and discerning truth from falsehood in all occurrences, even in subjects not mathematical. For which reason it is said, the Egyptians, Persians, and Lacedaemonians seldom elected any new kings, but such as had some knowledge in the mathematics, imagining those, who had not, men of imperfect judgments, and unfit to rule and govern.
From an article which appeared as 'The Usefulness of Mathematics', Pennsylvania Gazette (30 Oct 1735), No. 360. Collected, despite being without clear evidence of Franklin’s authorship, in The Works of Benjamin Franklin (1809), Vol. 4, 377. Evidence of actual authorship by Ben Franklin for the newspaper article has not been ascertained, and scholars doubt it. See Franklin documents at the website founders.archives.gov. The quote is included here to attach this caution.
~~[Misattributed ?]~~ Mathematical discoveries, like springtime violets in the woods, have their season which no human can hasten or retard.
Webmaster believes this quote is likely a misattributed paraphrase. The subject quote is as given in Israel Kleiner, 'Thinking the Unthinkable: The Story of Complex Numbers (with a Moral)', Mathematics Teacher (Oct 1988), 81, No. 7, 590. In Kleiner’s paper, alongside the quote is a citation, thus: “(Kline 1972)?” Notice the appended question mark. The reference at the end of the paper gives: Morris Kline, Mathematical Thought from Ancient to Modern Times (1972), but without page number. Webmaster checked a later edition, Vol. 3 (1990), 861, in which Kline has an epigraph, with different wording about violets, attributed - not to János - but to his father, “Wolfgang Bolyai” (who is also known as Farkas Bolyai). Translator Abe Shenitzer wrote an ambiguous passage in Herbert Meschkowski, NonEuclidean Geometry (1964), 33. In a discussion posted in the NCTM online Math Forum in 1998, Shenitzer clarified that the proper reading is that the “violet talk” is a simile used in advice given by the father to his son. Note that in the passage, János (Johann/John) reports about that advice in narrative form. Thus, one should also note that even in the original language, perhaps the father’s words are not verbatim. See Farkas Bolyai Quotes on another page of this website.
~~[Misquote]~~ Fourier is a mathematical poem.
Seen in various books and on the web. This seems to be a misquote based on Kelvin’s reference to Fourier’s mathematical theory of the conduction of heat as “Fourier's great mathematical poem.” More information on the latter quote on the Lord Kelvin Quotes page on this website.
~~[No known source]~~ Medicine makes people ill, mathematics make them sad and theology makes them sinful.
Webmaster strongly doubts that this is an authentic quote. As yet, Webmaster has found no primary source, no citation, and not even an early publication with this quote. Best guess is that it is a recent viral fake or mistake. Can you help? Meanwhile, Webmaster includes this quote here only to add this caution. At best, likely, it is barely a joke, by anonymous.
A general course in mathematics should be required of all officers for its practical value, but no less for its educational value in training the mind to logical forms of thought, in developing the sense of absolute truthfulness, together with a confidence in the accomplishment of definite results by definite means.
In 'Mathematics at West Point and Annapolis', United States Bureau of Education, Bulletin 1912, No. 2, 11.
A chemist who does not know mathematics is seriously handicapped.
Quoted in Albert Rosenfeld, Langmuir: The Man and the Scientist (1962), 293.
A chess problem is genuine mathematics, but it is in some way “trivial” mathematics. However, ingenious and intricate, however original and surprising the moves, there is something essential lacking. Chess problems are unimportant. The best mathematics is serious as well as beautiful—“important” if you like, but the word is very ambiguous, and “serious” expresses what I mean much better.
'A Mathematician's Apology', in James Roy Newman, The World of Mathematics (2000), 2029.
A colleague likened Mr Erdos to a honeybee: an industrious creature who buzzed about the world and pollinated the fields of mathematics.
— Magazine
The Economist, October 5th 1996, page 83.
A formal manipulator in mathematics often experiences the discomforting feeling that his pencil surpasses him in intelligence.
In An Introduction to the History of Mathematics (1953, 1976), 354. This same idea was said much earlier by Ernst Mach (1893). See the quote that begins, “The mathematician who pursues his studies,” on the Ernst Mach Quotes page on this website.
A fractal is a mathematical set or concrete object that is irregular or fragmented at all scales.
Cited as from Fractals: Form, Chance, and Dimension (1977), by J.W. Cannon, in review of The Fractal Geometry of Nature (1982) in The American Mathematical Monthly (Nov 1984), 91, No. 9, 594.
A good deal of my research in physics has consisted in not setting out to solve some particular problem, but simply examining mathematical quantities of a kind that physicists use and trying to fit them together in an interesting way, regardless of any application that the work may have. It is simply a search for pretty mathematics. It may turn out later to have an application. Then one has good luck. At age 78.
International Journal of Theoretical Physics (1982), 21, 603. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 110.
A good mathematical joke is better, and better mathematics, than a dozen mediocre papers.
In A Mathematician’s Miscellany (1953), reissued as Béla Bollobás, Littlewood’s Miscellany (1986), 24.
A good theoretical physicist today might find it useful to have a wide range of physical viewpoints and mathematical expressions of the same theory (for example, of quantum electrodynamics) available to him. This may be asking too much of one man. Then new students should as a class have this. If every individual student follows the same current fashion in expressing and thinking about electrodynamics or field theory, then the variety of hypotheses being generated to understand strong interactions, say, is limited. Perhaps rightly so, for possibly the chance is high that the truth lies in the fashionable direction. But, on the off-chance that it is in another direction—a direction obvious from an unfashionable view of field theory—who will find it?
In his Nobel Prize Lecture (11 Dec 1965), 'The Development of the Space-Time View of Quantum Electrodynamics'. Collected in Stig Lundqvist, Nobel Lectures: Physics, 1963-1970 (1998), 177.
A great deal of my work is just playing with equations and seeing what they give.
Quoted in Frank Wilczek, ',The Dirac Equation'. Proceedings of the Dirac Centennial Symposium (2003), 45.
A great department of thought must have its own inner life, however transcendent may be the importance of its relations to the outside. No department of science, least of all one requiring so high a degree of mental concentration as Mathematics, can be developed entirely, or even mainly, with a view to applications outside its own range. The increased complexity and specialisation of all branches of knowledge makes it true in the present, however it may have been in former times, that important advances in such a department as Mathematics can be expected only from men who are interested in the subject for its own sake, and who, whilst keeping an open mind for suggestions from outside, allow their thought to range freely in those lines of advance which are indicated by the present state of their subject, untrammelled by any preoccupation as to applications to other departments of science. Even with a view to applications, if Mathematics is to be adequately equipped for the purpose of coping with the intricate problems which will be presented to it in the future by Physics, Chemistry and other branches of physical science, many of these problems probably of a character which we cannot at present forecast, it is essential that Mathematics should be allowed to develop freely on its own lines.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 286.
A great man, [who] was convinced that the truths of political and moral science are capable of the same certainty as those that form the system of physical science, even in those branches like astronomy that seem to approximate mathematical certainty.
He cherished this belief, for it led to the consoling hope that humanity would inevitably make progress toward a state of happiness and improved character even as it has already done in its knowledge of the truth.
He cherished this belief, for it led to the consoling hope that humanity would inevitably make progress toward a state of happiness and improved character even as it has already done in its knowledge of the truth.
Describing administrator and economist Anne-Robert-Jacques Turgot in Essai sur l’application de l’analyse à la probabilité des décisions rendues à la pluralité des voix (1785), i. Cited epigraph in Charles Coulston Gillispie, Science and Polity in France: The End of the Old Regime (2004), 3
A great part of its [higher arithmetic] theories derives an additional charm from the peculiarity that important propositions, with the impress of simplicity on them, are often easily discovered by induction, and yet are of so profound a character that we cannot find the demonstrations till after many vain attempts; and even then, when we do succeed, it is often by some tedious and artificial process, while the simple methods may long remain concealed.
Quoted in H. Eves, Mathematical Circles (1977) .
A large part of mathematics which becomes useful developed with absolutely no desire to be useful, and in a situation where nobody could possibly know in what area it would become useful; and there were no general indications that it ever would be so.
From Address (1954) to Princeton Alumni, 'The Role of Mathematics in the Sciences and in Society', published in A.H. Taub (ed.), John von Neumann: Collected Works (1963), Vol. 6, 489. As quoted and cited in Rosemary Schmalz,Out of the Mouths of Mathematicians: A Quotation Book for Philomaths (1993), 123.
A large part of the training of the engineer, civil and military, as far as preparatory studies are concerned; of the builder of every fabric of wood or stone or metal designed to stand upon the earth, or bridge the stream, or resist or float upon the wave; of the surveyor who lays out a building lot in a city, or runs a boundary line between powerful governments across a continent; of the geographer, navigator, hydrographer, and astronomer,—must be derived from the mathematics.
In 'Academical Education', Orations and Speeches on Various Occasions (1870), Vol. 3, 513.
A marveilous newtrality have these things mathematicall, and also a strange participation between things supernaturall, immortall, intellectuall, simple and indivisible, and things naturall, mortall, sensible, componded and divisible.
— John Dee
In Mathematicall Praeface to the Elements of Geometrie of Euclid of Megara (1570).
A mathematical argument is, after all, only organized common sense, and it is well that men of science should not always expound their work to the few behind a veil of technical language, but should from time to time explain to a larger public the reasoning which lies behind their mathematical notation.
In The Tides and Kindred Phenomena in the Solar System: The Substance of Lectures Delivered in 1897 at the Lowell Institute, Boston, Massachusetts (1898), Preface, v.
Preface
A mathematical point is the most indivisble and unique thing which art can present.
Letters, 21. 1817. In Robert Édouard Moritz, Memorabilia Mathematica (1914), 295.
A mathematical problem should be difficult in order to entice us, yet not completely inaccessible, lest it mock at our efforts. It should be to us a guide post on the mazy paths to hidden truths, and ultimately a reminder of our pleasure in the successful solution.
In Mathematical Problems', Bulletin American Mathematical Society, 8, 438.
A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way.
In A Mathematician’s Apology (1940, 2012), 113.
A mathematical science is any body of propositions which is capable of an abstract formulation and arrangement in such a way that every proposition of the set after a certain one is a formal logical consequence of some or all the preceding propositions. Mathematics consists of all such mathematical sciences.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 222.
A mathematical theory is not to be considered complete until you have made it so clear that you can explain it to the first man whom you meet on the street.
…...
A mathematical truth is timeless, it does not come into being when we discover it. Yet its discovery is a very real event, it may be an emotion like a great gift from a fairy.
In 'Science and Religion', Mind and Matter (1958), collected in What is Life?: With Mind and Matter and Autobiographical Sketches (2012), 142.
A mathematician who can only generalise is like a monkey who can only climb UP a tree. ... And a mathematician who can only specialise is like a monkey who can only climb DOWN a tree. In fact neither the up monkey nor the down monkey is a viable creature. A real monkey must find food and escape his enemies and so must be able to incessantly climb up and down. A real mathematician must be able to generalise and specialise. ... There is, I think, a moral for the teacher. A teacher of traditional mathematics is in danger of becoming a down monkey, and a teacher of modern mathematics an up monkey. The down teacher dishing out one routine problem after another may never get off the ground, never attain any general idea. and the up teacher dishing out one definition after the other may never climb down from his verbiage, may never get down to solid ground, to something of tangible interest for his pupils.
From 'A Story With A Moral', Mathematical Gazette (Jun 1973), 57, No. 400, 86-87
A mind is accustomed to mathematical deduction, when confronted with the faulty foundations of astrology, resists a long, long time, like an obstinate mule, until compelled by beating and curses to put its foot into that dirty puddle.
As quoted in Arthur Koestler, The Sleep Walkers: A History of Man’s Changing Vision of the Universe (1959), 243, citing De Stella Nova in Pede Serpentarii (1606).
A modern branch of mathematics, having achieved the art of dealing with the infinitely small, can now yield solutions in other more complex problems of motion, which used to appear insoluble. This modern branch of mathematics, unknown to the ancients, when dealing with problems of motion, admits the conception of the infinitely small, and so conforms to the chief condition of motion (absolute continuity) and thereby corrects the inevitable error which the human mind cannot avoid when dealing with separate elements of motion instead of examining continuous motion. In seeking the laws of historical movement just the same thing happens. The movement of humanity, arising as it does from innumerable human wills, is continuous. To understand the laws of this continuous movement is the aim of history. … Only by taking an infinitesimally small unit for observation (the differential of history, that is, the individual tendencies of man) and attaining to the art of integrating them (that is, finding the sum of these infinitesimals) can we hope to arrive at the laws of history.
War and Peace (1869), Book 11, Chap. 1.
A modern mathematical proof is not very different from a modern machine, or a modern test setup: the simple fundamental principles are hidden and almost invisible under a mass of technical details.
Unterrichtsblätter für Mathematik und Naturwissenschaften (1932), 38, 177-188. As translated by Abe Shenitzer, in 'Part I. Topology and Abstract Algebra as Two Roads of Mathematical Comprehension', The American Mathematical Monthly (May 1995), 102, No. 7, 453.
A peculiar beauty reigns in the realm of mathematics, a beauty which resembles not so much the beauty of art as the beauty of nature and which affects the reflective mind, which has acquired an appreciation of it, very much like the latter.
From Berliner Monatsberichte (1867), 395. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 185.
A professor … may be to produce a perfect mathematical work of art, having every axiom stated, every conclusion drawn with flawless logic, the whole syllabus covered. This sounds excellent, but in practice the result is often that the class does not have the faintest idea of what is going on. … The framework is lacking; students do not know where the subject fits in, and this has a paralyzing effect on the mind.
In A Concrete Approach to Abstract Algebra (1959), 1-2.

A Russian with eyes on the stars,
Tsiolkovsky dreamed past the Mars,
With math as his guide,
He took in his stride,
To sketch out space voyages afar.
Tsiolkovsky dreamed past the Mars,
With math as his guide,
He took in his stride,
To sketch out space voyages afar.
Limerick created by Artificial Intelligence from: “Write a limerick about K.E. Tsiolkovsky, Russian pioneer space theorist,” and only two words tweaked by Webmaster (17 Sep 2023).
A short, broad man of tremendous vitality, the physical type of Hereward, the last of the English, and his brother-in-arms, Winter, Sylvester’s capacious head was ever lost in the highest cloud-lands of pure mathematics. Often in the dead of night he would get his favorite pupil, that he might communicate the very last product of his creative thought. Everything he saw suggested to him something new in the higher algebra. This transmutation of everything into new mathematics was a revelation to those who knew him intimately. They began to do it themselves. His ease and fertility of invention proved a constant encouragement, while his contempt for provincial stupidities, such as the American hieroglyphics for π and e, which have even found their way into Webster’s Dictionary, made each young worker apply to himself the strictest tests.
In Florian Cajori, Teaching and History of Mathematics in the United States (1890), 265.
A superficial knowledge of mathematics may lead to the belief that this subject can be taught incidentally, and that exercises akin to counting the petals of flowers or the legs of a grasshopper are mathematical. Such work ignores the fundamental idea out of which quantitative reasoning grows—the equality of magnitudes. It leaves the pupil unaware of that relativity which is the essence of mathematical science. Numerical statements are frequently required in the study of natural history, but to repeat these as a drill upon numbers will scarcely lend charm to these studies, and certainly will not result in mathematical knowledge.
In Primary Arithmetic: First Year, for the Use of Teachers (1897), 26-27.
A superficial knowledge of mathematics may lead to the belief that this subject can be taught incidentally, and that exercises akin to counting the petals of flowers or the legs of a grasshopper are mathematical. Such work ignores the fundamental idea out of which quantitative reasoning grows—the equality of magnitudes. It leaves the pupil unaware of that relativity which is the essence of mathematical science. Numerical statements are frequently required in the study of natural history, but to repeat these as a drill upon numbers will scarcely lend charm to these studies, and certainly will not result in mathematical knowledge.
In Primary Arithmetic: First Year, for the Use of Teachers (1897), 26-27.
A surprising proportion of mathematicians are accomplished musicians. Is it because music and mathematics share patterns that are beautiful?
In 'Introduction' contributed to Donald J. Albers and Gerald L. Alexanderson, More Mathematical People: Contemporary Conversations (1990), xi.
A teacher of mathematics has a great opportunity. If he fills his allotted time with drilling his students in routine operations he kills their interest, hampers their intellectual development, and misuses his opportunity. But if he challenges the curiosity of his students by setting them problems proportionate to their knowledge, and helps them to solve their problems with stimulating questions, he may give them a taste for, and some means of, independent thinking.
In How to Solve It (1948), Preface.
A theory of physics is not an explanation; it is a system of mathematical oppositions deduced from a small number of principles the aim of which is to represent as simply, as completely, and as exactly as possible, a group of experimental laws.
As quoted in Philipp Frank, Modern Science and its Philosophy (1949), 15, which cites Théorie Physique; Son Objet—Son Structure (1906), 24.
A theory with mathematical beauty is more likely to be correct than an ugly one that fits some experimental data. God is a mathematician of a very high order, and He used very advanced mathematics in constructing the universe.
In Scientific American (May 1963). As quoted and cited in The Hutchinson Encyclopedia of Science (1998), 468.
A thing is obvious mathematically after you see it.
Used as a filler, referring to Dean R.D. Carmichael, in Franz E. Hohn (ed.), Pi Mu Epsilon Journal (Fall 1956), 2, No. 5, 224. Carmichael was Dean of the Graduate School at the University of Illinois, from 1933 to his retirement in 1947. The journal was published at the University of Illinois. Webmaster has not yet found an earlier or primary source (can you help?) but would not rule out the quote being passed down by oral tradition at the university.
A troubling question for those of us committed to the widest application of intelligence in the study and solution of the problems of men is whether a general understanding of the social sciences will be possible much longer. Many significant areas of these disciplines have already been removed by the advances of the past two decades beyond the reach of anyone who does not know mathematics; and the man of letters is increasingly finding, to his dismay, that the study of mankind proper is passing from his hands to those of technicians and specialists. The aesthetic effect is admittedly bad: we have given up the belletristic “essay on man” for the barbarisms of a technical vocabulary, or at best the forbidding elegance of mathematical syntax.
Opening paragraph of 'The Study of Man: Sociology Learns the Language of Mathematics' in Commentary (1 Sep 1952). Reprinted in James Roy Newman, The World of Mathematics (1956), Vol. 2, 1294.
About the year 1821, I undertook to superintend, for the Government, the construction of an engine for calculating and printing mathematical and astronomical tables. Early in the year 1833, a small portion of the machine was put together, and was found to perform its work with all the precision which had been anticipated. At that period circumstances, which I could not control, caused what I then considered a temporary suspension of its progress; and the Government, on whose decision the continuance or discontinuance of the work depended, have not yet communicated to me their wishes on the question.
In The Ninth Bridgewater Treatise: A Fragment (1838), 186.
Abstractness, sometimes hurled as a reproach at mathematics, is its chief glory and its surest title to practical usefulness. It is also the source of such beauty as may spring from mathematics.
In 'General Prospectus', The Development of Mathematics (1940, 2017), Chap. 1, 9.
Abstruse mathematical researches … are … often abused for having no obvious physical application. The fact is that the most useful parts of science have been investigated for the sake of truth, and not for their usefulness. A new branch of mathematics, which has sprung up in the last twenty years, was denounced by the Astronomer Royal before the University of Cambridge as doomed to be forgotten, on account of its uselessness. Now it turns out that the reason why we cannot go further in our investigations of molecular action is that we do not know enough of this branch of mathematics.
In 'Conditions of Mental Development', Lectures and Essays (1901), Vol. 1, 115.
After an honest day’s work a mathematician goes off duty. Mathematics is very hard work, and dons tend to be above average in health and vigor. Below a certain threshold a man cracks up; but above it, hard mental work makes for health and vigor (also—on much historical evidence throughout the ages—for longevity). I have noticed lately that when I am working really hard I wake around 5.30 a.m. ready and eager to start; if I am slack, I sleep till I am called.
In 'The Mathematician’s Art of Work' (1967), collected in Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 195.
Again and again in reading even his [William Thomson] most abstract writings one is struck by the tenacity with which physical ideas control in him the mathematical form in which he expressed them. An instance of this is afforded by … an example of a mathematical result that is, in his own words, “not instantly obvious from the analytical form of my solution, but which we immediately see must be the case by thinking of the physical meaning of the result.”
As given in Life of Lord Kelvin (1910), Vol. 2, 1136. The ellipsis gives the reference to the quoted footnote, to a passage in his Mathematical and Physical Papers, Vol. 1, 457. [Note: William Thomson, later became Lord Kelvin. —Webmaster]
All science as it grows toward perfection becomes mathematical in its ideas.
In An Introduction to Mathematics (1911), 14. This is part of a longer quote that begins, “In modern times the belief that the ultimate explanation…”, on the Alfred North Whitehead Quotes page of this website.
All science requires mathematics.
[Editors' summary of Bacon's idea, not Bacon's wording.]
[Editors' summary of Bacon's idea, not Bacon's wording.]
These are not the exact words of Roger Bacon, but are from an editor's sub-heading, giving a summary for the topic of Chapter 2, for example, in Roger Bacon and Robert Belle Burke (ed.), Opus Maius (reproduction 2002), Vol. 1, Part 4, 117. Part 4 is devoted to a discourse on Mathematics. In its Chapter 1, as translated, Bacon states that 'There are four great sciences, without which the other sciences cannot be known nor a knowledge of things secured. ... Of these sciences the gate and key is mathematics.'
All the effects of Nature are only the mathematical consequences of a small number of immutable laws.
From the original French, “Tous les effets de la nature ne sont que résultats mathématiques d'un petit noinbre de lois immuables.”, in Oeuvres de Laplace, Vol. VII: Théorie des probabilités (1847), Introduction, cliv.
All the events which occur upon the earth result from Law: even those actions which are entirely dependent on the caprices of the memory, or the impulse of the passions, are shown by statistics to be, when taken in the gross, entirely independent of the human will. As a single atom, man is an enigma; as a whole, he is a mathematical problem. As an individual, he is a free agent; as a species, the offspring of necessity.
In The Martyrdom of Man (1876), 185-186.
All the mathematical sciences are founded on relations between physical laws and laws of numbers, so that the aim of exact science is to reduce the problems of nature to the determination of quantities by operations with numbers.
from Faraday's Lines of Force (1856)
All the modern higher mathematics is based on a calculus of operations, on laws of thought. All mathematics, from the first, was so in reality; but the evolvers of the modern higher calculus have known that it is so. Therefore elementary teachers who, at the present day, persist in thinking about algebra and arithmetic as dealing with laws of number, and about geometry as dealing with laws of surface and solid content, are doing the best that in them lies to put their pupils on the wrong track for reaching in the future any true understanding of the higher algebras. Algebras deal not with laws of number, but with such laws of the human thinking machinery as have been discovered in the course of investigations on numbers. Plane geometry deals with such laws of thought as were discovered by men intent on finding out how to measure surface; and solid geometry with such additional laws of thought as were discovered when men began to extend geometry into three dimensions.
In Lectures on the Logic of Arithmetic (1903), Preface, 18-19.
All the sciences have a relation, greater or less, to human nature; and...however wide any of them may seem to run from it, they still return back by one passage or another. Even Mathematics, Natural Philosophy, and Natural Religion, are in some measure dependent on the science of MAN; since they lie under the cognizance of men, and are judged of by their powers and faculties.
A Treatise on Human Nature (1739-40), ed. L. A. Selby-Bigge (1888), introduction, xix.
All the truths of mathematics are linked to each other, and all means of discovering them are equally admissible.
In article by Jean Itard, 'Legendre, Adrien-Marie', in Charles Coulston Gillespie (ed.), Dictionary of Scientific Biography (1973), Vol. 8, 142.
All things began in Order, so shall they end, and so shall they begin again, according to the Ordainer of Order, and the mystical mathematicks of the City of Heaven.
In 'Garden of Cyrus', Religio Medici and Other Writings (1909), 229.
Almost everything, which the mathematics of our century has brought forth in the way of original scientific ideas, attaches to the name of Gauss.
In Zahlentheorie, Teil 1 (1901), 43.
Although I was first drawn to math and science by the certainty they promised, today I find the unanswered questions and the unexpected connections at least as attractive.
In Warped Passages (2005), 65.
Although I was four years at the University [of Wisconsin], I did not take the regular course of studies, but instead picked out what I thought would be most useful to me, particularly chemistry, which opened a new world, mathematics and physics, a little Greek and Latin, botany and and geology. I was far from satisfied with what I had learned, and should have stayed longer.
[Enrolled in Feb 1861, left in 1863 without completing a degree, and began his first botanical foot journey.]
[Enrolled in Feb 1861, left in 1863 without completing a degree, and began his first botanical foot journey.]
The Story of My Boyhood and Youth (1913), 286.
Among all highly civilized peoples the golden age of art has always been closely coincident with the golden age of the pure sciences, particularly with mathematics, the most ancient among them.
This coincidence must not be looked upon as accidental, but as natural, due to an inner necessity. Just as art can thrive only when the artist, relieved of the anxieties of existence, can listen to the inspirations of his spirit and follow in their lead, so mathematics, the most ideal of the sciences, will yield its choicest blossoms only when life’s dismal phantom dissolves and fades away, when the striving after naked truth alone predominates, conditions which prevail only in nations while in the prime of their development.
This coincidence must not be looked upon as accidental, but as natural, due to an inner necessity. Just as art can thrive only when the artist, relieved of the anxieties of existence, can listen to the inspirations of his spirit and follow in their lead, so mathematics, the most ideal of the sciences, will yield its choicest blossoms only when life’s dismal phantom dissolves and fades away, when the striving after naked truth alone predominates, conditions which prevail only in nations while in the prime of their development.
From Die Entwickelung der Mathematik im Zusammenhange mit der Ausbreitung der Kultur (1893), 4. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 191-192. From the original German, “Bei allen Kulturvölkern ist die Blüthezeit der Kunst auch immer zeitlich eng verbunden mit einer Blüthezeit der reinen Wissenschaften, insbesondere der ältesten unter ihnen, der Mathematik.
Dieses Zusammentreffen dürfte auch nicht ein zufälliges, sondern ein natürliches, ein Ergebniss innerer Notwendigkeit sein. Wie die Kunst nur gedeihen kann, wenn der Künstler, unbekümmert um die Bedrängnisse des Daseins, den Eingebungen seines Geistes lauschen und ihnen folgen kann, so kann die idealste Wissenschaft, die Mathematik, erst dann ihre schönsten Blüthen treiben, wenn des Erdenlebens schweres Traumbild sinkt und sinkt und sinkt, wenn das Streben nach der nackten Wahrheit allein bestimmend ist, was nur bei Nationen in der Vollkraft ihrer Entwickelung vorkommt.”
Dieses Zusammentreffen dürfte auch nicht ein zufälliges, sondern ein natürliches, ein Ergebniss innerer Notwendigkeit sein. Wie die Kunst nur gedeihen kann, wenn der Künstler, unbekümmert um die Bedrängnisse des Daseins, den Eingebungen seines Geistes lauschen und ihnen folgen kann, so kann die idealste Wissenschaft, die Mathematik, erst dann ihre schönsten Blüthen treiben, wenn des Erdenlebens schweres Traumbild sinkt und sinkt und sinkt, wenn das Streben nach der nackten Wahrheit allein bestimmend ist, was nur bei Nationen in der Vollkraft ihrer Entwickelung vorkommt.”
Among the minor, yet striking characteristics of mathematics, may be mentioned the fleshless and skeletal build of its propositions; the peculiar difficulty, complication, and stress of its reasonings; the perfect exactitude of its results; their broad universality; their practical infallibility.
In Charles S. Peirce, Charles Hartshorne (ed.), Paul Weiss (ed.), Collected Papers of Charles Sanders Peirce (1931), Vol. 4, 197.
An announcement of [Christopher] Zeeman’s lecture at Northwestern University in the spring of 1977 contains a quote describing catastrophe theory as the most important development in mathematics since the invention of calculus 300 years ago.
In book review of Catastrophe Theory: Collected Papers, 1972-1977, in Bulletin of the American Mathematical Society (Nov 1978), 84, No. 6, 1360. Reprinted in Stephen Smale, Roderick Wong(ed.), The Collected Papers of Stephen Smale (2000), Vol. 2, 814.
An astronomer must be the wisest of men; his mind must be duly disciplined in youth; especially is mathematical study necessary; both an acquaintance with the doctrine of number, and also with that other branch of mathematics, which, closely connected as it is with the science of the heavens, we very absurdly call geometry, the measurement of the earth.
— Plato
From the 'Epilogue to the Laws' (Epinomis), 988-990. As quoted in William Whewell, History of the Inductive Sciences from the Earliest to the Present Time (1837), Vol. 1, 161. (Although referenced to Plato’s Laws, the Epinomis is regarded as a later addition, not by Plato himself.)
An essential [of an inventor] is a logical mind that sees analogies. No! No! not mathematical. No man of a mathematical habit of mind ever invented anything that amounted to much. He hasn’t the imagination to do it. He sticks too close to the rules, and to the things he is mathematically sure he knows, to create anything new.
As quoted in French Strother, 'The Modern Profession of Inventing', World's Work and Play (Jul 1905), 6, No. 32, 187.
An incidental remark from a German colleague illustrates the difference between Prussian ways and our own. He had apparently been studying the progress of our various crews on the river, and had been struck with the fact that though the masters in charge of the boats seemed to say and do very little, yet the boats went continually faster and faster, and when I mentioned Dr. Young’s book to him, he made the unexpected but suggestive reply: “Mathematics in Prussia! Ah, sir, they teach mathematics in Prussia as you teach your boys rowing in England: they are trained by men who have been trained by men who have themselves been trained for generations back.”
In John Perry (ed.), Discussion on the Teaching of Mathematics (1901), 43. The discussion took place on 14 Sep 1901 at the British Association at Glasgow, during a joint meeting of the mathematics and physics sections with the education section. The proceedings began with an address by John Perry. Langley related this anecdote during the Discussion which followed.
An old French geometer used to say that a mathematical theory was never to be considered complete till you had made it so clear that you could explain it to the first man you met in the street.
In Nature (1873), 8, 458.
And as for Mixed Mathematics, I may only make this prediction, that there cannot fail to be more kinds of them, as nature grows further disclosed.
In Advancement of Learning (1605), Book 2. Collected in The Works of Francis Bacon (1765), Vol. 1, 61.
And having thus passed the principles of arithmetic, geometry, astronomy, and geography, with a general compact of physics, they may descend in mathematics to the instrumental science of trigonometry, and from thence to fortification, architecture, engineering, or navigation. And in natural philosophy they may proceed leisurely from the history of meteors, minerals, plants, and living creatures, as far as anatomy. Then also in course might be read to them out of some not tedious writer the institution of physic. … To set forward all these proceedings in nature and mathematics, what hinders but that they may procure, as oft as shall be needful, the helpful experiences of hunters, fowlers, fishermen, shepherds, gardeners, apothecaries; and in other sciences, architects, engineers, mariners, anatomists.
In John Milton and Robert Fletcher (ed.), 'On Education', The Prose Works of John Milton: With an Introductory Review (1834), 100.
Angling may be said to be so like the Mathematics that it can never be fully learnt; at least not so fully but that there will still be more new experiments left for the trial of other men that succeed us.
In The Complete Angler (1653, 1915), 7.
Angling may be said to be so like the mathematics, that it can never be fully learnt.
In Izaak Walton and Charles Cotton, 'Walton to the Reader', The Complete Angler (1653, 1824), Vol. 1, lxv.
Another advantage of a mathematical statement is that it is so definite that it might be definitely wrong; and if it is found to be wrong, there is a plenteous choice of amendments ready in the mathematicians’ stock of formulae. Some verbal statements have not this merit; they are so vague that they could hardly be wrong, and are correspondingly useless.
From 'Mathematics of War and Foreign Politics', in James R. Newman, The World of Mathematics (1956), Vol. 2, 1248.
Another characteristic of mathematical thought is that it can have no success where it cannot generalize.
In Eberhard Zeidler, Applied Functional Analysis: main principles and their applications (1995), 282.
Another diversity of Methods is according to the subject or matter which is handled; for there is a great difference in delivery of the Mathematics, which are the most abstracted of knowledges, and Policy, which is the most immersed…, yet we see how that opinion, besides the weakness of it, hath been of ill desert towards learning, as that which taketh the way to reduce learning to certain empty and barren generalities; being but the very husks and shells of sciences, all the kernel being forced out and expulsed with the torture and press of the method.
Advancement of Learning, Book 2. In James Spedding, The Works of Francis Bacon (1863), Vol. 6, 292-293. Peter Pešić, explains that 'By Mathematics, he had in mind a sterile and rigid scheme of logical classifications, called dichotomies in his time,' inLabyrinth: A Search for the Hidden Meaning of Science (2001), 73.
Another great and special excellence of mathematics is that it demands earnest voluntary exertion. It is simply impossible for a person to become a good mathematician by the happy accident of having been sent to a good school; this may give him a preparation and a start, but by his own individual efforts alone can he reach an eminent position.
In Conflict of Studies (1873), 2.
Any conception which is definitely and completely determined by means of a finite number of specifications, say by assigning a finite number of elements, is a mathematical conception. Mathematics has for its function to develop the consequences involved in the definition of a group of mathematical conceptions. Interdependence and mutual logical consistency among the members of the group are postulated, otherwise the group would either have to be treated as several distinct groups, or would lie beyond the sphere of mathematics.
In 'Mathematics', Encyclopedia Britannica (9th ed.).
Anyone who cannot cope with mathematics is not fully human. At best he is a tolerable subhuman who has learned to wear shoes, bathe and not make messes in the house
In Time Enough for Love: The Lives of Lazarus Long (1973), 265.
Anyone who has had actual contact with the making of the inventions that built the radio art knows that these inventions have been the product of experiment and work based on physical reasoning, rather than on the mathematicians' calculations and formulae. Precisely the opposite impression is obtained from many of our present day text books and publications.
Attributed.
Anything at all that can be the object of scientific thought becomes dependent on the axiomatic method, and thereby indirectly on mathematics, as soon as it is ripe for the formation of a theory. By pushing ahead to ever deeper layers of axioms … we become ever more conscious of the unity of our knowledge. In the sign of the axiomatic method, mathematics is summoned to a leading role in science.
Address (11 Sep 1917), 'Axiomatisches Denken' delivered before the Swiss Mathematical Society in
Zürich. Translated by Ewald as 'Axiomatic Thought', (1918), in William Bragg Ewald, From Kant to Hilbert (1996), Vol. 2, 1115.
Archimedes will be remembered when Aeschylus is forgotten, because languages die and mathematical ideas do not. “Immortality” may be a silly word, but probably a mathematician has the best chance of whatever it may mean.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 81.
Archimedes, who combined a genius for mathematics with a physical insight, must rank with Newton, who lived nearly two thousand years later, as one of the founders of mathematical physics. … The day (when having discovered his famous principle of hydrostatics he ran through the streets shouting Eureka! Eureka!) ought to be celebrated as the birthday of mathematical physics; the science came of age when Newton sat in his orchard.
In An Introduction to Mathematics (1911), 37.
Arithmetically speaking, rabbits multiply faster than adders add.
In Evan Esar, 20,000 Quips and Quotes, 509.
Art is an expression of the world order and is, therefore, orderly, organic, subject to mathematical law, and susceptible to mathematical analysis.
In 'The Theosophic View of the Art of Architecture', The Beautiful Necessity, Seven Essays on Theosophy and Architecture (2nd ed., 1922), Preface to the Second Edition, 11.
Art is usually considered to be not of the highest quality if the desired object is exhibited in the midst of unnecessary lumber.
In Mathematics: Queen and Servant of Sciences (1938), 20. Bell is writing about the postulational method and the art of pruning a set of postulates to bare essentials without internal duplication.
As a little boy, I showed an abnormal aptitude for mathematics this gift played a horrible part in tussles with quinsy or scarlet fever, when I felt enormous spheres and huge numbers swell relentlessly in my aching brain.
In Speak, Memory: An Autobiography Revisited (1999), 2
As an Art, Mathematics has its own standard of beauty and elegance which can vie with the more decorative arts. In this it is diametrically opposed to a Baroque art which relies on a wealth of ornamental additions. Bereft of superfluous addenda, Mathematics may appear, on first acquaintance, austere and severe. But longer contemplation reveals the classic attributes of simplicity relative to its significance and depth of meaning.
In The Skeleton Key of Mathematics (1949), 12.
As an exercise of the reasoning faculty, pure mathematics is an admirable exercise, because it consists of reasoning alone, and does not encumber the student with an exercise of judgment: and it is well to begin with learning one thing at a time, and to defer a combination of mental exercises to a later period.
In Annotations to Bacon’s Essays (1873), Essay 1, 493.
As far as the laws of mathematics refer to reality, they are not certain; and as far as they are certain, they do not refer to reality.
Sidelights on Relativity (1920), 28.
As for everything else, so for a mathematical theory: beauty can be perceived but not explained.
President’s address (1883) to the British Association for the Advancement of Science, in The Collected Mathematical Papers (1895), Vol. 8, xxii.
As for the place of mathematics in relation to other sciences, mathematics can be seen as a big warehouse full of shelves. Mathematicians put things on the shelves and guarantee that they are true. They also explain how to use them and how to reconstruct them. Other sciences come and help themselves from the shelves; mathematicians are not concerned with what they do with what they have taken. This metaphor is rather coarse, but it reflects the situation well enough.
From interview with Marc Kirch, 'My First Fifty years at the Collège de France', collected in Helge Holden and Ragni Piene, The Abel Prize: 2003-2007 The First Five Years (2009), 15-29.
As history proves abundantly, mathematical achievement, whatever its intrinsic worth, is the most enduring of all.
In A Mathematician’s Apology (1940, 1967), 80.
As in Mathematicks, so in Natural Philosophy, the Investigation of difficult Things by the Method of Analysis, ought ever to precede the Method of Composition. This Analysis consists in making Experiments and Observations, and in drawing general Conclusions from them by Induction, and admitting of no Objections against the Conclusions, but such as are taken from Experiments, or other certain Truths. For Hypotheses are not to be regarded in experimental Philosophy.
From Opticks, (1704, 2nd ed. 1718), Book 3, Query 31, 380.
As in the domains of practical life so likewise in science there has come about a division of labor. The individual can no longer control the whole field of mathematics: it is only possible for him to master separate parts of it in such a manner as to enable him to extend the boundaries of knowledge by creative research.
In Die reine Mathematik in den Jahren 1884-99, 10. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 94.
As pure truth is the polar star of our science [mathematics], so it is the great advantage of our science over others that it awakens more easily the love of truth in our pupils. … If Hegel justly said, “Whoever does not know the works of the ancients, has lived without knowing beauty,” Schellbach responds with equal right, “Who does not know mathematics, and the results of recent scientific investigation, dies without knowing truth.”
From Didaktik und Methodik des Rechnens und der Mathematik (1908), 37. As quoted and translated in J.W.A. Young, Teaching of Mathematics in the Elementary and the Secondary School (1907), 44. From the original German, “Wenn Hegel mit Recht sagt: ‘Wer die Werke der Alten nicht kennt, der hat gelebt, ohne die Schönheit gekannt zu haben’, so erwidert Schellbach mit nicht minderem Recht: ‘Wer die Math. und die Resultate der neueren Naturforschung nicht gekannt hat, der stirbt, ohne die Wahrheit zu kennen.’”
As regards authority I so proceed. Boetius says in the second prologue to his Arithmetic, “If an inquirer lacks the four parts of mathematics, he has very little ability to discover truth.” And again, “Without this theory no one can have a correct insight into truth.” And he says also, “I warn the man who spurns these paths of knowledge that he cannot philosophize correctly.” And Again, “It is clear that whosoever passes these by, has lost the knowledge of all learning.”
Opus Majus [1266-1268], Part IV, distinction I, chapter I, trans. R. B. Burke, The Opus Majus of Roger Bacon (1928), Vol. I, 117.
As the prerogative of Natural Science is to cultivate a taste for observation, so that of Mathematics is, almost from the starting point, to stimulate the faculty of invention.
In 'A Plea for the Mathematician', Nature, 1, 261 in Collected Mathematical Papers, Vol. 2 (1908), 717.
As there is no study which may be so advantageously entered upon with a less stock of preparatory knowledge than mathematics, so there is none in which a greater number of uneducated men have raised themselves, by their own exertions, to distinction and eminence. … Many of the intellectual defects which, in such cases, are commonly placed to the account of mathematical studies, ought to be ascribed to the want of a liberal education in early youth.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 3, 183.
As to the need of improvement there can be no question whilst the reign of Euclid continues. My own idea of a useful course is to begin with arithmetic, and then not Euclid but algebra. Next, not Euclid, but practical geometry, solid as well as plane; not demonstration, but to make acquaintance. Then not Euclid, but elementary vectors, conjoined with algebra, and applied to geometry. Addition first; then the scalar product. Elementary calculus should go on simultaneously, and come into vector algebraic geometry after a bit. Euclid might be an extra course for learned men, like Homer. But Euclid for children is barbarous.
Electro-Magnetic Theory (1893), Vol. 1, 148. In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 130.
Astronomy and Pure Mathematics are the magnetic poles toward which the compass of my mind ever turns.
In Letter to Bolyai (30 Jun 1803), in Franz Schmidt and Paul Stäckel, Briefwechsel zwischen Carl Friedrich Gauss und Wolfgang Bolyai, (1899), Letter XXIII , 55.
At every major step physics has required, and frequently stimulated, the introduction of new mathematical tools and concepts. Our present understanding of the laws of physics, with their extreme precision and universality, is only possible in mathematical terms.
In Book Review 'Pulling the Strings,' of Lawrence Krauss's Hiding in the Mirror: The Mysterious Lure of Extra Dimensions, from Plato to String Theory and Beyond in Nature (22 Dec 2005), 438, 1081.
At the present time it is of course quite customary for physicists to trespass on chemical ground, for mathematicians to do excellent work in physics, and for physicists to develop new mathematical procedures. … Trespassing is one of the most successful techniques in science.
In Dynamics in Psychology (1940, 1973), 116.
Bacon himself was very ignorant of all that had been done by mathematics; and, strange to say, he especially objected to astronomy being handed over to the mathematicians. Leverrier and Adams, calculating an unknown planet into a visible existence by enormous heaps of algebra, furnish the last comment of note on this specimen of the goodness of Bacon’s view… . Mathematics was beginning to be the great instrument of exact inquiry: Bacon threw the science aside, from ignorance, just at the time when his enormous sagacity, applied to knowledge, would have made him see the part it was to play. If Newton had taken Bacon for his master, not he, but somebody else, would have been Newton.
In Budget of Paradoxes (1872), 53-54.
Be very vigilent over thy Childe … If he chuse the profession of a Scholler, advise him to study the most profitable Arts: Poetry, and the Mathematichs, take up too great a latitude of the Soule, and moderately used, are good Recreations, but bad Callings, bring nothing but their owne Reward.
From Alexander B. Grosart (ed), 'Enchyridion: The Fourth Book' (1641), The Complete Works in Prose and Verse of Francis Quarles (1880), Vol. 1, 48, Cap. XCIX.
Beauty is the first test: there is no permanent place in the world for ugly mathematics.
In A Mathematician’s Apology (1940, reprint with Foreward by C.P. Snow 1992), 85.
Before an experiment can be performed, it must be planned—the question to nature must be formulated before being posed. Before the result of a measurement can be used, it must be interpreted—nature's answer must be understood properly. These two tasks are those of the theorist, who finds himself always more and more dependent on the tools of abstract mathematics. Of course, this does not mean that the experimenter does not also engage in theoretical deliberations. The foremost classical example of a major achievement produced by such a division of labor is the creation of spectrum analysis by the joint efforts of Robert Bunsen, the experimenter, and Gustav Kirchoff, the theorist. Since then, spectrum analysis has been continually developing and bearing ever richer fruit.
'The Meaning and Limits of Exact Science', Science (30 Sep 1949), 110, No. 2857, 325. Advance reprinting of chapter from book Max Planck, Scientific Autobiography (1949), 110.
Before the introduction of the Arabic notation, multiplication was difficult, and the division even of integers called into play the highest mathematical faculties. Probably nothing in the modern world could have more astonished a Greek mathematician than to learn that, under the influence of compulsory education, the whole population of Western Europe, from the highest to the lowest, could perform the operation of division for the largest numbers. This fact would have seemed to him a sheer impossibility. … Our modern power of easy reckoning with decimal fractions is the most miraculous result of a perfect notation.
In Introduction to Mathematics (1911), 59.
Before you generalize, formalize, and axiomatize there must be mathematical substance.
In Eberhard Zeidler, Applied Functional Analysis: main principles and their applications (1995), 282.
Being a language, mathematics may be used not only to inform but also, among other things, to seduce.
From Fractals: Form, Chance and Dimension (1977), 20.
Bertrand, Darboux, and Glaisher have compared Cayley to Euler, alike for his range, his analytical power, and, not least, for his prolific production of new views and fertile theories. There is hardly a subject in the whole of pure mathematics at which he has not worked.
In Proceedings of London Royal Society (1895), 58, 21.
Besides a mathematical inclination, an exceptionally good mastery of one’s native tongue is the most vital asset of a competent programmer.
…...
Besides accustoming the student to demand, complete proof, and to know when he has not obtained it, mathematical studies are of immense benefit to his education by habituating him to precision. It is one of the peculiar excellencies of mathematical discipline, that the mathematician is never satisfied with à peu près. He requires the exact truth. Hardly any of the non-mathematical sciences, except chemistry, has this advantage. One of the commonest modes of loose thought, and sources of error both in opinion and in practice, is to overlook the importance of quantities. Mathematicians and chemists are taught by the whole course of their studies, that the most fundamental difference of quality depends on some very slight difference in proportional quantity; and that from the qualities of the influencing elements, without careful attention to their quantities, false expectation would constantly be formed as to the very nature and essential character of the result produced.
In An Examination of Sir William Hamilton’s Philosophy (1878), 611. [The French phrase, à peu près means “approximately”. —Webmaster]
Boltzmann was both a wizard of a mathematician and a physicist of international renown. The magnitude of his output of scientific papers was positively unnerving. He would publish two, three, sometimes four monographs a year; each one was forbiddingly dense, festooned with mathematics, and as much as a hundred pages in length.
In 'The Bulldog: A Profile of Ludwig Boltzmann', The American Scholar (1 Jan 1999), 99.
Bolyai [Janos] projected a universal language for speech as we have it for music and mathematics.
In János Bolyai, Science Absolute of Space, translated from the Latin by George Bruce Halsted (1896), Translator's Introduction, xxix.
Büchsel in his reminiscences from the life of a country parson relates that he sought his recreation in Lacroix’s Differential Calculus and thus found intellectual refreshment for his calling. Instances like this make manifest the great advantage which occupation with mathematics affords to one who lives remote from the city and is compelled to forego the pleasures of art. The entrancing charm of mathematics, which captivates every one who devotes himself to it, and which is comparable to the fine frenzy under whose ban the poet completes his work, has ever been incomprehensible to the spectator and has often caused the enthusiastic mathematician to be held in derision. A classic illustration is the example of Archimedes….
From Die Entwickelung der Mathematik im Zusammenhange mit der Ausbreitung der Kultur (1893), 22. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 186. From the original German, “Wenn Büchsel in seinen Erinnerungen aus dem Leben eines Landgeistlichen erzählt, dass er in der Differentialrechnung von Lacroix Erholung gesucht und geistige Erfrischung ftir seinen Beruf gefunden habe, so erkennen wir darin den grossen Vorzug, den die Beschaftigung mit der Mathematik für jemanden hat, der fern von einer Stadt lebt und auf ihre Kunstgenüsse verzichten muss. Der berückende Zauber der
Mathematik, dem jeder unterliegt, der sich ihr ergiebt, und der dem holden Wahnsinn vergleichbar ist, unter dessen Bann der Dichter sein Work vollendet, ist dem betrachtenden Mitmenschen immer unbegreiflich gewesen und hat den begeisterten Mathematiker oft zum Gespött werden lassen. Als klassisches Beispiel wird jedem Schüler Archimedes…”

Business, to be successful, must be based on science, for demand and supply are matters of mathematics, not guesswork.
The Book of Business (1913), 56.
But indeed, the English generally have been very stationary in latter times, and the French, on the contrary, so active and successful, particularly in preparing elementary books, in the mathematical and natural sciences, that those who wish for instruction, without caring from what nation they get it, resort universally to the latter language.
Letter (29 Jan 1824) to Patrick K. Rodgers. Collected in Andrew A. Lipscomb (ed.), The Writings of Thomas Jefferson (1904), Vol. 16, 2.
But it is precisely mathematics, and the pure science generally, from which the general educated public and independent students have been debarred, and into which they have only rarely attained more than a very meagre insight. The reason of this is twofold. In the first place, the ascendant and consecutive character of mathematical knowledge renders its results absolutely insusceptible of presentation to persons who are unacquainted with what has gone before, and so necessitates on the part of its devotees a thorough and patient exploration of the field from the very beginning, as distinguished from those sciences which may, so to speak, be begun at the end, and which are consequently cultivated with the greatest zeal. The second reason is that, partly through the exigencies of academic instruction, but mainly through the martinet traditions of antiquity and the influence of mediaeval logic-mongers, the great bulk of the elementary text-books of mathematics have unconsciously assumed a very repellant form,—something similar to what is termed in the theory of protective mimicry in biology “the terrifying form.” And it is mainly to this formidableness and touch-me-not character of exterior, concealing withal a harmless body, that the undue neglect of typical mathematical studies is to be attributed.
In Editor’s Preface to Augustus De Morgan and Thomas J. McCormack (ed.), Elementary Illustrations of the Differential and Integral Calculus (1899), v.
But of this I can assure you that there is not a movement of any body of Men however small whether on Horse-back or on foot, nor an operation or March of any description nor any Service in the field that is not formed upon some mathematical principle, and in the performance of which the knowledge and practical application of the mathematicks will be found not only useful but necessary. The application of the Mathematicks to Gunnery, Fortification, Tactics, the survey and knowledge of formal Castrenantion etc. cannot be acquired without study.
Duke of Wellington to his son Douro (1826). Quoted in A Selection of the Private Correspondence of the First Duke of Wellington (1952), 44.
But the creative principle resides in mathematics. In a certain sense, therefore, I hold it true that pure thought can grasp reality, as the ancients dreamed.
From Herbert Spencer Lecture, at University of Oxford (10 Jun 1933), 'On the Methods of Theoretical Physics'. Printed in Philosophy of Science, (Apr 1934), 1, No. 2. Quoted and cited in epigraph, A. H. Louie, More Than Life Itself: A Synthetic Continuation in Relational Biology (2013), 81.
Buttercups do not think, yet they are also built of mathematics. If buttercups do not cogitate, but we do, yet are built of the same ultimate stuff, then the difference must lie in the complexity of our structures that has emerged from the process of evolution.
In Creation Revisited: The Origin of Space, Time and the Universe (1992), 119.
By and large it is uniformly true in mathematics that there is a time lapse between a mathematical discovery and the moment when it is useful; and that this lapse of time can be anything from 30 to 100 years, in some cases even more.
From Address (1954) to Princeton Alumni, 'The Role of Mathematics in the Sciences and in Society', published in A.H. Taub (ed.), John von Neumann: Collected Works (1963), Vol. 6, 489. As quoted and cited in Rosemary Schmalz,Out of the Mouths of Mathematicians: A Quotation Book for Philomaths (1993), 123.
By keenly confronting the enigmas that surround us, and by considering and analyzing the observations that I had made I ended up in the domain of mathematics.
In M.C. Escher: The Graphic Work (1978), 8.
Can science ever be immune from experiments conceived out of prejudices and stereotypes, conscious or not? (Which is not to suggest that it cannot in discrete areas identify and locate verifiable phenomena in nature.) I await the study that says lesbians have a region of the hypothalamus that resembles straight men and I would not be surprised if, at this very moment, some scientist somewhere is studying brains of deceased Asians to see if they have an enlarged ‘math region’ of the brain.
— Kay Diaz
…...
Catastrophe Theory is a new mathematical method for describing the evolution of forms in nature. … It is particularly applicable where gradually changing forces produce sudden effects. We often call such effects catastrophes, because our intuition about the underlying continuity of the forces makes the very discontinuity of the effects so unexpected, and this has given rise to the name.
From Catastrophe Theory: Selected Papers, 1972-1977 (1977), 1. As quoted and cited in a Review by: Hector J. Sussmann, SIAM Review (Apr 1979), 21, No. 2, 269.
Cauchy is mad, and there is no way of being on good terms with him, although at present he is the only man who knows how mathematics should be treated. What he does is excellent, but very confused…
In Oeuvres (1826), Vol. 2, 259. As quoted and cited in Ernst Hairer and Gerhard Wanner Analysis by Its History (2008), 188. From the original French, “Cauchy est fou, et avec lui il n’y a pas moyen de s’entendre, bien que pour le moment il soit celui qui sait comment les mathématiques doivent être traitées. Ce qu’il fait est excellent, mais très brouillé….”
Cayley was singularly learned in the work of other men, and catholic in his range of knowledge. Yet he did not read a memoir completely through: his custom was to read only so much as would enable him to grasp the meaning of the symbols and understand its scope. The main result would then become to him a subject of investigation: he would establish it (or test it) by algebraic analysis and, not infrequently, develop it so to obtain other results. This faculty of grasping and testing rapidly the work of others, together with his great knowledge, made him an invaluable referee; his services in this capacity were used through a long series of years by a number of societies to which he was almost in the position of standing mathematical advisor.
In Proceedings of London Royal Society (1895), 58, 11-12.
Characteristically skeptical of the idea that living things would faithfully follow mathematical formulas, [Robert Harper] seized upon factors in corn which seemed to blend in the hybrid—rather than be represented by plus or minus signs, and put several seasons into throwing doubt upon the concept of immutable hypothetical units of inheritance concocted to account for selected results.
In 'Robert Almer Harper', National Academy Biographical Memoirs (1948), 25, 233-234.
Chemical engineering is the profession in which a knowledge of mathematics, chemistry and other natural sciences gained by study, experience and practice is applied with judgment to develop economic ways of using materials and energy for the benefit of mankind.
— AIChE
In Article III, 'Definition of the Profession', Constitution of the American Institute of
Chemical Engineers (as amended 17 Jan 2003). The same wording is found in the 1983 Constitution, as quoted in Nicholas A. Peppas (ed.), One Hundred Years of Chemical Engineering: From Lewis M. Norton (M.I.T. 1888) to Present (2012), 334.
Chess combines the beauty of mathematical structure with the recreational delights of a competitive game.
In 'Preface', Mathematics, Magic, and Mystery (1956), ix.
Chess problems are the hymn-tunes of mathematics.
'A Mathematician's Apology', in James Roy Newman, The World of Mathematics (2000), 2028.
Children are told that an apple fell on Isaac Newton’s head and he was led to state the law of gravity. This, of course, is pure foolishness. What Newton discovered was that any two particles in the universe attract each other with a force that is proportional to the product of their masses and inversely proportional to the square of the distance between them. This is not learned from a falling apple, but by observing quantities of data and developing a mathematical theory that can be verified by additional data. Data gathered by Galileo on falling bodies and by Johannes Kepler on motions of the planets were invaluable aids to Newton. Unfortunately, such false impressions about science are not universally outgrown like the Santa Claus myth, and some people who don’t study much science go to their graves thinking that the human race took until the mid-seventeenth century to notice that objects fall.
In How to Tell the Liars from the Statisticians (1983), 127.
Classes and concepts may, however, also be conceived as real objects, namely classes as “pluralities of things” or as structures consisting of a plurality of things and concepts as the properties and relations of things existing independently of our definitions and constructions. It seems to me that the assumption of such objects is quite as legitimate as the assumption of physical bodies and there is quite as much reason to believe in their existence. They are in the same sense necessary to obtain a satisfactory system of mathematics as physical bodies are necessary for a satisfactory theory of our sense perceptions…
In 'Russell's Mathematical Logic', in P.A. Schilpp (ed.), The Philosophy of Bertrand Russell (1944), Vol. 1, 137.
Common integration is only the memory of differentiation...
Confined to its true domain, mathematical reasoning is admirably adapted to perform the universal office of sound logic: to induce in order to deduce, in order to construct. … It contents itself to furnish, in the most favorable domain, a model of clearness, of precision, and consistency, the close contemplation of which is alone able to prepare the mind to render other conceptions also as perfect as their nature permits. Its general reaction, more negative than positive, must consist, above all, in inspiring us everywhere with an invincible aversion for vagueness, inconsistency, and obscurity, which may always be really avoided in any reasoning whatsoever, if we make sufficient effort.
In Synthèse Subjective (1856), 98. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 202-203. From the original French, “Bornée à son vrai domaine, la raison mathématique y peut admirablement remplir l’office universel de la saine logique: induire pour déduire, afin de construire. … Elle se contente de former, dans le domaine le plus favorable, un type de clarté, de précision, et de consistance, dont la contemplation familière peut seule disposer l’esprit à rendre les autres conceptions aussi parfaites que le comporte leur nature. Sa réaction générale, plus négative que positive, doit surtout consister à nous inspirer partout une invincible répugnance pour le vague, l’incohérence, et l’obscurité, que nous pouvons réellement éviter envers des pensées quelconques, si nous y faisons assez d’efforts.”
Coterminous with space and coeval with time is the kingdom of Mathematics; within this range her dominion is supreme; otherwise than according to her order nothing can exist; in contradiction to her laws nothing takes place. On her mysterious scroll is to be found written for those who can read it that which has been, that which is, and that which is to come.
From Presidential Address (Aug 1878) to the British Association, Dublin, published in the Report of the 48th Meeting of the British Association for the Advancement of Science (1878), 31.
Deductivism in mathematical literature and inductivism in scientific papers are simply the postures we choose to be seen in when the curtain goes up and the public sees us. The theatrical illusion is shattered if we ask what goes on behind the scenes. In real life discovery and justification are almost always different processes.
Induction and Intuition in Scientific Thought (1969), 26.
Definition of Mathematics.—It has now become apparent that the traditional field of mathematics in the province of discrete and continuous number can only be separated from the general abstract theory of classes and relations by a wavering and indeterminate line. Of course a discussion as to the mere application of a word easily degenerates into the most fruitless logomachy. It is open to any one to use any word in any sense. But on the assumption that “mathematics” is to denote a science well marked out by its subject matter and its methods from other topics of thought, and that at least it is to include all topics habitually assigned to it, there is now no option but to employ “mathematics” in the general sense of the “science concerned with the logical deduction of consequences from the general premisses of all reasoning.”
In article 'Mathematics', Encyclopedia Britannica (1911, 11th ed.), Vol. 17, 880. In the 2006 DVD edition of the encyclopedia, the definition of mathematics is given as “The science of structure, order, and relation that has evolved from elemental practices of counting, measuring, and describing the shapes of objects.” [Premiss is a variant form of “premise”. —Webmaster]
Descartes is the completest type which history presents of the purely mathematical type of mind—that in which the tendencies produced by mathematical cultivation reign unbalanced and supreme.
In An Examination of Sir William Hamilton’s Philosophy (1878), 626.
Difficulties [in defining mathematics with full generality, yet simplicity] are but consequences of our refusal to see that mathematics cannot be defined without acknowledging its most obvious feature: namely, that it is interesting. Nowhere is intellectual beauty so deeply felt and fastidiously appreciated.
In Personal Knowledge (1958, 2012), 200,
Dirichlet was not satisfied to study Gauss’ Disquisitiones arithmetical once or several times, but continued throughout life to keep in close touch with the wealth of deep mathematical thoughts which it contains by perusing it again and again. For this reason the book was never placed on the shelf but had an abiding place on the table at which he worked. … Dirichlet was the first one, who not only fully understood this work, but made it also accessible to others.
In Dirichlet, Werke, Bd. 2, 315. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 159.
Distrust even Mathematics; albeit so sublime and highly perfected, we have here a machine of such delicacy it can only work in vacuo, and one grain of sand in the wheels is enough to put everything out of gear. One shudders to think to what disaster such a grain of sand may bring a Mathematical brain. Remember Pascal.
The Garden of Epicurus (1894) translated by Alfred Allinson, in The Works of Anatole France in an English Translation (1920), 187.
Do not imagine that mathematics is harsh and crabbed, and repulsive to common sense. It is merely the etherealisation of common sense.
'The Six Gateways of Knowledge', Presidential Address to the Birmingham and Midland Institute, Birmingham (3 Oct 1883). In Popular Lectures and Addresses (1891), Vol. 1, 280.
Do not worry about your difficulties in Mathematics. I can assure you mine are still greater.
In letter (7 Jan 1943) to Barbara Wilson, a junior high school student, who had difficulties in school with mathematics. In Einstein Archives, 42-606. Quoted in Alice Calaprice, Dear Professor Einstein: Albert Einstein's Letters to and from Children (2002), 140.
Do not worry about your problems in mathematics. I assure you, my problems with mathematics are much greater than yours.
…...
Don’t talk to me of your Archimedes’ lever. He was an absent-minded person with a mathematical imagination. Mathematics commands all my respect, but I have no use for engines. Give me the right word and the right accent and I will move the world.
In 'Preface', A Personal Record (1912), 2.
Doubtless the reasoning faculty, the mind, is the leading and characteristic attribute of the human race. By the exercise of this, man arrives at the properties of the natural bodies. This is science, properly and emphatically so called. It is the science of pure mathematics; and in the high branches of this science lies the truly sublime of human acquisition. If any attainment deserves that epithet, it is the knowledge, which, from the mensuration of the minutest dust of the balance, proceeds on the rising scale of material bodies, everywhere weighing, everywhere measuring, everywhere detecting and explaining the laws of force and motion, penetrating into the secret principles which hold the universe of God together, and balancing worlds against worlds, and system against system. When we seek to accompany those who pursue studies at once so high, so vast, and so exact; when we arrive at the discoveries of Newton, which pour in day on the works of God, as if a second fiat had gone forth from his own mouth; when, further, we attempt to follow those who set out where Newton paused, making his goal their starting-place, and, proceeding with demonstration upon demonstration, and discovery upon discovery, bring new worlds and new systems of worlds within the limits of the known universe, failing to learn all only because all is infinite; however we may say of man, in admiration of his physical structure, that “in form and moving he is express and admirable,” it is here, and here without irreverence, we may exclaim, “In apprehension how like a god!” The study of the pure mathematics will of course not be extensively pursued in an institution, which, like this [Boston Mechanics’ Institute], has a direct practical tendency and aim. But it is still to be remembered, that pure mathematics lie at the foundation of mechanical philosophy, and that it is ignorance only which can speak or think of that sublime science as useless research or barren speculation.
In Works (1872), Vol. 1, 180.
During the last two centuries and a half, physical knowledge has been gradually made to rest upon a basis which it had not before. It has become mathematical. The question now is, not whether this or that hypothesis is better or worse to the pure thought, but whether it accords with observed phenomena in those consequences which can be shown necessarily to follow from it, if it be true
In Augustus De Morgan and Sophia Elizabeth De Morgan (ed.), A Budget of Paradoxes (1872), 2.
During the three years which I spent at Cambridge my time was wasted, as far as the academical studies were concerned…. I attempted mathematics, … but I got on very slowly. The work was repugnant to me, chiefly from my not being able to see any meaning in the early steps in algebra. This impatience was very foolish…
In Charles Darwin and Francis Darwin (ed.), 'Autobiography', The Life and Letters of Charles Darwin (1887, 1896), Vol. 1, 40.
Each generation has its few great mathematicians, and mathematics would not even notice the absence of the others. They are useful as teachers, and their research harms no one, but it is of no importance at all. A mathematician is great or he is nothing.
Reflections: Mathematics and Creativity', New Yorker (1972), 47, No. 53, 39-45. In Douglas M. Campbell, John C. Higgins (eds.), Mathematics: People, Problems, Results (1984), Vol. 2, 3.
Each thing in the world has names or unnamed relations to everything else. Relations are infinite in number and kind. To be is to be related. It is evident that the understanding of relations is a major concern of all men and women. Are relations a concern of mathematics? They are so much its concern that mathematics is sometimes defined to be the science of relations.
In Mole Philosophy and Other Essays (1927), 94-95.
Education is like a diamond with many facets: It includes the basic mastery of numbers and letters that give us access to the treasury of human knowledge, accumulated and refined through the ages; it includes technical and vocational training as well as instruction in science, higher mathematics, and humane letters.
In Proclamation 5463, for Education Day (19 Apr 1986). Collected in Public Papers of the Presidents of the United States: Ronald Reagan, 1986 (1988), 490.
Einstein, twenty-six years old, only three years away from crude privation, still a patent examiner, published in the Annalen der Physik in 1905 five papers on entirely different subjects. Three of them were among the greatest in the history of physics. One, very simple, gave the quantum explanation of the photoelectric effect—it was this work for which, sixteen years later, he was awarded the Nobel prize. Another dealt with the phenomenon of Brownian motion, the apparently erratic movement of tiny particles suspended in a liquid: Einstein showed that these movements satisfied a clear statistical law. This was like a conjuring trick, easy when explained: before it, decent scientists could still doubt the concrete existence of atoms and molecules: this paper was as near to a direct proof of their concreteness as a theoretician could give. The third paper was the special theory of relativity, which quietly amalgamated space, time, and matter into one fundamental unity.
This last paper contains no references and quotes no authority. All of them are written in a style unlike any other theoretical physicist’s. They contain very little mathematics. There is a good deal of verbal commentary. The conclusions, the bizarre conclusions, emerge as though with the greatest of ease: the reasoning is unbreakable. It looks as though he had reached the conclusions by pure thought, unaided, without listening to the opinions of others. To a surprisingly large extent, that is precisely what he had done.
This last paper contains no references and quotes no authority. All of them are written in a style unlike any other theoretical physicist’s. They contain very little mathematics. There is a good deal of verbal commentary. The conclusions, the bizarre conclusions, emerge as though with the greatest of ease: the reasoning is unbreakable. It looks as though he had reached the conclusions by pure thought, unaided, without listening to the opinions of others. To a surprisingly large extent, that is precisely what he had done.
In Variety of Men (1966), 100-101. First published in Commentary magazine.
ENGINEER, in the military art, an able expert man, who, by a perfect knowledge in mathematics, delineates upon paper, or marks upon the ground, all sorts of forts, and other works proper for offence and defence. He should understand the art of fortification, so as to be able, not only to discover the defects of a place, but to find a remedy proper for them; as also how to make an attack upon, as well as to defend, the place. Engineers are extremely necessary for these purposes: wherefore it is requisite that, besides being ingenious, they should be brave in proportion. When at a siege the engineers have narrowly surveyed the place, they are to make their report to the general, by acquainting him which part they judge the weakest, and where approaches may be made with most success. Their business is also to delineate the lines of circumvallation and contravallation, taking all the advantages of the ground; to mark out the trenches, places of arms, batteries, and lodgments, taking care that none of their works be flanked or discovered from the place. After making a faithful report to the general of what is a-doing, the engineers are to demand a sufficient number of workmen and utensils, and whatever else is necessary.
In Encyclopaedia Britannica or a Dictionary of Arts and Sciences (1771), Vol. 2, 497.
Engineering is quite different from science. Scientists try to understand nature. Engineers try to make things that do not exist in nature. Engineers stress invention. To embody an invention the engineer must put his idea in concrete terms, and design something that people can use. That something can be a device, a gadget, a material, a method, a computing program, an innovative experiment, a new solution to a problem, or an improvement on what is existing. Since a design has to be concrete, it must have its geometry, dimensions, and characteristic numbers. Almost all engineers working on new designs find that they do not have all the needed information. Most often, they are limited by insufficient scientific knowledge. Thus they study mathematics, physics, chemistry, biology and mechanics. Often they have to add to the sciences relevant to their profession. Thus engineering sciences are born.
Y.C. Fung and P. Tong, Classical and Computational Solid Mechanics (2001), 1.
Engineering is the application of scientific and mathematical principles to practical ends such as the design, manufacture, and operation of efficient and economical structures, machines, processes, and systems.
In Bernice Zeldin Schacter, Issues and Dilemmas of Biotechnology: A Reference Guide (1999), 1, citing the American Heritage Dictionary, 2nd College Edition.
Engineering is the profession in which a knowledge of the mathematical and natural sciences gained by study, experience, and practice is applied with judgment to develop ways to utilize, economically, the materials and forces of nature for the benefit of mankind.
— ABET
In EAC Criteria for 1999-2000 as cited in Charles R. Lord, Guide to Information Sources in Engineering (2000), 5. Found in many sources, and earlier, for example, Otis E. Lancaster, American Society for Engineering Education, Engineers' Council for Professional Development, Achieve Learning Objectives (1962), 8.

Engineering…is both an art and a science, and as a science it consists for the most part of mathematics applied to physics and mechanics. It is of necessity, therefore, a measuring science, and a congress of engineers ought, in the nature of things, to be interested in anything relating to progress in metrology.
From Address to the International Engineering Congress of the Columbia Exposition, Chicago, 1893. Published in Transactions of the American Society of Civil Engineers (Oct 1893), 120. Reprinted in 'Fundamental Units of Measure', Smithsonian Report for 1893 (1894), 135.
Engineers apply the theories and principles of science and mathematics to research and develop economical solutions to practical technical problems. Their work is the link between scientific discoveries and commercial applications. Engineers design products, the machinery to build those products, the factories in which those products are made, and the systems that ensure the quality of the product and efficiency of the workforce and manufacturing process. They design, plan, and supervise the construction of buildings, highways, and transit systems. They develop and implement improved ways to extract, process, and use raw materials, such as petroleum and natural gas. They develop new materials that both improve the performance of products, and make implementing advances in technology possible. They harness the power of the sun, the earth, atoms, and electricity for use in supplying the Nation’s power needs, and create millions of products using power. Their knowledge is applied to improving many things, including the
quality of health care, the safety of food products, and the efficient operation of financial systems.
Bureau of Labor Statistics, Occupational Outlook Handbook (2000) as quoted in Charles R. Lord. Guide to Information Sources in Engineering (2000), 5. This definition has been revised and expanded over time in different issues of the Handbook.
Eratosthenes of Cyrene, employing mathematical theories and geometrical methods, discovered from the course of the sun, the shadows cast by an equinoctial gnomon, and the inclination of the heaven that the circumference of the earth is two hundred and fifty-two thousand stadia, that is, thirty-one million five hundred thousand paces.
In De Architectura, Book 1, Chap 6, Sec. 9. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 27-28.
Essentially all civilizations that rose to the level of possessing an urban culture had need for two forms of science-related technology, namely, mathematics for land measurements and commerce and astronomy for time-keeping in agriculture and aspects of religious rituals.
From The Science Matrix: The Journey, Travails, Triumphs (1992, 1998), Preface, x.
Euclid and Archimedes are allowed to be knowing, and to have demonstrated what they say: and yet whosoever shall read over their writings without perceiving the connection of their proofs, and seeing what they show, though he may understand all their words, yet he is not the more knowing. He may believe, indeed, but does not know what they say, and so is not advanced one jot in mathematical knowledge by all his reading of those approved mathematicians.
In Conduct of the Understanding, sect. 24.
Euclid avoids it [the treatment of the infinite]; in modern mathematics it is systematically introduced, for only then is generality obtained.
'Geometry', Encyclopedia Britannica, 9th edition. In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 130. This is part of a longer quote, which begins “In Euclid each proposition…”, on the Arthur Cayley Quotes page of this website.
Euclidean mathematics assumes the completeness and invariability of mathematical forms; these forms it describes with appropriate accuracy and enumerates their inherent and related properties with perfect clearness, order, and completeness, that is, Euclidean mathematics operates on forms after the manner that anatomy operates on the dead body and its members. On the other hand, the mathematics of variable magnitudes—function theory or analysis—considers mathematical forms in their genesis. By writing the equation of the parabola, we express its law of generation, the law according to which the variable point moves. The path, produced before the eyes of the student by a point moving in accordance to this law, is the parabola.
If, then, Euclidean mathematics treats space and number forms after the manner in which anatomy treats the dead body, modern mathematics deals, as it were, with the living body, with growing and changing forms, and thus furnishes an insight, not only into nature as she is and appears, but also into nature as she generates and creates,—reveals her transition steps and in so doing creates a mind for and understanding of the laws of becoming. Thus modern mathematics bears the same relation to Euclidean mathematics that physiology or biology … bears to anatomy.
If, then, Euclidean mathematics treats space and number forms after the manner in which anatomy treats the dead body, modern mathematics deals, as it were, with the living body, with growing and changing forms, and thus furnishes an insight, not only into nature as she is and appears, but also into nature as she generates and creates,—reveals her transition steps and in so doing creates a mind for and understanding of the laws of becoming. Thus modern mathematics bears the same relation to Euclidean mathematics that physiology or biology … bears to anatomy.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 38. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 112-113.
Even if there is only one possible unified theory, it is just a set of rules and equations. What is it that breathes fire into the equations and makes a universe for them to describe? The usual approach of science of constructing a mathematical model cannot answer the questions of why there should be a universe for the model to describe. Why does the universe go to all the bother of existing?
A Brief History of Time (1998), 190.
Even now there is a very wavering grasp of the true position of mathematics as an element in the history of thought. I will not go so far as to say that to construct a history of thought without profound study of the mathematical ideas of successive epochs is like omitting Hamlet from the play which is named after him That would be claiming too much. But it is certainly analogous to cutting out the part of Ophelia. This simile is singularly exact. For Ophelia is quite essential to the play, she is very charming—and a little mad. Let us grant that the pursuit of mathematics is a divine madness of the human spirit, a refuge from the goading urgency of contingent happenings.
From Lecture to the Mathematical Society, Brown University, 'Mathematics as an Element in the History of Thought', collected as Chap. 2 in Science and the Modern World: Lowell Lectures, 1925 (1925), 31.
Every new body of discovery is mathematical in form, because there is no other guidance we can have.
(1931). As quoted, without citation, in Eric Temple Bell, 'They Say, What They Say, Let Them Say', Men of Mathematics (1937, 2014), Vol. 2, xvii. Webmaster has searched, but not yet found a primary source. Can you help?
Every discipline must be honored for reason other than its utility, otherwise it yields no enthusiasm for industry.
For both reasons, I consider mathematics the chief subject for the common school. No more highly honored exercise for the mind can be found; the buoyancy [Spannkraft] which it produces is even greater than that produced by the ancient languages, while its utility is unquestioned.
For both reasons, I consider mathematics the chief subject for the common school. No more highly honored exercise for the mind can be found; the buoyancy [Spannkraft] which it produces is even greater than that produced by the ancient languages, while its utility is unquestioned.
In 'Mathematischer Lehrplan für Realschulen' Werke [Kehrbach] (1890), Bd. 5, 167. (Mathematics Curriculum for Secondary Schools). As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 61.
Every human activity, good or bad, except mathematics, must come to an end.
Quoted as a favorite saying of Paul Erdös, by Béla Bollobás, 'The Life and Work of Paul Erdos', in Shiing-Shen Chern and Friedrich Hirzebruch (eds.) Wolf Prize in Mathematics (2000), Vol. 1, 292.
Every kind of science, if it has only reached a certain degree of maturity, automatically becomes a part of mathematics.
Eine jede Wissenschaft fällt, hat sie erst eine gewisse Reife erreicht, automatisch der Mathematik anheim.
Eine jede Wissenschaft fällt, hat sie erst eine gewisse Reife erreicht, automatisch der Mathematik anheim.
Identified as by “Anonymous” because Webmaster has so far found no source for these verbatim words. (Can you help?) Whenever seen attributed to David Hilbert, seemingly never is a primary source citation provided. However, expressed in totally different words, Hilbert expresses a similar idea in Address (11 Sep 1917), 'Axiomatisches Denken' delivered before the Swiss Mathematical Society in Zürich. See the quote that begins, “Anything at all that can be the object of scientific thought …”, on the David Hilbert Quotes page on this website.
Every mathematical book that is worth reading must be read “backwards and forwards”, if I may use the expression. I would modify Lagrange’s advice a little and say, “Go on, but often return to strengthen your faith.” When you come on a hard or dreary passage, pass it over; and come back to it after you have seen its importance or found the need for it further on.
In Algebra, Part 2 (1889), Preface, viii.
Every mathematical discipline goes through three periods of development: the naive, the formal, and the critical.
Quoted in R Remmert, Theory of complex functions (New York, 1989).
Every mathematician knows it is impossible to understand an elementary course in thermodynamics. The reason is that the thermodynamics is based—as Gibbs has explicitly proclaimed—on a rather complicated mathematical theory, on the contact geometry. Contact geometry is one of the few “simple geometries” of the so-called Cartan’s list, but it is still mostly unknown to the physicist.
The first sentence is often seen standing alone as a quote, to imply thermodynamics is a chimera beyond reach. When read in the full context, the point is that thermodynamics becomes possible to understand in an advanced course. It includes a reference to the work of mathematician Élie Cartan. From 'Contact Geometry: The Geometrical Method of Gibbs’ Thermodynamics', collected in D. Caldi and G. Mostow, (eds.) Proceedings of the Gibbs Symposium: Yale University, May 15-17, 1989, (1990), 163.
Every new theory as it arises believes in the flush of youth that it has the long sought goal; it sees no limits to its applicability, and believes that at last it is the fortunate theory to achieve the 'right' answer. This was true of electron theory—perhaps some readers will remember a book called The Electrical Theory of the Universe by de Tunzelman. It is true of general relativity theory with its belief that we can formulate a mathematical scheme that will extrapolate to all past and future time and the unfathomed depths of space. It has been true of wave mechanics, with its first enthusiastic claim a brief ten years ago that no problem had successfully resisted its attack provided the attack was properly made, and now the disillusionment of age when confronted by the problems of the proton and the neutron. When will we learn that logic, mathematics, physical theory, are all only inventions for formulating in compact and manageable form what we already know, like all inventions do not achieve complete success in accomplishing what they were designed to do, much less complete success in fields beyond the scope of the original design, and that our only justification for hoping to penetrate at all into the unknown with these inventions is our past experience that sometimes we have been fortunate enough to be able to push on a short distance by acquired momentum.
The Nature of Physical Theory (1936), 136.
Everybody firmly believes in it [Nomal Law of Errors] because the mathematicians imagine it is a fact of observation, and observers that it is a theory of mathematics.
…...
Everybody is to some small extent a philosopher of mathematics. Let him only exclaim on occasion: “But figures can’t lie!” and he joins the ranks of Plato and of Lakatos.
Co-author with Reuben Hersh, in The Mathematical Experience (1981), xi.
Everybody praises the incomparable power of the mathematical method, but so is everybody aware of its incomparable unpopularity.
In Jahresbericht der Deutschen Mathematiker Vereinigung, Bd. 13, 17.
Everyone makes for himself a clear idea of the motion of a point, that is to say, of the motion of a corpuscle which one supposes to be infinitely small, and which one reduces by thought in some way to a mathematical point.
Théorie Nouvelle de la Rotation des Corps (1834). As translated by Charles Thomas Whitley in Outlines of a New Theory of Rotatory Motion (1834), 1.
Everyone now agrees that a Physics where you banish all relationship with mathematics, to confine itself to a mere collection of observations and experiences, would be but an historical amusement, more fitting to entertain idle people, than to engage the mind of a true philosopher.
In 'Préface Contenant l’Exposition du Système', Dictionnaire de Physique (1761), Vol. 1, iii. English version via Google Translate, tweaked by Webmaster. From the original French, “Tout le monde convient maintenant qu’une Physique d’où l'on banniroit tout ce qui peut avoir quelque rapport avec les mathématiques, pour se borner à un simple recueil d’observations & d’experiences, ne seroit qu’un amusement historique, plus propre à récréer un cercle de personnes oisives, qu’à occuper un esprit véritablement philosophique.” Also seen translated as—“Everyone now agrees that a physics lacking all connection with mathematics…would only be an historical amusement, fitter for entertaining the idle than for occupying the mind of a philosopher,” in John L. Heilbron, Electricity in the 17th and 18th centuries: A Study of Early Modern Physics (1979), 74. In the latter source, the subject quote immediately follows a different one by Franz Karl Achard. An editor misreading that paragraph is the likely reason the subject quote will be found in Oxford Dictionary of Science Quotations attributed to Achard. Webmaster checked the original footnoted source, and corrected the author of this entry to Paulian (16 May 2014).
Everything is controlled by immutable mathematical laws, from which there is, and can be, no deviation whatsoever. We learn the complex from the simple. We arrive at the abstract by way of the concrete.
In The Science of Poetry and the Philosophy of Language (1910), xi.
Everything that the greatest minds of all times have accomplished toward the comprehension of forms by means of concepts is gathered into one great science, mathematics.
In 'Pestalozzi's Idee eines A B C der Anschauung', Werke[Kehrbach] (1890), Bd.l, 163. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 5.
Everywhere in nature we seek some certainty, but all this is nothing more than an arrangement of the dark feeling of our own. All the mathematical laws that we find in Nature are always suspicious to me, despite their beauty. They give me no pleasure. They are merely expedients. Everything is not true at close range.
From the original German, in Physikalische und Mathematische Schriften (1806), Vol. 4, 145, “Wir suchen in der Natur überall eine gewisse Bestimmtheit, aber das Alles ist weiter nichts, als Anordnung des dunkeln Gefühls unserer eigenen. Alle mathematischen Gesetze, die wir in der Natur finden, sind mir trotz ihrer Schönheit immer verdächtig. Sie Freuen mich nicht. Sie sind bloss Hülfsmittel. In der Nähe ist Alles nicht wahr.” English version by Webmaster using Google translate.
Examples ... show how difficult it often is for an experimenter to interpret his results without the aid of mathematics.
Quoted in E. T. Bell, Men of Mathematics, xvi.
Experimenters are the shock troops of science … An experiment is a question which science poses to Nature, and a measurement is the recording of Nature’s answer. But before an experiment can be performed, it must be planned–the question to nature must be formulated before being posed. Before the result of a measurement can be used, it must be interpreted–Nature’s answer must be understood properly. These two tasks are those of theorists, who find himself always more and more dependent on the tools of abstract mathematics.
'The Meaning and Limits of Exact Science', Science (30 Sep 1949), 110, No. 2857, 325. Advance reprinting of chapter from book Max Planck, Scientific Autobiography (1949), 110.
Experiments may be of two kinds: experiments of simple fact, and experiments of quantity. ...[In the latter] the conditions will ... vary, not in quality, but quantity, and the effect will also vary in quantity, so that the result of quantitative induction is also to arrive at some mathematical expression involving the quantity of each condition, and expressing the quantity of the result. In other words, we wish to know what function the effect is of its conditions. We shall find that it is one thing to obtain the numerical results, and quite another thing to detect the law obeyed by those results, the latter being an operation of an inverse and tentative character.
Principles of Science: A Treatise on Logic and Scientific Method (1874, 1892), 439.
Few will deny that even in the first scientific instruction in mathematics the most rigorous method is to be given preference over all others. Especially will every teacher prefer a consistent proof to one which is based on fallacies or proceeds in a vicious circle, indeed it will be morally impossible for the teacher to present a proof of the latter kind consciously and thus in a sense deceive his pupils. Notwithstanding these objectionable so-called proofs, so far as the foundation and the development of the system is concerned, predominate in our textbooks to the present time. Perhaps it will be answered, that rigorous proof is found too difficult for the pupil’s power of comprehension. Should this be anywhere the case,—which would only indicate some defect in the plan or treatment of the whole,—the only remedy would be to merely state the theorem in a historic way, and forego a proof with the frank confession that no proof has been found which could be comprehended by the pupil; a remedy which is ever doubtful and should only be applied in the case of extreme necessity. But this remedy is to be preferred to a proof which is no proof, and is therefore either wholly unintelligible to the pupil, or deceives him with an appearance of knowledge which opens the door to all superficiality and lack of scientific method.
In 'Stücke aus dem Lehrbuche der Arithmetik', Werke, Bd. 2 (1904), 296.
Finite systems of deterministic ordinary nonlinear differential equations may be designed to represent forced dissipative hydrodynamic flow. Solutions of these equations can be identified with trajectories in phase space. For those systems with bounded solutions, it is found that nonperiodic solutions are ordinarily unstable with respect to small modifications, so that slightly differing initial states can evolve into considerably different states. Systems with bounded solutions are shown to possess bounded numerical solutions.
A simple system representing cellular convection is solved numerically. All of the solutions are found to be unstable, and almost all of them are nonperiodic.
The feasibility of very-long-range weather prediction is examined in the light of these results
A simple system representing cellular convection is solved numerically. All of the solutions are found to be unstable, and almost all of them are nonperiodic.
The feasibility of very-long-range weather prediction is examined in the light of these results
Abstract from his landmark paper introducing Chaos Theory in relation to weather prediction, 'Deterministic Nonperiodic Flow', Journal of the Atmospheric Science (Mar 1963), 20, 130.
First of all, we ought to observe, that mathematical propositions, properly so called, are always judgments a priori, and not empirical, because they carry along with them necessity, which can never be deduced from experience. If people should object to this, I am quite willing to confine my statements to pure mathematics, the very concept of which implies that it does not contain empirical, but only pure knowledge a priori.
In Critique of Pure Reason (1900), 720.
First, [Newton’s Law of Universal Gravitation] is mathematical in its expression…. Second, it is not exact; Einstein had to modify it…. There is always an edge of mystery, always a place where we have some fiddling around to do yet…. But the most impressive fact is that gravity is simple…. It is simple, and therefore it is beautiful…. Finally, comes the universality of the gravitational law and the fact that it extends over such enormous distances…
In The Character of Physical Law (1965, 2001), 33.
First, as concerns the success of teaching mathematics. No instruction in the high schools is as difficult as that of mathematics, since the large majority of students are at first decidedly disinclined to be harnessed into the rigid framework of logical conclusions. The interest of young people is won much more easily, if sense-objects are made the starting point and the transition to abstract formulation is brought about gradually. For this reason it is psychologically quite correct to follow this course.
Not less to be recommended is this course if we inquire into the essential purpose of mathematical instruction. Formerly it was too exclusively held that this purpose is to sharpen the understanding. Surely another important end is to implant in the student the conviction that correct thinking based on true premises secures mastery over the outer world. To accomplish this the outer world must receive its share of attention from the very beginning.
Doubtless this is true but there is a danger which needs pointing out. It is as in the case of language teaching where the modern tendency is to secure in addition to grammar also an understanding of the authors. The danger lies in grammar being completely set aside leaving the subject without its indispensable solid basis. Just so in Teaching of Mathematics it is possible to accumulate interesting applications to such an extent as to stunt the essential logical development. This should in no wise be permitted, for thus the kernel of the whole matter is lost. Therefore: We do want throughout a quickening of mathematical instruction by the introduction of applications, but we do not want that the pendulum, which in former decades may have inclined too much toward the abstract side, should now swing to the other extreme; we would rather pursue the proper middle course.
Not less to be recommended is this course if we inquire into the essential purpose of mathematical instruction. Formerly it was too exclusively held that this purpose is to sharpen the understanding. Surely another important end is to implant in the student the conviction that correct thinking based on true premises secures mastery over the outer world. To accomplish this the outer world must receive its share of attention from the very beginning.
Doubtless this is true but there is a danger which needs pointing out. It is as in the case of language teaching where the modern tendency is to secure in addition to grammar also an understanding of the authors. The danger lies in grammar being completely set aside leaving the subject without its indispensable solid basis. Just so in Teaching of Mathematics it is possible to accumulate interesting applications to such an extent as to stunt the essential logical development. This should in no wise be permitted, for thus the kernel of the whole matter is lost. Therefore: We do want throughout a quickening of mathematical instruction by the introduction of applications, but we do not want that the pendulum, which in former decades may have inclined too much toward the abstract side, should now swing to the other extreme; we would rather pursue the proper middle course.
In Ueber den Mathematischen Unterricht an den hoheren Schulen; Jahresbericht der Deutschen Mathematiker Vereinigung, Bd. 11, 131.
For a physicist mathematics is not just a tool by means of which phenomena can be calculated, it is the main source of concepts and principles by means of which new theories can be created.
In 'Mathematics in the Physical Sciences', Scientific American (Sep 1964), 211, No. 3, 129.
For all their wealth of content, for all the sum of history and social institution invested in them, music, mathematics, and chess are resplendently useless (applied mathematics is a higher plumbing, a kind of music for the police band). They are metaphysically trivial, irresponsible. They refuse to relate outward, to take reality for arbiter. This is the source of their witchery.
In 'A Death of Kings', George Steiner at The New Yorker (2009), 209.
For he who knows not mathematics cannot know any other science; what is more, he cannot discover his own ignorance, or find its proper remedy.
For it being the nature of the mind of man (to the extreme prejudice of knowledge) to delight in the spacious liberty of generalities, as in a champion region, and not in the enclosures of particularity; the Mathematics were the goodliest fields to satisfy that appetite.
In De Augmentis, Bk. 8; Advancement of Learning, Bk. 2.
For many parts of Nature can neither be invented with sufficient subtlety, nor demonstrated with sufficient perspicuity, nor accommodated to use with sufficient dexterity, without the aid and intervention of Mathematic: of which sort are Perspective, Music, Astronomy, cosmography, Architecture, Machinery, and some others.
In De Augmentis, Bk. 3; The Advancement of Learning (1605), Book 3. As translated in Francis Bacon, James Spedding and Robert Leslie Ellis, 'Of the great Appendix of Natural Philosophy, both Speculative and Operative, namely Mathematic; and that it ought rather
to be placed among Appendices than among Substantive Sciences. Division of Mathematic into Pure and Mixed', The Works of Francis Bacon (1858), Vol. 4, Chap. 6, 371.
For mathematics, in a wilderness of tragedy and change, is a creature of the mind, born to the cry of humanity in search of an invariant reality, immutable in substance, unalterable with time.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
For scholars and laymen alike it is not philosophy but active experience in mathematics itself that can alone answer the question: What is mathematics?
As co-author with Herbert Robbins, in What Is Mathematics?: An Elementary Approach to Ideas and Methods (1941, 1996), xiii.
For the harmony of the world is made manifest in Form and Number, and the heart and soul and all the poetry of Natural Philosophy are embodied in the concept of mathematical beauty.
In 'Epilogue', On Growth and Form (1917), 778-9.
For the things of this world cannot be made known without a knowledge of mathematics.
(Sent to the Pope in 1267). As translated in Opus Majus (1928), Vol. 1, 128.
For twenty pages perhaps, he read slowly, carefully, dutifully, with pauses for self-examination and working out examples. Then, just as it was working up and the pauses should have been more scrupulous than ever, a kind of swoon and ecstasy would fall on him, and he read ravening on, sitting up till dawn to finish the book, as though it were a novel. After that his passion was stayed; the book went back to the Library and he was done with mathematics till the next bout. Not much remained with him after these orgies, but something remained: a sensation in the mind, a worshiping acknowledgment of something isolated and unassailable, or a remembered mental joy at the rightness of thoughts coming together to a conclusion, accurate thoughts, thoughts in just intonation, coming together like unaccompanied voices coming to a close.
In Mr. Fortune’s Maggot (1927), 161.
For we may remark generally of our mathematical researches, that these auxiliary quantities, these long and difficult calculations into which we are often drawn, are almost always proofs that we have not in the beginning considered the objects themselves so thoroughly and directly as their nature requires, since all is abridged and simplified, as soon as we place ourselves in a right point of view.
In Théorie Nouvelle de la Rotation des Corps (1834). As translated by Charles Thomas Whitley in Outlines of a New Theory of Rotatory Motion (1834), 4.
For, in mathematics or symbolic logic, reason can crank out the answer from the symboled equations—even a calculating machine can often do so—but it cannot alone set up the equations. Imagination resides in the words which define and connect the symbols—subtract them from the most aridly rigorous mathematical treatise and all meaning vanishes. Was it Eddington who said that we once thought if we understood 1 we understood 2, for 1 and 1 are 2, but we have since found we must learn a good deal more about “and”?
In 'The Biological Basis of Imagination', American Thought: 1947 (1947), 81.
For, Mathematical Demonstrations being built upon the impregnable Foundations of Geometry and Arithmetick, are the only Truths, that can sink into the Mind of Man, void of all Uncertainty; and all other Discourses participate more or less of Truth, according as their Subjects are more or less capable of Mathematical Demonstration.
Inaugural lecture of Christopher Wren in his chair of astronomy at Gresham College (1657). From Parentelia (1741, 1951), 200-201.
Formal thought, consciously recognized as such, is the means of all exact knowledge; and a correct understanding of the main formal sciences, Logic and Mathematics, is the proper and only safe foundation for a scientific education.
In Number and its Algebra (1896), 134.
Fractal is a word invented by Mandelbrot to bring together under one heading a large class of objects that have [played] … an historical role … in the development of pure mathematics. A great revolution of ideas separates the classical mathematics of the 19th century from the modern mathematics of the 20th. Classical mathematics had its roots in the regular geometric structures of Euclid and the continuously evolving dynamics of Newton. Modern mathematics began with Cantor’s set theory and Peano’s space-filling curve. Historically, the revolution was forced by the discovery of mathematical structures that did not fit the patterns of Euclid and Newton. These new structures were regarded … as “pathological,” .… as a “gallery of monsters,” akin to the cubist paintings and atonal music that were upsetting established standards of taste in the arts at about the same time. The mathematicians who created the monsters regarded them as important in showing that the world of pure mathematics contains a richness of possibilities going far beyond the simple structures that they saw in Nature. Twentieth-century mathematics flowered in the belief that it had transcended completely the limitations imposed by its natural origins.
Now, as Mandelbrot points out, … Nature has played a joke on the mathematicians. The 19th-century mathematicians may not have been lacking in imagination, but Nature was not. The same pathological structures that the mathematicians invented to break loose from 19th-century naturalism turn out to be inherent in familiar objects all around us.
Now, as Mandelbrot points out, … Nature has played a joke on the mathematicians. The 19th-century mathematicians may not have been lacking in imagination, but Nature was not. The same pathological structures that the mathematicians invented to break loose from 19th-century naturalism turn out to be inherent in familiar objects all around us.
From 'Characterizing Irregularity', Science (12 May 1978), 200, No. 4342, 677-678. Quoted in Benoit Mandelbrot, The Fractal Geometry of Nature (1977, 1983), 3-4.
From a mathematical standpoint it is possible to have infinite space. In a mathematical sense space is manifoldness, or combinations of numbers. Physical space is known as the 3-dimension system. There is the 4-dimension system, the 10-dimension system.
As quoted in 'Electricity Will Keep The World From Freezing Up', New York Times (12 Nov 1911), SM4.
From Pythagoras (ca. 550 BC) to Boethius (ca AD 480-524), when pure mathematics consisted of arithmetic and geometry while applied mathematics consisted of music and astronomy, mathematics could be characterized as the deductive study of “such abstractions as quantities and their consequences, namely figures and so forth” (Aquinas ca. 1260). But since the emergence of abstract algebra it has become increasingly difficult to formulate a definition to cover the whole of the rich, complex and expanding domain of mathematics.
In 100 Years of Mathematics: a Personal Viewpoint (1981), 2.
From the age of 13, I was attracted to physics and mathematics. My interest in these subjects derived mostly from popular science books that I read avidly. Early on I was fascinated by theoretical physics and determined to become a theoretical physicist. I had no real idea what that meant, but it seemed incredibly exciting to spend one's life attempting to find the secrets of the universe by using one's mind.
From 'Autobiography', in Tore Frängsmyr (ed.) Les Prix Nobel. The Nobel Prizes 2004, (2005).
Gauss [replied], when asked how soon he expected to reach certain mathematical conclusions, “that he had them long ago, all he was worrying about was how to reach them.”
In Louis Pasteur, Free Lance of Science (1950), 365. Also excerpted in 'Mechanisms of Discovery', collected in I.S. Gordon and S. Sorkin (eds.) The Armchair Science Reader (1959), 336.
Gauss once said, “Mathematics is the queen of the sciences and number theory the queen of mathematics.” If this is true we may add that the Disquisitions is the Magna Charter of number theory.
In Allgemeine Deutsche Biographie (1878, 8, 435. As cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 158.
Gel’fand amazed me by talking of mathematics as though it were poetry. He once said about a long paper bristling with formulas that it contained the vague beginnings of an idea which could only hint at and which he had never managed to bring out more clearly. I had always thought of mathematics as being much more straightforward: a formula is a formula, and an algebra is an algebra, but Gel’fand found hedgehogs lurking in the rows of his spectral sequences!
In '1991 Ruth Lyttle Satter Prize', Notices of the American Mathematical Society (Mar 1991), 38, No. 3, 186. This is from her acceptance of the 1991 Ruth Lyttle Satter Prize.
Generalisations which are fruitful because they reveal in a single general principle the rationale of a great many particular truths, the connections and common origins of which had not previously been seen, are found in all the sciences, and particularly in mathematics. Such generalisations are the most important of all, and their discovery is the work of genius.
From Essai sur les Fondements de nos Connaissances et sur les Caractères de la Critique Philosophique (1851), 28, as translated by Merritt H Moore in An Essay on the Foundations of Our Knowledge (1956), 24. From the original French: “Il y a dans toutes les sciences, et en mathématiques particulièrement, des généralisations fécondes, parce qu’elles nous montrent dans une vérité générale la raison d’une multitude de vérités particulières dont les liens et la commune origine n’étaient point aperçus. De telles généralisations sont des découvertes du génie, et les plus importantes de toutes.”
Generality of points of view and of methods, precision and elegance in presentation, have become, since Lagrange, the common property of all who would lay claim to the rank of scientific mathematicians. And, even if this generality leads at times to abstruseness at the expense of intuition and applicability, so that general theorems are formulated which fail to apply to a single special case, if furthermore precision at times degenerates into a studied brevity which makes it more difficult to read an article than it was to write it; if, finally, elegance of form has well-nigh become in our day the criterion of the worth or worthlessness of a proposition,—yet are these conditions of the highest importance to a wholesome development, in that they keep the scientific material within the limits which are necessary both intrinsically and extrinsically if mathematics is not to spend itself in trivialities or smother in profusion.
In Die Entwickdung der Mathematik in den letzten Jahrhunderten (1884), 14-15.
Genetics is the first biological science which got in the position in which physics has been in for many years. One can justifiably speak about such a thing as theoretical mathematical genetics, and experimental genetics, just as in physics. There are some mathematical geniuses who work out what to an ordinary person seems a fantastic kind of theory. This fantastic kind of theory nevertheless leads to experimentally verifiable prediction, which an experimental physicist then has to test the validity of. Since the times of Wright, Haldane, and Fisher, evolutionary genetics has been in a similar position.
Oral history memoir. Columbia University, Oral History Research Office, New York, 1962. Quoted in William B. Provine, Sewall Wright and Evolutionary Biology (1989), 277.
Geology has its peculiar difficulties, from which all other sciences are exempt. Questions in chemistry may be settled in the laboratory by experiment. Mathematical and philosophical questions may be discussed, while the materials for discussion are ready furnished by our own intellectual reflections. Plants, animals and minerals, may be arranged in the museum, and all questions relating to their intrinsic principles may be discussed with facility. But the relative positions, the shades of difference, the peculiar complexions, whether continuous or in subordinate beds, are subjects of enquiry in settling the character of rocks, which can be judged of while they are in situ only.
A Geological and Agricultural Survey of the District Adjoining the Erie Canal (1824), 8.
Get into any taxi and tell the driver you are a mathematician and the response is predictable … you will hear the immortal words: “I was never any good at mathematics.” My response is: “I was never any good at being a taxi driver so I went into mathematics.”
In paper, 'A Mathematician’s Survival Guide', pdf document linked from his homepage at math.missouri.edu (undated, but 2011 or earlier, indicated by an “accessed on” date elsewhere.) Collected in Peter Casazza, Steven G. Krantz and Randi D. Ruden (eds.) I, Mathematician (2005), 31.
Given any domain of thought in which the fundamental objective is a knowledge that transcends mere induction or mere empiricism, it seems quite inevitable that its processes should be made to conform closely to the pattern of a system free of ambiguous terms, symbols, operations, deductions; a system whose implications and assumptions are unique and consistent; a system whose logic confounds not the necessary with the sufficient where these are distinct; a system whose materials are abstract elements interpretable as reality or unreality in any forms whatsoever provided only that these forms mirror a thought that is pure. To such a system is universally given the name MATHEMATICS.
In 'Mathematics', National Mathematics Magazine (Nov 1937), 12, No. 2, 62.
Given for one instant an intelligence which could comprehend all the forces by which nature is animated and the respective situation of the beings which compose it—an intelligence sufficiently vast to submit these data to analysis, it would embrace in the same formula the movements of the greatest bodies in the universe and those of the lightest atom; to it nothing would be uncertain, and the future as the past would be present to its eyes.
Introduction to Oeuvres vol. VII, Theorie Analytique de Probabilites (1812-1820). As translated by Frederick Wilson Truscott and Frederick Lincoln Emory in A Philosophical Essay on Probabilities (1902), 4. [LaPlace is here expressing his belief in causal determinism.] From the original French, “Une intelligence qui, pour un instant donné, connaîtrait toutes les forces dont la nature est animée, et la situation respective des êtres qui la composent, si d’ailleurs elle était assez vaste pour soumettre ces données a l’analyse, embrasserait dans la même formula les mouvements des plus grand corps de l’univers et ceux du plus léger atome: rien ne serait incertain pour elle, et l’avenir comme le passé serait présent à ses yeux.”
God does not care about our mathematical difficulties. He integrates empirically.
Quoted, without citation, by Léopold Infeld in Quest (1942, 1980), 279. If you know the primary source, please contact Webmaster.
God exists since mathematics is consistent, and the Devil exists since we cannot prove it.
Given as “A. Weil has said…”, in Paul C. Rosenbloom, The Elements of Mathematical Logic (1950), 72. Note that Rosenbloom gives the quote in narrative form, not within quotation marks, which suggests these words may not be verbatim. Later texts have added quotation marks [which may not be justified. —Webmaster] As yet, Webmaster has not found an earlier source to validate whether the quotations marks can be used. (Can you help?)
God forbid that Truth should be confined to Mathematical Demonstration! He who does not know truth at sight is unworthy of Her Notice.
Marginal note (c. 1808) written in his copy of 'Discourse VII', The Works of Sir Joshua Reynolds (1798), beside “…as true as mathematical demonstration…”. As given in William Blake, Edwin John Ellis (ed.) and William Butler Yeats (ed.), The Works of William Blake (1893), Vol. 2, 340.
God is a child; and when he began to play, he cultivated mathematics. It is the most godly of man’s games.
Said by the fictional character, mathematics teacher, Professor Hirt, in Das Blinde Spiel (The Blind Game, 1954), 253. As translated in an epigraph, Stanley Gudder, A Mathematical Journey (1976), 269. From the original German, “Gott ist ein Kind, und als er zu spielen begann, trieb er Mathematik. Die Mathematik ist göttlichste Spielerei unter den Menschen”, as quoted in Herbert Meschkowski, Hundert Jahre Mengenlehre (1973), 119.
God used beautiful mathematics in creating the world.
Quoted in Behram Kursunoglu and Eugene Paul Wigner, Paul Adrien Maurice Dirac (1990), Preface, xv.
Gödel proved that the world of pure mathematics is inexhaustible; no finite set of axioms and rules of inference can ever encompass the whole of mathematics; given any finite set of axioms, we can find meaningful mathematical questions which the axioms leave unanswered. I hope that an analogous Situation exists in the physical world. If my view of the future is correct, it means that the world of physics and astronomy is also inexhaustible; no matter how far we go into the future, there will always be new things happening, new information coming in, new worlds to explore, a constantly expanding domain of life, consciousness, and memory.
From Lecture 1, 'Philosophy', in a series of four James Arthur Lectures, 'Lectures on Time and its Mysteries' at New York University (Autumn 1978). Printed in 'Time Without End: Physics and Biology in an Open Universe', Reviews of Modern Physics (Jul 1979), 51, 449.
Gradually, at various points in our childhoods, we discover different forms of conviction. There’s the rock-hard certainty of personal experience (“I put my finger in the fire and it hurt,”), which is probably the earliest kind we learn. Then there’s the logically convincing, which we probably come to first through maths, in the context of Pythagoras’s theorem or something similar, and which, if we first encounter it at exactly the right moment, bursts on our minds like sunrise with the whole universe playing a great chord of C Major.
In short essay, 'Dawkins, Fairy Tales, and Evidence', 2.
Greek mathematics is the real thing. The Greeks first spoke a language which modern mathematicians can understand… So Greek mathematics is ‘permanent’, more permanent even than Greek literature.
In A Mathematician’s Apology (1940, 1967), 81.
Guided only by their feeling for symmetry, simplicity, and generality, and an indefinable sense of the fitness of things, creative mathematicians now, as in the past, are inspired by the art of mathematics rather than by any prospect of ultimate usefulness.
In The Queen of the Sciences (1938), 2.
Hardly a pure science, history is closer to animal husbandry than it is to mathematics, in that it involves selective breeding. The principal difference between the husbandryman and the historian is that the former breeds sheep or cows or such, and the latter breeds (assumed) facts. The husbandryman uses his skills to enrich the future; the historian uses his to enrich the past. Both are usually up to their ankles in bullshit.
Another Roadside Attraction (1990), 127.
Has Matter more than Motion? Has it Thought,
Judgment, and Genius? Is it deeply learn’d
In Mathematics? Has it fram’d such Laws,
Which, but to guess, a Newton made immortal?—
If so, how each sage Atom laughs at me,
Who think a Clod inferior to a Man!
Judgment, and Genius? Is it deeply learn’d
In Mathematics? Has it fram’d such Laws,
Which, but to guess, a Newton made immortal?—
If so, how each sage Atom laughs at me,
Who think a Clod inferior to a Man!
The Complaint: or, Night-Thoughts on Life, Death, and Immortality (1742, 1750), Night 9, 279.
Having been the discoverer of many splendid things, he is said to have asked his friends and relations that, after his death, they should place on his tomb a cylinder enclosing a sphere, writing on it the proportion of the containing solid to that which is contained.
Plutarch, Life of Marcellus, 17.12. Trans. R. W. Sharples.
He [General Nathan Bedford Forrest] possessed a remarkable genius for mathematics, a subject in which he had absolutely no training. He could with surprising facility solve the most difficult problems in algebra, geometry, and trigonometry, only requiring that the theorem or rule be carefully read aloud to him.
In Life of General Nathan Bedford Forrest (1899), 627.
He [Sylvester] had one remarkable peculiarity. He seldom remembered theorems, propositions, etc., but had always to deduce them when he wished to use them. In this he was the very antithesis of Cayley, who was thoroughly conversant with everything that had been done in every branch of mathematics.
I remember once submitting to Sylvester some investigations that I had been engaged on, and he immediately denied my first statement, saying that such a proposition had never been heard of, let alone proved. To his astonishment, I showed him a paper of his own in which he had proved the proposition; in fact, I believe the object of his paper had been the very proof which was so strange to him.
I remember once submitting to Sylvester some investigations that I had been engaged on, and he immediately denied my first statement, saying that such a proposition had never been heard of, let alone proved. To his astonishment, I showed him a paper of his own in which he had proved the proposition; in fact, I believe the object of his paper had been the very proof which was so strange to him.
As quoted by Florian Cajori, in Teaching and History of Mathematics in the United States (1890), 268.
He that could teach mathematics well, would not be a bad teacher in any of [physics, chemistry, biology or psychology] unless by the accident of total inaptitude for experimental illustration; while the mere experimentalist is likely to fall into the error of missing the essential condition of science as reasoned truth; not to speak of the danger of making the instruction an affair of sensation, glitter, or pyrotechnic show.
In Education as a Science (1879), 298.
He was not a mathematician–he never even took a maths class after high school–yet Martin Gardner, who has died aged 95, was arguably the most influential and inspirational figure in mathematics in the second half of the last century.
In 'Martin Gardner Obituary', The Guardian (27 May 2010)
He who gives a portion of his time and talent to the investigation of mathematical truth will come to all other questions with a decided advantage over his opponents. He will be in argument what the ancient Romans were in the field: to them the day of battle was a day of comparative recreation, because they were ever accustomed to exercise with arms much heavier than they fought; and reviews differed from a real battle in two respects: they encountered more fatigue, but the victory was bloodless.
Reflection 352, in Lacon: or Many things in Few Words; Addressed to Those Who Think (1820), 159.
He who is unfamiliar with mathematics remains more or less a stranger to our time.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 39. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 122. From the original German, “Wer mathematisch ein Laie ist, geht mehr oder weniger als Fremder durch unsere Zeit”. More literally, the first phrase would be translated as, “He who is a layman in mathematics…”.
Helmholtz—the physiologist who learned physics for the sake of his physiology, and mathematics for the sake of his physics, and is now in the first rank of all three.
In 'Aims and Instruments of Scientific Thought', Lectures and Essays, Vol. 1 (1901), 165.
Here arises a puzzle that has disturbed scientists of all periods. How can it be that mathematics, being after all a product of human thought which is independent of experience, is so admirably appropriate to the objects of reality? Is human reason, then, without experience, merely by taking thought, able to fathom the properties of real things?
From 'Geometry and Experience', an expanded form of an Address by Albert Einstein to the Prussian Academy of Sciences in Berlin (27 Jan 1921). In Albert Einstein, translated by G. B. Jeffery and W. Perrett, Sidelights on Relativity (1923).
Here I shall present, without using Analysis [mathematics], the principles and general results of the Théorie, applying them to the most important questions of life, which are indeed, for the most part, only problems in probability. One may even say, strictly speaking, that almost all our knowledge is only probable; and in the small number of things that we are able to know with certainty, in the mathematical sciences themselves, the principal means of arriving at the truth—induction and analogy—are based on probabilities, so that the whole system of human knowledge is tied up with the theory set out in this essay.
Philosophical Essay on Probabilities (1814), 5th edition (1825), trans. Andrew I. Dale (1995), 1.
Here's to pure mathematics—may it never be of any use to anybody.
A toast, variously attributed as used of old at Cambridge University, or as used by G.N. Hardy (according to Arthur C. Clarke in 'The Joy of Maths', Greetings, Carbon-Based Bipeds!: Collected Essays, 1934-1998 (2001), 460).
Higher Mathematics is the art of reasoning about numerical relations between natural phenomena; and the several sections of Higher Mathematics are different modes of viewing these relations.
In Higher Mathematics for Students of Chemistry and Physics (1902), Prologue, xvii.
His “Mathematical Games” column in Scientific American is one of the few bridges over C.P. Snow’s famous “gulf of mutual incomprehension’' that lies between technical and literary cultures.
In 'Martin Gardner: A “Documentary”', collected in Elwyn Berlekamp and Tom Rodgers (eds.), The Mathematician and the Pied Puzzler: A Collection in Tribute to Martin Gardner (1999), 9.
His [Thomas Edison] method was inefficient in the extreme, for an immense ground had to be covered to get anything at all unless blind chance intervened and, at first, I was almost a sorry witness of his doings, knowing that just a little theory and calculation would have saved him 90 per cent of the labor. But he had a veritable contempt for book learning and mathematical knowledge, trusting himself entirely to his inventor's instinct and practical American sense. In view of this, the truly prodigious amount of his actual accomplishments is little short of a miracle.
As quoted in 'Tesla Says Edison Was an Empiricist', The New York Times (19 Oct 1931), 25. In 1884, Tesla had moved to America to assist Edison in the designing of motors and generators.
Histories make men wise; poets, witty; the mathematics, subtle; natural philosophy, deep; moral, grave; logic and rhetoric, able to contend.
'L. Of Studies,' Essays (1597). In Francis Bacon and Basil Montagu, The Works of Francis Bacon, Lord Chancellor of England (1852), 55.
How can a modern anthropologist embark upon a generalization with any hope of arriving at a satisfactory conclusion? By thinking of the organizational ideas that are present in any society as a mathematical pattern.
In Rethinking Anthropology (1961), 2.
How can you shorten the subject? That stern struggle with the multiplication table, for many people not yet ended in victory, how can you make it less? Square root, as obdurate as a hardwood stump in a pasture nothing but years of effort can extract it. You can’t hurry the process. Or pass from arithmetic to algebra; you can’t shoulder your way past quadratic equations or ripple through the binomial theorem. Instead, the other way; your feet are impeded in the tangled growth, your pace slackens, you sink and fall somewhere near the binomial theorem with the calculus in sight on the horizon. So died, for each of us, still bravely fighting, our mathematical training; except for a set of people called “mathematicians”—born so, like crooks.
In Too Much College: Or, Education Eating up Life, with Kindred Essays in Education and Humour (1939), 8.
How is it that there are so many minds that are incapable of understanding mathematics? ... the skeleton of our understanding, ... and actually they are the majority. ... We have here a problem that is not easy of solution, but yet must engage the attention of all who wish to devote themselves to education.
Science and Method (1914, 2003), 117-118.
Humanism is only another name for spiritual laziness, or a vague half-creed adopted by men of science and logicians whose heads are too occupied with the world of mathematics and physics to worry about religious categories.
In The Outsider (1956), 279.
I admit that mathematical science is a good thing. But excessive devotion to it is a bad thing.
Interview with J. W. N. Sullivan, Contemporary Mind (1934). Quoted in James Roy Newman, The World of Mathematics (2000), 2027.
I advise my students to listen carefully the moment they decide to take no more Mathematics courses. They might be able to hear the sound of closing doors.
From 'Everybody a Mathematician', CAIP Quarterly (Fall 1989), 2, as quoted and cited, as a space filler following article Reinhard C. Laubenbacher and Michael Siddoway, 'Great Problems of Mathematics: A Summer Workshop for High School Students', The College Mathematics Journal (Mar 1994), 25, No. 2, 114.
I also ask you my friends not to condemn me entirely to the mill of mathematical calculations, and allow me time for philosophical speculations, my only pleasures.
Letter to Vincenzo Bianchi (17 Feb 1619). Johannes Kepler Gesammelte Werke (1937- ), Vol. 17, letter 827, l. 249-51, p. 327.
I also require much time to ponder over the matters themselves, and particularly the principles of mechanics (as the very words: force, time, space, motion indicate) can occupy one severely enough; likewise, in mathematics, the meaning of imaginary quantities, of the infinitesimally small and infinitely large and similar matters.
In Davis Baird, R.I.G. Hughes and Alfred Nordmann, Heinrich Hertz: Classical Physicist, Modern Philosopher (1998), 159.
I am acutely aware of the fact that the marriage between mathematics and physics, which was so enormously fruitful in past centuries, has recently ended in divorce.
From 'Missed Opportunities', Josiah Willard Gibbs Lecture (17 Jan 1972), as published in Bulletin of the American Mathematical Society (Sep 1972), 78, No. 5. Collected in Jong-Ping Hsu and Dana Fine (eds.), 100 Years of Gravity and Accelerated Frames: The Deepest Insights of Einstein and Yang-Mills (2005), 347.
I am ever more intrigued by the correspondence between mathematics and physical facts. The adaptability of mathematics to the description of physical phenomena is uncanny.
From Nobel Banquet Speech (10 Dec 1981), in Wilhelm Odelberg (ed.), Les Prix Nobel 1981 (1981), 59.
I am interested in mathematics only as a creative art.
In A Mathematician’s Apology (1940, reprint with Foreward by C.P. Snow 1992), 115.
I am of the decided opinion, that mathematical instruction must have for its first aim a deep penetration and complete command of abstract mathematical theory together with a clear insight into the structure of the system, and doubt not that the instruction which accomplishes this is valuable and interesting even if it neglects practical applications. If the instruction sharpens the understanding, if it arouses the scientific interest, whether mathematical or philosophical, if finally it calls into life an esthetic feeling for the beauty of a scientific edifice, the instruction will take on an ethical value as well, provided that with the interest it awakens also the impulse toward scientific activity. I contend, therefore, that even without reference to its applications mathematics in the high schools has a value equal to that of the other subjects of instruction.
In 'Ueber das Lehrziel im mathemalischen Unterricht der höheren Realanstalten', Jahresbericht der Deutschen Mathematiker Vereinigung, 2, 192. (The Annual Report of the German Mathematical Association. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 73.

I beg to introduce myself to you as a clerk in the Accounts Department of the Port Trust Office at Madras on a salary of only £20 per annum. I am now about 23 years of age. … After leaving school I have been employing the spare time at my disposal to work at Mathematics.
Opening lines of first letter to G.H. Hardy (16 Jan 1913). In Collected Papers of Srinivasa Ramanujan (1927), xxiii. Hardy notes he did “seem to remember his telling me that his friends had given him some assistance” in writing the letter because Ramanujan's “knowledge of English, at that stage of his life, could scarcely have been sufficient.”
I believe that mathematical reality lies outside us, that our function is to discover or observe it, and that the theorems which we prove, and which we describe grandiloquently as our “creations,” are simply the notes of our observations.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 113.
I believe that the useful methods of mathematics are easily to be learned by quite young persons, just as languages are easily learned in youth. What a wondrous philosophy and history underlie the use of almost every word in every language—yet the child learns to use the word unconsciously. No doubt when such a word was first invented it was studied over and lectured upon, just as one might lecture now upon the idea of a rate, or the use of Cartesian co-ordinates, and we may depend upon it that children of the future will use the idea of the calculus, and use squared paper as readily as they now cipher. … When Egyptian and Chaldean philosophers spent years in difficult calculations, which would now be thought easy by young children, doubtless they had the same notions of the depth of their knowledge that Sir William Thomson might now have of his. How is it, then, that Thomson gained his immense knowledge in the time taken by a Chaldean philosopher to acquire a simple knowledge of arithmetic? The reason is plain. Thomson, when a child, was taught in a few years more than all that was known three thousand years ago of the properties of numbers. When it is found essential to a boy’s future that machinery should be given to his brain, it is given to him; he is taught to use it, and his bright memory makes the use of it a second nature to him; but it is not till after-life that he makes a close investigation of what there actually is in his brain which has enabled him to do so much. It is taken because the child has much faith. In after years he will accept nothing without careful consideration. The machinery given to the brain of children is getting more and more complicated as time goes on; but there is really no reason why it should not be taken in as early, and used as readily, as were the axioms of childish education in ancient Chaldea.
In Teaching of Mathematics (1902), 14.
I can only compare their [Hindu] astronomical and mathematical literature … to a mixture of pearl shells and sour dates, or of pearls and dung, or of costly crystals and common pebbles. Both kinds of things are equal in their eyes, since they cannot rise themselves to the methods of strictly scientific deduction.
As translated by Edward C. Sachau, in Alberuni’s India: An Account of the Religion, Philosophy, Literature, Geography, Chronology, Astronomy, Customs, Laws and Astrology of India about A.D. 1030 (1910), Vol. 1, 25.
I can see him [Sylvester] now, with his white beard and few locks of gray hair, his forehead wrinkled o’er with thoughts, writing rapidly his figures and formulae on the board, sometimes explaining as he wrote, while we, his listeners, caught the reflected sounds from the board. But stop, something is not right, he pauses, his hand goes to his forehead to help his thought, he goes over the work again, emphasizes the leading points, and finally discovers his difficulty. Perhaps it is some error in his figures, perhaps an oversight in the reasoning. Sometimes, however, the difficulty is not elucidated, and then there is not much to the rest of the lecture. But at the next lecture we would hear of some new discovery that was the outcome of that difficulty, and of some article for the Journal, which he had begun. If a text-book had been taken up at the beginning, with the intention of following it, that text-book was most likely doomed to oblivion for the rest of the term, or until the class had been made listeners to every new thought and principle that had sprung from the laboratory of his mind, in consequence of that first difficulty. Other difficulties would soon appear, so that no text-book could last more than half of the term. In this way his class listened to almost all of the work that subsequently appeared in the Journal. It seemed to be the quality of his mind that he must adhere to one subject. He would think about it, talk about it to his class, and finally write about it for the Journal. The merest accident might start him, but once started, every moment, every thought was given to it, and, as much as possible, he read what others had done in the same direction; but this last seemed to be his real point; he could not read without finding difficulties in the way of understanding the author. Thus, often his own work reproduced what had been done by others, and he did not find it out until too late.
A notable example of this is in his theory of cyclotomic functions, which he had reproduced in several foreign journals, only to find that he had been greatly anticipated by foreign authors. It was manifest, one of the critics said, that the learned professor had not read Rummer’s elementary results in the theory of ideal primes. Yet Professor Smith’s report on the theory of numbers, which contained a full synopsis of Kummer’s theory, was Professor Sylvester’s constant companion.
This weakness of Professor Sylvester, in not being able to read what others had done, is perhaps a concomitant of his peculiar genius. Other minds could pass over little difficulties and not be troubled by them, and so go on to a final understanding of the results of the author. But not so with him. A difficulty, however small, worried him, and he was sure to have difficulties until the subject had been worked over in his own way, to correspond with his own mode of thought. To read the work of others, meant therefore to him an almost independent development of it. Like the man whose pleasure in life is to pioneer the way for society into the forests, his rugged mind could derive satisfaction only in hewing out its own paths; and only when his efforts brought him into the uncleared fields of mathematics did he find his place in the Universe.
A notable example of this is in his theory of cyclotomic functions, which he had reproduced in several foreign journals, only to find that he had been greatly anticipated by foreign authors. It was manifest, one of the critics said, that the learned professor had not read Rummer’s elementary results in the theory of ideal primes. Yet Professor Smith’s report on the theory of numbers, which contained a full synopsis of Kummer’s theory, was Professor Sylvester’s constant companion.
This weakness of Professor Sylvester, in not being able to read what others had done, is perhaps a concomitant of his peculiar genius. Other minds could pass over little difficulties and not be troubled by them, and so go on to a final understanding of the results of the author. But not so with him. A difficulty, however small, worried him, and he was sure to have difficulties until the subject had been worked over in his own way, to correspond with his own mode of thought. To read the work of others, meant therefore to him an almost independent development of it. Like the man whose pleasure in life is to pioneer the way for society into the forests, his rugged mind could derive satisfaction only in hewing out its own paths; and only when his efforts brought him into the uncleared fields of mathematics did he find his place in the Universe.
In Florian Cajori, Teaching and History of Mathematics in the United States (1890), 266-267.
I can see him now at the blackboard, chalk in one hand and rubber in the other, writing rapidly and erasing recklessly, pausing every few minutes to face the class and comment earnestly, perhaps on the results of an elaborate calculation, perhaps on the greatness of the Creator, perhaps on the beauty and grandeur of Mathematics, always with a capital M. To him mathematics was not the handmaid of philosophy. It was not a humanly devised instrument of investigation, it was Philosophy itself, the divine revealer of TRUTH.
Writing as a Professor Emeritus at Harvard University, a former student of Peirce, in 'Benjamin Peirce: II. Reminiscences', The American Mathematical Monthly (Jan 1925), 32, No. 1, 5.
I can’t think of any definition of the words mathematician or scientist that would apply to me. I think of myself as a journalist who knows just enough about mathematics to be able to take low-level math and make it clear and interesting to nonmathematicians. Let me say that I think not knowing too much about a subject is an asset for a journalist, not a liability. The great secret of my column is that I know so little about mathematics that I have to work hard to understand the subject myself. Maybe I can explain things more clearly than a professional mathematician can.
In Scot Morris, 'Interview: Martin Gardner', Omni, 4, No. 4 (Jan 1982), 68.
I cannot find anything showing early aptitude for acquiring languages; but that he [Clifford] had it and was fond of exercising it in later life is certain. One practical reason for it was the desire of being able to read mathematical papers in foreign journals; but this would not account for his taking up Spanish, of which he acquired a competent knowledge in the course of a tour to the Pyrenees. When he was at Algiers in 1876 he began Arabic, and made progress enough to follow in a general way a course of lessons given in that language. He read modern Greek fluently, and at one time he was furious about Sanskrit. He even spent some time on hieroglyphics. A new language is a riddle before it is conquered, a power in the hand afterwards: to Clifford every riddle was a challenge, and every chance of new power a divine opportunity to be seized. Hence he was likewise interested in the various modes of conveying and expressing language invented for special purposes, such as the Morse alphabet and shorthand. … I have forgotten to mention his command of French and German, the former of which he knew very well, and the latter quite sufficiently; …
In paper, 'William Kingdon Clifford', The Fortnightly Review (1879), 31, 671. Published in advance of Leslie Stephen and Frederick Pollock (eds.), Clifford’s Lectures and Essays (1879), Vol. 1, Introduction, 9. The 'Introduction' was written by Pollock.
I confess, that after I began … to discern how useful mathematicks may be made to physicks, I have often wished that I had employed about the speculative part of geometry, and the cultivation of the specious Algebra I had been taught very young, a good part of that time and industry, that I had spent about surveying and fortification (of which I remember I once wrote an entire treatise) and other parts of practick mathematicks.
In 'The Usefulness of Mathematiks to Natural Philosophy', Works (1772), Vol. 3, 426.
I confess, that very different from you, I do find sometimes scientific inspiration in mysticism … but this is counterbalanced by an immediate sense for mathematics.
Letter to Niels Bohr (1955). Quoted in Robert J. Scully, The Demon and the Quantum (2007), 7.
I count Maxwell and Einstein, Eddington and Dirac, among “real” mathematicians. The great modern achievements of applied mathematics have been in relativity and quantum mechanics, and these subjects are at present at any rate, almost as “useless” as the theory of numbers.
In A Mathematician's Apology (1940, 2012), 131.
I did try “to make things clear,” first to myself (an important point) and then to my students and somehow to make “these dry bones live.”
His response on his 80th birthday (1929) recognition of his mathematical contributions and teachings by his former students. As quoted by R.T. Glazebrook in Obituary Notices of Fellows of the Royal Society (Dec 1935), 392.
I do not define time, space, place, and motion, as being well known to all. … [However] it will be convenient to distinguish them into Absolute and Relative, True and Apparent, Mathematical and Common.
Scholium following opening section of Definitions, Philosophia Naturalis Principia Mathematica (1687) as translated from the original Latin, in Andrew Motte, Newton's Principia: The Mathematical Principles of Natural Philosophy (1729), Vol. 1, 9.
I do not intend to go deeply into the question how far mathematical studies, as the representatives of conscious logical reasoning, should take a more important place in school education. But it is, in reality, one of the questions of the day. In proportion as the range of science extends, its system and organization must be improved, and it must inevitably come about that individual students will find themselves compelled to go through a stricter course of training than grammar is in a position to supply. What strikes me in my own experience with students who pass from our classical schools to scientific and medical studies, is first, a certain laxity in the application of strictly universal laws. The grammatical rules, in which they have been exercised, are for the most part followed by long lists of exceptions; accordingly they are not in the habit of relying implicitly on the certainty of a legitimate deduction from a strictly universal law. Secondly, I find them for the most part too much inclined to trust to authority, even in cases where they might form an independent judgment. In fact, in philological studies, inasmuch as it is seldom possible to take in the whole of the premises at a glance, and inasmuch as the decision of disputed questions often depends on an aesthetic feeling for beauty of expression, or for the genius of the language, attainable only by long training, it must often happen that the student is referred to authorities even by the best teachers. Both faults are traceable to certain indolence and vagueness of thought, the sad effects of which are not confined to subsequent scientific studies. But certainly the best remedy for both is to be found in mathematics, where there is absolute certainty in the reasoning, and no authority is recognized but that of one’s own intelligence.
In 'On the Relation of Natural Science to Science in general', Popular Lectures on Scientific Subjects, translated by E. Atkinson (1900), 25-26.
I do not know if God is a mathematician, but mathematics is the loom on which God weaves the universe.
In The Loom of God: Tapestries of Mathematics and Mysticism (1997, 2009), Introduction, 10.
I do not remember having felt, as a boy, any passion for mathematics, and such notions as I may have had of the career of a mathematician were far from noble. I thought of mathematics in terms of examinations and scholarships: I wanted to beat other boys, and this seemed to be the way in which I could do so most decisively.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 144.
I do not think the division of the subject into two parts - into applied mathematics and experimental physics a good one, for natural philosophy without experiment is merely mathematical exercise, while experiment without mathematics will neither sufficiently discipline the mind or sufficiently extend our knowledge in a subject like physics.
to Henry Roscoe, Professor of Chemistry at Owens College (2 Jun 1870), B.C.S Archive Quoted in R.H. Kargon, Science in Victorian Manchester (1977), 215.
I do present you with a man of mine
Cunning in music and the mathematics
To instruct her fully in those sciences.
Cunning in music and the mathematics
To instruct her fully in those sciences.
Referring to Hortensio in The Taming of the Shrew (1594), Act 2, Scene 1, in The Plays of William Shakespeare (1813), 242.
I don’t believe in mathematics.
A offhand remark when discussing the rigid rules of mathematics in a café conversation with engineer Gustave Ferrière. As quoted in Denis Brian, Einstein—A Life (1996), 76.
I don’t know anything about mathematics; can’t even do proportion. But I can hire all the good mathematicians I need for fifteen dollars a week.
As quoted in French Strother, 'The Modern Profession of Inventing', World's Work and Play (Jul 1905), 6, No. 32, 187.
I don’t think it is proper at all to take the position that C. P. Snow has: namely, that the science—the knowledge, the mathematical side of life—runs in an opposite direction to the life of spontaneous humanistic action. They supplement each other. In literature, for instance, writing sonnets: it takes a lot of practice to make that kind of structure become something that just pours out, but when it does pour out, it is possible to say things that cannot be said without the sonnet form. Form and expression are very close together.
In Diane K. Osbon (ed.), A Joseph Campbell Companion: Reflections on the Art of Living (Collected Works of Joseph Campbell) (1991, 1995), 261.
I grew up in Brooklyn, New York … a city neighborhood that included houses, lampposts, walls, and bushes. But with an early bedtime in the winter, I could look out my window and see the stars, and the stars were not like anything else in my neighborhood. [At age 5] I didn’t know what they were.
[At age 9] my mother … said to me, “You have a library card now, and you know how to read. Take the streetcar to the library and get a book on stars.” … I stepped up to the big librarian and asked for a book on stars. … I sat down and found out the answer, which was something really stunning.I found out that the stars are glowing balls of gas. I also found out that the Sun is a star but really close and that the stars are all suns except really far away I didn’t know any physics or mathematics at that time, but I could imagine how far you’d have to move the Sun away from us till it was only as bright as a star. It was in that library, reading that book, that the scale of the universe opened up to me. There was something beautiful about it.
At that young age, I already knew that I’d be very happy if I could devote my life to finding out more about the stars and the planets that go around them. And it’s been my great good fortune to do just that.
[At age 9] my mother … said to me, “You have a library card now, and you know how to read. Take the streetcar to the library and get a book on stars.” … I stepped up to the big librarian and asked for a book on stars. … I sat down and found out the answer, which was something really stunning.I found out that the stars are glowing balls of gas. I also found out that the Sun is a star but really close and that the stars are all suns except really far away I didn’t know any physics or mathematics at that time, but I could imagine how far you’d have to move the Sun away from us till it was only as bright as a star. It was in that library, reading that book, that the scale of the universe opened up to me. There was something beautiful about it.
At that young age, I already knew that I’d be very happy if I could devote my life to finding out more about the stars and the planets that go around them. And it’s been my great good fortune to do just that.
Quoted in interview with Jack Rightmyer, in 'Stars in His Eyes', Highlights For Children (1 Jan 1997). Ages as given in Tom Head (ed.), Conversations with Carl Sagan (2006), x.
I had a dislike for [mathematics], and ... was hopelessly short in algebra. ... [One extraordinary teacher of mathematics] got the whole year's course into me in exactly six [after-school] lessons of half an hour each. And how? More accurately, why? Simply because he was an algebra fanatic—because he believed that algebra was not only a science of the utmost importance, but also one of the greatest fascination. ... [H]e convinced me in twenty minutes that ignorance of algebra was as calamitous, socially and intellectually, as ignorance of table manners—That acquiring its elements was as necessary as washing behind the ears. So I fell upon the book and gulped it voraciously. ... To this day I comprehend the binomial theorem.
In Prejudices: third series (1922), 261-262.
For a longer excerpt, see H. L. Mencken's Recollections of School Algebra.
For a longer excerpt, see H. L. Mencken's Recollections of School Algebra.
I had a feeling once about Mathematics—that I saw it all. Depth beyond depth was revealed to me—the Byss and Abyss. I saw—as one might see the transit of Venus or even the Lord Mayor’s Show—a quantity passing through infinity and changing its sign from plus to minus. I saw exactly why it happened and why the tergiversation was inevitable but it was after dinner and I let it go.
In Sir Winston Churchill: A Self-Portrait (1954), 38.
I had begun it, it will now be unnecessary for me to finish it.[At a late age, expressing his enthusiasm for mathematics had gone, as when informed of some other mathematician's current work.]
As quoted by Charles Hutton in A Philosophical and Mathematical Dictionary (1815), Vol. 1, 708.
I had intended to major in physics … I could never seem to get the labs to come out right. So I switched to math and have been interested in it ever since.
In 'A Personal Profile of Karen K. Uhlenbeck', collected in Susan Ambrose et al., Journeys of Women in Science and Engineering, No Universal Constants (1999). Uhlenbeck’s father was an engineer and her father-in-law was a famous physicist.
I have a true aversion to teaching. The perennial business of a professor of mathematics is only to teach the ABC of his science; most of the few pupils who go a step further, and usually to keep the metaphor, remain in the process of gathering information, become only Halbwisser [one who has superficial knowledge of the subject], for the rarer talents do not want to have themselves educated by lecture courses, but train themselves. And with this thankless work the professor loses his precious time.
Letter to Heinrich Olbers (26 Oct 1802). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 414.
I have always been very fond of mathematics—for one short period, I even toyed with the possibility of abandoning chemistry in its favour. I enjoyed immensely both its conceptual and formal beauties, and the precision and elegance of its relationships and transformations. Why then did I not succumb to its charms? … because by and large, mathematics lacks the sensuous elements which play so large a role in my attraction to chemistry.I love crystals, the beauty of their forms and formation; liquids, dormant, distilling, sloshing! The fumes, the odors—good or bad, the rainbow of colors; the gleaming vessels of every size, shape and purpose.
In Arthur Clay Cope Address, Chicago (28 Aug 1973). In O. T. Benfey and P. J. T. Morris (eds.), Robert Burns Woodward. Architect and Artist in the World of Molecules (2001), 427.
I have before mentioned mathematics, wherein algebra gives new helps and views to the understanding. If I propose these it is not to make every man a thorough mathematician or deep algebraist; but yet I think the study of them is of infinite use even to grown men; first by experimentally convincing them, that to make anyone reason well, it is not enough to have parts wherewith he is satisfied, and that serve him well enough in his ordinary course. A man in those studies will see, that however good he may think his understanding, yet in many things, and those very visible, it may fail him. This would take off that presumption that most men have of themselves in this part; and they would not be so apt to think their minds wanted no helps to enlarge them, that there could be nothing added to the acuteness and penetration of their understanding.
In The Conduct of the Understanding, Sect. 7.
I have come to the conclusion that the exertion, without which a knowledge of mathematics cannot be acquired, is not materially increased by logical rigor in the method of instruction.
In Jahresbericht der Deutschen Mathematiker Vereinigung (1898), 143.
I have deeply regretted that I did not proceed far enough [as a Cambridge undergraduate] at least to understand something of the great leading principles of mathematics; for men thus endowed seem to have an extra sense.
In Charles Darwin and Francis Darwin (ed.), 'Autobiography', The Life and Letters of Charles Darwin (1887, 1896), Vol. 1, 40.
I have mentioned mathematics as a way to settle in the mind a habit of reasoning closely and in train; not that I think it necessary that all men should be deep mathematicians, but that, having got the way of reasoning which that study necessarily brings the mind to, they might be able to transfer it to other parts of knowledge, as they shall have occasion. For in all sorts of reasoning, every single argument should be managed as a mathematical demonstration; the connection and dependence of ideas should be followed till the mind is brought to the source on which it bottoms, and observes the coherence all along; …
In The Conduct of the Understanding, Sect. 7.
I have never done anything 'useful'. No discovery of mine has made, or is likely to make, directly or indirectly, for good or ill, the least difference to the amenity of the world... Judged by all practical standards, the value of my mathematical life is nil; and outside mathematics it is trivial anyhow. I have just one chance of escaping a verdict of complete triviality, that I may be judged to have created something worth creating. And that I have created something is undeniable: the question is about its value.
A Mathematician's Apology (1940), 90-1.
I have never done anything “useful.” No discovery of mine has made, or is likely to make, directly or indirectly, for good or ill, the least difference to the amenity of the world... Judged by all practical standards, the value of my mathematical life is nil; and outside mathematics it is trivial anyhow. I have just one chance of escaping a verdict of complete triviality, that I may be judged to have created something worth creating. And that I have created something is undeniable: the question is about its value. [The things I have added to knowledge do not differ from] the creations of the other artists, great or small, who have left some kind of memorial beind them.
Concluding remarks in A Mathmatician's Apology (1940, 2012), 150-151.
I have never thought a boy should undertake abstruse or difficult sciences, such as Mathematics in general, till fifteen years of age at soonest. Before that time they are best employed in learning the languages, which is merely a matter of memory.
Letter to Ralph Izard (17 July 1788).
I have no doubt that many small strikes of a hammer will finally have as much effect as one very heavy blow: I say as much in quantity, although they may be different in mode, but in my opinion, everything happens in nature in a mathematical way, and there is no quantity that is not divisible into an infinity of parts; and Force, Movement, Impact etc. are types of quantities.
From the original French, “Ie ne doute point que plusieurs petits coups de Marteau ne fassent enfin autant d’effet qu’vn fort grand coup, ie dis autant en quantité, bien qu’ils puissent estre différents, in modo; mais apud me omnia fiunt Mathematicè in Natura, & il n’y a point de quantité qui ne soit divisible en une infinité de parties; Or la Force, le Mouuement, la Percussion, &c. sont des Especes de quantitez,” in letter (11 Mar 1640) to Père Marin Mersenne (AT III 36), collected in Lettres de Mr Descartes (1659), Vol. 2, 211-212. English version by Webmaster using online resources.
I have often noticed that when people come to understand a mathematical proposition in some other way than that of the ordinary demonstration, they promptly say, “Oh, I see. That’s how it must be.” This is a sign that they explain it to themselves from within their own system.
Lichtenberg: A Doctrine of Scattered Occasions: Reconstructed From: Reconstructed From His Aphorisms and Reflections (1959), 291.
I have often thought that an interesting essay might be written on the influence of race on the selection of mathematical methods. methods. The Semitic races had a special genius for arithmetic
and algebra, but as far as I know have never produced a single geometrician of any eminence. The Greeks on the other hand adopted a geometrical procedure wherever it was possible, and they even treated arithmetic as a branch of geometry by means of the device of representing numbers by lines.
In A History of the Study of Mathematics at Cambridge (1889), 123
I have said that mathematics is the oldest of the sciences; a glance at its more recent history will show that it has the energy of perpetual youth. The output of contributions to the advance of the science during the last century and more has been so enormous that it is difficult to say whether pride in the greatness of achievement in this subject, or despair at his inability to cope with the multiplicity of its detailed developments, should be the dominant feeling of the mathematician. Few people outside of the small circle of mathematical specialists have any idea of the vast growth of mathematical literature. The Royal Society Catalogue contains a list of nearly thirty- nine thousand papers on subjects of Pure Mathematics alone, which have appeared in seven hundred serials during the nineteenth century. This represents only a portion of the total output, the very large number of treatises, dissertations, and monographs published during the century being omitted.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 285.
I know that the majority of those who are not only considered intellectual people, but actually are very intellectual, able to understand the most difficult scientic, mathematical, and philosophical discussions, are very rarely able to understand even the simplest and most obvious truth, if it involves a consequent admission that a judgment concerning some subject, which they have reached often with great effort, a judgment of which they are proud, with which they have indoctrinated others, and on the foundation of which they have built their whole lives-that this judgment may possibly be false.
First sentence of Chap. 14, 'The Sense of Art is Atrophied', What is Art? (1898), 207, as translated by Charles Johnston. [Note: scientic (sic) is as printed.]
I know, indeed, and can conceive of no pursuit so antagonistic to the cultivation of the oratorical faculty … as the study of Mathematics. An eloquent mathematician must, from the nature of things, ever remain as rare a phenomenon as a talking fish, and it is certain that the more anyone gives himself up to the study of oratorical effect the less will he find himself in a fit state to mathematicize.
In Address (22 Feb 1877) for Commemoration Day at Johns Hopkins University. Published as a pamphlet, and reprinted in The Collected Mathematical Papers of James Joseph Sylvester: (1870-1883) (1909), Vol. 3, 72.
https://books.google.com/books?id=wgVbAAAAQAAJ
James Joseph Sylvester - 1877
I learned easily mathematics and physics, as far as these sciences were taken in consideration in the school. I found in this ready help from my father, who loved science and had to teach it himself. He enjoyed any explanation he could give us about Nature and her ways. Unhappily, he had no laboratory and could not perform experiments.
About her childhood schooling. As translated by Charlotte and Vernon Kellogg in Marie Curie, 'Autobiographical Notes', Pierre Curie (1923), 188. [Her father, Władysław Skłodowski, had studied Physics and Mathematics at the St. Petersburg University. For the early years of his career, he was a teacher in Warsaw schools. —Webmaster]]
I learnt to distrust all physical concepts as the basis for a theory. Instead one should put one's trust in a mathematical scheme, even if the scheme does not appear at first sight to be connected with physics. One should concentrate on getting interesting mathematics.
From a 1977 lecture. Quoted in Pesi Rustom Masani, Norbert Wiener, 1894-1964 (1990), 6.
I like to look at mathematics almost more as an art than as a science; for the activity of the mathematician, constantly creating as he is, guided though not controlled by the external world of the senses, bears a resemblance, not fanciful I believe but real, to the activity of an artist, of a painter let us say. Rigorous deductive reasoning on the part of the mathematician may be likened here to technical skill in drawing on the part of the painter. Just as no one can become a good painter without a certain amount of skill, so no one can become a mathematician without the power to reason accurately up to a certain point. Yet these qualities, fundamental though they are, do not make a painter or mathematician worthy of the name, nor indeed are they the most important factors in the case. Other qualities of a far more subtle sort, chief among which in both cases is imagination, go to the making of a good artist or good mathematician.
From 'Fundamental Conceptions and Methods in Mathematics', Bulletin American Mathematical Society (1904), 9, 133. As cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 182.
I liked science. I wasn’t mathematically oriented, so I became an organic chemist.
As quoted in Columbia University Press Release, On Campus (21 Feb 2013).
I love mathematics not only because it is applicable to technology but also because it is beautiful.
In Eberhard Zeidler, Quantum Field Theory (2006), 955.
I maintain that in every special natural doctrine only so much science proper is to be met with as mathematics; for… science proper, especially [science] of nature, requires a pure portion, lying at the foundation of the empirical, and based upon a priori knowledge of natural things. … To the possibility of a determinate natural thing, and therefore to cognise it à priori, is further requisite that the intuition corresponding à priori to the conception should be given; in other words, that the conception should be constructed. But the cognition of the reason through construction of conceptions is mathematical. A pure philosophy of nature in general, namely, one that only investigates what constitutes a nature in general, may thus be possible without mathematics; but a pure doctrine of nature respecting determinate natural things (corporeal doctrine and mental doctrine), is only possible by means of mathematics; and as in every natural doctrine only so much science proper is to be met with therein as there is cognition à priori, a doctrine of nature can only contain so much science proper as there is in it of applied mathematics.
From Preface to The Metaphysical Foundations of Natural Science (1786), as translated by Ernest Belford Boax, in Kant’s Prolegomena: And The Metaphysical Foundations of Natural Science (1883), 140.
I never could do anything with figures, never had any talent for mathematics, never accomplished anything in my efforts at that rugged study, and to-day the only mathematics I know is multiplication, and the minute I get away up in that, as soon as I reach nine times seven— [He lapsed into deep thought, trying to figure nine times seven. Mr. McKelway whispered the answer to him.] I’ve got it now. It’s eighty-four. Well, I can get that far all right with a little hesitation. After that I am uncertain, and I can’t manage a statistic.
Speech at the New York Association for Promoting the Interests of the Blind (29 Mar 1906). In Mark Twain and William Dean Howells (ed.), Mark Twain’s Speeches? (1910), 323.
I presume that few who have paid any attention to the history of the Mathematical Analysis, will doubt that it has been developed in a certain order, or that that order has been, to a great extent, necessary—being determined, either by steps of logical deduction, or by the successive introduction of new ideas and conceptions, when the time for their evolution had arrived. And these are the causes that operate in perfect harmony. Each new scientific conception gives occasion to new applications of deductive reasoning; but those applications may be only possible through the methods and the processes which belong to an earlier stage.
Explaining his choice for the exposition in historical order of the topics in A Treatise on Differential Equations (1859), Preface, v-vi.
I propose to put forward an apology for mathematics; and I may be told that it needs none, since there are now few studies more generally recognized, for good reasons or bad, as profitable and praiseworthy.
In A Mathematician's Apology (1940, 2012), 63-64.
I read … that geometry is the art of making no mistakes in long calculations. I think that this is an underestimation of geometry. Our brain has two halves: one is responsible for the multiplication of polynomials and languages, and the other half is responsible for orientation of figures in space and all the things important in real life. Mathematics is geometry when you have to use both halves.
In S.H. Lui, 'An Interview with Vladimir Arnol’d', Notices of the AMS (Apr 1997) 44, No. 4, 438. Reprinted from the Hong Kong Mathematics Society (Feb 1996).
I should like to draw attention to the inexhaustible variety of the problems and exercises which it [mathematics] furnishes; these may be graduated to precisely the amount of attainment which may be possessed, while yet retaining an interest and value. It seems to me that no other branch of study at all compares with mathematics in this. When we propose a deduction to a beginner we give him an exercise in many cases that would have been admired in the vigorous days of Greek geometry. Although grammatical exercises are well suited to insure the great benefits connected with the study of languages, yet these exercises seem to me stiff and artificial in comparison with the problems of mathematics. It is not absurd to maintain that Euclid and Apollonius would have regarded with interest many of the elegant deductions which are invented for the use of our students in geometry; but it seems scarcely conceivable that the great masters in any other line of study could condescend to give a moment’s attention to the elementary books of the beginner.
In Conflict of Studies (1873), 10-11.
I should rejoice to see mathematics taught with that life and animation which the presence and example of her young and buoyant sister [natural and experimental science] could not fail to impart, short roads preferred to long ones.
From Presidential Address (1869) to the British Association, Exeter, Section A, collected in Collected Mathematical Papers of Lames Joseph Sylvester (1908), Vol. 2, 657.
I spent most of a lifetime trying to be a mathematician—and what did I learn. What does it take to be one? I think I know the answer: you have to be born right, you must continually strive to become perfect, you must love mathematics more than anything else, you must work at it hard and without stop, and you must never give up.
In I Want to be a Mathematician: an Automathography (1985), 400.
I started studying law, but this I could stand just for one semester. I couldn’t stand more. Then I studied languages and literature for two years. After two years I passed an examination with the result I have a teaching certificate for Latin and Hungarian for the lower classes of the gymnasium, for kids from 10 to 14. I never made use of this teaching certificate. And then I came to philosophy, physics, and mathematics. In fact, I came to mathematics indirectly. I was really more interested in physics and philosophy and thought about those. It is a little shortened but not quite wrong to say: I thought I am not good enough for physics and I am too good for philosophy. Mathematics is in between.
From interview on his 90th birthday. In D J Albers and G L Alexanderson (eds.), Mathematical People: Profiles and Interviews (1985), 245-254.
I tell them if they will occupy themselves with the study of mathematics they will find in it the best remedy against the lusts of the flesh.
The Magic Mountain (1924, 1965), 417.
I think I did pretty well, considering I started out with nothing but a bunch of blank paper.
As collected, without citation, in Lilless McPherson Shilling and Linda K. Fuller (eds.), Dictionary of Quotations in Communications (), 279.
I think it is a peculiarity of myself that I like to play about with equations, just looking for beautiful mathematical relations which maybe don’t have any physical meaning at all. Sometimes they do.
At age 60.
At age 60.
"Interview with T. Kuhn (7 May 1963), Niels Bohr Library, American Intitute of Physics, New York. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 109.
I think it would be desirable that this form of word [mathematics] should be reserved for the applications of the science, and that we should use mathematic in the singular to denote the science itself, in the same way as we speak of logic, rhetoric, or (own sister to algebra) music.
In Presidential Address to the British Association, Exeter British Association Report (1869); Collected Mathematical Papers, Vol. 2, 669.
I think of myself as a journalist who writes mainly about math and science, and a few other fields of interest.
In Kendrick Frazier, 'A Mind at Play: An Interview with Martin Gardner', Skeptical Inquirer (Mar/Apr 1998), 22, No. 2, 36.
I think that it is a relatively good approximation to truth—which is much too complicated to allow anything but approximations—that mathematical ideas originate in empirics.
From 'The Mathematician', collected in James Roy Newman (ed.), The World of Mathematics (1956), Vol. 4, 2063.
I think that the difference between pure and applied mathematics is social rather than scientific. A pure mathematician is paid for making mathematical discoveries. An applied mathematician is paid for the solution of given problems.
When Columbus set sail, he was like an applied mathematician, paid for the search of the solution of a concrete problem: find a way to India. His discovery of the New World was similar to the work of a pure mathematician.
When Columbus set sail, he was like an applied mathematician, paid for the search of the solution of a concrete problem: find a way to India. His discovery of the New World was similar to the work of a pure mathematician.
In S.H. Lui, 'An Interview with Vladimir Arnol’d', Notices of the AMS (Apr 1997) 44, No. 4, 438. Reprinted from the Hong Kong Mathematics Society (Feb 1996).
I transferred to … UCLA, … and I took several courses there. One was an acting class…; another was a course in television writing, which seemed practical. I also continued my studies in philosophy. I had done pretty well in symbolic logic at Long Beach, so I signed up for Advanced Symbolic Logic at my new school. Saying that I was studying Advanced Symbolic Logic at UCLA had a nice ring; what had been nerdy in high school now had mystique. However, I went to class the first day and discovered that UCLA used a different set of symbols from those I had learned at Long Beach. To catch
up, I added a class in Logic 101, which meant I was studying beginning logic and advanced logic at the same time. I was overwhelmed, and shocked to find that I couldn’t keep up. I had reached my math limit as well as my philosophy limit. I abruptly changed my major to theater and, free from the workload of my logic classes…. I realized that I was now investing in no other future but show business.
In Born Standing Up: A Comic’s Life (2007), 103.
I wanted certainty in the kind of way in which people want religious faith. I thought that certainty is more likely to be found in mathematics than elsewhere. But I discovered that many mathematical demonstrations, which my teachers expected me to accept, were full of fallacies, and that, if certainty were indeed discoverable in mathematics, it would be in a new field of mathematics, with more solid foundations than those that had hitherto been thought secure. But as the work proceeded, I was continually reminded of the fable about the elephant and the tortoise. Having constructed an elephant upon which the mathematical world could rest, I found the elephant tottering, and proceeded to construct a tortoise to keep the elephant from falling. But the tortoise was no more secure than the elephant, and after some twenty years of very arduous toil, I came to the conclusion that there was nothing more that I could do in the way of making mathematical knowledge indubitable.
In 'Reflections on my Eightieth Birthday', Portraits from Memory (1956), 54.
I was reading in an article on Bizet not long ago that music has ceased to be an art and has become a science—in which event it must have a mathematical future!
In letter to H.E. Krehbiel (1887), collected in Elizabeth Bisland The Writings of Lafcadio Hearn (1922), Vol. 14, 8.
I was x years old in the year x2.
When asked about his age (43).
When asked about his age (43).
Quoted in H. Eves, In Mathematical Circles (1969).
I will not go so far as to say that to construct a history of thought without profound study of the mathematical ideas of successive epochs is like omitting Hamlet from the play which is named after him. That would be claiming too much. But it is certainly analogous to cutting out the part of Ophelia. This simile is singularly exact. For Ophelia is quite essential to the play, she is very charming-and a little mad. Let us grant that the pursuit of mathematics is a divine madness of the human spirit, a refuge from the goading urgency of contingent happenings.
In Science and the Modern World (1926), 31.
I would have my son mind and understand business, read little history, study the mathematics and cosmography; these are good, with subordination to the things of God. … These fit for public services for which man is born.
In Letters and Speeches of Oliver Cromwell (1899), Vol. 1, 371.
I’m sorry to say that the subject I most disliked was mathematics. I have thought about it. I think the reason was that mathematics leaves no room for argument. If you made a mistake, that was all there was to it.
The Autobiography of Malcolm X (1965, 1999), 34.
If a lunatic scribbles a jumble of mathematical symbols it does not follow that the writing means anything merely because to the inexpert eye it is indistinguishable from higher mathematics.
In Men of Mathematics (1937), Vol. 2, 232. Also in J.R. Newman (ed.) The World of Mathematics (1956, 1960), Vol. 1, 308.
If a man is in any sense a real mathematician, then it is a hundred to one that his mathematics will be far better than anything else he can do, and that it would be silly if he surrendered any decent opportunity of exercising his one talent in order to do undistinguished work in other fields. Such a sacrifice could be justified only by economic necessity of age.
In A Mathematician's Apology (1940, 2012), 70.
If a man's wit be wandering, let him study the mathematics; for in demonstrations, if his wit be called away never so little, he must begin again.
Translation in Francis Bacon, James Spedding (ed.) et al., Works of Francis Bacon (1858) Vol. 6, 498.
If a nonnegative quantity was so small that it is smaller than any given one, then it certainly could not be anything but zero. To those who ask what the infinitely small quantity in mathematics is, we answer that it is actually zero. Hence there are not so many mysteries hidden in this concept as they are usually believed to be. These supposed mysteries have rendered the calculus of the infinitely small quite suspect to many people. Those doubts that remain we shall thoroughly remove in the following pages, where we shall explain this calculus.
…...
If all sentient beings in the universe disappeared, there would remain a sense in which mathematical objects and theorems would continue to exist even though there would be no one around to write or talk about them. Huge prime numbers would continue to be prime, even if no one had proved them prime.
In When You Were a Tadpole and I Was a Fish: And Other Speculations About This and That (), 124.
If Bacon erred here [in valuing mathematics only for its uses], we must acknowledge that we greatly prefer his error to the opposite error of Plato. We have no patience with a philosophy which, like those Roman matrons who swallowed abortives in order to preserve their shapes, takes pains to be barren for fear of being homely.
In 'Lord Bacon', Edinburgh Review (Jul 1837). Collected in Critical and Miscellaneous Essays: Contributed to the Edinburgh Review (1857), Vol. 1, 396.
If I feel unhappy, I do mathematics to become happy. If I am happy, I do mathematics to keep happy.
In P. Turán, 'The Work of Alfréd Rényi', Matematikai Lapok (1970), 21, 199-210.
If it is true as Whewell says, that the essence of the triumphs of Science and its progress consists in that it enables us to consider evident and necessary, views which our ancestors held to be unintelligible and were unable to comprehend, then the extension of the number concept to include the irrational, and we will at once add, the imaginary, is the greatest forward step which pure mathematics has ever taken.
In Theorie der Complexen Zahlensysteme (1867), 60. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 281. From the original German, “Wenn es wahr ist, dass, wie Whewell meint, das Wesen der Triumphe der Wissenschaft und ihres Fortschrittes darin besteht, dass wir veranlasst werden, Ansichten, welche unsere Vorfahren für unbegreiflich hielten und unfähig waren zu begreifen, für evident und nothwendig zu halten, so war die Erweiterung des Zahlenbegriffes auf das Irrationale, und wollen wir sogleich hinzufügen, das Imaginäre, der grösste Fortschritt, den die reine Mathematik jemals gemacht hat.”
If it’s green or wriggles, it’s biology. If it stinks, it’s chemistry. If it doesn’t work, it’s physics or engineering. If it’s green and wiggles and stinks and still doesn’t work, it’s psychology. If it’s incomprehensible, it’s mathematics. If it puts you to sleep, it’s statistics.
In Journal of the South African Institute of Mining and Metallurgy (1978), 79, 97, and additional lines from other sources.
If logical training is to consist, not in repeating barbarous scholastic formulas or mechanically tacking together empty majors and minors, but in acquiring dexterity in the use of trustworthy methods of advancing from the known to the unknown, then mathematical investigation must ever remain one of its most indispensable instruments. Once inured to the habit of accurately imagining abstract relations, recognizing the true value of symbolic conceptions, and familiarized with a fixed standard of proof, the mind is equipped for the consideration of quite other objects than lines and angles. The twin treatises of Adam Smith on social science, wherein, by deducing all human phenomena first from the unchecked action of selfishness and then from the unchecked action of sympathy, he arrives at mutually-limiting conclusions of transcendent practical importance, furnish for all time a brilliant illustration of the value of mathematical methods and mathematical discipline.
In 'University Reform', Darwinism and Other Essays (1893), 297-298.
If one be bird-witted, that is easily distracted and unable to keep his attention as long as he should, mathematics provides a remedy; for in them if the mind be caught away but a moment, the demonstration has to be commenced anew.
In De Augmentis, Bk. 6; Advancement of Learning, Bk. 2.
If others would but reflect on mathematical truths as deeply and as continuously as I have, they would make my discoveries.
As quoted, without citation, in Eric Temple Bell, Men of Mathematics (1945), 254.
If people do not believe that mathematics is simple, it is only because they do not realize how complicated life is.
In speech to first national meeting of the Association for Computing Machinery (1947), as quoted in Franz L. Alt, 'Archaeology of computers: Reminiscences, 1945-1947', Communications of the ACM (Jul 1972), 15, No. 7, 694.
If physics leads us today to a world view which is essentially mystical, it returns, in a way, to its beginning, 2,500 years ago. ... This time, however, it is not only based on intuition, but also on experiments of great precision and sophistication, and on a rigorous and consistent mathematical formalism.
In The Tao of Physics (1975), 19.
If scientific reasoning were limited to the logical processes of arithmetic, we should not get very far in our understanding of the physical world. One might as well attempt to grasp the game of poker entirely by the use of the mathematics of probability.
Endless Horizons (1946), 27.
If the average man in the street were asked to name the benefits derived from sunshine, he would probably say “light and warmth” and there he would stop. But, if we analyse the matter a little more deeply, we will soon realize that sunshine is the one great source of all forms of life and activity on this old planet of ours. … [M]athematics underlies present-day civilization in much the same far-reaching manner as sunshine underlies all forms of life, and that we unconsciously share the benefits conferred by the mathematical achievements of the race just as we unconsciously enjoy the blessings of the sunshine.
From Address (25 Feb 1928) to National Council of Teachers of Mathematics, Boston. Abstract published in 'Mathematics and Sunshine', The Mathematics Teacher (May 1928), 21, No. 5, 245.
If the NSF had never existed, if the government had never funded American mathematics, we would have half as many mathematicians as we now have, and I don’t see anything wrong with that.
From interview (1981) with Donald J. Albers. In John H. Ewing and Frederick W. Gehring, Paul Halmos Celebrating 50 Years of Mathematics (1991), 3.
If the proof starts from axioms, distinguishes several cases, and takes thirteen lines in the text book … it may give the youngsters the impression that mathematics consists in proving the most obvious things in the least obvious way.
Mathematical Discovery: on Understanding, Learning, and Teaching Problem Solving (1981), 129.
If there be some who, though ignorant of all mathematics, take upon them to judge of these, and dare to reprove this work, because of some passage of Scripture, which they have miserably warped to their purpose, I regard them not, and even despise their rash judgment. … What I have done in this matter, I submit principally to your Holiness, and then to the judgment of all learned mathematicians. And that I may not seem to promise your Holiness more concerning the utility of this work than I am able to perform, I pass now to the work itself.
The dedication to Pope Paul III in the Preface of De Revolutionibus Orbium Coelestium, (On the Revolutions of Heavenly Spheres), originally written in Latin. As translated and quoted in Sarah K. Bolton, Famous Men of Science (1926), 7-8. Webmaster, as yet, has not found this quotation, in these words verbatim, as part of a complete book translation of the original Latin by Copernicus. However, it is consistent with other translations.
If there is anything that can bind the heavenly mind of man to this dreary exile of our earthly home and can reconcile us with our fate so that one can enjoy living,—then it is verily the enjoyment of the mathematical sciences and astronomy.
In a letter to his son-in-law, Jakob Bartsch. Quoted in Norman Davidson, Sky Phenomena (2004), 131. Also see Johannes Kepler and Carola Baumgardt (ed.), Johannes Kepler: Life and Letters (1951), 190.
If there is something very slightly wrong in our definition of the theories, then the full mathematical rigor may convert these errors into ridiculous conclusions.
Feynman Lectures on Gravitation, edited by Brian Hatfield (2002), 21.
If we compare a mathematical problem with an immense rock, whose interior we wish to penetrate, then the work of the Greek mathematicians appears to us like that of a robust stonecutter, who, with indefatigable perseverance, attempts to demolish the rock gradually from the outside by means of hammer and chisel; but the modern mathematician resembles an expert miner, who first constructs a few passages through the rock and then explodes it with a single blast, bringing to light its inner treasures.
In Die Entwickelung der Mathematik in den letzten Jahrhunderten (1869), 9. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 114. From the original German, “Vergleichen wir ein mathematisches Problem mit einem gewaltigen Felsen, in dessen Inneres wir eindringen wollen, so erscheint die Arbeit der griechischen Mathematiker uns als die eines rüstigen Steinhauers, der mit Hammer und Meissel in unermüdlicher Ausdauer den Felsen langsam von aussen her zu zerbröckeln beginnt; der moderne Mathematiker aber als ein trefflicher Minirer, der diesen Felsen zunächst mit wenigen Gängen durchzieht, von denen aus er dann den Felsblock mit einem gewaltigem Schlage zersprengt und die Schätze des Inneren zu Tage fördert.”
If we consider the nature of a deductive proof, we recognize at once that there must be a hypothesis. It is clear, then, that the starting point of any mathematical science must be a set of one or more propositions which remain entirely unproved. This is essential: without it a vicious circle is unavoidable.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 3.
If we ever establish contact with intelligent aliens living on a planet around a distant star … They would be made of similar atoms to us. They could trace their origins back to the big bang 13.7 billion years ago, and they would share with us the universe's future. However, the surest common culture would be mathematics.
In 'Take Me to Your Mathematician', New Scientist (14 Feb 2009), 201, No. 2695.
If we go back to our chequer game, the fundamental laws are rules by which the chequers move. Mathematics may be applied in the complex situation to figure out what in given circumstances is a good move to make. But very little mathematics is needed for the simple fundamental character of the basic laws. They can be simply stated in English for chequers.
In The Character of Physical Law (1965), 36.
If we had a thorough knowledge of all the parts of the seed of any animal (e.g., man), we could from that alone, by reasons entirely mathematical and certain, deduce the whole conformation and figure of each of its members, and, conversely, if we knew several peculiarities of this conformation, we would from those deduce the nature of its seed.
If we survey the mathematical works of Sylvester, we recognize indeed a considerable abundance, but in contradistinction to Cayley—not a versatility toward separate fields, but, with few exceptions—a confinement to arithmetic-algebraic branches. …
The concept of Function of a continuous variable, the fundamental concept of modern mathematics, plays no role, is indeed scarcely mentioned in the entire work of Sylvester—Sylvester was combinatorist [combinatoriker].
The concept of Function of a continuous variable, the fundamental concept of modern mathematics, plays no role, is indeed scarcely mentioned in the entire work of Sylvester—Sylvester was combinatorist [combinatoriker].
In Mathematische Annalen (1898), Bd.50, 134-135. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 173.
If we view mathematical speculations with reference to their use, it appears that they should be divided into two classes. To the first belong those which furnish some marked advantage either to common life or to some art, and the value of such is usually determined by the magnitude of this advantage. The other class embraces those speculations which, though offering no direct advantage, are nevertheless valuable in that they extend the boundaries of analysis and increase our resources and skill. Now since many investigations, from which great advantage may be expected, must be abandoned solely because of the imperfection of analysis, no small value should be assigned to those speculations which promise to enlarge the field of anaylsis.
In Novi Comm. Petr., Vol. 4, Preface.
If we wish to foresee the future of mathematics, our proper course is to study the history and present condition of the science.
Science and Method (1914, 2003), 25.
If you ask ... the man in the street ... the human significance of mathematics, the answer of the world will be, that mathematics has given mankind a metrical and computatory art essential to the effective conduct of daily life, that mathematics admits of countless applications in engineering and the natural sciences, and finally that mathematics is a most excellent instrumentality for giving mental discipline... [A mathematician will add] that mathematics is the exact science, the science of exact thought or of rigorous thinking.
Address (28 Mar 1912), Michigan School Masters' Club, Ann Arbor, 'The Humanization of the Teaching of Mathematics. Printed in Science (26 Apr 1912). Collected in The Human Worth of Rigorous Thinking: Essays and Addresses (1916), 65-66.
If you disregard the very simplest cases, there is in all of mathematics not a single infinite series whose sum has been rigorously determined. In other words, the most important parts of mathematics stand without a foundation.
In Letter to a friend, as quoted in George Finlay Simmons, Calculus Gems (1992), 188.
If you look over my Scientific American columns you will see that they get progressively more sophisticated as I began reading math books and learning more about the subject. There is no better way to learn anything than to write about it!
In Kendrick Frazier, 'A Mind at Play: An Interview with Martin Gardner', Skeptical Inquirer (Mar/Apr 1998), 22, No. 2, 36.
If you want to be a physicist, you must do three things—first, study mathematics, second, study more mathematics, and third, do the same.
Interview with Paul H. Kirkpatrick, in Daniel J. Kevles, The Physicists (1978), 200.
If, unwarned by my example, any man shall undertake and shall succeed in really constructing an engine embodying in itself the whole of the executive department of mathematical analysis upon different principles or by simpler mechanical means, I have no fear of leaving my reputation in his charge, for he alone will be fully able to appreciate the nature of my efforts and the value of their results.
In Passages from the Life of a Philosopher (1864), 450.
Imagination is the Discovering Faculty, pre-eminently. … It is that which feels & discovers what is, the REAL which we see not, which exists not for our senses. … Mathematical science shows what is. It is the language of unseen relations between things. … Imagination too shows what is. … Hence she is or should be especially cultivated by the truly Scientific, those who wish to enter into the worlds around us!
Lovelace Papers, Bodleian Library, Oxford University, 175, folio 199, journal entry for 5 Jan 1841. As quoted and cited in Dorothy Stein (ed.), 'In Time I Will Do All, I Dare Say', Ada: A Life and a Legacy (1985), 128.
Imagine a person with a gift of ridicule [He might say] First that a negative quantity has no logarithm; secondly that a negative quantity has no square root; thirdly that the first non-existent is to the second as the circumference of a circle is to the diameter.
In [great mathematics] there is a very high degree of unexpectedness, combined with inevitability and economy.
In A Mathematician’s Apology (1940, reprint with Foreward by C.P. Snow 1992), 113.
In a class I was taking there was one boy who was much older than the rest. He clearly had no motive to work. I told him that, if he could produce for me, accurately to scale, drawings of the pieces of wood required to make a desk like the one he was sitting at, I would try to persuade the Headmaster to let him do woodwork during the mathematics hours—in the course of which, no doubt, he would learn something about measurement and numbers. Next day, he turned up with this task completed to perfection. This I have often found with pupils; it is not so much that they cannot do the work, as that they see no purpose in it.
In Mathematician's Delight (1943), 52.
In a lot of scientists, the ratio of wonder to skepticism declines in time. That may be connected with the fact that in some fields—mathematics, physics, some others—the great discoveries are almost entirely made by youngsters.
Quoted in interview with magazine staff, Psychology Today (Jan 1996).
In abstract mathematical theorems, the approximation to absolute truth is perfect. … In physical science, on the contrary, we treat of the least quantities which are perceptible.
In The Principles of Science: A Treatise on Logic and Scientific Method (1913), 478.
In addition to this it [mathematics] provides its disciples with pleasures similar to painting and music. They admire the delicate harmony of the numbers and the forms; they marvel when a new discovery opens up to them an unexpected vista; and does the joy that they feel not have an aesthetic character even if the senses are not involved at all? … For this reason I do not hesitate to say that mathematics deserves to be cultivated for its own sake, and I mean the theories which cannot be applied to physics just as much as the others.
(1897) From the original French, “Et surtout, leurs adeptes y trouvent des jouissances analogues á celles que donnent la peinture et la musique. Ils admirent la délicate harmonie des nombres et des formes; ils s’émerveillent quand une découverte nouvelle leur ouvre une perspective inattendue; et la joie qu’ils éprouvent ainsi n’a-t-elle pas le caractère esthétique, bien que les sens n’y prennent aucune part?...C’est pourquoi je n’hésite pas à dire que les mathématiques méritent d’être cultivées pour elles-mêmes et que les théories qui ne peuvent être appliquées á la physique doivent l’être comme les autres.” Address read for him at the First International Congress of Mathematicians in Zurich: '‘Sur les rapports de l’analyse pure et de la physique', in Proceedings of that Congress 81-90, (1898). Also published as 'L’Analyse et la Physique', in La Valeur de la Science (1905), 137-151. As translated in Armand Borel, 'On the Place of Mathematics in Culture', in Armand Borel: Œvres: Collected Papers (1983), Vol. 4, 420-421.
In all that has to do with the relations between man and the supernatural, we have to seek for a more than mathematical precision; this should be more exact than science.
In Gravity and Grace, (1947, 1952), 186.
In both social and natural sciences, the body of positive knowledge grows by the failure of a tentative hypothesis to predict phenomena the hypothesis professes to explain; by the patching up of that hypothesis until someone suggests a new hypothesis that more elegantly or simply embodies the troublesome phenomena, and so on ad infinitum. In both, experiment is sometimes possible, sometimes not (witness meteorology). In both, no experiment is ever completely controlled, and experience often offers evidence that is the equivalent of controlled experiment. In both, there is no way to have a self-contained closed system or to avoid interaction between the observer and the observed. The Gödel theorem in mathematics, the Heisenberg uncertainty principle in physics, the self-fulfilling or self-defeating prophecy in the social sciences all exemplify these limitations.
Inflation and Unemployment (1976), 348.
In destroying the predisposition to anger, science of all kind is useful; but the mathematics possess this property in the most eminent degree.
Quoted in Day, Collacon (no date).
In early times, medicine was an art, which took its place at the side of poetry and painting; to-day, they try to make a science of it, placing it beside mathematics, astronomy, and physics.
In Armand Trousseau and John Rose Cormack (trans.), Lectures on Clinical Medicine: Delivered at the Hôtel-Dieu, Paris (1869), Vol. 2, 40.
In Euclid each proposition stands by itself; its connection with others is never indicated; the leading ideas contained in its proof are not stated; general principles do not exist. In modern methods, on the other hand, the greatest importance is attached to the leading thoughts which pervade the whole; and general principles, which bring whole groups of theorems under one aspect, are given rather than separate propositions. The whole tendency is toward generalization. A straight line is considered as given in its entirety, extending both ways to infinity, while Euclid is very careful never to admit anything but finite quantities. The treatment of the infinite is in fact another fundamental difference between the two methods. Euclid avoids it, in modern mathematics it is systematically introduced, for only thus is generality obtained.
In 'Geometry', Encyclopedia Britannica (9th edition).
In every case the awakening touch has been the mathematical spirit, the attempt to count, to measure, or to calculate. What to the poet or the seer may appear to be the very death of all his poetry and all his visions—the cold touch of the calculating mind,—this has proved to be the spell by which knowledge has been born, by which new sciences have been created, and hundreds of definite problems put before the minds and into the hands of diligent students. It is the geometrical figure, the dry algebraical formula, which transforms the vague reasoning of the philosopher into a tangible and manageable conception; which represents, though it does not fully describe, which corresponds to, though it does not explain, the things and processes of nature: this clothes the fruitful, but otherwise indefinite, ideas in such a form that the strict logical methods of thought can be applied, that the human mind can in its inner chamber evolve a train of reasoning the result of which corresponds to the phenomena of the outer world.
In A History of European Thought in the Nineteenth Century (1896), Vol. 1, 314.
In every department of physical science there is only so much science, properly so-called, as there is mathematics.
…...
In fact, Gentlemen, no geometry without arithmetic, no mechanics without geometry... you cannot count upon success, if your mind is not sufficiently exercised on the forms and demonstrations of geometry, on the theories and calculations of arithmetic ... In a word, the theory of proportions is for industrial teaching, what algebra is for the most elevated mathematical teaching.
... a l'ouverture du cours de mechanique industrielle á Metz (1827), 2-3, trans. Ivor Grattan-Guinness.
In future times Tait will be best known for his work in the quaternion analysis. Had it not been for his expositions, developments and applications, Hamilton’s invention would be today, in all probability, a mathematical curiosity.
In Bibliotheca Mathematica (1903), 3, 189. As cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 178. [Note: Tait is Peter Guthrie Tait; Hamilton is Sir William Rowan Hamilton. —Webmaster]
In his wretched life of less than twenty-seven years Abel accomplished so much of the highest order that one of the leading mathematicians of the Nineteenth Century (Hermite, 1822-1901) could say without exaggeration, “Abel has left mathematicians enough to keep them busy for five hundred years.” Asked how he had done all this in the six or seven years of his working life, Abel replied, “By studying the masters, not the pupils.”
The Queen of the Sciences (1931, 1938), 10.
In many cases, mathematics is an escape from reality.
In Adventures of a Mathematician (1976), 120. Part of a longer quote that begins: “In many cases, mathematics…” on the Stanislaw Ulam Quotes page of this website.
In many cases, mathematics is an escape from reality. The mathematician finds his own monastic niche and happiness in pursuits that are disconnected from external affairs. Some practice it as if using a drug. Chess sometimes plays a similar role. In their unhappiness over the events of this world, some immerse themselves in a kind of self-sufficiency in mathematics. (Some have engaged in it for this reason alone.)
In Adventures of a Mathematician (1976), 120.
In mathematical analysis we call x the undetermined part of line a: the rest we don’t call y, as we do in common life, but a-x. Hence mathematical language has great advantages over the common language.
Lichtenberg: A Doctrine of Scattered Occasions: Reconstructed From: Reconstructed From His Aphorisms and Reflections (1959), 158.
In mathematics … we find two tendencies present. On the one hand, the tendency towards abstraction seeks to crystallise the logical relations inherent in the maze of materials ... being studied, and to correlate the material in a systematic and orderly
Geometry and the Imagination (New York, 1952).
In mathematics as in other fields, to find one self lost in wonder at some manifestation is frequently the half of a new discovery.
In Werke, Bd. 8 (1897), 233.
In mathematics it [sophistry] had no place from the beginning: Mathematicians having had the wisdom to define accurately the terms they use, and to lay down, as axioms, the first principles on which their reasoning is grounded. Accordingly we find no parties among mathematicians, and hardly any disputes.
In Essays on the Intellectual Powers of Man, Essay 1, chap. 1.
In mathematics it is notorious that we start from absurdities to reach a realm of law, and our whole (mathematical) conception of the world is based on a foundation which we believe to have no existence.
In The Dance of Life (1923), 86.
In mathematics the art of asking questions is more valuable than solving problems.
From doctoral dissertation, 'De aequationibus secundi gradus indeterminatis' (On indeterminate equations of the second degree, 1867), 26, Theses summary, III. As translated from the original Latin, “In re mathematica ars proponendi quaestionem pluris facienda est quam solvendi.” Other translations include: “In mathematics, the art of posing a problem is more important than solving it,” or “In mathematics the art of proposing a question must be held of higher value than solving it.”
In mathematics there are no true controversies. (1811)
This quote is usually seen without any specific source citation. The sense of it is given, not in quotation marks, as “In 1811 Gauss stated that there are no true controversies in mathematics,” in G. Waldo Dunnington, Jeremy Gray and Fritz-Egbert Dohse, Carl Friedrich Gauss: Titan of Science (2003), 418. If you know the primary source, please contact Webmaster.
In mathematics there is no ignorabimus!
This is part of a longer quote that begins, “This conviction of the solvability…”, which has the full citation. See the David Hilbert Quotes page on this website. Note that ignorabimus (first-person plural future active indicative of the Latin verb ignoro) refers to the future: “we will not know” or “we will not be ignorant of”. Compare ignoramus, (first-person plural present active indicative of ignoro) meaning in the present, “we do not know” or “we are ignorant of”.
In mathematics two ends are constantly kept in view: First, stimulation of the inventive faculty, exercise of judgment, development of logical reasoning, and the habit of concise statement; second, the association of the branches of pure mathematics with each other and with applied science, that the pupil may see clearly the true relations of principles and things.
In 'Aim of the Mathematical Instruction', International Commission on Teaching of Mathematics, American Report: United States Bureau of Education: Bulletin 1912, No. 4, 7.
In mathematics we find the primitive source of rationality; and to mathematics must the biologists resort for means to carry out their researches.
The Positive Philosophy, trans. Harriet Martineau (1853), Vol. 1, 388.
In mathematics, … and in natural philosophy since mathematics was applied to it, we see the noblest instance of the force of the human mind, and of the sublime heights to which it may rise by cultivation. An acquaintance with such sciences naturally leads us to think well of our faculties, and to indulge sanguine expectations concerning the improvement of other parts of knowledge. To this I may add, that, as mathematical and physical truths are perfectly uninteresting in their consequences, the understanding readily yields its assent to the evidence which is presented to it; and in this way may be expected to acquire the habit of trusting to its own conclusions, which will contribute to fortify it against the weaknesses of scepticism, in the more interesting inquiries after moral truth in which it may afterwards engage.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 3, 182.
In Mathematics, censorship and criticism can not be allowed to everyone; the speeches of the rhetoricians and the defenses of the lawyers are worthless.
From In Artem Analyticem Isagoge (1591, “Introduction to the Analytic Arts”), translated from the original Latin to French in Édouard Lucas, Récréations Mathématiques (1883), Vol. 2, 1, given as, “Dans les Mathématiques, la censure et la critique ne peuvent être permises à tout le monde; les discours des rhéteurs et les défenses des avocats n’y valent rien.” English version from the French by Webmaster using Google translate.
In mathematics, fractions speak louder than words.
In Evan Esar, 20,000 Quips and Quotes, 509.
In mathematics, if a pattern occurs, we can go on to ask, Why does it occur? What does it signify? And we can find answers to these questions. In fact, for every pattern that appears, a mathematician feels he ought to know why it appears.
in Prelude to mathematics (1955), 23.
In mathematics, which is but a mirror of the society in which it thrives or suffers, the pre-Athenian period is one of colorful men and important discoveries. Sparta, like most militaristic states before and after it, produced nothing. Athens, and the allied Ionians, produced a number of works by philosophers and mathematicians; some good, some controversial, some grossly overrated.
In A History of Pi (1970), 34.
In modern times the belief that the ultimate explanation of all things was to be found in Newtonian mechanics was an adumbration of the truth that all science, as it grows towards perfection, becomes mathematical in its ideas.
In An Introduction to Mathematics (1911), 13-14. [To suggest, disclose, or outline partially, produces an “adumbration”, which gives only the main facts and not the details. —Webmaster]
In most sciences one generation tears down what another has built, and what one has established, another undoes. In mathematics alone each generation adds a new storey to the old structure.
In Die Entwickelung der Mathematik in den letzten Jahrhunderten (1869), 34. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 14. From the original German, “In den meisten Wissenschaften pflegt eine Generation das niederzureissen, was die andere gebaut, und was jene gesetzt, hebt diese auf. In der Mathematik allein setzt jede Generation ein neues Stockwerk auf den alten Unterbau.”
In my opinion the English excel in the art of writing text-books for mathematical teaching; as regards the clear exposition of theories and the abundance of excellent examples, carefully selected, very few books exist in other countries which can compete with those of Salmon and many other distinguished English authors that could be named.
In Projective Geometry (1886), Preface.
In my opinion, there is absolutely no trustworthy proof that talents have been improved by their exercise through the course of a long series of generations. The Bach family shows that musical talent, and the Bernoulli family that mathematical power, can be transmitted from generation to generation, but this teaches us nothing as to the origin of such talents. In both families the high-watermark of talent lies, not at the end of the series of generations, as it should do if the results of practice are transmitted, but in the middle. Again, talents frequently appear in some member of a family which has not been previously distinguished.
In 'On Heredity', Essays upon Heredity and Kindred Biological Problems (1889), Vol. 1, 95-96.
In order to comprehend and fully control arithmetical concepts and methods of proof, a high degree of abstraction is necessary, and this condition has at times been charged against arithmetic as a fault. I am of the opinion that all other fields of knowledge require at least an equally high degree of abstraction as mathematics,—provided, that in these fields the foundations are also everywhere examined with the rigour and completeness which is actually necessary.
In 'Die Theorie der algebraischen Zahlkorper', Vorwort, Jahresbericht der Deutschen Mathematiker Vereinigung, Bd. 4.
In order to translate a sentence from English into French two things are necessary. First, we must understand thoroughly the English sentence. Second, we must be familiar with the forms of expression peculiar to the French language. The situation is very similar when we attempt to express in mathematical symbols a condition proposed in words. First, we must understand thoroughly the condition. Second, we must be familiar with the forms of mathematical expression.
In How to Solve It: A New Aspect of Mathematical Method (2004), 174.
In other branches of science, where quick publication seems to be so much desired, there may possibly be some excuse for giving to the world slovenly or ill-digested work, but there is no such excuse in mathematics. The form ought to be as perfect as the substance, and the demonstrations as rigorous as those of Euclid. The mathematician has to deal with the most exact facts of Nature, and he should spare no effort to render his interpretation worthy of his subject, and to give to his work its highest degree of perfection. “Pauca sed matura” was Gauss’s motto.
In Presidential Address British Association for the Advancement of Science, Section A, (1890), Nature, 42, 467. [The Latin motto translates as “Few, but ripe”. —Webmaster]
In our century the conceptions substitution and substitution group, transformation and transformation group, operation and operation group, invariant, differential invariant and differential parameter, appear more and more clearly as the most important conceptions of mathematics.
In Lapziger Berichte, No. 47 (1896), 261.
In physics, mathematics, and astronautics [elderly] means over thirty; in the other disciplines, senile decay is sometimes postponed to the forties. There are, of course, glorious exceptions; but as every researcher just out of college knows, scientists of over fifty are good for nothing but board meetings, and should at all costs be kept out of the laboratory!
Defining 'elderly scientist' as in Clarke's First Law.
Defining 'elderly scientist' as in Clarke's First Law.
'Hazards of Prophecy: The Failure of Imagination'. In the collection. Profiles of the Future: An Enquiry into the Limits of the Possible (1962, rev. 1973), 14-15.
In presenting a mathematical argument the great thing is to give the educated reader the chance to catch on at once to the momentary point and take details for granted: his successive mouthfuls should be such as can be swallowed at sight; in case of accidents, or in case he wishes for once to check in detail, he should have only a clearly circumscribed little problem to solve (e.g. to check an identity: two trivialities omitted can add up to an impasse). The unpractised writer, even after the dawn of a conscience, gives him no such chance; before he can spot the point he has to tease his way through a maze of symbols of which not the tiniest suffix can be skipped.
In A Mathematician's Miscellany (1953). Reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 49.
In pure mathematics we have a great structure of logically perfect deductions which constitutes an integral part of that great and enduring human heritage which is and should be largely independent of the perhaps temporary existence of any particular geographical location at any particular time. … The enduring value of mathematics, like that of the other sciences and arts, far transcends the daily flux of a changing world. In fact, the apparent stability of mathematics may well be one of the reasons for its attractiveness and for the respect accorded it.
In Fundamentals of Mathematics (1941), 463.
In Pure Mathematics, where all the various truths are necessarily connected with each other, (being all necessarily connected with those hypotheses which are the principles of the science), an arrangement is beautiful in proportion as the principles are few; and what we admire perhaps chiefly in the science, is the astonishing variety of consequences which may be demonstrably deduced from so small a number of premises.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 8, 186.
In scientific thought we adopt the simplest theory which will explain all the facts under consideration and enable us to predict new facts of the same kind. The catch in this criterion lies in the world “simplest.” It is really an aesthetic canon such as we find implicit in our criticisms of poetry or painting. The layman finds such a law as dx/dt = κ(d²x/dy²) much less simple than “it oozes,” of which it is the mathematical statement. The physicist reverses this judgment, and his statement is certainly the more fruitful of the two, so far as prediction is concerned. It is, however, a statement about something very unfamiliar to the plain man, namely the rate of change of a rate of change.
In 'Science and Theology as Art-Forms', Possible Worlds (1927), 227.
In the beginning (if there was such a thing), God created Newton’s laws of motion together with the necessary masses and forces. This is all; everything beyond this follows from the development of appropriate mathematical methods by means of deduction.
Autobiographical Notes (1946), 19. In Albert Einstein, Alice Calaprice, Freeman Dyson , The Ultimate Quotable Einstein (2011), 397.
In the company of friends, writers can discuss their books, economists the state of the economy, lawyers their latest cases, and businessmen their latest acquisitions, but mathematicians cannot discuss their mathematics at all. And the more profound their work, the less understandable it is.
Reflections: Mathematics and Creativity', New Yorker (1972), 47, No. 53, 39-45. In Douglas M. Campbell, John C. Higgins (eds.), Mathematics: People, Problems, Results (1984), Vol. 2, 7.
In the last two months I have been very busy with my own mathematical speculations, which have cost me much time, without my having reached my original goal. Again and again I was enticed by the frequently interesting prospects from one direction to the other, sometimes even by will-o'-the-wisps, as is not rare in mathematic speculations.
Letter to Ernst Weber (21 May 1843). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 416.
In the mathematical investigations I have usually employed such methods as present themselves naturally to a physicist. The pure mathematician will complain, and (it must be confessed) sometimes with justice, of deficient rigour. But to this question there are two sides. For, however important it may be to maintain a uniformly high standard in pure mathematics, the physicist may occasionally do well to rest content with arguments which are fairly satisfactory and conclusive from his point of view. To his mind, exercised in a different order of ideas, the more severe procedure of the pure mathematician may appear not more but less demonstrative. And further, in many cases of difficulty to insist upon the highest standard would mean the exclusion of the subject altogether in view of the space that would be required.
In Preface to second edition, The Theory of Sound (1894), Vol. 1, vii.
In the mathematics I can report no deficience, except that it be that men do not sufficiently understand the excellent use of the pure mathematics, in that they do remedy and cure many defects in the wit and faculties intellectual. For if the wit be too dull, they sharpen it; if too wandering, they fix it; if too inherent in the sense, they abstract it. So that as tennis is a game of no use in itself, but of great use in respect it maketh a quick eye and a body ready to put itself into all postures; so in the mathematics, that use which is collateral and intervenient is no less worthy than that which is principal and intended.
As translated in John Fauvel and Jeremy Gray (eds.) A History of Mathematics: A Reader (1987), 290-291. From De Augmentis, Book 3, The Advancement of Learning (1605), Book 2. Reprinted in The Two Books of Francis Bacon: Of the Proficience and Advancement of Learning, Divine and Human (2009), 97.
In the pure mathematics we contemplate absolute truths which existed in the divine mind before the morning stars sang together, and which will continue to exist there when the last of their radiant host shall have fallen from heaven.
From Address (22 Apr 1857) for Inauguration of Washington University, St. Louis, Missouri, collected in 'Academical Education', Orations and Speeches on Various Occasions (1870), Vol. 3, 514. This is seen misattributed to Eric Temple Bell, who only quoted it, attributing it to Everett, in for example, Mathematics: Queen and Servant of Sciences (1938), 20.
In the secondary schools mathematics should be a part of general culture and not contributory to technical training of any kind; it should cultivate space intuition, logical thinking, the power to rephrase in clear language thoughts recognized as correct, and ethical and esthetic effects; so treated, mathematics is a quite indispensable factor of general education in so far as the latter shows its traces in the comprehension of the development of civilization and the ability to participate in the further tasks of civilization.
The purposes of instruction in mathematics in secondary schools formulated by the German Society for the Advancement of Instruction. From Unterrichtsblätter fur Mathematik und Naturwissenschaft (1904), 128. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 72-73.
In the social equation, the value of a single life is nil; in the cosmic equation, it is infinite… Not only communism, but any political movement which implicitly relies on purely utilitarian ethics, must become a victim to the same fatal error. It is a fallacy as naïve as a mathematical teaser, and yet its consequences lead straight to Goya’s Disasters, to the reign of the guillotine, the torture chambers of the Inquisition, or the cellars of the Lubianka.
In 'The Invisible Writing', Arrow in the Blue: An Autobiography (1952), Vol. 2, 357.
In the spring of 1760, [I] went to William and Mary college, where I continued two years. It was my great good fortune, and what probably fixed the destinies of my life, that Dr. William Small of Scotland, was then Professor of Mathematics, a man profound in most of the useful branches of science, with a happy talent of communication, correct and gentlemanly manners, and an enlarged and liberal mind. He, most happily for me, became soon attached to me, and made me his daily companion when not engaged in the school; and from his conversation I got my first views of the expansion of science, and of the system of things in which we are placed.
In Thomas Jefferson and Andrew Adgate Lipscomb (ed.), The Writings of Thomas Jefferson (1904), Vol. 1, 3.
In this respect mathematics fails to reproduce with complete fidelity the obvious fact that experience is not composed of static bits, but is a string of activity, or the fact that the use of language is an activity, and the total meanings of terms are determined by the matrix in which they are embedded.
In The Nature of Physical Theory (1936), 58.
In using the present in order to reveal the past, we assume that the forces in the world are essentially the same through all time; for these forces are based on the very nature of matter, and could not have changed. The ocean has always had its waves, and those waves have always acted in the same manner. Running water on the land has ever had the same power of wear and transportation and mathematical value to its force. The laws of chemistry, heat, electricity, and mechanics have been the same through time. The plan of living structures has been fundamentally one, for the whole series belongs to one system, as much almost as the parts of an animal to the one body; and the relations of life to light and heat, and to the atmosphere, have ever been the same as now.
In 'Introduction', Manual of Geology: Treating of the Principles of the Science (1863), 7.
In working out physical problems there should be, in the first place, no pretence of rigorous formalism. The physics will guide the physicist along somehow to useful and important results, by the constant union of physical and geometrical or analytical ideas. The practice of eliminating the physics by reducing a problem to a purely mathematical exercise should be avoided as much as possible. The physics should be carried on right through, to give life and reality to the problem, and to obtain the great assistance which the physics gives to the mathematics.
In Electromagnetic Theory (1892), Vol. 2, 5.
Indeed the modern developments of mathematics constitute not only one of the most impressive, but one of the most characteristic, phenomena of our age. It is a phenomenon, however, of which the boasted intelligence of a “universalized” daily press seems strangely unaware; and there is no other great human interest, whether of science or of art, regarding which the mind of the educated public is permitted to hold so many fallacious opinions and inferior estimates.
In Lectures on Science, Philosophy and Arts (1908), 8.
Indeed, the aim of teaching [mathematics] should be rather to strengthen his [the pupil’s] faculties, and to supply a method of reasoning applicable to other subjects, than to furnish him with an instrument for solving practical problems.
In John Perry (ed.), Discussion on the Teaching of Mathematics (1901), 84. The discussion took place on 14 Sep 1901 at the British Association at Glasgow, during a joint meeting of the mathematics and physics sections with the education section. The proceedings began with an address by John Perry. Magnus spoke in the Discussion that followed.
Induction and analogy are the special characteristics of modern mathematics, in which theorems have given place to theories and no truth is regarded otherwise than as a link in an infinite chain. “Omne exit in infinitum” is their favorite motto and accepted axiom.
In 'A Plea for the Mathematician', Nature, Vol. 1, 861. [The Latin phrase “Omne exit in infinitum” means “Everything goes to infinity”.
Infinity…belonged in former days to philosophy, but belongs now to mathematics.
In 'Preface', Introduction to Mathematical Philosophy (1920), v.
Insofar as mathematics is about reality, it is not certain, and insofar as it is certain, it is not about reality.
…...
Inspiration plays no less a role in science than it does in the realm of art. It is a childish notion to think that a mathematician attains any scientifically valuable results by sitting at his desk with a ruler, calculating machines or other mechanical means. The mathematical imagination of a Weierstrass is naturally quite differently oriented in meaning and result than is the imagination of an artist, and differs basically in quality. But the psychological processes do not differ. Both are frenzy (in the sense of Plato’s “mania”) and “inspiration.”
From a Speech (1918) presented at Munich University, published in 1919, and collected in 'Wissenschaft als Beruf', Gessammelte Aufsätze zur Wissenschaftslehre (1922), 524-525. As given in H.H. Gerth and C. Wright-Mills (translators and eds.), 'Science as a Vocation', Max Weber: Essays in Sociology (1946), 136.
Integers are the fountainhead of all mathematics.
In Diophantische Approximationen, Preface. As cited in Claudi Alsina and Roger B. Nelsen, Charming Proofs: A Journey into Elegant Mathematics (2011), 1.
Is there perhaps some magical power in the subject [mathematics] that, although it had fought under the invincible banner of truth, has actually achieved its victories through some inner mysterious strength?
…...
It [mathematics] is in the inner world of pure thought, where all entia dwell, where is every type of order and manner of correlation and variety of relationship, it is in this infinite ensemble of eternal verities whence, if there be one cosmos or many of them, each derives its character and mode of being,—it is there that the spirit of mathesis has its home and its life.
Is it a restricted home, a narrow life, static and cold and grey with logic, without artistic interest, devoid of emotion and mood and sentiment? That world, it is true, is not a world of solar light, not clad in the colours that liven and glorify the things of sense, but it is an illuminated world, and over it all and everywhere throughout are hues and tints transcending sense, painted there by radiant pencils of psychic light, the light in which it lies. It is a silent world, and, nevertheless, in respect to the highest principle of art—the interpenetration of content and form, the perfect fusion of mode and meaning—it even surpasses music. In a sense, it is a static world, but so, too, are the worlds of the sculptor and the architect. The figures, however, which reason constructs and the mathematic vision beholds, transcend the temple and the statue, alike in simplicity and in intricacy, in delicacy and in grace, in symmetry and in poise. Not only are this home and this life thus rich in aesthetic interests, really controlled and sustained by motives of a sublimed and supersensuous art, but the religious aspiration, too, finds there, especially in the beautiful doctrine of invariants, the most perfect symbols of what it seeks—the changeless in the midst of change, abiding things hi a world of flux, configurations that remain the same despite the swirl and stress of countless hosts of curious transformations.
Is it a restricted home, a narrow life, static and cold and grey with logic, without artistic interest, devoid of emotion and mood and sentiment? That world, it is true, is not a world of solar light, not clad in the colours that liven and glorify the things of sense, but it is an illuminated world, and over it all and everywhere throughout are hues and tints transcending sense, painted there by radiant pencils of psychic light, the light in which it lies. It is a silent world, and, nevertheless, in respect to the highest principle of art—the interpenetration of content and form, the perfect fusion of mode and meaning—it even surpasses music. In a sense, it is a static world, but so, too, are the worlds of the sculptor and the architect. The figures, however, which reason constructs and the mathematic vision beholds, transcend the temple and the statue, alike in simplicity and in intricacy, in delicacy and in grace, in symmetry and in poise. Not only are this home and this life thus rich in aesthetic interests, really controlled and sustained by motives of a sublimed and supersensuous art, but the religious aspiration, too, finds there, especially in the beautiful doctrine of invariants, the most perfect symbols of what it seeks—the changeless in the midst of change, abiding things hi a world of flux, configurations that remain the same despite the swirl and stress of countless hosts of curious transformations.
In 'The Universe and Beyond', Hibbert Journal (1904-1906), 3, 314.
It always bothers me that according to the laws as we understand them today, it takes a computing machine an infinite number of logical operations to figure out what goes on in no matter how tiny a region of space and no matter how tiny a region of time … I have often made the hypothesis that ultimately physics will not require a mathematical statement, that in the end the machinery will be revealed and the laws will turn out to be simple, like the chequer board with all its apparent complexities. But this speculation is of the same nature as those other people make—“I like it”,“I don't like it”—and it is not good to be too prejudiced about these things.
In The Character of Physical Law (1965, 2001), 57.
It becomes the urgent duty of mathematicians, therefore, to meditate about the essence of mathematics, its motivations and goals and the ideas that must bind divergent interests together.
In 'Mathematics in the Modern World', Scientific American (Sep 1964) 211, No. 3, 42. Collected in Ronald J. Comer and Morris Kline, Mathematics in the Modern World: Readings from Scientific American (1988), 20.
It has been a fortunate fact in the modern history of physical science that the scientist constructing a new theoretical system has nearly always found that the mathematics he required for his system had already been worked out by pure mathematicians for their own amusement. … The moral for statesmen would seem to be that, for proper scientific “planning”, pure mathematics should be endowed fifty years ahead of scientists.
In 'Scientific Deductive Systems and Their Representations', Scientific Explanation: A Study of the Function of Theory, Probability and Law in Science (1968), 48-49. This book is elaborated and developed from his series of Tarner lectures (Lent 1946).
It has been asserted … that the power of observation is not developed by mathematical studies; while the truth is, that; from the most elementary mathematical notion that arises in the mind of a child to the farthest verge to which mathematical investigation has been pushed and applied, this power is in constant exercise. By observation, as here used, can only be meant the fixing of the attention upon objects (physical or mental) so as to note distinctive peculiarities—to recognize resemblances, differences, and other relations. Now the first mental act of the child recognizing the distinction between one and more than one, between one and two, two and three, etc., is exactly this. So, again, the first geometrical notions are as pure an exercise of this power as can be given. To know a straight line, to distinguish it from a curve; to recognize a triangle and distinguish the several forms—what are these, and all perception of form, but a series of observations? Nor is it alone in securing these fundamental conceptions of number and form that observation plays so important a part. The very genius of the common geometry as a method of reasoning—a system of investigation—is, that it is but a series of observations. The figure being before the eye in actual representation, or before the mind in conception, is so closely scrutinized, that all its distinctive features are perceived; auxiliary lines are drawn (the imagination leading in this), and a new series of inspections is made; and thus, by means of direct, simple observations, the investigation proceeds. So characteristic of common geometry is this method of investigation, that Comte, perhaps the ablest of all writers upon the philosophy of mathematics, is disposed to class geometry, as to its method, with the natural sciences, being based upon observation. Moreover, when we consider applied mathematics, we need only to notice that the exercise of this faculty is so essential, that the basis of all such reasoning, the very material with which we build, have received the name observations. Thus we might proceed to consider the whole range of the human faculties, and find for the most of them ample scope for exercise in mathematical studies. Certainly, the memory will not be found to be neglected. The very first steps in number—counting, the multiplication table, etc., make heavy demands on this power; while the higher branches require the memorizing of formulas which are simply appalling to the uninitiated. So the imagination, the creative faculty of the mind, has constant exercise in all original mathematical investigations, from the solution of the simplest problems to the discovery of the most recondite principle; for it is not by sure, consecutive steps, as many suppose, that we advance from the known to the unknown. The imagination, not the logical faculty, leads in this advance. In fact, practical observation is often in advance of logical exposition. Thus, in the discovery of truth, the imagination habitually presents hypotheses, and observation supplies facts, which it may require ages for the tardy reason to connect logically with the known. Of this truth, mathematics, as well as all other sciences, affords abundant illustrations. So remarkably true is this, that today it is seriously questioned by the majority of thinkers, whether the sublimest branch of mathematics,—the infinitesimal calculus—has anything more than an empirical foundation, mathematicians themselves not being agreed as to its logical basis. That the imagination, and not the logical faculty, leads in all original investigation, no one who has ever succeeded in producing an original demonstration of one of the simpler propositions of geometry, can have any doubt. Nor are induction, analogy, the scrutinization of premises or the search for them, or the balancing of probabilities, spheres of mental operations foreign to mathematics. No one, indeed, can claim preeminence for mathematical studies in all these departments of intellectual culture, but it may, perhaps, be claimed that scarcely any department of science affords discipline to so great a number of faculties, and that none presents so complete a gradation in the exercise of these faculties, from the first principles of the science to the farthest extent of its applications, as mathematics.
In 'Mathematics', in Henry Kiddle and Alexander J. Schem, The Cyclopedia of Education, (1877.) As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 27-29.
It has been proposed (in despair) to define mathematics as “what mathematicians do.” Only such a broad definition, it was felt, would cover all the things that might become embodied in mathematics; for mathematicians today attack many problems not regarded as mathematics in the past, and what they will do in the future there is no saying.
In 'The Extent of Mathematics', Prelude to Mathematics (1955), 11.
It has been said that no science is established on a firm basis unless its generalisations can be expressed in terms of number, and it is the special province of mathematics to assist the investigator in finding numerical relations between phenomena. After experiment, then mathematics. While a science is in the experimental or observational stage, there is little scope for discerning numerical relations. It is only after the different workers have “collected data” that the mathematician is able to deduce the required generalisation. Thus a Maxwell followed Faraday and a Newton completed Kepler.
In Higher Mathematics for Students of Chemistry and Physics (1902), 3.
It has come to pass, I know not how, that Mathematics and Logic, which ought to be but the handmaids of Physic, nevertheless presume on the strength of the certainty which they possess to exercise dominion over it.
From De Augmentis Scientiaurum as translated in Francis Guy Selby, The Advancement of Learning (1893), Vol. 2, 73.
It is a common observation that a science first begins to be exact when it is quantitatively treated. What are called the exact sciences are no others than the mathematical ones.
On The Doctrine of Chances, with Later Reflections (1878), 61.
It is a great pity Aristotle had not understood mathematics as well as Mr. Newton, and made use of it in his natural philosophy with good success: his example had then authorized the accommodating of it to material things.
In Second Reply to the Bishop of Worcester.
It is a mathematical fact that the casting of a pebble from my hand alters the centre of gravity of the universe.
In James Wood, Dictionary of Quotations from Ancient and Modern, English and Foreign Sources (1893), 190:1.
It is a melancholy experience for a professional mathematician to find him writing about mathematics. The function of a mathematician is to do something, to prove new theorems, to add to mathematics, and not to talk about what he or other mathematicians have done. Statesmen despise publicists, painters despise art-critics, and physiologists, physicists, or mathematicians have usually similar feelings; there is no scorn more profound, or on the whole more justifiable, than that of men who make for the men who explain. Exposition, criticism, appreciation, is work for second-rate minds.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 61 (Hardy's opening lines after Snow's foreward).
It is a safe rule to apply that, when a mathematical or philosophical author writes with a misty profoundity, he is talking nonsense.
In An Introduction to Mathematics (1911), 227.
It is above all the duty of the methodical text-book to adapt itself to the pupil’s power of comprehension, only challenging his higher efforts with the increasing development of his imagination, his logical power and the ability of abstraction. This indeed constitutes a test of the art of teaching, it is here where pedagogic tact becomes manifest. In reference to the axioms, caution is necessary. It should be pointed out comparatively early, in how far the mathematical body differs from the material body. Furthermore, since mathematical bodies are really portions of space, this space is to be conceived as mathematical space and to be clearly distinguished from real or physical space. Gradually the student will become conscious that the portion of the real space which lies beyond the visible stellar universe is not cognizable through the senses, that we know nothing of its properties and consequently have no basis for judgments concerning it. Mathematical space, on the other hand, may be subjected to conditions, for instance, we may condition its properties at infinity, and these conditions constitute the axioms, say the Euclidean axioms. But every student will require years before the conviction of the truth of this last statement will force itself upon him.
In Methodisches Lehrbuch der Elementar-Mathemalik (1904), Teil I, Vorwort, 4-5.
It is admitted by all that a finished or even a competent reasoner is not the work of nature alone; the experience of every day makes it evident that education develops faculties which would otherwise never have manifested their existence. It is, therefore, as necessary to learn to reason before we can expect to be able to reason, as it is to learn to swim or fence, in order to attain either of those arts. Now, something must be reasoned upon, it matters not much what it is, provided it can be reasoned upon with certainty. The properties of mind or matter, or the study of languages, mathematics, or natural history, may be chosen for this purpose. Now of all these, it is desirable to choose the one which admits of the reasoning being verified, that is, in which we can find out by other means, such as measurement and ocular demonstration of all sorts, whether the results are true or not. When the guiding property of the loadstone was first ascertained, and it was necessary to learn how to use this new discovery, and to find out how far it might be relied on, it would have been thought advisable to make many passages between ports that were well known before attempting a voyage of discovery. So it is with our reasoning faculties: it is desirable that their powers should be exerted upon objects of such a nature, that we can tell by other means whether the results which we obtain are true or false, and this before it is safe to trust entirely to reason. Now the mathematics are peculiarly well adapted for this purpose, on the following grounds:
1. Every term is distinctly explained, and has but one meaning, and it is rarely that two words are employed to mean the same thing.
2. The first principles are self-evident, and, though derived from observation, do not require more of it than has been made by children in general.
3. The demonstration is strictly logical, taking nothing for granted except self-evident first principles, resting nothing upon probability, and entirely independent of authority and opinion.
4. When the conclusion is obtained by reasoning, its truth or falsehood can be ascertained, in geometry by actual measurement, in algebra by common arithmetical calculation. This gives confidence, and is absolutely necessary, if, as was said before, reason is not to be the instructor, but the pupil.
5. There are no words whose meanings are so much alike that the ideas which they stand for may be confounded. Between the meaning of terms there is no distinction, except a total distinction, and all adjectives and adverbs expressing difference of degrees are avoided.
1. Every term is distinctly explained, and has but one meaning, and it is rarely that two words are employed to mean the same thing.
2. The first principles are self-evident, and, though derived from observation, do not require more of it than has been made by children in general.
3. The demonstration is strictly logical, taking nothing for granted except self-evident first principles, resting nothing upon probability, and entirely independent of authority and opinion.
4. When the conclusion is obtained by reasoning, its truth or falsehood can be ascertained, in geometry by actual measurement, in algebra by common arithmetical calculation. This gives confidence, and is absolutely necessary, if, as was said before, reason is not to be the instructor, but the pupil.
5. There are no words whose meanings are so much alike that the ideas which they stand for may be confounded. Between the meaning of terms there is no distinction, except a total distinction, and all adjectives and adverbs expressing difference of degrees are avoided.
In On the Study and Difficulties of Mathematics (1898), chap. 1.
It is always noteworthy that all those who seriously study this science [the theory of numbers] conceive a sort of passion for it.
Letter to Jonos Boyai (2 Sep 1808). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 413.
It is an obvious and imperative duty of every teacher of mathematics to study the masterpieces of mathematical literature.
In A Preface to Mathematics (1938), 76.
It is as great a mistake to maintain that a high development of the imagination is not essential to progress in mathematical studies as to hold with Ruskin and others that science and poetry are antagonistic pursuits.
In Sphere of Science (1898), 107.
It is by mathematical formulation of its observations and measurements that a science is able to form mathematically expressed hypotheses, and it is through its hypotheses that a natural science is able to make predictions.
The Nature of Science, and Other Essays (1971), 14.
It is by no means hopeless to expect to make a machine for really very difficult mathematical problems. But you would have to proceed step-by-step. I think electricity would be the best thing to rely on.
In Charles Sanders Peirce, Max Harold Fisch, Christian J. W. Kloesel Writings of Charles S. Peirce: 1884-1886 (1993), 422.
It is certainly true that all physical phenomena are subject to strictly mathematical conditions, and mathematical processes are unassailable in themselves. The trouble arises from the data employed. Most phenomena are so highly complex that one can never be quite sure that he is dealing with all the factors until the experiment proves it. So that experiment is rather the criterion of mathematical conclusions and must lead the way.
In Matter, Ether, Motion (1894), 89.
It is clear that the chief end of mathematical study must be to make the pupil think.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 4.
It is commonly considered that mathematics owes its certainty to its reliance on the immutable principles of formal logic. This … is only half the truth imperfectly expressed. The other half would be that the principles of formal logic owe such a degree of permanence as they have largely to the fact that they have been tempered by long and varied use by mathematicians. “A vicious circle!” you will perhaps say. I should rather describe it as an example of the process known by mathematicians as the method of successive approximation.
In 'The Fundamental Conceptions And Methods Of Mathematics', Bulletin of the American Mathematical Society (3 Nov 1904), 11, No. 3, 120.
It is customary in physics to take geometry for granted, as if it were a branch of mathematics. But in substance geometry is the noblest branch of physics.
In Introduction to the Calculus (1922), 348, footnote.
It is difficult even to attach a precise meaning to the term “scientific truth.” So different is the meaning of the word “truth” according to whether we are dealing with a fact of experience, a mathematical proposition or a scientific theory. “Religious truth” conveys nothing clear to me at all.
From 'Scientific Truth' in Essays in Science (1934, 2004), 11.
It is difficult to give an idea of the vast extent of modern mathematics. The word “extent” is not the right one: I mean extent crowded with beautiful detail—not an extent of mere uniformity such as an objectless plain, but of a tract of beautiful country seen at first in the distance, but which will bear to be rambled through and studied in every detail of hillside and valley, stream, rock, wood, and flower.
President’s address (1883) to the British Association for the Advancement of Science, in The Collected Mathematical Papers (1895), Vol. 8, xxii.
It is easy to make out three areas where scientists will be concentrating their efforts in the coming decades. One is in physics, where leading theorists are striving, with the help of experimentalists, to devise a single mathematical theory that embraces all the basic phenomena of matter and energy. The other two are in biology. Biologists—and the rest of us too—would like to know how the brain works and how a single cell, the fertilized egg cell, develops into an entire organism
Article 'The View From Mars', in Annals of the New York Academy of Sciences: Research Facilities of the Future (1994), 735, 37.
It is exceptional that one should be able to acquire the understanding of a process without having previously acquired a deep familiarity with running it, with using it, before one has assimilated it in an instinctive and empirical way. Thus any discussion of the nature of intellectual effort in any field is difficult, unless it presupposes an easy, routine familiarity with that field. In mathematics this limitation becomes very severe.
In 'The Mathematician', Works of the Mind (1947), 1, No. 1. Collected in James Roy Newman (ed.), The World of Mathematics (1956), Vol. 4, 2053.
It is from this absolute indifference and tranquility of the mind, that mathematical speculations derive some of their most considerable advantages; because there is nothing to interest the imagination; because the judgment sits free and unbiased to examine the point. All proportions, every arrangement of quantity, is alike to the understanding, because the same truths result to it from all; from greater from lesser, from equality and inequality.
In On the Sublime and Beautiful, Part 3, sect. 2.
It is hard to exaggerate Mr Erdos’s passion. For 19 hours a day, seven days a week, stimulated by coffee, and later by amphetamines, he worked on mathematics. He might start a game of chess, but would probably doze off until the conversation returned to maths. To find another life this century as intensely devoted to abstraction, one must reach back to Ludwig Wittgenstein (1889-1951), who stripped his life bare for philosophy. But whereas Wittgenstein discarded his family fortune as a form of self-torture, Mr Erdos gave away most of the money he earned because he simply did not need it. “Private property is a nuisance,” he would say. And where Wittgenstein was driven by near suicidal compulsions, Mr Erdos simply constructed his life to extract from his magnificent obsession the maximum amount of happiness.
— Magazine
In obituary 'Paul Erdos', The Economist (5 Oct 1996), 83.
It is hard to know what you are talking about in mathematics, yet no one questions the validity of what you say. There is no other realm of discourse half so queer.
In J.R. Newman (ed.), 'Commentary on The Foundations of Mathematics', The World of Mathematics (1956), Vol. 3, 1614.
It is here [in mathematics] that the artist has the fullest scope of his imagination.
In The Dance of Life (1923), 138-139.
It is interesting thus to follow the intellectual truths of analysis in the phenomena of nature. This correspondence, of which the system of the world will offer us numerous examples, makes one of the greatest charms attached to mathematical speculations.
Exposition du système du monde (1799)
It is known that the mathematics prescribed for the high school [Gymnasien] is essentially Euclidean, while it is modern mathematics, the theory of functions and the infinitesimal calculus, which has secured for us an insight into the mechanism and laws of nature. Euclidean mathematics is indeed, a prerequisite for the theory of functions, but just as one, though he has learned the inflections of Latin nouns and verbs, will not thereby be enabled to read a Latin author much less to appreciate the beauties of a Horace, so Euclidean mathematics, that is the mathematics of the high school, is unable to unlock nature and her laws.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 37-38. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 112.
It is mathematics that offers the exact natural sciences a certain measure of security which, without mathematics, they could not attain.
…...
It is not of the essence of mathematics to be conversant with the ideas of number and quantity. Whether as a general habit of mind it would be desirable to apply symbolic processes to moral argument, is another question.
An Investigation of the Laws of Thought (1854), 12.
It is not only a decided preference for synthesis and a complete denial of general methods which characterizes the ancient mathematics as against our newer Science [modern mathematics]: besides this extemal formal difference there is another real, more deeply seated, contrast, which arises from the different attitudes which the two assumed relative to the use of the concept of variability. For while the ancients, on account of considerations which had been transmitted to them from the Philosophie school of the Eleatics, never employed the concept of motion, the spatial expression for variability, in their rigorous system, and made incidental use of it only in the treatment of phonoromically generated curves, modern geometry dates from the instant that Descartes left the purely algebraic treatment of equations and proceeded to investigate the variations which an algebraic expression undergoes when one of its variables assumes a continuous succession of values.
In 'Untersuchungen über die unendlich oft oszillierenden und unstetigen Functionen', Ostwald’s Klassiker der exacten Wissenschaften (1905), No. 153, 44-45. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 115. From the original German, “Nicht allein entschiedene Vorliebe für die Synthese und gänzliche Verleugnung allgemeiner Methoden charakterisiert die antike Mathematik gegenüber unserer neueren Wissenschaft; es gibt neben diesem mehr äußeren, formalen, noch einen tiefliegenden realen Gegensatz, welcher aus der verschiedenen Stellung entspringt, in welche sich beide zu der wissenschaftlichen Verwendung des Begriffes der Veränderlichkeit gesetzt haben. Denn während die Alten den Begriff der Bewegung, des räumlichen Ausdruckes der Veränderlichkeit, aus Bedenken, die aus der philosophischen Schule der Eleaten auf sie übergegangen waren, in ihrem strengen Systeme niemals und auch in der Behandlung phoronomisch erzeugter Kurven nur vorübergehend verwenden, so datiert die neuere Mathematik von dem Augenblicke, als Descartes von der rein algebraischen Behandlung der Gleichungen dazu fortschritt, die Größenveränderungen zu untersuchen, welche ein algebraischer Ausdruck erleidet, indem eine in ihm allgemein bezeichnete Größe eine stetige Folge von Werten durchläuft.”
It is not so long since, during one of the meetings of the Association, one of the leading English newspapers briefly described a sitting of this Section in the words, “Saturday morning was devoted to pure mathematics, and so there was nothing of any general interest:” still, such toleration is better than undisguised and ill-informed hostility.
In Report of the 67th meeting of the British Association for the Advancement of Science.
It is not surprising that our language should be incapable of describing the processes occurring within the atoms, for, as has been remarked, it was invented to describe the experiences of daily life, and these consists only of processes involving exceedingly large numbers of atoms. Furthermore, it is very difficult to modify our language so that it will be able to describe these atomic processes, for words can only describe things of which we can form mental pictures, and this ability, too, is a result of daily experience. Fortunately, mathematics is not subject to this limitation, and it has been possible to invent a mathematical scheme—the quantum theory—which seems entirely adequate for the treatment of atomic processes; for visualization, however, we must content ourselves with two incomplete analogies—the wave picture and the corpuscular picture.
The Physical Principles of the Quantum Theory, trans. Carl Eckart and Frank C. Hoyt (1949), 11.
It is not surprising, in view of the polydynamic constitution of the genuinely mathematical mind, that many of the major heros of the science, men like Desargues and Pascal, Descartes and Leibnitz, Newton, Gauss and Bolzano, Helmholtz and Clifford, Riemann and Salmon and Plücker and Poincaré, have attained to high distinction in other fields not only of science but of philosophy and letters too. And when we reflect that the very greatest mathematical achievements have been due, not alone to the peering, microscopic, histologic vision of men like Weierstrass, illuminating the hidden recesses, the minute and intimate structure of logical reality, but to the larger vision also of men like Klein who survey the kingdoms of geometry and analysis for the endless variety of things that flourish there, as the eye of Darwin ranged over the flora and fauna of the world, or as a commercial monarch contemplates its industry, or as a statesman beholds an empire; when we reflect not only that the Calculus of Probability is a creation of mathematics but that the master mathematician is constantly required to exercise judgment—judgment, that is, in matters not admitting of certainty—balancing probabilities not yet reduced nor even reducible perhaps to calculation; when we reflect that he is called upon to exercise a function analogous to that of the comparative anatomist like Cuvier, comparing theories and doctrines of every degree of similarity and dissimilarity of structure; when, finally, we reflect that he seldom deals with a single idea at a tune, but is for the most part engaged in wielding organized hosts of them, as a general wields at once the division of an army or as a great civil administrator directs from his central office diverse and scattered but related groups of interests and operations; then, I say, the current opinion that devotion to mathematics unfits the devotee for practical affairs should be known for false on a priori grounds. And one should be thus prepared to find that as a fact Gaspard Monge, creator of descriptive geometry, author of the classic Applications de l’analyse à la géométrie; Lazare Carnot, author of the celebrated works, Géométrie de position, and Réflections sur la Métaphysique du Calcul infinitesimal; Fourier, immortal creator of the Théorie analytique de la chaleur; Arago, rightful inheritor of Monge’s chair of geometry; Poncelet, creator of pure projective geometry; one should not be surprised, I say, to find that these and other mathematicians in a land sagacious enough to invoke their aid, rendered, alike in peace and in war, eminent public service.
In Lectures on Science, Philosophy and Art (1908), 32-33.
It is now necessary to indicate more definitely the reason why mathematics not only carries conviction in itself, but also transmits conviction to the objects to which it is applied. The reason is found, first of all, in the perfect precision with which the elementary mathematical concepts are determined; in this respect each science must look to its own salvation .... But this is not all. As soon as human thought attempts long chains of conclusions, or difficult matters generally, there arises not only the danger of error but also the suspicion of error, because since all details cannot be surveyed with clearness at the same instant one must in the end be satisfied with a belief that nothing has been overlooked from the beginning. Every one knows how much this is the case even in arithmetic, the most elementary use of mathematics. No one would imagine that the higher parts of mathematics fare better in this respect; on the contrary, in more complicated conclusions the uncertainty and suspicion of hidden errors increases in rapid progression. How does mathematics manage to rid itself of this inconvenience which attaches to it in the highest degree? By making proofs more rigorous? By giving new rules according to which the old rules shall be applied? Not in the least. A very great uncertainty continues to attach to the result of each single computation. But there are checks. In the realm of mathematics each point may be reached by a hundred different ways; and if each of a hundred ways leads to the same point, one may be sure that the right point has been reached. A calculation without a check is as good as none. Just so it is with every isolated proof in any speculative science whatever; the proof may be ever so ingenious, and ever so perfectly true and correct, it will still fail to convince permanently. He will therefore be much deceived, who, in metaphysics, or in psychology which depends on metaphysics, hopes to see his greatest care in the precise determination of the concepts and in the logical conclusions rewarded by conviction, much less by success in transmitting conviction to others. Not only must the conclusions support each other, without coercion or suspicion of subreption, but in all matters originating in experience, or judging concerning experience, the results of speculation must be verified by experience, not only superficially, but in countless special cases.
In Werke [Kehrbach] (1890), Bd. 5, 105. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 19.
It is now quite lawful for a Catholic woman to avoid pregnancy by a resort to mathematics, though she is still forbidden to resort to physics and chemistry.
…...
It is only in mathematics, and to some extent in poetry, that originality may be attained at an early age, but even then it is very rare (Newton and Keats are examples), and it is not notable until adolescence is completed.
In A Study of British Genius (1904), 142
It is only necessary to check the comic books and Reader’s Digest to see the extent of the influence of applied science on the popular imagination. How much it is used to provide an atmosphere of endless thrill and excitement, quite apart from its accidental menace or utility, one can decide from such typical daily headlines as these:
London, March 10, 1947, Reuters: ROCKET TO MOON SEEN POSSIBLE BUT THOUSANDS TO DIE IN ATTEMPT
Cleveland, January 5, 1948.: LIFE SPAN OF 100, BE YOUNG AT 80, ATOM PREDICTION
Washington, June 11, 1947: SCIENTISTS AWAIT COW’S DEATH TO SOLVE MATHEMATICS PROBLEM
Needham Market, Suffolk, England. (U.P.): VICAR PROPOSES BABIES FOR YEARNING SPINSTERS, TEST-TUBE BABIES WILL PRODUCE ROBOTS
Washington, D.C., January 3, 1948. U.S. FLYER PASSING SONIC BARRIER OPENS NEW VISTAS OF DESTRUCTION ONE OF BRAVEST ACTS IN HISTORY
Those headlines represent “human interest” attempts to gear science to the human nervous system.
London, March 10, 1947, Reuters: ROCKET TO MOON SEEN POSSIBLE BUT THOUSANDS TO DIE IN ATTEMPT
Cleveland, January 5, 1948.: LIFE SPAN OF 100, BE YOUNG AT 80, ATOM PREDICTION
Washington, June 11, 1947: SCIENTISTS AWAIT COW’S DEATH TO SOLVE MATHEMATICS PROBLEM
Needham Market, Suffolk, England. (U.P.): VICAR PROPOSES BABIES FOR YEARNING SPINSTERS, TEST-TUBE BABIES WILL PRODUCE ROBOTS
Washington, D.C., January 3, 1948. U.S. FLYER PASSING SONIC BARRIER OPENS NEW VISTAS OF DESTRUCTION ONE OF BRAVEST ACTS IN HISTORY
Those headlines represent “human interest” attempts to gear science to the human nervous system.
In The Mechanical Bride: Folklore of Industrial Man (1967), 93.
It is perplexing to see the flexibility of the so-called 'exact sciences' which by cast-iron laws of logic and by the infallible help of mathematics can lead to conclusions which are diametrically opposite to one another.
In The Nature of Light: an Historical Survey (1970), 229
It is probably no exaggeration to suppose that in order to improve such an organ as the eye at all, it must be improved in ten different ways at once. And the improbability of any complex organ being produced and brought to perfection in any such way is an improbability of the same kind and degree as that of producing a poem or a mathematical demonstration by throwing letters at random on a table.
[Expressing his reservations about Darwin's proposed evolution of the eye by natural selection.]
[Expressing his reservations about Darwin's proposed evolution of the eye by natural selection.]
Opening address to the Belfast Natural History Society, as given in the 'Belfast Northern Whig,' (19 Nov 1866). As cited by Charles Darwin in The Variation of Animals & Plants Under Domestication (1868), 222.
It is quite possible that mathematics was invented in the ancient Middle East to keep track of tax receipts and grain stores. How odd that out of this should come a subtle scientific language that can effectively describe and predict the most arcane aspects of the Universe.
Epigraph in Isaac Asimov’s Book of Science and Nature Quotations (1988), 265.
It is said of Jacobi, that he attracted the particular attention and friendship of Böckh, the director of the philological seminary at Berlin, by the great talent he displayed for philology, and only at the end of two years’ study at the University, and after a severe mental struggle, was able to make his final choice in favor of mathematics.
In Collected Mathematical Papers, Vol. 2 (1908), 661.
It is said that in a certain grassy part of the world a man will walk a mile to catch a horse, whereon to ride a quarter of a mile to pay an afternoon call. Similarly, it is not quite respectable to arrive at a mathematical destination, under the gaze of a learned society, at the mere footpace of arithmetic. Even at the expense of considerable time and effort, one should be mounted on the swift steed of symbolic analysis.
Opening of 'How to Solve Differential Equations Approximately by Arithmetic', The Mathematical Gazette (Jul 1925), 12, No. 177, 415
It is said that the composing of the Lilavati was occasioned by the following circumstance. Lilavati was the name of the author’s daughter, concerning whom it appeared, from the qualities of the ascendant at her birth, that she was destined to pass her life unmarried, and to remain without children. The father ascertained a lucky hour for contracting her in marriage, that she might be firmly connected and have children. It is said that when that hour approached, he brought his daughter and his intended son near him. He left the hour cup on the vessel of water and kept in attendance a time-knowing astrologer, in order that when the cup should subside in the water, those two precious jewels should be united. But, as the intended arrangement was not according to destiny, it happened that the girl, from a curiosity natural to children, looked into the cup, to observe the water coming in at the hole, when by chance a pearl separated from her bridal dress, fell into the cup, and, rolling down to the hole, stopped the influx of water. So the astrologer waited in expectation of the promised hour. When the operation of the cup had thus been delayed beyond all moderate time, the father was in consternation, and examining, he found that a small pearl had stopped the course of the water, and that the long-expected hour was passed. In short, the father, thus disappointed, said to his unfortunate daughter, I will write a book of your name, which shall remain to the latest times—for a good name is a second life, and the ground-work of eternal existence.
In Preface to the Persian translation of the Lilavati by Faizi (1587), itself translated into English by Strachey and quoted in John Taylor (trans.) Lilawati, or, A Treatise on Arithmetic and Geometry by Bhascara Acharya (1816), Introduction, 3. [The Lilavati is the 12th century treatise on mathematics by Indian mathematician, Bhaskara Acharya, born 1114.]
It is the invaluable merit of the great Basle mathematician Leonhard Euler, to have freed the analytical calculus from all geometric bounds, and thus to have established analysis as an independent science, which from his time on has maintained an unchallenged leadership in the field of mathematics.
In Die Entwickelung der Mathematik in den letzten Jahrhunderten (1884), 12. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 153. Seen incorrectly attributed to Thomas Reid in N. Rose, Mathematical and Maxims and Minims (1988).
It is the merest truism, evident at once to unsophisticated observation, that mathematics is a human invention.
In The Logic of Modern Physics (1927, 1972), 60.
It is the perennial youthfulness of mathematics itself which marks it off with a disconcerting immortality from the other sciences.
In The Queen of the Sciences (1938), 5.
It is the structure of the universe that has taught this knowledge to man. That structure is an ever existing exhibition of every principle upon which every part of mathematical science is founded. The offspring of this science is mechanics; for mechanics are no other than the principles of science appplied practically.
In The Age of Reason: Being an Investigation of True and Fabulous Theology (27 Jan O.S. 1794), 42.
It is the symbolic language of mathematics only which has yet proved sufficiently accurate and comprehensive to demand familiarity with this conception of an inverse process.
In 'The Symbol of Division', Symbolic Logic (1894), 74, footnote.
It is therefore through the study of mathematics, and only by it, that one can form a fair and comprehensive idea of what science is. … Any scientific education which does not begin with such a study, necessarily is fundamentally flawed.
From Cours de Philosophie Positive (1830), Vol. 1, 132. Comte believed in a hierarchy of the sciences, ordered by degree of generality and simplicity of their ideas. Mathematics he placed first, followed by astronomy, physics, chemistry, biology, and sociology in the sixth and last place. This quote was included by T.H. Huxley, in 'The Scientific Aspects of Positivism', Fortnightly Review (1869), 11, 666-667, in which Huxley strongly disagreed with ranking the abstract discipline of mathematics at the top, since education should begin with the concrete based on investigation by observation, “from the easy to the difficult.” English translation by Webmaster using online resources, from the original French, “C’est donc par l’étude des mathématiques, et seulement par elle, que l’on peut se faire une idée juste et approfondie de ce que c’est qu’une science. … Toute éducation scientifique qui ne commence point par une telle étude pèche donc nécessairement par sa base.”
It is through it [intuition] that the mathematical world remains in touch with the real world, and even if pure mathematics could do without it, we should still have to have recourse to it to fill up the gulf that separates the symbol from reality.
…...
It is true that Fourier had the opinion that the principal end of mathematics was public utility and the explanation of natural phenomena; but a philosopher as he is should have known that the unique end of science is the honor of the human mind and that from this point of view a question of [the theory of] number is as important as a question of the system of the world.
From letter to Legendre, translation as given in F.R. Moulton, 'The Influence of Astronomy on Mathematics', Science (10 Mar 1911), N.S. Vol. 33, No. 845, 359. A different translation begins, “It is true that M. Fourier believed…” on the Karl Jacobi Quotes web page on this site.
It is true that M. Fourier believed that the main aim of mathematics was public utility and the explanation of natural phenomena; but a philosopher of his ability ought to have known that the sole aim of science is the honour of the human intellect, and that on this ground a problem in numbers is as important as a problem on the system of the world.
In Letter to Legendre, as quoted in an Address by Emile Picard to the Congress of Science and Art, St. Louis (22 Sep 1904), translated in 'Development of Mathematical Analysis', The Mathematical Gazette (Jul 1905), 3, No. 52, 200. A different translation begins, “It is true that Fourier had the opinion…” on the Karl Jacobi Quotes web page on this site.
It is true that mathematics, owing to the fact that its whole content is built up by means of purely logical deduction from a small number of universally comprehended principles, has not unfittingly been designated as the science of the self-evident [Selbstverständlichen]. Experience however, shows that for the majority of the cultured, even of scientists, mathematics remains the science of the incomprehensible [Unverständlichen].
In Ueber Wert und angeblichen Unwert der Mathematik, Jahresbericht der Deutschen Maihemaliker Vereinigung (1904), 357.
It is unsafe to talk mathematics. Folks don’t understand.
Martin H. Fischer, Howard Fabing (ed.) and Ray Marr (ed.), Fischerisms (1944), 3.
It is very interesting to observe how high the rank may be in the world of science, without the aid of wealth. I have before me a letter of the late Dr. Dalton, who, notwithstanding his great reputation as a chemist, until a late period of his life was a teacher of mathematics. He remarks in this note, that if the price of mercury continues so dear he shall not be able to afford the expense of filling the barometers which he requires for some experiments.
In Education and Manufactures (1863), 44-45.
It is with mathematics not otherwise than it is with music, painting or poetry. Anyone can become a lawyer, doctor or chemist, and as such may succeed well, provided he is clever and industrious, but not every one can become a painter, or a musician, or a mathematician: general cleverness and industry alone count here for nothing.
In Ueber die Anlage zur Mathematik (1900), 5. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 184.
It may be asserted without exaggeration that the domain of mathematical knowledge is the only one of which our otherwise omniscient journalism has not yet possessed itself.
In Ueber Wert und angeblichen Unwert der Mathematik'’ Jahresbericht der Deulschen Mathematiker Vereinigung (1904), 367.
It may be true that people who are merely mathematicians have certain specific shortcomings; however that is not the fault of mathematics, but is true of every exclusive occupation. Likewise a mere linguist, a mere jurist, a mere soldier, a mere merchant, and so forth. One could add such idle chatter that when a certain exclusive occupation is often connected with certain specific shortcomings, it is on the other hand always free of certain other shortcomings.
Letter to Heinrich Schumacher (1-5 Jan 1845). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 414.
It may be true, that men, who are mere mathematicians, have certain specific shortcomings, but that is not the fault of mathematics, for it is equally true of every other exclusive occupation. So there are mere philologists, mere jurists, mere soldiers, mere merchants, etc. To such idle talk it might further be added: that whenever a certain exclusive occupation is coupled with specific shortcomings, it is likewise almost certainly divorced from certain other shortcomings.
In Gauss-Schumacher Briefwechsel, Bd. 4, (1862), 387.
It may well be doubted whether, in all the range of science, there is any field so fascinating to the explorer—so rich in hidden treasures—so fruitful in delightful surprises—as that of Pure Mathematics. The charm lies chiefly, I think, in the absolute certainty of its results; for that is what, beyond all mental treasures, the human intellect craves for. Let us only be sure of something! More light, more light!
Written without pseudonym as Charles L. Dodgson. Opening remarks in Introduction to A New Theory of Parallels (1888, 1890), xv.
It may well be doubted whether, in all the range of Science, there is any field so fascinating to the explorer—so rich in hidden treasures—so fruitful in delightful surprises—as that of Pure Mathematics. The charm lies chiefly, I think, in the absolute certainty of its results: for that is what, beyond all mental treasures, the human intellect craves for. Let us only be sure of something! More light, more light … “And if our fate be death, give light and let us die” This is the cry that, through all the ages, is going up from perplexed Humanity, and Science has little else to offer, that will really meet the demands of its votaries, than the conclusions of Pure Mathematics.
Opening of 'Introduction', A New Theory of Parallels (1890), xv. As a non-fiction work, the author’s name on the title page of this book was Charles Lutwidge Dodgson. Being better known for his works of fiction as Lewis Carroll, all quotes relating to this one person, published under either name, are gathered on this single web page under his pen name.
It needs scarcely be pointed out that in placing Mathematics at the head of Positive Philosophy, we are only extending the application of the principle which has governed our whole Classification. We are simply carrying back our principle to its first manifestation. Geometrical and Mechanical phenomena are the most general, the most simple, the most abstract of all,— the most irreducible to others, the most independent of them; serving, in fact, as a basis to all others. It follows that the study of them is an indispensable preliminary to that of all others. Therefore must Mathematics hold the first place in the hierarchy of the sciences, and be the point of departure of all Education whether general or special.
In Auguste Comte and Harriet Martineau (trans.), The Positive Philosophy (1858), Introduction, Chap. 2, 50.
It often happens that men, even of the best understandings and greatest circumspection, are guilty of that fault in reasoning which the writers on logick call the insufficient, or imperfect enumeration of parts, or cases: insomuch that I will venture to assert, that this is the chief, and almost the only, source of the vast number of erroneous opinions, and those too very often in matters of great importance, which we are apt to form on all the subjects we reflect upon, whether they relate to the knowledge of nature, or the merits and motives of human actions. It must therefore be acknowledged, that the art which affords a cure to this weakness, or defect, of our understandings, and teaches us to enumerate all the possible ways in which a given number of things may be mixed and combined together, that we may be certain that we have not omitted anyone arrangement of them that can lead to the object of our inquiry, deserves to be considered as most eminently useful and worthy of our highest esteem and attention. And this is the business of the art, or doctrine of combinations ... It proceeds indeed upon mathematical principles in calculating the number of the combinations of the things proposed: but by the conclusions that are obtained by it, the sagacity of the natural philosopher, the exactness of the historian, the skill and judgement of the physician, and the prudence and foresight of the politician, may be assisted; because the business of all these important professions is but to form reasonable conjectures concerning the several objects which engage their attention, and all wise conjectures are the results of a just and careful examination of the several different effects that may possibly arise from the causes that are capable of producing them.
Ars conjectandi (1713). In F. Maseres, The Doctrine of Permutations and Combinations (1795), 36.
It seems perfectly clear that Economy, if it is to be a science at all, must be a mathematical science. There exists much prejudice against attempts to introduce the methods and language of mathematics into any branch of the moral sciences. Most persons appear to hold that the physical sciences form the proper sphere of mathematical method, and that the moral sciences demand some other method—I know not what.
The Theory of Political Economy (1871), 3.
It seems to me that the older subjects, classics and mathematics, are strongly to be recommended on the ground of the accuracy with which we can compare the relative performance of the students. In fact the definiteness of these subjects is obvious, and is commonly admitted. There is however another advantage, which I think belongs in general to these subjects, that the examinations can be brought to bear on what is really most valuable in these subjects.
In Conflict of Studies and other Essays (1873), 6-7.
It was a felicitous expression of Goethe’s to call a noble cathedral “frozen music,” but it might even better be called “petrified mathematics.”
In The Teaching of Mathematics in the Elementary and the Secondary School (1906), 44.
It was his [Leibnitz’s] love of method and order, and the conviction that such order and harmony existed in the real world, and that our success in understanding it depended upon the degree and order which we could attain in our own thoughts, that originally was probably nothing more than a habit which by degrees grew into a formal rule. This habit was acquired by early occupation with legal and mathematical questions. We have seen how the theory of combinations and arrangements of elements had a special interest for him. We also saw how mathematical calculations served him as a type and model of clear and orderly reasoning, and how he tried to introduce method and system into logical discussions, by reducing to a small number of terms the multitude of compound notions he had to deal with. This tendency increased in strength, and even in those early years he elaborated the idea of a general arithmetic, with a universal language of symbols, or a characteristic which would be applicable to all reasoning processes, and reduce philosophical investigations to that simplicity and certainty which the use of algebraic symbols had introduced into mathematics.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
In Leibnitz (1884), 44-45. [The first sentence is reworded to better introduce the quotation. —Webmaster]
It was not alone the striving for universal culture which attracted the great masters of the Renaissance, such as Brunellesco, Leonardo da Vinci, Raphael, Michelangelo and especially Albrecht Dürer, with irresistible power to the mathematical sciences. They were conscious that, with all the freedom of the individual fantasy, art is subject to necessary laws, and conversely, with all its rigor of logical structure, mathematics follows aesthetic laws.
From Lecture (5 Feb 1891) held at the Rathhaus, Zürich, printed as Ueber den Antheil der mathematischen Wissenschaft an der Kultur der Renaissance (1892), 19. (The Contribution of the Mathematical Sciences to the Culture of the Renaissance.) As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 183.
It would be difficult and perhaps foolhardy to analyze the chances of further progress in almost every part of mathematics one is stopped by unsurmountable difficulties, improvements in the details seem to be the only possibilities which are left… All these difficulties seem to announce that the power of our analysis is almost exhausted, even as the power of ordinary algebra with regard to transcendental geometry in the time of Leibniz and Newton, and that there is a need of combinations opening a new field to the calculation of transcendental quantities and to the solution of the equations including them.
From Rapport historique sur les progrès des sciences mathématiques depuis 1789, et sur leur état actuel (1810), 131. As translated in George Sarton, The Study of the History of Mathematics (1936), 13. In the original French: “Il seroit difficile et peut-être téméraire d’analyser les chances que l’avenir offre à l’avancement des mathématiques: dans presque toutes les parties, on est arrêté par des difficultés insurmontables; des perfectionnements de détail semblent la seule chose qui reste à faire… Toutes ces difficultés semblent annoncer que la puissance de notre analyse est à-peu-près épuisée, comme celle de l’algèbre ordinaire l’étoit par rapport à la géométrie transcendante au temps de Leibnitz et de Newton, et qu’il faut des combinaisons qui ouvrent un nouveau champ au calcul des transcendantes et à la résolution des équations qui les contiennent.” Sarton states this comes from “the report on mathematical progress prepared for the French Academy of Sciences at Napoleon’s request”.
It would be rash to say that nothing remains for discovery or improvement even in elementary mathematics, but it may be safely asserted that the ground has been so long and so thoroughly explored as to hold out little hope of profitable return for a casual adventurer.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 73.
It would be rash to say that nothing remains for discovery or improvement even in elementary mathematics, but it may be safely asserted that the ground has been so long and so thoroughly explored as to hold out little hope of profitable return for a casual adventurer.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 73.
It would seem at first sight as if the rapid expansion of the region of mathematics must be a source of danger to its future progress. Not only does the area widen but the subjects of study increase rapidly in number, and the work of the mathematician tends to become more and more specialized. It is, of course, merely a brilliant exaggeration to say that no mathematician is able to understand the work of any other mathematician, but it is certainly true that it is daily becoming more and more difficult for a mathematician to keep himself acquainted, even in a general way, with the progress of any of the branches of mathematics except those which form the field of his own labours. I believe, however, that the increasing extent of the territory of mathematics will always be counteracted by increased facilities in the means of communication. Additional knowledge opens to us new principles and methods which may conduct us with the greatest ease to results which previously were most difficult of access; and improvements in notation may exercise the most powerful effects both in the simplification and accessibility of a subject. It rests with the worker in mathematics not only to explore new truths, but to devise the language by which they may be discovered and expressed; and the genius of a great mathematician displays itself no less in the notation he invents for deciphering his subject than in the results attained. … I have great faith in the power of well-chosen notation to simplify complicated theories and to bring remote ones near and I think it is safe to predict that the increased knowledge of principles and the resulting improvements in the symbolic language of mathematics will always enable us to grapple satisfactorily with the difficulties arising from the mere extent of the subject.
In Presidential Address British Association for the Advancement of Science, Section A., (1890), Nature, 42, 466.
It’s important for students to be put in touch with real-world problems. The curriculum should include computer science. Mathematics should include statistics. The curriculums should really adjust.
From address at a conference on Google campus, co-hosted with Common Sense Media and the Joan Ganz Cooney Center at Sesame Workshop 'Breakthrough Learning in the Digital Age'. As quoted in Technology blog report by Dan Fost, 'Google co-founder Sergey Brin wants more computers in schools', Los Angeles Times (28 Oct 2009). On latimesblogs.latimes.com website.
As quoted, without citation, in Can Akdeniz, Fast MBA (2014), 280.
John Bahcall, an astronomer on the Institute of Advanced Study faculty since 1970 likes to tell the story of his first faculty dinner, when he found himself seated across from Kurt Gödel, … a man dedicated to logic and the clean certainties of mathematical abstraction. Bahcall introduced himself and mentioned that he was a physicist. Gödel replied, “I don’t believe in natural science.”
As stated in Adam Begley, 'The Lonely Genius Club', New York Magazine (30 Jan 1995), 63.
John Dalton was a very singular Man, a quaker by profession & practice: He has none of the manners or ways of the world. A tolerable mathematician He gained his livelihood I believe by teaching the mathematics to young people. He pursued science always with mathematical views. He seemed little attentive to the labours of men except when they countenanced or confirmed his own ideas... He was a very disinterested man, seemed to have no ambition beyond that of being thought a good Philosopher. He was a very coarse Experimenter & almost always found the results he required.—Memory & observation were subordinate qualities in his mind. He followed with ardour analogies & inductions & however his claims to originality may admit of question I have no doubt that he was one of the most original philosophers of his time & one of the most ingenious.
J. Z. Fullmer, 'Davy's Sketches of his Contemporaries', Chymia, 1967, 12, 133-134.
Just as mathematics aims to study such entities as numbers, functions, spaces, etc., the subject matter of metamathematics is mathematics itself.
In 'Mathematics: A Non-Technical Exposition', American Scientist (3 Jul 1954), 42, No. 3, 490.
Just by studying mathematics we can hope to make a guess at the kind of mathematics that will come into the physics of the future ... If someone can hit on the right lines along which to make this development, it m may lead to a future advance in which people will first discover the equations and then, after examining them, gradually learn how to apply the ... My own belief is that this is a more likely line of progress than trying to guess at physical pictures.
'The Evolution of the Physicist's Picture of Nature', Scientific American, May 1963, 208, 47. In Steve Adams, Frontiers (2000), 57.
Kant, discussing the various modes of perception by which the human mind apprehends nature, concluded that it is specially prone to see nature through mathematical spectacles. Just as a man wearing blue spectacles would see only a blue world, so Kant thought that, with our mental bias, we tend to see only a mathematical world.
In The Mysterious Universe (1930), 115.
Kirchhoff’s whole tendency, and its true counterpart, the form of his presentation, was different [from Maxwell’s “dramatic bulk”]. … He is characterized by the extreme precision of his hypotheses, minute execution, a quiet rather than epic development with utmost rigor, never concealing a difficulty, always dispelling the faintest obscurity. … he resembled Beethoven, the thinker in tones. — He who doubts that mathematical compositions can be beautiful, let him read his memoir on Absorption and Emission … or the chapter of his mechanics devoted to Hydrodynamics.
In Ceremonial Speech (15 Nov 1887) celebrating the 301st anniversary of the Karl-Franzens-University Graz. Published as Gustav Robert Kirchhoff: Festrede zur Feier des 301. Gründungstages der Karl-Franzens-Universität zu Graz (1888), 30, as translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 187. From the original German, “Kirchhoff … seine ganze Richtung war eine andere, und ebenso auch deren treues Abbild, die Form seiner Darstellung. … Ihn charakterisirt die schärfste Präcisirung der Hypothesen, feine Durchfeilung, ruhige mehr epische Fortentwicklung mit eiserner Consequenz ohne Verschweigung irgend einer Schwierigkeit, unter Aufhellung des leisesten Schattens. … er glich dem Denker in Tönen: Beethoven. – Wer in Zweifel zieht, dass mathematische Werke künstlerisch schön sein können, der lese seine Abhandlung über Absorption und Emission oder den der Hydrodynamik gewidmeten Abschnitt seiner Mechanik.” The memoir reference is Gesammelte Abhandlungen (1882), 571-598.
Lagrange, in one of the later years of his life, imagined that he had overcome the difficulty (of the parallel axiom). He went so far as to write a paper, which he took with him to the Institute, and began to read it. But in the first paragraph something struck him that he had not observed: he muttered: 'Il faut que j'y songe encore', and put the paper in his pocket.' [I must think about it again]
Budget of Paradoxes (1872), 173.
Laplace would have found it child's-play to fix a ratio of progression in mathematical science between Descartes, Leibnitz, Newton and himself
The Education of Henry Adams: An Autobiography? (1918), 491.
Laplace’s equation is the most famous and most universal of all partial differential equations. No other single equation has so many deep and diverse mathematical relationships and physical applications.
In G.F.D. Duff and D. Naylor (co-authors), Differential Equations of Applied Mathematics (1966), 133.
Large physical objects like stars consist of a rather limited array of parts, more or less haphazardly arranged. The behaviour of physical, nonbiological objects is so simple that it is feasible to use existing mathematical language to describe it, which is why physics books are full of mathematics.
In The Blind Watchmaker (1986, 1996), 2.
Leaving those [exercises] of the Body, I shall proceed to such Recreations as adorn the Mind; of which those of the Mathematicks are inferior to none.
In Pleasure with Profit: Consisting of Recreations of Divers Kinds (1694), v.
Leibnitz believed he saw the image of creation in his binary arithmetic in which he employed only two characters, unity and zero. Since God may be represented by unity, and nothing by zero, he imagined that the Supreme Being might have drawn all things from nothing, just as in the binary arithmetic all numbers are expressed by unity with zero. This idea was so pleasing to Leibnitz, that he communicated it to the Jesuit Grimaldi, President of the Mathematical Board of China, with the hope that this emblem of the creation might convert to Christianity the reigning emperor who was particularly attached to the sciences.
In 'Essai Philosophique sur les Probabiliés', Oeuvres (1896), t. 7, 119.
Let us now discuss the extent of the mathematical quality in Nature. According to the mechanistic scheme of physics or to its relativistic modification, one needs for the complete description of the universe not merely a complete system of equations of motion, but also a complete set of initial conditions, and it is only to the former of these that mathematical theories apply. The latter are considered to be not amenable to theoretical treatment and to be determinable only from observation.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 125.
Life is good for only two things: to do mathematics and to teach it.
From François Arago, 'Mort de Poisson', Œvres Complètes de François Arago (1854), Vol.2, 662, translated by Webmaster from the original French, “La vie n’est bonne qu’à deux choses: à faire des mathématiques et à les professer.” Quote introduced by Arago as “Poisson … avait l’habitude de dire” (“Poisson … used to say”). Also seen in a book of quotes, Alphonse Rebière (ed.), Mathématiques et Mathématiciens: Pensées et Curiosités (1893), 158, as “La vie n’est bonne qu'à étudier et à enseigner les mathématiques”, which Webmaster translates as “Life is good only to study and to teach mathematics”. Also currently found online as “La vie n’est bonne que pour deux choses: découvrir les mathématiques et enseigner les mathématiques”, translated as “Life is good for only two things, discovering mathematics and teaching mathematics”, in Howard Whitley Eves, Mathematical Circles Adieu: A Fourth Collection of Mathematical Stories and Anecdotes (1977), 22. Arago was a contemporary of Poisson, and Webmaster speculates from the context of the biography that perhaps Arago knew the quote firsthand from Poisson. Webmaster has not yet been able to trace either version to a primary source written by Poisson. (Can you help?)
Like almost every subject of human interest, this one [mathematics] is just as easy or as difficult as we choose to make it. A lifetime may be spent by a philosopher in discussing the truth of the simplest axiom. The simplest fact as to our existence may fill us with such wonder that our minds will remain overwhelmed with wonder all the time. A Scotch ploughman makes a working religion out of a system which appalls a mental philosopher. Some boys of ten years of age study the methods of the differential calculus; other much cleverer boys working at mathematics to the age of nineteen have a difficulty in comprehending the fundamental ideas of the calculus.
In Teaching of Mathematics (1902), 19-20.
Like Molière’s M. Jourdain, who spoke prose all his life without knowing it, mathematicians have been reasoning for at least two millennia without being aware of all the principles underlying what they were doing. The real nature of the tools of their craft has become evident only within recent times A renaissance of logical studies in modern times begins with the publication in 1847 of George Boole’s The Mathematical Analysis of Logic.
Co-authored with James R. Newman in Gödel's Proof (1986, 2005), 30.
Like the crest of a peacock, like the gem on the head of a snake, so is mathematics at the head of all knowledge.
From the oldest extant Indian astronomical text, Vedanga Jyotisa (c. 500 B.C.). Quoted, as cited by George Gheverghese Joseph, in Dick Teresi, Lost Discoveries (2003), 28. G. G. Joseph has written a book by the title Crest of the Peacock (1991).
Little can be understood of even the simplest phenomena of nature without some knowledge of mathematics, and the attempt to penetrate deeper into the mysteries of nature compels simultaneous development of the mathematical processes.
In Teaching of Mathematics in the Elementary and the Secondary School (1906), 16.
Logic issues in tautologies, mathematics in identities, philosophy in definitions; all trivial, but all part of the vital work of clarifying and organising our thought.
'Last Papers: Philosophy' (1929), in The Foundations of Mathematics and Other Logical Essays (1931), 264.
Logic it is called [referring to Whitehead and Russell’s Principia Mathematica] and logic it is, the logic of propositions and functions and classes and relations, by far the greatest (not merely the biggest) logic that our planet has produced, so much that is new in matter and in manner; but it is also mathematics, a prolegomenon to the science, yet itself mathematics in its most genuine sense, differing from other parts of the science only in the respects that it surpasses these in fundamentally, generality and precision, and lacks traditionality. Few will read it, but all will feel its effect, for behind it is the urgence and push of a magnificent past: two thousand five hundred years of record and yet longer tradition of human endeavor to think aright.
In Science (1912), 35, 110, from his book review on Alfred North Whitehead and Bertrand Russell, Principia Mathematica.
LOGIC, n. The art of thinking and reasoning in strict accordance with the limitations and incapacities of the human misunderstanding. The basic of logic is the syllogism, consisting of a major and a minor premise and a conclusion—thus:
Major Premise: Sixty men can do a piece of work sixty times as quickly as one man.
Minor Premise: One man can dig a post-hole in sixty seconds; therefore—
Conclusion: Sixty men can dig a post-hole in one second.
This may be called the syllogism arithmetical, in which, by combining logic and mathematics, we obtain a double certainty and are twice blessed.
Major Premise: Sixty men can do a piece of work sixty times as quickly as one man.
Minor Premise: One man can dig a post-hole in sixty seconds; therefore—
Conclusion: Sixty men can dig a post-hole in one second.
This may be called the syllogism arithmetical, in which, by combining logic and mathematics, we obtain a double certainty and are twice blessed.
The Collected Works of Ambrose Bierce (1911), Vol. 7, The Devil's Dictionary, 196.
Making mathematics accessible to the educated layman, while keeping high scientific standards, has always been considered a treacherous navigation between the Scylla of professional contempt and the Charybdis of public misunderstanding.
In Rota's 'Introduction' written (1980) to preface Philip J. Davis and Reuben Hersh, The Mathematical Experience (1981, 2012), xxiii.
Making out an income tax is a lesson in mathematics: addition, division, multiplication and extraction.
In Evan Esar, 20,000 Quips and Quotes, 419.
Man chooses either life or death, but he chooses; everything he does, from going to the toilet to mathematical speculation, is an act of religious worship, either of God or of himself.
Quoted in Kim Lim (ed.), 1,001 Pearls of Spiritual Wisdom: Words to Enrich, Inspire, and Guide Your Life (2014), 15
Man has never been a particularly modest or self-deprecatory animal, and physical theory bears witness to this no less than many other important activities. The idea that thought is the measure of all things, that there is such a thing as utter logical rigor, that conclusions can be drawn endowed with an inescapable necessity, that mathematics has an absolute validity and controls experience—these are not the ideas of a modest animal. Not only do our theories betray these somewhat bumptious traits of self-appreciation, but especially obvious through them all is the thread of incorrigible optimism so characteristic of human beings.
In The Nature of Physical Theory (1936), 135-136.
Man is full of desires: he loves only those who can satisfy them all. “This man is a good mathematician,” someone will say. But I have no concern for mathematics; he would take me for a proposition. “That one is a good soldier.” He would take me for a besieged town. I need, that is to say, a decent man who can accommodate himself to all my desires in a general sort of way.
From Pensées (1670), Sect. 6, Aphorism 18. As translated in W.H. Auden and L. Kronenberger (eds.) The Viking Book of Aphorisms (1966), 199. From the original French, “L’homme est plein de besoins: il n’aime que ceux qui peuvent les remplir tous. ‘C’est un bon mathématicien,’ dira-t-on. Mais je n’ai que faire de mathématiques; il me prendroit pour une proposition. ‘C’est un bon guerrier.’ Il me prendroit pour une place assiégée. Il faut donc un honnête homme qui puisse s’accommoder à tous mes besoins généralement,” in Oeuvres Complètes de Blaise Pascal (1858), Vol. 1, 276.
Many arts there are which beautify the mind of man; of all other none do more garnish and beautify it than those arts which are called mathematical.
The Elements of Geometric of the most ancient Philosopher Euclide of Megara (1570), Note to the Reader. In Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 44.
Many of the proofs in mathematics are very long and intricate. Others, though not long, are very ingeniously constructed.
In Mathematics for the General Reader (1959, 2017), 21.
Many who have never had an opportunity of knowing any more about mathematics confound it with arithmetic, and consider it an arid science. In reality, however, it is a science which requires a great amount of imagination.
In a letter to Madame Schabelskoy, quoted in Sónya Kovalévsky: Her Recollections of Childhood, translated by Isabel F. Hapgood (1895), 316.
Marx founded a new science: the science of history. … The sciences we are familiar with have been installed in a number of great “continents”. Before Marx, two such continents had been opened up to scientific knowledge: the continent of Mathematics and the continent of Physics. The first by the Greeks (Thales), the second by Galileo. Marx opened up a third continent to scientific knowledge: the continent of History.
In Lenin and Philosophy, and Other Writings (1971), 4.
Math is like love—a simple idea but it can get complicated.
Quoted in Jon Fripp, Michael Fripp, Deborah Fripp Speaking of Science: Notable Quotes on Science, Engineering, and the Environment (2000), 45, and attributed to “R. Drabek” with no further source information. Webmaster wonders if this is a typo for mathematician, Pavel Drábek.
Math was more fun than anything else. It was always a game to me.
In interview with Laurel M. Sheppard, 'An Interview with Mary Ross: First Native American Woman Engineer Aerospace Pioneer Returns to her Native American Roots', on website of Lash Publications.
Mathematic stands forth as that which unites, mediates between Man and Nature, Inner and Outer world, Thought and Perception, [as no other subject does].
In Die Erziehung der Menschheit (1826). Adapted and translated in William Henry Herford, 'The School: Mathematic', The Student’s Froebel (1894), Vol. 1, 84.
Mathematical “truth” is no “truer” than any other, and Pilate’s question is still meaningless. There are no absolutes, even in mathematics.
In 'What Mathematics Has Meant to Me', Mathematics Magazine (Jan-Feb 1951), 24, 161. [Note: Pontius Pilate questioned Jesus, “What is truth?” (John 18:38). —Webmaster]
Mathematical discoveries, like springtime violets in the woods, have their season which no human can hasten or retard.
Quoted in E.T. Bell, The Development of Mathematics (1945).
Mathematical discoveries, small or great … are never born of spontaneous generation. They always presuppose a soil seeded with preliminary knowledge and well prepared by labour, both conscious and subconscious.
As given, without citation, in Eric Temple Bell, Men of Mathematics (1937), 548.
Mathematical economics is old enough to be respectable, but not all economists respect it. It has powerful supporters and impressive testimonials, yet many capable economists deny that mathematics, except as a shorthand or expository device, can be applied to economic reasoning. There have even been rumors that mathematics is used in economics (and in other social sciences) either for the deliberate purpose of mystification or to confer dignity upon commonplaces as French was once used in diplomatic communications. …. To be sure, mathematics can be extended to any branch of knowledge, including economics, provided the concepts are so clearly defined as to permit accurate symbolic representation. That is only another way of saying that in some branches of discourse it is desirable to know what you are talking about.
In J.R. Newman (ed.), Commentary on Cournot, Jevons and the Mathematics of Money', The World of Mathematics (1956), Vol. 2, 1200.
Mathematical instruction, in this as well as in other countries, is laboring under a burden of century-old tradition. Especially is this so with reference to the teaching of geometry. Our texts in this subject are still patterned more or less closely after the model of Euclid, who wrote over two thousand years ago, and whose text, moreover, was not intended for the use of boys and girls, but for mature men.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 5.
Mathematical knowledge, therefore, appears to us of value not only in so far as it serves as means to other ends, but for its own sake as well, and we behold, both in its systematic external and internal development, the most complete and purest logical mind-activity, the embodiment of the highest intellect-esthetics.
In 'Ueber Wert und angeblichen Unwert der Mathematik', Jahresbericht der Deutschen Mathematiker Vereinigung, Bd. 13, 381.
Mathematical language is not only the simplest and most easily understood of any, but the shortest also.
In Works of Henry, Lord Brougham: Vol. 7: Rhetorical and literary Dissertions and Addresses (1856), 317, footnote.
Mathematical magic combines the beauty of mathematical structure with the entertainment value of a trick.
In 'Preface', Mathematics, Magic, and Mystery (1956), ix. Webmaster checked the actual page, in the 1956 edition, and there Gardner plainly uses the beginning words “Mathematical magic.” On page 113, Gardner credits Royal V. Heath for originating the term “Mathemagic,” used as Heath’s book title in 1933. Anywhere you see the quote incorrectly beginning with “Mathemagical mathematics…” or saying Gardner coined the term “mathemagical” obviously did not check the primary source.
Mathematical proofs are essentially of three different types: pre-formal; formal; post-formal. Roughly the first and third prove something about that sometimes clear and empirical, sometimes vague and ‘quasi-empirical’ stuff, which is the real though rather evasive subject of mathematics.
In Mathematics, Science and Epistemology (1980), Vol. 2, 69.
Mathematical proofs, like diamonds, are hard and clear, and will be touched with nothing but strict reasoning.
In 'Mr Locke’s Reply to the Bishop of Worcester’s Answer to his Second Letter', collected in The Works of John Locke (1824), Vol. 3, 428.
Mathematical reasoning is deductive in the sense that it is based upon definitions which, as far as the validity of the reasoning is concerned (apart from any existential import), needs only the test of self-consistency. Thus no external verification of definitions is required in mathematics, as long as it is considered merely as mathematics.
In Universal Algebra (1898), Preface, vi.
Mathematical research, with all its wealth of hidden treasure, is all too apt to yield nothing to our research: for it is haunted by certain ignes fatui—delusive phantoms, that float before us, and seem so fair, and are all but in our grasp, so nearly that it never seems to need more than one step further, and the prize shall be ours! Alas for him who has been turned aside from real research by one of these spectres—who has found a music in its mocking laughter—and who wastes his life and energy on the desperate chase!
Written without pseudonym as Charles L. Dodgson, in Introduction to A New Theory of Parallels (1888, 1890), xvi. Note: Ignes fatui, the plural of ignes fatuus (foolish fire), refers to a will-o'-the-wisp: something deceptive or deluding.
Mathematical rigor is like clothing; in its style it ought to suit the occasion, and it diminishes comfort and restrains freedom of movement if it is either too loose or too tight.
In Differential Equations: With Applications and Historical Notes (1972), ix.
Mathematical science is in my opinion an indivisible whole, an organism whose vitality is conditioned upon the connection of its parts. For with all the variety of mathematical knowledge, we are still clearly conscious of the similarity of the logical devices, the relationship of the ideas in mathematics as a whole and the numerous analogies in its different departments.
In 'Mathematical Problems', Bulletin American Mathematical Society, 8, 478.
Mathematical studies … when combined, as they now generally are, with a taste for physical science, enlarge infinitely our views of the wisdom and power displayed in the universe. The very intimate connexion indeed, which, since the date of the Newtonian philosophy, has existed between the different branches of mathematical and physical knowledge, renders such a character as that of a mere mathematician a very rare and scarcely possible occurrence.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 3, 184.
Mathematical theories have sometimes been used to predict phenomena that were not confirmed until years later. For example, Maxwell’s equations, named after physicist James Clerk Maxwell, predicted radio waves. Einstein’s field equations suggested that gravity would bend light and that the universe is expanding. Physicist Paul Dirac once noted that the abstract mathematics we study now gives us a glimpse of physics in the future. In fact, his equations predicted the existence of antimatter, which was subsequently discovered. Similarly, mathematician Nikolai Lobachevsky said that “there is no branch of mathematics, however abstract, which may not someday be applied to the phenomena of the real world.”
In 'Introduction', The Math Book: From Pythagoras to the 57th Dimension, 250 Milestones in the History of Mathematics (2009), 12.
Mathematicians attach great importance to the elegance of their methods and their results. This is not pure dilettantism. What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details. But this is exactly what yields great results, in fact the more we see this aggregate clearly and at a single glance, the better we perceive its analogies with other neighboring objects, consequently the more chances we have of divining the possible generalizations. Elegance may produce the feeling of the unforeseen by the unexpected meeting of objects we are not accustomed to bring together; there again it is fruitful, since it thus unveils for us kinships before unrecognized. It is fruitful even when it results only from the contrast between the simplicity of the means and the complexity of the problem set; it makes us then think of the reason for this contrast and very often makes us see that chance is not the reason; that it is to be found in some unexpected law. In a word, the feeling of mathematical elegance is only the satisfaction due to any adaptation of the solution to the needs of our mind, and it is because of this very adaptation that this solution can be for us an instrument. Consequently this esthetic satisfaction is bound up with the economy of thought.
In 'The Future of Mathematics', Monist, 20, 80. Translated from the French by George Bruce Halsted.
Mathematicians can and do fill in gaps, correct errors, and supply more detail and more careful scholarship when they are called on or motivated to do so. Our system is quite good at producing reliable theorems that can be backed up. It’s just that the reliability does not primarily come from mathematicians checking formal arguments; it come from mathematicians thinking carefully and critically about mathematical ideas.
Concerning revision of proofs. In 'On Proof and Progress in Mathematics', For the Learning of Mathematics (Feb 1995), 15, No. 1, 33. Reprinted from Bulletin of the American Mathematical Society (1994), 30, No. 2, 170.
Mathematicians create by acts of insight and intuition. Logic then sanctions the conquests of intuition. It is the hygiene that mathematics practices to keep its ideas healthy and strong. Moreover, the whole structure rests fundamentally on uncertain ground, the intuition of humans. Here and there an intuition is scooped out and replaced by a firmly built pillar of thought; however, this pillar is based on some deeper, perhaps less clearly defined, intuition. Though the process of replacing intuitions with precise thoughts does not change the nature of the ground on which mathematics ultimately rests, it does add strength and height to the structure.
In Mathematics in Western Culture (1964), 408.
Mathematicians have long since regarded it as demeaning to work on problems related to elementary geometry in two or three dimensions, in spite of the fact that it it precisely this sort of mathematics which is of practical value.
As coauthor with and G.C. Shephard, in Handbook of Applicable Mathematics, Volume V, Combinatorics and Geometry (1985), v.
Mathematics … above all other subjects, makes the student lust after knowledge, fills him, as it were, with a longing to fathom the cause of things and to employ his own powers independently; it collects his mental forces and concentrates them on a single point and thus awakens the spirit of individual inquiry, self-confidence and the joy of doing; it fascinates because of the view-points which it offers and creates certainty and assurance, owing to the universal validity of its methods. Thus, both what he receives and what he himself contributes toward the proper conception and solution of a problem, combine to mature the student and to make him skillful, to lead him away from the surface of things and to exercise him in the perception of their essence. A student thus prepared thirsts after knowledge and is ready for the university and its sciences. Thus it appears, that higher mathematics is the best guide to philosophy and to the philosophic conception of the world (considered as a self-contained whole) and of one’s own being.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 40. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 49.
Mathematics … belongs to every inquiry, moral as well as physical. Even the rules of logic, by which it is rigidly bound, could not be deduced without its aid. The laws of argument admit of simple statement, but they must be curiously transposed before they can be applied to the living speech and verified by observation. In its pure and simple form the syllogism cannot be directly compared with all experience, or it would not have required an Aristotle to discover it. It must be transmuted into all the possible shapes in which reasoning loves to clothe itself. The transmutation is the mathematical process in the establishment of the law.
From Memoir (1870) read before the National Academy of Sciences, Washington, printed in 'Linear Associative Algebra', American Journal of Mathematics (1881), 4, 97-98.
Mathematics … certainly would never have come into existence if mankind had known from the beginning that in all nature there is no perfectly straight line, no true circle, no standard of measurement.
From 'Of the First and Last Things', All Too Human: A Book For Free Spirits (1878, 1908), Part 1, section 11, 31.
Mathematics … engages, it fructifies, it quickens, compels attention, is as circumspect as inventive, induces courage and self-confidence as well as modesty and submission to truth. It yields the essence and kernel of all things, is brief in form and overflows with its wealth of content. It discloses the depth and breadth of the law and spiritual element behind the surface of phenomena; it impels from point to point and carries within itself the incentive toward progress; it stimulates the artistic perception, good taste in judgment and execution, as well as the scientific comprehension of things.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 40. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 49.
Mathematics … is man’s own handiwork, subject only to the limitations imposed by the laws of thought.
With co-author James R. Newman, in Mathematics and the Imagination (1940), 359.
Mathematics … is necessarily the foundation of exact thought as applied to natural phenomena.
In An Introduction to Mathematics (1911), 8.
MATHEMATICS … the general term for the various applications of mathematical thought, the traditional field of which is number and quantity. It has been usual to define mathematics as “the science of discrete and continuous magnitude.”
Opening statement in article 'Mathematics', Encyclopedia Britannica (1911, 11th ed.), Vol. 17, 878. Whitehead then indicated this was an inadequate definition, which he then discussed at length and tried to give an improved definition later in the article. See the quote beginning “Definition of Mathematics…” on the Alfred North Whitehead Quotes page on this website.
Mathematics is a language.
Remark at a Yale faculty discussion concerning the difference between languages and mathematics for elective course requirements. Quoted, “as he is reported to have stated,” in The Yale Scientific Magazine (1927), Vols. 1-4, 17.
Mathematics accomplishes really nothing outside of the realm of magnitude; marvellous, however, is the skill with which it masters magnitude wherever it finds it. We recall at once the network of lines which it has spun about heavens and earth; the system of lines to which azimuth and altitude, declination and right ascension, longitude and latitude are referred; those abscissas and ordinates, tangents and normals, circles of curvature and evolutes; those trigonometric and logarithmic functions which have been prepared in advance and await application. A look at this apparatus is sufficient to show that mathematicians are not magicians, but that everything is accomplished by natural means; one is rather impressed by the multitude of skilful machines, numerous witnesses of a manifold and intensely active industry, admirably fitted for the acquisition of true and lasting treasures.
In Werke [Kehrbach] (1890), Bd. 5, 101. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 13.
Mathematics and art are quite different. We could not publish so many papers that used, repeatedly, the same idea and still command the respect of our colleagues.
As given in essay, Ronald Coifman and Robert S. Strichartz, 'The School of Antoni Zygmund', collected in Peter Duren (ed.), A Century of Mathematics in America (1989), 348. The comment was made “after passing through several rooms in a museum filled with the paintings of a rather well-known modem painter”. The authors acknowledge students of Zygmund provided personal recollections to them for the essay in general. Webmaster speculates the quote is from a student recollection, and not necessarily verbatim.
Mathematics and music, the most sharply contrasted fields of scientific activity which can be found, and yet related, supporting each other, as if to show forth the secret connection which ties together all the activities of our mind, and which leads us to surmise that the manifestations of the artist’s genius are but the unconscious expressions of a mysteriously acting rationality.
In Vorträge und Reden (1884, 1896), Vol 1, 122. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 191. From the original German, “Mathematik und Musik, der schärfste Gegensatz geistiger Thätigkeit, den man auffinden kann, und doch verbunden, sich unterstützend, als wollten sie die geheime Consequenz nachweisen, die sich durch alle Thätigkeiten unseres Geistes hinzieht, und die auch in den Offenbarungen des künstlerischen Genius uns unbewusste Aeusserungen geheimnissvoll wirkender Vernunftmässigkeit ahnen lässt.”
Mathematics and Poetry are … the utterance of the same power of imagination, only that in the one case it is addressed to the head, and in the other, to the heart.
From a review of William Rowan Hamilton’s, Lectures on Quaternions (1853), in 'The Imagination in Mathematics', The North American Review (Jul 1857), 85, No. 176, 230. Also in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 189. The original text has “Mathematics…” but the latter text gives “Mathesis…”. The ellipsis is for the word “therefore”.
Mathematics appreciation is more than a course. It is an attitude that we should cultivate in every mathematics course.
In 'Loving Math Infinitely', The Chronicle of Higher Education (19 Jan 2001).
Mathematics as a science commenced when first someone, probably a Greek, proved propositions about any things or about some things, without specification of definite particular things. These propositions were first enunciated by the Greeks for geometry; and, accordingly, geometry was the great Greek mathematical science.
In An Introduction to Mathematics (1911), 15.
Mathematics as an expression of the human mind reflects the active will, the contemplative reason, and the desire for aesthetic perfection. Its basic elements are logic and intuition, analysis and construction, generality and individuality. Though different traditions may emphasize different aspects, it is only the interplay of these antithetic forces and the struggle for their synthesis that constitute the life, usefulness, and supreme value of mathematical science.
As co-author with Herbert Robbins, in What Is Mathematics?: An Elementary Approach to Ideas and Methods (1941, 1996), x.
Mathematics as we practice it is much more formally complete and precise than other sciences, but it is much less formally complete and precise for its content than computer programs.
In 'On Proof and Progress in Mathematics', For the Learning of Mathematics (Feb 1995), 15, No. 1, 33. Reprinted from Bulletin of the American Mathematical Society (1994), 30, No. 2, 170.
Mathematics associates new mental images with ... physical abstractions; these images are almost tangible to the trained mind but are far removed from those that are given directly by life and physical experience. For example, a mathematician represents the motion of planets of the solar system by a flow line of an incompressible fluid in a 54-dimensional phase space, whose volume is given by the Liouville measure
Mathematics and Physics (1981), Foreward. Reprinted in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 90.
Mathematics because of its nature and structure is peculiarly fitted for high school instruction [Gymnasiallehrfach]. Especially the higher mathematics, even if presented only in its elements, combines within itself all those qualities which are demanded of a secondary subject.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 40. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 49.
Mathematics began to seem too much like puzzle solving. Physics is puzzle solving, too, but of puzzles created by nature, not by the mind of man.
Quoted in Joan Dash, 'Maria Goeppert-Mayer', A Life of One's Own, 252.
Mathematics can remove no prejudices and soften no obduracy. It has no influence in sweetening the bitter strife of parties, and in the moral world generally its action is perfectly null.
In James Wood, Dictionary of Quotations from Ancient and Modern, English and Foreign Sources (1893), 271:3.
Mathematics contain a great number of premises, and there is perhaps a kind of intellect that can search with ease a few premises to the bottom, and cannot in the least penetrate those matters in which there are many premises.
In Pascal’s Pensées (1958), 3.
Mathematics contains much that will neither hurt one if one does not know it nor help one if one does know it.
…...
Mathematics education is much more complicated than you expected, even though you expected it to be more complicated than you expected.
Mathematics gives the young man a clear idea of demonstration and habituates him to form long trains of thought and reasoning methodically connected and sustained by the final certainty of the result; and it has the further advantage, from a purely moral point of view, of inspiring an absolute and fanatical respect for truth. In addition to all this, mathematics, and chiefly algebra and infinitesimal calculus, excite to a high degree the conception of the signs and symbols—necessary instruments to extend the power and reach of the human mind by summarizing an aggregate of relations in a condensed form and in a kind of mechanical way. These auxiliaries are of special value in mathematics because they are there adequate to their definitions, a characteristic which they do not possess to the same degree in the physical and mathematical [natural?] sciences.
There are, in fact, a mass of mental and moral faculties that can be put in full play only by instruction in mathematics; and they would be made still more available if the teaching was directed so as to leave free play to the personal work of the student.
There are, in fact, a mass of mental and moral faculties that can be put in full play only by instruction in mathematics; and they would be made still more available if the teaching was directed so as to leave free play to the personal work of the student.
In 'Science as an Instrument of Education', Popular Science Monthly (1897), 253.
Mathematics had never had more than a secondary interest for him [her husband, George Boole]; and even logic he cared for chiefly as a means of clearing the ground of doctrines imagined to be proved, by showing that the evidence on which they were supposed to give rest had no tendency to prove them. But he had been endeavoring to give a more active and positive help than this to the cause of what he deemed pure religion.
In Eleanor Meredith Cobham, Mary Everest Boole: Collected Works (1931), 40.
Mathematics has beauties of its own—a symmetry and proportion in its results, a lack of superfluity, an exact adaptation of means to ends, which is exceedingly remarkable and to be found only in the works of the greatest beauty. … When this subject is properly and concretely presented, the mental emotion should be that of enjoyment of beauty, not that of repulsion from the ugly and the unpleasant.
In The Teaching of Mathematics in the Elementary and the Secondary School (1906), 44-45.
Mathematics has not a foot to stand upon which is not purely metaphysical.
'Kant in His Miscellaneous Essays', Blackwood's Magazine, 1830, 28, 244-68.
Mathematics has often been characterized as the most conservative of all sciences. This is true in the sense of the immediate dependence of new upon old results. All the marvellous new advancements presuppose the old as indispensable steps in the ladder. … Inaccessibility of special fields of mathematics, except by the regular way of logically antecedent acquirements, renders the study discouraging or hateful to weak or indolent minds.
In Number and its Algebra (1896), 136.
Mathematics has the completely false reputation of yielding infallible conclusions. Its infallibility is nothing but identity. Two times two is not four, but it is just two times two, and that is what we call four for short. But four is nothing new at all. And thus it goes on and on in its conclusions, except that in the higher formulas the identity fades out of sight.
As quoted in Richard von Mises, 'Mathematical Postulates and Human Understanding', collected in J.R. Newman (ed.) The World of Mathematics (1956), Vol. 3, 1754.

Mathematics in general is fundamentally the science of self-evident things.
In Anwendung der Differenlial-und Integralrechnung auf Geometric (1902), 26.
Mathematics in gross, it is plain, are a grievance in natural philosophy, and with reason…Mathematical proofs are out of the reach of topical arguments, and are not to be attacked by the equivocal use of words or declamation, that make so great a part of other discourses; nay, even of controversies.
In 'Mr Locke’s Reply to the Bishop of Worcester’s Answer to his Second Letter', collected in The Works of John Locke (1824), Vol. 3, 428.
Mathematics in its pure form, as arithmetic, algebra, geometry, and the applications of the analytic method, as well as mathematics applied to matter and force, or statics and dynamics, furnishes the peculiar study that gives to us, whether as children or as men, the command of nature in this its quantitative aspect; mathematics furnishes the instrument, the tool of thought, which we wield in this realm.
In Psychologic Foundations of Education (1898), 325.
Mathematics in its widest signification is the development of all types of formal, necessary, deductive reasoning.
In Universal Algebra (1898), Preface, vi.
Mathematics is a body of knowledge, but it contains no truths.
In Mathematics in Western Culture (1954), 9.
Mathematics is a broad-ranging field of study in which the properties and interactions of idealized objects are examined
In CRC Concise Encyclopedia of Mathematics (1998, 2nd ed. 2002 ), 1862.
Mathematics is a dangerous profession; an appreciable proportion of us go mad.
In A Mathematician’s Miscellany (1953), reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 104.
Mathematics is a form of poetry which transcends poetry in that it proclaims a truth; a form of reasoning which transcends reasoning in that it wants to bring about the truth it proclaims; a form of action, of ritual behavior, which does not find fulfilment in the act but must proclaim and elaborate a poetic form of truth.
'Why Mathematics Grows', Journal of the History of Ideas (Jan-Mar 1965), 26, No. 1, 3. In Salomon Bochner and Robert Clifford Gunning (ed.) Collected Papers of Salomon Bochner (1992), Vol. 4, 191. Footnoted as restating about Mathematics what was written about Myth by Henri Frankfort, et al., in The Intellectual Adventures of Ancient Man (1946), 8.
Mathematics is a fundamental mode of thinking, impossible to evade.
In 'The Spirit of Research', III, 'Mathematical Research', in The Monist (Oct 1922), 32, No. 4, 543.
Mathematics is a game played according to certain simple rules with meaningless marks on paper.
Given as narrative, without quotation marks, in Eric Temple Bell, Mathematics, Queen and Servant of Science (1951, 1961), 21.
Mathematics is a logical method … Mathematical propositions express no thoughts. In life it is never a mathematical proposition which we need, but we use mathematical propositions only in order to infer from propositions which do not belong to mathematics to others which equally do not belong to mathematics.
In Tractatus Logico Philosophicus (1922), 169 (statements 6.2-6.211).
Mathematics is a public activity. It occurs in a social context and has social consequences. Posing a problem, formulating a definition, proving a theorem are none of them private acts. They are all part of that larger social process we call science.
In 'Mathematics as an Objective Science', The American Mathematical Monthly (Aug-Sep 1979), 86, No. 7, 542. Reprinted in The Mathematical Intelligencer (1983), 5, No. 3.
Mathematics is a science continually expanding; and its growth, unlike some political and industrial events, is attended by universal acclamation.
From remarks made while opening the proceedings for the Mathematics Section (20 Sep 1904), Congress of Arts and Sciences (1905), Vol. 1, 455.
Mathematics is a science of Observation, dealing with reals, precisely as all other sciences deal with reals. It would be easy to show that its Method is the same: that, like other sciences, having observed or discovered properties, which it classifies, generalises, co-ordinates and subordinates, it proceeds to extend discoveries by means of Hypothesis, Induction, Experiment and Deduction.
In Problems of Life and Mind: The Method of Science and its Application (1874), 423-424. [The reals are the relations of magnitude.]
Mathematics is a structure providing observers with a framework upon which to base healthy, informed, and intelligent judgment. Data and information are slung about us from all directions, and we are to use them as a basis for informed decisions. … Ability to critically analyze an argument purported to be logical, free of the impact of the loaded meanings of the terms involved, is basic to an informed populace.
In 'Mathematics Is an Edifice, Not a Toolbox', Notices of the AMS (Oct 1996), 43, No. 10, 1108.
Mathematics is a study which, when we start from its most familiar portions, may be pursued in either of two opposite directions. The more familiar direction is constructive, towards gradually increasing complexity: from integers to fractions, real numbers, complex numbers; from addition and multiplication to differentiation and integration, and on to higher mathematics. The other direction, which is less familiar, proceeds, by analysing, to greater and greater abstractness and logical simplicity; instead of asking what can be defined and deduced from what is assumed to begin with, we ask instead what more general ideas and principles can be found, in terms of which what was our starting-point can be defined or deduced. It is the fact of pursuing this opposite direction that characterises mathematical philosophy as opposed to ordinary mathematics.
In Introduction to Mathematical Philosophy (1920), 1.
Mathematics is a type of thought which seems ingrained in the human mind, which manifests itself to some extent with even the primitive races, and which is developed to a high degree with the growth of civilization. … A type of thought, a body of results, so essentially characteristic of the human mind, so little influenced by environment, so uniformly present in every civilization, is one of which no well-informed mind today can be ignorant.
In Teaching of Mathematics in the Elementary and the Secondary School (1906), 14.
Mathematics is an experimental science, and definitions do not come first, but later on.
In 'On Operators in Physical Mathematics, part II', Proceedings of the Royal Society of London (15 Jun 1893), 54, 121.
Mathematics is an infinity of flexibles forcing pure thought into a cosmos. It is an arc of austerity cutting realms of reason with geodesic grandeur.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
Mathematics is an interesting intellectual sport but it should not be allowed to stand in the way of obtaining sensible information about physical processes.
Quoted in Nicholas J. Rose, Mathematical Maxims and Minims (1988).
Mathematics is an obscure field, an abstruse science, complicated and exact; yet so many have attained perfection in it that we might conclude almost anyone who seriously applied himself would achieve a measure of success.
In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 82.
Mathematics is as little a science as grammar is a language.
…...
Mathematics is being lazy. Mathematics is letting the principles do the work for you so that you do not have to do the work for yourself
In Marion Walter and Tom O'Brien, 'Memories of George Polya', Mathematics Teaching (Sep 1986), 116, 4.
Mathematics is both the door and the key to the sciences.
In Opus Majus (1267).
Mathematics is concerned only with the enumeration and comparison of relations.
Quoted in E. T. Bell, The Development of Mathematics (1945).
Mathematics is crystallized clarity, precision personified, beauty distilled and rigorously sublimated.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
Mathematics is distinguished from all other sciences except only ethics, in standing in no need of ethics. Every other science, even logic—logic, especially—is in its early stages in danger of evaporating into airy nothingness, degenerating, as the Germans say, into an anachrioid [?] film, spun from the stuff that dreams are made of. There is no such danger for pure mathematics; for that is precisely what mathematics ought to be.
In Charles S. Peirce, Charles Hartshorne (ed.), Paul Weiss (ed.), Collected Papers of Charles Sanders Peirce (1931), Vol. 4, 200.
Mathematics is indeed dangerous in that it absorbs students to such a degree that it dulls their senses to everything else.
While a student, an observation made about his teacher, Professor Karl Schellbach. Quoted, without citation, in Howard W. Eves, Mathematical Circles Adieu, (1977).
Mathematics is like checkers in being suitable for the young, not too difficult, amusing, and without peril to the state.
— Plato
As given in Scripta Mathematica: A Quarterly Journal Devoted to the Philosophy, History, and Expository Treatment of Mathematics (1950), 17, 185.
Mathematics is like childhood diseases. The younger you get it, the better.
Quoted by Dudley Herschbach in 'Einstein as a Student', collected in Peter Galison (ed.), Gerald James Holton (ed.), Silvan S. Schweber (ed.), Einstein for the 21st Century: His Legacy in Science, Srt, and Modern Culture (2008), 236. The remark quoted was footnoted as heard by Dudley Herschbach many years earlier in a class by George Polya, but not found in print.
Mathematics is much more than a language for dealing with the physical world. It is a source of models and abstractions which will enable us to obtain amazing new insights into the way in which nature operates. Indeed, the beauty and elegance of the physical laws themselves are only apparent when expressed in the appropriate mathematical framework.
In Principles of Electrodynamics (1972, 1987), 105.
Mathematics is music for the mind; music is mathematics for the soul.
In Nat Shapiro (ed.) An Encyclopedia of Quotations About Music (1981), 3.
Mathematics is no more the art of reckoning and computation than architecture is the art of making bricks or hewing wood, no more than painting is the art of mixing colors on a palette, no more than the science of geology is the art of breaking rocks, or the science of anatomy the art of butchering.
In Lectures on Science, Philosophy and Art (1908), 29.
Mathematics is not a book confined within a cover and bound between brazen clasps, whose contents it needs only patience to ransack; it is not a mine, whose treasures may take long to reduce into possession, but which fill only a limited number of veins and lodes; it is not a soil, whose fertility can be exhausted by the yield of successive harvests; it is not a continent or an ocean, whose area can be mapped out and its contour defined: it is limitless as that space which it finds too narrow for its aspirations; its possibilities are as infinite as the worlds which are forever crowding in and multiplying upon the astronomer’s gaze; it is as incapable of being restricted within assigned boundaries or being reduced to definitions of permanent validity, as the consciousness of life, which seems to slumber in each monad, in every atom of matter, in each leaf and bud cell, and is forever ready to burst forth into new forms of vegetable and animal existence.
From Commemoration Day Address (22 Feb 1877) at Johns Hopkins University, Baltimore, collected in The Collected Mathematical Papers: (1870-1883) (1909), 77-78.
Mathematics is not a careful march down a well-cleared highway, but a journey into a strange wilderness, where the explorers often get lost. Rigour should be a signal to the historian that the maps have been made, and the real explorers have gone elsewhere.
'Mathematics and History', Mathematical Intelligencer (1992), 4, No. 4, 10.
Mathematics is not a contemplative but a creative subject.
In A Mathematician's Apology (1940, 2012), 43.
Mathematics is not a deductive science—that’s a cliché. When you try to prove a theorem, you don’t just list the hypotheses, and then start to reason. What you do is trial and error, experiment and guesswork.
In I Want to be a Mathematician: an Automathography in Three Parts (1985), 321.
Mathematics is not arithmetic. Though mathematics may have arisen from the practices of counting and measuring it really deals with logical reasoning in which theorems—general and specific statements—can be deduced from the starting assumptions. It is, perhaps, the purest and most rigorous of intellectual activities, and is often thought of as queen of the sciences.
Essay,'Private Games', in Lewis Wolpert, Alison Richards (eds.), A Passion for Science (1988), 53.
Mathematics is not only one of the most valuable inventions—or discoveries—of the human mind, but can have an aesthetic appeal equal to that of anything in art. Perhaps even more so, according to the poetess who proclaimed, “Euclid alone hath looked at beauty bare.”
From 'The Joy of Maths'. Collected in Arthur C. Clarke, Greetings, Carbon-Based Bipeds!: Collected Essays, 1934-1998, 460.
Mathematics is not only real, but it is the only reality. That is that entire universe is made of matter, obviously. And matter is made of particles. It’s made of electrons and neutrons and protons. So the entire universe is made out of particles. Now what are the particles made out of? They’re not made out of anything. The only thing you can say about the reality of an electron is to cite its mathematical properties. So there’s a sense in which matter has completely dissolved and what is left is just a mathematical structure.
In 'Gardner on Gardner: JPBM Communications Award Presentation', Focus-The Newsletter of the Mathematical Association of America (Dec 1994), 14, No. 6. Also, first sentence as filler, with citation, after Washek F. Pfeffer, 'A Devil's Platform', The American Mathematical Monthly (Dec 2008), 115, No. 10, 947.
Mathematics is not the discoverer of laws, for it is not induction; neither is it the framer of theories, for it is not hypothesis; but it is the judge over both, and it is the arbiter to which each must refer its claims; and neither law can rule nor theory explain without the sanction of mathematics.
In 'Linear Associative Algebra', American Journal of Mathematics (1881), 4, 97.
Mathematics is not yet capable of coping with the naivete of the mathematician himself.
In 'The Study of Man: Sociology Learns the Language of Mathematics', Commentary (1 Sep 1952). Reprinted in James Roy Newman, The World of Mathematics (1956), Vol. 2, 1301.
Mathematics is not yet ready for such problems.
Given, without citation, as the comment by Paul Erdös on the intractability of the 3x + 1 problem, by Jeffrey C. Lagarias in 'The 3x + 1 Problem and Its Generalizations', The American Mathematical Monthly, (Jan 1985), 92, No. 1, 3. Collected in Jeffrey C. Lagarias, The Ultimate Challenge: The 3x+1 Problem (2010), 31.
Mathematics is of two kinds, Rigorous and Physical. The former is Narrow: the latter Bold and Broad. To have to stop to formulate rigorous demonstrations would put a stop to most physico-mathematical inquiries. Am I to refuse to eat because I do not fully understand the mechanism of digestion?
As quoted by Charles Melbourne Focken in Dimensional Methods and Their Applications (1953), 17.
Mathematics is often considered a difficult and mysterious science, because of the numerous symbols which it employs. Of course, nothing is more incomprehensible than a symbolism which we do not understand. … But this is not because they are difficult in themselves. On the contrary they have invariably been introduced to make things easy. … [T]he symbolism is invariably an immense simplification. It … represents an analysis of the ideas of the subject and an almost pictorial representation of their relations to each other.
In Introduction to Mathematics (1911), 59-60.
Mathematics is often erroneously referred to as the science of common sense. Actually, it may transcend common sense and go beyond either imagination or intuition. It has become a very strange and perhaps frightening subject from the ordinary point of view, but anyone who penetrates into it will find a veritable fairyland, a fairyland which is strange, but makes sense, if not common sense.
With co-author James R. Newman, in Mathematics and the Imagination (1940), 359.
Mathematics is one of the oldest of the sciences; it is also one of the most active, for its strength is the vigour of perpetual youth.
In Presidential Address British Association for the Advancement of Science, Section A, (1897), Nature, 66, 378.
Mathematics is perfectly free in its development and is subject only to the obvious consideration, that its concepts must be free from contradictions in themselves, as well as definitely and orderly related by means of definitions to the previously existing and established concepts.
In Grundlagen einer allgemeinen Manigfaltigkeitslehre (1883), Sect. 8.
Mathematics is strange: many make thousands but not many make millions.
In Evan Esar, 20,000 Quips and Quotes, 250.
Mathematics is that form of intelligence in which we bring the objects of the phenomenal world under the control of the conception of quantity.
Offered as a provision definition, in 'The Departments of Mathematics, and their Mutual Relations', Journal of Speculative Philosophy (Apr 1871), 5, No. 2, 164.
Mathematics is that peculiar science in which the importance of a work can be measured by the number of earlier publications rendered superfluous by it.
As stated in narrative, without quotation marks, in Joong Fang, Bourbaki (1970), 18, citing “as Hilbert declared at the end of his famous paper on the twenty-three unsolved problems.” Webmaster has not identified this in that paper, however. Also quoted, without citation, in Harold Eves, Mathematical Circles Revisited (1971), as “One can measure the importance of a scientific work by the number of earlier publications rendered superfluous by it.”
Mathematics is the art of giving the same name to different things.
Henri Poincaré and George Bruce Halsted (trans.) The Foundations of Science: Science and Hypothesis (1921), 375.
Mathematics is the cheapest science. Unlike physics or chemistry, it does not require any expensive equipment. All one needs for mathematics is a pencil and paper.
Quoted in 'And Sometimes the Mathematician Wants a Powerful Computer', in Donald J. Albers and Gerald L. Alexanderson (eds.), Mathematical People (1985). In John De Pillis, 777 Mathematical Conversation Starters (2002), 193.
Mathematics is the classification and study of all possible patterns.
In Prelude to Mathematics (1955), 12.
Mathematics is the gate and key of the sciences. ... Neglect of mathematics works injury to all knowledge, since he who is ignorant of it cannot know the other sciences or the things of this world. And what is worse, men who are thus ignorant are unable to perceive their own ignorance and so do not seek a remedy.
In Opus Majus, Part 4, Distinctia Prima, cap. 1.
Mathematics is the key and door to the sciences.
Mathematics is the language in which God wrote the universe.
Quoted in Kim Lim (ed.), 1,001 Pearls of Spiritual Wisdom: Words to Enrich, Inspire, and Guide Your Life (2014), 33
Mathematics is the language of languages, the best school for sharpening thought and expression, is applicable to all processes in nature; and Germany needs mathematical gymnasia. Mathematics is God’s form of speech, and simplifies all things organic and inorganic. As knowledge becomes real, complete and great it approximates mathematical forms. It mediates between the worlds of mind and of matter.
Summarizing the ideas presented by Christian Heinrich Dillmann in Die Mathematik die Fackelträgerin einer neuen Zeit (1889). From book review, 'Recent Literature on Arithmetic and Arithmetical Teaching', in Granville Stanley Hall (ed.), The Pedagogical Seminary (1892), 2, 168. Dillmann’s book title translates as “Mathematics the Torchbearer of a New Era”. (However, Conant concluded that it was a “loosely-written, vague and incoherent book, which belies every anticipation awakened by its attractive title.”)
Mathematics is the life supreme. The life of the gods is mathematics. All divine messengers are mathematicians. Pure mathematics is religion. Its attainment requires a theophany.
In Schriften (1901), Bd.. 2, 223.
Mathematics is the most exact science, and its conclusions are capable of absolute proof. But this is so only because mathematics does not attempt to draw absolute conclusions. All mathematical truths are relative, conditional.
(1923). Quoted, without source, in E.T. Bell, Men of Mathematics (1937), Vol. 1, li.
Mathematics is the only good metaphysics.
Quoted in E. T. Bell, Men of Mathematics, xvii.
Mathematics is the only instructional material that can be presented in an entirely undogmatic way.
— Max Dehn
Address (18 Jan 1928) at the University of Frankfurt am Main, Germany. Trans. by Abe Schenitzer, and published in 'The Mentality of the Mathematician: A Characterization', The Mathematical Intelligencer (1983), 5, No. 2, 23.
Mathematics is the only true metaphysics.
Silvanus Phillips Thompson, Life of Lord Kelvin (1910), 10. In Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914)
Mathematics is the part of physics where experiments are cheap.
From an address (7 Mar 1997) at the discussion on 'Teaching of Mathematics' in Palais de Découverte, Paris. Quoted in Mathematical Understanding of Nature: Essays on Amazing Physical Phenomena and Their Understanding by Mathematicians (2014), Foreword, x.
Mathematics is the predominant science of our time; its conquests grow daily, though without noise; he who does not employ it for himself, will some day find it employed against himself.
In Werke [Kehrbach] (1890), Bd. 6, 105. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 12.
Mathematics is the queen of the sciences and arithmetic [number theory] is the queen of mathematics. She often condescends to render service to astronomy and other natural sciences, but in all relations, she is entitled to first rank.
I>Sartorius von
Waltershausen: Gauss zum Gedächtniss (1856), 79. Quoted in Robert Edouard Moritz, Memorabilia
Mathematica (1914), 271.
Mathematics is the queen of the sciences.
…...
Mathematics is the science of consistency; it is a picture of the universe; as Plato is said to have expressed the idea, “God eternally geometrizes.”
In 'The Poetry of Mathematics', The Mathematics Teacher (May 1926), 19, No. 5, 295.
Mathematics is the science of definiteness, the necessary vocabulary of those who know.
In A Scrap-book of Elementary Mathematics: Notes, Recreations, Essays (1908), 7.
Mathematics is the science of skillful operations with concepts and rules invented just for this purpose.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences,' Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1 (February 1960). Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 536.
Mathematics is the science of the connection of magnitudes. Magnitude is anything that can be put equal or unequal to another thing. Two things are equal when in every assertion each may be replaced by the other.
In Stücke aus dem Lehrbuche der Arithmetik, Werke (1904), Bd. 2, 298.
Mathematics is the science of the functional laws and transformations which enable us to convert figured extension and rated motion into number.
In 'The Departments of Mathematics, and their Mutual Relations', Journal of Speculative Philosophy (Apr 1871), 5, No. 2, 170.
Mathematics is the science of what is clear by itself.
As quoted in James R. Newman (ed.) The World of Mathematics (1956), 1747. From by the original German, “Die Math, als Wissenschaft von dem, was sich von selbst versteht”, in Maximilian Simon, Didaktik und Methodik des Rechnens und der Mathematik (1908), 37, which cites Jacobi, Inaugural Speech, Königsberg (7 Jul 1832).
Mathematics is the science which draws necessary conclusions.
First line of Linear Associative Algebra: read before the National Academy of Sciences, Washington City (1870), 2.
Mathematics is the science which uses easy words for hard ideas.
With co-author James R. Newman, in Mathematics and the Imagination (1940), 4.
Mathematics is the study of analogies between analogies. All science is. Scientists want to show that things that don’t look alike are really the same. That is one of their innermost Freudian motivations. In fact, that is what we mean by understanding.
In 'A Mathematician's Gossip', Indiscrete Thoughts (2008), 214.
Mathematics is the study which forms the foundation of the course [at West Point Military Academy]. This is necessary, both to impart to the mind that combined strength and versatility, the peculiar vigor and rapidity of comparison necessary for military action, and to pave the way for progress in the higher military sciences.
In Congressional Committee on Military Affairs, 1834, United States Bureau of Education, Bulletin 1912, No. 2, 10.
Mathematics is the tool specially suited for dealing with abstract concepts of any kind and there is no limit to its power in this field.
The Principles of Quantum Mechanics (1930, 1981), Preface, viii.
Mathematics is the universal art apodictic.
Quoted by C.J. Keyser, in Lectures on Science, Philosophy and Art (1908), 13. [“Apodictic” means clearly established or beyond dispute. —Webmaster]
Mathematics is written for mathematicians.
'To His Holiness Pope Paul III, in Copernicus: On the Revolutions of the Heavenly Spheres (1543), trans. A. M. Duncan (1976), 27.
Mathematics is, as it were, a sensuous logic, and relates to philosophy as do the arts, music, and plastic art to poetry.
Aphorism 365 from Selected Aphorisms from the Lyceum (1797-1800). In Friedrich Schlegel, translated by Ernst Behler and Roman Struc, Dialogue on Poetry and Literary Aphorisms (trans. 1968), 147.
Mathematics knows no races or geographic boundaries; for mathematics, the cultural world is one country.
In H. Eves, Mathematical Circles Squared (1972). As cited in Anton Zettl, Sturm-Liouville Theory (2005), 171.
Mathematics make the mind attentive to the objects which it considers. This they do by entertaining it with a great variety of truths, which are delightful and evident, but not obvious. Truth is the same thing to the understanding as music to the ear and beauty to the eye. The pursuit of it does really as much gratify a natural faculty implanted in us by our wise Creator as the pleasing of our senses: only in the former case, as the object and faculty are more spiritual, the delight is more pure, free from regret, turpitude, lassitude, and intemperance that commonly attend sensual pleasures.
In An Essay on the Usefulness of Mathematical Learning (1701), 3-4.
Mathematics may be compared to a mill of exquisite workmanship, which grinds you stuff of any degree of fineness; but, nevertheless, what you get out depends upon what you put in; and as the grandest mill in the world will not extract wheat-flour from peascods, so pages of formulae will not get a definite result out of loose data.
From Anniversary Address of the President to the Geological Society, Quarterly Journal of the Geological Society (1869), l. In 'Geological Reform', Collected Essays: Discourses, Biological and Geological (1894), Vol. 8, 333.
Mathematics may be defined as the the subject in which we never know what we are talking about, not whether what we are saying is true.
In 'Recent Work on the Principles of Mathematics', International Monthly (1901), 4, 84. This sentence is part of a longer quote that begins, “Pure mathematics consists entirely…”, on the Bertrand Russell Quotes page of this website.
Mathematics may be likened to a large rock whose interior composition we wish to examine. The older mathematicians appear as persevering stone cutters slowly attempting to demolish the rock from the outside with hammer and chisel. The later mathematicians resemble expert miners who seek vulnerable veins, drill into these strategic places, and then blast the rock apart with well placed internal charges.
From In Mathematical Circles (1969), 7.
Mathematics may, like poetry or music, “promote and sustain a lofty habit of mind.”
In A Mathematician's Apology (1940, 2012), 116.
Mathematics must subdue the flights of our reason; they are the staff of the blind; no one can take a step without them; and to them and experience is due all that is certain in physics.
In Oeuvres Completes (1880), t. 35, 219.
Mathematics pursues its own course unrestrained, not indeed with an unbridled licence which submits to no laws, but rather with the freedom which is determined by its own nature and in conformity with its own being.
In Die Entwickelung der Mathematik in den letzten Jahrhunderten (1869), 20. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 12. From the original German, “Die Mathematik folgt frei ihren eigenen Bahnen; zwar nicht mit der zügellosen Freiheit, die keinen Gesetzen unterliegt, sondern mit der Freiheit, die sich aus ihrer Natur heraus und mit ihr in Uebereinstimmung selbst determinirt.”
Mathematics renders its best service through the immediate furthering of rigorous thought and the spirit of invention.
In 'Mathematischer Lehrplan für Realschulen' Werke [Kehrbach] (1890), Bd. 5, 170. (Mathematics Curriculum for Secondary Schools). As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 51.
Mathematics seems to endow one with something like a new sense.
…...
Mathematics takes us still further from what is human, into the region of absolute necessity, to which not only the world, but every possible world, must conform.
In 'The Study of Mathematics', The New Quarterly (1907), reprinted in Philosophical Essays (1910). As quoted and cited in 'The Retreat From Pythagoras', The Basic Writings of Bertrand Russell, 1903-1959 (1992), 254.
Mathematics vindicates the right … to stand in the front rank of the pioneers that search the real truth and find it crystallized forever in brilliant gems.
In Lectures on the Philosophy of Mathematics (1918), 194.
Mathematics was born and nurtured in a cultural environment. Without the perspective which the cultural background affords, a proper appreciation of the content and state of present-day mathematics is hardly possible.
In Introduction to the Foundations of Mathematics (1952), 265.
Mathematics will not be properly esteemed in wider circles until more than the a b c of it is taught in the schools, and until the unfortunate impression is gotten rid of that mathematics serves no other purpose in instruction than the formal training of the mind. The aim of mathematics is its content, its form is a secondary consideration and need not necessarily be that historic form which is due to the circumstance that mathematics took permanent shape under the influence of Greek logic.
In Die Entivickelung der Mathematik in den letzten Jahrhunderten (1884), 6.
Mathematics—a wonderful science, but it hasn't yet come up with a way to divide one tricycle between three small boys.
In Bob Phillips, Phillips' Treasury of Humorous Quotations (2004), 171.
Mathematics—in a strict sense—is the abstract science which investigates deductively the conclusions implicit in the elementary conceptions of spatial and numerical relations.
In New English Dictionary as quoted in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 5. This definition of Mathematics appeared in Oxford English Dictionary (1933), as “The abstract science which investigates deductively the conclusions implicit in the elementary conceptions of spatial and numerical relations, and which includes as its main divisions geometry, arithmetic, and algebra.”
Mathematics, a creation of the mind, so accurately fits the outside world. … [There is a] fantastic amount of uniformity in the universe. The formulas of physics are compressed descriptions of nature's weird repetitions. The accuracy of those formulas, coupled with nature’s tireless ability to keep doing everything the same way, gives them their incredible power.
In book review, 'Adventures Of a Mathematician: The Man Who Invented the H-Bomb', New York Times (9 May 1976), 201. The book is a biography of Stanislaw Ulam, and this is Gardner’s description of one of Ulam’s reflections on nature and mathematics.
Mathematics, among all school subjects, is especially adapted to further clearness, definite brevity and precision in expression, although it offers no exercise in flights of rhetoric. This is due in the first place to the logical rigour with which it develops thought, avoiding every departure from the shortest, most direct way, never allowing empty phrases to enter. Other subjects excel in the development of expression in other respects: translation from foreign languages into the mother tongue gives exercise in finding the proper word for the given foreign word and gives knowledge of laws of syntax, the study of poetry and prose furnish fit patterns for connected presentation and elegant form of expression, composition is to exercise the pupil in a like presentation of his own or borrowed thoughtsand their development, the natural sciences teach description of natural objects, apparatus and processes, as well as the statement of laws on the grounds of immediate sense-perception. But all these aids for exercise in the use of the mother tongue, each in its way valuable and indispensable, do not guarantee, in the same manner as mathematical training, the exclusion of words whose concepts, if not entirely wanting, are not sufficiently clear. They do not furnish in the same measure that which the mathematician demands particularly as regards precision of expression.
In Anleitung zum mathematischen Unterricht in höheren Schulen (1906), 17.
Mathematics, as much as music or any other art, is one of the means by which we rise to a complete self-consciousness. The significance of mathematics resides precisely in the fact that it is an art; by informing us of the nature of our own minds it informs us of much that depends on our minds.
In Aspects of Science: Second Series (1926), 94.
Mathematics, from the earliest times to which the history of human reason can reach, has followed, among that wonderful people of the Greeks, the safe way of science. But it must not be supposed that it was as easy for mathematics as for logic, in which reason is concerned with itself alone, to find, or rather to make for itself that royal road. I believe, on the contrary, that there was a long period of tentative work (chiefly still among the Egyptians), and that the change is to be ascribed to a revolution, produced by the happy thought of a single man, whose experiments pointed unmistakably to the path that had to be followed, and opened and traced out for the most distant times the safe way of a science. The history of that intellectual revolution, which was far more important than the passage round the celebrated Cape of Good Hope, and the name of its fortunate author, have not been preserved to us. … A new light flashed on the first man who demonstrated the properties of the isosceles triangle (whether his name was Thales or any other name), for he found that he had not to investigate what he saw in the figure, or the mere concepts of that figure, and thus to learn its properties; but that he had to produce (by construction) what he had himself, according to concepts a priori, placed into that figure and represented in it, so that, in order to know anything with certainty a priori, he must not attribute to that figure anything beyond what necessarily follows from what he has himself placed into it, in accordance with the concept.
In Critique of Pure Reason, Preface to the Second Edition, (1900), 690.
Mathematics, including not merely Arithmetic, Algebra, Geometry, and the higher Calculus, but also the applied Mathematics of Natural Philosophy, has a marked and peculiar method or character; it is by preeminence deductive or demonstrative, and exhibits in a
nearly perfect form all the machinery belonging to this mode of obtaining truth. Laying down a very small number of first principles, either self-evident or requiring very little effort to prove them, it evolves a vast number of deductive truths and applications, by a procedure in the highest degree mathematical and systematic.
In Education as a Science (1879), 148.
Mathematics, indeed, is the very example of brevity, whether it be in the shorthand rule of the circle, c = πd, or in that fruitful formula of analysis, eiπ = -1, —a formula which fuses together four of the most important concepts of the science,—the logarithmic base, the
transcendental ratio π, and the imaginary and negative units.
In 'The Poetry of Mathematics', The Mathematics Teacher (May 1926), 19, No. 5, 293.
Mathematics, like dialectics, is an organ of the inner higher sense; in its execution it is an art like eloquence. Both alike care nothing for the content, to both nothing is of value but the form. It is immaterial to mathematics whether it computes pennies or guineas, to rhetoric whether it defends truth or error.
From Wilhelm Meislers Wanderjahre (1829), Zweites Buch. Collected in Goethe’s Werke (1830), Vol. 22, 252. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 36-37. The same book has another translation on p.202: “Mathematics, like dialectics, is an organ of the higher sense, in its execution it is an art like eloquence. To both nothing but the form is of value; neither cares anything for content. Whether mathematics considers pennies or guineas, whether rhetoric defends truth or error, is perfectly immaterial to either.” From the original German, “Die Mathematik ist, wie die Dialektik, ein Organ des inneren höheren Sinnes, in der Ausübung ist sie eine Kunst wie die Beredsamkeit. Für beide hat nichts Wert als die Form; der Gehalt ist ihnen gleichgültig. Ob die Mathematik Pfennige oder oder Guineen berechne, die Rhetorik Wahres oder Falsches verteidige, ist beiden vollkommen gleich.”
Mathematics, once fairly established on the foundation of a few axioms and definitions, as upon a rock, has grown from age to age, so as to become the most solid fabric that human reason can boast.
In Essays on the Intellectual Powers of Man, 4th. Ed., 461.
Mathematics, or the science of magnitudes, is that system which studies the quantitative relations between things; logic, or the science of concepts, is that system which studies the qualitative (categorical) relations between things.
In 'The Axioms of Logic', Tertium Organum: The Third Canon of Thought; a Key to the Enigmas of the World (1922), 246.
Mathematics, rightly viewed, possesses not only truth, but supreme beauty—a beauty cold and austere, like that of sculpture, without appeal to any part of our weaker nature, without the gorgeous trappings of painting or music, yet sublimely pure, and capable of a stern perfection such as only the greatest art can show.
Essay, 'The Study of Mathematics' (1902), collected in Philosophical Essays (1910), 73-74. Also collected in Mysticism and Logic: And Other Essays (1918), 60.
Mathematics, that giant pincers of scientific logic…
From Address to the Ohio Academy of Science, 'Biology and Mathematics', printed in Science (11 Aug 1905), New Series 22, No. 554, 162.
Mathematics, the priestess of definiteness and clearness.
In Werke [Kehrbach] (1890), Bd. 1, 171. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 14.
Mathematics, the science of the ideal, becomes the means of investigating, understanding and making known the world of the real. The complex is expressed in terms of the simple. From one point of view mathematics may be defined as the science of successive substitutions of simpler concepts for more complex.
In A Scrap-book of Elementary Mathematics (1908), 215.
Mathematics, too, is a language, and as concerns its structure and content it is the most perfect language which exists, superior to any vernacular; indeed, since it is understood by every people, mathematics may be called the language of languages. Through it, as it were, nature herself speaks; through it the Creator of the world has spoken, and through it the Preserver of the world continues to speak.
In Die Mathematik die Fackeltragerin einer neutn Zeit (1889), 5. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 194. From the original German, “Denn auch die Mathematik ist eine Sprache, und zwar nach Bau und Inhalt die vollkommenste Sprache, die es giebt, höher als jede Volkssprache; ja, weil alle Völker sie verstehen, kann sie die Sprache der Sprachen heißen. In ihr spricht sozusagen die Natur selbst, in ihr hat der Schöpfer der Welt geredet und in ihr redet noch immer ihr Erhalter.”
Mathematics, while giving no quick remuneration, like the art of stenography or the craft of bricklaying, does furnish the power for deliberate thought and accurate statement, and to speak the truth is one of the most social qualities a person can possess. Gossip, flattery, slander, deceit, all spring from a slovenly mind that has not been trained in the power of truthful statement, which is one of the highest utilities.
In Social Phases of Education in the School and the Home (1900), 30.
Mathematics: A science that cannot explain what happens to a man if his wife is his better half and he marries twice.
In Esar’s Comic Dictionary (1943, 4th ed. 1983), 373.
Mathematics… is the set of all possible self-consistent structures, and there are vastly more logical structures than physical principles.
In 'Conclusion', Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth Dimension (1995), 328.
May not Music be described as the Mathematic of sense, Mathematic as Music of the reason? the soul of each the same! Thus the musician feels Mathematic, the mathematician thinks Music, Music the dream, Mathematic the working life each to receive its consummation from the other when the human intelligence, elevated to its perfect type, shall shine forth glorified in some future Mozart-Dirichlet or Beethoven-Gauss a union already not indistinctly foreshadowed in the genius and labours of a Helmholtz!
In paper read 7 Apr 1864, printed in 'Algebraical Researches Containing a Disquisition On Newton’s Rule for the Discovery of Imaginary Roots', Philosophical Transactions of the Royal Society of London (1865), 154, 613, footnote. Also in Collected Mathematical Papers, Vol. 2, 419.
Men can construct a science with very few instruments, or with very plain instruments; but no one on earth could construct a science with unreliable instruments. A man might work out the whole of mathematics with a handful of pebbles, but not with a handful of clay which was always falling apart into new fragments, and falling together into new combinations. A man might measure heaven and earth with a reed, but not with a growing reed.
Heretics (1905), 146-7.
Men cannot be treated as units in operations of political arithmetic because they behave like the symbols for zero and the infinite, which dislocate all mathematical operations.
In The God That Failed: Six Studies in Communism (1965), 60.
Men of science belong to two different types—the logical and the intuitive. Science owes its progress to both forms of minds. Mathematics, although a purely logical structure, nevertheless makes use of intuition. Among the mathematicians there are intuitives and logicians, analysts and geometricians. Hermite and Weierstrass were intuitives. Riemann and Bertrand, logicians. The discoveries of intuition have always to be developed by logic.
In Man the Unknown (1935), 123.
Mere poets are sottish as mere drunkards are, who live in a continual mist, without seeing or judging anything clearly. A man should be learned in several sciences, and should have a reasonable, philosophical and in some measure a mathematical head, to be a complete and excellent poet.
In Notes and Observations on The Empress of Morocco (1674), 70.
Minus times Minus equals Plus:
The reason for this we need not discuss.
The reason for this we need not discuss.
The poet W.H. Auden recalled being asked to learn this mnemonic in school around 1919. As stated in 'Auden on Poetry: A Conversation with Stanley Kunitz', The Atlantic (1981), 218, 100.
Models so constructed, though of no practical value, serve a useful academic function. The oldest problem in economic education is how to exclude the incompetent. The requirement that there be an ability to master difficult models, including ones for which mathematical competence is required, is a highly useful screening device.
In Economics, Peace, and Laughter (1981), 40-41.
Modern bodybuilding is ritual, religion, sport, art, and science, awash in Western chemistry and mathematics. Defying nature, it surpasses it.
'Alice in Muscle Land,' Boston Globe (27 Jan 1991). Reprinted in Sex, Art, and American Culture (1992), 82.
Modern mathematics, that most astounding of intellectual creations, has projected the mind’s eye through infinite time and the mind’s hand into boundless space.
In 'What Knowledge is of Most Worth?', Presidential address to the National Education Association, Denver, Colorado (9 Jul 1895). In Educational Review (Sep 1895), 10, 108.
Moreover the perfection of mathematical beauty is such … that whatsoever is most beautiful and regular is also found to be most useful and excellent.
In 'Epilogue', On Growth and Form (1917), 779.
Most complex object in mathematics? The Mandelbrot Set, named after Benoit Mandelbrot, is represented by a unique pattern plotted from complex number coordinates. … A mathematical description of the shape’s outline would require an infinity of information and yet the pattern can be generated from a few lines of computer code. Used in the study of chaotic behavior, Mandelbrot’s work has found applications in fields such as fluid mechanics, economics and linguistics.
In Donald McFarlan (ed.), The Guinness Book of World Records: The 1991 Edition (1991), 187.
Most of the arts, as painting, sculpture, and music, have emotional appeal to the general public. This is because these arts can be experienced by some one or more of our senses. Such is not true of the art of mathematics; this art can be appreciated only by mathematicians, and to become a mathematician requires a long period of intensive training. The community of mathematicians is similar to an imaginary community of musical composers whose only satisfaction is obtained by the interchange among themselves of the musical scores they compose.
In Anton Z. Capri, Quips, Quotes and Quanta: An Anecdotal History of Physics (2007), 151. The author described Lanczos invited up on the platform at the Trieste Conference to celebrate Dirac’s 70th birthday, and gave an impromptu quote by Lanczos speaking about Pauli. The author followed that unrelated topic with another beginning, “Here is a comment by Lanczos…” followed by the subject quote above.
Most, if not all, of the great ideas of modern mathematics have had their origin in observation. Take, for instance, the arithmetical theory of forms, of which the foundation was laid in the diophantine theorems of Fermat, left without proof by their author, which resisted all efforts of the myriad-minded Euler to reduce to demonstration, and only yielded up their cause of being when turned over in the blow-pipe flame of Gauss’s transcendent genius; or the doctrine of double periodicity, which resulted from the observation of Jacobi of a purely analytical fact of transformation; or Legendre’s law of reciprocity; or Sturm’s theorem about the roots of equations, which, as he informed me with his own lips, stared him in the face in the midst of some mechanical investigations connected (if my memory serves me right) with the motion of compound pendulums; or Huyghen’s method of continued fractions, characterized by Lagrange as one of the principal discoveries of that great mathematician, and to which he appears to have been led by the construction of his Planetary Automaton; or the new algebra, speaking of which one of my predecessors (Mr. Spottiswoode) has said, not without just reason and authority, from this chair, “that it reaches out and indissolubly connects itself each year with fresh branches of mathematics, that the theory of equations has become almost new through it, algebraic geometry transfigured in its light, that the calculus of variations, molecular physics, and mechanics” (he might, if speaking at the present moment, go on to add the theory of elasticity and the development of the integral calculus) “have all felt its influence”.
In 'A Plea for the Mathematician', Nature, 1, 238 in Collected Mathematical Papers, Vol. 2, 655-56.
Mother of all the sciences, it [mathematics] is a builder of the imagination, a weaver of patterns of sheer thought, an intuitive dreamer, a poet.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
Mr. Bertrand Russell tells us that it can be shown that a mathematical web of some kind can be woven about any universe containing several objects. If this be so, then the fact that our universe lends itself to mathematical treatment is not a fact of any great philosophical significance.
In The Limitations of Science (1933), 229. [Notice that there are no quotation marks in the narrative statement by Sullivan. Therefore, Webmaster believes they are not necessarily, and likely not, the verbatim words from Russell. The first sentence is more likely to be Sullivan expressing in his own words an idea from Russell, and most likely the second sentence is Sullivan’s comment on that idea. (Be cautioned that quotation marks, perhaps spurious, have appeared when re-stated in later publications by other authors.) Webmaster has so far been unable to identify a primary source for these words in a text by Russell. If you know the primary source, please contact Webmaster.]
Music and poesy use to quicken you;
The mathematics and the metaphysics—
Fall to them as you find your stomach serves you.
No profit grows where is no pleasure ta’en:
In brief, sir, study what you most affect.
The mathematics and the metaphysics—
Fall to them as you find your stomach serves you.
No profit grows where is no pleasure ta’en:
In brief, sir, study what you most affect.
In Taming of the Shrew, Act 1, Scene 1.
My decision to leave applied mathematics for economics was in part tied to the widely-held popular belief in the 1960s that macroeconomics had made fundamental inroads into controlling business cycles and stopping dysfunctional unemployment and inflation.
Nobel Banquet Speech (1995). Collected in Tore Frängsmyr (ed.), Les Prix Nobel/Nobel Lectures/The Nobel Prizes.
My interest in the sciences started with mathematics in the very beginning, and later with chemistry in early high school and the proverbial home chemistry set.
In Tore Frängsmyr (ed.), Les Prix Nobel/The Nobel Prizes 1992.
My Math teacher called me average. How mean!
My two Jamaican cousins … were studying engineering. “That’s where the money is,” Mom advised. … I was to be an engineering major, despite my allergy to science and math. … Those who preceded me at CCNY include the polio vaccine discoverer, Dr. Jonas Salk … and eight Nobel Prize winners. … In class, I stumbled through math, fumbled through physics, and did reasonably well in, and even enjoyed, geology. All I ever looked forward to was ROTC.
Explaining his original reason for going to the City College of New York, where he shortly turned to his military career, in My American Journey (1996), 23-26. ROTC is the Reserve Officers’ Training Corps (ROTC) school-based program of the U.S. military. From there, the self-described “C-average student out of middling Morris High School” went on to become a four-star general.
My view, the skeptical one, holds that we may be as far away from an understanding of elementary particles as Newton's successors were from quantum mechanics. Like them, we have two tremendous tasks ahead of us. One is to study and explore the mathematics of the existing theories. The existing quantum field-theories may or may not be correct, but they certainly conceal mathematical depths which will take the genius of an Euler or a Hamilton to plumb. Our second task is to press on with the exploration of the wide range of physical phenomena of which the existing theories take no account. This means pressing on with experiments in the fashionable area of particle physics. Outstanding among the areas of physics which have been left out of recent theories of elementary particles are gravitation and cosmology
In Scientific American (Sep 1958). As cited in '50, 100 & 150 years ago', Scientific American (Sep 2008), 299, No. 3, 14.
Nature's economy shall be the base for our own, for it is immutable, but ours is secondary. An economist without knowledge of nature is therefore like a physicist without knowledge of mathematics.
'Tankar om grunden til oeconomien', 1740, 406. Trans. Lisbet Koerner, Linnaeus: Nature and Nation (1999), 103.
Nearly every subject has a shadow, or imitation. It would, I suppose, be quite possible to teach a deaf and dumb child to play the piano. When it played a wrong note, it would see the frown of its teacher, and try again. But it would obviously have no idea of what it was doing, or why anyone should devote hours to such an extraordinary exercise. It would have learnt an imitation of music. and it would fear the piano exactly as most students fear what is supposed to be mathematics.
In Mathematician's Delight (1943), 8.
Neither in the subjective nor in the objective world can we find a criterion for the reality of the number concept, because the first contains no such concept, and the second contains nothing that is free from the concept. How then can we arrive at a criterion? Not by evidence, for the dice of evidence are loaded. Not by logic, for logic has no existence independent of mathematics: it is only one phase of this multiplied necessity that we call mathematics.
How then shall mathematical concepts be judged? They shall not be judged. Mathematics is the supreme arbiter. From its decisions there is no appeal. We cannot change the rules of the game, we cannot ascertain whether the game is fair. We can only study the player at his game; not, however, with the detached attitude of a bystander, for we are watching our own minds at play.
How then shall mathematical concepts be judged? They shall not be judged. Mathematics is the supreme arbiter. From its decisions there is no appeal. We cannot change the rules of the game, we cannot ascertain whether the game is fair. We can only study the player at his game; not, however, with the detached attitude of a bystander, for we are watching our own minds at play.
In Number: The Language of Science; a Critical Survey Written for the Cultured Non-Mathematician (1937), 244-245.
Never leave an unsolved difficulty behind. I mean, don’t go any further in that book till the difficulty is conquered. In this point, Mathematics differs entirely from most other subjects. Suppose you are reading an Italian book, and come to a hopelessly obscure sentence—don’t waste too much time on it, skip it, and go on; you will do very well without it. But if you skip a mathematical difficulty, it is sure to crop up again: you will find some other proof depending on it, and you will only get deeper and deeper into the mud.
From letter to Edith Rix with hints for studying (about Mar 1885), in Stuart Dodgson Collingwood, The Life and Letters of Lewis Carroll (1898), 241.
Newton's health, and confusion to mathematics.
28 Dec 1817, in Tom Taylor (ed.), The Autobiography and Memoirs of Benjamin Robert Haydon (1786-1846), intro. Aldous Huxley (1926), Vol. 1, 269.
No discovery of mine has made, or is likely to make, directly or indirectly, for good or ill, the least difference to the amenity of the world.
In A Mathematician’s Apology (1941, reprint with Foreward by C.P. Snow 1992), 150.
No irrational exaggeration of the claims of Mathematics can ever deprive that part of philosophy of the property of being the natural basis of all logical education, through its simplicity, abstractness, generality, and freedom from disturbance by human passion. There, and there alone, we find in full development the art of reasoning, all the resources of which, from the most spontaneous to the most sublime, are continually applied with far more variety and fruitfulness than elsewhere;… The more abstract portion of mathematics may in fact be regarded as an immense repository of logical resources, ready for use in scientific deduction and co-ordination.
In Auguste Comte and Harriet Martineau (trans.), Positive Philosophy (1854), Vol. 2, 528-529.
No man who has not a decently skeptical mind can claim to be civilized. Euclid taught me that without assumptions there is no proof. Therefore, in any argument, examine the assumptions. Then, in the alleged proof, be alert for inexplicit assumptions. Euclid’s notorious oversights drove this lesson home. Thanks to him, I am (I hope!) immune to all propaganda, including that of mathematics itself.
In 'What Mathematics Has Meant to Me', Mathematics Magazine (Jan-Feb 1951), 24, 161.
No mathematician should ever allow him to forget that mathematics, more than any other art or science, is a young man's game. … Galois died at twenty-one, Abel at twenty-seven, Ramanujan at thirty-three, Riemann at forty. There have been men who have done great work later; … [but] I do not know of a single instance of a major mathematical advance initiated by a man past fifty. … A mathematician may still be competent enough at sixty, but it is useless to expect him to have original ideas.
In A Mathematician's Apology (1941, reprint with Foreward by C.P. Snow 1992), 70-71.
No mathematician should ever allow himself to forget that mathematics, more than any other art or science, is a young man's game.
A Mathematician's Apology (1940), 10.
No matter how correct a mathematical theorem may appear to be, one ought never to be satisfied that there was not something imperfect about it until it also gives the impression of being beautiful.
As quoted in Desmond MacHale. Comic Sections (1993), 107, without citation. Please contact the Webmaster if you know the primary source.
No old Men (excepting Dr. Wallis) love Mathematicks.
Comment made by Newton to William Whiston. Quoted in Richard Westfall, Never at Rest: A Biography of Isaac Newton (1980), 139.
No one really understood music unless he was a scientist, her father had declared, and not just a scientist, either, oh, no, only the real ones, the theoreticians, whose language mathematics. She had not understood mathematics until he had explained to her that it was the symbolic language of relationships. “And relationships,” he had told her, “contained the essential meaning of life.”
In The Goddess Abides, (1972), 20.
No one shall expel us from the paradise which Cantor has created for us.
Expressing the importance of Cantor's set theory in the development of mathematics.
Expressing the importance of Cantor's set theory in the development of mathematics.
In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 33.
No other part of science has contributed as much to the liberation of the human spirit as the Second Law of Thermodynamics. Yet, at the same time, few other parts of science are held to be so recondite. Mention of the Second Law raises visions of lumbering steam engines, intricate mathematics, and infinitely incomprehensible entropy. Not many would pass C.P. Snow’s test of general literacy, in which not knowing the Second Law is equivalent to not having read a work of Shakespeare.
In The Second Law (1984), Preface, vii.
No other subject has such clear-cut or unanimously accepted standards, and the men who are remembered are almost always the men who merit it. Mathematical fame, if you have the cash to pay for it, is one of the soundest and steadiest of investments.
In A Mathematician's Apology (1940, 2012), 82.
No other theory known to science [other than superstring theory] uses such powerful mathematics at such a fundamental level. …because any unified field theory first must absorb the Riemannian geometry of Einstein’s theory and the Lie groups coming from quantum field theory… The new mathematics, which is responsible for the merger of these two theories, is topology, and it is responsible for accomplishing the seemingly impossible task of abolishing the infinities of a quantum theory of gravity.
In 'Conclusion', Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth Dimension (1995), 326.
No part of Mathematics suffers more from the triviality of its initial presentation to beginners than the great subject of series. Two minor examples of series, namely arithmetic and geometric series, are considered; these examples are important because they are the simplest examples of an important general theory. But the general ideas are never disclosed; and thus the examples, which exemplify nothing, are reduced to silly trivialities.
In An Introduction to Mathematics (1911), 194.
No school subject so readily furnishes tasks whose purpose can be made so clear, so immediate and so appealing to the sober second-thought of the immature learner as the right sort of elementary school mathematics.
In Arithmetic in Public Education (1909), 8. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 50.
No shreds of dignity encumber
The undistinguished Random Number
He has, so sad a lot is his,
No reason to be what he is.
The undistinguished Random Number
He has, so sad a lot is his,
No reason to be what he is.
In Kenneth Ewart Boulding and Richard P. Beilock (Ed.), Illustrating Economics: Beasts, Ballads and Aphorisms (1980, 2009), 153.
Nobody before the Pythagoreans had thought that mathematical relations held the secret of the universe. Twenty-five centuries later, Europe is still blessed and cursed with their heritage. To non-European civilizations, the idea that numbers are the key to both wisdom and power, seems never to have occurred.
In The Sleepwalkers: A History of Man's Changing Vision of the Universe (1959), Preface, 40.
Non-standard analysis frequently simplifies substantially the proofs, not only of elementary theorems, but also of deep results. This is true, e.g., also for the proof of the existence of invariant subspaces for compact operators, disregarding the improvement of the result; and it is true in an even higher degree in other cases. This state of affairs should prevent a rather common misinterpretation of non-standard analysis, namely the idea that it is some kind of extravagance or fad of mathematical logicians. Nothing could be farther from the truth. Rather, there are good reasons to believe that non-standard analysis, in some version or other, will be the analysis of the future.
In 'Remark on Non-standard Analysis' (1974), in S. Feferman (ed.), Kurt Gödel Collected Works: Publications 1938-1974 (1990), Vol. 2, 311.
Nonmathematical people sometimes ask me, “You know math, huh? Tell me something I’ve always wondered, What is infinity divided by infinity?” I can only reply, “The words you just uttered do not make sense. That was not a mathematical sentence. You spoke of ‘infinity’ as if it were a number. It’s not. You may as well ask, 'What is truth divided by beauty?’ I have no clue. I only know how to divide numbers. ‘Infinity,’ ‘truth,’ ‘beauty’—those are not numbers.”
From Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics (2003), 16.
Nor do I know any study which can compete with mathematics in general in furnishing matter for severe and continued thought. Metaphysical problems may be even more difficult; but then they are far less definite, and, as they rarely lead to any precise conclusion, we miss the power of checking our own operations, and of discovering whether we are thinking and reasoning or merely fancying and dreaming.
In Conflict of Studies (1873), 13.
Not everything is an idea. Otherwise psychology would contain all the sciences within it or at least it would be the highest judge over all the sciences. Otherwise psychology would rule over logic and mathematics. But nothing would be a greater misunderstanding of mathematics than its subordination to psychology.
In Elmer Daniel Klemke, Essays on Frege (1968), 531.
Not seldom did he [Sir William Thomson], in his writings, set down some mathematical statement with the prefacing remark “it is obvious that” to the perplexity of mathematical readers, to whom the statement was anything but obvious from such mathematics as preceded it on the page. To him it was obvious for physical reasons that might not suggest themselves at all to the mathematician, however competent.
As given in Life of Lord Kelvin (1910), Vol. 2, 1136. [Note: William Thomson, later became Lord Kelvin —Webmaster]
Nothing afflicted Marcellus so much as the death of Archimedes, who was then, as fate would have it, intent upon working out some problem by a diagram, and having fixed his mind alike and his eyes upon the subject of his speculation, he never noticed the incursion of the Romans, nor that the city was taken. In this transport of study and contemplation, a soldier, unexpectedly coming up to him, commanded him to follow to Marcellus, which he declined to do before he had worked out his problem to a demonstration; the soldier, enraged, drew his sword and ran him through. Others write, that a Roman soldier, running upon him with a drawn sword, offered to kill him; and that Archimedes, looking back, earnestly besought him to hold his hand a little while, that he might not leave what he was at work upon inconclusive and imperfect; but the soldier, nothing moved by his entreaty, instantly killed him. Others again relate, that as Archimedes was carrying to Marcellus mathematical instruments, dials, spheres, and angles, by which the magnitude of the sun might be measured to the sight, some soldiers seeing him, and thinking that he carried gold in a vessel, slew him. Certain it is, that his death was very afflicting to Marcellus; and that Marcellus ever after regarded him that killed him as a murderer; and that he sought for his kindred and honoured them with signal favours.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
Nothing can be more fatal to progress than a too confident reliance upon mathematical symbols; for the student is only too apt to take the easier course, and consider the formula and not the fact as the physical reality.
In William Thomson and Peter Guthrie Tait, Treatise on Natural Philosophy (1867), Vol. 1, viii.
Nothing can he learned as to the physical world save by observation and experiment, or by mathematical deductions from data so obtained.
From Heat (1884), 1.
Nothing enrages me more than when people criticize my criticism of school by telling me that schools are not just places to learn math and spelling, they are places where children learn a vaguely defined thing called socialization. I know. I think schools generally do an effective and terribly damaging job of teaching children to be infantile, dependent, intellectually dishonest, passive and disrespectful to their own developmental capacities. (1981)
Quoted in K.P. Yaday and Malti Sundram, Encyclopaedia Of Child And Primary Education Development, Vol. 2, 99.
Nothing has afforded me so convincing a proof of the unity of the Deity as these purely mental conceptions of numerical and mathematical science which have been by slow degrees vouchsafed to man, and are still granted in these latter times by the Differential Calculus, now superseded by the Higher Algebra, all of which must have existed in that sublimely omniscient Mind from eternity.
Martha Somerville (ed.) Personal Recollections, from Early Life to Old Age, of Mary Somerville (1874), 140-141.
Nothing is less applicable to life than mathematical reasoning. A proposition in mathematics is decidedly false or true. Everywhere else the true is mingled with the false.
Quoted, without citation, in David Eugene Smith, The Teaching of Elementary Mathematics (1904), 170.
Now do you not see that the eye embraces the beauty of the whole world? It counsels and corrects all the arts of mankind... it is the prince of mathematics, and the sciences founded on it are absolutely certain. It has measured the distances and sizes of the stars it has discovered the elements and their location... it has given birth to architecture and to perspective and to the divine art of painting.
…...
Now I feel as if I should succeed in doing something in mathematics, although I cannot see why it is so very important… The knowledge doesn’t make life any sweeter or happier, does it?
In Letter (29 May 1898), at age almost 18, to Mrs. Lawrence Hutton, excerpted in The Story of My Life: With her Letters (1887-1901) (1903, 1921), 242.
Now this establishment of correspondence between two aggregates and investigation of the propositions that are carried over by the correspondence may be called the central idea of modern mathematics.
In 'Philosophy of the Pure Sciences', Lectures and Essays (1901), Vol. 1, 402.
Now this supreme wisdom, united to goodness that is no less infinite, cannot but have chosen the best. For as a lesser evil is a kind of good, even so a lesser good is a kind of evil if it stands in the way of a greater good; and the would be something to correct in the actions of God if it were possible to the better. As in mathematics, when there is no maximum nor minimum, in short nothing distinguished, everything is done equally, or when that is not nothing at all is done: so it may be said likewise in respect of perfect wisdom, which is no less orderly than mathematics, that if there were not the best (optimum) among all possible worlds, God would not have produced any.
Theodicy: Essays on the Goodness of God and Freedom of Man and the Origin of Evil (1710), 128.
Now, in the development of our knowledge of the workings of Nature out of the tremendously complex assemblage of phenomena presented to the scientific inquirer, mathematics plays in some respects a very limited, in others a very important part. As regards the limitations, it is merely necessary to refer to the sciences connected with living matter, and to the ologies generally, to see that the facts and their connections are too indistinctly known to render mathematical analysis practicable, to say nothing of the complexity.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
Number, place, and combination … the three intersecting but distinct spheres of thought to which all mathematical ideas admit of being referred.
In Philosophical Magazine (1844), 84, 285; Collected Mathematical Papers, Vol. 1, 91.
Numbers written on restaurant checks [bills] within the confines of restaurants do not follow the same mathematical laws as numbers written on any other pieces of paper in any other parts of the Universe.
This single statement took the scientific world by storm. It completely revolutionized it. So many mathematical conferences got held in such good restaurants that many of the finest minds of a generation died of obesity and heart failure and the science of math was put back by years.
This single statement took the scientific world by storm. It completely revolutionized it. So many mathematical conferences got held in such good restaurants that many of the finest minds of a generation died of obesity and heart failure and the science of math was put back by years.
Life, the Universe and Everything (1982, 1995), 49.
Objections … inspired Kronecker and others to attack Weierstrass’ “sequential” definition of irrationals. Nevertheless, right or wrong, Weierstrass and his school made the theory work. The most useful results they obtained have not yet been questioned, at least on the ground of their great utility in mathematical analysis and its implications, by any competent judge in his right mind. This does not mean that objections cannot be well taken: it merely calls attention to the fact that in mathematics, as in everything else, this earth is not yet to be confused with the Kingdom of Heaven, that perfection is a chimaera, and that, in the words of Crelle, we can only hope for closer and closer approximations to mathematical truth—whatever that may be, if anything—precisely as in the Weierstrassian theory of convergent sequences of rationals defining irrationals.
In Men of Mathematics (1937), 431-432.
Of all regions of the earth none invites speculation more than that which lies beneath our feet, and in none is speculation more dangerous; yet, apart from speculation, it is little that we can say regarding the constitution of the interior of the earth. We know, with sufficient accuracy for most purposes, its size and shape: we know that its mean density is about 5½ times that of water, that the density must increase towards the centre, and that the temperature must be high, but beyond these facts little can be said to be known. Many theories of the earth have been propounded at different times: the central substance of the earth has been supposed to be fiery, fluid, solid, and gaseous in turn, till geologists have turned in despair from the subject, and become inclined to confine their attention to the outermost crust of the earth, leaving its centre as a playground for mathematicians.
'The Constitution of the Interior of the Earth, as Revealed by Earthquakes', Quarterly Journal of the Geological Society (1906), 62, 456.
Of all the intellectual faculties, judgment is the last to arrive at maturity. The child should give its attention either to subjects where no error is possible at all, such as mathematics, or to those in which there is no particular danger in making a mistake, such as languages, natural science, history, and so on.
In Arthur Schopenhauer and T. Bailey (ed., trans.) Essays of Arthur Schopenhauer (1902), 67.
Of all the sciences that pertain to reason, Metaphysics and Geometry are those in which imagination plays the greatest part. … Imagination acts no less in a geometer who creates than in a poet who invents. It is true that they operate differently on their object. The first shears it down and analyzes it, the second puts it together and embellishes it. … Of all the great men of antiquity, Archimedes is perhaps the one who most deserves to be placed beside Homer.
From the original French: “La Métaphysique & la Géométrie sont de toutes les Sciences qui appartiennent à la raison, celles où l’imagination à le plus de part. … L’imagination dans un Géometre qui crée, n’agit pas moins que dans un Poëte qui invente. Il est vrai qu’ils operent différemment sur leur objet; le premier le dépouille & l’analyse, le second le compose & l’embellit. … De tous les grands hommes de l’antiquité, Archimede est peut-être celui qui mérite le plus d’être placé à côté d’Homere.” In Discours Preliminaire de L'Encyclopedie (1751), xvi. As translated by Richard N. Schwab and Walter E. Rex, Preliminary Discourse to the Encyclopedia of Diderot (1963, 1995), xxxvi. A footnote states “Note that ‘geometer’ in d’Alembert’s definition is a term that includes all mathematicians and is not strictly limited to practitioners of geometry alone.” Also seen in a variant extract and translation: “Thus metaphysics and mathematics are, among all the sciences that belong to reason, those in which imagination has the greatest role. I beg pardon of those delicate spirits who are detractors of mathematics for saying this …. The imagination in a mathematician who creates makes no less difference than in a poet who invents…. Of all the great men of antiquity, Archimedes may be the one who most deserves to be placed beside Homer.” This latter translation may be from The Plan of the French Encyclopædia: Or Universal Dictionary of Arts, Sciences, Trades and Manufactures (1751). Webmaster has not yet been able to check for a verified citation for this translation. Can you help?
Of my own age I may say … I was x years old in the year x × x. … I dare say Professor De Morgan, or some of your mathematical correspondents, will be able to find my age.
In Notes and Queries: Volume Twelve: July—December 1855 (4 Aug 1855), Vol. 12 No. 301, 94. The reply is signed as by M. However De Morgan is identified as author in C.O. Tuckey, 'Noughts and Crosses', The Mathematical Gazette (Dec 1929), 14, No. 204, 577, which points out: M “contributed other replies that were certainly from the pen of De Morgan.” Furthermore, De Morgan, mathematician, born in 1806, was 43 in the year 1849 (43 × 43, which is the only reasonable solution for an adult writing in 1855 since 42² = 1764).
Of these austerer virtues the love of truth is the chief, and in mathematics, more than elsewhere, the love of truth may find encouragement for waning faith. Every great study is not only an end in itself, but also a means of creating and sustaining a lofty habit of mind; and this purpose should be kept always in view throughout the teaching and learning of mathematics.
Essay, 'The Study of Mathematics' (1902), collected in Philosophical Essays (1910), 73-74. Also collected in Mysticism and Logic: And Other Essays (1919), 73.
On all levels primary, and secondary and undergraduate - mathematics is taught as an isolated subject with few, if any, ties to the real world. To students, mathematics appears to deal almost entirely with things whlch are of no concern at all to man.
In editorial in Focus, a Journal of the Mathematical Association of America (1986), quoted in obituary by Eric Pace, New York Times (11 Jun 1992).
On foundations we believe in the reality of mathematics, but of course, when philosophers attack us with their paradoxes, we rush to hide behind formalism and say 'mathematics is just a combination of meaningless symbols,'... Finally we are left in peace to go back to our mathematics and do it as we have always done, with the feeling each mathematician has that he is working with something real. The sensation is probably an illusion, but it is very convenient.
'The Work of Nicholas Bourbaki'American Mathematical Monthly (1970), 77, 134. In Carl C. Gaither, Alma E. Cavazos-Gaither, Mathematically Speaking: a Dictionary of Quotations (), 194.
One can argue that mathematics is a human activity deeply rooted in reality, and permanently returning to reality. From counting on one’s fingers to moon-landing to Google, we are doing mathematics in order to understand, create, and handle things, … Mathematicians are thus more or less responsible actors of human history, like Archimedes helping to defend Syracuse (and to save a local tyrant), Alan Turing cryptanalyzing Marshal Rommel’s intercepted military dispatches to Berlin, or John von Neumann suggesting high altitude detonation as an efficient tactic of bombing.
In 'Mathematical Knowledge: Internal, Social and Cultural Aspects', Mathematics As Metaphor: Selected Essays (2007), 3.
One cannot escape the feeling that these mathematical formulas have an independent existence and an intelligence of their own, that they are wiser that we are, wiser even than their discoverers, that we get more out of them than was originally put into them.
Quoted, without citation, in Men of Mathematics (1937), Vol. 2, 16.
One doesn’t really understand what mathematics is until at least halfway through college when one takes abstract math courses and learns about proofs.
In 'A Personal Profile of Karen K. Uhlenbeck', collected in Susan Ambrose et al., Journeys of Women in Science and Engineering, No Universal Constants (1999).
One may be a mathematician of the first rank without being able to compute. It is possible to be a great computer without having the slightest idea of mathematics.
In Schriften, Zweiter Teil (1901), 223.
One merit of mathematics few will deny: it says more in fewer words than any other science.
In 'The Poetry of Mathematics', The Mathematics Teacher (May 1926), 19, No. 5, 293. This is a paraphrase from Voltaire: “One merit of poetry few will deny; it says more and in fewer words than prose.”
One might describe the mathematical quality in Nature by saying that the universe is so constituted that mathematics is a useful tool in its description. However, recent advances in physical science show that this statement of the case is too trivial. The connection between mathematics and the description of the universe goes far deeper than this, and one can get an appreciation of it only from a thorough examination of the various facts that make it up.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 122.
One of the attractions of mathematics … that had a great impact on my choosing a career—I wanted a career where I didn’t have to work with other people … to work in an area where I compete only with myself and didn’t have to deal with the negative aspects of competition.
In 'A Personal Profile of Karen K. Uhlenbeck', collected in Susan Ambrose et al., Journeys of Women in Science and Engineering, No Universal Constants (1999).
One of the big misapprehensions about mathematics that we perpetrate in our classrooms is that the teacher always seems to know the answer to any problem that is discussed. This gives students the idea that there is a book somewhere with all the right answers to all of the interesting questions, and that teachers know those answers. And if one could get hold of the book, one would have everything settled. That’s so unlike the true nature of mathematics.
As quoted in L.A. Steen and D.J. Albers (eds.), Teaching Teachers, Teaching Students (1981), 89.
One of the chiefest triumphs of modern mathematics consists in having discovered what mathematics really is.
International Monthly (1901), 4, 84. In Robert Édoward Moritz, Memorabilia Mathematica (1914), 109.
One of the endlessly alluring aspects of mathematics is that its thorniest paradoxes have a way of blooming into beautiful theories.
In 'Number', Scientific American (Sep 1964), 211, No. 3, 55.
One of the first and foremost duties of the teacher is not to give his students the impression that mathematical problems have little connection with each other, and no connection at all with anything else. We have a natural opportunity to investigate the connections of a problem when looking back at its solution.
In How to Solve It: A New Aspect of Mathematical Method (2004), 15.
One of the most conspicuous and distinctive features of mathematical thought in the nineteenth century is its critical spirit. Beginning with the calculus, it soon permeates all analysis, and toward the close of the century it overhauls and recasts the foundations of geometry and aspires to further conquests in mechanics and in the immense domains of mathematical physics. … A searching examination of the foundations of arithmetic and the calculus has brought to light the insufficiency of much of the reasoning formerly considered as conclusive.
In History of Mathematics in the Nineteenth Century', Congress of Arts and Sciences (1906), Vol. 1, 482. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 113-114.
One rarely hears of the mathematical recitation as a preparation for public speaking. Yet mathematics shares with these studies [foreign languages, drawing and natural science] their advantages, and has another in a higher degree than either of them.
Most readers will agree that a prime requisite for healthful experience in public speaking is that the attention of the speaker and hearers alike be drawn wholly away from the speaker and concentrated upon the thought. In perhaps no other classroom is this so easy as in the mathematical, where the close reasoning, the rigorous demonstration, the tracing of necessary conclusions from given hypotheses, commands and secures the entire mental power of the student who is explaining, and of his classmates. In what other circumstances do students feel so instinctively that manner counts for so little and mind for so much? In what other circumstances, therefore, is a simple, unaffected, easy, graceful manner so naturally and so healthfully cultivated? Mannerisms that are mere affectation or the result of bad literary habit recede to the background and finally disappear, while those peculiarities that are the expression of personality and are inseparable from its activity continually develop, where the student frequently presents, to an audience of his intellectual peers, a connected train of reasoning. …
One would almost wish that our institutions of the science and art of public speaking would put over their doors the motto that Plato had over the entrance to his school of philosophy: “Let no one who is unacquainted with geometry enter here.”
Most readers will agree that a prime requisite for healthful experience in public speaking is that the attention of the speaker and hearers alike be drawn wholly away from the speaker and concentrated upon the thought. In perhaps no other classroom is this so easy as in the mathematical, where the close reasoning, the rigorous demonstration, the tracing of necessary conclusions from given hypotheses, commands and secures the entire mental power of the student who is explaining, and of his classmates. In what other circumstances do students feel so instinctively that manner counts for so little and mind for so much? In what other circumstances, therefore, is a simple, unaffected, easy, graceful manner so naturally and so healthfully cultivated? Mannerisms that are mere affectation or the result of bad literary habit recede to the background and finally disappear, while those peculiarities that are the expression of personality and are inseparable from its activity continually develop, where the student frequently presents, to an audience of his intellectual peers, a connected train of reasoning. …
One would almost wish that our institutions of the science and art of public speaking would put over their doors the motto that Plato had over the entrance to his school of philosophy: “Let no one who is unacquainted with geometry enter here.”
In A Scrap-book of Elementary Mathematics: Notes, Recreations, Essays (1908), 210-211.
One reason why mathematics enjoys special esteem, above all other sciences, is that its laws are absolutely certain and indisputable, while those of other sciences are to some extent debatable and in constant danger of being overthrown by newly discovered facts.
In Albert Einstein, translated by G.B. Jeffery and W. Perrett, 'Geometry and Experience',Sidelights on Relativity (1922), 27.
One should first discourage people from doing mathematics; there is no need for too many mathematicians. But, if after that, they still insist on doing mathematics, then one should indeed encourage them, and help them.
Reply to question, “How could we encourage young people to take up mathematics, especially in the schools?” C.T. Chong and Y.K. Leong, 'An Interview With Jean-Pierre Serre', Singapore Mathematical Society Mathematical Medley (1985), 13, No. 1, 11-19.
One striking peculiarity of mathematics is its unlimited power of evolving examples and problems. A student may read a book of Euclid, or a few chapters of Algebra, and within that limited range of knowledge it is possible to set him exercises as real and as interesting as the propositions themselves which he has studied; deductions which might have pleased the Greek geometers, and algebraic propositions which Pascal and Fermat would not have disdained to investigate.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 82.
One would have to have completely forgotten the history of science so as not to remember that the desire to know nature has had the most constant and the happiest influence on the development of mathematics.
In Henri Poincaré and George Bruce Halsted (trans.), The Value of Science: Essential Writings of Henri Poincare (1907), 79.
Only dead mathematics can be taught where the attitude of competition prevails: living mathematics must always be a communal possession.
In Mary Everest Boole: Collected Works (1931), Vol. 3, 1008.
Only go on working so long as the brain is quite clear. The moment you feel the ideas getting confused leave off and rest, or your penalty will be that you will never learn Mathematics at all!
From letter to Edith Rix with hints for studying (about Mar 1885), in Stuart Dodgson Collingwood, The Life and Letters of Lewis Carroll (1898), 241.
Only mathematics and mathematical logic can say as little as the physicist means to say. (1931)
In The Scientific Outlook (1931, 2009), 57.
Only the mathematically minded can really teach mathematics; and it takes a great deal of mathematics to teach any mathematics well.
In A Preface to Mathematics (1938), 75.
Only the privileged few are called to enjoy it [mathematics] fully, it is true; but is it not the same with all the noblest arts?
From 'The Relation of Analysis and Mathematical Physics', Bulletin American Mathematical Society (1899), 4 (1899), 248. As cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 181.
Ordinary language is totally unsuited for expressing what physics really asserts, since the words of everyday life are not sufficiently abstract. Only mathematics and mathematical logic can say as little as the physicist means to say.
In The Scientific Outlook (1931, 2001), 61.
Our experience up to date justifies us in feeling sure that in Nature is actualized the ideal of mathematical simplicity. It is my conviction that pure mathematical construction enables us to discover the concepts and the laws connecting them, which gives us the key to understanding nature… In a certain sense, therefore, I hold it true that pure thought can grasp reality, as the ancients dreamed.
In Herbert Spencer Lecture at Oxford (10 Jun 1933), 'On the Methods of Theoretical Physics'. Printed in Discovery (Jul 1933), 14, 227. Also quoted in Stefano Zambelli and Donald A. R. George, Nonlinearity, Complexity and Randomness in Economics (2012).
Our federal income tax law defines the tax y to be paid in terms of the income x; it does so in a clumsy enough way by pasting several linear functions together, each valid in another interval or bracket of income. An archaeologist who, five thousand years from now, shall unearth some of our income tax returns together with relics of engineering works and mathematical books, will probably date them a couple of centuries earlier, certainly before Galileo and Vieta.
From Address (1940), given at the Bicentennial Conference at the University of Pennsylvania, 'The Mathematical Way of Thinking'. Collected in Hermann Weyl and Peter Pesic (ed.), Levels of Infinity: Selected Writings on Mathematics and Philosophy (2012), 67.
Our knowledge of the external world must always consist of numbers, and our picture of the universe—the synthesis of our knowledge—must necessarily be mathematical in form. All the concrete details of the picture, the apples, the pears and bananas, the ether and atoms and electrons, are mere clothing that we ourselves drape over our mathematical symbols— they do not belong to Nature, but to the parables by which we try to make Nature comprehensible. It was, I think, Kronecker who said that in arithmetic God made the integers and man made the rest; in the same spirit, we may add that in physics God made the mathematics and man made the rest.
From Address (1934) to the British Association for the Advancement of Science, Aberdeen, 'The New World—Picture of Modern Physics'. Printed in Nature (Sep 1934) 134, No. 3384, 356. As quoted and cited in Wilbur Marshall Urban, Language and Reality: The Philosophy of Language and the Principles of Symbolism (2004), Vol. 15, 542.
Our present work sets forth mathematical principles of philosophy. For the basic problem of philosophy seems to be to discover the forces of nature from the phenomena of motions and then to demonstrate the other phenomena from these forces. It is to these ends that the general propositions in books 1 and 2 are directed, while in book 3 our explanation of the system of the world illustrates these propositions.
The Principia: Mathematical Principles of Natural Philosophy (1687), 3rd edition (1726), trans. I. Bernard Cohen and Anne Whitman (1999), Preface to the first edition, 382.
Our remote ancestors tried to interpret nature in terms of anthropomorphic concepts of their own creation and failed. The efforts of our nearer ancestors to interpret nature on engineering lines proved equally inadequate. Nature refused to accommodate herself to either of these man-made moulds. On the other hand, our efforts to interpret nature in terms of the concepts of pure mathematics have, so far, proved brilliantly successful. It would now seem to be beyond dispute that in some way nature is more closely allied to the concepts of pure mathematics than to those of biology or of engineering, and…the mathematical interpretation…fits objective nature incomparably better than the two previously tried.
In The Mysterious Universe (1930, Rev. Ed. 1942), 158.
Our school curricula, by stripping mathematics of its cultural content and leaving a bare skeleton of technicalities, have repelled many a fine mind.
In Number, the Language of Science: A Critical Survey Written for the Cultured Non-mathematician (1930), vii.

Our science, in contrast with others, is not founded on a single period of human history, but has accompanied the development of culture through all its stages. Mathematics is as much interwoven with Greek culture as with the most modern problems in Engineering. She not only lends a hand to the progressive natural sciences but participates at the same time in the abstract investigations of logicians and philosophers.
In Klein und Riecke: Ueber angewandte Mathematik und Physik (1900), 228.
Out of the interaction of form and content in mathematics grows an acquaintance with methods which enable the student to produce independently within certain though moderate limits, and to extend his knowledge through his own reflection. The deepening of the consciousness of the intellectual powers connected with this kind of activity, and the gradual awakening of the feeling of intellectual self-reliance may well be considered as the most beautiful and highest result of mathematical training.
In 'Ueber Wert und angeblichen Unwert der Mathematik', Jahresbericht der Deutschen Mathematiker Vereinigung (1904), 374.
Perhaps I can best describe my experience of doing mathematics in terms of a journey through a dark unexplored mansion. You enter the first room of the mansion and it’s completely dark. You stumble around bumping into the furniture, but gradually you learn where each piece of furniture is. Finally, after six months or so, you find the light switch, you turn it on, and suddenly it’s all illuminated. You can see exactly where you were. Then you move into the next room and spend another six months in the dark. So each of these breakthroughs, while sometimes they’re momentary, sometimes over a period of a day or two, they are the culmination of—and couldn’t exist without—the many months of stumbling around in the dark that proceed them.
Quoted in interview for website for PBS TV Nova program, 'The Proof'.
Perhaps I may without immodesty lay claim to the appellation of Mathematical Adam, as I believe that I have given more names (passed into general circulation) of the creatures of the mathematical reason than all the other mathematicians of the age combined.
In Nature (1887-1888), 87, 162. As quoted and cited in As cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 178
Perhaps the best reason for regarding mathematics as an art is not so much that it affords an outlet for creative activity as that it provides spiritual values. It puts man in touch with the highest aspirations and lofiest goals. It offers intellectual delight and the exultation of resolving the mysteries of the universe.
Mathematics: a Cultural Approach (1962), 671. Quoted in H. E. Hunter, The Divine Proportion (1970), 6.
Perhaps the greatest paradox of all is that there are paradoxes in mathematics.
With co-author James R. Newman, in Mathematics and the Imagination (1940), 193.
Perhaps the least inadequate description of the general scope of modern Pure Mathematics—I will not call it a definition—would be to say that it deals with form, in a very general sense of the term; this would include algebraic form, functional relationship, the relations of order in any ordered set of entities such as numbers, and the analysis of the peculiarities of form of groups of operations.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 287.
Perhaps the most surprising thing about mathematics is that it is so surprising. The rules which we make up at the beginning seem ordinary and inevitable, but it is impossible to foresee their consequences. These have only been found out by long study, extending over many centuries. Much of our knowledge is due to a comparatively few great mathematicians such as Newton, Euler, Gauss, or Riemann; few careers can have been more satisfying than theirs. They have contributed something to human thought even more lasting than great literature, since it is independent of language.
Quoted in a space filler, without citation, in The Pentagon: A Mathematics Magazine for Students (Fall 1951), 11, No. 1, 12. Primary source needed (can you help).
Perhaps the strongest bond of sympathy between mathematics and poetry, however, is the endless invention of each. Dr. Johnson remarked, “The essence of poetry is invention; such invention as, by producing something unexpected, surprises and delights”; but he might have said the same of mathematics.
In 'The Poetry of Mathematics', The Mathematics Teacher (May 1926), 19, No. 5, 295.
Perhaps we see equations as simple because they are easily expressed in terms of mathematical notation already invented at an earlier stage of development of the science, and thus what appears to us as elegance of description really reflects the interconnectedness of Nature's laws at different levels.
Nobel Banquet Speech (10 Dec 1969), in Wilhelm Odelberg (ed.),Les Prix Nobel en 1969 (1970).
Persons, who have a decided mathematical talent, constitute, as it were, a favored class. They bear the same relation to the rest of mankind that those who are academically trained bear to those who are not.
In Ueber die Anlage zur Mathematik (1900), 4.
Perspective is a most subtle discovery in mathematical studies, for by means of lines it causes to appear distant that which is near, and large that which is small.
Attributed.
Philosophy [the universe] is written in that great book which ever lies before our eyes ... We cannot understand it if we do not first learn the language and grasp the symbols in which it is written. The book is written in the mathematical language ... without whose help it is humanly impossible to comprehend a single word of it, and without which one wanders in vain through a dark labyrinth.
…...
Philosophy is a game with objectives and no rules. Mathematics is a game with rules and no objectives.
In Wieslaw Krawcewicz, Bindhyachal Rai, Calculus with Maple Labs (2003), 328. In this book, and also in Julian Havil, Nonplussed!: Mathematical Proof of Implausible Ideas? (2007), 68, the quote is attributed to Ian Ellis, but most sources vite it as Anonymous.
Philosophy is written in this grand book, the universe, which stands continually open to our gaze. But the book cannot be understood unless one first learns to comprehend the language and read the letters in which it is composed. It is written in the language of mathematics, and its characters are triangles, circles, and other geometric figures without which it is humanly impossible to understand a single word of it; without these, one wanders about in a dark labyrinth.
In 'The Assayer' (1623), trans. Stillman Drake, Discoveries and Opinions of Galileo (1957), 237-8.
Physicists still tend to regard biologists as men condemned by their lack of mathematics to follow an imprecise science. Some biologists think that, life is too complex to be amenable to mathematical study.
From A.S. Curtis, 'The Value of Mathematics in Biology',New Scientist (25 Jan 1962), 223.
Physics is NOT a body of indisputable and immutable Truth; it is a body of well-supported probable opinion only .... Physics can never prove things the way things are proved in mathematics, by eliminating ALL of the alternative possibilities. It is not possible to say what the alternative possibilities are.... Write down a number of 20 figures; if you multiply this by a number of, say, 30 figures, you would arrive at some enormous number (of either 49 or 50 figures). If you were to multiply the 30-figure number by the 20-figure number you would arrive at the same enormous 49- or 50-figure number, and you know this to be true without having to do the multiplying. This is the step you can never take in physics.
In Science is a Sacred Cow (1950), 68, 88, 179.
Physics is to mathematics what sex is to masturbation.
In Fear of Physics by Lawrence M. Krauss (1993).
Physics without mathematics is meaningless.
In Edward Teller, Wendy Teller and Wilson Talley, Conversations on the Dark Secrets of Physics (1991, 2013), 1.
Plenty of mathematicians, Hardy knew, could follow a step-by-step discursus unflaggingly—yet counted for nothing beside Ramanujan. Years later, he would contrive an informal scale of natural mathematical ability on which he assigned himself a 25 and Littlewood a 30. To David Hilbert, the most eminent mathematician of the day, he assigned an 80. To Ramanujan he gave 100.
In The Man who Knew Infinity: A Life of the Genius Ramanujan (1975), 226.
Poincaré was a vigorous opponent of the theory that all mathematics can be rewritten in terms of the most elementary notions of classical logic; something more than logic, he believed, makes mathematics what it is.
In Men of Mathematics (1937), 552.
Poincaré was the last man to take practically all mathematics, pure and applied, as his province. … Few mathematicians have had the breadth of philosophic vision that Poincaré had, and none is his superior in the gift of clear exposition.
In Eric Temple Bell, Men of Mathematics (1937, 1986), 527.
Poor teaching leads to the inevitable idea that the subject [mathematics] is only adapted to peculiar minds, when it is the one universal science and the one whose four ground-rules are taught us almost in infancy and reappear in the motions of the universe.
In Mathematical Teaching (1907), 19.
Probably among all the pursuits of the University, mathematics pre-eminently demand self-denial, patience, and perseverance from youth, precisely at that period when they have liberty to act for themselves, and when on account of obvious temptations, habits of restraint and application are peculiarly valuable.
In The Conflict of Studies and other Essays (1873), 12.
Professor [Max] Planck, of Berlin, the famous originator of the Quantum Theory, once remarked to me that in early life he had thought of studying economics, but had found it too difficult! Professor Planck could easily master the whole corpus of mathematical economics in a few days. He did not mean that! But the amalgam of logic and intuition and the wide knowledge of facts, most of which are not precise, which is required for economic interpretation in its highest form is, quite truly, overwhelmingly difficult for those whose gift mainly consists in the power to imagine and pursue to their furthest points the implications and prior conditions of comparatively simple facts which are known with a high degree of precision.
'Alfred Marshall: 1842-1924' (1924). In Geoffrey Keynes (ed.), Essays in Biography (1933), 191-2
Professor Cayley has since informed me that the theorem about whose origin I was in doubt, will be found in Schläfli’s De Eliminatione. This is not the first unconscious plagiarism I have been guilty of towards this eminent man whose friendship I am proud to claim. A more glaring case occurs in a note by me in the Comptes Rendus, on the twenty-seven straight lines of cubic surfaces, where I believe I have followed (like one walking in his sleep), down to the very nomenclature and notation, the substance of a portion of a paper inserted by Schlafli in the Mathematical Journal, which bears my name as one of the editors upon the face.
In Philosophical Transactions of the Royal Society (1864), 642.
Programming is one of the most difficult branches of applied mathematics; the poorer mathematicians had better remain pure mathematicians.
…...
Prolonged commitment to mathematical exercises in economics can be damaging. It leads to the atrophy of judgement and intuition which are indispensable for real solutions and, on occasion, leads also to a habit of mind which simply excludes the mathematically inconvenient factors from consideration.
In Economics, Peace, and Laughter (1981), 41, footnote.
Proper Experiments have always Truth to defend them; also Reasoning join’d with Mathematical Evidence, and founded upon Experiment, will hold equally true; but should it be true, without those Supports it must be altogether useless.
In Academical Lectures on the Theory of Physic (1751), Vol. 1. As quoted in Thomas Steele Hall, A Source Book in Animal Biology (1951), 485.
Pure mathematics … reveals itself as nothing but symbolic or formal logic. It is concerned with implications, not applications. On the other hand, natural science, which is empirical and ultimately dependent upon observation and experiment, and therefore incapable of absolute exactness, cannot become strictly mathematical. The certainty of geometry is thus merely the certainty with which conclusions follow from non-contradictory premises. As to whether these conclusions are true of the material world or not, pure mathematics is indifferent.
In 'Non-Euclidian Geometry of the Fourth Dimension', collected in Henry Parker Manning (ed.), The Fourth Dimension Simply Explained (1910), 58.
Pure mathematics and physics are becoming ever more closely connected, though their methods remain different. One may describe the situation by saying that the mathematician plays a game in which he himself invents the rules while the while the physicist plays a game in which the rules are provided by Nature, but as time goes on it becomes increasingly evident that the rules which the mathematician finds interesting are the same as those which Nature has chosen. … Possibly, the two subjects will ultimately unify, every branch of pure mathematics then having its physical application, its importance in physics being proportional to its interest in mathematics.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 124.
Pure mathematics consists entirely of such asseverations as that, if such and such is a proposition is true of anything, then such and such another propositions is true of that thing. It is essential not to discuss whether the first proposition is really true, and not to mention what the anything is of which it is supposed to be true. Both these points would belong to applied mathematics. … If our hypothesis is about anything and not about some one or more particular things, then our deductions constitute mathematics. Thus mathematics may be defined as the the subject in which we never know what we are talking about, not whether what we are saying is true. People who have been puzzled by the beginnings of mathematics will, I hope, find comfort in this definition, and will probably agree that it is accurate.
In 'Recent Work on the Principles of Mathematics', International Monthly (1901), 4, 84.
Pure mathematics is a collection of hypothetical, deductive theories, each consisting of a definite system of primitive, undefined, concepts or symbols and primitive, unproved, but self-consistent assumptions (commonly called axioms) together with their logically deducible consequences following by rigidly deductive processes without appeal to intuition.
In 'Non-Euclidian Geometry of the Fourth Dimension', collected in Henry Parker Manning (ed.), The Fourth Dimension Simply Explained (1910), 58.
Pure mathematics is much more than an armoury of tools and techniques for the applied mathematician. On the other hand, the pure mathematician has ever been grateful to applied mathematics for stimulus and inspiration. From the vibrations of the violin string they have drawn enchanting harmonies of Fourier Series, and to study the triode valve they have invented a whole theory of non-linear oscillations.
In 100 Years of Mathematics: a Personal Viewpoint (1981), 3.
Pure mathematics is not concerned with magnitude. It is merely the doctrine of notation of relatively ordered thought operations which have become mechanical.
In Schriften (1901), Zweiter Teil, 282.
Pure Mathematics is the class of all propositions of the form “p implies q,” where p and q are propositions containing one or more variables, the same in the two propositions, and neither p nor q contains any constants except logical constants. And logical constants are all notions definable in terms of the following: Implication, the relation of a term to a class of which it is a member, the notion of such that, the notion of relation, and such further notions as may be involved in the general notion of propositions of the above form. In addition to these, mathematics uses a notion which is not a constituent of the propositions which it considers, namely the notion of truth.
In 'Definition of Pure Mathematics', Principles of Mathematics (1903), 3.
Pure mathematics is, in its way, the poetry of logical ideas. One seeks the most general ideas of operation which will bring together in simple, logical and unified form the largest possible circle of formal relationships. In this effort toward logical beauty spiritual formulas are discovered necessary for the deeper penetration into the laws of nature.
In letter (1 May 1935), Letters to the Editor, 'The Late Emmy Noether: Professor Einstein Writes in Appreciation of a Fellow-Mathematician', New York Times (4 May 1935), 12.
Pure mathematics proves itself a royal science both through its content and form, which contains within itself the cause of its being and its methods of proof. For in complete independence mathematics creates for itself the object of which it treats, its magnitudes and laws, its formulas and symbols.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 94. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 11.
Pure mathematics; may it never be of any use to anyone.
A banquet toast, quoted as “I believe that it was at a banquet of the Red Lions that he proposed the toast…”, in Alexander Macfarlane, 'Henry John Stephen Smith', Lectures on Ten British Mathematicians of the Nineteenth Century (1916), 100.
Putting together the mysteries of nature with the laws of mathematics, he dared to hope to be able to unlock the secrets of both with the same key.
Epitaph of René Descartes
Epitaph of René Descartes
— Epitaph
In Peter Pešic, Labyrinth: A Search for the Hidden Meaning of Science (2001), 73.
Questions that pertain to the foundations of mathematics, although treated by many in recent times, still lack a satisfactory solution. Ambiguity of language is philosophy's main source of problems. That is why it is of the utmost importance to examine attentively the very words we use.
Arithmetices Principia, (1889)
Quite distinct from the theoretical question of the manner in which mathematics will rescue itself from the perils to which it is exposed by its own prolific nature is the practical problem of finding means of rendering available for the student the results which have been already accumulated, and making it possible for the learner to obtain some idea of the present state of the various departments of mathematics. … The great mass of mathematical literature will be always contained in Journals and Transactions, but there is no reason why it should not be rendered far more useful and accessible than at present by means of treatises or higher text-books. The whole science suffers from want of avenues of approach, and many beautiful branches of mathematics are regarded as difficult and technical merely because they are not easily accessible. … I feel very strongly that any introduction to a new subject written by a competent person confers a real benefit on the whole science. The number of excellent text-books of an elementary kind that are published in this country makes it all the more to be regretted that we have so few that are intended for the advanced student. As an example of the higher kind of text-book, the want of which is so badly felt in many subjects, I may mention the second part of Prof. Chrystal’s Algebra published last year, which in a small compass gives a great mass of valuable and fundamental knowledge that has hitherto been beyond the reach of an ordinary student, though in reality lying so close at hand. I may add that in any treatise or higher text-book it is always desirable that references to the original memoirs should be given, and, if possible, short historic notices also. I am sure that no subject loses more than mathematics by any attempt to dissociate it from its history.
In Presidential Address British Association for the Advancement of Science, Section A (1890), Nature, 42, 466.
Reductio ad absurdum, which Euclid loved so much, is one of a mathematician's finest weapons. It is a far finer gambit than any chess play: a chess player may offer the sacrifice of a pawn or even a piece, but a mathematician offers the game.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 94.
Remote from human passions, remote even from the pitiful facts of nature, the generations have gradually created an ordered cosmos [mathematics], where pure thought can dwell in its natural home...
'The Study of Mathematics', Philosophical Essays (1910), 73-74. In J. E. Creighton (Ed.), Evander Bradley McGilvary, 'Reviews of Books', The Philosophical Review (1911), Vol 20, 422.
Revolutions never occur in mathematics.
Tenth Law in 'Ten “Laws” Concerning Patterns of Change in the History of Mathematics', Historia Mathematica (1975), 2, 165.
Rigor is the gilt on the lily of real mathematics.
In 'The Ancients Versus the Moderns, a New Battle of the Books', The Mathematics Teacher (Oct 1958), 51, No. 6, 423.
Said M. Waldman, “…Chemistry is that branch of natural philosophy in which the greatest improvements have been and may be made; it is on that account that I have made it my peculiar study; but at the same time, I have not neglected the other branches of science. A man would make but a very sorry chemist if he attended to that department of human knowledge alone. If your wish is to become really a man of science and not merely a petty experimentalist, I should advise you to apply to every branch of natural philosophy, including mathematics.”
In Frankenstein: Or, The Modern Prometheus (1823), Vol. 1, 73-74. Webmaster note: In the novel, when the fictional characters meet, M. Waldman, professor of chemistry, sparks Victor Frankenstein’s interest in science. Shelley was age 20 when the first edition of the novel was published anonymously (1818).
Sample recommendation letter:
Dear Search Committee Chair,
I am writing this letter for Mr. John Smith who has applied for a position in your department. I should start by saying that I cannot recommend him too highly.
In fact, there is no other student with whom I can adequately compare him, and I am sure that the amount of mathematics he knows will surprise you.
His dissertation is the sort of work you don’t expect to see these days.
It definitely demonstrates his complete capabilities.
In closing, let me say that you will be fortunate if you can get him to work for you.
Sincerely,
A. D. Visor (Prof.)
Dear Search Committee Chair,
I am writing this letter for Mr. John Smith who has applied for a position in your department. I should start by saying that I cannot recommend him too highly.
In fact, there is no other student with whom I can adequately compare him, and I am sure that the amount of mathematics he knows will surprise you.
His dissertation is the sort of work you don’t expect to see these days.
It definitely demonstrates his complete capabilities.
In closing, let me say that you will be fortunate if you can get him to work for you.
Sincerely,
A. D. Visor (Prof.)
In A Mathematician Reads the Newspaper (1995), 43
Science and mathematics [are] much more compelling and exciting than the doctrines of pseudoscience, whose practitioners were condemned as early as the fifth century B.C. by the Ionian philosopher Heraclitus as “night walkers, magicians, priests of Bacchus, priestesses of the wine-vat, mystery-mongers.” But science is more intricate and subtle, reveals a much richer universe, and powerfully evokes our sense of wonder. And it has the additional and important virtue—to whatever extent the word has any meaning—of being true.
Broca's Brain: Reflections on the Romance of Science (1979, 1986), 76.
Science attempts to find logic and simplicity in nature. Mathematics attempts to establish order and simplicity in human thought.
The Pursuit of Simplicity (1980), 17.
Science has never shaken off the impress of its origin In the historical revolt of the later Renaissance, It has remained predominately an anti-rationalistic movement, based on a naive faith. What reasoning it has wanted it borrowed from mathematics, which is a surviving relic of Greek rationalism, following the deductive method. Science repudiated Philosophy. In other words, it never cared to justify its faith or explain its meaning; it has remained blandly indifferent to its refutation by Hume.
In Science and the Modern World: Lowell Lectures, 1925 (1926), 23-24.
Science is complex and chilling. The mathematical language of science is understood by very few. The vistas it presents are scary—an enormous universe ruled by chance and impersonal rules, empty and uncaring, ungraspable and vertiginous. How comfortable to turn instead to a small world, only a few thousand years old, and under God's personal; and immediate care; a world in which you are His peculiar concern.
The 'Threat' of Creationism. In Ashley Montagu (ed.), Science and Creationism (1984), 192.
Science should be taught the way mathematics is taught today. Science education should begin in kindergarten. In the first grade one would learn a little more, in the second grade, a little more, and so on. All students should get this basic science training.
From interview with Neil A. Campbell, in 'Crossing the Boundaries of Science', BioScience (Dec 1986), 36, No. 11, 738.
Scientific subjects do not progress necessarily on the lines of direct usefulness. Very many applications of the theories of pure mathematics have come many years, sometimes centuries, after the actual discoveries themselves. The weapons were at hand, but the men were not able to use them.
In Perry, Teaching of Mathematics (1902), 35.
Scientific work, especially mathematical work which is purely conceptual, may indeed possess the appearance of beauty, because of the inner coherence which it shares with fine art, or may resemble a piece of architecture.
From 'Characters of the Beautiful', Beauty, Chap. 3, collected in Collected Works Of Samuel Alexander (2000), 51-52.
Secondly, the study of mathematics would show them the necessity there is in reasoning, to separate all the distinct ideas, and to see the habitudes that all those concerned in the present inquiry have to one another, and to lay by those which relate not to the proposition in hand, and wholly to leave them out of the reckoning. This is that which, in other respects besides quantity is absolutely requisite to just reasoning, though in them it is not so easily observed and so carefully practised. In those parts of knowledge where it is thought demonstration has nothing to do, men reason as it were in a lump; and if upon a summary and confused view, or upon a partial consideration, they can raise the appearance of a probability, they usually rest content; especially if it be in a dispute where every little straw is laid hold on, and everything that can but be drawn in any way to give color to the argument is advanced with ostentation. But that mind is not in a posture to find truth that does not distinctly take all the parts asunder, and, omitting what is not at all to the point, draws a conclusion from the result of all the particulars which in any way influence it.
In Conduct of the Understanding, Sect. 7.
See skulking Truth to her old cavern fled,
Mountains of Casuistry heap’d o’er her head!
Philosophy, that lean’d on Heav’n before,
Shrinks to her second cause, and is no more.
Physic of Metaphysic begs defence,
And Metaphysic calls for aid on Sense!
See Mystery to Mathematics fly!
Mountains of Casuistry heap’d o’er her head!
Philosophy, that lean’d on Heav’n before,
Shrinks to her second cause, and is no more.
Physic of Metaphysic begs defence,
And Metaphysic calls for aid on Sense!
See Mystery to Mathematics fly!
In The Dunciad, collected in The Poetical Works of Alexander Pope (1828), Vol. 3, 211.
Seeing there is nothing that is so troublesome to mathematical practice … than the multiplications, divisions, square and cubical extractions of great numbers, which besides the tedious expense of time are … subject to many slippery errors, I began therefore to consider [how] I might remove those hindrances.
From Mirifici Logarithmorum Canonis Descriptio (1614), as translated by William Rae Macdonald in A Description of the Wonderful Canon of Logarithms (1889),
She knew only that if she did or said thus-and-so, men would unerringly respond with the complimentary thus-and-so. It was like a mathematical formula and no more difficult, for mathematics was the one subject that had come easy to Scarlett in her schooldays.
In Gone With the Wind (1936), 60.
Since the seventeenth century, physical intuition has served as a vital source for mathematical porblems and methods. Recent trends and fashions have, however, weakened the connection between mathematics and physics; mathematicians, turning away from their roots of mathematics in intuition, have concentrated on refinement and emphasized the postulated side of mathematics, and at other times have overlooked the unity of their science with physics and other fields. In many cases, physicists have ceased to appreciate the attitudes of mathematicians. This rift is unquestionably a serious threat to science as a whole; the broad stream of scientific development may split into smaller and smaller rivulets and dry out. It seems therefore important to direct our efforts towards reuniting divergent trends by classifying the common features and interconnections of many distinct and diverse scientific facts.
As co-author with David Hilbert, in Methods of Mathematical Physics (1937, 1989), Preface, v.
Sir Arthur Eddington deduces religion from the fact that atoms do not obey the laws of mathematics. Sir James Jeans deduces it from the fact that they do.
In The Scientific Outlook (1931, 2009), 77.
So is not mathematical analysis then not just a vain game of the mind? To the physicist it can only give a convenient language; but isn’t that a mediocre service, which after all we could have done without; and, it is not even to be feared that this artificial language be a veil, interposed between reality and the physicist’s eye? Far from that, without this language most of the intimate analogies of things would forever have remained unknown to us; and we would never have had knowledge of the internal harmony of the world, which is, as we shall see, the only true objective reality.
From La valeur de la science. In Anton Bovier, Statistical Mechanics of Disordered Systems (2006), 3, giving translation "approximately" in the footnote of the opening epigraph in the original French: “L’analyse mathématique, n’est elle donc qu’un vain jeu d’esprit? Elle ne peut pas donner au physicien qu’un langage commode; n’est-ce pa là un médiocre service, dont on aurait pu se passer à la rigueur; et même n’est il pas à craindre que ce langage artificiel ne soit pas un voile interposé entre la réalité at l’oeil du physicien? Loin de là, sans ce langage, la pluspart des anaologies intimes des choses nous seraient demeurées à jamais inconnues; et nous aurions toujours ignoré l’harmonie interne du monde, qui est, nous le verrons, la seule véritable réalité objective.” Another translation, with a longer quote, beginning “Without this language…”, is on the Henri Poincaré Quotes" page of this website.
Some books are to be tasted, others to be swallowed, and some few to be chewed and digested; that is, some books are to be read only in parts; other to be read, but not curiously; and some few to be read wholly, and with diligence and attention. Some books also may be read by deputy, and extracts made of them by others; but that would be only in the less important arguments, and the meaner sort of books; else distilled books are like common distilled waters, flashy things. Reading maketh a full man; conference a ready man; and writing an exact man. And therefore, if a man write little, he had need have a great memory; if he confer little, he had need have a present wit: and if he read little, he had need have much cunning, to seem to know that he doth not. Histories make men wise; poets witty; the mathematics subtile; natural philosophy deep; moral grave; logic and rhetoric able to contend. Abeunt studia in mores. [The studies pass into the manners.]
'Of Studies' (1625) in James Spedding, Robert Ellis and Douglas Heath (eds.), The Works of Francis Bacon (1887-1901), Vol. 6, 498.
Some mathematics problems look simple, and you try them for a year or so, and then you try them for a hundred years, and it turns out that they're extremely hard to solve. There's no reason why these problems shouldn't be easy, and yet they turn out to be extremely intricate. [Fermat's] Last Theorem is the most beautiful example of this.
From interview for PBS website on the NOVA program, 'The Proof'.
Some of my cousins who had the great advantage of University education used to tease me with arguments to prove that nothing has any existence except what we think of it. … These amusing mental acrobatics are all right to play with. They are perfectly harmless and perfectly useless. ... I always rested on the following argument. … We look up to the sky and see the sun. Our eyes are dazzled and our senses record the fact. So here is this great sun standing apparently on no better foundation than our physical senses. But happily there is a method, apart altogether from our physical senses, of testing the reality of the sun. It is by mathematics. By means of prolonged processes of mathematics, entirely separate from the senses, astronomers are able to calculate when an eclipse will occur. They predict by pure reason that a black spot will pass across the sun on a certain day. You go and look, and your sense of sight immediately tells you that their calculations are vindicated. So here you have the evidence of the senses reinforced by the entirely separate evidence of a vast independent process of mathematical reasoning. We have taken what is called in military map-making “a cross bearing.” When my metaphysical friends tell me that the data on which the astronomers made their calculations, were necessarily obtained originally through the evidence of the senses, I say, “no.” They might, in theory at any rate, be obtained by automatic calculating-machines set in motion by the light falling upon them without admixture of the human senses at any stage. When it is persisted that we should have to be told about the calculations and use our ears for that purpose, I reply that the mathematical process has a reality and virtue in itself, and that onie discovered it constitutes a new and independent factor. I am also at this point accustomed to reaffirm with emphasis my conviction that the sun is real, and also that it is hot— in fact hot as Hell, and that if the metaphysicians doubt it they should go there and see.
In My Early Life (1930).
Some of the men stood talking in this room, and at the right of the door a little knot had formed round a small table, the center of which was the mathematics student, who was eagerly talking. He had made the assertion that one could draw through a given point more than one parallel to a straight line; Frau Hagenström had cried out that this was impossible, and he had gone on to prove it so conclusively that his hearers were constrained to behave as though they understood.
In Little Herr Friedemann (1961), 25.
Some persons have contended that mathematics ought to be taught by making the illustrations obvious to the senses. Nothing can be more absurd or injurious: it ought to be our never-ceasing effort to make people think, not feel.
Seven Lectures on Shakespeare and Milton (1856) 24.
Srinivasa Ramanujan was the strangest man in all of mathematics, probably in the entire history of science. He has been compared to a bursting supernova, illuminating the darkest, most profound corners of mathematics, before being tragically struck down by tuberculosis at the age of
33... Working in total isolation from the main currents of his field, he was able to rederive 100 years’ worth of Western mathematics on his own. The tragedy of his life is that much of his work was wasted rediscovering known mathematics.
In Hyperspace:A Scientific Odyssey through Parallel Universes, Time Warps, and the Tenth Dimension (1994), 172.
Standard mathematics has recently been rendered obsolete by the discovery that for years we have been writing the numeral five backward. This has led to reevaluation of counting as a method of getting from one to ten. Students are taught advanced concepts of Boolean algebra, and formerly unsolvable equations are dealt with by threats of reprisals.
Getting Even (1978), 44.
Statistics is, or should be, about scientific investigation and how to do it better, but many statisticians believe it is a branch of mathematics.
In Technometrics (1990), 32, 251-252.
Statistics: the mathematical theory of ignorance.
From chapter title, 'The Mathematical Theory of Ignorance: The Statistical Approach to the Study of Man', in Mathematics in Western Culture (1953, 1964), 340.
Strange as it may sound, the power of mathematics rests on its evasion of all unnecessary thought and on its wonderful saving of mental operations.
As quoted, without source, in E.T. Bell, Men of Mathematics (1937), Vol. 1, l (Roman numeral 'l').
Stripping down to essentials is the essence of mathematics. … Reduction to essentials is what makes…a mathematician. … He doesn’t need roughage in his diet if he is a mathematician.
From Lecture (28 Nov 1957) in the Queen’s University, Belfast, 'Is the Study of its History a Brake on the Progress of Science', printed in Hermethena (1958), 19, 25.
Such is the character of mathematics in its profounder depths and in its higher and remoter zones that it is well nigh impossible to convey to one who has not devoted years to its exploration a just impression of the scope and magnitude of the existing body of the science. An imagination formed by other disciplines and accustomed to the interests of another field may scarcely receive suddenly an apocalyptic vision of that infinite interior world. But how amazing and how edifying were such a revelation, if it only could be made.
In Lectures on Science, Philosophy and Art (1908), 6.
Superman corresponds to the medieval speculations about the nature of angels. The economist Werner Sombart argued that modern abstract finance and mathematical science was a realization at the material level of the elaborate speculations of medieval philosophy. In the same way it could be argued that Superman is the comic-strip brother of the medieval angels. For the angels, as explained by Thomas Aquinas, are quite superior to time or space, yet can exert a local and material energy of superhuman kind.
In The Mechanical Bride: Folklore of Industrial Man (1967), 103.
Suppose then I want to give myself a little training in the art of reasoning; suppose I want to get out of the region of conjecture and probability, free myself from the difficult task of weighing evidence, and putting instances together to arrive at general propositions, and simply desire to know how to deal with my general propositions when I get them, and how to deduce right inferences from them; it is clear that I shall obtain this sort of discipline best in those departments of thought in which the first principles are unquestionably true. For in all our thinking, if we come to erroneous conclusions, we come to them either by accepting false premises to start with—in which case our reasoning, however good, will not save us from error; or by reasoning badly, in which case the data we start from may be perfectly sound, and yet our conclusions may be false. But in the mathematical or pure sciences,—geometry, arithmetic, algebra, trigonometry, the calculus of variations or of curves,— we know at least that there is not, and cannot be, error in our first principles, and we may therefore fasten our whole attention upon the processes. As mere exercises in logic, therefore, these sciences, based as they all are on primary truths relating to space and number, have always been supposed to furnish the most exact discipline. When Plato wrote over the portal of his school. “Let no one ignorant of geometry enter here,” he did not mean that questions relating to lines and surfaces would be discussed by his disciples. On the contrary, the topics to which he directed their attention were some of the deepest problems,— social, political, moral,—on which the mind could exercise itself. Plato and his followers tried to think out together conclusions respecting the being, the duty, and the destiny of man, and the relation in which he stood to the gods and to the unseen world. What had geometry to do with these things? Simply this: That a man whose mind has not undergone a rigorous training in systematic thinking, and in the art of drawing legitimate inferences from premises, was unfitted to enter on the discussion of these high topics; and that the sort of logical discipline which he needed was most likely to be obtained from geometry—the only mathematical science which in Plato’s time had been formulated and reduced to a system. And we in this country [England] have long acted on the same principle. Our future lawyers, clergy, and statesmen are expected at the University to learn a good deal about curves, and angles, and numbers and proportions; not because these subjects have the smallest relation to the needs of their lives, but because in the very act of learning them they are likely to acquire that habit of steadfast and accurate thinking, which is indispensable to success in all the pursuits of life.
In Lectures on Teaching (1906), 891-92.
Suppose we loosely define a religion as any discipline whose foundations rest on an element of faith, irrespective of any element of reason which may be present. Quantum mechanics for example would be a religion under this definition. But mathematics would hold the unique position of being the only branch of theology possessing a rigorous demonstration of the fact that it should be so classified.
Concluding remark in 'Consistency and Completeness—A Résumé', The American Mathematical Monthly (May 1956), 63, No.5, 305.
Surely the claim of mathematics to take a place among the liberal arts must now be admitted as fully made good. Whether we look at the advances made in modern geometry, in modern integral calculus, or in modern algebra, in each of these three a free handling of the material employed is now possible, and an almost unlimited scope is left to the regulated play of fancy. It seems to me that the whole of aesthetic (so far as at present revealed) may be regarded as a scheme having four centres, which may be treated as the four apices of a tetrahedron, namely Epic, Music, Plastic, and Mathematic. There will be found a common plane to every three of these, outside of which lies the fourth; and through every two may be drawn a common axis opposite to the axis passing through the other two. So far is certain and demonstrable. I think it also possible that there is a centre of gravity to each set of three, and that the line joining each such centre with the outside apex will intersect in a common point the centre of gravity of the whole body of aesthetic; but what that centre is or must be I have not had time to think out.
In 'Proof of the Hitherto Undemonstrated Fundamental Theorem of Invariants', Collected Mathematical Papers (1909), Vol. 3, 123.
Surely this is the golden age of mathematics.
In 'History of Mathematics in the Nineteenth Century', Congress of Arts and Sciences (1905), Vol. 1, 493. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 108.
Sylvester was incapable of reading mathematics in a purely receptive way. Apparently a subject either fired in his brain a train of active and restless thought, or it would not retain his attention at all. To a man of such a temperament, it would have been peculiarly helpful to live in an atmosphere in which his human associations would have supplied the stimulus which he could not find in mere reading. The great modern work in the theory of functions and in allied disciplines, he never became acquainted with …
What would have been the effect if, in the prime of his powers, he had been surrounded by the influences which prevail in Berlin or in Gottingen? It may be confidently taken for granted that he would have done splendid work in those domains of analysis, which have furnished the laurels of the great mathematicians of Germany and France in the second half of the present century.
What would have been the effect if, in the prime of his powers, he had been surrounded by the influences which prevail in Berlin or in Gottingen? It may be confidently taken for granted that he would have done splendid work in those domains of analysis, which have furnished the laurels of the great mathematicians of Germany and France in the second half of the present century.
In Address delivered at a memorial meeting at the Johns Hopkins University (2 May 1897), published in Bulletin of the American Mathematical Society (Jun 1897), 303. Also in Johns Hopkins University Circulars, 16 (1897), 54.
Symbolic Logic…has been disowned by many logicians on the plea that its interest is mathematical, and by many mathematicians on the plea that its interest is logical.
In 'Preface', A Treatise on Universal Algebra: With Applications (1898), Vol. 1, vi.
Taking … the mathematical faculty, probably fewer than one in a hundred really possess it, the great bulk of the population having no natural ability for the study, or feeling the slightest interest in it*. And if we attempt to measure the amount of variation in the faculty itself between a first-class mathematician and the ordinary run of people who find any kind of calculation confusing and altogether devoid of interest, it is probable that the former could not be estimated at less than a hundred times the latter, and perhaps a thousand times would more nearly measure the difference between them.
[* This is the estimate furnished me by two mathematical masters in one of our great public schools of the proportion of boys who have any special taste or capacity for mathematical studies. Many more, of course, can be drilled into a fair knowledge of elementary mathematics, but only this small proportion possess the natural faculty which renders it possible for them ever to rank high as mathematicians, to take any pleasure in it, or to do any original mathematical work.]
[* This is the estimate furnished me by two mathematical masters in one of our great public schools of the proportion of boys who have any special taste or capacity for mathematical studies. Many more, of course, can be drilled into a fair knowledge of elementary mathematics, but only this small proportion possess the natural faculty which renders it possible for them ever to rank high as mathematicians, to take any pleasure in it, or to do any original mathematical work.]
In Darwinism, chap. 15.
Taking mathematics from the beginning of the world to the time when Newton lived, what he had done was much the better half.
As quoted in Edmund Fillingham King, A Biographical Sketch of Sir Isaac Newton (1858), 97, stating this was Leibniz’s reply “when asked at the royal table in Berlin his opinion of Newton.” No source citation was given, although all the next quotes that followed had footnotes. The lack of citation leaves the accuracy of the quote unverified. If you know a primary source, please contact the Webmaster.
That man can interrogate as well as observe nature was a lesson slowly learned in his evolution. Of the two methods by which he can do this, the mathematical and the experimental, both have been equally fruitful—by the one he has gauged the starry heights and harnessed the cosmic forces to his will; by the other he has solved many of the problems of life and lightened many of the burdens of humanity.
In 'The Evolution of the Idea of Experiment in Medicine', in C.G. Roland, Sir William Osler, 1849-1919: A Selection for Medical Students (1982), 103. As cited in William Osler and Mark E. Silverman (ed.), The Quotable Osler (2002), 249
That mathematics “do not cultivate the power of generalization,”; … will be admitted by no person of competent knowledge, except in a very qualified sense. The generalizations of mathematics, are, no doubt, a different thing from the generalizations of physical science; but in the difficulty of seizing them, and the mental tension they require, they are no contemptible preparation for the most arduous efforts of the scientific mind. Even the fundamental notions of the higher mathematics, from those of the differential calculus upwards are products of a very high abstraction. … To perceive the mathematical laws common to the results of many mathematical operations, even in so simple a case as that of the binomial theorem, involves a vigorous exercise of the same faculty which gave us Kepler’s laws, and rose through those laws to the theory of universal gravitation. Every process of what has been called Universal Geometry—the great creation of Descartes and his successors, in which a single train of reasoning solves whole classes of problems at once, and others common to large groups of them—is a practical lesson in the management of wide generalizations, and abstraction of the points of agreement from those of difference among objects of great and confusing diversity, to which the purely inductive sciences cannot furnish many superior. Even so elementary an operation as that of abstracting from the particular configuration of the triangles or other figures, and the relative situation of the particular lines or points, in the diagram which aids the apprehension of a common geometrical demonstration, is a very useful, and far from being always an easy, exercise of the faculty of generalization so strangely imagined to have no place or part in the processes of mathematics.
In An Examination of Sir William Hamilton’s Philosophy (1878), 612-13.
That sometimes clear … and sometimes vague stuff … which is … mathematics.
In Mathematics, Science and Epistemology (1980), Vol. 2, 69. This quote condensed from a longer quote which begins, “Mathematical proofs are…”, on the Imre Lakatos Quotes page of this website.
The ‘mad idea’ which will lie at the basis of a future fundamental physical theory will come from a realization that physical meaning has some mathematical form not previously associated with reality. From this point of view the problem of the ‘mad idea’ is the problem of choosing, not of generating, the right idea. One should not understand that too literally. In the 1960s it was said (in a certain connection) that the most important discovery of recent years in physics was the complex numbers. The author [Yuri Manin] has something like that in mind.
Mathematics and Physics (1981), Foreward. Reprinted in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 90.
The “seriousness” of a mathematical theorem lies, not in its practical consequences, which are usually negligible, but in the significance of the mathematical ideas which it connects.
In A Mathematician's Apology (1940, 2012), 89.
The arithmetization of mathematics … which began with Weierstrass … had for its object the separation of purely mathematical concepts, such as number and correspondence and aggregate, from intuitional ideas, which mathematics had acquired from long association with geometry and mechanics. These latter, in the opinion of the formalists, are so firmly entrenched in mathematical thought that in spite of the most careful circumspection in the choice of words, the meaning concealed behind these words, may influence our reasoning. For the trouble with human words is that they possess content, whereas the purpose of mathematics is to construct pure thought. But how can we avoid the use of human language? The … symbol. Only by using a symbolic language not yet usurped by those vague ideas of space, time, continuity which have their origin in intuition and tend to obscure pure reason—only thus may we hope to build mathematics on the solid foundation of logic.
In Tobias Dantzig and Joseph Mazur (ed.), Number: The Language of Science (1930, ed. by Joseph Mazur 2007), 99.
The Mathematics are Friends to Religion, inasmuch as they charm the Passions, restrain the Impetuosity of the Imagination, and purge the Mind from Error and Prejudice. Vice is Error, Confusion, and false Reasoning; and all Truth is more or less opposite to it. Besides, Mathematical Studies may serve for a pleasant Entertainment for those Hours which young Men are apt to throw away upon their Vices; the Delightfulness of them being such as to make Solitude not only easy, but desirable.
In An Essay On the Usefulness of Mathematical Learning, (1701) 8-9.
The Principia Mathematica developed an overall scheme of the universe, one far more elegant and enlightening than any the ancients had devised. And the Newtonian scheme was based on a set of assumptions, so few and so simple, developed through so clear and so enticing a line of mathematics that conservatives could scarcely find the heart and courage to fight it.
In Entry 231, 'Newton, Sir Isaac', Asimov’s Biographical Encyclopedia of Science and Technology (2nd rev ed., 1982), 152.
The ability to imagine relations is one of the most indispensable conditions of all precise thinking. No subject can be named, in the investigation of which it is not imperatively needed; but it can be nowhere else so thoroughly acquired as in the study of mathematics.
In Darwinism and other Essays (1893), 296.
The actual evolution of mathematical theories proceeds by a process of induction strictly analogous to the method of induction employed in building up the physical sciences; observation, comparison, classification, trial, and generalisation are essential in both cases. Not only are special results, obtained independently of one another, frequently seen to be really included in some generalisation, but branches of the subject which have been developed quite independently of one another are sometimes found to have connections which enable them to be synthesised in one single body of doctrine. The essential nature of mathematical thought manifests itself in the discernment of fundamental identity in the mathematical aspects of what are superficially very different domains. A striking example of this species of immanent identity of mathematical form was exhibited by the discovery of that distinguished mathematician … Major MacMahon, that all possible Latin squares are capable of enumeration by the consideration of certain differential operators. Here we have a case in which an enumeration, which appears to be not amenable to direct treatment, can actually be carried out in a simple manner when the underlying identity of the operation is recognised with that involved in certain operations due to differential operators, the calculus of which belongs superficially to a wholly different region of thought from that relating to Latin squares.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 290.
The advancement and perfection of mathematics are intimately connected with the prosperity of the State.
Correspondance de Napoléon, t. 24 (1868), 112. In Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 42.
The advantage is that mathematics is a field in which one’s blunders tend to show very clearly and can be corrected or erased with a stroke of the pencil. It is a field which has often been compared with chess, but differs from the latter in that it is only one’s best moments that count and not one’s worst. A single inattention may lose a chess game, whereas a single successful approach to a problem, among many which have been relegated to the wastebasket, will make a mathematician’s reputation.
In Ex-Prodigy: My Childhood and Youth (1953), 21.
The analysis of variance is not a mathematical theorem, but rather a convenient method of arranging the arithmetic.
Remarking on the paper, ‘Statistics in Agricultural Research’ by J. Wishart, Journal of the Royal Statistical Society, Supplement (1934), 1, 52. As cited in Michael Cowle, Statistics in Psychology: An Historical Perspective (2005), 210.
The analytical geometry of Descartes and the calculus of Newton and Leibniz have expanded into the marvelous mathematical method—more daring than anything that the history of philosophy records—of Lobachevsky and Riemann, Gauss and Sylvester. Indeed, mathematics, the indispensable tool of the sciences, defying the senses to follow its splendid flights, is demonstrating today, as it never has been demonstrated before, the supremacy of the pure reason.
In 'What Knowledge is of Most Worth?', Presidential address to the National Education Association, Denver, Colorado (9 Jul 1895). In Educational Review (Sep 1895), 10, 109.
The ancients devoted a lifetime to the study of arithmetic; it required days to extract a square root or to multiply two numbers together. Is there any harm in skipping all that, in letting the school boy learn multiplication sums, and in starting his more abstract reasoning at a more advanced point? Where would be the harm in letting the boy assume the truth of many propositions of the first four books of Euclid, letting him assume their truth partly by faith, partly by trial? Giving him the whole fifth book of Euclid by simple algebra? Letting him assume the sixth as axiomatic? Letting him, in fact, begin his severer studies where he is now in the habit of leaving off? We do much less orthodox things. Every here and there in one’s mathematical studies one makes exceedingly large assumptions, because the methodical study would be ridiculous even in the eyes of the most pedantic of teachers. I can imagine a whole year devoted to the philosophical study of many things that a student now takes in his stride without trouble. The present method of training the mind of a mathematical teacher causes it to strain at gnats and to swallow camels. Such gnats are most of the propositions of the sixth book of Euclid; propositions generally about incommensurables; the use of arithmetic in geometry; the parallelogram of forces, etc., decimals.
In Teaching of Mathematics (1904), 12.
The Anglo-Dane appears to possess an aptitude for mathematics which is not shared by the native of any other English district as a whole, and it is in the exact sciences that the Anglo-Dane triumphs.
In A Study of British Genius (1904), 69. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 131. Moritz adds an editorial footnote: “The mathematical tendencies of Cambridge are due to the fact that Cambridge drains the ability of nearly the whole Anglo-Danish district.”
The anxious precision of modern mathematics is necessary for accuracy, … it is necessary for research. It makes for clearness of thought and for fertility in trying new combinations of ideas. When the initial statements are vague and slipshod, at every subsequent stage of thought, common sense has to step in to limit applications and to explain meanings. Now in creative thought common sense is a bad master. Its sole criterion for judgment is that the new ideas shall look like the old ones, in other words it can only act by suppressing originality.
In Introduction to Mathematics (1911), 157.
The apex of mathematical achievement occurs when two or more fields which were thought to be entirely unrelated turn out to be closely intertwined. Mathematicians have never decided whether they should feel excited or upset by such events.
In 'A Mathematician's Gossip', Indiscrete Thoughts (2008), 214.
The apodictic quality of mathematical thought, the certainty and correctness of its conclusions, are due, not to a special mode of ratiocination, but to the character of the concepts with which it deals. What is that distinctive characteristic? I answer: precision, sharpness, completeness,* of definition. But how comes your mathematician by such completeness? There is no mysterious trick involved; some ideas admit of such precision, others do not; and the mathematician is one who deals with those that do.
In 'The Universe and Beyond', Hibbert Journal (1904-1905), 3, 309. An editorial footnote indicates “precision, sharpness, completeness” — i.e., in terms of the absolutely clear and indefinable.
The art of doing mathematics consists in finding that special case which contains all the germs of generality.
Given as “attributed (apocryphally perhaps)” and no further citation; stated without quotation marks, in M. Kac, 'Wiener and Integration in Function Spaces', Bulletin of the American Mathematical Society (Jan 1966), 72, No. 1, Part 2, 65. This issue of the Bulletin, subtitled 'Norbert Wiener
1894-1964', Felix E. Browder (ed.), was dedicated to the memory of Norbert Wiener.
The astronomer who studies the motion of the stars is surely like a blind man who, with only a staff [mathematics] to guide him, must make a great, endless, hazardous journey that winds through innumerable desolate places. What will be the result? Proceeding anxiously for a while and groping his way with his staff, he will at some time, leaning upon it, cry out in despair to Heaven, Earth and all the Gods to aid him in his misery.
In The Sleepwalkers by Arthur Koestler (1968).
The average English author [of mathematical texts] leaves one under the impression that he has made a bargain with his reader to put before him the truth, the greater part of the truth, and nothing but the truth; and that if he has put the facts of his subject into his book, however difficult it may be to unearth them, he has fulfilled his contract with his reader. This is a very much mistaken view, because effective teaching requires a great deal more than a bare recitation of facts, even if these are duly set forth in logical order—as in English books they often are not. The probable difficulties which will occur to the student, the objections which the intelligent student will naturally and necessarily raise to some statement of fact or theory—these things our authors seldom or never notice, and yet a recognition and anticipation of them by the author would be often of priceless value to the student. Again, a touch of humour (strange as the contention may seem) in mathematical works is not only possible with perfect propriety, but very helpful; and I could give instances of this even from the pure mathematics of Salmon and the physics of Clerk Maxwell.
In Perry, Teaching of Mathematics (1902), 59-61.
The average gambler will say “The player who stakes his whole fortune on a single play is a fool, and the science of mathematics can not prove him to be otherwise.” The reply is obvious: “The science of mathematics never attempts the impossible, it merely shows that other players are greater fools.”
Concluding remarks to his mathematical proof, with certain assumptions, that the best betting strategy for “Gambler’s Ruin” would be to always make his largest stake on his first play. In 'Gambler’s Ruin', Annals of Mathematics (Jul 1909), 2nd Series, 10, No. 4, 189. This is also seen, without primary source, quoted as “It is true that a man who does this is a fool. I have only proved that a man who does anything else is an even bigger fool,” in Harold Eves, Return to Mathematical Circles (1988), 39.
The Babylonian and Assyrian civilizations have perished; Hammurabi, Sargon and Nebuchadnezzar are empty names; yet Babylonian mathematics is still interesting, and the Babylonian scale of 60 is still used in Astronomy.
In A Mathematician's Apology (1940, 2012), 80.
The basic ideas and simplest facts of set-theoretic topology are needed in the most diverse areas of mathematics; the concepts of topological and metric spaces, of compactness, the properties of continuous functions and the like are often indispensable.
As co-author with H. Hopf, Topologie I (1935), 23. As quoted, translated and cited in Reinhold Remmert, Theory of Complex Functions (1991), 9-10. From the original German, “Die Grundbegriffe und die einfachsten Tatsachen aus der mengentheoretischen Topologie braucht man in sehr verschiedenen Gebieten der Mathematik; die Begriffe des topologischen und des metrischen Raumes, der Kompaktheit, die Eigenschaften stetiger Abbildungen u. dgl. sind oft unentbehrlich.”
The beautiful has its place in mathematics as elsewhere. The prose of ordinary intercourse and of business correspondence might be held to be the most practical use to which language is put, but we should be poor indeed without the literature of imagination. Mathematics too has its triumphs of the Creative imagination, its beautiful theorems, its proofs and processes whose perfection of form has made them classic. He must be a “practical” man who can see no poetry in mathematics.
In A Scrap-book of Elementary Mathematics: Notes, Recreations, Essays (1908), 208.
The belief that mathematics, because it is abstract, because it is static and cold and gray, is detached from life, is a mistaken belief. Mathematics, even in its purest and most abstract estate, is not detached from life. It is just the ideal handling of the problems of life, as sculpture may idealize a human figure or as poetry or painting may idealize a figure or a scene. Mathematics is precisely the ideal handling of the problems of life, and the central ideas of the science, the great concepts about which its stately doctrines have been built up, are precisely the chief ideas with which life must always deal and which, as it tumbles and rolls about them through time and space, give it its interests and problems, and its order and rationality. That such is the case a few indications will suffice to show. The mathematical concepts of constant and variable are represented familiarly in life by the notions of fixedness and change. The concept of equation or that of an equational system, imposing restriction upon variability, is matched in life by the concept of natural and spiritual law, giving order to what were else chaotic change and providing partial freedom in lieu of none at all. What is known in mathematics under the name of limit is everywhere present in life in the guise of some ideal, some excellence high-dwelling among the rocks, an “ever flying perfect” as Emerson calls it, unto which we may approximate nearer and nearer, but which we can never quite attain, save in aspiration. The supreme concept of functionality finds its correlate in life in the all-pervasive sense of interdependence and mutual determination among the elements of the world. What is known in mathematics as transformation—that is, lawful transfer of attention, serving to match in orderly fashion the things of one system with those of another—is conceived in life as a process of transmutation by which, in the flux of the world, the content of the present has come out of the past and in its turn, in ceasing to be, gives birth to its successor, as the boy is father to the man and as things, in general, become what they are not. The mathematical concept of invariance and that of infinitude, especially the imposing doctrines that explain their meanings and bear their names—What are they but mathematicizations of that which has ever been the chief of life’s hopes and dreams, of that which has ever been the object of its deepest passion and of its dominant enterprise, I mean the finding of the worth that abides, the finding of permanence in the midst of change, and the discovery of a presence, in what has seemed to be a finite world, of being that is infinite? It is needless further to multiply examples of a correlation that is so abounding and complete as indeed to suggest a doubt whether it be juster to view mathematics as the abstract idealization of life than to regard life as the concrete realization of mathematics.
In 'The Humanization of Teaching of Mathematics', Science, New Series, 35, 645-46.
The bottom line for mathematicians is that the architecture has to be right. In all the mathematics that I did, the essential point was to find the right architecture. It’s like building a bridge. Once the main lines of the structure are right, then the details miraculously fit. The problem is the overall design.
In interview by Donald J. Albers, in 'Freeman Dyson: Mathematician, Physicist, and Writer', The College Mathematics Journal (Jan 1994), 25, No. 1, 20.
The British Mathematical Colloquium consists of three days of mathematics with no dogs and no wives.
Quoted in Des MacHale, Comic Sections (1993)
The business of concrete mathematics is to discover the equations which express the mathematical laws of the phenomenon under consideration; and these equations are the starting-point of the calculus, which must obtain from them certain quantities by means of others.
In Positive Philosophy, Bk. 1, chap. 2.
The calculus is to mathematics no more than what experiment is to physics, and all the truths produced solely by the calculus can be treated as truths of experiment. The sciences must proceed to first causes, above all mathematics where one cannot assume, as in physics, principles that are unknown to us. For there is in mathematics, so to speak, only what we have placed there… If, however, mathematics always has some essential obscurity that one cannot dissipate, it will lie, uniquely, I think, in the direction of the infinite; it is in that direction that mathematics touches on physics, on the innermost nature of bodies about which we know little….
In Elements de la géométrie de l'infini (1727), Preface, ciii. Quoted as a footnote to Michael S. Mahoney, 'Infinitesimals and Transcendent Relations: The Mathematics of Motion in the Late Seventeenth Century', collected in David C. Lindberg and Robert S. Westman (eds.), Reappraisals of the Scientific Revolution (1990), 489-490, footnote 46
The calculus was the first achievement of modern mathematics and it is difficult to overestimate its importance. I think it defines more unequivocally than anything else the inception of modern mathematics; and the system of mathematical analysis, which is its logical development, still constitutes the greatest technical advance in exact thinking.
In 'The Mathematician', Works of the Mind (1947), 1, No. 1. Collected in James Roy Newman (ed.), The World of Mathematics (1956), Vol. 4, 2055.
The chief aim of all investigations of the external world should be to discover the rational order and harmony which has been imposed on it by God and which He revealed to us in the language of mathematics.
Epigraph, without citation, in Morris Kline, Mathematical Thought from Ancient to Modern Times (1972), 231. Need primary source. Can you help?
The chief forms of beauty are order and symmetry and definiteness, which the mathematical sciences demonstrate in a special degree.
As translated in Book 13, 1078.a3, Aristotle’s Metaphysics, a Revised Text with Introduction and Commentary by W. D. Ross (1924), Vol. 2, 416.
The computational formalism of mathematics is a thought process that is externalised to such a degree that for a time it becomes alien and is turned into a technological process. A mathematical concept is formed when this thought process, temporarily removed from its human vessel, is transplanted back into a human mold. To think ... means to calculate with critical awareness.
Mathematics and Physics (1981), Foreward. Reprinted in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 90.
The computer is important, but not to mathematics.
From interview (1981) with Donald J. Albers. In John H. Ewing and Frederick W. Gehring, Paul Halmos Celebrating 50 Years of Mathematics (1991), 3.
The conception of correspondence plays a great part in modern mathematics. It is the fundamental notion in the science of order as distinguished from the science of magnitude. If the older mathematics were mostly dominated by the needs of mensuration, modern mathematics are dominated by the conception of order and arrangement. It may be that this tendency of thought or direction of reasoning goes hand in hand with the modern discovery in physics, that the changes in nature depend not only or not so much on the quantity of mass and energy as on their distribution or arrangement.
In History of European Thought in the Nineteenth Century (1903), Vol. 2, 736.
The conception of objective reality … has thus evaporated … into the transparent clarity of mathematics that represents no longer the behavior of particles but rather our knowledge of this behavior.
In 'The Representation of Nature in Contemporary Physics', Daedalus (1958), 87, 95-108. As cited in Karl Popper, Quantum Theory and the Schism in Physics (1992), 85.
The concepts of ‘soul’ or ‘life’ do not occur in atomic physics, and they could not, even indirectly, be derived as complicated consequences of some natural law. Their existence certainly does not indicate the presence of any fundamental substance other than energy, but it shows only the action of other kinds of forms which we cannot match with the mathematical forms of modern atomic physics ... If we want to describe living or mental processes, we shall have to broaden these structures. It may be that we shall have to introduce yet other concepts.
In 'Fundamental Problems of Atomic Physics', collected in Philip P. Wiener (Ed.), Readings in Philosophy of Science: Introduction to the Foundations and Cultural Aspects of the Sciences (1953), 96. From a Lecture delivered at the Eidgenössische Technische Hochschule, Zürich (9 Jul 1948). Published Wandlungen in den Grundlagen der Naturwissenschaft, as translated by F. C. Hayes in Philosophic Problems of Nuclear Science (1952).
The consideration of mathematics is at the base of knowledge of the mind as it is at the base of the natural sciences, and for the same reason: the free and fertile work of thought dates from that epoch when mathematics brought to man the true norm of truth.
As translated in James Byrnie Shaw, Lectures on the Philosophy of Mathematics (1918), 193. From Léon Brunschvicg, Les Étapes de La Philosophie Mathématique (1912), 577, “La considération de la mathématique est à la base de la connaissance de l’esprit comme elle est à la
base des sciences de la nature, et pour une même raison: l’œuvre libre et féconde de la pensée date de l’époque où la mathématique vint apporter à l’homme la norme véritable de la vérité.”
The constructions of the mathematical mind are at the same time free and necessary. The individual mathematician feels free to define his notions and set up his axioms as he pleases. But the question is will he get his fellow-mathematician interested in the constructs of his imagination. We cannot help the feeling that certain mathematical structures which have evolved through the combined efforts of the mathematical community bear the stamp of a necessity not affected by the accidents of their historical birth. Everybody who looks at the spectacle of modern algebra will be struck by this complementarity of freedom and necessity.
In 'A Half-Century of Mathematics',The American Mathematical Monthly (Oct 1951), 58, No. 8, 538-539.
The critical mathematician has abandoned the search for truth. He no longer flatters himself that his propositions are or can be known to him or to any other human being to be true; and he contents himself with aiming at the correct, or the consistent. The distinction is not annulled nor even blurred by the reflection that consistency contains immanently a kind of truth. He is not absolutely certain, but he believes profoundly that it is possible to find various sets of a few propositions each such that the propositions of each set are compatible, that the propositions of each such set imply other propositions, and that the latter can be deduced from the former with certainty. That is to say, he believes that there are systems of coherent or consistent propositions, and he regards it his business to discover such systems. Any such system is a branch of mathematics.
In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 94. Also in Science (1912), New Series, 35, 107.
The deep study of nature is the most fruitful source of mathematical discoveries. By offering to research a definite end, this study has the advantage of excluding vague questions and useless calculations; besides it is a sure means of forming analysis itself and of discovering the elements which it most concerns us to know, and which natural science ought always to conserve.
Théorie Analytique de la Chaleur, Discours Préliminaire. Translation as in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 89.
The definition of a good mathematical problem is the mathematics it generates rather than the problem itself.
From interview for PBS website on the NOVA program, 'The Proof'.
The development of abstract methods during the past few years has given mathematics a new and vital principle which furnishes the most powerful instrument for exhibiting the essential unity of all its branches.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 225.
The development of mathematics is largely a natural, not a purely logical one: mathematicians are continually answering questions suggested by astronomers or physicists; many essential mathematical theories are but the reflex outgrowth from physical puzzles.
In 'The Teaching of the History of Science', The Scientific Monthly (Sep 1918), 194.
The development of mathematics toward greater precision has led, as is well known, to the formalization of large tracts of it, so that one can prove any theorem using nothing but a few mechanical rules... One might therefore conjecture that these axioms and rules of inference are sufficient to decide any mathematical question that can at all be formally expressed in these systems. It will be shown below that this is not the case, that on the contrary there are in the two systems mentioned relatively simple problems in the theory of integers that cannot be decided on the basis of the axioms.
'On Formally Undecidable Propositions of Principia Mathematica and Related Systems I' (193 1), in S. Feferman (ed.), Kurt Gödel Collected Works: Publications 1929-1936 (1986), Vol. I, 145.
The dexterous management of terms and being able to fend and prove with them, I know has and does pass in the world for a great part of learning; but it is learning distinct from knowledge, for knowledge consists only in perceiving the habitudes and relations of ideas one to another, which is done without words; the intervention of sounds helps nothing to it. And hence we see that there is least use of distinction where there is most knowledge: I mean in mathematics, where men have determined ideas with known names to them; and so, there being no room for equivocations, there is no need of distinctions.
In Conduct of the Understanding, Sect. 31.
The discoveries of Newton have done more for England and for the race, than has been done by whole dynasties of British monarchs; and we doubt not that in the great mathematical birth of 1853, the Quaternions of Hamilton, there is as much real promise of benefit to mankind as in any event of Victoria’s reign.
In 'Imagination in Mathematics', North American Review, 85, 228.
The discovery in 1846 of the planet Neptune was a dramatic and spectacular achievement of mathematical astronomy. The very existence of this new member of the solar system, and its exact location, were demonstrated with pencil and paper; there was left to observers only the routine task of pointing their telescopes at the spot the mathematicians had marked.
In J.R. Newman (ed.), 'Commentary on John Couch Adams', The World of Mathematics (1956), 820.
The domain of mathematics is the sole domain of certainty. There and there alone prevail the standards by which every hypothesis respecting the external universe and all observation and all experiment must be finally judged. It is the realm to which all speculation and thought must repair for chastening and sanitation, the court of last resort, I say it reverently, for all intellection whatsoever, whether of demon, or man, or deity. It is there that mind as mind attains its highest estate.
In 'The Universe and Beyond', Hibbert Journal (1904-1906), 3, 314.
The effort of the economist is to see, to picture the interplay of economic elements. The more clearly cut these elements appear in his vision, the better; the more elements he can grasp and hold in his mind at once, the better. The economic world is a misty region. The first explorers used unaided vision. Mathematics is the lantern by which what before was dimly visible now looms up in firm, bold outlines. The old phantasmagoria disappear. We see better. We also see further.
In Mathematical Investigations in the Theory of Value and Prices (1892), 119.
The elegance of a mathematical theorem is directly proportional to the number of independent ideas one can see in the theorem and inversely proportional to the effort it takes to see them.
In Mathematical Discovery: On Understanding, Learning, and Teaching Problem Solving (1981). As cited, with no more details, in Yi Ma, An Invitation to 3-D Vision (2004), 228.
The employment of mathematical symbols is perfectly natural when the relations between magnitudes are under discussion; and even if they are not rigorously necessary, it would hardly be reasonable to reject them, because they are not equally familiar to all readers and because they have sometimes been wrongly used, if they are able to facilitate the exposition of problems, to render it more concise, to open the way to more extended developments, and to avoid the digressions of vague argumentation.
From Recherches sur les Principes Mathématiques de la Théorie des Richesses (1838), as translated by Nathaniel T. Bacon in 'Preface', Researches Into Mathematical Principles of the Theory of Wealth (1897), 3-4. From the original French, “L’emploi des signes mathématiques est chose naturelle toutes les fois qu'il s'agit de discuter des relations entre des grandeurs ; et lors même qu’ils ne seraient pas rigoureusement nécessaires, s’ils peuvent faciliter l’exposition, la rendre plus concise, mettre sur la voie de développements plus étendus, prévenir les écarts d’une vague argumentation, il serait peu philosophique de les rebuter, parce qu'ils ne sont pas également familiers à tous les lecteurs et qu'on s'en est quelquefois servi à faux.”
The end of the eighteenth and the beginning of the nineteenth century were remarkable for the small amount of scientific movement going on in this country, especially in its more exact departments. ... Mathematics were at the last gasp, and Astronomy nearly so—I mean in those members of its frame which depend upon precise measurement and systematic calculation. The chilling torpor of routine had begun to spread itself over all those branches of Science which wanted the excitement of experimental research.
Quoted in Sophia Elizabeth De Morgan, Memoir of Augustus De Morgan (1882), 41
The ends to be attained [in Teaching of Mathematics in the secondary schools] are the knowledge of a body of geometrical truths, the power to draw correct inferences from given premises, the power to use algebraic processes as a means of finding results in practical problems, and the awakening of interest in the science of mathematics.
In 'Aim of the Mathematical Instruction', International Commission on Teaching of Mathematics, American Report: United States Bureau of Education: Bulletin 1912, No. 4, 7.
The engineer is concerned to travel from the abstract to the concrete. He begins with an idea and ends with an object. He journeys from theory to practice. The scientist’s job is the precise opposite. He explores nature with his telescopes or microscopes, or much more sophisticated techniques, and feeds into a computer what he finds or sees in an attempt to define mathematically its significance and relationships. He travels from the real to the symbolic, from the concrete to the abstract. The scientist and the engineer are the mirror image of each other.
In The Development of Design (1981), 19-20.
The entire mathematical arsenal that our modern sages command cannot establish facts. Practical people should always keep this in mind when they ask mathematicians for help.
As translated from Literaturnaya Gazeta (5 Dec 1979), 49, 12, in 'Miscellanea', The American Mathematical Monthly (Aug-Sep 1980), 87, No. 7, 589.
The equation eπi = -1 has been called the eutectic point of mathematics, for no matter how you boil down and explain this equation, which relates four of the most remarkable numbers of mathematics, it still has a certain mystery about it that cannot be explained away.
The essence of mathematics is not to make simple things complicated, but to make complicated things simple.
In A Mathematical Journey (1976), xi.
The essence of mathematics lies in its freedom.
In Mathematische Annalen, Bd. 21, 564.
The essence of mathematics lies precisely in its freedom.
Gesammelte Abhandlungen (1932), 182, trans. Ivor Grattan-Guinness. Also, givened as: 'The essence of mathematics lies in its freedom,' in Mathematische Annalen, 21, 564. In Robert Édouard Moritz, Memorabilia Mathematica (1914), 12.
The essential fact is simply that all the pictures which science now draws of nature, and which alone seem capable of according with observational facts, are mathematical pictures. … It can hardly be disputed that nature and our conscious mathematical minds work according to the same laws.
In The Mysterious Universe (1930, 1932), 149 & 162.
The existence of an extensive Science of Mathematics, requiring the highest scientific genius in those who contributed to its creation, and calling for the most continued and vigorous exertion of intellect in order to appreciate it when created, etc.
In System of Logic, Bk. 2, chap. 4, sect. 4.
The extraordinary development of mathematics in the last century is quite unparalleled in the long history of this most ancient of sciences. Not only have those branches of mathematics which were taken over from the eighteenth century steadily grown, but entirely new ones have sprung up in almost bewildering profusion, and many of them have promptly assumed proportions of vast extent.
In The History of Mathematics in the Nineteenth Century', Congress of Arts and Sciences (1905), Vol. 1, 474. As cited and wuoted in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 110.
The fact is that there are few more “popular” subjects than mathematics. Most people have some appreciation of mathematics, just as most people can enjoy a pleasant tune; and there are probably more people really interested in mathematics than in music. Appearances may suggest the contrary, but there are easy explanations. Music can be used to stimulate mass emotion, while mathematics cannot; and musical incapacity is recognized (no doubt rightly) as mildly discreditable, whereas most people are so frightened of the name of mathematics that they are ready, quite unaffectedly, to exaggerate their own mathematical stupidity.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 86.
The fact that all Mathematics is Symbolic Logic is one of the greatest discoveries of our age; and when this fact has been established, the remainder of the principles of mathematics consists of the analysis of Symbolic Logic itself.
In Bertrand Russell, The Principles of Mathematics (1903), 5.
The fact that the proof of a theorem consists in the application of certain simple rules of logic does not dispose of the creative element in mathematics, which lies in the choice of the possibilities to be examined.
As co-author with Herbert Robbins, in What Is Mathematics?: An Elementary Approach to Ideas and Methods (1941, 1996), 15.
The faculty of resolution is possibly much invigorated by mathematical study, and especially by that highest branch of it which, unjustly, merely on account of its retrograde operations, has been called, as if par excellence, analysis.
In The Murders in Rue Morgue.
The faith of scientists in the power and truth of mathematics is so implicit that their work has gradually become less and less observation, and more and more calculation. The promiscuous collection and tabulation of data have given way to a process of assigning possible meanings, merely supposed real entities, to mathematical terms, working out the logical results, and then staging certain crucial experiments to check the hypothesis against the actual empirical results. But the facts which are accepted by virtue of these tests are not actually observed at all. With the advance of mathematical technique in physics, the tangible results of experiment have become less and less spectacular; on the other hand, their significance has grown in inverse proportion. The men in the laboratory have departed so far from the old forms of experimentation—typified by Galileo's weights and Franklin's kite—that they cannot be said to observe the actual objects of their curiosity at all; instead, they are watching index needles, revolving drums, and sensitive plates. No psychology of 'association' of sense-experiences can relate these data to the objects they signify, for in most cases the objects have never been experienced. Observation has become almost entirely indirect; and readings take the place of genuine witness.
Philosophy in a New Key; A Study in Inverse the Symbolism of Reason, Rite, and Art (1942), 19-20.
The farther a mathematical theory is developed, the more harmoniously and uniformly does its construction proceed, and unsuspected relations are disclosed between hitherto separated branches of the science.
In 'Mathematical Problems', Lecture at the International Congress of Mathematics, Paris, (8 Aug 1900). Translated by Dr. Maby Winton Newson in Bulletin of the American Mathematical Society (1902), 8, 479.
The fear of mathematics is a tradition handed down from days when the majority of teachers knew little about human nature and nothing at all about the nature of mathematics itself. What they did teach was an imitation.
in Mathematician’s Delight (1946), 12.
The feudal model of agriculture collided, first, with environmental limits and then with a massive external shock – the Black Death. After that, there was a demographic shock: too few workers for the land, which raised their wages and made the old feudal obligation system impossible to enforce. The labour shortage also forced technological innovation. The new technologies that underpinned the rise of merchant capitalism were the ones that stimulated commerce (printing and accountancy), the creation of tradeable wealth (mining, the compass and fast ships) and productivity (mathematics and the scientific method).
In 'The End of Capitalism Has Begun', The Guardian (17 Jul 2015) (online).
The first acquaintance which most people have with mathematics is through arithmetic. That two and two make four is usually taken as the type of a simple mathematical proposition which everyone will have heard of. … The first noticeable fact about arithmetic is that it applies to everything, to tastes and to sounds, to apples and to angels, to the ideas of the mind and to the bones of the body.
In An Introduction to Mathematics (1911), 9.
The first law of Engineering Mathematics: All infinite series converge, and moreover converge to the first term.
The first nonabsolute number is the number of people for whom the table is reserved. This will vary during the course of the first three telephone calls to the restaurant, and then bear no apparent relation to the number of people who actually turn up, or to the number of people who subsequently join them after the show/match/party/gig, or to the number of people who leave when they see who else has turned up.
The second nonabsolute number is the given time of arrival, which is now known to be one of the most bizarre of mathematical concepts, a recipriversexcluson, a number whose existence can only be defined as being anything other than itself. In other words, the given time of arrival is the one moment of time at which it is impossible that any member of the party will arrive. Recipriversexclusons now play a vital part in many branches of math, including statistics and accountancy and also form the basic equations used to engineer the Somebody Else’s Problem field.
The third and most mysterious piece of nonabsoluteness of all lies in the relationship between the number of items on the check [bill], the cost of each item, the number of people at the table and what they are each prepared to pay for. (The number of people who have actually brought any money is only a subphenomenon of this field.)
The second nonabsolute number is the given time of arrival, which is now known to be one of the most bizarre of mathematical concepts, a recipriversexcluson, a number whose existence can only be defined as being anything other than itself. In other words, the given time of arrival is the one moment of time at which it is impossible that any member of the party will arrive. Recipriversexclusons now play a vital part in many branches of math, including statistics and accountancy and also form the basic equations used to engineer the Somebody Else’s Problem field.
The third and most mysterious piece of nonabsoluteness of all lies in the relationship between the number of items on the check [bill], the cost of each item, the number of people at the table and what they are each prepared to pay for. (The number of people who have actually brought any money is only a subphenomenon of this field.)
Life, the Universe and Everything (1982, 1995), 47-48.
The first successes were such that one might suppose all the difficulties of science overcome in advance, and believe that the mathematician, without being longer occupied in the elaboration of pure mathematics, could turn his thoughts exclusively to the study of natural laws.
From Preface to Traité de calcul différentiel et de calcul intégral (1864-70), i. Quoted in address to the section of Algebra and Analysis, International Congress of Arts and Sciences, St. Louis (22 Sep 1904), 'On the Development of Mathematical Analysis and its Relation to Certain Other Sciences,' as translated by M.W. Haskell in Bulletin of the American Mathematical Society (May 1905), 11, 408.
The flights of the imagination which occur to the pure mathematician are in general so much better described in his formulas than in words, that it is not remarkable to find the subject treated by outsiders as something essentially cold and uninteresting— … the only successful attempt to invest mathematical reasoning with a halo of glory—that made in this section by Prof. Sylvester—is known to a comparative few, …
In Presidential Address British Association for the Advancement of Science (1871), Nature Vol. 4, 271,
The following is one of the many stories told of “old Donald McFarlane” the faithful assistant of Sir William Thomson.
The father of a new student when bringing him to the University, after calling to see the Professor [Thomson] drew his assistant to one side and besought him to tell him what his son must do that he might stand well with the Professor. “You want your son to stand weel with the Profeessorr?” asked McFarlane. “Yes.” “Weel, then, he must just have a guid bellyful o’ mathematics!”
The father of a new student when bringing him to the University, after calling to see the Professor [Thomson] drew his assistant to one side and besought him to tell him what his son must do that he might stand well with the Professor. “You want your son to stand weel with the Profeessorr?” asked McFarlane. “Yes.” “Weel, then, he must just have a guid bellyful o’ mathematics!”
As given in Life of Lord Kelvin (1910), Vol. 1, 420, footnote. [Note: William Thomson, later became Lord Kelvin. —Webmaster]
The following story (here a little softened from the vernacular) was narrated by Lord Kelvin himself when dining at Trinity Hall:
A certain rough Highland lad at the university had done exceedingly well, and at the close of the session gained prizes both in mathematics and in metaphysics. His old father came up from the farm to see his son receive the prizes, and visited the College. Thomson was deputed to show him round the place. “Weel, Mr. Thomson,” asked the old man, “and what may these mathematics be, for which my son has getten a prize?” “I told him,” replied Thomson, “that mathematics meant reckoning with figures, and calculating.” “Oo ay,” said the old man, “he’ll ha’ getten that fra’ me: I were ever a braw hand at the countin’.” After a pause he resumed: “And what, Mr. Thomson, might these metapheesics be?” “I endeavoured,” replied Thomson, “to explain how metaphysics was the attempt to express in language the indefinite.” The old Highlander stood still and scratched his head. “Oo ay: may be he’ll ha’ getten that fra’ his mither. She were aye a bletherin’ body."
A certain rough Highland lad at the university had done exceedingly well, and at the close of the session gained prizes both in mathematics and in metaphysics. His old father came up from the farm to see his son receive the prizes, and visited the College. Thomson was deputed to show him round the place. “Weel, Mr. Thomson,” asked the old man, “and what may these mathematics be, for which my son has getten a prize?” “I told him,” replied Thomson, “that mathematics meant reckoning with figures, and calculating.” “Oo ay,” said the old man, “he’ll ha’ getten that fra’ me: I were ever a braw hand at the countin’.” After a pause he resumed: “And what, Mr. Thomson, might these metapheesics be?” “I endeavoured,” replied Thomson, “to explain how metaphysics was the attempt to express in language the indefinite.” The old Highlander stood still and scratched his head. “Oo ay: may be he’ll ha’ getten that fra’ his mither. She were aye a bletherin’ body."
As given in Life of Lord Kelvin (1910), Vol. 2, 1124, footnote. [Note: William Thomson, later became Lord Kelvin. —Webmaster]
The formulation of a problem is often more essential than its solution, which may be merely a matter of mathematical or experimental skill. To raise new questions, new possibilities, to regard old problems from a new angle requires creative imagination and marks real advances in science.
In Albert Einstein and Léopold Infeld, The Evolution of Physics: The Growth of Ideas from Early Concepts to Relativity and Quanta (1938, 1966), 92.
The foundations of population genetics were laid chiefly by mathematical deduction from basic premises contained in the works of Mendel and Morgan and their followers. Haldane, Wright, and Fisher are the pioneers of population genetics whose main research equipment was paper and ink rather than microscopes, experimental fields, Drosophila bottles, or mouse cages. Theirs is theoretical biology at its best, and it has provided a guiding light for rigorous quantitative experimentation and observation.
'A Review of Some Fundamental Concepts and Problems of Population Genetics', Cold Spring Harbor Symposia on Quantitative Biology, 1955, 20, 13-14.
The fundamental laws necessary for the mathematical treatment of a large part of physics and the whole of chemistry are thus completely known, and the difficulty lies only in the fact that application of these laws leads to equations that are too complex to be solved.
'Quantum Mechanics of Many-Electron Systems', Proceedings of the Royal Society (1929), A, 123, 714-733. Quoted in Steven M. Bachrach, Computational Organic Chemistry, Preface, xiii.
The gambling reasoner is incorrigible; if he would but take to the squaring of the circle, what a load of misery would be saved.
Quoted in D. MacHale, Comic Sections (1993).
The game of chess has always fascinated mathematicians, and there is reason to suppose that the possession of great powers of playing that game is in many features very much like the possession of great mathematical ability. There are the different pieces to learn, the pawns, the knights, the bishops, the castles, and the queen and king. The board possesses certain possible combinations of squares, as in rows, diagonals, etc. The pieces are subject to certain rules by which their motions are governed, and there are other rules governing the players. … One has only to increase the number of pieces, to enlarge the field of the board, and to produce new rules which are to govern either the pieces or the player, to have a pretty good idea of what mathematics consists.
In Book review, 'What is Mathematics?', Bulletin American Mathematical Society (May 1912), 18, 386-387.
The general knowledge of our author [Leonhard Euler] was more extensive than could well be expected, in one who had pursued, with such unremitting ardor, mathematics and astronomy as his favorite studies. He had made a very considerable progress in medical, botanical, and chemical science. What was still more extraordinary, he was an excellent scholar, and possessed in a high degree what is generally called erudition. He had attentively read the most eminent writers of ancient Rome; the civil and literary history of all ages and all nations was familiar to him; and foreigners, who were only acquainted with his works, were astonished to find in the conversation of a man, whose long life seemed solely occupied in mathematical and physical researches and discoveries, such an extensive acquaintance with the most interesting branches of literature. In this respect, no doubt, he was much indebted to an uncommon memory, which seemed to retain every idea that was conveyed to it, either from reading or from meditation.
In Philosophical and Mathematical Dictionary (1815), 493-494.
The general mental qualification necessary for scientific advancement is that which is usually denominated “common sense,” though added to this, imagination, induction, and trained logic, either of common language or of mathematics, are important adjuncts.
From presidential address (24 Nov 1877) to the Philosophical Society of Washington. As cited by L.A. Bauer in his retiring president address (5 Dec 1908), 'The Instruments and Methods of Research', published in Philosophical Society of Washington Bulletin, 15, 103. Reprinted in William Crookes (ed.) The Chemical News and Journal of Industrial Science (30 Jul 1909), 59.
The genesis of mathematical creation is a problem which should intensely interest the psychologist.
In 'Mathematical Creation', The Value of Science, collected in Henri Poincaré and George bruce Halsted (trans.), The Foundations of Science (1913), 383.
The genesis of mathematical invention is a problem that must inspire the psychologist with the keenest interest. For this is the process in which the human mind seems to borrow least from the exterior world, in which it acts, or appears to act, only by itself and on itself, so that by studying the process of geometric thought, we may hope to arrive at what is most essential in the human mind
As translated in Arthur I. Miller, Imagery in Scientific Thought Creating 20th-Century Physics (1984, 2013), 307. Opening of Paper delivered at Conference at the Institut Général Psychologique, Paris, 'L’Invention Mathématique', published in Enseignment Mathématique (1908), 10, 357. From the original French, “La genèse do l’Invention mathématique est un problème qui doit inspirer le plus vif intérêt au psychologue. C’est l’acte dans lequel l’esprit humain semble le moins emprunter au monde extérieur, où il n’agit ou ne paraît agir que par lui-même et sur lui-même, de sorte, qu’en étudiant le processus de la pensée géométrique, c’est ce qu’il y a de plus essentiel dans l’esprit humain que nous pouvons espérer atteindre.”
The geneticist to-day is in a rather difficult position. He must have at least a bowing acquaintance with anatomy, cytology, and mathematics. He must dabble in taxonomy, physics, and even psychology.
In 'The Biochemistry of the Individual' (1937), collected in Neurath Hans (ed.), Perspectives in Biochemistry (1989), 6.
The genius of Laplace was a perfect sledge hammer in bursting purely mathematical obstacles; but, like that useful instrument, it gave neither finish nor beauty to the results. In truth, in truism if the reader please, Laplace was neither Lagrange nor Euler, as every student is made to feel. The second is power and symmetry, the third power and simplicity; the first is power without either symmetry or simplicity. But, nevertheless, Laplace never attempted investigation of a subject without leaving upon it the marks of difficulties conquered: sometimes clumsily, sometimes indirectly, always without minuteness of design or arrangement of detail; but still, his end is obtained and the difficulty is conquered.
In 'Review of “Théorie Analytique des Probabilites” par M. le Marquis de Laplace, 3eme edition. Paris. 1820', Dublin Review (1837), 2, 348.
The golden age of mathematics—that was not the age of Euclid, it is ours. Ours is the age when no less than six international congresses have been held in the course of nine years. It is in our day that more than a dozen mathematical societies contain a growing membership of more than two thousand men representing the centers of scientific light throughout the great culture nations of the world. It is in our time that over five hundred scientific journals are each devoted in part, while more than two score others are devoted exclusively, to the publication of mathematics. It is in our time that the Jahrbuch über die Fortschritte der Mathematik, though admitting only condensed abstracts with titles, and not reporting on all the journals, has, nevertheless, grown to nearly forty huge volumes in as many years. It is in our time that as many as two thousand books and memoirs drop from the mathematical press of the world in a single year, the estimated number mounting up to fifty thousand in the last generation. Finally, to adduce yet another evidence of a similar kind, it requires not less than seven ponderous tomes of the forthcoming Encyclopaedie der Mathematischen Wissenschaften to contain, not expositions, not demonstrations, but merely compact reports and bibliographic notices sketching developments that have taken place since the beginning of the nineteenth century.
In Lectures on Science, Philosophy and Art (1908), 8.
The Golden Gate Bridge is a giant moving math problem.
Quoted on web site for PBS American Experience episode for 'Golden Gate Bridge.'
The Good Spirit never cared for the colleges, and though all men and boys were now drilled in Greek, Latin, and Mathematics, it had quite left these shells high on the beach, and was creating and feeding other matters [science] at other ends of the world.
The Prose Works of Ralph Waldo Emerson (1870), 553.
The great problem of today is, how to subject all physical phenomena to dynamical laws. With all the experimental devices, and all the mathematical appliances of this generation, the human mind has been baffled in its attempts to construct a universal science of physics.
'President's Address', Proceedings of the American Association for the Advancement of Science (1874), 23, 34-5.
The great science [mathematics] occupies itself at least just as much with the power of imagination as with the power of logical conclusion.
In 'Pestalozzi's Idee eines A B C der Anschauung', Werke[Kehrbach] (1890), Bd.l, 174. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 31.
The great truths with which it [mathematics] deals, are clothed with austere grandeur, far above all purposes of immediate convenience or profit. It is in them that our limited understandings approach nearest to the conception of that absolute and infinite, towards which in most other things they aspire in vain. In the pure mathematics we contemplate absolute truths, which existed in the divine mind before the morning stars sang together, and which will continue to exist there, when the last of their radiant host shall have fallen from heaven. They existed not merely in metaphysical possibility, but in the actual contemplation of the supreme reason. The pen of inspiration, ranging all nature and life for imagery to set forth the Creator’s power and wisdom, finds them best symbolized in the skill of the surveyor. "He meted out heaven as with a span;" and an ancient sage, neither falsely nor irreverently, ventured to say, that “God is a geometer”.
In Orations and Speeches (1870), Vol. 3, 614.
The greatest unsolved theorem in mathematics is why some people are better at it than others.
In Howard W. Eves Return to Mathematical Circles, (1988), 158.
The Greeks in the first vigour of their pursuit of mathematical truth, at the time of Plato and soon after, had by no means confined themselves to those propositions which had a visible bearing on the phenomena of nature; but had followed out many beautiful trains of research concerning various kinds of figures, for the sake of their beauty alone; as for instance in their doctrine of Conic Sections, of which curves they had discovered all the principal properties. But it is curious to remark, that these investigations, thus pursued at first as mere matters of curiosity and intellectual gratification, were destined, two thousand years later, to play a very important part in establishing that system of celestial motions which succeeded the Platonic scheme of cycles and epicycles. If the properties of conic sections had not been demonstrated by the Greeks and thus rendered familiar to the mathematicians of succeeding ages, Kepler would probably not have been able to discover those laws respecting the orbits and motions of planets which were the occasion of the greatest revolution that ever happened in the history of science.
In History of Scientific Ideas, Bk. 9, chap. 14, sect. 3.
The Handmaiden of the Sciences.
Title of one of his books (1937) on mathematics for the lay reader. Compare “Science the Handmaid of Religion” as the title of an address by J.J. Coxhead in St. Paul’s Cathedral, London, to the Lay Helpers’ Association in 1877, which began with this “statement has often been made.”
The highest mathematical principles may be involved in the production of the simplest mechanical result.
In 'Academical Education', Orations and Speeches on Various Occasions (1870), Vol. 3, 513. This is seen misattributed to Eric Temple Bell, but he only quoted it, attributing it to Everett, in for example, Mathematics: Queen and Servant of Sciences (1938), 20.
The history of mathematics is exhilarating, because it unfolds before us the vision of an endless series of victories of the human mind, victories without counterbalancing failures, that is, without dishonorable and humiliating ones, and without atrocities.
In The Study of the History of Mathematics (1936), 13.
The history of mathematics is important also as a valuable contribution to the history of civilization. Human progress is closely identified with scientific thought. Mathematical and physical researches are a reliable record of intellectual progress.
In History of Mathematics (1897), 4.
The history of mathematics may be instructive as well as agreeable; it may not only remind us of what we have, but may also teach us to increase our store. Says De Morgan, “The early history of the mind of men with regards to mathematics leads us to point out our own errors; and in this respect it is well to pay attention to the history of mathematics.” It warns us against hasty conclusions; it points out the importance of a good notation upon the progress of the science; it discourages excessive specialization on the part of the investigator, by showing how apparently distinct branches have been found to possess unexpected connecting links; it saves the student from wasting time and energy upon problems which were, perhaps, solved long since; it discourages him from attacking an unsolved problem by the same method which has led other mathematicians to failure; it teaches that fortifications can be taken by other ways than by direct attack, that when repulsed from a direct assault it is well to reconnoiter and occupy the surrounding ground and to discover the secret paths by which the apparently unconquerable position can be taken.
In History of Mathematics (1897), 1-2.
The history of mathematics, as of any science, is to some extent the story of the continual replacement of one set of misconceptions by another. This is of course no cause for despair, for the newly instated assumptions very often possess the merit of being closer approximations to truth than those that they replace.
In 'Consistency and Completeness—A Résumé', The American Mathematical Monthly (May 1956), 63, No.5, 295.
The history of mathematics, lacking the guidance of philosophy, [is] blind, while the philosophy of mathematics, turning its back on the most intriguing phenomena in the history of mathematics, is empty.
In Proofs and Refutations: The Logic of Mathematical Discovery (1976), 2
The idea that aptitude for mathematics is rarer than aptitude for other subjects is merely an illusion which is caused by belated or neglected beginners.
In 'Umriss pädagogischer Vorlesungen', Werke [Kehrbach] (1902), Bd. 10, 101. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 74.
The ideal of mathematics should be to erect a calculus to facilitate reasoning in connection with every province of thought, or of external experience, in which the succession of thoughts, or of events can be definitely ascertained and precisely stated. So that all serious thought which is not philosophy, or inductive reasoning, or imaginative literature, shall be mathematics developed by means of a calculus.
In Universal Algebra (1898), Preface.
The ideas which these sciences, Geometry, Theoretical Arithmetic and Algebra involve extend to all objects and changes which we observe in the external world; and hence the consideration of mathematical relations forms a large portion of many of the sciences which treat of the phenomena and laws of external nature, as Astronomy, Optics, and Mechanics. Such sciences are hence often termed Mixed Mathematics, the relations of space and number being, in these branches of knowledge, combined with principles collected from special observation; while Geometry, Algebra, and the like subjects, which involve no result of experience, are called Pure Mathematics.
In The Philosophy of the Inductive Sciences (1868), Part 1, Bk. 2, chap. 1, sect. 4.
The imaginary expression √(-a) and the negative expression -b, have this resemblance, that either of them occurring as the solution of a problem indicates some inconsistency or absurdity. As far as real meaning is concerned, both are imaginary, since 0 - a is as inconceivable as √(-a).
The influence of his [Leibnitz’s] genius in forming that peculiar taste both in pure and in mixed mathematics which has prevailed in France, as well as in Germany, for a century past, will be found, upon examination, to have been incomparably greater than that of any other individual.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 3, 187.
The instruction of children should aim gradually to combine knowing and doing [Wissen und Konnen]. Among all sciences mathematics seems to be the only one of a kind to satisfy this aim most completely.
In Werke, Bd. 9 (1888), 409.
The intensity and quantity of polemical literature on scientific problems frequently varies inversely as the number of direct observations on which the discussions are based: the number and variety of theories concerning a subject thus often form a coefficient of our ignorance. Beyond the superficial observations, direct and indirect, made by geologists, not extending below about one two-hundredth of the Earth's radius, we have to trust to the deductions of mathematicians for our ideas regarding the interior of the Earth; and they have provided us successively with every permutation and combination possible of the three physical states of matter—solid, liquid, and gaseous.
'Address delivered by the President of Section [Geology] at Sydney (Friday, Aug 21), Report of the Eighty-Fourth Meeting of the British Association for the Advancement of Science: Australia 1914, 1915, 345.
The interpretation of messages from the earth’s interior demands all the resources of ordinary physics and of extraordinary mathematics. The geophysicist is of a noble company, all of whom are reading messages from the untouchable reality of things. The inwardness of things—atoms, crystals, mountains, planets, stars, nebulas, universes—is the quarry of these hunters of genius and Promethean boldness.
The interrelations of mathematics with science are as rich and various as the texture of science itself.
In 'Mathematics in the Physical Sciences', Scientific American (Sep 1964), 211, No. 3, 129.
The intrinsic character of mathematical research and knowledge is based essentially on three properties: first, on its conservative attitude towards the old truths and discoveries of mathematics; secondly, on its progressive mode of development, due to the incessant acquisition of new knowledge on the basis of the old; and thirdly, on its self-sufficiency and its consequent absolute independence.
In Mathematical Essays and Recreations (1898), 87.
The invention of the differential calculus marks a crisis in the history of mathematics. The progress of science is divided between periods characterized by a slow accumulation of ideas and periods, when, owing to the new material for thought thus patiently collected, some genius by the invention of a new method or a new point of view, suddenly transforms the whole subject on to a higher level.
In An Introduction to Mathematics (1911), 217. Whitehead continued by quoting the poet, Percy Shelley, who compared the slow accumulation of thoughts leading to an avalanche following the laying down of a great truth. See the poetic quote beginning, “The sun-awakened avalanche…” on the Percy Shelley Quotations page.
The invention of what we may call primary or fundamental notation has been but little indebted to analogy, evidently owing to the small extent of ideas in which comparison can be made useful. But at the same time analogy should be attended to, even if for no other reason than that, by making the invention of notation an art, the exertion of individual caprice ceases to be allowable. Nothing is more easy than the invention of notation, and nothing of worse example and consequence than the confusion of mathematical expressions by unknown symbols. If new notation be advisable, permanently or temporarily, it should carry with it some mark of distinction from that which is already in use, unless it be a demonstrable extension of the latter.
In 'Calculus of Functions', Encyclopaedia of Pure Mathematics (1847), Addition to Article 26, 388.
The iron labor of conscious logical reasoning demands great perseverance and great caution; it moves on but slowly, and is rarely illuminated by brilliant flashes of genius. It knows little of that facility with which the most varied instances come thronging into the memory of the philologist or historian. Rather is it an essential condition of the methodical progress of mathematical reasoning that the mind should remain concentrated on a single point, undisturbed alike by collateral ideas on the one hand, and by wishes and hopes on the other, and moving on steadily in the direction it has deliberately chosen.
In Ueber das Verhältniss der Naturwissenschaften zur Gesammtheit der Wissenschaft, Vorträge und Reden (1896), Bd. 1, 178.
The large collection of problems which our modern Cambridge books supply will be found to be almost an exclusive peculiarity of these books; such collections scarcely exist in foreign treatises on mathematics, nor even in English treatises of an earlier date. This fact shows, I think, that a knowledge of mathematics may be gained without the perpetual working of examples. … Do not trouble yourselves with the examples, make it your main business, I might almost say your exclusive business, to understand the text of your author.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 74.
The latest authors, like the most ancient, strove to subordinate the phenomena of nature to the laws of mathematics.
From 'Auctoris Præfatio', Principia Mathematica (1687). As translated by Andrew Motte in 'Author’s Preface', The Mathematical Principles of Natural Philosophy (1729), Vol. 1, first page of the Preface, unpaginated. From the original Latin: “Cum Veteres Mechanicam (uti Auctor est Pappus) in rerum Naturalium investigatione maximi fecerint, & Recentiores, missis formis substantialibus & qualitatibus occultis, Phenomena Naturæ ad leges Mathematicas revocare aggressi sint : Visum est in hoc Tractatu Mathesin excolere quatenus ea ad Philosophiam spectat.”
The laws of logic and pure mathematics, by their very nature, cannot be used as a basis for a scientific explanation because they tell us nothing that distinguishes the actual world from some other possible world. When we ask for the explanation of a fact, a particular observation in the actual world, we must use of empirical laws. They do not possess the certainty of logical and mathematical laws, but they do tell us something about the structure of the world.
In An Introduction to the Philosophy of Science (1966, 1995), 11-12.
The life and soul of science is its practical application, and just as the great advances in mathematics have been made through the desire of discovering the solution of problems which were of a highly practical kind in mathematical science, so in physical science many of the greatest advances that have been made from the beginning of the world to the present time have been made in the earnest desire to turn the knowledge of the properties of matter to some purpose useful to mankind.
From 'Electrical Units of Measurement', a lecture delivered at the Institution of Civil Engineers, London (3 May 1883), Popular Lectures and Addresses Vol. 1 (1891), 86-87.
The life of the spirit is a life of thought; the ideal of thought is truth; everlasting truth is the goal of mathematics.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
The line between entertaining math and serious math is a blurry one.
His final retrospective article, 'A Quarter-Century of Recreational Mathematics', Scientific American (Aug 1998).
The longer mathematics lives the more abstract—and therefore, possibly also the more practical—it becomes.
As quoted in The Mathematical Intelligencer (Winter 1991), 13, No. 1.
The love of mathematics is daily on the increase, not only with us but in the army. The result of this was unmistakably apparent in our last campaigns. Bonaparte himself has a mathematical head, and though all who study this science may not become geometricians like Laplace or Lagrange, or heroes like Bonaparte, there is yet left an influence upon the mind which enables them to accomplish more than they could possibly have achieved without this training.
In Letter (26 Jan 1798) to Von Zach. As quoted in translation in Karl Bruhns (ed.), Jane Lassell (trans.) and Caroline Lassell (trans.), Life of Alexander von Humboldt (1872), Vol. 1, 232. [Webmaster assigns this quote to Jérôme Lalande as an informed guess for the following reasons. The cited text gives only the last names, Lalande and von Zach, but it does also give a source footnote to a Allgemeine geographische Ephemeriden, 1, 340. The journal editor, Franz Xaver von Zach, was a Hungarian astronomer. Jérôme Lalande was a French astronomer, living at the same time, who called himself Jérôme Le Français de la Lande. Their names are seen referred to together in the same journal, Vol. 6, 360.]
The main duty of the historian of mathematics, as well as his fondest privilege, is to explain the humanity of mathematics, to illustrate its greatness, beauty and dignity, and to describe how the incessant efforts and accumulated genius of many generations have built up that magnificent monument, the object of our most legitimate pride as men, and of our wonder, humility and thankfulness, as individuals.
In The Study of the History of Mathematics (1936), 28.
The main sources of mathematical invention seem to be within man rather than outside of him: his own inveterate and insatiable curiosity, his constant itching for intellectual adventure; and likewise the main obstacles to mathematical progress seem to be also within himself; his scandalous inertia and laziness, his fear of adventure, his need of conformity to old standards, and his obsession by mathematical ghosts.
In The Study of the History of Mathematics (1936), 16.
The main species of beauty are orderly arrangement, proportion, and definiteness; and these are especially manifested by the mathematical sciences.
In Metaphysics, 13-1078a-b, as translated by Hugh Tredennick (1933). Also seen translated as, “The mathematical sciences particularly exhibit order, symmetry, and limitation; and these are the greatest forms of the beautiful.”
The majority of mathematical truths now possessed by us presuppose the intellectual toil of many centuries. A mathematician, therefore, who wishes today to acquire a thorough understanding of modern research in this department, must think over again in quickened tempo the mathematical labors of several centuries. This constant dependence of new truths on old ones stamps mathematics as a science of uncommon exclusiveness and renders it generally impossible to lay open to uninitiated readers a speedy path to the apprehension of the higher mathematical truths. For this reason, too, the theories and results of mathematics are rarely adapted for popular presentation … This same inaccessibility of mathematics, although it secures for it a lofty and aristocratic place among the sciences, also renders it odious to those who have never learned it, and who dread the great labor involved in acquiring an understanding of the questions of modern mathematics. Neither in the languages nor in the natural sciences are the investigations and results so closely interdependent as to make it impossible to acquaint the uninitiated student with single branches or with particular results of these sciences, without causing him to go through a long course of preliminary study.
In Mathematical Essays and Recreations (1898), 32.
The manuscript looks chaotic, even by mathematics standards.
[About newly-found late work of Srinivasa Ramanujan.]
[About newly-found late work of Srinivasa Ramanujan.]
Quoted in John Noble Wilford, 'Mathematician's Final Equations Praised', New York Times (9 Jun 1981), C1.
The material world begins to seem so trivial, so arbitrary, so ephemeral when contrasted with the timeless beauty of mathematics.
In The Mathematical Universe: An Alphabetical Journey Through the Great Proofs, Problems, and Personalities (1994), 160.
The mathematic, then, is an art. As such it has its styles and style periods. It is not, as the layman and the philosopher (who is in this matter a layman too) imagine, substantially unalterable, but subject like every art to unnoticed changes form epoch to epoch. The development of the great arts ought never to be treated without an (assuredly not unprofitable) side-glance at contemporary mathematics.
In Oswald Spengler and Charles Francis Atkinson (trans.), The Decline of the West (1926), 62.
The mathematical conception is, from its very nature, abstract; indeed its abstractness is usually of a higher order than the abstractness of the logician.
In 'Mathematics', Encyclopedia Britannica (1883), Vol. 15, 636.
The mathematical facts worthy of being studied are those which, by their analogy with other facts, are capable of leading us to the knowledge of a physical law. They reveal the kinship between other facts, long known, but wrongly believed to be strangers to one another.
From Lecture to the Psychological Society, Paris, 'Mathematical Creation', translation collected in James Roy Newman The World of Mathematics (1956), Vol. 4, 2043.
The mathematical formulation of the physicist’s often crude experience leads in an uncanny number of cases to an amazingly accurate description of a large class of phenomena. This shows that the mathematical language has more to commend it than being the only language which we can speak; it shows that it is, in a very real sense, the correct language.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences,' Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1 (February 1960). Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 542.
The mathematical framework of quantum theory has passed countless successful tests and is now universally accepted as a consistent and accurate description of all atomic phenomena. The verbal interpretation, on the other hand – i.e., the metaphysics of quantum theory – is on far less solid ground. In fact, in more than forty years physicists have not been able to provide a clear metaphysical model.
In The Tao of Physics (1975), 132.
The mathematical framework of quantum theory has passed countless successful tests and is now universally accepted as a consistent and accurate description of all atomic phenomena. The verbal interpretation, on the other hand, i.e. the metaphysics of quantum physics, is on far less solid ground. In fact, in more than forty years physicists have not been able to provide a clear metaphysical model.
In The Tao of Physics: An Exploration of the Parallels Between Modern Physics (1975), 132.
The mathematical intellectualism is henceforth a positive doctrine, but one that inverts the usual doctrines of positivism: in place of originating progress in order, dynamics in statics, its goal is to make logical order the product of intellectual progress. The science of the future is not enwombed, as Comte would have had it, as Kant had wished it, in the forms of the science already existing; the structure of these forms reveals an original dynamism whose onward sweep is prolonged by the synthetic generation of more and more complicated forms. No speculation on number considered as a category a priori enables one to account for the questions set by modern mathematics … space affirms only the possibility of applying to a multiplicity of any elements whatever, relations whose type the intellect does not undertake to determine in advance, but, on the contrary, it asserts their existence and nourishes their unlimited development.
As translated in James Byrnie Shaw, Lectures on the Philosophy of Mathematics (1918), 193. From Léon Brunschvicg, Les Étapes de La Philosophie Mathématique (1912), 567-568, “L’intellectualisme mathématique est désormais une doctrine positive, mais qui intervertira les formules habituelles du positivisme: au lieu de faire sortir le progrès de l’ordre, ou le dynamique du statique, il tend à faire de l'ordre logique le produit du progrès intellectuel. La science à venir n'est pas enfermée, comme l’aurait voulu Comte, comme le voulait déjà Kant, dans les formes de la science déjà faite; la constitution de ces formes révèle un dynamisme originel dont l’élan se prolonge par la génération synthétique de notions de plus en plus compliquées. Aucune spéculation sur le nombre, considéré comme catégorie a priori, ne permet de rendre compte des questions qui se sont posées pour la mathématique moderne … … l’espace ne fait qu'affirmer la possibilité d'appliquer sur une multiplicité d’éléments quelconques des relations dont l’intelligence ne cherche pas à déterminer d’avance le type, dont elle constate, au contraire, dont elle suscite le développement illimité.”
The mathematical method is the essence of mathematics. He who fully comprehends the method is a mathematician.
In Schriften (1901), Zweiter Teil, 190.
The mathematical sciences particularly exhibit order, symmetry, and limitation; and these are the greatest forms of the beautiful.
In Metaphysica, 3-1078b.
The mathematical take-over of physics has its dangers, as it could tempt us into realms of thought which embody mathematical perfection but might be far removed, or even alien to, physical reality. Even at these dizzying heights we must ponder the same deep questions that troubled both Plato and Immanuel Kant. What is reality? Does it lie in our mind, expressed by mathematical formulae, or is it “out there”.
In Book Review 'Pulling the Strings,' of Lawrence Krauss's Hiding in the Mirror: The Mysterious Lure of Extra Dimensions, from Plato to String Theory and Beyond in Nature (22 Dec 2005), 438, 1081.
The mathematical talent of Cayley was characterized by clearness and extreme elegance of analytical form; it was re-enforced by an incomparable capacity for work which has caused the distinguished scholar to be compared with Cauchy.
In Comptes Rendus (1895), 120, 234.
The mathematical universe is already so large and diversified that it is hardly possible for a single mind to grasp it, or, to put it in another way, so much energy would be needed for grasping it that there would be none left for creative research. A mathematical congress of today reminds one of the Tower of Babel, for few men can follow profitably the discussions of sections other than their own, and even there they are sometimes made to feel like strangers.
In The Study Of The History Of Mathematics (1936), 14.
The mathematically formulated laws of quantum theory show clearly that our ordinary intuitive concepts cannot be unambiguously applied to the smallest particles. All the words or concepts we use to describe ordinary physical objects, such as position, velocity, color, size, and so on, become indefinite and problematic if we try to use them of elementary particles.
In Across the Frontiers (1974), 114.
The mathematician is entirely free, within the limits of his imagination, to construct what worlds he pleases. What he is to imagine is a matter for his own caprice; he is not thereby discovering the fundamental principles of the universe nor becoming acquainted with the ideas of God. If he can find, in experience, sets of entities which obey the same logical scheme as his mathematical entities, then he has applied his mathematics to the external world; he has created a branch of science.
Aspects of Science: Second Series (1926), 92.
The mathematician lives in a purely conceptual sphere, and mathematics is but the higher development of Symbolic Logic.
In Recent Development of Physical Science (1904), 34. The second half of the sentence appears in Robert Édouard Moritz, Memorabilia Mathematica (1914), 206.
The mathematician requires tact and good taste at every step of his work, and he has to learn to trust to his own instinct to distinguish between what is really worthy of his efforts and what is not; he must take care not to be the slave of his symbols, but always to have before his mind the realities which they merely serve to express. For these and other reasons it seems to me of the highest importance that a mathematician should be trained in no narrow school; a wide course of reading in the first few years of his mathematical study cannot fail to influence for good the character of the whole of his subsequent work.
In Presidential Address British Association for the Advancement of Science, Section A, (1890), Nature, 42, 467.
The mathematician's patterns … must be beautiful … Beauty is the first test; there is no permanent place in the world for ugly mathematics.
In A Mathematician's Apology (1940, 2012), 85.
The mathematician's patterns, like the painter's or the poet's must be beautiful; the ideas, like the colours or the words must fit together in a harmonious way.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 85.
The mathematician’s best work is art, a high and perfect art, as daring as the most secret dreams of imagination, clear, and limpid. Mathematical genius and artistic genius touch each other.
As quoted in Havelock Ellis, The Dance of Life (1923), 139.
The mathematician’s best work is art, a high perfect art, as daring as the most secret dreams of imagination, clear and limpid. Mathematical genius and artistic genius touch one another.
As quoted, without citation, in Havelock Ellis, The Dance of Life (1923), 139.
The Mathematics are usually considered as being the very antipodes of Poesy. Yet Mathesis and Poesy are of the closest kindred, for they are both works of imagination. Poetry is a creation, a making, a fiction; and the Mathematics have been called, by an admirer of them, the sublimest and the most stupendous of fictions. It is true, they are not only μάθησις learning, but ποίησις, a creation.
From a review of William Rowan Hamilton’s, Lectures on Quaternions (1853), in 'The Imagination in Mathematics', The North American Review (Jul 1857), 85, No. 176, 229. Also in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 189. The original text has “Poetry is a creation…” but the latter text gives “Poesy is a creation…”.
The mathematics clearly called for a set of underlying elementary objects—at that time we needed three types of them—elementary objects that could be combined three at a time in different ways to make all the heavy particles we knew. ... I needed a name for them and called them quarks, after the taunting cry of the gulls, “Three quarks for Muster Mark,” from Finnegan's Wake by the Irish writer James Joyce.
From asppearance in the BBC-TV program written by Nigel Calder, 'The Key to the Universe,' (27 Jan 1977). As cited in Arthur Lewis Caso, 'The Production of New Scientific Terms', American Speech (Summer 1980), 55, No. 2, 101-102.
The mathematics have always been the implacable enemies of scientific romances.
In Oeuvres (1866), t. 3, 498.
The mathematics involved in string theory … in subtlety and sophistication vastly exceeds previous uses of mathematics in physical theories. … String theory has led to a whole host of amazing results in mathematics in areas that seem far removed from physics. To many this indicates that string theory must be on the right track.
In Book Review 'Pulling the Strings,' of Lawrence Krauss's Hiding in the Mirror: The Mysterious Lure of Extra Dimensions, from Plato to String Theory and Beyond in Nature (22 Dec 2005), 438, 1082.
The mathematics is not there till we put it there.
In 'The Concept of Structure', The Philosophy of Physical Science (1938, 2012), 137.
The mathematics of cooperation of men and tools is interesting. Separated men trying their individual experiments contribute in proportion to their numbers and their work may be called mathematically additive. The effect of a single piece of apparatus given to one man is also additive only, but when a group of men are cooperating, as distinct from merely operating, their work raises with some higher power of the number than the first power. It approaches the square for two men and the cube for three. Two men cooperating with two different pieces of apparatus, say a special furnace and a pyrometer or a hydraulic press and new chemical substances, are more powerful than their arithmetical sum. These facts doubtless assist as assets of a research laboratory.
Quoted from a speech delivered at the fiftieth anniversary of granting of M.I.T's charter, in Guy Suits, 'Willis Rodney Whitney', National Academy of Sciences, Biographical Memoirs (1960), 352.
The mathematics of the twenty-first century may be very different from our own; perhaps the schoolboy will begin algebra with the theory of substitution groups, as he might now but for inherited habits.
From Address before the New York Mathematical Society, Bulletin of the New York Mathematical Society (1893), 3, 107. As cited in G.A. Miller, 'Appreciative Remarks on the Theory of Groups', The American Mathematical Monthly (1903), 10, No. 4, 89.
https://books.google.com/books?id=hkM0AQAAMAAJ
1903
The Mathematics, I say, which effectually exercises, not vainly deludes or vexatiously torments studious Minds with obscure Subtilties, perplexed Difficulties, or contentious Disquisitions; which overcomes without Opposition, triumphs without Pomp, compels without Force, and rules absolutely without Loss of Liberty; which does not privately over-reach a weak Faith, but openly assaults an armed Reason, obtains a total Victory, and puts on inevitable Chains; whose Words are so many Oracles, and Works as many Miracles; which blabs out nothing rashly, nor designs anything from the Purpose, but plainly demonstrates and readily performs all Things within its Verge; which obtrudes no false Shadow of Science, but the very Science itself, the Mind firmly adhering to it, as soon as possessed of it, and can never after desert it of its own Accord, or be deprived of it by any Force of others: Lastly the Mathematics, which depends upon Principles clear to the Mind, and agreeable to Experience; which draws certain Conclusions, instructs by profitable Rules, unfolds pleasant Questions; and produces wonderful Effects; which is the fruitful Parent of, I had almost said all, Arts, the unshaken Foundation of Sciences, and the plentiful Fountain of Advantage to human Affairs.
Address to the University of Cambridge upon being elected Lucasian Professor of Mathematics (14 Mar 1664). In Mathematical Lectures (1734), xxviii.
The mere man of pleasure is miserable in old age, and the mere drudge in business is but little better, whereas, natural philosophy, mathematical and mechanical science, are a continual source of tranquil pleasure, and in spite of the gloomy dogmas of priests and of superstition, the study of these things is the true theology; it teaches man to know and admire the Creator, for the principles of science are in the creation, and are unchangeable and of divine origin.
Age of Reason (1794, 1818), 35.
The metaphysical philosopher from his point of view recognizes mathematics as an instrument of education, which strengthens the power of attention, develops the sense of order and the faculty of construction, and enables the mind to grasp under the simple formulae the quantitative differences of physical phenomena.
In Dialogues of Plato (1897), Vol. 2, 78.
The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift which we neither understand nor deserve.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences', Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1, 14. Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 537.
The modern development of mathematical logic dates from Boole’s Laws of Thought (1854). But in him and his successors, before Peano and Frege, the only thing really achieved, apart from certain details, was the invention of a mathematical symbolism for deducing consequences from the premises which the newer methods shared with Aristotle.
From a Lowell Lecture delivered in Boston (Apr 1914), 'Logic as the Essence of Philosophy". Published in Our Knowledge of the External World: As A Field For Scientific Method in Philosophy (1914), Lecture II, 40. Also quoted in William Bragg Ewald, From Kant to Hilbert: A Source Book in the Foundations of Mathematics (1996), Vol. 1, footnote, 442. In the Footnote, Ewalt contrasts a more “romantic” view of Boole written by Russell for a popular audience. Refer to the latter quote elsewhere on this Bertrand Russell webpage, which begins “Pure mathematics was discovered by Boole….”
The modern, and to my mind true, theory is that mathematics is the abstract form of the natural sciences; and that it is valuable as a training of the reasoning powers not because it is abstract, but because it is a representation of actual things.
From 'Introduction', Mathematical Teaching and its Modern Methods (1886), 9-10.
The Moon and its phases gave man his first calendar. Trying to match that calendar with the seasons helped give him mathematics. The usefulness of the calendar helped give rise to the thought of beneficent gods. And with all that the Moon is beautiful, too.
Epigraph in Isaac Asimov’s Book of Science and Nature Quotations (1988), 164.
The more a science advances, the more will it be possible to understand immediately results which formerly could be demonstrated only by means of lengthy intermediate considerations: a mathematical subject cannot be considered as finally completed until this end has been attained.
In Formensystem binärer Formen (1875), 2.
The more I study the things of the mind the more mathematical I find them. In them as in mathematics it is a question of quantities; they must be treated with precision. I have never had more satisfaction than in proving this in the realms of art, politics and history.
Notes made after the completion of the third chapter of Vol. 3 of La Rivolution, 22 April 1883. In E. Sparvel-Bayly (trans.), Life and Letters of H. Taine (1902-1908), Vol. 3, 239.
The more progress physical sciences make, the more they tend to enter the domain of mathematics, which is a kind of center to which they all converge. We may even judge of the degree of perfection to which a science has arrived by the facility with which it may be submitted to calculation.
In Eulogy of Quetelet by E. Mailly (1874).
The most difficult problem in mathematics is to make the date of a woman's birth agree with her present age.
In Evan Esar, 20,000 Quips and Quotes, 22.
The most distinct and beautiful statement of any truth [in science] must take at last the mathematical form.
A Week on the Concord and Merrimack Rivers (1862), 381.
The most distinctive characteristic which differentiates mathematics from the various branches of empirical science, and which accounts for its fame as the queen of the sciences, is no doubt the peculiar certainty and necessity of its results.
First sentence of 'Geometry and Empirical Science', collected in Carl Hempel and James H. Fetzer (ed.), The Philosophy of Carl G. Hempel: Studies in Science, Explanation, and Rationality (2001), Chap. 2, 18. Also Carl Hempel, 'Geometry and Empirical Science', collected in J.R. Newman (ed.), The World of Mathematics (1956), Vol. 3, 1635.
The most important thing we can do is inspire young minds and to advance the kind of science, math and technology education that will help youngsters take us to the next phase of space travel.
As summarized on a CNN web page - without quotation marks - from a statement by Glenn about the fourth National Space Day (4 May 2000). 'All systems go for National Space Day' on CNN website.
The most obvious and easy things in mathematics are not those that come logically at the beginning; they are things that, from the point of view of logical deduction, come somewhere in the middle. Just as the easiest bodies to see are those that are neither very near nor very far…
In Introduction to Mathematical Philosophy (1920), 2.
The most painful thing about mathematics is how far away you are from being able to use it after you have learned it.
In J.R. Newman (ed.), 'Commentary on The Tears of Mathematics', The World of Mathematics (1956), 1978.
The most useless investigation may prove to have the most startling practical importance: Wireless telegraphy might not yet have come if Clerk Maxwell had been drawn away from his obviously “useless” equations to do something of more practical importance. Large branches of chemistry would have remained obscure had Willard Gibbs not spent his time at mathematical calculations which only about two men of his generation could understand.
Quoted in Larry R. Squire (ed.), The History of Neuroscience in Autobiography (1996), Vol. 1, 350-351. The above is a highlight excerpted from a longer quote beginning “To prove to an indignant questioner ….” in this same collection for A. V. Hill.
The motive for the study of mathematics is insight into the nature of the universe. Stars and strata, heat and electricity, the laws and processes of becoming and being, incorporate mathematical truths. If language imitates the voice of the Creator, revealing His heart, mathematics discloses His intellect, repeating the story of how things came into being. And Value of Mathematics, appealing as it does to our energy and to our honor, to our desire to know the truth and thereby to live as of right in the household of God, is that it establishes us in larger and larger certainties. As literature develops emotion, understanding, and sympathy, so mathematics develops observation, imagination, and reason.
In A Theory of Motives, Ideals and Values in Education (1907), 406.
The moving power of mathematical invention is not reasoning but imagination.
Quoted in Robert Perceval Graves, Life of Sir W. R. Hamilton, Vol. 3 (1889), 219.
The nearer man approaches mathematics the farther away he moves from the animals.
In Progress and Catastrophe: An Anatomy of Human Adventure (1937), 62.
The new mathematics is a sort of supplement to language, affording a means of thought about form and quantity and a means of expression, more exact, compact, and ready than ordinary language. The great body of physical science, a great deal of the essential facts of financial science, and endless social and political problems are only accessible and only thinkable to those who have had a sound training in mathematical analysis, and the time may not be very remote when it will be understood that for complete initiation as an efficient citizen of the great complex world-wide States that are now developing, it is as necessary to be able to compute, to think in averages and maxima and minima, as it is now to be able to read and write.
Mankind in the Making (1903), 204. This is seen in a shorter form, somewhat misquoted in a paraphrase as: “Statistical thinking will one day be as necessary for efficient citizenship as the ability to read and write.” However, note that in fact, Wells refers only to “mathematical analysis” such as “averages and maxima and minima” — and did not specify (more complex) “statistics” at all! For citation of the paraphrase, see Samuel Wilks Quotations on this site.
The nineteenth century which prides itself upon the invention of steam and evolution, might have derived a more legitimate title to fame from the discovery of pure mathematics.
In International Monthly (1901), 4, 83.
The number of mathematical students … would be much augmented if those who hold the highest rank in science would condescend to give more effective assistance in clearing the elements of the difficulties which they present.
In Study and Difficulties of Mathematics (1902), Preface.
The object of pure mathematics is those relations which may be conceptually established among any conceived elements whatsoever by assuming them contained in some ordered manifold; the law of order of this manifold must be subject to our choice; the latter is the case in both of the only conceivable kinds of manifolds, in the discrete as well as in the continuous.
In Über das System der rein mathematischen Wissenschaften, Jahresbericht der Deutschen Mathematiker-Vereinigung, Bd. 1, 36. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 3.
The only place where a dollar is still worth one hundred cents today is in the problems in an arithmetic book.
In Evan Esar, 20,000 Quips and Quotes, 509.
The only way to learn mathematics is to do mathematics.
In 'Preface', A Hilbert Space Problem Book (1974, 1982), vii.
The opinion appears to be gaining ground that this very general conception of functionality, born on mathematical ground, is destined to supersede the narrower notion of causation, traditional in connection with the natural sciences. As an abstract formulation of the idea of determination in its most general sense, the notion of functionality includes and transcends the more special notion of causation as a one-sided determination of future phenomena by means of present conditions; it can be used to express the fact of the subsumption under a general law of past, present, and future alike, in a sequence of phenomena. From this point of view the remark of Huxley that Mathematics “knows nothing of causation” could only be taken to express the whole truth, if by the term “causation” is understood “efficient causation.” The latter notion has, however, in recent times been to an increasing extent regarded as just as irrelevant in the natural sciences as it is in Mathematics; the idea of thorough-going determinancy, in accordance with formal law, being thought to be alone significant in either domain.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 290.
The opinion of Bacon on this subject [geometry] was diametrically opposed to that of the ancient philosophers. He valued geometry chiefly, if not solely, on account of those uses, which to Plato appeared so base. And it is remarkable that the longer Bacon lived the stronger this feeling became. When in 1605 he wrote the two books on the Advancement of Learning, he dwelt on the advantages which mankind derived from mixed mathematics; but he at the same time admitted that the beneficial effect produced by mathematical study on the intellect, though a collateral advantage, was “no less worthy than that which was principal and intended.” But it is evident that his views underwent a change. When near twenty years later, he published the De Augmentis, which is the Treatise on the Advancement of Learning, greatly expanded and carefully corrected, he made important alterations in the part which related to mathematics. He condemned with severity the pretensions of the mathematicians, “delidas et faslum mathematicorum.” Assuming the well-being of the human race to be the end of knowledge, he pronounced that mathematical science could claim no higher rank than that of an appendage or an auxiliary to other sciences. Mathematical science, he says, is the handmaid of natural philosophy; she ought to demean herself as such; and he declares that he cannot conceive by what ill chance it has happened that she presumes to claim precedence over her mistress.
In 'Lord Bacon', Edinburgh Review (Jul 1837). Collected in Critical and Miscellaneous Essays: Contributed to the Edinburgh Review (1857), Vol. 1, 395.
The origin of a science is usually to be sought for not in any systematic treatise, but in the investigation and solution of some particular problem. This is especially the case in the ordinary history of the great improvements in any department of mathematical science. Some problem, mathematical or physical, is proposed, which is found to be insoluble by known methods. This condition of insolubility may arise from one of two causes: Either there exists no machinery powerful enough to effect the required reduction, or the workmen are not sufficiently expert to employ their tools in the performance of an entirely new piece of work. The problem proposed is, however, finally solved, and in its solution some new principle, or new application of old principles, is necessarily introduced. If a principle is brought to light it is soon found that in its application it is not necessarily limited to the particular question which occasioned its discovery, and it is then stated in an abstract form and applied to problems of gradually increasing generality.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
In A Treatise on Projections (1880), Introduction, xi. Published as United States Coast and Geodetic Survey, Treasury Department Document, No. 61.
The originality of mathematics consists in the fact that in mathematical science connections between things are exhibited which, apart from the agency of human reason, are extremely unobvious.
In Science and the Modern World (1938), 32.
The participation in the general development of the mental powers without special reference to his future vocation must be recognized as the essential aim of mathematical instruction.
In Anleitung zum Mathematischen Unterricht an höheren Schulen (1906), 12.
The peculiar character of mathematical truth is, that it is necessarily and inevitably true; and one of the most important lessons which we learn from our mathematical studies is a knowledge that there are such truths, and a familiarity with their form and character.
This lesson is not only lost, but read backward, if the student is taught that there is no such difference, and that mathematical truths themselves are learned by experience.
This lesson is not only lost, but read backward, if the student is taught that there is no such difference, and that mathematical truths themselves are learned by experience.
In Thoughts on the Study of Mathematics. Principles of English University Education (1838).
The philosophy of mathematics still consists essentially in discerning the rational order of dependence of as many abstract truths as the sagacity of inventive minds has successfully and laboriously discovered, often by very roundabout means.
From Essai sur les Fondements de nos Connaissances et sur les Caractères de la Critique Philosophique (1851), Vol. 2, 235, as translated by Merritt H Moore in An Essay on the Foundations of Our Knowledge (1956), 475. From the original French: “La philosophie des mathématiques consiste encore essentiellement à discerner l'ordre et la dépendance rationnelle de tant de vérités abstraites que la sagacité des inventeurs a successivement et laborieusement découvertes, souvent par des voies si détournées.”
The physicist, in his study of natural phenomena, has two methods of making progress: (1) the method of experiment and observation, and (2) the method of mathematical reasoning. The former is just the collection of selected data; the latter enables one to infer results about experiments that have not been performed. There is no logical reason why the second method should be possible at all, but one has found in practice that it does work and meets with reasonable success.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 122.
The point of mathematics is that in it we have always got rid of the particular instance, and even of any particular sorts of entities. So that for example, no mathematical truths apply merely to fish, or merely to stones, or merely to colours. … Mathematics is thought moving in the sphere of complete abstraction from any particular instance of what it is talking about.
In 'Mathematics', Science and the Modern World (1926, 2011), 27.
The point of mathematics is that in it we have always got rid of the particular instance, and even of any particular sorts of entities. So that for example, no mathematical truths apply merely to fish, or merely to stones, or merely to colours. So long as you are dealing with pure mathematics, you are in the realm of complete and absolute abstraction. … Mathematics is thought moving in the sphere of complete abstraction from any particular instance of what it is talking about.
In Science and the Modern World: Lowell Lectures, 1925 (1925), 31.
The present gigantic development of the mathematical faculty is wholly unexplained by the theory of natural selection, and must be due to some altogether distinct cause.
In Darwinism, chap. 15.
The present state of electrical science seems peculiarly unfavorable to speculation … to appreciate the requirements of the science, the student must make himself familiar with a considerable body of most intricate mathematics, the mere retention of which in the memory materially interferes with further progress. The first process therefore in the effectual study of the science, must be one of simplification and reduction of the results of previous investigation to a form in which the mind can grasp them.
First sentence of Maxwell’s first paper (read 10 Dec 1855), 'On Faraday’s Lines of Force', Transactions of the Cambridge Philosophical Society (1857), Vol. X, part I. Collected in William Davidson Niven (ed.), The Scientific Papers of James Clerk Maxwell (1890), Vol. 1, 155.
The presentation of mathematics where you start with definitions, for example, is simply wrong. Definitions aren't the places where things start. Mathematics starts with ideas and general concepts, and then definitions are isolated from concepts. Definitions occur somewhere in the middle of a progression or the development of a mathematical concept. The same thing applies to theorems and other icons of mathematical progress. They occur in the middle of a progression of how we explore the unknown.
Interview for website of the Mathematical Association of America.
The prevailing trend in modern physics is thus much against any sort of view giving primacy to ... undivided wholeness of flowing movement. Indeed, those aspects of relativity theory and quantum theory which do suggest the need for such a view tend to be de-emphasized and in fact hardly noticed by most physicists, because they are regarded largely as features of the mathematical calculus and not as indications of the real nature of things.
Wholeness and the Implicate Order? (1981), 14.
The principles of logic and mathematics are true universally simply because we never allow them to be anything else. And the reason for this is that we cannot abandon them without contradicting ourselves, without sinning against the rules which govern the use of language, and so making our utterances self-stultifying. In other words, the truths of logic and mathematics are analytic propositions or tautologies.
Language, Truth and Logic (1960), 77.
The professor may choose familiar topics as a starting point. The students collect material, work problems, observe regularities, frame hypotheses, discover and prove theorems for themselves. … the student knows what he is doing and where he is going; he is secure in his mastery of the subject, strengthened in confidence of himself. He has had the experience of discovering mathematics. He no longer thinks of mathematics as static dogma learned by rote. He sees mathematics as something growing and developing, mathematical concepts as something continually revised and enriched in the light of new knowledge. The course may have covered a very limited region, but it should leave the student ready to explore further on his own.
In A Concrete Approach to Abstract Algebra (1959), 1-2.
The profound mathematical ability of Bolyai János showed itself physically not only in his handling of the violin, where he was a master, but also of arms, where he was unapproachable.
In János Bolyai, Science Absolute of Space, translated from the Latin by George Bruce Halsted (1896), Translator's Introduction, xxix. [Bolyai was the victor in many duels. —Webmaster]
The progress of mathematics can be viewed as progress from the infinite to the finite.
In 'A Mathematician's Gossip', Indiscrete Thoughts, (2008), 214.
The prominent reason why a mathematician can be judged by none but mathematicians, is that he uses a peculiar language. The language of mathesis is special and untranslatable. In its simplest forms it can be translated, as, for instance, we say a right angle to mean a square corner. But you go a little higher in the science of mathematics, and it is impossible to dispense with a peculiar language. It would defy all the power of Mercury himself to explain to a person ignorant of the science what is meant by the single phrase “functional exponent.” How much more impossible, if we may say so, would it be to explain a whole treatise like Hamilton’s Quaternions, in such a wise as to make it possible to judge of its value! But to one who has learned this language, it is the most precise and clear of all modes of expression. It discloses the thought exactly as conceived by the writer, with more or less beauty of form, but never with obscurity. It may be prolix, as it often is among French writers; may delight in mere verbal metamorphoses, as in the Cambridge University of England; or adopt the briefest and clearest forms, as under the pens of the geometers of our Cambridge; but it always reveals to us precisely the writer’s thought.
In North American Review (Jul 1857), 85, 224-225.
The proof given by Wright, that non-adaptive differentiation will occur in small populations owing to “drift,” or the chance fixation of some new mutation or recombination, is one of the most important results of mathematical analysis applied to the facts of neo-mendelism. It gives accident as well as adaptation a place in evolution, and at one stroke explains many facts which puzzled earlier selectionists, notably the much greater degree of divergence shown by island than mainland forms, by forms in isolated lakes than in continuous river-systems.
Evolution: The Modern Synthesis (1942), 199-200.
The propositions of mathematics have, therefore, the same unquestionable certainty which is typical of such propositions as “All bachelors are unmarried,” but they also share the complete lack of empirical content which is associated with that certainty: The propositions of mathematics are devoid of all factual content; they convey no information whatever on any empirical subject matter.
From 'On the Nature of Mathematical Truth', collected in Carl Hempel and James H. Fetzer (ed.), The Philosophy of Carl G. Hempel: Studies in Science, Explanation, and Rationality (2001), Chap. 1, 13. Also Carl Hempel, 'On the Nature of Mathematical Truth', collected in J.R. Newman (ed.), The World of Mathematics (1956), Vol. 3, 1631.
The public does not need to be convinced that there is something in mathematics.
In A Mathematician's Apology (1940, 2012), 63-65.
The purely formal sciences, logic and mathematics, deal with such relations which are independent of the definite content, or the substance of the objects, or at least can be. In particular, mathematics involves those relations of objects to each other that involve the concept of size, measure, number.
In Theorie der Complexen Zahlensysteme, (1867), 1. Translated by Webmaster using Google Translate from the original German, “Die rein formalen Wissenschaften, Logik und Mathematik, haben solche Relationen zu behandeln, welche unabhängig von dem bestimmten Inhalte, der Substanz der Objecte sind oder es wenigstens sein können.”
The purely formal Sciences, logic and mathematics, deal with those relations which are, or can be, independent of the particular content or the substance of objects. To mathematics in particular fall those relations between objects which involve the concepts of magnitude, of measure and of number.
In Theorie der Complexen Zahlensysteme (1867), 1. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 4. From the original German, “Die rein formalen Wissenschaften, Logik und Mathematik, haben solche Relationen zu behandeln, welche unabhängig von dem bestimmten Inhalte, der Substanz der Objecte sind oder es wenigstens sein können. Der Mathematik fallen ins Besondere diejenigen Beziehungen der Objecte zu einander zu, die den Begriff der Grösse, des Maasses, der Zahl involviren.”
The pursuit of mathematical science makes its votary appear singularly indifferent to the ordinary interests and cares of men. Seeking eternal truths, and finding his pleasures in the realities of form and number, he has little interest in the disputes and contentions of the passing hour. His views on social and political questions partake of the grandeur of his favorite contemplations, and, while careful to throw his mite of influence on the side of right and truth, he is content to abide the workings of those general laws by which he doubts not that the fluctuations of human history are as unerringly guided as are the perturbations of the planetary hosts.
In 'Imagination in Mathematics', North American Review, 85, 227.
The question of the origin of the hypothesis belongs to a domain in which no very general rules can be given; experiment, analogy and constructive intuition play their part here. But once the correct hypothesis is formulated, the principle of mathematical induction is often sufficient to provide the proof.
As co-author with Herbert Robbins, in What Is Mathematics?: An Elementary Approach to Ideas and Methods (1941, 1996), 15.
The Reader may here observe the Force of Numbers, which can be successfully applied, even to those things, which one would imagine are subject to no Rules. There are very few things which we know, which are not capable of being reduc’d to a Mathematical Reasoning, and when they cannot, it’s a sign our Knowledge of them is very small and confus’d; and where a mathematical reasoning can be had, it’s as great folly to make use of any other, as to grope for a thing in the dark when you have a Candle standing by you.
From 'Preface' to Of the Laws of Chance, or, a Method of the Hazards of Game (1692), The book was Arbuthnot’s translation of Christiaan Huygen, Tractatus de Rationciniis in Aleae Ludo, in which Huygen expanded on the work of Blaise Pascal in probability and statistics.
The real mathematician is an enthusiast per se. Without enthusiasm no mathematics.
In Schriften (1901), Zweiter Teil, 223.
The real trouble with this world of ours is not that it is an unreasonable world, nor even that it is a reasonable one. The commonest kind of trouble is that it is nearly reasonable, but not quite. … It looks just a little more mathematical and regular than it is; its exactitude is obvious, but its inexactitude is hidden; its wilderness lies in wait.
In Orthodoxy (1908), 148.
The reasoning of mathematics is a type of perfect reasoning.
In Common Sense in Education and Teaching (1905), 222.
The results of mathematics are seldom directly applied; it is the definitions that are really useful. Once you learn the concept of a differential equation, you see differential equations all over, no matter what you do. This you cannot see unless you take a course in abstract differential equations. What applies is the cultural background you get from a course in differential equations, not the specific theorems. If you want to learn French, you have to live the life of France, not just memorize thousands of words. If you want to apply mathematics, you have to live the life of differential equations. When you live this life, you can then go back to molecular biology with a new set of eyes that will see things you could not otherwise see.
In 'A Mathematician's Gossip', Indiscrete Thoughts (2008), 213.
The rudest numerical scales, such as that by which the mineralogists distinguish different degrees of hardness, are found useful. The mere counting of pistils and stamens sufficed to bring botany out of total chaos into some kind of form. It is not, however, so much from counting as from measuring, not so much from the conception of number as from that of continuous quantity, that the advantage of mathematical treatment comes. Number, after all, only serves to pin us down to a precision in our thoughts which, however beneficial, can seldom lead to lofty conceptions, and frequently descend to pettiness.
On the Doctrine of Chances, with Later Reflections (1878), 61-2.
The science [of mathematics] has grown to such vast proportion that probably no living mathematician can claim to have achieved its mastery as a whole.
In An Introduction to Mathematics (1911), 262.
The science of government is my duty. … I must study politics and war that my sons may have liberty to study mathematics and philosophy. My sons ought to study mathematics and philosophy, geography, natural history, naval architecture, navigation, commerce, and agriculture, in order to give their children a right to study painting, poetry, music, architecture, statuary, tapestry, and porcelain.
Letter to Abigail Adams, (1780). In John Adams and Charles Francis Adams, Letters of John Adams, Addressed to His Wife (1841), 68.
The science of mathematics performs more than it promises, but the science of metaphysics promises more than it performs.
Reflection 342, Lacon: Many Things in Few Words (1820), 161-162.
The science of mathematics performs more than it promises…. The study of the mathematics, like the Nile, begins in minuteness, but ends in magnificence.
Reflection 342, in Lacon: or Many things in Few Words; Addressed to Those Who Think (1820), 162.
The science of mathematics presents the most brilliant example of how pure reason may successfully enlarge its domain without the aid of experience.
In Immanuel Kant and F. Max Müller (trans.), 'Method of Transcendentalism', Critique of Pure Reason (1881), Vol. 2, 610.
The science of pure mathematics … may claim to be the most original creation of the human spirit.
In Science and the Modern World: Lowell Lectures, 1925 (1925), 29.
The sciences are taught in following order: morality, arithmetic, accounts, agriculture, geometry, longimetry, astronomy, geomancy, economics, the art of government, physic, logic, natural philosophy, abstract mathematics, divinity, and history.
From Ain-i-Akbery (c.1590). As translated from the original Persian, by Francis Gladwin in 'Akbar’s Conduct and Administrative Rules', 'Regulations For Teaching in the Public Schools', Ayeen Akbery: Or, The Institutes of the Emperor Akber (1783), Vol. 1, 290. Note: Akbar (Akber) was a great ruler; he was an enlightened statesman. He instituted a great system for general education.
The sciences do not try to explain, they hardly even try to interpret, they mainly make models. By a model is meant a mathematical construct which, with the addition of certain verbal interpretations, describes observed phenomena. The justification of such a mathematical construct is solely and precisely that it is expected to work—that is, correctly to describe phenomena from a reasonably wide area. Furthermore, it must satisfy certain esthetic criteria—that is, in relation to how much it describes, it must be rather simple.
From 'Method in the Physical Sciences', in John von Neumann and L. Leary (ed.), The Unity of Knowledge (1955), 158. Reprinted in John Von Neumann, F. Bródy (ed.) and Tibor Vámos (ed.), The Neumann Compendium (2000), 628.
The sciences, even the best,—mathematics and astronomy,—are like sportsmen, who seize whatever prey offers, even without being able to make any use of it.
Emerson's Complete Works (1883),62.
The second [argument about motion] is the so-called Achilles, and it amounts to this, that in a race the quickest runner can never overtake the slowest, since the pursuer must first reach the point whence the pursued started, so that the slower must always hold a lead.
Statement of the Achilles and the Tortoise paradox in the relation of the discrete to the continuous.; perhaps the earliest example of the reductio ad absurdum method of proof.
Statement of the Achilles and the Tortoise paradox in the relation of the discrete to the continuous.; perhaps the earliest example of the reductio ad absurdum method of proof.
— Zeno
Aristotle, Physics, 239b, 14-6. In Jonathan Barnes (ed.), The Complete Works of Aristotle (1984), Vol. 1, 404.
The seventeenth century witnessed the birth of modern science as we know it today. This science was something new, based on a direct confrontation of nature by experiment and observation. But there was another feature of the new science—a dependence on numbers, on real numbers of actual experience.
From The Triumph of Numbers: How Counting Shaped Modern Life (2005), 37.
The smallest particles of matter were said [by Plato] to be right-angled triangles which, after combining in pairs, ... joined together into the regular bodies of solid geometry; cubes, tetrahedrons, octahedrons and icosahedrons. These four bodies were said to be the building blocks of the four elements, earth, fire, air and water ... [The] whole thing seemed to be wild speculation. ... Even so, I was enthralled by the idea that the smallest particles of matter must reduce to some mathematical form ... The most important result of it all, perhaps, was the conviction that, in order to interpret the material world we need to know something about its smallest parts.
[Recalling how as a teenager at school, he found Plato's Timaeus to be a memorable poetic and beautiful view of atoms.]
[Recalling how as a teenager at school, he found Plato's Timaeus to be a memorable poetic and beautiful view of atoms.]
In Werner Heisenberg and A.J. Pomerans (trans.) The Physicist's Conception of Nature (1958), 58-59. Quoted in Jagdish Mehra and Helmut Rechenberg, The Historical Development of Quantum Theory (2001), Vol. 2, 12. Cited in Mauro Dardo, Nobel Laureates and Twentieth-Century Physics (2004), 178.
The so-called Pythagoreans applied themselves to mathematics, and were the first to develop this science; and through studying it they came to believe that its principles are the principles of everything.
In Metaphysics, 1-985b, as translated by Hugh Tredennick (1933). Also seen translated as, “The so-called Pythagoreans, who were the first to take up mathematics, not only advanced this subject, but saturated with it, they fancied that the principles of mathematics were the principles of all things.”
The social sciences mathematically developed are to be the controlling factors in civilization.
In A Scrap-book of Elementary Mathematics: Notes, Recreations, Essays (1908), 208.
The solution of fallacies, which give rise to absurdities, should be to him who is not a first beginner in mathematics an excellent means of testing for a proper intelligible insight into mathematical truth, of sharpening the wit, and of confining the judgment and reason within strictly orderly limits
In 'Vorwort', Mathematische Sophismen (1864), 3. As translated and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-Book (1914), 89. From the original German, “Das Aufsuchen der Trugschlüsse, durch welche Ungereimtheiten entstellen, dürfte nun für den nicht ganz ersten Anfänger in der Mathematik ein vorzügliches Mittel sein, eine richtige begriffliche Einsicht in die mathematischen Wahrheiten zu erproben, den Verstand zu schärfen und das Urtheilen und Schliessen in streng geregelte Grenzen zu dämmen.”
The solution of problems is one of the lowest forms of mathematical research, … yet its educational value cannot be overestimated. It is the ladder by which the mind ascends into higher fields of original research and investigation. Many dormant minds have been aroused into activity through the mastery of a single problem.
With co-editor J. M. Colaw, Editorial introducing the first issue of The American Mathematical Monthly (Jan 1894), 1, No. 1, 2.
The solution of the difficulties which formerly surrounded the mathematical infinite is probably the greatest achievement of which our age has to boast.
In 'The Study of Mathematics', Philosophical Essays (1910), 77.
The speculative propositions of mathematics do not relate to facts; … all that we are convinced of by any demonstration in the science, is of a necessary connection subsisting between certain suppositions and certain conclusions. When we find these suppositions actually take place in a particular instance, the demonstration forces us to apply the conclusion. Thus, if I could form a triangle, the three sides of which were accurately mathematical lines, I might affirm of this individual figure, that its three angles are equal to two right angles; but, as the imperfection of my senses puts it out of my power to be, in any case, certain of the exact correspondence of the diagram which I delineate, with the definitions given in the elements of geometry, I never can apply with confidence to a particular figure, a mathematical theorem. On the other hand, it appears from the daily testimony of our senses that the speculative truths of geometry may be applied to material objects with a degree of accuracy sufficient for the purposes of life; and from such applications of them, advantages of the most important kind have been gained to society.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 3, 180.
The steady progress of physics requires for its theoretical formulation a mathematics which get continually more advanced. ... it was expected that mathematics would get more and more complicated, but would rest on a permanent basis of axioms and definitions, while actually the modern physical developments have required a mathematics that continually shifts its foundation and gets more abstract. Non-euclidean geometry and noncommutative algebra, which were at one time were considered to be purely fictions of the mind and pastimes of logical thinkers, have now been found to be very necessary for the description of general facts of the physical world. It seems likely that this process of increasing abstraction will continue in the future and the advance in physics is to be associated with continual modification and generalisation of the axioms at the base of mathematics rather than with a logical development of any one mathematical scheme on a fixed foundation.
Introduction to a paper on magnetic monopoles, 'Quantised singularities in the electromagnetic field', Proceedings of the Royal Society of Lonndon (1931), A, 133 60. In Helge Kragh, Dirac: a Scientific Biography (1990), 208.
The student of mathematics often finds it hard to throw off the uncomfortable feeling that his science, in the person of his pencil, surpasses him in intelligence,—an impression which the great Euler confessed he often could not get rid of. This feeling finds a sort of justification when we reflect that the majority of the ideas we deal with were conceived by others, often centuries ago. In a great measure it is really the intelligence of other people that confronts us in science.
In Popular Scientific Lectures (1910), 196.
The student of medicine can no more hope to advance in the mastery of his subject with a loose and careless mind than the student of mathematics. If the laws of abstract truth require such rigid precision from those who study them, we cannot believe the laws of nature require less. On the contrary, they would seem to require more; for the facts are obscure, the means of inquiry imperfect, and in every exercise of the mind there are peculiar facilities to err.
From Address (Oct 1874) delivered at Guy’s Hospital, 'On The Study of Medicine', printed in British Medical journal (1874), 2, 425. Collected in Sir William Withey Gull and Theodore Dyke Acland (ed.), A Collection of the Published Writings of William Withey Gull (1896), 6.
The student should not lose any opportunity of exercising himself in numerical calculation and particularly in the use of logarithmic tables. His power of applying mathematics to questions of practical utility is in direct proportion to the facility which he possesses in computation.
In Study and Difficulties of Mathematics (1902), chap. 12.
The student should read his author with the most sustained attention, in order to discover the meaning of every sentence. If the book is well written, it will endure and repay his close attention: the text ought to be fairly intelligible, even without illustrative examples. Often, far too often, a reader hurries over the text without any sincere and vigorous effort to understand it; and rushes to some example to clear up what ought not to have been obscure, if it had been adequately considered. The habit of scrupulously investigating the text seems to me important on several grounds. The close scrutiny of language is a very valuable exercise both for studious and practical life. In the higher departments of mathematics the habit is indispensable: in the long investigations which occur there it would be impossible to interpose illustrative examples at every stage, the student must therefore encounter and master, sentence by sentence, an extensive and complicated argument.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 67.
The study of geometry is a petty and idle exercise of the mind, if it is applied to no larger system than the starry one. Mathematics should be mixed not only with physics but with ethics; that is mixed mathematics.
A Week on the Concord and Merrimack Rivers (1862), 381-382.
The study of mathematics cannot be replaced by any other activity that will train and develop man’s purely logical faculties to the same level of rationality.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
The study of mathematics is apt to commence in disappointment. … We are told that by its aid the stars are weighed and the billions of molecules in a drop of water are counted. Yet, like the ghost of Hamlet's father, this greatest science eludes the efforts of our mental weapons to grasp it.
Opening of Chap 1, in An Introduction to Mathematics (1911), 7.
The study of mathematics is apt to commence in disappointment. The important applications of the science, the theoretical interest of its ideas, and the logical rigour of its methods all generate the expectation of a speedy introduction to processes of interest. We are told that by its aid the stars are weighed and the billions of molecules in a drop of water are counted. Yet, like the ghost of Hamlet's father, this great science eludes the efforts of our mental weapons to grasp it.
Opening to An Introduction to Mathematics (1911), 7.
The study of mathematics is, if an unprofitable, a perfectly harmless and innocent occupation.
From Inaugural Lecture, Oxford (1920). Recalled in A Mathematician’s Apology (1940, 1967), 74.
The study of mathematics—from ordinary reckoning up to the higher processes—must be connected with knowledge of nature, and at the same time with experience, that it may enter the pupil’s circle of thought.
In Johann Friedrich Herbart, Henry M. Felkin (trans.) and Emmie Felkin (trans.), Letters and Lectures on Education [Felkin] (1898), 117.
The study of the history of mathematics will not make better mathematicians but gentler ones, it will enrich their minds, mellow their hearts, and bring out their finer qualities.
In The Study of the History of Mathematics (1936), 28.
The study of the mathematics is like climbing up a steep and craggy mountain; when once you reach the top, it fully recompenses your trouble, by opening a fine, clear, and extensive prospect.
In Tryon Edwards (ed.), A Dictionary of Thoughts (1908), 337.
The supply of problems in mathematics is inexhaustible, and as soon as one problem is solved numerous others come forth in its place.
From Lecture at Second International Congress of Mathematicians in Paris (1900), 'Mathematische Probleme.' English translation by Mary Winston Newson, 'Mathematical Problems', Bulletin of the American Mathematical Society (Jul 1902), 445.
The teaching of elementary mathematics should be conducted so that the way should be prepared for the building upon them of the higher mathematics. The teacher should always bear in mind and look forward to what is to come after. The pupil should not be taught what may be sufficient for the time, but will lead to difficulties in the future. … I think the fault in teaching arithmetic is that of not attending to general principles and teaching instead of particular rules. … I am inclined to attack Teaching of Mathematics on the grounds that it does not dwell sufficiently on a few general axiomatic principles.
In John Perry (ed.), Discussion on the Teaching of Mathematics (1901), 33. The discussion took place on 14 Sep 1901 at the British Association at Glasgow, during a joint meeting of the mathematics and physics sections with the education section. The proceedings began with an address by John Perry. Professor Hudson was the first speak in the Discussion which followed.
The Theory of Groups is a branch of mathematics in which one does something to something and then compares the result with the result obtained from doing the same thing to something else, or something else to the same thing.
In J.R. Newman (ed.) The World of Mathematics (1956), Vol. 3, 1534.
The theory of probability is the only mathematical tool available to help map the unknown and the uncontrollable. It is fortunate that this tool, while tricky, is extraordinarily powerful and convenient.
The Fractal Geometry of Nature (1977, 1983), 201.
The tool which serves as intermediary between theory and practice, between thought and observation, is mathematics; it is mathematics which builds the linking bridges and gives the ever more reliable forms. From this it has come about that our entire contemporary culture, inasmuch as it is based on the intellectual penetration and the exploitation of nature, has its foundations in mathematics. Already Galileo said: one can understand nature only when one has learned the language and the signs in which it speaks to us; but this language is mathematics and these signs are mathematical figures.
Radio broadcast (8 Sep 1930). As quoted in Michael Fitzgerald and Ioan James, The Mind of the Mathematician (2007), 6-7.
The totality of our so-called knowledge or beliefs, from the most casual matters of geography and history to the profoundest laws of atomic physics or even of pure mathematics and logic, is a man-made fabric which impinges on experience only along the edges. Or, to change the figure, total science is like a field of force whose boundary conditions are experience. A conflict with experience at the periphery occasions readjustments in the interior of the field. Truth values have to be redistributed over some of our statements. Reevaluation of some statements entails reevaluation of others, because of their logical interconnections—the logical laws being in turn simply certain further statements of the system, certain further elements of the field.
'Two Dogmas of Experience,' in Philosophical Review (1951). Reprinted in From a Logical Point of View (1953), 42.
The traditional mathematics professor of the popular legend is absentminded. He usually appears in public with a lost umbrella in each hand. He prefers to face a blackboard and to turn his back on the class. He writes a, he says b, he means c, but it should be d. Some of his sayings are handed down from generation to generation:
“In order to solve this differential equation you look at it till a solution occurs to you.”
“This principle is so perfectly general that no particular application of it is possible.”
“Geometry is the science of correct reasoning on incorrect figures.”
“My method to overcome a difficulty is to go round it.”
“What is the difference between method and device? A method is a device which you used twice.”
“In order to solve this differential equation you look at it till a solution occurs to you.”
“This principle is so perfectly general that no particular application of it is possible.”
“Geometry is the science of correct reasoning on incorrect figures.”
“My method to overcome a difficulty is to go round it.”
“What is the difference between method and device? A method is a device which you used twice.”
In How to Solve It: A New Aspect of Mathematical Method (2004), 208.
The training which mathematics gives in working with symbols is an excellent preparation for other sciences; … the world’s work requires constant mastery of symbols.
In Teaching of Mathematics in the Elementary and the Secondary School (1906), 42.
The transfinite numbers are in a sense the new irrationalities [ ... they] stand or fall with the finite irrational numbers.
Gesammelte Abhandlungen (1932),395, trans. Ivor Grattan-Guinness.
The treatises [of Archimedes] are without exception, monuments of mathematical exposition; the gradual revelation of the plan of attack, the masterly ordering of the propositions, the stern elimination of everything not immediately relevant to the purpose, the finish of the whole, are so impressive in their perfection as to create a feeling akin to awe in the mind of the reader.
In A History of Greek Mathematics (1921), Vol. 1, 20.
The trend of mathematics and physics towards unification provides the physicist with a powerful new method of research into the foundations of his subject. … The method is to begin by choosing that branch of mathematics which one thinks will form the basis of the new theory. One should be influenced very much in this choice by considerations of mathematical beauty. It would probably be a good thing also to give a preference to those branches of mathematics that have an interesting group of transformations underlying them, since transformations play an important role in modern physical theory, both relativity and quantum theory seeming to show that transformations are of more fundamental importance than equations.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 122.
The true spirit of delight, the exaltation, the sense of being more than man, which is the touchstone of highest excellence, is to be found in mathematics as surely as in poetry.
Essay, 'The Study of Mathematics' (1902), collected in Philosophical Essays (1910), 73-74. Also collected in Mysticism and Logic: And Other Essays (1919), 60.
The truth of the matter is that, though mathematics truth may be beauty, it can be only glimpsed after much hard thinking. Mathematics is difficult for many human minds to grasp because of its hierarchical structure: one thing builds on another and depends on it.
As co-author with D.T.E. Marjoram, Mathematics in a Changing World (1973).
The two great components of the critical movement, though distinct in origin and following separate paths, are found to converge at last in the thesis: Symbolic Logic is Mathematics, Mathematics is Symbolic Logic, the twain are one.
In Lecture delivered at Columbia University (16 Oct 1907), 'Mathematics', the first of a series published as Lectures on Science, Philosophy and Art (1908), 19.
The union of philosophical and mathematical productivity, which besides in Plato we find only in Pythagoras, Descartes and Leibnitz, has always yielded the choicest fruits to mathematics; To the first we owe scientific mathematics in general, Plato discovered the analytic method, by means of which mathematics was elevated above the view-point of the elements, Descartes created the analytical geometry, our own illustrious countryman discovered the infinitesimal calculus—and just these are the four greatest steps in the development of mathematics.
In Geschichte der Mathematik im Altertum und im Mittelalter (1874), 149-150. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 210. From the original German, “Die Verbindung philosophischer und mathematischer Productivität, wie wir sie ausser in Platon wohl nur noch in Pythagoras, Descartes, Leibnitz vorfinden, hat der Mathematik immer die schönsten Früchte gebracht: Ersterem verdanken wir die wissenschaftliche Mathematik überhaupt, Platon erfand die analytische Methode, durch welche sich die Mathematik über den Standpunct der Elemente erhob, Descartes schuf die analytische Geometrie, unser berühmter Landsmann den Infinitesimalcalcül—und eben daß sind die vier grössten Stufen in der Entwickelung der Mathematik.”
The universe is made of particles and fields about which nothing can be said except to describe their mathematical structures. In a sense, the entire universe is made of mathematics. If the particles and fields are not made of mathematical structure, then please tell me what you think they are made of!
As quoted in Kendrick Frazier, 'A Mind at Play: An Interview with Martin Gardner', Skeptical Inquirer (Mar/Apr 1998), 22, No. 2, 39.
The universe…cannot be read until we have learnt the language and become familiar with the characters in which it is written. It is written in mathematical language, and the letters are triangles, circles and other geometrical figures, without which means it is humanly impossible to comprehend a single word.
From Opere Il Saggiatore (1623), Sec. 6. (Title in English, The Assayer.) As translated in D. D. Raphael, Hobbes: Morals and Politics (2014), Vol. 6, 19.
The unreasonable efficiency of mathematics in science is a gift we neither understand nor deserve.
Quoted in Robert J. Scully, The Demon and the Quantum (2007), 191.
The usefulness of mathematics in furthering the sciences is commonly acknowledged: but outside the ranks of the experts there is little inquiry into its nature and purpose as a deliberate human activity. Doubtless this is due to the inevitable drawback that mathematical study is saturated with technicalities from beginning to end.
Opening remark in Preface to The Great Mathmaticians (1929). Collected in James Newman, The World of Mathematics (1956), Vol. 1, 75.
The validity of mathematical propositions is independent of the actual world—the world of existing subject-matters—is logically prior to it, and would remain unaffected were it to vanish from being. Mathematical propositions, if true, are eternal verities.
In The Pastures of Wonder: The Realm of Mathematics and the Realm of Science (1929), 99.
The value of mathematical instruction as a preparation for those more difficult investigations, consists in the applicability not of its doctrines but of its methods. Mathematics will ever remain the past perfect type of the deductive method in general; and the applications of mathematics to the simpler branches of physics furnish the only school in which philosophers can effectually learn the most difficult and important of their art, the employment of the laws of simpler phenomena for explaining and predicting those of the more complex. These grounds are quite sufficient for deeming mathematical training an indispensable basis of real scientific education, and regarding with Plato, one who is … as wanting in one of the most essential qualifications for the successful cultivation of the higher branches of philosophy
In System of Logic, Bk. 3, chap. 24, sect. 9.
The whole of Mathematics consists in the organization of a series of aids to the imagination in the process of reasoning.
In Universal Algebra (1898), 12.
The word “definition” has come to have a dangerously reassuring sound, owing no doubt to its frequent occurrence in logical and mathematical writings.
In 'Two Dogmas of Empiricism', From a Logical Point of View: Nine Logico-Philosophical Essays (1953, 1961), 26.
The word “mathematics” is a Greek word and, by origin, it means “something that has been learned or understood,” or perhaps “acquired knowledge,” or perhaps even, somewhat against grammar, “acquirable knowledge,” that is, “learnable knowledge,” that is, “knowledge acquirable by learning.”
'Why Mathematics Grows', Journal of the History of Ideas (Jan-Mar 1965), 26, No. 1, 4. In Salomon Bochner and Robert Clifford Gunning (ed.) Collected Papers of Salomon Bochner (1992), Vol. 4, 192.
The works of Lavoisier and his associates operated upon many of us at that time like the Sun's rising after a night of moonshine: but Chemistry is now betrothed to the Mathematics, and is in consequence grown somewhat shy of her former admirers.
In Luke Howard, Johann Wolfgang von Goethe and D.F.S. Scott (ed.), Luke Howard (1772-1864): His Correspondence with Goethe and his Continental Journey of 1816(1976), 2.
The world is anxious to admire that apex and culmination of modern mathematics: a theorem so perfectly general that no particular application of it is feasible.
In 'A Story With a Moral', Mathematical Gazette (Jun 1973), 57, No. 400, 87.
The world looks like a multiplication-table, or a mathematical equation, which, turn it how you will, balances itself.
From 'Compensation', collected in The Complete Works of Ralph Waldo Emerson (1903), 102.
The world of ideas which it [mathematics] discloses or illuminates, the contemplation of divine beauty and order which it induces, the harmonious connexion of its parts, the infinite hierarchy and absolute evidence of the truths with which it is concerned, these, and such like, are the surest grounds of the title of mathematics to human regard, and would remain unimpeached and unimpaired were the plan of the universe unrolled like a map at our feet, and the mind of man qualified to take in the whole scheme of creation at a glance.
In Presidential Address to British Association (19 Aug 1869), 'A Plea for the Mathematician', published in Nature (6 Jan 1870), 1, 262. Collected in Collected Mathematical Papers (1908), Vol. 2, 659.
The world of mathematics and theoretical physics is hierarchical. That was my first exposure to it. There's a limit beyond which one cannot progress. The differences between the limiting abilities of those on successively higher steps of the pyramid are enormous. I have not seen described anywhere the shock a talented man experiences when he finds, late in his academic life, that there are others enormously more talented than he. I have personally seen more tears shed by grown men and women over this discovery than I would have believed possible. Most of those men and women shift to fields where they can compete on more equal terms
Alvarez: Adventures of a Physicist (1987), 20.
The world of mathematics, which you condemn, is really a beautiful world; it has nothing to do with life and death and human sordidness, but is eternal, cold and passionless. To me, pure, mathematics is one of the highest forms of art; it has a sublimity quite special to itself, and an immense dignity derived, from the fact that its world is exempt I, from change and time. I am quite serious in this. The only difficulty is that none but mathematicians can enter this enchanted region, and they hardly ever have a sense of beauty. And mathematics is the only thing we know of that is capable of perfection; in thinking about it we become Gods.
Letter to Helen Thomas (30 Dec 1901). Quoted in Nicholas Griffin (ed.), The Selected Letters of Bertrand Russell (1992), Vol. 1, 224.
Theology, Mr. Fortune found, is a more accommodating subject than mathematics; its technique of exposition allows greater latitude. For instance when you are gravelled for matter there is always the moral to fall back upon. Comparisons too may be drawn, leading cases cited, types and antetypes analysed and anecdotes introduced. Except for Archimedes mathematics is singularly naked of anecdotes.
In Mr. Fortune’s Maggot (1927), 168.
Theorems are not to mathematics what successful courses are to a meal.
In Rota's 'Introduction' written (1980) to preface Philip J. Davis and Reuben Hersh, The Mathematical Experience (1981, 2012), xxii-xxiii.
Theoretical physicists accept the need for mathematical beauty as an act of faith... For example, the main reason why the theory of relativity is so universally accepted is its mathematical beauty.
'Methods in Theoretical Physics', From A Life of Physics: Evening Lectures at the International Centre for Theoretical Physics, Trieste, Italy. A Special Supplement of the IAEA Bulletin (1968), 22.
There are diverse views as to what makes a science, but three constituents will be judged essential by most, viz: (1) intellectual content, (2) organization into an understandable form, (3) reliance upon the test of experience as the ultimate standard of validity. By these tests, mathematics is not a science, since its ultimate standard of validity is an agreed-upon sort of logical consistency and provability.
In 'The Future of Data Analysis', Annals of Mathematical Statistics (1962), 33, No. 1, 5-6.
There are few humanities that could surpass in discipline, in beauty, in emotional and aesthetic satisfaction, those humanities which are called mathematics, and the natural sciences.
'Scientist and Citizen', Speech to the Empire Club of Canada (29 Jan 1948), The Empire Club of Canada Speeches (29 Jan 1948), 209-221.
There are four great sciences, without which the other sciences cannot be known nor a knowledge of things secured … Of these sciences the gate and key is mathematics … He who is ignorant of this [mathematics] cannot know the other sciences nor the affairs of this world.
Opus Majus [1266-1268], Part IV, distinction I, chapter I, trans. R. B. Burke, The Opus Majus of Roger Bacon (1928), Vol. I, 116.
There are many arts and sciences of which a miner should not be ignorant. First there is Philosophy, that he may discern the origin, cause, and nature of subterranean things; for then he will be able to dig out the veins easily and advantageously, and to obtain more abundant results from his mining. Secondly there is Medicine, that he may be able to look after his diggers and other workman ... Thirdly follows astronomy, that he may know the divisions of the heavens and from them judge the directions of the veins. Fourthly, there is the science of Surveying that he may be able to estimate how deep a shaft should be sunk … Fifthly, his knowledge of Arithmetical Science should be such that he may calculate the cost to be incurred in the machinery and the working of the mine. Sixthly, his learning must comprise Architecture, that he himself may construct the various machines and timber work required underground … Next, he must have knowledge of Drawing, that he can draw plans of his machinery. Lastly, there is the Law, especially that dealing with metals, that he may claim his own rights, that he may undertake the duty of giving others his opinion on legal matters, that he may not take another man’s property and so make trouble for himself, and that he may fulfil his obligations to others according to the law.
In De Re Metallica (1556), trans. H.C. and L.H. Hoover (1950), 3-4.
There are notable examples enough of demonstration outside of mathematics, and it may be said that Aristotle has already given some in his “Prior Analytics.” In fact logic is as susceptible of demonstration as geometry, … Archimedes is the first, whose works we have, who has practised the art of demonstration upon an occasion where he is treating of physics, as he has done in his book on Equilibrium. Furthermore, jurists may be said to have many good demonstrations; especially the ancient Roman jurists, whose fragments have been preserved to us in the Pandects.
In G.W. Leibniz and Alfred Gideon Langley (trans.), New Essay on Human Understanding (1896), Bk. 4, Chap. 2, Sec. 9, 414-415.
There are only two kinds of math books. Those you cannot read beyond the first sentence, and those you cannot read beyond the first page.
Attributed, but without reference. For example, in John Mitchinson, John Lloyd, If Ignorance Is Bliss, Why Aren't There More Happy People? (2009), 31. If you know the primary source, please contact Webmaster.
There are problems to whose solution I would attach an infinitely greater importance than to those of mathematics, for example touching ethics, or our relation to God, or concerning our destiny and our future; but their solution lies wholly beyond us and completely outside the province of science.
Quoted in J.R. Newman, The World of Mathematics (1956), 314.
There are several kinds of truths, and it is customary to place in the first order mathematical truths, which are, however, only truths of definition. These definitions rest upon simple, but abstract, suppositions, and all truths in this category are only constructed, but abstract, consequences of these definitions ... Physical truths, to the contrary, are in no way arbitrary, and do not depend on us.
'Premier Discours: De la Manière d'Étudier et de Traiter l'Histoire naturelle', Histoire Naturelle, Generale et Particulière, Avec la Description du Cabinet du Roi (1749), Vol. I, 53-4. Trans. Phillip R. Sloan.
There are then two kinds of intellect: the one able to penetrate acutely and deeply into the conclusions of given premises, and this is the precise intellect; the other able to comprehend a great number of premises without confusing them, and this is the mathematical intellect. The one has force and exactness, the other comprehension. Now the one quality can exist without the other; the intellect can be strong and narrow, and can also be comprehensive and weak.
In Pascal’s Pensées (1958), 3.
There are things which seem incredible to most men who have not studied mathematics.
As given, without source, in epigraph, Herbert Meschkowski Ways of Thought of Great Mathematicians: An Approach to the History of Mathematics (1964), 13.
There are three ruling ideas, three so to say, spheres of thought, which pervade the whole body of mathematical science, to some one or other of which, or to two or all three of them combined, every mathematical truth admits of being referred; these are the three cardinal notions, of Number, Space and Order.
Arithmetic has for its object the properties of number in the abstract. In algebra, viewed as a science of operations, order is the predominating idea. The business of geometry is with the evolution of the properties of space, or of bodies viewed as existing in space.
Arithmetic has for its object the properties of number in the abstract. In algebra, viewed as a science of operations, order is the predominating idea. The business of geometry is with the evolution of the properties of space, or of bodies viewed as existing in space.
In 'A Probationary Lecture on Geometry, York British Association Report (1844), Part 2; Collected Mathematical Papers, Vol. 2, 5.
There are two types of mind … the mathematical, and what might be called the intuitive. The former arrives at its views slowly, but they are firm and rigid; the latter is endowed with greater flexibility and applies itself simultaneously to the diverse lovable parts of that which it loves.
In Discours sur les passions de l’amour (1653).
There are, at present, fundamental problems in theoretical physics … the solution of which … will presumably require a more drastic revision of our fundmental concepts than any that have gone before. Quite likely, these changes will be so great that it will be beyond the power of human intelligence to get the necessary new ideas by direct attempts to formulate the experimental data in mathematical terms. The theoretical worker in the future will, therefore, have to proceed in a more direct way. The most powerful method of advance that can be suggested at present is to employ all the resources of pure mathematics in attempts to perfect and generalize the mathematical formalism that forms the existing basis of theoretical physics, and after each success in this direction, to try to interpret the new mathematical features in terms of physical entities.
At age 28.
At age 28.
Proceedings of the Royal Society (1931), A133, 60. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 109.
There can be but one opinion as to the beauty and utility of this analysis of Laplace; but the manner in which it has been hitherto presented has seemed repulsive to the ablest mathematicians, and difficult to ordinary mathematical students.[Co-author with Peter Guthrie Tait.]
In William Thomson Baron Kelvin, Peter Guthrie Tait, Treatise on Natural Philosophy (1879), Vol. 1, Preface, vii.
There exists a passion for comprehension, just as there exists a passion for music. That passion is rather common in children but gets lost in most people later on. Without this passion, there would be neither mathematics nor natural science.
'On the Generalized Theory of Gravitation', Scientific American (Apr 1950). Collected in David H. Levy (ed.), The Scientific American Book of the Cosmos (2000), 13.
There exists, if I am not mistaken, an entire world which is the totality of mathematical truths, to which we have access only with our mind, just as a world of physical reality exists, the one like the other independent of ourselves, both of divine creation.
As quoted, without citation, in the original French, “Il existe, si je ne me trompe, tout un monde qui est l'ensemble des vérités mathématiques, dans lequel nous n’avons accès que par l'intelligence, comme existe le monde des réalités physiques; l’un et l’autre indépendants de nous, tous deux de création divine,” in Gaston Darboux, 'La Vie et l’Oeuvre de Charles Hermite', La Revue du Mois (10 Jan 1906), 46. As translated in Armand Borel, 'On the Place of Mathematics in Culture', in Armand Borel: Œvres: Collected Papers (1983), Vol. 4, 428.
There has come about a general public awareness that America is not automatically, and effortlessly, and unquestionably the leader of the world in science and technology. This comes as no surprise to those of us who have watched and tried to warn against the steady deterioration in the teaching of science and mathematics in the schools for the past quarter century. It comes as no surprise to those who have known of dozens of cases of scientists who have been hounded out of jobs by silly disloyalty charges, and kept out of all professional employment by widespread blacklisting practices.
Banquet speech at American Physical Society, St. Louis, Missouri. (29 Nov 1957). In "Time to Stop Baiting Scientists", Bulletin of the Atomic Scientists (Feb 1958), 80.
There has not been any science so much esteemed and honored as this of mathematics, nor with so much industry and vigilance become the care of great men, and labored in by the potentates of the world, viz. emperors, kings, princes, etc.
In 'On the Usefulness of Mathematics', in Works (1840), Vol. 2, 28.
There have been many authorities who have asserted that the basis of science lies in counting or measuring, i.e. in the use of mathematics. Neither counting nor measuring can however be the most fundamental processes in our study of the material universe—before you can do either to any purpose you must first select what you propose to count or measure, which presupposes a classification.
Classification and Biology (1970), 2.
There is a certain way of searching for the truth in mathematics that Plato is said first to have discovered. Theon called this analysis.
…...
There is a noble vision of the great Castle of Mathematics, towering somewhere in the Platonic World of Ideas, which we humbly and devotedly discover (rather than invent). The greatest mathematicians manage to grasp outlines of the Grand Design, but even those to whom only a pattern on a small kitchen tile is revealed, can be blissfully happy. … Mathematics is a proto-text whose existence is only postulated but which nevertheless underlies all corrupted and fragmentary copies we are bound to deal with. The identity of the writer of this proto-text (or of the builder of the Castle) is anybody’s guess. …
In 'Mathematical Knowledge: Internal, Social, and Cultural Aspects', Mathematics As Metaphor: Selected Essays (2007), 4.
There is a science which investigates being as being and the attributes which belong to this in virtue of its own nature. Now this is not the same as any of the so-called special sciences; for none of these treats universally of being as being. They cut off a part of being and investigate the attribute of this part; this is what the mathematical sciences for instance do. Now since we are seeking the first principles and the highest causes, clearly there must be some thing to which these belong in virtue of its own nature. If then those who sought the elements of existing things were seeking these same principles, it is necessary that the elements must be elements of being not by accident but just because it is being. Therefore it is of being as being that we also must grasp the first causes.
'Book Gamma (1003a17-1011b23' in Metaphysics, trans. W.D. Ross (1924). Excerpt 'Being Qua Being', in Joseph Margolis and Jacques Catudal, The Quarrel between Invariance and Flux (2001), 18-19.
There is a strange disparity between the sciences of inert matter and those of life. Astronomy, mechanics, and physics are based on concepts which can be expressed, tersely and elegantly, in mathematical language. They have built up a universe as harmonious as the monuments of ancient Greece. They weave about it a magnificent texture of calculations and hypotheses. They search for reality beyond the realm of common thought up to unutterable abstractions consisting only of equations of symbols. Such is not the position of biological sciences. Those who investigate the phenomena of life are as if lost in an inextricable jungle, in the midst of a magic forest, whose countless trees unceasingly change their place and their shape. They are crushed under a mass of facts, which they can describe but are incapable of defining in algebraic equations.
Man the Unknown (1935), 1.
There is an astonishing imagination, even in the science of mathematics. … We repeat, there was far more imagination in the head of Archimedes than in that of Homer.
In A Philosophical Dictionary: from the French (1824), 126.
There is beauty in discovery. There is mathematics in music, a kinship of science and poetry in the description of nature, and exquisite form in a molecule. Attempts to place different disciplines in different camps are revealed as artificial in the face of the unity of knowledge. All illiterate men are sustained by the philosopher, the historian, the political analyst, the economist, the scientist, the poet, the artisan, and the musician.
From address (1958), upon being appointed Chancellor of the University of California.
There is inherent in nature a hidden harmony that reflects itself in our minds under the image of simple mathematical laws. That then is the reason why events in nature are predictable by a combination of observation and mathematical analysis. Again and again in the history of physics this conviction, or should I say this dream, of harmony in nature has found fulfillments beyond our expectations.
…...
There is no branch of mathematics, however abstract, which may not some day be applied to phenomena of the real world.
As quoted, without source, in D’Arcy Wentworth Thompson, On Growth and Form (1942), Vol. 1, 10. If you know the primary source, please contact Webmaster.
There is no certainty where one can neither apply any of the mathematical sciences nor any of those which are based upon the mathematical sciences.
(G 96 v.) In Edward McCurdy (ed., trans.), Leonardo da Vinci’s Note-Books: Arranged and Rendered into English (1908), 54. Also translated elsewhere as, “No knowledge can be certain if it is not based upon mathematics or upon some other knowledge which is itself based upon the mathematical sciences.”
There is no more common error than to assume that, because prolonged and accurate mathematical calculations have been made, the application of the result to some fact of nature is absolutely certain.
In An Introduction to Mathematics (1911), 27.
There is no national science, just as there is no national multiplication table; what is national is no longer science.
In Anton Chekhov, S. S. Koteliansky (trans.) and Leonard Woolf (trans.), Note-Book of Anton Chekhov (1921), 18.
There is no prophet which preaches the superpersonal God more plainly than mathematics.
In 'Reflections on Magic Squares', Monist (1906), 147.
There is no science which teaches the harmonies of nature more clearly than mathematics.
In Andrews, Magic Squares and Cubes (1908), Introduction.
There is no study in the world which brings into more harmonious action all the faculties of the mind than [mathematics], … or, like this, seems to raise them, by successive steps of initiation, to higher and higher states of conscious intellectual being.
In Presidential Address to British Association (19 Aug 1869), 'A Plea for the Mathematician', published in Nature (6 Jan 1870), 1, 261.
There is no thing as a man who does not create mathematics and yet is a fine mathematics teacher. Textbooks, course material—these do not approach in importance the communication of what mathematics is really about, of where it is going, and of where it currently stands with respect to the specific branch of it being taught. What really matters is the communication of the spirit of mathematics. It is a spirit that is active rather than contemplative—a spirit of disciplined search for adventures of the intellect. Only as adventurer can really tell of adventures.
Reflections: Mathematics and Creativity', New Yorker (1972), 47, No. 53, 39-45. In Douglas M. Campbell, John C. Higgins (eds.), Mathematics: People, Problems, Results (1984), Vol. 2, 7.
There is no way to guarantee in advance what pure mathematics will later find application. We can only let the process of curiosity and abstraction take place, let mathematicians obsessively take results to their logical extremes, leaving relevance far behind, and wait to see which topics turn out to be extremely useful. If not, when the challenges of the future arrive, we won’t have the right piece of seemingly pointless mathematics to hand.
In 'The Unplanned Impact of Mathematics', Nature (14 Jul 2011), 475, No. 7355, 167.
There is not wholly unexpected surprise, but surprise nevertheless, that mathematics has direct application to the physical world about us.
In The American Mathematical Monthly (1949), 56, 19. Excerpted in John Ewing (ed,), A Century of Mathematics: Through the Eyes of the Monthly (1996), 186.
There is nothing mysterious, as some have tried to maintain, about the applicability of mathematics. What we get by abstraction from something can be returned.
In Introduction to the Foundations of Mathematics (1952), 175.
There is plenty of room left for exact experiment in art, and the gate has been opened for some time. What had been accomplished in music by the end of the eighteenth century has only begun in the fine arts. Mathematics and physics have given us a clue in the form of rules to be strictly observed or departed from, as the case may be. Here salutary discipline is come to grips first of all with the function of forms, and not with form as the final result … in this way we learn how to look beyond the surface and get to the root of things.
Quoted in The Bulletin of the Atomic Scientists (Feb 1959), 59, citing Bauhaus-Zeitschrijt (1928).
There is probably no other science which presents such different appearances to one who cultivates it and to one who does not, as mathematics. To this person it is ancient, venerable, and complete; a body of dry, irrefutable, unambiguous reasoning. To the mathematician, on the other hand, his science is yet in the purple bloom of vigorous youth, everywhere stretching out after the “attainable but unattained” and full of the excitement of nascent thoughts; its logic is beset with ambiguities, and its analytic processes, like Bunyan’s road, have a quagmire on one side and a deep ditch on the other and branch off into innumerable by-paths that end in a wilderness.
In 'The Theory of Transformation Groups', (A review of Erster Abschnitt, Theorie der Transformationsgruppen (1888)), Bulletin New York Mathematical Society (1893), 2 (First series), 61.
There is thus a possibility that the ancient dream of philosophers to connect all Nature with the properties of whole numbers will some day be realized. To do so physics will have to develop a long way to establish the details of how the correspondence is to be made. One hint for this development seems pretty obvious, namely, the study of whole numbers in modern mathematics is inextricably bound up with the theory of functions of a complex variable, which theory we have already seen has a good chance of forming the basis of the physics of the future. The working out of this idea would lead to a connection between atomic theory and cosmology.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 129.
There was a young fellow from Trinity,
Who took the square root of infinity.
But the number of digits,
Gave him the fidgets;
He dropped Math and took up Divinity.
Who took the square root of infinity.
But the number of digits,
Gave him the fidgets;
He dropped Math and took up Divinity.
Epigraph on title page of One, Two, Three… Infinity: Facts and Speculations of Science (1947, 1988), i. The original text shows symbols instead of the words which appear above as “square root of infinity.”
There was, I think, a feeling that the best science was that done in the simplest way. In experimental work, as in mathematics, there was “style” and a result obtained with simple equipment was more elegant than one obtained with complicated apparatus, just as a mathematical proof derived neatly was better than one involving laborious calculations. Rutherford's first disintegration experiment, and Chadwick's discovery of the neutron had a “style” that is different from that of experiments made with giant accelerators.
From 'Physics in a University Laboratory Before and After World War II', Proceedings of the Royal Society of London, Series A, (1975), 342, 463. As cited in Alan McComas, Galvani's Spark: The Story of the Nerve Impulse (2011), 107.
There’s a fine line between a numerator and a denominator. Only a fraction of people know this.
Therefore O students study mathematics and do not build without foundations.
…...
Therefore on long pondering this uncertainty of mathematical traditions on the deduction of the motions of the system of the spheres, I began to feel disgusted that no more certain theory of the motions of the mechanisms of the universe, which has been established for us by the best and most systematic craftsman of all, was agreed by the philosophers, who otherwise theorised so minutely with most careful attention to the details of this system. I therefore set myself the task of reading again the books of all philosophers which were available to me, to search out whether anyone had ever believed that the motions of the spheres of the, universe were other than was supposed by those who professed mathematics in the schools.
'To His Holiness Pope Paul III', in Copernicus: On the Revolutions of the Heavenly Spheres (1543), trans. A. M. Duncan (1976), 25.
These Disciplines [mathematics] serve to inure and corroborate the Mind to a constant Diligence in Study; to undergo the Trouble of an attentive Meditation, and cheerfully contend with such Difficulties as lie in the Way. They wholly deliver us from a credulous Simplicity, most strongly fortify us against the Vanity of Scepticism, effectually restrain from a rash Presumption, most easily incline us to a due Assent, perfectly subject us to the Government of right Reason, and inspire us with Resolution to wrestle against the unjust Tyranny of false Prejudices. If the Fancy be unstable and fluctuating, it is to be poized by this Ballast, and steadied by this Anchor, if the Wit be blunt it is sharpened upon this Whetstone; if luxuriant it is pared by this Knife; if headstrong it is restrained by this Bridle; and if dull it is rouzed by this Spur. The Steps are guided by no Lamp more clearly through the dark Mazes of Nature, by no Thread more surely through the intricate Labyrinths of Philosophy, nor lastly is the Bottom of Truth sounded more happily by any other Line. I will not mention how plentiful a Stock of Knowledge the Mind is furnished from these, with what wholesome Food it is nourished, and what sincere Pleasure it enjoys. But if I speak farther, I shall neither be the only Person, nor the first, who affirms it; that while the Mind is abstracted and elevated from sensible Matter, distinctly views pure Forms, conceives the Beauty of Ideas, and investigates the Harmony of Proportions; the Manners themselves are sensibly corrected and improved, the Affections composed and rectified, the Fancy calmed and settled, and the Understanding raised and excited to more divine Contemplations. All which I might defend by Authority, and confirm by the Suffrages of the greatest Philosophers.
Prefatory Oration in Mathematical Lectures (1734), xxxi.
These estimates may well be enhanced by one from F. Klein (1849-1925), the leading German mathematician of the last quarter of the nineteenth century. “Mathematics in general is fundamentally the science of self-evident things.” ... If mathematics is indeed the science of self-evident things, mathematicians are a phenomenally stupid lot to waste the tons of good paper they do in proving the fact. Mathematics is abstract and it is hard, and any assertion that it is simple is true only in a severely technical sense—that of the modern postulational method which, as a matter of fact, was exploited by Euclid. The assumptions from which mathematics starts are simple; the rest is not.
Mathematics: Queen and Servant of Science (1952),19-20.
These specimens, which I could easily multiply, may suffice to justify a profound distrust of Auguste Comte, wherever he may venture to speak as a mathematician. But his vast general ability, and that personal intimacy with the great Fourier, which I most willingly take his own word for having enjoyed, must always give an interest to his views on any subject of pure or applied mathematics.
In R. Graves, Life of W. R. Hamilton (1882-89), Vol. 3, 475.
This [the fact that the pursuit of mathematics brings into harmonious action all the faculties of the human mind] accounts for the extraordinary longevity of all the greatest masters of the Analytic art, the Dii Majores of the mathematical Pantheon. Leibnitz lived to the age of 70; Euler to 76; Lagrange to 77; Laplace to 78; Gauss to 78; Plato, the supposed inventor of the conic sections, who made mathematics his study and delight, who called them the handles or aids to philosophy, the medicine of the soul, and is said never to have let a day go by without inventing some new theorems, lived to 82; Newton, the crown and glory of his race, to 85; Archimedes, the nearest akin, probably, to Newton in genius, was 75, and might have lived on to be 100, for aught we can guess to the contrary, when he was slain by the impatient and ill mannered sergeant, sent to bring him before the Roman general, in the full vigour of his faculties, and in the very act of working out a problem; Pythagoras, in whose school, I believe, the word mathematician (used, however, in a somewhat wider than its present sense) originated, the second founder of geometry, the inventor of the matchless theorem which goes by his name, the pre-cognizer of the undoubtedly mis-called Copernican theory, the discoverer of the regular solids and the musical canon who stands at the very apex of this pyramid of fame, (if we may credit the tradition) after spending 22 years studying in Egypt, and 12 in Babylon, opened school when 56 or 57 years old in Magna Græcia, married a young wife when past 60, and died, carrying on his work with energy unspent to the last, at the age of 99. The mathematician lives long and lives young; the wings of his soul do not early drop off, nor do its pores become clogged with the earthy particles blown from the dusty highways of vulgar life.
In Presidential Address to the British Association, Collected Mathematical Papers, Vol. 2 (1908), 658.
This conviction of the solvability of every mathematical problem is a powerful incentive to the worker. We hear within us the perpetual call: There is the problem. Seek its solution. You can find it by pure reason, for in mathematics there is no ignorabimus!
Ignorabimus as used here, means “we will not know”, in the future (which is slightly different from ignoramus meaning ignorance in the present, “we do not know”). In Lecture (1900), 'Mathematische Probleme' (Mathematical Problems), to the International Congress of Mathematicians, Paris. From the original German reprinted in David Hilbert: Gesammelte Abhandlungen (Collected Treatises, 1970), Vol. 3, 298, “Diese Überzeugung von der Lösbarkeit eines jeden mathematischer Problems ist uns ein kräftiger Ansporn während der Arbeit ; wir hören in uns den steten Zuruf: Da ist das Problem, suche die Lösung. Du kannst sie durch reines Denken finden; denn in der Mathematik gibt es kein Ignorabimus. English version as translated by Dr. Maby Winton Newson for Bulletin of the American Mathematical Society (1902), 8, 437-479. The address was first published in Göttinger Nachrichten is Nachrichten von der Königl. Gesellschaft der Wiss. zu Göttingen (1900), 253-297; and Archiv der Mathematik und Physik (1901), 3, No. 1, 44-63.
This property of human languages—their resistance to algorithmic processing— is perhaps the ultimate reason why only mathematics can furnish an adequate language for physics. It is not that we lack words for expressing all this E = mc² and ∫eiS(Φ)DΦ … stuff … , the point is that we still would not be able to do anything with these great discoveries if we had only words for them. … Miraculously, it turns out that even very high level abstractions can somehow reflect reality: knowledge of the world discovered by physicists can be expressed only in the language of mathematics.
In 'Mathematical Knowledge: Internal, Social, And Cultural Aspects', Mathematics As Metaphor: Selected Essays (2007), 5.
This quality of genius is, sometimes, difficult to be distinguished from talent, because high genius includes talent. It is talent, and something more. The usual distinction between genius and talent is, that one represents creative thought, the other practical skill: one invents, the other applies. But the truth is, that high genius applies its own inventions better than talent alone can do. A man who has mastered the higher mathematics, does not, on that account, lose his knowledge of arithmetic. Hannibal, Napoleon, Shakespeare, Newton, Scott, Burke, Arkwright, were
they not men of talent as well as men of genius?
In 'Genius', Wellman’s Miscellany (Dec 1871), 4, No. 6, 203.
This relation logical implication is probably the most rigorous and powerful of all the intellectual enterprises of man. From a properly selected set of the vast number of prepositional functions a set can be selected from which an infinitude of prepositional functions can be implied. In this sense all postulational thinking is mathematics. It can be shown that doctrines in the sciences, natural and social, in history, in jurisprudence and in ethics are constructed on the postulational thinking scheme and to that extent are mathematical. Together the proper enterprise of Science and the enterprise of Mathematics embrace the whole knowledge-seeking activity of mankind, whereby “knowledge” is meant the kind of knowledge that admits of being made articulate in the form of propositions.
In Mathematics as a Culture Clue: And Other Essays (1947), 127.
This splendid subject [mathematics], queen of all exact sciences, and the ideal and norm of all careful thinking...
Educational Problems (1911), Vol. 2, 393.
This therefore is Mathematics:
She reminds you of the invisible forms of the soul;
She gives life to her own discoveries;
She awakens the mind and purifies the intellect;
She brings light to our intrinsic ideas;
She abolishes oblivion and ignorance which are ours by birth...
She reminds you of the invisible forms of the soul;
She gives life to her own discoveries;
She awakens the mind and purifies the intellect;
She brings light to our intrinsic ideas;
She abolishes oblivion and ignorance which are ours by birth...
— Proclus
Quoted in Benjamin Franklin Finkel, Mathematical Association of America, The American Mathematical Monthly (1947), Vol. 54, 425.
This trend [emphasizing applied mathematics over pure mathematics] will make the queen of the sciences into the quean of the sciences.
As given, without citation, in Howard W. Eves Mathematical Circles Squared (1972), 158, which attributes it (via Dirk J. Struik) to a memorandum in which Passano wrote of the trend in the Dept. of Mathematics at M.I.T. Webmaster has as yet been unable to identify a primary source. (Can you help?) [Note: “quean” is an archaic word for: a disreputable woman; specifically : prostitute.—Merriam-Webster. “Thus the semantic spread between queen and quean could not be greater: from a woman of the highest repute to one of the lowest.” —alphadictionary.com]
Those that can readily master the difficulties of Mathematics find a considerable charm in the study, sometimes amounting to fascination. This is far from universal; but the subject contains elements of strong interest of a kind that constitutes the pleasures of knowledge. The marvellous devices for solving problems elate the mind with the feeling of intellectual power; and the innumerable constructions of the science leave us lost in wonder.
In Education as a Science (1879), 153.
Those who assert that the mathematical sciences make no affirmation about what is fair or good make a false assertion; for they do speak of these and frame demonstrations of them in the most eminent sense of the word. For if they do not actually employ these names, they do not exhibit even the results and the reasons of these, and therefore can be hardly said to make any assertion about them. Of what is fair, however, the most important species are order and symmetry, and that which is definite, which the mathematical sciences make manifest in a most eminent degree. And since, at least, these appear to be the causes of many things—now, I mean, for example, order, and that which is a definite thing, it is evident that they would assert, also, the existence of a cause of this description, and its subsistence after the same manner as that which is fair subsists in.
In Metaphysics [MacMahon] Bk. 12, chap. 3.
Thought-economy is most highly developed in mathematics, that science which has reached the highest formal development, and on which natural science so frequently calls for assistance. Strange as it may seem, the strength of mathematics lies in the avoidance of all unnecessary thoughts, in the utmost economy of thought-operations. The symbols of order, which we call numbers, form already a system of wonderful simplicity and economy. When in the multiplication of a number with several digits we employ the multiplication table and thus make use of previously accomplished results rather than to repeat them each time, when by the use of tables of logarithms we avoid new numerical calculations by replacing them by others long since performed, when we employ determinants instead of carrying through from the beginning the solution of a system of equations, when we decompose new integral expressions into others that are familiar,—we see in all this but a faint reflection of the intellectual activity of a Lagrange or Cauchy, who with the keen discernment of a military commander marshalls a whole troop of completed operations in the execution of a new one.
In Populär-wissenschafliche Vorlesungen (1903), 224-225.
Through most of his existence man’s survival depended on his ability to cope with nature. If the mind evolved as an aid in human survival it was primarily as an instrument for the mastery of nature. The mind is still at its best when tinkering with the mathematics that rule nature.
In Before the Sabbath (1979), 26.
Throughout his life Newton must have devoted at least as much attention to chemistry and theology as to mathematics.
In History of Mathematics (3rd Ed., 1901), 335.
Till the fifteenth century little progress appears to have been made in the science or practice of music; but since that era it has advanced with marvelous rapidity, its progress being curiously parallel with that of mathematics, inasmuch as great musical geniuses appeared suddenly among different nations, equal in their possession of this special faculty to any that have since arisen. As with the mathematical so with the musical faculty it is impossible to trace any connection between its possession and survival in the struggle for existence.
In 'Darwinism Applied to Man', Darwinism: An Exposition of the Theory of Natural Selection with Some of Its Applications (1901), Chap. 15, 468.
To a mind of sufficient intellectual power, the whole of mathematics would appear trivial, as trivial as the statement that a four-footed animal is an animal. (1959)
My Philosophical Development (1995), 207.
To be a scholar of mathematics you must be born with talent, insight, concentration, taste, luck, drive and the ability to visualize and guess.
In I Want to be a Mathematician: An Automathography (1985), 400.
To be always poring over the same Object, dulls the Intellects and tires the Mind, which is delighted and improved by a Variety: and therefore it ought, at times, to be relaxed from the more severe mathematical Contemplations, and to be employed upon something more light and agreeable, as Poetry, Physic, History, &c
In Dr. Boerhaave's Academical Lectures on the Theory of Physic (1746), Vol. 6, 264.
To be placed on the title-page of my collected works: Here it will be perceived from innumerable examples what is the use of mathematics for judgement in the natural sciences and how impossible it is to philosophise correctly without the guidance of Geometry, as the wise maxim of Plato has it.
Epigraph before title page, T. W. Körner, The Pleasures of Counting (1996).
To complete a PhD[,] I took courses in the history of philosophy. … As a result of my studies, I concluded that the traditional philosophy of science had little if anything to do with biology. … I had no use for a philosophy based on such an occult force as the vis vitalis. … But I was equally disappointed by the traditional philosophy of science, which was all based on logic, mathematics, and the physical sciences, and had adopted Descartes’ conclusion that an organism was nothing but a machine. This Cartesianism left me completely dissatisfied.
In 'Introduction', What Makes Biology Unique?: Considerations on the Autonomy of a Scientific Discipline (2007), 2.
To complete a PhD[,] I took courses in the history of philosophy. … As a result of my studies, I concluded that the traditional philosophy of science had little if anything to do with biology. … I had no use for a philosophy based on such an occult force as the vis vitalis. … But I was equally disappointed by the traditional philosophy of science, which was all based on logic, mathematics, and the physical sciences, and had adopted Descartes’ conclusion that an organism was nothing but a machine. This Cartesianism left me completely dissatisfied.
In 'Introduction', What Makes Biology Unique?: Considerations on the Autonomy of a Scientific Discipline (2007), 2.
To emphasize this opinion that mathematicians would be unwise to accept practical issues as the sole guide or the chief guide in the current of their investigations, ... let me take one more instance, by choosing a subject in which the purely mathematical interest is deemed supreme, the theory of functions of a complex variable. That at least is a theory in pure mathematics, initiated in that region, and developed in that region; it is built up in scores of papers, and its plan certainly has not been, and is not now, dominated or guided by considerations of applicability to natural phenomena. Yet what has turned out to be its relation to practical issues? The investigations of Lagrange and others upon the construction of maps appear as a portion of the general property of conformal representation; which is merely the general geometrical method of regarding functional relations in that theory. Again, the interesting and important investigations upon discontinuous two-dimensional fluid motion in hydrodynamics, made in the last twenty years, can all be, and now are all, I believe, deduced from similar considerations by interpreting functional relations between complex variables. In the dynamics of a rotating heavy body, the only substantial extension of our knowledge since the time of Lagrange has accrued from associating the general properties of functions with the discussion of the equations of motion. Further, under the title of conjugate functions, the theory has been applied to various questions in electrostatics, particularly in connection with condensers and electrometers. And, lastly, in the domain of physical astronomy, some of the most conspicuous advances made in the last few years have been achieved by introducing into the discussion the ideas, the principles, the methods, and the results of the theory of functions. … the refined and extremely difficult work of Poincare and others in physical astronomy has been possible only by the use of the most elaborate developments of some purely mathematical subjects, developments which were made without a thought of such applications.
In Presidential Address British Association for the Advancement of Science, Section A, (1897), Nature, 56, 377.
To fully understand the mathematical genius of Sophus Lie, one must not turn to books recently published by him in collaboration with Dr. Engel, but to his earlier memoirs, written during the first years of his scientific career. There Lie shows himself the true geometer that he is, while in his later publications, finding that he was but imperfectly understood by the mathematicians accustomed to the analytic point of view, he adopted a very general analytic form of treatment that is not always easy to follow.
In Lectures on Mathematics (1911), 9.
To function efficiently in today’s world, you need math. The world is so technical, if you plan to work in it, a math background will let you go farther and faster.
In 'Ross, Mary Golda :The Cherokee Nation Remembers the First Woman Engineer for Lockheed', Indian Country News, article published online (13 May 2008) on the indiancountrynews.com website.
To isolate mathematics from the practical demands of the sciences is to invite the sterility of a cow shut away from the bulls.
As quoted, without citation, in In J.E. Littlewood, A Mathematician’s Miscellany (1953), , reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986).
To keep pace with the growth of mathematics, one would have to read about fifteen papers a day, most of them packed with technical details and of considerable length. No one dreams of attempting this task.
In 'The Extent of Mathematics', Prelude to Mathematics (1955), 11.
To my mathematical brain, the numbers alone make thinking about aliens perfectly rational. The real challenge is to work out what aliens might actually be like.
From Discovery TV series, Into the Universe With Stephen Hawking (2010). As quoted on nbcnews.com webpage, 'Hawking: Aliens may pose risks to Earth' (25 Apr 2010). He warned that rather than actively trying to communicate with extra-terrestrials, humans should do everything possible to avoid contact.
To the average mathematician who merely wants to know his work is securely based, the most appealing choice is to avoid difficulties by means of Hilbert's program. Here one regards mathematics as a formal game and one is only concerned with the question of consistency ... . The Realist position is probably the one which most mathematicians would prefer to take. It is not until he becomes aware of some of the difficulties in set theory that he would even begin to question it. If these difficulties particularly upset him, he will rush to the shelter of Formalism, while his normal position will be somewhere between the two, trying to enjoy the best of two worlds.
In Axiomatic Set Theory (1971), 9-15. In Thomas Tymoczko, New Directions in the Philosophy of Mathematics: an Anthology (), 11-12.
To what purpose should People become fond of the Mathematicks and Natural Philosophy? … People very readily call Useless what they do not understand. It is a sort of Revenge… One would think at first that if the Mathematicks were to be confin’d to what is useful in them, they ought only to be improv'd in those things which have an immediate and sensible Affinity with Arts, and the rest ought to be neglected as a Vain Theory. But this would be a very wrong Notion. As for Instance, the Art of Navigation hath a necessary Connection with Astronomy, and Astronomy can never be too much improv'd for the Benefit of Navigation. Astronomy cannot be without Optics by reason of Perspective Glasses: and both, as all parts of the Mathematicks are grounded upon Geometry … .
Of the Usefulness of Mathematical Learning (1699)
To-day, science has withdrawn into realms that are hardly understanded of the people. Biology means very largely histology, the study of the cell by difficult and elaborate microscopical processes. Chemistry has passed from the mixing of simple substances with ascertained reactions, to an experimentation of these processes under varying conditions of temperature, pressure, and electrification—all requiring complicated apparatus and the most delicate measurement and manipulation. Similarly, physics has outgrown the old formulas of gravity, magnetism, and pressure; has discarded the molecule and atom for the ion, and may in its recent generalizations be followed only by an expert in the higher, not to say the transcendental mathematics.
‘Exit the Amateur Scientist.’ Editorial, The Nation, 23 August 1906, 83, 160.
Today, it is not only that our kings do not know mathematics, but our philosophers do not know mathematics and—to go a step further—our mathematicians do not know mathematics.
'The Tree of Knowledge', Harper's Magazine (1958), 217, 55.
Today’s scientists have substituted mathematics for experiments, and they wander off through equation after equation, and eventually build a structure which has no relation to reality.
In 'Radio Power Will Revolutionize the World', Modern Mechanix and Inventions (Jul 1934), 117-118.
Two extreme views have always been held as to the use of mathematics. To some, mathematics is only measuring and calculating instruments, and their interest ceases as soon as discussions arise which cannot benefit those who use the instruments for the purposes of application in mechanics, astronomy, physics, statistics, and other sciences. At the other extreme we have those who are animated exclusively by the love of pure science. To them pure mathematics, with the theory of numbers at the head, is the only real and genuine science, and the applications have only an interest in so far as they contain or suggest problems in pure mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
In History of European Thought in the Nineteenth Century (1903), 630.
Two kinds of symbol must surely be distinguished. The algebraic symbol comes naked into the world of mathematics and is clothed with value by its masters. A poetic symbol—like the Rose, for Love, in Guillaume de Lorris—comes trailing clouds of glory from the real world, clouds whose shape and colour largely determine and explain its poetic use. In an equation, x and y will do as well as a and b; but the Romance of the Rose could not, without loss, be re-written as the Romance of the Onion, and if a man did not see why, we could only send him back to the real world to study roses, onions, and love, all of them still untouched by poetry, still raw.
C.S. Lewis and E.M. Tillyard, The Personal Heresy: A Controversy (1936), 97.
Ultra-modern physicists [are tempted to believe] that Nature in all her infinite variety needs nothing but mathematical clothing [and are] strangely reluctant to contemplate Nature unclad. Clothing she must have. At the least she must wear a matrix, with here and there a tensor to hold the queer garment together.
As quoted by Stephen T. Keith and Pierre Quédec, in 'Magnetism and Magnetic Materials', an article collected in Out of the Crystal Maze: Chapters from The History of Solid State Physics (1992), 361.
Undeterred by poverty, failure, domestic tragedy, and persecution, but sustained by his mystical belief in an attainable mathematical harmony and perfection of nature, Kepler persisted for fifteen years before finding the simple regularity [of planetary orbits] he sought… . What stimulated Kepler to keep slaving all those fifteen years? An utter absurdity. In addition to his faith in the mathematical perfectibility of astronomy, Kepler also believed wholeheartedly in astrology. This was nothing against him. For a scientist of Kepler’s generation astrology was as respectable scientifically and mathematically as the quantum theory or relativity is to theoretical physicists today. Nonsense now, astrology was not nonsense in the sixteenth century.
In The Handmaiden of the Sciences (1937), 30.

Undoubtedly, the capstone of every mathematical theory is a convincing proof of all of its assertions. Undoubtedly, mathematics inculpates itself when it foregoes convincing proofs. But the mystery of brilliant productivity will always be the posing of new questions, the anticipation of new theorems that make accessible valuable results and connections. Without the creation of new viewpoints, without the statement of new aims, mathematics would soon exhaust itself in the rigor of its logical proofs and begin to stagnate as its substance vanishes. Thus, in a sense, mathematics has been most advanced by those who distinguished themselves by intuition rather than by rigorous proofs.
As quoted in Hermann Weyl, Unterrichtsblätter für Mathematik und Naturwissenschaften (1932), 38, 177-188. As translated by Abe Shenitzer, in 'Part I. Topology and Abstract Algebra as Two Roads of Mathematical Comprehension', The American Mathematical Monthly (May 1995), 102, No. 7, 453.
Unless the chemist learns the language of mathematics, he will become a provincial and the higher branches of chemical work, that require reason as well as skill, will gradually pass out of his hands.
Quoted in Journal of the Chemical Society, 1929, 6, 254.
Until now, physical theories have been regarded as merely models with approximately describe the reality of nature. As the models improve, so the fit between theory and reality gets closer. Some physicists are now claiming that supergravity is the reality, that the model and the real world are in mathematically perfect accord.
Superforce (1984, 1985), 149.
Upon this ground it is that I am bold to think that morality is capable of demonstration, as well as mathematics: since the precise real essence of the things moral words stand for may be perfectly known, and so the congruity and incongruity of the things themselves be certainly discussed; in which consists perfect knowledge.
From An Essay in Human Understanding (1777), as quoted and cited in Philip Davis with Reuben Hersh, in The Mathematical Experience (1981), 110.
Very few people realize the enormous bulk of contemporary mathematics. Probably it would be easier to learn all the languages of the world than to master all mathematics at present known. The languages could, I imagine, be learnt in a lifetime; mathematics certainly could not. Nor is the subject static.
In 'The Extent of Mathematics', Prelude to Mathematics (1955), 11.
We all use math every day; to predict weather, to tell time, to handle money. Math is more than formulas or equations; it’s logic, it’s rationality, it’s using your mind to solve the biggest mysteries we know.
— NUM3ERS
Voice-over for each episode opening title of the TV show, “NUM3ERS” (2005-2010).
We are concerned to understand the motivation for the development of pure mathematics, and it will not do simply to point to aesthetic qualities in the subject and leave it at that. It must be remembered that there is far more excitement to be had from creating something than from appreciating it after it has been created. Let there be no mistake about it, the fact that the mathematician is bound down by the rules of logic can no more prevent him from being creative than the properties of paint can prevent the artist. … We must remember that the mathematician not only finds the solutions to his problems, he creates the problems themselves.
In A Signpost to Mathematics (1951), 19. As quoted and cited in William L. Schaaf, 'Memorabilia Mathematica', The Mathematics Teacher (Mar 1957), 50, No. 3, 230. Note that this paper incorrectly attributes “A.H. Head”.
We are not very pleased when we are forced to accept a mathematical truth by virtue of a complicated chain of formal conclusions and computations, which we traverse blindly, link by link, feeling our way by touch. We want first an overview of the aim and of the road; we want to understand the idea of the proof, the deeper context.
Unterrichtsblätter für Mathematik und Naturwissenschaften (1932), 38, 177-188. As translated by Abe Shenitzer, in 'Part I. Topology and Abstract Algebra as Two Roads of Mathematical Comprehension', The American Mathematical Monthly (May 1995), 102, No. 7, 453.
We are servants rather than masters in mathematics.
From the original French, “Nous sommes serviteurs plutôt que maîtres en mathématiques,” as quoted, without citation, in Jacques Hadamard, 'L’Œvre d'Henri Poincaré: Le Mathématician', Revue de Métaphysique et de Morale (Sep 1913), 21, No. 5, 618. As translated in Harold Chapman Brown, 'The Work of Henri Poincaré', The Journal of Philosophy, Psychology and Scientific Methods (23 Apr 1914), 11, No. 9, 226.
We are told that “Mathematics is that study which knows nothing of observation, nothing of experiment, nothing of induction, nothing of causation.” I think no statement could have been made more opposite to the facts of the case; that mathematical analysis is constantly invoking the aid of new principles, new ideas, and new methods, not capable of being defined by any form of words, but springing direct from the inherent powers and activities of the human mind, and from continually renewed introspection of that inner world of thought of which the phenomena are as varied and require as close attention to discern as those of the outer physical world (to which the inner one in each individual man may, I think, be conceived to stand somewhat in the same relation of correspondence as a shadow to the object from which it is projected, or as the hollow palm of one hand to the closed fist which it grasps of the other), that it is unceasingly calling forth the faculties of observation and comparison, that one of its principal weapons is induction, that it has frequent recourse to experimental trial and verification, and that it affords a boundless scope for the exercise of the highest efforts of the imagination and invention.
In Presidential Address to British Association, Exeter British Association Report (1869), pp. 1-9, in Collected Mathematical Papers, Vol. 2, 654.
We cannot get more out of the mathematical mill than we put into it, though we may get it in a form infinitely more useful for our purpose.
From James Forrest Lecture (3 May 1894) at an Extra Meeting of the Institution of Civil Engineers, 'The Relation of Mathematics to Engineering', collected in Minutes of Proceedings of the Institution of Civil Engineers (1894), 346.
We cannot take anything for granted, beyond the first mathematical formula. Question everything else.
In Phebe Mitchell Kendall (ed.), Maria Mitchell: Life, Letters, and Journals (1896), 188.
We do not learn by inference and deduction, and the application of mathematics to philosophy, but by direct intercourse and sympathy.
In 'Natural History of Massachusetts', The Dial: A Magazine for Literature, Philosophy, and Religion (Jul 1842), 3, No. 1, 40.
We especially need imagination in science. It is not all mathematics, nor all logic, but it is somewhat beauty and poetry.
In Phebe Mitchell Kendall (ed.), Maria Mitchell: Life, Letters, and Journals (1896), 186.
We have heard much about the poetry of mathematics, but very little of it has yet been sung. The ancients had a juster notion of their poetic value than we. The most distinct and beautiful statements of any truth must take at last the mathematical form.
In A Week on the Concord and Merrimack Rivers (1862), 381.
We have overcome the notion that mathematical truths have an existence independent and apart from our own minds. It is even strange to us that such a notion could ever have existed.
With co-author James R. Newman, Mathematics and the Imagination (1940), 359.
We have overcome the notion that mathematical truths have an existence independent and apart from our own minds. It is even strange to us that such a notion could ever have existed. [Coauthor with James R. Newman]
In Edward Kasner and James Newman, Mathematics and the Imagination (1940, 1949), 359.
We have to come back to something like ordinary language after all when we want to talk “about” mathematics!
As co-author with Bertha Swirles Jeffreys, in Methods of Mathematical Physics (1946, 1999), 2.
We know that mathematicians care no more for logic than logicians for mathematics. The two eyes of science are mathematics and logic; the mathematical set puts out the logical eye, the logical set puts out the mathematical eye; each believing that it sees better with one eye than with two.
Note that De Morgan, himself, only had sight with only one eye.
Note that De Morgan, himself, only had sight with only one eye.
Review of a book on geometry in the Athenaeum, 1868, Vol. 2, 71-73.
We know the laws of trial and error, of large numbers and probabilities. We know that these laws are part of the mathematical and mechanical fabric of the universe, and that they are also at play in biological processes. But, in the name of the experimental method and out of our poor knowledge, are we really entitled to claim that everything happens by chance, to the exclusion of all other possibilities?
From Nobel Prize Lecture (Dec 1974), 'The Coming Age of the Cell'. Collected in Jan Lindsten (ed.) Nobel Lectures, Physiology or Medicine 1971-1980 (1992).
We love to discover in the cosmos the geometrical forms that exist in the depths of our consciousness. The exactitude of the proportions of our monuments and the precision of our machines express a fundamental character of our mind. Geometry does not exist in the earthly world. It has originated in ourselves. The methods of nature are never so precise as those of man. We do not find in the universe the clearness and accuracy of our thought. We attempt, therefore, to abstract from the complexity of phenomena some simple systems whose components bear to one another certain relations susceptible of being described mathematically.
In Man the Unknown (1935), 8.
We may always depend on it that algebra, which cannot be translated into good English and sound common sense, is bad algebra.
In Common Sense in the Exact Sciences (1885), 21.
We may as well cut out group theory. That is a subject that will never be of any use in physics.
Discussing mathematics curriculum reform at Princeton University (1910), as quoted in Abraham P. Hillman, Gerald L. Alexanderson, Abstract Algebra: A First Undergraduate Course (1994), 94.
We may discover resources on the moon or Mars that will boggle the imagination, that will test our limits to dream. And the fascination generated by further exploration will inspire our young people to study math, and science, and engineering and create a new generation of innovators and pioneers.
Speech, NASA Headquarters (14 Jan 2004). In Office of the Federal Register (U.S.) Staff (eds.), Public Papers of the Presidents of the United States, George W. Bush (2007), 58-59.
We may safely say, that the whole form of modern mathematical thinking was created by Euler. It is only with the greatest difficulty that one is able to follow the writings of any author immediately preceding Euler, because it was not yet known how to let the formulas speak for themselves. This art Euler was the first one to teach.
As quoted in W. Ahrens Scherz und Ernst in der Mathematik (1904), 251. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 183.
We may see how unexpectedly recondite parts of pure mathematics may bear upon physical science, by calling to mind the circumstance that Fresnel obtained one of the most curious confirmations of the theory (the laws of Circular Polarization by reflection) through an interpretation of an algebraical expression, which, according to the original conventional meaning of the symbols, involved an impossible quantity.
In History of Scientific Ideas, Bk. 2, chap. 14, sect. 8.
We may summarize … the fundamental characteristics and limitations of mathematics as follows: mathematics is ultimately an experimental science, for freedom from contradiction cannot be proved, but only postulated and checked by observation, and similarly existence can only be postulated and checked by observation. Furthermore, mathematics requires the fundamental device of all thought, of analyzing experience into static bits with static meanings.
In The Nature of Physical Theory (1936), 58.
We often hear that mathematics consists mainly of “proving theorems.” Is a writer's job mainly that of “writing sentences?”
In Rota's 'Introduction' written (1980) to preface Philip J. Davis and Reuben Hersh, The Mathematical Experience (1981, 2012), xxii.
We pass with admiration along the great series of mathematicians, by whom the science of theoretical mechanics has been cultivated, from the time of Newton to our own. There is no group of men of science whose fame is higher or brighter. The great discoveries of Copernicus, Galileo, Newton, had fixed all eyes on those portions of human knowledge on which their successors employed their labors. The certainty belonging to this line of speculation seemed to elevate mathematicians above the students of other subjects; and the beauty of mathematical relations and the subtlety of intellect which may be shown in dealing with them, were fitted to win unbounded applause. The successors of Newton and the Bernoullis, as Euler, Clairaut, D’Alembert, Lagrange, Laplace, not to introduce living names, have been some of the most remarkable men of talent which the world has seen.
In History of the Inductive Sciences, Vol. 1, Bk. 4, chap. 6, sect. 6.
We perfectly know what is Good, and what is Evil; and may be as certain in Morals as in Mathematics.
No. 5452 in Gnomologia: Adagies and Proverbs, Wise Sentences and Witty Sayings (1732), 238.
We receive it as a fact, that some minds are so constituted as absolutely to require for their nurture the severe logic of the abstract sciences; that rigorous sequence of ideas which leads from the premises to the conclusion, by a path, arduous and narrow, it may be, and which the youthful reason may find it hard to mount, but where it cannot stray; and on which, if it move at all, it must move onward and upward… . Even for intellects of a different character, whose natural aptitude is for moral evidence and those relations of ideas which are perceived and appreciated by taste, the study of the exact sciences may be recommended as the best protection against the errors into which they are most likely to fall. Although the study of language is in many respects no mean exercise in logic, yet it must be admitted that an eminently practical mind is hardly to be formed without mathematical training.
In Orations and Speeches (1870), Vol. 8, 510.
What appear to be the most valuable aspects of the theoretical physics we have are the mathematical descriptions which enable us to predict events. These equations are, we would argue, the only realities we can be certain of in physics; any other ways we have of thinking about the situation are visual aids or mnemonics which make it easier for beings with our sort of macroscopic experience to use and remember the equations.
In The Lost Cause: Causation and the Mind-body Problem (2003).
What binds us to space-time is our rest mass, which prevents us from flying at the speed of light, when time stops and space loses meaning. In a world of light there are neither points nor moments of time; beings woven from light would live “nowhere” and “nowhen”; only poetry and mathematics are capable of speaking meaningfully about such things.
In 'Mathematics and Physics', collected in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 130.
What is best in mathematics deserves not merely to be learnt as a task, but to assimilated as a part of daily thought, and brought again and again before the mind with ever-renewed encouragement.
Essay, 'The Study of Mathematics' (1902), collected in Philosophical Essays (1910), 73-74. Also collected in Mysticism and Logic: And Other Essays (1919), 60.
What is exact about mathematics but exactness? And is not this a consequence of the inner sense of truth?
In 'Sprüche in Prosa', Natur, 6, 948.
What Is Mathematics? This question, if asked in earnest, has no answer.
'Why Mathematics Grows', Journal of the History of Ideas (Jan-Mar 1965), 26, No. 1, 3. In Salomon Bochner and Robert Clifford Gunning (ed.) Collected Papers of Salomon Bochner (1992), Vol. 4, 191.
What is mathematics? To give a satisfactory definition is difficult, if not impossible.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 1.
What is mathematics? What is it for? What are mathematicians doing nowadays? Wasn't it all finished long ago? How many new numbers can you invent anyway? Is today’s mathematics just a matter of huge calculations, with the mathematician as a kind of zookeeper, making sure the precious computers are fed and watered? If it’s not, what is it other than the incomprehensible outpourings of superpowered brainboxes with their heads in the clouds and their feet dangling from the lofty balconies of their ivory towers?
Mathematics is all of these, and none. Mostly, it’s just different. It’s not what you expect it to be, you turn your back for a moment and it's changed. It's certainly not just a fixed body of knowledge, its growth is not confined to inventing new numbers, and its hidden tendrils pervade every aspect of modern life.
Mathematics is all of these, and none. Mostly, it’s just different. It’s not what you expect it to be, you turn your back for a moment and it's changed. It's certainly not just a fixed body of knowledge, its growth is not confined to inventing new numbers, and its hidden tendrils pervade every aspect of modern life.
Opening paragraphs of 'Preface', From Here to Infinity (1996), vii.
What is this subject, which may be called indifferently either mathematics or logic? Is there any way in which we can define it? Certain characteristics of the subject are clear. To begin with, we do not, in this subject, deal with particular things or particular properties: we deal formally with what can be said about any thing or any property. We are prepared to say that one and one are two, but not that Socrates and Plato are two, because, in our capacity of logicians or pure mathematicians, we have never heard of Socrates or Plato. A world in which there were no such individuals would still be a world in which one and one are two. It is not open to us, as pure mathematicians or logicians, to mention anything at all, because, if we do so we introduce something irrelevant and not formal.
In Introduction to Mathematical Philosophy (1920), 196-197.
What makes the theory of relativity so acceptable to physicists in spite of its going against the principle of simplicity is its great mathematical beauty. This is a quality which cannot be defined, any more than beauty in art can be defined, but which people who study mathematics usually have no difficulty in appreciating. … The restricted theory changed our ideas of space and time in a way that may be summarised by stating that the group of transformations to which the space-time continuum is subject must be changed from the Galilean group to the Lorentz group.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 123.
What renders a problem definite, and what leaves it indefinite, may best be understood from mathematics. The very important idea of solving a problem within limits of error is an element of rational culture, coming from the same source. The art of totalizing fluctuations by curves is capable of being carried, in conception, far beyond the mathematical domain, where it is first learnt. The distinction between laws and co-efficients applies in every department of causation. The theory of Probable Evidence is the mathematical contribution to Logic, and is of paramount importance.
In Education as a Science (1879), 151-152.
What science can there be more noble, more excellent, more useful for men, more admirably high and demonstrative, than this of the mathematics?
In 'On the Usefulness of Mathematics', Works (1840), Vol. 2, 69.
What, in fact, is mathematical discovery? It does not consist in making new combinations with mathematical entities that are already known. That can be done by anyone, and the combinations that could be so formed would be infinite in number, and the greater part of them would be absolutely devoid of interest. Discovery consists precisely in not constructing useless combinations, but in constructing those that are useful, which are an infinitely small minority. Discovery is discernment, selection.
In Science et Méthode (1920), 48, as translated by Francis Maitland, in Science and Method (1908, 1952), 50-51. Also seen elsewhere translated with “invention” in place of “discovery”.
What’s the best part of being a mathematician? I'm not a religious man, but it’s almost like being in touch with God when you’re thinking about mathematics. God is keeping secrets from us, and it’s fun to try to learn some of the secrets.
From interview with Donald J. Albers. In John H. Ewing and Frederick W. Gehring, Paul Halmos Celebrating 50 Years of Mathematics (1991), 21.
Whatever advantage can be attributed to logic in directing and strengthening the action of the understanding is found in a higher degree in mathematical study, with the immense added advantage of a determinate subject, distinctly circumscribed, admitting of the utmost precision, and free from the danger which is inherent in all abstract logic—of leading to useless and puerile rules, or to vain ontological speculations. The positive method, being everywhere identical, is as much at home in the art of reasoning as anywhere else: and this is why no science, whether biology or any other, can offer any kind of reasoning, of which mathematics does not supply a simpler and purer counterpart. Thus, we are enabled to eliminate the only remaining portion of the old philosophy which could even appear to offer any real utility; the logical part, the value of which is irrevocably absorbed by mathematical science.
In Auguste Comte and Harriet Martineau (trans.), Positive Philosophy (1858), Vol. 1, 326-327.
Whatever may happen to the latest theory of Dr. Einstein, his treatise represents a mathematical effort of overwhelming proportions. It is the more remarkable since Einstein is primarily a physicist and only incidentally a mathematician. He came to mathematics rather of necessity than by predilection, and yet he has here developed mathematical formulae and calculations springing from a colossal knowledge.
In 'Marvels at Einstein For His Mathematics', New York Times (4 Feb 1929), 3.
Whatever may have been imputed to some other studies under the notion of insignificancy and loss of time, yet these [mathematics], I believe, never caused repentance in any, except it was for their remissness in the prosecution of them.
In 'On the Usefulness of Mathematics', Works (1840), Vol. 2, 69.
When all the discoveries [relating to the necessities and some to the pastimes of life] were fully developed, the sciences which relate neither to pleasure nor yet to the necessities of life were invented, and first in those places where men had leisure. Thus the mathematical sciences originated in the neighborhood of Egypt, because there the priestly class was allowed leisure.
In Metaphysics, 1-981b, as translated by Hugh Tredennick (1933). Also seen translated as “Now that practical skills have developed enough to provide adequately for material needs, one of these sciences which are not devoted to utilitarian ends [mathematics] has been able to arise in Egypt, the priestly caste there having the leisure necessary for disinterested research.”
When ever we turn in these days of iron, steam and electricity we find that Mathematics has been the pioneer. Were its back bone removed, our material civilization would inevitably collapse. Modern thought and belief would have been altogether different, had Mathematics not made the various sciences exact.
The Teaching of Mathematics in the Elementary and the Secondary School (1907), 13.
When Faraday filled space with quivering lines of force, he was bringing mathematics into electricity. When Maxwell stated his famous laws about the electromagnetic field it was mathematics. The relativity theory of Einstein which makes gravity a fiction, and reduces the mechanics of the universe to geometry, is mathematical research.
In 'The Spirit of Research', III, 'Mathematical Research', in The Monist (Oct 1922), 32, No. 4, 542-543.
When first I applied my mind to Mathematics I read straight away most of what is usually given by the mathematical writers, and I paid special attention to Arithmetic and Geometry because they were said to be the simplest and so to speak the way to all the rest. But in neither case did I then meet with authors who fully satisfied me. I did indeed learn in their works many propositions about numbers which I found on calculation to be true. As to figures, they in a sense exhibited to my eyes a great number of truths and drew conclusions from certain consequences. But they did not seem to make it sufficiently plain to the mind itself why these things are so, and how they discovered them. Consequently I was not surprised that many people, even of talent and scholarship, should, after glancing at these sciences, have either given them up as being empty and childish or, taking them to be very difficult and intricate, been deterred at the very outset from learning them. … But when I afterwards bethought myself how it could be that the earliest pioneers of Philosophy in bygone ages refused to admit to the study of wisdom any one who was not versed in Mathematics … I was confirmed in my suspicion that they had knowledge of a species of Mathematics very different from that which passes current in our time.
In Elizabeth S. Haldane (trans.) and G.R.T. Ross (trans.), 'Rules for the Direction of the Mind', The Philosophical Works of Descartes (1911, 1973), Vol. 1, Rule 4, 11.
When I asked Sir Isaac how the study of the mathematics flourished in England, he said, “Not so much as it has done here; but more than it does in any other country.”
As recalled and recorded in Joseph Spence and Edmund Malone (ed.) Anecdotes, Observations, and Characters of Books and Men (1858), 160.
When I came back from Munich, it was September, and I was Professor of Mathematics at the Eindhoven University of Technology. Later I learned that I had been the Department’s third choice, after two numerical analysts had turned the invitation down; the decision to invite me had not been an easy one, on the one hand because I had not really studied mathematics, and on the other hand because of my sandals, my beard and my ‘arrogance’ (whatever that may be).
…...
When I was reading Mathematics for University honours, I would sometimes, after working a week or two at some new book, and mastering ten or twenty pages, get into a hopeless muddle, and find it just as bad the next morning. My rule was to begin the book again. And perhaps in another fortnight I had come to the old difficulty with impetus enough to get over it. Or perhaps not. I have several books that I have begun over and over again.
From letter to Edith Rix with hints for studying (about Mar 1885), in Stuart Dodgson Collingwood, The Life and Letters of Lewis Carroll (1898), 240-241.
When one considers how hard it is to write a computer program even approaching the intellectual scope of a good paper, and how much greater time and effort have to be put in to make it “almost” formally correct, it is preposterous to claim that mathematics as we practice it is anywhere near formally correct.
In 'On Proof and Progress in Mathematics', For the Learning of Mathematics (Feb 1995), 15, No. 1, 33. Reprinted from Bulletin of the American Mathematical Society (1994), 30, No. 2, 170-171.
When physicists speak of “beauty” in their theories, they really mean that their theory possesses at least two essential features: 1. A unifying symmetry 2. The ability to explain vast amounts of experimental data with the most economical mathematical expressions.
In 'Quantum Heresy', Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth Dimension (1995), 127.
When Ramanujan was sixteen, he happened upon a copy of Carr’s Synopsis of Mathematics. This chance encounter secured immortality for the book, for it was this book that suddenly woke Ramanujan into full mathematical activity and supplied him essentially with his complete mathematical equipment in analysis and number theory. The book also gave Ramanujan his general direction as a dealer in formulas, and it furnished Ramanujan the germs of many of his deepest developments.
In Mathematical Circles Squared (1972), 158. George Shoobridge Carr (1837-1914) wrote his Synopsis of Elementary Results in Mathematics in 1886.
When the difficulty of a problem lies only in finding out what follows from certain fixed premises, mathematical methods furnish invaluable wings for flying over intermediate obstructions.
From The Economic Theory of the Location of Railways (1887, 1914), viii.
When the first mathematical, logical, and natural uniformities, the first laws, were discovered, men were so carried away by the clearness, beauty and simplification that resulted, that they believed themselves to have deciphered authentically the eternal thoughts of the Almighty.
From Lecture (Nov 1906) at the Lowell Institute, Boston. Published in 'The Present Dilemma in Philosophy',Pragmatism: A New Name for Some Old Ways of Thinking: Popular Lectures on Philosophy (1907), 56.
When the mathematician says that such and such a proposition is true of one thing, it may be interesting, and it is surely safe. But when he tries to extend his proposition to everything, though it is much more interesting, it is also much more dangerous. In the transition from one to all, from the specific to the general, mathematics has made its greatest progress, and suffered its most serious setbacks, of which the logical paradoxes constitute the most important part. For, if mathematics is to advance securely and confidently, it must first set its affairs in order at home.
With co-author James R. Newman, in Mathematics and the Imagination (1940), 219.
When the war finally came to an end, 1 was at a loss as to what to do. ... I took stock of my qualifications. A not-very-good degree, redeemed somewhat by my achievements at the Admiralty. A knowledge of certain restricted parts of magnetism and hydrodynamics, neither of them subjects for which I felt the least bit of enthusiasm.
No published papers at all … [Only gradually did I realize that this lack of qualification could be an advantage. By the time most scientists have reached age thirty they are trapped by their own expertise. They have invested so much effort in one particular field that it is often extremely difficult, at that time in their careers, to make a radical change. I, on the other hand, knew nothing, except for a basic training in somewhat old-fashioned physics and mathematics and an ability to turn my hand to new things. … Since I essentially knew nothing, I had an almost completely free choice. …
No published papers at all … [Only gradually did I realize that this lack of qualification could be an advantage. By the time most scientists have reached age thirty they are trapped by their own expertise. They have invested so much effort in one particular field that it is often extremely difficult, at that time in their careers, to make a radical change. I, on the other hand, knew nothing, except for a basic training in somewhat old-fashioned physics and mathematics and an ability to turn my hand to new things. … Since I essentially knew nothing, I had an almost completely free choice. …
In What Mad Pursuit (1988).
When the world is mad, a mathematician may find in mathematics an incomparable anodyne. For mathematics is, of all the arts and sciences, the most austere and the most remote, and a mathematician should be of all men the one who can most easily take refuge where, as Bertrand Russell says, “one at least of our nobler impulses can best escape from the dreary exile of
the actual world.”
In A Mathematician's Apology (1940, 2012), 43.
When we talk mathematics, we may be discussing a secondary language built on the primary language of the nervous system.
As quoted in John C. Oxtoby and B. J. Pettis (eds.), 'John von Neumann, 1903-1957', Bulletin of the American Mathematical Society (May 1958), 64, No. 3, Part 2, 128.
When we think of giving a child a mathematical education we are apt to ask whether he has special aptitudes fitting him to receive it. Do we ask any such questions when we talk of teaching him to read and write?
In 'Mathematics for Children', Popular Science Monthly (Oct 1899), 187, citing “translated for the Popular Science Monthly from the Revue Scientifique. Also seen paraphrased as “To ask whether a child has an aptitude for mathematics is equivalent to asking whether he has an aptitude for reading and writing,” in William L. Schaaf, 'Memorabilia Mathematica', The Mathematics Teacher (Mar 1957), 50, No. 3, 231.
Whenever … a controversy arises in mathematics, the issue is not whether a thing is true or not, but whether the proof might not be conducted more simply in some other way, or whether the proposition demonstrated is sufficiently important for the advancement of the science as to deserve especial enunciation and emphasis, or finally, whether the proposition is not a special case of some other and more general truth which is as easily discovered.
In Mathematical Essays and Recreations (1898), 88.
Where we reach the sphere of mathematics we are among processes which seem to some the most inhuman of all human activities and the most remote from poetry. Yet it is just here that the artist has the fullest scope for his imagination. … We are in the imaginative sphere of art, and the mathematician is engaged in a work of creation which resembles music in its orderliness, … It is not surprising that the greatest mathematicians have again and again appealed to the arts in order to find some analogy to their own work. They have indeed found it in the most varied arts, in poetry, in painting, and in sculpture, although it would certainly seem that it is in music, the most abstract of all the arts, the art of number and time, that we find the closest analogy.
In The Dance of Life (1923), 138-139.
Whereas, to borrow an illustration from mathematics, life was formerly an equation of, say, 100 unknown quantities, it is now one of 99 only, inasmuch as memory and heredity have been shown to be one and the same thing.
Samuel Butler, Henry Festing Jones (ed.), The Note-Books of Samuel Butler (1917), 57.
Wherever it was, I did not come to know it through the bodily senses; the only things we know through the bodily senses are material objects, which we have found are not truly and simply one. Moreover, if we do not perceive one by the bodily sense, then we do not perceive any number by that sense, at least of those numbers that we grasp by understanding.
De Ubero Arbitrio (On Free Choice of the Will) [386], trans. T. Williams (1993), 45.
While, on the one hand, the end of scientific investigation is the discovery of laws, on the other, science will have reached its highest goal when it shall have reduced ultimate laws to one or two, the necessity of which lies outside the sphere of our cognition. These ultimate laws—in the domain of physical science at least—will be the dynamical laws of the relations of matter to number, space, and time. The ultimate data will be number, matter, space, and time themselves. When these relations shall be known, all physical phenomena will be a branch of pure mathematics.
'Address to the section of Mathematical and Physical Science', Reports of the British Association for the Advancement of Science (1895), 595.
Who knows not mathematics and the results of recent scientific investigation dies without knowing truth.
As qoted in Jacob William Albert Young, Teaching of Mathematics in the Elementary and the Secondary School (1907), 44. Footnoted with cite to Simon, Mathematischer Unterricht, 21
Who made me the genius I am today,
The mathematician that others all quote?
Who’s the professor that made me that way,
The greatest that ever got chalk on his coat?
One man deserves the credit;
One man deserves the blame,
And Nicolai Ivanovich Lobachevsky is his name. Oy!
Nicolai Ivanovich Lobach…
I am never forget the day I first meet the great Lobachevsky.
In one word he told me the secret of success in mathematics:
Plagiarize!
Plagiarize,
Let no one else’s work evade your eyes.
Remember why the good Lord made your eyes,
So don’t shade your eyes.
But plagiarize, plagiarize, plagiarize —
Only be sure always to call it please “research”.
And ever since I meet this man, my life is not the same.
And Nicolai Ivanovich Lobachevsky is his name. Oy!
Nicolai Ivanovich Lobach…
The mathematician that others all quote?
Who’s the professor that made me that way,
The greatest that ever got chalk on his coat?
One man deserves the credit;
One man deserves the blame,
And Nicolai Ivanovich Lobachevsky is his name. Oy!
Nicolai Ivanovich Lobach…
I am never forget the day I first meet the great Lobachevsky.
In one word he told me the secret of success in mathematics:
Plagiarize!
Plagiarize,
Let no one else’s work evade your eyes.
Remember why the good Lord made your eyes,
So don’t shade your eyes.
But plagiarize, plagiarize, plagiarize —
Only be sure always to call it please “research”.
And ever since I meet this man, my life is not the same.
And Nicolai Ivanovich Lobachevsky is his name. Oy!
Nicolai Ivanovich Lobach…
In lyrics of his song 'Lobachevski' recorded on his first album Songs by Tom Lehrer (1953). First performed as part a musical revue, The Physical Revue (1951–1952).
Who, by vigor of mind almost divine, the motions and figures of the planets, the paths of comets, and the tides of the seas, his mathematics first demonstrated.
English translation of the epitaph inscribed in Latin on the monument beside his grave in Westminster Abbey. Seen, for example as epigraph, without citation, in Morris Kline, Mathematical Thought from Ancient to Modern Times (1972), 342. The original Latin is, “Qui, animi vi prope divinâ, Planetarum Motus, Figuras, Cometarum semitas, Oceanique Aestus, Suâ Mathesi facem praeferente Primus demonstravit:” as given in Le journal des sçavans, pour l'année MDCCXXXI (Jul 1731), 438. The words “his mathematics” are missing from most quotes of this epitaph, but have been added by Webmaster for the Latin words “Suâ Mathesi” which are present in the verbatim epitaph.
Whoever despises the high wisdom of mathematics nourishes himself on delusion.
As quoted, without citation, in Nicholas J. Rose, Mathematical Maxims and Minims (1988).
Winwood Reade … remarks that while a man is an insoluble puzzle, in the aggregate he becomes a mathematical certainty. You can, for example, never foretell what any one man will do, but you can say with precision what an average number will be up to. Individuals vary, but percentages remain constant. So says the statistician.
Character Sherlock Holmes recommends Winwood Reade’s book The Martyrdom of Man to Dr. Watson in The Sign of the Four (1890), 196. Earlier in the novel, Holmes calls Reade’s book “one of the most remarkable ever penned.” Reade is a real person and his book was published in 1872. The actual statement in it reads: “As a single atom man is an enigma: as a whole he is a mathematical problem.”
With the exception of the geometrical series, there does not exist in all of mathematics a single infinite series the sum of which has been rigorously determined. In other words, the things which are the most important in mathematics are also those which have the least foundation.
From letter (Jan 1828) to his former teacher Berndt Holmböe. In Morris Kline, Mathematics: The Loss of Certainty (1982), 170.
With the extension of mathematical knowledge will it not finally become impossible for the single investigator to embrace all departments of this knowledge? In answer let me point out how thoroughly it is ingrained in mathematical science that every real advance goes hand in hand with the invention of sharper tools and simpler methods which, at the same time, assist in understanding earlier theories and in casting aside some more complicated developments.
In 'Mathematical Problems', Lecture at the International Congress of Mathematics, Paris, (8 Aug 1900). Translated by Dr. Maby Winton Newson in Bulletin of the American Mathematical Society (1902), 8, 479. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 94-95. It is reprinted in Jeremy Gray, The Hilbert Challenge (2000), 282.
Without doubt one of the most characteristic features of mathematics in the last century is the systematic and universal use of the complex variable. Most of its great theories received invaluable aid from it, and many owe their very existence to it.
In 'History of Mathematics in the Nineteenth Century', Congress of Arts and Sciences (1905), Vol. 1, 474. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 115.
Without the concepts, methods and results found and developed by previous generations right down to Greek antiquity one cannot understand either the aims or achievements of mathematics in the last fifty years.
In 'A Half-Century of Mathematics', The American Mathematical Monthly, 58, No. 8, 523.
Without this language [mathematics] most of the intimate analogies of things would have remained forever unknown to us; and we should forever have been ignorant of the internal harmony of the world, which is the only true objective reality. …
This harmony … is the sole objective reality, the only truth we can attain; and when I add that the universal harmony of the world is the source of all beauty, it will be understood what price we should attach to the slow and difficult progress which little by little enables us to know it better.
This harmony … is the sole objective reality, the only truth we can attain; and when I add that the universal harmony of the world is the source of all beauty, it will be understood what price we should attach to the slow and difficult progress which little by little enables us to know it better.
From La Valeur de la Science, as translated by George Bruce Halsted, in 'The Value of Science', Popular Science Monthly (Sep 1906), 69 195-196.
Would it sound too presumptuous to speak of perception as a quintessence of sensation, language (that is, communicable thought) of perception, mathematics of language? We should then have four terms differentiating from inorganic matter and from each other the Vegetable, Animal, Rational, and Super-sensual modes of existence.
From Presidential Address (1869) to the British Association, Exeter, Section A, collected in Collected Mathematical Papers of James Joseph Sylvester (1908), Vol. 2, 652, footnote.
Would you have a man reason well, you must use him to it betimes; exercise his mind in observing the connection between ideas, and following them in train. Nothing does this better than mathematics, which therefore, I think should be taught to all who have the time and opportunity, not so much to make them mathematicians, as to make them reasonable creatures; for though we all call ourselves so, because we are born to it if we please, yet we may truly say that nature gives us but the seeds of it, and we are carried no farther than industry and application have carried us.
In Conduct of the Understanding, Sect. 6.
Yes, we have to divide up our time like that, between our politics and our equations. But to me our equations are far more important, for politics are only a matter of present concern. A mathematical equation stands forever.
…...
You are surprised at my working simultaneously in literature and in mathematics. Many people who have never had occasion to learn what mathematics is confuse it with arithmetic and consider it a dry and arid science. In actual fact it is the science which demands the utmost imagination. One of the foremost mathematicians of our century says very justly that it is impossible to be a mathematician without also being a poet in spirit. It goes without saying that to understand the truth of this statement one must repudiate the old prejudice by which poets are supposed to fabricate what does not exist, and that imagination is the same as “making things up”. It seems to me that the poet must see what others do not see, and see more deeply than other people. And the mathematician must do the same.
In letter (1890), quoted in S. Kovalevskaya and Beatrice Stillman (trans. and ed.), Sofia Kovalevskaya: A Russian Childhood (2013), 35. Translated the Russian edition of Vospominaniya detstva (1974).
You can't go by mathematics: the dollar you borrow is never as big as the dollar you pay back.
In Evan Esar, 20,000 Quips and Quotes, 240.
You know we’re constantly taking. We don’t make most of the food we eat, we don’t grow it, anyway. We wear clothes other people make, we speak a language other people developed, we use a mathematics other people evolved and spent their lives building. I mean we’re constantly taking things. It’s a wonderful ecstatic feeling to create something and put it into the pool of human experience and knowledge.
Expressing the driving force behind his passion. Interview with Rolling Stone writer, Steven Levy (late Nov 1983). As quoted in Nick Bilton, 'The 30-Year-Old Macintosh and a Lost Conversation With Steve Jobs' (24 Jan 2014), on New York Times blog web page. Levy appended a transcript of the interview to an updated Kindle version of his book, Insanely Great: The Life and Times of Macintosh, the Computer that Changed Everything.
You may object that by speaking of simplicity and beauty I am introducing aesthetic criteria of truth, and I frankly admit that I am strongly attracted by the simplicity and beauty of mathematical schemes which nature presents us. You must have felt this too: the almost frightening simplicity and wholeness of the relationship, which nature suddenly spreads out before us.
Letter to Albert Einstein. In Ian Stewart, Why Beauty is Truth (), 278.
You propound a complicated arithmetical problem: say cubing a number containing four digits. Give me a slate and half an hour’s time, and I can produce a wrong answer.
Cashel Byron's Profession (1886, 1901), xxiii.
You treat world history as a mathematician does mathematics, in which nothing but laws and formulae exist, no reality, no good and evil, no time, no yesterday, no tomorrow, nothing but an eternal, shallow, mathematical present.
From Das Glasperlemspeil (1943) translated as The Glass Bead Game (1969, 1990), 168.
You’re aware the boy failed my grade school math class, I take it? And not that many years later he’s teaching college. Now I ask you: Is that the sorriest indictment of the American educational system you ever heard? [pauses to light cigarette.] No aptitude at all for long division, but never mind. It’s him they ask to split the atom. How he talked his way into the Nobel prize is beyond me. But then, I suppose it’s like the man says, it’s not what you know...
Karl Arbeiter (former teacher of Albert Einstein)






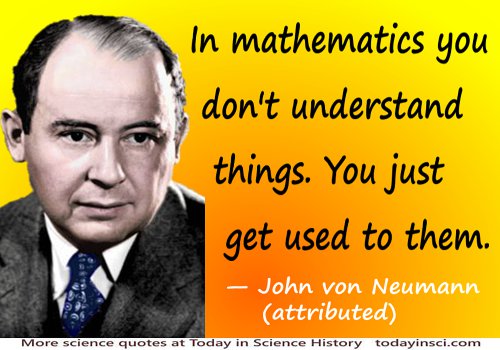



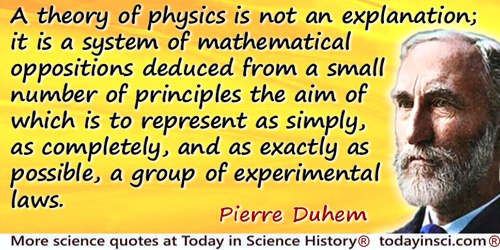
























![Carl Friedrich Gauss quote: Mathematics is the queen of the sciences and arithmetic [number theory] is the queen of mathematics.](https://todayinsci.com/G/Gauss_Carl/GaussCarl-QueenThm.jpg)


![Martin Gardner quote: Mathematics, a creation of the mind, so accurately fits the outside world. … [There is a] fantastic amount](https://todayinsci.com/G/Gardner_Martin/GardnerMartin-FormulasThm.jpg)













/AdamsJohn(1735-1826)-StudyThm.jpg)










 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


