Rigour Quotes (21 quotes)
[The Royal Society] is quite simply the voice of science in Britain. It is intellectually rigorous, not afraid to be outspoken on controversial issues such as climate change, but it is not aggressively secular either, insisting on a single view of the world. In fact, there are plenty of eminent scientists – Robert Winston, for instance – who are also men of faith.
Quoted in Max Davidson, 'Bill Bryson: Have faith, science can solve our problems', Daily Telegraph (26 Sep 2010)
As for methods I have sought to give them all the rigour that one requires in geometry, so as never to have recourse to the reasons drawn from the generality of algebra.
In Cours d’analyse (1821), Preface, trans. Ivor Grattan-Guinness.
As in the experimental sciences, truth cannot be distinguished from error as long as firm principles have not been established through the rigorous observation of facts.
Ésur la maladie des vers ásoie (1870), 39.
Besides it is an error to believe that rigour is the enemy of simplicity. On the contrary we find it confirmed by numerous examples that the rigorous method is at the same time the simpler and the more easily comprehended. The very effort for rigor forces us to find out simpler methods of proof.
'Mathematical Problems', Bulletin of the American Mathematical Society (Jul 1902), 8, 441.
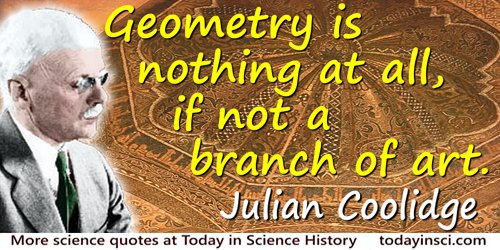
Every writer must reconcile, as best he may, the conflicting claims of consistency and variety, of rigour in detail and elegance in the whole. The present author humbly confesses that, to him, geometry is nothing at all, if not a branch of art.
Concluding remark in preface to Treatise on Algebraic Plane Curves (1931), x.
Exercise in the most rigorous thinking that is possible will of its own accord strengthen the sense of truth and right, for each advance in the ability to distinguish between correct and false thoughts, each habit making for rigour in thought development will increase in the sound pupil the ability and the wish to ascertain what is right in life and to defend it.
In Anleitung zum mathematischen Unterricht in den höheren Schulen (1906), 28.
Harvard Law: Under the most rigorously controlled conditions of pressure, temperature, humidity, and other variables, the organism will do as it damn well pleases.
The Coevolution Quarterly, Nos. 8-12 (1975), 138.
I shall devote all my efforts to bring light into the immense obscurity that today reigns in Analysis. It so lacks any plan or system, that one is really astonished that so many people devote themselves to it—and, still worse, it is absolutely devoid of any rigour.
In Oeuvres (1826), Vol. 2, 263. As translated and cited in Ernst Hairer and Gerhard Wanner Analysis by Its History (2008), 188. From the original French, “Je consacrerai toutes mes forces à répandre de la lumière sur l’immense obscurité qui règne aujourd’hui dans l’Analyse. Elle est tellement dépourvue de tout plan et de tout système, qu’on s’étonne seulement qu’il y ait tant de gens qui s’y livrent—et ce qui pis est, elle manque absolument de rigueur.”
In order to comprehend and fully control arithmetical concepts and methods of proof, a high degree of abstraction is necessary, and this condition has at times been charged against arithmetic as a fault. I am of the opinion that all other fields of knowledge require at least an equally high degree of abstraction as mathematics,—provided, that in these fields the foundations are also everywhere examined with the rigour and completeness which is actually necessary.
In 'Die Theorie der algebraischen Zahlkorper', Vorwort, Jahresbericht der Deutschen Mathematiker Vereinigung, Bd. 4.
In the mathematical investigations I have usually employed such methods as present themselves naturally to a physicist. The pure mathematician will complain, and (it must be confessed) sometimes with justice, of deficient rigour. But to this question there are two sides. For, however important it may be to maintain a uniformly high standard in pure mathematics, the physicist may occasionally do well to rest content with arguments which are fairly satisfactory and conclusive from his point of view. To his mind, exercised in a different order of ideas, the more severe procedure of the pure mathematician may appear not more but less demonstrative. And further, in many cases of difficulty to insist upon the highest standard would mean the exclusion of the subject altogether in view of the space that would be required.
In Preface to second edition, The Theory of Sound (1894), Vol. 1, vii.
It may be observed of mathematicians that they only meddle with such things as are certain, passing by those that are doubtful and unknown. They profess not to know all things, neither do they affect to speak of all things. What they know to be true, and can make good by invincible arguments, that they publish and insert among their theorems. Of other things they are silent and pass no judgment at all, chusing [choosing] rather to acknowledge their ignorance, than affirm anything rashly. They affirm nothing among their arguments or assertions which is not most manifestly known and examined with utmost rigour, rejecting all probable conjectures and little witticisms. They submit nothing to authority, indulge no affection, detest subterfuges of words, and declare their sentiments, as in a Court of Judicature [Justice], without passion, without apology; knowing that their reasons, as Seneca testifies of them, are not brought to persuade, but to compel.
Mathematical Lectures (1734), 64.
It really is worth the trouble to invent a new symbol if we can thus remove not a few logical difficulties and ensure the rigour of the proofs. But many mathematicians seem to have so little feeling for logical purity and accuracy that they will use a word to mean three or four different things, sooner than make the frightful decision to invent a new word.
Grundgesetz der Arithmetik(1893), Vol. 2, Section 60, In P. Greach and M. Black (eds., Translations from the Philosophical Writings of Gottlob Frege (1952), 144.
Mathematics is not a careful march down a well-cleared highway, but a journey into a strange wilderness, where the explorers often get lost. Rigour should be a signal to the historian that the maps have been made, and the real explorers have gone elsewhere.
'Mathematics and History', Mathematical Intelligencer (1992), 4, No. 4, 10.
Mathematics, among all school subjects, is especially adapted to further clearness, definite brevity and precision in expression, although it offers no exercise in flights of rhetoric. This is due in the first place to the logical rigour with which it develops thought, avoiding every departure from the shortest, most direct way, never allowing empty phrases to enter. Other subjects excel in the development of expression in other respects: translation from foreign languages into the mother tongue gives exercise in finding the proper word for the given foreign word and gives knowledge of laws of syntax, the study of poetry and prose furnish fit patterns for connected presentation and elegant form of expression, composition is to exercise the pupil in a like presentation of his own or borrowed thoughtsand their development, the natural sciences teach description of natural objects, apparatus and processes, as well as the statement of laws on the grounds of immediate sense-perception. But all these aids for exercise in the use of the mother tongue, each in its way valuable and indispensable, do not guarantee, in the same manner as mathematical training, the exclusion of words whose concepts, if not entirely wanting, are not sufficiently clear. They do not furnish in the same measure that which the mathematician demands particularly as regards precision of expression.
In Anleitung zum mathematischen Unterricht in höheren Schulen (1906), 17.
One of the principal obstacles to the rapid diffusion of a new idea lies in the difficulty of finding suitable expression to convey its essential point to other minds. Words may have to be strained into a new sense, and scientific controversies constantly resolve themselves into differences about the meaning of words. On the other hand, a happy nomenclature has sometimes been more powerful than rigorous logic in allowing a new train of thought to be quickly and generally accepted.
Opening Address to the Annual Meeting of the British Association by Prof. Arthur Schuster, in Nature (4 Aug 1892), 46, 325.
The great artifice of regarding small deviations from the truth as being the truth itself is at the same time the foundation of wit, where the whole thing would often collapse if we were to regard these deviations in a spirit of philosophical rigor.
Aphorism from Georg Christoph Lichtenberg and R.J. Hollingdale (trans.) 'Notebook A: 1765-1770', The Waste Books (1990), 4. Also seen translated as, “The great trick of regarding small departures from the truth as the truth itself—on which is founded the entire integral calculus—is also the basis of our witty speculations, where the whole thing would often collapse if we considered the departures with philosophical rigour,” for example, as quoted in FractalVision: Put Fractals to Work For You (1992), 5, citing Aphorisms: 1764-1799.
The study of mathematics is apt to commence in disappointment. The important applications of the science, the theoretical interest of its ideas, and the logical rigour of its methods all generate the expectation of a speedy introduction to processes of interest. We are told that by its aid the stars are weighed and the billions of molecules in a drop of water are counted. Yet, like the ghost of Hamlet's father, this great science eludes the efforts of our mental weapons to grasp it.
Opening to An Introduction to Mathematics (1911), 7.
There is no rigorous definition of rigor.
In Mathematics: The Loss of Certainty (1982), 315.
These sciences, Geometry, Theoretical Arithmetic and Algebra, have no principles besides definitions and axioms, and no process of proof but deduction; this process, however, assuming a most remarkable character; and exhibiting a combination of simplicity and complexity, of rigour and generality, quite unparalleled in other subjects.
In The Philosophy of the Inductive Sciences (1858), Part 1, Bk. 2, chap. 1, sect. 2.
Those of us who were familiar with the state of inorganic chemistry in universities twenty to thirty years ago will recall that at that time it was widely regarded as a dull and uninteresting part of the undergraduate course. Usually, it was taught almost entirely in the early years of the course and then chiefly as a collection of largely unconnected facts. On the whole, students concluded that, apart from some relationships dependent upon the Periodic table, there was no system in inorganic chemistry comparable with that to be found in organic chemistry, and none of the rigour and logic which characterised physical chemistry. It was widely believed that the opportunities for research in inorganic chemistry were few, and that in any case the problems were dull and uninspiring; as a result, relatively few people specialized in the subject... So long as inorganic chemistry is regarded as, in years gone by, as consisting simply of the preparations and analysis of elements and compounds, its lack of appeal is only to be expected. The stage is now past and for the purpose of our discussion we shall define inorganic chemistry today as the integrated study of the formation, composition, structure and reactions of the chemical elements and compounds, excepting most of those of carbon.
Inaugural Lecture delivered at University College, London (1 Mar 1956). In The Renaissance of Inorganic Chemistry (1956), 4-5.
While it is never safe to affirm that the future of Physical Science has no marvels in store even more astonishing than those of the past, it seems probable that most of the grand underlying principles have been firmly established and that further advances are to be sought chiefly in the rigorous application of these principles to all the phenomena which come under our notice.
'Spectroscopy, Molecular Orbitals, and Chemical Bonding', Nobel Lecture (12 Dec 1966). In Nobel Lectures: Chemistry 1963-1970 (1972), 159.
 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


