“But in the binary system,” Dale points out, handing back the squeezable glass, “the alternative to one isn’t minus one, it’s zero. That’s the beauty of it, mechanically.” “O.K. Gotcha. You’re asking me, What’s this minus one? I’ll tell you. It’s a plus one moving backward in time. This is all in the space-time foam, inside the Planck duration, don’t forget. The dust of points gives birth to time, and time gives birth to the dust of points. Elegant, huh? It has to be. It’s blind chance, plus pure math. They’re proving it, every day. Astronomy, particle physics, it’s all coming together. Relax into it, young fella. It feels great. Space-time foam.”

[About mathematicians’ writings] Extreme external elegance, sometimes a somewhat weak skeleton of conclusions characterizes the French; the English, above all Maxwell, are distinguished by the greatest dramatic bulk.

[Thomas Jefferson] played a major role in one of the great tragedies of recent World history, a tragedy which he so elegantly mourned: the dispossession and decimation of the first Americans.

[About Sir Roderick Impey Murchison:] The enjoyments of elegant life you early chose to abandon, preferring to wander for many successive years over the rudest portions of Europe and Asia—regions new to Science—in the hope, happily realized, of winning new truths.
By a rare union of favourable circumstances, and of personal qualifications equally rare, you have thus been enabled to become the recognized Interpreter and Historian (not without illustrious aid) of the Silurian Period.

~~[Unverified]~~ Why has elegance found so little following? Elegance has the disadvantage that hard work is needed to achieve it and a good education to appreciate it.

A troubling question for those of us committed to the widest application of intelligence in the study and solution of the problems of men is whether a general understanding of the social sciences will be possible much longer. Many significant areas of these disciplines have already been removed by the advances of the past two decades beyond the reach of anyone who does not know mathematics; and the man of letters is increasingly finding, to his dismay, that the study of mankind proper is passing from his hands to those of technicians and specialists. The aesthetic effect is admittedly bad: we have given up the belletristic “essay on man” for the barbarisms of a technical vocabulary, or at best the forbidding elegance of mathematical syntax.

As an Art, Mathematics has its own standard of beauty and elegance which can vie with the more decorative arts. In this it is diametrically opposed to a Baroque art which relies on a wealth of ornamental additions. Bereft of superfluous addenda, Mathematics may appear, on first acquaintance, austere and severe. But longer contemplation reveals the classic attributes of simplicity relative to its significance and depth of meaning.

Elegance is not a dispensable luxury but a quality that decides between success and failure.

Equations are Expressions of Arithmetical Computation, and properly have no place in Geometry, except as far as Quantities truly Geometrical (that is, Lines, Surfaces, Solids, and Proportions) may be said to be some equal to others. Multiplications, Divisions, and such sort of Computations, are newly received into Geometry, and that unwarily, and contrary to the first Design of this Science. For whosoever considers the Construction of a Problem by a right Line and a Circle, found out by the first Geometricians, will easily perceive that Geometry was invented that we might expeditiously avoid, by drawing Lines, the Tediousness of Computation. Therefore these two Sciences ought not to be confounded. The Ancients did so industriously distinguish them from one another, that they never introduced Arithmetical Terms into Geometry. And the Moderns, by confounding both, have lost the Simplicity in which all the Elegance of Geometry consists. Wherefore that is Arithmetically more simple which is determined by the more simple Equation, but that is Geometrically more simple which is determined by the more simple drawing of Lines; and in Geometry, that ought to be reckoned best which is geometrically most simple.

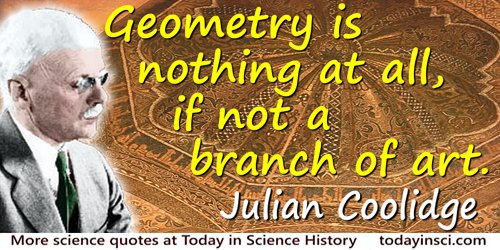
Every writer must reconcile, as best he may, the conflicting claims of consistency and variety, of rigour in detail and elegance in the whole. The present author humbly confesses that, to him, geometry is nothing at all, if not a branch of art.

Fossils, be it understood, are given botanical names and spoken of as if they were species, but they are merely the bits, however wonderfully preserved, of extinct beings: we are apt to forget in the explicit elegance of the structures made by cell-walls, that it is the living protoplasm which is plant life and makes species.

Generality of points of view and of methods, precision and elegance in presentation, have become, since Lagrange, the common property of all who would lay claim to the rank of scientific mathematicians. And, even if this generality leads at times to abstruseness at the expense of intuition and applicability, so that general theorems are formulated which fail to apply to a single special case, if furthermore precision at times degenerates into a studied brevity which makes it more difficult to read an article than it was to write it; if, finally, elegance of form has well-nigh become in our day the criterion of the worth or worthlessness of a proposition,—yet are these conditions of the highest importance to a wholesome development, in that they keep the scientific material within the limits which are necessary both intrinsically and extrinsically if mathematics is not to spend itself in trivialities or smother in profusion.

He was an admirable marksman, an expert swimmer, a clever rider, possessed of great activity [and] prodigious strength, and was notable for the elegance of his figure and the beauty of his features, and he aided nature by a careful attendance to his dress. Besides other accomplishments he was musical, a good fencer, danced well, and had some acquaintance with legerdemain tricks, worked in hair, and could plait willow baskets.

How do we convince people that in programming simplicity and clarity–in short: what mathematicians call ‘elegance’–are not a dispensable luxury, but a crucial matter that decides between success and failure?

I have always been very fond of mathematics—for one short period, I even toyed with the possibility of abandoning chemistry in its favour. I enjoyed immensely both its conceptual and formal beauties, and the precision and elegance of its relationships and transformations. Why then did I not succumb to its charms? … because by and large, mathematics lacks the sensuous elements which play so large a role in my attraction to chemistry.I love crystals, the beauty of their forms and formation; liquids, dormant, distilling, sloshing! The fumes, the odors—good or bad, the rainbow of colors; the gleaming vessels of every size, shape and purpose.

I have the vagary of taking a lively interest in mathematical subjects only where I may anticipate ingenious association of ideas and results recommending themselves by elegance or generality.

If you are out to describe the truth, leave elegance to the tailor.
On being reproached that his formula of gravitation was longer and more cumbersome than Newton’s.

In some respects, science has far surpassed religion in delivering awe. How is it that hardly any major religion has looked at science and concluded, “This is better than we thought! The Universe is much bigger than our prophets said, grander, more subtle, more elegant. God must be even greater than we dreamed”? Instead they say, 'No, no, no! My god is a little god, and I want him to stay that way.'

Mathematicians attach great importance to the elegance of their methods and their results. This is not pure dilettantism. What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details. But this is exactly what yields great results, in fact the more we see this aggregate clearly and at a single glance, the better we perceive its analogies with other neighboring objects, consequently the more chances we have of divining the possible generalizations. Elegance may produce the feeling of the unforeseen by the unexpected meeting of objects we are not accustomed to bring together; there again it is fruitful, since it thus unveils for us kinships before unrecognized. It is fruitful even when it results only from the contrast between the simplicity of the means and the complexity of the problem set; it makes us then think of the reason for this contrast and very often makes us see that chance is not the reason; that it is to be found in some unexpected law. In a word, the feeling of mathematical elegance is only the satisfaction due to any adaptation of the solution to the needs of our mind, and it is because of this very adaptation that this solution can be for us an instrument. Consequently this esthetic satisfaction is bound up with the economy of thought.

Mathematics is much more than a language for dealing with the physical world. It is a source of models and abstractions which will enable us to obtain amazing new insights into the way in which nature operates. Indeed, the beauty and elegance of the physical laws themselves are only apparent when expressed in the appropriate mathematical framework.

Much as I admired the elegance of physical theories, which at that time geology wholly lacked, I preferred a life in the woods to one in the laboratory.

My colleagues in elementary particle theory in many lands [and I] are driven by the usual insatiable curiosity of the scientist, and our work is a delightful game. I am frequently astonished that it so often results in correct predictions of experimental results. How can it be that writing down a few simple and elegant formulae, like short poems governed by strict rules such as those of the sonnet or the waka, can predict universal regularities of Nature?

Nature seems to take advantage of the simple mathematical representations of the symmetry laws. When one pauses to consider the elegance and the beautiful perfection of the mathematical reasoning involved and contrast it with the complex and far-reaching physical consequences, a deep sense of respect for the power of the symmetry laws never fails to develop.

Nothing could be more admirable than the manner in which for forty years he [Joseph Black] performed this useful and dignified office. His style of lecturing was as nearly perfect as can well be conceived; for it had all the simplicity which is so entirely suited to scientific discourse, while it partook largely of the elegance which characterized all he said or did … I have heard the greatest understandings of the age giving forth their efforts in its most eloquent tongues—have heard the commanding periods of Pitt’s majestic oratory—the vehemence of Fox’s burning declamation—have followed the close-compacted chain of Grant’s pure reasoning—been carried away by the mingled fancy, epigram, and argumentation of Plunket; but I should without hesitation prefer, for mere intellectual gratification (though aware how much of it is derived from association), to be once more allowed the privilege which I in those days enjoyed of being present while the first philosopher of his age was the historian of his own discoveries, and be an eyewitness of those experiments by which he had formerly made them, once more performed with his own hands.

Perhaps we see equations as simple because they are easily expressed in terms of mathematical notation already invented at an earlier stage of development of the science, and thus what appears to us as elegance of description really reflects the interconnectedness of Nature's laws at different levels.

Since natural selection demands only adequacy, elegance of design is not relevant; any combination of behavioural adjustment, physiological regulation, or anatomical accommodation that allows survival and reproduction may be favoured by selection. Since all animals are caught in a phylogenetic trap by the nature of past evolutionary adjustments, it is to be expected that a given environmental challenge will be met in a variety of ways by different animals. The delineation of the patterns of the accommodations of diverse types of organisms to the environment contributes much of the fascination of ecologically relevant physiology.

Since the beginning of the century, computational procedures have become so complicated that any progress by those means has become impossible, without the elegance which modern mathematicians have brought to bear on their research, and by means of which the spirit comprehends quickly and in one step a great many computations.
It is clear that elegance, so vaunted and so aptly named, can have no other purpose. …
[But, the simplifications produced by this elegance will soon outrun the problems supplied by analysis. What happens then?]
Go to the roots, of these calculations! Group the operations. Classify them according to their complexities rather than their appearances! This, I believe, is the mission of future mathematicians. This is the road on which I am embarking in this work.

The elegance of a mathematical theorem is directly proportional to the number of independent ideas one can see in the theorem and inversely proportional to the effort it takes to see them.

The framing of hypotheses is, for the enquirer after truth, not the end, but the beginning of his work. Each of his systems is invented, not that he may admire it and follow it into all its consistent consequences, but that he may make it the occasion of a course of active experiment and observation. And if the results of this process contradict his fundamental assumptions, however ingenious, however symmetrical, however elegant his system may be, he rejects it without hesitation. He allows no natural yearning for the offspring of his own mind to draw him aside from the higher duty of loyalty to his sovereign, Truth, to her he not only gives his affections and his wishes, but strenuous labour and scrupulous minuteness of attention.

The mathematical talent of Cayley was characterized by clearness and extreme elegance of analytical form; it was re-enforced by an incomparable capacity for work which has caused the distinguished scholar to be compared with Cauchy.

The moment you encounter string theory and realise that almost all of the major developments in physics over the last hundred years emerge—and emerge with such elegance—from such a simple starting point, you realise that this incredibly compelling theory is in a class of its own.

There is a great deal of emotional satisfaction in the elegant demonstration, in the elegant ordering of facts into theories, and in the still more satisfactory, still more emotionally exciting discovery that the theory is not quite right and has to be worked over again, very much as any other work of art—a painting, a sculpture has to be worked over in the interests of aesthetic perfection. So there is no scientist who is not to some extent worthy of being described as artist or poet.

There was, I think, a feeling that the best science was that done in the simplest way. In experimental work, as in mathematics, there was “style” and a result obtained with simple equipment was more elegant than one obtained with complicated apparatus, just as a mathematical proof derived neatly was better than one involving laborious calculations. Rutherford's first disintegration experiment, and Chadwick's discovery of the neutron had a “style” that is different from that of experiments made with giant accelerators.

Think, for a moment, of a cheetah, a sleek, beautiful animal, one of the fastest on earth, which roams freely on the savannas of Africa. In its natural habitat, it is a magnificent animal, almost a work of art, unsurpassed in speed or grace by any other animal. Now, think of a cheetah that has been captured and thrown into a miserable cage in a zoo. It has lost its original grace and beauty, and is put on display for our amusement. We see only the broken spirit of the cheetah in the cage, not its original power and elegance. The cheetah can be compared to the laws of physics, which are beautiful in their natural setting. The natural habitat of the laws of physics is a higher-dimensional space-time. However, we can only measure the laws of physics when they have been broken and placed on display in a cage, which is our three-dimensional laboratory. We only see the cheetah when its grace and beauty have been stripped away.

This is true of all science. Successes were largely due to forgetting completely about what one ultimately wanted, or whether one wanted anything ultimately; in refusing to investigate things which profit, and in relying solely on guidance by criteria of intellectual elegance. … And I think it extremely instructive to watch the role of science in everyday life, and to note how in this area the principle of laissez faire has led to strange and wonderful results.

What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details.

What the scientists have always found by physical experiment was an a priori orderliness of nature, or Universe always operating at an elegance level that made the discovering scientist’s working hypotheses seem crude by comparison. The discovered reality made the scientists’ exploratory work seem relatively disorderly.

Whatever is Natural doth by that appear, adorned with all imaginable Elegance and Beauty. There are such inimitable gildings and embroideries in the smallest seeds of Plants, but especially in the parts of Animals, in the head or eye of a small Fly: such accurate order and symmetry in the frame of the most minute creatures, a Lowse or a Mite, as no man were able to conceive without seeing of them. Whereas the most curious works of Art, the sharpest finest Needle, doth appear as a blunt rough bar of iron, coming from the furnace or the forge. The most accurate engravings or embossments, seem such rude bungling deformed works, as if they had been done with a Mattock or a Trowel.

When I saw the alpha-helix and saw what a beautiful, elegant structure it was, I was thunderstruck and was furious with myself for not having built this, but on the other hand, I wondered, was it really right?
So I cycled home for lunch and was so preoccupied with the turmoil in my mind that didn’t respond to anything. Then I had an idea, so I cycled back to the lab. I realized that I had a horse hair in a drawer. I set it up on the X-ray camera and gave it a two hour exposure, then took the film to the dark room with my heart in my mouth, wondering what it showed, and when I developed it, there was the 1.5 angstrom reflection which I had predicted and which excluded all structures other than the alpha-helix.
So on Monday morning I stormed into my professor’s office, into Bragg’s office and showed him this, and Bragg said, 'Whatever made you think of that?' And I said, 'Because I was so furious with myself for having missed that beautiful structure.' To which Bragg replied coldly, 'I wish I had made you angry earlier.'

While Occam’s razor is a useful tool in the physical sciences, it can be a very dangerous implement in biology. It is thus very rash to use simplicity and elegance as a guide in biological research.