Simple Quotes (426 quotes)
...it would be a simple way of solving the goiter problem. And in addition to that it would be the biggest thing in a medical proposition to be carried out in the state of Michigan, and Michigan is a large place. And as I thought of the thing the more convinced I became that this oughtn't to be a personal thing, This ought to be something done by the Michigan State Medical Society as a body.
Recommending the addition of a trace of iodine to table salt.
Recommending the addition of a trace of iodine to table salt.
Opening address to the Medical Department of the University of Michigan, Sep 1914. Quoted by Howard Markel in 'When it Rains it Pours' : Endemic Goiter, Iodized Salt, and David Murray Cowie, M.D. American Journal of Public Health, Feb 1987, vol.77, No.2, page 222.
“If there are two theories, one simpler man the other, the simpler one is to be preferred.” At first sight this does not seem quite so bad, but a little thought shows that our tendency to prefer the simpler possibility is psychological rather than scientific. It is less trouble to think that way. Experience invariably shows that the more correct a theory becomes, the more complex does it seem. … So this … interpretation of [Ockham’s Razor] is … worthless.
With co-author Nalin Chandra Wickramasinghe, Evolution from Space (1981), 135.
“Planning” is simply the result of experience read backward and projected into the future. To me the “purposive” action of a beehive is simply the summation and integration of its units, and Natural Selection has put higher and higher premiums on the most “purposeful” integration. It is the same way (to me) in the evolution of the middle ear, the steps in the Cynodonts (clearly shown by me in 1910 and by you later in Oudenodon) make it easier to see how such a wonderful device as the middle ear could arise without any predetermination or human-like planning, and in fact in the good old Darwinian way, if only we admit that as the “twig is bent the tree’s inclined” and that each stage conserves the advantages of its predecessors… The simple idea that planning is only experience read backward and combined by selection in suitable or successful combinations takes the mystery out of Nature and out of men’s minds.
Letter to Robert Broom [1933]. In Ronald Rainger, An Agenda for Antiquity (1991), 238.
[After the flash of the atomic bomb test explosion] Fermi got up and dropped small pieces of paper … a simple experiment to measure the energy liberated by the explosion … [W]hen the front of the shock wave arrived (some seconds after the flash) the pieces of paper were displaced a few centimeters in the direction of propagation of the shock wave. From the distance of the source and from the displacement of the air due to the shock wave, he could calculate the energy of the explosion. This Fermi had done in advance having prepared himself a table of numbers, so that he could tell immediately the energy liberated from this crude but simple measurement. … It is also typical that his answer closely approximated that of the elaborate official measurements. The latter, however, were available only after several days’ study of the records, whereas Fermi had his within seconds.
In Enrico Fermi: Physicist (1970), 147-148.
[Decimal currency is desirable because] by that means all calculations of interest, exchange, insurance, and the like are rendered much more simple and accurate, and, of course, more within the power of the great mass of people. Whenever such things require much labor, time, and reflection, the greater number who do not know, are made the dupes of the lesser number who do.
Letter to Congress (15 Jan 1782). 'Coinage Scheme Proposed by Robert Morris, Superintendent of Finance', from MS. letters and reports of the Superintendent of Finance, No, 137, Vol. 1, 289-300. Reprinted as Appendix, in Executive Documents, Senate of the U.S., Third Session of the Forty-Fifth Congress, 1878-79 (1879), 430.
[Freud's] great strength, though sometimes also his weakness, was the quite extraordinary respect he had for the singular fact... When he got hold of a simple but significant fact he would feel, and know, that it was an example of something general or universal, and the idea of collecting statistics on the matter was quite alien to him.
The Life and Work of Sigmund Freud (1953), Vol 1, 96-7.
[In 18th-century Britain] engineers for the most began as simple workmen, skilful and ambitious but usually illiterate and self-taught. They were either millwrights like Bramah, mechanics like Murdoch and George Stephenson, or smiths like Newcomen and Maudslay.
In Science in History (1969), Vol. 2, 591.
[It] may be laid down as a general rule that, if the result of a long series of precise observations approximates a simple relation so closely that the remaining difference is undetectable by observation and may be attributed to the errors to which they are liable, then this relation is probably that of nature.
'Mémoire sur les Inégalites Séculaires des Planètes et des Satellites' (I 785, published 1787). In Oeuvres completes de Laplace, 14 Vols. (1843-1912), Vol. 11, 57, trans. Charles Coulston Gillispie, Pierre-Simon Laplace 1749-1827: A Life in Exact Science (1997), 130.
[John] Dalton was a man of regular habits. For fifty-seven years he walked out of Manchester every day; he measured the rainfall, the temperature—a singularly monotonous enterprise in this climate. Of all that mass of data, nothing whatever came. But of the one searching, almost childlike question about the weights that enter the construction of these simple molecules—out of that came modern atomic theory. That is the essence of science: ask an impertinent question, and you are on the way to the pertinent answer.
The Ascent of Man (1973), 153.
[On Oxygen, Chlorine, Iodine, Fluorine:] The most important division of ponderable substances seems to be that which represents their electrical energies or their respective inherent states. When the poles of a voltaic apparatus are introduced into a mixture of the simple substances, it is found that four of them go to the positive, while the rest evince their state by passing to the negative pole. As this division coincides with one resulting from a consideration of their most important properties, it is that which I shall adopt as the first.
From 5th Lecture in 1816, in Bence Jones, The Life and Letters of Faraday (1870), Vol. 1, 217-218.
[Science moves] with the spirit of an adventure characterized both by youthful arrogance and by the belief that the truth, once found, would be simple as well as pretty.
In The Double Helix: A Personal Account of the Discovery of the Structure of DNA (1968, 2001), Preface, xi.
[The steamboat] will answer for sea voyages as well as for inland navigation, in particular for packets, where there may be a great number of passengers. He is also of opinion, that fuel for a short voyage would not exceed the weight of water for a long one, and it would produce a constant supply of fresh water. ... [T]he boat would make head against the most violent tempests, and thereby escape the danger of a lee shore; and that the same force may be applied to a pump to free a leaky ship of her water. ... [T]he good effects of the machine, is the almost omnipotent force by which it is actuated, and the very simple, easy, and natural way by which the screws or paddles are turned to answer the purpose of oars.
[This letter was written in 1785, before the first steamboat carried a man (Fitch) on 27 Aug 1787.]
[This letter was written in 1785, before the first steamboat carried a man (Fitch) on 27 Aug 1787.]
Letter to Benjamin Franklin (12 Oct 1785), in The Works of Benjamin Franklin (1882), Vol. 10, 232.
[The surplus of basic knowledge of the atomic nucleus was] largely used up [during the war with the atomic bomb as the dividend.] We must, without further delay restore this surplus in preparation for the important peacetime job for the nucleus - power production. ... Many of the proposed applications of atomic power - even for interplanetary rockets - seem to be within the realm of possibility provided the economic factor is ruled out completely, and the doubtful physical and chemical factors are weighted heavily on the optimistic side. ... The development of economic atomic power is not a simple extrapolation of knowledge gained during the bomb work. It is a new and difficult project to reach a satisfactory answer. Needless to say, it is vital that the atomic policy legislation now being considered by the congress recognizes the essential nature of this peacetime job, and that it not only permits but encourages the cooperative research-engineering effort of industrial, government and university laboratories for the task. ... We must learn how to generate the still higher energy particles of the cosmic rays - up to 1,000,000,000 volts, for they will unlock new domains in the nucleus.
Addressing the American Institute of Electrical Engineering, in New York (24 Jan 1946). In Schenectady Gazette (25 Jan 1946),
[Zoophytes (Protists, or simple life forms) are] the primitive types from which all the organisms of the higher classes had arisen by gradual development.
Entry for Gottfried Reinhold Treviranus in Encyclopedia Britannica (1911), Vol. 27, 255-256.
[When asked “Dr. Einstein, why is it that when the mind of man has stretched so far as to discover the structure of the atom we have been unable to devise the political means to keep the atom from destroying us?”] That is simple, my friend. It is because politics is more difficult than physics.
Einstein’s answer to a conferee at a meeting at Princeton, N.J. (Jan 1946), as recalled by Greenville Clark in 'Letters to the Times', in New York Times (22 Apr 1955), 24.
Are coral reefs growing from the depths of the oceans? ... [The] reply is a simple negative; and a single fact establishes its truth. The reef-forming coral zoophytes, as has been shown, cannot grow at greater depths than 100 or 120 feet; and therefore in seas deeper than this, the formation or growth of reefs over the bottom is impossible.
On Coral Reefs and Islands (1853), 138.
Il ne peut y avoir de langage plus universel et plus simple, plus exempt d’erreurs et d’obscurités, c'est-à-dire plus digne d'exprimer les rapports invariables des êtres naturels.
There cannot be a language more universal and more simple, more free from errors and obscurities, … more worthy to express the invariable relations of all natural things. [About mathematical analysis.]
There cannot be a language more universal and more simple, more free from errors and obscurities, … more worthy to express the invariable relations of all natural things. [About mathematical analysis.]
From Théorie Analytique de la Chaleur (1822), xiv, translated by Alexander Freeman in The Analytical Theory of Heat (1878), 7.
In primis, hominis est propria VERI inquisitio atque investigato. Itaque cum sumus negotiis necessariis, curisque vacui, tum avemus aliquid videre, audire, ac dicere, cognitionemque rerum, aut occultarum aut admirabilium, ad benè beatéque vivendum necessariam ducimus; —ex quo intelligitur, quod VERUM, simplex, sincerumque sit, id esse naturæ hominis aptissimum. Huic veri videndi cupiditati adjuncta est appetitio quædam principatûs, ut nemini parere animus benè a naturâ informatus velit, nisi præcipienti, aut docenti, aut utilitatis causâ justè et legitimè imperanti: ex quo animi magnitudo existit, et humanarum rerum contemtio.
Before all other things, man is distinguished by his pursuit and investigation of TRUTH. And hence, when free from needful business and cares, we delight to see, to hear, and to communicate, and consider a knowledge of many admirable and abstruse things necessary to the good conduct and happiness of our lives: whence it is clear that whatsoever is TRUE, simple, and direct, the same is most congenial to our nature as men. Closely allied with this earnest longing to see and know the truth, is a kind of dignified and princely sentiment which forbids a mind, naturally well constituted, to submit its faculties to any but those who announce it in precept or in doctrine, or to yield obedience to any orders but such as are at once just, lawful, and founded on utility. From this source spring greatness of mind and contempt of worldly advantages and troubles.
Before all other things, man is distinguished by his pursuit and investigation of TRUTH. And hence, when free from needful business and cares, we delight to see, to hear, and to communicate, and consider a knowledge of many admirable and abstruse things necessary to the good conduct and happiness of our lives: whence it is clear that whatsoever is TRUE, simple, and direct, the same is most congenial to our nature as men. Closely allied with this earnest longing to see and know the truth, is a kind of dignified and princely sentiment which forbids a mind, naturally well constituted, to submit its faculties to any but those who announce it in precept or in doctrine, or to yield obedience to any orders but such as are at once just, lawful, and founded on utility. From this source spring greatness of mind and contempt of worldly advantages and troubles.
In De Officiis, Book 1. Sect. 13. As given in epigraph to John Frederick William Herschel, A Preliminary Discourse on the Study of Natural Philosophy (1830), viii.
Les causes primordiales ne nous sont point connues; mais elles sont assujetties à des lois simples et constantes, que l’on peut découvrir par l’observation, et dont l’étude est l’objet de la philosophie naturelle.
Primary causes are unknown to us; but are subject to simple and constant laws, which may be discovered by observation, the study of them being the object of natural philosophy.
Primary causes are unknown to us; but are subject to simple and constant laws, which may be discovered by observation, the study of them being the object of natural philosophy.
Opening statement from 'Discours Préliminaire' to Théorie Analytique de la Chaleur (1822), i, translated by Alexander Freeman in The Analytical Theory of Heat (1878), 1.
Les mathématiciens parviennent à la solution d’un problême par le simple arrangement des données, & en réduisant le raisonnement à des opérations si simples, à des jugemens si courts, qu’ils ne perdent jamais de vue l’évidence qui leur sert de guide.
Mathematicians come to the solution of a problem by the simple arrangement of the data, and reducing the reasoning to such simple operations, to judgments so brief, that they never lose sight of the evidence that serves as their guide.
Mathematicians come to the solution of a problem by the simple arrangement of the data, and reducing the reasoning to such simple operations, to judgments so brief, that they never lose sight of the evidence that serves as their guide.
From a paper read to the Académie Royales des Sciences (18 Apr 1787), printed in Méthode de Nomenclature Chimique (1787), 12. Translation from the French by Webmaster.
Neumann, to a physicist seeking help with a difficult problem: Simple. This can be solved by using the method of characteristics.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Attributed, as related by Dr. Felix Smith (Head of Molecular Physics, Stanford Research Institute) to author Gary Zukav, who quoted it in The Dancing Wu Li Masters: An Overview of the New Physics (1979, 2001), 208, footnote. The physicist (a friend of Dr. Smith) worked at Los Alamos after WW II. It should be noted that although the author uses quotation marks around the spoken remarks, that they represent the author's memory of Dr. Smith's recollection, who heard it from the physicist. Therefore the fourth-hand wording is very likely not verbatim. Webmaster finds Zukav's book seems to be the only source for this quote.
Newsreader: A huge asteroid could destroy Earth! And by coincidence, that's the subject of tonight's miniseries.
Dogbert: In science, researchers proved that this simple device can keep idiots off your television screen. [TV remote control] Click.
Dogbert: In science, researchers proved that this simple device can keep idiots off your television screen. [TV remote control] Click.
Dilbert cartoon strip (30 Apr 1993).
Simplicibus itaque verbis gaudet Mathematica Veritas, cum etiam per se simplex sit Veritatis oratio. (So Mathematical Truth prefers simple words since the language of Truth is itself simple.)
Epistolarum astronomicarum liber primus (1596)
Toutes les fois que dans une équation finale on trouve deux quantités inconnues, on a un lieu, l'extrémité de l'une d’elles décrivant une ligne droite ou courbe. La ligne droite est simple et unique dans son genre; les espèces des courbes sont en nombre indéfini, cercle, parabole, hyperbole, ellipse, etc.
Whenever two unknown magnitudes appear in a final equation, we have a locus, the extremity of one of the unknown magnitudes describing a straight line or a curve. The straight line is simple and unique; the classes of curves are indefinitely many,—circle, parabola, hyperbola, ellipse, etc.
Whenever two unknown magnitudes appear in a final equation, we have a locus, the extremity of one of the unknown magnitudes describing a straight line or a curve. The straight line is simple and unique; the classes of curves are indefinitely many,—circle, parabola, hyperbola, ellipse, etc.
Introduction aux Lieux Plans et Solides (1679) collected in OEuvres de Fermat (1896), Vol. 3, 85. Introduction to Plane and Solid Loci, as translated by Joseph Seidlin in David E. Smith(ed.)A Source Book in Mathematics (1959), 389. Alternate translation using Google Translate: “Whenever in a final equation there are two unknown quantities, there is a locus, the end of one of them describing a straight line or curve. The line is simple and unique in its kind, species curves are indefinite in number,—circle, parabola, hyperbola, ellipse, etc.”
… the really fundamental things have a way of appearing to be simple once they have been stated by a genius. ...
In 'Analytical Function and Mathematical Physics,' Bulletin of the American Mathematical Society, October 1931.
~~[Misattributed]~~ If the human mind were simple enough to understand, we’d be too simple to understand it.
— Pat Bahn
Seen labelled as “Bahn’s Conundrum to Cognitive Theory,” but this is not original to Pat Bahn. It is a variant of a quote traced in 1978 to c.1938 by Emerson M. Pugh, beginning, “If the brain were so simple…,” (q.v., on another page of this website).
1839—The fermentation satire
THE MYSTERY OF ALCOHOLIC FERMENTATION RESOLVED
(Preliminary Report by Letter) Schwindler
I am about to develop a new theory of wine fermentation … Depending on the weight, these seeds carry fermentation to completion somewhat less than as in the beginning, which is understandable … I shall develop a new theory of wine fermentation [showing] what simple means Nature employs in creating the most amazing phenomena. I owe it to the use of an excellent microscope designed by Pistorius.
When brewer’s yeast is mixed with water the microscope reveals that the yeast dissolves into endless small balls, which are scarcely 1/800th of a line in diameter … If these small balls are placed in sugar water, it can be seen that they consist of the eggs of animals. As they expand, they burst, and from them develop small creatures that multiply with unbelievable rapidity in a most unheard of way. The form of these animals differs from all of the 600 types described up until now. They possess the shape of a Beinsdorff still (without the cooling apparatus). The head of the tube is a sort of proboscis, the inside of which is filled with fine bristles 1/2000th of a line long. Teeth and eyes are not discernible; however, a stomach, intestinal canal, anus (a rose red dot), and organs for secretion of urine are plainly discernible. From the moment they are released from the egg one can see these animals swallow the sugar from the solution and pass it to the stomach. It is digested immediately, a process recognized easily by the resultant evacuation of excrements. In a word, these infusors eat sugar, evacuate ethyl alcohol from the intestinal canal, and carbon dioxide from the urinary organs. The bladder, in the filled state, has the form of a champagne bottle; when empty, it is a small button … As soon as the animals find no more sugar present, they eat each other up, which occurs through a peculiar manipulation; everything is digested down to the eggs which pass unchanged through the intestinal canal. Finally, one again fermentable yeast, namely the seed of the animals, which remain over.
THE MYSTERY OF ALCOHOLIC FERMENTATION RESOLVED
(Preliminary Report by Letter) Schwindler
I am about to develop a new theory of wine fermentation … Depending on the weight, these seeds carry fermentation to completion somewhat less than as in the beginning, which is understandable … I shall develop a new theory of wine fermentation [showing] what simple means Nature employs in creating the most amazing phenomena. I owe it to the use of an excellent microscope designed by Pistorius.
When brewer’s yeast is mixed with water the microscope reveals that the yeast dissolves into endless small balls, which are scarcely 1/800th of a line in diameter … If these small balls are placed in sugar water, it can be seen that they consist of the eggs of animals. As they expand, they burst, and from them develop small creatures that multiply with unbelievable rapidity in a most unheard of way. The form of these animals differs from all of the 600 types described up until now. They possess the shape of a Beinsdorff still (without the cooling apparatus). The head of the tube is a sort of proboscis, the inside of which is filled with fine bristles 1/2000th of a line long. Teeth and eyes are not discernible; however, a stomach, intestinal canal, anus (a rose red dot), and organs for secretion of urine are plainly discernible. From the moment they are released from the egg one can see these animals swallow the sugar from the solution and pass it to the stomach. It is digested immediately, a process recognized easily by the resultant evacuation of excrements. In a word, these infusors eat sugar, evacuate ethyl alcohol from the intestinal canal, and carbon dioxide from the urinary organs. The bladder, in the filled state, has the form of a champagne bottle; when empty, it is a small button … As soon as the animals find no more sugar present, they eat each other up, which occurs through a peculiar manipulation; everything is digested down to the eggs which pass unchanged through the intestinal canal. Finally, one again fermentable yeast, namely the seed of the animals, which remain over.
In 'Das entriithselle Geheimiss der geisligen Giihrung', Annalen der Pharmacie und Chemie (1839), 29, 100-104; adapted from English translalion by Ralph E. Oesper, The Human Side of Scientists (1975), 203-205.
A casual glance at crystals may lead to the idea that they were pure sports of nature, but this is simply an elegant way of declaring one’s ignorance. With a thoughtful examination of them, we discover laws of arrangement. With the help of these, calculation portrays and links up the observed results. How variable and at the same time how precise and regular are these laws! How simple they are ordinarily, without losing anything of their significance! The theory which has served to develop these laws is based entirely on a fact, whose existence has hitherto been vaguely discerned rather than demonstrated. This fact is that in all minerals which belong to the same species, these little solids, which are the crystal elements and which I call their integrant molecules, have an invariable form, in which the faces lie in the direction of the natural fracture surfaces corresponding to the mechanical division of the crystals. Their angles and dimensions are derived from calculations combined with observation.
Traité de mineralogie … Publié par le conseil des mines (1801), Vol. 1, xiii-iv, trans. Albert V. and Marguerite Carozzi.
A central lesson of science is that to understand complex issues (or even simple ones), we must try to free our minds of dogma and to guarantee the freedom to publish, to contradict, and to experiment. Arguments from authority are unacceptable.
Billions and Billions: Thoughts on Life and Death at the Brink of the Millenium (1998), 190.
A DNA sequence for the genome of bacteriophage ΦX174 of approximately 5,375 nucleotides has been determined using the rapid and simple “plus and minus” method. The sequence identifies many of the features responsible for the production of the proteins of the nine known genes of the organism, including initiation and termination sites for the proteins and RNAs. Two pairs of genes are coded by the same region of DNA using different reading frames.
[Paper co-author]
[Paper co-author]
Frederick Sanger, et al., 'Nucleotide Sequence of Bacteriophage ΦX174 DNA', Nature (1977), 265, 687.
A fact is a simple statement that everyone believes. It is innocent, unless found guilty. A hypothesis is a novel suggestion that no one wants to believe. It is guilty until found effective.
Edward Teller, Wendy Teller, Wilson Talley, Conversations on the Dark Secrets of Physics (1991, 2002), Footnote, 69.
A great part of its [higher arithmetic] theories derives an additional charm from the peculiarity that important propositions, with the impress of simplicity on them, are often easily discovered by induction, and yet are of so profound a character that we cannot find the demonstrations till after many vain attempts; and even then, when we do succeed, it is often by some tedious and artificial process, while the simple methods may long remain concealed.
Quoted in H. Eves, Mathematical Circles (1977) .
A hypothesis may be simply defined as a guess. A scientific hypothesis is an intelligent guess.
In Isaac Asimov’s Book of Science and Nature Quotations (1988), 114.
A lot of prizes have been awarded for showing the universe is not as simple as we might have thought.
In A Brief History of Time, (1988, 1998), 80.
A marveilous newtrality have these things mathematicall, and also a strange participation between things supernaturall, immortall, intellectuall, simple and indivisible, and things naturall, mortall, sensible, componded and divisible.
— John Dee
In Mathematicall Praeface to the Elements of Geometrie of Euclid of Megara (1570).
A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way.
In A Mathematician’s Apology (1940, 2012), 113.
A modern mathematical proof is not very different from a modern machine, or a modern test setup: the simple fundamental principles are hidden and almost invisible under a mass of technical details.
Unterrichtsblätter für Mathematik und Naturwissenschaften (1932), 38, 177-188. As translated by Abe Shenitzer, in 'Part I. Topology and Abstract Algebra as Two Roads of Mathematical Comprehension', The American Mathematical Monthly (May 1995), 102, No. 7, 453.
A moment’s consideration of this case shows what a really great advance in the theory and practise of breeding has been obtained through the discovery of Mendel’s law. What a puzzle this case would have presented to the biologist ten years ago! Agouti crossed with chocolate gives in the second filial generation (not in the first) four varieties, viz., agouti, chocolate, black and cinnamon. We could only have shaken our heads and looked wise (or skeptical).
Then we had no explanation to offer for such occurrences other than the “instability of color characters under domestication,” the “effects of inbreeding,” “maternal impressions.” Serious consideration would have been given to the proximity of cages containing both black and cinnamon-agouti mice.
Now we have a simple, rational explanation, which anyone can put to the test. We are able to predict the production of new varieties, and to produce them.
We must not, of course, in our exuberance, conclude that the powers of the hybridizer know no limits. The result under consideration consists, after all, only in the making of new combinations of unit characters, but it is much to know that these units exist and that all conceivable combinations of them are ordinarily capable of production. This valuable knowledge we owe to the discoverer and to the rediscoverers of Mendel’s law.
Then we had no explanation to offer for such occurrences other than the “instability of color characters under domestication,” the “effects of inbreeding,” “maternal impressions.” Serious consideration would have been given to the proximity of cages containing both black and cinnamon-agouti mice.
Now we have a simple, rational explanation, which anyone can put to the test. We are able to predict the production of new varieties, and to produce them.
We must not, of course, in our exuberance, conclude that the powers of the hybridizer know no limits. The result under consideration consists, after all, only in the making of new combinations of unit characters, but it is much to know that these units exist and that all conceivable combinations of them are ordinarily capable of production. This valuable knowledge we owe to the discoverer and to the rediscoverers of Mendel’s law.
'New Colour Variety of the Guinea Pig', Science, 1908, 28, 250-252.
A neurotic person can be most simply described as someone who, while he was growing up, learned ways of behaving that are self-defeating in his society.
In Margaret Mead and Rhoda Bubendey Métraux (ed.), Margaret Mead, Some Personal Views (1979), 216.
A physician’s subject of study is necessarily the patient, and his first field for observation is the hospital. But if clinical observation teaches him to know the form and course of diseases, it cannot suffice to make him understand their nature; to this end he must penetrate into the body to find which of the internal parts are injured in their functions. That is why dissection of cadavers and microscopic study of diseases were soon added to clinical observation. But to-day these various methods no longer suffice; we must push investigation further and, in analyzing the elementary phenomena of organic bodies, must compare normal with abnormal states. We showed elsewhere how incapable is anatomy alone to take account of vital phenenoma, and we saw that we must add study of all physico-chemical conditions which contribute necessary elements to normal or pathological manifestations of life. This simple suggestion already makes us feel that the laboratory of a physiologist-physician must be the most complicated of all laboratories, because he has to experiment with phenomena of life which are the most complex of all natural phenomena.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 140-141.
All scientists must focus closely on limited targets. Whether or not one’s findings on a limited subject will have wide applicability depends to some extent on chance, but biologists of superior ability repeatedly focus on questions the answers to which either have wide ramifications or lead to new areas of investigation. One procedure that can be effective is to attempt both reduction and synthesis; that is, direct a question at a phenomenon on one integrative level, identify its mechanism at a simpler level, then extrapolate its consequences to a more complex level of integration.
In 'Scientific innovation and creativity: a zoologist’s point of view', American Zoologist (1982), 22, 230-231,
All that can be said upon the number and nature of elements is, in my opinion, confined to discussions entirely of a metaphysical nature. The subject only furnishes us with indefinite problems, which may be solved in a thousand different ways, not one of which, in all probability, is consistent with nature. I shall therefore only add upon this subject, that if, by the term elements, we mean to express those simple and indivisible atoms of which matter is composed, it is extremely probable we know nothing at all about them; but, if we apply the term elements, or principles of bodies, to express our idea of the last point which analysis is capable of reaching, we must admit, as elements, all the substances into which we are capable, by any means, to reduce bodies by decomposition.
Elements of Chemistry (1790), trans. R. Kerr, Preface, xxiv.
All the inventions and devices ever constructed by the human hand or conceived by the human mind, no matter how delicate, how intricate and complicated, are simple, childish toys compared with that most marvelously wrought mechanism, the human body. Its parts are far more delicate, and their mutual adjustments infinitely more accurate, than are those of the most perfect chronometer ever made.
In Plain Facts For Old and Young: Embracing the Natural History and Hygiene of Organic Life (1879, 1887), Revised Ed., 332.
Although the cooking of food presents some unsolved problems, the quick warming of cooked food and the thawing of frozen food both open up some attractive uses. ... There is no important reason why the the housewife of the future should not purchase completely frozen meals at the grocery store just as she buys quick frozen vegetables. With a quick heating, high-frequency unit in her kitchen, food preparation from a pre-cooked, frozen meal becomes a simple matter.
[Predicting home kitchen appliances could be developed from the radionic tube employed to jam enemy radar in World War II.]
[Predicting home kitchen appliances could be developed from the radionic tube employed to jam enemy radar in World War II.]
In 'Physics of Today Become the Engineering of Tomorrow', Proceedings of the National Electronics Conference (1947), Vols. 1-2, 24-25. Note: by 1947 Ratheon was able to demonstrate a refrigerator-sized commercial microwave oven.
Among natural bodies some have, and some have not, life; and by life we mean the faculties of self-nourishment, self-growth and self-decay. Thus every natural body partaking of life may be regarded as an essential existence; … but then it is an existence only in combination. … And since the organism is such a combination, being possessed of life, it cannot be the Vital Principle. Therefore it follows that the Vital Principle most be an essence, as being the form of a natural body, holding life in potentiality; but essence is a reality (entetechie). The Vital Principle is the original reality of a natural body endowed with potential life; this, however, is to be understood only of a body which may be organized. Thus the parts even of plants are organs, but they are organs that are altogether simple; as the leaf which is the covering of the pericarp, the pericarp of the fruit. If, then, there be any general formula for every kind of Vital Principle, it is—tthe primary reality of an organism.
In George Henry Lewes, Aristotle (1864), 231.
An irrefutable proof that such single-celled primaeval animals really existed as the direct ancestors of Man, is furnished according to the fundamental law of biogeny by the fact that the human egg is nothing more than a simple cell.
Natürlche Schöpfungsgeschichte, trans. E. R. Lankester, The History of Creation (1892), Vol. 2, 381.
Anaxagoras of Clazomenae, son of Hegesiboulos, held that the first principles of things were the homoeomeries. For it seemed to him quite impossible that anything should come into being from the non-existent or be dissolved into it. Anyhow we take in nourishment which is simple and homogeneous, such as bread or water, and by this are nourished hair, veins, arteries, flesh, sinews, bones and all the other parts of the body. Which being so, we must agree that everything that exists is in the nourishment we take in, and that everything derives its growth from things that exist. There must be in that nourishment some parts that are productive of blood, some of sinews, some of bones, and so on-parts which reason alone can apprehend. For there is no need to refer the fact that bread and water produce all these things to sense-perception; rather, there are in bread and water parts which only reason can apprehend.
Aetius 1.3.5. In G. S. Kirk, J. E. Raven and M. Schofield (eds), The Presocratic Philosophers: A Critical History with a Selection of Texts (1983), p. 375.
Anaximenes son of Eurystratus, of Miletus, was a pupil of Anaximander; some say he was also a pupil of Parmenides. He said that the material principle was air and the infinite; and that the stars move, not under the earth, but round it. He used simple and economical Ionic speech. He was active, according to what Apollodorus says, around the time of the capture of Sardis, and died in the 63rd Olympiad.
Diogenes Laertius 2.3. In G. S. Kirk, J. E. Raven and M. Schofield (eds), The Presocratic Philosophers: A Critical History with a Selection of Texts(1983), p. 143.
And do you know what “the world” is to me? Shall I show it to you in my mirror? This world: a monster of energy, without beginning, without end; a firm, iron magnitude of force that does not grow bigger or smaller, that does not expend itself but only transforms itself; as a whole, of unalterable size, a household without expenses or losses, but likewise without increase or income; enclosed by “nothingness”' as by a boundary; not by something blurry or wasted, not something endlessly extended, but set in a definite space as a definite force, and not a space that might be “empty” here or there, but rather as force throughout, as a play of forces and waves of forces, at the same time one and many, increasing here and at the same time decreasing there; a sea of forces flowing and rushing together, eternally changing, eternally flooding back, with tremendous years of recurrence, with an ebb and a flood of its forms; out of the simplest forms striving toward the most complex, out of the stillest, most rigid, coldest forms toward the hottest, most turbulent, most self-contradictory, and then again returning home to the simple out of this abundance, out of the play of contradictions back to the joy of concord, still affirming itself in this uniformity of its courses and its years, blessing itself as that which must return eternally, as a becoming that knows no satiety, no disgust, no weariness: this, my Dionysian world of the eternally self-creating, the eternally self-destroying, this mystery world of the twofold voluptuous delight, my “beyond good and evil,” without goal, unless the joy of the circle itself is a goal; without will, unless a ring feels good will toward itself-do you want a name for this world? A solution for all its riddles? A light for you, too, you best-concealed, strongest, most intrepid, most midnightly men?—This world is the will to power—and nothing besides! And you yourselves are also this will to power—and nothing besides!
The Will to Power (Notes written 1883-1888), book 4, no. 1067. Trans. W. Kaufmann and R. J. Hollingdale and ed. W. Kaufmann (1968), 549-50.
And thus Nature will be very conformable to her self and very simple, performing all the great Motions of the heavenly Bodies by the Attraction of Gravity which intercedes those Bodies, and almost all the small ones of their Particles by some other attractive and repelling Powers which intercede the Particles. The Vis inertiae is a passive Principle by which Bodies persist in their Motion or Rest, receive Motion in proportion to the Force impressing it, and resist as much as they are resisted. By this Principle alone there never could have been any Motion in the World. Some other Principle was necessary for putting Bodies into Motion; and now they are in Motion, some other Principle is necessary for conserving the Motion.
From Opticks, (1704, 2nd ed. 1718), Book 3, Query 31, 372-3.
And, to prevent mistakes, I must advertize you, that I now mean by elements, as those chymists that speak plainest do by their principles, certain primitive or simple, or perfectly unmingled bodies; which not being made of any other bodies, or of one another, are the ingredients of which all those called perfectly mixt bodies are immediately compounded, and into which they are ultimately resolved: now whether there be any such body to be constantly met with in all, and each, of those that are said to be elemented bodies, is the thing I now question.
The Sceptical Chemist (2nd ed., 1661), Appendix, 354. As given in Henry M. Leicester and Herbert S. Klickstein, A Source Book in Chemistry 1400-1900 (1952), 42.
Anybody can make the simple complicated. Creativity is making the complicated simple.
In David Pressman. Patent it Yourself (2008), 37.
As is well known the principle of virtual velocities transforms all statics into a mathematical assignment, and by D'Alembert's principle for dynamics, the latter is again reduced to statics. Although it is is very much in order that in gradual training of science and in the instruction of the individual the easier precedes the more difficult, the simple precedes the more complicated, the special precedes the general, yet the min, once it has arrived at the higher standpoint, demands the reverse process whereby all statics appears only as a very special case of mechanics.
Collected Works (1877), Vol. 5, 25-26. Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 412.
As man advances in civilisation, and small tribes are united into larger communities, the simplest reason would tell each individual that he ought to extend his social instincts and sympathies to all the members of the same nation, though personally unknown to him. This point being once reached, there is only an artificial barrier to prevent his sympathies extending to the men of all nations and races.
…...
Ask a scientist a very profound question on his science, and he will be silent. Ask a religious person a very simple question on his religion, and he will be frenzied.
Quotations: Superultramodern Science and Philosophy (2005).
At last gleams of light have come, and I am almost convinced (quite contrary to opinion I started with) that species are not (it is like confessing a murder) immutable. Heaven forfend me from Lamarck nonsense of a “tendency to progression”, “adaptations from the slow willing of animals”, &c! But the conclusions I am led to are not widely different from his; though the means of change are wholly so. I think I have found out (here’s presumption!) the simple way by which species become exquisitely adapted to various ends.
Letter to Sir Joseph Hooker (11 Jan 1844). In Charles Darwin and Francis Darwin (ed.), Charles Darwin: His Life Told in an Autobiographical Chapter, and in a Selected Series of His Published Letters (1892), 173-174.
At terrestrial temperatures matter has complex properties which are likely to prove most difficult to unravel; but it is reasonable to hope that in the not too distant future we shall be competent to understand so simple a thing as a star.
The Internal Constitution of Stars, Cambridge. (1926, 1988), 393.
At the outset do not be worried about this big question—Truth. It is a very simple matter if each one of you starts with the desire to get as much as possible. No human being is constituted to know the truth, the whole truth, and nothing but the truth; and even the best of men must be content with fragments, with partial glimpses, never the full fruition. In this unsatisfied quest the attitude of mind, the desire, the thirst—a thirst that from the soul must arise!—the fervent longing, are the be-all and the end-all.
'The Student Life' (1905). In G. L. Keynes (ed.), Selected Writings of Sir William Osler (1951), 172.
Attaching significance to invariants is an effort to recognize what, because of its form or colour or meaning or otherwise, is important or significant in what is only trivial or ephemeral. A simple instance of failing in this is provided by the poll-man at Cambridge, who learned perfectly how to factorize a²-b² but was floored because the examiner unkindly asked for the factors of p²–q².
In 'Recent Developments in Invariant Theory', The Mathematical Gazette (Dec 1926), 13, No. 185, 217. [Note: A poll-man is a student who takes the ordinary university degree, without honours. -Webmaster]
Avoid complexities. Make everything as simple as possible.
As quoted in Joseph Wickham Roe, English and American Tool Builders (1916), 49.
But how is one to determine what is pleasing to God? ... Whatever is unpleasant to man is pleasant to God. The test is the natural instinct of man. If there arises within one’s dark recesses a hot desire to do this or that, then it is the paramount duty of a Christian to avoid doing this or that. And if, on the contrary, one cherishes an abhorrence of the business, then one must tackle it forthwith, all the time shouting ‘Hallelujah!’ A simple enough religion, surely–simple, satisfying and idiotic.
…...
But it is just this characteristic of simplicity in the laws of nature hitherto discovered which it would be fallacious to generalize, for it is obvious that simplicity has been a part cause of their discovery, and can, therefore, give no ground for the supposition that other undiscovered laws are equally simple.
From Herbert Spencer lecture delivered at Oxford (1914) 'On Scientific Method in Philosophy', collected in Mysticism and Logic and Other Essays (1919), 102.
But shall gravity be therefore called an occult cause, and thrown out of philosophy, because the cause of gravity is occult and not yet discovered? Those who affirm this, should be careful not to fall into an absurdity that may overturn the foundations of all philosophy. For causes usually proceed in a continued chain from those that are more compounded to those that are more simple; when we are arrived at the most simple cause we can go no farther ... These most simple causes will you then call occult and reject them? Then you must reject those that immediately depend on them.
Mathematical Principles (1729), 27.
By destroying the biological character of phenomena, the use of averages in physiology and medicine usually gives only apparent accuracy to the results. From our point of view, we may distinguish between several kinds of averages: physical averages, chemical averages and physiological and pathological averages. If, for instance, we observe the number of pulsations and the degree of blood pressure by means of the oscillations of a manometer throughout one day, and if we take the average of all our figures to get the true or average blood pressure and to learn the true or average number of pulsations, we shall simply have wrong numbers. In fact, the pulse decreases in number and intensity when we are fasting and increases during digestion or under different influences of movement and rest; all the biological characteristics of the phenomenon disappear in the average. Chemical averages are also often used. If we collect a man's urine during twenty-four hours and mix all this urine to analyze the average, we get an analysis of a urine which simply does not exist; for urine, when fasting, is different from urine during digestion. A startling instance of this kind was invented by a physiologist who took urine from a railroad station urinal where people of all nations passed, and who believed he could thus present an analysis of average European urine! Aside from physical and chemical, there are physiological averages, or what we might call average descriptions of phenomena, which are even more false. Let me assume that a physician collects a great many individual observations of a disease and that he makes an average description of symptoms observed in the individual cases; he will thus have a description that will never be matched in nature. So in physiology, we must never make average descriptions of experiments, because the true relations of phenomena disappear in the average; when dealing with complex and variable experiments, we must study their various circumstances, and then present our most perfect experiment as a type, which, however, still stands for true facts. In the cases just considered, averages must therefore be rejected, because they confuse, while aiming to unify, and distort while aiming to simplify. Averages are applicable only to reducing very slightly varying numerical data about clearly defined and absolutely simple cases.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 134-135.
Chemistry as a science is still in its infancy. I hold to my view because there is still so much beyond our understanding even in the simplest systems the chemist has cared to deal with.
Speech at the Nobel Banquet (10 Dec 1983) for his Nobel Prize in Chemistry. In Wilhelm Odelberg (ed.), Les Prix Nobel: The Nobel Prizes (1984), 43.
Compounds of gaseous substances with each other are always formed in very simple ratios, so that representing one of the terms by unity, the other is 1, 2, or at most 3 ... The apparent contraction of volume suffered by gas on combination is also very simply related to the volume of one of them.
Mémoires de la Société d' Arcueil, 1809, 2, 233-4. Trans. Foundations of the Molecular Theory, Alembic Club Reprint, no. 4 (1950), 24.
Computers and rocket ships are examples of invention, not of understanding. … All that is needed to build machines is the knowledge that when one thing happens, another thing happens as a result. It’s an accumulation of simple patterns. A dog can learn patterns. There is no “why” in those examples. We don’t understand why electricity travels. We don’t know why light travels at a constant speed forever. All we can do is observe and record patterns.
In God's Debris: A Thought Experiment (2004), 22.
Creation science has not entered the curriculum for a reason so simple and so basic that we often forget to mention it: because it is false, and because good teachers understand why it is false. What could be more destructive of that most fragile yet most precious commodity in our entire intellectual heritage—good teaching—than a bill forcing our honorable teachers to sully their sacred trust by granting equal treatment to a doctrine not only known to be false, but calculated to undermine any general understanding of science as an enterprise?.
In 'The Verdict on Creationism' The Sketical Inquirer (Winter 1987/88), 12, 186.
Direct observation of the testimony of the earth … is a matter of the laboratory, of the field naturalist, of indefatigable digging among the ancient archives of the earth’s history. If Mr. Bryan, with an open heart and mind, would drop all his books and all the disputations among the doctors and study first hand the simple archives of Nature, all his doubts would disappear; he would not lose his religion; he would become an evolutionist.
'Evolution and Religion', New York Times (5 Mar 1922), 91. Written in response to an article a few days earlier in which William Jennings Bryan challenged the theory of evolution as lacking proof.
During a conversation with the writer in the last weeks of his life, Sylvester remarked as curious that notwithstanding he had always considered the bent of his mind to be rather analytical than geometrical, he found in nearly every case that the solution of an analytical problem turned upon some quite simple geometrical notion, and that he was never satisfied until he could present the argument in geometrical language.
In Proceedings London Royal Society, 63, 17.
During the time that [Karl] Landsteiner gave me an education in the field of imununology, I discovered that he and I were thinking about the serologic problem in very different ways. He would ask, What do these experiments force us to believe about the nature of the world? I would ask, What is the most. simple and general picture of the world that we can formulate that is not ruled by these experiments? I realized that medical and biological investigators were not attacking their problems the same way that theoretical physicists do, the way I had been in the habit of doing.
‘Molecular Disease’, Pfizer Spectrum (1958), 6:9, 234.
Each of us has read somewhere that in New Guinea pidgin the word for 'piano' is (I use English spelling) 'this fellow you hit teeth belonging to him he squeal all same pig'. I am inclined to doubt whether this expression is authentic; it looks just like the kind of thing a visitor to the Islands would facetiously invent. But I accept 'cut grass belong head belong me' for 'haircut' as genuine... Such phrases seem very funny to us, and make us feel very superior to the ignorant foreigners who use long winded expressions for simple matters. And then it is our turn to name quite a simple thing, a small uncomplicated molecule consisting of nothing more than a measly 11 carbons, seven hydrogens, one nitrogen and six oxygens. We sharpen our pencils, consult our rule books and at last come up with 3-[(1, 3- dihydro-1, 3-dioxo-2H-isoindol-2-yl) oxy]-3-oxopropanoic acid. A name like that could drive any self-respecting Papuan to piano-playing.
The Chemist's English (1990), 3rd Edition, 57.
Ecology has not yet explicitly developed the kind of cohesive, simplifying generalizations exemplified by, say, the laws of physics. Nevertheless there are a number of generalizations that are already evident in what we now know about the ecosphere and that can be organized into a kind of informal set of laws of ecology.
In The Closing Circle: Nature, Man, and Technology (2014).
Einstein, twenty-six years old, only three years away from crude privation, still a patent examiner, published in the Annalen der Physik in 1905 five papers on entirely different subjects. Three of them were among the greatest in the history of physics. One, very simple, gave the quantum explanation of the photoelectric effect—it was this work for which, sixteen years later, he was awarded the Nobel prize. Another dealt with the phenomenon of Brownian motion, the apparently erratic movement of tiny particles suspended in a liquid: Einstein showed that these movements satisfied a clear statistical law. This was like a conjuring trick, easy when explained: before it, decent scientists could still doubt the concrete existence of atoms and molecules: this paper was as near to a direct proof of their concreteness as a theoretician could give. The third paper was the special theory of relativity, which quietly amalgamated space, time, and matter into one fundamental unity.
This last paper contains no references and quotes no authority. All of them are written in a style unlike any other theoretical physicist’s. They contain very little mathematics. There is a good deal of verbal commentary. The conclusions, the bizarre conclusions, emerge as though with the greatest of ease: the reasoning is unbreakable. It looks as though he had reached the conclusions by pure thought, unaided, without listening to the opinions of others. To a surprisingly large extent, that is precisely what he had done.
This last paper contains no references and quotes no authority. All of them are written in a style unlike any other theoretical physicist’s. They contain very little mathematics. There is a good deal of verbal commentary. The conclusions, the bizarre conclusions, emerge as though with the greatest of ease: the reasoning is unbreakable. It looks as though he had reached the conclusions by pure thought, unaided, without listening to the opinions of others. To a surprisingly large extent, that is precisely what he had done.
In Variety of Men (1966), 100-101. First published in Commentary magazine.
Either one or the other [analysis or synthesis] may be direct or indirect. The direct procedure is when the point of departure is known-direct synthesis in the elements of geometry. By combining at random simple truths with each other, more complicated ones are deduced from them. This is the method of discovery, the special method of inventions, contrary to popular opinion.
Ampère gives this example drawn from geometry to illustrate his meaning for “direct synthesis” when deductions following from more simple, already-known theorems leads to a new discovery. In James R. Hofmann, André-Marie Ampère (1996), 159. Cites Académie des Sciences Ampère Archives, box 261.
Endowed with two qualities, which seemed incompatible with each other, a volcanic imagination and a pertinacity of intellect which the most tedious numerical calculations could not daunt, Kepler conjectured that the movements of the celestial bodies must be connected together by simple laws, or, to use his own expression, by harmonic laws. These laws he undertook to discover. A thousand fruitless attempts, errors of calculation inseparable from a colossal undertaking, did not prevent him a single instant from advancing resolutely toward the goal of which he imagined he had obtained a glimpse. Twenty-two years were employed by him in this investigation, and still he was not weary of it! What, in reality, are twenty-two years of labor to him who is about to become the legislator of worlds; who shall inscribe his name in ineffaceable characters upon the frontispiece of an immortal code; who shall be able to exclaim in dithyrambic language, and without incurring the reproach of anyone, “The die is cast; I have written my book; it will be read either in the present age or by posterity, it matters not which; it may well await a reader, since God has waited six thousand years for an interpreter of his words.”
In 'Eulogy on Laplace', in Smithsonian Report for the year 1874 (1875), 131-132.
Equations are Expressions of Arithmetical Computation, and properly have no place in Geometry, except as far as Quantities truly Geometrical (that is, Lines, Surfaces, Solids, and Proportions) may be said to be some equal to others. Multiplications, Divisions, and such sort of Computations, are newly received into Geometry, and that unwarily, and contrary to the first Design of this Science. For whosoever considers the Construction of a Problem by a right Line and a Circle, found out by the first Geometricians, will easily perceive that Geometry was invented that we might expeditiously avoid, by drawing Lines, the Tediousness of Computation. Therefore these two Sciences ought not to be confounded. The Ancients did so industriously distinguish them from one another, that they never introduced Arithmetical Terms into Geometry. And the Moderns, by confounding both, have lost the Simplicity in which all the Elegance of Geometry consists. Wherefore that is Arithmetically more simple which is determined by the more simple Equation, but that is Geometrically more simple which is determined by the more simple drawing of Lines; and in Geometry, that ought to be reckoned best which is geometrically most simple.
In 'On the Linear Construction of Equations', Universal Arithmetic (1769), Vol. 2, 470.
Ethnologists regard man as the primitive element of tribes, races, and peoples. The anthropologist looks at him as a member of the fauna of the globe, belonging to a zoölogical classification, and subject to the same laws as the rest of the animal kingdom. To study him from the last point of view only would be to lose sight of some of his most interesting and practical relations; but to be confined to the ethnologist’s views is to set aside the scientific rule which requires us to proceed from the simple to the compound, from the known to the unknown, from the material and organic fact to the functional phenomenon.
'Paul Broca and the French School of Anthropology'. Lecture delivered in the National Museum, Washington, D.C., 15 April 1882, by Dr. Robert Fletcher. In The Saturday Lectures (1882), 118.
Eventually the process of aging, which is unlikely to be simple, should be understandable. Hopefully some of its processes can be slowed down or avoided. In fact, in the next century, we shall have to tackle the question of the preferred form of death.
(1986).
Every rule has its limits, and every concept its ambiguities. Most of all is this true in the science of life, where nothing quite corresponds to our ideas; similar ends are reached by varied means, and no causes are simple.
In Internal Factors in Evolution (1965), 13.
Everyone now agrees that a Physics where you banish all relationship with mathematics, to confine itself to a mere collection of observations and experiences, would be but an historical amusement, more fitting to entertain idle people, than to engage the mind of a true philosopher.
In 'Préface Contenant l’Exposition du Système', Dictionnaire de Physique (1761), Vol. 1, iii. English version via Google Translate, tweaked by Webmaster. From the original French, “Tout le monde convient maintenant qu’une Physique d’où l'on banniroit tout ce qui peut avoir quelque rapport avec les mathématiques, pour se borner à un simple recueil d’observations & d’experiences, ne seroit qu’un amusement historique, plus propre à récréer un cercle de personnes oisives, qu’à occuper un esprit véritablement philosophique.” Also seen translated as—“Everyone now agrees that a physics lacking all connection with mathematics…would only be an historical amusement, fitter for entertaining the idle than for occupying the mind of a philosopher,” in John L. Heilbron, Electricity in the 17th and 18th centuries: A Study of Early Modern Physics (1979), 74. In the latter source, the subject quote immediately follows a different one by Franz Karl Achard. An editor misreading that paragraph is the likely reason the subject quote will be found in Oxford Dictionary of Science Quotations attributed to Achard. Webmaster checked the original footnoted source, and corrected the author of this entry to Paulian (16 May 2014).
Everything is controlled by immutable mathematical laws, from which there is, and can be, no deviation whatsoever. We learn the complex from the simple. We arrive at the abstract by way of the concrete.
In The Science of Poetry and the Philosophy of Language (1910), xi.
Everything should be made as simple as possible, but not simpler.
Attributed.
Experiments may be of two kinds: experiments of simple fact, and experiments of quantity. ...[In the latter] the conditions will ... vary, not in quality, but quantity, and the effect will also vary in quantity, so that the result of quantitative induction is also to arrive at some mathematical expression involving the quantity of each condition, and expressing the quantity of the result. In other words, we wish to know what function the effect is of its conditions. We shall find that it is one thing to obtain the numerical results, and quite another thing to detect the law obeyed by those results, the latter being an operation of an inverse and tentative character.
Principles of Science: A Treatise on Logic and Scientific Method (1874, 1892), 439.
Finally, to the theme of the respiratory chain, it is especially noteworthy that David Kellin's chemically simple view of the respiratory chain appears now to have been right all along–and he deserves great credit for having been so reluctant to become involved when the energy-rich chemical intermediates began to be so fashionable. This reminds me of the aphorism: 'The obscure we see eventually, the completely apparent takes longer'.
'David Kellin's Respiratory Chain Concept and Its Chemiosmotic Consequences', Nobel Lecture (8 Dec 1978). In Nobel Lectures: Chemistry 1971-1980 (1993), 325.
Finite systems of deterministic ordinary nonlinear differential equations may be designed to represent forced dissipative hydrodynamic flow. Solutions of these equations can be identified with trajectories in phase space. For those systems with bounded solutions, it is found that nonperiodic solutions are ordinarily unstable with respect to small modifications, so that slightly differing initial states can evolve into considerably different states. Systems with bounded solutions are shown to possess bounded numerical solutions.
A simple system representing cellular convection is solved numerically. All of the solutions are found to be unstable, and almost all of them are nonperiodic.
The feasibility of very-long-range weather prediction is examined in the light of these results
A simple system representing cellular convection is solved numerically. All of the solutions are found to be unstable, and almost all of them are nonperiodic.
The feasibility of very-long-range weather prediction is examined in the light of these results
Abstract from his landmark paper introducing Chaos Theory in relation to weather prediction, 'Deterministic Nonperiodic Flow', Journal of the Atmospheric Science (Mar 1963), 20, 130.
First you guess. Don’t laugh, this is the most important step. Then you compute the consequences. Compare the consequences to experience. If it disagrees with experience, the guess is wrong. In that simple statement is the key to science. It doesn’t matter how beautiful your guess is or how smart you are or what your name is. If it disagrees with experience, it’s wrong.
As condensed in Florentin Smarandache, V. Christianto, Multi-Valued Logic, Neutrosophy, and Schrodinger Equation? (2006), 73 & 160 (footnote), paraphrasing from Lecture No. 7, 'Seeking New Laws', Messenger Lectures, Cornell (1964). The original verbatim quote, taken from the transcript is elsewhere on the Richard Feynman Quotations webpage, beginning: “In general, we look for a new law…”.
First, [Newton’s Law of Universal Gravitation] is mathematical in its expression…. Second, it is not exact; Einstein had to modify it…. There is always an edge of mystery, always a place where we have some fiddling around to do yet…. But the most impressive fact is that gravity is simple…. It is simple, and therefore it is beautiful…. Finally, comes the universality of the gravitational law and the fact that it extends over such enormous distances…
In The Character of Physical Law (1965, 2001), 33.
Five centuries ago the printing press sparked a radical reshaping of the nature of education. By bringing a master’s words to those who could not hear a master’s voice, the technology of printing dissolved the notion that education must be reserved for those with the means to hire personal tutors. Today we are approaching a new technological revolution, one whose impact on education may be as far-reaching as that of the printing press: the emergence of powerful computers that are sufficiently inexpensive to be used by students for learning, play and exploration. It is our hope that these powerful but simple tools for creating and exploring richly interactive environments will dissolve the barriers to the production of knowledge as the printing press dissolved the barriers to its transmission.
As co-author with A.A. diSessa, from 'Preface', Turtle Geometry: The Computer as a Medium for Exploring Mathematics (1986), xiii.
For every complex question there is a simple answer–and it's wrong.
Although often seen attributed to H.L. Mencken, webmaster has not found found a primary source, and no authoritative quote collection containing it. If you have a primary source, please contact webmaster, who meanwhile lists this quote as only being author unknown.
Forests … are in fact the world’s air-conditioning system—the very lungs of the planet—and help to store the largest body of freshwater on the planet … essential to produce food for our planet’s growing population. The rainforests of the world also provide the livelihoods of more than a billion of the poorest people on this Earth… In simple terms, the rainforests, which encircle the world, are our very life-support system—and we are on the verge of switching it off.
Presidential Lecture (3 Nov 2008) at the Presidential Palace, Jakarta, Indonesia. On the Prince of Wales website.
Four college students taking a class together, had done so well through the semester, and each had an “A”. They were so confident, the weekend before finals, they went out partying with friends. Consequently, on Monday, they overslept and missed the final. They explained to the professor that they had gone to a remote mountain cabin for the weekend to study, but, unfortunately, they had a flat tire on the way back, didn’t have a spare, and couldn’t get help for a long time. As a result, they missed the final. The professor kindly agreed they could make up the final the following day. When they arrived the next morning, he placed them each in separate rooms, handed each one a test booklet, and told them to begin. The the first problem was simple, worth 5 points. Turning the page they found the next question, written: “(For 95 points): Which tire?”
Fractal is a word invented by Mandelbrot to bring together under one heading a large class of objects that have [played] … an historical role … in the development of pure mathematics. A great revolution of ideas separates the classical mathematics of the 19th century from the modern mathematics of the 20th. Classical mathematics had its roots in the regular geometric structures of Euclid and the continuously evolving dynamics of Newton. Modern mathematics began with Cantor’s set theory and Peano’s space-filling curve. Historically, the revolution was forced by the discovery of mathematical structures that did not fit the patterns of Euclid and Newton. These new structures were regarded … as “pathological,” .… as a “gallery of monsters,” akin to the cubist paintings and atonal music that were upsetting established standards of taste in the arts at about the same time. The mathematicians who created the monsters regarded them as important in showing that the world of pure mathematics contains a richness of possibilities going far beyond the simple structures that they saw in Nature. Twentieth-century mathematics flowered in the belief that it had transcended completely the limitations imposed by its natural origins.
Now, as Mandelbrot points out, … Nature has played a joke on the mathematicians. The 19th-century mathematicians may not have been lacking in imagination, but Nature was not. The same pathological structures that the mathematicians invented to break loose from 19th-century naturalism turn out to be inherent in familiar objects all around us.
Now, as Mandelbrot points out, … Nature has played a joke on the mathematicians. The 19th-century mathematicians may not have been lacking in imagination, but Nature was not. The same pathological structures that the mathematicians invented to break loose from 19th-century naturalism turn out to be inherent in familiar objects all around us.
From 'Characterizing Irregularity', Science (12 May 1978), 200, No. 4342, 677-678. Quoted in Benoit Mandelbrot, The Fractal Geometry of Nature (1977, 1983), 3-4.
From the war of nature, from famine and death, the most exalted object which we are capable of conceiving, namely, the production of the higher animals, directly follows. There is grandeur in this view of life, with its several powers, having been originally breathed into a few forms or into one; and that, whilst this planet has gone cycling on according to the fixed law of gravity, from so simple a beginning endless forms most beautiful and most wonderful have been, and are being, evolved.
Concluding paragraph in The Origin of Species (1859), 490. In the second edition, Darwin changed “breathed” to “breathed by the Creator”.
Furthermore, it’s equally evident that what goes on is actually one degree better than self-reproduction, for organisms appear to have gotten more elaborate in the course of time. Today's organisms are phylogenetically descended from others which were vastly simpler than they are, so much simpler, in fact, that it’s inconceivable, how any kind of description of the latter, complex organism could have existed in the earlier one. It’s not easy to imagine in what sense a gene, which is probably a low order affair, can contain a description of the human being which will come from it. But in this case you can say that since the gene has its effect only within another human organism, it probably need not contain a complete description of what is to happen, but only a few cues for a few alternatives. However, this is not so in phylogenetic evolution. That starts from simple entities, surrounded by an unliving amorphous milieu, and produce, something more complicated. Evidently, these organisms have the ability to produce something more complicated than themselves.
From lecture series on self-replicating machines at the University of Illinois, Lecture 5 (Dec 1949), 'Re-evaluation of the Problems of Complicated Automata—Problems of Hierarchy and Evolution', Theory of Self-Reproducing Automata (1966).
He [Heinrich Rose] looked upon the various substances that he was manipulating, as well as their reactions, under a thoroughly familial point of view: they were like so many children entrusted to his tutelage. Every time he explained simple, clear, well-defined phenomena, he assumed a jovial and smiling countenance; on the other hand, he almost got angry at certain mischievous bodies, the properties of which did not obey ordinary laws and troubled general theoretical views; in his eyes, this was unruly behavior.
As his student, about the lectures of Heinrich Rose, as quoted in entry by Stuart Pierson, 'Rose, Heinrich', in Charles Coulston Gillespie (ed.), Dictionary of Scientific Biography (1975), Vol.11, 541, citing Adolphe Remelé, 'Notice biographique sur le Professeur Henri Rose', in Moniteur Scientifique (1864), 2nd ser., 6, 385–389. [Remelé’s italics.]
He who knows not, and knows not he knows not, he is a fool—shun him;
He who knows not, and knows he knows not, he is simple—teach him;
He who knows, and knows not he knows, he is asleep—wake him;
He who knows, and knows he knows, he is wise—follow him.
He who knows not, and knows he knows not, he is simple—teach him;
He who knows, and knows not he knows, he is asleep—wake him;
He who knows, and knows he knows, he is wise—follow him.
Hesiod, 'Works and Days,' 293-7. In William White, Notes and Queries (1904), Series 10, Vol. 1, 235, the correspondent H.A. Strong says that the origin of these lines is to be found in Hesiod [Greek, 8th Century B.C.], Works and Days, 293-7; that the passage was very celebrated in antiquity, and is quoted by Aristotle, Nic. Eth., i. 4; and that both Livy (xxii. 29) and Cicero (Pro Cluent., 31) refer to it. Another correspondent (J.H.K.) said it was stated to be an Arab proverb in Lady [Isabel] Burton, Life of [Captain] Sir Richard [F.] Burton (1893), Vol. 1, 548, footnote, which prepends to the quote, “Men are four.”
High technology has done us one great service: It has retaught us the delight of performing simple and primordial tasks—chopping wood, building a fire, drawing water from a spring.
In 'Science and Technology', A Voice Crying in the Wilderness (Vox Clamantis in Deserto) (1989), 91.
How to start on my adventure—how to become a forester—was not so simple. There were no schools of Forestry in America. … Whoever turned his mind toward Forestry in those days thought little about the forest itself and more about its influences, and about its influence on rainfall first of all. So I took a course in meteorology, which has to do with weather and climate. and another in botany, which has to do with the vegetable kingdom—trees are unquestionably vegetable. And another in geology, for forests grow out of the earth. Also I took a course in astronomy, for it is the sun which makes trees grow. All of which is as it should be, because science underlies the forester’s knowledge of the woods. So far I was headed right. But as for Forestry itself, there wasn’t even a suspicion of it at Yale. The time for teaching Forestry as a profession was years away.
In Breaking New Ground (1947, 1998), 3.
I … share an excitement and a certain pride in the wonders opened up by scientific investigation …, and also a recognition of the value in scientific method of keeping the hypotheses as simple as possible—my Oxford tutor gave me a great respect for Occam’s razor.
In Letter to periodical, Chemistry and Industry (17 Feb 1997).
I am a great believer in the simplicity of things and as you probably know I am inclined to hang on to broad & simple ideas like grim death until evidence is too strong for my tenacity.
Letter to Irving Langmuir (10 Jun 1919). Quoted in Nathan Reingold and Ida H. Reingold, Science in America: A Documentary History 1900-1939 (1981), 354.
I am a naturalist rather than a scientist. Simply looking at a flower or a frog has always seemed to me to be just about the most interesting thing there is. Others say human beings are pretty interesting, which they are, but as a child you’re not interested in Auntie Flo’s psychology; you’re interested in how a dragonfly larva turns into a dragonfly.
From 'Interview: Of Mind and Matter: David Attenborough Meets Richard Dawkins', The Guardian (11 Sep 2010).
I am a simple man and I want simple answers.
Said to be a favorite line a favorite line of his when challenging his students. As stated, without citation in Robert Slater, Portraits in Silicon (1987), 88.
I am curious in a super-apish way. I like finding out things. That … is all that the “noble self-sacrificing devotion to truth” of 99-44/100% of all scientists amounts to—simple curiosity. That is the spirit in which nearly all productive scientific research is carried on.
Letter from London (20 Apr 1937), No. 81, in George Gaylord Simpson and Léo F. LaPorte (ed.), Simple Curiosity: Letters from George Gaylord Simpson to His Family, 1921-1970 (1987), 34.
I am much occupied with the investigation of the physical causes [of motions in the Solar System]. My aim in this is to show that the celestial machine is to be likened not to a divine organism but rather to a clockwork … insofar as nearly all the manifold movements are carried out by means of a single, quite simple magnetic force. This physical conception is to be presented through calculation and geometry.
Letter to Ilerwart von Hohenburg (10 Feb 1605) Quoted in Holton, Johannes Kepler's Universe: Its Physics and Metaphysics, 342, as cited by Hylarie Kochiras, Force, Matter, and Metaphysics in Newton's Natural Philosophy (2008), 57.
I believe in “intelligence,” and I believe also that there are inherited differences in intellectual ability, but I do not believe that intelligence is a simple scalar endowment that can be quantified by attaching a single figure to it—an I.Q. or the like.
In Advice to a Young Scientist (1979), 25. Footnoted with reference to his own earlier review article of books about IQ, in which he stated “misgivings about whether it is indeed possible to attach a single-number valuation to an endowment as complex and as various as intelligence.” That review was titled 'Unnatural Science', in New York Review of Books (3 Feb 1977), 24, No. 1, 13,
I believe it’s worth emphasizing that a scientist and a graduate student in college, and a kid in grammar school all can start with understanding something new by exploring even the simplest and most common forms of life you find right in the heart of the city. Along a fringe of a street, along the edges and into a city park, is a multitude of species, of associations, of phenomena going on that scientists themselves have not fully come to understand.
From interview with National Geographic, in Andrew Revkin, 'Conservation Legend Has Big Plans For Future', on nationalgeographic.com website.
I believe that the useful methods of mathematics are easily to be learned by quite young persons, just as languages are easily learned in youth. What a wondrous philosophy and history underlie the use of almost every word in every language—yet the child learns to use the word unconsciously. No doubt when such a word was first invented it was studied over and lectured upon, just as one might lecture now upon the idea of a rate, or the use of Cartesian co-ordinates, and we may depend upon it that children of the future will use the idea of the calculus, and use squared paper as readily as they now cipher. … When Egyptian and Chaldean philosophers spent years in difficult calculations, which would now be thought easy by young children, doubtless they had the same notions of the depth of their knowledge that Sir William Thomson might now have of his. How is it, then, that Thomson gained his immense knowledge in the time taken by a Chaldean philosopher to acquire a simple knowledge of arithmetic? The reason is plain. Thomson, when a child, was taught in a few years more than all that was known three thousand years ago of the properties of numbers. When it is found essential to a boy’s future that machinery should be given to his brain, it is given to him; he is taught to use it, and his bright memory makes the use of it a second nature to him; but it is not till after-life that he makes a close investigation of what there actually is in his brain which has enabled him to do so much. It is taken because the child has much faith. In after years he will accept nothing without careful consideration. The machinery given to the brain of children is getting more and more complicated as time goes on; but there is really no reason why it should not be taken in as early, and used as readily, as were the axioms of childish education in ancient Chaldea.
In Teaching of Mathematics (1902), 14.
I believed that, instead of the multiplicity of rules that comprise logic, I would have enough in the following four, as long as I made a firm and steadfast resolution never to fail to observe them.
The first was never to accept anything as true if I did not know clearly that it was so; that is, carefully to avoid prejudice and jumping to conclusions, and to include nothing in my judgments apart from whatever appeared so clearly and distinctly to my mind that I had no opportunity to cast doubt upon it.
The second was to subdivide each on the problems I was about to examine: into as many parts as would be possible and necessary to resolve them better.
The third was to guide my thoughts in an orderly way by beginning, as if by steps, to knowledge of the most complex, and even by assuming an order of the most complex, and even by assuming an order among objects in! cases where there is no natural order among them.
And the final rule was: in all cases, to make such comprehensive enumerations and such general review that I was certain not to omit anything.
The long chains of inferences, all of them simple and easy, that geometers normally use to construct their most difficult demonstrations had given me an opportunity to think that all the things that can fall within the scope of human knowledge follow from each other in a similar way, and as long as one avoids accepting something as true which is not so, and as long as one always observes the order required to deduce them from each other, there cannot be anything so remote that it cannot be reached nor anything so hidden that it cannot be uncovered.
The first was never to accept anything as true if I did not know clearly that it was so; that is, carefully to avoid prejudice and jumping to conclusions, and to include nothing in my judgments apart from whatever appeared so clearly and distinctly to my mind that I had no opportunity to cast doubt upon it.
The second was to subdivide each on the problems I was about to examine: into as many parts as would be possible and necessary to resolve them better.
The third was to guide my thoughts in an orderly way by beginning, as if by steps, to knowledge of the most complex, and even by assuming an order of the most complex, and even by assuming an order among objects in! cases where there is no natural order among them.
And the final rule was: in all cases, to make such comprehensive enumerations and such general review that I was certain not to omit anything.
The long chains of inferences, all of them simple and easy, that geometers normally use to construct their most difficult demonstrations had given me an opportunity to think that all the things that can fall within the scope of human knowledge follow from each other in a similar way, and as long as one avoids accepting something as true which is not so, and as long as one always observes the order required to deduce them from each other, there cannot be anything so remote that it cannot be reached nor anything so hidden that it cannot be uncovered.
Discourse on Method in Discourse on Method and Related Writings (1637), trans. Desmond M. Clarke, Penguin edition (1999), Part 2, 16.
I claim that relativity and the rest of modern physics is not complicated. It can be explained very simply. It is only unusual or, put another way, it is contrary to common sense.
In Edward Teller, Wendy Teller and Wilson Talley, Conversations on the Dark Secrets of Physics (1991, 2013), 2.
I consider the study of medicine to have been that training which preached more impressively and more convincingly than any other could have done, the everlasting principles of all scientific work; principles which are so simple and yet are ever forgotten again, so clear and yet always hidden by a deceptive veil.
In Lecture (2 Aug 1877) delivered on the anniversary of the foundation of the Institute for the Education of Army Surgeons, 'On Thought in Medicine', collected in 'Popular Scientific Lectures', The Humboldt Library of Popular Science Literature (1 Jul 1881), 1, No. 24, 18, (renumbered as p.748 in reprint volume of Nos. 1-24).
I could not help laughing at the ease with which he explained his process of deduction. “When I hear you give your reasons,” I remarked, “the thing always appears to me to be so ridiculously simple that I could easily do it myself, though at each successive instance of your reasoning I am baffled, until you explain your process. And yet I believe that my eyes are as good as yours.”
“Quite so,” he answered, lighting a cigarette, and throwing himself down into an arm-chair. “You see, but you do not observe. The distinction is clear. For example, you have frequently seen the steps which lead up from the hall to this room.”
“Frequently.”
“How often?”
“'Well, some hundreds of times.”
“Then how many are there?”
“How many! I don't know.”
“Quite so! You have not observed. And yet you have seen. That is just my point. Now, I know that there are seventeen steps, because I have both seen and observed.”
“Quite so,” he answered, lighting a cigarette, and throwing himself down into an arm-chair. “You see, but you do not observe. The distinction is clear. For example, you have frequently seen the steps which lead up from the hall to this room.”
“Frequently.”
“How often?”
“'Well, some hundreds of times.”
“Then how many are there?”
“How many! I don't know.”
“Quite so! You have not observed. And yet you have seen. That is just my point. Now, I know that there are seventeen steps, because I have both seen and observed.”
From 'Adventure I.—A Scandal in Bohemia', Adventures of Sherlock Holmes, in The Strand Magazine: An Illustrated Monthly (Jul 1891), 2, 62.
I had a Meccano set with which I “played” endlessly. Meccano which was invented by Frank Hornby around 1900, is called Erector Set in the US. New toys (mainly Lego) have led to the extinction of Meccano and this has been a major disaster as far as the education of our young engineers and scientists is concerned. Lego is a technically trivial plaything and kids love it partly because it is so simple and partly because it is seductively coloured. However it is only a toy, whereas Meccano is a real engineering kit and it teaches one skill which I consider to be the most important that anyone can acquire: This is the sensitive touch needed to thread a nut on a bolt and tighten them with a screwdriver and spanner just enough that they stay locked, but not so tightly that the thread is stripped or they cannot be unscrewed. On those occasions (usually during a party at your house) when the handbasin tap is closed so tightly that you cannot turn it back on, you know the last person to use the washroom never had a Meccano set.
Nobel laureate autobiography in Les Prix Nobel/Nobel Lectures 1996 (1997), 189.
I have deep faith that the principle of the universe will be beautiful and simple.
…...
I have now reached the point where I may indicate briefly what to me constitutes the essence of the crisis of our time. It concerns the relationship of the individual to society. The individual has become more conscious than ever of his dependence upon society. But he does not experience this dependence as a positive asset, as an organic tie, as a protective force, but rather as a threat to his natural rights, or even to his economic existence. Moreover, his position in society is such that the egotistical drives of his make-up are constantly being accentuated, while his social drives, which are by nature weaker, progressively deteriorate. All human beings, whatever their position in society, are suffering from this process of deterioration. Unknowingly prisoners of their own egotism, they feel insecure, lonely, and deprived of the naive, simple, and unsophisticated enjoyment of life. Man can find meaning in life, short and perilous as it is, only through devoting himself to society.
…...
I know of no department of natural science more likely to reward a man who goes into it thoroughly than anthropology. There is an immense deal to be done in the science pure and simple, and it is one of those branches of inquiry which brings one into contact with the great problems of humanity in every direction.
…...
I know two people who have found it [the secret of success]. … Getting ready. Getting prepared. There were Edison and Lindbergh,—they both got ready before they started. I had to find that out too. I had to stop for ten years after I had started; I had to stop for ten years and get ready. I made my first car in 1893, but it was 1903 before I had it ready to sell. It is these simple things that young men ought to know, and they are hardest to grasp. Before everything else, get ready.
From Henry Ford and Ralph Waldo Trine, The Power that Wins (1929), 147. Often seen paraphrased as “Before everything else, getting ready is the secret of success,” for example, in Connie Robertson (ed.), The Wordsworth Dictionary of Quotations (1998), 129.
I know with sure and certain knowledge that a man’s work is nothing but this slow trek to rediscover, through the detours of art, those two or three great and simple images in whose presence his heart first opened.
In Lyrical and Critical Essays (1967), 14.
I now think the answer is very simple: it’s true. God did create the universe about 13.7 billion years ago, and of necessity has involved Himself with His creation ever since. The purpose of this universe is something that only God knows for sure, but it is increasingly clear to modern science that the universe was exquisitely fine-tuned to enable human life.
In Letter (May 2005), sent to the Hope College 2005 Alumni Banquet, read in lieu of accepting an award in person, because of declining health from cancer.
I realized both the upper and lower body must be held securely in place with one strap across the chest and one across the hips. The belt also needed an immovable anchorage point for the buckle as far down beside the occupant’s hip, so it could hold the body properly during a collision. It was just a matter of finding a solution that was simple, effective and could be put on conveniently with one hand.
as quoted in New York Times obituary, 26 Sep 2002
I shall explain a System of the World differing in many particulars from any yet known, answering in all things to the common Rules of Mechanical Motions: This depends upon three Suppositions. First, That all Cœlestial Bodies whatsoever, have an attraction or gravitating power towards their own Centers, whereby they attract not only their own parts, and keep them from flying from them, as we may observe the Earth to do, but that they do also attract all the other Cœlestial bodies that are within the sphere of their activity; and consequently that not only the Sun and Moon have an influence upon the body and motion the Earth, and the Earth upon them, but that Mercury also Venus, Mars, Saturn and Jupiter by their attractive powers, have a considerable influence upon its motion in the same manner the corresponding attractive power of the Earth hath a considerable influence upon every one of their motions also. The second supposition is this, That all bodies whatsoever that are put into a direct and simple motion, will continue to move forward in a streight line, till they are by some other effectual powers deflected and bent into a Motion, describing a Circle, Ellipse, or some other more compounded Curve Line. The third supposition is, That these attractive powers are so much the more powerful in operating, by how much the nearer the body wrought upon is to their own Centers. Now what these several degrees are I have not yet experimentally verified; but it is a notion, which if fully prosecuted as it ought to be, will mightily assist the Astronomer to reduce all the Cœlestial Motions to a certain rule, which I doubt will never be done true without it. He that understands the nature of the Circular Pendulum and Circular Motion, will easily understand the whole ground of this Principle, and will know where to find direction in Nature for the true stating thereof. This I only hint at present to such as have ability and opportunity of prosecuting this Inquiry, and are not wanting of Industry for observing and calculating, wishing heartily such may be found, having myself many other things in hand which I would first compleat and therefore cannot so well attend it. But this I durst promise the Undertaker, that he will find all the Great Motions of the World to be influenced by this Principle, and that the true understanding thereof will be the true perfection of Astronomy.
An Attempt to Prove the Motion of the Earth from Observations (1674), 27-8. Based on a Cutlerian Lecture delivered by Hooke at the Royal Society four years earlier.
I suppose that I tend to be optimistic about the future of physics. And nothing makes me more optimistic than the discovery of broken symmetries. In the seventh book of the Republic, Plato describes prisoners who are chained in a cave and can see only shadows that things outside cast on the cave wall. When released from the cave at first their eyes hurt, and for a while they think that the shadows they saw in the cave are more real than the objects they now see. But eventually their vision clears, and they can understand how beautiful the real world is. We are in such a cave, imprisoned by the limitations on the sorts of experiments we can do. In particular, we can study matter only at relatively low temperatures, where symmetries are likely to be spontaneously broken, so that nature does not appear very simple or unified. We have not been able to get out of this cave, but by looking long and hard at the shadows on the cave wall, we can at least make out the shapes of symmetries, which though broken, are exact principles governing all phenomena, expressions of the beauty of the world outside.
In Nobel Lecture (8 Dec 1989), 'Conceptual Foundations of the Unified Theory of Weak and Electromagnetic Interactions.' Nobel Lectures: Physics 1971-1980 (1992), 556.
I took him [Lawrence Bragg] to a young zoologist working on pattern formation in insect cuticles. The zoologist explained how disturbances introduced into these regular patterns pointed to their formation being governed by some kind of gradient. Bragg listened attentively and then exclaimed: “Your disturbed gradient behaves like a stream of sand running downhill and encountering an obstacle.” “Good heavens,” replied the zoologist, “I had been working on this problem for years before this simple analogy occurred to me and you think of it after twenty minutes.”
As quoted in David Phillips, Biographical Memoirs of Fellows of the Royal Society (Nov 1979), 25, 132, citing: Perutz, M.F. 1971 New Sci. & Sci. J. 8 July 1967.
I was an impostor, the worthy associate of a brigand, &c., &c., and all this for an atom of chlorine put in the place of an atom of hydrogen, for the simple correction of a chemical formula!
Chemical Method (1855), 203.
I was led to the conclusion that at the most extreme dilutions all salts would consist of simple conducting molecules. But the conducting molecules are, according to the hypothesis of Clausius and Williamson, dissociated; hence at extreme dilutions all salt molecules are completely disassociated. The degree of dissociation can be simply found on this assumption by taking the ratio of the molecular conductivity of the solution in question to the molecular conductivity at the most extreme dilution.
Letter to Van’t Hoff, 13 April 1887. In J. R. Partington, A History of Chemistry (1961), Vol. 4, 678.
I was sitting in a chair in the patent office at Bern when all of a sudden a thought occurred to me: “If a person falls freely he will not feel his own weight.” I was startled. This simple thought made a deep impression on me. It impelled me toward a theory of gravitation.
Lecture in Japan (1922). The quote is footnoted in Michael White, John Gribbin, Einstein: a Life in Science (1995), 128, saying the talk is known as the 'Kyoto address', reported in J. Ishiwara, Einstein Koen-Roku (1977).
I was very impressed that one simple theory could incorporate so much physics
…...
I wasn’t aware of Chargaff’s rules when he said them, but the effect on me was quite electric because I realized immediately that if you had this sort of scheme that John Griffith was proposing, of adenine being paired with thymine, and guanine being paired with cytosine, then you should get Chargaff’s rules.
I was very excited, but I didn’t actually tell Chargaff because it was something I was doing with John Griffith. There was a sort of musical comedy effect where I forgot what the bases were and I had to go to the library to check, and I went back to John Griffith to find out which places he said. Low and behold, it turned out that John Griffith’s ideas fitted in with Chargaff’s rules!
This was very exciting, and we thought “ah ha!” and we realized—I mean what anyone who is familiar with the history of science ought to realize—that when you have one-to-one ratios, it means things go to together. And how on Earth no one pointed out this simple fact in those years, I don’t know.
I was very excited, but I didn’t actually tell Chargaff because it was something I was doing with John Griffith. There was a sort of musical comedy effect where I forgot what the bases were and I had to go to the library to check, and I went back to John Griffith to find out which places he said. Low and behold, it turned out that John Griffith’s ideas fitted in with Chargaff’s rules!
This was very exciting, and we thought “ah ha!” and we realized—I mean what anyone who is familiar with the history of science ought to realize—that when you have one-to-one ratios, it means things go to together. And how on Earth no one pointed out this simple fact in those years, I don’t know.
From Transcript of documentary by VSM Productions, The DNA Story (1973). As excerpted on web page 'Chargaff’s Rules', Linus Pauling and the Race for DNA on website scarc.library.oregonstate.edu
If a little less time was devoted to the translation of letters by Julius Caesar describing Britain 2000 years ago and a little more time was spent on teaching children how to describe (in simple modern English) the method whereby ethylene was converted into polythene in 1933 in the ICI laboratories at Northwich, and to discussing the enormous social changes which have resulted from this discovery, then I believe that we should be training future leaders in this country to face the world of tomorrow far more effectively than we are at the present time.
Quoted in an Obituary, D. P. Craig, Biographical Memoirs of Fellows of the Royal Society (1972), 18, 461.
If I go out into nature, into the unknown, to the fringes of knowledge, everything seems mixed up and contradictory, illogical, and incoherent. This is what research does; it smooths out contradictions and makes things simple, logical, and coherent.
In 'Dionysians and Apollonians', Science (2 Jun 1972), 176, 966. Reprinted in Mary Ritchie Key, The Relationship of Verbal and Nonverbal Communication (1980), 318.
If it [a hypothesis] disagrees with experiment, it’s wrong. In that simple statement, is the key to science: it doesn’t make any difference how beautiful your guess is; it doesn’t make any difference how smart you are, who made the guess, or what his name is—if it disagrees with experiment, it’s wrong; that’s all there is to it.
Verbatim from Lecture No. 7, 'Seeking New Laws', Messenger Lectures, Cornell, (1964) in video and transcript online at caltech.edu website. Also in Christopher Sykes, No Ordinary Genius: The Illustrated Richard Feynman (1994), 143. This quote continues one elsewhere on the Richard Feynman Quotations webpage, which begins: “In general, we look for a new law by…”.
If people do not believe that mathematics is simple, it is only because they do not realize how complicated life is.
In speech to first national meeting of the Association for Computing Machinery (1947), as quoted in Franz L. Alt, 'Archaeology of computers: Reminiscences, 1945-1947', Communications of the ACM (Jul 1972), 15, No. 7, 694.
If the brain were simple enough for us to understand it, we would be too simple to understand it.
— Ken Hill
…...
If the brain were so simple
That we could understand it,
We would be so simple
That we couldn’t.
That we could understand it,
We would be so simple
That we couldn’t.
From c. 1938. Quoted and cited by his son, George Edgin Pugh, as a footnoted epigraph, in The Biological Origin of Human Values (1978), 154. The quote is also widely seen slightly paraphrased, attributed to Lyall Watson (q.v. on the Lyall Watson web page of this site, beginning, “If the brain were so simple…”). For example, introduced as “Biologist Lyall Watson spoke of the Catch-22 of brain research:” in Marilyn Ferguson The Aquarian Conspiracy: Personal and Social Transformation in the 1980s (1987), 539. The quote is also widely seen in various paraphrases, attributed to others, including Pat Bahn and Ian Stewart.
If the matter is one that can be settled by observation, make the observation yourself. Aristotle could have avoided the mistake of thinking that women have fewer teeth than men, by the simple device of asking Mrs. Aristotle to keep her mouth open while he counted.
In An Outline of Intellectual Rubbish (1943), 22.
If this seems complex, the reason is because Tao is both simple and complex. It is complex when we try to understand it, and simple when we allow ourselves to experience it.
In Gary William Flake, The Computational Beauty of Nature (2000), 327.
If three simple questions and one well chosen laboratory test lead to an unambiguous diagnosis, why harry the patient with more?
Editorial, 'Clinical decision by numbers'. Lancet (1975) 1, 1077.
If we ascribe the ejection of the proton to a Compton recoil from a quantum of 52 x 106 electron volts, then the nitrogen recoil atom arising by a similar process should have an energy not greater than about 400,000 volts, should produce not more than about 10,000 ions, and have a range in the air at N.T.P. of about 1-3mm. Actually, some of the recoil atoms in nitrogen produce at least 30,000 ions. In collaboration with Dr. Feather, I have observed the recoil atoms in an expansion chamber, and their range, estimated visually, was sometimes as much as 3mm. at N.T.P.
These results, and others I have obtained in the course of the work, are very difficult to explain on the assumption that the radiation from beryllium is a quantum radiation, if energy and momentum are to be conserved in the collisions. The difficulties disappear, however, if it be assumed that the radiation consists of particles of mass 1 and charge 0, or neutrons. The capture of the a-particle by the Be9 nucleus may be supposed to result in the formation of a C12 nucleus and the emission of the neutron. From the energy relations of this process the velocity of the neutron emitted in the forward direction may well be about 3 x 109 cm. per sec. The collisions of this neutron with the atoms through which it passes give rise to the recoil atoms, and the observed energies of the recoil atoms are in fair agreement with this view. Moreover, I have observed that the protons ejected from hydrogen by the radiation emitted in the opposite direction to that of the exciting a-particle appear to have a much smaller range than those ejected by the forward radiation.
This again receives a simple explanation on the neutron hypothesis.
These results, and others I have obtained in the course of the work, are very difficult to explain on the assumption that the radiation from beryllium is a quantum radiation, if energy and momentum are to be conserved in the collisions. The difficulties disappear, however, if it be assumed that the radiation consists of particles of mass 1 and charge 0, or neutrons. The capture of the a-particle by the Be9 nucleus may be supposed to result in the formation of a C12 nucleus and the emission of the neutron. From the energy relations of this process the velocity of the neutron emitted in the forward direction may well be about 3 x 109 cm. per sec. The collisions of this neutron with the atoms through which it passes give rise to the recoil atoms, and the observed energies of the recoil atoms are in fair agreement with this view. Moreover, I have observed that the protons ejected from hydrogen by the radiation emitted in the opposite direction to that of the exciting a-particle appear to have a much smaller range than those ejected by the forward radiation.
This again receives a simple explanation on the neutron hypothesis.
'Possible Existence of a Neutron', Letter to the Editor, Nature, 1932, 129, 312.
If we go back to our chequer game, the fundamental laws are rules by which the chequers move. Mathematics may be applied in the complex situation to figure out what in given circumstances is a good move to make. But very little mathematics is needed for the simple fundamental character of the basic laws. They can be simply stated in English for chequers.
In The Character of Physical Law (1965), 36.
If we wish to give an account of the atomic constitution of the aromatic compounds, we are bound to explain the following facts:
1) All aromatic compounds, even the most simple, are relatively richer in carbon than the corresponding compounds in the class of fatty bodies.
2) Among the aromatic compounds, as well as among the fatty bodies, a large number of homologous substances exist.
3) The most simple aromatic compounds contain at least six atoms of carbon.
4) All the derivatives of aromatic substances exhibit a certain family likeness; they all belong to the group of 'Aromatic compounds'. In cases where more vigorous reactions take place, a portion of the carbon is often eliminated, but the chief product contains at least six atoms of carbon These facts justify the supposition that all aromatic compounds contain a common group, or, we may say, a common nucleus consisting of six atoms of carbon. Within this nucleus a more intimate combination of the carbon atoms takes place; they are more compactly placed together, and this is the cause of the aromatic bodies being relatively rich in carbon. Other carbon atoms can be joined to this nucleus in the same way, and according to the same law, as in the case of the group of fatty bodies, and in this way the existence of homologous compounds is explained.
1) All aromatic compounds, even the most simple, are relatively richer in carbon than the corresponding compounds in the class of fatty bodies.
2) Among the aromatic compounds, as well as among the fatty bodies, a large number of homologous substances exist.
3) The most simple aromatic compounds contain at least six atoms of carbon.
4) All the derivatives of aromatic substances exhibit a certain family likeness; they all belong to the group of 'Aromatic compounds'. In cases where more vigorous reactions take place, a portion of the carbon is often eliminated, but the chief product contains at least six atoms of carbon These facts justify the supposition that all aromatic compounds contain a common group, or, we may say, a common nucleus consisting of six atoms of carbon. Within this nucleus a more intimate combination of the carbon atoms takes place; they are more compactly placed together, and this is the cause of the aromatic bodies being relatively rich in carbon. Other carbon atoms can be joined to this nucleus in the same way, and according to the same law, as in the case of the group of fatty bodies, and in this way the existence of homologous compounds is explained.
Bulletin de la Societé Chimique de France (1865), 1, 98. Trans. W. H. Brock.
Imagine the chaos that would arise if time machines were as common as automobiles, with tens of millions of them commercially available. Havoc would soon break loose, tearing at the fabric of our universe. Millions of people would go back in time to meddle with their own past and the past of others, rewriting history in the process. … It would thus be impossible to take a simple census to see how many people there were at any given time.
In Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and The Tenth Dimension (1994, 1995), 234.
In a sense, of course, probability theory in the form of the simple laws of chance is the key to the analysis of warfare;… My own experience of actual operational research work, has however, shown that its is generally possible to avoid using anything more sophisticated. … In fact the wise operational research worker attempts to concentrate his efforts in finding results which are so obvious as not to need elaborate statistical methods to demonstrate their truth. In this sense advanced probability theory is something one has to know about in order to avoid having to use it.
In 'Operations Research', Physics Today (Nov 1951), 19. As cited by Maurice W. Kirby and Jonathan Rosenhead, 'Patrick Blackett (1897)' in Arjang A. Assad (ed.) and Saul I. Gass (ed.),Profiles in Operations Research: Pioneers and Innovators (2011), 25.
In a way, cancer is so simple and so natural. The older you get, this is just one of the things that happens as the clock ticks.
As quoted in Eric Berger, Houston Chronicle (28 Oct 2005)
In all chemical investigations, it has justly been considered an important object to ascertain the relative weights of the simples which constitute a compound. But unfortunately the enquiry has terminated here; whereas from the relative weights in the mass, the relative weights of the ultimate particles or atoms of the bodies might have been inferred, from which their number and weight in various other compounds would appear, in order to assist and to guide future investigations, and to correct their results. Now it is one great object of this work, to shew the importance and advantage of ascertaining the relative weights of the ultimate particles, both of simple and compound bodies, the number of simple elementary particles which constitute one compound particle, and the number of less compound particles which enter into the formation of one more compound particle.
If there are two bodies, A and B, which are disposed to combine, the following is the order in which the combinations may take place, beginning with the most simple: namely,
1 atom of A + 1 atom of B = 1 atom of C, binary
1 atom of A + 2 atoms of B = 1 atom of D, ternary
2 atoms of A + 1 atom of B = 1 atom of E, ternary
1 atom of A + 3 atoms of B = 1 atom of F, quaternary
3 atoms of A and 1 atom of B = 1 atom of G, quaternary
If there are two bodies, A and B, which are disposed to combine, the following is the order in which the combinations may take place, beginning with the most simple: namely,
1 atom of A + 1 atom of B = 1 atom of C, binary
1 atom of A + 2 atoms of B = 1 atom of D, ternary
2 atoms of A + 1 atom of B = 1 atom of E, ternary
1 atom of A + 3 atoms of B = 1 atom of F, quaternary
3 atoms of A and 1 atom of B = 1 atom of G, quaternary
A New System of Chemical Philosophy (1808), Vol. 1, 212-3.
In all likelihood, it is the local conditions of society, which determine the form of the disease, and we can so far think of it as a fairly general result, that the simplest form is the more common, the more paltry and unbalanced the food, and the worse the dwellings are.
From the original German, “Aller Wahrscheinlichkeit nach sind es die lokalen Verhältnisse der Gesellschaft, welche die Form der Krankheit bestimmen, und wir können bis jetzt als ein ziemlich allgemeines Resultathinstellen, daß die einfache Form umso häufiger ist, je armseliger und einseitiger die Nahrungsmittel und je schlechter die Wohnungen sind,” in 'Mittheilungen über die in Oberschlesien herrschende Typhus-Epidemie', R. Virchow and B. Reinhardt, Archiv für pathologische Anatomie und Physiologie und für klinische Medicin (1848), 2, No. 2, 248. English version by Webmaster with Google translate.
In biology, nothing is clear, everything is too complicated, everything is a mess, and just when you think you understand something, you peel off a layer and find deeper complications beneath. Nature is anything but simple.
The Hot Zone
In every living being there exists a capacity for endless diversity of form; each possesses the power of adapting its organization to the variations of the external world, and it is this power, called into activity by cosmic changes, which has enabled the simple zoophytes of the primitive world to climb to higher and higher stages of organization, and has brought endless variety into nature.
From Gottfried Reinold Treviranus, Biologie, oder Philosophie der lebenden Natur [Biology, or Philosophy of Animate Nature], quoted in Lecture 1, August Weismann (1904, 2nd German ed.) as translated in August Weismann, Margaret R. Thomson (trans.), The Evolution Theory, Vol 1., 18-19.
In fact, we will have to give up taking things for granted, even the apparently simple things. We have to learn to understand nature and not merely to observe it and endure what it imposes on us. Stupidity, from being an amiable individual defect, has become a social crime.
The Origin of Life (1967), 163.
In inorganic chemistry the radicals are simple; in organic chemistry they are compounds—that is the sole difference.
Joint paper with Liebig, but written by Dumas, Comptes Rendus 1837, 5, 567. Trans. J. R. Partington, A History of Chemistry, Vol. 4, 351.
In its earliest development knowledge is self-sown. Impressions force themselves upon men’s senses whether they will or not, and often against their will. The amount of interest in which these impressions awaken is determined by the coarser pains and pleasures which they carry in their train or by mere curiosity; and reason deals with the materials supplied to it as far as that interest carries it, and no further. Such common knowledge is rather brought than sought; and such ratiocination is little more than the working of a blind intellectual instinct. It is only when the mind passes beyond this condition that it begins to evolve science. When simple curiosity passes into the love of knowledge as such, and the gratification of the æsthetic sense of the beauty of completeness and accuracy seems more desirable that the easy indolence of ignorance; when the finding out of the causes of things becomes a source of joy, and he is accounted happy who is successful in the search, common knowledge passes into what our forefathers called natural history, whence there is but a step to that which used to be termed natural philosophy, and now passes by the name of physical science.
In this final state of knowledge the phenomena of nature are regarded as one continuous series of causes and effects; and the ultimate object of science is to trace out that series, from the term which is nearest to us, to that which is at the farthest limit accessible to our means of investigation.
The course of nature as it is, as it has been, and as it will be, is the object of scientific inquiry; whatever lies beyond, above, or below this is outside science. But the philosopher need not despair at the limitation on his field of labor; in relation to the human mind Nature is boundless; and, though nowhere inaccessible, she is everywhere unfathomable.
In this final state of knowledge the phenomena of nature are regarded as one continuous series of causes and effects; and the ultimate object of science is to trace out that series, from the term which is nearest to us, to that which is at the farthest limit accessible to our means of investigation.
The course of nature as it is, as it has been, and as it will be, is the object of scientific inquiry; whatever lies beyond, above, or below this is outside science. But the philosopher need not despair at the limitation on his field of labor; in relation to the human mind Nature is boundless; and, though nowhere inaccessible, she is everywhere unfathomable.
The Crayfish: an Introduction to the Study of Zoölogy (1880), 2-3. Excerpted in Popular Science (Apr 1880), 16, 789-790.
In many cases a dull proof can be supplemented by a geometric analogue so simple and beautiful that the truth of a theorem is almost seen at a glance.
In 'Mathematical Games', Scientific American (Oct 1973), 229, 114.
In order to discover Truth in this manner by observation and reason, it is requisite we should fix on some principles whose certainty and effects are demonstrable to our senses, which may serve to explain the phenomena of natural bodies and account for the accidents that arise in them; such only are those which are purely material in the human body with mechanical and physical experiments … a physician may and ought to furnish himself with, and reason from, such things as are demonstrated to be true in anatomy, chemistry, and mechanics, with natural and experimental philosophy, provided he confines his reasoning within the bounds of truth and simple experiment.
As quoted in selection from the writings of Herman Boerhaave, collected in Oliver Joseph Thatcher (ed.), The Ideas that Have Influenced Civilization, in the Original Documents (1800), Vol. 6, 242.
In science as in life the greatest truths are the simplest.
In 'Mathematical Emancipations', The Monist (Jan 1906), 16, No. 1, 65.
In scientific thought we adopt the simplest theory which will explain all the facts under consideration and enable us to predict new facts of the same kind. The catch in this criterion lies in the world “simplest.” It is really an aesthetic canon such as we find implicit in our criticisms of poetry or painting. The layman finds such a law as dx/dt = κ(d²x/dy²) much less simple than “it oozes,” of which it is the mathematical statement. The physicist reverses this judgment, and his statement is certainly the more fruitful of the two, so far as prediction is concerned. It is, however, a statement about something very unfamiliar to the plain man, namely the rate of change of a rate of change.
In 'Science and Theology as Art-Forms', Possible Worlds (1927), 227.
In the case of the Sun, we have a new understanding of the cosmological meaning of sacrifice. The Sun is, with each second, transforming four million tons of itself into light—giving itself over to become energy that we, with every meal, partake of. The Sun converts itself into a flow of energy that photosynthesis changes into plants that are consumed by animals. Humans have been feasting on the Sun’s energy stored in the form of wheat or maize or reindeer as each day the Sun dies as Sun and is reborn as the vitality of Earth. These solar flares are in fact the very power of the vast human enterprise. Every child of ours needs to learn the simple truth: she is the energy of the Sun. And we adults should organize things so her face shines with the same radiant joy.
In The Hidden Heart of the Cosmos: Humanity and the New Story (1996), 40-41.
In the discussion of the. energies involved in the deformation of nuclei, the concept of surface tension of nuclear matter has been used and its value had been estimated from simple considerations regarding nuclear forces. It must be remembered, however, that the surface tension of a charged droplet is diminished by its charge, and a rough estimate shows that the surface tension of nuclei, decreasing with increasing nuclear charge, may become zero for atomic numbers of the order of 100. It seems therefore possible that the uranium nucleus has only small stability of form, and may, after neutron capture, divide itself into two nuclei of roughly equal size (the precise ratio of sizes depending on liner structural features and perhaps partly on chance). These two nuclei will repel each other and should gain a total kinetic energy of c. 200 Mev., as calculated from nuclear radius and charge. This amount of energy may actually be expected to be available from the difference in packing fraction between uranium and the elements in the middle of the periodic system. The whole 'fission' process can thus be described in an essentially classical way, without having to consider quantum-mechanical 'tunnel effects', which would actually be extremely small, on account of the large masses involved.
[Co-author with Otto Robert Frisch]
[Co-author with Otto Robert Frisch]
Lise Meitner and O. R. Frisch, 'Disintegration of Uranium by Neutrons: a New Type of Nuclear Reaction', Nature (1939), 143, 239.
In the following pages I offer nothing more than simple facts, plain arguments, and common sense; and have no other preliminaries to settle with the reader, than that he will divest himself of prejudice and repossession, and suffer his reason and feelings to determine for themselves; and that he will put on, or rather that he will not put off, the true character of man, and generously enlarge his view beyond the present day.
In Common Sense: Addressed to the Inhabitants of America (1792), 15.
In the modern interpretation of Mendelism, facts are being transformed into factors at a rapid rate. If one factor will not explain the facts, then two are involved; if two prove insufficient, three will sometimes work out. The superior jugglery sometimes necessary to account for the results may blind us, if taken too naively, to the common-place that the results are often so excellently 'explained' because the explanation was invented to explain them. We work backwards from the facts to the factors, and then, presto! explain the facts by the very factors that we invented to account for them. I am not unappreciative of the distinct advantages that this method has in handling the facts. I realize how valuable it has been to us to be able to marshal our results under a few simple assumptions, yet I cannot but fear that we are rapidly developing a sort of Mendelian ritual by which to explain the extraordinary facts of alternative inheritance. So long as we do not lose sight of the purely arbitrary and formal nature of our formulae, little harm will be done; and it is only fair to state that those who are doing the actual work of progress along Mendelian lines are aware of the hypothetical nature of the factor-assumption.
'What are 'Factors' in Mendelian Explanations?', American Breeders Association (1909), 5, 365.
In this lecture I would like to conclude with … some characteristics [of] gravity … The most impressive fact is that gravity is simple. It is simple to state the principles completely and not have left any vagueness for anybody to change the ideas of the law. It is simple, and therefore it is beautiful. It is simple in its pattern. I do not mean it is simple in its action—the motions of the various planets and the perturbations of one on the other can be quite complicated to work out, and to follow how all those stars in a globular cluster move is quite beyond our ability. It is complicated in its actions, but the basic pattern or the system beneath the whole thing is simple. This is common to all our laws; they all turn out to be simple things, although complex in their actual actions.
In 'The Law of Gravitation, as Example of Physical Law', the first of his Messenger Lectures (1964), Cornell University. Collected in The Character of Physical Law (1967), 33-34.
Indeed, the most important part of engineering work—and also of other scientific work—is the determination of the method of attacking the problem, whatever it may be, whether an experimental investigation, or a theoretical calculation. … It is by the choice of a suitable method of attack, that intricate problems are reduced to simple phenomena, and then easily solved.
In Engineering Mathematics: A Series of Lectures Delivered at Union College (1911, 1917), Vol. 2, 275.
It always bothers me that according to the laws as we understand them today, it takes a computing machine an infinite number of logical operations to figure out what goes on in no matter how tiny a region of space and no matter how tiny a region of time … I have often made the hypothesis that ultimately physics will not require a mathematical statement, that in the end the machinery will be revealed and the laws will turn out to be simple, like the chequer board with all its apparent complexities. But this speculation is of the same nature as those other people make—“I like it”,“I don't like it”—and it is not good to be too prejudiced about these things.
In The Character of Physical Law (1965, 2001), 57.
It appears, nevertheless, that all such simple solutions of the problem of vertebrate ancestry are without warrant. They arise from a very common tendency of the mind, against which the naturalist has to guard himself,—a tendency which finds expression in the very widespread notion that the existing anthropoid apes, and more especially the gorilla, must be looked upon as the ancestors of mankind, if once the doctrine of the descent of man from ape-like forefathers is admitted. A little reflexion suffices to show that any given living form, such as the gorilla, cannot possibly be the ancestral form from which man was derived, since ex-hypothesi that ancestral form underwent modification and development, and in so doing, ceased to exist.
'Vertebrata', entry in Encyclopaedia Britannica, 9th edition (1899), Vol. 24, 180.
It behooves us always to remember that in physics it has taken great men to discover simple things. They are very great names indeed which we couple with the explanation of the path of a stone, the droop of a chain, the tints of a bubble, the shadows of a cup.
In On Growth and Form (1917).
It follows from the supreme perfection of God, that in creating the universe has chosen the best possible plan, in which there is the greatest variety together with the greatest order; the best arranged ground, place, time; the most results produced in the most simple ways; the most of power, knowledge, happiness and goodness the creatures that the universe could permit. For since all the possibles in I understanding of God laid claim to existence in proportion to their perfections, the actual world, as the resultant of all these claims, must be the most perfect possible. And without this it would not be possible to give a reason why things have turned out so rather than otherwise.
The Principles of Nature and Grace (1714), The Philosophical Works of Leibnitz (1890), ed. G. M. Duncan, 213-4.
It has been asserted … that the power of observation is not developed by mathematical studies; while the truth is, that; from the most elementary mathematical notion that arises in the mind of a child to the farthest verge to which mathematical investigation has been pushed and applied, this power is in constant exercise. By observation, as here used, can only be meant the fixing of the attention upon objects (physical or mental) so as to note distinctive peculiarities—to recognize resemblances, differences, and other relations. Now the first mental act of the child recognizing the distinction between one and more than one, between one and two, two and three, etc., is exactly this. So, again, the first geometrical notions are as pure an exercise of this power as can be given. To know a straight line, to distinguish it from a curve; to recognize a triangle and distinguish the several forms—what are these, and all perception of form, but a series of observations? Nor is it alone in securing these fundamental conceptions of number and form that observation plays so important a part. The very genius of the common geometry as a method of reasoning—a system of investigation—is, that it is but a series of observations. The figure being before the eye in actual representation, or before the mind in conception, is so closely scrutinized, that all its distinctive features are perceived; auxiliary lines are drawn (the imagination leading in this), and a new series of inspections is made; and thus, by means of direct, simple observations, the investigation proceeds. So characteristic of common geometry is this method of investigation, that Comte, perhaps the ablest of all writers upon the philosophy of mathematics, is disposed to class geometry, as to its method, with the natural sciences, being based upon observation. Moreover, when we consider applied mathematics, we need only to notice that the exercise of this faculty is so essential, that the basis of all such reasoning, the very material with which we build, have received the name observations. Thus we might proceed to consider the whole range of the human faculties, and find for the most of them ample scope for exercise in mathematical studies. Certainly, the memory will not be found to be neglected. The very first steps in number—counting, the multiplication table, etc., make heavy demands on this power; while the higher branches require the memorizing of formulas which are simply appalling to the uninitiated. So the imagination, the creative faculty of the mind, has constant exercise in all original mathematical investigations, from the solution of the simplest problems to the discovery of the most recondite principle; for it is not by sure, consecutive steps, as many suppose, that we advance from the known to the unknown. The imagination, not the logical faculty, leads in this advance. In fact, practical observation is often in advance of logical exposition. Thus, in the discovery of truth, the imagination habitually presents hypotheses, and observation supplies facts, which it may require ages for the tardy reason to connect logically with the known. Of this truth, mathematics, as well as all other sciences, affords abundant illustrations. So remarkably true is this, that today it is seriously questioned by the majority of thinkers, whether the sublimest branch of mathematics,—the infinitesimal calculus—has anything more than an empirical foundation, mathematicians themselves not being agreed as to its logical basis. That the imagination, and not the logical faculty, leads in all original investigation, no one who has ever succeeded in producing an original demonstration of one of the simpler propositions of geometry, can have any doubt. Nor are induction, analogy, the scrutinization of premises or the search for them, or the balancing of probabilities, spheres of mental operations foreign to mathematics. No one, indeed, can claim preeminence for mathematical studies in all these departments of intellectual culture, but it may, perhaps, be claimed that scarcely any department of science affords discipline to so great a number of faculties, and that none presents so complete a gradation in the exercise of these faculties, from the first principles of the science to the farthest extent of its applications, as mathematics.
In 'Mathematics', in Henry Kiddle and Alexander J. Schem, The Cyclopedia of Education, (1877.) As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 27-29.
It is because simplicity and vastness are both beautiful that we seek by preference simple facts and vast facts; that we take delight, now in following the giant courses of the stars, now in scrutinizing the microscope that prodigious smallness which is also a vastness, and now in seeking in geological ages the traces of a past that attracts us because of its remoteness.
…...
It is contrary to the usual order of things, that events so harmonious as those of the system of the world, should depend on such diversified agents as are supposed to exist in our artificial arrangements; and there is reason to anticipate a great reduction in the number of undecompounded bodies, and to expect that the analogies of nature will be found conformable to the refined operations of art. The more the phenomena of the universe are studied, the more distinct their connection appears, and the more simple their causes, the more magnificent their design, and the more wonderful the wisdom and power of their Author.
Elements of Chemical Philosophy (1812), in J. Davy (ed.), The Collected Works of Sir Humphry Davy(1839-40), Vol. 4, 42.
It is easy to create an interstellar radio message which can be recognized as emanating unambiguously from intelligent beings. A modulated signal (‘beep,’ ‘beep-beep,’…) comprising the numbers 1, 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, for example, consists exclusively of the first 12 prime numbers…. A signal of this kind, based on a simple mathematical concept, could only have a biological origin. … But by far the most promising method is to send pictures.
From 'The Quest for Extraterrestrial Intelligence', in the magazine Smithsonian (May 1978), 43-44. Reprinted in Cosmic Search (Mar 1979), 1, No. 2, 5.
It is India that gave us the ingenious method of expressing all numbers by means of ten symbols, each symbol receiving a value of position as well as an absolute value; a profound and important idea which appears so simple to us now that we ignore its true merit. But its very simplicity and the great ease which it has lent to computations put our arithmetic in the first rank of useful inventions; and we shall appreciate the grandeur of the achievement the more when we remember that it escaped the genius of Archimedes and Apollonius, two of the greatest men produced by antiquity.
Quoted in Return to Mathematical Circles H. Eves (Boston 1988).
It is natural for man to relate the units of distance by which he travels to the dimensions of the globe that he inhabits. Thus, in moving about the earth, he may know by the simple denomination of distance its proportion to the whole circuit of the earth. This has the further advantage of making nautical and celestial measurements correspond. The navigator often needs to determine, one from the other, the distance he has traversed from the celestial arc lying between the zeniths at his point of departure and at his destination. It is important, therefore, that one of these magnitudes should be the expression of the other, with no difference except in the units. But to that end, the fundamental linear unit must be an aliquot part of the terrestrial meridian. ... Thus, the choice of the metre was reduced to that of the unity of angles.
Lecture at the École Normale to the Year III (Apr 1795), Oeuvres Completes de Laplace (1878-1912), Vol. 14, 141. In Charles Coulston Gillispie, Dictionary of Scientific Biography (1978), Vol. 15, 335.
It is necessary that a surgeon should have a temperate and moderate disposition. That he should have well-formed hands, long slender fingers, a strong body, not inclined to tremble and with all his members trained to the capable fulfilment of the wishes of his mind. He should be of deep intelligence and of a simple, humble, brave, but not audacious disposition. He should be well grounded in natural science, and should know not only medicine but every part of philosophy; should know logic well, so as to be able to understand what is written, to talk properly, and to support what he has to say by good reasons.
Chirurgia Magna (1296, printed 1479), as translated by James Joseph Walsh in Old-Time Makers of Medicine (1911), 261.
It is not a simple life to be a single cell, although I have no right to say so, having been a single cell so long ago myself that I have no memory at all of that stage in my life.
In A Long Line of Cells: Collected Essays (1990), 244.
It is not merely as an investigator and discoverer, but as a high-principled and unassuming man, that Scheele merits our warmest admiration. His aim and object was the discovery of truth. The letters of the man reveal to us in the most pleasant way his high scientific ideal, his genuinely philosophic temper, and his simple mode of thought. “It is the truth alone that we desire to know, and what joy there is in discovering it!” With these words he himself characterizes his own efforts.
From History of Chemistry (1899). As quoted in Victor Robinson, Pathfinders in Medicine (1912), 121.
It is not necessary for all men to be great in action. The greatest and sublimest power is often simple patience.
From 'The Efficiency of the Passive Virtues', New Life, Sermons for the New Life (1869), 412.
It is not possible to find in all geometry more difficult and more intricate questions or more simple and lucid explanations [than those given by Archimedes]. Some ascribe this to his natural genius; while others think that incredible effort and toil produced these, to all appearance, easy and unlaboured results. No amount of investigation of yours would succeed in attaining the proof, and yet, once seen, you immediately believe you would have discovered it; by so smooth and so rapid a path he leads you to the conclusion required.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
It is the simple hypotheses of which one must be most wary; because these are the ones that have the most chances of passing unnoticed.
…...
It must … be admitted that very simple relations … exist between the volumes of gaseous substances and the numbers of simple or compound molecules which form them. The first hypothesis to present itself in this connection, and apparently even the only admissible one, is the supposition that the number of integral molecules in any gases is always the same for equal volumes, or always proportional to the volumes. Indeed, if we were to suppose that the number of molecules contained in a given volume were different for different gases, it would scarcely be possible to conceive that the law regulating the distance of molecules could give in all cases relations so simple as those which the facts just detailed compel us to acknowledge between the volume and the number of molecules.
In 'Essay on a Manner of Determining the Relative Masses of the Elementary Molecules of Bodies, and the Proportions in which they enter into these Compounds', Journal de Physique, 1811, 73, 58-76. In Foundations of the Molecular Theory; Alembic Club Reprints, Number 4 (1923), 28-9.
It needs scarcely be pointed out that in placing Mathematics at the head of Positive Philosophy, we are only extending the application of the principle which has governed our whole Classification. We are simply carrying back our principle to its first manifestation. Geometrical and Mechanical phenomena are the most general, the most simple, the most abstract of all,— the most irreducible to others, the most independent of them; serving, in fact, as a basis to all others. It follows that the study of them is an indispensable preliminary to that of all others. Therefore must Mathematics hold the first place in the hierarchy of the sciences, and be the point of departure of all Education whether general or special.
In Auguste Comte and Harriet Martineau (trans.), The Positive Philosophy (1858), Introduction, Chap. 2, 50.
It seems to me that the view toward which we are tending is that the specificity in gene action is always a chemical specificity, probably the production of enzymes which guide metabolic processes along particular channels. A given array of genes thus determines the production of a particular kind of protoplasm with particular properties—such, for example, as that of responding to surface forces by the formation of a special sort of semipermeable membrane, and that of responding to trivial asymmetries in the play of external stimuli by polarization, with consequent orderly quantitative gradients in all physiologic processes. Different genes may now be called into play at different points in this simple pattern, either through the local formation of their specific substrates for action, or by activation of a mutational nature. In either case the pattern becomes more complex and qualitatively differentiated. Successive interactions of differentiated regions and the calling into play of additional genes may lead to any degree of complexity of pattern in the organism as a largely self-contained system. The array of genes, assembled in the course of evolution, must of course be one which determines a highly selfregulatory system of reactions. On this view the genes are highly specific chemically, and thus called into play only under very specific conditions; but their morphological effects, if any, rest on quantitative influences of immediate or remote products on growth gradients, which are resultants of all that has gone on before in the organism.
In 'Genetics of Abnormal Growth in the Guinea Pig', Cold Spring Harbor Symposia on Quantitative Biology (1934), 2, 142.
It seems to me that you are solving a problem which goes beyond the limits of physiology in too simple a way. Physiology has realized its problem with fortitude, breaking man down into endless actions and counteractions and reducing him to a crossing, a vortex of reflex acts. Let it now permit sociology to restore him as a whole. Sociology will wrest man from the anatomical theatre and return him to history.
Letter to his son, Alexander, July-Aug 1868. Trans. Roger Smith, Inhibition: History and Meaning in the Sciences of Mind and Brain (1992), 223.
Just as Darwin discovered the law of evolution in organic nature, so Marx discovered the law of evolution in human history; he discovered the simple fact, hitherto concealed by an overgrowth of idealogy [sic], that mankind must first of all eat and drink, have shelter and clothing, before it can pursue politics, science, religion, art etc.
Engels' Speech over the Grave of Karl Marx, delivered at Highgate Cemetery, London, 17 Mar 1883. Quoted in Karl Marx 1818-1883, for the Anniversary of his Death (1942), 27.
Kids who aren’t even allowed to have hazardous toys, … Hazardous toys…! Whatever happened to natural selection? Survival of the fittest? The kid who swallows too many marbles doesn’t grow up to have kids of his own. Simple stuff. Nature knows best!
In Napalm and Silly Putty (2001), 56.
Knowledge leads us from the simple to the complex; wisdom leads us from the complex to the simple.
In Dianna Daniels Booher, Your Signature Life: Pursuing God's Best Every Day (2003), 30.
Lavoisier was right in the deepest, almost holy, way. His passion harnessed feeling to the service of reason; another kind of passion was the price. Reason cannot save us and can even persecute us in the wrong hands; but we have no hope of salvation without reason. The world is too complex, too intransigent; we cannot bend it to our simple will.
…...
Leaving aside genetic surgery applied humans, I foresee that the coming century will place in our hands two other forms of biological technology which are less dangerous but still revolutionary enough to transform the conditions of our existence. I count these new technologies as powerful allies in the attack on Bernal's three enemies. I give them the names “biological engineering” and “self-reproducing machinery.” Biological engineering means the artificial synthesis of living organisms designed to fulfil human purposes. Self-reproducing machinery means the imitation of the function and reproduction of a living organism with non-living materials, a computer-program imitating the function of DNA and a miniature factory imitating the functions of protein molecules. After we have attained a complete understanding of the principles of organization and development of a simple multicellular organism, both of these avenues of technological exploitation should be open to us.
From 3rd J.D. Bernal Lecture, Birkbeck College London (16 May 1972), The World, the Flesh and the Devil (1972), 6. Collected in The Scientist as Rebel (2006), 292. (The World, the Flesh & the Devil: An Enquiry into the Future of the Three Enemies of the Rational Soul is the title of a book by J. D Bernal, a scientist who pioneered X-ray crystallography.)
Lecturing after a fashion is easy enough ; teaching is a very different affair. ... The transmission of ideas from one mind to another, in a simple unequivocal form, is not always easy ; but in teaching, the object is not merely to convey the idea, but to give a lively and lasting impression; something that should not merely cause the retention of the image, but in such connection as to excite another process, ' thought.'
Memoirs of John Abernethy (1854), 253.
Let any man reflect on the revolution produced in society by two simple and common things, glass and gunpowder.
Reflection 328, in Lacon: or Many things in Few Words; Addressed to Those Who Think (1820), 155.
Let me suggest to you a simple test one can apply to scientific activities to determine whether or not they can constitute the practice of physics. Is what you are doing beautiful? Many beautiful things are created without the use of physical knowledge, but I know of no really worthwhile physics that isn’t beautiful. Indeed, one of the most distressing symptoms of scientific illiteracy is the impression so often given to school children that science is a mechanistic activity subject to algorithmic description.
In 'Physics and the APS in 1979', Physics Today (Apr 1980), 33, No. 4, 50.
Let NEWTON, pure Intelligence, whom GOD
To Mortals lent, to trace his boundless Works
From Laws sublimely simple, speak thy Fame
In all Philosophy.
To Mortals lent, to trace his boundless Works
From Laws sublimely simple, speak thy Fame
In all Philosophy.
The Seasons (1746), 130.
Let us ... consider the ovum [egg] as a physical system. Its potentialities are prodigious and one's first impulse is to expect that such vast potentialities would find expression in complexity of
structure. But what do we find? The substance is clouded with particles, but these can be
centrifuged away leaving it optically structureless but still capable of development.... On the
surface of the egg there is a fine membrane, below it fluid of high viscosity, next fluid of
relatively low viscosity, and within this the nucleus, which in the resting stage is simply a bag
of fluid enclosed in a delicate membrane.... The egg's simplicity is not that of a machine or a
crystal, but that of a nebula. Gathered into it are units relatively simple but capable by their
combinations of forming a vast number of dynamical systems...
As guest of honour, closing day address (Jun 1928), Sixth Colloid Symposium, Toronto, Canada, 'Living Matter', printed in Harry Boyer Weiser (ed.), Colloid Symposium Monograph (1928), Vol. 6, 15. Quoted in Joseph Needham, Chemical Embryology (1931), Vol. 1, 612-613.
Let us now declare the means whereby our understanding can rise to knowledge without fear of error. There are two such means: intuition and deduction. By intuition I mean not the varying testimony of the senses, nor the deductive judgment of imagination naturally extravagant, but the conception of an attentive mind so distinct and so clear that no doubt remains to it with regard to that which it comprehends; or, what amounts to the same thing, the self-evidencing conception of a sound and attentive mind, a conception which springs from the light of reason alone, and is more certain, because more simple, than deduction itself. …
It may perhaps be asked why to intuition we add this other mode of knowing, by deduction, that is to say, the process which, from something of which we have certain knowledge, draws consequences which necessarily follow therefrom. But we are obliged to admit this second step; for there are a great many things which, without being evident of themselves, nevertheless bear the marks of certainty if only they are deduced from true and incontestable principles by a continuous and uninterrupted movement of thought, with distinct intuition of each thing; just as we know that the last link of a long chain holds to the first, although we can not take in with one glance of the eye the intermediate links, provided that, after having run over them in succession, we can recall them all, each as being joined to its fellows, from the first up to the last. Thus we distinguish intuition from deduction, inasmuch as in the latter case there is conceived a certain progress or succession, while it is not so in the former; … whence it follows that primary propositions, derived immediately from principles, may be said to be known, according to the way we view them, now by intuition, now by deduction; although the principles themselves can be known only by intuition, the remote consequences only by deduction.
It may perhaps be asked why to intuition we add this other mode of knowing, by deduction, that is to say, the process which, from something of which we have certain knowledge, draws consequences which necessarily follow therefrom. But we are obliged to admit this second step; for there are a great many things which, without being evident of themselves, nevertheless bear the marks of certainty if only they are deduced from true and incontestable principles by a continuous and uninterrupted movement of thought, with distinct intuition of each thing; just as we know that the last link of a long chain holds to the first, although we can not take in with one glance of the eye the intermediate links, provided that, after having run over them in succession, we can recall them all, each as being joined to its fellows, from the first up to the last. Thus we distinguish intuition from deduction, inasmuch as in the latter case there is conceived a certain progress or succession, while it is not so in the former; … whence it follows that primary propositions, derived immediately from principles, may be said to be known, according to the way we view them, now by intuition, now by deduction; although the principles themselves can be known only by intuition, the remote consequences only by deduction.
In Rules for the Direction of the Mind, Philosophy of Descartes. [Torrey] (1892), 64-65.
Life and business are rather simple after all—to make a success of either, you've got to hang on to the knack of putting yourself into the other person's place.
c. 1891. On Wrigley Company web site.
Like almost every subject of human interest, this one [mathematics] is just as easy or as difficult as we choose to make it. A lifetime may be spent by a philosopher in discussing the truth of the simplest axiom. The simplest fact as to our existence may fill us with such wonder that our minds will remain overwhelmed with wonder all the time. A Scotch ploughman makes a working religion out of a system which appalls a mental philosopher. Some boys of ten years of age study the methods of the differential calculus; other much cleverer boys working at mathematics to the age of nineteen have a difficulty in comprehending the fundamental ideas of the calculus.
In Teaching of Mathematics (1902), 19-20.
Little can be understood of even the simplest phenomena of nature without some knowledge of mathematics, and the attempt to penetrate deeper into the mysteries of nature compels simultaneous development of the mathematical processes.
In Teaching of Mathematics in the Elementary and the Secondary School (1906), 16.
Making the simple complicated is commonplace; making the complicated simple, awesomely simple, that’s creativity.
As quoted, without citation, in Richard D Pepperman, The Eye is Quicker (2004), xv.
Man is the Reasoning Animal. Such is the claim. I think it is open to dispute. Indeed, my experiments have proven to me that he is the Unreasoning Animal. … It seems plain to me that whatever he is he is not a reasoning animal. His record is the fantastic record of a maniac. I consider that the strongest count against his intelligence is the fact that with that record back of him he blandly sets himself up as the head animal of the lot: whereas by his own standards he is the bottom one.
In truth, man is incurably foolish. Simple things which the other animals easily learn, he is incapable of learning. Among my experiments was this. In an hour I taught a cat and a dog to be friends. I put them in a cage. In another hour I taught them to be friends with a rabbit. In the course of two days I was able to add a fox, a goose, a squirrel and some doves. Finally a monkey. They lived together in peace; even affectionately.
Next, in another cage I confined an Irish Catholic from Tipperary, and as soon as he seemed tame I added a Scotch Presbyterian from Aberdeen. Next a Turk from Constantinople; a Greek Christian from Crete; an Armenian; a Methodist from the wilds of Arkansas; a Buddhist from China; a Brahman from Benares. Finally, a Salvation Army Colonel from Wapping. Then I stayed away two whole days. When I came back to note results, the cage of Higher Animals was all right, but in the other there was but a chaos of gory odds and ends of turbans and fezzes and plaids and bones and flesh—not a specimen left alive. These Reasoning Animals had disagreed on a theological detail and carried the matter to a Higher Court.
In truth, man is incurably foolish. Simple things which the other animals easily learn, he is incapable of learning. Among my experiments was this. In an hour I taught a cat and a dog to be friends. I put them in a cage. In another hour I taught them to be friends with a rabbit. In the course of two days I was able to add a fox, a goose, a squirrel and some doves. Finally a monkey. They lived together in peace; even affectionately.
Next, in another cage I confined an Irish Catholic from Tipperary, and as soon as he seemed tame I added a Scotch Presbyterian from Aberdeen. Next a Turk from Constantinople; a Greek Christian from Crete; an Armenian; a Methodist from the wilds of Arkansas; a Buddhist from China; a Brahman from Benares. Finally, a Salvation Army Colonel from Wapping. Then I stayed away two whole days. When I came back to note results, the cage of Higher Animals was all right, but in the other there was but a chaos of gory odds and ends of turbans and fezzes and plaids and bones and flesh—not a specimen left alive. These Reasoning Animals had disagreed on a theological detail and carried the matter to a Higher Court.
In Mark Twain and Bernard DeVoto (ed.), Letters from the Earth: Uncensored Writings (1962), 180-181. [Note: As a humorous, irreverent consideration of Man and Christianity, these essays (written c.1909) remained unpublished for over 50 years after Twain’s death (1910), because his daughter and literary executor (Clara Clemens Samossoud) felt that some of the pieces did not accurately represent her father’s beliefs, but eventually, she consented to their publication.]
Mankind have been slow to believe that order reigns in the universe—that the world is a cosmos and a chaos.
… The divinities of heathen superstition still linger in one form or another in the faith of the ignorant, and even intelligent men shrink from the contemplation of one supreme will acting regularly, not fortuitously, through laws beautiful and simple rather than through a fitful and capricious system of intervention.
... The scientific spirit has cast out the demons, and presented us with nature clothed in her right mind and living under the reign of law. It has given us, for the sorceries of the alchemist, the beautiful laws of chemistry; for the dreams of the astrologer, the sublime truths of astronomy; for the wild visions of cosmogony, the monumental records of geology; for the anarchy of diabolism, the laws of God.
… The divinities of heathen superstition still linger in one form or another in the faith of the ignorant, and even intelligent men shrink from the contemplation of one supreme will acting regularly, not fortuitously, through laws beautiful and simple rather than through a fitful and capricious system of intervention.
... The scientific spirit has cast out the demons, and presented us with nature clothed in her right mind and living under the reign of law. It has given us, for the sorceries of the alchemist, the beautiful laws of chemistry; for the dreams of the astrologer, the sublime truths of astronomy; for the wild visions of cosmogony, the monumental records of geology; for the anarchy of diabolism, the laws of God.
Speech (16 Dec 1867) given while a member of the U.S. House of Representatives, introducing resolution for the appointment of a committee to examine the necessities for legislation upon the subject of the ninth census to be taken the following year. Quoted in John Clark Ridpath, The Life and Work of James A. Garfield (1881), 216.
Many inventions are not suitable for the people at large because of their carelessness. Before a thing can be marketed to the masses, it must be made practically fool-proof. Its operation must be made extremely simple. That is one reason, I think, why the phonograph has been so universally adopted. Even a child can operate it. … Another reason is that people are far more willing to pay for being amused than for anything else.
As quoted from an interview by B.C. Forbes in The American Magazine (Jan 1921), 86.
Math is like love—a simple idea but it can get complicated.
Quoted in Jon Fripp, Michael Fripp, Deborah Fripp Speaking of Science: Notable Quotes on Science, Engineering, and the Environment (2000), 45, and attributed to “R. Drabek” with no further source information. Webmaster wonders if this is a typo for mathematician, Pavel Drábek.
Mathematical language is not only the simplest and most easily understood of any, but the shortest also.
In Works of Henry, Lord Brougham: Vol. 7: Rhetorical and literary Dissertions and Addresses (1856), 317, footnote.
Mathematician: A scientist who can figure out anything except such simple things as squaring the circle and trisecting an angle.
In Esar’s Comic Dictionary (1943, 4th ed. 1983), 372-373.
Mathematics … belongs to every inquiry, moral as well as physical. Even the rules of logic, by which it is rigidly bound, could not be deduced without its aid. The laws of argument admit of simple statement, but they must be curiously transposed before they can be applied to the living speech and verified by observation. In its pure and simple form the syllogism cannot be directly compared with all experience, or it would not have required an Aristotle to discover it. It must be transmuted into all the possible shapes in which reasoning loves to clothe itself. The transmutation is the mathematical process in the establishment of the law.
From Memoir (1870) read before the National Academy of Sciences, Washington, printed in 'Linear Associative Algebra', American Journal of Mathematics (1881), 4, 97-98.
Mathematics is a game played according to certain simple rules with meaningless marks on paper.
Given as narrative, without quotation marks, in Eric Temple Bell, Mathematics, Queen and Servant of Science (1951, 1961), 21.
Mathematics, the science of the ideal, becomes the means of investigating, understanding and making known the world of the real. The complex is expressed in terms of the simple. From one point of view mathematics may be defined as the science of successive substitutions of simpler concepts for more complex.
In A Scrap-book of Elementary Mathematics (1908), 215.
Men go into space to see whether it is the kind of place where other men, and their families and their children, can eventually follow them. A disturbingly high proportion of the intelligent young are discontented because they find the life before them intolerably confining. The moon offers a new frontier. It is as simple and splendid as that.
— Magazine
Editorial on the moon landing, The Economist (1969).
Men of science, fit to teach, hardly exist. There is no demand for such men. The sciences make up life; they are important to life. The highly educated man fails to understand the simplest things of science, and has no peculiar aptitude for grasping them. I find the grown-up mind coming back to me with the same questions over and over again.
Giving Evidence (18 Nov 1862) to the Public Schools Commission. As quoted in John L. Lewis, 125 Years: The Physical Society & The Institute of Physics (1999), 168.
Mere numbers cannot bring out … the intimate essence of the experiment. This conviction comes naturally when one watches a subject at work. … What things can happen! What reflections, what remarks, what feelings, or, on the other hand, what blind automatism, what absence of ideas! … The experimenter judges what may be going on in [the subject’s] mind, and certainly feels difficulty in expressing all the oscillations of a thought in a simple, brutal number, which can have only a deceptive precision. How, in fact, could it sum up what would need several pages of description!
In La Suggestibilité (1900), 119-20.
Most of the fundamental ideas of science are essentially simple, and may, as a rule, be expressed in a language comprehensible to everyone.
Co-authored with Leopold Infeld.
Co-authored with Leopold Infeld.
The Evolution of Physics: The Growth of Ideas from the Early Concepts to Relativity and Quanta (1938), 29. Infeld was a Polish physicist (1898-1968).
Most of us have had moments in childhood when we touched the divine presence. We did not think it extraordinary because it wasn’t; it was just a beautiful moment filled with love. In those simple moments our hearts were alive, and we saw the poignant beauty of life vividly with wonder and appreciation.
David McArthur and Bruce McArthur
Mssr. Fermat—what have you done?
Your simple conjecture has everyone
Churning out proofs,
Which are nothing but goofs!
Could it be that your statement’s an erudite spoof?
A marginal hoax
That you’ve played on us folks?
But then you’re really not known for your practical jokes.
Or is it then true
That you knew what to do
When n was an integer greater than two?
Oh then why can’t we find
That same proof…are we blind?
You must be reproved, for I’m losing my mind.
Your simple conjecture has everyone
Churning out proofs,
Which are nothing but goofs!
Could it be that your statement’s an erudite spoof?
A marginal hoax
That you’ve played on us folks?
But then you’re really not known for your practical jokes.
Or is it then true
That you knew what to do
When n was an integer greater than two?
Oh then why can’t we find
That same proof…are we blind?
You must be reproved, for I’m losing my mind.
In 'Fermat's Last Theorem', Mathematics Magazine (Apr 1986), 59, No. 2, 76.
My aim is to say that the machinery of the heavens is not like a divine animal but like a clock (and anyone who believes a clock has a soul gives the work the honour due to its maker) and that in it almost all the variety of motions is from one very simple magnetic force acting on bodies, as in the clock all motions are from a very simple weight.
Letter to J. G. Herwart von Hohenburg (16 Feb 1605). Johannes Kepler Gesammelte Werke (1937- ), Vol. 15, letter 325, l. 57-61, p. 146.
My colleagues in elementary particle theory in many lands [and I] are driven by the usual insatiable curiosity of the scientist, and our work is a delightful game. I am frequently astonished that it so often results in correct predictions of experimental results. How can it be that writing down a few simple and elegant formulae, like short poems governed by strict rules such as those of the sonnet or the waka, can predict universal regularities of Nature?
Nobel Banquet Speech (10 Dec 1969), in Wilhelm Odelberg (ed.),Les Prix Nobel en 1969 (1970).
My experiments with single traits all lead to the same result: that from the seeds of hybrids, plants are obtained half of which in turn carry the hybrid trait (Aa), the other half, however, receive the parental traits A and a in equal amounts. Thus, on the average, among four plants two have the hybrid trait Aa, one the parental trait A, and the other the parental trait a. Therefore, 2Aa+ A +a or A + 2Aa + a is the empirical simple series for two differing traits.
Letter to Carl Nägeli, 31 Dec 1866. In Curt Stern and Eva R. Sherwood (eds.), The Origin of Genetics: A Mendel Source Book (1966), 63.
My goal is simple. It is a complete understanding of the universe, why it is as it is and why it exists at all.
In the Washington Post, April 15, 1988.
My main thesis will be that in the study of the intermediate processes of metabolism we have to deal not with complex substances which elude ordinary chemical methods, but with the simple substances undergoing comprehensible reactions... I intend also to emphasise the fact that it is not alone with the separation and identification of products from the animal that our present studies deal; but with their reactions in the body; with the dynamic side of biochemical phenomena.
'The Dynamic Side of Biochemistry', Address (11 Sep 1913) in Report on the 83rd Meeting of the British Association for the Advancement of Science (1914), 653.
My own thinking (and that of many of my colleagues) is based on two general principles, which I shall call the Sequence Hypothesis and the Central Dogma. The direct evidence for both of them is negligible, but I have found them to be of great help in getting to grips with these very complex problems. I present them here in the hope that others can make similar use of them. Their speculative nature is emphasized by their names. It is an instructive exercise to attempt to build a useful theory without using them. One generally ends in the wilderness.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
'On Protein Synthesis', Symposia of the Society for Experimental Biology: The Biological Replication of Macromolecules, 1958, 12, 152-3.
Nature becomes fertile only by virtue of laws that oblige matter to organize itself into one of a number of necessarily very simple primitive forms. Because of their very simplicity, these are capable of constituting the basis for increasingly complex bodies, by the addition of organs calculated according to identical laws of possibility.
'Matiere', Dictionnaire Classique d' Histoire Naturelle (1822-31), Vol. 10, 277, trans. J. Mandelbaum. Quoted in Pietro Corsi, The Age of Lamarck (1988), 225.
Nature offers us a thousand simple pleasures—plays of light and color, fragrance in the air, the sun’s warmth on skin and muscle, the audible rhythm of life’s stir and push—for the price of merely paying attention. What joy! But how unwilling or unable many of us are to pay this price in an age when manufactured sources of stimulation and pleasure are everywhere at hand. For me, enjoying nature’s pleasures takes conscious choice, a choice to slow down to seed time or rock time, to still the clamoring ego, to set aside plans and busyness, and to simply to be present in my body, to offer myself up.
In Sisters of the Earth: Women’s Prose and Poetry (1991), 43.
Nature seems to take advantage of the simple mathematical representations of the symmetry laws. When one pauses to consider the elegance and the beautiful perfection of the mathematical reasoning involved and contrast it with the complex and far-reaching physical consequences, a deep sense of respect for the power of the symmetry laws never fails to develop.
Nobel Lecture (11 Dec 1957). In Nobel Lectures: Physics, 1981-1990) (1998), 394-395.
Nature, displayed in its full extent, presents us with an immense tableau, in which all the order of beings are each represented by a chain which sustains a continuous series of objects, so close and so similar that their difference would be difficult to define. This chain is not a simple thread which is only extended in length, it is a large web or rather a network, which, from interval to interval, casts branches to the side in order to unite with the networks of another order.
'Les Oiseaux Qui Ne Peuvent Voler', Histoire Naturelle des Oiseaux (1770), Vol. I, 394. Trans. Phillip R. Sloan.
Never believe that the atom is a complex mystery—it is not. The atom is what we find when we look for the underlying architecture in nature, whose bricks are as few, as simple and orderly as possible.
Quoted in Andrew Jon Rotter, Hiroshima (2008), 7, without citation. Also in 'ABC of the Atom'. Reader's Digest (Feb 1952), 40, 25.
No hypothesis concerning the nature of this 'something' shall be advanced thereby or based thereon. Therefore it appears as most simple to use the last syllable 'gen' taken from Darwin's well-known word pangene since it alone is of interest to use, in order thereby to replace the poor, more ambiguous word, 'Anlage'. Thus, we will say for 'das pangene' and 'die pangene' simply 'Das Gen' and 'Die Gene,' The word Gen is fully free from every hypothesis; it expresses only the safely proved fact that in any case many properties of organisms are conditioned by separable and hence independent 'Zustiinde,' 'Grundlagen,' 'Anlagen'—in short what we will call 'just genes'—which occur specifically in the gametes.
Elemente der Exakten Erblichkeitslehre (1909), 124. Trans. G. E. Allen and quoted in G. E. Allen, Thomas Hunt Morgan: The Man and His Science (1978), 209-10 (Footnote 79).
No part of Mathematics suffers more from the triviality of its initial presentation to beginners than the great subject of series. Two minor examples of series, namely arithmetic and geometric series, are considered; these examples are important because they are the simplest examples of an important general theory. But the general ideas are never disclosed; and thus the examples, which exemplify nothing, are reduced to silly trivialities.
In An Introduction to Mathematics (1911), 194.
No problem can be solved until it is reduced to some simple form. The changing of a vague difficulty into a specific, concrete form is a very essential element in thinking.
Seen, for example, in The Grain and Feed Review (1931), 21, 34.
No substantial part of the universe is so simple that it can be grasped and controlled without abstraction. Abstraction consists in replacing the part of the universe under consideration by a model of similar but simpler structure. Models, formal and intellectual on the one hand, or material on the other, are thus a central necessity of scientific procedure.
As coauthor with Norbert Wiener in 'The Role of Models in Science', Philosophy of Science (Oct 1945), 12, No. 4, 316.
Now, we propose in the first place to show, that this law of organic progress is the law of all progress. Whether it be in the development of the Earth, in the development in Life upon its surface, in the development of Society, of Government, of Manufactures, of Commerce, of Language, Literature, Science, Art, this same evolution of the simple into the complex, through a process of continuous differentiation, holds throughout. From the earliest traceable cosmical changes down to the latest results of civilization, we shall find that the transformation of the homogeneous into the heterogeneous is that in which Progress essentially consists.
'Progress: Its Law and Cause', Westminster Review (1857), 67, 446-7.
Numbers have neither substance, nor meaning, nor qualities. They are nothing but marks, and all that is in them we have put into them by the simple rule of straight succession.
In 'Mathematics and the Laws of Nature', The Armchair Science Reader (1959), 301.
O. Hahn and F. Strassmann have discovered a new type of nuclear reaction, the splitting into two smaller nuclei of the nuclei of uranium and thorium under neutron bombardment. Thus they demonstrated the production of nuclei of barium, lanthanum, strontium, yttrium, and, more recently, of xenon and caesium. It can be shown by simple considerations that this type of nuclear reaction may be described in an essentially classical way like the fission of a liquid drop, and that the fission products must fly apart with kinetic energies of the order of hundred million electron-volts each.
'Products of the Fission of the Urarium Nucleus', Nature (1939), 143, 471.
Observation is simple, indefatigable, industrious, upright, without any preconceived opinion. Experiment is artificial, impatient, busy, digressive; passionate, unreliable. We see every day one experiment after another, the second outweighing the impression gained from the first, both, often enough, carried out by men who are neither much distinguished for their spirit, nor for carrying with them the truth of personality and self denial. Nothing is easier than to make a series of so-called interesting experiments. Nature can only in some way be forced, and in her distress, she will give her suffering answer. Nothing is more difficult than to explain it, nothing is more difficult than a valid physiological experiment. We consider as the first task of current physiology to point at it and comprehend it.
Inaugural lecture as docent of physiology at the University of Bonn (19 Oct) 1824. Published in Johannes Muller, Zur vergleichenden Physiologie des Gesichtssinnes des Menschen und der Thiere (1826), 20.
Of course, Behaviourism “works.” So does torture. Give me a no-nonsense, down-to-earth behaviourist, a few drugs, and simple electrical appliances, and in six months I will have him reciting the Athanasian Creed in public.
A Certain World: A Commonplace Book (1971),33.
Ohm found that the results could be summed up in such a simple law that he who runs may read it, and a schoolboy now can predict what a Faraday then could only guess at roughly. By Ohm's discovery a large part of the domain of electricity became annexed by Coulomb's discovery of the law of inverse squares, and completely annexed by Green's investigations. Poisson attacked the difficult problem of induced magnetisation, and his results, though differently expressed, are still the theory, as a most important first approximation. Ampere brought a multitude of phenomena into theory by his investigations of the mechanical forces between conductors supporting currents and magnets. Then there were the remarkable researches of Faraday, the prince of experimentalists, on electrostatics and electrodynamics and the induction of currents. These were rather long in being brought from the crude experimental state to a compact system, expressing the real essence. Unfortunately, in my opinion, Faraday was not a mathematician. It can scarely be doubted that had he been one, he would have anticipated much later work. He would, for instance, knowing Ampere's theory, by his own results have readily been led to Neumann’s theory, and the connected work of Helmholtz and Thomson. But it is perhaps too much to expect a man to be both the prince of experimentalists and a competent mathematician.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
On May 15, 1957 Linus Pauling made an extraordinary speech to the students of Washington University. ... It was at this time that the idea of the scientists' petition against nuclear weapons tests was born. That evening we discussed it at length after dinner at my house and various ones of those present were scribbling and suggesting paragraphs. But it was Linus Pauling himself who contributed the simple prose of the petition that was much superior to any of the suggestions we were making.
Speech, "The 1962 Nobel Peace Prize," at Unitarian Church, Boulder, Colorado (20 Oct 1963). On Oregon State University Library website.
One feature which will probably most impress the mathematician accustomed to the rapidity and directness secured by the generality of modern methods is the deliberation with which Archimedes approaches the solution of any one of his main problems. Yet this very characteristic, with its incidental effects, is calculated to excite the more admiration because the method suggests the tactics of some great strategist who foresees everything, eliminates everything not immediately conducive to the execution of his plan, masters every position in its order, and then suddenly (when the very elaboration of the scheme has almost obscured, in the mind of the spectator, its ultimate object) strikes the final blow. Thus we read in Archimedes proposition after proposition the bearing of which is not immediately obvious but which we find infallibly used later on; and we are led by such easy stages that the difficulties of the original problem, as presented at the outset, are scarcely appreciated. As Plutarch says: “It is not possible to find in geometry more difficult and troublesome questions, or more simple and lucid explanations.” But it is decidedly a rhetorical exaggeration when Plutarch goes on to say that we are deceived by the easiness of the successive steps into the belief that anyone could have discovered them for himself. On the contrary, the studied simplicity and the perfect finish of the treatises involve at the same time an element of mystery. Though each step depends on the preceding ones, we are left in the dark as to how they were suggested to Archimedes. There is, in fact, much truth in a remark by Wallis to the effect that he seems “as it were of set purpose to have covered up the traces of his investigation as if he had grudged posterity the secret of his method of inquiry while he wished to extort from them assent to his results.” Wallis adds with equal reason that not only Archimedes but nearly all the ancients so hid away from posterity their method of Analysis (though it is certain that they had one) that more modern mathematicians found it easier to invent a new Analysis than to seek out the old.
In The Works of Archimedes (1897), Preface, vi.
One hardly knows where, in the history of science, to look for an important movement that had its effective start in so pure and simple an accident as that which led to the building of the great Washington telescope, and went on to the discovery of the satellites of Mars.
In The Reminiscences of an Astronomer (1903), Vol. 3, 128.
One rarely hears of the mathematical recitation as a preparation for public speaking. Yet mathematics shares with these studies [foreign languages, drawing and natural science] their advantages, and has another in a higher degree than either of them.
Most readers will agree that a prime requisite for healthful experience in public speaking is that the attention of the speaker and hearers alike be drawn wholly away from the speaker and concentrated upon the thought. In perhaps no other classroom is this so easy as in the mathematical, where the close reasoning, the rigorous demonstration, the tracing of necessary conclusions from given hypotheses, commands and secures the entire mental power of the student who is explaining, and of his classmates. In what other circumstances do students feel so instinctively that manner counts for so little and mind for so much? In what other circumstances, therefore, is a simple, unaffected, easy, graceful manner so naturally and so healthfully cultivated? Mannerisms that are mere affectation or the result of bad literary habit recede to the background and finally disappear, while those peculiarities that are the expression of personality and are inseparable from its activity continually develop, where the student frequently presents, to an audience of his intellectual peers, a connected train of reasoning. …
One would almost wish that our institutions of the science and art of public speaking would put over their doors the motto that Plato had over the entrance to his school of philosophy: “Let no one who is unacquainted with geometry enter here.”
Most readers will agree that a prime requisite for healthful experience in public speaking is that the attention of the speaker and hearers alike be drawn wholly away from the speaker and concentrated upon the thought. In perhaps no other classroom is this so easy as in the mathematical, where the close reasoning, the rigorous demonstration, the tracing of necessary conclusions from given hypotheses, commands and secures the entire mental power of the student who is explaining, and of his classmates. In what other circumstances do students feel so instinctively that manner counts for so little and mind for so much? In what other circumstances, therefore, is a simple, unaffected, easy, graceful manner so naturally and so healthfully cultivated? Mannerisms that are mere affectation or the result of bad literary habit recede to the background and finally disappear, while those peculiarities that are the expression of personality and are inseparable from its activity continually develop, where the student frequently presents, to an audience of his intellectual peers, a connected train of reasoning. …
One would almost wish that our institutions of the science and art of public speaking would put over their doors the motto that Plato had over the entrance to his school of philosophy: “Let no one who is unacquainted with geometry enter here.”
In A Scrap-book of Elementary Mathematics: Notes, Recreations, Essays (1908), 210-211.
One reason which has led the organic chemist to avert his mind from the problems of Biochemistry is the obsession that the really significant happenings in the animal body are concerned in the main with substances of such high molecular weight and consequent vagueness of molecular structure as to make their reactions impossible of study by his available and accurate methods. There remains, I find, pretty widely spread, the feeling—due to earlier biological teaching—that, apart from substances which are obviously excreta, all the simpler products which can be found in cells or tissues are as a class mere objects, already too remote from the fundamental biochemical events to have much significance. So far from this being the case, recent progress points in the clearest way to the fact that the molecules with which a most important and significant part of the chemical dynamics of living tissues is concerned are of a comparatively simple character.
In 'The Dynamic Side of Biochemistry', Address (11 Sep 1913) in Report on the 83rd Meeting of the British Association for the Advancement of Science (1914), 657-8.
Organisms are not billiard balls, propelled by simple and measurable external forces to predictable new positions on life’s pool table. Sufficiently complex systems have greater richness. Organisms have a history that constrains their future in myriad, subtle ways.
In The Panda’s Thumb: More Reflections in Natural History (1987, 2010), 16.
Our job in physics is to see things simply, to understand a great many complicated phenomena in a unified way, in terms of a few simple principles.
In Nobel Lecture (8 Dec 1989), 'Conceptual Foundations of the Unified Theory of Weak and Electromagnetic Interactions.'
Our new idea is simple: to build a physics valid for all coordinate systems.
Out of all possible universes, the only one which can exist, in the sense that it can be known, is simply the one which satisfies the narrow conditions necessary for the development of intelligent life.
From In the Centre of Immensities: Creation (1979), as cited in Bill Swainson, The Encarta Book of Quotations (2000), 579.
People say to me, “Are you looking for the ultimate laws of physics?” No, I’m not; I’m just looking to find out more about the world and if it turns out there is a simple ultimate law which explains everything, so be it; that would be very nice to discover. If it turns out it’s like an onion with millions of layers, and we’re just sick and tired of looking at the layers, then that’s the way it is …
From Interview in BBC TV program Horizon (1981). As quoted in The Pleasure of Finding Things Out: The Best Short Works of Richard P. Feynman 1983, (1999), 23.
People wonder why the novel is the most popular form of literature; people wonder why it is read more than books of science or books of metaphysics. The reason is very simple; it is merely that the novel is more true than they are. … In the fiery alphabet of every sunset is written “to be continued in our next.”
'On Certain Modern Writers and the institution of the Family' Heretics (1903). Collected in G. K. Chesterton and Dale Ahlquist (ed.), In Defense of Sanity: The Best Essays of G.K. Chesterton (2011), 82.
Perhaps we see equations as simple because they are easily expressed in terms of mathematical notation already invented at an earlier stage of development of the science, and thus what appears to us as elegance of description really reflects the interconnectedness of Nature's laws at different levels.
Nobel Banquet Speech (10 Dec 1969), in Wilhelm Odelberg (ed.),Les Prix Nobel en 1969 (1970).
Philosophically, I liked the steady-state cosmology. So I thought that we should report our results as a simple measurement; the measurement might be true after the cosmology was no longer true!
Remarking on the measurement he made with Arno Penzias of the 3 K cosmic background radiation. From Proceedings of workshop, National Radio Astronomy Observatory, Green Bank, West Virginia (4-6 May 1983), 'Discovery of the Cosmic Microwave Background', Serendipitous Discoveries in Radio Astronomy (1983), 195. Also collected in B. Bertotti (ed.) Modern Cosmology in Retrospect (1990), 303.
Philosophy asks the simple question, What is it all about?
In 'Remarks: Analysis of Meaning', The Philosophical Review (Mar 1937), 46,
No. 2, 178. Collected in Barbara MacKinnon, American Philosophy: A Historical Anthology (1985), 406.
Physical science is like simple addition: it is either infallible or it is false.
In All Things Considered (1908), 187.
Probably the simple facts about health are that all of us form bad dietary habits when we have young stomachs, and continue in them when our stomachs show the natural wear of long use. Stomachs weaken, as do eyes; but we cannot buy spectacles for our stomachs.
In Sinner Sermons: A Selection of the Best Paragraphs of E. W. Howe (1926), 12.
Producing food for 6.2 billion people, adding a population of 80 million more a year, is not simple. We better develop an ever improved science and technology, including the new biotechnology, to produce the food that’s needed for the world today. In response to the fraction of the world population that could be fed if current farmland was convered to organic-only crops: “We are 6.6 billion people now. We can only feed 4 billion. I don’t see 2 billion volunteers to disappear.” In response to extreme critics: “These are utopian people that live on Cloud 9 and come into the third world and cause all kinds of confusion and negative impacts on the developing countries.”
…...
Professor [Max] Planck, of Berlin, the famous originator of the Quantum Theory, once remarked to me that in early life he had thought of studying economics, but had found it too difficult! Professor Planck could easily master the whole corpus of mathematical economics in a few days. He did not mean that! But the amalgam of logic and intuition and the wide knowledge of facts, most of which are not precise, which is required for economic interpretation in its highest form is, quite truly, overwhelmingly difficult for those whose gift mainly consists in the power to imagine and pursue to their furthest points the implications and prior conditions of comparatively simple facts which are known with a high degree of precision.
'Alfred Marshall: 1842-1924' (1924). In Geoffrey Keynes (ed.), Essays in Biography (1933), 191-2
PROJECTILE, n. The final arbiter in international disputes. Formerly these disputes were settled by physical contact of the disputants, with such simple arguments as the rudimentary logic of the times could supply —the sword, the spear, and so forth. With the growth of prudence in military affairs the projectile came more and more into favor, and is now held in high esteem by the most courageous. Its capital defect is that it requires personal attendance at the point of propulsion.
The Collected Works of Ambrose Bierce (1911), Vol. 7, The Devil's Dictionary, 268.
Psychoanalysis makes quite simple people feel they’re complex.
Spoken by character Harold Sigrift in the play Brief Moment, produced on Broadway 1932-33. In Terry Reed and Kenneth T. Reed, S. N. Behrman (1975), 129.
Pure mathematics is, in its way, the poetry of logical ideas. One seeks the most general ideas of operation which will bring together in simple, logical and unified form the largest possible circle of formal relationships. In this effort toward logical beauty spiritual formulas are discovered necessary for the deeper penetration into the laws of nature.
In letter (1 May 1935), Letters to the Editor, 'The Late Emmy Noether: Professor Einstein Writes in Appreciation of a Fellow-Mathematician', New York Times (4 May 1935), 12.
Radioimmunoassay (RIA) is simple in principle.
'Radioimmunoassay: A Probe for the Fine Structure of Biologic Systems', Nobel Lecture, 1977. In Nobel Lectures: Physiology or Medicine 1971-1980 (1992), 450.
Run the tape again, and let the tiny twig of Homo sapiens expire in Africa. Other hominids may have stood on the threshold of what we know as human possibilities, but many sensible scenarios would never generate our level of mentality. Run the tape again, and this time Neanderthal perishes in Europe and Homo erectus in Asia (as they did in our world). The sole surviving human stock, Homo erectus in Africa, stumbles along for a while, even prospers, but does not speciate and therefore remains stable. A mutated virus then wipes Homo erectus out, or a change in climate reconverts Africa into inhospitable forest. One little twig on the mammalian branch, a lineage with interesting possibilities that were never realized, joins the vast majority of species in extinction. So what? Most possibilities are never realized, and who will ever know the difference? Arguments of this form lead me to the conclusion that biology's most profound insight into human nature, status, and potential lies in the simple phrase, the embodiment of contingency: Homo sapiens is an entity, not a tendency.
Wonderful Life (1989), 320.
Rutherford was as straightforward and unpretentious as a physicist as he was elsewhere in life, and that no doubt was one of the secrets of his success. “I was always a believer in simplicity, being a simple man myself,” he said. If a principle of physics could not be explained to a barmaid, he insisted, the problem was with the principle, not the barmaid.
In Great Physicists (2001), 328.
Science gains from it [the pendulum] more than one can expect. With its huge dimensions, the apparatus presents qualities that one would try in vain to communicate by constructing it on a small [scale], no matter how carefully. Already the regularity of its motion promises the most conclusive results. One collects numbers that, compared with the predictions of theory, permit one to appreciate how far the true pendulum approximates or differs from the abstract system called 'the simple pendulum'.
In 'Demonstration Experimentale du Movement de Rotation de la Terre' (31 May 1851). In C.M. Gariel (ed.), J. Bertrand (ed.) and Harold Burstyn (trans.), Recueil des Travaux Scientifiques de Lion Foucault (1878), Vol. 2, 527.
Science has a simple faith, which transcends utility. Nearly all men of science, all men of learning for that matter, and men of simple ways too, have it in some form and in some degree. It is the faith that it is the privilege of man to learn to understand, and that this is his mission. If we abandon that mission under stress we shall abandon it forever, for stress will not cease. Knowledge for the sake of understanding, not merely to prevail, that is the essence of our being. None can define its limits, or set its ultimate boundaries.
Science is Not Enough (1967), 191.
Science is always simple and profound. It is only the half truths that are dangerous.
…...
Science is beautiful when it makes simple explanations of phenomena or connections between different observations. Examples include the double helix in biology, and the fundamental equations of physics.
[Answer to question: What are the things you find most beautiful in science?]
[Answer to question: What are the things you find most beautiful in science?]
'Stephen Hawking: "There is no heaven; it's a fairy story"', interview in newspaper The Guardian (15 May 2011).
Science is simply common sense at its best—that is, rigidly accurate in observation, and merciless to fallacy in logic.
In The Crayfish: An Introduction to the Study of Zoology (1880), 2.
Science is triumphant with far-ranging success, but its triumph is somehow clouded by growing difficulties in providing for the simple necessities of human life on earth.
In Science and Survival (1966).
Scientific discovery, or the formulation of scientific theory, starts in with the unvarnished and unembroidered evidence of the senses. It starts with simple observation—simple, unbiased, unprejudiced, naive, or innocent observation—and out of this sensory evidence, embodied in the form of simple propositions or declarations of fact, generalizations will grow up and take shape, almost as if some process of crystallization or condensation were taking place. Out of a disorderly array of facts, an orderly theory, an orderly general statement, will somehow emerge.
In 'Is the Scientific Paper Fraudulent?', The Saturday Review (1 Aug 1964), 42.
Shall an invention be patented or donated to the public freely? I have known some well-meaning scientific men to look askance at the patenting of inventions, as if it were a rather selfish and ungracious act, essentially unworthy. The answer is very simple. Publish an invention freely, and it will almost surely die from lack of interest in its development. It will not be developed and the world will not be benefited. Patent it, and if valuable, it will be taken up and developed into a business.
Address as M.I.T. acting president, to the graduating class (11 Jun 1920). Published in Massachusetts Institute of Technology, Technology Review (Jul 1920), 22, 420.
Simple as the law of gravity now appears, and beautifully in accordance with all the observations of past and of present times, consider what it has cost of intellectual study. Copernicus, Galileo, Kepler, Euler, Lagrange, Laplace, all the great names which have exalted the character of man, by carrying out trains of reasoning unparalleled in every other science; these, and a host of others, each of whom might have been the Newton of another field, have all labored to work out, the consequences which resulted from that single law which he discovered. All that the human mind has produced—the brightest in genius, the most persevering in application, has been lavished on the details of the law of gravity.
in The Ninth Bridgewater Treatise: A Fragment (1838), 57.
Simple diet is best.
Louis Klopsch, Many Thoughts of Many Minds (1896), 67.
Simple goitre is so simple to prevent that it can disappear as soon as society makes the choice.
(1915) As quoted in Basil S. Hetzel, Chance and Commitment: Memoirs of a Medical Scientist (2005), 195.
Simple molecules combine to make powerful chemicals. Simple cells combine to make powerful life-forms. Simple electronics combine to make powerful computers. Logically, all things are created by a combination of simpler, less capable components. Therefore, a supreme being must be in our future, not our origin. What if “God” is the consciousness that will be created when enough of us are connected by the Internet?!!
Thoughts by character Dogbert in Dilbert cartoon strip (11 Feb 1996).
Some mathematics problems look simple, and you try them for a year or so, and then you try them for a hundred years, and it turns out that they're extremely hard to solve. There's no reason why these problems shouldn't be easy, and yet they turn out to be extremely intricate. [Fermat's] Last Theorem is the most beautiful example of this.
From interview for PBS website on the NOVA program, 'The Proof'.
Space travel is at the frontier of my profession. It is going to be accomplished and I want to be in on it. There is also an element of simple duty involved. I am convinced that I have something to give this project.
As he wrote in an article for Life (14 Sep 1959), 38.
Suppose [an] imaginary physicist, the student of Niels Bohr, is shown an experiment in which a virus particle enters a bacterial cell and 20 minutes later the bacterial cell is lysed and 100 virus particles are liberated. He will say: “How come, one particle has become 100 particles of the same kind in 20 minutes? That is very interesting. Let us find out how it happens! How does the particle get in to the bacterium? How does it multiply? Does it multiply like a bacterium, growing and dividing, or does it multiply by an entirely different mechanism ? Does it have to be inside the bacterium to do this multiplying, or can we squash the bacterium and have the multiplication go on as before? Is this multiplying a trick of organic chemistry which the organic chemists have not yet discovered ? Let us find out. This is so simple a phenomenon that the answers cannot be hard to find. In a few months we will know. All we have to do is to study how conditions will influence the multiplication. We will do a few experiments at different temperatures, in different media, with different viruses, and we will know. Perhaps we may have to break into the bacteria at intermediate stages between infection and lysis. Anyhow, the experiments only take a few hours each, so the whole problem can not take long to solve.”
[Eight years later] he has not got anywhere in solving the problem he set out to solve. But [he may say to you] “Well, I made a slight mistake. I could not do it in a few months. Perhaps it will take a few decades, and perhaps it will take the help of a few dozen other people. But listen to what I have found, perhaps you will be interested to join me.”
[Eight years later] he has not got anywhere in solving the problem he set out to solve. But [he may say to you] “Well, I made a slight mistake. I could not do it in a few months. Perhaps it will take a few decades, and perhaps it will take the help of a few dozen other people. But listen to what I have found, perhaps you will be interested to join me.”
From 'Experiments with Bacterial Viruses (Bacteriophages)', Harvey Lecture (1946), 41, 161-162. As cited in Robert Olby, The Path of the Double Helix: The Discovery of DNA (1974, 1994), 237.
Take the rose—most people think it very beautiful: I don’t care for it at all. I prefer the cactus, for the simple reason that it has a more interesting personality. It has wonderfully adapted itself to its surroundings! It is the best illustration of the theory of evolution in plant life.
From George MacAdam, 'Steinmetz, Electricity’s Mastermind, Enters Politics', New York Times (2 Nov 1913), SM3.
That mathematics “do not cultivate the power of generalization,”; … will be admitted by no person of competent knowledge, except in a very qualified sense. The generalizations of mathematics, are, no doubt, a different thing from the generalizations of physical science; but in the difficulty of seizing them, and the mental tension they require, they are no contemptible preparation for the most arduous efforts of the scientific mind. Even the fundamental notions of the higher mathematics, from those of the differential calculus upwards are products of a very high abstraction. … To perceive the mathematical laws common to the results of many mathematical operations, even in so simple a case as that of the binomial theorem, involves a vigorous exercise of the same faculty which gave us Kepler’s laws, and rose through those laws to the theory of universal gravitation. Every process of what has been called Universal Geometry—the great creation of Descartes and his successors, in which a single train of reasoning solves whole classes of problems at once, and others common to large groups of them—is a practical lesson in the management of wide generalizations, and abstraction of the points of agreement from those of difference among objects of great and confusing diversity, to which the purely inductive sciences cannot furnish many superior. Even so elementary an operation as that of abstracting from the particular configuration of the triangles or other figures, and the relative situation of the particular lines or points, in the diagram which aids the apprehension of a common geometrical demonstration, is a very useful, and far from being always an easy, exercise of the faculty of generalization so strangely imagined to have no place or part in the processes of mathematics.
In An Examination of Sir William Hamilton’s Philosophy (1878), 612-13.
That the fundamental aspects of heredity should have turned out to be so extraordinarily simple supports us in the hope that nature may, after all, be entirely approachable. Her much-advertised inscrutability has once more been found to be an illusion due to our ignorance. This is encouraging, for, if the world in which we live were as complicated as some of our friends would have us believe we might well despair that biology could ever become an exact science.
The Physical Basis of Heredity (1919), 15.
That the machine of Heaven is not a hard and impervious body full of various real spheres, as up to now has been believed by most people. It will be proved that it extends everywhere, most fluid and simple, and nowhere presents obstacles as was formerly held, the circuits of the Planets being wholly free and without the labour and whirling round of any real spheres at all, being divinely governed under a given law.
De Mundi Aetherei Recentioribus Phaenomenis (On Recent Phenomena in the Aetherial World) (1588). Quoted in M. Boas Hall, The Scientific Renaissance 1450-1630 (1962), 117.
The Principia Mathematica developed an overall scheme of the universe, one far more elegant and enlightening than any the ancients had devised. And the Newtonian scheme was based on a set of assumptions, so few and so simple, developed through so clear and so enticing a line of mathematics that conservatives could scarcely find the heart and courage to fight it.
In Entry 231, 'Newton, Sir Isaac', Asimov’s Biographical Encyclopedia of Science and Technology (2nd rev ed., 1982), 152.
The actual evolution of mathematical theories proceeds by a process of induction strictly analogous to the method of induction employed in building up the physical sciences; observation, comparison, classification, trial, and generalisation are essential in both cases. Not only are special results, obtained independently of one another, frequently seen to be really included in some generalisation, but branches of the subject which have been developed quite independently of one another are sometimes found to have connections which enable them to be synthesised in one single body of doctrine. The essential nature of mathematical thought manifests itself in the discernment of fundamental identity in the mathematical aspects of what are superficially very different domains. A striking example of this species of immanent identity of mathematical form was exhibited by the discovery of that distinguished mathematician … Major MacMahon, that all possible Latin squares are capable of enumeration by the consideration of certain differential operators. Here we have a case in which an enumeration, which appears to be not amenable to direct treatment, can actually be carried out in a simple manner when the underlying identity of the operation is recognised with that involved in certain operations due to differential operators, the calculus of which belongs superficially to a wholly different region of thought from that relating to Latin squares.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 290.
The advance from the simple to the complex, through a process of successive differentiations, is seen alike in the earliest changes of the Universe to which we can reason our way back, and in the earliest changes which we can inductively establish; it is seen in the geologic and climatic evolution of the Earth; it is seen in the unfolding of every single organism on its surface, and in the multiplication of kinds of organisms; it is seen in the evolution of Humanity, whether contemplated in the civilized individual, or in the aggregate of races; it is seen in the evolution of Society in respect alike of its political, its religious, and its economical organization; and it is seen in the evolution of all those endless concrete and abstract products of human activity which constitute the environment of our daily life. From the remotest past which Science can fathom, up to the novelties of yesterday, that in which Progress essentially consists, is the transformation of the homogeneous into the heterogeneous.
Progress: Its Law and Cause (1857), 35.
The aim of science is to seek the simplest explanations of complex facts. We are apt to fall into the error of thinking that the facts are simple because simplicity is the goal of our quest. The guiding motto in the life of every natural philosopher should be, Seek simplicity and distrust it.
In The Concept of Nature: Tarner Lectures Delivered in Trinity College, November 1919 (1920), 163.
The ancestors of the higher animals must be regarded as one-celled beings, similar to the Amœbæ which at the present day occur in our rivers, pools, and lakes. The incontrovertible fact that each human individual develops from an egg, which, in common with those of all animals, is a simple cell, most clearly proves that the most remote ancestors of man were primordial animals of this sort, of a form equivalent to a simple cell. When, therefore, the theory of the animal descent of man is condemned as a “horrible, shocking, and immoral” doctrine, tho unalterable fact, which can be proved at any moment under the microscope, that the human egg is a simple cell, which is in no way different to those of other mammals, must equally be pronounced “horrible, shocking, and immoral.”
Translated from his Ueber die Entstehung und den Stammbaum des Menschengeschlechts, (1873), Vol. 2, as an epigraph to Chap. 6, The Evolution of Man, (1879), Vol 1, 120-121.
The ancients devoted a lifetime to the study of arithmetic; it required days to extract a square root or to multiply two numbers together. Is there any harm in skipping all that, in letting the school boy learn multiplication sums, and in starting his more abstract reasoning at a more advanced point? Where would be the harm in letting the boy assume the truth of many propositions of the first four books of Euclid, letting him assume their truth partly by faith, partly by trial? Giving him the whole fifth book of Euclid by simple algebra? Letting him assume the sixth as axiomatic? Letting him, in fact, begin his severer studies where he is now in the habit of leaving off? We do much less orthodox things. Every here and there in one’s mathematical studies one makes exceedingly large assumptions, because the methodical study would be ridiculous even in the eyes of the most pedantic of teachers. I can imagine a whole year devoted to the philosophical study of many things that a student now takes in his stride without trouble. The present method of training the mind of a mathematical teacher causes it to strain at gnats and to swallow camels. Such gnats are most of the propositions of the sixth book of Euclid; propositions generally about incommensurables; the use of arithmetic in geometry; the parallelogram of forces, etc., decimals.
In Teaching of Mathematics (1904), 12.
The basic ideas and simplest facts of set-theoretic topology are needed in the most diverse areas of mathematics; the concepts of topological and metric spaces, of compactness, the properties of continuous functions and the like are often indispensable.
As co-author with H. Hopf, Topologie I (1935), 23. As quoted, translated and cited in Reinhold Remmert, Theory of Complex Functions (1991), 9-10. From the original German, “Die Grundbegriffe und die einfachsten Tatsachen aus der mengentheoretischen Topologie braucht man in sehr verschiedenen Gebieten der Mathematik; die Begriffe des topologischen und des metrischen Raumes, der Kompaktheit, die Eigenschaften stetiger Abbildungen u. dgl. sind oft unentbehrlich.”
The chemical compounds are comparable to a system of planets in that the atoms are held together by chemical affinity. They may be more or less numerous, simple or complex in composition, and in the constitution of the materials, they play the same role as Mars and Venus do in our planetary system, or the compound members such as our earth with its moon, or Jupiter with its satellites... If in such a system a particle is replaced by one of different character, the equilibrium can persist, and then the new compound will exhibit properties similar to those shown by the original substance.
Quoted in Ralph Oesper, The Human Side of Scientists (1975), 55.
The complexity of the world is the outcome of huge numbers of sometimes conflicting simple events.
In 'Religion - The Antithesis to Science', Chemistry & Industry (Feb 1997).
The constant conditions which are maintained in the body might be termed equilibria. That word, however, has come to have fairly exact meaning as applied to relatively simple physico-chemical states, in closed systems, where known forces are balanced. The coordinated physiological processes which maintain most of the steady states in the organism are so complex and so peculiar to living beings—involving, as they may, the brain and nerves, the heart, lungs, kidneys and spleen, all working cooperatively—that I have suggested a special designation for these states, homeostasis. The word does not imply something set and immobile, a stagnation. It means a condition—a condition which may vary, but which is relatively constant.
In The Wisdom of the Body (1932), 24.
The construction of an analogue computer or a supersonic airplane is simple when compared to the mixture of space and evolutionary eons represented by a cell.
In 'The Wisdom of Wilderness', Life (22 Dec 1967), 63, No. 25, 10.
The curiosity remains… to grasp more clearly how the same matter, which in physics and chemistry displays orderly and reproducible and relatively simple properties, arranges itself in the most astounding fashions as soon as it is drawn into the orbit of the living organism. The closer one looks at these performances of matter in living organisms the more impressive the show becomes. The meanest living cell becomes a magic puzzle box full of elaborate and changing molecules.
From 'Life: The Magic Puzzle Box', Transactions of the Connecticut Academy of Arts and Sciences (Dec 1949), 38, 173-190.
The development of mathematics toward greater precision has led, as is well known, to the formalization of large tracts of it, so that one can prove any theorem using nothing but a few mechanical rules... One might therefore conjecture that these axioms and rules of inference are sufficient to decide any mathematical question that can at all be formally expressed in these systems. It will be shown below that this is not the case, that on the contrary there are in the two systems mentioned relatively simple problems in the theory of integers that cannot be decided on the basis of the axioms.
'On Formally Undecidable Propositions of Principia Mathematica and Related Systems I' (193 1), in S. Feferman (ed.), Kurt Gödel Collected Works: Publications 1929-1936 (1986), Vol. I, 145.
The electron is not as simple as it looks.
As quoted in Alan L. Mackay, “The Harvest of a Quiet Eye” (1977), 23. Cited as “recounted by Sir George Paget Thompson at the Electron Diffraction Conference, Imperial College, 1967.”
The essence of mathematics is not to make simple things complicated, but to make complicated things simple.
In A Mathematical Journey (1976), xi.
The essential characteristic of science is the simple idea of attempting “to ascertain objective truth without regard to personal desires.”
The Nature of Science and Other Lectures (1954), 8.
The examples which a beginner should choose for practice should be simple and should not contain very large numbers. The powers of the mind cannot be directed to two things at once; if the complexity of the numbers used requires all the student’s attention, he cannot observe the principle of the rule which he is following.
In Study and Difficulties of Mathematics (1902), chap. 3.
The experiments made on the mutual electrical relations of bodies have taught us that they can be divided into two classes: electropositive and electronegative. The simple bodies which belong to the first class, as well as their oxides, always take up positive electricity when they meet simple bodies or oxides belonging to the second class; and the oxides of the first class always behave with the oxides of the other like salifiable bases with acids.
Essai sur le théorie des proportions chimiques (1819). Translated in Henry M. Leicester and Herbert S. Klickstein, A Source Book in Chemistry 1400-1900 (1952), 260.
The fact that the proof of a theorem consists in the application of certain simple rules of logic does not dispose of the creative element in mathematics, which lies in the choice of the possibilities to be examined.
As co-author with Herbert Robbins, in What Is Mathematics?: An Elementary Approach to Ideas and Methods (1941, 1996), 15.
The final results [of work on the theory of relativity] appear almost simple; any intelligent undergraduate can understand them without much trouble. But the years of searching in the dark for a truth that one feels, but cannot express; the intense effort and the alternations of confidence and misgiving, until one breaks through to clarity and understanding, are only known to him who has himself experienced them.
Concluding remark of George Gibson lecture at the University of Glasgow, 'The Origins of the General Theory of Relativity', (20 Jun 1933). Published by Glasgow University as The Origins of the General Theory of Relativity: Being the First Lecture on the George A. Gibson Foundation in the University of Glasgow, Delivered on June 20th, 1933 (1933), 11. Also quoted in 'No Hitching Posts' The Atlantic (1936), 157, 251.
The first [quality] to be named must always be the power of attention, of giving one's whole mind to the patient without the interposition of anything of oneself. It sounds simple but only the very greatest doctors ever fully attain it. … The second thing to be striven for is intuition. This sounds an impossibility, for who can control that small quiet monitor? But intuition is only interference from experience stored and not actively recalled. … The last aptitude I shall mention that must be attained by the good physician is that of handling the sick man's mind.
In 'Art and Science in Medicine', The Collected Papers of Wilfred Trotter, FRS (1941), 98.
The first acquaintance which most people have with mathematics is through arithmetic. That two and two make four is usually taken as the type of a simple mathematical proposition which everyone will have heard of. … The first noticeable fact about arithmetic is that it applies to everything, to tastes and to sounds, to apples and to angels, to the ideas of the mind and to the bones of the body.
In An Introduction to Mathematics (1911), 9.
The frequency of disastrous consequences in compound fracture, contrasted with the complete immunity from danger to life or limb in simple fracture, is one of the most striking as well as melancholy facts in surgical practice.
'On a New Method of Treating Compound Fracture, Abscesses, etc: With Observations on the Conditions of Supperation', Part I, The Lancet (1867), 326.
The general disposition of the land [in the Periodic Kingdom] is one of metals in the west, giving way, as you travel eastward, to a varied landscape of nonmetals, which terminates in largely inert elements at the eastern shoreline. To the south of the mainland, there is an offshore island, which we shall call the Southern Island. It consists entirely of metals of subtly modulated personality. North of the mainland, situated rather like Iceland off the northwestern edge of Europe, lies a single, isolated region-hydrogen. This simple but gifted element is an essential outpost of the kingdom, for despite its simplicity it is rich in chemical personality. It is also the most abundant element in the universe and the fuel of the stars.
In The Periodic Kingdom: A Journey Into the Land of the Chemical Elements (1995), 9.
The great beauty of Darwin’s theory of evolution is that it explains how complex, difficult to understand things could have arisen step by plausible step, from simple, easy to understand beginnings. We start our explanation from almost infinitely simple beginnings: pure hydrogen and a huge amount of energy. Our scientific, Darwinian explanations carry us through a series of well-understood gradual steps to all the spectacular beauty and complexity of life.
From speech at the Edinburgh International Science Festival (15 Apr 1992), published in the Independent newspaper. Included in excerpt in Alec Fisher, The Logic of Real Arguments (2004), 84-85. The full speech was reprinted in The Nullifidian, (Dec 1994). Transcribed online in the Richard Dawkins archive, article 89, titled: Lecture from 'The Nullifidian' (Dec 94).
The greater the mind, the greater are the truths self-evident to it, and the greater also is its power to induce complex from simple truths—complex truths of which we may be as certain
as we are of the primary self-evident truths themselves.
In The Science of Poetry and the Philosophy of Language (1910), x.
The highest mathematical principles may be involved in the production of the simplest mechanical result.
In 'Academical Education', Orations and Speeches on Various Occasions (1870), Vol. 3, 513. This is seen misattributed to Eric Temple Bell, but he only quoted it, attributing it to Everett, in for example, Mathematics: Queen and Servant of Sciences (1938), 20.
The idea that the universe is running down comes from a simple observation about machines. Every machine consumes more energy than it renders.
In The Ascent of Man (1973).
The language of the genes has a simple alphabet, not with twenty-six letters, but just four. These are the four different DNA bases—adenine, guanine, cytosine and thymine (A, G, C and T for short). The bases are arranged in words of three letters such as CGA or TGG. Most of the words code for different amino acids, which themselves are joined together to make proteins, the building blocks of the body.
The Language of the Genes: Biology, History and the Evolutionary Future (1993), 3.
The man who inspired me most, I think, was Dr. Alfred Blalock, who was professor of surgery at Johns Hopkins. He was a rather simple man with a burning curiosity. It was through his curiosity that he made many real contributions to medical science.
The man who is thoroughly convinced of the universal operation of the law of causation cannot for a moment entertain the idea of a being who interferes in the course of events–provided, of course, that he takes the hypothesis of causality really seriously. He has no use for the religion of fear and equally little for social or moral religion. A God who rewards and punishes is inconceivable to him for the simple reason that a man’s actions are determined by necessity, external and internal, so that in God’s eyes he cannot be responsible, any more than an inanimate object is responsible for the motions it undergoes. Science has therefore been charged with undermining morality, but the charge is unjust. A man’s ethical behavior should be based effectually on sympathy, education, and social ties and needs; no religious basis is necessary. Man would indeed be in a poor way if he had to be restrained by fear of punishment and hopes of reward after death.
From 'Religion And Science', as collected in Ideas And Opinions (1954), 39, given its source as: “Written expressly for the New York Times Magazine. Appeared there November 9, 1930 (pp. 1-4). The German text was published in the Berliner Tageblatt, November 11, 1930.” The NYT Magazine article in full, is reprinted in Edward H. Cotton (ed.), Has Science Discovered God? A Symposium of Modern Scientific Opinion (1931), 101. This original version directly from the magazine has significantly different wording, beginning, “For anyone who is pervaded with the sense of causal law….” See this alternate form on the Albert Einstein Quotes page on this website. As for why the difference, Webmaster speculates the book form editor perhaps used a revised translation from Einstein’s German article.
The maxim of science is simply that of common sense—simple cases first; begin with seeing how the main force acts when there is as little as possible to impede it, and when you thoroughly comprehend that, add to it in succession the separate effects of each of the incumbering and interfering agencies.
Collected in The Works of Walter Bagehot (1889), Vol. 5, 319-320.
The metaphysical philosopher from his point of view recognizes mathematics as an instrument of education, which strengthens the power of attention, develops the sense of order and the faculty of construction, and enables the mind to grasp under the simple formulae the quantitative differences of physical phenomena.
In Dialogues of Plato (1897), Vol. 2, 78.
The method of science depends on our attempts to describe the world with simple theories: theories that are complex may become untestable, even if they happen to be true. Science may be described as the art of systematic over-simplification—the art of discerning what we may with advantage omit.
Karl Raimund Popper and William Warren Bartley (ed.), The Open Universe: an Argument for Indeterminism (1991), 44.
by Karl Raimund Popper, William Warren Bartley - Science - 1991
The mighty steam-engine has its germ in the simple boiler in which the peasant prepares his food. The huge ship is but the expansion of the floating leaf freighted with its cargo of atmospheric dust; and the flying balloon is but the infant's soap-bubble lightly laden and overgrown. But the Telescope, even in its most elementary form, embodies a novel and gigantic idea, without an analogue in nature, and without a prototype in experience
Stories of Inventors and Discoverers in Science and the Useful Arts (1860), 145.
The moment one has offered an original explanation for a phenomenon which seems satisfactory, that moment affection for his intellectual child springs into existence, and as the explanation grows into a definite theory his parental affections cluster about his offspring and it grows more and more dear to him. ... There springs up also unwittingly a pressing of the theory to make it fit the facts and a pressing of the facts to make them fit the theory... To avoid this grave danger, the method of multiple working hypotheses is urged. It differs from the simple working hypothesis in that it distributes the effort and divides the affections... In developing the multiple hypotheses, the effort is to bring up into view every rational exploration of the phenomenon in hand and to develop every tenable hypothesis relative to its nature, cause or origin, and to give to all of these as impartially as possible a working form and a due place in the investigation. The investigator thus becomes the parent of a family of hypotheses; and by his parental relations to all is morally forbidden to fasten his affections unduly upon anyone. ... Each hypothesis suggests its own criteria, its own method of proof, its own method of developing the truth, and if a group of hypotheses encompass the subject on all sides, the total outcome of means and of methods is full and rich.
'Studies for Students. The Method of Multiple Working Hypotheses', Journal of Geology (1897), 5, 840-6.
The moment you encounter string theory and realise that almost all of the major developments in physics over the last hundred years emerge—and emerge with such elegance—from such a simple starting point, you realise that this incredibly compelling theory is in a class of its own.
…...
The more important fundamental laws and facts of physical science have all been discovered, and these are now so firmly established that the possibility of their ever being supplanted in consequence of new discoveries is exceedingly remote. Nevertheless, it has been found that there are apparent exceptions to most of these laws, and this is particularly true when the observations are pushed to a limit, i.e., whenever the circumstances of experiment are such that extreme cases can be examined. Such examination almost surely leads, not to the overthrow of the law, but to the discovery of other facts and laws whose action produces the apparent exceptions. As instances of such discoveries, which are in most cases due to the increasing order of accuracy made possible by improvements in measuring instruments, may be mentioned: first, the departure of actual gases from the simple laws of the so-called perfect gas, one of the practical results being the liquefaction of air and all known gases; second, the discovery of the velocity of light by astronomical means, depending on the accuracy of telescopes and of astronomical clocks; third, the determination of distances of stars and the orbits of double stars, which depend on measurements of the order of accuracy of one-tenth of a second-an angle which may be represented as that which a pin's head subtends at a distance of a mile. But perhaps the most striking of such instances are the discovery of a new planet or observations of the small irregularities noticed by Leverrier in the motions of the planet Uranus, and the more recent brilliant discovery by Lord Rayleigh of a new element in the atmosphere through the minute but unexplained anomalies found in weighing a given volume of nitrogen. Many other instances might be cited, but these will suffice to justify the statement that “our future discoveries must be looked for in the sixth place of decimals.”
In Light Waves and Their Uses (1903), 23-4. Michelson had some years earlier referenced “an eminent physicist” that he did not name who had “remarked that the future truths of physical science are to be looked for in the sixth place of decimals,” near the end of his Convocation Address at the Dedication of the Ryerson Physical Laboratory at the University of Chicago, 'Some of the Objects and Methods of Physical Science' (4 Jul 1894), published in University of Chicago Quarterly Calendar (Aug 1894), 3, No.2, 15. Also
The more man inquires into the laws which regulate the material universe, the more he is convinced that all its varied forms arise from the action of a few simple principles. These principles themselves converge, with accelerating force, towards some still more comprehensive law to which all matter seems to be submitted. Simple as that law may possibly be, it must be remembered that it is only one amongst an infinite number of simple laws: that each of these laws has consequences at least as extensive as the existing one, and therefore that the Creator who selected the present law must have foreseen the consequences of all other laws.
In Passages from the Life of a Philosopher (1864), 402.
The more we learn of science, the more we see that its wonderful mysteries are all explained by a few simple laws so connected together and so dependent upon each other, that we see the same mind animating them all.
Sermon (c. 13 Jan. 1895), Mukwonago, Wisconsin, published in Olympia Brown and Gwendolen B. Willis (ed.), Olympia Brown, An Autobiography (1960). Reprinted in Annual Journal of the Universalist Historical Society (1963), vol. 4, 103.
The more you understand the significance of evolution, the more you are pushed away from the agnostic position and towards atheism. Complex, statistically improbable things are by their nature more difficult to explain than simple, statistically probable things.
From edited version of a speech, at the Edinburgh International Science Festival (15 Apr 1992), as reprinted from the Independent newspaper in Alec Fisher, The Logic of Real Arguments (2004), 84.
The night before Easter Sunday of that year (1920) I awoke, turned on the light, and jotted down a few notes on a tiny slip of thin paper. Then I fell asleep again. It occurred to me at six o’clock in the morning that during the night I had written down something most important, but I was unable to decipher the scrawl. The next night, at three o’clock, the idea returned. It was the design of an experiment to determine whether the hypothesis of chemical transmission that I had uttered seventeen years ago was correct. I got up immediately, went to the laboratory, and performed a simple experiment on a frog heart according to the nocturnal design. I have to describe this experiment briefly since its results became the foundation of the theory of chemical transmission of the nervous impulse. The hearts of two frogs were isolated, the first with its nerves, the second without. Both hearts were attached to Straub cannulas filled with a little Ringer solution. The vagus nerve of the first heart was stimulated for a few minutes. Then the Ringer solution that had been in the first heart during the stimulation of the vagus was transferred to the second heart. It slowed and its beats diminished just as if its vagus had been stimulated. Similarly, when the accelerator nerve was stimulated and the Ringer from this period transferred, the second heart speeded up and its beats increased. These results unequivocally proved that the nerves do not influence the heart directly but liberate from their terminals specific chemical substances which, in their turn, cause the well-known modifications of the function of the heart characteristic of the stimulation of its nerves.
'An Autobiographic Sketch', Perspectives in Biology and Medicine (1960), 4, 17.
The old saying of the two kinds of truth. To the one kind belongs statements so simple and clear that the opposite assertion obviously could not be defended. The other kind, the so-called “deep truths”, are statements in which the opposite also contains deep truth.
Niels Bohr, 'Discussion with Einstein on Epistemological Problems in Atomic Physics', in P. A. Schilpp (ed.), Albert Einstein: Philosopher-Scientist (1949), 240.
The origin of a science is usually to be sought for not in any systematic treatise, but in the investigation and solution of some particular problem. This is especially the case in the ordinary history of the great improvements in any department of mathematical science. Some problem, mathematical or physical, is proposed, which is found to be insoluble by known methods. This condition of insolubility may arise from one of two causes: Either there exists no machinery powerful enough to effect the required reduction, or the workmen are not sufficiently expert to employ their tools in the performance of an entirely new piece of work. The problem proposed is, however, finally solved, and in its solution some new principle, or new application of old principles, is necessarily introduced. If a principle is brought to light it is soon found that in its application it is not necessarily limited to the particular question which occasioned its discovery, and it is then stated in an abstract form and applied to problems of gradually increasing generality.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
In A Treatise on Projections (1880), Introduction, xi. Published as United States Coast and Geodetic Survey, Treasury Department Document, No. 61.
The origin of species is therefore simply the evolution of some difference—any difference at all—that prevents the production of fertile hybrids between populations under natural conditions.
In 'Biodiversity Rising', The Diversity of Life (1992), 56.
The peculiar taste both in pure and in mixed nature of those relations about which it is conversant, from its simple and definite phraseology, and from the severe logic so admirably displayed in the concatenation of its innumerable theorems, are indeed immense, and well entitled to separate and ample illustration.
In Philosophy of the Human Mind (1816), Vol. 2, Chap. 2, Sec. 3, 157.
The plain fact is that education is itself a form of propaganda–a deliberate scheme to outfit the pupil, not with the capacity to weigh ideas, but with a simple appetite for gulping ideas readymade. The aim is to make ‘good’ citizens, which is to say, docile and uninquisitive citizens.
…...
The plexus called rectiform [rete mirabile] by anatomists, is the most wonderful of the bodies located in this region. It encircles the gland [the hypophysis] itself and extends far to the rear; for nearly the whole base of the encephalon has this plexus lying beneath it. It is not a simple network but [looks] as if you had taken several fisherman’s nets and superimposed them. It is characteristic of this net of Nature’s, however, that the meshes of one layer are always attached to those of another, and it is impossible to remove anyone of them alone; for, one after another, the rest follow the one you are removing, because they are all attached to one another successively.
— Galen
On the Usefulness of the Parts of the Body, Book IX, 4. Trans. Margaret Tallmadge May (1968), Vol. 1, 430-1.
The point of philosophy is to start with something so simple as not to seem worth stating and to end with something so paradoxical that no one will believe it
The Philosophy of Logical Atomism (1959), 10.
The pre-Darwinian age had come to be regarded as a Dark Age in which men still believed that the book of Genesis was a standard scientific treatise, and that the only additions to it were Galileo’s demonstration of Leonardo da Vinci’s simple remark that the earth is a moon of the sun, Newton’s theory of gravitation, Sir Humphry Davy's invention of the safety-lamp, the discovery of electricity, the application of steam to industrial purposes, and the penny post.
Back to Methuselah: a Metabiological Pentateuch (1921), viii.
The problems of analyzing war operations are … rather nearer, in general, to many problems, say of biology or of economics, than to most problems of physics, where usually a great deal of numerical data are ascertainable about relatively simple phenomena.
In report at the British Association Annual Meeting, Dundee (30 Aug 1947), published in 'Operational Research in War and Peace', The Advancement of Science (1948), 17, 320-332. Collected in P.M.S. Blackett, Studies of War: Nuclear and Conventional (1962), 177.
The process of discovery is very simple. An unwearied and systematic application of known laws to nature, causes the unknown to reveal themselves. Almost any mode of observation will be successful at last, for what is most wanted is method.
In A Week on the Concord and Merrimack Rivers (1862), 382.
The processes concerned in simple descent are those of Family Variability and Reversion. It is well to define these words clearly. By family variability is meant the departure of the children of the same or similarly descended families from the ideal mean type of all of them. Reversion is the tendency of that ideal mean type to depart from the parent type, 'reverting' towards what may be roughly and perhaps fairly described as the average ancestral type. If family variability had been the only process in simple descent, the dispersion of the race would indefinitely increase with the number of the generations, but reversion checks this increase, and brings it to a standstill.
Typical Laws of Heredity (1877), 513.
The prominent reason why a mathematician can be judged by none but mathematicians, is that he uses a peculiar language. The language of mathesis is special and untranslatable. In its simplest forms it can be translated, as, for instance, we say a right angle to mean a square corner. But you go a little higher in the science of mathematics, and it is impossible to dispense with a peculiar language. It would defy all the power of Mercury himself to explain to a person ignorant of the science what is meant by the single phrase “functional exponent.” How much more impossible, if we may say so, would it be to explain a whole treatise like Hamilton’s Quaternions, in such a wise as to make it possible to judge of its value! But to one who has learned this language, it is the most precise and clear of all modes of expression. It discloses the thought exactly as conceived by the writer, with more or less beauty of form, but never with obscurity. It may be prolix, as it often is among French writers; may delight in mere verbal metamorphoses, as in the Cambridge University of England; or adopt the briefest and clearest forms, as under the pens of the geometers of our Cambridge; but it always reveals to us precisely the writer’s thought.
In North American Review (Jul 1857), 85, 224-225.
The reasoning of mathematicians is founded on certain and infallible principles. Every word they use conveys a determinate idea, and by accurate definitions they excite the same ideas in the mind of the reader that were in the mind of the writer. When they have defined the terms they intend to make use of, they premise a few axioms, or self-evident principles, that every one must assent to as soon as proposed. They then take for granted certain postulates, that no one can deny them, such as, that a right line may be drawn from any given point to another, and from these plain, simple principles they have raised most astonishing speculations, and proved the extent of the human mind to be more spacious and capacious than any other science.
In Diary, Works (1850), Vol. 2, 21.
The risk of developing carcinoma of the lung increases steadily as the amount smoked increases. If the risk among non-smokers is taken as unity and the resulting ratios in the three age groups in which a large number of patients were interviewed (ages 45 to 74) are averaged, the relative risks become 6, 19, 26, 49, and 65 when the number of cigarettes smoked a day are 3, 10, 20, 35, and, say, 60—that is, the mid-points of each smoking group. In other words, on the admittedly speculative assumptions we have made, the risk seems to vary in approximately simple proportion with the amount smoked.
William Richard Shaboe Doll and Austin Bradford Hill (1897-1991 British medical statistician) 'Smoking and Carcinoma of the Lung', British Medical Journal, 1950, ii, 746.
The sciences do not try to explain, they hardly even try to interpret, they mainly make models. By a model is meant a mathematical construct which, with the addition of certain verbal interpretations, describes observed phenomena. The justification of such a mathematical construct is solely and precisely that it is expected to work—that is, correctly to describe phenomena from a reasonably wide area. Furthermore, it must satisfy certain esthetic criteria—that is, in relation to how much it describes, it must be rather simple.
From 'Method in the Physical Sciences', in John von Neumann and L. Leary (ed.), The Unity of Knowledge (1955), 158. Reprinted in John Von Neumann, F. Bródy (ed.) and Tibor Vámos (ed.), The Neumann Compendium (2000), 628.
The simple and plain fact is that the scientific method wins its success by ignoring parts of reality as given in experience; it is perfectly right to do this for its own purposes; but it must not be permitted by a kind of bluff to create the impression that what it ignores is non-existent.
In Nature, Man and God: Being the Gifford Lectures Delivered in the University of Glasgow in the Academical Years 1932-1933 and 1933-1934 (1934), 51.
The simple fact is that the world is not paying for the services the forests provide. At the moment, they are worth more dead than alive–for soya, for beef, for palm oil and for logging, feeding the demand from other countries. … I think we need to be clear that the drivers of rainforest destruction do not originate in the rainforest nations, but in the more developed countries which, unwittingly or not, have caused climate change.
Presidential Lecture (3 Nov 2008) at the Presidential Palace, Jakarta, Indonesia. On the Prince of Wales website.
The simple rule about weapons is that if thery can be built, they will be built.
Accidental Empires (1992), 79.
The simplest schoolboy is now familiar with facts for which Archimedes would have sacrificed his life. What would we not give to make it possible for us to steal a look at a book that will serve primary schools in a hundred years?
In Souvenirs d’Enfance et de Jeunesse (1846), Preface, 13. From the original French, “Le simple écolier sait maintenant des vérités pour lesquelles Archimède eût sacrifié sa vie. Que ne donnerions-nous pas pour qu’il nous fût possible de jeter un coup d’œil furtif sur tel livre qui servira aux écoles primaires dans cent ans?”
The simplicity of nature is not to be measured by that of our conceptions. Infinitely varied in its effects, nature is simple only in its causes, and its economy consists in producing a great number of phenomena, often very complicated, by means of a small number of general laws.
Philosophical Essay on Probabilities (1825), trans. Andrew I. Dale (1995), book 1, chap. 14.
The steady states of the fluid matrix of the body are commonly preserved by physiological reactions, i.e., by more complicated processes than are involved in simple physico-chemical equilibria. Special designations, therefore, are appropriate:—“homeostasis” to designate stability of the organism; “homeostatic conditions,” to indicate details of the stability; and “homeostatic reactions,” to signify means for maintaining stability.
'Physiological Regulation of Normal States: Some Tentative Postulates Concerning Biological Homeostatics', 1926. Reprinted in L. L. Langley (ed.), Homeostasis: Origins of the Concept (1973), 246.
The study of … simple cases would, I think, often be of advantage even to students whose mathematical attainments are sufficient to enable them to follow the solution of the more general cases. For in these simple cases the absence of analytical difficulties allows attention to be more easily concentrated on the physical aspects of the question, and thus gives the student a more vivid idea and a more manageable grasp of the subject than he would be likely to attain if he merely regarded electrical phenomena through a cloud of analytical symbols.
Elements of the Mathematical Theory of Electricity and Magnetism (189S), v-vi.
The supreme goal of all theory is to make the irreducible basic elements as simple and as few as possible without having to surrender the adequate representation of a single datum of experience.
…...
The technologies which have had the most profound effects on human life are usually simple. A good example of a simple technology with profound historical consequences is hay. ... It was hay that allowed populations to grow and civilizations to flourish among the forests of Northern Europe. Hay moved the greatness of Rome to Paris and London, and later to Berlin and Moscow and New York.
[The year-round growth of green grass in the Mediterranean climate meant that hay was not needed by the Romans. North of the Alps, hay maintained horses and oxen and thus their motive power, and productivity.]
[The year-round growth of green grass in the Mediterranean climate meant that hay was not needed by the Romans. North of the Alps, hay maintained horses and oxen and thus their motive power, and productivity.]
In 'Quick is Beautiful', Infinite in All Directions: Gifford Lectures Given at Aberdeen, Scotland (1988, 2004), 135.
The technologies which have had the most profound effects on human life are usually simple. A good example of a simple technology with profound historical consequences is hay. Nobody knows who invented hay, the idea of cutting grass in the autumn and storing it in large enough quantities to keep horses and cows alive through the winter. All we know is that the technology of hay was unknown to the Roman Empire but was known to every village of medieval Europe. Like many other crucially important technologies, hay emerged anonymously during the so-called Dark Ages. According to the Hay Theory of History, the invention of hay was the decisive event which moved the center of gravity of urban civilization from the Mediterranean basin to Northern and Western Europe. The Roman Empire did not need hay because in a Mediterranean climate the grass grows well enough in winter for animals to graze. North of the Alps, great cities dependent on horses and oxen for motive power could not exist without hay. So it was hay that allowed populations to grow and civilizations to flourish among the forests of Northern Europe. Hay moved the greatness of Rome to Paris and London, and later to Berlin and Moscow and New York. ... Great inventions like hay and printing, whatever their immediate social costs may be, result in a permanent expansion of our horizons, a lasting acquisition of new territory for human bodies and minds to cultivate.
Infinite In All Directions (1988, 2004), 135. The book is a revised version of a series of the Gifford Lectures under the title 'In Praise of Diversity', given at Aberdeen, Scotland.
The ultimate aim of those who are devoted to science is to penetrate beyond the phenomena observed on the surface to the ultimate causes, and to reduce the whole … to a simple deductive system of mechanics, in which the phenomena observed shall be shown to flow naturally from the few simple laws that underlie the structure of the universe.
In article, 'Meteorolgy', Encyclopaedia Britannica, (11th ed., 1911), Vol. 18, 281
The underlying concepts that unlock nature must be shown to arise early and in the simplest cultures of man from his basic and specific faculties. And the development of science which joins them in more and more complex conjunctions must be seen to be equally human: discoveries are made by men, not merely by minds, so that they are alive and charged with individuality.
In 'Foreward', The Ascent of Man, (1973), 13-14.
The Unexpected stalks a farm in big boots like a vagrant bent on havoc. Not every farmer is an inventor, but the good ones have the seeds of invention within them. Economy and efficiency move their relentless tinkering and yet the real motive often seems to be aesthetic. The mind that first designed a cutter bar is not far different from a mind that can take the intractable steel of an outsized sickle blade and make it hum in the end. The question is how to reduce the simplicity that constitutes a problem (“It's simple; it’s broke.”) to the greater simplicity that constitutes a solution.
In Making Hay (2003), 33-34.
The universe is of the nature of a thought or sensation in a universal Mind… To put the conclusion crudely—the stuff of the world is mind-stuff. As is often the way with crude statements, I shall have to explain that by “mind” I do not exactly mean mind and by “stuff” I do not at all mean stuff. Still that is about as near as we can get to the idea in a simple phrase. The mind-stuff of the world is something more general than our individual conscious minds; but we may think of its nature as not altogether foreign to feelings in our consciousness… Having granted this, the mental activity of the part of world constituting ourselves occasions no great surprise; it is known to us by direct self-knowledge, and we do not explain it away as something other than we know it to be—or rather, it knows itself to be.
From Gifford Lecture, Edinburgh, (1927), 'Reality', collected in The Nature of the Physical World (1928), 276.
The universe is simple; it’s the explanation that’s complex.
The value of fundamental research does not lie only in the ideas it produces. There is more to it. It affects the whole intellectual life of a nation by determining its way of thinking and the standards by which actions and intellectual production are judged. If science is highly regarded and if the importance of being concerned with the most up-to-date problems of fundamental research is recognized, then a spiritual climate is created which influences the other activities. An atmosphere of creativity is established which penetrates every cultural frontier. Applied sciences and technology are forced to adjust themselves to the highest intellectual standards which are developed in the basic sciences. This influence works in many ways: some fundamental students go into industry; the techniques which are applied to meet the stringent requirements of fundamental research serve to create new technological methods. The style, the scale, and the level of scientific and technical work are determined in pure research; that is what attracts productive people and what brings scientists to those countries where science is at the highest level. Fundamental research sets the standards of modern scientific thought; it creates the intellectual climate in which our modern civilization flourishes. It pumps the lifeblood of idea and inventiveness not only into the technological laboratories and factories, but into every cultural activity of our time. The case for generous support for pure and fundamental science is as simple as that.
In 'Why Pure Science?' in Bulletin of the Atomic Scientists, 1965.
The value of mathematical instruction as a preparation for those more difficult investigations, consists in the applicability not of its doctrines but of its methods. Mathematics will ever remain the past perfect type of the deductive method in general; and the applications of mathematics to the simpler branches of physics furnish the only school in which philosophers can effectually learn the most difficult and important of their art, the employment of the laws of simpler phenomena for explaining and predicting those of the more complex. These grounds are quite sufficient for deeming mathematical training an indispensable basis of real scientific education, and regarding with Plato, one who is … as wanting in one of the most essential qualifications for the successful cultivation of the higher branches of philosophy
In System of Logic, Bk. 3, chap. 24, sect. 9.
The visible figures by which principles are illustrated should, so far as possible, have no accessories. They should be magnitudes pure and simple, so that the thought of the pupil may not be distracted, and that he may know what features of the thing represented he is to pay attention to.
In National Education Association of the United States, Report of the Committee of Ten on Secondary School Subjects, (1894), 109.
The way a child discovers the world constantly replicates the way science began. You start to notice what’s around you, and you get very curious about how things work. How things interrelate. It’s as simple as seeing a bug that intrigues you. You want to know where it goes at night; who its friends are; what it eats.
In David Chronenberg and Chris Rodley (ed.), Chronenberg on Chronenberg (1992), 5.
The wisest man could ask no more of fate
Than to be simple, modest, manly, true,
Safe from the many, honored by the few;
Nothing to count in world, or church, or state,
But inwardly in secret to be great;
To feel mysterious nature ever new,
To touch, if not to grasp, her endless clue,
And learn by each discovery how to wait,
To widen knowledge and escape the praise;
Wisely to teach because more wise to learn;
To toil for science, not to draw men’s gate,
But for her love of self denial stern;
That such a man could spring from our decays
Fans the soul’s nobler faith until it burn.
Than to be simple, modest, manly, true,
Safe from the many, honored by the few;
Nothing to count in world, or church, or state,
But inwardly in secret to be great;
To feel mysterious nature ever new,
To touch, if not to grasp, her endless clue,
And learn by each discovery how to wait,
To widen knowledge and escape the praise;
Wisely to teach because more wise to learn;
To toil for science, not to draw men’s gate,
But for her love of self denial stern;
That such a man could spring from our decays
Fans the soul’s nobler faith until it burn.
Elegy to Dr. Leidy for introduction to the inauguration of the Joseph Leidy Memorial Lectureship. As published in 'The Joseph Leidy Lecture: Introduction by the Provost of the University of Pennsylvania', Science (30 May 1913), 37, No. 961, 809.
The world is very complicated and it is clearly impossible for the human mind to understand it completely. Man has therefore devised an artifice which permits the complicated nature of the world to be blamed on something which is called accidental and thus permits him to abstract a domain in which simple laws can be found.
In Floyd Merrell, Unthinking Thinking: Jorge Luis Borges, Mathematics, and the New Physics (1991), 156.
Theorem I. The first and most simple manifestation and representation of things, non-existent as well as latent in the folds of Nature, happened by means of straight line and circle.
Theorem II. Yet the circle cannot be artificially produced without the straight line, or the straight line without the point. Hence, things first began to be by way of a point, and a monad. And things related to the periphery (however big they may be) can in no way exist without the aid of the central point.
Theorem II. Yet the circle cannot be artificially produced without the straight line, or the straight line without the point. Hence, things first began to be by way of a point, and a monad. And things related to the periphery (however big they may be) can in no way exist without the aid of the central point.
— John Dee
From Monas Hierogyphica: Ioannia Dee, Londinensis (The Hieroglyphic Monad of John Dee, of London) translated by C. H.Josten, in Ambix, vol. 12 (1964). As quoted and cited in Philip Davis with Reuben Hersh, in The Mathematical Experience (1981), 103.
There are also two kinds of truths, those of reasoning and those of fact. Truths of reasoning are necessary and their opposite is impossible: truths of fact are contingent and their opposite is possible. When a truth is necessary, reason can be found by analysis, resolving it into more simple ideas and truths, until we come to those which are primary.
The Monadology and Other Philosophical Writings (1714), trans. Robert Latta (1898), 235-6.
There are no better terms available to describe the difference between the approach of the natural and the social sciences than to call the former ‘objective’ and the latter ‘subjective.’ ... While for the natural scientist the contrast between objective facts and subjective opinions is a simple one, the distinction cannot as readily be applied to the object of the social sciences. The reason for this is that the object, the ‘facts’ of the social sciences are also opinions—not opinions of the student of the social phenomena, of course, but opinions of those whose actions produce the object of the social scientist.
…...
There are several kinds of truths, and it is customary to place in the first order mathematical truths, which are, however, only truths of definition. These definitions rest upon simple, but abstract, suppositions, and all truths in this category are only constructed, but abstract, consequences of these definitions ... Physical truths, to the contrary, are in no way arbitrary, and do not depend on us.
'Premier Discours: De la Manière d'Étudier et de Traiter l'Histoire naturelle', Histoire Naturelle, Generale et Particulière, Avec la Description du Cabinet du Roi (1749), Vol. I, 53-4. Trans. Phillip R. Sloan.
There are things out there that are very simple and you never think would work. … Wikipedia is one of those that it would never occur to me that something like that would work. … But it does work. … People who have taken fairly simple ideas, … at a certain scale and after they gain a certain amount of momentum, they can really take off and work. And that’s really an amazing thing.
Guest Lecture, UC Berkeley, 'Search Engines, Technology, and Business' (3 Oct 2005). At 1:13 in the YouTube video.
There are, I believe, very few maxims in philosophy that have laid firmer hold upon the mind, than that air, meaning atmospherical air (free from various foreign matters, which were always supposed to be dissolved, and intermixed with it) is a simple elementary substance, indestructible, and unalterable, at least as much so as water is supposed to be. In the course of my enquiries, I was, however, soon satisfied that atmospherical air is not an unalterable thing; for that the phlogiston with which it becomes loaded from bodies burning in it, and animals breathing it, and various other chemical processes, so far alters and depraves it, as to render it altogether unfit for inflammation, respiration, and other purposes to which it is subservient; and I had discovered that agitation in water, the process of vegetation, and probably other natural processes, by taking out the superfluous phlogiston, restore it to its original purity.
'On Dephlogisticated Air, and the Constitution of the Atmosphere', in The Discovery of Oxygen, Part I, Experiments by Joseph Priestley 1775 (Alembic Club Reprint, 1894), 6.
There can be no doubt that science is in many ways the natural enemy of language. Language, either literary or colloquial, demands a rich store of living and vivid words—words that are “thoughtpictures,” and appeal to the senses, and also embody our feelings about the objects they describe. But science cares nothing about emotion or vivid presentation; her ideal is a kind of algebraic notation, to be used simply as an instrument of analysis; and for this she rightly prefers dry and abstract terms, taken from some dead language, and deprived of all life and personality.
In The English Language (1912), 124-125.
There could not be a language more universal and more simple, more exempt from errors and obscurities, that is to say, more worthy of expressing the invariable relations of natural objects. Considered from this point of view, it is coextensive with nature itself; it defines all the sensible relations, measures the times, the spaces, the forces, the temperatures; this difficult science is formed slowly, but it retains all the principles it has once acquired. It grows and becomes more certain without limit in the midst of so many errors of the human mind.
From Théorie Analytique de la Chaleur, Discours Préliminaire (Theory of Heat, Introduction), quoted as translated in F.R. Moulton, 'The Influence of Astronomy on Mathematics', Science (10 Mar 1911), N.S. Vol. 33, No. 845, 359.
There is synthesis when, in combining therein judgments that are made known to us from simpler relations, one deduces judgments from them relative to more complicated relations.
There is analysis when from a complicated truth one deduces more simple truths.
There is analysis when from a complicated truth one deduces more simple truths.
In James R. Hofmann, André-Marie Ampère (1996), 158. Cites Académie des Sciences Ampère Archives, lecture notes, box 261.
There is always a well-known solution to every human problem—neat, plausible, and wrong.
As given in 'The Divine Afflatus', Prejudices: Second Series (1920), 157. Various paraphrases changing Mencken’s original words, are also found. For example, “…an easy solution…” or “Every complex problem has a solution that is simple, neat, and wrong.” Also sometimes seen both paraphrased and misattributed, for example, to Thomas Huxley. [See further discussion on quoteinvestigator.com website.]
There is another approach to the extraterrestrial hypothesis of UFO origins. This assessment depends on a large number of factors about which we know little, and a few about which we know literally nothing. I want to make some crude numerical estimate of the probability that we are frequently visited by extraterrestrial beings.
Now, there is a range of hypotheses that can be examined in such a way. Let me give a simple example: Consider the Santa Claus hypothesis, which maintains that, in a period of eight hours or so on December 24-25 of each year, an outsized elf visits one hundred million homes in the United States. This is an interesting and widely discussed hypothesis. Some strong emotions ride on it, and it is argued that at least it does no harm.
We can do some calculations. Suppose that the elf in question spends one second per house. This isn't quite the usual picture—“Ho, Ho, Ho,” and so on—but imagine that he is terribly efficient and very speedy; that would explain why nobody ever sees him very much-only one second per house, after all. With a hundred million houses he has to spend three years just filling stockings. I have assumed he spends no time at all in going from house to house. Even with relativistic reindeer, the time spent in a hundred million houses is three years and not eight hours. This is an example of hypothesis-testing independent of reindeer propulsion mechanisms or debates on the origins of elves. We examine the hypothesis itself, making very straightforward assumptions, and derive a result inconsistent with the hypothesis by many orders of magnitude. We would then suggest that the hypothesis is untenable.
We can make a similar examination, but with greater uncertainty, of the extraterrestrial hypothesis that holds that a wide range of UFOs viewed on the planet Earth are space vehicles from planets of other stars.
Now, there is a range of hypotheses that can be examined in such a way. Let me give a simple example: Consider the Santa Claus hypothesis, which maintains that, in a period of eight hours or so on December 24-25 of each year, an outsized elf visits one hundred million homes in the United States. This is an interesting and widely discussed hypothesis. Some strong emotions ride on it, and it is argued that at least it does no harm.
We can do some calculations. Suppose that the elf in question spends one second per house. This isn't quite the usual picture—“Ho, Ho, Ho,” and so on—but imagine that he is terribly efficient and very speedy; that would explain why nobody ever sees him very much-only one second per house, after all. With a hundred million houses he has to spend three years just filling stockings. I have assumed he spends no time at all in going from house to house. Even with relativistic reindeer, the time spent in a hundred million houses is three years and not eight hours. This is an example of hypothesis-testing independent of reindeer propulsion mechanisms or debates on the origins of elves. We examine the hypothesis itself, making very straightforward assumptions, and derive a result inconsistent with the hypothesis by many orders of magnitude. We would then suggest that the hypothesis is untenable.
We can make a similar examination, but with greater uncertainty, of the extraterrestrial hypothesis that holds that a wide range of UFOs viewed on the planet Earth are space vehicles from planets of other stars.
The Cosmic Connection: An Extraterrestrial Perspective (1973), 200.
There is inherent in nature a hidden harmony that reflects itself in our minds under the image of simple mathematical laws. That then is the reason why events in nature are predictable by a combination of observation and mathematical analysis. Again and again in the history of physics this conviction, or should I say this dream, of harmony in nature has found fulfillments beyond our expectations.
…...
There is no art so difficult as the art of observation: it requires a skillful, sober spirit and a well-trained experience, which can only be acquired by practice; for he is not an observer who only sees the thing before him with his eyes, but he who sees of what parts the thing consists, and in what connexion the parts stand to the whole. One person overlooks half from inattention; another relates more than he sees while he confounds it with that which he figures to himself; another sees the parts of the whole, but he throws things together that ought to be separated. ... When the observer has ascertained the foundation of a phenomenon, and he is able to associate its conditions, he then proves while he endeavours to produce the phenomena at his will, the correctness of his observations by experiment. To make a series of experiments is often to decompose an opinion into its individual parts, and to prove it by a sensible phenomenon. The naturalist makes experiments in order to exhibit a phenomenon in all its different parts. When he is able to show of a series of phenomena, that they are all operations of the same cause, he arrives at a simple expression of their significance, which, in this case, is called a Law of Nature. We speak of a simple property as a Law of Nature when it serves for the explanation of one or more natural phenomena.
'The Study of the Natural Sciences: An Introductory Lecture to the Course of Experimental Chemistry in the University of Munich, for the Winter Session of 1852-53,' as translated and republished in The Medical Times and Gazette (22 Jan 1853), N.S. Vol. 6, 82.
There is no foundation in geological facts, for the popular theory of the successive development of the animal and vegetable world, from the simplest to the most perfect forms.
Principles of Geology (1830-3), Vol. 1, 153.
There is no sharp boundary line separating the reactions of the immune bodies from chemical processes between crystalloids, just as in nature there exists every stage between crystalloid and colloid. The nearer the colloid particle approximates to the normal electrolyte, the nearer its compounds must obviously come to conforming to the law of simple stoichiometric proportions, and the compounds themselves to simple chemical compounds. At this point, it should be recalled that Arrhenius has shown that the quantitative relationship between toxin and antitoxin is very similar to that between acid and base.
Landsteiner and Nicholas von Jagic, 'Uber Reaktionen anorganischer Kolloide und Immunkorper', Münchener medizinischer Wochenschrift (1904), 51, 1185-1189. Trans. Pauline M. H. Mazumdar.
There was, I think, a feeling that the best science was that done in the simplest way. In experimental work, as in mathematics, there was “style” and a result obtained with simple equipment was more elegant than one obtained with complicated apparatus, just as a mathematical proof derived neatly was better than one involving laborious calculations. Rutherford's first disintegration experiment, and Chadwick's discovery of the neutron had a “style” that is different from that of experiments made with giant accelerators.
From 'Physics in a University Laboratory Before and After World War II', Proceedings of the Royal Society of London, Series A, (1975), 342, 463. As cited in Alan McComas, Galvani's Spark: The Story of the Nerve Impulse (2011), 107.
These creators, makers of the new, can never become obsolete, for in the arts there is no correct answer. The story of discoverers could be told in simple chronological order, since the latest science replaces what went before. But the arts are another story—a story of infinite addition. We must find order in the random flexings of the imagination.
In The Creators: A History of Heroes of the Imagination (1992), xv.
These estimates may well be enhanced by one from F. Klein (1849-1925), the leading German mathematician of the last quarter of the nineteenth century. “Mathematics in general is fundamentally the science of self-evident things.” ... If mathematics is indeed the science of self-evident things, mathematicians are a phenomenally stupid lot to waste the tons of good paper they do in proving the fact. Mathematics is abstract and it is hard, and any assertion that it is simple is true only in a severely technical sense—that of the modern postulational method which, as a matter of fact, was exploited by Euclid. The assumptions from which mathematics starts are simple; the rest is not.
Mathematics: Queen and Servant of Science (1952),19-20.
These were errors in thinking which caused me two years of hard work before at last, in 1915, I recognised them as such. … The final results appear almost simple; any intelligent undergraduate can understand them without much trouble. But the years of searching in the dark for a truth that one feels, but cannot express; the intense desire and the alternations of confidence and misgiving, until one breaks through to clarity and understanding, are only known to him who has himself experienced them.
From address, 'Notes on the Origin of the General Theory of Relativity', part of the collection of essays in Mein Weltbild (1934), translated from the original German. This translation as quoted in W.I.B. Beveridge, The Art of Scientific Investigation (1957), 60. Another translation is given on this web page, beginning: “These were errors of thought…”.
This law [of gravitation] has been called “the greatest generalization achieved by the human mind”. … I am interested not so much in the human mind as in the marvel of a nature which can obey such an elegant and simple law as this law of gravitation. Therefore our main concentration will not be on how clever we are to have found it all out, but on how clever nature is to pay attention to it.
In The Character of Physical Law (1965), 14.
This leads us to ask for the reasons which call for this new theory of transmutation. The beginning of things must needs lie in obscurity, beyond the bounds of proof, though within those of conjecture or of analogical inference. Why not hold fast to the customary view, that all species were directly, instead of indirectly, created after their respective kinds, as we now behold them,--and that in a manner which, passing our comprehension, we intuitively refer to the supernatural? Why this continual striving after “the unattained and dim,”—these anxious endeavors, especially of late years, by naturalists and philosophers of various schools and different tendencies, to penetrate what one of them calls “the mystery of mysteries,” the origin of species? To this, in general, sufficient answer may be found in the activity of the human intellect, “the delirious yet divine desire to know,” stimulated as it has been by its own success in unveiling the laws and processes of inorganic Nature,—in the fact that the principal triumphs of our age in physical science have consisted in tracing connections where none were known before, in reducing heterogeneous phenomena to a common cause or origin, in a manner quite analogous to that of the reduction of supposed independently originated species to a common ultimate origin,—thus, and in various other ways, largely and legitimately extending the domain of secondary causes. Surely the scientific mind of an age which contemplates the solar system as evolved from a common, revolving, fluid mass,— which, through experimental research, has come to regard light, heat, electricity, magnetism, chemical affinity, and mechanical power as varieties or derivative and convertible forms of one force, instead of independent species,—which has brought the so-called elementary kinds of matter, such as the metals, into kindred groups, and raised the question, whether the members of each group may not be mere varieties of one species,—and which speculates steadily in the direction of the ultimate unity of matter, of a sort of prototype or simple element which may be to the ordinary species of matter what the protozoa or component cells of an organism are to the higher sorts of animals and plants,—the mind of such an age cannot be expected to let the old belief about species pass unquestioned.
— Asa Gray
'Darwin on the Origin of Species', The Atlantic Monthly (Jul 1860), 112-3. Also in 'Natural Selection Not Inconsistent With Natural Theology', Darwiniana: Essays and Reviews Pertaining to Darwinism (1876), 94-95.
This missing science of heredity, this unworked mine of knowledge on the borderland of biology and anthropology, which for all practical purposes is as unworked now as it was in the days of Plato, is, in simple truth, ten times more important to humanity than all the chemistry and physics, all the technical and indsutrial science that ever has been or ever will be discovered.
Mankind in the Making (1903), 72.
This science, Geometry, is one of indispensable use and constant reference, for every student of the laws of nature; for the relations of space and number are the alphabet in which those laws are written. But besides the interest and importance of this kind which geometry possesses, it has a great and peculiar value for all who wish to understand the foundations of human knowledge, and the methods by which it is acquired. For the student of geometry acquires, with a degree of insight and clearness which the unmathematical reader can but feebly imagine, a conviction that there are necessary truths, many of them of a very complex and striking character; and that a few of the most simple and self-evident truths which it is possible for the mind of man to apprehend, may, by systematic deduction, lead to the most remote and unexpected results.
In The Philosophy of the Inductive Sciences Part 1, Bk. 2, chap. 4, sect. 8 (1868).
Those who have taken upon them to lay down the law of nature as a thing already searched out and understood, whether they have spoken in simple assurance or professional affectation, have therein done philosophy and the sciences great injury. For as they have been successful in inducing belief, so they have been effective in quenching and stopping inquiry; and have done more harm by spoiling and putting an end to other men's efforts than good by their own. Those on the other hand who have taken a contrary course, and asserted that absolutely nothing can be known — whether it were from hatred of the ancient sophists, or from uncertainty and fluctuation of mind, or even from a kind of fullness of learning, that they fell upon this opinion — have certainly advanced reasons for it that are not to be despised; but yet they have neither started from true principles nor rested in the just conclusion, zeal and affectation having carried them much too far...
Now my method, though hard to practice, is easy to explain; and it is this. I propose to establish progressive stages of certainty. The evidence of the sense, helped and guarded by a certain process of correction, I retain. But the mental operation which follows the act of sense I for the most part reject; and instead of it I open and lay out a new and certain path for the mind to proceed in, starting directly from the simple sensuous perception.
Now my method, though hard to practice, is easy to explain; and it is this. I propose to establish progressive stages of certainty. The evidence of the sense, helped and guarded by a certain process of correction, I retain. But the mental operation which follows the act of sense I for the most part reject; and instead of it I open and lay out a new and certain path for the mind to proceed in, starting directly from the simple sensuous perception.
Novum Organum (1620)
Though human ingenuity may make various inventions which, by the help of various machines answering the same end, it will never devise any inventions more beautiful, nor more simple, nor more to the purpose than Nature does; because in her inventions nothing is wanting, and nothing is superfluous, and she needs no counterpoise when she makes limbs proper for motion in the bodies of animals.
W. An. IV. 184a (7). Translated by Jean Paul Richter, in 'Physiology', The Literary Works of Leonardo da Vinci: Compiled and Edited from the Original Manuscripts (1883), Vol. 2, 126, selection 837.
Three passions, simple but overwhelmingly strong, have governed my life: the longing for love, the search for knowledge, and unbearable pity for the suffering of mankind.
The Autobiography of Betrand Russell (1998), 9, first sentence of the Prologue.
Time is defined so that motion looks simple.
In Gravitation (1973).
To arrive at the simplest truth, as Newton knew and practiced, requires years of contemplation. Not activity Not reasoning. Not calculating. Not busy behaviour of any kind. Not reading. Not talking. Not making an effort. Not thinking. Simply bearing in mind what it is one needs to know. And yet those with the courage to tread this path to real discovery are not only offered practically no guidance on how to do so, they are actively discouraged and have to set about it in secret, pretending meanwhile to be diligently engaged in the frantic diversions and to conform with the deadening personal opinions which are continually being thrust upon them.
In 'Appendix 1', The Laws of Form (1969), 110.
To me the hypothesis of a Creator is no less simple than the hypothesis of no Creator…
In Letter to periodical, Chemistry and Industry (17 Feb 1997).
To pick a hole–say in the 2nd law of Ωcs, that if two things are in contact the hotter cannot take heat from the colder without external agency.
Now let A & B be two vessels divided by a diaphragm and let them contain elastic molecules in a state of agitation which strike each other and the sides. Let the number of particles be equal in A & B but let those in A have equal velocities, if oblique collisions occur between them their velocities will become unequal & I have shown that there will be velocities of all magnitudes in A and the same in B only the sum of the squares of the velocities is greater in A than in B.
When a molecule is reflected from the fixed diaphragm CD no work is lost or gained.
If the molecule instead of being reflected were allowed to go through a hole in CD no work would be lost or gained, only its energy would be transferred from the one vessel to the other.
Now conceive a finite being who knows the paths and velocities of all the molecules by simple inspection but who can do no work, except to open and close a hole in the diaphragm, by means of a slide without mass.
Let him first observe the molecules in A and when lie sees one coming the square of whose velocity is less than the mean sq. vel. of the molecules in B let him open a hole & let it go into B. Next let him watch for a molecule in B the square of whose velocity is greater than the mean sq. vel. in A and when it comes to the hole let him draw and slide & let it go into A, keeping the slide shut for all other molecules.
Then the number of molecules in A & B are the same as at first but the energy in A is increased and that in B diminished that is the hot system has got hotter and the cold colder & yet no work has been done, only the intelligence of a very observant and neat fingered being has been employed. Or in short if heat is the motion of finite portions of matter and if we can apply tools to such portions of matter so as to deal with them separately then we can take advantage of the different motion of different portions to restore a uniformly hot system to unequal temperatures or to motions of large masses. Only we can't, not being clever enough.
Now let A & B be two vessels divided by a diaphragm and let them contain elastic molecules in a state of agitation which strike each other and the sides. Let the number of particles be equal in A & B but let those in A have equal velocities, if oblique collisions occur between them their velocities will become unequal & I have shown that there will be velocities of all magnitudes in A and the same in B only the sum of the squares of the velocities is greater in A than in B.
When a molecule is reflected from the fixed diaphragm CD no work is lost or gained.
If the molecule instead of being reflected were allowed to go through a hole in CD no work would be lost or gained, only its energy would be transferred from the one vessel to the other.
Now conceive a finite being who knows the paths and velocities of all the molecules by simple inspection but who can do no work, except to open and close a hole in the diaphragm, by means of a slide without mass.
Let him first observe the molecules in A and when lie sees one coming the square of whose velocity is less than the mean sq. vel. of the molecules in B let him open a hole & let it go into B. Next let him watch for a molecule in B the square of whose velocity is greater than the mean sq. vel. in A and when it comes to the hole let him draw and slide & let it go into A, keeping the slide shut for all other molecules.
Then the number of molecules in A & B are the same as at first but the energy in A is increased and that in B diminished that is the hot system has got hotter and the cold colder & yet no work has been done, only the intelligence of a very observant and neat fingered being has been employed. Or in short if heat is the motion of finite portions of matter and if we can apply tools to such portions of matter so as to deal with them separately then we can take advantage of the different motion of different portions to restore a uniformly hot system to unequal temperatures or to motions of large masses. Only we can't, not being clever enough.
Letter to Peter Guthrie Tait (11 Dec 1867). In P. M. Harman (ed.), The Scientific Letters and Papers of James Clerk Maxwell (1995), Vol. 2, 331-2.
To solve a problem means to reduce it to something simpler than itself.
In 'On Groups', Prelude to Mathematics (1955), 203.
To suppose that the eye, with all its inimitable contrivances for adjusting the focus to different distances, for admitting different amounts of light, and for the correction of spherical and chromatic aberration, could have been formed by natural selection, seems, I freely confess, absurd in the highest possible degree. When it was first said that the sun stood still and the world turned round, the common sense of mankind declared the doctrine false; but the old saying of Vox populi, vox Dei, as every philosopher knows, cannot be trusted in science. Reason tells me, that if numerous gradations from a perfect and complex eye to one very imperfect and simple, each grade being useful to its possessor, can be shown to exist; if further, the eye does vary ever so slightly, and the variations be inherited, which is certainly the case; and if any variation or modification in the organ be ever useful to an animal under changing conditions of life, then the difficulty of believing that a perfect and complex eye could be formed by natural selection, though insuperable by our imagination, can hardly be considered real.
On The Origin of Species by Means of Natural Selection (1859, 1882), 143-144.
To-day, science has withdrawn into realms that are hardly understanded of the people. Biology means very largely histology, the study of the cell by difficult and elaborate microscopical processes. Chemistry has passed from the mixing of simple substances with ascertained reactions, to an experimentation of these processes under varying conditions of temperature, pressure, and electrification—all requiring complicated apparatus and the most delicate measurement and manipulation. Similarly, physics has outgrown the old formulas of gravity, magnetism, and pressure; has discarded the molecule and atom for the ion, and may in its recent generalizations be followed only by an expert in the higher, not to say the transcendental mathematics.
‘Exit the Amateur Scientist.’ Editorial, The Nation, 23 August 1906, 83, 160.
Undeterred by poverty, failure, domestic tragedy, and persecution, but sustained by his mystical belief in an attainable mathematical harmony and perfection of nature, Kepler persisted for fifteen years before finding the simple regularity [of planetary orbits] he sought… . What stimulated Kepler to keep slaving all those fifteen years? An utter absurdity. In addition to his faith in the mathematical perfectibility of astronomy, Kepler also believed wholeheartedly in astrology. This was nothing against him. For a scientist of Kepler’s generation astrology was as respectable scientifically and mathematically as the quantum theory or relativity is to theoretical physicists today. Nonsense now, astrology was not nonsense in the sixteenth century.
In The Handmaiden of the Sciences (1937), 30.
Unity of plan everywhere lies hidden under the mask: of diversity of structure—the complex is everywhere evolved out of the simple.
From lecture, 'A Lobster; or, the Study of Zoology' (1861). In Collected Essays (1894). Vol. 8, 205-6.
Very simple was my explanation, and plausible enough—as most wrong theories are!
In The Time Machine (1898), 77.
We are living in an age of awesome agricultural enterprise that needs to be interpreted. We find our simple faith in science dominated by the Religion of PhDeism under the reign of Data; so narrow in people and often so meaningless in context as to be worthless to the scientific farmer.
Letter to Joshua Lederberg (19 Apr 1970), Joshua Lederberg papers, National Library of Medicine (online). Hildebrand was a response to a Lederberg's letter published in the Washington Post (18 Apr 1970) about 'Ecology Has All Requisites of an Authentic Religion.' Note that Sam Murchid claimed this term PhDeism in another context in his diaries (as seen in diaries of 1964 and others).
We come back then to our records of nervous messages with a reasonable assurance that they do tell us what the message is like. It is a succession of brief waves of surface breakdown, each allowing a momentary leakage of ions from the nerve fibre. The waves can be set up so that they follow one another in rapid or in slow succession, and this is the only form of gradation of which the message is capable. Essentially the same kind of activity is found in all sorts of nerve fibres from all sorts of animals and there is no evidence to suggest that any other kind of nervous transmission is possible. In fact we may conclude that the electrical method can tell us how the nerve fibre carries out its function as the conducting unit of the nervous system, and that it does so by reactions of a fairly simple type.
The Mechanism of Nervous Action (1932), 21.
We come therefore now to that knowledge whereunto the ancient oracle directeth us, which is the knowledge of ourselves; which deserveth the more accurate handling, by how much it toucheth us more nearly. This knowledge, as it is the end and term of natural philosophy in the intention of man, so notwithstanding it is but a portion of natural philosophy in the continent of nature. And generally let this be a rule, that all partitions of knowledges be accepted rather for lines and veins, than for sections and separations; and that the continuance and entireness of knowledge be preserved. For the contrary hereof hath made particular sciences to become barren, shallow, and erroneous; while they have not been nourished and maintained from the common fountain. So we see Cicero the orator complained of Socrates and his school, that he was the first that separated philosophy and rhetoric; whereupon rhetoric became an empty and verbal art. So we may see that the opinion of Copernicus touching the rotation of the earth, which astronomy itself cannot correct because it is not repugnant to any of the phenomena, yet natural philosophy may correct. So we see also that the science of medicine, if it be destituted and forsaken by natural philosophy, it is not much better than an empirical practice. With this reservation therefore we proceed to Human Philosophy or Humanity, which hath two parts: the one considereth man segregate, or distributively; the other congregate, or in society. So as Human Philosophy is either Simple and Particular, or Conjugate and Civil. Humanity Particular consisteth of the same parts whereof man consisteth; that is, of knowledges that respect the Body, and of knowledges that respect the Mind. But before we distribute so far, it is good to constitute. For I do take the consideration in general and at large of Human Nature to be fit to be emancipate and made a knowledge by itself; not so much in regard of those delightful and elegant discourses which have been made of the dignity of man, of his miseries, of his state and life, and the like adjuncts of his common and undivided nature; but chiefly in regard of the knowledge concerning the sympathies and concordances between the mind and body, which, being mixed, cannot be properly assigned to the sciences of either.
The Advancement of Learning (1605) in James Spedding, Robert Ellis and Douglas Heath (eds.), The Works of Francis Bacon (1887-1901), Vol. 3, 366-7.
We have hitherto considered those Ideas, in the reception whereof, the Mind is only passive, which are those simple ones received from Sensation and Reflection before-mentioned, whereof the Mind cannot make anyone to it self, nor have any Idea which does not wholy consist of them. But as these simple Ideas are observed to exist in several Combinations united together; so the Mind has a power to consider several of them united together, as one Idea; and that not only as they are united in external Objects, but as it self has joined them. Ideas thus made up of several simple ones put together, I call Complex; such as are Beauty, Gratitude, a Man, an Army, the Universe; which tough complicated various simple Ideas, made up of simple ones, yet are, when the Mind pleases, considered each by if self, as one entire thing, and signified by one name.
An Essay Concerning Human Understanding (1690). Edited by Peter Nidditch (1975), Book 2, Chapter 12, Section 1, 163-4.
We have taken to the Moon the wealth of this nation,
the vision of its political leaders,
the intelligence of its scientists,
the dedication of its engineers,
the careful craftsmanship of its workers,
and the enthusiastic support of its people.
We have brought back rocks, and I think it is a fair trade . . .
Man has always gone where he has been able to go. It’s that simple.
He will continue pushing back his frontier,
no matter how far it may carry him from his homeland.
the vision of its political leaders,
the intelligence of its scientists,
the dedication of its engineers,
the careful craftsmanship of its workers,
and the enthusiastic support of its people.
We have brought back rocks, and I think it is a fair trade . . .
Man has always gone where he has been able to go. It’s that simple.
He will continue pushing back his frontier,
no matter how far it may carry him from his homeland.
…...
We hope to explain the universe in a single, simple formula that you can wear on your T-shirt.
In 'Quark City' by Richard Wolkomir, Omni, February 1984.
We love to discover in the cosmos the geometrical forms that exist in the depths of our consciousness. The exactitude of the proportions of our monuments and the precision of our machines express a fundamental character of our mind. Geometry does not exist in the earthly world. It has originated in ourselves. The methods of nature are never so precise as those of man. We do not find in the universe the clearness and accuracy of our thought. We attempt, therefore, to abstract from the complexity of phenomena some simple systems whose components bear to one another certain relations susceptible of being described mathematically.
In Man the Unknown (1935), 8.
We may need simple and heroic legends for that peculiar genre of literature known as the textbook. But historians must also labor to rescue human beings from their legends in science–if only so that we may understand the process of scientific thought aright.
…...
We may regard geometry as a practical logic, for the truths which it considers, being the most simple and most sensible of all, are, for this reason, the most susceptible to easy and ready application of the rules of reasoning.
From 'De la Géométrie', Pensées de Monsieur d’Alembert (1774), 137. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 203. From the original French, “On peut regarder la géométrie comme une logique pratique, parce que les vérités dont elle s'occupe, étant les plus simples et les plus sensibles de toutes, sont par cette raison, les plus susceptibles d'une application facile et palpable des règles du raisonnement.”
We must examine the moral alchemy through which the in-group readily transmutes virtue into vice and vice into virtue, as the occasion may demand. … We begin with the engagingly simple formula of moral alchemy: the same behavior must be differently evaluated according to the person who exhibits it. For example, the proficient alchemist will at once know that the word “firm” is properly declined as follows:
I am firm,
Thou art obstinate,
He is pig-headed.
There are some, unversed in the skills of this science, who will tell you that one and the same term should be applied to all three instances of identical behavior.
I am firm,
Thou art obstinate,
He is pig-headed.
There are some, unversed in the skills of this science, who will tell you that one and the same term should be applied to all three instances of identical behavior.
In article, 'The Self-Fulfilling Prophecy', The Antioch Review (Summer 1948), 8, No. 2, 195-196. Included as Chap. 7 of Social Theory and Social Structure (1949), 201.
We must not forget … that “influence” is not a simple, but on the contrary, a very complex, bilateral relation. We are not influenced by everything we read or learn. In one sense, and perhaps the deepest, we ourselves determine the influences we are submitting to; our intellectual ancestors are by no means given to, but are freely chosen by, us.
In From the Closed World to the Infinite Universe (1957) 5-6.
We must not only prepare [students] in sciences, we must prepare them in other areas. For example, I teach Chemistry but on every test I give I have an English question. And I give a simple question. I say, “Discuss your understanding of this topic.”
In address, to the Economic Club of Detroit (14 Jan 1990), 'Where Do We Go From Here?' on the massiechairs.com website.
We see only the simple motion of descent, since that other circular one common to the Earth, the tower, and ourselves remains imperceptible. There remains perceptible to us only that of the stone, which is not shared by us; and, because of this, sense shows it as by a straight line, always parallel to the tower, which is built upright and perpendicular upon the terrestrial surface.
Dialogue on the Great World Systems (1632). Revised and Annotated by Giorgio De Santillana (1953), 177.
We should make things as simple as possible, but not simpler.
…...
We suppose ... that the constituent molecules of any simple gas whatever (i.e., the molecules which are at such a distance from each other that they cannot exercise their mutual action) are not formed of a solitary elementary molecule, but are made up of a certain number of these molecules united by attraction to form a single one.
'Essay on a Manner of Determining the Relative Masses of the Elementary Molecules of Bodies, and the Proportions in which they enter into these Compounds', Journal de Physique, 1811, 73, 58-76. In Foundations of the Molecular Theory: Alembic Club Reprints, Number 4 (1923), 31.
What attracted me to immunology was that the whole thing seemed to revolve around a very simple experiment: take two different antibody molecules and compare their primary sequences. The secret of antibody diversity would emerge from that. Fortunately at the time I was sufficiently ignorant of the subject not to realise how naive I was being.
From Nobel Lecture (8 Dec 1984), collected in Tore Frängsmyr and Jan Lindsten (eds.), Nobel Lectures in Physiology Or Medicine: 1981-1990 (1993), 248.
What is science? We have all this mess around us. Things are totally incomprehensible. And then eventually we find simple laws, simple formulas. In a way, a very simple formula, Newton’s Law, which is just also a few symbols, can by hard work explain the motion of the planets around the sun and many, many other things to the 50th decimal. It’s marvellous: a very simple formula explains all these very complicated things
As quoted in Nigel Lesmoir-Gordon, 'Benoît Mandelbrot Obituary', The Guardian (17 Oct 2010).
What we usually consider as impossible are simply engineering problems … there’s no law of physics preventing them.
As quoted in Alok Ajh, 'Science Weekly with Michio Kaku: Impossibility is Relative' (15 Jun 2009), on website of The Guardian.
Whatever advantage can be attributed to logic in directing and strengthening the action of the understanding is found in a higher degree in mathematical study, with the immense added advantage of a determinate subject, distinctly circumscribed, admitting of the utmost precision, and free from the danger which is inherent in all abstract logic—of leading to useless and puerile rules, or to vain ontological speculations. The positive method, being everywhere identical, is as much at home in the art of reasoning as anywhere else: and this is why no science, whether biology or any other, can offer any kind of reasoning, of which mathematics does not supply a simpler and purer counterpart. Thus, we are enabled to eliminate the only remaining portion of the old philosophy which could even appear to offer any real utility; the logical part, the value of which is irrevocably absorbed by mathematical science.
In Auguste Comte and Harriet Martineau (trans.), Positive Philosophy (1858), Vol. 1, 326-327.
Whatever the subject of any investigation may be, whether poetry, biology, ethics or torpedo warfare, the same scientific method of procedure must be followed. We must first unravel the complex and heterogeneous back to first principles, and then reason forward from the simple to the complex, from the homogeneous to the heterogeneous, from what we know to what we would learn. Such are the methods pursued by all successful inventors, scientific investigators and discoverers.
In The Science of Poetry and the Philosophy of Language (1910), ix.
Wheeler’s First Moral Principle: Never make a calculation until you know the answer. Make an estimate before every calculation, try a simple physical argument (symmetry! invariance! conservation!) before every derivation, guess the answer to every paradox and puzzle. Courage: No one else needs to know what the guess is. Therefore make it quickly, by instinct. A right guess reinforces this instinct. A wrong guess brings the refreshment of surprise. In either case life as a spacetime expert, however long, is more fun!
In E.F. Taylor and J.A. Wheeler, Spacetime Physics (1992), 20.
When Cayley had reached his most advanced generalizations he proceeded to establish them directly by some method or other, though he seldom gave the clue by which they had first been obtained: a proceeding which does not tend to make his papers easy reading. …
His literary style is direct, simple and clear. His legal training had an influence, not merely upon his mode of arrangement but also upon his expression; the result is that his papers are severe and present a curious contrast to the luxuriant enthusiasm which pervades so many of Sylvester’s papers. He used to prepare his work for publication as soon as he carried his investigations in any subject far enough for his immediate purpose. … A paper once written out was promptly sent for publication; this practice he maintained throughout life. … The consequence is that he has left few arrears of unfinished or unpublished papers; his work has been given by himself to the world.
His literary style is direct, simple and clear. His legal training had an influence, not merely upon his mode of arrangement but also upon his expression; the result is that his papers are severe and present a curious contrast to the luxuriant enthusiasm which pervades so many of Sylvester’s papers. He used to prepare his work for publication as soon as he carried his investigations in any subject far enough for his immediate purpose. … A paper once written out was promptly sent for publication; this practice he maintained throughout life. … The consequence is that he has left few arrears of unfinished or unpublished papers; his work has been given by himself to the world.
In Proceedings of London Royal Society (1895), 58, 23-24.
When I behold a fashionable table set out in all its magnificence, I fancy that I see gouts and dropsies, fevers and lethargies, with other innumerable distempers lying in ambuscade among the dishes. Nature delights in the most plain and simple diet. Every animal but man keeps to one dish. Herbs are the food of this species, fish of that, and flesh of a third. Man falls upon everything that comes in his way; not the smallest fruit or excrescence of the earth, scarce a berry or a mushroom can escape him.
Spectator, No. 195. In Samuel Austin Allibone, Prose Quotations from Socrates to Macaulay (1880), 363.
When it comes to understanding the planet’s blue [ocean] frontier, one of the largest challenges we face can be encapsulated by a simple phrase: Out of sight, out of mind.
huffingtonpost.com/philippe-cousteau/ocean-oases-protecting-ca_b_873016.html
When puzzled, it never hurts to read the primary documents–a rather simple and self-evident principle that has, nonetheless, completely disappeared from large sectors of the American experience.
…...
When someone says “I am thinking, therefore I am, or I exist,” he does not deduce existence from thought by means of a syllogism, but recognises it as something self-evident by a simple intuition of the mind. This is clear from the fact that if he were deducing it by means of a syllogism, he would have to have had previous knowledge of the major premiss 'Everything which thinks is, or exists'; yet in fact he learns it from experiencing in his own case that it is impossible that he should think without existing. It is in the nature of our mind to construct general propositions on the basis of our knowledge of particular ones.
Author's Replies to the Second set of Objections to Meditations on the First Philosophy (1641), in The Philosophical Writings of Descartes (1985), trans. J. Cottingham, R. Stoothoff and D. Murdoch, Vol. 2, 100.
When the solution is simple, God is answering. Where the world ceases to be the scene of our personal hopes and wishes, where we face it as free beings admiring, asking and observing, there we enter the realm of Art and Science.
…...
Whenever there is a simple error that most laymen fall for, there is always a slightly more sophisticated version of the same problem that experts fall for.
As quoted in Brooks Jackson and Kathleen Hall Jamieson, unSpun: Finding Facts in a World of Disinformation (2007), 70-71.
While DNA could be claimed to be both simple and elegant, it must be remembered that DNA almost certainly originated fairly close to the origin of life when things were necessarily simple or they would not have got going.
In What Mad Pursuit: A Personal View of Scientific Discovery (1988), 138.
Why does this magnificent applied science which saves work and makes life easier bring us so little happiness? … The simple answer runs: “Because we have not yet learned to make sensible use of it.”
Address to students of the California Institute of Technology, Pasadena, California (16 Feb 1931). In New York Times (17 Feb 1931), p. 6.
Why it is that animals, instead of developing in a simple and straightforward way, undergo in the course of their growth a series of complicated changes, during which they often acquire organs which have no function, and which, after remaining visible for a short time, disappear without leaving a trace ... To the Darwinian, the explanation of such facts is obvious. The stage when the tadpole breathes by gills is a repetition of the stage when the ancestors of the frog had not advanced in the scale of development beyond a fish.
In The Works of Francis Maitland Balfour (1885), Vol. 1, 702.
William Blake called division the sin of man; Faraday was a great man because he was utterly undivided. His whole, very harmonious, very well balanced, … and used the brain in the limited
way in which it is useful…. [H]e built up his few but precious speculations. Their simplicity rivals with their forcefulness.
In 'The Scientific Grammar of Michael Faraday’s Diaries', Part I, 'The Classic of Science', A Classic and a Founder (1937), collected in Rosenstock-Huessy Papers (1981), Vol. 1, 7.
With the extension of mathematical knowledge will it not finally become impossible for the single investigator to embrace all departments of this knowledge? In answer let me point out how thoroughly it is ingrained in mathematical science that every real advance goes hand in hand with the invention of sharper tools and simpler methods which, at the same time, assist in understanding earlier theories and in casting aside some more complicated developments.
In 'Mathematical Problems', Lecture at the International Congress of Mathematics, Paris, (8 Aug 1900). Translated by Dr. Maby Winton Newson in Bulletin of the American Mathematical Society (1902), 8, 479. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 94-95. It is reprinted in Jeremy Gray, The Hilbert Challenge (2000), 282.
With the nervous system intact the reactions of the various parts of that system, the 'simple reflexes', are ever combined into great unitary harmonies, actions which in their sequence one upon another constitute in their continuity what may be termed the 'behaviour'.
The Integrative Action of the Nervous System (1906), 237.
Without any doubt, the regularity which astronomy shows us in the movements of the comets takes place in all phenomena. The trajectory of a simple molecule of air or vapour is regulated in a manner as certain as that of the planetary orbits; the only difference between them is that which is contributed by our ignorance. Probability is relative in part to this ignorance, and in part to our knowledge.
Philosophical Essay on Probabilities (1814), 5th edition (1825), trans. Andrew I. Dale (1995), 3.
Would not [an] uncluttered mind also see the attempts to reconcile science and religion by disparaging the reduction of the complex to the simple as attempts guided by muddle-headed sentiment and intellectually dishonest emotion?
Essay collected in John Cornwell (ed.), 'The Limitless Power of Science', Nature's Imagination: The Frontiers of Scientific Vision (1995), 123.
You can recognize truth by its beauty and simplicity. When you get it right, it is obvious that it is right—at least if you have any experience—because usually what happens is that more comes out than goes in. … The inexperienced, the crackpots, and people like that, make guesses that are simple, but you can immediately see that they are wrong, so that does not count. Others, the inexperienced students, make guesses that are very complicated, and it sort of looks as if it is all right, but I know it is not true because the truth always turns out to be simpler than you thought.
In The Character of Physical Law (1965), 171.
Zoocentrism is the primary fallacy of human sociobiology, for this view of human behavior rests on the argument that if the actions of ‘lower’ animals with simple nervous systems arise as genetic products of natural selection, then human behavior should have a similar basis.
…...


![James Watson quote: [Science moves] with the spirit of an adventure characterized both by youthful arrogance and by the belief t](https://todayinsci.com/W/Watson_James/WatsonJames-Pretty500x250px.jpg)

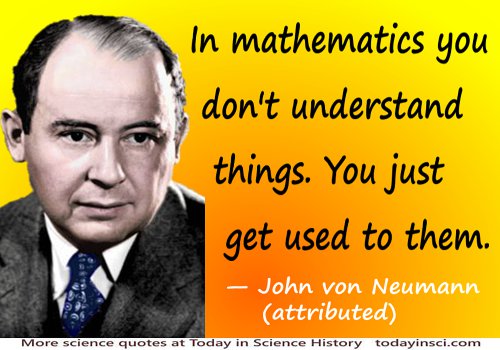















 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


