Prove Quotes (265 quotes)
Proved Quotes, Proving Quotes
Proved Quotes, Proving Quotes

… how the real proof should run. The main thing is the content, not the mathematics. With mathematics one can prove anything.
Commenting on a mistake in some work from Planck, for which “the result is correct but the proof is faulty.” As quoted in Denis Brian, Einstein—A Life (1996), 76.
… the truth is that the knowledge of external nature and of the sciences which that knowledge requires or includes, is not the great or the frequent business of the human mind. Whether we provide for action or conversation, whether we wish to be useful or pleasing, the first requisite is the religious and moral knowledge of right and wrong; the next is an acquaintance with the history of mankind, and with those examples which may be said to embody truth, and prove by events the reasonableness of opinions. Prudence and justice are virtues, and excellencies, of all times and of all places; we are perpetually moralists, but we are geometricians only by chance. Our intercourse with intellectual nature is necessary; our speculations upon matter are voluntary, and at leisure. Physical knowledge is of such rare emergence, that one man may know another half his life without being able to estimate his skill in hydrostatics or astronomy; but his moral and prudential character immediately appears.
In Lives of the Poets (1779-81).
… There can be no doubt about faith and not reason being the ultima ratio. Even Euclid, who has laid himself as little open to the charge of credulity as any writer who ever lived, cannot get beyond this. He has no demonstrable first premise. He requires postulates and axioms which transcend demonstration, and without which he can do nothing. His superstructure indeed is demonstration, but his ground his faith. Nor again can he get further than telling a man he is a fool if he persists in differing from him. He says “which is absurd,” and declines to discuss the matter further. Faith and authority, therefore, prove to be as necessary for him as for anyone else.
In The Way of All Flesh (1917), 319-320.
“Divide et impera” is as true in algebra as in statecraft; but no less true and even more fertile is the maxim “auge et impera”.The more to do or to prove, the easier the doing or the proof.
In 'Proof of the Fundamental Theorem of Invariants', Philosophic Magazine (1878), 186. In Collected Mathematical Papers, 3, 126. [The Latin phrases, “Divide/auge et impera” translate as “Divide/increase and rule”.
“I should have more faith,” he said; “I ought to know by this time that when a fact appears opposed to a long train of deductions it invariably proves to be capable of bearing some other interpretation.”
Spoken by character, Sherlock Holmes, in A Study in Scarlet (1887), in Works of Arthur Conan Doyle (1902), Vol. 11, 106.
[E.H.] Moore was presenting a paper on a highly technical topic to a large gathering of faculty and graduate students from all parts of the country. When half way through he discovered what seemed to be an error (though probably no one else in the room observed it). He stopped and re-examined the doubtful step for several minutes and then, convinced of the error, he abruptly dismissed the meeting—to the astonishment of most of the audience. It was an evidence of intellectual courage as well as honesty and doubtless won for him the supreme admiration of every person in the group—an admiration which was in no wise diminished, but rather increased, when at a later meeting he announced that after all he had been able to prove the step to be correct.
In Obituary, 'Eliakim Hastings Moore', The American Mathematical Monthly (Apr 1933), 40, 191.
[J.J.] Sylvester’s methods! He had none. “Three lectures will be delivered on a New Universal Algebra,” he would say; then, “The course must be extended to twelve.” It did last all the rest of that year. The following year the course was to be Substitutions-Théorie, by Netto. We all got the text. He lectured about three times, following the text closely and stopping sharp at the end of the hour. Then he began to think about matrices again. “I must give one lecture a week on those,” he said. He could not confine himself to the hour, nor to the one lecture a week. Two weeks were passed, and Netto was forgotten entirely and never mentioned again. Statements like the following were not unfrequent in his lectures: “I haven’t proved this, but I am as sure as I can be of anything that it must be so. From this it will follow, etc.” At the next lecture it turned out that what he was so sure of was false. Never mind, he kept on forever guessing and trying, and presently a wonderful discovery followed, then another and another. Afterward he would go back and work it all over again, and surprise us with all sorts of side lights. He then made another leap in the dark, more treasures were discovered, and so on forever.
As quoted by Florian Cajori, in Teaching and History of Mathematics in the United States (1890), 265-266.
[Someone] remarked to me once: Physicians should not say, I have cured this man, but, This man didn’t die in my care. In physics too one might say, For such and such a phenomenon I have determined causes whose absurdity cannot finally be proved, instead of saying, I have explained it.
As quoted in Joseph Peter Stern, Lichtenberg: A Doctrine of Scattered Occasions: Reconstructed From His Aphorisms and Reflections (1959), 297.
[This] may prove to be the beginning of some embracing generalization, which will throw light, not only on radioactive processes, but on elements in general and the Periodic Law.... Chemical homogeneity is no longer a guarantee that any supposed element is not a mixture of several of different atomic weights, or that any atomic weight is not merely a mean number.
From Chemical Society's Annual Reports (1910), Vol. 7, 285. As quoted in Francis Aston in Lecture (1936) on 'Forty Years of Atomic Theory', collected in Needham and Pagel (eds.) in Background to Modern Science: Ten Lectures at Cambridge Arranged by the History of Science Committee, (1938), 100. Cited in Alfred Walter Stewart, Recent Advances in Physical and Inorganic Chemistry (1920), 198.
[In refutation of evolution] They use carbon dating ... to prove that something was millions of years old. Well, we have the eruption of Mt. Saint Helens and the carbon dating test that they used then would have to then prove that these were hundreds of millions of years younger, when what happened was they had the exact same results on the fossils and canyons that they did the tests on that were supposedly 100 millions of years old. And it’s the kind of inconsistent tests like this that they’re basing their “facts” on.
[Citing results from a solitary young-Earth creationist, questioning whether the lava dome at Mount St. Helens is really a million years old.]
[Citing results from a solitary young-Earth creationist, questioning whether the lava dome at Mount St. Helens is really a million years old.]
From interview by Miles O''Brien on CNN (30 Mar 1996). Reported from transcript, via Nexis, in New York Magazine (15 Sep 2010).
Goldsmith: If you put a tub full of blood into a stable, the horses are like to go mad.
Johnson: I doubt that.
Goldsmith: Nay, sir, it is a fact well authenticated.
Thrale: You had better prove it before you put it into your book on natural history. You may do it in my stable if you will.
Johnson: Nay, sir, I would not have him prove it. If he is content to take his information from others, he may get through his book with little trouble, and without much endangering his reputation. But if he makes experiments for so comprehensive a book as his, there would be no end to them; his erroneous assertions would then fall upon himself: and he might be blamed for not having made experiments as to every particular.
Johnson: I doubt that.
Goldsmith: Nay, sir, it is a fact well authenticated.
Thrale: You had better prove it before you put it into your book on natural history. You may do it in my stable if you will.
Johnson: Nay, sir, I would not have him prove it. If he is content to take his information from others, he may get through his book with little trouble, and without much endangering his reputation. But if he makes experiments for so comprehensive a book as his, there would be no end to them; his erroneous assertions would then fall upon himself: and he might be blamed for not having made experiments as to every particular.
In James Boswell, The Life of Samuel Johnson, LL.D.: Comprehending an Account of His Studies and Numerous Works (1785, 1830), 229-230.
La vérité ne diffère de l'erreur qu'en deux points: elle est un peu plus difficile à prouver et beaucoup plus difficile à faire admettre. (Dec 1880)
Truth is different from error in two respects: it is a little harder to prove and more difficult to admit.
Truth is different from error in two respects: it is a little harder to prove and more difficult to admit.
In Recueil d'Œuvres de Léo Errera: Botanique Générale (1908), 193. Google translation by Webmaster.
~~[Misquoted ?]~~ It gives me the same pleasure when someone else proves a good theorem as when I do it myself.
Webmaster believes this is a first person paraphrase misquoted from “It was said round 1912 that it gave him the same pleasure when someone else proved a good theorem as if he had done it himself,” as given in Littlewood, A Mathematician’s Miscellany (1953), reissued as Béla Bollobás, Littlewood’s Miscellany (1986), 24. Notice that Littlewood states this in the third person—about Landau. As a first person quote, it is sometimes cited to a much later author, Desmond MacHale, Comic Sections (1993). Webmaster has not yet found a primary source to authenticate this first person version, and since the earlier occurrence is hedged with “it was said”, Webmaster suggests this first person version is a misquote, and is not an authentic verbatim quote.
A primâ facie argument in favour of the efficacy of prayer is therefore to be drawn from the very general use of it. The greater part of mankind, during all the historic ages, have been accustomed to pray for temporal advantages. How vain, it may be urged, must be the reasoning that ventures to oppose this mighty consensus of belief! Not so. The argument of universality either proves too much, or else it is suicidal.
'Statistical Inquiries into the Efficacy of Prayer', Fortnightly Review, 1872, 12, 126.
A discussion between Haldane and a friend began to take a predictable turn. The friend said with a sigh, “It’s no use going on. I know what you will say next, and I know what you will do next.” The distinguished scientist promptly sat down on the floor, turned two back somersaults, and returned to his seat. “There,” he said with a smile. “That’s to prove that you’re not always right.”
As quoted in Clifton Fadiman (ed.), André Bernard (ed.), Bartlett's Book of Anecdotes (2000), 253.
A fateful process is set in motion when the individual is released “to the freedom of his own impotence” and left to justify his existence by his own efforts. The autonomous individual, striving to realize himself and prove his worth, has created all that is great in literature, art, music, science and technology. The autonomous individual, also, when he can neither realize himself nor justify his existence by his own efforts, is a breeding call of frustration, and the seed of the convulsions which shake our world to its foundations.
In The Passionate State of Mind (1955), 18.
A few years ago, Science endeavored to show that it was not inconsistent with the bible. The tables have been turned, and now, Religion is endeavoring to prove that the bible is not inconsistent with Science.
In Some Mistakes of Moses (1879), 242.
A Frenchman who arrives in London, will find Philosophy, like every Thing else, very much chang’d there. He had left the World a plenum, and he now finds it a vacuum. At Paris the Universe is seen, compos’d of Vortices of subtile Matter; but nothing like it is seen in London. In France, ‘tis the Pressure of the Moon that causes the Tides; but in England ‘tis the Sea that gravitates towards the Moon; so what when you think that the Moon should make it flood with us, those Gentlemen fancy it should be Ebb, which, very unluckily, cannot be prov’d. For to be able to do this, ‘tis necessary the Moon and the Tides should have been enquir’d into, at the very instant of the Creation.
Letter XIV. 'On DesCartes and Sir Isaac Newton', in Letters Concerning the English Nation (1733), 109-110.
A physicist will tell me that this armchair is made of vibrations and that it’s not really here at all. But when Samuel Johnson was asked to prove the material existence of reality, he just went up to a big stone and kicked it. I'm with him.
Commenting on string theory. From 'Interview: Of Mind and Matter: David Attenborough Meets Richard Dawkins', The Guardian (11 Sep 2010).
A witty statesman said you might prove anything with figures.
In Chartism (1839, 1840), 9.
After we came out of the church, we stood talking for some time together of Bishop Berkeley’s ingenious sophistry to prove the non-existence of matter, and that every thing in the universe is merely ideal. I observed, that though we are satisfied his doctrine is not true, it is impossible to refute it. I never shall forget the alacrity with which Johnson answered, striking his foot with mighty force against a large stone, till he rebounded from it, “I refute it thus.”
In Boswell’s Life of Johnson (1820), Vol. 1, 218.
Alexander Langmuir was quoted in the early 1960s instructing incoming Epidemic Intelligence Service (EIS) officers that the only need for the laboratory in an outbreak investigation was to “prove their conclusions were right.” (2011)
As given in epigraph to Chap. 9, in Centers for Disease Control and Prevention, The CDC Field Epidemiology Manual (2018), 188. A footnoted reference explains: “Langmuir’s point was not to denigrate the laboratory but to emphasize the power of an investigation based on a solid clinical case definition and established field epidemiologic principles,” in Walter R. Dowdle (corresponding author), Leonard W. Mayer, Karen K. Steinberg, Neelam D. Ghiya and Tanja Popovic (co-authors), 'CDC Laboratory Contributions to Public Health', Morbidity and Mortality Weekly Report, Supplements (7 Oct 2011), 60, 27.
All fossil anthropoids found hitherto have been known only from mandibular or maxillary fragments, so far as crania are concerned, and so the general appearance of the types they represented had been unknown; consequently, a condition of affairs where virtually the whole face and lower jaw, replete with teeth, together with the major portion of the brain pattern, have been preserved, constitutes a specimen of unusual value in fossil anthropoid discovery. Here, as in Homo rhodesiensis, Southern Africa has provided documents of higher primate evolution that are amongst the most complete extant. Apart from this evidential completeness, the specimen is of importance because it exhibits an extinct race of apes intermediate between living anthropoids and man ... Whether our present fossil is to be correlated with the discoveries made in India is not yet apparent; that question can only be solved by a careful comparison of the permanent molar teeth from both localities. It is obvious, meanwhile, that it represents a fossil group distinctly advanced beyond living anthropoids in those two dominantly human characters of facial and dental recession on one hand, and improved quality of the brain on the other. Unlike Pithecanthropus, it does not represent an ape-like man, a caricature of precocious hominid failure, but a creature well advanced beyond modern anthropoids in just those characters, facial and cerebral, which are to be anticipated in an extinct link between man and his simian ancestor. At the same time, it is equally evident that a creature with anthropoid brain capacity and lacking the distinctive, localised temporal expansions which appear to be concomitant with and necessary to articulate man, is no true man. It is therefore logically regarded as a man-like ape. I propose tentatively, then, that a new family of Homo-simidæ be created for the reception of the group of individuals which it represents, and that the first known species of the group be designated Australopithecus africanus, in commemoration, first, of the extreme southern and unexpected horizon of its discovery, and secondly, of the continent in which so many new and important discoveries connected with the early history of man have recently been made, thus vindicating the Darwinian claim that Africa would prove to be the cradle of mankind.
'Australopithicus africanus: The Man-Ape of South Africa', Nature, 1925, 115, 195.
All sorts of dung and compost contain some matter which, when mixed with the soil, ferments therein; and by such ferment dissolves, crumbles, and divides the earth very much. This is the chief and almost only use of dung. … This proves, that its (manure) use is not to nourish, but to dissolve, i.e., divide the terrestrial matter, which affords nourishment to the Mouths of vegetable roots.
His underestimate of the value of manure. In The Horse-Hoeing Husbandry (1733), 18.
All that Eddington and Millikan achieve, when they attempt their preposterous reconciliation of science and theology, is to prove that they themselves, for all their technical skill, are scientists only by trade, not by conviction. They practice science diligently and to some effect, but only in the insensate way in which Blind Tom played the piano. … they can’t get rid of a congenital incredulity. Science, to them, remains a bit strange and shocking. They are somewhat in the position of a Christian clergyman who finds himself unable to purge himself of a suspicion that Jonah, after all, probably did not swallow the whale.
Minority Report (1956, 2006 reprint), 140.
All the experiments which have been hitherto carried out, and those that are still being daily performed, concur in proving that between different bodies, whether principles or compounds, there is an agreement, relation, affinity or attraction (if you will have it so), which disposes certain bodies to unite with one another, while with others they are unable to contract any union: it is this effect, whatever be its cause, which will help us to give a reason for all the phenomena furnished by chemistry, and to tie them together.
From Elemens de Chymie Theorique (1749). As quoted, in Trevor Harvey Levere, Affinity and Matter: Elements of Chemical Philosophy, 1800-1865 (1971), 17.
All those things [scientific research] which you would think would recommend me to the trustees my opponent is using against me. I am shamefully abused as being an atheist, an infidel. It has been positively asserted that I seek to make proselytes to infidelity, and that in my writings I have tried to prove that geology overthrows the Mosaic account of the creation. You may judge of my feelings.”
In letter (1853) to Spencer F. Baird, describing competing for the opening for chair of anatomy. As quoted, without detailed source citation, in Henry Fairfield Osborn, 'Biographical Memoir of Joseph Leidy', collected in National Academy of Sciences, Biographical Memoirs: Volume 7 (1913), 346.
Ampère was a mathematician of various resources & I think might rather be called excentric [sic] than original. He was as it were always mounted upon a hobby horse of a monstrous character pushing the most remote & distant analogies. This hobby horse was sometimes like that of a child ['s] made of heavy wood, at other times it resembled those [?] shapes [?] used in the theatre [?] & at other times it was like a hypogrif in a pantomime de imagie. He had a sort of faith in animal magnetism & has published some refined & ingenious memoirs to prove the identity of electricity & magnetism but even in these views he is rather as I said before excentric than original. He has always appeared to me to possess a very discursive imagination & but little accuracy of observation or acuteness of research.
'Davy’s Sketches of his Contemporaries', Chymia, 1967, 12, 135-6.
An experiment is never a failure solely because it fails to achieve predicted results. An experiment is a failure only when it also fails adequately to test the hypothesis in question, when the data it produces don’t prove anything one way or another.
In Zen and the Art of Motorcycle Maintenance: An Inquiry Into Values (1974), 102.
Any priest or shaman must be presumed guilty until proved innocent.
In 'From the Notebooks of Lazarus Long', Time Enough for Love: The Lives of Lazarus Long (1973), 256.
Archaeology is the science that proves you can’t keep a good man down.
In Bob Phillips, Phillips' Book of Great Thoughts & Funny Sayings (1993), 24.
Archaeology is the science that proves you can't keep a good man down.
In Bob Phillips, Phillips' Book of Great Thoughts and Funny Sayings (1993), 25
As history proves abundantly, mathematical achievement, whatever its intrinsic worth, is the most enduring of all.
In A Mathematician’s Apology (1940, 1967), 80.
At first men try with magic charms
To fertilize the earth,
To keep their flocks and herds from harm
And bring new young to birth.
Then to capricious gods they turn
To save from fire or flood;
Their smoking sacrifices burn
On altars red with blood.
Next bold philosopher and sage
A settled plan decree
And prove by thought or sacred page
What Nature ought to be.
But Nature smiles—a Sphinx-like smile
Watching their little day
She waits in patience for a while—
Their plans dissolve away.
Then come those humbler men of heart
With no completed scheme,
Content to play a modest part,
To test, observe, and dream.
Till out of chaos come in sight
Clear fragments of a Whole;
Man, learning Nature’s ways aright
Obeying, can control.
To fertilize the earth,
To keep their flocks and herds from harm
And bring new young to birth.
Then to capricious gods they turn
To save from fire or flood;
Their smoking sacrifices burn
On altars red with blood.
Next bold philosopher and sage
A settled plan decree
And prove by thought or sacred page
What Nature ought to be.
But Nature smiles—a Sphinx-like smile
Watching their little day
She waits in patience for a while—
Their plans dissolve away.
Then come those humbler men of heart
With no completed scheme,
Content to play a modest part,
To test, observe, and dream.
Till out of chaos come in sight
Clear fragments of a Whole;
Man, learning Nature’s ways aright
Obeying, can control.
Epigraph in A History of Science and Its Relation with Philosophy & Religion (1968), vi.
At terrestrial temperatures matter has complex properties which are likely to prove most difficult to unravel; but it is reasonable to hope that in the not too distant future we shall be competent to understand so simple a thing as a star.
The Internal Constitution of Stars, Cambridge. (1926, 1988), 393.
Borel makes the amusing supposition of a million monkeys allowed to play upon the keys of a million typewriters. What is the chance that this wanton activity should reproduce exactly all of the volumes which are contained in the library of the British Museum? It certainly is not a large chance, but it may be roughly calculated, and proves in fact to be considerably larger than the chance that a mixture of oxygen and nitrogen will separate into the two pure constituents. After we have learned to estimate such minute chances, and after we have overcome our fear of numbers which are very much larger or very much smaller than those ordinarily employed, we might proceed to calculate the chance of still more extraordinary occurrences, and even have the boldness to regard the living cell as a result of random arrangement and rearrangement of its atoms. However, we cannot but feel that this would be carrying extrapolation too far. This feeling is due not merely to a recognition of the enormous complexity of living tissue but to the conviction that the whole trend of life, the whole process of building up more and more diverse and complex structures, which we call evolution, is the very opposite of that which we might expect from the laws of chance.
The Anatomy of Science (1926), 158-9.
But it seems to me equally obvious that the orderliness is not all-pervasive. There are streaks of order to be found among the chaos, and the nature of scientific method is to seek these out and to stick to them when found and to reject or neglect the chaos. It is obvious that we have succeeded in finding some order in nature, but this fact in itself does not prove anything farther.
Scientific Method: An Inquiry into the Character and Validy of Natural Law (1923), 200.
Concepts that have proven useful in ordering thi ngs easily achieve such authority over us that we forget their earthly origins and accept them as unalterable givens.
…...
Dear Professor Rutherford, We students of our university physics club elect you our honorary president because you proved that atoms have balls.
In Letter (10 Oct 1929) received from students in Rostow na Donu, USSR, asking Ernest Rutherford USSR to become honorary president of their physics club. As quoted in George Gamow, My World Line: An Informal Autobiography (1970), 77-78. Gamow explained to Rutherford that the phrases for atomic “nucleus” and “cannonball” shared the use of the word “iadro” in Russian. The students had made faulty use of their Russian-English dictionary, but they were aware of Rutherford’s gold foil experiment. [Peter Atkins may have repeated the anecdote somewhere, but he did not originate it, yet the quote is seen attributed to him around the Web. —Webmaster]
Deaths, births, and marriages, considering how much they are separately dependent on the freedom of the human will, should seem to be subject to no law according to which any calculation could be made beforehand of their amount; and yet the yearly registers of these events in great countries prove that they go on with as much conformity to the laws of nature as the oscillations of the weather.
'Idea of a Universal history on a Cosmo-Political Plan' (1784). As translated by Thomas De Quinsey in The London Magazine (Oct 1824), 10, 385. Reprinted in 1859 by De Quincey in Vol. 8 of his Collective Edition of his writings.
Detest it as lewd intercourse, it can deprive you of all your leisure, your health, your rest, and the whole happiness of your life.
Having himself spent a lifetime unsuccessfully trying to prove Euclid’s postulate that parallel lines do not meet, Farkas discouraged his son János from any further attempt.
Having himself spent a lifetime unsuccessfully trying to prove Euclid’s postulate that parallel lines do not meet, Farkas discouraged his son János from any further attempt.
Letter (1820), to his son, János Bolyai. Translation as in Dirk Jan Struik, A concise history of mathematics (2nd Ed., 1948), 253.
Do not try the parallels in that way: I know that way all along. I have measured that bottomless night, and all the light and all the joy of my life went out there.
Having himself spent a lifetime unsuccessfully trying to prove Euclid's postulate that parallel lines do not meet, Farkas discouraged his son János from any further attempt.
Having himself spent a lifetime unsuccessfully trying to prove Euclid's postulate that parallel lines do not meet, Farkas discouraged his son János from any further attempt.
Letter (4 Apr 1820), to his son, János Bolyai. In J. J. O'Connor and E. F. Robertson, 'Farkas Wolfgang Bolyai' (Mar 2004), web article in MacTutor..
Even a wise experiment when made by a fool generally leads to a false conclusion, but that fools’ experiments conducted by a genius often prove to be leaps through the dark into great discoveries.
Commenting on Charles Darwin’s “fool’s experiments”, in 'Charles Robert Darwin', collected in C.D. Warner (ed.), Library of the World’s Best Literature Ancient and Modern (1896), Vol. 2, 4391-4392.
Every definition implies an axiom, since it asserts the existence of the object defined. The definition then will not be justified, from the purely logical point of view, until we have ‘proved’ that it involves no contradiction either in its terms or with the truths previously admitted.
…...
Every man will be a poet if he can; otherwise a philosopher or man of science. This proves the superiority of the poet.
Odell Shepard (Ed.), The Heart of Thoreau's Journals (1961), 84.
Facts are meaningless. You could use facts to prove anything that's even remotely true! Facts, shmacts.
Spoken by character Homer Simpson in TV episode written by David X. Cohen, 'Lisa the Skeptic', of the animated comedy, The Simpsons (23 Nov 1997). Quoted in Kee Malesky, All Facts Considered: The Essential Library of Inessential Knowledge (2010), 201.
Far must thy researches go
Wouldst thou learn the world to know;
Thou must tempt the dark abyss
Wouldst thou prove what Being is;
Naught but firmness gains the prize,—
Naught but fullness makes us wise,—
Buried deep truth ever lies!
Wouldst thou learn the world to know;
Thou must tempt the dark abyss
Wouldst thou prove what Being is;
Naught but firmness gains the prize,—
Naught but fullness makes us wise,—
Buried deep truth ever lies!
In Edgar A. Bowring (trans.), The Poems of Schiller (1875), 260.
For centuries knowledge meant proven knowledge…. Einstein’s results again turned the tables and now very few philosophers or scientists still think that scientific knowledge is, or can be, proven knowledge. But few realize that with this the whole classical structure of intellectual values falls in ruins and has to be replaced.
In 'Falsification and the Methodology of Scientific Research Programmes', in I. Lakatos and A. Musgrave (eds.), Criticism and the Growth of Knowledge: Proceedings of the International Colloquium in the Philosophy of Science, London 1965 (1970), Vol. 4, 91-92.
For God’s sake, please give it up. Fear it no less than the sensual passion, because it, too, may take up all your time and deprive you of your health, peace of mind and happiness in life.
Having himself spent a lifetime unsuccessfully trying to prove Euclid's postulate that parallel lines do not meet, Farkas discouraged his son János from any further attempt.
Having himself spent a lifetime unsuccessfully trying to prove Euclid's postulate that parallel lines do not meet, Farkas discouraged his son János from any further attempt.
Letter (1820) to his son, János Bolyai. Translation as in Philip J. Davis and Reuben Hersh, The Mathematical Experience (1981), 220. In Bill Swainson, Encarta Book of Quotations (2000), 124.
For if as scientists we seek simplicity, then obviously we try the simplest surviving theory first, and retreat from it only when it proves false. Not this course, but any other, requires explanation. If you want to go somewhere quickly, and several alternate routes are equally likely to be open, no one asks why you take the shortest. The simplest theory is to be chosen not because it is the most likely to be true but because it is scientifically the most rewarding among equally likely alternatives. We aim at simplicity and hope for truth.
Problems and Projects (1972), 352.
For it is too bad that there are so few who seek the truth and so few who do not follow a mistaken method in philosophy. This is not, however, the place to lament the misery of our century, but to rejoice with you over such beautiful ideas for proving the truth. So I add only, and I promise, that I shall read your book at leisure; for I am certain that I shall find the noblest things in it. And this I shall do the more gladly, because I accepted the view of Copernicus many years ago, and from this standpoint I have discovered from their origins many natural phenomena, which doubtless cannot be explained on the basis of the more commonly accepted hypothesis.
Letter (4 Aug 1597) to Kepler, expressing thanks and interest in the book Kepler sent him. As quoted in translation in Jackson J. Spielvogel, Western Civilization: Alternate Volume: Since 1300 (2010), Vol. 2, 494.
For the holy Bible and the phenomena of nature proceed alike from the divine Word, the former as the dictate of the Holy Ghost and the latter as the observant executrix of God's commands. It is necessary for the Bible, in order to be accommodated to the understanding of every man, to speak many things which appear to differ from the absolute truth so far as the bare meaning of the words is concerned. But Nature, on the other hand, is inexorable and immutable; she never transgresses the laws imposed upon her, or cares a whit whether her abstruse reasons and methods of operation are understandable to men. For that reason it appears that nothing physical which sense-experience sets before our eyes, or which necessary demonstrations prove to us, ought to be called in question (much less condemned) upon the testimony of biblical passages which may have some different meaning beneath their words.
Letter to Madame Christina of Lorraine, Grand Duchess of Tuscany: Concerning the Use of Biblical Quotations in Matters of Science (1615), trans. Stillman Drake, Discoveries and Opinions of Galileo (1957), 182-3.
For the saving the long progression of the thoughts to remote and first principles in every case, the mind should provide itself several stages; that is to say, intermediate principles, which it might have recourse to in the examining those positions that come in its way. These, though they are not self-evident principles, yet, if they have been made out from them by a wary and unquestionable deduction, may be depended on as certain and infallible truths, and serve as unquestionable truths to prove other points depending upon them, by a nearer and shorter view than remote and general maxims. … And thus mathematicians do, who do not in every new problem run it back to the first axioms through all the whole train of intermediate propositions. Certain theorems that they have settled to themselves upon sure demonstration, serve to resolve to them multitudes of propositions which depend on them, and are as firmly made out from thence as if the mind went afresh over every link of the whole chain that tie them to first self-evident principles.
In The Conduct of the Understanding, Sect. 21.
For there are two modes of acquiring knowledge, namely, by reasoning and experience. Reasoning draws a conclusion and makes us grant the conclusion, but does not make the conclusion certain, nor does it remove doubt so that the mind may rest on the intuition of truth, unless the mind discovers it by the path of experience; since many have the arguments relating to what can be known, but because they lack experience they neglect the arguments, and neither avoid what is harmful nor follow what is good. For if a man who has never seen fire should prove by adequate reasoning that fire burns and injures things and destroys them, his mind would not be satisfied thereby, nor would he avoid fire, until he placed his hand or some combustible substance in the fire, so that he might prove by experience that which reasoning taught. But when he has had actual experience of combustion his mind is made certain and rests in the full light of truth. Therefore reasoning does not suffice, but experience does.
Opus Majus [1266-1268], Part VI, chapter I, trans. R. B. Burke, The Opus Majus of Roger Bacon (1928), Vol. 2, 583.
Frogs will eat red-flannel worms fed to them by biologists; this proves a great deal about both parties concerned.
Quoted in Joseph Wood Krutch, The Best of Two Worlds (1953), 105.
Gauss was not the son of a mathematician; Handel’s father was a surgeon, of whose musical powers nothing is known; Titian was the son and also the nephew of a lawyer, while he and his brother, Francesco Vecellio, were the first painters in a family which produced a succession of seven other artists with diminishing talents. These facts do not, however, prove that the condition of the nerve-tracts and centres of the brain, which determine the specific talent, appeared for the first time in these men: the appropriate condition surely existed previously in their parents, although it did not achieve expression. They prove, as it seems to me, that a high degree of endowment in a special direction, which we call talent, cannot have arisen from the experience of previous generations, that is, by the exercise of the brain in the same specific direction.
In 'On Heredity', Essays upon Heredity and Kindred Biological Problems (1889), Vol. 1, 96.
Gentlemen, that is surely true, it is absolutely paradoxical; we cannot understand it, and we don’t know what it means. But we have proved it, and therefore we know it is the truth.
In a lecture, after establishing the relation eπ/2 = i√i in a lecture, “which evidently had a strong hold on his imagination. He dropped his chalk and rubber, put his hands in his pockets, and after contemplating the formula a few minutes turned to his class and said [this quote] very slowly and impressively.” As quoted in W. E. Byerly (writing as a Professor Emeritus at Harvard University, but a former student of Peirce), 'Benjamin Peirce: II. Reminiscences', The American Mathematical Monthly (Jan 1925), 32, No. 1, 6.
Geology fully proves that organic creation passed through a series of stages before the highest vegetable and animal forms appeared.
Explanations (1845), 31
God exists since mathematics is consistent, and the Devil exists since we cannot prove it.
Given as “A. Weil has said…”, in Paul C. Rosenbloom, The Elements of Mathematical Logic (1950), 72. Note that Rosenbloom gives the quote in narrative form, not within quotation marks, which suggests these words may not be verbatim. Later texts have added quotation marks [which may not be justified. —Webmaster] As yet, Webmaster has not found an earlier source to validate whether the quotations marks can be used. (Can you help?)
God pity the man of science who believes in nothing but what he can prove by scientific methods; for if ever a human being needed divine pity, he does.
Every-Day Topics, a Book of Briefs (1882), 5.
Gödel proved that the world of pure mathematics is inexhaustible; no finite set of axioms and rules of inference can ever encompass the whole of mathematics; given any finite set of axioms, we can find meaningful mathematical questions which the axioms leave unanswered. I hope that an analogous Situation exists in the physical world. If my view of the future is correct, it means that the world of physics and astronomy is also inexhaustible; no matter how far we go into the future, there will always be new things happening, new information coming in, new worlds to explore, a constantly expanding domain of life, consciousness, and memory.
From Lecture 1, 'Philosophy', in a series of four James Arthur Lectures, 'Lectures on Time and its Mysteries' at New York University (Autumn 1978). Printed in 'Time Without End: Physics and Biology in an Open Universe', Reviews of Modern Physics (Jul 1979), 51, 449.
He [Sylvester] had one remarkable peculiarity. He seldom remembered theorems, propositions, etc., but had always to deduce them when he wished to use them. In this he was the very antithesis of Cayley, who was thoroughly conversant with everything that had been done in every branch of mathematics.
I remember once submitting to Sylvester some investigations that I had been engaged on, and he immediately denied my first statement, saying that such a proposition had never been heard of, let alone proved. To his astonishment, I showed him a paper of his own in which he had proved the proposition; in fact, I believe the object of his paper had been the very proof which was so strange to him.
I remember once submitting to Sylvester some investigations that I had been engaged on, and he immediately denied my first statement, saying that such a proposition had never been heard of, let alone proved. To his astonishment, I showed him a paper of his own in which he had proved the proposition; in fact, I believe the object of his paper had been the very proof which was so strange to him.
As quoted by Florian Cajori, in Teaching and History of Mathematics in the United States (1890), 268.
He made an instrument to know If the moon shine at full or no;
That would, as soon as e’er she shone straight,
Whether ‘twere day or night demonstrate;
Tell what her d’ameter to an inch is,
And prove that she’s not made of green cheese.
That would, as soon as e’er she shone straight,
Whether ‘twere day or night demonstrate;
Tell what her d’ameter to an inch is,
And prove that she’s not made of green cheese.
He thought he saw an Argument
That proved he was the Pope:
He looked again and found it was
A Bar of Mottled Soap.
“A fact so dread.” he faintly said,
“Extinguishes all hope!”
That proved he was the Pope:
He looked again and found it was
A Bar of Mottled Soap.
“A fact so dread.” he faintly said,
“Extinguishes all hope!”
In Sylvie and Bruno Concluded (1893), 319.
However, if we consider that all the characteristics which have been cited are only differences in degree of structure, may we not suppose that this special condition of organization of man has been gradually acquired at the close of a long period of time, with the aid of circumstances which have proved favorable? What a subject for reflection for those who have the courage to enter into it!
In Recherches sur l'Organization des corps vivans (1802), as translated in Alpheus Spring Packard, Lamarck, the Founder of Evolution: His Life and Work (1901), 363. Packard's italics.
I am an atheist, out and out. It took me a long time to say it. I’ve been an atheist for years and years, but somehow I felt it was intellectually unrespectable to say one was an atheist, because it assumed knowledge that one didn't have. Somehow, it was better to say one was a humanist or an agnostic. I finally decided that I’m a creature of emotion as well as of reason. Emotionally, I am an atheist. I don't have the evidence to prove that God doesn’t exist, but I so strongly suspect he doesn’t that I don’t want to waste my time.
'Isaac Asimov on Science and the Bible'. In Sidney Hook, et. al. On the Barricades: Religion and Free Inquiry in Conflict (1989), 329.
I am an organic chemist, albeit one who adheres to the definition of organic chemistry given by the great Swedish chemist Berzelius, namely, the chemistry of substances found in living matter, and my science is one of the more abstruse insofar as it rests on concepts and employs a jargon neither of which is a part of everyday experience. Nevertheless, organic chemistry deals with matters of truly vital Importance and in some of its aspects with which I myself have been particularly concerned it may prove to hold the keys to Life itself.
In 'Synthesis in the Study of Nucleotides', Nobel Lecture, 11 December 1957. In Nobel Lectures: Chemistry 1942-1962 (1964), 522.
I am coming more and more to the conviction that the necessity of our geometry cannot be proved, at least neither by, nor for, the human intelligence … One would have to rank geometry not with arithmetic, which stands a priori, but approximately with mechanics.
From Letter (28 Apr 1817) to Olbers, as quoted in Guy Waldo Dunnington, Carl Friedrich Gauss, Titan of Science: A Study of His Life and Work (1955), 180.
I am more fond of achieving than striving. My theories must prove to be facts or be discarded as worthless. My efforts must soon be crowned with success, or discontinued.
The Rest of My Life, ch. 4 (1937).Wells wrote, in addition to other works, the popular 'Fleming Stone' detective series.
I believe in an immortal soul. science has proved that nothing disintegrates into nothingness. Life and soul, therefore, cannot disintegrate into nothingness, and so are immortal.
…...
I believe that mathematical reality lies outside us, that our function is to discover or observe it, and that the theorems which we prove, and which we describe grandiloquently as our “creations,” are simply the notes of our observations.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 113.
I believe that the science of chemistry alone almost proves the existence of an intelligent creator.
…...
I can’t prove it, but I’m pretty sure that people gain a selective advantage from believing in things they can’t prove.
In David Stokes, Nicholas Wilson and Martha Mador, Entrepreneurship (2009), 190.
I can’t recall a single problem in my life, of any sort, that I ever started on that I didn't solve, or prove that I couldn’t solve it. I never let up, until I had done everything that I could think of, no matter how absurd it might seem as a means to the end I was after.
As quoted in French Strother, 'The Modern Profession of Inventing', World's Work and Play (Jul 1905), 6, No. 32, 186.
I cannot but be astonished that Sarsi should persist in trying to prove by means of witnesses something that I may see for myself at any time by means of experiment. Witnesses are examined in doutbful matters which are past and transient, not in those which are actual and present. A judge must seek by means of witnesses to determine whether Peter injured John last night, but not whether John was injured, since the judge can see that for himself.
'The Assayer' (1623), trans. Stillman Drake, Discoveries and Opinions of Galileo (1957), 271.
I claim to have proved that the sea itself provides sufficient food and drink to enable the battle for survival to be fought with perfect confidence.
In Bombard’s Voyage: Abridged from The Bombard Story (1959), 143.
I confess that Fermat’s Theorem as an isolated proposition has very little interest for me, because I could easily lay down a multitude of such propositions, which one could neither prove nor dispose of.
From Letter (Mar 1816), replying to Olbers’ attempt to entice him to work on Fermat’s Theorem. In James R. Newman (ed.) The World of Mathematics (1956), Vol. 1, 312-313.
I confess that Fermat’s Theorem as an isolated proposition has very little interest for me, for a multitude of such theorems can easily be set up, which one could neither prove nor disprove. But I have been stimulated by it to bring our again several old ideas for a great extension of the theory of numbers. Of course, this theory belongs to the things where one cannot predict to what extent one will succeed in reaching obscurely hovering distant goals. A happy star must also rule, and my situation and so manifold distracting affairs of course do not permit me to pursue such meditations as in the happy years 1796-1798 when I created the principal topics of my Disquisitiones arithmeticae. But I am convinced that if good fortune should do more than I expect, and make me successful in some advances in that theory, even the Fermat theorem will appear in it only as one of the least interesting corollaries.
In reply to Olbers' attempt in 1816 to entice him to work on Fermat's Theorem. The hope Gauss expressed for his success was never realised.
In reply to Olbers' attempt in 1816 to entice him to work on Fermat's Theorem. The hope Gauss expressed for his success was never realised.
Letter to Heinrich Olbers (21 Mar 1816). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 413.
I have been arranging certain experiments in reference to the notion that Gravity itself may be practically and directly related by experiment to the other powers of matter and this morning proceeded to make them. It was almost with a feeling of awe that I went to work, for if the hope should prove well founded, how great and mighty and sublime in its hitherto unchangeable character is the force I am trying to deal with, and how large may be the new domain of knowledge that may be opened up to the mind of man.
In Thomas Martin (ed.) Faraday’s Diary: Sept. 6, 1847 - Oct. 17, 1851 (1934), 156.
I have said that science is impossible without faith. … Inductive logic, the logic of Bacon, is rather something on which we can act than something which we can prove, and to act on it is a supreme assertion of faith … Science is a way of life which can only fluorish when men are free to have faith.
In Calyampudi Radhakrishna Rao, Statistics and Truth (1997), 31.
I hereby bequeath to the Bide-a-Wee Home all people who have statistics to prove that a human
Is nothing but a combination of iron and water and potash and albumen.
That may very well be the truth
But it’s just like saying that a cocktail is nothing but ice and gin and vermouth.
Is nothing but a combination of iron and water and potash and albumen.
That may very well be the truth
But it’s just like saying that a cocktail is nothing but ice and gin and vermouth.
From 'Lines in Dispraise of Dispraise', Hard Lines (1931), 40.
I know of scarcely anything so apt to impress the imagination as the wonderful form of cosmic order expressed by the “Law of Frequency of Error.” The law would have been personified by the Greeks and deified, if they had known of it. It reigns with serenity and in complete self-effacement, amidst the wildest confusion. The huger the mob, and the greater the apparent anarchy, the more perfect is its sway. It is the supreme law of Unreason. Whenever a large sample of chaotic elements are taken in hand and marshaled in the order of their magnitude, an unsuspected and most beautiful form of regularity proves to have been latent all along.
In Natural Inheritance (1894), 66.
I must admit that when I chose the name, “vitamine,” I was well aware that these substances might later prove not to be of an amine nature. However, it was necessary for me to choose a name that would sound well and serve as a catchword, since I had already at that time no doubt about the importance and the future popularity of the new field.
The Vitamines translated by Harry Ennis Dubin (1922), 26, footnote.
I ought to call myself an agnostic; but, for all practical purposes, I am an atheist. I do not think the existence of the Christian God any more probable than the existence of the Gods of Olympus or Valhalla. To take another illustration: nobody can prove that there is not between the Earth and Mars a china teapot revolving in an elliptical orbit, but nobody thinks this sufficiently likely to be taken into account in practice. I think the Christian God just as unlikely.
Letter (1958) to Mr Major. Collected in Dear Bertrand Russell: A Selection of his
Correspondence with the General Public, 1950 - 1968 (1969), 41-42.
I refrained from writing another one, thinking to myself: Never mind, I will prove that I am able to become a greater scientist than some of you, even without the title of doctor.
Reaction when his thesis (1922) on rocket experiments was rejected as too cursory. In Astronautics (1959), 4, No. 6, 103.
I was pretty good in science. But again, because of the small budget, in science class we couldn’t do experiments in order to prove theories. We just believed everything. Actually I think that class was call Religion. Religion was always an easy class. All you had to do was suspend the logic and reasoning you were taught in all the other classes.
In autobiography, Brain Droppings (1998), 227.
I've come loaded with statistics, for I've noticed that a man can't prove anything without statistics. No man can.
Speech at the Republican Rally, Hartford Opera House (26 Oct 1880). In Mark Twain and Paul Fatout (ed.,) Mark Twain Speaking (2006), 140.
If [in a rain forest] the traveler notices a particular species and wishes to find more like it, he must often turn his eyes in vain in every direction. Trees of varied forms, dimensions, and colors are around him, but he rarely sees any of them repeated. Time after time he goes towards a tree which looks like the one he seeks, but a closer examination proves it to be distinct.
In 'Equitorial Vegetation', Natural Selection and Tropical Nature Essays on Descriptive and Theoretical Biology (1891), 267.
If anyone could prove to me that Christ is outside the truth, and if the truth really did exclude Christ, I should prefer to stay with Christ and not with truth.
Quoted in Kim Lim (ed.), 1,001 Pearls of Spiritual Wisdom: Words to Enrich, Inspire, and Guide Your Life (2014), 155
If basketball was going to enable Bradley to make friends, to prove that a banker’s son is as good as the next fellow, to prove that he could do without being the greatest-end-ever at Missouri, to prove that he was not chicken, and to live up to his mother’s championship standards, and if he was going to have some moments left over to savor his delight in the game, he obviously needed considerable practice, so he borrowed keys to the gym and set a schedule for himself that he adhereded to for four full years—in the school year, three and a half hours every day after school, nine to five on Saturday, one-thirty to five on Sunday, and, in the summer, about three hours a day.
A Sense of Where You Are: Bill Bradley at Princeton
If Einstein’s theory [of relativity] should prove to be correct, as I expect it will, he will be considered the Copernicus of the twentieth century.
As quoted in Philipp Frank and Shuichi Kusaka, Einstein, His Life and Times (1947), 101.
If finally, the science should prove that society at a certain time revert to the church and recover its old foundation of absolute faith in a personal providence and a revealed religion, it commits suicide.
In The Degradation of the Democratic Dogma (1919), 131.
If I set out to prove something, I am no real scientist—I have to learn to follow where the facts lead me—I have to learn to whip my prejudices.
Attributed, as cited in Peter McDonald (ed.), Oxford Dictionary of Medical Quotations (2004), 95. Quoted earlier without citation as “If I want to find truth, I must have an open mind. I have to learn to follow where the facts lead me—I have to learn to whip my prejudices,” in World Order (1948), 14, 76.
If I were to awaken after having slept for a thousand years, my first question would be: Has the Riemann hypothesis been proven?
…...
If my theory of relativity is proven successful, Germany will claim me as a German and France will declare I am a citizen of the world. Should my theory prove untrue, France will say I am a German and Germany will declare I am a Jew.
In Speech (6 Apr 1922) to the French Philosophical Society, Sorbonne. Quoted in Alice Calaprice, The Quotable Einstein (1996), 8.
If one proves the equality of two numbers a and b by showing first that “a is less than or equal to b” and then “a is greater than or equal to b”, it is unfair, one should instead show that they are really equal by disclosing the inner ground for their equality.
As quoted, without citation, in biography by Hermann Wehl, Emmy Noether (1935), 18.
If to be the Author of new things, be a crime; how will the first Civilizers of Men, and makers of Laws, and Founders of Governments escape? Whatever now delights us in the Works of Nature, that excells the rudeness of the first Creation, is New. Whatever we see in Cities, or Houses, above the first wildness of Fields, and meaness of Cottages, and nakedness of Men, had its time, when this imputation of Novelty, might as well have bin laid to its charge. It is not therefore an offence, to profess the introduction of New things, unless that which is introduc'd prove pernicious in itself; or cannot be brought in, without the extirpation of others, that are better.
The History of the Royal Society (1667), 322.
If we consider that part of the theory of relativity which may nowadays in a sense be regarded as bone fide scientific knowledge, we note two aspects which have a major bearing on this theory. The whole development of the theory turns on the question of whether there are physically preferred states of motion in Nature (physical relativity problem). Also, concepts and distinctions are only admissible to the extent that observable facts can be assigned to them without ambiguity (stipulation that concepts and distinctions should have meaning). This postulate, pertaining to epistemology, proves to be of fundamental importance.
'Fundamental ideas and problems of the theory of relativity', Lecture delivered to the Nordic Assembly of Naturalists at Gothenburg, 11 Jul 1923. In Nobel Physics 1901-1921 (1998), 482.
If you have to prove a theorem, do not rush. First of all, understand fully what the theorem says, try to see clearly what it means. Then check the theorem; it could be false. Examine the consequences, verify as many particular instances as are needed to convince yourself of the truth. When you have satisfied yourself that the theorem is true, you can start proving it.
In How to Solve It: A New Aspect of Mathematical Method (2004), 15.
If you were going to risk all that, not just risk the hardship and the pain but risk your life. Put everything on line for a dream, for something that’s worth nothing, that can’t be proved to anybody. You just have the transient moment on a summit and when you come back down to the valley it goes. It is actually a completely illogical thing to do. It is not justifiable by any rational terms. That’s probably why you do it.
The Beckoning Silence
In every case the awakening touch has been the mathematical spirit, the attempt to count, to measure, or to calculate. What to the poet or the seer may appear to be the very death of all his poetry and all his visions—the cold touch of the calculating mind,—this has proved to be the spell by which knowledge has been born, by which new sciences have been created, and hundreds of definite problems put before the minds and into the hands of diligent students. It is the geometrical figure, the dry algebraical formula, which transforms the vague reasoning of the philosopher into a tangible and manageable conception; which represents, though it does not fully describe, which corresponds to, though it does not explain, the things and processes of nature: this clothes the fruitful, but otherwise indefinite, ideas in such a form that the strict logical methods of thought can be applied, that the human mind can in its inner chamber evolve a train of reasoning the result of which corresponds to the phenomena of the outer world.
In A History of European Thought in the Nineteenth Century (1896), Vol. 1, 314.
In order that an inventory of plants may be begun and a classification of them correctly established, we must try to discover criteria of some sort for distinguishing what are called “species”. After a long and considerable investigation, no surer criterion for determining species had occurred to me than distinguishing features that perpetuate themselves in propagation from seed. Thus, no matter what variations occur in the individuals or the species, if they spring from the seed of one and the same plant, they are accidental variations and not such as to distinguish a species. For these variations do not perpetuate themselves in subsequent seeding. Thus, for example, we do not regard caryophylli with full or multiple blossoms as a species distinct from caryophylli with single blossoms, because the former owe their origin to the seed of the latter and if the former are sown from their own seed, they once more produce single-blossom caryophylli. But variations that never have as their source seed from one and the same species may finally be regarded as distinct species. Or, if you make a comparison between any two plants, plants which never spring from each other's seed and never, when their seed is sown, are transmuted one into the other, these plants finally are distinct species. For it is just as in animals: a difference in sex is not enough to prove a difference of species, because each sex is derived from the same seed as far as species is concerned and not infrequently from the same parents; no matter how many and how striking may be the accidental differences between them; no other proof that bull and cow, man and woman belong to the same species is required than the fact that both very frequently spring from the same parents or the same mother. Likewise in the case of plants, there is no surer index of identity of species than that of origin from the seed of one and the same plant, whether it is a matter of individuals or species. For animals that differ in species preserve their distinct species permanently; one species never springs from the seed of another nor vice versa.
— John Ray
Historia Plantarum (1686), Vol. 1, 40. Trans. Edmund Silk. Quoted in Barbara G. Beddall, 'Historical Notes on Avian Classification', Systematic Zoology (1957), 6, 133-4.
In teaching man, experimental science results in lessening his pride more and more by proving to him every day that primary causes, like the objective reality of things, will be hidden from him forever and that he can only know relations.
…...
In the course of centuries the naïve self-love of men has had to submit to two major blows at the hands of science. The first was when they learnt that our earth was not the centre of the universe but only a tiny fragment of a cosmic system of scarcely imaginable vastness… the second blow fell when biological research destroyed man’s supposedly privileged place in creation and proved his descent from the animal kingdom and his ineradicable animal nature… But human megalomania will have suffered its third and most wounding blow from the psychological research of the present time which seeks to prove to the ego that it is not even master in its own house, but must content itself with scanty information of what is going on unconsciously in its mind.
Introductory Lectures on Psychoanalyis (1916), in James Strachey (ed.), The Standard Edition of the Complete Psychological Works of Sigmund Freud (1963), Vol. 16, 284-5.
In the expressions we adopt to prescribe physical phenomena we necessarily hover between two extremes. We either have to choose a word which implies more than we can prove, or we have to use vague and general terms which hide the essential point, instead of bringing it out. The history of electrical theories furnishes a good example.
Opening Address to the Annual Meeting of the British Association by Prof. Arthur Schuster, in Nature (4 Aug 1892), 46, 325.
In the modern interpretation of Mendelism, facts are being transformed into factors at a rapid rate. If one factor will not explain the facts, then two are involved; if two prove insufficient, three will sometimes work out. The superior jugglery sometimes necessary to account for the results may blind us, if taken too naively, to the common-place that the results are often so excellently 'explained' because the explanation was invented to explain them. We work backwards from the facts to the factors, and then, presto! explain the facts by the very factors that we invented to account for them. I am not unappreciative of the distinct advantages that this method has in handling the facts. I realize how valuable it has been to us to be able to marshal our results under a few simple assumptions, yet I cannot but fear that we are rapidly developing a sort of Mendelian ritual by which to explain the extraordinary facts of alternative inheritance. So long as we do not lose sight of the purely arbitrary and formal nature of our formulae, little harm will be done; and it is only fair to state that those who are doing the actual work of progress along Mendelian lines are aware of the hypothetical nature of the factor-assumption.
'What are 'Factors' in Mendelian Explanations?', American Breeders Association (1909), 5, 365.
In the same sense that our judicial system presumes us to be innocent until proven guilty, a medical care system may work best if it starts with the presumption that most people are healthy. Left to themselves, computers may try to do it in the opposite way, taking it as given that some sort of direct, continual, professional intervention is required all the time, in order to maintain the health of each citizen, and we will end up spending all our money on nothing but this.
In 'Aspects of Biomedical Science Policy', The New England Journal of Medicine (12 Oct 1972), 4. Also published as Occasional Paper of the Institute of Medicine.
In the sphere of natural science let us remember that we have always to deal with an insoluble problem. Let us prove keen and honest in attending to anything which is in any way brought to our notice, most of all when it does not fit in with our previous ideas. For it is only thereby that we perceive the problem, which does indeed lie in nature, but still more in man.
In The Maxims and Reflections of Goethe (1906), 183.
In the summer of 1937, … I told Banach about an expression Johnny [von Neumann] had once used in conversation with me in Princeton before stating some non-Jewish mathematician’s result, “Die Goim haben den folgendenSatzbewiesen” (The goys have proved the following theorem). Banach, who was pure goy, thought it was one of the funniest sayings he had ever heard. He was enchanted by its implication that if the goys could do it, Johnny and I ought to be able to do it better. Johnny did not invent this joke, but he liked it and we started using it.
In Adventures of a Mathematician (1976, 1991), 107. Von Neumann, who was raised in Budapest by a Jewish family, knew the Yiddish word “goy” was equivalent to “gentile” or a non-Jew. Stefan Banach, a Polish mathematician, was raised in a Catholic family, hence “pure goy”. Ulam thus gives us the saying so often elsewhere seen attributed to von Neumann without the context: “The goys have proved the following theorem.” It is seen anecdotally as stated by von Neumann to begin a classroom lecture.
In the X-ray laboratory we are exposed, not only to the direct action of the rays, but to the effects of ionized air. This may be proved by hanging a charged silk tassel anywhere in the room. It will suddenly collapse when the current is turned on through the focus tube.
In 'Protection in X-Ray Work', Archives of the Roentgen Ray (July 1905), 10, No. 2, 38. [Note that this concern for protection, written in 1905, comes within 10 years of the discovery of X-Rays in 1895. —Webmaster]
Induction may be defined, the operation of discovering and proving general propositions.
In A System of Logic, Ratiocinative and Inductive: Being a Connected View of the Principles of Evidence, and the Methods of Scientific Investigation (1843), Vol. 1, 347.
It appears to me that those who rely simply on the weight of authority to prove any assertion, without searching out the arguments to support it, act absurdly. I wish to question freely and to answer freely without any sort of adulation. That well becomes any who are sincere in the search for truth.
Quoted in James Reston, Jr., Galileo, a Life, p. 9.
It is a melancholy experience for a professional mathematician to find him writing about mathematics. The function of a mathematician is to do something, to prove new theorems, to add to mathematics, and not to talk about what he or other mathematicians have done. Statesmen despise publicists, painters despise art-critics, and physiologists, physicists, or mathematicians have usually similar feelings; there is no scorn more profound, or on the whole more justifiable, than that of men who make for the men who explain. Exposition, criticism, appreciation, is work for second-rate minds.
In A Mathematician's Apology (1940, reprint with Foreward by C.P. Snow 1992), 61 (Hardy's opening lines after Snow's foreward).
It is beyond all doubt that during the sixteenth century, and the years immediately preceding and following it, poisoning had been brought to a pitch of perfection which remains unknown to modern chemistry, but which is indisputably proved by history. Italy, the cradle of modern science, was at that time the inventor and mistress of these secrets, many of which are lost. Romancers have made such extravagant use of this fact, that whenever they introduce Italians they make them play the part of assassins and poisoners.
In Catherine De Medici (1842), 274-275.
It is by logic that we prove, but by intuition that we discover.
In Science and Method (1908) translated by Francis Maitland (1914, 2007), 129.
It is certainly true that all physical phenomena are subject to strictly mathematical conditions, and mathematical processes are unassailable in themselves. The trouble arises from the data employed. Most phenomena are so highly complex that one can never be quite sure that he is dealing with all the factors until the experiment proves it. So that experiment is rather the criterion of mathematical conclusions and must lead the way.
In Matter, Ether, Motion (1894), 89.
It is imperative in the design process to have a full and complete understanding of how failure is being obviated in order to achieve success. Without fully appreciating how close to failing a new design is, its own designer may not fully understand how and why a design works. A new design may prove to be successful because it has a sufficiently large factor of safety (which, of course, has often rightly been called a “factor of ignorance”), but a design's true factor of safety can never be known if the ultimate failure mode is unknown. Thus the design that succeeds (ie, does not fail) can actually provide less reliable information about how or how not to extrapolate from that design than one that fails. It is this observation that has long motivated reflective designers to study failures even more assiduously than successes.
In Design Paradigms: Case Histories of Error and Judgment in Engineering (1994), 31.
books.google.comHenry Petroski - 1994
It is not enough to discover and prove a useful truth previously unknown, but that it is necessary also to be able to propagate it and get it recognized.
Philosophie Zoologique (1809), Vol. 2, 450, trans. Hugh Elliot (1914), 404
It is one of the chief merits of proofs that they instil a certain scepticism as to the result proved.
In The Principles of Mathematics (1903), Vol. 1, 360.
It is perhaps difficult sufficiently to emphasise Seeking without disparaging its correlative Finding. But I must risk this, for Finding has a clamorous voice that proclaims its own importance; it is definite and assured, something that we can take hold of —that is what we all want, or think we want. Yet how transitory it proves.
The finding of one generation will not serve for the next. It tarnishes rapidly except it be reserved with an ever-renewed spirit of seeking.
The finding of one generation will not serve for the next. It tarnishes rapidly except it be reserved with an ever-renewed spirit of seeking.
Swarthmore Lecture (1929) at Friends’ House, London, printed in Science and the Unseen World (1929), 87-88.
It is through science that we prove, but through intuition that we discover.
…...
It is time, therefore, to abandon the superstition that natural science cannot be regarded as logically respectable until philosophers have solved the problem of induction. The problem of induction is, roughly speaking, the problem of finding a way to prove that certain empirical generalizations which are derived from past experience will hold good also in the future.
Language, Truth and Logic (1960), 49.
It is very different to make a practical system and to introduce it. A few experiments in the laboratory would prove the practicability of system long before it could be brought into general use. You can take a pipe and put a little coal in it, close it up, heat it and light the gas that comes out of the stem, but that is not introducing gas lighting. I'll bet that if it were discovered to-morrow in New York that gas could be made out of coal it would be at least five years before the system would be in general use.
From the New York Herald (30 Jan 1879), as cited in Leslie Tomory, 'Building the First Gas Network, 1812-1820', Technology and Culture (Jan 2011), 52, No. 1, 75-102.
It must be granted that in every syllogism, considered as an argument to prove the conclusion, there is a petitio principii. When we say, All men are mortal Socrates is a man therefore Socrates is mortal; it is unanswerably urged by the adversaries of the syllogistic theory, that the proposition, Socrates is mortal.
A System of Logic, Ratiocinative and Inductive (1858), 122.
It usually develops that after much laborious and frustrating effort the investigator of environmental physiology succeeds in proving that the animal in question can actually exist where it lives. It is always somewhat discouraging for an investigator to realize that his efforts can be made to appear so trite, but this statement does not belittle the ecological physiologist. If his data assist the understanding of the ways in which an animal manages to live where it does, he makes an important contribution to the study of distribution, for the present is necessarily a key to the past.”
From 'The role of physiology in the distribution of terrestrial vertebrates', collected in C.L. Hubbs (ed.), Zoogeography: Publ. 51 (1958), 84.
It was his [Leibnitz’s] love of method and order, and the conviction that such order and harmony existed in the real world, and that our success in understanding it depended upon the degree and order which we could attain in our own thoughts, that originally was probably nothing more than a habit which by degrees grew into a formal rule. This habit was acquired by early occupation with legal and mathematical questions. We have seen how the theory of combinations and arrangements of elements had a special interest for him. We also saw how mathematical calculations served him as a type and model of clear and orderly reasoning, and how he tried to introduce method and system into logical discussions, by reducing to a small number of terms the multitude of compound notions he had to deal with. This tendency increased in strength, and even in those early years he elaborated the idea of a general arithmetic, with a universal language of symbols, or a characteristic which would be applicable to all reasoning processes, and reduce philosophical investigations to that simplicity and certainty which the use of algebraic symbols had introduced into mathematics.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
In Leibnitz (1884), 44-45. [The first sentence is reworded to better introduce the quotation. —Webmaster]
It was said round 1912 that it gave him [Edmund Landau] the same pleasure when someone else proved a good theorem as if he had done it himself.
In A Mathematician’s Miscellany (1953), reissued as Béla Bollobás, Littlewood’s Miscellany (1986), 24. Notice that Littlewood states this in the third person—about Landau. The words are found on the web paraphrased as a first person quote (It gives me … as when I do it myself), sometimes citing a later author, Desmond MacHale, Comic Sections (1993). Webmaster has not yet found a primary source to authenticate the first person version, and since the earlier occurrence is hedged with “it was said”, Webmaster suggests the first person version is not an authentic verbatim quote.
Keep some souvenirs of your past, or how will you ever prove it wasn’t all a dream?
…...
Let us now recapitulate all that has been said, and let us conclude that by hermetically sealing the vials, one is not always sure to prevent the birth of the animals in the infusions, boiled or done at room temperature, if the air inside has not felt the ravages of fire. If, on the contrary, this air has been powerfully heated, it will never allow the animals to be born, unless new air penetrates from outside into the vials. This means that it is indispensable for the production of the animals that they be provided with air which has not felt the action of fire. And as it would not be easy to prove that there were no tiny eggs disseminated and floating in the volume of air that the vials contain, it seems to me that suspicion regarding these eggs continues, and that trial by fire has not entirely done away with fears of their existence in the infusions. The partisans of the theory of ovaries will always have these fears and will not easily suffer anyone's undertaking to demolish them.
Nouvelles Recherches sur les Découvertes Microscopiques, et la Génération des Corps Organisés (1769), 134-5. Quoted in Jacques Roger, The Life Sciences in Eighteenth-Century French Thought, ed. Keith R. Benson and trans. Robert Ellrich (1997), 510-1.
Logicians have but ill defined
As rational the human mind;
Reason, they say, belongs to man,
But let them prove it if they can.
As rational the human mind;
Reason, they say, belongs to man,
But let them prove it if they can.
In 'The Logicians Refuted', The Poems and Plays of Oliver Goldsmith (1818), 58.
Lord Kelvin was so satisfied with this triumph of science that he declared himself to be as certain of the existence of the ether as a man can be about anything.... “When you can measure what you are speaking about, and express it in numbers, you know something about it....” Thus did Lord Kelvin lay down the law. And though quite wrong, this time he has the support of official modern Science. It is NOT true that when you can measure what you are speaking about, you know something about it. The fact that you can measure something doesn't even prove that that something exists.... Take the ether, for example: didn't they measure the ratio of its elasticity to its density?
In Science is a Sacred Cow (1950), 69-70; 85.
Many 'hard' scientists regard the term 'social science' as an oxymoron. Science means hypotheses you can test, and prove or disprove. Social science is little more than observation putting on airs.
'A Cuba Policy That's Stuck On Plan A', opinion column, The Washington Post (17 Apr 2009)
Many orthodox people speak as though it were the business of sceptics to disprove received dogmas rather than of dogmatists to prove them. This is, of course, a mistake.
In unpublished manuscript, 'Is There a God', (5 Mar 1952) written for the magazine, Illustrated. Collected in Bertrand Russell, John G. Slater (ed.) and Peter Köllner (ed.) The Collected Papers of Bertrand Russell: Volume II: Last Philosophical Testament: 1943-68 (1997), 547.
Mathematical proofs are essentially of three different types: pre-formal; formal; post-formal. Roughly the first and third prove something about that sometimes clear and empirical, sometimes vague and ‘quasi-empirical’ stuff, which is the real though rather evasive subject of mathematics.
In Mathematics, Science and Epistemology (1980), Vol. 2, 69.
Mathematics as a science commenced when first someone, probably a Greek, proved propositions about any things or about some things, without specification of definite particular things. These propositions were first enunciated by the Greeks for geometry; and, accordingly, geometry was the great Greek mathematical science.
In An Introduction to Mathematics (1911), 15.
Mathematics had never had more than a secondary interest for him [her husband, George Boole]; and even logic he cared for chiefly as a means of clearing the ground of doctrines imagined to be proved, by showing that the evidence on which they were supposed to give rest had no tendency to prove them. But he had been endeavoring to give a more active and positive help than this to the cause of what he deemed pure religion.
In Eleanor Meredith Cobham, Mary Everest Boole: Collected Works (1931), 40.
Mathematics is a public activity. It occurs in a social context and has social consequences. Posing a problem, formulating a definition, proving a theorem are none of them private acts. They are all part of that larger social process we call science.
In 'Mathematics as an Objective Science', The American Mathematical Monthly (Aug-Sep 1979), 86, No. 7, 542. Reprinted in The Mathematical Intelligencer (1983), 5, No. 3.
Mathematics is not a deductive science—that’s a cliché. When you try to prove a theorem, you don’t just list the hypotheses, and then start to reason. What you do is trial and error, experiment and guesswork.
In I Want to be a Mathematician: an Automathography in Three Parts (1985), 321.
Mathematics, including not merely Arithmetic, Algebra, Geometry, and the higher Calculus, but also the applied Mathematics of Natural Philosophy, has a marked and peculiar method or character; it is by preeminence deductive or demonstrative, and exhibits in a
nearly perfect form all the machinery belonging to this mode of obtaining truth. Laying down a very small number of first principles, either self-evident or requiring very little effort to prove them, it evolves a vast number of deductive truths and applications, by a procedure in the highest degree mathematical and systematic.
In Education as a Science (1879), 148.
Metaphysics: An attempt to prove the incredible by an appeal to the unintelligible.
…...
My crystal ball or intuition tells me that in the '80s the impact of RIA [radioimmunoassay] on the study of infectious diseases may prove as revolutionary as its impact on endocrinology in the 60s.
Radioimmunoassay: A probe for fine structure of biological systems Nobel Lecture, 8 Dec 1977
My Design in this Book is not to explain the Properties of Light by Hypotheses, but to propose and prove them by Reason and Experiments: In order to which, I shall premise the following Definitions and Axioms.
Opticks (1704), Book 1, Part 1, Introduction, 1.
My experiences with science led me to God. They challenge science to prove the existence of God. But must we really light a candle to see the sun?
In letter to California State board of Education (14 Sep 1972).
No amount of experimentation can ever prove me right; a single experiment can prove me wrong.
Attributed to Einstein. Quoted in Alice Calaprice, The Quotable Einstein (1996), 224.
No science doth make known the first principles whereon it buildeth; but they are always taken as plain and manifest in themselves, or as proved and granted already, some former knowledge having made them evident.
In The of Mr. Richard Hooker in Eight Books Of the Laws of Ecclesiastical Polity (1723), Book 3, 69.
Noise proves nothing. Often a hen who has merely laid an egg cackles as if she laid an asteroid. — Pudd’nhead Wilson’s New Calendar
Epigraph to Chap. 5, In More Tramps Abroad (1897), Vol. 1, 40. Also published under the title Following the Equator (1897).
None of us deny evolution. We know it and study it with pleasure. Catholic universities do not see anything in evolution to prevent a Christian accepting it, but with the reservation that the great problem of the origin of the world and of the creation of man is the secret of God. The Catholic church accepts what science gives it on condition that science reports facts which can be proved, for it is a fact that there is no scientific truth which can contradict eternal truth.
The position of the Catholic Church on the theory of evolution, as stated by Monseigneur Piette, as he introduced the next speaker Dr. Thomas Hunt Morgan, professor of experimental zoology at Columbia University. As given in The School of Education Record of the University of North Dakota (Jun 1926), Vol.11, No. 9, 72.
Not long ago the head of what should be a strictly scientific department in one of the major universities commented on the odd (and ominous) phenomenon that persons who can claim to be scientists on the basis of the technical training that won them the degree of Ph.D. are now found certifying the authenticity of the painted rag that is called the “Turin Shroud” or adducing “scientific” arguments to support hoaxes about the “paranormal” or an antiquated religiosity. “You can hire a scientist [sic],” he said, “to prove anything.” He did not adduce himself as proof of his generalization, but he did boast of his cleverness in confining his own research to areas in which the results would not perturb the Establishment or any vociferous gang of shyster-led fanatics. If such is indeed the status of science and scholarship in our darkling age, Send not to ask for whom the bell tolls.
In 'The Price of the Head', Instauration Magazine (Mar 1980).
Not only are there meaningless questions, but many of the problems with which the human intellect has tortured itself turn out to be only 'pseudo problems,' because they can be formulated only in terms of questions which are meaningless. Many of the traditional problems of philosophy, of religion, or of ethics, are of this character. Consider, for example, the problem of the freedom of the will. You maintain that you are free to take either the right- or the left-hand fork in the road. I defy you to set up a single objective criterion by which you can prove after you have made the turn that you might have made the other. The problem has no meaning in the sphere of objective activity; it only relates to my personal subjective feelings while making the decision.
The Nature of Physical Theory (1936), 12.
Nothing can so quickly blur and distort the facts as desire—the wish to use the facts for some purpose of your own—and nothing can so surely destroy the truth. As soon as the witness wants to prove something he is no longer impartial and his evidence is no longer to be trusted.
From 'Getting at the Truth', The Saturday Review (19 Sep 1953), 36, No. 38, 12. Excerpted in Meta Riley Emberger and Marian Ross Hall, Scientific Writing (1955), 400.
Of possible quadruple algebras the one that had seemed to him by far the most beautiful and remarkable was practically identical with quaternions, and that he thought it most interesting that a calculus which so strongly appealed to the human mind by its intrinsic beauty and symmetry should prove to be especially adapted to the study of natural phenomena. The mind of man and that of Nature’s God must work in the same channels.
As quoted in W. E. Byerly (writing as a Professor Emeritus at Harvard University, but a former student at a Peirce lecture on Hamilton’s new calculus of quaternions), 'Benjamin Peirce: II. Reminiscences', The American Mathematical Monthly (Jan 1925), 32, No. 1, 6.
Of Science generally we can remark, first, that it is the most perfect embodiment of Truth, and of the ways of getting at Truth. More than anything else does it impress the mind with the nature of Evidence, with the labour and precautions necessary to prove a thing. It is the grand corrective of the laxness of the natural man in receiving unaccredited facts and conclusions. It exemplifies the devices for establishing a fact, or a law, under every variety of circumstances; it saps the credit of everything that is affirmed without being properly attested.
In Education as a Science (1879), 147-148.
Of the many forms of false culture, a premature converse with abstractions is perhaps the most likely to prove fatal to the growth of a masculine vigour of intellect.
In A Treatise on Differential Equations (1859), Preface, vi.
One aim of physical sciences had been to give an exact picture the material world. One achievement of physics in the twentieth century has been to prove that that aim is unattainable.
From The Ascent of Man (1973), 353.
One could not by any experience whatsoever demonstrate that the heavens, and not the earth, are moved with a diurnal motion.
In Livre du ciel et du monde (On the Book of the Heavens and the World of Aristotle) (completed 1377), Book 2, Chap 25. Translated by Menut and Denomy (1968), as excerpted in Marshall Clagett, The Science of Mechanics in the Middle Ages, 600. Also found translated as, “No experience whatsoever could prove that the heavens rotate daily and not the earth.”
One of the principal results of civilization is to reduce more and more the limits within which the different elements of society fluctuate. The more intelligence increases the more these limits are reduced, and the nearer we approach the beautiful and the good. The perfectibility of the human species results as a necessary consequence of all our researches. Physical defects and monstrosities are gradually disappearing; the frequency and severity of diseases are resisted more successfully by the progress of modern science; the moral qualities of man are proving themselves not less capable of improvement; and the more we advance, the less we shall have need to fear those great political convulsions and wars and their attendant results, which are the scourges of mankind.
…...
One of the ways of stopping science would be only to do experiments in the region where you know the law. … In other words we are trying to prove ourselves wrong as quickly as possible, because only in that way can we find progress.
In The Character of Physical Law (1965, 2001), 158.
Our earth is very old, an old warrior that has lived through many battles. Nevertheless, the face of it is still changing, and science sees no certain limit of time for its stately evolution. Our solid earth, apparently so stable, inert, and finished, is changing, mobile, and still evolving. Its major quakings are largely the echoes of that divine far-off event, the building of our noble mountains. The lava floods and intriguing volcanoes tell us of the plasticity, mobility, of the deep interior of the globe. The slow coming and going of ancient shallow seas on the continental plateaus tell us of the rhythmic distortion of the deep interior-deep-seated flow and changes of volume. Mountain chains prove the earth’s solid crust itself to be mobile in high degree. And the secret of it all—the secret of the earthquake, the secret of the “temple of fire,” the secret of the ocean basin, the secret of the highland—is in the heart of the earth, forever invisible to human eyes.
In Our Mobile Earth (1926), 320.
Our world is not an optimal place, fine tuned by omnipotent forces of selection. It is a quirky mass of imperfections, working well enough (often admirably); a jury-rigged set of adaptations built of curious parts made available by past histories in different contexts ... A world optimally adapted to current environments is a world without history, and a world without history might have been created as we find it. History matters; it confounds perfection and proves that current life transformed its own past.
…...
Persons possessing great intellect and a capacity for excelling in the creative arts and also in the sciences are generally likely to have heavier brains than the ordinary individual. Arguing from this we might expect to find a corresponding lightness in the brain of the criminal, but this is not always the case ... Many criminals show not a single anomaly in their physical or mental make-up, while many persons with marked evidences of morphological aberration have never exhibited the criminal tendency.
Every attempt to prove crime to be due to a constitution peculiar only to criminals has failed signally. It is because most criminals are drawn from the ranks of the low, the degraded, the outcast, that investigators were ever deceived into attempting to set up a 'type' of criminal. The social conditions which foster the great majority of crimes are more needful of study and improvement.
From study of known normal brains we have learned that there is a certain range of variation. No two brains are exactly alike, and the greatest source of error in the assertions of Benedict and Lombroso has been the finding of this or that variation in a criminal’s brains, and maintaining such to be characteristic of the 'criminal constitution,' unmindful of the fact that like variations of structure may and do exist in the brains of normal, moral persons.
Every attempt to prove crime to be due to a constitution peculiar only to criminals has failed signally. It is because most criminals are drawn from the ranks of the low, the degraded, the outcast, that investigators were ever deceived into attempting to set up a 'type' of criminal. The social conditions which foster the great majority of crimes are more needful of study and improvement.
From study of known normal brains we have learned that there is a certain range of variation. No two brains are exactly alike, and the greatest source of error in the assertions of Benedict and Lombroso has been the finding of this or that variation in a criminal’s brains, and maintaining such to be characteristic of the 'criminal constitution,' unmindful of the fact that like variations of structure may and do exist in the brains of normal, moral persons.
Address to the American Association for the Advancement of Science in Philadelphia (28 Dec 1904), as quoted in 'Americans of Future Will Have Best Brains', New York Times (29 Dec 1904), 6.
Physics is NOT a body of indisputable and immutable Truth; it is a body of well-supported probable opinion only .... Physics can never prove things the way things are proved in mathematics, by eliminating ALL of the alternative possibilities. It is not possible to say what the alternative possibilities are.... Write down a number of 20 figures; if you multiply this by a number of, say, 30 figures, you would arrive at some enormous number (of either 49 or 50 figures). If you were to multiply the 30-figure number by the 20-figure number you would arrive at the same enormous 49- or 50-figure number, and you know this to be true without having to do the multiplying. This is the step you can never take in physics.
In Science is a Sacred Cow (1950), 68, 88, 179.
Presumptions are of vast importance in medicine, as in law. A man is presumed innocent until he is proved guilty. A medicine—that is, a noxious agent, like a blister, a seton, an emetic, or a cathartic-should always be presumed to be hurtful. It always is directly hurtful; it may sometimes be indirectly beneficial. If this presumption were established, and disease always assumed to be the innocent victim of circumstances, and not punishable by medicines, that is, noxious agents, or poisons, until the contrary was shown, we should not so frequently hear the remark commonly, perhaps erroneously, attributed to Sir Astley Cooper, but often repeated by sensible persons, that, on the whole, more harm than good is done by medication.
From Address to Annual Meeting of Massachusetts Medical Society (30 May 1860), 'Currents and Counter-Currents in Medical Science'. Collected in The Writings of Oliver Wendell Holmes: Medical Essays, 1842-1882 (1891), 202.
Prove all things; hold fast that which is good.
— Bible
In Thessalonians 5:21, King James Version, Bible. For example, in Robert Carroll and Stephen Prickett, eds., The Bible: Authorized King James Version (2008), 255.
Psychoanalysis is a science conducted by lunatics for lunatics. They are generally concerned with proving that people are irresponsible; and they certainly succeed in proving that some people are.
From Illustrated London News (23 Jun 1928). In Dale Ahlquist (ed.) The Universe According to G.K. Chesterton: A Dictionary of the Mad, Mundane and Metaphysical (2013), 93.
Pure mathematics proves itself a royal science both through its content and form, which contains within itself the cause of its being and its methods of proof. For in complete independence mathematics creates for itself the object of which it treats, its magnitudes and laws, its formulas and symbols.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 94. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 11.
Round about the accredited and orderly facts of every science there ever floats a sort of dust-cloud of exceptional observations, of occurrences minute and irregular and seldom met with, which it always proves more easy to ignore than to attend to … Anyone will renovate his science who will steadily look after the irregular phenomena, and when science is renewed, its new formulas often have more of the voice of the exceptions in them than of what were supposed to be the rules.
In 'The Hidden Self', Scribner’s Magazine (1890), Vol. 7, 361.
Round about the accredited and orderly facts of every science there ever floats a sort of dustcloud of exceptional observations, of occurrences minute and irregular and seldom met with, which it always proves more easy to ignore than to attend to.
The Fractal Geometry of Nature (1977, 1983), 28.
Science deals with judgments on which it is possible to obtain universal agreement. These judgments do not concern individual facts and events, but the invariable association of facts and events known as the laws of science. Agreement is secured by observation and experiment—impartial courts of appeal to which all men must submit if they wish to survive. The laws are grouped and explained by theories of ever increasing generality. The theories at first are ex post facto—merely plausible interpretations of existing bodies of data. However, they frequently lead to predictions that can be tested by experiments and observations in new fields, and, if the interpretations are verified, the theories are accepted as working hypotheses until they prove untenable. The essential requirements are agreement on the subject matter and the verification of predictions. These features insure a body of positive knowledge that can be transmitted from person to person, and that accumulates from generation to generation.
From manuscript on English Science in the Renaissance (1937), Edwin Hubble collection, Box 2, Huntington Library, San Marino, California. As cited by Norriss S. Hetherington in 'Philosophical Values and Observation in Edwin Hubble's Choice of a Model of the Universe', Historical Studies in the Physical Sciences (1982), 13, No. 1, 41. (Hetherington comments parenthetically that the references to court, judgment and appeal may be attributable to his prior experiences as a Rhodes Scholar reading Roman law at Oxford, and to a year's practice as an attorney in Louisville, Kentucky.)
Science is like society and trade, in resting at bottom upon a basis of faith. There are some things here, too, that we can not prove, otherwise there would be nothing we can prove. Science is busy with the hither-end of things, not the thither-end. It is a mistake to contrast religion and science in this respect, and to think of religion as taking everything for granted, and science as doing only
clean work, and having all the loose ends gathered up and tucked in. We never reach the roots of things in science more than in religion.
From 'Walking by Faith', The Pattern in the Mount: And Other Sermons (1885), 49. The sentence “Science is busy with the hither-end of things, not the thither-end” is quoted alone in collections such as James Wood, Dictionary of Quotations from Ancient and Modern, English and Foreign Sources (1893), 382:35.
Science is often regarded as the most objective and truth-directed of human enterprises, and since direct observation is supposed to be the favored route to factuality, many people equate respectable science with visual scrutiny–just the facts ma’am, and palpably before my eyes. But science is a battery of observational and inferential methods, all directed to the testing of propositions that can, in principle, be definitely proven false ... At all scales, from smallest to largest, quickest to slowest, many well-documented conclusions of science lie beyond the strictly limited domain of direct observation. No one has ever seen an electron or a black hole, the events of a picosecond or a geological eon.
In Eight Little Piggies: Reflections in Natural History (1994), 200.
Science is really in the business of disproving current models or changing them to conform to new information. In essence, we are constantly proving our latest ideas wrong.
John Mitchinson and John Lloyd, If Ignorance Is Bliss, Why Aren't There More Happy People?: Smart Quotes for Dumb Times (2009), 274.
Science is the study of the admitted laws of existence, which cannot prove a universal negative about whether those laws could ever be suspended by something admittedly above them. It is as if we were to say that a lawyer was so deeply learned in the American Constitution that he knew there could never be a revolution in America..
From 'The Early Bird in History',The Thing: Why I Am Catholic (1929), 207. In Collected Works (1990), Vol. 3, 296.
Science, by itself, cannot supply us with an ethic. It can show us how to achieve a given end, and it may show us that some ends cannot be achieved. But among ends that can be achieved our choice must be decided by other than purely scientific considerations. If a man were to say, “I hate the human race, and I think it would be a good thing if it were exterminated,” we could say, “Well, my dear sir, let us begin the process with you.” But this is hardly argument, and no amount of science could prove such a man mistaken.
'The Science to Save us from Science', New York Times Magazine (19 Mar 1950). Collected in M. Gardner (ed.), Great Essays in Science (1950), 396-397.
Scientific findings do not threaten anyone (except to the extent that Homo sapiens may prove incapable of controlling what science makes possible). But what is critical to understand is that our species (or, for that matter, God) is not in the least diminished by the idea that we emerged thanks to the processes of evolution.
In The Monkey in the Mirror: Essays on the Science of What Makes Us Human (2003), 55.
Scientific knowledge scarcely exists amongst the higher classes of society. The discussion in the Houses of Lords or of Commons, which arise on the occurrence of any subjects connected with science, sufficiently prove this fact…
In Reflections on the Decline of Science in England (1830), 8.
Scientists are supposed to make predictions, probably to prove they are human and can be as mistaken as anyone else. Long-range predictions are better to make because the audience to whom the prediction was made is no longer around to ask questions. The alternative... is to make conflicting predictions, so that one prediction may prove right.
'Fossils—The How and Why of Collecting and Storing', Proceedings of the Biological Society of Washington (1969), 82, 597.
Scripture and Nature agree in this, that all things were covered with water; how and when this aspect began, and how long it lasted, Nature says not, Scripture relates. That there was a watery fluid, however, at a time when animals and plants were not yet to be found, and that the fluid covered all things, is proved by the strata of the higher mountains, free from all heterogeneous material. And the form of these strata bears witness to the presence of a fluid, while the substance bears witness to the absence of heterogeneous bodies. But the similarity of matter and form in the strata of mountains which are different and distant from each other, proves that the fluid was universal.
The Prodromus of Nicolaus Steno's Dissertation Concerning a Solid Body enclosed by Process of Nature within a Solid (1669), trans. J. G. Winter (1916), 263-4.
Since the examination of consistency is a task that cannot be avoided, it appears necessary to axiomatize logic itself and to prove that number theory and set theory are only parts of logic. This method was prepared long ago (not least by Frege’s profound investigations); it has been most successfully explained by the acute mathematician and logician Russell. One could regard the completion of this magnificent Russellian enterprise of the axiomatization of logic as the crowning achievement of the work of axiomatization as a whole.
Address (11 Sep 1917), 'Axiomatisches Denken' delivered before the Swiss Mathematical Society in
Zürich. Translated by Ewald as 'Axiomatic Thought', (1918), in William Bragg Ewald, From Kant to Hilbert (1996), Vol. 2, 1113.
Slavery in America was perpetuated not merely by human badness but also by human blindness. … Men convinced themselves that a system that was so economically profitable must be morally justifiable. … Science was commandeered to prove the biological inferiority of the Negro. Even philosophical logic was manipulated [exemplified by] an Aristotlian syllogism:
All men are made in the image of God;
God, as everyone knows, is not a Negro;
Therefore, the Negro is not a man.
All men are made in the image of God;
God, as everyone knows, is not a Negro;
Therefore, the Negro is not a man.
'Love in Action', Strength To Love (1963, 1981), 44.
Some of my cousins who had the great advantage of University education used to tease me with arguments to prove that nothing has any existence except what we think of it. … These amusing mental acrobatics are all right to play with. They are perfectly harmless and perfectly useless. ... I always rested on the following argument. … We look up to the sky and see the sun. Our eyes are dazzled and our senses record the fact. So here is this great sun standing apparently on no better foundation than our physical senses. But happily there is a method, apart altogether from our physical senses, of testing the reality of the sun. It is by mathematics. By means of prolonged processes of mathematics, entirely separate from the senses, astronomers are able to calculate when an eclipse will occur. They predict by pure reason that a black spot will pass across the sun on a certain day. You go and look, and your sense of sight immediately tells you that their calculations are vindicated. So here you have the evidence of the senses reinforced by the entirely separate evidence of a vast independent process of mathematical reasoning. We have taken what is called in military map-making “a cross bearing.” When my metaphysical friends tell me that the data on which the astronomers made their calculations, were necessarily obtained originally through the evidence of the senses, I say, “no.” They might, in theory at any rate, be obtained by automatic calculating-machines set in motion by the light falling upon them without admixture of the human senses at any stage. When it is persisted that we should have to be told about the calculations and use our ears for that purpose, I reply that the mathematical process has a reality and virtue in itself, and that onie discovered it constitutes a new and independent factor. I am also at this point accustomed to reaffirm with emphasis my conviction that the sun is real, and also that it is hot— in fact hot as Hell, and that if the metaphysicians doubt it they should go there and see.
In My Early Life (1930).
Some of the men stood talking in this room, and at the right of the door a little knot had formed round a small table, the center of which was the mathematics student, who was eagerly talking. He had made the assertion that one could draw through a given point more than one parallel to a straight line; Frau Hagenström had cried out that this was impossible, and he had gone on to prove it so conclusively that his hearers were constrained to behave as though they understood.
In Little Herr Friedemann (1961), 25.
Some recent work by E. Fermi and L. Szilard, which has been communicated to me in manuscript, leads me to expect that the element uranium may be turned into a new and important source of energy in the immediate future. Certain aspects of the situation seem to call for watchfulness and, if necessary, quick action on the part of the Administration. …
In the course of the last four months it has been made probable … that it may become possible to set up nuclear chain reactions in a large mass of uranium, by which vast amounts of power and large quantities of new radium-like elements would be generated. Now it appears almost certain that this could be achieved in the immediate future.
This new phenomenon would also lead to the construction of bombs, and it is conceivable—though much less certain—that extremely powerful bombs of a new type may thus be constructed. A single bomb of this type, carried by boat or exploded in a port, might well destroy the whole port altogether with some of the surrounding territory. However, such bombs might well prove to be too heavy for transportation by air.
In the course of the last four months it has been made probable … that it may become possible to set up nuclear chain reactions in a large mass of uranium, by which vast amounts of power and large quantities of new radium-like elements would be generated. Now it appears almost certain that this could be achieved in the immediate future.
This new phenomenon would also lead to the construction of bombs, and it is conceivable—though much less certain—that extremely powerful bombs of a new type may thus be constructed. A single bomb of this type, carried by boat or exploded in a port, might well destroy the whole port altogether with some of the surrounding territory. However, such bombs might well prove to be too heavy for transportation by air.
Letter to President Franklin P. Roosevelt, (2 Aug 1939, delivered 11 Oct 1939). In Otto Nathan and Heinz Norden (Eds.) Einstein on Peace (1960, reprinted 1981), 294-95.
Statistics can be made to prove anything—even the truth.
In Evan Esar, 20,000 Quips and Quotes (1995), 765.
Success in the solution of a problem generally depends in a great measure on the selection of the most appropriate method of approaching it; many properties of conic sections (for instance) being demonstrable by a few steps of pure geometry which would involve the most laborious operations with trilinear co-ordinates, while other properties are almost self-evident under the method of trilinear co-ordinates, which it would perhaps be actually impossible to prove by the old geometry.
In Trilinear Coordinates and Other Methods of Modern Analytical Geometry of Two Dimensions (1866), 154.
That is the way of the scientist. He will spend thirty years in building up a mountain range of facts with the intent to prove a certain theory; then he is so happy with his achievement that as a rule he overlooks the main chief fact of all—that all his accumulation proves an entirely different thing.
'The Bee'. In What is Man? and Other Essays? (1917), 283.
The [mechanical] bird I have described ought to be able by the help of the wind to rise to a great height, and this will prove to be its safety; since even if… revolutions [of the winds] were to befall it, it would still have time to regain a condition of equilibrium; provided that its various parts have a great power of resistance, so that they can safely withstand the fury and violence of the descent, by the aid of the defenses which I have mentioned; and its joints should be made of strong tanned hide, and sewn with cords of strong raw silk. And let no one encumber himself with iron bands, for these are very soon broken at the joints or else they become worn out, and consequently it is well not to encumber oneself with them.
'Of the Bird’s Movement' from Sul Voio degli Uccelli, 8 [7] r. in Leonardo da Vinci's Notebooks, trans. E. MacCurdy (1906), 153-4.
The ancestors of the higher animals must be regarded as one-celled beings, similar to the Amœbæ which at the present day occur in our rivers, pools, and lakes. The incontrovertible fact that each human individual develops from an egg, which, in common with those of all animals, is a simple cell, most clearly proves that the most remote ancestors of man were primordial animals of this sort, of a form equivalent to a simple cell. When, therefore, the theory of the animal descent of man is condemned as a “horrible, shocking, and immoral” doctrine, tho unalterable fact, which can be proved at any moment under the microscope, that the human egg is a simple cell, which is in no way different to those of other mammals, must equally be pronounced “horrible, shocking, and immoral.”
Translated from his Ueber die Entstehung und den Stammbaum des Menschengeschlechts, (1873), Vol. 2, as an epigraph to Chap. 6, The Evolution of Man, (1879), Vol 1, 120-121.
The average gambler will say “The player who stakes his whole fortune on a single play is a fool, and the science of mathematics can not prove him to be otherwise.” The reply is obvious: “The science of mathematics never attempts the impossible, it merely shows that other players are greater fools.”
Concluding remarks to his mathematical proof, with certain assumptions, that the best betting strategy for “Gambler’s Ruin” would be to always make his largest stake on his first play. In 'Gambler’s Ruin', Annals of Mathematics (Jul 1909), 2nd Series, 10, No. 4, 189. This is also seen, without primary source, quoted as “It is true that a man who does this is a fool. I have only proved that a man who does anything else is an even bigger fool,” in Harold Eves, Return to Mathematical Circles (1988), 39.
The central dogma, enunciated by Crick in 1958 and the keystone of molecular biology ever since, is likely to prove a considerable over-simplification. That is the heretical but inescapable conclusion stemming from experiments done in the past few months in two laboratories in the United States.
'News and Views', Nature, 1970, 226, 1198.
The cult of individual personalities is always, in my view, unjustified. To be sure, nature distributes her gifts variously among her children. But there are plenty of the well-endowed ones too, thank God, and I am firmly convinced that most of them live quiet, unregarded lives. It strikes me as unfair, and even in bad taste, to select a few of them for boundless admiration, attributing superhuman powers of mind and character to them. This has been my fate, and the contrast between the popular estimate of my powers and achievements and the reality is simply grotesque. The consciousness of this extraordinary state of affairs would be unbearable but for one great consoling thought: it is a welcome symptom in an age which is commonly denounced as materialistic, that it makes heroes of men whose ambitions lie wholly in the intellectual and moral sphere. This proves that knowledge and justice are ranked above wealth and power by a large section of the human race. My experience teaches me that this idealistic outlook is particularly prevalent in America, which is usually decried as a particularly materialistic country.
From Mein Weltbild, as translated by Alan Harris (trans.), 'Some Notes on my American Impressions', The World as I See It (1956, 1993), 37-38.
The decadence of the Greeks and Romans without change of race proves the influence of institutions upon customs.
From Appendix A, 'Extracts From the Unpublished Writings of Carnot', Reflections on the Motive Power of Heat (1890, 2nd ed. 1897), 211.
The development of mathematics toward greater precision has led, as is well known, to the formalization of large tracts of it, so that one can prove any theorem using nothing but a few mechanical rules... One might therefore conjecture that these axioms and rules of inference are sufficient to decide any mathematical question that can at all be formally expressed in these systems. It will be shown below that this is not the case, that on the contrary there are in the two systems mentioned relatively simple problems in the theory of integers that cannot be decided on the basis of the axioms.
'On Formally Undecidable Propositions of Principia Mathematica and Related Systems I' (193 1), in S. Feferman (ed.), Kurt Gödel Collected Works: Publications 1929-1936 (1986), Vol. I, 145.
The dexterous management of terms and being able to fend and prove with them, I know has and does pass in the world for a great part of learning; but it is learning distinct from knowledge, for knowledge consists only in perceiving the habitudes and relations of ideas one to another, which is done without words; the intervention of sounds helps nothing to it. And hence we see that there is least use of distinction where there is most knowledge: I mean in mathematics, where men have determined ideas with known names to them; and so, there being no room for equivocations, there is no need of distinctions.
In Conduct of the Understanding, Sect. 31.
The existence of trousers proves that God meant us to be bipeds.
Caption to cartoon showing one 19th century man holding a book, speaking to another holding a book titled “Darwin.” Accompanying article by Ralph Estling, 'The Trouble With Thinking Backwards', New Scientist (2 Jun 1983), 620.
The fact that man knows right from wrong proves his intellectual superiority to other creatures; but the fact that he can do wrong proves his moral inferiority to any creature that cannot.
Spoken by Old Man in What is Man? In What is Man? and Other Essays (1917), 89.
The fact that man produces a concept ‘I’ besides the totality of his mental and emotional experiences or perceptions does not prove that there must be any specific existence behind such a concept. We are succumbing to illusions produced by our self-created language, without reaching a better understanding of anything. Most of so-called philosophy is due to this kind of fallacy.
…...
The fact that your patient gets well does not prove that your diagnosis was correct.
…...
The facts proved by geology are briefly these: that during an immense, but unknown period, the surface of the earth has undergone successive changes; land has sunk beneath the ocean, while fresh land has risen up from it; mountain chains have been elevated; islands have been formed into continents, and continents submerged till they have become islands; and these changes have taken place, not once merely, but perhaps hundreds, perhaps thousands of times.
In 'On the Law which has regulated the Introduction of New Species', The Annals and Magazine of Natural History (1855), 16, No. 93, 184.
The history of civilization proves beyond doubt just how sterile the repeated attempts of metaphysics to guess at nature’s laws have been. Instead, there is every reason to believe that when the human intellect ignores reality and concentrates within, it can no longer explain the simplest inner workings of life’s machinery or of the world around us.
From Reglas y Consejos sobre Investigacíon Cientifica: Los tónicos de la voluntad. (1897), as translated by Neely and Larry W. Swanson, in Advice for a Young Investigator (1999), 2.
The individual on his own is stable only so long as he is possessed of self-esteem. The maintenance of self-esteem is a continuous task which taxes all of the individual’s powers and inner resources. We have to prove our worth and justify our existence anew each day. When, for whatever reason, self-esteem is unattainable, the autonomous individual becomes a highly explosive entity. He turns away from an unpromising self and plunges into the pursuit of pride—the explosive substitute for self-esteem. All social disturbances and upheavals have their roots in crises of individual self-esteem, and the great endeavor in which the masses most readily unite is basically a search for pride.
In The Passionate State of Mind (1955), 18
The laws of nature, as we understand them, are the foundation of our knowledge in natural things. So much as we know of them has been developed by the successive energies of the highest intellects, exerted through many ages. After a most rigid and scrutinizing examination upon principle and trial, a definite expression has been given to them; they have become, as it were, our belief or trust. From day to day we still examine and test our expressions of them. We have no interest in their retention if erroneous. On the contrary, the greatest discovery a man could make would be to prove that one of these accepted laws was erroneous, and his greatest honour would be the discovery.
Experimental researches in chemistry and physics (1859), 469.
The limitations of archaeology are galling. It collects phenomena, but hardly ever can isolate them so as to interpret scientifically; it can frame any number of hypotheses, but rarely, if ever, scientifically prove.
In Times Literary Supplement (29 Dec 1921), 869, as cited in J.A. Macgillivray, Minotaur: Sir Arthur Evans and the Archaeology of the Minoan Myth (2000), 271.
The mathematicians have been very much absorbed with finding the general solution of algebraic equations, and several of them have tried to prove the impossibility of it. However, if I am not mistaken, they have not as yet succeeded. I therefore dare hope that the mathematicians will receive this memoir with good will, for its purpose is to fill this gap in the theory of algebraic equations.
Opening of Memoir on Algebraic Equations, Proving the Impossibility of a Solution of the General Equation of the Fifth Degree. The paper was originally published (1824) in French, as a pamphlet, in Oslo. Collected in Œuvres Complètes (1881), Vol. 1, 28. Translation by W.H. Langdon collected in David Eugene Smith, A Source Book in Mathematics (2012), 261. In this work, he showed why—despite two centuries of efforts by mathematicians—solving equations of the fifth degree would remain futile. The insights from this paper led to the modern theory of equations.
The mind can proceed only so far upon what it knows and can prove. There comes a point where the mind takes a higher plane of knowledge, but can never prove how it got there. All great discoveries have involved such a leap
As recollected from a visit some months earlier, and quoted in William Miller, 'Old Man’s Advice to Youth: “Never Lose a Holy Curiosity”', Life (2 May 1955), 64.
The motto in the pursuit of knowledge, of whatever kind, has always been, “Hope all things;—Prove all things.”
From Address (Oct 1874) delivered at Guy’s Hospital, 'On The Study of Medicine', printed in British Medical journal (1874), 2, 425. Collected in Sir William Withey Gull and Theodore Dyke Acland (ed.), A Collection of the Published Writings of William Withey Gull (1896), 5.
The oceans are the planet’s last great living wilderness, man’s only remaining frontier on Earth, and perhaps his last chance to prove himself as a rational species.
The Forests of the Sea
The one quality that seems to be so universal among eccentrics is … so subjective as to be incapable of being proved or disproved, yet … eccentrics appear to be happier than the rest of us.
From a summary his study of 1,000 people, done at Royal Edinburgh Hospital in Scotland. In David Weeks, David Joseph Weeks and Jamie James, Eccentrics (1995), 38.
The picture of scientific method drafted by modern philosophy is very different from traditional conceptions. Gone is the ideal of a universe whose course follows strict rules, a predetermined cosmos that unwinds itself like an unwinding clock. Gone is the ideal of the scientist who knows the absolute truth. The happenings of nature are like rolling dice rather than like revolving stars; they are controlled by probability laws, not by causality, and the scientist resembles a gambler more than a prophet. He can tell you only his best posits—he never knows beforehand whether they will come true. He is a better gambler, though, than the man at the green table, because his statistical methods are superior. And his goal is staked higher—the goal of foretelling the rolling dice of the cosmos. If he is asked why he follows his methods, with what title he makes his predictions, he cannot answer that he has an irrefutable knowledge of the future; he can only lay his best bets. But he can prove that they are best bets, that making them is the best he can do—and if a man does his best, what else can you ask of him?
The Rise of Scientific Philosophy (1951, 1973), 248-9. Collected in James Louis Jarrett and Sterling M. McMurrin (eds.), Contemporary Philosophy: A Book of Readings (1954), 376.
The professor may choose familiar topics as a starting point. The students collect material, work problems, observe regularities, frame hypotheses, discover and prove theorems for themselves. … the student knows what he is doing and where he is going; he is secure in his mastery of the subject, strengthened in confidence of himself. He has had the experience of discovering mathematics. He no longer thinks of mathematics as static dogma learned by rote. He sees mathematics as something growing and developing, mathematical concepts as something continually revised and enriched in the light of new knowledge. The course may have covered a very limited region, but it should leave the student ready to explore further on his own.
In A Concrete Approach to Abstract Algebra (1959), 1-2.
The profoundest facts in the earth’s history prove that the oceans have always been oceans.
In Corals and Coral Islands (1879), 371.
The proving power of the intellect or the senses was questioned by the skeptics more than two thousand years ago; but they were browbeaten into confusion by the glory of Newtonian physics.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 8.
The rainbow, “the bridge of the gods,” proved to be the bridge to our understanding of light—much more important.
Epigraph in Isaac Asimov’s Book of Science and Nature Quotations (1988), 189.
The Reason of making Experiments is, for the Discovery of the Method of Nature, in its Progress and Operations. Whosoever, therefore doth rightly make Experiments, doth design to enquire into some of these Operations; and, in order thereunto, doth consider what Circumstances and Effects, in the Experiment, will be material and instructive in that Enquiry, whether for the confirming or destroying of any preconceived Notion, or for the Limitation and Bounding thereof, either to this or that Part of the Hypothesis, by allowing a greater Latitude and Extent to one Part, and by diminishing or restraining another Part within narrower Bounds than were at first imagin'd, or hypothetically supposed. The Method therefore of making Experiments by the Royal Society I conceive should be this.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
'Dr Hooke's Method of Making Experiments' (1664-5). In W. Derham (ed.), Philosophical Experiments and Observations Of the Late Eminent Dr. Robert Hooke, F.R.S. And Geom. Prof. Gresh. and Other Eminent Virtuoso's in his Time (1726), 26-8.
The reasoning of mathematicians is founded on certain and infallible principles. Every word they use conveys a determinate idea, and by accurate definitions they excite the same ideas in the mind of the reader that were in the mind of the writer. When they have defined the terms they intend to make use of, they premise a few axioms, or self-evident principles, that every one must assent to as soon as proposed. They then take for granted certain postulates, that no one can deny them, such as, that a right line may be drawn from any given point to another, and from these plain, simple principles they have raised most astonishing speculations, and proved the extent of the human mind to be more spacious and capacious than any other science.
In Diary, Works (1850), Vol. 2, 21.
The regularity with which we conclude that further advances in a particular field are impossible seems equaled only by the regularity with which events prove that we are of too limited vision. And it always seems to be those who have the fullest opportunity to know who are the most limited in view. What, then, is the trouble? I think that one answer should be: we do not realize sufficiently that the unknown is absolutely infinite, and that new knowledge is always being produced.
Quoted in Guy Suits, 'Willis Rodney Whitney', National Academy of Sciences, Biographical Memoirs (1960), 357.
The story of a theory’s failure often strikes readers as sad and unsatisfying. Since science thrives on self-correction, we who practice this most challenging of human arts do not share such a feeling. We may be unhappy if a favored hypothesis loses or chagrined if theories that we proposed prove inadequate. But refutation almost always contains positive lessons that overwhelm disappointment, even when no new and comprehensive theory has yet filled the void.
…...
The strongest arguments prove nothing so long as the conclusions are not verified by experience. Experimental science is the queen of sciences and the goal of all speculation.
Opus Tertium. Translation as stated in Popular Science (Aug 1901), 337.
The Superfund legislation set up a system of insurance premiums collected from the chemical industry to clean up toxic wastes. This new program may prove to be as far-reaching and important as any accomplishment of my administration. The reduction of the threat to America's health and safety from thousands of toxic-waste sites will continue to be an urgent but bitterly fought issue—another example for the conflict between the public welfare and the profits of a few private despoilers of our nation's environment.
Keeping Faith: Memoirs of a President (1980), 591.
The Synthesis consists in assuming the Causes discovered and established as Principles, and by them explaining the Phænomena proceeding from them, and proving the Explanations.
From 'Query 31', Opticks (1704, 2nd ed., 1718), 380-381.
The trouble is that all the investigators proceeded in exactly the same spirit, the spirit that is of scientific curiosity, and with no possibility of telling whether the issue of their work would prove them to be fiends, or dreamers, or angels.
'The Presidential Address: Part II Science and Warfare', Reports of the British Association for the Advancement of Science (1938), 18-9.
The understanding must not however be allowed to jump and fly from particulars to axioms remote and of almost the highest generality (such as the first principles, as they are called, of arts and things), and taking stand upon them as truths that cannot be shaken, proceed to prove and frame the middle axioms by reference to them; which has been the practice hitherto, the understanding being not only carried that way by a natural impulse, but also by the use of syllogistic demonstration trained and inured to it. But then, and then only, may we hope well of the sciences when in a just scale of ascent, and by successive steps not interrupted or broken, we rise from particulars to lesser axioms; and then to middle axioms, one above the other; and last of all to the most general. For the lowest axioms differ but slightly from bare experience, while the highest and most general (which we now have) are notional and abstract and without solidity. But the middle are the true and solid and living axioms, on which depend the affairs and fortunes of men; and above them again, last of all, those which are indeed the most general; such, I mean, as are not abstract, but of which those intermediate axioms are really limitations.
The understanding must not therefore be supplied with wings, but rather hung with weights, to keep it from leaping and flying. Now this has never yet been done; when it is done, we may entertain better hopes of science.
The understanding must not therefore be supplied with wings, but rather hung with weights, to keep it from leaping and flying. Now this has never yet been done; when it is done, we may entertain better hopes of science.
From Novum Organum (1620), Book 1, Aphorism 104. Translated as The New Organon: Aphorisms Concerning the Interpretation of Nature and the Kingdom of Man), collected in James Spedding, Robert Ellis and Douglas Heath (eds.), The Works of Francis Bacon (1857), Vol. 4, 97.
The use of thesis-writing is to train the mind, or to prove that the mind has been trained; the former purpose is, I trust, promoted, the evidences of the latter are scanty and occasional.
From Preface to First Edition to Notes on the Composition of Scientific Papers (1904), v.
The vortex theory [of the atom] is only a dream. Itself unproven, it can prove nothing, and any speculations founded upon it are mere dreams about dreams.
Quoted in Henry Smith Williams, 'Some Unsolved Scientific Problems', Harper's New Monthly Magazine (1899-1900), Vol. 100, 779.
The whole history of physics proves that a new discovery is quite likely lurking at the next decimal place.
In 'The Romance of the Next Decimal Place', Science (1 Jan 1932), 75, No. 1931, 3.
The work of Planck and Einstein proved that light behaved as particles in some ways and that the ether therefore was not needed for light to travel through a vacuum. When this was done, the ether was no longer useful and it was dropped with a glad cry. The ether has never been required since. It does not exist now; in fact, it never existed.
In Asimov on Physics (1976), 85. Also in Isaac Asimov’s Book of Science and Nature Quotations (1988), 212.
Theological or anti-theological argument to prove or disprove the existence of a deity seems to me to occupy itself largely with skating among the difficulties caused by our making a fetish of this word [existence].
Swarthmore Lecture (1929) at Friends’ House, London, printed in Science and the Unseen World (1929), 69.
There is a theory that creativity arises when individuals are out of sync with their environment. To put it simply, people who fit in with their communities have insufficient motivation to risk their psyches in creating something truly new, while those who are out of sync are driven by the constant need to prove their worth.
In 'Beyond the Soapsuds Universe', Discover Magazine (1997). The author explains (in a blog found online) that this “idea comes from a theory by Mihaly Csikszentmihalyi, who wrote a book called Flow: the Psychology of Optimal Experience. You do need someone to come in from outside. One of the theories about why mathematicians do their best work when they’re young is because they’re not yet educated enough to know what seems obviously wrong. So they try new things.”
There is more evidence to prove that saltiness [of the sea] is due to the admixture of some substance ... It is this stuff which makes salt water heavy (it weighs more than fresh water) and thick. The difference in consistency is such that ships with the same cargo very nearly sink in a river when they are quite fit to navigate in the sea. This circumstance has before now caused loss to shippers freighting their ships in a river. That the thicker consistency is due to an admixture of something is proved by the fact that if you make strong brine by the admixture of salt, eggs, even when they are full, float in it. It almost becomes like mud; such a quantity of earthy matter is there in the sea.
[Aristotle recognised the different density of fresh (river) or salty (sea) water. He describes an experiment using an egg (which sinks in fresh water) that floats in a strong brine solution.]
[Aristotle recognised the different density of fresh (river) or salty (sea) water. He describes an experiment using an egg (which sinks in fresh water) that floats in a strong brine solution.]
Meteorology (350 B.C.), Book II, translated by E. W. Webster. Internet Classics Archive, (classics.mit.edu).
There is more evidence to prove that saltness [of the sea] is due to the admixture of some substance, besides that which we have adduced. Make a vessel of wax and put it in the sea, fastening its mouth in such a way as to prevent any water getting in. Then the water that percolates through the wax sides of the vessel is sweet, the earthy stuff, the admixture of which makes the water salt, being separated off as it were by a filter.
[This is an example of Aristotle giving proof by experiment, in this case, of desalination by osmosis.]
[This is an example of Aristotle giving proof by experiment, in this case, of desalination by osmosis.]
Meteorology (350 B.C.), Book II, translated by E. W. Webster. Internet Classics Archive, (classics.mit.edu).
There is no art so difficult as the art of observation: it requires a skillful, sober spirit and a well-trained experience, which can only be acquired by practice; for he is not an observer who only sees the thing before him with his eyes, but he who sees of what parts the thing consists, and in what connexion the parts stand to the whole. One person overlooks half from inattention; another relates more than he sees while he confounds it with that which he figures to himself; another sees the parts of the whole, but he throws things together that ought to be separated. ... When the observer has ascertained the foundation of a phenomenon, and he is able to associate its conditions, he then proves while he endeavours to produce the phenomena at his will, the correctness of his observations by experiment. To make a series of experiments is often to decompose an opinion into its individual parts, and to prove it by a sensible phenomenon. The naturalist makes experiments in order to exhibit a phenomenon in all its different parts. When he is able to show of a series of phenomena, that they are all operations of the same cause, he arrives at a simple expression of their significance, which, in this case, is called a Law of Nature. We speak of a simple property as a Law of Nature when it serves for the explanation of one or more natural phenomena.
'The Study of the Natural Sciences: An Introductory Lecture to the Course of Experimental Chemistry in the University of Munich, for the Winter Session of 1852-53,' as translated and republished in The Medical Times and Gazette (22 Jan 1853), N.S. Vol. 6, 82.
There is no existing ‘standard of protein intake’ that is based on the sure ground of experimental evidence. ... Between the two extremes of a very high and a very low protein intake it is difficult to prove that one level of intake is preferable to another. ... Physiologists, in drawing up dietary standards, are largely influenced by the dietary habits of their time and country.
Nutrition and Public Health', League of Nations Health Organization Quarterly Bulletin (1935) 4, 323–474. In Kenneth J. Carpenter, 'The Work of Wallace Aykroyd: International Nutritionist and Author', The Journal of Nutrition (2007), 137, 873-878.
There is one experiment which I always like to try, because it proves something whichever way it goes. A solution of iodine in water is shaken with bone-black, filtered and tested with starch paste. If the colorless solution does not turn the starch blue, the experiment shows how completely charcoal extracts iodine from aqueous solution. If the starch turns blue, the experiment shows that the solution, though apparently colorless, still contains iodine which can be detected by means of a sensitive starch test.
Applied Colloid Chemistry (1921), 111.
There may be fairies at the bottom of the garden. There is no evidence of it, but you can’t prove that there aren’t any so shouldn’t we be agnostic with respect to fairies? The trouble with the agnostic argument is that it can be applied to anything. There is an infinite number of hypothetical beliefs we could hold which we can’t positively disprove. On the whole, people don’t believe in most of them, such as fairies, unicorns, dragons, Father Christmas, and so on. But on the whole they do believe in a creator God, together with whatever particular baggage goes with the religion of their parents.
From speech at the Edinburgh International Science Festival (15 Apr 1992), published in the Independent newspaper. Included in excerpt in Alec Fisher, The Logic of Real Arguments (2004), 84. The full speech was reprinted in The Nullifidian, (Dec 1994). Transcribed online in the Richard Dawkins archive, article 89, titled: Lecture from 'The Nullifidian' (Dec 94).
Things sweet to taste prove in digestion sour.
Louis Klopsch, Many Thoughts of Many Minds (1896), 67.
This alleged damage which the small radioactivity is causing—supposedly cancer and leukemia—has not been proved, to the best of my knowledge, by decent and clear statistics. It is possible that there is damage. It is even possible, to my mind, that there is no damage; and there is the possibility, further, that very small amounts of radioactivity are helpful.
From debate (20 Feb 1958) between Linus Pauling and Edward Teller on WQED-TV, San Francisco. Transcript published as Fallout and Disarmament: The Pauling-Teller Debate (1958). Reprinted in 'Fallout and Disarmament: A Debate between Linus Pauling and Edward Teller', Daedalus (Spring 1958), 87, No. 2, 155.
This new integral of Lebesgue is proving itself a wonderful tool. I might compare it with a modern Krupp gun, so easily does it penetrate barriers which were impregnable.
In 'Current Tendencies of Mathematical Research', Bulletin of the American Mathematical Society (Oct 1916), 23, 7. Previously given as an address read at the Quarter Centennial of the University of Chicago, before a conference of the mathematical, physical and astronomical departments.
Those who work standing ... carpenters, sawyers, carvers, blacksmiths, masons ... are liable to varicose veins ... [because] the strain on the muscles is such that the circulation of the blood is retarded. Standing even for a short time proves exhausting compared with walking and running though it be for a long time ... Nature delights and is restored by alternating and varied actions.
Thus, be it understood, to demonstrate a theorem, it is neither necessary nor even advantageous to know what it means. The geometer might be replaced by the logic piano imagined by Stanley Jevons; or, if you choose, a machine might be imagined where the assumptions were put in at one end, while the theorems came out at the other, like the legendary Chicago machine where the pigs go in alive and come out transformed into hams and sausages. No more than these machines need the mathematician know what he does.
From 'Les Mathématiques et la Logique', Science et Méthode (1908, 1920), Livre 2, Chap. 3, Sec. 2, 157. English as in Henri Poincaré and George Bruce Halsted (trans.), 'Mathematics and Logic', Science and Method collected in The Foundations of Science: Science and Hypothesis, The Value of Science, Science and Method (1913), 451. From the French, “Ainsi, c’est bien entendu, pour démontrer un théorème, il n’est pas nécessaire ni même utile de savoir ce qu’il veut dire. On pourrait remplacer le géomètre par le piano à raisonner imaginé par Stanley Jevons; ou, si l’on aime mieux, on pourrait imaginer une machine où l’on introduirait les axiomes par un bout pendant qu’on recueillerait les théorèmes à l’autre bout, comme cette machine légendaire de Chicago où les porcs entrent vivants et d’où ils sortent transformés en jambons et en saucisses. Pas plus que ces machines, le mathématicien n’a besoin de comprendre ce qu’il fait”.
To illustrate the apparent contrast between statistics and truth … may I quote a remark I once overheard: “There are three kinds of lies: white lies, which are justifiable; common lies—these have no justification; and statistics.” Our meaning is similar when we say: “Anything can be proved by figures”; or, modifying a well-known quotation from Goethe, with numbers “all men may contend their charming systems to defend.”
In Probability, Statistics, and Truth (1939), 1.
To prove that tuberculosis is caused by the invasion of bacilli, and that it is a parasitic disease primarily caused by the growth and multiplication of bacilli, it is necessary to isolate the bacilli from the body, to grow them in pure culture until they are freed from every disease product of the animal organism, and, by introducing isolated bacilli into animals, to reproduce the same morbid condition that is known to follow from inoculation with spontaneously developed tuberculous material.
'The Etiology of Tuberculosis' (1882), Essays of Robert Koch (1987), trans. K. Codell Carter, 87.
To prove to an indignant questioner on the spur of the moment that the work I do was useful seemed a thankless task and I gave it up. I turned to him with a smile and finished, “To tell you the truth we don’t do it because it is useful but because it’s amusing.” The answer was thought of and given in a moment: it came from deep down in my soul, and the results were as admirable from my point of view as unexpected. My audience was clearly on my side. Prolonged and hearty applause greeted my confession. My questioner retired shaking his head over my wickedness and the newspapers next day, with obvious approval, came out with headlines “Scientist Does It Because It’s Amusing!” And if that is not the best reason why a scientist should do his work, I want to know what is. Would it be any good to ask a mother what practical use her baby is? That, as I say, was the first evening I ever spent in the United States and from that moment I felt at home. I realised that all talk about science purely for its practical and wealth-producing results is as idle in this country as in England. Practical results will follow right enough. No real knowledge is sterile. The most useless investigation may prove to have the most startling practical importance: Wireless telegraphy might not yet have come if Clerk Maxwell had been drawn away from his obviously “useless” equations to do something of more practical importance. Large branches of chemistry would have remained obscure had Willard Gibbs not spent his time at mathematical calculations which only about two men of his generation could understand. With this faith in the ultimate usefulness of all real knowledge a man may proceed to devote himself to a study of first causes without apology, and without hope of immediate return.
From lecture to a scientific society in Philadelphia on “The Mechanism of the Muscle” given by invitation after he received a Nobel Prize for that work. The quote is Hill’s response to a post-talk audience question asking disapprovingly what practical use the speaker thought there was in his research. The above quoted answer, in brief, is—for the intellectual curiosity. As quoted about Hill by Bernard Katz in his own autobiographical chapter, 'Sir Bernard Katz', collected in Larry R. Squire (ed.), The History of Neuroscience in Autobiography (1996), Vol. 1, 350-351. Two excerpts from the above have been highlighted as standalone quotes here in this same quote collection for A. V. Hill. They begin “All talk about science…” and “The most useless investigation may prove…”.
True science and true religion are twin sisters, and the separation of either from the other is sure to prove the death of both. Science prospers exactly in proportion as it is religious; and religion flourishes in exact proportion to the scientific depth and firmness of its basis.
As quoted from the close of a recent lecture by Huxley in 'What Knowledge is of Most Worth'. Lectures in Education, by Herbert Spencer, delivered at the Royal Institution (1855). In The Westminster Review (Jul 1859), 22. Collected in Essays on Education and Kindred Subjects (1911), 41.
We cannot … prove geometrical truths by arithmetic.
In Posterior Analytics.
We know that there exist true propositions which we can never formally prove. What about propositions whose proofs require arguments beyond our capabilities? What about propositions whose proofs require millions of pages? Or a million, million pages? Are there proofs that are possible, but beyond us?
Mathematical Mysteries (1999), 295.
We may summarize … the fundamental characteristics and limitations of mathematics as follows: mathematics is ultimately an experimental science, for freedom from contradiction cannot be proved, but only postulated and checked by observation, and similarly existence can only be postulated and checked by observation. Furthermore, mathematics requires the fundamental device of all thought, of analyzing experience into static bits with static meanings.
In The Nature of Physical Theory (1936), 58.
We must make the following remark: a proof, that after a certain time t1, the spheres must necessarily be mixed uniformly, whatever may be the initial distribution of states, cannot be given. This is in fact a consequence of probability theory, for any non-uniform distribution of states, no matter how improbable it may be, is still not absolutely impossible. Indeed it is clear that any individual uniform distribution, which might arise after a certain time from some particular initial state, is just as improbable as an individual non-uniform distribution; just as in the game of Lotto, any individual set of five numbers is as improbable as the set 1, 2, 3, 4, 5. It is only because there are many more uniform distributions than non-uniform ones that the distribution of states will become uniform in the course of time. One therefore cannot prove that, whatever may be the positions and velocities of the spheres at the beginning, the distributions must become uniform after a long time; rather one can only prove that infinitely many more initial states will lead to a uniform one after a definite length of time than to a non-uniform one. Loschmidt's theorem tells us only about initial states which actually lead to a very non-uniform distribution of states after a certain time t1; but it does not prove that there are not infinitely many more initial conditions that will lead to a uniform distribution after the same time. On the contrary, it follows from the theorem itself that, since there are infinitely many more uniform distributions, the number of states which lead to uniform distributions after a certain time t1, is much greater than the number that leads to non-uniform ones, and the latter are the ones that must be chosen, according to Loschmidt, in order to obtain a non-uniform distribution at t1.
From 'On the Relation of a General Mechanical Theorem to the Second Law of Thermodynamics' (1877), in Stephen G. Brush (ed.), Selected Readings in Physics (1966), Vol. 2, Irreversible Processes, 191-2.
We must not forget that when radium was discovered no one knew that it would prove useful in hospitals. The work was one of pure science. And this is a proof that scientific work must not be considered from the point of view of the direct usefulness of it. It must be done for itself, for the beauty of science, and then there is always the chance that a scientific discovery may become like the radium a benefit for humanity.
Lecture at Vassar College, Poughkeepsie, New York (14 May 1921). In Cambridge Editorial Partnership, Speeches that Changed the World, 53.
We must reject the false choice between combating climate change and fostering strong economic growth. If any country can prove that, it’s the United States.
In Hillary Clinton, 'Hillary Clinton: America Must Lead at Paris Climate Talks', Time (29 Nov 2015).
We must somehow keep the dreams of space exploration alive, for in the long run they will prove to be of far more importance to the human race than the attainment of material benefits. Like Darwin, we have set sail upon an ocean: the cosmic sea of the Universe. There can be no turning back. To do so could well prove to be a guarantee of extinction. When a nation, or a race or a planet turns its back on the future, to concentrate on the present, it cannot see what lies ahead. It can neither plan nor prepare for the future, and thus discards the vital opportunity for determining its evolutionary heritage and perhaps its survival.
…...
We should not argue with the blind man who maintained that sight was an illusion to which some abnormal people were subject. Therefore in speaking of religious experience I do not attempt to prove the existence of religious experience…
Swarthmore Lecture (1929) at Friends’ House, London, printed in Science and the Unseen World (1929), 48-49.
WEATHER, n. The climate of an hour. A permanent topic of conversation among persons whom it does not interest, but who have inherited the tendency to chatter about it from naked arboreal ancestors whom it keenly concerned. The setting up of official weather bureaus and their maintenance in mendacity prove that even governments are accessible to suasion by the rude forefathers of the jungle.
The Collected Works of Ambrose Bierce (1911), Vol. 7, The Devil's Dictionary, 362-363.
What I have done is to show that it is possible for the way the universe began to be determined by the laws of science. In that case, it would not be necessary to appeal to God to decide how the universe began. This doesn't prove that there is no God, only that God is not necessary. (17 Oct 1988)
Der Spiegel (17 Oct 1988). Quoted in Clifford A. Pickover, Archimedes to Hawking (2008), 483.
What is now proved was once only imagin’d.
In 'Proverbs', The Poems: With Specimens of the Prose Writings of William Blake (1885), 280.
What is possible can never be demonstrated to be false; and 'tis possible the course of nature may change, since we can conceive such a change. Nay, I will go farther, and assert, that he could not so much as prove by any probable arguments, that the future must be conformable to the past. All probable arguments are built on the supposition, that there is this conformity betwixt the future and the past, and therefore can never prove it. This conformity is a matter of fact, and if it must be proved, will admit of no proof but from experience. But our experience in the past can be a proof of nothing for the future, but upon a supposition, that there is a resemblance betwixt them. This therefore is a point, which can admit of no proof at all, and which we take for granted without any proof.
An Abstract of A Treatise on Human Nature (1740), ed. John Maynard Keynes and Piero Sraffa (1938), 15.
What is truth ? A man may prove much that has no other truth but in him, and all be a turnip lantern leading to a precipice over the sea.
In Sir William Withey Gull and Theodore Dyke Acland (ed.), A Collection of the Published Writings of William Withey Gull (1896), li.
What vexes me most is, that my female friends, who could bear me very well a dozen years ago, have now forsaken me, although I am not so old in proportion to them as I formerly was: which I can prove by arithmetic, for then I was double their age, which now I am not.
In Letter (7 Feb 1736) to Alexander Pope, The Works of Jonathan Swift (1841), Vol. 2, 764.
When asked what it was like to set about proving something, the mathematician likened proving a theorem to seeing the peak of a mountain and trying to climb to the top. One establishes a base camp and begins scaling the mountain’s sheer face, encountering obstacles at every turn, often retracing one’s steps and struggling every foot of the journey. Finally when the top is reached, one stands examining the peak, taking in the view of the surrounding countryside and then noting the automobile road up the other side!
Space-filler in The Two-Year College Mathematics Journal (Nov 1980), 11, No. 5, 295.
While seeing any number of black crows does not prove all the crows are black, seeing one white crow disproves it. Thus science proceeds not by proving models correct but by discarding false ones or improving incomplete ones.
In 'On the Nature of Science', Physics in Canada (Jan/Feb 2007), 63, No. 1, 7.
Whoever … proves his point and demonstrates the prime truth geometrically should be believed by all the world, for there we are captured.
In J. Heidrich (ed.), 'Von Menschlicher Proportion', Albrecht Dürer’s Schriftlicher Nachlass (1920), 270. From the original German: “Welcher aber … durch die Geometria sein Ding beweist und die gründliche Wahrheit anzeigt, dem soll alle Welt glauben. Denn da ist man gefangen.”
With crystals we are in a situation similar to an attempt to investigate an optical grating merely from the spectra it produces... But a knowledge of the positions and intensities of the spectra does not suffice for the determination of the structure. The phases with which the diffracted waves vibrate relative to one another enter in an essential way. To determine a crystal structure on the atomic scale, one must know the phase differences between the different interference spots on the photographic plate, and this task may certainly prove to be rather difficult.
Physikalische Zeitschrift (1913), 14. Translated in Walter Moore, Schrödinger. Life and Thought (1989), 73.
X-rays will prove to be a hoax.
In Marc J. Madou, Fundamentals of Microfabrication: the Science of Miniaturization (2nd ed., 2002), 325.
You can hardly convince a man of error in a life-time, but must content yourself with the reflection that the progress of science is slow. If he is not convinced, his grand-children may be. The geologists tell us that it took one hundred years to prove that fossils are organic, and one hundred and fifty more, to prove that they are not to be referred to the Noachian deluge.
In A Week on the Concord and Merrimack Rivers (1862), 68.
You have heard of the new chemical nomenclature endeavored to be introduced by Lavoisier, Fourcroy, &c. Other chemists of this country, of equal note, reject it, and prove in my opinion that it is premature, insufficient and false. These latter are joined by the British chemists; and upon the whole, I think the new nomenclature will be rejected, after doing more harm than good. There are some good publications in it, which must be translated into the ordinary chemical language before they will be useful.
Letter to Dr. Currie (Paris, 1788). In Thomas Jefferson and John P. Foley (ed.), The Jeffersonian Cyclopedia (1900), 135. From H.A. Washington, The Writings of Thomas Jefferson (1853-54). Vol 2, 544.
You Surgeons of London, who puzzle your Pates,
To ride in your Coaches, and purchase Estates,
Give over, for Shame, for your Pride has a Fall,
And ye Doctress of Epsom has outdone you all.
Dame Nature has given her a doctor's degree,
She gets all the patients and pockets the fee;
So if you don't instantly prove it a cheat,
She'll loll in a chariot whilst you walk the street.
Cautioning doctors about the quack bone-setter, Mrs. Mapp (d. 22 Dec 1737), who practiced in Epsom town once a week, arriving in a coach-and-four.
To ride in your Coaches, and purchase Estates,
Give over, for Shame, for your Pride has a Fall,
And ye Doctress of Epsom has outdone you all.
Dame Nature has given her a doctor's degree,
She gets all the patients and pockets the fee;
So if you don't instantly prove it a cheat,
She'll loll in a chariot whilst you walk the street.
Cautioning doctors about the quack bone-setter, Mrs. Mapp (d. 22 Dec 1737), who practiced in Epsom town once a week, arriving in a coach-and-four.
Verses from a song in a comedy at the Lincoln's Inn Fields Theatre, called The Husband's Relief, or The Female Bone-setter and the Worm-doctor. In Robert Chambers, The Book of Days (1832), 729.
Young men should prove theorems, old men should write books.
Quoted by Freeman Dyson as the answer from G.H. Hardy about the book An Introduction to the Theory of Numbers. Dyson, while at Cambridge, had asked him why “he spent so much time and effort writing that marvellous book when he might be doing serious mathematics.” In Freeman Dyson 'A Walk Through Ramanujan's Garden', Lecture by Dyson at Ramanujan Centenary Conference (2 Jun 1987). Collected in Selected Papers of Freeman Dyson with Commentary (1996), 189. Also as quoted in 'Mathematician, Physicist, and Writer.' Interview (Jun 1990) with Donald J. Albers, The College Mathematics Journal (Jan 1994), 25, No. 1, 4.

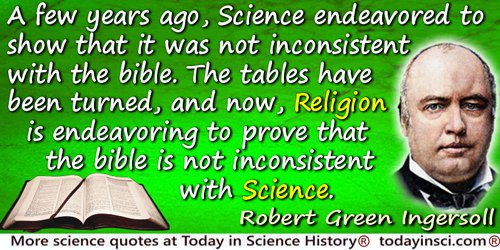











 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


