Solution Quotes (282 quotes)
“Yes,” he said. “But these things (the solutions to problems in solid geometry such as the duplication of the cube) do not seem to have been discovered yet.” “There are two reasons for this,” I said. “Because no city holds these things in honour, they are investigated in a feeble way, since they are difficult; and the investigators need an overseer, since they will not find the solutions without one. First, it is hard to get such an overseer, and second, even if one did, as things are now those who investigate these things would not obey him, because of their arrogance. If however a whole city, which did hold these things in honour, were to oversee them communally, the investigators would be obedient, and when these problems were investigated continually and with eagerness, their solutions would become apparent.”
— Plato
In The Republic 7 528bc, trans. R.W. Sharples.
[A scientist] naturally and inevitably … mulls over the data and guesses at a solution. [He proceeds to] testing of the guess by new data—predicting the consequences of the guess and then dispassionately inquiring whether or not the predictions are verified.
From manuscript on Francis Bacon as a scientist (1942), Edwin Hubble collection, Box 2, Huntington Library, San Marino, California. As cited by Norriss S. Hetherington in 'Philosophical Values and Observation in Edwin Hubble's Choice of a Model of the Universe', Historical Studies in the Physical Sciences (1982), 13, No. 1, 42.
[Certain students] suppose that because science has penetrated the structure of the atom it can solve all the problems of the universe. ... They are known in every ... college as the most insufferable, cocksure know-it-alls. If you want to talk to them about poetry, they are likely to reply that the "emotive response" to poetry is only a conditioned reflex .... If they go on to be professional scientists, their sharp corners are rubbed down, but they undergo no fundamental change. They most decidedly are not set apart from the others by their intellectual integrity and faith, and their patient humility in front of the facts of nature.... They are uneducated, in the fullest sense of the word, and they certainly are no advertisement for the claims of science teachers.
In Science is a Sacred Cow (1950), 18-19.
[O]ur own existence once presented the greatest of all mysteries, but … it is a mystery no longer because it is solved. Darwin and Wallace solved it … I was surprised that so many people seemed not only unaware of the elegant and beautiful solution to this deepest of problems but, incredibly, in many cases actually unaware that there was a problem in the first place!
The Blind Watchmaker (1996), front matter.
[The religion of science was] an implicit faith that by the methods of physical science, and by these methods alone, could be solved all the problems arising out of the relation of man to man and of man towards the universe.
In My Apprenticeship (1926), 89.
Die nicht wãsserigen Losungen leiten ja nicht.
Non-aqueous solutions don't conduct.
Non-aqueous solutions don't conduct.
Translation by W. H. Brock of Ostwald inZeitschrift für Physikalische Chemie (1901), 5, 341.
Douter de tout ou tout croire, ce sont deux solutions également commodes, qui l’une et l’autre nous dispensent de défléchir.
To doubt everything and to believe everything are two equally convenient solutions; each saves us from thinking.
To doubt everything and to believe everything are two equally convenient solutions; each saves us from thinking.
From 'Introduction', La Science et l’Hypothèse (1902), 2. Translation by George Bruce Halsted, 'Introduction', Science and Hypothesis (New York, 1905), 1. In 'Author’s Preface', Science and Hypothesis (London 1905), xxii, it is translated more closely as “To doubt everything and to believe everything are two equally convenient solutions; both dispense with the necessity of reflection.”
Il est impossible de contempler le spectacle de l’univers étoilé sans se demander comment il s’est formé: nous devions peut-être attendre pour chercher une solution que nous ayons patiemment rassemblé les éléments …mais si nous étions si raisonnables, si nous étions curieux sans impatience, il est probable que nous n’avions jamais créé la Science et que nous nous serions toujours contentés de vivre notre petite vie. Notre esprit a donc reclamé impérieusement cette solution bien avant qu’elle fut mûre, et alors qu’il ne possédait que de vagues lueurs, lui permettant de la deviner plutôt que de l’attendre.
It is impossible to contemplate the spectacle of the starry universe without wondering how it was formed: perhaps we ought to wait, and not look for a solution until have patiently assembled the elements … but if we were so reasonable, if we were curious without impatience, it is probable we would never have created Science and we would always have been content with a trivial existence. Thus the mind has imperiously laid claim to this solution long before it was ripe, even while perceived in only faint glimmers—allowing us to guess a solution rather than wait for it.
It is impossible to contemplate the spectacle of the starry universe without wondering how it was formed: perhaps we ought to wait, and not look for a solution until have patiently assembled the elements … but if we were so reasonable, if we were curious without impatience, it is probable we would never have created Science and we would always have been content with a trivial existence. Thus the mind has imperiously laid claim to this solution long before it was ripe, even while perceived in only faint glimmers—allowing us to guess a solution rather than wait for it.
From Leçons sur les Hypothèses Consmogoniques (1913) as cited in D. Ter Haar and A.G.W. Cameron, 'Historical Review of Theories of the Origin of the Solar System', collected in Robert Jastrow and A. G. W. Cameron (eds.), Origin of the Solar System: Proceedings of a Conference Held at the Goddard Institute for Space Studies, New York, January 23-24, 1962, (1963), 3.
'Cosmogonical Hypotheses' (1913), collected in Harlow Shapley, Source Book in Astronomy, 1900-1950 (1960), 347.
Les mathématiciens parviennent à la solution d’un problême par le simple arrangement des données, & en réduisant le raisonnement à des opérations si simples, à des jugemens si courts, qu’ils ne perdent jamais de vue l’évidence qui leur sert de guide.
Mathematicians come to the solution of a problem by the simple arrangement of the data, and reducing the reasoning to such simple operations, to judgments so brief, that they never lose sight of the evidence that serves as their guide.
Mathematicians come to the solution of a problem by the simple arrangement of the data, and reducing the reasoning to such simple operations, to judgments so brief, that they never lose sight of the evidence that serves as their guide.
From a paper read to the Académie Royales des Sciences (18 Apr 1787), printed in Méthode de Nomenclature Chimique (1787), 12. Translation from the French by Webmaster.
Neumann, to a physicist seeking help with a difficult problem: Simple. This can be solved by using the method of characteristics.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Attributed, as related by Dr. Felix Smith (Head of Molecular Physics, Stanford Research Institute) to author Gary Zukav, who quoted it in The Dancing Wu Li Masters: An Overview of the New Physics (1979, 2001), 208, footnote. The physicist (a friend of Dr. Smith) worked at Los Alamos after WW II. It should be noted that although the author uses quotation marks around the spoken remarks, that they represent the author's memory of Dr. Smith's recollection, who heard it from the physicist. Therefore the fourth-hand wording is very likely not verbatim. Webmaster finds Zukav's book seems to be the only source for this quote.
Quand les physiciens nous demandent la solution d'un problème, ce n'est pas une corvée qu'ils nous impsent, c'est nous au contraire qui leur doivent des remercîments.
When the physicists ask us for the solution of a problem, it is not drudgery that they impose on us, on the contrary, it is us who owe them thanks.
When the physicists ask us for the solution of a problem, it is not drudgery that they impose on us, on the contrary, it is us who owe them thanks.
La valeur de la science. In Anton Bovier, Statistical Mechanics of Disordered Systems (2006), 111.
1839—The fermentation satire
THE MYSTERY OF ALCOHOLIC FERMENTATION RESOLVED
(Preliminary Report by Letter) Schwindler
I am about to develop a new theory of wine fermentation … Depending on the weight, these seeds carry fermentation to completion somewhat less than as in the beginning, which is understandable … I shall develop a new theory of wine fermentation [showing] what simple means Nature employs in creating the most amazing phenomena. I owe it to the use of an excellent microscope designed by Pistorius.
When brewer’s yeast is mixed with water the microscope reveals that the yeast dissolves into endless small balls, which are scarcely 1/800th of a line in diameter … If these small balls are placed in sugar water, it can be seen that they consist of the eggs of animals. As they expand, they burst, and from them develop small creatures that multiply with unbelievable rapidity in a most unheard of way. The form of these animals differs from all of the 600 types described up until now. They possess the shape of a Beinsdorff still (without the cooling apparatus). The head of the tube is a sort of proboscis, the inside of which is filled with fine bristles 1/2000th of a line long. Teeth and eyes are not discernible; however, a stomach, intestinal canal, anus (a rose red dot), and organs for secretion of urine are plainly discernible. From the moment they are released from the egg one can see these animals swallow the sugar from the solution and pass it to the stomach. It is digested immediately, a process recognized easily by the resultant evacuation of excrements. In a word, these infusors eat sugar, evacuate ethyl alcohol from the intestinal canal, and carbon dioxide from the urinary organs. The bladder, in the filled state, has the form of a champagne bottle; when empty, it is a small button … As soon as the animals find no more sugar present, they eat each other up, which occurs through a peculiar manipulation; everything is digested down to the eggs which pass unchanged through the intestinal canal. Finally, one again fermentable yeast, namely the seed of the animals, which remain over.
THE MYSTERY OF ALCOHOLIC FERMENTATION RESOLVED
(Preliminary Report by Letter) Schwindler
I am about to develop a new theory of wine fermentation … Depending on the weight, these seeds carry fermentation to completion somewhat less than as in the beginning, which is understandable … I shall develop a new theory of wine fermentation [showing] what simple means Nature employs in creating the most amazing phenomena. I owe it to the use of an excellent microscope designed by Pistorius.
When brewer’s yeast is mixed with water the microscope reveals that the yeast dissolves into endless small balls, which are scarcely 1/800th of a line in diameter … If these small balls are placed in sugar water, it can be seen that they consist of the eggs of animals. As they expand, they burst, and from them develop small creatures that multiply with unbelievable rapidity in a most unheard of way. The form of these animals differs from all of the 600 types described up until now. They possess the shape of a Beinsdorff still (without the cooling apparatus). The head of the tube is a sort of proboscis, the inside of which is filled with fine bristles 1/2000th of a line long. Teeth and eyes are not discernible; however, a stomach, intestinal canal, anus (a rose red dot), and organs for secretion of urine are plainly discernible. From the moment they are released from the egg one can see these animals swallow the sugar from the solution and pass it to the stomach. It is digested immediately, a process recognized easily by the resultant evacuation of excrements. In a word, these infusors eat sugar, evacuate ethyl alcohol from the intestinal canal, and carbon dioxide from the urinary organs. The bladder, in the filled state, has the form of a champagne bottle; when empty, it is a small button … As soon as the animals find no more sugar present, they eat each other up, which occurs through a peculiar manipulation; everything is digested down to the eggs which pass unchanged through the intestinal canal. Finally, one again fermentable yeast, namely the seed of the animals, which remain over.
In 'Das entriithselle Geheimiss der geisligen Giihrung', Annalen der Pharmacie und Chemie (1839), 29, 100-104; adapted from English translalion by Ralph E. Oesper, The Human Side of Scientists (1975), 203-205.
A cosmic mystery of immense proportions, once seemingly on the verge of solution, has deepened and left astronomers and astrophysicists more baffled than ever. The crux ... is that the vast majority of the mass of the universe seems to be missing.
[Reporting a Nature article discrediting explanation of invisible mass being due to neutrinos]
[Reporting a Nature article discrediting explanation of invisible mass being due to neutrinos]
In 'If Theory is Right, Most of Universe is Still “Missing”', New York Times (11 Sep 1984).
A great discovery solves a great problem, but there is a grain of discovery in the solution of any problem. Your problem may be modest, but if it challenges your curiosity and brings into play your inventive faculties, and if you solve it by your own means, you may experience the tension and enjoy the triumph of discovery.
From Preface to the first printing, reprinted in How to Solve It: A New Aspect of Mathematical Method (2004), v.
A human being should be able to change a diaper, plan an invasion, butcher a hog, conn a ship, design a building, write a sonnet, balance accounts, build a wall, set a bone, comfort the dying, take orders, give orders, cooperate, act alone, solve equations, analyze a new problem, pitch manure, program a computer, cook a tasty meal, fight efficiently, die gallantly. Specialization is for insects.
In Time Enough for Love: The Lives of Lazarus Long (1973), 265.
A mathematical problem should be difficult in order to entice us, yet not completely inaccessible, lest it mock at our efforts. It should be to us a guide post on the mazy paths to hidden truths, and ultimately a reminder of our pleasure in the successful solution.
In Mathematical Problems', Bulletin American Mathematical Society, 8, 438.
A modern branch of mathematics, having achieved the art of dealing with the infinitely small, can now yield solutions in other more complex problems of motion, which used to appear insoluble. This modern branch of mathematics, unknown to the ancients, when dealing with problems of motion, admits the conception of the infinitely small, and so conforms to the chief condition of motion (absolute continuity) and thereby corrects the inevitable error which the human mind cannot avoid when dealing with separate elements of motion instead of examining continuous motion. In seeking the laws of historical movement just the same thing happens. The movement of humanity, arising as it does from innumerable human wills, is continuous. To understand the laws of this continuous movement is the aim of history. … Only by taking an infinitesimally small unit for observation (the differential of history, that is, the individual tendencies of man) and attaining to the art of integrating them (that is, finding the sum of these infinitesimals) can we hope to arrive at the laws of history.
War and Peace (1869), Book 11, Chap. 1.
A problem well stated is a problem half-solved.
A scientist works largely by intuition. Given enough experience, a scientist examining a problem can leap to an intuition as to what the solution ‘should look like.’ ... Science is ultimately based on insight, not logic.
…...
A time will come, when fields will be manured with a solution of glass (silicate of potash), with the ashes of burnt straw, and with the salts of phosphoric acid, prepared in chemical manufactories, exactly as at present medicines are given for fever and goitre.
Agricultural Chemistry (1847), 4th edn., 186.
A troubling question for those of us committed to the widest application of intelligence in the study and solution of the problems of men is whether a general understanding of the social sciences will be possible much longer. Many significant areas of these disciplines have already been removed by the advances of the past two decades beyond the reach of anyone who does not know mathematics; and the man of letters is increasingly finding, to his dismay, that the study of mankind proper is passing from his hands to those of technicians and specialists. The aesthetic effect is admittedly bad: we have given up the belletristic “essay on man” for the barbarisms of a technical vocabulary, or at best the forbidding elegance of mathematical syntax.
Opening paragraph of 'The Study of Man: Sociology Learns the Language of Mathematics' in Commentary (1 Sep 1952). Reprinted in James Roy Newman, The World of Mathematics (1956), Vol. 2, 1294.
After Darwin had conceived the basic idea of evolution, … there was still one important point not accounted for, namely, the tendency in organic beings descended from the same stock to diverge as they become modified. … “I can remember the very spot in the road, whilst in my carriage, when to my joy the solution occurred to me.”
In The Art of Scientific Investigation (1950), 69.
After the discovery of spectral analysis no one trained in physics could doubt the problem of the atom would be solved when physicists had learned to understand the language of spectra. So manifold was the enormous amount of material that has been accumulated in sixty years of spectroscopic research that it seemed at first beyond the possibility of disentanglement. An almost greater enlightenment has resulted from the seven years of Röntgen spectroscopy, inasmuch as it has attacked the problem of the atom at its very root, and illuminates the interior. What we are nowadays hearing of the language of spectra is a true 'music of the spheres' in order and harmony that becomes ever more perfect in spite of the manifold variety. The theory of spectral lines will bear the name of Bohr for all time. But yet another name will be permanently associated with it, that of Planck. All integral laws of spectral lines and of atomic theory spring originally from the quantum theory. It is the mysterious organon on which Nature plays her music of the spectra, and according to the rhythm of which she regulates the structure of the atoms and nuclei.
Atombau und Spektrallinien (1919), viii, Atomic Structure and Spectral Lines, trans. Henry L. Brose (1923), viii.
Again and again in reading even his [William Thomson] most abstract writings one is struck by the tenacity with which physical ideas control in him the mathematical form in which he expressed them. An instance of this is afforded by … an example of a mathematical result that is, in his own words, “not instantly obvious from the analytical form of my solution, but which we immediately see must be the case by thinking of the physical meaning of the result.”
As given in Life of Lord Kelvin (1910), Vol. 2, 1136. The ellipsis gives the reference to the quoted footnote, to a passage in his Mathematical and Physical Papers, Vol. 1, 457. [Note: William Thomson, later became Lord Kelvin. —Webmaster]
All that can be said upon the number and nature of elements is, in my opinion, confined to discussions entirely of a metaphysical nature. The subject only furnishes us with indefinite problems, which may be solved in a thousand different ways, not one of which, in all probability, is consistent with nature. I shall therefore only add upon this subject, that if, by the term elements, we mean to express those simple and indivisible atoms of which matter is composed, it is extremely probable we know nothing at all about them; but, if we apply the term elements, or principles of bodies, to express our idea of the last point which analysis is capable of reaching, we must admit, as elements, all the substances into which we are capable, by any means, to reduce bodies by decomposition.
Elements of Chemistry (1790), trans. R. Kerr, Preface, xxiv.
Ammonia is furnished from all animal substances by decomposition. The horns of cattle, especially those of deer, yield it in abundance, and it is from this circumstance that a solution of ammonia in water has been termed hartshorn.
From 'Artist and Mechanic', The artist & Tradesman’s Guide: embracing some leading facts & principles of science, and a variety of matter adapted to the wants of the artist, mechanic, manufacturer, and mercantile community (1827), 14.
An undefined problem has an infinite number of solutions.
…...
And by the influence of heat, light, and electrical powers, there is a constant series of changes [in animal and vegetal substances]; matter assumes new forms, the destruction of one order of beings tends to the conservation of another, solution and consolidation, decay and renovation, are connected, and whilst the parts of the system, continue in a state of fluctuation and change, the order and harmony of the whole remain unalterable.
The Elements of Agricultural Chemistry (1813), in J. Davy (ed.) The Collected Works of Sir Humphry Davy(1839-40), Vol 7, 182.
And do you know what “the world” is to me? Shall I show it to you in my mirror? This world: a monster of energy, without beginning, without end; a firm, iron magnitude of force that does not grow bigger or smaller, that does not expend itself but only transforms itself; as a whole, of unalterable size, a household without expenses or losses, but likewise without increase or income; enclosed by “nothingness”' as by a boundary; not by something blurry or wasted, not something endlessly extended, but set in a definite space as a definite force, and not a space that might be “empty” here or there, but rather as force throughout, as a play of forces and waves of forces, at the same time one and many, increasing here and at the same time decreasing there; a sea of forces flowing and rushing together, eternally changing, eternally flooding back, with tremendous years of recurrence, with an ebb and a flood of its forms; out of the simplest forms striving toward the most complex, out of the stillest, most rigid, coldest forms toward the hottest, most turbulent, most self-contradictory, and then again returning home to the simple out of this abundance, out of the play of contradictions back to the joy of concord, still affirming itself in this uniformity of its courses and its years, blessing itself as that which must return eternally, as a becoming that knows no satiety, no disgust, no weariness: this, my Dionysian world of the eternally self-creating, the eternally self-destroying, this mystery world of the twofold voluptuous delight, my “beyond good and evil,” without goal, unless the joy of the circle itself is a goal; without will, unless a ring feels good will toward itself-do you want a name for this world? A solution for all its riddles? A light for you, too, you best-concealed, strongest, most intrepid, most midnightly men?—This world is the will to power—and nothing besides! And you yourselves are also this will to power—and nothing besides!
The Will to Power (Notes written 1883-1888), book 4, no. 1067. Trans. W. Kaufmann and R. J. Hollingdale and ed. W. Kaufmann (1968), 549-50.
Any problem can be solved using the materials in the room.
In Peter C. Wensberg, Land's Polaroid: A Company and the Man Who Invented It (1987).
As he [Clifford] spoke he appeared not to be working out a question, but simply telling what he saw. Without any diagram or symbolic aid he described the geometrical conditions on which the solution depended, and they seemed to stand out visibly in space. There were no longer consequences to be deduced, but real and evident facts which only required to be seen. … So whole and complete was his vision that for the time the only strange thing was that anybody should fail to see it in the same way. When one endeavored to call it up again, and not till then, it became clear that the magic of genius had been at work, and that the common sight had been raised to that higher perception by the power that makes and transforms ideas, the conquering and masterful quality of the human mind which Goethe called in one word das Dämonische.
In Leslie Stephen and Frederick Pollock (eds.), Lectures and Essays by William Kingdon Clifford(1879), Vol. 1, Introduction, 4-5.
As soon as we touch the complex processes that go on in a living thing, be it plant or animal, we are at once forced to use the methods of this science [chemistry]. No longer will the microscope, the kymograph, the scalpel avail for the complete solution of the problem. For the further analysis of these phenomena which are in flux and flow, the investigator must associate himself with those who have labored in fields where molecules and atoms, rather than multicellular tissues or even unicellular organisms, are the units of study.
'Experimental and Chemical Studies of the Blood with an Appeal for More Extended Chemical Training for the Biological and Medical Investigator', Science (6 Aug 1915), 42, 176.
As the saying goes, the Stone Age did not end because we ran out of stones; we transitioned to better solutions. The same opportunity lies before us with energy efficiency and clean energy.
In letter (1 Feb 2013) to Energy Department employees announcing his decision not to serve a second term.
At a given instant everything the surgeon knows suddenly becomes important to the solution of the problem. You can't do it an hour later, or tomorrow. Nor can you go to the library and look it up.
Quoted in 'The Best Hope of All', Time (3 May 1963)
At its heart, engineering is about using science to find creative, practical solutions. It is a noble profession!
From a speech by The Queen at The Queen Elizabeth Prize for Engineering (25 Jun 2013).
Change requires experimentation. But no problem can be solved by the same consciousness that created it. Our job is to dream—and to make those dreams happen.
In interview article, 'Designing For The Future', Newsweek (15 May 2005).
Changes, cyclic or otherwise, within the solar system or within our galaxy, would seem to be the easy and incontrovertible solution for everything that I have found remarkable in the stratigraphical record.
In The Nature of the Stratigraphical Record (1973), 83.
Chess is not a game. Chess is a well-defined form of computation. You may not be able to work out the answers, but in theory there must be a solution, a right procedure in any position. Now real games are not like that at all. Real life is not like that. Real life consists of bluffing, of little tactics of deception, of asking yourself what is the other man going to think I mean to do.
In Jacob Bronowski, The Ascent of Man (2011), 324. Although printed within single quote marks, these words are presumably not verbatim, but only a narrative recollection of a conversation with the author (during WWII, in a taxi, about von Neumann’s Theory of Games).
Common-sense contents itself with the unreconciled contradiction, laughs when it can, and weeps when it must, and makes, in short, a practical compromise, without trying a theoretical solution.
From Essay, 'German Pessimism', a book review (of Der Modern Pessimismus by Edmund Pfleiderer) in Nation (7 Oct 1875), 21, No. 536, 233. Reprinted in Ralph Barton Perry (ed.), Collected Essays and Reviews by William James (1920), 17.
Consider a cow. A cow doesn’t have the problem-solving skill of a chimpanzee, which has discovered how to get termites out of the ground by putting a stick into a hole. Evolution has developed the brain’s ability to solve puzzles, and at the same time has produced in our brain a pleasure of solving problems.
In John Tierney, 'For Decades, Puzzling People With Mathematics', New York Times (20 Oct 2009), D2.
Crowds are somewhat like the sphinx of ancient fable: It is necessary to arrive at a solution of the problems offered by their psychology or to resign ourselves to being devoured by them.
From Psychologie des Foules (1895), 90. English text in The Crowd: A Study of the Popular Mind (1897), Book 2, Chap. 2, 95. Original French text: “Les foules sont un peu comme le sphinx de la fable antique: il faut savoir résoudre les problèmes que leur psychologie nous pose, ou se résigner à être dévoré par elles.”
Curves that have no tangents are the rule. … Those who hear of curves without tangents, or of functions without derivatives, often think at first that Nature presents no such complications. … The contrary however is true. … Consider, for instance, one of the white flakes that are obtained by salting a solution of soap. At a distance its contour may appear sharply defined, but as we draw nearer its sharpness disappears. The eye can no longer draw a tangent at any point. … The use of a magnifying glass or microscope leaves us just as uncertain, for fresh irregularities appear every time we increase the magnification. … An essential characteristic of our flake … is that we suspect … that any scale involves details that absolutely prohibit the fixing of a tangent.
(1906). As quoted “in free translation” in Benoit B. Mandelbrot, The Fractal Geometry of Nature (1977, 1983), 7.
Dad [Walter C. Alvarez] … advised me to sit every few months in my reading chair for an entire evening, close my eyes and try to think of new problems to solve. I took his advice very seriously and have been glad ever since that he did.
In Alvarez: Adventures of a Physicist (1987), 58.
Daniel Bernoulli used to tell two little adventures, which he said had given him more pleasure than all the other honours he had received. Travelling with a learned stranger, who, being pleased with his conversation, asked his name; “I am Daniel Bernoulli,” answered he with great modesty; “and I,” said the stranger (who thought he meant to laugh at him) “am Isaac Newton.” Another time, having to dine with the celebrated Koenig, the mathematician, who boasted, with some degree of self-complacency, of a difficult problem he had solved with much trouble, Bernoulli went on doing the honours of his table, and when they went to drink coffee he presented Koenig with a solution of the problem more elegant than his own.
In A Philosophical and Mathematical Dictionary (1815), 1, 226.
Does there truly exist an insuperable contradiction between religion and science? Can religion be superseded by science? The answers to these questions have, for centuries, given rise to considerable dispute and, indeed, bitter fighting. Yet, in my own mind there can be no doubt that in both cases a dispassionate consideration can only lead to a negative answer. What complicates the solution, however, is the fact that while most people readily agree on what is meant by ‘science,’ they are likely to differ on the meaning of ‘religion.’
…...
During a conversation with the writer in the last weeks of his life, Sylvester remarked as curious that notwithstanding he had always considered the bent of his mind to be rather analytical than geometrical, he found in nearly every case that the solution of an analytical problem turned upon some quite simple geometrical notion, and that he was never satisfied until he could present the argument in geometrical language.
In Proceedings London Royal Society, 63, 17.
Each species has evolved a special set of solutions to the general problems that all organisms must face. By the fact of its existence, a species demonstrates that its members are able to carry out adequately a series of general functions. … These general functions offer a framework within which one can integrate one’s view of biology and focus one’s research. Such a view helps one to avoid becoming lost in a morass of unstructured detail—even though the ways in which different species perform these functions may differ widely. A few obvious examples will suffice. Organisms must remain functionally integrated. They must obtain materials from their environments, and process and release energy from these materials. … They must differentiate and grow, and they must reproduce. By focusing one’s questions on one or another of these obligatory and universal capacities, one can ensure that one’s research will not be trivial and that it will have some chance of achieving broad general applicability.
In 'Integrative Biology: An Organismic Biologist’s Point of View', Integrative and Comparative Biology (2005), 45, 331.
Engineering is not merely knowing and being knowledgeable, like a walking encyclopedia; engineering is not merely analysis; engineering is not merely the possession of the capacity to get elegant solutions to non-existent engineering problems; engineering is practicing the art of the organizing forces of technological change ... Engineers operate at the interface between science and society.
In Bert Scalzo, et al., Database Benchmarking: Practical Methods for Oracle & SQL Server (2007), 37.
Engineering is quite different from science. Scientists try to understand nature. Engineers try to make things that do not exist in nature. Engineers stress invention. To embody an invention the engineer must put his idea in concrete terms, and design something that people can use. That something can be a device, a gadget, a material, a method, a computing program, an innovative experiment, a new solution to a problem, or an improvement on what is existing. Since a design has to be concrete, it must have its geometry, dimensions, and characteristic numbers. Almost all engineers working on new designs find that they do not have all the needed information. Most often, they are limited by insufficient scientific knowledge. Thus they study mathematics, physics, chemistry, biology and mechanics. Often they have to add to the sciences relevant to their profession. Thus engineering sciences are born.
Y.C. Fung and P. Tong, Classical and Computational Solid Mechanics (2001), 1.
Engineers apply the theories and principles of science and mathematics to research and develop economical solutions to practical technical problems. Their work is the link between scientific discoveries and commercial applications. Engineers design products, the machinery to build those products, the factories in which those products are made, and the systems that ensure the quality of the product and efficiency of the workforce and manufacturing process. They design, plan, and supervise the construction of buildings, highways, and transit systems. They develop and implement improved ways to extract, process, and use raw materials, such as petroleum and natural gas. They develop new materials that both improve the performance of products, and make implementing advances in technology possible. They harness the power of the sun, the earth, atoms, and electricity for use in supplying the Nation’s power needs, and create millions of products using power. Their knowledge is applied to improving many things, including the
quality of health care, the safety of food products, and the efficient operation of financial systems.
Bureau of Labor Statistics, Occupational Outlook Handbook (2000) as quoted in Charles R. Lord. Guide to Information Sources in Engineering (2000), 5. This definition has been revised and expanded over time in different issues of the Handbook.
Even fairly good students, when they have obtained the solution of the problem and written down neatly the argument, shut their books and look for something else. Doing so, they miss an important and instructive phase of the work. ... A good teacher should understand and impress on his students the view that no problem whatever is completely exhausted.
In How to Solve It: A New Aspect of Mathematical Method (2004), 14.
Everybody’s a mad scientist, and life is their lab. We’re all trying to experiment to find a way to live, to solve problems, to fend off madness and chaos.
In David Chronenberg and Chris Rodley (ed.), Chronenberg on Chronenberg (1992), 7. As cited in Carl Royer, B Lee Cooper, The Spectacle of Isolation in Horror Films: Dark Parades (2013), 55.
Everything in nature is a puzzle until it finds its solution in man, who solves it in some way with God, and so completes the circle of creation.
The Appeal to Life (1891), 315.
Finite systems of deterministic ordinary nonlinear differential equations may be designed to represent forced dissipative hydrodynamic flow. Solutions of these equations can be identified with trajectories in phase space. For those systems with bounded solutions, it is found that nonperiodic solutions are ordinarily unstable with respect to small modifications, so that slightly differing initial states can evolve into considerably different states. Systems with bounded solutions are shown to possess bounded numerical solutions.
A simple system representing cellular convection is solved numerically. All of the solutions are found to be unstable, and almost all of them are nonperiodic.
The feasibility of very-long-range weather prediction is examined in the light of these results
A simple system representing cellular convection is solved numerically. All of the solutions are found to be unstable, and almost all of them are nonperiodic.
The feasibility of very-long-range weather prediction is examined in the light of these results
Abstract from his landmark paper introducing Chaos Theory in relation to weather prediction, 'Deterministic Nonperiodic Flow', Journal of the Atmospheric Science (Mar 1963), 20, 130.
For the environmentalists, The Space Option is the ultimate environmental solution. For the Cornucopians, it is the technological fix that they are relying on. For the hard core space community, the obvious by-product would be the eventual exploration and settlement of the solar system. For most of humanity however, the ultimate benefit is having a realistic hope in a future with possibilities.... If our species does not soon embrace this unique opportunity with sufficient commitment, it may miss its one and only chance to do so. Humanity could soon be overwhelmed by one or more of the many challenges it now faces. The window of opportunity is closing as fast as the population is increasing. Our future will be either a Space Age or a Stone Age.
Arthur Woods and Marco Bernasconi
Forces of nature act in a mysterious manner. We can but solve the mystery by deducing the unknown result from the known results of similar events.
In The Words of Gandhi (2001), 87.
FORTRAN —’the infantile disorder’—, by now nearly 20 years old, is hopelessly inadequate for whatever computer application you have in mind today: it is now too clumsy, too risky, and too expensive to use. PL/I —’the fatal disease’— belongs more to the problem set than to the solution set. It is practically impossible to teach good programming to students that have had a prior exposure to BASIC: as potential programmers they are mentally mutilated beyond hope of regeneration. The use of COBOL cripples the mind; its teaching should, therefore, be regarded as a criminal offence. APL is a mistake, carried through to perfection. It is the language of the future for the programming techniques of the past: it creates a new generation of coding bums.
…...
Free men are aware of the imperfection inherent in human affairs, and they are willing to fight and die for that which is not perfect. They know that basic human problems can have no final solutions, that our freedom, justice, equality, etc. are far from absolute, and that the good life is compounded of half measures, compromises, lesser evils, and gropings toward the perfect. The rejection of approximations and the insistence on absolutes are the manifestation of a nihilism that loathes freedom, tolerance, and equity.
In The Temper of Our Time (1967), 103.
From the point of view of the pure morphologist the recapitulation theory is an instrument of research enabling him to reconstruct probable lines of descent; from the standpoint of the student of development and heredity the fact of recapitulation is a difficult problem whose solution would perhaps give the key to a true understanding of the real nature of heredity.
Form and Function: A Contribution to the History of Animal Morphology (1916), 312-3.
He [Lord Bacon] appears to have been utterly ignorant of the discoveries which had just been made by Kepler’s calculations … he does not say a word about Napier’s Logarithms, which had been published only nine years before and reprinted more than once in the interval. He complained that no considerable advance had been made in Geometry beyond Euclid, without taking any notice of what had been done by Archimedes and Apollonius. He saw the importance of determining accurately the specific gravities of different substances, and himself attempted to form a table of them by a rude process of his own, without knowing of the more scientific though still imperfect methods previously employed by Archimedes, Ghetaldus and Porta. He speaks of the εὕρηκα of Archimedes in a manner which implies that he did not clearly appreciate either the problem to be solved or the principles upon which the solution depended. In reviewing the progress of Mechanics, he makes no mention either of Archimedes, or Stevinus, Galileo, Guldinus, or Ghetaldus. He makes no allusion to the theory of Equilibrium. He observes that a ball of one pound weight will fall nearly as fast through the air as a ball of two, without alluding to the theory of acceleration of falling bodies, which had been made known by Galileo more than thirty years before. He proposed an inquiry with regard to the lever,—namely, whether in a balance with arms of different length but equal weight the distance from the fulcrum has any effect upon the inclination—though the theory of the lever was as well understood in his own time as it is now. … He speaks of the poles of the earth as fixed, in a manner which seems to imply that he was not acquainted with the precession of the equinoxes; and in another place, of the north pole being above and the south pole below, as a reason why in our hemisphere the north winds predominate over the south.
From Spedding’s 'Preface' to De Interpretations Naturae Proœmium, in The Works of Francis Bacon (1857), Vol. 3, 511-512. [Note: the Greek word “εὕρηκα” is “Eureka” —Webmaster.]
Honest investigation is but the application of common sense to the solution of the unknown. Science does not wait on Genius, but is the companion of Industry.
From address (1 Oct 1884), at inauguration of the Corcoran School of Science and Arts, Columbian University, Washington, D.C. Published in 'The Larger Import of Scientific Education', Popular Science Monthly (Feb 1885), 26, 453.
How is it that there are so many minds that are incapable of understanding mathematics? ... the skeleton of our understanding, ... and actually they are the majority. ... We have here a problem that is not easy of solution, but yet must engage the attention of all who wish to devote themselves to education.
Science and Method (1914, 2003), 117-118.
How often people speak of art and science as though they were two entirely different things, with no interconnection. An artist is emotional, they think, and uses only his intuition; he sees all at once and has no need of reason. A scientist is cold, they think, and uses only his reason; he argues carefully step by step, and needs no imagination. That is all wrong. The true artist is quite rational as well as imaginative and knows what he is doing; if he does not, his art suffers. The true scientist is quite imaginative as well as rational, and sometimes leaps to solutions where reason can follow only slowly; if he does not, his science suffers.
'Prometheus.' The Roving Mind (1983), Chap 25.
I believe that, as men occupied with the study and treatment of disease, we cannot have too strong a conviction that the problems presented to us are physical problems, which perhaps we may never solve, but still admitting of solution only in one way, namely, by regarding them as part of an unbroken series, running up from the lowest elementary conditions of matter to the highest composition of organic structure.
From Address (7 Aug 1868), the Hunterian Oration, 'Clinical Observation in Relation to medicine in Modern Times' delivered to a meeting of the British Medical Association, Oxford. Collected in Sir William Withey Gull and Theodore Dyke Acland (ed.), A Collection of the Published Writings of William Withey Gull (1896), 4.
I consider that I understand an equation when I can predict the properties of its solutions, without actually solving it.
Quoted in F Wilczek, B Devine, Longing for the Harmonies.
I distinguish two kinds of "applied" research: problem-solving research — government or commercially initiated, centrally managed and institutionally coupled to a plan for application of the results, useful science—investigator-initiated, competitively evaluated and widely communicated. Then we have basic science—useful also, also investigator-initiated, competitively evaluated and widely communicated.
In Confessions of a Technophile (1994), 31.
I don’t know if I would call it a miracle. I would call it a spectacular example of what people can do. To me, it’s like putting the first man on the moon or splitting the atom. We’ve shown that if the right treatment is given to people who have a catastrophic injury that they could walk away from it.
Expressing optimism for further recovery for Kevin Everett, a Buffalo Bills football player who suffered a paralyzing spinal injury during a game (9 Sep 2007), but after two days of hospital treatment had begun voluntarily moving his arms and legs. Green credits as significant to the recovery was that within minutes of his injury, the patient was quickly treated with intravenous ice-cold saline solution to induce hypothermia.
Expressing optimism for further recovery for Kevin Everett, a Buffalo Bills football player who suffered a paralyzing spinal injury during a game (9 Sep 2007), but after two days of hospital treatment had begun voluntarily moving his arms and legs. Green credits as significant to the recovery was that within minutes of his injury, the patient was quickly treated with intravenous ice-cold saline solution to induce hypothermia.
Quoted in John Wawrow, 'Bills' Everett Improves, May Walk Again', Associated Press news report, Washington Post (12 Sep 2007).
I feel that I have at last struck the solution of a great problem—and the day is coming when telegraph wires will be laid on to houses just like water or gas—and friends converse with each other without leaving home.
Letter (10 Mar 1876) to his father on the day his first words were sent by wire to Mr. Watson. As quoted in Robert V. Bruce, Bell: Alexander Graham Bell and the Conquest of Solitude (1973, 1990), 181.
I have been able to solve a few problems of mathematical physics on which the greatest mathematicians since Euler have struggled in vain … But the pride I might have held in my conclusions was perceptibly lessened by the fact that I knew that the solution of these problems had almost always come to me as the gradual generalization of favorable examples, by a series of fortunate conjectures, after many errors. I am fain to compare myself with a wanderer on the mountains who, not knowing the path, climbs slowly and painfully upwards and often has to retrace his steps because he can go no further—then, whether by taking thought or from luck, discovers a new track that leads him on a little till at length when he reaches the summit he finds to his shame that there is a royal road by which he might have ascended, had he only the wits to find the right approach to it. In my works, I naturally said nothing about my mistake to the reader, but only described the made track by which he may now reach the same heights without difficulty.
(1891) As quoted in translation in Leo Koenigsberger and Frances A. Welby (trans.), Hermann von Helmholtz (1906), 180-181.
I have found a wonderful solution to Fermats’ Last Theorem—but my train is leaving.
Graffiti on wall of subway, New York. As quoted in William Reville, 'The Science of Writing a Good Joke', The Irish Times (5 Jun 2000).
I have often been amused by our vulgar tendency to take complex issues, with solutions at neither extreme of a continuum of possibilities, and break them into dichotomies, assigning one group to one pole and the other to an opposite end, with no acknowledgment of subtleties and intermediate positions–and nearly always with moral opprobrium attached to opponents.
…...
I never allow myself to become discouraged under any circumstances. … After we had conducted
thousands of experiments on a certain project without solving the problem, … we had learned something. For we had learned for a certainty that the thing couldn’t be done that way, and that we would have to try some other way. We sometimes learn a lot from our failures if we have put into the effort the best thought and work we are capable of.
As quoted from an interview by B.C. Forbes in The American Magazine (Jan 1921), 89.
I ought to say that one of our first joint researches, so far as publication was concerned, had the peculiar effect of freeing me forever from the wiles of college football, and if that is a defect, make the most of it! Dr. Noyes and I conceived an idea on sodium aluminate solutions on the morning of the day of a Princeton-Harvard game (as I recall it) that we had planned to attend. It looked as though a few days' work on freezing-point determinations and electrical conductivities would answer the question. We could not wait, so we gave up the game and stayed in the laboratory. Our experiments were successful. I think that this was the last game I have ever cared about seeing. I mention this as a warning, because this immunity might attack anyone. I find that I still complainingly wonder at the present position of football in American education.
Address upon receiving the Perkin Medal Award, 'The Big Things in Chemistry', The Journal of Industrial and Engineering Chemistry (Feb 1921), 13, No. 2, 162-163.
I prefer the spagyric chemical physicians, for they do not consort with loafers or go about gorgeous in satins, silks and velvets, gold rings on their fingers, silver daggers hanging at their sides and white gloves on their hands, but they tend their work at the fire patiently day and night. They do not go promenading, but seek their recreation in the laboratory, wear plain learthern dress and aprons of hide upon which to wipe their hands, thrust their fingers amongst the coals, into dirt and rubbish and not into golden rings. They are sooty and dirty like the smiths and charcoal burners, and hence make little show, make not many words and gossip with their patients, do not highly praise their own remedies, for they well know that the work must praise the master, not the master praise his work. They well know that words and chatter do not help the sick nor cure them... Therefore they let such things alone and busy themselves with working with their fires and learning the steps of alchemy. These are distillation, solution, putrefaction, extraction, calcination, reverberation, sublimination, fixation, separation, reduction, coagulation, tinction, etc.
Quoted in R. Oesper, The Human Side of Scientists (1975), 150. [Spagyric is a form of herbalism based on alchemic procedures of preparation.]
I realized both the upper and lower body must be held securely in place with one strap across the chest and one across the hips. The belt also needed an immovable anchorage point for the buckle as far down beside the occupant’s hip, so it could hold the body properly during a collision. It was just a matter of finding a solution that was simple, effective and could be put on conveniently with one hand.
as quoted in New York Times obituary, 26 Sep 2002
I regard sex as the central problem of life. And now that the problem of religion has practically been settled, and that the problem of labor has at least been placed on a practical foundation, the question of sex—with the racial questions that rest on it—stands before the coming generations as the chief problem for solution. Sex lies at the root of life, and we can never learn to reverence life until we know how to understand sex.
Studies in the Psychology of Sex (1897), Vol. 1, xxx.
I thank you for your Expt on the Hedge Hog; but why do you ask me such a question, by way of solving it. I think your solution is just; but why think, why not try the Expt.
[Often seen, without context, briefly as: But why think, why not try the experiment?']
[Often seen, without context, briefly as: But why think, why not try the experiment?']
Letter to Edward Jenner (2 Aug 1775). In A. J. Harding Rains (ed.), Letters From the Past: From John Hunter to Edward Jenner (1976), 9.
I think that the difference between pure and applied mathematics is social rather than scientific. A pure mathematician is paid for making mathematical discoveries. An applied mathematician is paid for the solution of given problems.
When Columbus set sail, he was like an applied mathematician, paid for the search of the solution of a concrete problem: find a way to India. His discovery of the New World was similar to the work of a pure mathematician.
When Columbus set sail, he was like an applied mathematician, paid for the search of the solution of a concrete problem: find a way to India. His discovery of the New World was similar to the work of a pure mathematician.
In S.H. Lui, 'An Interview with Vladimir Arnol’d', Notices of the AMS (Apr 1997) 44, No. 4, 438. Reprinted from the Hong Kong Mathematics Society (Feb 1996).
I wanted to preserve the spontaneity of thought in speech… [and to] guard the spontaneity of the argument. A spoken argument is informal and heuristic; it singles out the heart of the matter and shows in what way it is crucial and new; and it gives the direction and line of the solution so that, simplified as it is, still the logic is right. For me, this philosophic form of argument is the foundation of science, and nothing should be allowed to obscure it.
On his philosophy in presenting the TV series, from which the book followed. In 'Foreward', The Ascent of Man, (1973), 14-15.
I was led to the conclusion that at the most extreme dilutions all salts would consist of simple conducting molecules. But the conducting molecules are, according to the hypothesis of Clausius and Williamson, dissociated; hence at extreme dilutions all salt molecules are completely disassociated. The degree of dissociation can be simply found on this assumption by taking the ratio of the molecular conductivity of the solution in question to the molecular conductivity at the most extreme dilution.
Letter to Van’t Hoff, 13 April 1887. In J. R. Partington, A History of Chemistry (1961), Vol. 4, 678.
I was working with these very long-chain … extended-chain polymers, where you had a lot of benzene rings in them. … Transforming a polymer solution from a liquid to a fiber requires a process called spinning. … We spun it and it spun beautifully. It [Kevlar] was very strong and very stiff—unlike anything we had made before. I knew that I had made a discovery. I didn’t shout “Eureka!” but I was very excited, as was the whole laboratory excited, and management was excited, because we were looking for something new. Something different. And this was it.
From transcript for video interview (2007, published Aug 2012), 'Stephanie Kwolek: Curiosity and the Discovery of Kevlar', in the series Women in Chemistry, on Chemical Heritage Foundation website.
I, Galileo Galilei, son of the late Vincenzo Galilei, of Florence, aged seventy years, being brought personally to judgment, and kneeling before your Most Eminent and Most Reverend Lords Cardinals, General Inquisitors of the universal Christian republic against heretical depravity, having before my eyes the Holy Gospels, which I touch with my own hands, swear that I have always believed, and now believe, and with the help of God will in future believe, every article which the Holy Catholic and Apostolic Church of Rome holds, teaches, and preaches. But because I have been enjoined by this Holy Office altogether to abandon the false opinion which maintains that the sun is the centre and immovable, and forbidden to hold, defend, or teach the said false doctrine in any manner, and after it hath been signified to me that the said doctrine is repugnant with the Holy Scripture, I have written and printed a book, in which I treat of the same doctrine now condemned, and adduce reasons with great force in support of the same, without giving any solution, and therefore have been judged grievously suspected of heresy; that is to say, that I held and believed that the sun is the centre of the universe and is immovable, and that the earth is not the centre and is movable; willing, therefore, to remove from the minds of your Eminences, and of every Catholic Christian, this vehement suspicion rightfully entertained toward me, with a sincere heart and unfeigned faith, I abjure, curse, and detest the said errors and heresies, and generally every other error and sect contrary to Holy Church; and I swear that I will never more in future say or assert anything verbally, or in writing, which may give rise to a similar suspicion of me; but if I shall know any heretic, or anyone suspected of heresy, that I will denounce him to this Holy Office, or to the Inquisitor or Ordinary of the place where I may be; I swear, moreover, and promise, that I will fulfil and observe fully, all the penances which have been or shall be laid on me by this Holy Office. But if it shall happen that I violate any of my said promises, oaths, and protestations (which God avert!), I subject myself to all the pains and punishments which have been decreed and promulgated by the sacred canons, and other general and particular constitutions, against delinquents of this description. So may God help me, and his Holy Gospels which I touch with my own hands. I, the above-named Galileo Galilei, have abjured, sworn, promised, and bound myself as above, and in witness thereof with my own hand have subscribed this present writing of my abjuration, which I have recited word for word. At Rome, in the Convent of Minerva, June 22, 1633. I, Galileo Galilei, have abjured as above with my own hand.
Abjuration, 22 Jun 1633. In J.J. Fahie, Galileo, His Life and Work (1903), 319-321.
I’m convinced that the best solutions are often the ones that are counterintuitive—that challenge conventional thinking—and end in breakthroughs. It is always easier to do things the same old way … why change? To fight this, keep your dissatisfaction index high and break with tradition. Don’t be too quick to accept the way things are being done. Question whether there’s a better way. Very often you will find that once you make this break from the usual way - and incidentally, this is probably the hardest thing to do—and start on a new track your horizon of new thoughts immediately broadens. New ideas flow in like water. Always keep your interests broad - don’t let your mind be stunted by a limited view.
1988
I’m not sure what solutions we’ll find to deal with all our environmental problems, but I’m sure of this: They will be provided by industry; they will be products of technology. Where else can they come from?
Nation's Business (12 Jun 1988).
If a solution fails to appear … and yet we feel success is just around the corner, try resting for a while. … Like the early morning frost, this intellectual refreshment withers the parasitic and nasty vegetation that smothers the good seed. Bursting forth at last is the flower of truth.
From Reglas y Consejos sobre Investigacíon Cientifica: Los tónicos de la voluntad. (1897), as translated by Neely and Larry W. Swanson, in Advice for a Young Investigator (1999), 35.
If any student comes to me and says he wants to be useful to mankind and go into research to alleviate human suffering, I advise him to go into charity instead. Research wants real egotists who seek their own pleasure and satisfaction, but find it in solving the puzzles of nature.
In Science Today (May 1980), 35. In Vladimir Burdyuzha, The Future of Life and the Future of Our Civilization (2006), 374.
If there is a problem you can’t solve, then there is an easier problem you can solve: find it.
Quoted in Preface, How to Solve It: A New Aspect of Mathematical Method (2004), xxi.
If thou art able, O stranger, to find out all these things and gather them together in your mind, giving all the relations, thou shalt depart crowned with glory and knowing that thou hast been adjudged perfect in this species of wisdom.
From a letter to Eratosthenes, the chief librarian at Alexandria, containing the Cattle Problem, an exceedingly difficult calculation involving huge numbers (which was not solved exactly until the use of a supercomputer in 1981). In David J. Darling, The Universal Book of Mathematics (2004), 23. The debate by scholars regarding whether Archimedes is the true author is in T. L. Heath (ed.), The Works of Archimedes (1897), xxxiv.
If we want to solve a problem that we have never solved before, we must leave the door to the unknown ajar.
In 'The Value of Science,' What Do You Care What Other People Think? (1988, 2001), 247. Collected in The Pleasure of Finding Things Out (2000), 149.
If you’re not part of the solution, you’re part of the precipitate.
…...
In a famous passage, René Descartes tells us that he considered himself to be placed in three simultaneous domiciles, patiently recognizing his loyalties to the social past, fervidly believing in a final solution of nature’s secrets and in the meantime consecrated to the pursuit of scientific doubt. Here we have the half way house of the scientific laboratory, of the scientific mind in the midst of its campaign.
In 'The Three Dimensions of Time', Part I, 'The Classic of Science', A Classic and a Founder (1937), collected in Rosenstock-Huessy Papers (1981), Vol. 1, 14.
In a great number of the cosmogonic myths the world is said to have developed from a great water, which was the prime matter. In many cases, as for instance in an Indian myth, this prime matter is indicated as a solution, out of which the solid earth crystallized out.
In Theories of Solutions (1912), 1.
In general the position as regards all such new calculi is this That one cannot accomplish by them anything that could not be accomplished without them. However, the advantage is, that, provided such a calculus corresponds to the inmost nature of frequent needs, anyone who masters it thoroughly is able—without the unconscious inspiration of genius which no one can command—to solve the respective problems, yea, to solve them mechanically in complicated cases in which, without such aid, even genius becomes powerless. Such is the case with the invention of general algebra, with the differential calculus, and in a more limited region with Lagrange’s calculus of variations, with my calculus of congruences, and with Möbius’s calculus. Such conceptions unite, as it were, into an organic whole countless problems which otherwise would remain isolated and require for their separate solution more or less application of inventive genius.
Letter (15 May 1843) to Schumacher, collected in Carl Friedrich Gauss Werke (1866), Vol. 8, 298, as translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 197-198. From the original German, “Überhaupt verhält es sich mit allen solchen neuen Calculs so, dass man durch sie nichts leisten kann, was nicht auch ohne sie zu leisten wäre; der Vortheil ist aber der, dass, wenn ein solcher Calcul dem innersten Wesen vielfach vorkommender Bedürfnisse correspondirt, jeder, der sich ihn ganz angeeignet hat, auch ohne die gleichsam unbewussten Inspirationen des Genies, die niemand erzwingen kann, die dahin gehörigen Aufgaben lösen, ja selbst in so verwickelten Fällen gleichsam mechanisch lösen kann, wo ohne eine solche Hülfe auch das Genie ohnmächtig wird. So ist es mit der Erfindung der Buchstabenrechnung überhaupt; so mit der Differentialrechnung gewesen; so ist es auch (wenn auch in partielleren Sphären) mit Lagranges Variationsrechnung, mit meiner Congruenzenrechnung und mit Möbius' Calcul. Es werden durch solche Conceptionen unzählige Aufgaben, die sonst vereinzelt stehen, und jedesmal neue Efforts (kleinere oder grössere) des Erfindungsgeistes erfordern, gleichsam zu einem organischen Reiche.”
In less than eight years “The Origin of Species” has produced conviction in the minds of a majority of the most eminent living men of science. New facts, new problems, new difficulties as they arise are accepted, solved, or removed by this theory; and its principles are illustrated by the progress and conclusions of every well established branch of human knowledge.
From a review of four books on the subject 'Mimicry, and Other Protective Resemblances Among Animals', in The Westminster Review (Jul 1867), 88, 1. Wallace is identified as the author in the article as reprinted in William Beebe, The Book of Naturalists: An Anthology of the Best Natural History (1988), 108.
In short, the greatest contribution to real security that science can make is through the extension of the scientific method to the social sciences and a solution of the problem of complete avoidance of war.
In "Science and Security", Science (25 Jun 1948), 107, 665. Written while Director of the U.S. National Bureau of Standards.
In the conception of a machine or the product of a machine there is a point where one may leave off for parsimonious reasons, without having reached aesthetic perfection; at this point perhaps every mechanical factor is accounted for, and the sense of incompleteness is due to the failure to recognize the claims of the human agent. Aesthetics carries with it the implications of alternatives between a number of mechanical solutions of equal validity; and unless this awareness is present at every stage of the process … it is not likely to come out with any success in the final stage of design.
From 'The Esthetic Assimilation of the Machine', Technics and Civilization (1934), 349.
In the discovery of lemmas the best aid is a mental aptitude for it. For we may see many who are quick at solutions and yet do not work by method ; thus Cratistus in our time was able to obtain the required result from first principles, and those the fewest possible, but it was his natural gift which helped him to the discovery.
— Proclus
As given in Euclid, The Thirteen Books of Euclid's Elements, translated from the text of Johan Ludvig Heiberg by Sir Thomas Little Heath, Vol. 1, Introduction and Books 1,2 (1908), 133. The passage also states that Proclus gives the definition of the term lemma as a proposition not proved beforehand. Glenn Raymond Morrow in A Commentary on the First Book of Euclid's Elements (1992), 165, states nothing more seems to be known of Cratistus.
In the next twenty centuries … humanity may begin to understand its most baffling mystery—where are we going? The earth is, in fact, traveling many thousands of miles per hour in the direction of the constellation Hercules—to some unknown destination in the cosmos. Man must understand his universe in order to understand his destiny. Mystery, however, is a very necessary ingredient in our lives. Mystery creates wonder and wonder is the basis for man’s desire to understand. Who knows what mysteries will be solved in our lifetime, and what new riddles will become the challenge of the new generation? Science has not mastered prophesy. We predict too much for the next year yet far too little for the next ten. Responding to challenges is one of democracy’s great strengths. Our successes in space can be used in the next decade in the solution of many of our planet’s problems.
In a speech to a Joint Meeting of the Two Houses of Congress to Receive the Apollo 11 Astronauts (16 Sep 1969), in the Congressional Record.
In the search for truth there are certain questions that are not important. Of what material is the universe constructed? Is the universe eternal? Are there limits or not to the universe? ... If a man were to postpone his search and practice for Enlightenment until such questions were solved, he would die before he found the path.
— Budha
…...
Increasingly, our leaders must deal with dangers that threaten the entire world, where an understanding of those dangers and the possible solutions depend on a good grasp of science. The ozone layer, the greenhouse effect, acid rain, questions of diet and of heredity--all require scientific literacy. Can Americans choose the proper leaders and support the proper programs if they are scientifically illiterate?
articles.latimes.com/1989-03-31/news/vw-543_1_scientific-literacy
Increasingly, our leaders must deal with dangers that threaten the entire world, where an understanding of those dangers and the possible solutions depends on a good grasp of science. The ozone layer, the greenhouse effect, acid rain, questions of diet and heredity. All require scientific literacy. Can Americans choose the proper leaders and support the proper programs if they themselves are scientifically illiterate? The whole premise of democracy is that it is safe to leave important questions to the court of public opinion—but is it safe to leave them to the court of public ignorance?
In Los Angeles Times (31 Mar 1989).
Indeed, while Nature is wonderfully inventive of new structures, her conservatism in holding on to old ones is still more remarkable. In the ascending line of development she tries an experiment once exceedingly thorough, and then the question is solved for all time. For she always takes time enough to try the experiment exhaustively. It took ages to find how to build a spinal column or brain, but when the experiment was finished she had reason to be, and was, satisfied.
In The Whence and Whither of Man; a Brief History of his Origin and Development through Conformity to Environment; being the Morse Lectures of 1895. (1896), 173. The Morse lectureship was founded by Prof. Samuel F.B. Morse in 1865 at Union Theological Seminary, the lectures to deal with “the relation of the Bible to any of the sciences.”
Intelligence is an extremely subtle concept. It’s a kind of understanding that flourishes if it’s combined with a good memory, but exists anyway even in the absence of good memory. It’s the ability to draw consequences from causes, to make correct inferences, to foresee what might be the result, to work out logical problems, to be reasonable, rational, to have the ability to understand the solution from perhaps insufficient information. You know when a person is intelligent, but you can be easily fooled if you are not yourself intelligent.
In Irv Broughton (ed.), The Writer's Mind: Interviews with American Authors (1990), Vol. 2, 57.
Investigation may be likened to the long months of pregnancy, and solving a problem to the day of birth. To investigate a problem is, indeed, to solve it.
In Winberg Chai, The Foreign Relations of the People's Republic of China (1972), 46.
It appears that the solution of the problem of time and space is reserved to philosophers who, like Leibniz, are mathematicians, or to mathematicians who, like Einstein, are philosophers.
Collected in Paul Arthur Schilpp (ed.), Albert Einstein: Philosopher-Scientist (1959), Vol. 1, 307. Also, in James Louis Jarrett and Sterling M. McMurrin (eds.), Contemporary Philosophy: A Book of Readings (1954), 71.
It appears, nevertheless, that all such simple solutions of the problem of vertebrate ancestry are without warrant. They arise from a very common tendency of the mind, against which the naturalist has to guard himself,—a tendency which finds expression in the very widespread notion that the existing anthropoid apes, and more especially the gorilla, must be looked upon as the ancestors of mankind, if once the doctrine of the descent of man from ape-like forefathers is admitted. A little reflexion suffices to show that any given living form, such as the gorilla, cannot possibly be the ancestral form from which man was derived, since ex-hypothesi that ancestral form underwent modification and development, and in so doing, ceased to exist.
'Vertebrata', entry in Encyclopaedia Britannica, 9th edition (1899), Vol. 24, 180.
It has been asserted … that the power of observation is not developed by mathematical studies; while the truth is, that; from the most elementary mathematical notion that arises in the mind of a child to the farthest verge to which mathematical investigation has been pushed and applied, this power is in constant exercise. By observation, as here used, can only be meant the fixing of the attention upon objects (physical or mental) so as to note distinctive peculiarities—to recognize resemblances, differences, and other relations. Now the first mental act of the child recognizing the distinction between one and more than one, between one and two, two and three, etc., is exactly this. So, again, the first geometrical notions are as pure an exercise of this power as can be given. To know a straight line, to distinguish it from a curve; to recognize a triangle and distinguish the several forms—what are these, and all perception of form, but a series of observations? Nor is it alone in securing these fundamental conceptions of number and form that observation plays so important a part. The very genius of the common geometry as a method of reasoning—a system of investigation—is, that it is but a series of observations. The figure being before the eye in actual representation, or before the mind in conception, is so closely scrutinized, that all its distinctive features are perceived; auxiliary lines are drawn (the imagination leading in this), and a new series of inspections is made; and thus, by means of direct, simple observations, the investigation proceeds. So characteristic of common geometry is this method of investigation, that Comte, perhaps the ablest of all writers upon the philosophy of mathematics, is disposed to class geometry, as to its method, with the natural sciences, being based upon observation. Moreover, when we consider applied mathematics, we need only to notice that the exercise of this faculty is so essential, that the basis of all such reasoning, the very material with which we build, have received the name observations. Thus we might proceed to consider the whole range of the human faculties, and find for the most of them ample scope for exercise in mathematical studies. Certainly, the memory will not be found to be neglected. The very first steps in number—counting, the multiplication table, etc., make heavy demands on this power; while the higher branches require the memorizing of formulas which are simply appalling to the uninitiated. So the imagination, the creative faculty of the mind, has constant exercise in all original mathematical investigations, from the solution of the simplest problems to the discovery of the most recondite principle; for it is not by sure, consecutive steps, as many suppose, that we advance from the known to the unknown. The imagination, not the logical faculty, leads in this advance. In fact, practical observation is often in advance of logical exposition. Thus, in the discovery of truth, the imagination habitually presents hypotheses, and observation supplies facts, which it may require ages for the tardy reason to connect logically with the known. Of this truth, mathematics, as well as all other sciences, affords abundant illustrations. So remarkably true is this, that today it is seriously questioned by the majority of thinkers, whether the sublimest branch of mathematics,—the infinitesimal calculus—has anything more than an empirical foundation, mathematicians themselves not being agreed as to its logical basis. That the imagination, and not the logical faculty, leads in all original investigation, no one who has ever succeeded in producing an original demonstration of one of the simpler propositions of geometry, can have any doubt. Nor are induction, analogy, the scrutinization of premises or the search for them, or the balancing of probabilities, spheres of mental operations foreign to mathematics. No one, indeed, can claim preeminence for mathematical studies in all these departments of intellectual culture, but it may, perhaps, be claimed that scarcely any department of science affords discipline to so great a number of faculties, and that none presents so complete a gradation in the exercise of these faculties, from the first principles of the science to the farthest extent of its applications, as mathematics.
In 'Mathematics', in Henry Kiddle and Alexander J. Schem, The Cyclopedia of Education, (1877.) As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 27-29.
It has the property of detonating very violently in certain circumstances. On one occasion a small amount of ether solution of pyroglycerin condensed in a glass bowl. ... When the bowl was heated over a spirit lamp, an extremely violent explosion occurred, which shattered it into small fragments. On another occasion a drop was heated in a test-tube, and exploded with such violence that the glass splinters cut deep into my face and hands, and hurt other people who were standing some distance off in the room.
[Describing early experiments on his discovery of nitroglycerin.]
[Describing early experiments on his discovery of nitroglycerin.]
From speech to the Royal Academy of Turin (1847). In Robert Shaplen, 'Annals of Science, Adventures of a Pacifist,' The New Yorker (15 Mar 1958), 49.
It is a commonplace of modern technology that problems have solutions before there is knowledge of how they are to be solved.
In The New Industrial State (1977).
It is a curious property of research activity that after the problem has been solved the solution seems obvious. This is true not only for those who have not previously been acquainted with the problem, but also for those who have worked over it for years.
Address at the Franklin Institute (1937). Journal of the Franklin Institute (1937), 224, 277. Also see Paul C. Wensberg, Land's Polaroid: A Company and the Man who Invented It (1987), 31.
It is an occupational risk of biologists to claim, towards the end of their careers, that the problems which they have not solved are insoluble.
'Popper's World', The London Review of Books (18-31 August 1983), 12.
It is apparent to me that the possibilities of the aeroplane, which two or three years ago were thought to hold the solution to the [flying machine] problem, have been exhausted, and that we must turn elsewhere.
…...
It is better to do the right problem the wrong way than the wrong problem the right way.
Quoted in Julie K. Petersen, Fiber Optics Illustrated Dictionary (2003), 435.
It is not possession of the solution, but the recognition of the problem itself that provides a resource and the answers.
In Take Today: The Executive as Dropout (1972), 92.
It is the business of science to offer rational explanations for all the events in the real world, and any scientist who calls on God to explain something is falling down on his job. This applies as much to the start of the expansion as to any other event. If the explanation is not forthcoming at once, the scientist must suspend judgment: but if he is worth his salt he will always maintain that a rational explanation will eventually be found. This is the one piece of dogmatism that a scientist can allow himself—and without it science would be in danger of giving way to superstition every time that a problem defied solution for a few years.
The Mystery of the Expanding Universe (1964), 122.
It isn't that they can't see the solution. It is that they can't see the problem.
'The Point of a Pin', in The Scandal of Father Brown (1935,2000), 142.
It isn’t that they can’t see the solution. It is that they can’t see the problem.
In 'The Point of a Pin', The Scandal of Father Brown (1935), Chap. 7, 209.
It may very properly be asked whether the attempt to define distinct species, of a more or less permanent nature, such as we are accustomed to deal with amongst the higher plants and animals, is not altogether illusory amongst such lowly organised forms of life as the bacteria. No biologist nowadays believes in the absolute fixity of species … but there are two circumstances which here render the problem of specificity even more difficult of solution. The bacteriologist is deprived of the test of mutual fertility or sterility, so valuable in determining specific limits amongst organisms in which sexual reproduction prevails. Further, the extreme rapidity with which generation succeeds generation amongst bacteria offers to the forces of variation and natural selection a field for their operation wholly unparalleled amongst higher forms of life.
'The Evolution of the Streptococci', The Lancet, 1906, 2, 1415-6.
It would be difficult and perhaps foolhardy to analyze the chances of further progress in almost every part of mathematics one is stopped by unsurmountable difficulties, improvements in the details seem to be the only possibilities which are left… All these difficulties seem to announce that the power of our analysis is almost exhausted, even as the power of ordinary algebra with regard to transcendental geometry in the time of Leibniz and Newton, and that there is a need of combinations opening a new field to the calculation of transcendental quantities and to the solution of the equations including them.
From Rapport historique sur les progrès des sciences mathématiques depuis 1789, et sur leur état actuel (1810), 131. As translated in George Sarton, The Study of the History of Mathematics (1936), 13. In the original French: “Il seroit difficile et peut-être téméraire d’analyser les chances que l’avenir offre à l’avancement des mathématiques: dans presque toutes les parties, on est arrêté par des difficultés insurmontables; des perfectionnements de détail semblent la seule chose qui reste à faire… Toutes ces difficultés semblent annoncer que la puissance de notre analyse est à-peu-près épuisée, comme celle de l’algèbre ordinaire l’étoit par rapport à la géométrie transcendante au temps de Leibnitz et de Newton, et qu’il faut des combinaisons qui ouvrent un nouveau champ au calcul des transcendantes et à la résolution des équations qui les contiennent.” Sarton states this comes from “the report on mathematical progress prepared for the French Academy of Sciences at Napoleon’s request”.
It would be irresponsible to expect quick results, or to base our energy policy on the expectation of fusion will solve our problem in the next 10 or 20 0r 30 years.
In address at City College, reported in Victor K. McElheny, 'Hans Bethe Urges U.S. Drive for Atom Power and Coal', The New York Times (14 Dec 1974), 58.
It’s much more effective to allow solutions to problems to emerge from the people close to the problem rather than to impose them from higher up.
Interviewed in 'Simple, Yet Complex', CIO (15 Apr 1998), 64.
Knowledge and ability must be combined with ambition as well as with a sense of honesty and a severe conscience. Every analyst occasionally has doubts about the accuracy of his results, and also there are times when he knows his results to be incorrect. Sometimes a few drops of the solution were spilt, or some other slight mistake made. In these cases it requires a strong conscience to repeat the analysis and to make a rough estimate of the loss or apply a correction. Anyone not having sufficient will-power to do this is unsuited to analysis no matter how great his technical ability or knowledge. A chemist who would not take an oath guaranteeing the authenticity, as well as the accuracy of his work, should never publish his results, for if he were to do so, then the result would be detrimental not only to himself, but to the whole of science.
Anleitung zur Quantitativen Analyse (1847), preface. F. Szabadvary, History of Analytical Chemistry (1966), trans. Gyula Svehla, 176.
Language is a guide to 'social reality.' Though language is not ordinarily thought of as essential interest to the students of social science, it powerfully conditions all our thinking about social problems and processes. Human beings do not live in the objective world alone, nor alone in the world of social activity as ordinarily understood, but are very much at the mercy of the particular language which has become the medium of expression for their society. It is quite an illusion to imagine that one adjusts to reality essentially without the use of language and that language is merely an incidental means of solving specific problems of communication or reflection. The fact of the matter is that the 'real world' is to a large extent unconsciously built up on the language habits of the group. No two languages are ever sufficiently similar to be considered as representing the same social reality. The worlds in which different societies live are distinct worlds, not merely the same world with different labels attached.
'The Status of Linguistics as a Science', Language (1929), 5, 207-14. In David Mandelbaum (ed.), Selected Writings of Edward Sapir in Language, Culture, and Personality (1949), 162.
Later scientific theories are better than earlier ones for solving puzzles in the often quite different environments to which they are applied. That is not a relativist's position, and it displays the sense in which I am a convinced believer in scientific progress.
The Structure of Scientific Revolutions, 2nd edition (1970), 206.
Leibnitz’s discoveries lay in the direction in which all modern progress in science lies, in establishing order, symmetry, and harmony, i.e., comprehensiveness and perspicuity,—rather than in dealing with single problems, in the solution of which followers soon attained greater dexterity than himself.
In Leibnitz (1884), 112.
Life arose as a living molecule or protogene, the progression from this stage to that of the ameba is at least as great as from ameba to man. All the essential problems of living organisms are already solved in the one-celled (or, as many now prefer to say, noncellular) protozoan and these are only elaborated in man or the other multicellular animals. The step from nonlife to life may not have been so complex, after all, and that from cell to multicellular organism is readily comprehensible. The change from protogene to protozoan was probably the most complex that has occurred in evolution, and it may well have taken as long as the change from protozoan to man.
The Meaning of Evolution: A Study of the History of Life and of its Significance for Man (1949), 16
M.D.—Make Do.— Quaint idea! … Work for the handicapped … who is handicapped, your patients, or you? Both. Helping the survival of the unfit.… With more to come. What in the world was the solution. Where to find a formula for head and heart too?
Quoted in M.C. Winternitz, 'Alan Gregg, Physician', Science (20 Dec 1957), 1279.
Mankind always takes up only such problems as it can solve; since, looking at the matter more closely, we will always find that the problem itself arises only when the material conditions necessary for its solution already exist or are at least in the process of formation.
In Karl Marx and N.I. Stone (trans.), A Contribution to the Critique of Political Economy (1904), 12.
Many people know everything they know in the way we know the solution of a riddle after we have read it or been told it, and that is the worst kind of knowledge and the kind least to be cultivated; we ought rather to cultivate that kind of knowledge which enables us to discover for ourselves in case of need that which others have to read or be told of in order to know it.
Aphorism 89 in Notebook D (1773-1775), as translated by R.J. Hollingdale in Aphorisms (1990). Reprinted as The Waste Books (2000), 58.
Marxists are more right than wrong when they argue that the problems scientists take up,. the way they go about solving them, and even the solutions they arc inclined to accept, arc conditioned by the intellectual, social, and economic environments in which they live and work.
In Mankind Evolving: The Evolution of the Human Species, 128. As cited in Ted Woods & Alan Grant, Reason in Revolt - Dialectical Philosophy and Modern Science (2003), Vol. 2, 183.
Mathematicians attach great importance to the elegance of their methods and their results. This is not pure dilettantism. What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details. But this is exactly what yields great results, in fact the more we see this aggregate clearly and at a single glance, the better we perceive its analogies with other neighboring objects, consequently the more chances we have of divining the possible generalizations. Elegance may produce the feeling of the unforeseen by the unexpected meeting of objects we are not accustomed to bring together; there again it is fruitful, since it thus unveils for us kinships before unrecognized. It is fruitful even when it results only from the contrast between the simplicity of the means and the complexity of the problem set; it makes us then think of the reason for this contrast and very often makes us see that chance is not the reason; that it is to be found in some unexpected law. In a word, the feeling of mathematical elegance is only the satisfaction due to any adaptation of the solution to the needs of our mind, and it is because of this very adaptation that this solution can be for us an instrument. Consequently this esthetic satisfaction is bound up with the economy of thought.
In 'The Future of Mathematics', Monist, 20, 80. Translated from the French by George Bruce Halsted.
Mathematics … above all other subjects, makes the student lust after knowledge, fills him, as it were, with a longing to fathom the cause of things and to employ his own powers independently; it collects his mental forces and concentrates them on a single point and thus awakens the spirit of individual inquiry, self-confidence and the joy of doing; it fascinates because of the view-points which it offers and creates certainty and assurance, owing to the universal validity of its methods. Thus, both what he receives and what he himself contributes toward the proper conception and solution of a problem, combine to mature the student and to make him skillful, to lead him away from the surface of things and to exercise him in the perception of their essence. A student thus prepared thirsts after knowledge and is ready for the university and its sciences. Thus it appears, that higher mathematics is the best guide to philosophy and to the philosophic conception of the world (considered as a self-contained whole) and of one’s own being.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 40. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 49.
Motion with respect to the universal ocean of aether eludes us. We say, “Let V be the velocity of a body through the aether”, and form the various electromagnetic equations in which V is scattered liberally. Then we insert the observed values, and try to eliminate everything which is unknown except V. The solution goes on famously; but just as we have got rid of all the other unknowns, behold! V disappears as well, and we are left with the indisputable but irritating conclusion —
0 = 0
This is a favourite device that mathematical equations resort to, when we propound stupid questions.
0 = 0
This is a favourite device that mathematical equations resort to, when we propound stupid questions.
From Gifford Lecture, Edinburgh, (1927), 'Relativity', collected in The Nature of the Physical World (1928), 30.
Natural selection produces systems that function no better than necessary. It results in ad hoc adaptive solutions to immediate problems. Whatever enhances fitness is selected. The product of natural selection is not perfection but adequacy, not final answers but limited, short-term solutions.
In 'The role of natural history in contemporary biology', BioScience (1986), 36, 325.
Never confuse a fool’s gold opportunity with a silver bullet solution.
Nevertheless, it is necessary to remember that a planned economy is not yet socialism. A planned economy as such may be accompanied by the complete enslavement of the individual. The achievement of socialism requires the solution of some extremely difficult socio-political problems: how is it possible, in view of the far-reaching centralisation of political and economic power, to prevent bureaucracy from becoming all-powerful and overweening? How can the rights of the individual be protected and therewith a democratic counterweight to the power of bureaucracy be assured?
…...
No problem can be solved until it is reduced to some simple form. The changing of a vague difficulty into a specific, concrete form is a very essential element in thinking.
Seen, for example, in The Grain and Feed Review (1931), 21, 34.
No research will answer all queries that the future may raise. It is wiser to praise the work for what it has accomplished and then to formulate the problems still to be solved.
Letter to Dr. E. B. Krumhaar (11 Oct 1933), in Journal of Bacteriology (Jan 1934), 27, No. 1, 19.
No scientist is admired for failing in the attempt to solve problems that lie beyond his competence. … Good scientists study the most important problems they think they can solve. It is, after all, their professional business to solve problems, not merely to grapple with them.
The Art of the Soluble: Creativity and Originality in Science (1967), 7.
Obviously we biologists should fit our methods to our materials. An interesting response to this challenge has been employed particularly by persons who have entered biology from the physical sciences or who are distressed by the variability in biology; they focus their research on inbred strains of genetically homogeneous laboratory animals from which, to the maximum extent possible, variability has been eliminated. These biologists have changed the nature of the biological system to fit their methods. Such a bold and forthright solution is admirable, but it is not for me. Before I became a professional biologist, I was a boy naturalist, and I prefer a contrasting approach; to change the method to fit the system. This approach requires that one employ procedures which allow direct scientific utilization of the successful long-term evolutionary experiments which are documented by the fascinating diversity and variability of the species of animals which occupy the earth. This is easy to say and hard to do.
In 'Scientific innovation and creativity: a zoologist’s point of view', American Zoologist (1982), 22, 232.
One feature which will probably most impress the mathematician accustomed to the rapidity and directness secured by the generality of modern methods is the deliberation with which Archimedes approaches the solution of any one of his main problems. Yet this very characteristic, with its incidental effects, is calculated to excite the more admiration because the method suggests the tactics of some great strategist who foresees everything, eliminates everything not immediately conducive to the execution of his plan, masters every position in its order, and then suddenly (when the very elaboration of the scheme has almost obscured, in the mind of the spectator, its ultimate object) strikes the final blow. Thus we read in Archimedes proposition after proposition the bearing of which is not immediately obvious but which we find infallibly used later on; and we are led by such easy stages that the difficulties of the original problem, as presented at the outset, are scarcely appreciated. As Plutarch says: “It is not possible to find in geometry more difficult and troublesome questions, or more simple and lucid explanations.” But it is decidedly a rhetorical exaggeration when Plutarch goes on to say that we are deceived by the easiness of the successive steps into the belief that anyone could have discovered them for himself. On the contrary, the studied simplicity and the perfect finish of the treatises involve at the same time an element of mystery. Though each step depends on the preceding ones, we are left in the dark as to how they were suggested to Archimedes. There is, in fact, much truth in a remark by Wallis to the effect that he seems “as it were of set purpose to have covered up the traces of his investigation as if he had grudged posterity the secret of his method of inquiry while he wished to extort from them assent to his results.” Wallis adds with equal reason that not only Archimedes but nearly all the ancients so hid away from posterity their method of Analysis (though it is certain that they had one) that more modern mathematicians found it easier to invent a new Analysis than to seek out the old.
In The Works of Archimedes (1897), Preface, vi.
One of the first and foremost duties of the teacher is not to give his students the impression that mathematical problems have little connection with each other, and no connection at all with anything else. We have a natural opportunity to investigate the connections of a problem when looking back at its solution.
In How to Solve It: A New Aspect of Mathematical Method (2004), 15.
One wonders whether a generation that demands instant satisfaction of all its needs and instant solution of the world’s problems will produce anything of lasting value. Such a generation, even when equipped with the most modern technology, will be essentially primitive - it will stand in awe of nature, and submit to the tutelage of medicine men.
In Reflections on the Human Condition (1973), 38.
Our atom of carbon enters the leaf, colliding with other innumerable (but here useless) molecules of nitrogen and oxygen. It adheres to a large and complicated molecule that activates it, and simultaneously receives the decisive message from the sky, in the flashing form of a packet of solar light; in an instant, like an insect caught by a spider, it is separated from its oxygen, combined with hydrogen and (one thinks) phosphorus, and finally inserted in a chain, whether long or short does not matter, but it is the chain of life. All this happens swiftly, in silence, at the temperature and pressure of the atmosphere, and gratis: dear colleagues, when we learn to do likewise we will be sicut Deus [like God], and we will have also solved the problem of hunger in the world.
Levi Primo and Raymond Rosenthal (trans.), The Periodic Table (1975, 1984), 227-228. In this final section of his book, Levi imagines the life of a carbon atom. He calls this his first “literary dream”. It came to him at Auschwitz.
Pain is a sensation produced by something contrary to the course of nature and this sensation is set up by one of two circumstances: either a very sudden change of the temperament (or the bad effect of a contrary temperament) or a solution of continuity.
— Avicenna
'A General Discussion of the Causes of Pain', in The Canon of Medicine, adapted by L. Bakhtiar (1999), 246.
Part of the charm in solving a differential equation is in the feeling that we are getting something for nothing. So little information appears to go into the solution that there is a sense of surprise over the extensive results that are derived.
Co-author with Jules Alphonse Larrivee, Mathematics and Computers (1957), 40.
Particular and contingent inventions in the solution of problems, which, though many times more concise than a general method would allow, yet, in my judgment, are less proper to instruct a learner, as acrostics, and such kind of artificial poetry, though never so excellent, would be but improper examples to instruct one that aims at Ovidean poetry.
In Letter to Collins (Macclesfield, 1670), Correspondence of Scientific Men (1841), Vol. 2, 307.
People who are unused to learning, learn little, and that slowly, while those more accustomed do much more and do it more easily. The same thing also happens in connection with research. Those who are altogether unfamiliar with this become blinded and bewildered as soon as their minds begin to work: they readily withdraw from the inquiry, in a state of mental fatigue and exhaustion, much like people who attempt to race without having been trained. He, on the other hand, who is accustomed to research, seeks and penetrates everywhere mentally, passing constantly from one topic to another; nor does he ever give up his investigation; he pursues it not merely for a matter of days, but throughout his whole life. Also by transferring his mind to other ideas which are yet not foreign to the questions at issue, he persists till he reaches the solution.
'On Paralysis'. Quoted in A. J. Brock, Greek Medicine: Being Extracts Illustrative of Medical Writers from Hippocrates to Galen (1929), 185.
Perhaps I can best describe my experience of doing mathematics in terms of a journey through a dark unexplored mansion. You enter the first room of the mansion and it’s completely dark. You stumble around bumping into the furniture, but gradually you learn where each piece of furniture is. Finally, after six months or so, you find the light switch, you turn it on, and suddenly it’s all illuminated. You can see exactly where you were. Then you move into the next room and spend another six months in the dark. So each of these breakthroughs, while sometimes they’re momentary, sometimes over a period of a day or two, they are the culmination of—and couldn’t exist without—the many months of stumbling around in the dark that proceed them.
Quoted in interview for website for PBS TV Nova program, 'The Proof'.
Physics is becoming so unbelievably complex that it is taking longer and longer to train a physicist. It is taking so long, in fact, to train a physicist to the place where he understands the nature of physical problems that he is already too old to solve them.
As quoted by Colin Pittendrigh (1971). In George C. Beakley, Ernest G. Chilton, Introduction to Engineering Design and Graphics (1973), 40
Prolonged commitment to mathematical exercises in economics can be damaging. It leads to the atrophy of judgement and intuition which are indispensable for real solutions and, on occasion, leads also to a habit of mind which simply excludes the mathematically inconvenient factors from consideration.
In Economics, Peace, and Laughter (1981), 41, footnote.
Questions that pertain to the foundations of mathematics, although treated by many in recent times, still lack a satisfactory solution. Ambiguity of language is philosophy's main source of problems. That is why it is of the utmost importance to examine attentively the very words we use.
Arithmetices Principia, (1889)
Religious leaders and men of science have the same ideals; they want to understand and explain the universe of which they are part; they both earnestly desire to solve, if a solution be ever possible, that great riddle: Why are we here?
Concerning Man's Origin (1927), viii.
Research may start from definite problems whose importance it recognizes and whose solution is sought more or less directly by all forces. But equally legitimate is the other method of research which only selects the field of its activity and, contrary to the first method, freely reconnoitres in the search for problems which are capable of solution. Different individuals will hold different views as to the relative value of these two methods. If the first method leads to greater penetration it is also easily exposed to the danger of unproductivity. To the second method we owe the acquisition of large and new fields, in which the details of many things remain to be determined and explored by the first method.
In Zum Gedächtniss an Julius Plucker', Göttinger Abhandlungen (1871), 16, Mathematische Classe, 6.
Round about what is, lies a whole mysterious world of might be, — a psychological romance of possibilities and things that do not happen. By going out a few minutes sooner or later, by stopping to speak with a friend at a corner, by meeting this man or that, or by turning down this street instead of the other, we may let slip some great occasion good, or avoid some impending evil, by which the whole current of our lives would have been changed. There is no possible solution to the dark enigma but the one word, “Providence.”
In 'Table-Talk', The Poetical Works of Henry Wadsworth Longfellow: Volume 3 (1883), 1354.
Rules of Thumb
Thumb’s First Postulate: It is better to use a crude approximation and know the truth, plus or minus 10 percent, than demand an exact solution and know nothing at all.
Thumb’s Second Postulate: An easily understood, workable falsehood is more useful than a complex incomprehensible truth.
Thumb’s First Postulate: It is better to use a crude approximation and know the truth, plus or minus 10 percent, than demand an exact solution and know nothing at all.
Thumb’s Second Postulate: An easily understood, workable falsehood is more useful than a complex incomprehensible truth.
In Arthur Bloch, The Complete Murphy's Law: A Definitive Collection (1991), 126.
Salt water when it turns into vapour becomes sweet, and the vapour does not form salt water when it condenses again. This I know by experiment. The same thing is true in every case of the kind: wine and all fluids that evaporate and condense back into a liquid state become water. They all are water modified by a certain admixture, the nature of which determines their flavour.
[Aristotle describing his distillation experiment.]
[Aristotle describing his distillation experiment.]
Meteorology (350 B.C.), Book II, translated by E. W. Webster. Internet Classics Archive, (classics.mit.edu).
Samoa culture demonstrates how much the tragic or the easy solution of the Oedipus situation depends upon the inter-relationship between parents and children, and is not created out of whole cloth by the young child’s biological impulses.
Male and Female: A Study of the Sexes in a Changing World (1949), 119.
Science by itself produces a very badly deformed man who becomes rounded out into a useful creative being only with great difficulty and large expenditure of time. … It is a much smaller matter to both teach and learn pure science than it is to intelligently apply this science to the solution of problems as they arise in daily life.
As quoted in Gary W. Matkin, Technology Transfer and the University (1990), 24.
Science cannot solve the ultimate mystery of nature. And that is because, in the last analysis, we ourselves are part of nature and therefore part of the mystery that we are trying to solve. Music and art are, to an extent, also attempts to solve or at least express the mystery. But to my mind the more we progress with either the more we are brought into harmony with all nature itself. And that is one of the great services of science to the individual.
In Max Planck and James Vincent Murphy (trans.), Where is Science Going?, (1932), Epilogue, 217.
Science fiction writers foresee the inevitable, and although problems and catastrophes may be inevitable, solutions are not.
'How Easy to See the Future'. In Asimov on Science Fiction (1981), 86.
Science is a game—but a game with reality, a game with sharpened knives … If a man cuts a picture carefully into 1000 pieces, you solve the puzzle when you reassemble the pieces into a picture; in the success or failure, both your intelligences compete. In the presentation of a scientific problem, the other player is the good Lord. He has not only set the problem but also has devised the rules of the game—but they are not completely known, half of them are left for you to discover or to deduce. The experiment is the tempered blade which you wield with success against the spirits of darkness—or which defeats you shamefully. The uncertainty is how many of the rules God himself has permanently ordained, and how many apparently are caused by your own mental inertia, while the solution generally becomes possible only through freedom from its limitations.
Quoted in Walter Moore, Schrödinger: Life and Thought (1989), 348.
Science is bound by the everlasting law of honour, to face fearlessly every problem which can fairly be presented to it. If a probable solution, consistent with the ordinary course of nature, can be found, we must not invoke an abnormal act of Creative Power.
From Inaugural Address of the President (2 Aug 1871) to the Edinburgh meeting of the British Association. Published in Chemical News and Journal of Industrial Science (4 Aug 1871), 24, 55.
Science is the search for truth. It is not a game in which one tries to beat his opponent, to do harm to others. We need to have the spirit of science in international affairs, to make the conduct of international affairs the effort to find the right solution, the just solution of international problems, not the effort by each nation to get the better of other nations, to do harm to them when it is possible.
In No More War! (1958).
Science tells us how very far we are from attaining our industrial aims with anything approaching the theoretical expenditure of force. Science also tells us in what directions we may look forward to arriving at improvements. I might say that we are on the eve of creating a science of invention, that is, of developing scientific methods for solving industrial problems.
From paper at inaugural meeting of the Society of the Chemical Industry, London (1881), 'Patent Laws as Applied to Chemical Invention', quoted in obituary by Charles Baskerville, 'Ludwig Mond', The Engineering and Mining Journal (1 Jan 1910), 89, No. 1, 3.
Scientists don’t really ever grow up. I read, as a 10-or-so-year-old, a book for kids by Einstein. I think it was The Meaning of Relativity. It was exciting! Science was compared to a detective story, replete with clues, and the solution was the search for a coherent account of all the known events. Then I remember some very entrapping biographies: Crucibles, by Bernard Jaffe, was the story of chemistry told through the lives of great chemists; Microbe Hunters, by Paul de Kruif, did the same for biologists. Also, the novel Arrowsmith, by Sinclair Lewis, about a medical researcher. These books were a crucial component of getting hooked into science.
When asked by Discover magazine what books helped inspire his passion as a scientist.
When asked by Discover magazine what books helped inspire his passion as a scientist.
In 'The 1998 Discover Science Gift Guide: Fantastic Voyages Children's Books That Mattered', Discover (Dec 1998).
Scientists often have a naive faith that if only they could discover enough facts about a problem, these facts would somehow arrange themselves in a compelling and true solution.
In Mankind Evolving: The Evolution of the Human Species, 128. As cited in Ted Woods & Alan Grant, Reason in Revolt - Dialectical Philosophy and Modern Science (2003), Vol. 2, 183.
Search the scriptures of human achievement and you cannot find any to equal in beneficence the introduction of Anæsthesia, Sanitation, with ail that it includes, and Asepsis—a short half century’s contribution towards the practical solution of the problems of human suffering, regarded as eternal and insoluble.
Address to the Canadian Medical association, Montreal (1902). Collected in 'Chavinism in Medicine', Aequanimitas (1904), 283.
Sociobiology is not just any statement that biology, genetics, and evolutionary theory have something to do with human behavior. Sociobiology is a specific theory about the nature of genetic and evolutionary input into human behavior. It rests upon the view that natural selection is a virtually omnipotent architect, constructing organisms part by part as best solutions to problems of life in local environments. It fragments organisms into “traits,” explains their existence as a set of best solutions, and argues that each trait is a product of natural selection operating “for” the form or behavior in question. Applied to humans, it must view specific behaviors (not just general potentials) as adaptations built by natural selection and rooted in genetic determinants, for natural selection is a theory of genetic change. Thus, we are presented with unproved and unprovable speculations about the adaptive and genetic basis of specific human behaviors: why some (or all) people are aggressive, xenophobic, religious, acquisitive, or homosexual.
In Hen's Teeth and Horses Toes (1983, 2010), 242-243.
Some men said the atomic bomb should never have been built; researchers should have stopped working when they had realized that the bomb was feasible. Enrico did not think this would have been a sensible solution. It is no good trying to stop knowledge from going forward. Whatever Nature has in store for mankind, unpleasant as it may be, men must accept, for ignorance is never better than knowledge.
Note: Although attributed as his viewpoint to Enrico Fermi, it is probably not a direct quote by him.
Note: Although attributed as his viewpoint to Enrico Fermi, it is probably not a direct quote by him.
This is the viewpoint of Enrico Fermi, as written in her own words by his wife, Laura Fermi, in Atoms in the Family: My Life with Enrico Fermi (1954), 244. The last sentence is also seen on its own, contracted as: “It is no good to try to stop knowledge from going forward. Ignorance is never better that knowledge.” Webmaster has therefore flags each of these wordings as “not a direct quote”, with this cautionary note. If you know a primary source of the quote in this wording, please contact Webmaster.
Some problems are just too complicated for rational logical solutions. They admit of insights, not answers.
In 'Profiles: A Scientist’s Advice II' by D. Lang, The New Yorker (26 Jan 1963).
Success in the solution of a problem generally depends in a great measure on the selection of the most appropriate method of approaching it; many properties of conic sections (for instance) being demonstrable by a few steps of pure geometry which would involve the most laborious operations with trilinear co-ordinates, while other properties are almost self-evident under the method of trilinear co-ordinates, which it would perhaps be actually impossible to prove by the old geometry.
In Trilinear Coordinates and Other Methods of Modern Analytical Geometry of Two Dimensions (1866), 154.
Taking advantage of the method, found by me, of the black staining of the elements of the brain, staining obtained by the prolonged immersion of the pieces, previously hardened with potassium or ammonium bichromate, in a 0.50 or 1.0% solution of silver nitrate, I happened to discover some facts concerning the structure of the cerebral gray matter that I believe merit immediate communication.
'On the Structure of the Gray Matter of the Brain', Gazetta Medica Italiana, 2 Aug 1873. Trans. Maurizio Santini (ed.), Golgi Centennial Symposium: Perspectives in Neurobiology (1975), 647.
Technology can relieve the symptoms of a problem without affecting the underlying causes. Faith in technology as the ultimate solution to all problems can thus divert our attention from the most fundamental problem—the problem of growth in a finite system
et al., The Limits to Growth (1972).
The alternative to the Big Bang is not, in my opinion, the steady state; it is instead the more general theory of continuous creation. Continuous creation can occur in bursts and episodes. These mini-bangs can produce all the wonderful element-building that Fred Hoyle discovered and contributed to cosmology. This kind of element and galaxy formation can take place within an unbounded, non-expanding universe. It will also satisfy precisely the Friedmann solutions of general relativity. It can account very well for all the facts the Big Bang explains—and also for those devastating, contradictory observations which the Big Bang must, at all costs, pretend are not there
In 'Letters: Wrangling Over the Bang', Science News (27 Jul 1991), 140, No. 4, 51. Also quoted in Roy C. Martin, Astronomy on Trial: A Devastating and Complete Repudiation of the Big Bang Fiasco (1999), Appendix I, 217.
The art of research [is] the art of making difficult problems soluble by devising means of getting at them.
Pluto's Republic (1982), 2.
The century of biology upon which we are now well embarked is no matter of trivialities. It is a movement of really heroic dimensions, one of the great episodes in man’s intellectual history. The scientists who are carrying the movement forward talk in terms of nucleo-proteins, of ultracentrifuges, of biochemical genetics, of electrophoresis, of the electron microscope, of molecular morphology, of radioactive isotopes. But do not be misled by these horrendous terms, and above all do not be fooled into thinking this is mere gadgetry. This is the dependable way to seek a solution of the cancer and polio problems, the problems of rheumatism and of the heart. This is the knowledge on which we must base our solution of the population and food problems. This is the understanding of life.
Letter to H. M. H. Carsan (17 Jun 1949). Quoted in Raymond B. Fosdick, The Story of the Rockefeller Foundation (1952), 166.
The contingency of history (both for life in general and for the cultures of Homo sapiens) and human free will (in the factual rather than theological sense) are conjoined concepts, and no better evidence can be produced than the ‘experimental’ production of markedly different solutions in identical environments.
…...
The difficulty lies not in solving problems but expressing them.
From 'The Evolution of Chastity' (Feb 1934), as translated by René Hague in Toward the Future (1975), 86.
The discovery of an interaction among the four hemes made it obvious that they must be touching, but in science what is obvious is not necessarily true. When the structure of hemoglobin was finally solved, the hemes were found to lie in isolated pockets on the surface of the subunits. Without contact between them how could one of them sense whether the others had combined with oxygen? And how could as heterogeneous a collection of chemical agents as protons, chloride ions, carbon dioxide, and diphosphoglycerate influence the oxygen equilibrium curve in a similar way? It did not seem plausible that any of them could bind directly to the hemes or that all of them could bind at any other common site, although there again it turned out we were wrong. To add to the mystery, none of these agents affected the oxygen equilibrium of myoglobin or of isolated subunits of hemoglobin. We now know that all the cooperative effects disappear if the hemoglobin molecule is merely split in half, but this vital clue was missed. Like Agatha Christie, Nature kept it to the last to make the story more exciting. There are two ways out of an impasse in science: to experiment or to think. By temperament, perhaps, I experimented, whereas Jacques Monod thought.
From essay 'The Second Secret of Life', collected in I Wish I'd Made You Angry Earlier (1998), 263-5.
The discrepancy between what was expected and what has been observed has grown over the years, and we're straining harder and harder to fill the gap.
[Commenting on the 1984 article in Nature discrediting neutrinos as the explanation for the missing mass of the universe, leaving astrophysicists more baffled for a solution.]
[Commenting on the 1984 article in Nature discrediting neutrinos as the explanation for the missing mass of the universe, leaving astrophysicists more baffled for a solution.]
In 'If Theory is Right, Most of Universe is Still “Missing”', New York Times (11 Sep 1984).
The efforts of the great philosopher [Newton] were always superhuman; the questions which he did not solve were incapable of solution in his time
In 'Eulogy on Laplace', Smithsonian Report (1874), 133.
The equation of animal and vegetable life is too complicated a problem for human intelligence to solve, and we can never know how wide a circle of disturbance we produce in the harmonies of nature when we throw the smallest pebble into the ocean of organic life.
Man and Nature, (1864), 103.
The essence of engineering consists not so much in the mere construction of the spectacular layouts or developments, but in the invention required—the analysis of the problem, the design, the solution by the mind which directs it all.
As quoted, “he said to the writer in effect,” Robert Fletcher, 'William Hood '67, Chief Engineer of the Southern Pacific Railroad Lines, Dartmouth Alumni Magazine (1919), Vol. 11, 223.
The Excellence of Modern Geometry is in nothing more evident, than in those full and adequate Solutions it gives to Problems; representing all possible Cases in one view, and in one general Theorem many times comprehending whole Sciences; which deduced at length into Propositions, and demonstrated after the manner of the Ancients, might well become the subjects of large Treatises: For whatsoever Theorem solves the most complicated Problem of the kind, does with a due Reduction reach all the subordinate Cases.
In 'An Instance of the Excellence of Modern Algebra, etc', Philosophical Transactions, 1694, 960.
The first step in finding the solution to a problem often involves discovering a problem with the existing solution.
The formulation of a problem is often more essential than its solution, which may be merely a matter of mathematical or experimental skill. To raise new questions, new possibilities, to regard old problems from a new angle requires creative imagination and marks real advances in science.
In Albert Einstein and Léopold Infeld, The Evolution of Physics: The Growth of Ideas from Early Concepts to Relativity and Quanta (1938, 1966), 92.
THE fundamental questions in chemistry,—those questions the answers to which would convert chemistry into a branch of exact science, and enable us to predict with absolute certainty the result of every reaction—are (1) What is the nature of the forces which retain the several molecules or atoms of a compound together? and (2) How may their direction and amount be determined? We may safely say that, in the present state of the science, these questions cannot be answered; and it is extremely doubtful whether any future advances will render their solution possible.
Opening paragraph of his University of Edinburgh M.D. thesis, 'On the Theory of Chemical Combination' (1861).
The future mathematician ... should solve problems, choose the problems which are in his line, meditate upon their solution, and invent new problems. By this means, and by all other means, he should endeavor to make his first important discovery: he should discover his likes and dislikes, his taste, his own line.
How to Solve it: A New Aspect of Mathematical Method (1957), 206.
The greatest achievements in the science of this [twentieth] century are themselves the sources of more puzzlement than human beings have ever experienced. Indeed, it is likely that the twentieth century will be looked back at as the time when science provided the first close glimpse of the profundity of human ignorance. We have not reached solutions; we have only begun to discover how to ask questions.
In 'On Science and Certainty', Discover Magazine (Oct 1980).
The greatest challenge facing mankind is the challenge of distinguishing reality from fantasy, truth from propaganda. We must daily decide whether the threats we face are real, whether the solutions we are offered will do any good, whether the problems we’re told exist are in fact real problems, or non-problems.
…...
The greatest spiritual revolutionary Western history, Saint Francis, proposed what he thought was an alternative Christian view of nature and man’s relation to it: he tried to substitute the idea of the equality of creatures, including man, for the idea of man’s limitless rule of creation. He failed. Both our present science and our present technology are so tinctured with orthodox Christian arrogance toward nature that no solution for our ecologic crisis can be expected from them alone. Since the roots of our trouble are so largely religious, the remedy must also be essentially religious, whether we call it that or not. We must rethink and refeel our nature and destiny. The profoundly religious, but heretical, sense of the primitive Franciscans for the spiritual autonomy of all parts of nature may point a direction. I propose Francis as a patron saint for ecologists.
In The Historical Roots of our Ecologic Crisis (1967), 1207.
The idea formed itself in my mind that if I could get a solution of alumina in something which contained no water, and in a solvent which was chemically more stable than the alumina, this would probably give a bath from which aluminum could be obtained by electrolysis.
In 'The Perkin Medal Award', The Journal of Industrial and Engineering Chemistry (Mar 1911), 3, No. 3, 147.
The imaginary expression √(-a) and the negative expression -b, have this resemblance, that either of them occurring as the solution of a problem indicates some inconsistency or absurdity. As far as real meaning is concerned, both are imaginary, since 0 - a is as inconceivable as √(-a).
The importance of a result is largely relative, is judged differently by different men, and changes with the times and circumstances. It has often happened that great importance has been attached to a problem merely on account of the difficulties which it presented; and indeed if for its solution it has been necessary to invent new methods, noteworthy artifices, etc., the science has gained more perhaps through these than through the final result. In general we may call important all investigations relating to things which in themselves are important; all those which have a large degree of generality, or which unite under a single point of view subjects apparently distinct, simplifying and elucidating them; all those which lead to results that promise to be the source of numerous consequences; etc.
From 'On Some Recent Tendencies in Geometric Investigations', Rivista di Matematica (1891), 44. In Bulletin American Mathematical Society (1904), 444.
The intellect has little to do on the road to discovery. There comes a leap in consciousness, call it intuition or what you will, and the solution comes to you and you don’t know why or how.
Quoted in Forbes (15 Sep 1974). In Larry Chang, Wisdom for the Soul (2006), 179.
The life and soul of science is its practical application, and just as the great advances in mathematics have been made through the desire of discovering the solution of problems which were of a highly practical kind in mathematical science, so in physical science many of the greatest advances that have been made from the beginning of the world to the present time have been made in the earnest desire to turn the knowledge of the properties of matter to some purpose useful to mankind.
From 'Electrical Units of Measurement', a lecture delivered at the Institution of Civil Engineers, London (3 May 1883), Popular Lectures and Addresses Vol. 1 (1891), 86-87.
The logic of the subject [algebra], which, both educationally and scientifically speaking, is the most important part of it, is wholly neglected. The whole training consists in example grinding. What should have been merely the help to attain the end has become the end itself. The result is that algebra, as we teach it, is neither an art nor a science, but an ill-digested farrago of rules, whose object is the solution of examination problems. … The result, so far as problems worked in examinations go, is, after all, very miserable, as the reiterated complaints of examiners show; the effect on the examinee is a well-known enervation of mind, an almost incurable superficiality, which might be called Problematic Paralysis—a disease which unfits a man to follow an argument extending beyond the length of a printed octavo page.
In Presidential Address British Association for the Advancement of Science (1885), Nature, 32, 447-448.
The man in the street will, therefore, twist the statement that the scientist has come to the end of meaning into the statement that the scientist has penetrated as far as he can with the tools at his command, and that there is something beyond the ken of the scientist. This imagined beyond, which the scientist has proved he cannot penetrate, will become the playground of the imagination of every mystic and dreamer. The existence of such a domain will be made the basis of an orgy of rationalizing. It will be made the substance of the soul; the spirits of the dead will populate it; God will lurk in its shadows; the principle of vital processes will have its seat here; and it will be the medium of telepathic communication. One group will find in the failure of the physical law of cause and effect the solution of the age-long problem of the freedom of the will; and on the other hand the atheist will find the justification of his contention that chance rules the universe.
Reflections of a Physicist (1950),102-3.
The mathematicians have been very much absorbed with finding the general solution of algebraic equations, and several of them have tried to prove the impossibility of it. However, if I am not mistaken, they have not as yet succeeded. I therefore dare hope that the mathematicians will receive this memoir with good will, for its purpose is to fill this gap in the theory of algebraic equations.
Opening of Memoir on Algebraic Equations, Proving the Impossibility of a Solution of the General Equation of the Fifth Degree. The paper was originally published (1824) in French, as a pamphlet, in Oslo. Collected in Œuvres Complètes (1881), Vol. 1, 28. Translation by W.H. Langdon collected in David Eugene Smith, A Source Book in Mathematics (2012), 261. In this work, he showed why—despite two centuries of efforts by mathematicians—solving equations of the fifth degree would remain futile. The insights from this paper led to the modern theory of equations.
The method of inquiry which all our ingenious Theorists of the Earth have pursued is certainly erroneous. They first form an hypothesis to solve the phenomena, but in fact the Phenomena are always used as a prop to the hypothesis.
Instead therefore of attempting to cut the gordian knot by Hypothetical analysis, we shall follow the synthetic method of inquiry and content ourselves with endeavouring to establish facts rather than attempt solutions and try by experiments how far that method may leave us thro' the mazes of this subject
Instead therefore of attempting to cut the gordian knot by Hypothetical analysis, we shall follow the synthetic method of inquiry and content ourselves with endeavouring to establish facts rather than attempt solutions and try by experiments how far that method may leave us thro' the mazes of this subject
Introduction to his lecture course. In Robert Jameson, edited by H. W. Scott, Lectures on Geology, (1966), 27. In Patrick Wyse Jackson, Four Centuries of Geological Travel (2007), 33.
The mind of man may be compared to a musical instrument with a certain range of notes, beyond which in both directions we have an infinitude of silence. The phenomena of matter and force lie within our intellectual range, and as far as they reach we will at all hazards push our inquiries. But behind, and above, and around all, the real mystery of this universe [Who made it all?] lies unsolved, and, as far as we are concerned, is incapable of solution.
In 'Matter and Force', Fragments of Science for Unscientific People (1871), 93.
The moment philosophy supposes it can find a final and comprehensive solution, it ceases to be inquiry and becomes either apologetics or propaganda.
Logic (1938), 42.
The most direct, and in a sense the most important, problem which our conscious knowledge of Nature should enable us to solve is the anticipation of future events, so that we may arrange our present affairs in accordance with such anticipation. As a basis for the solution of this problem we always make use of our knowledge of events which have already occurred, obtained by chance observation or by prearranged experiment.
In Heinrich Hertz, D.E. Jones (trans.) and J.T. Walley (trans.), 'Introduction', The Principles of Mechanics (1899), 1.
The most practical solution is a good theory.
Epigraph, without citation, in Eberhard Zeidler, Applied Functional Analysis: main principles and their applications (1995), 1.
The mystery of life is certainly the most persistent problem ever placed before the thought of man. There is no doubt that from the time humanity began to think it has occupied itself with the problem of its origin and its future which undoubtedly is the problem of life. The inability of science to solve it is absolute. This would be truly frightening were it not for faith.
Address (10 Sep 1934) to the International Congress of Electro-Radio Biology, Venice. In Associated Press, 'Life a Closed Book, Declares Marconi', New York Times (11 Sep 1934), 15.
The night before Easter Sunday of that year (1920) I awoke, turned on the light, and jotted down a few notes on a tiny slip of thin paper. Then I fell asleep again. It occurred to me at six o’clock in the morning that during the night I had written down something most important, but I was unable to decipher the scrawl. The next night, at three o’clock, the idea returned. It was the design of an experiment to determine whether the hypothesis of chemical transmission that I had uttered seventeen years ago was correct. I got up immediately, went to the laboratory, and performed a simple experiment on a frog heart according to the nocturnal design. I have to describe this experiment briefly since its results became the foundation of the theory of chemical transmission of the nervous impulse. The hearts of two frogs were isolated, the first with its nerves, the second without. Both hearts were attached to Straub cannulas filled with a little Ringer solution. The vagus nerve of the first heart was stimulated for a few minutes. Then the Ringer solution that had been in the first heart during the stimulation of the vagus was transferred to the second heart. It slowed and its beats diminished just as if its vagus had been stimulated. Similarly, when the accelerator nerve was stimulated and the Ringer from this period transferred, the second heart speeded up and its beats increased. These results unequivocally proved that the nerves do not influence the heart directly but liberate from their terminals specific chemical substances which, in their turn, cause the well-known modifications of the function of the heart characteristic of the stimulation of its nerves.
'An Autobiographic Sketch', Perspectives in Biology and Medicine (1960), 4, 17.
The Ocean Health Index is like a thermometer of ocean health, which will allow us to determine how the patient is doing. The Index will be a measure of whether our policies are working, or whether we need new solutions.
As quoted in press release (14 Aug 2012), 'Ocean Health Index Provides First-Ever Global Benchmark of 171 Coastal Regions', on web page of Conservation International, conservation.org.
The only difference between a problem and a solution is that people understand the solution.
The origin of a science is usually to be sought for not in any systematic treatise, but in the investigation and solution of some particular problem. This is especially the case in the ordinary history of the great improvements in any department of mathematical science. Some problem, mathematical or physical, is proposed, which is found to be insoluble by known methods. This condition of insolubility may arise from one of two causes: Either there exists no machinery powerful enough to effect the required reduction, or the workmen are not sufficiently expert to employ their tools in the performance of an entirely new piece of work. The problem proposed is, however, finally solved, and in its solution some new principle, or new application of old principles, is necessarily introduced. If a principle is brought to light it is soon found that in its application it is not necessarily limited to the particular question which occasioned its discovery, and it is then stated in an abstract form and applied to problems of gradually increasing generality.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
In A Treatise on Projections (1880), Introduction, xi. Published as United States Coast and Geodetic Survey, Treasury Department Document, No. 61.
The present knowledge of the biochemical constitution of the cell was achieved largely by the use of destructive methods. Trained in the tradition of the theory of solutions, many a biochemist tends, even today, to regard the cell as a “bag of enzymes”. However, everyone realizes now that the biochemical processes studied in vitro may have only a remote resemblance to the events actually occurring in the living cell.
Nucleo-cytoplasmic Relations in Micro-Organisms: Their Bearing on Cell Heredity and Differentiation (1953), 108.
The problem of distinguishing prime numbers from composite numbers and of resolving the latter into their prime factors is known to be one of the most important and useful in arithmetic. It has engaged the industry and wisdom of ancient and modern geometers to such an extent that it would be superfluous to discuss the problem at length... Further, the dignity of the science itself seems to require that every possible means be explored for the solution of a problem so elegant and so celebrated.
Disquisitiones Arithmeticae (1801), Article 329
The question, What is cholera? is left unsolved. Concerning this, the fundamental point, all is darkness and confusion, vague theory, and a vain speculation. Is it a fungus, an insect, a miasm,
an electrical disturbance, a deficiency of ozone, a morbid offscouring from the intestinal canal? We know nothing; we are at sea, in a whirlpool of conjecture.
Editorial in Lancet 1 (1853), 393. As quoted in Peter Vinten-Johansen, et al., Cholera, Chloroform, and the Science of Medicine: A Life of John Snow (2003), 116.
The real achievement in discoveries … is seeing an analogy where no one saw one before. … The essence of discovery is that unlikely marriage of … previously unrelated forms of reference or universes of discourse, whose union will solve the previously insoluble problem.
In Act of Creation (1964), 201.
The real value of science is in the getting, and those who have tasted the pleasure of discovery alone know what science is. A problem solved is dead. A world without problems to be solved would be devoid of science.
In Matter and Energy (1912), 18.
The reason Dick's [Richard Feynman] physics was so hard for ordinary people to grasp was that he did not use equations. The usual theoretical physics was done since the time of Newton was to begin by writing down some equations and then to work hard calculating solutions of the equations. This was the way Hans [Bethe] and Oppy [Oppenheimer] and Julian Schwinger did physics. Dick just wrote down the solutions out of his head without ever writing down the equations. He had a physical picture of the way things happen, and the picture gave him the solutions directly with a minimum of calculation. It was no wonder that people who had spent their lives solving equations were baffled by him. Their minds were analytical; his was pictorial.
Quoted in Michio Kaku and Jennifer Trainer Thompson, Beyond Einstein: the Cosmic Quest for the Theory of the Universe (1987, 1999), 56-57, citing Freeman Dyson, Disturbing the Universe (1979, 1981), 55-56.
The riddles of God are more satisfying than the solutions of man.
In 'The Book of Job: An Introduction', Putnam’s Magazine and the Critic (Jun 1907), 2, No. 3, 356.
The scientist has marched in and taken the place of the poet. But one day somebody will find the solution to the problems of the world and remember, it will be a poet, not a scientist.
As quoted in The Star (1959). Collected in Jonathon Green, Morrow's International Dictionary of Contemporary Quotations (1982).
The scientist is not much given to talking of the riddle of the universe. “Riddle” is not a scientific term. The conception of a riddle is “something which can he solved.” And hence the scientist does not use that popular phrase. We don’t know the why of anything. On that matter we are no further advanced than was the cavedweller. The scientist is contented if he can contribute something toward the knowledge of what is and how it is.
As quoted in 'Electricity Will Keep The World From Freezing Up', New York Times (12 Nov 1911), SM4.
The skeptic does not mean him who doubts, but him who investigates or researches, as opposed to him who asserts and thinks that he has found. The one is the man who studies the problem and the other is the man who gives us a formula, correct or incorrect, as the solution of it.
'My Religion', Essays and Soliloquies, translated by John Ernest Crawford Flitch (1925), 56. In Robert Andrews, The Columbia Dictionary of Quotations (1993), 844:9.
The solution is dilution.
Aphorism, seen for example, in Frank R. Spellman and Nancy E. Whiting, Environmental Engineer’s Mathematics Handbook (2004), 291.
The solution of fallacies, which give rise to absurdities, should be to him who is not a first beginner in mathematics an excellent means of testing for a proper intelligible insight into mathematical truth, of sharpening the wit, and of confining the judgment and reason within strictly orderly limits
In 'Vorwort', Mathematische Sophismen (1864), 3. As translated and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-Book (1914), 89. From the original German, “Das Aufsuchen der Trugschlüsse, durch welche Ungereimtheiten entstellen, dürfte nun für den nicht ganz ersten Anfänger in der Mathematik ein vorzügliches Mittel sein, eine richtige begriffliche Einsicht in die mathematischen Wahrheiten zu erproben, den Verstand zu schärfen und das Urtheilen und Schliessen in streng geregelte Grenzen zu dämmen.”
The solution of problems is one of the lowest forms of mathematical research, … yet its educational value cannot be overestimated. It is the ladder by which the mind ascends into higher fields of original research and investigation. Many dormant minds have been aroused into activity through the mastery of a single problem.
With co-editor J. M. Colaw, Editorial introducing the first issue of The American Mathematical Monthly (Jan 1894), 1, No. 1, 2.
The solution of the difficulties which formerly surrounded the mathematical infinite is probably the greatest achievement of which our age has to boast.
In 'The Study of Mathematics', Philosophical Essays (1910), 77.
The solution, as all thoughtful people recognize, must lie in properly melding the themes of inborn predisposition and shaping through life’s experiences. This fruitful joining cannot take the false form of percentages adding to 100–as in ‘intelligence is 80 percent nature and 20 percent nurture,’ or ‘homosexuality is 50 percent inborn and 50 percent learned,’ and a hundred other harmful statements in this foolish format. When two ends of such a spectrum are commingled, the result is not a separable amalgam (like shuffling two decks of cards with different backs), but an entirely new and higher entity that cannot be decomposed (just as adults cannot be separated into maternal and paternal contributions to their totality).
…...
The solutions put forth by imperialism are the quintessence of simplicity...When they speak of the problems of population and birth, they are in no way moved by concepts related to the interests of the family or of society...Just when science and technology are making incredible advances in all fields, they resort to technology to suppress revolutions and ask the help of science to prevent population growth. In short, the peoples are not to make revolutions, and women are not to give birth. This sums up the philosophy of imperialism.
From Fidel Castro (1968).
The spectral density of black body radiation ... represents something absolute, and since the search for the absolutes has always appeared to me to be the highest form of research, I applied myself vigorously to its solution.
In Michael Dudley Sturge , Statistical and Thermal Physics (2003), 201.
The study of … simple cases would, I think, often be of advantage even to students whose mathematical attainments are sufficient to enable them to follow the solution of the more general cases. For in these simple cases the absence of analytical difficulties allows attention to be more easily concentrated on the physical aspects of the question, and thus gives the student a more vivid idea and a more manageable grasp of the subject than he would be likely to attain if he merely regarded electrical phenomena through a cloud of analytical symbols.
Elements of the Mathematical Theory of Electricity and Magnetism (189S), v-vi.
The technologists claim that if everything works [in a nuclear fission reactor] according to their blueprints, fission energy will be a safe and very attractive solution to the energy needs of the world. ... The real issue is whether their blueprints will work in the real world and not only in a “technological paradise.”...
Opponents of fission energy point out a number of differences between the real world and the “technological paradise.” ... No acts of God can be permitted.
Opponents of fission energy point out a number of differences between the real world and the “technological paradise.” ... No acts of God can be permitted.
'Energy and Environment', Bulletin of the Atomic Scientists (May 1972), 6.
The traditional mathematics professor of the popular legend is absentminded. He usually appears in public with a lost umbrella in each hand. He prefers to face a blackboard and to turn his back on the class. He writes a, he says b, he means c, but it should be d. Some of his sayings are handed down from generation to generation:
“In order to solve this differential equation you look at it till a solution occurs to you.”
“This principle is so perfectly general that no particular application of it is possible.”
“Geometry is the science of correct reasoning on incorrect figures.”
“My method to overcome a difficulty is to go round it.”
“What is the difference between method and device? A method is a device which you used twice.”
“In order to solve this differential equation you look at it till a solution occurs to you.”
“This principle is so perfectly general that no particular application of it is possible.”
“Geometry is the science of correct reasoning on incorrect figures.”
“My method to overcome a difficulty is to go round it.”
“What is the difference between method and device? A method is a device which you used twice.”
In How to Solve It: A New Aspect of Mathematical Method (2004), 208.
The traditional method of confronting the student not with the problem but with the finished solution means depriving him of all excitement, to shut off the creative impulse, to reduce the adventure of mankind to a dusty heap of theorems.
In The Act of Creation (1964), 266.
The transition from a paradigm in crisis to a new one from which a new tradition of normal science can emerge is far from a cumulative process, one achieved by an articulation or extension of the old paradigm. Rather it is a reconstruction of the field from new fundamentals, a reconstruction that changes some of the field's most elementary theoretical generalizations as well as many of its paradigm methods and applications. During the transition period there will be a large but never complete overlap between the problems that can be solved by the old and by the new paradigm. But there will also be a decisive difference in the modes of solution. When the transition is complete, the profession will have changed its view of the field, its methods, and its goals.
The Structure of Scientific Revolutions (1962), 84-5.
The Unexpected stalks a farm in big boots like a vagrant bent on havoc. Not every farmer is an inventor, but the good ones have the seeds of invention within them. Economy and efficiency move their relentless tinkering and yet the real motive often seems to be aesthetic. The mind that first designed a cutter bar is not far different from a mind that can take the intractable steel of an outsized sickle blade and make it hum in the end. The question is how to reduce the simplicity that constitutes a problem (“It's simple; it’s broke.”) to the greater simplicity that constitutes a solution.
In Making Hay (2003), 33-34.
The universe is governed by science. But science tells us that we can’t solve the equations, directly in the abstract. We need to use the effective theory of Darwinian natural selection of those societies most likely to survive. We assign them higher value.
[Answer to question: What is the value in knowing “Why are we here?”]
[Answer to question: What is the value in knowing “Why are we here?”]
'Stephen Hawking: "There is no heaven; it’s a fairy story"', interview in newspaper The Guardian (15 May 2011).
The Wegener hypothesis has been so stimulating and has such fundamental implications in geology as to merit respectful and sympathetic interest from every geologist. Some striking arguments in his favor have been advanced, and it would be foolhardy indeed to reject any concept that offers a possible key to the solution of profound problems in the Earth’s history.
Published while geologists remained sceptical of Alfred Wegener’s idea of Continental Drift, Though unconvinced, he published these thoughts suggesting that critics should be at least be open-minded. His patience was proven justified when two decades later, the theory of plate tectonics provided a mechanism for the motion of the continents.
Published while geologists remained sceptical of Alfred Wegener’s idea of Continental Drift, Though unconvinced, he published these thoughts suggesting that critics should be at least be open-minded. His patience was proven justified when two decades later, the theory of plate tectonics provided a mechanism for the motion of the continents.
Some Thoughts on the Evidence for Continental Drift (1944).
The work of a pioneer in science of technique often consists of finding a correct solution, or creating a working mechanism, based on laws that are not yet discovered.
In The Story of the Winged-S: The Autobiography of Igor I. Sikorsky (2011).
The work of the inventor consists of conceptualizing, combining, and ordering what is possible according to the laws of nature. This inner working out which precedes the external has a twofold characteristic: the participation of the subconscious in the inventing subject; and that encounter with an external power which demands and obtains complete subjugation, so that the way to the solution is experienced as the fitting of one's own imagination to this power.
Philosophie der Technik (1927). 'Technology in Its Proper Sphere' translated by William Carroll. In Carl Mitcham (ed.) and Robert Mackey (ed.), Philosophy and Technology: Readings in the Philosophical Problems of Technology, (1972), Vol. 14, 321. In David Lovekin, Technique, Discourse, and Consciousness (1991), 73.
The world is devoted to physical science, because it believes theses discoveries will increase its capacity of luxury and self-indulgence. But the pursuit of science only leads to the insoluble.
In Lothair (1879), 70.
There are problems to whose solution I would attach an infinitely greater importance than to those of mathematics, for example touching ethics, or our relation to God, or concerning our destiny and our future; but their solution lies wholly beyond us and completely outside the province of science.
Quoted in J.R. Newman, The World of Mathematics (1956), 314.
There are some four million different kinds of animals and plants in the world. Four million different solutions to the problems of staying alive.
As quoted in Jack Shepherd, "David Attenborough: 15 of the naturalist’s best quotes: In celebration of his 94th birthday", Independent (8 May 2017), on independent.co.uk website.
There are, at present, fundamental problems in theoretical physics … the solution of which … will presumably require a more drastic revision of our fundmental concepts than any that have gone before. Quite likely, these changes will be so great that it will be beyond the power of human intelligence to get the necessary new ideas by direct attempts to formulate the experimental data in mathematical terms. The theoretical worker in the future will, therefore, have to proceed in a more direct way. The most powerful method of advance that can be suggested at present is to employ all the resources of pure mathematics in attempts to perfect and generalize the mathematical formalism that forms the existing basis of theoretical physics, and after each success in this direction, to try to interpret the new mathematical features in terms of physical entities.
At age 28.
At age 28.
Proceedings of the Royal Society (1931), A133, 60. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 109.
There is always a well-known solution to every human problem—neat, plausible, and wrong.
As given in 'The Divine Afflatus', Prejudices: Second Series (1920), 157. Various paraphrases changing Mencken’s original words, are also found. For example, “…an easy solution…” or “Every complex problem has a solution that is simple, neat, and wrong.” Also sometimes seen both paraphrased and misattributed, for example, to Thomas Huxley. [See further discussion on quoteinvestigator.com website.]
There is more evidence to prove that saltiness [of the sea] is due to the admixture of some substance ... It is this stuff which makes salt water heavy (it weighs more than fresh water) and thick. The difference in consistency is such that ships with the same cargo very nearly sink in a river when they are quite fit to navigate in the sea. This circumstance has before now caused loss to shippers freighting their ships in a river. That the thicker consistency is due to an admixture of something is proved by the fact that if you make strong brine by the admixture of salt, eggs, even when they are full, float in it. It almost becomes like mud; such a quantity of earthy matter is there in the sea.
[Aristotle recognised the different density of fresh (river) or salty (sea) water. He describes an experiment using an egg (which sinks in fresh water) that floats in a strong brine solution.]
[Aristotle recognised the different density of fresh (river) or salty (sea) water. He describes an experiment using an egg (which sinks in fresh water) that floats in a strong brine solution.]
Meteorology (350 B.C.), Book II, translated by E. W. Webster. Internet Classics Archive, (classics.mit.edu).
There is one experiment which I always like to try, because it proves something whichever way it goes. A solution of iodine in water is shaken with bone-black, filtered and tested with starch paste. If the colorless solution does not turn the starch blue, the experiment shows how completely charcoal extracts iodine from aqueous solution. If the starch turns blue, the experiment shows that the solution, though apparently colorless, still contains iodine which can be detected by means of a sensitive starch test.
Applied Colloid Chemistry (1921), 111.
There was yet another disadvantage attaching to the whole of Newton’s physical inquiries, ... the want of an appropriate notation for expressing the conditions of a dynamical problem, and the general principles by which its solution must be obtained. By the labours of LaGrange, the motions of a disturbed planet are reduced with all their complication and variety to a purely mathematical question. It then ceases to be a physical problem; the disturbed and disturbing planet are alike vanished: the ideas of time and force are at an end; the very elements of the orbit have disappeared, or only exist as arbitrary characters in a mathematical formula
Address to the Mechanics Institute, 'An Address on the Genius and Discoveries of Sir Isaac Newton' (1835), excerpted in paper by Luis M. Laita, Luis de Ledesma, Eugenio Roanes-Lozano and
Alberto Brunori, 'George Boole, a Forerunner of Symbolic Computation', collected in John A. Campbell and Eugenio Roanes-Lozano (eds.), Artificial Intelligence and Symbolic Computation: International Conference AISC 2000 (2001), 3.
Think of a single problem confronting the world today. Disease, poverty, global warming… If the problem is going to be solved, it is science that is going to solve it. Scientists tend to be unappreciated in the world at large, but you can hardly overstate the importance of the work they do. If anyone ever cures cancer, it will be a guy with a science degree. Or a woman with a science degree.
Quoted in Max Davidson, 'Bill Bryson: Have faith, science can solve our problems', Daily Telegraph (26 Sep 2010)
This conviction of the solvability of every mathematical problem is a powerful incentive to the worker. We hear within us the perpetual call: There is the problem. Seek its solution. You can find it by pure reason, for in mathematics there is no ignorabimus!
Ignorabimus as used here, means “we will not know” (which is slightly different from ignoramus meaning present ignorance, “we do not know”). In Lecture (1900), 'Mathematische Probleme' (Mathematical Problems), to the International Congress of Mathematicians, Paris. From the original German reprinted in David Hilbert: Gesammelte Abhandlungen (Collected Treatises, 1970), Vol. 3, 298, “Diese Überzeugung von der Lösbarkeit eines jeden mathematischer Problems ist uns ein kräftiger Ansporn während der Arbeit ; wir hören in uns den steten Zuruf: Da ist das Problem, suche die Lösung. Du kannst sie durch reines Denken finden; denn in der Mathematik gibt es kein Ignorabimus. English version as translated by Dr. Maby Winton Newson for Bulletin of the American Mathematical Society (1902), 8, 437-479. The address was first published in Göttinger Nachrichten is Nachrichten von der Königl. Gesellschaft der Wiss. zu Göttingen (1900), 253-297; and Archiv der Mathematik und Physik (1901), 3, No. 1, 44-63.
This interpretation of the atomic number [as the number of orbital electrons] may be said to signify an important step toward the solution of the boldest dreams of natural science, namely to build up an understanding of the regularities of nature upon the consideration of pure number.
Atomic Theory and the Description of Nature (1934), 103-104Cited in Gerald James Holton, Thematic Origins of Scientific Thought: Kepler to Einstein (1985), 74.
This paper gives wrong solutions to trivial problems. The basic error, however, is not new.
In Mathematical Reviews 12, 561. As quoted and cited in P.R. Halmos, I Want to be a Mathematician: An Automathography (2013), 120
Thought-economy is most highly developed in mathematics, that science which has reached the highest formal development, and on which natural science so frequently calls for assistance. Strange as it may seem, the strength of mathematics lies in the avoidance of all unnecessary thoughts, in the utmost economy of thought-operations. The symbols of order, which we call numbers, form already a system of wonderful simplicity and economy. When in the multiplication of a number with several digits we employ the multiplication table and thus make use of previously accomplished results rather than to repeat them each time, when by the use of tables of logarithms we avoid new numerical calculations by replacing them by others long since performed, when we employ determinants instead of carrying through from the beginning the solution of a system of equations, when we decompose new integral expressions into others that are familiar,—we see in all this but a faint reflection of the intellectual activity of a Lagrange or Cauchy, who with the keen discernment of a military commander marshalls a whole troop of completed operations in the execution of a new one.
In Populär-wissenschafliche Vorlesungen (1903), 224-225.
Through the discovery of Buchner, Biology was relieved of another fragment of mysticism. The splitting up of sugar into CO2 and alcohol is no more the effect of a 'vital principle' than the splitting up of cane sugar by invertase. The history of this problem is instructive, as it warns us against considering problems as beyond our reach because they have not yet found their solution.
The Dynamics of Living Matter (1906), 22.
Throughout history, engineers have served their neighbours, their towns and their countries by making tools, machines and countless other things that improve every aspect of life. From information technology to medical science and mining, from building roads to space travel, engineers are working to make a difference to our standard of living, and with it our health, wealth and happiness. At its heart, engineering is about using science to find creative, practical solutions. It is a noble profession.
In Speech (25 Jun 2013), for the 2013 Queen Elizabeth Prize for Engineering.
To appreciate a work of art we need bring with us nothing from life, no knowledge of its ideas and affairs, no familiarity with its emotions. Art transports us from the world of man’s activity to a world of æsthetic exaltation. For a moment we are shut off from human interests; our anticipations and memories are arrested; we are lifted above the stream of life. The pure mathematician rapt in his studies knows a state of mind which I take to be similar, if not identical. He feels an emotion for his speculations which arises from no perceived relation between them and the lives of men, but springs, inhuman or super-human, from the heart of an abstract science. I wonder, sometimes, whether the appreciators of art and of mathematical solutions are not even more closely allied.
In Art (1913), 25.
To solve a problem is to create new problems, new knowledge immediately reveals new areas of ignorance, and the need for new experiments. At least, in the field of fast reactions, the experiments do not take very long to perform.
From Nobel Lecture (11 Dec 1967), 'Flash Photolysis and Some of its Applications.' In Nobel Lectures: Chemistry 1963-1970 (1972), 261.
Today's water institutions—the policies and laws, government agencies and planning and engineering practices that shape patterns of water use—are steeped in a supply-side management philosophy no longer appropriate to solving today's water problems.
From a study Postel wrote for Worldwatch Institute, quoted in New York Times (22 Sep 1985), 19.
Train yourselves. Don’t wait to be fed knowledge out of a book. Get out and seek it. Make explorations. Do your own research work. Train your hands and your mind. Become curious. Invent your own problems and solve them. You can see things going on all about you. Inquire into them. Seek out answers to your own questions. There are many phenomena going on in nature the explanation of which cannot be found in books. Find out why these phenomena take place. Information a boy gets by himself is enormously more valuable than that which is taught to him in school.
In 'Dr. Irving Langmuir', Boys' Life (Jul 1941), 12.
Typical of the fundamental scientific problems whose solution should lead to important industrial consequences are, for example, the release of atomic energy, which experiment has shown to exist in quantities millions of times greater than is liberated by combustion.
An early speculation on using the amount of energy that could be released from uranium atoms. In a letter to Henry Ford (18 May 1931). He recorded earlier thoughts on the subject in his Research Notebook, entry for 23 Jul 1930, in Arthur H. Compton Notebooks, Washington University, St. Louis, and AIP. Cited by Stanley Coben, in 'The Scientific Establishment and the Transmission of Quantum Mechanics to the United States, 1919-32', The American Historical Review (Apr 1971), 76, No. 2, 466.
Understanding a theory has, indeed, much in common with understanding a human personality. We may know or understand a man's system of dispositions pretty well; that is to say, we may be able to predict how he would act in a number of different situations. But since there are infinitely many possible situations, of infinite variety, a full understanding of a man's dispositions does not seem to be possible.
Objective Knowledge: an Evolutionary Approach (1972), 299.
Very little comes easily to our poor, benighted species (the first creature, after all, to experiment with the novel evolutionary inventions of self-conscious philosophy and art). Even the most ‘obvious,’ ‘accurate,’ and ‘natural’ style of thinking or drawing must be regulated by history and won by struggle. Solutions must therefore arise within a social context and record the complex interactions of mind and environment that define the possibility of human improvement.
…...
We academic scientists move within a certain sphere, we can go on being useless up to a point, in the confidence that sooner or later some use will be found for our studies. The mathematician, of course, prides himself on being totally useless, but usually turns out to be the most useful of the lot. He finds the solution but he is not interested in what the problem is: sooner or later, someone will find the problem to which his solution is the answer.
'Concluding Remarks', Proceedings of the Royal Society of London, Series A, A Discussion of New Materials, 1964, 282, 152-3.
We are at the very beginning of time for the human race. It is not unreasonable that we grapple with problems. But there are tens of thousands of years in the future. Our responsibility is to do what we can, learn what we can, improve the solutions, and pass them on.
…...
We are concerned to understand the motivation for the development of pure mathematics, and it will not do simply to point to aesthetic qualities in the subject and leave it at that. It must be remembered that there is far more excitement to be had from creating something than from appreciating it after it has been created. Let there be no mistake about it, the fact that the mathematician is bound down by the rules of logic can no more prevent him from being creative than the properties of paint can prevent the artist. … We must remember that the mathematician not only finds the solutions to his problems, he creates the problems themselves.
In A Signpost to Mathematics (1951), 19. As quoted and cited in William L. Schaaf, 'Memorabilia Mathematica', The Mathematics Teacher (Mar 1957), 50, No. 3, 230. Note that this paper incorrectly attributes “A.H. Head”.
We call the one side [of humanity] religion, and we call the other science. Religion is always right. ... Science is always wrong; it is the very artifice of men. Science can never solve one problem without raising ten more problems.
Speech at the Einstein Dinner, Savoy Hotel, London (28 Oct 1930). Reproduced in George Bernard Shaw and Warren Sylvester Smith (ed.), The Religious Speeches of George Bernard Shaw (1963), 83.
We called the new [fourth] quark the “charmed quark” because we were pleased, and fascinated by the symmetry it brought to the subnuclear world. “Charm” also means a “a magical device to avert evil,” and in 1970 it was realized that the old three quark theory ran into very serious problems. ... As if by magic the existence of the charmed quark would [solve those problems].
From asppearance in the BBC-TV program written by Nigel Calder, 'The Key to the Universe,' (27 Jan 1977). As cited in Arthur Lewis Caso, 'The Production of New Scientific Terms', American Speech (Summer 1980), 55, No. 2, 102.
We have elves here, and they help me. … While I’m digging in the tunnel, the elves will often come to me with solutions to my problem.
Revealing the secrets of his success, in a legend told by colleague, John Rollwagen, about Cray showing his house to a visiting French scientist, and a tunnel that Cray was building under it. Cray explained that when he reached an impasse in his computer design, he would retire to the tunnel to dig. As quoted in Toby Howard, 'Seymour Cray: An Appreciation', Computer World (Feb 1997).
We have gone a long way towards solving a problem when we are able to formulate it.
In Le Phénomène Humain (1955) as translated by Bernard Wall in 'The Expansion of Life',The Phenomenon of Man (1959, 2008), 115.
We may, I think, draw a yet higher and deeper teaching from the phenomena of degeneration. We seem to learn from it the absolute necessity of labour and effort, of struggle and difficulty, of discomfort and pain, as the condition of all progress, whether physical or mental, and that the lower the organism the more need there is of these ever-present stimuli, not only to effect progress, but to avoid retrogression. And if so, does not this afford us the nearest attainable solution of the great problem of the origin of evil? What we call evil is the essential condition of progress in the lower stages of the development of conscious organisms, and will only cease when the mind has become so thoroughly healthy, so well balanced, and so highly organised, that the happiness derived from mental activity, moral harmony, and the social affections, will itself be a sufficient stimulus to higher progress and to the attainment of a more perfect life.
In 'Two Darwinian Essays', Nature (1880), 22, 142.
We need a number of solutions - we need more efficiency and conservation. Efficiency is a big one. I think car companies need to do a lot better in producing more efficient cars. They have the technology, we just need to demand them as consumers.
…...
What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details.
From 'L’Avenir des Mathématiques', Science et Méthode (1908, 1920), Livre Premier, Chap. 2, 25. English as in Henri Poincaré and George Bruce Halsted (trans.), 'The Future of Mathematics', Science and Method collected in The Foundations of Science: Science and Hypothesis, The Value of Science, Science and Method (1913), 372. From the French, “Qu’est-ce qui nous donne en effet dans une solution, dans une démonstration, le sentiment de l’élégance? C’est l’harmonie des diverses parties, leur symétrie, leur heureux balancement; c’est en un mot tout ce qui y met de l'ordre, tout ce qui leur donne de l’unité, ce qui nous permet par conséquent d’y voir clair et d’en comprendre l’ensemble en même temps que les détails”.
When an inquiry becomes so convoluted, we must suspect that we are proceeding in the wrong way. We must return to go, change gears, and reformulate the problem, not pursue every new iota of information or nuance of argument jn the old style, hoping all the time that our elusive solution simply awaits a crucial item, yet undiscovered.
In The Flamingo’s Smile (1985).
When he [Wilhelm His] set a problem it was concisely stated; he outlined the general plan by which it was to be solved. All of the details were left to the pupil and it annoyed him to be consulted regarding them. He desired that the pupil should have full freedom to work out his own solution and aided him mainly through severe criticism.
As quoted, without citation, in Florence R. Sabin, Franklin Paine Mall: The Story of a Mind. (1934), 39.
When I am working on a problem, I never think about beauty … but when I have finished, if the solution is not beautiful, I know it is wrong.
Quoted in David J. Darling, The Universal Book of Mathematics (2004). 34.
When the solution is simple, God is answering. Where the world ceases to be the scene of our personal hopes and wishes, where we face it as free beings admiring, asking and observing, there we enter the realm of Art and Science.
…...
When we are young, we think that science has to do with facts, with finding answers and solutions, and that it proceeds like an arrow from the primitive to the sophisticated, from mystery to light. But as we get older, we find that, while science does have to do with facts and laws, it has equally to do with wisdom.
In Introduction to Isaac Asimov and Jason A. Shulman (eds.), Isaac Asimov’s Book of Science and Nature Quotations (1988), xix.
Whereas the chemico-chemists always find in industry a beautiful field of gold-laden soil, the physico-chemists stand somewhat farther off, especially those who seek only the greatest dilution, for in general there is little to make with watery solutions.
Attributed.
Whether we like it or not, the ultimate goal of every science is to become trivial, to become a well-controlled apparatus for the solution of schoolbook exercises or for practical application in the construction of engines.
'Nonequilibrium Thermodynamics', International Science and Technology (Oct 1963), 44.
While knowledge can create problems, it is not through ignorance that we can solve them.
In Asimov's New Guide to Science (1984), 15.
Why do they [Americans] quarrel, why do they hate Negroes, Indians, even Germans, why do they not have science and poetry commensurate with themselves, why are there so many frauds and so much nonsense? I cannot soon give a solution to these questions ... It was clear that in the United States there was a development not of the best, but of the middle and worst sides of European civilization; the notorious general voting, the tendency to politics... all the same as in Europe. A new dawn is not to be seen on this side of the ocean.
The Oil Industry in the North American State of Pennsylvania and in the Caucasus (1877). Translated by H. M. Leicester, from the original in Russian, in 'Mendeleev's Visit to America', Journal of Chemical Education (1957), 34, 333.
Why is it so easy to acquire the solutions of past problems and so difficult to solve current ones
(Attributed ??) This quote is often seen, but without a citation, even on the official Marshall McLuhan website. If you known a primary print source, please contact Webmaster.
Will it be possible to solve these problems? It is certain that nobody has thus far observed the transformation of dead into living matter, and for this reason we cannot form a definite plan for the solution of this problem of transformation. But we see that plants and animals during their growth continually transform dead into living matter, and that the chemical processes in living matter do not differ in principle from those in dead matter. There is, therefore, no reason to predict that abiogenesis is impossible, and I believe that it can only help science if the younger investigators realize that experimental abiogenesis is the goal of biology.
The Dynamics of Living Matter (1906), 223.
Without a commitment to science and rationality in its proper domain, there can be no solution to the problems that engulf us. Still, the Yahoos never rest.
Ever Since Darwin (1980),146.
You propound a complicated arithmetical problem: say cubing a number containing four digits. Give me a slate and half an hour’s time, and I can produce a wrong answer.
Cashel Byron's Profession (1886, 1901), xxiii.
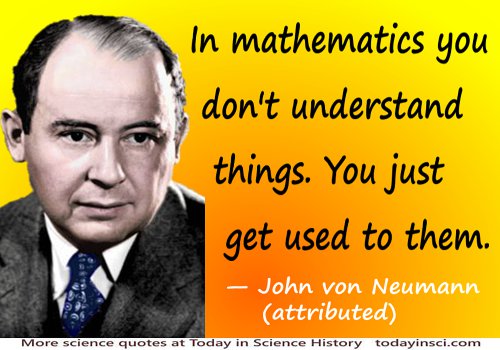


![Luis W. Alvarez quote: Dad [Walter C. Alvarez] … advised me to sit every few months in my reading chair for an entire evening, c](https://todayinsci.com/A/Alvarez_Luis/AlvarezLuis-Problems500x250px.jpg)












 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


