... one of the main functions of an analogy or model is to suggest extensions of the theory by considering extensions of the analogy, since more is known about the analogy than is known about the subject matter of the theory itself … A collection of observable concepts in a purely formal hypothesis suggesting no analogy with anything would consequently not suggest either any directions for its own development.

[Presently, science undergraduates] do not learn to write clearly and briefly, marshalling their points in due and aesthetically satisfying order, and eliminating inessentials. They are inept at those turns of phrase or happy analogy which throw a flying bridge across a chasm of misunderstanding and make contact between mind and mind.

A mathematician’s work is mostly a tangle of guesswork, analogy, wishful thinking and frustration, and proof, far from being the core of discovery, is more often than not a way of making sure that our minds are not playing tricks.

According to the historian D. B. McIntyre (1963), James Hutton, often known as the father of geology, said in a lecture before the Royal Society of Edinburgh in the 1790s that he thought of the Earth as a superorganism and that its proper study would be by physiology. Hutton went on to make the analogy between the circulation of the blood, discovered by Harvey, and the circulation of the nutrient elements of the Earth and of the way that sunlight distills water from the oceans so that it may later fall as rain and so refresh the earth.

An essential [of an inventor] is a logical mind that sees analogies. No! No! not mathematical. No man of a mathematical habit of mind ever invented anything that amounted to much. He hasn’t the imagination to do it. He sticks too close to the rules, and to the things he is mathematically sure he knows, to create anything new.

An immune system of enormous complexity is present in all vertebrate animals. When we place a population of lymphocytes from such an animal in appropriate tissue culture fluid, and when we add an antigen, the lymphocytes will produce specific antibody molecules, in the absence of any nerve cells. I find it astonishing that the immune system embodies a degree of complexity which suggests some more or less superficial though striking analogies with human language, and that this cognitive system has evolved and functions without assistance of the brain.

Analogies, it is true, decide nothing but they can make one feel more at home.

Analogy cannot serve as proof.

Analogy is a wonderful, useful and most important form of thinking, and biology is saturated with it. Nothing is worse than a horrible mass of undigested facts, and facts are indigestible unless there is some rhyme or reason to them. The physicist, with his facts, seeks reason; the biologist seeks something very much like rhyme, and rhyme is a kind of analogy.... This analogizing, this fine sweeping ability to see likenesses in the midst of differences is the great glory of biology, but biologists don't know it.... They have always been so fascinated and overawed by the superior prestige of exact physical science that they feel they have to imitate it.... In its central content, biology is not accurate thinking, but accurate observation and imaginative thinking, with great sweeping generalizations.

Astronomy, as the science of cyclical motions, has nothing in common with Geology. But look at Astronomy where she has an analogy with Geology; consider our knowledge of the heavens as a palaetiological science;—as the study of a past condition, from which the present is derived by causes acting in time. Is there no evidence of a beginning, or of a progress?

Catastrophe Theory is—quite likely—the first coherent attempt (since Aristotelian logic) to give a theory on analogy. When narrow-minded scientists object to Catastrophe Theory that it gives no more than analogies, or metaphors, they do not realise that they are stating the proper aim of Catastrophe Theory, which is to classify all possible types of analogous situations.

Deprived, therefore, as regards this period, of any assistance from history, but relieved at the same time from the embarrassing interference of tradition, the archaeologist is free to follow the methods which have been so successfully pursued in geology—the rude bone and stone implements of bygone ages being to the one what the remains of extinct animals are to the other. The analogy may be pursued even further than this. Many mammalia which are extinct in Europe have representatives still living in other countries. Our fossil pachyderms, for instance, would be almost unintelligible but for the species which still inhabit some parts of Asia and Africa; the secondary marsupials are illustrated by their existing representatives in Australia and South America; and in the same manner, if we wish clearly to understand the antiquities of Europe, we must compare them with the rude implements and weapons still, or until lately, used by the savage races in other parts of the world. In fact, the Van Diemaner and South American are to the antiquary what the opossum and the sloth are to the geologist.

Ecosystems are always in some state of dynamics, sometimes very obviously so, sometimes not. An analogy can be drawn with watching the second and hour hands on a clock. Both hands move, but you can only detect movement in the second hand. Stare at the hour hand all you want: it never seems to move, though it obviously does. Ecosystem change ranges from “watching the second hand” to “watching the hour hand,” depending on what sort of change is occurring.

He who studies it [Nature] has continually the exquisite pleasure of discerning or half discerning and divining laws; regularities glimmer through an appearance of confusion, analogies between phenomena of a different order suggest themselves and set the imagination in motion; the mind is haunted with the sense of a vast unity not yet discoverable or nameable. There is food for contemplation which never runs short; you are gazing at an object which is always growing clearer, and yet always, in the very act of growing clearer, presenting new mysteries.

Here I shall present, without using Analysis [mathematics], the principles and general results of the Théorie, applying them to the most important questions of life, which are indeed, for the most part, only problems in probability. One may even say, strictly speaking, that almost all our knowledge is only probable; and in the small number of things that we are able to know with certainty, in the mathematical sciences themselves, the principal means of arriving at the truth—induction and analogy—are based on probabilities, so that the whole system of human knowledge is tied up with the theory set out in this essay.

However much we may enlarge our ideas of the time which has elapsed since the Niagara first began to drain the waters of the upper lakes, we have seen that this period was one only of a series, all belonging to the present zoological epoch; or that in which the living testaceous fauna, whether freshwater or marine, had already come into being. If such events can take place while the zoology of the earth remains almost stationary and unaltered, what ages may not be comprehended in those successive tertiary periods during which the Flora and Fauna of the globe have been almost entirely changed. Yet how subordinate a place in the long calendar of geological chronology do the successive tertiary periods themselves occupy! How much more enormous a duration must we assign to many antecedent revolutions of the earth and its inhabitants! No analogy can be found in the natural world to the immense scale of these divisions of past time, unless we contemplate the celestial spaces which have been measured by the astronomer.

I often use the analogy of a chess game: one can learn all the rules of chess, but one doesn’t know how to play well…. The present situation in physics is as if we know chess, but we don’t know one or two rules. But in this part of the board where things are in operation, those one or two rules are not operating much and we can get along pretty well without understanding those rules. That’s the way it is, I would say, regarding the phenomena of life, consciousness and so forth.

I took him [Lawrence Bragg] to a young zoologist working on pattern formation in insect cuticles. The zoologist explained how disturbances introduced into these regular patterns pointed to their formation being governed by some kind of gradient. Bragg listened attentively and then exclaimed: “Your disturbed gradient behaves like a stream of sand running downhill and encountering an obstacle.” “Good heavens,” replied the zoologist, “I had been working on this problem for years before this simple analogy occurred to me and you think of it after twenty minutes.”

If men of science owe anything to us, we may learn much from them that is essential. For they can show how to test proof, how to secure fulness and soundness in induction, how to restrain and to employ with safety hypothesis and analogy.

If the scientist has during the whole of his life observed carefully, trained himself to be on the look out for analogy, and possessed himself of relevant knowledge, then the ‘instrument of feeling’ … will become a powerful divining rod … in creative science feeling plays a leading part.

If we consider our earth as a spaceship and the earthly astronauts as the crew of that spaceship, I would say wars can be analogous to mutinies aboard the ship.

In no subject is there a rule, compliance with which will lead to new knowledge or better understanding. Skilful observations, ingenious ideas, cunning tricks, daring suggestions, laborious calculations, all these may be required to advance a subject. Occasionally the conventional approach in a subject has to be studiously followed; on other occasions it has to be ruthlessly disregarded. Which of these methods, or in what order they should be employed is generally unpredictable. Analogies drawn from the history of science are frequently claimed to be a guide; but, as with forecasting the next game of roulette, the existence of the best analogy to the present is no guide whatever to the future. The most valuable lesson to be learnt from the history of scientific progress is how misleading and strangling such analogies have been, and how success has come to those who ignored them.

Induction and analogy are the special characteristics of modern mathematics, in which theorems have given place to theories and no truth is regarded otherwise than as a link in an infinite chain. “Omne exit in infinitum” is their favorite motto and accepted axiom.

It has been asserted … that the power of observation is not developed by mathematical studies; while the truth is, that; from the most elementary mathematical notion that arises in the mind of a child to the farthest verge to which mathematical investigation has been pushed and applied, this power is in constant exercise. By observation, as here used, can only be meant the fixing of the attention upon objects (physical or mental) so as to note distinctive peculiarities—to recognize resemblances, differences, and other relations. Now the first mental act of the child recognizing the distinction between one and more than one, between one and two, two and three, etc., is exactly this. So, again, the first geometrical notions are as pure an exercise of this power as can be given. To know a straight line, to distinguish it from a curve; to recognize a triangle and distinguish the several forms—what are these, and all perception of form, but a series of observations? Nor is it alone in securing these fundamental conceptions of number and form that observation plays so important a part. The very genius of the common geometry as a method of reasoning—a system of investigation—is, that it is but a series of observations. The figure being before the eye in actual representation, or before the mind in conception, is so closely scrutinized, that all its distinctive features are perceived; auxiliary lines are drawn (the imagination leading in this), and a new series of inspections is made; and thus, by means of direct, simple observations, the investigation proceeds. So characteristic of common geometry is this method of investigation, that Comte, perhaps the ablest of all writers upon the philosophy of mathematics, is disposed to class geometry, as to its method, with the natural sciences, being based upon observation. Moreover, when we consider applied mathematics, we need only to notice that the exercise of this faculty is so essential, that the basis of all such reasoning, the very material with which we build, have received the name observations. Thus we might proceed to consider the whole range of the human faculties, and find for the most of them ample scope for exercise in mathematical studies. Certainly, the memory will not be found to be neglected. The very first steps in number—counting, the multiplication table, etc., make heavy demands on this power; while the higher branches require the memorizing of formulas which are simply appalling to the uninitiated. So the imagination, the creative faculty of the mind, has constant exercise in all original mathematical investigations, from the solution of the simplest problems to the discovery of the most recondite principle; for it is not by sure, consecutive steps, as many suppose, that we advance from the known to the unknown. The imagination, not the logical faculty, leads in this advance. In fact, practical observation is often in advance of logical exposition. Thus, in the discovery of truth, the imagination habitually presents hypotheses, and observation supplies facts, which it may require ages for the tardy reason to connect logically with the known. Of this truth, mathematics, as well as all other sciences, affords abundant illustrations. So remarkably true is this, that today it is seriously questioned by the majority of thinkers, whether the sublimest branch of mathematics,—the infinitesimal calculus—has anything more than an empirical foundation, mathematicians themselves not being agreed as to its logical basis. That the imagination, and not the logical faculty, leads in all original investigation, no one who has ever succeeded in producing an original demonstration of one of the simpler propositions of geometry, can have any doubt. Nor are induction, analogy, the scrutinization of premises or the search for them, or the balancing of probabilities, spheres of mental operations foreign to mathematics. No one, indeed, can claim preeminence for mathematical studies in all these departments of intellectual culture, but it may, perhaps, be claimed that scarcely any department of science affords discipline to so great a number of faculties, and that none presents so complete a gradation in the exercise of these faculties, from the first principles of the science to the farthest extent of its applications, as mathematics.

It is a natural inquiry to ask—To what most nearly are these new phenomena [the newly-born science of radioactivity and the spontaneous disintegration of elements] correlated? Is it possible to give, by the help of an analogy to familiar phenomena, any correct idea of the nature of this new phenomenon “Radioactivity”? The answer may surprise those who hold to the adage that there is nothing new under the sun. Frankly, it is not possible, because in these latest developments science has broken fundamentally new ground, and has delved one distinct step further down into the foundations of knowledge.

It is my thesis that the physical functioning of the living individual and the operation of some of the newer communication machines are precisely parallel in their analogous attempts to control entropy through feedback. Both of them have sensory receptors as one stage in their cycle of operation: that is, in both of them there exists a special apparatus for collecting information from the outer world at low energy levels, and for making it available in the operation of the individual or of the machine. In both cases these external messages are not taken neat, but through the internal transforming powers of the apparatus, whether it be alive or dead. The information is then turned into a new form available for the further stages of performance. In both the animal and the machine this performance is made to be effective on the outer world. In both of them, their performed action on the outer world, and not merely their intended aetion, is reported back to the central regulatory apparatus.

It is often in our Power to obtain an Analogy where we cannot have an Induction.

It is to geometry that we owe in some sort the source of this discovery [of beryllium]; it is that [science] that furnished the first idea of it, and we may say that without it the knowledge of this new earth would not have been acquired for a long time, since according to the analysis of the emerald by M. Klaproth and that of the beryl by M. Bindheim one would not have thought it possible to recommence this work without the strong analogies or even almost perfect identity that Citizen Haüy found for the geometrical properties between these two stony fossils.

It must be gently but firmly pointed out that analogy is the very corner-stone of scientific method. A root-and-branch condemnation would invalidate any attempt to explain the unknown in terms of the known, and thus prune away every hypothesis.

Its [mathematical analysis] chief attribute is clearness; it has no means for expressing confused ideas. It compares the most diverse phenomena and discovers the secret analogies which unite them. If matter escapes us, as that of air and light because of its extreme tenuity, if bodies are placed far from us in the immensity of space, if man wishes to know the aspect of the heavens at successive periods separated by many centuries, if gravity and heat act in the interior of the solid earth at depths which will forever be inaccessible, mathematical analysis is still able to trace the laws of these phenomena. It renders them present and measurable, and appears to be the faculty of the human mind destined to supplement the brevity of life and the imperfection of the senses, and what is even more remarkable, it follows the same course in the study of all phenomena; it explains them in the same language, as if in witness to the unity and simplicity of the plan of the universe, and to make more manifest the unchangeable order which presides over all natural causes.

Let us suppose that an ichthyologist is exploring the life of the ocean. He casts a net into the water and brings up a fishy assortment. Surveying his catch, he proceeds in the usual manner of a scientist to systematise what it reveals. He arrives at two generalisations:
(1) No sea-creature is less than two inches long.
(2) All sea-creatures have gills.
These are both true of his catch, and he assumes tentatively that they will remain true however often he repeats it.
In applying this analogy, the catch stands for the body of knowledge which constitutes physical science, and the net for the sensory and intellectual equipment which we use in obtaining it. The casting of the net corresponds to observation; for knowledge which has not been or could not be obtained by observation is not admitted into physical science.
An onlooker may object that the first generalisation is wrong. “There are plenty of sea-creatures under two inches long, only your net is not adapted to catch them.” The icthyologist dismisses this objection contemptuously. “Anything uncatchable by my net is ipso facto outside the scope of icthyological knowledge. In short, what my net can't catch isn't fish.” Or—to translate the analogy—“If you are not simply guessing, you are claiming a knowledge of the physical universe discovered in some other way than by the methods of physical science, and admittedly unverifiable by such methods. You are a metaphysician. Bah!”

Mathematical science is in my opinion an indivisible whole, an organism whose vitality is conditioned upon the connection of its parts. For with all the variety of mathematical knowledge, we are still clearly conscious of the similarity of the logical devices, the relationship of the ideas in mathematics as a whole and the numerous analogies in its different departments.

Mathematicians attach great importance to the elegance of their methods and their results. This is not pure dilettantism. What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details. But this is exactly what yields great results, in fact the more we see this aggregate clearly and at a single glance, the better we perceive its analogies with other neighboring objects, consequently the more chances we have of divining the possible generalizations. Elegance may produce the feeling of the unforeseen by the unexpected meeting of objects we are not accustomed to bring together; there again it is fruitful, since it thus unveils for us kinships before unrecognized. It is fruitful even when it results only from the contrast between the simplicity of the means and the complexity of the problem set; it makes us then think of the reason for this contrast and very often makes us see that chance is not the reason; that it is to be found in some unexpected law. In a word, the feeling of mathematical elegance is only the satisfaction due to any adaptation of the solution to the needs of our mind, and it is because of this very adaptation that this solution can be for us an instrument. Consequently this esthetic satisfaction is bound up with the economy of thought.

Mathematics is the study of analogies between analogies. All science is. Scientists want to show that things that don’t look alike are really the same. That is one of their innermost Freudian motivations. In fact, that is what we mean by understanding.

Microbiology is usually regarded as having no relevance to the feelings and aspirations of the man of flesh and bone. Yet, never in my professional life do I find myself far removed from the man of flesh and bone. It is not only because microbes are ubiquitous in our environment, and therefore must be studied for the sake of human welfare. More interesting, and far more important in the long run, is the fact that microbes exhibit profound resemblances to man. They resemble him in their physical makeup, in their properties, in their responses to various stimuli; they also display associations with other living things which have perplexing and illuminating analogies with human societies.

Money, mechanization, algebra. The three monsters of contemporary civilization. Complete analogy.

More about the selection theory: Jerne meant that the Socratic idea of learning was a fitting analogy for 'the logical basis of the selective theories of antibody formation': Can the truth (the capability to synthesize an antibody) be learned? If so, it must be assumed not to pre-exist; to be learned, it must be acquired. We are thus confronted with the difficulty to which Socrates calls attention in Meno [ ... ] namely, that it makes as little sense to search for what one does not know as to search for what one knows; what one knows, one cannot search for, since one knows it already, and what one does not know, one cannot search for, since one does not even know what to search for. Socrates resolves this difficulty by postulating that learning is nothing but recollection. The truth (the capability to synthesize an antibody) cannot be brought in, but was already inherent.

Natural science is founded on minute critical views of the general order of events taking place upon our globe, corrected, enlarged, or exalted by experiments, in which the agents concerned are placed under new circumstances, and their diversified properties separately examined. The body of natural science, then, consists of facts; is analogy,—the relation of resemblance of facts by which its different parts are connected, arranged, and employed, either for popular use, or for new speculative improvements.

Newton supposed that the case of the planet was similar to that of [a ball spun around on the end of an elastic string]; that it was always pulled in the direction of the sun, and that this attraction or pulling of the sun produced the revolution of the planet, in the same way that the traction or pulling of the elastic string produces the revolution of the ball. What there is between the sun and the planet that makes each of them pull the other, Newton did not know; nobody knows to this day; and all we are now able to assert positively is that the known motion of the planet is precisely what would be produced if it were fastened to the sun by an elastic string, having a certain law of elasticity. Now observe the nature of this discovery, the greatest in its consequences that has ever yet been made in physical science:—
I. It begins with an hypothesis, by supposing that there is an analogy between the motion of a planet and the motion of a ball at the end of a string.
II. Science becomes independent of the hypothesis, for we merely use it to investigate the properties of the motion, and do not trouble ourselves further about the cause of it.

Now, it may be stretching an analogy to compare epidemics of cholera—caused by a known agent—with that epidemic of violent crime which is destroying our cities. It is unlikely that our social problems can be traced to a single, clearly defined cause in the sense that a bacterial disease is ‘caused’ by a microbe. But, I daresay, social science is about as advanced in the late twentieth century as bacteriological science was in the mid nineteenth century. Our forerunners knew something about cholera; they sensed that its spread was associated with misdirected sewage, filth, and the influx of alien poor into crowded, urban tenements. And we know something about street crime; nowhere has it been reported that a member of the New York Stock Exchange has robbed ... at the point of a gun. Indeed, I am naively confident that an enlightened social scientist of the next century will be able to point out that we had available to us at least some of the clues to the cause of urban crime.

Of our three principal instruments for interrogating Nature,—observation, experiment, and comparison,—the second plays in biology a quite subordinate part. But while, on the one hand, the extreme complication of causes involved in vital processes renders the application of experiment altogether precarious in its results, on the other hand, the endless variety of organic phenomena offers peculiar facilities for the successful employment of comparison and analogy.

Probably there was a beginning—it is a metaphysical question, worthy a theologian—species have begun and ended—but the analogy is faint and distant.

Psychology, as the behaviorist views it, is a purely objective, experimental branch of natural science which needs introspection as little as do the sciences of chemistry and physics. It is granted that the behavior of animals can be investigated without appeal to consciousness. Heretofore the viewpoint has been that such data have value only in so far as they can be interpreted by analogy in terms of consciousness. The position is taken here that the behavior of man and the behavior of animals must be considered in the same plane.

Saturated with that speculative spirit then pervading the Greek mind, he [Pythagoras] endeavoured to discover some principle of homogeneity in the universe. Before him, the philosophers of the Ionic school had sought it in the matter of things; Pythagoras looked for it in the structure of things. He observed the various numerical relations or analogies between numbers and the phenomena of the universe. Being convinced that it was in numbers and their relations that he was to find the foundation to true philosophy, he proceeded to trace the origin of all things to numbers. Thus he observed that musical strings of equal lengths stretched by weights having the proportion of 1/2, 2/3, 3/4, produced intervals which were an octave, a fifth and a fourth. Harmony, therefore, depends on musical proportion; it is nothing but a mysterious numerical relation. Where harmony is, there are numbers. Hence the order and beauty of the universe have their origin in numbers. There are seven intervals in the musical scale, and also seven planets crossing the heavens. The same numerical relations which underlie the former must underlie the latter. But where number is, there is harmony. Hence his spiritual ear discerned in the planetary motions a wonderful “Harmony of spheres.”

Science is simply the classification of the common knowledge of the common people. It is bringing together the things we all know and putting them together so we can use them. This is creation and finds its analogy in Nature, where the elements are combined in certain ways to give us fruits or flowers or grain.

Science’s defenders have identified five hallmark moves of pseudoscientists. They argue that the scientific consensus emerges from a conspiracy to suppress dissenting views. They produce fake experts, who have views contrary to established knowledge but do not actually have a credible scientific track record. They cherry-pick the data and papers that challenge the dominant view as a means of discrediting an entire field. They deploy false analogies and other logical fallacies. And they set impossible expectations of research: when scientists produce one level of certainty, the pseudoscientists insist they achieve another.

Since biologists deal constantly in analogies, they are easily misled by them.

Such is the tendency of the human mind to speculation, that on the least idea of an analogy between a few phenomena, it leaps forward, as it were, to a cause or law, to the temporary neglect of all the rest; so that, in fact, almost all our principal inductions must be regarded as a series of ascents and descents, and of conclusions from a few cases, verified by trial on many.

The analogies between science and art are very good as long as you are talking about the creation and the performance. The creation is certainly very analogous. The aesthetic pleasure of the craftsmanship of performance is also very strong in science.

The elementary parts of all tissues are formed of cells in an analogous, though very diversified manner, so that it may be asserted, that there is one universal principle of development for the elementary parts of organisms, however different, and that this principle is the formation of cells.

The fact that astronomies change while the stars abide is a true analogy of every realm of human life and thought, religion not least of all.

The generation of seeds ... is therefore marvelous and analogous to the other productions of living things. For first of all an umbilicus appears. ... Its extremity gradually expands and after gathering a colliquamentous ichor becomes analogous to an amnion. ... In the course of time the seed or fetus begins to become visible.

The habitat of an organism is the place where it lives, or the place where one would go to find it. The ecological niche, on the other hand, is the position or status of an organism within its community and ecosystem resulting from the organism’s structural adaptations, physiological responses and specific behavior (inherited and/or learned). The ecological niche of an organism depends not only on where it lives, but also on what it does. By analogy, it may be said that the habitat is the organism’s ‘address,’ and the niche is its ‘profession,’ biologically speaking.

The human mind delights in finding pattern–so much so that we often mistake coincidence or forced analogy for profound meaning. No other habit of thought lies so deeply within the soul of a small creature trying to make sense of a complex world not constructed for it.

The invention of what we may call primary or fundamental notation has been but little indebted to analogy, evidently owing to the small extent of ideas in which comparison can be made useful. But at the same time analogy should be attended to, even if for no other reason than that, by making the invention of notation an art, the exertion of individual caprice ceases to be allowable. Nothing is more easy than the invention of notation, and nothing of worse example and consequence than the confusion of mathematical expressions by unknown symbols. If new notation be advisable, permanently or temporarily, it should carry with it some mark of distinction from that which is already in use, unless it be a demonstrable extension of the latter.

The mathematical facts worthy of being studied are those which, by their analogy with other facts, are capable of leading us to the knowledge of a physical law. They reveal the kinship between other facts, long known, but wrongly believed to be strangers to one another.

The methods of science may be described as the discovery of laws, the explanation of laws by theories, and the testing of theories by new observations. A good analogy is that of the jigsaw puzzle, for which the laws are the individual pieces, the theories local patterns suggested by a few pieces, and the tests the completion of these patterns with pieces previously unconsidered. … The scientist likes to fancy … that sufficient pieces may be assembled to indicate eventually the entire pattern of the puzzle, and thus to reveal the structure and behavior of the physical universe as it appears to man.

The principal result of my investigation is that a uniform developmental principle controls the individual elementary units of all organisms, analogous to the finding that crystals are formed by the same laws in spite of the diversity of their forms.

The question of the origin of the hypothesis belongs to a domain in which no very general rules can be given; experiment, analogy and constructive intuition play their part here. But once the correct hypothesis is formulated, the principle of mathematical induction is often sufficient to provide the proof.

The real achievement in discoveries … is seeing an analogy where no one saw one before. … The essence of discovery is that unlikely marriage of … previously unrelated forms of reference or universes of discourse, whose union will solve the previously insoluble problem.

The results have exhibited one striking feature which has been frequently emphasized, namely that at high pressures all twelve liquids become more nearly like each other. This suggests that it might be useful in developing a theory of liquids to arbitrarily construct a 'perfect liquid' and to discuss its properties. Certainly the conception of a 'perfect gas' has been of great service in the kinetic theory of gases; and the reason is that all actual gases approximate closely to the 'perfect gas.' In the same way, at high pressures all liquids approximate to one and the same thing, which may be called by analogy the 'perfect liquid.' It seems to offer at least a promising line of attack to discuss the properties of this 'perfect liquid,' and then to invent the simplest possible mechanism to explain them.

The scientific discovery appears first as the hypothesis of an analogy; and science tends to become independent of the hypothesis.

The tendency of the sciences has long been an increasing proclivity of separation and dismemberment … The mathematician turns away from the chemist; the chemist from the naturalist; the mathematician, left to himself divides himself into a pure mathematician and a mixed mathematician, who soon part company … And thus science, even mere physical science, loses all traces of unity. A curious illustration of this result may be observed in the want of any name by which we can designate the students of the knowledge of the material world collectively. We are informed that this difficulty was felt very oppressively by the members of the British Association for the Advancement of Science, at their meetings at York, Oxford and Cambridge, in the last three summers. There was no general term by which these gentlemen could describe themselves with reference to their pursuits … some ingenious gentleman [William Whewell] proposed that, by analogy with artist, they might form Scientist, and added that there could be no scruple … when we have words such as sciolist, economist, and atheist—but this was not generally palatable.

There are 60 sub-atomic particles they’ve discovered that can explain the thousands of other sub-atomic particles, and the model is too ugly. This is my analogy: it’s like taking Scotch tape and taping a giraffe to a mule to a whale to a tiger and saying this is the ultimate theory of particles. … We have so many particles that Oppenheimer once said you could give a Nobel Prize to the physicist that did not discover a particle that year. We were drowning in sub-atomic particles.
Now we realize that this whole zoo of sub-atomic particles, thousands of them coming out of our accelerators, can be explained by little vibrating strings.

To the extent that remaining old-growth Douglas fir ecosystems possess unique structural and functional characteristics distinct from surrounding managed forests, the analogy between forest habitat islands and oceanic islands applies. Forest planning decision variables such as total acreage to be maintained, patch size frequency distribution, spatial distribution of patches, specific locations, and protective measures all need to be addressed.

We have seen that a proton of energy corresponding to 30,000 volts can effect the transformation of lithium into two fast α-particles, which together have an energy equivalent of more than 16 million volts. Considering the individual process, the output of energy in the transmutation is more than 500 times greater than the energy carried by the proton. There is thus a great gain of energy in the single transmutation, but we must not forget that on an average more than 1000 million protons of equal energy must be fired into the lithium before one happens to hit and enter the lithium nucleus. It is clear in this case that on the whole the energy derived from transmutation of the atom is small compared with the energy of the bombarding particles. There thus seems to be little prospect that we can hope to obtain a new source of power by these processes. It has sometimes been suggested, from analogy with ordinary explosives, that the transmutation of one atom might cause the transmutation of a neighbouring nucleus, so that the explosion would spread throughout all the material. If this were true, we should long ago have had a gigantic explosion in our laboratories with no one remaining to tell the tale. The absence of these accidents indicates, as we should expect, that the explosion is confined to the individual nucleus and does not spread to the neighbouring nuclei, which may be regarded as relatively far removed from the centre of the explosion.

We may therefore say in the future, strictly within the limits of observation, that in certain respects the fossil species of a class traverse in their historical succession metamorphoses similar to those which the embryos undergo in themselves. … The development of a class in the history of the earth offers, in many respects, the greatest analogy with the development of an individual at different periods of his life. The demonstration of this truth is one of the most beautiful results of modern paleontology.

What has been learned in physics stays learned. People talk about scientific revolutions. The social and political connotations of revolution evoke a picture of a body of doctrine being rejected, to be replaced by another equally vulnerable to refutation. It is not like that at all. The history of physics has seen profound changes indeed in the way that physicists have thought about fundamental questions. But each change was a widening of vision, an accession of insight and understanding. The introduction, one might say the recognition, by man (led by Einstein) of relativity in the first decade of this century and the formulation of quantum mechanics in the third decade are such landmarks. The only intellectual casualty attending the discovery of quantum mechanics was the unmourned demise of the patchwork quantum theory with which certain experimental facts had been stubbornly refusing to agree. As a scientist, or as any thinking person with curiosity about the basic workings of nature, the reaction to quantum mechanics would have to be: “Ah! So that’s the way it really is!” There is no good analogy to the advent of quantum mechanics, but if a political-social analogy is to be made, it is not a revolution but the discovery of the New World.

What I chiefly admired, and thought altogether unaccountable, was the strong disposition I observed in them [the mathematicians of Laputa] towards news and politics; perpetually inquiring into public affairs; giving their judgments in matters of state; and passionately disputing every inch of party opinion. I have indeed observed the same disposition among most of the mathematicians I have known in Europe, although I could never discover the least analogy between the two sciences.

When men are ignorant of the natural causes producing things, and cannot even explain them by analogy with similar things, they attribute their own nature to them. The vulgar, for example, say the magnet loves the iron.

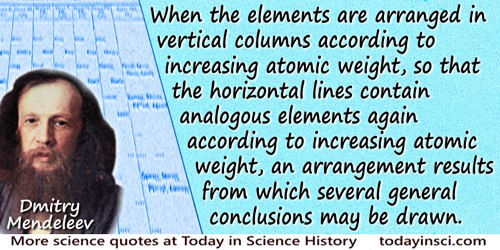
When the elements are arranged in vertical columns according to increasing atomic weight, so that the horizontal lines contain analogous elements again according to increasing atomic weight, an arrangement results from which several general conclusions may be drawn.

Where we reach the sphere of mathematics we are among processes which seem to some the most inhuman of all human activities and the most remote from poetry. Yet it is just here that the artist has the fullest scope for his imagination. … We are in the imaginative sphere of art, and the mathematician is engaged in a work of creation which resembles music in its orderliness, … It is not surprising that the greatest mathematicians have again and again appealed to the arts in order to find some analogy to their own work. They have indeed found it in the most varied arts, in poetry, in painting, and in sculpture, although it would certainly seem that it is in music, the most abstract of all the arts, the art of number and time, that we find the closest analogy.

Whoever wishes to acquire a deep acquaintance with Nature must observe that there are analogies which connect whole branches of science in a parallel manner, and enable us to infer of one class of phenomena what we know of another. It has thus happened on several occasions that the discovery of an unsuspected analogy between two branches of knowledge has been the starting point for a rapid course of discovery.

Why, it is asked, since the scientist, by means of classification and experiment, can predict the “action of the physical world, shall not the historian do as much for the moral world”! The analogy is false at many points; but the confusion arises chiefly from the assumption that the scientist can predict the action of the physical world. Certain conditions precisely given, the scientist can predict the result; he cannot say when or where in the future those conditions will obtain.

Without this language [mathematics] most of the intimate analogies of things would have remained forever unknown to us; and we should forever have been ignorant of the internal harmony of the world, which is the only true objective reality. …
This harmony … is the sole objective reality, the only truth we can attain; and when I add that the universal harmony of the world is the source of all beauty, it will be understood what price we should attach to the slow and difficult progress which little by little enables us to know it better.

Your admission of the late appearance of the great intellectual crown of the whole animal race [man] strikes me as perfectly fatal to the analogy of your system of a continually recurring series of identical terms.