Difficult Quotes (263 quotes)
…it ought to be remembered that there is nothing more difficult to take in hand, more perilous to conduct, or more uncertain in its success, than to take the lead in the introduction of a new order of things. Because the innovator has for enemies all those who have done well under the old conditions, and lukewarm defenders in those who may do well under the new.
The Prince (1532). W. K. Marriott (translator) and Rob McMahon (editor), The Prince (2008), 71.
“Yes,” he said. “But these things (the solutions to problems in solid geometry such as the duplication of the cube) do not seem to have been discovered yet.” “There are two reasons for this,” I said. “Because no city holds these things in honour, they are investigated in a feeble way, since they are difficult; and the investigators need an overseer, since they will not find the solutions without one. First, it is hard to get such an overseer, and second, even if one did, as things are now those who investigate these things would not obey him, because of their arrogance. If however a whole city, which did hold these things in honour, were to oversee them communally, the investigators would be obedient, and when these problems were investigated continually and with eagerness, their solutions would become apparent.”
— Plato
In The Republic 7 528bc, trans. R.W. Sharples.
[I find it as difficult] to understand a scientist who does not acknowledge the presence of a superior rationality behind the existence of the universe as it is to comprehend a theologian who would deny the advances of science.
Speech, Huntsville Ministerial Association, in Wernher Von Braun and Irene E. Powell-Willhite (ed.), The Voice of Dr. Wernher Von Braun: An Anthology (2007), 89.
[The surplus of basic knowledge of the atomic nucleus was] largely used up [during the war with the atomic bomb as the dividend.] We must, without further delay restore this surplus in preparation for the important peacetime job for the nucleus - power production. ... Many of the proposed applications of atomic power - even for interplanetary rockets - seem to be within the realm of possibility provided the economic factor is ruled out completely, and the doubtful physical and chemical factors are weighted heavily on the optimistic side. ... The development of economic atomic power is not a simple extrapolation of knowledge gained during the bomb work. It is a new and difficult project to reach a satisfactory answer. Needless to say, it is vital that the atomic policy legislation now being considered by the congress recognizes the essential nature of this peacetime job, and that it not only permits but encourages the cooperative research-engineering effort of industrial, government and university laboratories for the task. ... We must learn how to generate the still higher energy particles of the cosmic rays - up to 1,000,000,000 volts, for they will unlock new domains in the nucleus.
Addressing the American Institute of Electrical Engineering, in New York (24 Jan 1946). In Schenectady Gazette (25 Jan 1946),
[The toughest part of being in charge is] killing ideas that are great but poorly timed. And delivering tough feedback that’s difficult to hear but that I know will help people—and the team—in the long term.
In Issie Lapowsky, 'Scott Belsky', Inc. (Nov 2013), 140. Biography in Context,
[Describing the effects of over-indulgence in wine:]
But most too passive, when the blood runs low
Too weakly indolent to strive with pain,
And bravely by resisting conquer fate,
Try Circe's arts; and in the tempting bowl
Of poisoned nectar sweet oblivion swill.
Struck by the powerful charm, the gloom dissolves
In empty air; Elysium opens round,
A pleasing frenzy buoys the lightened soul,
And sanguine hopes dispel your fleeting care;
And what was difficult, and what was dire,
Yields to your prowess and superior stars:
The happiest you of all that e'er were mad,
Or are, or shall be, could this folly last.
But soon your heaven is gone: a heavier gloom
Shuts o'er your head; and, as the thundering stream,
Swollen o'er its banks with sudden mountain rain,
Sinks from its tumult to a silent brook,
So, when the frantic raptures in your breast
Subside, you languish into mortal man;
You sleep, and waking find yourself undone,
For, prodigal of life, in one rash night
You lavished more than might support three days.
A heavy morning comes; your cares return
With tenfold rage. An anxious stomach well
May be endured; so may the throbbing head;
But such a dim delirium, such a dream,
Involves you; such a dastardly despair
Unmans your soul, as maddening Pentheus felt,
When, baited round Citheron's cruel sides,
He saw two suns, and double Thebes ascend.
But most too passive, when the blood runs low
Too weakly indolent to strive with pain,
And bravely by resisting conquer fate,
Try Circe's arts; and in the tempting bowl
Of poisoned nectar sweet oblivion swill.
Struck by the powerful charm, the gloom dissolves
In empty air; Elysium opens round,
A pleasing frenzy buoys the lightened soul,
And sanguine hopes dispel your fleeting care;
And what was difficult, and what was dire,
Yields to your prowess and superior stars:
The happiest you of all that e'er were mad,
Or are, or shall be, could this folly last.
But soon your heaven is gone: a heavier gloom
Shuts o'er your head; and, as the thundering stream,
Swollen o'er its banks with sudden mountain rain,
Sinks from its tumult to a silent brook,
So, when the frantic raptures in your breast
Subside, you languish into mortal man;
You sleep, and waking find yourself undone,
For, prodigal of life, in one rash night
You lavished more than might support three days.
A heavy morning comes; your cares return
With tenfold rage. An anxious stomach well
May be endured; so may the throbbing head;
But such a dim delirium, such a dream,
Involves you; such a dastardly despair
Unmans your soul, as maddening Pentheus felt,
When, baited round Citheron's cruel sides,
He saw two suns, and double Thebes ascend.
The Art of Preserving Health: a Poem in Four Books (2nd. ed., 1745), Book IV, 108-110.
[When asked “Dr. Einstein, why is it that when the mind of man has stretched so far as to discover the structure of the atom we have been unable to devise the political means to keep the atom from destroying us?”] That is simple, my friend. It is because politics is more difficult than physics.
Einstein’s answer to a conferee at a meeting at Princeton, N.J. (Jan 1946), as recalled by Greenville Clark in 'Letters to the Times', in New York Times (22 Apr 1955), 24.
Ac astronomye is an hard thyng,
And yvel for to knowe;
Geometrie and geomesie,
So gynful of speche,
Who so thynketh werche with tho two
Thryveth ful late,
For sorcerie is the sovereyn book
That to tho sciences bilongeth.
Now, astronomy is a difficult discipline, and the devil to learn;
And geometry and geomancy have confusing terminology:
If you wish to work in these two, you will not succeed quickly.
For sorcery is the chief study that these sciences entail.
And yvel for to knowe;
Geometrie and geomesie,
So gynful of speche,
Who so thynketh werche with tho two
Thryveth ful late,
For sorcerie is the sovereyn book
That to tho sciences bilongeth.
Now, astronomy is a difficult discipline, and the devil to learn;
And geometry and geomancy have confusing terminology:
If you wish to work in these two, you will not succeed quickly.
For sorcery is the chief study that these sciences entail.
In William Langland and B. Thomas Wright (ed.) The Vision and Creed of Piers Ploughman (1842), 186. Modern translation by Terrence Tiller in Piers Plowman (1981, 1999), 94.
La vérité ne diffère de l'erreur qu'en deux points: elle est un peu plus difficile à prouver et beaucoup plus difficile à faire admettre. (Dec 1880)
Truth is different from error in two respects: it is a little harder to prove and more difficult to admit.
Truth is different from error in two respects: it is a little harder to prove and more difficult to admit.
In Recueil d'Œuvres de Léo Errera: Botanique Générale (1908), 193. Google translation by Webmaster.
Neumann, to a physicist seeking help with a difficult problem: Simple. This can be solved by using the method of characteristics.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Attributed, as related by Dr. Felix Smith (Head of Molecular Physics, Stanford Research Institute) to author Gary Zukav, who quoted it in The Dancing Wu Li Masters: An Overview of the New Physics (1979, 2001), 208, footnote. The physicist (a friend of Dr. Smith) worked at Los Alamos after WW II. It should be noted that although the author uses quotation marks around the spoken remarks, that they represent the author's memory of Dr. Smith's recollection, who heard it from the physicist. Therefore the fourth-hand wording is very likely not verbatim. Webmaster finds Zukav's book seems to be the only source for this quote.
Qu'une goutee de vin tombe dans un verre d'eau; quelle que soit la loi du movement interne du liquide, nous verrons bientôt se colorer d'une teinte rose uniforme et à partir de ce moment on aura beau agiter le vase, le vin et l'eau ne partaîtront plus pouvoir se séparer. Tout cela, Maxwell et Boltzmann l'ont expliqué, mais celui qui l'a vu plus nettement, dans un livre trop peu lu parce qu'il est difficile à lire, c'est Gibbs dans ses principes de la Mécanique Statistique.
Let a drop of wine fall into a glass of water; whatever be the law that governs the internal movement of the liquid, we will soon see it tint itself uniformly pink and from th at moment on, however we may agitate the vessel, it appears that the wine and water can separate no more. All this, Maxwell and Boltzmann have explained, but the one who saw it in the cleanest way, in a book that is too little read because it is difficult to read, is Gibbs, in his Principles of Statistical Mechanics.
Let a drop of wine fall into a glass of water; whatever be the law that governs the internal movement of the liquid, we will soon see it tint itself uniformly pink and from th at moment on, however we may agitate the vessel, it appears that the wine and water can separate no more. All this, Maxwell and Boltzmann have explained, but the one who saw it in the cleanest way, in a book that is too little read because it is difficult to read, is Gibbs, in his Principles of Statistical Mechanics.
La valeur de la science. In Anton Bovier, Statistical Mechanics of Disordered Systems (2006), 3.
The Charms of Statistics.—It is difficult to understand why statisticians commonly limit their inquiries to Averages, and do not revel in more comprehensive views. Their souls seem as dull to the charm of variety as that of the native of one of our flat English counties, whose retrospect of Switzerland was that, if its mountains could be thrown into its lakes, two nuisances would be got rid of at once. An Average is but a solitary fact, whereas if a single other fact be added to it, an entire Normal Scheme, which nearly corresponds to the observed one, starts potentially into existence. Some people hate the very name of statistics, but I find them full of beauty and interest. Whenever they are not brutalised, but delicately handled by the higher methods, and are warily interpreted, their power of dealing with complicated phenomena is extraordinary. They are the only tools by which an opening can be cut through the formidable thicket of difficulties that bars the path of those who pursue the Science of man.
Natural Inheritance (1889), 62-3.
~~[Misattributed?]~~ Prediction is difficult, especially the future.
Seen variously attributed, including to Yogi Berra or Mark Twain. The association with Bohr is unverified, and is, for example, given as a quote without citation, in Alan G. Mencher, 'On the Social Deployment of Science', Bulletin of the Atomic Scientists (1971), 27, No. 10, 37. Bohr may perhaps have uttered these words, but probably did not originate them. Bohr was Danish, and the quote is known as a Danish saying. It appears in Danish as “Det er vanskeligt at spaa, især naar det gælder Fremtiden,” in Karl Kristian Steincke, 'Og saa til Slut et Par parlamentariske Sprogblomster' (Parliamentary Howlers), Farvel Og Tak (1948), Vol. 4, 227. Background in depth is on the quoteinvestigator.com website.
A cat finds it easy to be a cat, as nearly as we can tell. It isn’t afraid to be a cat. But being a full human being is difficult, frightening, and problematical. While human beings love knowledge and seek it—they are curious—they also fear it. The closer to the personal it is, the more they fear
In The Psychology of Science: A Reconnaissance (1966), 16.
A fossil hunter needs sharp eyes and a keen search image, a mental template that subconsciously evaluates everything he sees in his search for telltale clues. A kind of mental radar works even if he isn’t concentrating hard. A fossil mollusk expert has a mollusk search image. A fossil antelope expert has an antelope search image. … Yet even when one has a good internal radar, the search is incredibly more difficult than it sounds. Not only are fossils often the same color as the rocks among which they are found, so they blend in with the background; they are also usually broken into odd-shaped fragments. … In our business, we don’t expect to find a whole skull lying on the surface staring up at us. The typical find is a small piece of petrified bone. The fossil hunter’s search therefore has to have an infinite number of dimensions, matching every conceivable angle of every shape of fragment of every bone on the human body.
Describing the skill of his co-worker, Kamoya Kimeu, who discovered the Turkana Boy, the most complete specimen of Homo erectus, on a slope covered with black lava pebbles.
Describing the skill of his co-worker, Kamoya Kimeu, who discovered the Turkana Boy, the most complete specimen of Homo erectus, on a slope covered with black lava pebbles.
Richard Leakey and Roger Lewin, Origins Reconsidered: In Search of What Makes Us Human (1992), 26.
A mathematical problem should be difficult in order to entice us, yet not completely inaccessible, lest it mock at our efforts. It should be to us a guide post on the mazy paths to hidden truths, and ultimately a reminder of our pleasure in the successful solution.
In Mathematical Problems', Bulletin American Mathematical Society, 8, 438.
About eight days ago I discovered that sulfur in burning, far from losing weight, on the contrary, gains it; it is the same with phosphorus; this increase of weight arises from a prodigious quantity of air that is fixed during combustion and combines with the vapors. This discovery, which I have established by experiments, that I regard as decisive, has led me to think that what is observed in the combustion of sulfur and phosphorus may well take place in the case of all substances that gain in weight by combustion and calcination; and I am persuaded that the increase in weight of metallic calxes is due to the same cause... This discovery seems to me one of the most interesting that has been made since Stahl and since it is difficult not to disclose something inadvertently in conversation with friends that could lead to the truth I have thought it necessary to make the present deposit to the Secretary of the Academy to await the time I make my experiments public.
Sealed note deposited with the Secretary of the French Academy 1 Nov 1772. Oeuvres de Lavoisier, Correspondance, Fasc. II. 1770-75 (1957), 389-90. Adapted from translation by A. N. Meldrum, The Eighteenth-Century Revolution in Science (1930), 3.
After I had addressed myself to this very difficult and almost insoluble problem, the suggestion at length came to me how it could be solved with fewer and much simpler constructions than were formerly used, if some assumptions (which are called axioms) were granted me. They follow in this order.
There is no one center of all the celestial circles or spheres.
The center of the earth is not the center of the universe, but only of gravity and of the lunar sphere.
All the spheres revolve about the sun as their mid-point, and therefore the sun is the center of the universe.
The ratio of the earth’s distance from the sun to the height of the firmament is so much smaller than the ratio of the earth’s radius to its distance from the sun that the distance from the earth to the sun is imperceptible in comparison with the height of the firmament.
Whatever motion appears in the firmament arises not from any motion of the firmament, but from the earth’s motion. The earth together with its circumjacent elements performs a complete rotation on its fixed poles in a daily motion, while the firmament and highest heaven abide unchanged.
What appears to us as motions of the sun arise not from its motion but from the motion of the earth and our sphere, with which we revolve about the sun like any other planet. The earth has, then, more than one motion.
The apparent retrograde and direct motion of the planets arises not from their motion but from the earth’s. The motion of the earth alone, therefore, suffices to explain so many apparent inequalities in the heavens.
There is no one center of all the celestial circles or spheres.
The center of the earth is not the center of the universe, but only of gravity and of the lunar sphere.
All the spheres revolve about the sun as their mid-point, and therefore the sun is the center of the universe.
The ratio of the earth’s distance from the sun to the height of the firmament is so much smaller than the ratio of the earth’s radius to its distance from the sun that the distance from the earth to the sun is imperceptible in comparison with the height of the firmament.
Whatever motion appears in the firmament arises not from any motion of the firmament, but from the earth’s motion. The earth together with its circumjacent elements performs a complete rotation on its fixed poles in a daily motion, while the firmament and highest heaven abide unchanged.
What appears to us as motions of the sun arise not from its motion but from the motion of the earth and our sphere, with which we revolve about the sun like any other planet. The earth has, then, more than one motion.
The apparent retrograde and direct motion of the planets arises not from their motion but from the earth’s. The motion of the earth alone, therefore, suffices to explain so many apparent inequalities in the heavens.
'The Commentariolus', in Three Copernican Treatises (c.1510), trans. E. Rosen (1939), 58-9.
After physiology has taken Humpty Dumpty apart, it is difficult perhaps even unfashionable to put him back together again.
In 'The role of natural history in contemporary biology', BioScience (1986), 36, 327.
Alexander the king of the Macedonians, began like a wretch to learn geometry, that he might know how little the earth was, whereof he had possessed very little. Thus, I say, like a wretch for this, because he was to understand that he did bear a false surname. For who can be great in so small a thing? Those things that were delivered were subtile, and to be learned by diligent attention: not which that mad man could perceive, who sent his thoughts beyond the ocean sea. Teach me, saith he, easy things. To whom his master said: These things be the same, and alike difficult unto all. Think thou that the nature of things saith this. These things whereof thou complainest, they are the same unto all: more easy things can be given unto none; but whosoever will, shall make those things more easy unto himself. How? With uprightness of mind.
In Thomas Lodge (trans.), 'Epistle 91', The Workes of Lucius Annaeus Seneca: Both Morrall and Naturall (1614), 383. Also in Robert Édouard Moritz, Memorabilia Mathematica (1914), 135.
All things are difficult before they are easy.
No. 560 in Gnomologia: Adagies and Proverbs, Wise Sentences and Witty Sayings (1732), 21.
Altering a gene in the gene line to produce improved offspring is likely to be very difficult because of the danger of unwanted side effects. It would also raise obvious ethical problems.
Among our sensations it is difficult not to confuse what comes from the part of objects with what comes from the part of our senses. … Supposing this, one clearly sees that it is not easy to say much about colors,… and that all one can expect in such a difficult subject is to give some general rules and to derive from them consequences that can be of some use in the arts and satisfy somewhat the natural desire we have to render account of everything that appears to us.
'De la Nature des Couleurs', collected in Oeuvres (1717), 196–197.
As for what I have done as a poet, I take no pride in whatever. Excellent poets have lived at the same time with me, poets more excellent lived before me, and others will come after me. But that in my country I am the only person who knows the truth in the difficult science of colors—of that, I say, I am not a little proud, and here have a consciousness of superiority to many.
Wed 18 Feb 1829. Johann Peter Eckermann, Conversations with Goethe, ed. J. K. Moorhead and trans. J. Oxenford, (1971), 302.
As in Mathematicks, so in Natural Philosophy, the Investigation of difficult Things by the Method of Analysis, ought ever to precede the Method of Composition. This Analysis consists in making Experiments and Observations, and in drawing general Conclusions from them by Induction, and admitting of no Objections against the Conclusions, but such as are taken from Experiments, or other certain Truths. For Hypotheses are not to be regarded in experimental Philosophy.
From Opticks, (1704, 2nd ed. 1718), Book 3, Query 31, 380.
As is well known the principle of virtual velocities transforms all statics into a mathematical assignment, and by D'Alembert's principle for dynamics, the latter is again reduced to statics. Although it is is very much in order that in gradual training of science and in the instruction of the individual the easier precedes the more difficult, the simple precedes the more complicated, the special precedes the general, yet the min, once it has arrived at the higher standpoint, demands the reverse process whereby all statics appears only as a very special case of mechanics.
Collected Works (1877), Vol. 5, 25-26. Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 412.
At terrestrial temperatures matter has complex properties which are likely to prove most difficult to unravel; but it is reasonable to hope that in the not too distant future we shall be competent to understand so simple a thing as a star.
The Internal Constitution of Stars, Cambridge. (1926, 1988), 393.
At that point, my sense of dissatisfaction was so strong that I firmly resolved to start thinking until I should find a purely arithmetic and absolutely rigorous foundation of the principles of infinitesimal analysis. … I achieved this goal on November 24th, 1858, … but I could not really decide upon a proper publication, because, firstly, the subject is not easy to present, and, secondly, the material is not very fruitful.
(1872). As quoted in Ernst Hairer and Gerhard Wanner, Analysis by Its History (2008), 177.
At the age of eleven, I began Euclid, with my brother as my tutor. ... I had not imagined that there was anything so delicious in the world. After I had learned the fifth proposition, my brother told me that it was generally considered difficult, but I had found no difficulty whatsoever. This was the first time it had dawned on me that I might have some intelligence.
In Autobiography: 1872-1914 (1967), Vol. 1, 37-38.
Augustine's Law XVI: Software is like entropy. It is difficult to grasp, weighs nothing, and obeys the second law of thermodynamics; i.e. it always increases.
In Augustine's Laws (1997), 114.
Be of good cheer. Do not think of today’s failures, but of the success that may come tomorrow. You have set yourself a difficult task, but you will succeed if you persevere; and you will find a joy in overcoming obstacles.
…...
Before the introduction of the Arabic notation, multiplication was difficult, and the division even of integers called into play the highest mathematical faculties. Probably nothing in the modern world could have more astonished a Greek mathematician than to learn that, under the influence of compulsory education, the whole population of Western Europe, from the highest to the lowest, could perform the operation of division for the largest numbers. This fact would have seemed to him a sheer impossibility. … Our modern power of easy reckoning with decimal fractions is the most miraculous result of a perfect notation.
In Introduction to Mathematics (1911), 59.
Bradley is one of the few basketball players who have ever been appreciatively cheered by a disinterested away-from-home crowd while warming up. This curious event occurred last March, just before Princeton eliminated the Virginia Military Institute, the year’s Southern Conference champion, from the NCAA championships. The game was played in Philadelphia and was the last of a tripleheader. The people there were worn out, because most of them were emotionally committed to either Villanova or Temple-two local teams that had just been involved in enervating battles with Providence and Connecticut, respectively, scrambling for a chance at the rest of the country. A group of Princeton players shooting basketballs miscellaneously in preparation for still another game hardly promised to be a high point of the evening, but Bradley, whose routine in the warmup time is a gradual crescendo of activity, is more interesting to watch before a game than most players are in play. In Philadelphia that night, what he did was, for him, anything but unusual. As he does before all games, he began by shooting set shots close to the basket, gradually moving back until he was shooting long sets from 20 feet out, and nearly all of them dropped into the net with an almost mechanical rhythm of accuracy. Then he began a series of expandingly difficult jump shots, and one jumper after another went cleanly through the basket with so few exceptions that the crowd began to murmur. Then he started to perform whirling reverse moves before another cadence of almost steadily accurate jump shots, and the murmur increased. Then he began to sweep hook shots into the air. He moved in a semicircle around the court. First with his right hand, then with his left, he tried seven of these long, graceful shots-the most difficult ones in the orthodoxy of basketball-and ambidextrously made them all. The game had not even begun, but the presumably unimpressible Philadelphians were applauding like an audience at an opera.
A Sense of Where You Are: Bill Bradley at Princeton
But I must confess I am jealous of the term atom; for though it is very easy to talk of atoms, it is very difficult to form a clear idea of their nature, especially when compounded bodies are under consideration.
'On the Absolute Quantity of Electricity Associated with the Particles or Atoms of Matter,' (31 Dec 1833), published in Philosophical Transactions (Jan 1834) as part of Series VII. Collected in Experimental Researches in Electricity: Reprinted from the Philosophical Transactions of 1831-1838 (1839), 256.
But if anyone, well seen in the knowledge, not onely of Sacred and exotick History, but of Astronomical Calculation, and the old Hebrew Kalendar, shall apply himself to these studies, I judge it indeed difficult, but not impossible for such a one to attain, not onely the number of years, but even, of dayes from the Creation of the World.
In 'Epistle to the Reader', The Annals of the World (1658). As excerpted in Wallen Yep, Man Before Adam: A Correction to Doctrinal Theology, "The Missing Link Found" (2002), 18.
But just as much as it is easy to find the differential of a given quantity, so it is difficult to find the integral of a given differential. Moreover, sometimes we cannot say with certainty whether the integral of a given quantity can be found or not.
Webmaster has looked and found no citation, and no example, in books with this wording, earlier than in a list of quotes, without citation, in Baumslag Benjamin, Fundamentals Of Teaching Mathematics At University Level (2000), 214. The original would be in native French, so different translations are possible. Can you help?
Common to all these types is the anthropomorphic character of their conception of God. In general, only individuals of exceptional endowments, and exceptionally high-minded communities, rise to any considerable extent above this level. But there is a third stage of religious experience which belongs to all of them, even though it is rarely found in a pure form: I shall call it cosmic religious feeling. It is very difficult to elucidate this feeling to anyone who is entirely without it, especially as there is no anthropomorphic conception of God corresponding to it.
…...
Concerning alchemy it is more difficult to discover the actual state of things, in that the historians who specialise in this field seem sometimes to be under the wrath of God themselves; for, like those who write of the Bacon-Shakespeare controversy or on Spanish politics, they seem to become tinctured with the kind of lunacy they set out to describe.
The Origins of Modern Science (1949), 115.
Daniel Bernoulli used to tell two little adventures, which he said had given him more pleasure than all the other honours he had received. Travelling with a learned stranger, who, being pleased with his conversation, asked his name; “I am Daniel Bernoulli,” answered he with great modesty; “and I,” said the stranger (who thought he meant to laugh at him) “am Isaac Newton.” Another time, having to dine with the celebrated Koenig, the mathematician, who boasted, with some degree of self-complacency, of a difficult problem he had solved with much trouble, Bernoulli went on doing the honours of his table, and when they went to drink coffee he presented Koenig with a solution of the problem more elegant than his own.
In A Philosophical and Mathematical Dictionary (1815), 1, 226.
Diamond, for all its great beauty, is not nearly as interesting as the hexagonal plane of graphite. It is not nearly as interesting because we live in a three-dimensional space, and in diamond each atom is surrounded in all three directions in space by a full coordination. Consequently, it is very difficult for an atom inside the diamond lattice to be confronted with anything else in this 3D world because all directions are already taken up.
From Nobel Lecture (7 Dec 1996), 'Discovering the Fullerenes', collected in Ingmar Grenthe (ed.), Nobel Lectures, Chemistry 1996-2000 (2003).
England and all civilised nations stand in deadly peril of not having enough to eat. As mouths multiply, food resources dwindle. Land is a limited quantity, and the land that will grow wheat is absolutely dependent on difficult and capricious natural phenomena... I hope to point a way out of the colossal dilemma. It is the chemist who must come to the rescue of the threatened communities. It is through the laboratory that starvation may ultimately be turned into plenty... The fixation of atmospheric nitrogen is one of the great discoveries, awaiting the genius of chemists.
Presidential Address to the British Association for the Advancement of Science 1898. Published in Chemical News, 1898, 78, 125.
Even more difficult to explain, than the breaking-up of a single mass into fragments, and the drifting apart of these blocks to form the foundations of the present-day continents, is the explanation of the original production of the single mass, or PANGAEA, by the concentration of the former holosphere of granitic sial into a hemisphere of compressed and crushed gneisses and schists. Creep and the effects of compression, due to shrinking or other causes, have been appealed to but this is hardly a satisfactory explanation. The earth could no more shrug itself out of its outer rock-shell unaided, than an animal could shrug itself out of its hide, or a man wriggle out of his skin, or even out of his closely buttoned coat, without assistance either of his own hands or those of others.
The Rhythm of Ages (1940), 9-10.
Every discovery, every enlargement of the understanding, begins as an imaginative preconception of what the truth might be. The imaginative preconception—a “hypothesis”—arises by a process as easy or as difficult to understand as any other creative act of mind; it is a brainwave, an inspired guess, a product of a blaze of insight. It comes anyway from within and cannot be achieved by the exercise of any known calculus of discovery.
In Advice to a Young Scientist (1979), 84.
Everyone is aware of the difficult and menacing situation in which human society–shrunk into one community with a common fate–now finds itself, but only a few act accordingly. Most people go on living their every-day life: half frightened, half indifferent, they behold the ghostly tragicomedy which is being performed on the international stage before the eyes and ears of the world. But on that stage, on which the actors under the floodlights play their ordained parts, our fate of tomorrow, life or death of the nations, is being decided.
…...
Examples ... show how difficult it often is for an experimenter to interpret his results without the aid of mathematics.
Quoted in E. T. Bell, Men of Mathematics, xvi.
Experience hobbles progress and leads to abandonment of difficult problems; it encourages the initiated to walk on the shady side of the street in the direction of experiences that have been pleasant. Youth without experience attacks the unsolved problems which maturer age with experience avoids, and from the labors of youth comes progress. Youth has dreams and visions, and will not be denied.
From speech 'In the Time of Henry Jacob Bigelow', given to the Boston Surgical Society, Medalist Meeting (6 Jun 1921). Printed in Journal of the Medical Association (1921), 77, 599.
Experiments in geology are far more difficult than in physics and chemistry because of the greater size of the objects, commonly outside our laboratories, up to the earth itself, and also because of the fact that the geologic time scale exceeds the human time scale by a million and more times. This difference in time allows only direct observations of the actual geologic processes, the mind having to imagine what could possibly have happened in the past.
In 'The Scientific Character of Geology', The Journal of Geology (Jul 1961), 69, No. 4, 455-6.
Experiments on ornamental plants undertaken in previous years had proven that, as a rule, hybrids do not represent the form exactly intermediate between the parental strains. Although the intermediate form of some of the more striking traits, such as those relating to shape and size of leaves, pubescence of individual parts, and so forth, is indeed nearly always seen, in other cases one of the two parental traits is so preponderant that it is difficult or quite impossible, to detect the other in the hybrid. The same is true for Pisum hybrids. Each of the seven hybrid traits either resembles so closely one of the two parental traits that the other escapes detection, or is so similar to it that no certain distinction can be made. This is of great importance to the definition and classification of the forms in which the offspring of hybrids appear. In the following discussion those traits that pass into hybrid association entirely or almost entirely unchanged, thus themselves representing the traits of the hybrid, are termed dominating and those that become latent in the association, recessive. The word 'recessive' was chosen because the traits so designated recede or disappear entirely in the hybrids, but reappear unchanged in their progeny, as will be demonstrated later.
'Experiments on Plant Hybrids' (1865). In Curt Stern and Eva R. Sherwood (eds.), The Origin of Genetics: A Mendel Source Book (1966), 9.
Falsehood is so easy, truth so difficult.
In Adam Bede (1859, 1860), 151.
Fanatical ethnic or religious or national chauvinisms are a little difficult to maintain when we see our planet as a fragile blue crescent fading to become an inconspicuous point of light against a bastion and citadel of the stars.
Cosmos
Few will deny that even in the first scientific instruction in mathematics the most rigorous method is to be given preference over all others. Especially will every teacher prefer a consistent proof to one which is based on fallacies or proceeds in a vicious circle, indeed it will be morally impossible for the teacher to present a proof of the latter kind consciously and thus in a sense deceive his pupils. Notwithstanding these objectionable so-called proofs, so far as the foundation and the development of the system is concerned, predominate in our textbooks to the present time. Perhaps it will be answered, that rigorous proof is found too difficult for the pupil’s power of comprehension. Should this be anywhere the case,—which would only indicate some defect in the plan or treatment of the whole,—the only remedy would be to merely state the theorem in a historic way, and forego a proof with the frank confession that no proof has been found which could be comprehended by the pupil; a remedy which is ever doubtful and should only be applied in the case of extreme necessity. But this remedy is to be preferred to a proof which is no proof, and is therefore either wholly unintelligible to the pupil, or deceives him with an appearance of knowledge which opens the door to all superficiality and lack of scientific method.
In 'Stücke aus dem Lehrbuche der Arithmetik', Werke, Bd. 2 (1904), 296.
First, as concerns the success of teaching mathematics. No instruction in the high schools is as difficult as that of mathematics, since the large majority of students are at first decidedly disinclined to be harnessed into the rigid framework of logical conclusions. The interest of young people is won much more easily, if sense-objects are made the starting point and the transition to abstract formulation is brought about gradually. For this reason it is psychologically quite correct to follow this course.
Not less to be recommended is this course if we inquire into the essential purpose of mathematical instruction. Formerly it was too exclusively held that this purpose is to sharpen the understanding. Surely another important end is to implant in the student the conviction that correct thinking based on true premises secures mastery over the outer world. To accomplish this the outer world must receive its share of attention from the very beginning.
Doubtless this is true but there is a danger which needs pointing out. It is as in the case of language teaching where the modern tendency is to secure in addition to grammar also an understanding of the authors. The danger lies in grammar being completely set aside leaving the subject without its indispensable solid basis. Just so in Teaching of Mathematics it is possible to accumulate interesting applications to such an extent as to stunt the essential logical development. This should in no wise be permitted, for thus the kernel of the whole matter is lost. Therefore: We do want throughout a quickening of mathematical instruction by the introduction of applications, but we do not want that the pendulum, which in former decades may have inclined too much toward the abstract side, should now swing to the other extreme; we would rather pursue the proper middle course.
Not less to be recommended is this course if we inquire into the essential purpose of mathematical instruction. Formerly it was too exclusively held that this purpose is to sharpen the understanding. Surely another important end is to implant in the student the conviction that correct thinking based on true premises secures mastery over the outer world. To accomplish this the outer world must receive its share of attention from the very beginning.
Doubtless this is true but there is a danger which needs pointing out. It is as in the case of language teaching where the modern tendency is to secure in addition to grammar also an understanding of the authors. The danger lies in grammar being completely set aside leaving the subject without its indispensable solid basis. Just so in Teaching of Mathematics it is possible to accumulate interesting applications to such an extent as to stunt the essential logical development. This should in no wise be permitted, for thus the kernel of the whole matter is lost. Therefore: We do want throughout a quickening of mathematical instruction by the introduction of applications, but we do not want that the pendulum, which in former decades may have inclined too much toward the abstract side, should now swing to the other extreme; we would rather pursue the proper middle course.
In Ueber den Mathematischen Unterricht an den hoheren Schulen; Jahresbericht der Deutschen Mathematiker Vereinigung, Bd. 11, 131.
For a dying man it is not a difficult decision [to agree to become the world's first heart transplant] … because he knows he is at the end. If a lion chases you to the bank of a river filled with crocodiles, you will leap into the water convinced you have a chance to swim to the other side. But you would not accept such odds if there were no lion.
In Janie B. Butts and Karen Rich, Nursing Ethics (2005), 59.
For terrestrial vertebrates, the climate in the usual meteorological sense of the term would appear to be a reasonable approximation of the conditions of temperature, humidity, radiation, and air movement in which terrestrial vertebrates live. But, in fact, it would be difficult to find any other lay assumption about ecology and natural history which has less general validity. … Most vertebrates are much smaller than man and his domestic animals, and the universe of these small creatures is one of cracks and crevices, holes in logs, dense underbrush, tunnels, and nests—a world where distances are measured in yards rather than miles and where the difference between sunshine and shadow may be the difference between life and death. Actually, climate in the usual sense of the term is little more than a crude index to the physical conditions in which most terrestrial animals live.
From 'Interaction of physiology and behavior under natural conditions', collected in R.I. Bowman (ed.), The Galapagos (1966), 40.
For we may remark generally of our mathematical researches, that these auxiliary quantities, these long and difficult calculations into which we are often drawn, are almost always proofs that we have not in the beginning considered the objects themselves so thoroughly and directly as their nature requires, since all is abridged and simplified, as soon as we place ourselves in a right point of view.
In Théorie Nouvelle de la Rotation des Corps (1834). As translated by Charles Thomas Whitley in Outlines of a New Theory of Rotatory Motion (1834), 4.
Fossil bones and footsteps and ruined homes are the solid facts of history, but the surest hints, the most enduring signs, lie in those miniscule genes. For a moment we protect them with our lives, then like relay runners with a baton, we pass them on to be carried by our descendents. There is a poetry in genetics which is more difficult to discern in broken bomes, and genes are the only unbroken living thread that weaves back and forth through all those boneyards.
The Self-Made Man: Human Evolution From Eden to Extinction (1996), 13.
From Pythagoras (ca. 550 BC) to Boethius (ca AD 480-524), when pure mathematics consisted of arithmetic and geometry while applied mathematics consisted of music and astronomy, mathematics could be characterized as the deductive study of “such abstractions as quantities and their consequences, namely figures and so forth” (Aquinas ca. 1260). But since the emergence of abstract algebra it has become increasingly difficult to formulate a definition to cover the whole of the rich, complex and expanding domain of mathematics.
In 100 Years of Mathematics: a Personal Viewpoint (1981), 2.
From the point of view of the pure morphologist the recapitulation theory is an instrument of research enabling him to reconstruct probable lines of descent; from the standpoint of the student of development and heredity the fact of recapitulation is a difficult problem whose solution would perhaps give the key to a true understanding of the real nature of heredity.
Form and Function: A Contribution to the History of Animal Morphology (1916), 312-3.
General practice is at least as difficult, if it is to be carried on well and successfully, as any special practice can be, and probably more so; for the G.P. has to live continually, as it were, with the results of his handiwork.
The Corner of Harley Street: Being Some Familiar Correspondence of Peter Harding, M. D., Ch. 26.
Generality of points of view and of methods, precision and elegance in presentation, have become, since Lagrange, the common property of all who would lay claim to the rank of scientific mathematicians. And, even if this generality leads at times to abstruseness at the expense of intuition and applicability, so that general theorems are formulated which fail to apply to a single special case, if furthermore precision at times degenerates into a studied brevity which makes it more difficult to read an article than it was to write it; if, finally, elegance of form has well-nigh become in our day the criterion of the worth or worthlessness of a proposition,—yet are these conditions of the highest importance to a wholesome development, in that they keep the scientific material within the limits which are necessary both intrinsically and extrinsically if mathematics is not to spend itself in trivialities or smother in profusion.
In Die Entwickdung der Mathematik in den letzten Jahrhunderten (1884), 14-15.
Gifford Pinchot points out that in colonial and pioneer days the forest was a foe and an obstacle to the settler. It had to be cleared away... But [now] as a nation we have not yet come to have a proper respect for the forest and to regard it as an indispensable part of our resources—one which is easily destroyed but difficult to replace; one which confers great benefits while it endures, but whose disappearance is accompanied by a train of evil consequences not readily foreseen and positively irreparable.
Concluding remark, in 'A Country that has Used up its Trees', The Outlook (24 Mar 1906), 82, 700. The topic of the article is the extensive deforestation in China, its consequences, and that America must avoid such massive problems.
Good people are seldom fully recognised during their lifetimes, and here, there are serious problems of corruption. One day it will be realised that my findings should have been acknowledged.
It was difficult, but she always smiled when asked why she went on when recognition eluded her in her own country.
It was difficult, but she always smiled when asked why she went on when recognition eluded her in her own country.
Quoted in obituary by Anthony Tucker, 'Alice Stewart', The Guardian newspaper (28 Jun 2002).
Good sense is, of all things among men, the most equally distributed ; for every one thinks himself so abundantly provided with it, that those even who are the most difficult to satisfy in everything else, do not usually desire a larger measure of this quality than they already possess.
In Discours de la Méthode (1637), Part 1. English version as given in John Veitch (trans.), The Method, Meditations, and Selections from the Principles of Descartes (1880), 3. Also seen translated as “Of all things, good sense is the most fairly distributed: everyone thinks he is so well supplied with it that even those who are the hardest to satisfy in every other respect never desire more of it than they already have,” or “Good sense is of all things in the world the most equally distributed, for everybody thinks he is so well supplied with it that even those most difficult to please in all other matters never desire more of it than they already possess.” From the original French, “Le bon sens est la chose du monde la mieux partagée; car chacun pense en être si bien pourvu, que ceux même qui sont les plus difficiles à contenter en toute autre chose n'ont point coutume d'en désirer plus qu'ils en ont.”
Good work is no done by “humble” men. It is one of the first duties of a professor, for example, in any subject, to exaggerate a little both the importance of his subject and his own importance in it. A man who is always asking “Is what I do worth while?” and “Am I the right person to do it?” will always be ineffective himself and a discouragement to others. He must shut his eyes a little and think a little more of his subject and himself than they deserve. This is not too difficult: it is harder not to make his subject and himself ridiculous by shutting his eyes too tightly.
In A Mathematician’s Apology (1940, 1967), 66.
He [General Nathan Bedford Forrest] possessed a remarkable genius for mathematics, a subject in which he had absolutely no training. He could with surprising facility solve the most difficult problems in algebra, geometry, and trigonometry, only requiring that the theorem or rule be carefully read aloud to him.
In Life of General Nathan Bedford Forrest (1899), 627.
I believe scientists have a duty to share the excitement and pleasure of their work with the general public, and I enjoy the challenge of presenting difficult ideas in an understandable way.
From Autobiography in Wilhelm Odelberg (ed.), Les Prix Nobel en 1974/Nobel Lectures (1975)
I believe that the useful methods of mathematics are easily to be learned by quite young persons, just as languages are easily learned in youth. What a wondrous philosophy and history underlie the use of almost every word in every language—yet the child learns to use the word unconsciously. No doubt when such a word was first invented it was studied over and lectured upon, just as one might lecture now upon the idea of a rate, or the use of Cartesian co-ordinates, and we may depend upon it that children of the future will use the idea of the calculus, and use squared paper as readily as they now cipher. … When Egyptian and Chaldean philosophers spent years in difficult calculations, which would now be thought easy by young children, doubtless they had the same notions of the depth of their knowledge that Sir William Thomson might now have of his. How is it, then, that Thomson gained his immense knowledge in the time taken by a Chaldean philosopher to acquire a simple knowledge of arithmetic? The reason is plain. Thomson, when a child, was taught in a few years more than all that was known three thousand years ago of the properties of numbers. When it is found essential to a boy’s future that machinery should be given to his brain, it is given to him; he is taught to use it, and his bright memory makes the use of it a second nature to him; but it is not till after-life that he makes a close investigation of what there actually is in his brain which has enabled him to do so much. It is taken because the child has much faith. In after years he will accept nothing without careful consideration. The machinery given to the brain of children is getting more and more complicated as time goes on; but there is really no reason why it should not be taken in as early, and used as readily, as were the axioms of childish education in ancient Chaldea.
In Teaching of Mathematics (1902), 14.
I believed that, instead of the multiplicity of rules that comprise logic, I would have enough in the following four, as long as I made a firm and steadfast resolution never to fail to observe them.
The first was never to accept anything as true if I did not know clearly that it was so; that is, carefully to avoid prejudice and jumping to conclusions, and to include nothing in my judgments apart from whatever appeared so clearly and distinctly to my mind that I had no opportunity to cast doubt upon it.
The second was to subdivide each on the problems I was about to examine: into as many parts as would be possible and necessary to resolve them better.
The third was to guide my thoughts in an orderly way by beginning, as if by steps, to knowledge of the most complex, and even by assuming an order of the most complex, and even by assuming an order among objects in! cases where there is no natural order among them.
And the final rule was: in all cases, to make such comprehensive enumerations and such general review that I was certain not to omit anything.
The long chains of inferences, all of them simple and easy, that geometers normally use to construct their most difficult demonstrations had given me an opportunity to think that all the things that can fall within the scope of human knowledge follow from each other in a similar way, and as long as one avoids accepting something as true which is not so, and as long as one always observes the order required to deduce them from each other, there cannot be anything so remote that it cannot be reached nor anything so hidden that it cannot be uncovered.
The first was never to accept anything as true if I did not know clearly that it was so; that is, carefully to avoid prejudice and jumping to conclusions, and to include nothing in my judgments apart from whatever appeared so clearly and distinctly to my mind that I had no opportunity to cast doubt upon it.
The second was to subdivide each on the problems I was about to examine: into as many parts as would be possible and necessary to resolve them better.
The third was to guide my thoughts in an orderly way by beginning, as if by steps, to knowledge of the most complex, and even by assuming an order of the most complex, and even by assuming an order among objects in! cases where there is no natural order among them.
And the final rule was: in all cases, to make such comprehensive enumerations and such general review that I was certain not to omit anything.
The long chains of inferences, all of them simple and easy, that geometers normally use to construct their most difficult demonstrations had given me an opportunity to think that all the things that can fall within the scope of human knowledge follow from each other in a similar way, and as long as one avoids accepting something as true which is not so, and as long as one always observes the order required to deduce them from each other, there cannot be anything so remote that it cannot be reached nor anything so hidden that it cannot be uncovered.
Discourse on Method in Discourse on Method and Related Writings (1637), trans. Desmond M. Clarke, Penguin edition (1999), Part 2, 16.
I concluded that I might take as a general rule the principle that all things which we very clearly and obviously conceive are true: only observing, however, that there is some difficulty in rightly determining the objects which we distinctly conceive.
In Discours de la Méthode (1637), as translated by J. Veitch, A Discourse on Method (1912), 27.
I devoted myself to studying the texts—the original and commentaries—in the natural sciences and metaphysics, and the gates of knowledge began opening for me. Next I sought to know medicine, and so read the books written on it. Medicine is not one of the difficult sciences, and therefore, I excelled in it in a very short time, to the point that distinguished physicians began to read the science of medicine under me. I cared for the sick and there opened to me some of the doors of medical treatment that are indescribable and can be learned only from practice. In addition I devoted myself to jurisprudence and used to engage in legal disputations, at that time being sixteen years old.
— Avicenna
W. E. Gohhnan, The Life of Ibn Sina: A Critical Edition and Annotated Translation (1974), 25-7.
I do ... humbly conceive (tho' some possibly may think there is too much notice taken of such a trivial thing as a rotten Shell, yet) that Men do generally rally too much slight and pass over without regard these Records of Antiquity which Nature have left as Monuments and Hieroglyphick Characters of preceding Transactions in the like duration or Transactions of the Body of the Earth, which are infinitely more evident and certain tokens than any thing of Antiquity that can be fetched out of Coins or Medals, or any other way yet known, since the best of those ways may be counterfeited or made by Art and Design, as may also Books, Manuscripts and Inscriptions, as all the Learned are now sufficiently satisfied, has often been actually practised; but those Characters are not to be Counterfeited by all the Craft in the World, nor can they be doubted to be, what they appear, by anyone that will impartially examine the true appearances of them: And tho' it must be granted, that it is very difficult to read them, and to raise a Chronology out of them, and to state the intervalls of the Times wherein such, or such Catastrophies and Mutations have happened; yet 'tis not impossible, but that, by the help of those joined to ' other means and assistances of Information, much may be done even in that part of Information also.
Lectures and Discourses of Earthquakes (1668). In The Posthumous Works of Robert Hooke, containing his Cutlerian Lectures and other Discourses read at the Meetings of the Illustrious Royal Society (1705), 411.
I do not think it is possible really to understand the successes of science without understanding how hard it is—how easy it is to be led astray, how difficult it is to know at any time what is the next thing to be done.
In The First Three Minutes: A Modern View of the Origin of the Universe (1977), 132.
I feel very strongly indeed that a Cambridge education for our scientists should include some contact with the humanistic side. The gift of expression is important to them as scientists; the best research is wasted when it is extremely difficult to discover what it is all about ... It is even more important when scientists are called upon to play their part in the world of affairs, as is happening to an increasing extent.
From essay in Thomas Rice Henn, The Apple and the Spectroscope: Being Lectures on Poetry Designed (in the Main) for Science Students (1951), 142.
I have never thought a boy should undertake abstruse or difficult sciences, such as Mathematics in general, till fifteen years of age at soonest. Before that time they are best employed in learning the languages, which is merely a matter of memory.
Letter to Ralph Izard (17 July 1788).
I have said that mathematics is the oldest of the sciences; a glance at its more recent history will show that it has the energy of perpetual youth. The output of contributions to the advance of the science during the last century and more has been so enormous that it is difficult to say whether pride in the greatness of achievement in this subject, or despair at his inability to cope with the multiplicity of its detailed developments, should be the dominant feeling of the mathematician. Few people outside of the small circle of mathematical specialists have any idea of the vast growth of mathematical literature. The Royal Society Catalogue contains a list of nearly thirty- nine thousand papers on subjects of Pure Mathematics alone, which have appeared in seven hundred serials during the nineteenth century. This represents only a portion of the total output, the very large number of treatises, dissertations, and monographs published during the century being omitted.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 285.
I like a deep and difficult investigation when I happen to have made it easy to myself, if not to all others; and there is a spirit of gambling in this, whether, as by the cast of a die, a calculation è perte de vue shall bring out a beautiful and perfect result or shall be wholly thrown away. Scientific investigations are a sort of warfare carried on in the closet or on the couch against all one's contemporaries and predecessors; I have often gained a signal victory when I have been half asleep, but more frequently have found, upon being thoroughly awake, that the enemy had still the advantage of me, when I thought I had him fast in a corner, and all this you see keeps me alive.
Letter to Hudson Gurney, quoted in George Peacock, The Life of Thomas Young (1855), 239.
I must not pass by Dr. Young called Phaenomenon Young at Cambridge. A man of universal erudition, & almost universal accomplishments. Had he limited himself to anyone department of knowledge, he must have been first in that department. But as a mathematician, a scholar, a hieroglyphist, he was eminent; & he knew so much that it is difficult to say what he did not know. He was a most amiable & good-tempered man; too fond, perhaps, of the society of persons of rank for a true philosopher.
J. Z. Fullmer, 'Davy's Sketches of his Contemporaries', Chymia (1967), 12, 135.
I regret that it has been necessary for me in this lecture to administer such a large dose of four-dimensional geometry. I do not apologize, because I am really not responsible for the fact that nature in its most fundamental aspect is four-dimensional. Things are what they are; and it is useless to disguise the fact that “what things are” is often very difficult for our intellects to follow.
From The Concept of Nature (1920, 1964), 118.
I took this view of the subject. The medulla spinalis has a central division, and also a distinction into anterior and posterior fasciculi, corresponding with the anterior and posterior portions of the brain. Further we can trace down the crura of the cerebrum into the anterior fasciculus of the spinal marrow, and the crura of the cerebellum into the posterior fasciculus. I thought that here I might have an opportunity of touching the cerebellum, as it were, through the posterior portion of the spinal marrow, and the cerebrum by the anterior portion. To this end I made experiments which, though they were not conclusive, encouraged me in the view I had taken. I found that injury done to the anterior portion of the spinal marrow, convulsed the animal more certainly than injury done to the posterior portion; but I found it difficult to make the experiment without injuring both portions.
Idea of a New Anatomy of the Brain (1811), 21-22.
I watched Baeyer activating magnesium with iodine for a difficult Grignard reaction; it was done in a test tube, which he watched carefully as he moved it gently by hand over a flame for three quarters of an hour. The test tube was the apparatus to Baeyer.
In Richard Willstätter, Arthur Stoll (ed. of the original German) and Lilli S. Hornig (trans.), From My Life: The Memoirs of Richard Willstätter (1958), 140.
I would not leave anything to a man of action; as he would be tempted to give up work. On the other hand I would like to help dreamers as they find it difficult to get on in life.
A few months before his death. As translated and stated in H. Schück and Ragnar Sohlman, The Life of Alfred Nobel (1929), 241.
I’ll deal with the most difficult problem first. Creation ex nihilo. The adipose argument is that “God did it.” That of course is the lazy man’s elixir. Sort of a cocktail made up of a swig of credulity and a teaspoon full of unwillingness to think. In short, it’s an explanation that avoids explanation.
From transcript of debate (Apr 1998) with William Lane Craig at the Carter Presidential Center, Atlanta, Georgia, 'What is the evidence for/against the existence of God?' on reasonablefaith.org website.
If I were working in astrophysics I would find it quite hard to explain to people what I was doing. Natural history is a pretty easy thing to explain. It does have its complexities, but nowhere do you speak about things that are outside people’s experience. You might speak about a species that is outside their experience, but nothing as remote as astrophysics.
From interview with Michael Bond, 'It’s a Wonderful Life', New Scientist (14 Dec 2002), 176, No. 2373, 48.
If man were by nature a solitary animal, the passions of the soul by which he was conformed to things so as to have knowledge of them would be sufficient for him; but since he is by nature a political and social animal it was necessary that his conceptions be made known to others. This he does through vocal sound. Therefore there had to be significant vocal sounds in order that men might live together. Whence those who speak different languages find it difficult to live together in social unity.
As quoted in Jeffrey J. Maciejewski, Thomas Aquinas on Persuasion: Action, Ends, and Natural Rhetoric (2013), 36.
If one looks with a cold eye at the mess man has made of history, it is difficult to avoid the conclusion that he has been afflicted by some built-in mental disorder which drives him towards self-destruction.
In The Ghost in the Machine (1967).
If the observation of the amount of heat the sun sends the earth is among the most important and difficult in astronomical physics, it may also be termed the fundamental problem of meteorology, nearly all whose phenomena would become predictable, if we knew both the original quantity and kind of this heat.
In Report of the Mount Whitney Expedition, quoted in Charles Greeley Abbot, Adventures in the World of Science (1958), 17. Also quoted and cited in David H. Devorkin, 'Charles Greeley Abbot', Biographical Memoirs (1998), 4.
If the world has begun with a single quantum, the notions of space and would altogether fail to have any meaning at the beginning; they would only begin to have a sensible meaning when the original quantum had been divided into a sufficient number of quanta. If this suggestion is correct, the beginning of the world happened a little before the beginning of space and time. I think that such a beginning of the world is far enough from the present order of Nature to be not at all repugnant. It may be difficult to follow up the idea in detail as we are not yet able to count the quantum packets in every case. For example, it may be that an atomic nucleus must be counted as a unique quantum, the atomic number acting as a kind of quantum number. If the future development of quantum theory happens to turn in that direction, we could conceive the beginning of the universe in the form of a unique atom, the atomic weight of which is the total mass of the universe. This highly unstable atom would divide in smaller and smaller atoms by a kind of super-radioactive process.
In a seminal short letter (457 words), 'The Beginning of the World from the Point of View of Quantum Theory', Nature (9 May 1931), 127, 706.
If there is real love, it is not difficult to exercise tolerance, for tolerance is the daughter of love—it is the truly Christian trait, which, of course, Christians of today do not practice.
English translation of the original German, “Liebt man sich wirklich, so ist es ja nicht schwer, die Toleranz zu üben, denn die Toleranz ist die Tochter der Liebe—es ist die eigentlich christliche Eigenschaft, die freilich von der heutigen Christenwelt nicht geübt wird.” In a letter to his father (7 Apr 1851), published in Briefe an seite Eltern, 1839 bis 1864 (1907).
If we ascribe the ejection of the proton to a Compton recoil from a quantum of 52 x 106 electron volts, then the nitrogen recoil atom arising by a similar process should have an energy not greater than about 400,000 volts, should produce not more than about 10,000 ions, and have a range in the air at N.T.P. of about 1-3mm. Actually, some of the recoil atoms in nitrogen produce at least 30,000 ions. In collaboration with Dr. Feather, I have observed the recoil atoms in an expansion chamber, and their range, estimated visually, was sometimes as much as 3mm. at N.T.P.
These results, and others I have obtained in the course of the work, are very difficult to explain on the assumption that the radiation from beryllium is a quantum radiation, if energy and momentum are to be conserved in the collisions. The difficulties disappear, however, if it be assumed that the radiation consists of particles of mass 1 and charge 0, or neutrons. The capture of the a-particle by the Be9 nucleus may be supposed to result in the formation of a C12 nucleus and the emission of the neutron. From the energy relations of this process the velocity of the neutron emitted in the forward direction may well be about 3 x 109 cm. per sec. The collisions of this neutron with the atoms through which it passes give rise to the recoil atoms, and the observed energies of the recoil atoms are in fair agreement with this view. Moreover, I have observed that the protons ejected from hydrogen by the radiation emitted in the opposite direction to that of the exciting a-particle appear to have a much smaller range than those ejected by the forward radiation.
This again receives a simple explanation on the neutron hypothesis.
These results, and others I have obtained in the course of the work, are very difficult to explain on the assumption that the radiation from beryllium is a quantum radiation, if energy and momentum are to be conserved in the collisions. The difficulties disappear, however, if it be assumed that the radiation consists of particles of mass 1 and charge 0, or neutrons. The capture of the a-particle by the Be9 nucleus may be supposed to result in the formation of a C12 nucleus and the emission of the neutron. From the energy relations of this process the velocity of the neutron emitted in the forward direction may well be about 3 x 109 cm. per sec. The collisions of this neutron with the atoms through which it passes give rise to the recoil atoms, and the observed energies of the recoil atoms are in fair agreement with this view. Moreover, I have observed that the protons ejected from hydrogen by the radiation emitted in the opposite direction to that of the exciting a-particle appear to have a much smaller range than those ejected by the forward radiation.
This again receives a simple explanation on the neutron hypothesis.
'Possible Existence of a Neutron', Letter to the Editor, Nature, 1932, 129, 312.
If you ask mathematicians what they do, you always get the same answer. They think. They think about difficult and unusual problems. (They never think about ordinary problems—they just write down the answers.)
As translated from Russian in 'A byl li brak?', Literaturnaya Gazeta (5 Dec 1979), 49, 12, as quoted and cited in The American Mathematical Monthly (Nov 1980), 87, No. 97, 696.
In an age of egoism, it is so difficult to persuade man that of all studies, the most important is that of himself. This is because egoism, like all passions, is blind. The attention of the egoist is directed to the immediate needs of which his senses give notice, and cannot be raised to those reflective needs that reason discloses to us; his aim is satisfaction, not perfection. He considers only his individual self; his species is nothing to him. Perhaps he fears that in penetrating the mysteries of his being he will ensure his own abasement, blush at his discoveries, and meet his conscience. True philosophy, always at one with moral science, tells a different tale. The source of useful illumination, we are told, is that of lasting content, is in ourselves. Our insight depends above all on the state of our faculties; but how can we bring our faculties to perfection if we do not know their nature and their laws! The elements of happiness are the moral sentiments; but how can we develop these sentiments without considering the principle of our affections, and the means of directing them? We become better by studying ourselves; the man who thoroughly knows himself is the wise man. Such reflection on the nature of his being brings a man to a better awareness of all the bonds that unite us to our fellows, to the re-discovery at the inner root of his existence of that identity of common life actuating us all, to feeling the full force of that fine maxim of the ancients: 'I am a man, and nothing human is alien to me.'
Considerations sur les diverses méthodes à suivre dans l'observation des peuples sauvages (1800) The Observation of Savage Peoples, trans. F. C. T. Moore (1969), 61.
In clinical investigation the sick individual is at the centre of the picture. The physician must have a deep interest in his patient’s economic and social structure as well as in his physical and psychic state. If attention is not paid to the diagnosis of the person the clinical investigator is apt to fail in studies of the patient’s disease. Without a consideration of the patient as a human being it would have been difficult to have fed patients daily large amounts of liver.
In Nobel Banquet speech (10 Dec 1934). Collected in Gustaf Santesson (ed.) Les Prix Nobel en 1934 (1935).
In describing a protein it is now common to distinguish the primary, secondary and tertiary structures. The primary structure is simply the order, or sequence, of the amino-acid residues along the polypeptide chains. This was first determined by Sanger using chemical techniques for the protein insulin, and has since been elucidated for a number of peptides and, in part, for one or two other small proteins. The secondary structure is the type of folding, coiling or puckering adopted by the polypeptide chain: the a-helix structure and the pleated sheet are examples. Secondary structure has been assigned in broad outline to a number of librous proteins such as silk, keratin and collagen; but we are ignorant of the nature of the secondary structure of any globular protein. True, there is suggestive evidence, though as yet no proof, that a-helices occur in globular proteins, to an extent which is difficult to gauge quantitatively in any particular case. The tertiary structure is the way in which the folded or coiled polypeptide chains are disposed to form the protein molecule as a three-dimensional object, in space. The chemical and physical properties of a protein cannot be fully interpreted until all three levels of structure are understood, for these properties depend on the spatial relationships between the amino-acids, and these in turn depend on the tertiary and secondary structures as much as on the primary. Only X-ray diffraction methods seem capable, even in principle, of unravelling the tertiary and secondary structures.
Co-author with G. Bodo, H. M. Dintzis, R. G. Parrish, H. Wyckoff, and D. C. Phillips
Co-author with G. Bodo, H. M. Dintzis, R. G. Parrish, H. Wyckoff, and D. C. Phillips
'A Three-Dimensional Model of the Myoglobin Molecule Obtained by X-ray Analysis', Nature (1958) 181, 662.
In the sciences hypothesis always precedes law, which is to say, there is always a lot of tall guessing before a new fact is established. The guessers are often quite as important as the factfinders; in truth, it would not be difficult to argue that they are more important.
From Baltimore Evening Sun (6 Apr 1931). Collected in A Mencken Chrestomathy (1949, 1956), 329.
In this age of specialization men who thoroughly know one field are often incompetent to discuss another. … The old problems, such as the relation of science and religion, are still with us, and I believe present as difficult dilemmas as ever, but they are not often publicly discussed because of the limitations of specialization.
Opening statement, in transcript of talk to the Caltech Lunch Forum (2 May 1956), 'The Relation of Science and Religion', collected in Richard Phillips Feynman and Jeffrey Robbins (ed.), The Pleasure of Finding Things Out: The Best Short Works of Richard P. Feynman (1999, 2005), 245-246.
Infinite space cannot be conceived by anybody; finite but unbounded space is difficult to conceive but not impossible. … [We] are using a conception of space which must have originated a million years ago and has become rather firmly imbedded in human thought. But the space of Physics ought not to be dominated by this creation of the dawning mind of an enterprising ape."
In The Nature of the Physical World (1929), 80-81.
Inventions are best developed on your own. When you work for other people or borrow money from them, maintaining freedom of intellect is difficult.
As quoted by Franz Lidz in 'Dr. NakaMats, the Man With 3300 Patents to His Name', Smithsonian Magazine (Dec 2012).
Is it not evident, that if the child is at any epoch of his long period of helplessness inured into any habit or fixed form of activity belonging to a lower stage of development, the tendency will be to arrest growth at that standpoint and make it difficult or next to impossible to continue the growth of the child?
In 'The Old Psychology vs. the New', Journal of Pedagogy (1894), 8, 76.
It is a scale of proportions which makes the bad difficult and the good easy.
…...
It is almost as difficult to make a man unlearn his errors, as his knowledge. Mal-information is more hopeless than non-information: for error is always more busy than ignorance. Ignorance is a blank sheet on which we may write; but error is a scribbled one on which we first erase. Ignorance is contented to stand still with her back to the truth; but error is more presumptuous, and proceeds, in the same direction. Ignorance has no light, but error follows a false one. The consequence is, that error, when she retraces her footsteps, has farther to go, before we can arrive at the truth, than ignorance.
Reflection 1, in Lacon: or Many things in Few Words; Addressed to Those Who Think (1820), 15.
It is by no means hopeless to expect to make a machine for really very difficult mathematical problems. But you would have to proceed step-by-step. I think electricity would be the best thing to rely on.
In Charles Sanders Peirce, Max Harold Fisch, Christian J. W. Kloesel Writings of Charles S. Peirce: 1884-1886 (1993), 422.
It is difficult even to attach a precise meaning to the term “scientific truth.” So different is the meaning of the word “truth” according to whether we are dealing with a fact of experience, a mathematical proposition or a scientific theory. “Religious truth” conveys nothing clear to me at all.
From 'Scientific Truth' in Essays in Science (1934, 2004), 11.
It is difficult for the matter-of-fact physicist to accept the view that the substratum of everything is of mental character. But no one can deny that mind is the first and most direct thing in our experience, and all else is remote inference—inference either intuitive or deliberate.
From Gifford Lecture, Edinburgh, (1927), 'Reality', collected in The Nature of the Physical World (1928), 281.
It is difficult to conceive a grander mass of vegetation:—the straight shafts of the timber-trees shooting aloft, some naked and clean, with grey, pale, or brown bark; others literally clothed for yards with a continuous garment of epiphytes, one mass of blossoms, especially the white Orchids Caelogynes, which bloom in a profuse manner, whitening their trunks like snow. More bulky trunks were masses of interlacing climbers, Araliaceae, Leguminosae, Vines, and Menispermeae, Hydrangea, and Peppers, enclosing a hollow, once filled by the now strangled supporting tree, which has long ago decayed away. From the sides and summit of these, supple branches hung forth, either leafy or naked; the latter resembling cables flung from one tree to another, swinging in the breeze, their rocking motion increased by the weight of great bunches of ferns or Orchids, which were perched aloft in the loops. Perpetual moisture nourishes this dripping forest: and pendulous mosses and lichens are met with in profusion.
Himalayan Journals (1854), vol. 1, 110-1.
It is difficult to discriminate the voice of truth from amid the clamour raised by heated partisans.
From Schillers Sammtliche Werke (1834), 934. Given with translation, in James Wood, Dictionary of Quotations from Ancient and Modern, English and Foreign Sources (1893), 382. The original German is: Schwer ist es, aus dem Geschrei erhitzter Parteien die Stimme der Wahrheit zu unterscheiden.
It is difficult to give an idea of the vast extent of modern mathematics. The word “extent” is not the right one: I mean extent crowded with beautiful detail—not an extent of mere uniformity such as an objectless plain, but of a tract of beautiful country seen at first in the distance, but which will bear to be rambled through and studied in every detail of hillside and valley, stream, rock, wood, and flower.
President’s address (1883) to the British Association for the Advancement of Science, in The Collected Mathematical Papers (1895), Vol. 8, xxii.
It is difficult to imagine a greater imposition [than adding] genes to future generations that changes the nature of future people.
in The Second Creation: Dolly and the Age of Biological Control
It is difficult to know how to treat the errors of the age. If a man oppose them, he stands alone; if he surrender to them, they bring him neither joy nor credit.
In The Maxims and Reflections of Goethe (1906), 185.
It is difficult to say what is impossible, for “The dream of yesterday is the hope of today and the reality of tomorrow.”
The opening clause is his own paraphrase of his own introductory remark, followed by quoting directly from his Oration (21 Jun 1904), 'On Taking Things for Granted', at his own graduation from South High School, Worcester, Massachusetts, in 'Material for an Autobiography of R.H. Goddard: written in July 1923 with interpolations made in 1933', The Papers of Robert H. Goddard: Vol. 1: 1898-1924 (1970), 11. The source of the paraphrase is seen in a longer direct quote from his Oration: “In the sciences we have learned that we are too ignorant to pronounce anything impossible,… The dream of yesterday is the hope of today and the reality of tomorrow”, in the epigraph on page v. The full Oration is on pp 63-66.
It is difficult to see anything but infatuation in the destructive temperament which leads to the action … that each of us is to rejoice that our several units are to be distinguished at death into countless millions of organisms; for such, it seems, is the latest revelation delivered from the fragile tripod of a modern Delphi.
In 'Dawn and the Creation of Worship', The Nineteenth Century, 1885, 18, 706.
It is easy for men to give advice, but difficult for one’s self to follow; we have an example in physicians: for their patients they order a strict regime, for themselves, on going to bed, they do all that they have forbidden to others.
— Philemon
'The Sicilian.' In Gustave Jules Witkowski, The Evil that Has Been Said of Doctors (1889), 4-5
It is exceptional that one should be able to acquire the understanding of a process without having previously acquired a deep familiarity with running it, with using it, before one has assimilated it in an instinctive and empirical way. Thus any discussion of the nature of intellectual effort in any field is difficult, unless it presupposes an easy, routine familiarity with that field. In mathematics this limitation becomes very severe.
In 'The Mathematician', Works of the Mind (1947), 1, No. 1. Collected in James Roy Newman (ed.), The World of Mathematics (1956), Vol. 4, 2053.
It is not possible to find in all geometry more difficult and more intricate questions or more simple and lucid explanations [than those given by Archimedes]. Some ascribe this to his natural genius; while others think that incredible effort and toil produced these, to all appearance, easy and unlaboured results. No amount of investigation of yours would succeed in attaining the proof, and yet, once seen, you immediately believe you would have discovered it; by so smooth and so rapid a path he leads you to the conclusion required.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
It is not so difficult a task as to plant new truths, as to root out old errors
Lacon: Many Things in Few Words (1820-22, 1866), 276.
It is not surprising that our language should be incapable of describing the processes occurring within the atoms, for, as has been remarked, it was invented to describe the experiences of daily life, and these consists only of processes involving exceedingly large numbers of atoms. Furthermore, it is very difficult to modify our language so that it will be able to describe these atomic processes, for words can only describe things of which we can form mental pictures, and this ability, too, is a result of daily experience. Fortunately, mathematics is not subject to this limitation, and it has been possible to invent a mathematical scheme—the quantum theory—which seems entirely adequate for the treatment of atomic processes; for visualization, however, we must content ourselves with two incomplete analogies—the wave picture and the corpuscular picture.
The Physical Principles of the Quantum Theory, trans. Carl Eckart and Frank C. Hoyt (1949), 11.
It is notoriously difficult to define the word living.
Opening sentence in Of Molecules and Men (1966, 2004), 3.
It is now necessary to indicate more definitely the reason why mathematics not only carries conviction in itself, but also transmits conviction to the objects to which it is applied. The reason is found, first of all, in the perfect precision with which the elementary mathematical concepts are determined; in this respect each science must look to its own salvation .... But this is not all. As soon as human thought attempts long chains of conclusions, or difficult matters generally, there arises not only the danger of error but also the suspicion of error, because since all details cannot be surveyed with clearness at the same instant one must in the end be satisfied with a belief that nothing has been overlooked from the beginning. Every one knows how much this is the case even in arithmetic, the most elementary use of mathematics. No one would imagine that the higher parts of mathematics fare better in this respect; on the contrary, in more complicated conclusions the uncertainty and suspicion of hidden errors increases in rapid progression. How does mathematics manage to rid itself of this inconvenience which attaches to it in the highest degree? By making proofs more rigorous? By giving new rules according to which the old rules shall be applied? Not in the least. A very great uncertainty continues to attach to the result of each single computation. But there are checks. In the realm of mathematics each point may be reached by a hundred different ways; and if each of a hundred ways leads to the same point, one may be sure that the right point has been reached. A calculation without a check is as good as none. Just so it is with every isolated proof in any speculative science whatever; the proof may be ever so ingenious, and ever so perfectly true and correct, it will still fail to convince permanently. He will therefore be much deceived, who, in metaphysics, or in psychology which depends on metaphysics, hopes to see his greatest care in the precise determination of the concepts and in the logical conclusions rewarded by conviction, much less by success in transmitting conviction to others. Not only must the conclusions support each other, without coercion or suspicion of subreption, but in all matters originating in experience, or judging concerning experience, the results of speculation must be verified by experience, not only superficially, but in countless special cases.
In Werke [Kehrbach] (1890), Bd. 5, 105. As quoted, cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 19.
It is perhaps difficult for a modern student of Physics to realize the basic taboo of the past period (before 1956) … it was unthinkable that anyone would question the validity of symmetries under “space inversion,” “charge conjugation” and “time reversal.” It would have been almost sacrilegious to do experiments to test such unholy thoughts.
In paper presented to the International Conference on the History of Original Ideas and Basic Discoveries, Erice, Sicily (27 Jul-4 Aug 1994), 'Parity Violation' collected in Harvey B. Newman, Thomas Ypsilantis History of Original Ideas and Basic Discoveries in Particle Physics (1996), 381.
It is perhaps difficult sufficiently to emphasise Seeking without disparaging its correlative Finding. But I must risk this, for Finding has a clamorous voice that proclaims its own importance; it is definite and assured, something that we can take hold of —that is what we all want, or think we want. Yet how transitory it proves.
The finding of one generation will not serve for the next. It tarnishes rapidly except it be reserved with an ever-renewed spirit of seeking.
The finding of one generation will not serve for the next. It tarnishes rapidly except it be reserved with an ever-renewed spirit of seeking.
Swarthmore Lecture (1929) at Friends’ House, London, printed in Science and the Unseen World (1929), 87-88.
It is so very difficult for a sick man not to be a scoundrel.
…...
It is the reciprocity of these appearances—that each party should think the other has contracted—that is so difficult to realise. Here is a paradox beyond even the imagination of Dean Swift. Gulliver regarded the Lilliputians as a race of dwarfs; and the Lilliputians regarded Gulliver as a giant. That is natural. If the Lilliputians had appeared dwarfs to Gulliver, and Gulliver had appeared a dwarf to the Lilliputians—but no! that is too absurd for fiction, and is an idea only to be found in the sober pages of science. …It is not only in space but in time that these strange variations occur. If we observed the aviator carefully we should infer that he was unusually slow in his movements; and events in the conveyance moving with him would be similarly retarded—as though time had forgotten to go on. His cigar lasts twice as long as one of ours. …But here again reciprocity comes in, because in the aviator’s opinion it is we who are travelling at 161,000 miles a second past him; and when he has made all allowances, he finds that it is we who are sluggish. Our cigar lasts twice as long as his.
In Space, Time and Gravitation: An Outline of the General Relativity Theory (1920, 1921), 23-24.
It is very difficult not to be excited by 10,000 king penguins.
From interview with Michael Bond, 'It’s a Wonderful Life', New Scientist (14 Dec 2002), 176, No. 2373, 48.
It is very difficult to say nowadays where the suburbs of London come to an end and where the country begins. The railways, instead of enabling Londoners to live in the country have turned the countryside into a city.
In The Three Clerks (1857, 1904), 30-31.
It is well-known that those who have charge of young infants, that it is difficult to feel sure when certain movements about their mouths are really expressive; that is when they really smile. Hence I carefully watched my own infants. One of them at the age of forty-five days, and being in a happy frame of mind, smiled... I observed the same thing on the following day: but on the third day the child was not quite well and there was no trace of a smile, and this renders it probable that the previous smiles were real.
The Expression of Emotions in Man and Animals
It may very properly be asked whether the attempt to define distinct species, of a more or less permanent nature, such as we are accustomed to deal with amongst the higher plants and animals, is not altogether illusory amongst such lowly organised forms of life as the bacteria. No biologist nowadays believes in the absolute fixity of species … but there are two circumstances which here render the problem of specificity even more difficult of solution. The bacteriologist is deprived of the test of mutual fertility or sterility, so valuable in determining specific limits amongst organisms in which sexual reproduction prevails. Further, the extreme rapidity with which generation succeeds generation amongst bacteria offers to the forces of variation and natural selection a field for their operation wholly unparalleled amongst higher forms of life.
'The Evolution of the Streptococci', The Lancet, 1906, 2, 1415-6.
It might be thought … that evolutionary arguments would play a large part in guiding biological research, but this is far from the case. It is difficult enough to study what is happening now. To figure out exactly what happened in evolution is even more difficult. Thus evolutionary achievements can be used as hints to suggest possible lines of research, but it is highly dangerous to trust them too much. It is all too easy to make mistaken inferences unless the process involved is already very well understood.
In What Mad Pursuit: A Personal View of Scientific Discovery (1988), 138-139.
It was indeed very difficult to explain the concept of a lake as a large body of fresh water which fills a whole district. In Egypt and the Sudan there is no expression for it: birket, fula, tirra etc. refer rather to pond, rain pond, marsh etc.
Their Arabic had no word for lake. In August Petermann, Petermann’s Geographische Mittheilungen (1871), 13. As quoted with implied citation, in Kathrin Fritsch, '"You Have Everything Confused And Mixed Up…!" Georg Schweinfurth, Knowledge And Cartography Of Africa In The 19th Century', History in Africa (2009), 36, 94. Fritsch comments on their misunderstandings caused by “different spatial conceptions [and] language barriers.”
It would be difficult and perhaps foolhardy to analyze the chances of further progress in almost every part of mathematics one is stopped by unsurmountable difficulties, improvements in the details seem to be the only possibilities which are left… All these difficulties seem to announce that the power of our analysis is almost exhausted, even as the power of ordinary algebra with regard to transcendental geometry in the time of Leibniz and Newton, and that there is a need of combinations opening a new field to the calculation of transcendental quantities and to the solution of the equations including them.
From Rapport historique sur les progrès des sciences mathématiques depuis 1789, et sur leur état actuel (1810), 131. As translated in George Sarton, The Study of the History of Mathematics (1936), 13. In the original French: “Il seroit difficile et peut-être téméraire d’analyser les chances que l’avenir offre à l’avancement des mathématiques: dans presque toutes les parties, on est arrêté par des difficultés insurmontables; des perfectionnements de détail semblent la seule chose qui reste à faire… Toutes ces difficultés semblent annoncer que la puissance de notre analyse est à-peu-près épuisée, comme celle de l’algèbre ordinaire l’étoit par rapport à la géométrie transcendante au temps de Leibnitz et de Newton, et qu’il faut des combinaisons qui ouvrent un nouveau champ au calcul des transcendantes et à la résolution des équations qui les contiennent.” Sarton states this comes from “the report on mathematical progress prepared for the French Academy of Sciences at Napoleon’s request”.
It would be difficult to name a man more remarkable for the greatness and the universality of his intellectual powers than Leibnitz.
In System of Logic, Bk. 2, chap. 5, sect. 6.
It would not be difficult to come to an agreement as to what we understand by science. Science is the century-old endeavor to bring together by means of systematic thought the perceptible phenomena of this world into as thoroughgoing an association as possible. To put it boldly, it is the attempt at the posterior reconstruction of existence by the process of conceptualization. But when asking myself what religion is I cannot think of the answer so easily. And even after finding an answer which may satisfy me at this particular moment, I still remain convinced that I can never under any circumstances bring together, even to a slight extent, the thoughts of all those who have given this question serious consideration.
…...
It would seem at first sight as if the rapid expansion of the region of mathematics must be a source of danger to its future progress. Not only does the area widen but the subjects of study increase rapidly in number, and the work of the mathematician tends to become more and more specialized. It is, of course, merely a brilliant exaggeration to say that no mathematician is able to understand the work of any other mathematician, but it is certainly true that it is daily becoming more and more difficult for a mathematician to keep himself acquainted, even in a general way, with the progress of any of the branches of mathematics except those which form the field of his own labours. I believe, however, that the increasing extent of the territory of mathematics will always be counteracted by increased facilities in the means of communication. Additional knowledge opens to us new principles and methods which may conduct us with the greatest ease to results which previously were most difficult of access; and improvements in notation may exercise the most powerful effects both in the simplification and accessibility of a subject. It rests with the worker in mathematics not only to explore new truths, but to devise the language by which they may be discovered and expressed; and the genius of a great mathematician displays itself no less in the notation he invents for deciphering his subject than in the results attained. … I have great faith in the power of well-chosen notation to simplify complicated theories and to bring remote ones near and I think it is safe to predict that the increased knowledge of principles and the resulting improvements in the symbolic language of mathematics will always enable us to grapple satisfactorily with the difficulties arising from the mere extent of the subject.
In Presidential Address British Association for the Advancement of Science, Section A., (1890), Nature, 42, 466.
It’s a common occurrence in a forefront area of science, where the questions are tough and the measurements extremely difficult. You have different groups using different methods and they get different answers. You see it all the time, and the public rarely notices. But when it happens to be in cosmology, it makes headlines.
As quoted in John Moble Wilford, 'Astronomers Debate Conflicting Answers for the Age of the Universe', New York Times (27 Dec 1994), C9.
It's hard to imagine anything more difficult to study than human sexuality, on every level from the technical to the political. One has only to picture monitoring orgasm in the lab to begin to grasp the challenge of developing testing techniques that are thorough and precise, yet respectful.
If you know an original print source for this quote, please contact webmaster.
Knowledge and wisdom are indeed not identical; and every man’s experience must have taught him that there may be much knowledge with little wisdom, and much wisdom with little knowledge. But with imperfect knowledge it is difficult or impossible to arrive at right conclusions. Many of the vices, many of the miseries, many of the follies and absurdities by which human society has been infested and disgraced may be traced to a want of knowledge.
Presidential Address to Anniversary meeting of the Royal Society (30 Nov 1859), Proceedings of the Royal Society of London (1860), 10, 163.
Life is short, and the Art long; the occasion fleeting; experience fallacious, and judgment difficult. The physician must not only be prepared to do what is right himself, but also to make the patient, the attendants, and externals cooperate.
The Genuine Works of Hippocrates, trans. Francis Adams (1886), Vol. 2, 192.
Life is short, the Art long, opportunity fleeting, experience treacherous, judgment difficult. The physician must be ready, not only to do his duty himself, but also to secure the co-operation of the patient, of the attendants and of externals.
Aphorisms, in Hippocrates, trans. W. H. S. Jones (1931), Vol. 4, 99.
Like almost every subject of human interest, this one [mathematics] is just as easy or as difficult as we choose to make it. A lifetime may be spent by a philosopher in discussing the truth of the simplest axiom. The simplest fact as to our existence may fill us with such wonder that our minds will remain overwhelmed with wonder all the time. A Scotch ploughman makes a working religion out of a system which appalls a mental philosopher. Some boys of ten years of age study the methods of the differential calculus; other much cleverer boys working at mathematics to the age of nineteen have a difficulty in comprehending the fundamental ideas of the calculus.
In Teaching of Mathematics (1902), 19-20.
Man is a rational animal—so at least I have been told. … Aristotle, so far as I know, was the first man to proclaim explicitly that man is a rational animal. His reason for this view was … that some people can do sums. … It is in virtue of the intellect that man is a rational animal. The intellect is shown in various ways, but most emphatically by mastery of arithmetic. The Greek system of numerals was very bad, so that the multiplication table was quite difficult, and complicated calculations could only be made by very clever people.
From An Outline of Intellectual Rubbish (1937, 1943), 5. Collected in The Basic Writings of Bertrand Russell (2009), 45.
Mathematical analysis is as extensive as nature itself; it defines all perceptible relations, measures times, spaces, forces, temperatures; this difficult science is formed slowly, but it preserves every principle which it has once acquired; it grows and strengthens itself incessantly in the midst of the many variations and errors of the human mind.
From Théorie Analytique de la Chaleur (1822), Discours Préliminaire, xiv, (Theory of Heat, Introduction), as translated by Alexander Freeman in The Analytical Theory of Heat (1878), 7. From the original French, “L’analyse mathématique est aussi étendue que la nature elle-même; elle définit tous les rapports sensibles, mesure les temps y les espaces, les forces, les températures; cette science difficile se forme avec lenteur, mais elle conserve tous les principes quelle a une fois acquis; elle s’accroît et s’affermit sans cesse au milieu de tant de variations et d’erreurs de l’esprit humain.”
Mathematics is like checkers in being suitable for the young, not too difficult, amusing, and without peril to the state.
— Plato
As given in Scripta Mathematica: A Quarterly Journal Devoted to the Philosophy, History, and Expository Treatment of Mathematics (1950), 17, 185.
Mathematics is often considered a difficult and mysterious science, because of the numerous symbols which it employs. Of course, nothing is more incomprehensible than a symbolism which we do not understand. … But this is not because they are difficult in themselves. On the contrary they have invariably been introduced to make things easy. … [T]he symbolism is invariably an immense simplification. It … represents an analysis of the ideas of the subject and an almost pictorial representation of their relations to each other.
In Introduction to Mathematics (1911), 59-60.
Models so constructed, though of no practical value, serve a useful academic function. The oldest problem in economic education is how to exclude the incompetent. The requirement that there be an ability to master difficult models, including ones for which mathematical competence is required, is a highly useful screening device.
In Economics, Peace, and Laughter (1981), 40-41.
Most impediments to scientific understanding are conceptual locks, not factual lacks. Most difficult to dislodge are those biases that escape our scrutiny because they seem so obviously, even ineluctably, just. We know ourselves best and tend to view other creatures as mirrors of our own constitution and social arrangements. (Aristotle, and nearly two millennia of successors, designated the large bee that leads the swarm as a king.)
…...
My dear friend, to be both powerful and fair has always been difficult for mankind. Power and justice have always been seen like day and night; this being the case, when one of them is there the other disappears.
From the play Galileo Galilei (2001) .
My mother, my dad and I left Cuba when I was two [January, 1959]. Castro had taken control by then, and life for many ordinary people had become very difficult. My dad had worked [as a personal bodyguard for the wife of Cuban president Batista], so he was a marked man. We moved to Miami, which is about as close to Cuba as you can get without being there. It’s a Cuba-centric society. I think a lot of Cubans moved to the US thinking everything would be perfect. Personally, I have to say that those early years were not particularly happy. A lot of people didn’t want us around, and I can remember seeing signs that said: “No children. No pets. No Cubans.” Things were not made easier by the fact that Dad had begun working for the US government. At the time he couldn’t really tell us what he was doing, because it was some sort of top-secret operation. He just said he wanted to fight against what was happening back at home. [Estefan’s father was one of the many Cuban exiles taking part in the ill-fated, anti-Castro Bay of Pigs invasion to overthrow dictator Fidel Castro.] One night, Dad disappeared. I think he was so worried about telling my mother he was going that he just left her a note. There were rumors something was happening back home, but we didn’t really know where Dad had gone. It was a scary time for many Cubans. A lot of men were involved—lots of families were left without sons and fathers. By the time we found out what my dad had been doing, the attempted coup had taken place, on April 17, 1961. Initially he’d been training in Central America, but after the coup attempt he was captured and spent the next two years as a political prisoner in Cuba. That was probably the worst time for my mother and me. Not knowing what was going to happen to Dad. I was only a kid, but I had worked out where my dad was. My mother was trying to keep it a secret, so she used to tell me Dad was on a farm. Of course, I thought that she didn’t know what had really happened to him, so I used to keep up the pretense that Dad really was working on a farm. We used to do this whole pretending thing every day, trying to protect each other. Those two years had a terrible effect on my mother. She was very nervous, just going from church to church. Always carrying her rosary beads, praying her little heart out. She had her religion, and I had my music. Music was in our family. My mother was a singer, and on my father’s side there was a violinist and a pianist. My grandmother was a poet.
…...
Nature, displayed in its full extent, presents us with an immense tableau, in which all the order of beings are each represented by a chain which sustains a continuous series of objects, so close and so similar that their difference would be difficult to define. This chain is not a simple thread which is only extended in length, it is a large web or rather a network, which, from interval to interval, casts branches to the side in order to unite with the networks of another order.
'Les Oiseaux Qui Ne Peuvent Voler', Histoire Naturelle des Oiseaux (1770), Vol. I, 394. Trans. Phillip R. Sloan.
Nevertheless, it is necessary to remember that a planned economy is not yet socialism. A planned economy as such may be accompanied by the complete enslavement of the individual. The achievement of socialism requires the solution of some extremely difficult socio-political problems: how is it possible, in view of the far-reaching centralisation of political and economic power, to prevent bureaucracy from becoming all-powerful and overweening? How can the rights of the individual be protected and therewith a democratic counterweight to the power of bureaucracy be assured?
…...
No question is so difficult as that to which the answer is obvious.
Saying.
No question is so difficult to answer as that which the answer is obvious.
…...
Nor can it be supposed that the diversity of chemical structure and process stops at the boundary of the species, and that within that boundary, which has no real finality, rigid uniformity reigns. Such a conception is at variance with any evolutionary conception of the nature and origin of species. The existence of chemical individuality follows of necessity from that of chemical specificity, but we should expect the differences between individuals to be still more subtle and difficult of detection. Indications of their existence are seen, even in man, in the various tints of skin, hair, and eyes, and in the quantitative differences in those portions of the end-products of metabolism which are endogenous and are not affected by diet, such as recent researches have revealed in increasing numbers. Even those idiosyncrasies with regard to drugs and articles of food which are summed up in the proverbial saying that what is one man's meat is another man's poison presumably have a chemical basis.
Inborn Errors of Metabolism: The Croonian Lectures delivered before the Royal College of Physicians of London, in June, 1908 (1909), 2-3.
Nor do I know any study which can compete with mathematics in general in furnishing matter for severe and continued thought. Metaphysical problems may be even more difficult; but then they are far less definite, and, as they rarely lead to any precise conclusion, we miss the power of checking our own operations, and of discovering whether we are thinking and reasoning or merely fancying and dreaming.
In Conflict of Studies (1873), 13.
Observation is simple, indefatigable, industrious, upright, without any preconceived opinion. Experiment is artificial, impatient, busy, digressive; passionate, unreliable. We see every day one experiment after another, the second outweighing the impression gained from the first, both, often enough, carried out by men who are neither much distinguished for their spirit, nor for carrying with them the truth of personality and self denial. Nothing is easier than to make a series of so-called interesting experiments. Nature can only in some way be forced, and in her distress, she will give her suffering answer. Nothing is more difficult than to explain it, nothing is more difficult than a valid physiological experiment. We consider as the first task of current physiology to point at it and comprehend it.
Inaugural lecture as docent of physiology at the University of Bonn (19 Oct) 1824. Published in Johannes Muller, Zur vergleichenden Physiologie des Gesichtssinnes des Menschen und der Thiere (1826), 20.
Of the nucleosides from deoxyribonucleic acids, all that was known with any certainty [in the 1940s] was that they were 2-deoxy-D-ribosides of the bases adenine, guanine, thymine and cytosine and it was assumed that they were structurally analogous to the ribonucleosides. The chemistry of the nucleotides—the phosphates of the nucleosides—was in a correspondingly primitive state. It may well be asked why the chemistry of these groups of compounds was not further advanced, particularly since we recognize today that they occupy a central place in the history of the living cell. True, their full significance was for a long time unrecognized and emerged only slowly as biochemical research got into its stride but I think a more important reason is to be found in the physical properties of compounds of the nucleotide group. As water-soluble polar compounds with no proper melting points they were extremely difficult to handle by the classic techniques of organic chemistry, and were accordingly very discouraging substances to early workers. It is surely no accident that the major advances in the field have coincided with the appearance of new experimental techniques such as paper and ion-exchange chromatography, paper electrophoresis, and countercurrent distribution, peculiarly appropriate to the compounds of this group.
In 'Synthesis in the Study of Nucleotides', Nobel Lecture, 11 December 1957. In Nobel Lectures: Chemistry 1942-1962 (1964), 524.
Ohm found that the results could be summed up in such a simple law that he who runs may read it, and a schoolboy now can predict what a Faraday then could only guess at roughly. By Ohm's discovery a large part of the domain of electricity became annexed by Coulomb's discovery of the law of inverse squares, and completely annexed by Green's investigations. Poisson attacked the difficult problem of induced magnetisation, and his results, though differently expressed, are still the theory, as a most important first approximation. Ampere brought a multitude of phenomena into theory by his investigations of the mechanical forces between conductors supporting currents and magnets. Then there were the remarkable researches of Faraday, the prince of experimentalists, on electrostatics and electrodynamics and the induction of currents. These were rather long in being brought from the crude experimental state to a compact system, expressing the real essence. Unfortunately, in my opinion, Faraday was not a mathematician. It can scarely be doubted that had he been one, he would have anticipated much later work. He would, for instance, knowing Ampere's theory, by his own results have readily been led to Neumann’s theory, and the connected work of Helmholtz and Thomson. But it is perhaps too much to expect a man to be both the prince of experimentalists and a competent mathematician.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
On the whole, I cannot help saying that it appears to me not a little extraordinary, that a theory so new, and of such importance, overturning every thing that was thought to be the best established in chemistry, should rest on so very narrow and precarious a foundation, the experiments adduced in support of it being not only ambiguous or explicable on either hypothesis, but exceedingly few. I think I have recited them all, and that on which the greatest stress is laid, viz. That of the formation of water from the decomposition of the two kinds of air, has not been sufficiently repeated. Indeed it required so difficult and expensive an apparatus, and so many precautions in the use of it, that the frequent repetition of the experiment cannot be expected; and in these circumstances the practised experimenter cannot help suspecting the accuracy of the result and consequently the certainty of the conclusion.
Considerations on the Doctrine of Phlogiston (1796), 57-8.
One can ask: “If I crystallize a virus to obtain a crystal consisting of the molecules that make up the virus, are those molecules lifeless or not?” … The properties of living organisms are those of aggregates of molecules. It’s very difficult to draw a line between molecules that are lifeless and molecules that are not lifeless.
From interview with Neil A. Campbell, in 'Crossing the Boundaries of Science', BioScience (Dec 1986), 36, No. 11, 738.
One feature which will probably most impress the mathematician accustomed to the rapidity and directness secured by the generality of modern methods is the deliberation with which Archimedes approaches the solution of any one of his main problems. Yet this very characteristic, with its incidental effects, is calculated to excite the more admiration because the method suggests the tactics of some great strategist who foresees everything, eliminates everything not immediately conducive to the execution of his plan, masters every position in its order, and then suddenly (when the very elaboration of the scheme has almost obscured, in the mind of the spectator, its ultimate object) strikes the final blow. Thus we read in Archimedes proposition after proposition the bearing of which is not immediately obvious but which we find infallibly used later on; and we are led by such easy stages that the difficulties of the original problem, as presented at the outset, are scarcely appreciated. As Plutarch says: “It is not possible to find in geometry more difficult and troublesome questions, or more simple and lucid explanations.” But it is decidedly a rhetorical exaggeration when Plutarch goes on to say that we are deceived by the easiness of the successive steps into the belief that anyone could have discovered them for himself. On the contrary, the studied simplicity and the perfect finish of the treatises involve at the same time an element of mystery. Though each step depends on the preceding ones, we are left in the dark as to how they were suggested to Archimedes. There is, in fact, much truth in a remark by Wallis to the effect that he seems “as it were of set purpose to have covered up the traces of his investigation as if he had grudged posterity the secret of his method of inquiry while he wished to extort from them assent to his results.” Wallis adds with equal reason that not only Archimedes but nearly all the ancients so hid away from posterity their method of Analysis (though it is certain that they had one) that more modern mathematicians found it easier to invent a new Analysis than to seek out the old.
In The Works of Archimedes (1897), Preface, vi.
One of my surgical giant friends had in his operating room a sign “If the operation is difficult, you aren’t doing it right.” What he meant was, you have to plan every operation You cannot ever be casual You have to realize that any operation is a potential fatality.
From Cornelia Dean, 'A Conversation with Joseph E. Murray', New York Times (25 Sep 2001), F5.
One of the most curious and interesting reptiles which I met with in Borneo was a large tree-frog, which was brought me by one of the Chinese workmen. He assured me that he had seen it come down in a slanting direction from a high tree, as if it flew. On examining it, I found the toes very long and fully webbed to their very extremity, so that when expanded they offered a surface much larger than the body. The forelegs were also bordered by a membrane, and the body was capable of considerable inflation. The back and limbs were of a very deep shining green colour, the undersurface and the inner toes yellow, while the webs were black, rayed with yellow. The body was about four inches long, while the webs of each hind foot, when fully expanded, covered a surface of four square inches, and the webs of all the feet together about twelve square inches. As the extremities of the toes have dilated discs for adhesion, showing the creature to be a true tree frog, it is difficult to imagine that this immense membrane of the toes can be for the purpose of swimming only, and the account of the Chinaman, that it flew down from the tree, becomes more credible. This is, I believe, the first instance known of a “flying frog,” and it is very interesting to Darwinians as showing that the variability of the toes which have been already modified for purposes of swimming and adhesive climbing, have been taken advantage of to enable an allied species to pass through the air like the flying lizard. It would appear to be a new species of the genus Rhacophorus, which consists of several frogs of a much smaller size than this, and having the webs of the toes less developed.
Malay Archipelago
One precept for the scientist-to-be is already obvious. Do not place yourself in an environment where your advisor is already suffering from scientific obsolescence. If one is so unfortunate as to receive his training under a person who is either technically or intellectually obsolescent, one finds himself to be a loser before he starts. It is difficult to move into a position of leadership if one’s launching platform is a scientific generation whose time is already past.
In 'Scientific innovation and creativity: a zoologist’s point of view', American Zoologist (1982), 22, 229.
Palaeontology is the Aladdin’s lamp of the most deserted and lifeless regions of the earth; it touches the rocks and there spring forth in orderly succession the monarchs of the past and the ancient river streams and savannahs wherein they flourished. The rocks usually hide their story in the most difficult and inaccessible places.
In On the Trail of Ancient Man (1926), x.
Paris ... On this side of the ocean it is difficult to understand the susceptibility of American citizens on the subject and precisely why they should so stubbornly cling to the biblical version. It is said in Genesis the first man came from mud and mud is not anything very clean. In any case if the Darwinian hypothesis should irritate any one it should only be the monkey. The monkey is an innocent animal—a vegetarian by birth. He never placed God on a cross, knows nothing of the art of war, does not practice lynch law and never dreams of assassinating his fellow beings. The day when science definitely recognizes him as the father of the human race the monkey will have no occasion to be proud of his descendants. That is why it must be concluded that the American Association which is prosecuting the teacher of evolution can be no other than the Society for Prevention of Cruelty to Animals.
[A cynical article in the French press on the Scopes Monkey Trial, whether it will decide “a monkey or Adam was the grandfather of Uncle Sam.”]
[A cynical article in the French press on the Scopes Monkey Trial, whether it will decide “a monkey or Adam was the grandfather of Uncle Sam.”]
Article from a French daily newspaper on the day hearings at the Scopes Monkey Trial began, Paris Soir (13 Jul 1925), quoted in 'French Satirize the Case', New York Times (14 Jul 1925), 3.
People will work every bit as hard to fool themselves as they will to fool others—which makes it very difficult to tell just where the line between foolishness and fraud is located.
Voodoo Science. In Marc J. Madou, Fundamentals of Microfabrication: the Science of Miniaturization (2nd ed., 2002), 77.
Physicians are rather like undescended testicles, they are difficult to locate and when they are found, they are pretty ineffective.
Susi Greenwood, Book of Humorous Medical Anecdotes (1989), 47.
Physics is becoming too difficult for the physicists.
As quoted in Constance Reid, Hilbert (1970), 127.
Precedents are treated by powerful minds as fetters with which to bind down the weak, as reasons with which to mistify the moderately informed, and as reeds which they themselves fearlessly break through whenever new combinations and difficult emergencies demand their highest efforts.
A Word to the Wise (1833), 3-6. Quoted in Anthony Hyman (ed.), Science and Reform: Selected Works of Charles Babbage (1989), 202.
Predictions can be very difficult—especially about the future.
Quoted in H. Rosovsky, The University: An Owners Manual (1991), 147. It is said that Bohr used to quote this saying to illustrate the differences between Danish and Swedish humour. Bohr always attributed the saying to Robert Storm Petersen (1882-1949), a well-known Danish artist and writer. However, the saying did NOT originate from Petersen. It may have been said in the Danish Parliament between 1935 and 1939 [Information supplied courtesy of Professor Erik Rüdinger, Niels Bohr Archive, Copenhagen].
Professor [Max] Planck, of Berlin, the famous originator of the Quantum Theory, once remarked to me that in early life he had thought of studying economics, but had found it too difficult! Professor Planck could easily master the whole corpus of mathematical economics in a few days. He did not mean that! But the amalgam of logic and intuition and the wide knowledge of facts, most of which are not precise, which is required for economic interpretation in its highest form is, quite truly, overwhelmingly difficult for those whose gift mainly consists in the power to imagine and pursue to their furthest points the implications and prior conditions of comparatively simple facts which are known with a high degree of precision.
'Alfred Marshall: 1842-1924' (1924). In Geoffrey Keynes (ed.), Essays in Biography (1933), 191-2
Programming is one of the most difficult branches of applied mathematics; the poorer mathematicians had better remain pure mathematicians.
…...
Quite distinct from the theoretical question of the manner in which mathematics will rescue itself from the perils to which it is exposed by its own prolific nature is the practical problem of finding means of rendering available for the student the results which have been already accumulated, and making it possible for the learner to obtain some idea of the present state of the various departments of mathematics. … The great mass of mathematical literature will be always contained in Journals and Transactions, but there is no reason why it should not be rendered far more useful and accessible than at present by means of treatises or higher text-books. The whole science suffers from want of avenues of approach, and many beautiful branches of mathematics are regarded as difficult and technical merely because they are not easily accessible. … I feel very strongly that any introduction to a new subject written by a competent person confers a real benefit on the whole science. The number of excellent text-books of an elementary kind that are published in this country makes it all the more to be regretted that we have so few that are intended for the advanced student. As an example of the higher kind of text-book, the want of which is so badly felt in many subjects, I may mention the second part of Prof. Chrystal’s Algebra published last year, which in a small compass gives a great mass of valuable and fundamental knowledge that has hitherto been beyond the reach of an ordinary student, though in reality lying so close at hand. I may add that in any treatise or higher text-book it is always desirable that references to the original memoirs should be given, and, if possible, short historic notices also. I am sure that no subject loses more than mathematics by any attempt to dissociate it from its history.
In Presidential Address British Association for the Advancement of Science, Section A (1890), Nature, 42, 466.
Recollections [his autobiographical work] might possibly interest my children or their children. I know that it would have interested me greatly to have read even so short and dull a sketch of the mind of my grandfather, written by himself, and what he thought and did, and how he worked. I have attempted to write the following account of myself as if I were a dead man in another world looking back at my own life. Nor have I found this difficult, for life is nearly over with me.
Research has deserted the individual and entered the group. The individual worker find the problem too large, not too difficult. He must learn to work with others.
Letter to Dr. E. B. Krumhaar (11 Oct 1933), in Journal of Bacteriology (Jan 1934), 27, No. 1, 20.
Revere those things beyond science which really matter and about which it is so difficult to speak.
…...
She [Rosalind Franklin] discovered in a series of beautifully executed researches the fundamental distinction between carbons that turned on heating into graphite and those that did not. Further she related this difference to the chemical constitution of the molecules from which carbon was made. She was already a recognized authority in industrial physico-chemistry when she chose to abandon this work in favour of the far more difficult and more exciting fields of biophysics.
Comment in The Times, 19 Apr 1958, shortly after Franklin's death. In Jenifer Glynn, 'Rosalind Franklin', in E. Shils and C. Blacker (eds.), Cambridge Women: Twelve Portraits (1996), 206.
She knew only that if she did or said thus-and-so, men would unerringly respond with the complimentary thus-and-so. It was like a mathematical formula and no more difficult, for mathematics was the one subject that had come easy to Scarlett in her schooldays.
In Gone With the Wind (1936), 60.
So much goes into doing a transplant operation. All the way from preparing the patient, to procuring the donor. It's like being an astronaut. The astronaut gets all the credit, he gets the trip to the moon, but he had nothing to do with the creation of the rocket, or navigating the ship. He's the privileged one who gets to drive to the moon. I feel that way in some of these more difficult operations, like the heart transplant.
Some one once asked Rutherford how it was that he always managed to keep on the crest of the wave. “Well” said Rutherford “that isn’t difficult. I made the wave, why shouldn’t I be at the top of it.” I hasten to say that my own subject is a very minor ripple compared to Rutherford’s.
From Speech (10 Dec 1963) at the Nobel Banquet in Stockholm, Sweden. Collected inGöran Liljestrand (ed.), Les Prix Nobel en 1963, (1964).
Sometimes one has got to say difficult things, but one ought to say them as simply as one knows how.
In A Mathematician's Apology (1940, 2012), 47.
Suddenly there was an enormous explosion, like a violent volcano. The nuclear reactions had led to overheating in the underground burial grounds. The explosion poured radioactive dust and materials high up into the sky. It was just the wrong weather for such a tragedy. Strong winds blew the radioactive clouds hundreds of miles away. It was difficult to gauge the extent of the disaster immediately, and no evacuation plan was put into operation right away. Many villages and towns were only ordered to evacuate when the symptoms of radiation sickness were already quite apparent. Tens of thousands of people were affected, hundreds dying, though the real figures have never been made public. The large area, where the accident happened, is still considered dangerous and is closed to the public.
'Two Decades of Dissidence', New Scientist (4 Nov 1976), 72, No. 72, 265.
Suppose it were perfectly certain that the life and fortune of every one of us would, one day or other, depend upon his winning or losing a game of chess. Don't you think that we should all consider it to be a primary duty to learn at least the names and the moves of the pieces; to have a notion of a gambit, and a keen eye for all the means of giving and getting out of check? Do you not think that we should look with a disapprobation amounting to scorn upon the father who allowed his son, or the state which allowed its members, to grow up without knowing a pawn from a knight?
Yet, it is a very plain and elementary truth that the life, the fortune, and the happiness of every one of us, and, more or less, of those who are connected with us, do depend upon our knowing something of the rules of a game infinitely more difficult and complicated than chess. It is a game which has been played for untold ages, every man and woman of us being one of the two players in a game of his or her own. The chess-board is the world, the pieces are the phenomena of the universe, the rules of the game are what we call the laws of nature. The player on the other side is hidden from us. We know that his play is always fair, just, and patient. But also we know, to our cost, that he never overlooks a mistake, or makes the smallest allowance for ignorance. To the man who plays well the highest stakes are paid with that sort of overflowing generosity with which the strong shows delight in strength. And one who plays ill is checkmated—without haste, but without remorse.
Yet, it is a very plain and elementary truth that the life, the fortune, and the happiness of every one of us, and, more or less, of those who are connected with us, do depend upon our knowing something of the rules of a game infinitely more difficult and complicated than chess. It is a game which has been played for untold ages, every man and woman of us being one of the two players in a game of his or her own. The chess-board is the world, the pieces are the phenomena of the universe, the rules of the game are what we call the laws of nature. The player on the other side is hidden from us. We know that his play is always fair, just, and patient. But also we know, to our cost, that he never overlooks a mistake, or makes the smallest allowance for ignorance. To the man who plays well the highest stakes are paid with that sort of overflowing generosity with which the strong shows delight in strength. And one who plays ill is checkmated—without haste, but without remorse.
Address to the South London Working Men’s College. 'A Liberal Education; and Where to Find It', in David Masson, (ed.), Macmillan’s Magazine (Mar 1868), 17, 369. Also in 'A Liberal Education and Where to Find it' (1868). In Collected Essays (1893), Vol. 3, 82.
Suppose then I want to give myself a little training in the art of reasoning; suppose I want to get out of the region of conjecture and probability, free myself from the difficult task of weighing evidence, and putting instances together to arrive at general propositions, and simply desire to know how to deal with my general propositions when I get them, and how to deduce right inferences from them; it is clear that I shall obtain this sort of discipline best in those departments of thought in which the first principles are unquestionably true. For in all our thinking, if we come to erroneous conclusions, we come to them either by accepting false premises to start with—in which case our reasoning, however good, will not save us from error; or by reasoning badly, in which case the data we start from may be perfectly sound, and yet our conclusions may be false. But in the mathematical or pure sciences,—geometry, arithmetic, algebra, trigonometry, the calculus of variations or of curves,— we know at least that there is not, and cannot be, error in our first principles, and we may therefore fasten our whole attention upon the processes. As mere exercises in logic, therefore, these sciences, based as they all are on primary truths relating to space and number, have always been supposed to furnish the most exact discipline. When Plato wrote over the portal of his school. “Let no one ignorant of geometry enter here,” he did not mean that questions relating to lines and surfaces would be discussed by his disciples. On the contrary, the topics to which he directed their attention were some of the deepest problems,— social, political, moral,—on which the mind could exercise itself. Plato and his followers tried to think out together conclusions respecting the being, the duty, and the destiny of man, and the relation in which he stood to the gods and to the unseen world. What had geometry to do with these things? Simply this: That a man whose mind has not undergone a rigorous training in systematic thinking, and in the art of drawing legitimate inferences from premises, was unfitted to enter on the discussion of these high topics; and that the sort of logical discipline which he needed was most likely to be obtained from geometry—the only mathematical science which in Plato’s time had been formulated and reduced to a system. And we in this country [England] have long acted on the same principle. Our future lawyers, clergy, and statesmen are expected at the University to learn a good deal about curves, and angles, and numbers and proportions; not because these subjects have the smallest relation to the needs of their lives, but because in the very act of learning them they are likely to acquire that habit of steadfast and accurate thinking, which is indispensable to success in all the pursuits of life.
In Lectures on Teaching (1906), 891-92.
Surprisingly, history is much more difficult than chemistry.
Louis Hammett Symposium (31 Aug 1983), Advances in Physical Organic Chemistry (1985), 21, 2.
Symbolism is useful because it makes things difficult. Now in the beginning everything is self-evident, and it is hard to see whether one self-evident proposition follows from another or not. Obviousness is always the enemy to correctness. Hence we must invent a new and difficult symbolism in which nothing is obvious. … Thus the whole of Arithmetic and Algebra has been shown to require three indefinable notions and five indemonstrable propositions.
In International Monthly (1901), 4, 85-86.
Talent, in difficult situations, strives to untie knots, which genius instantly cuts with one swift decision.
In 'Genius', Wellman’s Miscellany (Dec 1871), 4, No. 6, 203.
Thanks to the sharp eyes of a Minnesota man, it is possible that two identical snowflakes may finally have been observed. While out snowmobiling, Oley Skotchgaard noticed a snowflake that looked familiar to him. Searching his memory, he realized it was identical to a snowflake he had seen as a child in Vermont. Weather experts, while excited, caution that the match-up will be difficult to verify.
In Napalm and Silly Putty (2002), 105.
That the Anatomy of the Nerves yields more pleasant and profitable Speculations, than the Theory of any parts besides in the animated Body: for from hence the true and genuine Reasons are drawn of very many Actions and Passions that are wont to happen in our Body, which otherwise seem most difficult and unexplicable; and no less from this Fountain the hidden Causes of Diseases and their Symptoms, which commonly are ascribed to the Incantations of Witches, may be found out and clearly laid open. But as to our observations about the Nerves, from our following Discourse it will plainly appear, that I have not trod the paths or footsteps of others, nor repeated what hath been before told.
In Anatomy of the Brain and Nerves (1664), trans. Samuel Pordage (1681), reprinted in William Peindel (ed.), Thomas Willis: Anatomy of the Brain and Nerves (1965), Vol. 2, 125.
That the master manufacturer, by dividing the work to be executed into different processes, each requiring different degrees of skill or of force, can purchase precisely the precise quantity of both which is necessary for each process; whereas, if the whole work were executed by one workman, that person must possess sufficient skill to perform the most difficult, and sufficient strength to execute the most laborious, of the operations into which the art is divided.
In 'On the Division of Labour', Economy of Machinery and Manufactures (1st ed., 1832), chap. 18, 127.
The advances of biology during the past 20 years have been breathtaking, particularly in cracking the mystery of heredity. Nevertheless, the greatest and most difficult problems still lie ahead. The discoveries of the 1970‘s about the chemical roots of memory in nerve cells or the basis of learning, about the complex behavior of man and animals, the nature of growth, development, disease and aging will be at least as fundamental and spectacular as those of the recent past.
As quoted in 'H. Bentley Glass', New York Times (12 Jan 1970), 96.
The art of drawing conclusions from experiments and observations consists in evaluating probabilities and in estimating whether they are sufficiently great or numerous enough to constitute proofs. This kind of calculation is more complicated and more difficult than it is commonly thought to be. … It is above all in medicine that the difficulty of evaluating the probabilities is greater.
Translated by I.M.L. Donaldson, from an undated document collected in Mémoires de Lavoisier, Oeuvres (1865), Tome III, 509. From the original French, “L’art de conclure d’après des expériences et des observations consiste à évaluer des probabilités, et à estimer si elles sont assez grandes ou assez multipliées pour constituer des preuves. Ce genre de calcul est plus compliqué et plus difficile qu’on ne pense. … C’est surtout en médecine que la difficulté d’évaluer les probabilités est plus grande.”
The art of research [is] the art of making difficult problems soluble by devising means of getting at them.
Pluto's Republic (1982), 2.
The average English author [of mathematical texts] leaves one under the impression that he has made a bargain with his reader to put before him the truth, the greater part of the truth, and nothing but the truth; and that if he has put the facts of his subject into his book, however difficult it may be to unearth them, he has fulfilled his contract with his reader. This is a very much mistaken view, because effective teaching requires a great deal more than a bare recitation of facts, even if these are duly set forth in logical order—as in English books they often are not. The probable difficulties which will occur to the student, the objections which the intelligent student will naturally and necessarily raise to some statement of fact or theory—these things our authors seldom or never notice, and yet a recognition and anticipation of them by the author would be often of priceless value to the student. Again, a touch of humour (strange as the contention may seem) in mathematical works is not only possible with perfect propriety, but very helpful; and I could give instances of this even from the pure mathematics of Salmon and the physics of Clerk Maxwell.
In Perry, Teaching of Mathematics (1902), 59-61.
The ball of rumor and criticism, once it starts rolling, is difficult to stop.
In letter to mother, as quoted in Beverly Gherman, Anne Morrow Lindbergh: Between the Sea and the Stars (2007), 104.
The calculus was the first achievement of modern mathematics and it is difficult to overestimate its importance. I think it defines more unequivocally than anything else the inception of modern mathematics; and the system of mathematical analysis, which is its logical development, still constitutes the greatest technical advance in exact thinking.
In 'The Mathematician', Works of the Mind (1947), 1, No. 1. Collected in James Roy Newman (ed.), The World of Mathematics (1956), Vol. 4, 2055.
The custom of eating the lover after consummation of the nuptials, of making a meal of the exhausted pigmy, who is henceforth good for nothing, is not so difficult to understand, since insects can hardly be accused of sentimentality; but to devour him during the act surpasses anything the most morbid mind could imagine. I have seen the thing with my own eyes, and I have not yet recovered from my surprise.
In Jean-Henri Fabre and B. Miall (trans.), Social Life in the Insect World (1912), 84.
The discovery of the famous original [Rosetta Stone] enabled Napoleon’s experts to begin the reading of Egypt’s ancient literature. In like manner the seismologists, using the difficult but manageable Greek of modern physics, are beginning the task of making earthquakes tell the nature of the earth’s interior and translating into significant speech the hieroglyphics written by the seismograph.
The easiest thing to be in the world is you. The most difficult thing to be is what other people want you to be. Don’t let them put you in that position.
…...
The geneticist to-day is in a rather difficult position. He must have at least a bowing acquaintance with anatomy, cytology, and mathematics. He must dabble in taxonomy, physics, and even psychology.
In 'The Biochemistry of the Individual' (1937), collected in Neurath Hans (ed.), Perspectives in Biochemistry (1989), 6.
The great beauty of Darwin’s theory of evolution is that it explains how complex, difficult to understand things could have arisen step by plausible step, from simple, easy to understand beginnings. We start our explanation from almost infinitely simple beginnings: pure hydrogen and a huge amount of energy. Our scientific, Darwinian explanations carry us through a series of well-understood gradual steps to all the spectacular beauty and complexity of life.
From speech at the Edinburgh International Science Festival (15 Apr 1992), published in the Independent newspaper. Included in excerpt in Alec Fisher, The Logic of Real Arguments (2004), 84-85. The full speech was reprinted in The Nullifidian, (Dec 1994). Transcribed online in the Richard Dawkins archive, article 89, titled: Lecture from 'The Nullifidian' (Dec 94).
The great masters of modern analysis are Lagrange, Laplace, and Gauss, who were contemporaries. It is interesting to note the marked contrast in their styles. Lagrange is perfect both in form and matter, he is careful to explain his procedure, and though his arguments are general they are easy to follow. Laplace on the other hand explains nothing, is indifferent to style, and, if satisfied that his results are correct, is content to leave them either with no proof or with a faulty one. Gauss is as exact and elegant as Lagrange, but even more difficult to follow than Laplace, for he removes every trace of the analysis by which he reached his results, and studies to give a proof which while rigorous shall be as concise and synthetical as possible.
In History of Mathematics (3rd Ed., 1901), 468.
The great secret of happiness consists not in enjoying, but in renouncing. But it is hard, very hard.
From Book III, 'A Talk on the Stairs', Hyperion: A Romance (1853), 213.
The idea that something in food might be of advantage to patients with pernicious anemia was in my mind in 1912, when I was a house officer at the Massachusetts General Hospital…. Ever since my student days, when I had the opportunity, in my father’s wards at the Massachusetts General Hospital, … I have taken a deep interest in this disease. … Prolonged observation permitted me to become acquainted with the multiple variations and many aspects of the disease, and to realize that from a few cases it was difficult to determine the effect of therapeutic procedures.
From Nobel Prize Lecture (12 Dec 1934), collected in Nobel Lectures, Physiology or Medicine 1922-1941 (1965).
The idea that time may vary from place to place is a difficult one, but it is the idea Einstein used, and it is correct—believe it or not.
…...
The indescribable pleasure—which pales the rest of life's joys—is abundant compensation for the investigator who endures the painful and persevering analytical work that precedes the appearance of the new truth, like the pain of childbirth. It is true to say that nothing for the scientific scholar is comparable to the things that he has discovered. Indeed, it would be difficult to find an investigator willing to exchange the paternity of a scientific conquest for all the gold on earth. And if there are some who look to science as a way of acquiring gold instead of applause from the learned, and the personal satisfaction associated with the very act of discovery, they have chosen the wrong profession.
From Reglas y Consejos sobre Investigacíon Cientifica: Los tónicos de la voluntad. (1897), as translated by Neely and Larry W. Swanson, in Advice for a Young Investigator (1999), 50.
The Internet is like a herd of performing elephants with diarrhea—massive, difficult to redirect, awe-inspiring, entertaining and a source of mind-boggling amounts of excrement when you least expect it.
Posted to a mailing list (1992), and circulated from there by some newsgroups. As authenticated in 'Quotable Spaf' on his faculty webpage at purdue.com
The layman is delighted to learn that after all, in spite of science being so impossibly difficult to understand, Scientists Are Human!
In What Mad Pursuit (1988), 83.
The more you understand the significance of evolution, the more you are pushed away from the agnostic position and towards atheism. Complex, statistically improbable things are by their nature more difficult to explain than simple, statistically probable things.
From edited version of a speech, at the Edinburgh International Science Festival (15 Apr 1992), as reprinted from the Independent newspaper in Alec Fisher, The Logic of Real Arguments (2004), 84.
The most difficult problem in mathematics is to make the date of a woman's birth agree with her present age.
In Evan Esar, 20,000 Quips and Quotes, 22.
The only objections that have occurred to me are, 1st that you have loaded yourself with an unnecessary difficulty in adopting Natura non facit saltum so unreservedly. … And 2nd, it is not clear to me why, if continual physical conditions are of so little moment as you suppose, variation should occur at all. However, I must read the book two or three times more before I presume to begin picking holes.
Comments after reading Darwin's book, Origin of Species.]
Comments after reading Darwin's book, Origin of Species.]
Letter to Charles Darwin (23 Nov 1859). In Charles Darwin and Francis Darwin (ed.), Charles Darwin: His Life Told in an Autobiographical Chapter, and in a Selected Series of His Published Letters (1892), 214.
The originator of a new concept … finds, as a rule, that it is much more difficult to find out why other people do not understand him than it was to discover the new truths.
From address on Michael Faraday. As given in Michael Pupin, From Immigrant to Inventor (1923), 225.
The path towards sustainable energy sources will be long and sometimes difficult. But America cannot resist this transition, we must lead it. We cannot cede to other nations the technology that will power new jobs and new industries, we must claim its promise. That’s how we will maintain our economic vitality and our national treasure—our forests and waterways, our crop lands and snow-capped peaks. That is how we will preserve our planet, commanded to our care by God. That’s what will lend meaning to the creed our fathers once declared.
In Second Inaugural Address (21 Jan 2013) at the United States Capitol.
The physicist cannot simply surrender to the philosopher the critical contemplation of the theoretical foundations for he himself knows best and feels most surely where the shoe pinches. … he must try to make clear in his own mind just how far the concepts which he uses are justified … The whole of science is nothing more than a refinement of everyday thinking. It is for this reason that the critical thinking of the physicist cannot possibly be restricted by the examination of the concepts of his own specific field. He cannot proceed without considering critically a much more difficult problem, the problem of analyzing the nature of everyday thinking.
‘Physics and Reality’, Franklin Institute Journal (Mar 1936). Collected in Out of My Later Years (1950), 59.
The progressive development of man is vitally dependent on invention. It is the most important product of his creative brain. Its ultimate purpose is the complete mastery of mind over the material world, the harnessing of the forces of nature to human needs. This is the difficult task of the inventor.
Opening lines of 'My Early Life, Part 1 of 'My Inventions', first of a series of articles in Electrical Experimenter (May 1919), 7, No. 73, 16. Collected in 'My Early Life', My Inventions: And Other Writings (2016), 1.
The Reason of making Experiments is, for the Discovery of the Method of Nature, in its Progress and Operations. Whosoever, therefore doth rightly make Experiments, doth design to enquire into some of these Operations; and, in order thereunto, doth consider what Circumstances and Effects, in the Experiment, will be material and instructive in that Enquiry, whether for the confirming or destroying of any preconceived Notion, or for the Limitation and Bounding thereof, either to this or that Part of the Hypothesis, by allowing a greater Latitude and Extent to one Part, and by diminishing or restraining another Part within narrower Bounds than were at first imagin'd, or hypothetically supposed. The Method therefore of making Experiments by the Royal Society I conceive should be this.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
'Dr Hooke's Method of Making Experiments' (1664-5). In W. Derham (ed.), Philosophical Experiments and Observations Of the Late Eminent Dr. Robert Hooke, F.R.S. And Geom. Prof. Gresh. and Other Eminent Virtuoso's in his Time (1726), 26-8.
The rich can pay when they have to pay [for medical care]. The poor receive free treatment from skilled specialists and can go, when necessary, to hospitals free of charge. But between seventy-five and ninety per cent. of our population, that which constitutes our very backbone, find it difficult to be relieved from the intolerable burden of illness.
In Genevieve Parkhurst, 'Dr. Sabin, Scientist: Winner Of Pictorial Review’s Achievement Award', Pictorial Review (Jan 1930), 70.
The star spectra present such varieties that it is difficult to point out any mode of classification. For the present, I divide them into three groups: first, those having many lines and bands and most nearly resembling the sun viz., Capella, β Geminorum, α Orionis, Aldeberan, γ Leonis, Arcturus, and β Pegasi. These are all reddish or golden stars. The second group, of which Sirius is the type, presents spectra wholly unlike that of the sun, and are white stars. The third group, comprising α Virginis, Rigel &c., are also white stars, but show no lines; perhaps they contain no mineral substance or are incandescent without flame.
L.M. Rutherfurd, 'Astronomical Observations with the Spectroscope' (4 Dec 1862), American Journal of Science and Arts (May 1863), 2nd Series, 35, No. 103, 77. His obituarist, Johns K. Rees, wrote (1892) “This paper was the first published work on star spectra.”
The surgeon should not love difficult cases and should not allow himself to be tempted to undertake those that are desperate. He should help the poor as far as he can, but he should not hesitate to ask for good fees from the rich.
Chirurgia Magna (1296, printed 1479), as translated by James Joseph Walsh in Old-Time Makers of Medicine (1911), 262.
The traditional disputes of philosophers are, for the most part, as unwarranted as they are unfruitful. The surest way to end them is to establish beyond question what should be the purpose and method of a philosophical enquiry. And this is by no means so difficult a task as the history of philosophy would lead one to suppose. For if there are any questions which science leaves it to philosophy to answer, a straightforward process of elimination must lead to their discovery.
Language, Truth and Logic (1960), 33.
The trouble about always trying to preserve the health of the body is that it is so difficult to do it without destroying the health of the mind.
In 'The Health of the Mind', Illustrated London News (10 Aug 1929), collected in Selected Essays (1955), 22.
The truth of the matter is that, though mathematics truth may be beauty, it can be only glimpsed after much hard thinking. Mathematics is difficult for many human minds to grasp because of its hierarchical structure: one thing builds on another and depends on it.
As co-author with D.T.E. Marjoram, Mathematics in a Changing World (1973).
The unprecedented identification of the spectrum of an apparently stellar object in terms of a large red-shift suggests either of the two following explanations.
The stellar object is a star with a large gravitational red-shift. Its radius would then be of the order of 10km. Preliminary considerations show that it would be extremely difficult, if not impossible, to account for the occurrence of permitted lines and a forbidden line with the same red-shift, and with widths of only 1 or 2 per cent of the wavelength.
The stellar object is the nuclear region of a galaxy with a cosmological red-shift of 0.158, corresponding to an apparent velocity of 47,400 km/sec. The distance would be around 500 megaparsecs, and the diameter of the nuclear region would have to be less than 1 kiloparsec. This nuclear region would be about 100 times brighter optically than the luminous galaxies which have been identified with radio sources thus far. If the optical jet and component A of the radio source are associated with the galaxy, they would be at a distance of 50 kiloparsecs implying a time-scale in excess of 105 years. The total energy radiated in the optical range at constant luminosity would be of the order of 1059 ergs.
Only the detection of irrefutable proper motion or parallax would definitively establish 3C 273 as an object within our Galaxy. At the present time, however, the explanation in terms of an extragalactic origin seems more direct and less objectionable.
The stellar object is a star with a large gravitational red-shift. Its radius would then be of the order of 10km. Preliminary considerations show that it would be extremely difficult, if not impossible, to account for the occurrence of permitted lines and a forbidden line with the same red-shift, and with widths of only 1 or 2 per cent of the wavelength.
The stellar object is the nuclear region of a galaxy with a cosmological red-shift of 0.158, corresponding to an apparent velocity of 47,400 km/sec. The distance would be around 500 megaparsecs, and the diameter of the nuclear region would have to be less than 1 kiloparsec. This nuclear region would be about 100 times brighter optically than the luminous galaxies which have been identified with radio sources thus far. If the optical jet and component A of the radio source are associated with the galaxy, they would be at a distance of 50 kiloparsecs implying a time-scale in excess of 105 years. The total energy radiated in the optical range at constant luminosity would be of the order of 1059 ergs.
Only the detection of irrefutable proper motion or parallax would definitively establish 3C 273 as an object within our Galaxy. At the present time, however, the explanation in terms of an extragalactic origin seems more direct and less objectionable.
'3C 273: A Star-like Object with Large Red-Shift', Nature (1963), 197, 1040.
The value of mathematical instruction as a preparation for those more difficult investigations, consists in the applicability not of its doctrines but of its methods. Mathematics will ever remain the past perfect type of the deductive method in general; and the applications of mathematics to the simpler branches of physics furnish the only school in which philosophers can effectually learn the most difficult and important of their art, the employment of the laws of simpler phenomena for explaining and predicting those of the more complex. These grounds are quite sufficient for deeming mathematical training an indispensable basis of real scientific education, and regarding with Plato, one who is … as wanting in one of the most essential qualifications for the successful cultivation of the higher branches of philosophy
In System of Logic, Bk. 3, chap. 24, sect. 9.
The whole question of imagination in science is often misunderstood by people in other disciplines. They try to test our imagination in the following way. They say, “Here is a picture of some people in a situation. What do you imagine will happen next?” When we say, “I can’t imagine,” they may think we have a weak imagination. They overlook the fact that whatever we are allowed to imagine in science must be consistent with everything else we know; that the electric fields and the waves we talk about are not just some happy thoughts which we are free to make as we wish, but ideas which must be consistent with all the laws of physics we know. We can’t allow ourselves to seriously imagine things which are obviously in contradiction to the laws of nature. And so our kind of imagination is quite a difficult game. One has to have the imagination to think of something that has never been seen before, never been heard of before. At the same time the thoughts are restricted in a strait jacket, so to speak, limited by the conditions that come from our knowledge of the way nature really is. The problem of creating something which is new, but which is consistent with everything which has been seen before, is one of extreme difficulty
In The Feynman Lectures in Physics (1964), Vol. 2, Lecture 20, p.20-10 to p.20-11.
There are four subjects which must be taught: reading, writing and arithmetic, and the fear of God. The most difficult of these is arithmetic.
Quoted as a filler, without citation in The Record (3 Nov 1948), 40, No. 8, 2. (Student newspaper of the New York State College for Teachers.)
There are no shortcuts to moral insight. Nature is not intrinsically anything that can offer comfort or solace in human terms–if only because our species is such an insignificant latecomer in a world not constructed for us. So much the better. The answers to moral dilemmas are not lying out there, waiting to be discovered. They reside, like the kingdom of God, within us–the most difficult and inaccessible spot for any discovery or consensus.
…...
There can be but one opinion as to the beauty and utility of this analysis of Laplace; but the manner in which it has been hitherto presented has seemed repulsive to the ablest mathematicians, and difficult to ordinary mathematical students.[Co-author with Peter Guthrie Tait.]
In William Thomson Baron Kelvin, Peter Guthrie Tait, Treatise on Natural Philosophy (1879), Vol. 1, Preface, vii.
There could not be a language more universal and more simple, more exempt from errors and obscurities, that is to say, more worthy of expressing the invariable relations of natural objects. Considered from this point of view, it is coextensive with nature itself; it defines all the sensible relations, measures the times, the spaces, the forces, the temperatures; this difficult science is formed slowly, but it retains all the principles it has once acquired. It grows and becomes more certain without limit in the midst of so many errors of the human mind.
From Théorie Analytique de la Chaleur, Discours Préliminaire (Theory of Heat, Introduction), quoted as translated in F.R. Moulton, 'The Influence of Astronomy on Mathematics', Science (10 Mar 1911), N.S. Vol. 33, No. 845, 359.
There is no art or science that is too difficult for industry to attain to; it is the gift of tongues, and makes a man understood and valued in all countries, and by all nations; it is the philosopher's stone, that turns all metals, and even stones, into gold, and suffers not want to break into its dwelling; it is the northwest passage, that brings the merchant's ships as soon to him as he can desire: in a word, it conquers all enemies, and makes fortune itself pay contribution.
'Essay on Industry' (1670). In Thomas Henry Lister, Life and Administration of Edward, first Earl of Clarendon (1838), Vol. 2, 566.
There is no art so difficult as the art of observation: it requires a skillful, sober spirit and a well-trained experience, which can only be acquired by practice; for he is not an observer who only sees the thing before him with his eyes, but he who sees of what parts the thing consists, and in what connexion the parts stand to the whole. One person overlooks half from inattention; another relates more than he sees while he confounds it with that which he figures to himself; another sees the parts of the whole, but he throws things together that ought to be separated. ... When the observer has ascertained the foundation of a phenomenon, and he is able to associate its conditions, he then proves while he endeavours to produce the phenomena at his will, the correctness of his observations by experiment. To make a series of experiments is often to decompose an opinion into its individual parts, and to prove it by a sensible phenomenon. The naturalist makes experiments in order to exhibit a phenomenon in all its different parts. When he is able to show of a series of phenomena, that they are all operations of the same cause, he arrives at a simple expression of their significance, which, in this case, is called a Law of Nature. We speak of a simple property as a Law of Nature when it serves for the explanation of one or more natural phenomena.
'The Study of the Natural Sciences: An Introductory Lecture to the Course of Experimental Chemistry in the University of Munich, for the Winter Session of 1852-53,' as translated and republished in The Medical Times and Gazette (22 Jan 1853), N.S. Vol. 6, 82.
There is no existing ‘standard of protein intake’ that is based on the sure ground of experimental evidence. ... Between the two extremes of a very high and a very low protein intake it is difficult to prove that one level of intake is preferable to another. ... Physiologists, in drawing up dietary standards, are largely influenced by the dietary habits of their time and country.
Nutrition and Public Health', League of Nations Health Organization Quarterly Bulletin (1935) 4, 323–474. In Kenneth J. Carpenter, 'The Work of Wallace Aykroyd: International Nutritionist and Author', The Journal of Nutrition (2007), 137, 873-878.
There is no form of prose more difficult to understand and more tedious to read than the average scientific paper.
The Astonishing Hypothesis: The Scientific Search for the Soul (1995), xiii.
There is no short cut, nor “royal road” to the attainment of medical knowledge. The path which we have to pursue is long, difficult, and unsafe. In our progress, we must frequently take up our abode with death and corruption, we must adopt loathsome diseases for our familiar associates, or we shall never be acquainted with their nature and dispositions ; we must risk, nay, even injure our own health, in order to be able to preserve, or restore that of others.
Hunterian Oration (1819). Quoted in Clement Carlyon, Early Years and Late Reflections (1856), 110-111.
There may be a golden ignorance. If Professor Bell had known how difficult a task he was attempting, he would never have given us the telephone.
From chapter 'Jottings from a Note-book', in Canadian Stories (1918), 178.
There was a golden period that I look back upon with great regret, in which the cheapest of experimental animals were medical students. Graduate students were even better. In the old days, if you offered a graduate student a thiamine-deficient diet, he gladly went on it, for that was the only way he could eat. Science is getting to be more and more difficult.
In talk, 'Origin of Death' (1970).
There was a seminar for advanced students in Zürich that I was teaching and von Neumann was in the class. I came to a certain theorem, and I said it is not proved and it may be difficult. Von Neumann didn’t say anything but after five minutes he raised his hand. When I called on him he went to the blackboard and proceeded to write down the proof. After that I was afraid of von Neumann.
In George Pólya and Gerald L. Alexanderson (ed.) The Pólya Picture Album: Encounters of a Mathematician (1987), 154. Also footnoted in Matti Tedre, The Development of Computer Science: a Sociocultural Perspective (2006), 198, cited as from How to Solve It: A New Aspect of Mathematical Method (1957), xv.
These facts shaw that mitosis is due to the co-ordinate play of an extremely complex system of forces which are as yet scarcely comprehended. Its purpose is, however, as obvious as its physiological explanation is difficult. It is the end of mitosis to divide every part of the chromatin of the mother-cell equally between the daughter-nuclei. All the other operations are tributary to this. We may therefore regard the mitotic figure as essentially an apparatus for the distribution of the hereditary substance, and in this sense as the especial instrument of inheritance.
The Cell in Development and Inheritance (1896), 86.
This is the reason why all attempts to obtain a deeper knowledge of the foundations of physics seem doomed to me unless the basic concepts are in accordance with general relativity from the beginning. This situation makes it difficult to use our empirical knowledge, however comprehensive, in looking for the fundamental concepts and relations of physics, and it forces us to apply free speculation to a much greater extent than is presently assumed by most physicists.
…...
This quality of genius is, sometimes, difficult to be distinguished from talent, because high genius includes talent. It is talent, and something more. The usual distinction between genius and talent is, that one represents creative thought, the other practical skill: one invents, the other applies. But the truth is, that high genius applies its own inventions better than talent alone can do. A man who has mastered the higher mathematics, does not, on that account, lose his knowledge of arithmetic. Hannibal, Napoleon, Shakespeare, Newton, Scott, Burke, Arkwright, were
they not men of talent as well as men of genius?
In 'Genius', Wellman’s Miscellany (Dec 1871), 4, No. 6, 203.
Though we must not without further consideration condemn a body of reasoning merely because it is easy, nevertheless we must not allow ourselves to be lured on merely by easiness; and we should take care that every problem which we choose for attack, whether it be easy or difficult, shall have a useful purpose, that it shall contribute in some measure to the up-building of the great edifice.
From 'On Some Recent Tendencies in Geometric Investigation', Rivista di Matematica (1891), 63. In Bulletin American Mathematical Society (1904), 465.
To ask what qualities distinguish good from routine scientific research is to address a question that should be of central concern to every scientist. We can make the question more tractable by rephrasing it, “What attributes are shared by the scientific works which have contributed importantly to our understanding of the physical world—in this case the world of living things?” Two of the most widely accepted characteristics of good scientific work are generality of application and originality of conception. . These qualities are easy to point out in the works of others and, of course extremely difficult to achieve in one’s own research. At first hearing novelty and generality appear to be mutually exclusive, but they really are not. They just have different frames of reference. Novelty has a human frame of reference; generality has a biological frame of reference. Consider, for example, Darwinian Natural Selection. It offers a mechanism so widely applicable as to be almost coexistent with reproduction, so universal as to be almost axiomatic, and so innovative that it shook, and continues to shake, man’s perception of causality.
In 'Scientific innovation and creativity: a zoologist’s point of view', American Zoologist (1982), 22, 230.
To do easily what is difficult for others is the mark of talent. To do what is impossible for talent is the mark of genius.
Entry for 17 Dec 1856 in Amiel’s Journal: The Journal Intime of Henri-Frédéric Amiel, trans. Humphry Ward (1893), 60.
To emphasize this opinion that mathematicians would be unwise to accept practical issues as the sole guide or the chief guide in the current of their investigations, ... let me take one more instance, by choosing a subject in which the purely mathematical interest is deemed supreme, the theory of functions of a complex variable. That at least is a theory in pure mathematics, initiated in that region, and developed in that region; it is built up in scores of papers, and its plan certainly has not been, and is not now, dominated or guided by considerations of applicability to natural phenomena. Yet what has turned out to be its relation to practical issues? The investigations of Lagrange and others upon the construction of maps appear as a portion of the general property of conformal representation; which is merely the general geometrical method of regarding functional relations in that theory. Again, the interesting and important investigations upon discontinuous two-dimensional fluid motion in hydrodynamics, made in the last twenty years, can all be, and now are all, I believe, deduced from similar considerations by interpreting functional relations between complex variables. In the dynamics of a rotating heavy body, the only substantial extension of our knowledge since the time of Lagrange has accrued from associating the general properties of functions with the discussion of the equations of motion. Further, under the title of conjugate functions, the theory has been applied to various questions in electrostatics, particularly in connection with condensers and electrometers. And, lastly, in the domain of physical astronomy, some of the most conspicuous advances made in the last few years have been achieved by introducing into the discussion the ideas, the principles, the methods, and the results of the theory of functions. … the refined and extremely difficult work of Poincare and others in physical astronomy has been possible only by the use of the most elaborate developments of some purely mathematical subjects, developments which were made without a thought of such applications.
In Presidential Address British Association for the Advancement of Science, Section A, (1897), Nature, 56, 377.
To know how to grow old is the master-work of wisdom, and one of the most difficult chapters in the great art of living.
Amiel's Journal The Journal Intime of Henri-Frederic Amiel, (21 Sep 1874), trans. By Mrs Humphry Ward (1889),177.
To-day, science has withdrawn into realms that are hardly understanded of the people. Biology means very largely histology, the study of the cell by difficult and elaborate microscopical processes. Chemistry has passed from the mixing of simple substances with ascertained reactions, to an experimentation of these processes under varying conditions of temperature, pressure, and electrification—all requiring complicated apparatus and the most delicate measurement and manipulation. Similarly, physics has outgrown the old formulas of gravity, magnetism, and pressure; has discarded the molecule and atom for the ion, and may in its recent generalizations be followed only by an expert in the higher, not to say the transcendental mathematics.
‘Exit the Amateur Scientist.’ Editorial, The Nation, 23 August 1906, 83, 160.
Traditions may be very important, but they can be extremely hampering as well, and whether or not tradition is of really much value I have never been certain. Of course when they are very fine, they do good, but it is very difficult of course ever to repeat the conditions under which good traditions are formed, so they may be and are often injurious and I think the greatest progress is made outside of traditions.
Quoted as the notations of an unnamed student in the auditorium when Councilman made impromptu biographical remarks at his last lecture as a teacher of undergraduates in medicine (19 Dec 1921). As quoted in obituary, 'William Thomas Councilman', by Harvey Cushing, Science (30 Jun 1933), 77, No. 2009, 613-618. Reprinted in National Academy Biographical Memoirs, Vol. 18, 159-160. The transcribed lecture was published privately in a 23-page booklet, A Lecture Delivered to the Second-Year Class of the Harvard Medical School at the Conclusion of the Course in Pathology, Dec. 19, 1921.
Two extreme views have always been held as to the use of mathematics. To some, mathematics is only measuring and calculating instruments, and their interest ceases as soon as discussions arise which cannot benefit those who use the instruments for the purposes of application in mechanics, astronomy, physics, statistics, and other sciences. At the other extreme we have those who are animated exclusively by the love of pure science. To them pure mathematics, with the theory of numbers at the head, is the only real and genuine science, and the applications have only an interest in so far as they contain or suggest problems in pure mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
In History of European Thought in the Nineteenth Century (1903), 630.
We have also here an acting cause to account for that balance so often observed in nature,—a deficiency in one set of organs always being compensated by an increased development of some others—powerful wings accompanying weak feet, or great velocity making up for the absence of defensive weapons; for it has been shown that all varieties in which an unbalanced deficiency occurred could not long continue their existen The action of this principle is exactly like that of the centrifugal governor of the steam engine, which checks and corrects any irregularities almost before they become evident; and in like manner no unbalanced deficiency in the animal kingdom can ever reach any conspicuous magnitude, because it would make itself felt at the very first step, by rendering existence difficult and extinction almost sure soon to follow.
In 'On the Tendency of Varieties to Depart Indefinitely from the Original Type', Journal of the Proceedings of the Linnean Society, Zoology (1858), 3, 61-62.
We live in a glass-soaked civilization, but as for the bird in the Chinese proverb who finds it so difficult to discover air, the substance is almost invisible to us. To use a metaphor drawn from glass, it may be revealing for us to re-focus, to stop looking through glass, and let our eyes dwell on it for a moment to contemplate its wonder. [Co-author with Gerry Martin.]
Glass: A World History (2002), 4.
We were trying. In spite of several failures, we felt we were getting close. It’s difficult to translate the optimism of the Brigham staff and hospital. The administration really backed us.
Concerning the early years trying to solve the problem of rejection of a transplanted kidney. As quoted by Alvin Powell in 'A Transplant Makes History', Harvard Gazette (22 Sep 2011). The writer of the articles stated: “Through those dark years, he and his colleagues pressed on, inspired by the dying patients who volunteered for surgery in hopes that, even if they didn’t make it, enough could be learned that success would come one day.”
What certainty can there be in a Philosophy which consists in as many Hypotheses as there are Phaenomena to be explained. To explain all nature is too difficult a task for any one man or even for any one age. 'Tis much better to do a little with certainty, & leave the rest for others that come after you, than to explain all things by conjecture without making sure of any thing.
Quoted in Richard S. Westfall, The Life of Isaac Newton (1994), 256.
What is mathematics? To give a satisfactory definition is difficult, if not impossible.
In Lectures on Fundamental Concepts of Algebra and Geometry (1911), 1.
What is more difficult, to think of an encampment on the moon or of Harlem rebuilt? Both are now within the reach of our resources. Both now depend upon human decision and human will.
…...
When a man of science speaks of his “data,” he knows very well in practice what he means. Certain experiments have been conducted, and have yielded certain observed results, which have been recorded. But when we try to define a “datum” theoretically, the task is not altogether easy. A datum, obviously, must be a fact known by perception. But it is very difficult to arrive at a fact in which there is no element of inference, and yet it would seem improper to call something a “datum” if it involved inferences as well as observation. This constitutes a problem. …
In The Analysis of Matter (1954).
When first I applied my mind to Mathematics I read straight away most of what is usually given by the mathematical writers, and I paid special attention to Arithmetic and Geometry because they were said to be the simplest and so to speak the way to all the rest. But in neither case did I then meet with authors who fully satisfied me. I did indeed learn in their works many propositions about numbers which I found on calculation to be true. As to figures, they in a sense exhibited to my eyes a great number of truths and drew conclusions from certain consequences. But they did not seem to make it sufficiently plain to the mind itself why these things are so, and how they discovered them. Consequently I was not surprised that many people, even of talent and scholarship, should, after glancing at these sciences, have either given them up as being empty and childish or, taking them to be very difficult and intricate, been deterred at the very outset from learning them. … But when I afterwards bethought myself how it could be that the earliest pioneers of Philosophy in bygone ages refused to admit to the study of wisdom any one who was not versed in Mathematics … I was confirmed in my suspicion that they had knowledge of a species of Mathematics very different from that which passes current in our time.
In Elizabeth S. Haldane (trans.) and G.R.T. Ross (trans.), 'Rules for the Direction of the Mind', The Philosophical Works of Descartes (1911, 1973), Vol. 1, Rule 4, 11.
When I undertake the dissection of a human cadaver I pass a stout rope tied like a noose beneath the lower jaw and through the two zygomas up to the top of the head, either more toward the forehead or more toward the occiput according as I want the cadaver to hang with its head up or down. The longer end of the noose I run through a pulley fixed to a beam in the room so that I may raise or lower the cadaver as it hangs there or may turn it round in any direction to suit my purpose; and should I so wish I can allow it to recline at an angle upon a table, since a table can easily be placed underneath the pulley. This is how the cadaver was suspended for drawing all the muscle tables... though while that one was being drawn the rope was passed around the occiput so as to show the muscles in the neck. If the lower jaw has been removed in the course of dissection, or the zygomas have been broken, the hollows for the temporal muscles will nonetheless hold the noose sufficiently firmly. You must take care not to put the noose around the neck, unless some of the muscles connected to the occipital bone have already been cut away. It is best to suspend the cadaver like this because a human body lying on a table is very difficult to turn over on to its chest or its back.
From De Humani Corporis Fabrica Libri Septem (1543), Book II, 268, as translated by William Frank Richardson and John Burd Carman, in 'How the Cadaver Can Be Held Erect While These Muscles are Dissected', On The Fabric of the Human Body: Book II: The Ligaments and Muscles (1998), 234.
When one considers in its length and in its breadth the importance of this question of the education of the nation's young, the broken lives, the defeated hopes, the national failures, which result from the frivolous inertia with which it is treated, it is difficult to restrain within oneself a savage rage. In the conditions of modern life the rule is absolute, the race which does not value trained intelligence is doomed. Not all your heroism, not all your social charm, not all your wit, not all your victories on land or at sea, can move back the finger of fate. To-day we maintain ourselves. To-morrow science will have moved forward yet one more step, and there will be no appeal from the judgment which will then be pronounced on the uneducated.
In 'Organisation of Thought', The Aims of Education: & Other Essays (1917), 22.
When the war finally came to an end, 1 was at a loss as to what to do. ... I took stock of my qualifications. A not-very-good degree, redeemed somewhat by my achievements at the Admiralty. A knowledge of certain restricted parts of magnetism and hydrodynamics, neither of them subjects for which I felt the least bit of enthusiasm.
No published papers at all … [Only gradually did I realize that this lack of qualification could be an advantage. By the time most scientists have reached age thirty they are trapped by their own expertise. They have invested so much effort in one particular field that it is often extremely difficult, at that time in their careers, to make a radical change. I, on the other hand, knew nothing, except for a basic training in somewhat old-fashioned physics and mathematics and an ability to turn my hand to new things. … Since I essentially knew nothing, I had an almost completely free choice. …
No published papers at all … [Only gradually did I realize that this lack of qualification could be an advantage. By the time most scientists have reached age thirty they are trapped by their own expertise. They have invested so much effort in one particular field that it is often extremely difficult, at that time in their careers, to make a radical change. I, on the other hand, knew nothing, except for a basic training in somewhat old-fashioned physics and mathematics and an ability to turn my hand to new things. … Since I essentially knew nothing, I had an almost completely free choice. …
In What Mad Pursuit (1988).
When you start in science, you are brainwashed into believing how careful you must be, and how difficult it is to discover things. There’s something that might be called the “graduate student syndrome”; graduate students hardly believe they can make a discovery.
Quotation supplied by Professor Francis Crick.
Whenever a textbook is written of real educational worth, you may be quite certain that some reviewer will say that it will be difficult to teach from it. Of course it will be difficult to teach from it. It it were easy, the book ought to be burned; for it cannot be educational. In education as elsewhere, the broad primrose path leads to a nasty place. This evil path is represented by a book or a set of lectures which will practically enable the student to learn by heart all the questions likely to be asked at the next external examination.
In 'The Aims of Education', The Aims of Education: & Other Essays (1917), 6-7.
Why is it so easy to acquire the solutions of past problems and so difficult to solve current ones
(Attributed ??) This quote is often seen, but without a citation, even on the official Marshall McLuhan website. If you known a primary print source, please contact Webmaster.
Will fluorine ever have practical applications?
It is very difficult to answer this question. I may, however, say in all sincerity that I gave this subject little thought when I undertook my researches, and I believe that all the chemists whose attempts preceded mine gave it no more consideration.
A scientific research is a search after truth, and it is only after discovery that the question of applicability can be usefully considered.
It is very difficult to answer this question. I may, however, say in all sincerity that I gave this subject little thought when I undertook my researches, and I believe that all the chemists whose attempts preceded mine gave it no more consideration.
A scientific research is a search after truth, and it is only after discovery that the question of applicability can be usefully considered.
Proceedings of the Royal Institution (1897). In Annual Report of the Board of Regents of the Smithsonian Institution to July 1897 (1898), 261.
With crystals we are in a situation similar to an attempt to investigate an optical grating merely from the spectra it produces... But a knowledge of the positions and intensities of the spectra does not suffice for the determination of the structure. The phases with which the diffracted waves vibrate relative to one another enter in an essential way. To determine a crystal structure on the atomic scale, one must know the phase differences between the different interference spots on the photographic plate, and this task may certainly prove to be rather difficult.
Physikalische Zeitschrift (1913), 14. Translated in Walter Moore, Schrödinger. Life and Thought (1989), 73.
Without this language [mathematics] most of the intimate analogies of things would have remained forever unknown to us; and we should forever have been ignorant of the internal harmony of the world, which is the only true objective reality. …
This harmony … is the sole objective reality, the only truth we can attain; and when I add that the universal harmony of the world is the source of all beauty, it will be understood what price we should attach to the slow and difficult progress which little by little enables us to know it better.
This harmony … is the sole objective reality, the only truth we can attain; and when I add that the universal harmony of the world is the source of all beauty, it will be understood what price we should attach to the slow and difficult progress which little by little enables us to know it better.
From La Valeur de la Science, as translated by George Bruce Halsted, in 'The Value of Science', Popular Science Monthly (Sep 1906), 69 195-196.
You may drink the ocean dry; you may uproot from its base the mountain Meru: you may swallow fire. But more difficult than all these, oh Good One! is control over the mind.
Quoted in Kim Lim (ed.), 1,001 Pearls of Spiritual Wisdom: Words to Enrich, Inspire, and Guide Your Life (2014), 258.
![Christiaan Barnard quote: For a dying man it is not a difficult decision [to agree to become the world’s first heart transplant]](https://todayinsci.com/B/Barnard_Christiaan/BarnardChristiaan-Decision500x250px.jpg)


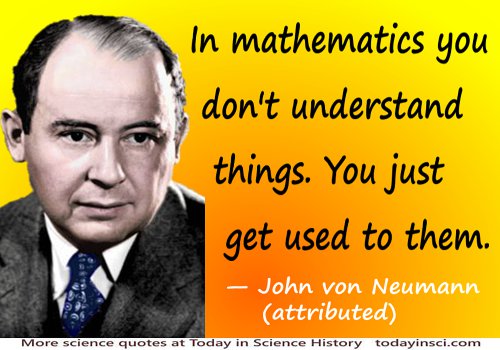










 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


