Theory Quotes (1023 quotes)
… however useful the words may have been in the past, they have now become handicaps to the further development of knowledge. Words like botany and zoology imply that plants and animals are quite different things. … But the differences rapidly become blurred when we start looking at the world through a microscope. … The similarities between plants and animals became more important than their differences with the discoveries that both were built up of cells, had sexual reproduction,… nutrition and respiration … and with the development of evolutionary theory.
In The Forest and the Sea (1960), 7.
... one of the main functions of an analogy or model is to suggest extensions of the theory by considering extensions of the analogy, since more is known about the analogy than is known about the subject matter of the theory itself … A collection of observable concepts in a purely formal hypothesis suggesting no analogy with anything would consequently not suggest either any directions for its own development.
'Operational Definition and Analogy in Physical Theories', British Journal for the Philosophy of Science (Feb 1952), 2, No. 8, 291.
... there is an external world which can in principle be exhaustively described in scientific language. The scientist, as both observer and language-user, can capture the external facts of the world in prepositions that are true if they correspond to the facts and false if they do not. Science is ideally a linguistic system in which true propositions are in one-to-one relation to facts, including facts that are not directly observed because they involve hidden entities or properties, or past events or far distant events. These hidden events are described in theories, and theories can be inferred from observation, that is the hidden explanatory mechnism of the world can be discovered from what is open to observation. Man as scientist is regarded as standing apart from the world and able to experiment and theorize about it objectively and dispassionately.
'Introduction', Revolutions and Reconstructions in the Philosophy of Science (1981), xii. In John Templeton and Robert L. Herrmann, Is God the Only Reality (1994), 11-12.
…The present revolution of scientific thought follows in natural sequence on the great revolutions at earlier epochs in the history of science. Einstein’s special theory of relativity, which explains the indeterminateness of the frame of space and time, crowns the work of Copernicus who first led us to give up our insistence on a geocentric outlook on nature; Einstein's general theory of relativity, which reveals the curvature or non-Euclidean geometry of space and time, carries forward the rudimentary thought of those earlier astronomers who first contemplated the possibility that their existence lay on something which was not flat. These earlier revolutions are still a source of perplexity in childhood, which we soon outgrow; and a time will come when Einstein’s amazing revelations have likewise sunk into the commonplaces of educated thought.
In The Theory of Relativity and its Influence on Scientific Thought (1922), 31-32
“Does one error disappear only to make room for another?” … [L]et us look at the science of astronomy. How grand and magnificent have been the discoveries in that field of knowledge. What victories over error have been achieved by the telescope. That instrument did … bring down and dispel vast clouds of error, both in respect of the sky and of our planet. It must be confessed, too, that it took something from the importance of our planet. The idea that all the hosts of heaven are mere appendages to this earth is no longer entertained by average men, and … [almost no men] now stand by the old theory for which the church proposed to murder Galileo. Men are compelled to admit that the Genesis by Moses is less trustworthy as to the time of creating the heavens and the earth than are the rocks and the stars.
From speech (20 Nov 1883) delivered to the Bethel Literary and Historical Association, Washington D.C.,'It Moves, or Philosophy of Reform', collected in The Essential Douglass: Selected Writings and Speeches (2016), 290.
“If there are two theories, one simpler man the other, the simpler one is to be preferred.” At first sight this does not seem quite so bad, but a little thought shows that our tendency to prefer the simpler possibility is psychological rather than scientific. It is less trouble to think that way. Experience invariably shows that the more correct a theory becomes, the more complex does it seem. … So this … interpretation of [Ockham’s Razor] is … worthless.
With co-author Nalin Chandra Wickramasinghe, Evolution from Space (1981), 135.
“Science studies everything,” say the scientists. But, really, everything is too much. Everything is an infinite quantity of objects; it is impossible at one and the same time to study all. As a lantern cannot light up everything, but only lights up the place on which it is turned or the direction in which the man carrying it is walking, so also science cannot study everything, but inevitably only studies that to which its attention is directed. And as a lantern lights up most strongly the place nearest to it, and less and less strongly objects that are more and more remote from it, and does not at all light up those things its light does not reach, so also human science, of whatever kind, has always studied and still studies most carefully what seems most important to the investigators, less carefully what seems to them less important, and quite neglects the whole remaining infinite quantity of objects. ... But men of science to-day ... have formed for themselves a theory of “science for science's sake,” according to which science is to study not what mankind needs, but everything.
In 'Modern Science', Essays and Letters (1903), 223.
“Unless,” said I [Socrates], “either philosophers become kings in our states or those whom we now call our kings and rulers take to the pursuit of' philosophy seriously and adequately, and there is a conjunction of these two things, political power and philosophic intelligence, while the motley horde of the natures who at present pursue either apart from the other are compulsorily excluded, there can be no cessation of troubles, dear Glaucon, for our states, nor, I fancy for the human race either. Nor, until this happens, will this constitution which we have been expounding in theory ever be put into practice within the limits of possibility and see the light of the sun.”
— Plato
From The Republic 5 473 c-e, in Paul Shorey (trans.), Plato in Twelve Volumes (1930, 1969), Vol. 5, 509.
“Wu Li” was more than poetic. It was the best definition of physics that the conference would produce. It caught that certain something, that living quality that we were seeking to express in a book, that thing without which physics becomes sterile. “Wu” can mean either “matter” or “energy.” “Li” is a richly poetic word. It means “universal order” or “universal law.” It also means “organic patterns.” The grain in a panel of wood is Li. The organic pattern on the surface of a leaf is also Li, and so is the texture of a rose petal. In short, Wu Li, the Chinese word for physics, means “patterns of organic energy” (“matter/ energy” [Wu] + “universal order/organic patterns” [Li]). This is remarkable since it reflects a world view which the founders of western science (Galileo and Newton) simply did not comprehend, but toward which virtually every physical theory of import in the twentieth century is pointing!
In The Dancing Wu Li Masters: An Overview of the New Physics (1979), 5.
[A] theory is a Fact; a Fact is a familiar Theory.
In The Philosophy of the Inductive Sciences (1840), Vol. I, 40.
[Alfred Russell] Wallace's sales agent, back in London, heard mutterings from some naturalists that young Mr. Wallace ought to quit theorizing and stick to gathering facts. Besides expressing their condescension toward him in particular, that criticism also reflected a common attitude that fact-gathering, not theory, was the proper business of all naturalists.
In The Song of the Dodo: Island Biogeography in an Age of Extinctions (1996), 41.
[An outsider views a scientist] as a type of unscrupulous opportunist: he appears as a realist, insofar as he seeks to describe the world independent of the act of perception; as idealist insofar as he looks upon the concepts and theories as the free inventions of the human spirit (not logically derivable from that which is empirically given); as positivist insofar as he considers his concepts and theories justified only to the extent to which they furnish a logical representation of relations among sense experiences. He may even appear as Platonist or Pythagorean insofar as he considers the viewpoint of logical simplicity as an indispensable and effective tool of his research.
In 'Reply to Critcisms', Paul Arthur Schilpp (ed.), Albert Einstein: Philosopher-Scientist (1949, 1959), Vol. 2, 684.
[As a science hobbyist, hoping to become famous someday, Artie Pinsetter (Lou Costello):] They also laughed at Einstein and his theory of relativity. Now everyone has relatives.
From movie The 30-foot Bride of Candy Rock (1959). Writers, Rowland Barber and Arthur A. Ross. In Larry Langman and Paul Gold, Comedy Quotes from the Movies (2001), 289. This movie, (with its rare appearance of Costello without Bud Abott, his usual comedy partner), was released later in the year of Costello's death.
[Cantor’s set theory is] The finest product of mathematical genius and one of the supreme achievements of purely intellectual human activity.
As quoted in Constance Reid, Hilbert (1970), 176.
[Creationists] make it sound as though a “theory” is something you dreamt up after being drunk all night.
Remark to the National Center Against Censorship (NCAC)(1980). In Norman A. Johnson, Darwinian Detectives (2007), 27.
[Experimental Physicist] Phys. I know that it is often a help to represent pressure and volume as height and width on paper; and so geometry may have applications to the theory of gases. But is it not going rather far to say that geometry can deal directly with these things and is not necessarily concerned with lengths in space?
[Mathematician] Math. No. Geometry is nowadays largely analytical, so that in form as well as in effect, it deals with variables of an unknown nature. …It is literally true that I do not want to know the significance of the variables x, y, z, t that I am discussing. …
Phys. Yours is a strange subject. You told us at the beginning that you are not concerned as to whether your propositions are true, and now you tell us you do not even care to know what you are talking about.
Math. That is an excellent description of Pure Mathematics, which has already been given by an eminent mathematician [Bertrand Russell].
[Mathematician] Math. No. Geometry is nowadays largely analytical, so that in form as well as in effect, it deals with variables of an unknown nature. …It is literally true that I do not want to know the significance of the variables x, y, z, t that I am discussing. …
Phys. Yours is a strange subject. You told us at the beginning that you are not concerned as to whether your propositions are true, and now you tell us you do not even care to know what you are talking about.
Math. That is an excellent description of Pure Mathematics, which has already been given by an eminent mathematician [Bertrand Russell].
In Space, Time and Gravitation: An Outline of the General Relativity Theory (1920, 1921), 14.
[F. Werner, while a student in Princeton,] came to me and expressed his bewilderment with the fact that we make a rather narrow selection when choosing the data on which we test our theories. “How do we know that, if we made a theory which focuses its attention on phenomena we disregard and disregards some of the phenomena now commanding our attention, that we could not build another theory which has little in common with the present one but which, nevertheless, explains just as many phenomena as the present theory?” It has to be admitted that we have no definite evidence that there is no such theory.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences,' Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1 (February 1960). Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 535.
[Gauss calculated the elements of the planet Ceres] and his analysis proved him to be the first of theoretical astronomers no less than the greatest of “arithmeticians.”
In History of Mathematics (3rd Ed., 1901), 458.
[In mathematics] There are two kinds of mistakes. There are fatal mistakes that destroy a theory, but there are also contingent ones, which are useful in testing the stability of a theory.
In 'Ten Lessons I Wish I Had Been Taught', Indiscrete Thoughts (2008), 202.
[In] the evolution of ideas… New ideas are thrown up spontaneously like mutations; the vast majority of them are useless crank theories, the equivalent of biological freaks without survival-value.
In Epilogue, The Sleepwalkers: A History of Man’s Changing Vision of the Universe (1959), 515.
[John] Dalton was a man of regular habits. For fifty-seven years he walked out of Manchester every day; he measured the rainfall, the temperature—a singularly monotonous enterprise in this climate. Of all that mass of data, nothing whatever came. But of the one searching, almost childlike question about the weights that enter the construction of these simple molecules—out of that came modern atomic theory. That is the essence of science: ask an impertinent question, and you are on the way to the pertinent answer.
The Ascent of Man (1973), 153.
[Kepler] had to realize clearly that logical-mathematical theoretizing, no matter how lucid, could not guarantee truth by itself; that the most beautiful logical theory means nothing in natural science without comparison with the exactest experience. Without this philosophic attitude, his work would not have been possible.
From Introduction that Einstein wrote for Carola Baumgardt and Jamie Callan, Johannes Kepler Life and Letters (1953), 13.
[Modern science] passed through a long period of uncertainty and inconclusive experiment, but as the instrumental aids to research improved, and the results of observation accumulated, phantoms of the imagination were exorcised, idols of the cave were shattered, trustworthy materials were obtained for logical treatment, and hypotheses by long and careful trial were converted into theories.
In The Present Relations of Science and Religion (1913, 2004), 3
[My uncle said to me…] When I read, forty years ago, that shells from Syria were found in the Alps, I say, I admit, with a slightly mocking tone, that these shells were apparently brought by pilgrims who were returning from Jerusalem. M. de Buffon reprimanded me very sharply in his Theory of the Earth, page 281. I did not want to quarrel with him over shells, but I remain of the same opinion, because the impossibility that the sea formed the mountains is evident to me. Some may tell me that porphyry is made of sea urchin spikes, I’ll believe it when I see white marble is made of ostrich feathers.
Voltaire is recounting one of the reasons his uncle (Abbe Ambroise Bazing) had told him, for disputing Buffon’s claim that the sea that made the mountains. Translated by Webmaster from 'La Défense de Mon Oncle: Des montagnes et des Coquilles', collected in Oeuvres complètes: Histoire.- Mélanges historiques (1817), Tome 15, Chap. 19, 133-134. From the original French; “Quand je lus, il y a quarante ans, qu’on avait trouvé dans les Alpes des coquilles de Syrie, je dis, je l’avoue , d’un ton un peu goguenard, que ces coquilles avaient été apparemment apportées par des pélerins qui revenaient de Jérusalem. M. de Buffon m’en reprit très-vertement dans sa Théorie de la terre, page 281. Je n’ai pas voulu me brouiller avec lui pour des coquilles, mais je suis demeuré dans non opinion, parce que l’impossibilité que la mer ait formé les montagnes m’est démontrée. On a beau me dire que le porphyre est fait de pointes d’oursin, je le croirai quand je verrai que le marbre blanc est fait de plumes d’autruche.” [Note: porphyry is a form of igneous rock. Also note, this passage does NOT appear in 'Les Singularités de la Nature', which may be found elsewhere incorrectly cited as the source. —Webmaster]
[Referring to Fourier’s mathematical theory of the conduction of heat] … Fourier's great mathematical poem…
In W. Thomson and P. G. Tait, Treatise on Natural Philosophy. Reprinted as Principles of Mechanics and Dynamics (2000), 470.
[The infinitely small] neither have nor can have theory; it is a dangerous instrument in the hands of beginners [ ... ] anticipating, for my part, the judgement of posterity, I would dare predict that this method will be accused one day, and rightly, of having retarded the progress of the mathematical sciences.
Annales des Mathematiques Pures et Appliquées (1814-5), 5, 148.
[The] structural theory is of extreme simplicity. It assumes that the molecule is held together by links between one atom and the next: that every kind of atom can form a definite small number of such links: that these can be single, double or triple: that the groups may take up any position possible by rotation round the line of a single but not round that of a double link: finally that with all the elements of the first short period [of the periodic table], and with many others as well, the angles between the valencies are approximately those formed by joining the centre of a regular tetrahedron to its angular points. No assumption whatever is made as to the mechanism of the linkage. Through the whole development of organic chemistry this theory has always proved capable of providing a different structure for every different compound that can be isolated. Among the hundreds of thousands of known substances, there are never more isomeric forms than the theory permits.
Presidential Address to the Chemical Society (16 Apr 1936), Journal of the Chemical Society (1936), 533.
[Theory is] an explanation that has been confirmed to such a degree, by observation and experiment, that knowledgeable experts accept it as fact. That’s what scientists mean when they talk about a theory: not a dreamy and unreliable speculation, but an explanatory statement that fits the evidence. They embrace such an explanation confidently but provisionally—taking it as their best available view of reality, at least until some severely conflicting data or some better explanation might come along.
In 'Was Darwin Wrong?', National Geographic (Nov 2004), 206, 4.
[In refutation of evolution] There is not enough evidence, consistent evidence to make it as fact, and I say that because for theory to become a fact, it needs to consistently have the same results after it goes through a series of tests. The tests that they put—that they use to support evolution do not have consistent results. Now too many people are blindly accepting evolution as fact. But when you get down to the hard evidence, it’s merely a theory.
[In favor of the teaching of creationism alongside evolution in schools.]
[In favor of the teaching of creationism alongside evolution in schools.]
From interview by Miles O'Brien on CNN (30 Mar 1996). Reported from transcript, via Nexis, in New York Magazine (15 Sep 2010).
Dilbert: It took weeks but I’ve calculated a new theory about the origin of the universe. According to my calculations it didn’t start with a “Big Bang” at all—it was more of “Phhbwt” sound. You may be wondering about the practical applications of the “Little Phhbwt” theory.
Dogbert: I was wondering when you’ll go away.
Dogbert: I was wondering when you’ll go away.
Dilbert comic strip (1 Jan 1993)
Dilbert: You joined the “Flat Earth Society?”
Dogbert: I believe the earth must be flat. There is no good evidence to support the so-called “round earth theory.”
Dilbert: I think Christopher Columbus would disagree.
Dogbert: How convenient that your best witness is dead.
Dogbert: I believe the earth must be flat. There is no good evidence to support the so-called “round earth theory.”
Dilbert: I think Christopher Columbus would disagree.
Dogbert: How convenient that your best witness is dead.
Dilbert comic strip (9 Oct 1989).
Discovery always carries an honorific connotation. It is the stamp of approval on a finding of lasting value. Many laws and theories have come and gone in the history of science, but they are not spoken of as discoveries. Kepler is said to have discovered the laws of planetary motion named after him, but no the many other 'laws' which he formulated. ... Theories are especially precarious, as this century profoundly testifies. World views can and do often change. Despite these difficulties, it is still true that to count as a discovery a finding must be of at least relatively permanent value, as shown by its inclusion in the generally accepted body of scientific knowledge.
Discovery in the Physical Sciences (1969). In Rodney P. Carlisle, Scientific American Inventions and Discoveries (2004), 179.
Dogbert: So, Since Columbus is dead, you have no evidence that the earth is round.
Dilbert: Look. You can Ask Senator John Glenn. He orbited the earth when he was an astronaut.
Dogbert: So, your theory depends on the honesty of politicians.
Dilbert: Yes... no, wait...
Dilbert: Look. You can Ask Senator John Glenn. He orbited the earth when he was an astronaut.
Dogbert: So, your theory depends on the honesty of politicians.
Dilbert: Yes... no, wait...
Dilbert comic strip (10 Oct 1989).
Drosophila melanogaster has been more extensively used in the study of genetics than any other organism, and the theory of heredity that is now generally accepted is based chiefly on the results obtained with this fly. … Not only has Drosophila been the most productive material for research in the subject, but it is now the standard object for laboratory instruction, and is used as such in many colleges and universities.
In The North American Species of Drosophila (1921), 12.
La chaleur pénètre, comme la gravité, toutes les substances de l’univers, ses rayons occupent toutes les parties de l’espace. Le but de notre ouvrage est d’exposer les lois mathématiques que suit cet élément. Cette théorie formera désormais une des branches les plus importantes de la physique générale.
Heat, like gravity, penetrates every substance of the universe, its rays occupy all parts of space. The object of our work is to set forth the mathematical laws which this element obeys. The theory of heat will hereafter form one of the most important branches of general physics.
Heat, like gravity, penetrates every substance of the universe, its rays occupy all parts of space. The object of our work is to set forth the mathematical laws which this element obeys. The theory of heat will hereafter form one of the most important branches of general physics.
From 'Discours Préliminaire' to Théorie Analytique de la Chaleur (1822), i, translated by Alexander Freeman in The Analytical Theory of Heat (1878), 1.
La théorie est l’hypothèse vérifiée, après qu’elle a été soumise au contrôle du raisonnement et de la critique expérimentale. La meilleure théorie est celle qui a été vérifiée par le plus grand nombre de faits. Mais une théorie, pour rester bonne, doit toujours se modifier avec les progrès de la science et demeurer constamment soumise à la vérification et à la critique des faits nouveaux qui apparaissent.
A theory is a verified hypothesis, after it has been submitted to the control of reason and experimental criticism. The soundest theory is one that has been verified by the greatest number of facts. But to remain valid, a theory must be continually altered to keep pace with the progress of science and must be constantly resubmitted to verification and criticism as new facts appear.
A theory is a verified hypothesis, after it has been submitted to the control of reason and experimental criticism. The soundest theory is one that has been verified by the greatest number of facts. But to remain valid, a theory must be continually altered to keep pace with the progress of science and must be constantly resubmitted to verification and criticism as new facts appear.
Original work in French, Introduction à l'Étude de la Médecine Expérimentale (1865), 385. English translation by Henry Copley Green in An Introduction to the Study of Experimental Medicine (1927, 1957), 220.
La théorie n’est que l’idée scientifique contrôlée par l’expérience.
A theory is merely a scientific idea controlled by experiment.
A theory is merely a scientific idea controlled by experiment.
Original work in French, Introduction à l'Étude de la Médecine Expérimentale (1865), 40. English translation by Henry Copley Green in An Introduction to the Study of Experimental Medicine (1927, 1957), 26.
Les médecins les plus savans en théorie sont rarement les plus habile practiciens.
The doctors most learned in theory are seldom the most skilled practitioners.
The doctors most learned in theory are seldom the most skilled practitioners.
Maxim No. 281 in Maximes, Réflexions et Pensées Diverses (1819), 236.
Nous avons l’obligation aux Anciens de nous avoir épuisé la plus grande partie des idées fausses qu’on le pouvait faire
We are under obligation to the ancients for having exhausted all the false theories that could be formed.
We are under obligation to the ancients for having exhausted all the false theories that could be formed.
In Digression sur les Anciens et les Modernes (1688), 165. Collected in Oeuvres Diverses (1727), Vol. 3, 139. English version as quoted in John Bagnell Bury, The Idea of Progress: An Inquiry Into Its Origin and Growth (1920), 104.
Ron Hutcheson, a Knight-Ridder reporter: [Mr. President, what are your] personal views [about the theory of] intelligent design?
President George W. Bush: [Laughing. You're] doing a fine job of dragging me back to the past [days as governor of Texas]. ... Then, I said that, first of all, that decision should be made to local school districts, but I felt like both sides ought to be properly taught...”
Hutcheson: Both sides ought to be properly taught?
President: Yes ... so people can understand what the debate is about.
Hutcheson: So the answer accepts the validity of “intelligent design” as an alternative to evolution?
President: I think that part of education is to expose people to different schools of thought, and I'm not suggesting—you're asking me whether or not people ought to be exposed to different ideas, and the answer is yes.
Hutcheson: So we've got to give these groups—...
President: [interrupting] Very interesting question, Hutch. [Laughter from other reporters]
President George W. Bush: [Laughing. You're] doing a fine job of dragging me back to the past [days as governor of Texas]. ... Then, I said that, first of all, that decision should be made to local school districts, but I felt like both sides ought to be properly taught...”
Hutcheson: Both sides ought to be properly taught?
President: Yes ... so people can understand what the debate is about.
Hutcheson: So the answer accepts the validity of “intelligent design” as an alternative to evolution?
President: I think that part of education is to expose people to different schools of thought, and I'm not suggesting—you're asking me whether or not people ought to be exposed to different ideas, and the answer is yes.
Hutcheson: So we've got to give these groups—...
President: [interrupting] Very interesting question, Hutch. [Laughter from other reporters]
From conversation with reporters at the White House (1 Aug 2005), as quoted by Matthew Cooper in 'Fanning the Controversy Over “Intelligent Design”', Time (3 Aug 2005). The Time writer stated, “The president has gone farther in questioning the widely-taught theories of evolution and natural selection than any president since Ronald Reagan, who advocated teaching creationism in public schools alongside evolution.” Just a few months later, in the nation's first case on that point, on 20 Dec 2005, “a federal judge [John E. Jones] ruled it was unconstitutional for a Pennsylvania school district to present intelligent design as an alternative in high school biology courses, because it is a religious viewpoint,” as reported by Laurie Goodstein in 'Judge Rejects Teaching Intelligent Design', New York Times (21 Dec 2005). Goodstein also wrote “Judge Jones, a Republican appointed by President Bush, concluded that intelligent design was not science,” and that “the evidence in the trial proved that intelligent design was 'creationism relabeled.' The Supreme Court has already ruled that creationism ... cannot be taught as science in a public school.”
Theories thus become instruments, not answers to enigmas, in which we can rest. We don’t lie back upon them, we move forward, and, on occasion, make nature over again by their aid.
Pragmatism, a New Name for Some Old Ways of Thinking: Popular Lectures on Philosophy (1907), 53.
To Wheeler's comment, If you haven't found something strange during the day, it hasn't been much of a day, a student responded, I can't believe that space is that crummy. Wheeler replied: To disagree leads to study, to study leads to understanding, to understand is to appreciate, to appreciate is to love. So maybe I'll end up loving your theory.
Quoted in Charles Birch, Biology and the Riddle of Life (1999), 10.
Une même expression, dont les géomètres avaient considéré les propriétés abstraites, … représente'aussi le mouvement de la lumière dans l’atmosphère, quelle détermine les lois de la diffusion de la chaleur dans la matière solide, et quelle entre dans toutes les questions principales de la théorie des probabilités.
The same expression whose abstract properties geometers had considered … represents as well the motion of light in the atmosphere, as it determines the laws of diffusion of heat in solid matter, and enters into all the chief problems of the theory of probability.
The same expression whose abstract properties geometers had considered … represents as well the motion of light in the atmosphere, as it determines the laws of diffusion of heat in solid matter, and enters into all the chief problems of the theory of probability.
From Théorie Analytique de la Chaleur (1822), translated by Alexander Freeman in The Analytical Theory of Heat (1878), 7.
Von Theorie wild man nicht heller.
Gott geb' täglich unsern Teller.
When theory's light is less than stellar.
Give us, O Lord, our daily Teller.
This rhyme from an alphabet ditty describing various physicists was written for a party at Göttingen.
Gott geb' täglich unsern Teller.
When theory's light is less than stellar.
Give us, O Lord, our daily Teller.
This rhyme from an alphabet ditty describing various physicists was written for a party at Göttingen.
Quoted in Edward Teller with Judith L. Shoolery, Memoirs: A Twentieth-Century Journey in Science and Politics (2001), 75. The first clause, translated more literally than poetically, is given as, 'When theory does not enlighten us,' in Nina Byers and Gary Williams, Out of the Shadows (2006),130.
~~[Attributed without source]~~ A theory that you can’t explain to a bartender is probably no damn good.
Variant “…explain to a cleaning lady….” Often seen, virally spread, but always without a source citation. If you can provide a primary source, please contact Webmaster. Until then the quote should be regarded as not authenticated.
~~[Attributed]~~ That theory is worthless. It isn’t even wrong!
Probably a feral variant of “This isn’t right. This isn’t even wrong,” which can only be cited as “Attributed,” since there is no known primary source.
~~[No known source]~~ Later generations will regard Mengenlehre [set theory] as a disease from which one has recovered.
Webmaster finds zero support for the authenticity of this quote. Speculations on how it may have evolved include reference to a beau cas pathologique (a beautiful pathological case) in a very different quote which begins “I think, and I am not the only one…”. (See the Henri Poincaré Quotes web page of this site.) More details readily available with a Google search (for example): Did Poincaré say “set theory is a disease”. The quote is included here to provide this caution.
~~[Orphan]~~ If the facts don’t fit the theory, change the facts.
Although in recent years seen attributed to Einstein, there seems to be no authentic source. So treat it as by “Anonymous”. Included here under Einstein to link it to this warning. Compare with, “If the facts do not agree with your theory … then so much the worse for the facts.” See quote beginning, “An enthusiastic philosopher…” on the Charles Mackay Quotes page on this website.
~~[Orphan]~~ The truth of a theory is in your mind, not in your eyes.
Attributed, without citation, in Howard W. Eves Mathematical Circles Squared (1972), 57. Webmaster has so far not been able to verify from a primary source. Can you help?
~~[Unverified]~~ [Louis Pasteur’s] … theory of germs is a ridiculous fiction. How do you think that these germs in the air can be numerous enough to develop into all these organic infusions? If that were true, they would be numerous enough to form a thick fog, as dense as iron.
Webmaster has not yet been able to verify this quote - and is very suspicious of it - but includes it to provide this cautionary note. It appears in several books, such as Rob Kaplan, Science Says (2001), 201-202, which cites “Professor of Physiology at Toulouse, The Universe: The Infinitely Great and the Infinitely Small (1872).” Webmaster has not yet found any information on Pierre Pochet as a 19th-century scientist, anywhere. A similar book title was found as The Universe: The Infinitely Great and the Infinitely Little (1870) but that author is Félix-Archimède Pochet, Director of the Natural History Museum at Rouen. This book, translated from the French, presents germs in the normal way, with no trace of the subject quote to be found in it. The Preface (dated 1867) begins, “My sole object in writing this work was…” implying it was totally authored by F.A. Pochet with no reference to taking over from earlier work by another person. It does, however, have this curious statement: “One of my learned colleagues at the Academy of Sciences lately brought out a similar work, but under a fictitious name,” which F.A. Pochet thought was a questionable practice, and did not provide any name of the individual involved. If you find more definitive information, please contact Webmaster.
1839—The fermentation satire
THE MYSTERY OF ALCOHOLIC FERMENTATION RESOLVED
(Preliminary Report by Letter) Schwindler
I am about to develop a new theory of wine fermentation … Depending on the weight, these seeds carry fermentation to completion somewhat less than as in the beginning, which is understandable … I shall develop a new theory of wine fermentation [showing] what simple means Nature employs in creating the most amazing phenomena. I owe it to the use of an excellent microscope designed by Pistorius.
When brewer’s yeast is mixed with water the microscope reveals that the yeast dissolves into endless small balls, which are scarcely 1/800th of a line in diameter … If these small balls are placed in sugar water, it can be seen that they consist of the eggs of animals. As they expand, they burst, and from them develop small creatures that multiply with unbelievable rapidity in a most unheard of way. The form of these animals differs from all of the 600 types described up until now. They possess the shape of a Beinsdorff still (without the cooling apparatus). The head of the tube is a sort of proboscis, the inside of which is filled with fine bristles 1/2000th of a line long. Teeth and eyes are not discernible; however, a stomach, intestinal canal, anus (a rose red dot), and organs for secretion of urine are plainly discernible. From the moment they are released from the egg one can see these animals swallow the sugar from the solution and pass it to the stomach. It is digested immediately, a process recognized easily by the resultant evacuation of excrements. In a word, these infusors eat sugar, evacuate ethyl alcohol from the intestinal canal, and carbon dioxide from the urinary organs. The bladder, in the filled state, has the form of a champagne bottle; when empty, it is a small button … As soon as the animals find no more sugar present, they eat each other up, which occurs through a peculiar manipulation; everything is digested down to the eggs which pass unchanged through the intestinal canal. Finally, one again fermentable yeast, namely the seed of the animals, which remain over.
THE MYSTERY OF ALCOHOLIC FERMENTATION RESOLVED
(Preliminary Report by Letter) Schwindler
I am about to develop a new theory of wine fermentation … Depending on the weight, these seeds carry fermentation to completion somewhat less than as in the beginning, which is understandable … I shall develop a new theory of wine fermentation [showing] what simple means Nature employs in creating the most amazing phenomena. I owe it to the use of an excellent microscope designed by Pistorius.
When brewer’s yeast is mixed with water the microscope reveals that the yeast dissolves into endless small balls, which are scarcely 1/800th of a line in diameter … If these small balls are placed in sugar water, it can be seen that they consist of the eggs of animals. As they expand, they burst, and from them develop small creatures that multiply with unbelievable rapidity in a most unheard of way. The form of these animals differs from all of the 600 types described up until now. They possess the shape of a Beinsdorff still (without the cooling apparatus). The head of the tube is a sort of proboscis, the inside of which is filled with fine bristles 1/2000th of a line long. Teeth and eyes are not discernible; however, a stomach, intestinal canal, anus (a rose red dot), and organs for secretion of urine are plainly discernible. From the moment they are released from the egg one can see these animals swallow the sugar from the solution and pass it to the stomach. It is digested immediately, a process recognized easily by the resultant evacuation of excrements. In a word, these infusors eat sugar, evacuate ethyl alcohol from the intestinal canal, and carbon dioxide from the urinary organs. The bladder, in the filled state, has the form of a champagne bottle; when empty, it is a small button … As soon as the animals find no more sugar present, they eat each other up, which occurs through a peculiar manipulation; everything is digested down to the eggs which pass unchanged through the intestinal canal. Finally, one again fermentable yeast, namely the seed of the animals, which remain over.
In 'Das entriithselle Geheimiss der geisligen Giihrung', Annalen der Pharmacie und Chemie (1839), 29, 100-104; adapted from English translalion by Ralph E. Oesper, The Human Side of Scientists (1975), 203-205.
A … difference between most system-building in the social sciences and systems of thought and classification of the natural sciences is to be seen in their evolution. In the natural sciences both theories and descriptive systems grow by adaptation to the increasing knowledge and experience of the scientists. In the social sciences, systems often issue fully formed from the mind of one man. Then they may be much discussed if they attract attention, but progressive adaptive modification as a result of the concerted efforts of great numbers of men is rare.
The Study of Man (1941), 19-20.
A casual glance at crystals may lead to the idea that they were pure sports of nature, but this is simply an elegant way of declaring one’s ignorance. With a thoughtful examination of them, we discover laws of arrangement. With the help of these, calculation portrays and links up the observed results. How variable and at the same time how precise and regular are these laws! How simple they are ordinarily, without losing anything of their significance! The theory which has served to develop these laws is based entirely on a fact, whose existence has hitherto been vaguely discerned rather than demonstrated. This fact is that in all minerals which belong to the same species, these little solids, which are the crystal elements and which I call their integrant molecules, have an invariable form, in which the faces lie in the direction of the natural fracture surfaces corresponding to the mechanical division of the crystals. Their angles and dimensions are derived from calculations combined with observation.
Traité de mineralogie … Publié par le conseil des mines (1801), Vol. 1, xiii-iv, trans. Albert V. and Marguerite Carozzi.
A closer look at the course followed by developing theory reveals for a start that it is by no means as continuous as one might expect, but full of breaks and at least apparently not along the shortest logical path. Certain methods often afforded the most handsome results only the other day, and many might well have thought that the development of science to infinity would consist in no more than their constant application. Instead, on the contrary, they suddenly reveal themselves as exhausted and the attempt is made to find other quite disparate methods. In that event there may develop a struggle between the followers of the old methods and those of the newer ones. The former's point of view will be termed by their opponents as out-dated and outworn, while its holders in turn belittle the innovators as corrupters of true classical science.
In 'On the Development of the Methods of Theoretical Physics in Recent Times', Populäre Schriften, Essay 14. Address (22 Sep 1899) to the Meeting of Natural Scientists at Munich. Collected in Brian McGuinness (ed.), Ludwig Boltzmann: Theoretical Physics and Philosophical Problems, Selected Writings (1974), 79.
A complete theory of evolution must acknowledge a balance between ‘external’ forces of environment imposing selection for local adaptation and ‘internal’ forces representing constraints of inheritance and development. Vavilov placed too much emphasis on internal constraints and downgraded the power of selection. But Western Darwinians have erred equally in practically ignoring (while acknowledging in theory) the limits placed on selection by structure and development–what Vavilov and the older biologists would have called ‘laws of form.’
…...
A conceptual scheme is never discarded merely because of a few stubborn facts with which it cannot be reconciled; a conceptual scheme is either modified or replaced by a better one, never abandoned with nothing left to take its place.
Science and Common Sense (1951), 173.
A discovery in science, or a new theory, even when it appears most unitary and most all-embracing, deals with some immediate element of novelty or paradox within the framework of far vaster, unanalysed, unarticulated reserves of knowledge, experience, faith, and presupposition. Our progress is narrow; it takes a vast world unchallenged and for granted. This is one reason why, however great the novelty or scope of new discovery, we neither can, nor need, rebuild the house of the mind very rapidly. This is one reason why science, for all its revolutions, is conservative. This is why we will have to accept the fact that no one of us really will ever know very much. This is why we shall have to find comfort in the fact that, taken together, we know more and more.
Science and the Common Understanding (1954), 53-4.
A discovery is generally an unforeseen relation not included in theory.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 38.
A distinguished writer [Siméon Denis Poisson] has thus stated the fundamental definitions of the science:
“The probability of an event is the reason we have to believe that it has taken place, or that it will take place.”
“The measure of the probability of an event is the ratio of the number of cases favourable to that event, to the total number of cases favourable or contrary, and all equally possible” (equally like to happen).
From these definitions it follows that the word probability, in its mathematical acceptation, has reference to the state of our knowledge of the circumstances under which an event may happen or fail. With the degree of information which we possess concerning the circumstances of an event, the reason we have to think that it will occur, or, to use a single term, our expectation of it, will vary. Probability is expectation founded upon partial knowledge. A perfect acquaintance with all the circumstances affecting the occurrence of an event would change expectation into certainty, and leave neither room nor demand for a theory of probabilities.
“The probability of an event is the reason we have to believe that it has taken place, or that it will take place.”
“The measure of the probability of an event is the ratio of the number of cases favourable to that event, to the total number of cases favourable or contrary, and all equally possible” (equally like to happen).
From these definitions it follows that the word probability, in its mathematical acceptation, has reference to the state of our knowledge of the circumstances under which an event may happen or fail. With the degree of information which we possess concerning the circumstances of an event, the reason we have to think that it will occur, or, to use a single term, our expectation of it, will vary. Probability is expectation founded upon partial knowledge. A perfect acquaintance with all the circumstances affecting the occurrence of an event would change expectation into certainty, and leave neither room nor demand for a theory of probabilities.
An Investigation of the Laws of Thought (1854), 243-244. The Poisson quote is footnoted as from Recherches sur la Probabilité des Jugemens.
A formative influence on my undergraduate self was the response of a respected elder statesmen of the Oxford Zoology Department when an American visitor had just publicly disproved his favourite theory. The old man strode to the front of the lecture hall, shook the American warmly by the hand and declared in ringing, emotional tones: “My dear fellow, I wish to thank you. I have been wrong these fifteen years.” And we clapped our hands red. Can you imagine a Government Minister being cheered in the House of Commons for a similar admission? “Resign, Resign” is a much more likely response!
From a talk, 'Science, Delusion and the Appetite for Wonder', (21 Dec 1996), published on edge.org website.
A good theoretical physicist today might find it useful to have a wide range of physical viewpoints and mathematical expressions of the same theory (for example, of quantum electrodynamics) available to him. This may be asking too much of one man. Then new students should as a class have this. If every individual student follows the same current fashion in expressing and thinking about electrodynamics or field theory, then the variety of hypotheses being generated to understand strong interactions, say, is limited. Perhaps rightly so, for possibly the chance is high that the truth lies in the fashionable direction. But, on the off-chance that it is in another direction—a direction obvious from an unfashionable view of field theory—who will find it?
In his Nobel Prize Lecture (11 Dec 1965), 'The Development of the Space-Time View of Quantum Electrodynamics'. Collected in Stig Lundqvist, Nobel Lectures: Physics, 1963-1970 (1998), 177.
A man who is all theory is like “a rudderless ship on a shoreless sea.” … Theories and speculations may be indulged in with safety only as long as they are based on facts that we can go back to at all times and know that we are on solid ground.
In Nature's Miracles: Familiar Talks on Science (1899), Vol. 1, Introduction, vii.
A mathematical theory is not to be considered complete until you have made it so clear that you can explain it to the first man whom you meet on the street.
…...
A mere inference or theory must give way to a truth revealed; but a scientific truth must be maintained, however contradictory it may appear to the most cherished doctrines of religion.
More Worlds Than One: The Creed of the Philosopher and the Hope of the Christian (1856), 132.
A metaphysical conclusion is either a false conclusion or a concealed experimental conclusion.
'On Thought in Medicine' (1877). Trans. E. Atkinson, Popular Lectures on Scientific Subjects (1881), 234.
A moment’s consideration of this case shows what a really great advance in the theory and practise of breeding has been obtained through the discovery of Mendel’s law. What a puzzle this case would have presented to the biologist ten years ago! Agouti crossed with chocolate gives in the second filial generation (not in the first) four varieties, viz., agouti, chocolate, black and cinnamon. We could only have shaken our heads and looked wise (or skeptical).
Then we had no explanation to offer for such occurrences other than the “instability of color characters under domestication,” the “effects of inbreeding,” “maternal impressions.” Serious consideration would have been given to the proximity of cages containing both black and cinnamon-agouti mice.
Now we have a simple, rational explanation, which anyone can put to the test. We are able to predict the production of new varieties, and to produce them.
We must not, of course, in our exuberance, conclude that the powers of the hybridizer know no limits. The result under consideration consists, after all, only in the making of new combinations of unit characters, but it is much to know that these units exist and that all conceivable combinations of them are ordinarily capable of production. This valuable knowledge we owe to the discoverer and to the rediscoverers of Mendel’s law.
Then we had no explanation to offer for such occurrences other than the “instability of color characters under domestication,” the “effects of inbreeding,” “maternal impressions.” Serious consideration would have been given to the proximity of cages containing both black and cinnamon-agouti mice.
Now we have a simple, rational explanation, which anyone can put to the test. We are able to predict the production of new varieties, and to produce them.
We must not, of course, in our exuberance, conclude that the powers of the hybridizer know no limits. The result under consideration consists, after all, only in the making of new combinations of unit characters, but it is much to know that these units exist and that all conceivable combinations of them are ordinarily capable of production. This valuable knowledge we owe to the discoverer and to the rediscoverers of Mendel’s law.
'New Colour Variety of the Guinea Pig', Science, 1908, 28, 250-252.
A new theory is guilty until proven innocent, and the pre-existing theory innocent until proven guilty ... Continental drift was guilty until proven innocent.
The Nemesis Affair: A Story of the Death of the Dinosaurs and the Ways of Science (1986), 195-205.
A physical theory remains an empty shell until we have found a reasonable physical interpretation.
At the 4th Soviet Gravitational Conference, Minsk, USSR (Jul 1976). Quoted in Anton Z. Capri, From Quanta to Quarks: More Anecdotal History of Physics (2007), 120.
A principle of induction would be a statement with the help of which we could put inductive inferences into a logically acceptable form. In the eyes of the upholders of inductive logic, a principle of induction is of supreme importance for scientific method: “... this principle”, says Reichenbach, “determines the truth of scientific theories. To eliminate it from science would mean nothing less than to deprive science of the power to decide the truth or falsity of its theories. Without it, clearly, science would no longer have the right to distinguish its theories from the fanciful and arbitrary creations of the poet’s mind.” Now this principle of induction cannot be a purely logical truth like a tautology or an analytic statement. Indeed, if there were such a thing as a purely logical principle of induction, there would be no problem of induction; for in this case, all inductive inferences would have to be regarded as purely logical or tautological transformations, just like inferences in inductive logic. Thus the principle of induction must be a synthetic statement; that is, a statement whose negation is not self-contradictory but logically possible. So the question arises why such a principle should be accepted at all, and how we can justify its acceptance on rational grounds.
…...
A scientifically unimportant discovery is one which, however true and however interesting for other reasons, has no consequences for a system of theory with which scientists in that field are concerned.
The Structure of Social Action (1937), Vol. 1, 7.
A sound Physics of the Earth should include all the primary considerations of the earth's atmosphere, of the characteristics and continual changes of the earth's external crust, and finally of the origin and development of living organisms. These considerations naturally divide the physics of the earth into three essential parts, the first being a theory of the atmosphere, or Meteorology, the second, a theory of the earth's external crust, or Hydrogeology, and the third, a theory of living organisms, or Biology.
Hydrogéologie (1802), trans. A. V. Carozzi (1964), 18.
A story about the Jack Spratts of medicine [was] told recently by Dr. Charles H. Best, co-discoverer of insulin. He had been invited to a conference of heart specialists in North America. On the eve of the meeting, out of respect for the fat-clogs-the-arteries theory, the delegates sat down to a special banquet served without fats. It was unpalatable but they all ate it as a duty. Next morning Best looked round the breakfast room and saw these same specialists—all in the 40-60 year old, coronary age group—happily tucking into eggs, bacon, buttered toast and coffee with cream.
'Objections To High-Fat Diets', Eat Fat And Grow Slim (1958), Ch. 3.
A taxonomy of abilities, like a taxonomy anywhere else in science, is apt to strike a certain type of impatient student as a gratuitous orgy of pedantry. Doubtless, compulsions to intellectual tidiness express themselves prematurely at times, and excessively at others, but a good descriptive taxonomy, as Darwin found in developing his theory, and as Newton found in the work of Kepler, is the mother of laws and theories.
From Intelligence: Its Structure, Growth and Action: Its Structure, Growth and Action (1987), 61.
A theory can be proved by experiment; but no path leads from experiment to the birth of a theory.
As quoted in Antonina Vallentin, Einstein: A Biography (1954), 105. The author,
a close friend of Einstein’s family, cites the quote only as “which he has recently made public.”
A theory has only the alternative of being right or wrong. A model has a third possibility: it may be right, but irrelevant.
Manfred Eigen, 'The Origin of Biological Information', in Jagdish Mehra (ed.), The Physicists's Conception of Nature (1973), 618.
A theory is a supposition which we hope to be true, a hypothesis is a supposition which we expect to be useful; fictions belong to the realm of art; if made to intrude elsewhere, they become either make-believes or mistakes.
As quoted by William Ramsay, in 'Radium and Its Products', Harper’s Magazine (Dec 1904), 52. The first part, about suppositions, appears in a paper read by G. Johnson Stoney to the American Philosophical Society, Philadelphia (3 Apr 1903), printed in 'On the Dependence of What Apparently Takes Place in Nature Upon What Actually Occurs in the Universe of Real Existences', Proceedings of the American Philosophical Society Held at Philadelphia for Promoting Useful Knowledge (Apr-May 1903) 42, No. 173, 107. If you know a primary source for the part on fictions and mistakes, please contact Webmaster.
A theory is scientific only if it can be disproved. But the moment you try to cover absolutely everything the chances are that you cover nothing.
From Assumption and Myth in Physical Theory (1967), 12.
A theory is the more impressive the greater the simplicity of its premises is, the more different kinds of things it relates, and the more extended is its area of applicability. Therefore the deep impression which classical thermodynamics made upon me. It is the only physical theory of universal content concerning which I am convinced that within the framework of the applicability of its basic concepts, it will never be overthrown.
Autobiographical Notes (1946), 33. Quoted in Gerald Holton and Yehuda Elkana, Albert Einstein: Historical and Cultural Perspectives (1997), 227.
A theory of physics is not an explanation; it is a system of mathematical oppositions deduced from a small number of principles the aim of which is to represent as simply, as completely, and as exactly as possible, a group of experimental laws.
As quoted in Philipp Frank, Modern Science and its Philosophy (1949), 15, which cites Théorie Physique; Son Objet—Son Structure (1906), 24.
A theory which cannot be mortally endangered cannot be alive.
Personal communication quoted by John R. Platt in 'Science, Strong Inference', Science (16 Oct 1964), 146, No. 3642, 349.
A theory with mathematical beauty is more likely to be correct than an ugly one that fits some experimental data. God is a mathematician of a very high order, and He used very advanced mathematics in constructing the universe.
In Scientific American (May 1963). As quoted and cited in The Hutchinson Encyclopedia of Science (1998), 468.
About ten months ago [1609] a report reached my ears that a certain Fleming [Hans Lippershey] had constructed a spyglass, by means of which visible objects, though very distant from the eye of the observer, were distinctly seen as if nearby... Of this truly remarkable effect several experiences were related, to which some persons gave credence while others denied them. A few days later the report was confirmed to me in a letter from a noble Frenchman at Paris, Jacques Badovere, which caused me to apply myself wholeheartedly to enquire into the means by which I might arrive at the invention of a similar instrument. This I did shortly afterwards, my basis being the theory of refraction. First I prepared a tube of lead, at the ends of which I fitted two glass lenses, both plane on one side while on the other side one was spherically convex and the other concave.
The Starry Messenger (1610), trans. Stillman Drake, Discoveries and Opinions of Galileo (1957), 28-9.
About thirty years ago there was much talk that geologists ought only to observe and not theorise; and I well remember some one saying that at this rate a man might as well go into a gravel-pit and count the pebbles and describe the colours. How odd it is that anyone should not see that all observation must be for or against some view if it is to be of any service!
Letter to Henry Fawcett (18 Sep 1861). In Charles Darwin, Francis Darwin, Albert Charles Seward, More Letters of Charles Darwin (1903), Vol. 1, 195.
According to the estimate of a prominent advertising firm, above 90 per cent, of the earning capacity of the prominent nostrums is represented by their advertising. And all this advertising is based on the well-proven theory of the public's pitiable ignorance and gullibility in the vitally important matter of health.
'The Fundamental Fakes', Collier's Weekly (17 Feb 1906). Reprinted in The Great American Fraud (1907), 55.
According to the theory of aerodynamics, as may be readily demonstrated through wind tunnel experiments, the bumblebee is unable to fly. This is because the size, weight and shape of his body in relation to the total wingspread make flying impossible. But the bumblebee, being ignorant of these scientific truths, goes ahead and flies anyway—and makes a little honey every day.
Sign in a General Motors Corporation factory. As quoted in Ralph L. Woods, The Businessman's
Book of Quotations (1951), 249-50. Cited in Suzy Platt (ed)., Respectfully Quoted: A Dictionary of Quotations (1989), 118.
After … the general experimental knowledge has been acquired, accompanied with just a sufficient amount of theory to connect it together…, it becomes possible to consider the theory by itself, as theory. The experimental facts then go out of sight, in a great measure, not because they are unimportant, but because … they are fundamental, and the foundations are always hidden from view in well-constructed buildings.
In Electromagnetic Theory (1892), Vol. 2, 1.
After the discovery of spectral analysis no one trained in physics could doubt the problem of the atom would be solved when physicists had learned to understand the language of spectra. So manifold was the enormous amount of material that has been accumulated in sixty years of spectroscopic research that it seemed at first beyond the possibility of disentanglement. An almost greater enlightenment has resulted from the seven years of Röntgen spectroscopy, inasmuch as it has attacked the problem of the atom at its very root, and illuminates the interior. What we are nowadays hearing of the language of spectra is a true 'music of the spheres' in order and harmony that becomes ever more perfect in spite of the manifold variety. The theory of spectral lines will bear the name of Bohr for all time. But yet another name will be permanently associated with it, that of Planck. All integral laws of spectral lines and of atomic theory spring originally from the quantum theory. It is the mysterious organon on which Nature plays her music of the spectra, and according to the rhythm of which she regulates the structure of the atoms and nuclei.
Atombau und Spektrallinien (1919), viii, Atomic Structure and Spectral Lines, trans. Henry L. Brose (1923), viii.
All change is relative. The universe is expanding relatively to our common material standards; our material standards are shrinking relatively to the size of the universe. The theory of the “expanding universe” might also be called the theory of the “shrinking atom”. …
:Let us then take the whole universe as our standard of constancy, and adopt the view of a cosmic being whose body is composed of intergalactic spaces and swells as they swell. Or rather we must now say it keeps the same size, for he will not admit that it is he who has changed. Watching us for a few thousand million years, he sees us shrinking; atoms, animals, planets, even the galaxies, all shrink alike; only the intergalactic spaces remain the same. The earth spirals round the sun in an ever-decreasing orbit. It would be absurd to treat its changing revolution as a constant unit of time. The cosmic being will naturally relate his units of length and time so that the velocity of light remains constant. Our years will then decrease in geometrical progression in the cosmic scale of time. On that scale man’s life is becoming briefer; his threescore years and ten are an ever-decreasing allowance. Owing to the property of geometrical progressions an infinite number of our years will add up to a finite cosmic time; so that what we should call the end of eternity is an ordinary finite date in the cosmic calendar. But on that date the universe has expanded to infinity in our reckoning, and we have shrunk to nothing in the reckoning of the cosmic being.
We walk the stage of life, performers of a drama for the benefit of the cosmic spectator. As the scenes proceed he notices that the actors are growing smaller and the action quicker. When the last act opens the curtain rises on midget actors rushing through their parts at frantic speed. Smaller and smaller. Faster and faster. One last microscopic blurr of intense agitation. And then nothing.
:Let us then take the whole universe as our standard of constancy, and adopt the view of a cosmic being whose body is composed of intergalactic spaces and swells as they swell. Or rather we must now say it keeps the same size, for he will not admit that it is he who has changed. Watching us for a few thousand million years, he sees us shrinking; atoms, animals, planets, even the galaxies, all shrink alike; only the intergalactic spaces remain the same. The earth spirals round the sun in an ever-decreasing orbit. It would be absurd to treat its changing revolution as a constant unit of time. The cosmic being will naturally relate his units of length and time so that the velocity of light remains constant. Our years will then decrease in geometrical progression in the cosmic scale of time. On that scale man’s life is becoming briefer; his threescore years and ten are an ever-decreasing allowance. Owing to the property of geometrical progressions an infinite number of our years will add up to a finite cosmic time; so that what we should call the end of eternity is an ordinary finite date in the cosmic calendar. But on that date the universe has expanded to infinity in our reckoning, and we have shrunk to nothing in the reckoning of the cosmic being.
We walk the stage of life, performers of a drama for the benefit of the cosmic spectator. As the scenes proceed he notices that the actors are growing smaller and the action quicker. When the last act opens the curtain rises on midget actors rushing through their parts at frantic speed. Smaller and smaller. Faster and faster. One last microscopic blurr of intense agitation. And then nothing.
In The Expanding Universe (1933) , 90-92.
All good intellects have repeated, since Bacon’s time, that there can be no real knowledge but that which is based on observed facts. This is incontestable, in our present advanced stage; but, if we look back to the primitive stage of human knowledge, we shall see that it must have been otherwise then. If it is true that every theory must be based upon observed facts, it is equally true that facts cannot be observed without the guidance of some theory. Without such guidance, our facts would be desultory and fruitless; we could not retain them: for the most part we could not even perceive them.
The Positive Philosophy, trans. Harriet Martineau (1853), Vol. 1, 3-4.
All interesting issues in natural history are questions of relative frequency, not single examples. Everything happens once amidst the richness of nature. But when an unanticipated phenomenon occurs again and again–finally turning into an expectation–then theories are overturned.
…...
All science has one aim, namely, to find a theory of nature.
In Nature (1849), 2.
All scientific theories are provisional and may be changed, but ... on the whole, they are accepted from Washington to Moscow because of their practical success. Where religion has opposed the findings of science, it has almost always had to retreat.
Essay 'Science Will Never Give Us the Answers to All Our Questions', collected in Henry Margenau, and Roy Abraham Varghese (eds.), Cosmos, Bios, Theos (1992), 65.
All that stuff I was taught about evolution, embryology, Big Bang theory, all that is lies straight from the pit of hell. It’s lies to try to keep me and all the folks who are taught that from understanding that they need a savior.
[Revealing his anti-science views, contrary to the qualifications needed to make important public policy on matters of science.]
[Revealing his anti-science views, contrary to the qualifications needed to make important public policy on matters of science.]
From speech (27 Sep 2012) to a sportman’s banquet at Liberty Baptist Church, Hartwell, Georgia, as quoted in Matt Pearce, 'U.S. Rep. Paul Broun: Evolution a lie ‘from the pit of hell’', Los Angeles Times (7 Oct 2012).
Almost everyone... seems to be quite sure that the differences between the methodologies of history and of the natural sciences are vast. For, we are assured, it is well known that in the natural sciences we start from observation and proceed by induction to theory. And is it not obvious that in history we proceed very differently? Yes, I agree that we proceed very differently. But we do so in the natural sciences as well.
In both we start from myths—from traditional prejudices, beset with error—and from these we proceed by criticism: by the critical elimination of errors. In both the role of evidence is, in the main, to correct our mistakes, our prejudices, our tentative theories—that is, to play a part in the critical discussion, in the elimination of error. By correcting our mistakes, we raise new problems. And in order to solve these problems, we invent conjectures, that is, tentative theories, which we submit to critical discussion, directed towards the elimination of error.
In both we start from myths—from traditional prejudices, beset with error—and from these we proceed by criticism: by the critical elimination of errors. In both the role of evidence is, in the main, to correct our mistakes, our prejudices, our tentative theories—that is, to play a part in the critical discussion, in the elimination of error. By correcting our mistakes, we raise new problems. And in order to solve these problems, we invent conjectures, that is, tentative theories, which we submit to critical discussion, directed towards the elimination of error.
The Myth of the Framework: In Defence of Science and Rationality (1993), 140.
Among the current discussions, the impact of new and sophisticated methods in the study of the past occupies an important place. The new 'scientific' or 'cliometric' history—born of the marriage contracted between historical problems and advanced statistical analysis, with economic theory as bridesmaid and the computer as best man—has made tremendous advances in the last generation.
Co-author with Geoffrey Rudolph Elton (1921-94), British historian. Which Road to the Past? Two Views of History (1983), 2.
An announcement of [Christopher] Zeeman’s lecture at Northwestern University in the spring of 1977 contains a quote describing catastrophe theory as the most important development in mathematics since the invention of calculus 300 years ago.
In book review of Catastrophe Theory: Collected Papers, 1972-1977, in Bulletin of the American Mathematical Society (Nov 1978), 84, No. 6, 1360. Reprinted in Stephen Smale, Roderick Wong(ed.), The Collected Papers of Stephen Smale (2000), Vol. 2, 814.
An enthusiastic philosopher, of whose name we are not informed, had constructed a very satisfactory theory on some subject or other, and was not a little proud of it. “But the facts, my dear fellow,” said his friend, “the facts do not agree with your theory.”—“Don't they?” replied the philosopher, shrugging his shoulders, “then, tant pis pour les faits;”—so much the worse for the facts!
From Memoirs of Extraordinary Popular Delusions (1841), Vol. 3, 313, footnote.
An old French geometer used to say that a mathematical theory was never to be considered complete till you had made it so clear that you could explain it to the first man you met in the street.
In Nature (1873), 8, 458.
And yet I think that the Full House model does teach us to treasure variety for its own sake–for tough reasons of evolutionary theory and nature’s ontology, and not from a lamentable failure of thought that accepts all beliefs on the absurd rationale that disagreement must imply disrespect. Excellence is a range of differences, not a spot. Each location on the range can be occupied by an excellent or an inadequate representative– and we must struggle for excellence at each of these varied locations. In a society driven, of ten unconsciously, to impose a uniform mediocrity upon a former richness of excellence–where McDonald’s drives out the local diner, and the mega-Stop & Shop eliminates the corner Mom and Pop–an understanding and defense of full ranges as natural reality might help to stem the tide and preserve the rich raw material of any evolving system: variation itself.
…...
Anthropology has reached that point of development where the careful investigation of facts shakes our firm belief in the far-reaching theories that have been built up. The complexity of each phenomenon dawns on our minds, and makes us desirous of proceeding more cautiously. Heretofore we have seen the features common to all human thought. Now we begin to see their differences. We recognize that these are no less important than their similarities, and the value of detailed studies becomes apparent. Our aim has not changed, but our method must change. We are still searching for the laws that govern the growth of human culture, of human thought; but we recognize the fact that before we seek for what is common to all culture, we must analyze each culture by careful and exact methods, as the geologist analyzes the succession and order of deposits, as the biologist examines the forms of living matter. We see that the growth of human culture manifests itself in the growth of each special culture. Thus we have come to understand that before we can build up the theory of the growth of all human culture, we must know the growth of cultures that we find here and there among the most primitive tribes of the Arctic, of the deserts of Australia, and of the impenetrable forests of South America; and the progress of the civilization of antiquity and of our own times. We must, so far as we can, reconstruct the actual history of mankind, before we can hope to discover the laws underlying that history.
The Jesup North Pacific Expedition: Memoir of the American Museum of Natural History (1898), Vol. 1, 4.
Any fundamental theory of physics is beautiful. If it isn’t, it’s probably wrong.
In the University of Toronto Bulletin, May 5, 1986.
Any one whose disposition leads him to attach more weight to unexplained difficulties than to the explanation of facts will certainly reject my theory.
The Origin of Species (1859), 482.
Any theory is better than no theory.
In Barry G. Firkin, Judith A. Whitworth, Dictionary of Medical Eponyms (1996), 5.
Anybody who looks at living organisms knows perfectly well that they can produce other organisms like themselves. This is their normal function, they wouldn’t exist if they didn’t do this, and it’s not plausible that this is the reason why they abound in the world. In other words, living organisms are very complicated aggregations of elementary parts, and by any reasonable theory of probability or thermodynamics highly improbable. That they should occur in the world at all is a miracle of the first magnitude; the only thing which removes, or mitigates, this miracle is that they reproduce themselves. Therefore, if by any peculiar accident there should ever be one of them, from there on the rules of probability do not apply, and there will be many of them, at least if the milieu is reasonable. But a reasonable milieu is already a thermodynamically much less improbable thing. So, the operations of probability somehow leave a loophole at this point, and it is by the process of self-reproduction that they are pierced.
From lecture series on self-replicating machines at the University of Illinois, Lecture 5 (Dec 1949), 'Re-evaluation of the Problems of Complicated Automata—Problems of Hierarchy and Evolution', Theory of Self-Reproducing Automata (1966).
Anyone who has examined into the history of the theories of earth evolution must have been astounded to observe the manner in which the unique and the difficultly explainable has been made to take the place of the common and the natural in deriving the framework of these theories.
Earth Evolution and Facial Expression (1921), 174.
Anyone who is not shocked by the quantum theory has not understood it. [Attributed.]
Webmaster is not alone in failing to find a primary source. Regardless of how widely quoted, the few citations to be found merely reference other books in which it is stated without a valid citation. For example, this quote is an epigraph in Eric Middleton, The New Flatlanders (2007), 19, with a note (p.151) citing Niels Bohr, Atomic Physics and Human Knowledge (1958), but Webmaster’s search of that text does not find it. If you know the primary source, or an early citation, please contact Webmaster.
Anything at all that can be the object of scientific thought becomes dependent on the axiomatic method, and thereby indirectly on mathematics, as soon as it is ripe for the formation of a theory. By pushing ahead to ever deeper layers of axioms … we become ever more conscious of the unity of our knowledge. In the sign of the axiomatic method, mathematics is summoned to a leading role in science.
Address (11 Sep 1917), 'Axiomatisches Denken' delivered before the Swiss Mathematical Society in
Zürich. Translated by Ewald as 'Axiomatic Thought', (1918), in William Bragg Ewald, From Kant to Hilbert (1996), Vol. 2, 1115.
Architects who have aimed at acquiring manual skill without scholarship have never been able to reach a position of authority to correspond to their pains, while those who relied only upon theories and scholarship were obviously hunting the shadow, not the substance. But those who have a thorough knowledge of both, like men armed at all points, have the sooner attained their object and carried authority with them.
In De Architectura, Book 1, Chap 1, Sec. 2. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 3.
As Crystallography was born of a chance observation by Haüy of the cleavage-planes of a single fortunately fragile specimen, … so out of the slender study of the Norwich Spiral has sprung the vast and interminable Calculus of Cyclodes, which strikes such far-spreading and tenacious roots into the profoundest strata of denumeration, and, by this and the multitudinous and multifarious dependent theories which cluster around it, reminds one of the Scriptural comparison of the Kingdom of Heaven “to a grain of mustard-seed which a man took and cast into his garden, and it grew and waxed a great tree, and the fowls of the air lodged in the branches of it.”
From 'Outline Trace of the Theory of Reducible Cyclodes', Proceedings of the London Mathematical Society (1869), 2, 155, collected in Collected Mathematical Papers of James Joseph Sylvester (1908), Vol. 2, 683-684.
As every circumstance relating to so capital a discovery as this (the greatest, perhaps, that has been made in the whole compass of philosophy, since the time of Sir Isaac Newton) cannot but give pleasure to all my readers, I shall endeavour to gratify them with the communication of a few particulars which I have from the best authority. The Doctor [Benjamin Franklin], after having published his method of verifying his hypothesis concerning the sameness of electricity with the matter lightning, was waiting for the erection of a spire in Philadelphia to carry his views into execution; not imagining that a pointed rod, of a moderate height, could answer the purpose; when it occurred to him, that, by means of a common kite, he could have a readier and better access to the regions of thunder than by any spire whatever. Preparing, therefore, a large silk handkerchief, and two cross sticks, of a proper length, on which to extend it, he took the opportunity of the first approaching thunder storm to take a walk into a field, in which there was a shed convenient for his purpose. But dreading the ridicule which too commonly attends unsuccessful attempts in science, he communicated his intended experiment to no body but his son, who assisted him in raising the kite.
The kite being raised, a considerable time elapsed before there was any appearance of its being electrified. One very promising cloud passed over it without any effect; when, at length, just as he was beginning to despair of his contrivance, he observed some loose threads of the hempen string to stand erect, and to avoid one another, just as if they had been suspended on a common conductor. Struck with this promising appearance, he inmmediately presented his knuckle to the key, and (let the reader judge of the exquisite pleasure he must have felt at that moment) the discovery was complete. He perceived a very evident electric spark. Others succeeded, even before the string was wet, so as to put the matter past all dispute, and when the rain had wetted the string, he collected electric fire very copiously. This happened in June 1752, a month after the electricians in France had verified the same theory, but before he had heard of any thing that they had done.
The kite being raised, a considerable time elapsed before there was any appearance of its being electrified. One very promising cloud passed over it without any effect; when, at length, just as he was beginning to despair of his contrivance, he observed some loose threads of the hempen string to stand erect, and to avoid one another, just as if they had been suspended on a common conductor. Struck with this promising appearance, he inmmediately presented his knuckle to the key, and (let the reader judge of the exquisite pleasure he must have felt at that moment) the discovery was complete. He perceived a very evident electric spark. Others succeeded, even before the string was wet, so as to put the matter past all dispute, and when the rain had wetted the string, he collected electric fire very copiously. This happened in June 1752, a month after the electricians in France had verified the same theory, but before he had heard of any thing that they had done.
The History and Present State of Electricity, with Original Experiments (1767, 3rd ed. 1775), Vol. 1, 216-7.
As far as I see, such a theory [of the primeval atom] remains entirely outside any metaphysical or religious question. It leaves the materialist free to deny any transcendental Being. He may keep, for the bottom of space-time, the same attitude of mind he has been able to adopt for events occurring in non-singular places in space-time. For the believer, it removes any attempt to familiarity with God, as were Laplace’s chiquenaude or Jeans’ finger. It is consonant with the wording of Isaiah speaking of the “Hidden God” hidden even in the beginning of the universe … Science has not to surrender in face of the Universe and when Pascal tries to infer the existence of God from the supposed infinitude of Nature, we may think that he is looking in the wrong direction.
From 'The Primeval Atom Hypothesis and the Problem of Clusters of Galaxies', in R. Stoops (ed.), La Structure et l'Evolution de l'Univers (1958), 1-32. As translated in Helge Kragh, Cosmology and Controversy: The Historical Development of Two Theories of the Universe (1996), 60.
As for everything else, so for a mathematical theory: beauty can be perceived but not explained.
President’s address (1883) to the British Association for the Advancement of Science, in The Collected Mathematical Papers (1895), Vol. 8, xxii.
As is well known the principle of virtual velocities transforms all statics into a mathematical assignment, and by D'Alembert's principle for dynamics, the latter is again reduced to statics. Although it is is very much in order that in gradual training of science and in the instruction of the individual the easier precedes the more difficult, the simple precedes the more complicated, the special precedes the general, yet the min, once it has arrived at the higher standpoint, demands the reverse process whereby all statics appears only as a very special case of mechanics.
Collected Works (1877), Vol. 5, 25-26. Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 412.
As regards authority I so proceed. Boetius says in the second prologue to his Arithmetic, “If an inquirer lacks the four parts of mathematics, he has very little ability to discover truth.” And again, “Without this theory no one can have a correct insight into truth.” And he says also, “I warn the man who spurns these paths of knowledge that he cannot philosophize correctly.” And Again, “It is clear that whosoever passes these by, has lost the knowledge of all learning.”
Opus Majus [1266-1268], Part IV, distinction I, chapter I, trans. R. B. Burke, The Opus Majus of Roger Bacon (1928), Vol. I, 117.
As soon … as it was observed that the stars retained their relative places, that the times of their rising and setting varied with the seasons, that sun, moon, and planets moved among them in a plane, … then a new order of things began.… Science had begun, and the first triumph of it was the power of foretelling the future; eclipses were perceived to recur in cycles of nineteen years, and philosophers were able to say when an eclipse was to be looked for. The periods of the planets were determined. Theories were invented to account for their eccentricities; and, false as those theories might be, the position of the planets could be calculated with moderate certainty by them.
Lecture delivered to the Royal Institution (5 Feb 1864), 'On the Science of History'. Collected in Notices of the Proceedings at the Meetings of the Members of the Royal Institution of Great Britain with Abstracts of the Discourses (1866), Vol. 4, 187.
As usual, nature’s imagination far surpasses our own, as we have seen from the other theories which are subtle and deep.
In The Character of Physical Law (1965, 2001), 162.
Asked in 1919 whether it was true that only three people in the world understood the theory of general relativity, [Eddington] allegedly replied: “Who's the third?”
As described in Brian Stableford, Science Fact and Science Fiction: An Encyclopedia (2006), 150.
Astronomy was thus the cradle of the natural sciences and the starting point of geometrical theories. The stars themselves gave rise to the concept of a ‘point’; triangles, quadrangles and other geometrical figures appeared in the constellations; the circle was realized by the disc of the sun and the moon. Thus in an essentially intuitive fashion the elements of geometrical thinking came into existence.
In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 72.
Astrophysicists closing in on the grand structure of matter and emptiness in the universe are ruling out the meatball theory, challenging the soap bubble theory, and putting forward what may be the strongest theory of all: that the cosmos is organized like a sponge.
'Rethinking Clumps and Voids in the Universe', New York Times (9 Nov 1986), A1.
At fertilization, these two “haploid” nuclei are added together to make a “diploid” nucleus that now contains 2a, 2b and so on; and, by the splitting of each chromosome and the regulated karyokinetic separation of the daughter chromosomes, this double series is inherited by both of the primary blastomeres. In the resulting resting nuclei the individual chromosomes are apparently destroyed. But we have the strongest of indications that, in the stroma of the resting nucleus, every one of the chromosomes that enters the nucleus survives as a well-defined region; and as the cell prepares for its next division this region again gives rise to the same chromosome (Theory of the Individuality of the Chromosomes). In this way the two sets of chromosomes brought together at fertilization are inherited by all the cells of the new individual. It is only in the germinal cells that the so called reduction division converts the double series into a single one. Out of the diploid state, the haploid is once again generated.
In Arch. Zellforsch, 1909, 3, 181, trans. Henry Harris, The Birth of the Cell (1999), 171-2.
Atoms are round balls of wood invented by Dr. Dalton.
Answer given by a pupil to a question on atomic theory, as reported by Sir Henry Enfield Roscoe.
Answer given by a pupil to a question on atomic theory, as reported by Sir Henry Enfield Roscoe.
Reports of the British Association for the Advancement of Science, 57th report, 1887, 7.
Be suspicious of a theory if more and more hypotheses are needed to support it as new facts become available, or as new considerations are brought to bear.
Given as the authors’ preferred interpretation of Ockham’s Razor. With co-author Nalin Chandra Wickramasinghe, Evolution from Space (1981), 135.
Being of the opinion that, if an object is the class of some a (e.g., people, points, square circles), then it actually consists of a, I always rejected … the existence of theoretical monstrosities like the class of square circles, understanding only too well that nothing can consist of something which does not even exist.
(1927). As quoted in Rafal Urbaniak, Leśniewski’s Systems of Logic and Foundations of Mathematics (2013), 113.
Bertrand, Darboux, and Glaisher have compared Cayley to Euler, alike for his range, his analytical power, and, not least, for his prolific production of new views and fertile theories. There is hardly a subject in the whole of pure mathematics at which he has not worked.
In Proceedings of London Royal Society (1895), 58, 21.
Berzelius' symbols are horrifying. A young student in chemistry might as soon learn Hebrew as make himself acquainted with them... They appear to me equally to perplex the adepts in science, to discourage the learner, as well as to cloud the beauty and simplicity of the atomic theory.
Quoted in H. E. Roscoe, 'Presidential Address', Reports of the British Association for the Advancement of Science, 57th report, 1887, 7.
Besides electrical engineering theory of the transmission of messages, there is a larger field [cybernetics] which includes not only the study of language but the study of messages as a means of controlling machinery and society, the development of computing machines and other such automata, certain reflections upon psychology and the nervous system, and a tentative new theory of scientific method.
In Cybernetics (1948).
Biological determinism is, in its essence, a theory of limits. It takes the current status of groups as a measure of where they should and must be ... We inhabit a world of human differences and predilections, but the extrapolation of these facts to theories of rigid limits is ideology.
The Mismeasure of Man (1981), 28-9.
Blind commitment to a theory is not an intellectual virtue: it is an intellectual crime.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 1.
Buffon, who, with all his theoretical ingenuity and extraordinary eloquence, I suspect had little actual information in the science on which he wrote so admirably For instance, he tells us that the cow sheds her horns every two years; a most palpable error. ... It is wonderful that Buffon who lived so much in the country at his noble seat should have fallen into such a blunder I suppose he has confounded the cow with the deer.
In The Life of Samuel Johnson, LL.D. (1826), Vol. 3, 70, footnote.
But although in theory physicists realize that their conclusions are ... not certainly true, this ... does not really sink into their consciousness. Nearly all the time ... they ... act as if Science were indisputably True, and what's more, as if only science were true.... Any information obtained otherwise than by the scientific method, although it may be true, the scientists will call “unscientific,” using this word as a smear word, by bringing in the connotation from its original [Greek] meaning, to imply that the information is false, or at any rate slightly phony.
In Science is a Sacred Cow (1950), 176-77.
But for the persistence of a student of this university in urging upon me his desire to study with me the modern algebra I should never have been led into this investigation; and the new facts and principles which I have discovered in regard to it (important facts, I believe), would, so far as I am concerned, have remained still hidden in the womb of time. In vain I represented to this inquisitive student that he would do better to take up some other subject lying less off the beaten track of study, such as the higher parts of the calculus or elliptic functions, or the theory of substitutions, or I wot not what besides. He stuck with perfect respectfulness, but with invincible pertinacity, to his point. He would have the new algebra (Heaven knows where he had heard about it, for it is almost unknown in this continent), that or nothing. I was obliged to yield, and what was the consequence? In trying to throw light upon an obscure explanation in our text-book, my brain took fire, I plunged with re-quickened zeal into a subject which I had for years abandoned, and found food for thoughts which have engaged my attention for a considerable time past, and will probably occupy all my powers of contemplation advantageously for several months to come.
In Johns Hopkins Commemoration Day Address, Collected Mathematical Papers, Vol. 3, 76.
But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.
In Van Gogh, the Man Suicided by Society (1947). As translated in Jack Hirschman (ed.) Artaud Anthology (1965), 149.
But in its [the corpuscular theory of radiation] relation to the wave theory there is one extraordinary and, at present, insoluble problem. It is not known how the energy of the electron in the X-ray bulb is transferred by a wave motion to an electron in the photographic plate or in any other substance on which the X-rays fall. It is as if one dropped a plank into the sea from the height of 100 ft. and found that the spreading ripple was able, after travelling 1000 miles and becoming infinitesimal in comparison with its original amount, to act upon a wooden ship in such a way that a plank of that ship flew out of its place to a height of 100 ft. How does the energy get from one place to the other?
'Aether Waves and Electrons' (Summary of the Robert Boyle Lecture), Nature, 1921, 107, 374.
But it is precisely mathematics, and the pure science generally, from which the general educated public and independent students have been debarred, and into which they have only rarely attained more than a very meagre insight. The reason of this is twofold. In the first place, the ascendant and consecutive character of mathematical knowledge renders its results absolutely insusceptible of presentation to persons who are unacquainted with what has gone before, and so necessitates on the part of its devotees a thorough and patient exploration of the field from the very beginning, as distinguished from those sciences which may, so to speak, be begun at the end, and which are consequently cultivated with the greatest zeal. The second reason is that, partly through the exigencies of academic instruction, but mainly through the martinet traditions of antiquity and the influence of mediaeval logic-mongers, the great bulk of the elementary text-books of mathematics have unconsciously assumed a very repellant form,—something similar to what is termed in the theory of protective mimicry in biology “the terrifying form.” And it is mainly to this formidableness and touch-me-not character of exterior, concealing withal a harmless body, that the undue neglect of typical mathematical studies is to be attributed.
In Editor’s Preface to Augustus De Morgan and Thomas J. McCormack (ed.), Elementary Illustrations of the Differential and Integral Calculus (1899), v.
But no other theory can explain so much. Continental drift is without a cause or a physical theory. It has never been applied to any but the last part of geological time.
In 'Geophysics and Continental Growth', American Scientist (1959), 47, 23.
But while I accept specialization in the practice, I reject it utterly in the theory of science.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 25.
But, but, but … if anybody says he can think about quantum theory without getting giddy it merely shows that he hasn’t understood the first thing about it!
Quoted in Otto R. Frisch, What Little I Remember (1979), 95.
But, contrary to the lady’s prejudices about the engineering profession, the fact is that quite some time ago the tables were turned between theory and applications in the physical sciences. Since World War II the discoveries that have changed the world are not made so much in lofty halls of theoretical physics as in the less-noticed labs of engineering and experimental physics. The roles of pure and applied science have been reversed; they are no longer what they were in the golden age of physics, in the age of Einstein, Schrödinger, Fermi and Dirac.
'The Age of Computing: a Personal Memoir', Daedalus (1992), 121, 120.
By an application of the theory of relativity to the taste of readers, today in Germany I am called a German man of science, and in England I am represented as a Swiss Jew. If I come to be regarded as a bête noire the descriptions will be reversed, and I shall become a Swiss Jew for the Germans and a German man of science for the English!
Times (28 Nov 1919). In Robert Andrews Famous Lines: a Columbia Dictionary of Familiar Quotations (1997), 414. Variations of this quote were made by Einstein on other occasions.
By far the most important consequence of the conceptual revolution brought about in physics by relativity and quantum theory lies not in such details as that meter sticks shorten when they move or that simultaneous position and momentum have no meaning, but in the insight that we had not been using our minds properly and that it is important to find out how to do so.
'Quo Vadis'. In Gerald Holton (ed.), Science and the Modern Mind (1971), 84.
Catastrophe Theory is—quite likely—the first coherent attempt (since Aristotelian logic) to give a theory on analogy. When narrow-minded scientists object to Catastrophe Theory that it gives no more than analogies, or metaphors, they do not realise that they are stating the proper aim of Catastrophe Theory, which is to classify all possible types of analogous situations.
From 'La Théorie des catastrophes État présent et perspective', as quoted in Erick Christopher Zeeman, (ed.), Catastrophe Theory: Selected Papers, 1972-1977 (1977), 637, as cited in Martin Krampe (ed.), Classics of Semiotics (1987), 214.
Cell genetics led us to investigate cell mechanics. Cell mechanics now compels us to infer the structures underlying it. In seeking the mechanism of heredity and variation we are thus discovering the molecular basis of growth and reproduction. The theory of the cell revealed the unity of living processes; the study of the cell is beginning to reveal their physical foundations.
Recent Advances in Cytology (1937), 562.
Cells are required to stick precisely to the point. Any ambiguity, any tendency to wander from the matter at hand, will introduce grave hazards for the cells, and even more for the host in which they live. … There is a theory that the process of aging may be due to the cumulative effect of imprecision, a gradual degrading of information. It is not a system that allows for deviating.
In 'Information', The Lives of a Cell: Notes of a Biology Watcher (1974), 110-111.
Chaos theory is a new theory invented by scientists panicked by the thought that the public were beginning to understand the old ones.
John Mitchinson and John Lloyd, If Ignorance Is Bliss, Why Aren't There More Happy People?: Smart Quotes for Dumb Times (2009), 273.
Chemistry and physics are experimental sciences; and those who are engaged in attempting to enlarge the boundaries of science by experiment are generally unwilling to publish speculations; for they have learned, by long experience, that it is unsafe to anticipate events. It is true, they must make certain theories and hypotheses. They must form some kind of mental picture of the relations between the phenomena which they are trying to investigate, else their experiments would be made at random, and without connection.
From 'Radium and Its Products', Harper’s Magazine (Dec 1904), 52.
Chemistry is like a majestic skyscraper. The concrete secure foundation of chemistry consists of countless experimentally observed facts. The theories, principles and laws developed from these observations are like an elevator which runs from the bottom to the top of the edifice.
Ernest R. Toon and George L. Ellis (eds.), Foundations of Chemistry (1968), 1.
Chess is not a game. Chess is a well-defined form of computation. You may not be able to work out the answers, but in theory there must be a solution, a right procedure in any position. Now real games are not like that at all. Real life is not like that. Real life consists of bluffing, of little tactics of deception, of asking yourself what is the other man going to think I mean to do.
In Jacob Bronowski, The Ascent of Man (2011), 324. Although printed within single quote marks, these words are presumably not verbatim, but only a narrative recollection of a conversation with the author (during WWII, in a taxi, about von Neumann’s Theory of Games).
Children are told that an apple fell on Isaac Newton’s head and he was led to state the law of gravity. This, of course, is pure foolishness. What Newton discovered was that any two particles in the universe attract each other with a force that is proportional to the product of their masses and inversely proportional to the square of the distance between them. This is not learned from a falling apple, but by observing quantities of data and developing a mathematical theory that can be verified by additional data. Data gathered by Galileo on falling bodies and by Johannes Kepler on motions of the planets were invaluable aids to Newton. Unfortunately, such false impressions about science are not universally outgrown like the Santa Claus myth, and some people who don’t study much science go to their graves thinking that the human race took until the mid-seventeenth century to notice that objects fall.
In How to Tell the Liars from the Statisticians (1983), 127.
Classes and concepts may, however, also be conceived as real objects, namely classes as “pluralities of things” or as structures consisting of a plurality of things and concepts as the properties and relations of things existing independently of our definitions and constructions. It seems to me that the assumption of such objects is quite as legitimate as the assumption of physical bodies and there is quite as much reason to believe in their existence. They are in the same sense necessary to obtain a satisfactory system of mathematics as physical bodies are necessary for a satisfactory theory of our sense perceptions…
In 'Russell's Mathematical Logic', in P.A. Schilpp (ed.), The Philosophy of Bertrand Russell (1944), Vol. 1, 137.
Classical thermodynamics ... is the only physical theory of universal content which I am convinced ... will never be overthrown.
Quoted in Albert Einstein and Stephen Hawking (ed.), A Stubbornly Persistent Illusion (2007), 353.
Common sense is only the application of theories which have grown and been formulated unconsciously as result of experience.
From 'For Mans Use of God's Gifts', collected in Robert C. Goodpasture (ed.), Engineers and Ivory Towers (1952), 107.
Considered in its entirety, psychoanalysis won’t do. It is an end product, moreover, like a dinosaur or a zeppelin, no better theory can ever be erected on its ruins, which will remain for ever one of the saddest and strangest of all landmarks in the history of twentieth century thought.
From 'Further Comments on Psychoanalysis', The Hope of Progress: A Scientist Looks at Problems in Philosophy, Literature and Science (1973), 69.
Cosmology, for centuries consisting of speculation based on a minimum of observational evidence and a maximum of philosophical predilection, became in the twentieth century an observational science, its theories now subject to verification or refutation to a degree previously unimaginable.
Opening sentence in 'Philosophical Values and Observation in Edwin Hubble's Choice of a Model of the Universe', Historical Studies in the Physical Sciences (1982), 13, No. 1, 41.
Creating a new theory is not like destroying an old barn and erecting a skyscraper in its place. It is rather like climbing a mountain, gaining new and wider views, discovering unexpected connections between our starting point and its rich environment. But the point from which we started out still exists and can be seen, although it appears smaller and forms a tiny part of our broad view gained by the mastery of the obstacles on our adventurous way up.
Dalton transformed the atomic concept from a philosophical speculation into a scientific theory—framed to explain quantitative observations, suggesting new tests and experiments, and capable of being given quantitative form through the establishment of relative masses of atomic particles.
Development of Concepts of Physics. In Clifford A. Pickover, Archimedes to Hawking: Laws of Science and the Great Minds Behind Them (2008), 175.
Darwin's theory was received in Russia with profound sympathy. While in Western Europe it met firmly established old traditions which it had first to overcome, in Russia its appearance coincided with the awakening of our society after the Crimean War and here it immediately received the status of full citizenship and ever since has enjoyed widespread popularity.
Quoted in Thomas F. Glick (ed.), The Comparative Reception of Darwinism (1988), 229-30.
Definition of Mathematics.—It has now become apparent that the traditional field of mathematics in the province of discrete and continuous number can only be separated from the general abstract theory of classes and relations by a wavering and indeterminate line. Of course a discussion as to the mere application of a word easily degenerates into the most fruitless logomachy. It is open to any one to use any word in any sense. But on the assumption that “mathematics” is to denote a science well marked out by its subject matter and its methods from other topics of thought, and that at least it is to include all topics habitually assigned to it, there is now no option but to employ “mathematics” in the general sense of the “science concerned with the logical deduction of consequences from the general premisses of all reasoning.”
In article 'Mathematics', Encyclopedia Britannica (1911, 11th ed.), Vol. 17, 880. In the 2006 DVD edition of the encyclopedia, the definition of mathematics is given as “The science of structure, order, and relation that has evolved from elemental practices of counting, measuring, and describing the shapes of objects.” [Premiss is a variant form of “premise”. —Webmaster]
Democracy can’t work. Mathematicians, peasants, and animals, that’s all there is—so democracy, a theory based on the assumption that mathematicians and peasants are equal, can never work.
In Glory Road (1963, 1981), 233.
Democracy might therefore almost in a sense be termed that practice of which science is the theory.
In The Grand Titration (1969).
Do not say hypothesis, and even less theory: say way of thinking.
Aphorism 263 in Notebook J (1789-1793), as translated by R. J. Hollingdale in Aphorisms (1990). Reprinted as The Waste Books (2000), 181.
Do you see this egg? With this you can topple every theological theory, every church or temple in the world.
'Conversation Between d'Alembert and Diderot,' D'Alembert's Dream (written 1769, published 1830). Reprinted in Selected Writings, ed. Lester G. Crocker (1966).
Don’t confuse hypothesis and theory. The former is a possible explanation; the latter, the correct one. The establishment of theory is the very purpose of science.
Martin H. Fischer, Howard Fabing (ed.) and Ray Marr (ed.), Fischerisms (1944), 7.
During my stay in London I resided for a considerable time in Clapham Road in the neighbourhood of Clapham Common... One fine summer evening I was returning by the last bus 'outside' as usual, through the deserted streets of the city, which are at other times so full of life. I fell into a reverie (Träumerei), and 10, the atoms were gambolling before my eyes! Whenever, hitherto, these diminutive beings had appeared to me, they had always been in motion: but up to that time I had never been able to discern the nature of their motion. Now, however, I saw how, frequently, two smaller atoms united to form a pair: how the larger one embraced the two smaller ones: how still larger ones kept hold of three or even four of the smaller: whilst the whole kept whirling in a giddy dance. I saw how the larger ones formed a chain, dragging the smaller ones after them but only at the ends of the chain. I saw what our past master, Kopp, my highly honoured teacher and friend has depicted with such charm in his Molekular-Welt: but I saw it long before him. The cry of the conductor 'Clapham Road', awakened me from my dreaming: but I spent part of the night in putting on paper at least sketches of these dream forms. This was the origin of the 'Structural Theory'.
Kekule at Benzolfest in Berichte (1890), 23, 1302.
During the half-century that has elapsed since the enunciation of the cell-theory by Schleiden and Schwann, in 1838-39, it has became ever more clearly apparent that the key to all ultimate biological problems must, in the last analysis, be sought in the cell. It was the cell-theory that first brought the structure of plants and animals under one point of view by revealing their common plan of organization. It was through the cell-theory that Kolliker and Remak opened the way to an understanding of the nature of embryological development, and the law of genetic continuity lying at the basis of inheritance. It was the cell-theory again which, in the hands of Virchaw and Max Schultze, inaugurated a new era in the history of physiology and pathology, by showing that all the various functions of the body, in health and in disease, are but the outward expression of cell-activities. And at a still later day it was through the cell-theory that Hertwig, Fol, Van Beneden, and Strasburger solved the long-standing riddle of the fertilization of the egg, and the mechanism of hereditary transmission. No other biological generalization, save only the theory of organic evolution, has brought so many apparently diverse phenomena under a common point of view or has accomplished more far the unification of knowledge. The cell-theory must therefore be placed beside the evolution-theory as one of the foundation stones of modern biology.
In The Cell in Development and Inheritance (1896), 1.
Each worldview was a cultural product, but evolution is true and separate creation is not ... Worldviews are social constructions, and they channel the search for facts. But facts are found and knowledge progresses, however fitfully. Fact and theory are intertwined, and all great scientists understand the interaction.
…...
Earlier theories … were based on the hypothesis that all the matter in the universe was created in one big bang at a particular time in the remote past. [Coining the “big bang” expression.]
From microfilmed Speaker's Copy of a radio script held at the BBC Written Archive Centre, for Hoyle's radio talk on the BBC Third Programme (28 Mar 1949). The date and time of the broadcast, 6:30pm, are given in that week’s Radio Times. The quote, with these references given in footnotes, in Simon Mitton, Fred Hoyle: A Life in Science (2011), 127-128 and 332. The text of the talk, the first printed use of the “big bang” expression, in the BBC’s The Listener magazine (7 Apr 1949), Vol.41, 568.
Einstein, my upset stomach hates your theory [of General Relativity]—it almost hates you yourself! How am I to provide for my students? What am I to answer to the philosophers?!!
Letter to Albert Einstein, 20 Nov 1919. In Martin J. Klein, Paul Ehrenfest: The Making of a Theoretical Physicist (1970), Vol. 1, 315.
Electric and magnetic forces. May they live for ever, and never be forgot, if only to remind us that the science of electromagnetics, in spite of the abstract nature of its theory, involving quantities whose nature is entirely unknown at the present, is really and truly founded on the observations of real Newtonian forces, electric and magnetic respectively.
From 'Electromagnetic Theory, CXII', The Electrician (23 Feb 1900), Vol. 44, 615.
Emission of lava … during geological time … would produce more contraction than any reasonable amount of cooling of the Earth. It has been shown that contraction could lead to fracturing of a kind which might show many of the principal features observed in existing and past mountains. A vast amount remains to be done, but no other theory can explain so much. Continental drift is without a cause or a physical theory. It has never been applied to any but the last part of geological time.
In Sigma XI National Lecture (1957-58), published in 'Geophysics and Continental Growth', American Scientist (Mar 1959), 47, No. 1, 23.
Engineering is the art of directing the great sources of power in nature for the use and the convenience of people. In its modern form engineering involves people, money, materials, machines, and energy. It is differentiated from science because it is primarily concerned with how to direct to useful and economical ends the natural phenomena which scientists discover and formulate into acceptable theories. Engineering therefore requires above all the creative imagination to innovate useful applications of natural phenomena. It seeks newer, cheaper, better means of using natural sources of energy and materials.
In McGraw Hill, Science and Technology Encyclopedia
Engineers apply the theories and principles of science and mathematics to research and develop economical solutions to practical technical problems. Their work is the link between scientific discoveries and commercial applications. Engineers design products, the machinery to build those products, the factories in which those products are made, and the systems that ensure the quality of the product and efficiency of the workforce and manufacturing process. They design, plan, and supervise the construction of buildings, highways, and transit systems. They develop and implement improved ways to extract, process, and use raw materials, such as petroleum and natural gas. They develop new materials that both improve the performance of products, and make implementing advances in technology possible. They harness the power of the sun, the earth, atoms, and electricity for use in supplying the Nation’s power needs, and create millions of products using power. Their knowledge is applied to improving many things, including the
quality of health care, the safety of food products, and the efficient operation of financial systems.
Bureau of Labor Statistics, Occupational Outlook Handbook (2000) as quoted in Charles R. Lord. Guide to Information Sources in Engineering (2000), 5. This definition has been revised and expanded over time in different issues of the Handbook.
Enough research will tend to support your theory.
In Murphy’s Law by Arthur Bloch (1977), and titled 'Murphy’s Law of Research'.
Entropy theory is indeed a first attempt to deal with global form; but it has not been dealing with structure. All it says is that a large sum of elements may have properties not found in a smaller sample of them.
In Entropy and Art: An Essay on Disorder and Order (1974), 21.
Entropy theory, on the other hand, is not concerned with the probability of succession in a series of items but with the overall distribution of kinds of items in a given arrangement.
In Entropy and Art: An Essay on Disorder and Order (1974), 19.
Euclidean mathematics assumes the completeness and invariability of mathematical forms; these forms it describes with appropriate accuracy and enumerates their inherent and related properties with perfect clearness, order, and completeness, that is, Euclidean mathematics operates on forms after the manner that anatomy operates on the dead body and its members. On the other hand, the mathematics of variable magnitudes—function theory or analysis—considers mathematical forms in their genesis. By writing the equation of the parabola, we express its law of generation, the law according to which the variable point moves. The path, produced before the eyes of the student by a point moving in accordance to this law, is the parabola.
If, then, Euclidean mathematics treats space and number forms after the manner in which anatomy treats the dead body, modern mathematics deals, as it were, with the living body, with growing and changing forms, and thus furnishes an insight, not only into nature as she is and appears, but also into nature as she generates and creates,—reveals her transition steps and in so doing creates a mind for and understanding of the laws of becoming. Thus modern mathematics bears the same relation to Euclidean mathematics that physiology or biology … bears to anatomy.
If, then, Euclidean mathematics treats space and number forms after the manner in which anatomy treats the dead body, modern mathematics deals, as it were, with the living body, with growing and changing forms, and thus furnishes an insight, not only into nature as she is and appears, but also into nature as she generates and creates,—reveals her transition steps and in so doing creates a mind for and understanding of the laws of becoming. Thus modern mathematics bears the same relation to Euclidean mathematics that physiology or biology … bears to anatomy.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 38. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 112-113.
Even if there is only one possible unified theory, it is just a set of rules and equations. What is it that breathes fire into the equations and makes a universe for them to describe? The usual approach of science of constructing a mathematical model cannot answer the questions of why there should be a universe for the model to describe. Why does the universe go to all the bother of existing?
A Brief History of Time (1998), 190.
Even in the dark times between experimental breakthroughs, there always continues a steady evolution of theoretical ideas, leading almost imperceptibly to changes in previous beliefs.
In Nobel Lecture (8 Dec 1989), 'Conceptual Foundations of the Unified Theory of Weak and Electromagnetic Interactions.'
Even mistaken hypotheses and theories are of use in leading to discoveries. This remark is true in all the sciences. The alchemists founded chemistry by pursuing chimerical problems and theories which are false. In physical science, which is more advanced than biology, we might still cite men of science who make great discoveries by relying on false theories. It seems, indeed, a necessary weakness of our mind to be able to reach truth only across a multitude of errors and obstacles.
An Introduction to the Study of Experimental Medicine (1865, translation 1927, 1957), 170.
Every discovery opens a new field for investigation of facts, shows us the imperfection of our theories. It has justly been said, that the greater the circle of light, the greater the boundary of darkness by which it is surrounded.
Humphry Davy and John Davy, 'Consolations in Travel—Dialogue V—The Chemical Philosopher', The Collected Works of Sir Humphry Davy (1840), Vol. 9, 362.
Every mathematician knows it is impossible to understand an elementary course in thermodynamics. The reason is that the thermodynamics is based—as Gibbs has explicitly proclaimed—on a rather complicated mathematical theory, on the contact geometry. Contact geometry is one of the few “simple geometries” of the so-called Cartan’s list, but it is still mostly unknown to the physicist.
The first sentence is often seen standing alone as a quote, to imply thermodynamics is a chimera beyond reach. When read in the full context, the point is that thermodynamics becomes possible to understand in an advanced course. It includes a reference to the work of mathematician Élie Cartan. From 'Contact Geometry: The Geometrical Method of Gibbs’ Thermodynamics', collected in D. Caldi and G. Mostow, (eds.) Proceedings of the Gibbs Symposium: Yale University, May 15-17, 1989, (1990), 163.
Every new theory as it arises believes in the flush of youth that it has the long sought goal; it sees no limits to its applicability, and believes that at last it is the fortunate theory to achieve the 'right' answer. This was true of electron theory—perhaps some readers will remember a book called The Electrical Theory of the Universe by de Tunzelman. It is true of general relativity theory with its belief that we can formulate a mathematical scheme that will extrapolate to all past and future time and the unfathomed depths of space. It has been true of wave mechanics, with its first enthusiastic claim a brief ten years ago that no problem had successfully resisted its attack provided the attack was properly made, and now the disillusionment of age when confronted by the problems of the proton and the neutron. When will we learn that logic, mathematics, physical theory, are all only inventions for formulating in compact and manageable form what we already know, like all inventions do not achieve complete success in accomplishing what they were designed to do, much less complete success in fields beyond the scope of the original design, and that our only justification for hoping to penetrate at all into the unknown with these inventions is our past experience that sometimes we have been fortunate enough to be able to push on a short distance by acquired momentum.
The Nature of Physical Theory (1936), 136.
Every theoretical physicist who is any good knows six or seven different theoretical representations for exactly the same physics. He knows that they are all equivalent, and that nobody is ever going to be able to decide which one is right at that level, but he keeps them in his head, hoping that they will give him different ideas for guessing.
In The Character of Physical Law (1965, 2001), 168.
Every theory of love, from Plato down, teaches that each individual loves in the other sex what he lacks in himself.
Quoted in Values of the Wise: Humanity's Highest Aspirations (2004), 195.
Everybody firmly believes in it [Nomal Law of Errors] because the mathematicians imagine it is a fact of observation, and observers that it is a theory of mathematics.
…...
Everything should be made as simple as possible, but not simpler.
Attributed.
Evolution ... is really two theories, the vague theory and the precise theory. The vague theory has been abundantly proved.... The precise theory has never been proved at all. However, like relativity, it is accepted on faith.... On getting down to actual details, difficulties begin.
In Science is a Sacred Cow (1950), 101 & 103.
Evolution is a theory of organic change, but it does not imply, as many people assume, that ceaseless flux is the irreducible state of nature and that structure is but a temporary incarnation of the moment. Change is more often a rapid transition between stable states than a continuous transformation at slow and steady rates. We live in a world of structure and legitimate distinction. Species are the units of nature’s morphology.
…...
Experience teaches nothing without theory.
In On the Management of Statistical Techniques for Quality and Productivity (1981), 86.
Facts alone, no matter how numerous or verifiable, do not automatically arrange themselves into an intelligible, or truthful, picture of the world. It is the task of the human mind to invent a theoretical framework to account for them.
In Francis Bello, Lawrence Lessing and George A.W. Boehm, Great American Scientists (1960, 1961), 116.
Facts and theories are different things, not rungs in a hierarchy of increasing certainty. Facts are the world's data. Theories are structures of ideas that explain and interpret facts. Facts do not go away while scientists debate rival theories for explaining them. Einstein's theory of gravitation replaced Newton's, but apples did not suspend themselves in mid-air pending the outcome.
'Evolution as Fact and Theory', in Hen's Teeth and Horse's Toes (1983, 1994), Chap. 19.
Facts are a heap of bricks and timber. It is only a successful theory that can convert the heap into a stately mansion
Epigraph in Isaac Asimov’s Book of Science and Nature Quotations (1988), 324.
Facts are not pure unsullied bits of information; culture also influences what we see and how we see it. Theories, moreover, are not inexorable inductions from facts. The most creative theories are often imaginative visions imposed upon facts; the source of imagination is also strongly cultural.
In The Mismeasure of Man (1981, 1996), 54.
Facts are of not much use, considered as facts. They bewilder by their number and their apparent incoherency. Let them be digested into theory, however, and brought into mutual harmony, and it is another matter.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
Facts do not “speak for themselves”; they are read in the light of a theory. Creative thought, in science as much as in the arts, is the motor of changing opinion.
In Perspectives in Biological Medicine (1985).
Factual assertions and fundamental principles are... merely parts of theories: they are given within the framework of a theory; they are chosen and valid within this framework; and subsequently they are dependent upon it. This holds for all empirical sciences—for the natural sciences as well as those pertaining to history.
Critique of Scientific Reason (1983), 106.
Finally I got to carbon, and as you all know, in the case of carbon the reaction works out beautifully. One goes through six reactions, and at the end one comes back to carbon. In the process one has made four hydrogen atoms into one of helium. The theory, of course, was not made on the railway train from Washington to Ithaca … It didn’t take very long, it took about six weeks, but not even the Trans-Siberian railroad [has] taken that long for its journey.
'Pleasure from Physics', From A Life of Physics: Evening Lectures at the International Centre for Theoretical Physics, Trieste, Italy. A Special Supplement of the IAEA Bulletin (1968), 14.
Finding the world would not accommodate to his theory, he wisely determined to accommodate the theory to the world.
Under the pseudonym of Diedrich Knickerbocker, in A History of New York (1809). Collected in The Works of Washington Irving (1840), Vol. 1, 24.
First... a new theory is attacked as absurd; then it is admitted to be true, but obvious and insignificant; finally it is seen to be so important that its adversaries claim that they themselves discovered it.
'Pragmatism's Conception of Truth', in Pragmatism: A New Name for some Old Ways of Thinking, Popular Lectures on Philosophy (1907), 198.
Following the original proposal of Belinfante, “the writer has in a recent note on the meson theory of nuclear forces” used the word “nuclon” as a common notation for the heavy nuclear constituents, neutrons and protons. In the meantime, however, it has been pointed out to me that, since the root of the word nucleus is “nucle”, the notation “nucleon” would from a philological point of view be more appropriate for this purpose….
In Physical Review (1 Feb 1941), 59, 323. For book using the word “nuclon”, see Frederik Jozef Belinfante, Theory of Heavy Quanta: Proefschrift (1939), 40.
For a physicist mathematics is not just a tool by means of which phenomena can be calculated, it is the main source of concepts and principles by means of which new theories can be created.
In 'Mathematics in the Physical Sciences', Scientific American (Sep 1964), 211, No. 3, 129.
For chemistry is no science form’d à priori; ’tis no production of the human mind, framed by reasoning and deduction: it took its rise from a number of experiments casually made, without any expectation of what follow’d; and was only reduced into an art or system, by collecting and comparing the effects of such unpremeditated experiments, and observing the uniform tendency thereof. So far, then, as a number of experimenters agree to establish any undoubted truth; so far they may be consider'd as constituting the theory of chemistry.
From 'The Author's Preface', in A New Method of Chemistry (1727), vi.
For if as scientists we seek simplicity, then obviously we try the simplest surviving theory first, and retreat from it only when it proves false. Not this course, but any other, requires explanation. If you want to go somewhere quickly, and several alternate routes are equally likely to be open, no one asks why you take the shortest. The simplest theory is to be chosen not because it is the most likely to be true but because it is scientifically the most rewarding among equally likely alternatives. We aim at simplicity and hope for truth.
Problems and Projects (1972), 352.
For if there is any truth in the dynamical theory of gases the different molecules in a gas at uniform temperature are moving with very different velocities. Put such a gas into a vessel with two compartments [A and B] and make a small hole in the wall about the right size to let one molecule through. Provide a lid or stopper for this hole and appoint a doorkeeper, very intelligent and exceedingly quick, with microscopic eyes but still an essentially finite being.
Whenever he sees a molecule of great velocity coming against the door from A into B he is to let it through, but if the molecule happens to be going slow he is to keep the door shut. He is also to let slow molecules pass from B to A but not fast ones ... In this way the temperature of B may be raised and that of A lowered without any expenditure of work, but only by the intelligent action of a mere guiding agent (like a pointsman on a railway with perfectly acting switches who should send the express along one line and the goods along another).
I do not see why even intelligence might not be dispensed with and the thing be made self-acting.
Moral The 2nd law of Thermodynamics has the same degree of truth as the statement that if you throw a tumblerful of water into the sea you cannot get the same tumblerful of water out again.
Whenever he sees a molecule of great velocity coming against the door from A into B he is to let it through, but if the molecule happens to be going slow he is to keep the door shut. He is also to let slow molecules pass from B to A but not fast ones ... In this way the temperature of B may be raised and that of A lowered without any expenditure of work, but only by the intelligent action of a mere guiding agent (like a pointsman on a railway with perfectly acting switches who should send the express along one line and the goods along another).
I do not see why even intelligence might not be dispensed with and the thing be made self-acting.
Moral The 2nd law of Thermodynamics has the same degree of truth as the statement that if you throw a tumblerful of water into the sea you cannot get the same tumblerful of water out again.
Letter to John William Strutt (6 Dec 1870). In P. M. Hannan (ed.), The Scientific Letters and Papers of James Clerk Maxwell (1995), Vol. 2, 582-3.
For more than ten years, my theory was in limbo. Then, finally, in the late 1980s, physicists at Princeton said, “There’s nothing wrong with this theory. It’s the only one that works, and we have to open out minds to hyperspace.” We weren’t destined to discover this theory for another 100 years because it’s so bizarre, so different from everything we’d been doing. We didn’t use the normal sequence of discoveries to get to it.
Describing reaction to his superstring theory of hyperspace which mathematically relates the universe’s basic forces.
Describing reaction to his superstring theory of hyperspace which mathematically relates the universe’s basic forces.
Quoted in Nina L. Diamond, Voices of Truth (2000), 326.
For strictly scientific or technological purposes all this is irrelevant. On a pragmatic view, as on a religious view, theory and concepts are held in faith. On the pragmatic view the only thing that matters is that the theory is efficacious, that it “works” and that the necessary preliminaries and side issues do not cost too much in time and effort. Beyond that, theory and concepts go to constitute a language in which the scientistic matters at issue can be formulated and discussed.
In Nobel Lecture (8 Dec 1994), 'Slow Neutron Spectroscopy and the Grand Atlas of the Physical World', Nobel Lectures: Physics 1991-1995 (1997), 111.
Fortunately I experienced Max Wertheimer's teaching in Berlin and collaborated for over a decade with Wolfgang Köhler. I need not emphasize my debts to these outstanding personalities. The fundamental ideas of Gestalt theory are the foundation of all our investigations in the field of the will, of affection, and of the personality.
From A Dynamic Theory of Personality. Selected papers (1935), 240.
Fractal is a word invented by Mandelbrot to bring together under one heading a large class of objects that have [played] … an historical role … in the development of pure mathematics. A great revolution of ideas separates the classical mathematics of the 19th century from the modern mathematics of the 20th. Classical mathematics had its roots in the regular geometric structures of Euclid and the continuously evolving dynamics of Newton. Modern mathematics began with Cantor’s set theory and Peano’s space-filling curve. Historically, the revolution was forced by the discovery of mathematical structures that did not fit the patterns of Euclid and Newton. These new structures were regarded … as “pathological,” .… as a “gallery of monsters,” akin to the cubist paintings and atonal music that were upsetting established standards of taste in the arts at about the same time. The mathematicians who created the monsters regarded them as important in showing that the world of pure mathematics contains a richness of possibilities going far beyond the simple structures that they saw in Nature. Twentieth-century mathematics flowered in the belief that it had transcended completely the limitations imposed by its natural origins.
Now, as Mandelbrot points out, … Nature has played a joke on the mathematicians. The 19th-century mathematicians may not have been lacking in imagination, but Nature was not. The same pathological structures that the mathematicians invented to break loose from 19th-century naturalism turn out to be inherent in familiar objects all around us.
Now, as Mandelbrot points out, … Nature has played a joke on the mathematicians. The 19th-century mathematicians may not have been lacking in imagination, but Nature was not. The same pathological structures that the mathematicians invented to break loose from 19th-century naturalism turn out to be inherent in familiar objects all around us.
From 'Characterizing Irregularity', Science (12 May 1978), 200, No. 4342, 677-678. Quoted in Benoit Mandelbrot, The Fractal Geometry of Nature (1977, 1983), 3-4.
Freudian psychoanalytical theory is a mythology that answers pretty well to Levi-Strauss's descriptions. It brings some kind of order into incoherence; it, too, hangs together, makes sense, leaves no loose ends, and is never (but never) at a loss for explanation. In a state of bewilderment it may therefore bring comfort and relief … give its subject a new and deeper understanding of his own condition and of the nature of his relationship to his fellow men. A mythical structure will be built up around him which makes sense and is believable-in, regardless of whether or not it is true.
From 'Science and Literature', The Hope of Progress: A Scientist Looks at Problems in Philosophy, Literature and Science (1973), 29.
From a certain temperature on, the molecules 'condense' without attractive forces; that is, they accumulate at zero velocity. The theory is pretty, but is there some truth in it.
Letter to Ehrenfest (Dec 1924). Quoted in Abraham Pais, Roger Penrose, Subtle Is the Lord: The Science and the Life of Albert Einstein (2005), 432.
From astronomy we find the east, west, south, and north, as well as the theory of the heavens, the equinox, solstice, and courses of the stars. If one has no knowledge of these matters, he will not be able to have any comprehension of the theory of sundials.
In De Architectura, Book 1, Chap 1, Sec. 10. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 10.
From the medical theoretical standpoint, it suffices to deliberate and speculate regarding most illnesses. However, therapeutically, speculation is not adequate, and true and correct knowledge is imperative.
As quoted in Fred Rosner, The Medical Legacy of Moses Maimonides (1998), 54.
From the point of view of the physicist, a theory of matter is a policy rather than a creed; its object is to connect or co-ordinate apparently diverse phenomena, and above all to suggest, stimulate and direct experiment. It ought to furnish a compass which, if followed, will lead the observer further and further into previously unexplored regions.
The Corpuscular Theory of Matter (1907), 1.
From the point of view of the pure morphologist the recapitulation theory is an instrument of research enabling him to reconstruct probable lines of descent; from the standpoint of the student of development and heredity the fact of recapitulation is a difficult problem whose solution would perhaps give the key to a true understanding of the real nature of heredity.
Form and Function: A Contribution to the History of Animal Morphology (1916), 312-3.
Gauss once said, “Mathematics is the queen of the sciences and number theory the queen of mathematics.” If this is true we may add that the Disquisitions is the Magna Charter of number theory.
In Allgemeine Deutsche Biographie (1878, 8, 435. As cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 158.
General Theory of Relativity = Gravity too; referentially, eh?
— Anagram
Anagram by V. Rabin (2003) on anagramgenius.com website.
Generally speaking, geologists seem to have been much more intent on making little worlds of their own, than in examining the crust of that which they inhabit. It would be much more desirable that facts should be placed in the foreground and theories in the distance, than that theories should be brought forward at the expense of facts. So that, in after times, when the speculations of the present day shall have passed away, from a greater accumulation of information, the facts may be readily seized and converted to account.
Sections and Views Illustrative of Geological Phenomena (1830), iv.
Genetics is the first biological science which got in the position in which physics has been in for many years. One can justifiably speak about such a thing as theoretical mathematical genetics, and experimental genetics, just as in physics. There are some mathematical geniuses who work out what to an ordinary person seems a fantastic kind of theory. This fantastic kind of theory nevertheless leads to experimentally verifiable prediction, which an experimental physicist then has to test the validity of. Since the times of Wright, Haldane, and Fisher, evolutionary genetics has been in a similar position.
Oral history memoir. Columbia University, Oral History Research Office, New York, 1962. Quoted in William B. Provine, Sewall Wright and Evolutionary Biology (1989), 277.
Genetics is to biology what atomic theory is to physics. Its principle is clear: that inheritance is based on particles and not on fluids. Instead of the essence of each parent mixing, with each child the blend of those who made him, information is passed on as a series of units. The bodies of successive generations transport them through time, so that a long-lost character may emerge in a distant descendant. The genes themselves may be older than the species that bear them.
Almost Like a Whale: The Origin of Species Updated (1999), 115.
Genetics was, I would say, the first part of biology to become a pretty good theoretical subject, based on the theory of the gene and patterns of inheritance of characteristics.
From interview with Neil A. Campbell, in 'Crossing the Boundaries of Science', BioScience (Dec 1986), 36, No. 11, 738.
Geologists have not been slow to admit that they were in error in assuming that they had an eternity of past time for the evolution of the earth’s history. They have frankly acknowledged the validity of the physical arguments which go to place more or less definite limits to the antiquity of the earth. They were, on the whole, disposed to acquiesce in the allowance of 100 millions of years granted to them by Lord Kelvin, for the transaction of the whole of the long cycles of geological history. But the physicists have been insatiable and inexorable. As remorseless as Lear’s daughters, they have cut down their grant of years by successive slices, until some of them have brought the number to something less than ten millions. In vain have the geologists protested that there must somewhere be a flaw in a line of argument which tends to results so entirely at variance with the strong evidence for a higher antiquity, furnished not only by the geological record, but by the existing races of plants and animals. They have insisted that this evidence is not mere theory or imagination, but is drawn from a multitude of facts which become hopelessly unintelligible unless sufficient time is admitted for the evolution of geological history. They have not been able to disapprove the arguments of the physicists, but they have contended that the physicists have simply ignored the geological arguments as of no account in the discussion.
'Twenty-five years of Geological Progress in Britain', Nature, 1895, 51, 369.
Germs of a theory, though in their present condition they are vague and formless … may be said to resemble stones in the quarry, rough and unhewn, but which may some time become corner-stones, columns, and entablatures in the future edifice.
In Report on the Geology of the High Plateaus of Utah (1880), 114.
Given a large mass of data, we can by judicious selection construct perfectly plausible unassailable theories—all of which, some of which, or none of which may be right.
I-Ching and the citric acid cycle. Unpublished manuscript/seminar notes quoted in Frederick Grinnell, Everyday Practice of Science (2008), 86.
God runs electromagnetics on Monday, Wednesday, and Friday by the wave theory, and the devil runs it by quantum theory on Tuesday, Thursday, and Saturday.
In Daniel J. Kevles, The Physicists (1978), 159, citing interview by David Webster, AIP, p.35.
Good lawyers know that in many cases where the decisions are correct, the reasons that are given to sustain them may be entirely wrong. This is a thousand times more likely to be true in the practice of medicine than in that of the law, and hence the impropriety, not to say the folly, in spending your time in the discussion of medical belief and theories of cure that are more ingenious and seductive than they are profitable.
Introductory lecture (22 Sep 1885), Hahnemann Medical College, Chicago, printed in United States Medical Investigator (1885), 21, 526.
Great scientific discoveries have been made by men seeking to verify quite erroneous theories about the nature of things.
From 'Wordsworth in the Tropics', in Life and Letters and the London Mercury (1928), Vol. 1, 349.
Great theories are expansive; failures mire us in dogmatism and tunnel vision.
…...
Half a century ago Oswald (1910) distinguished classicists and romanticists among the scientific investigators: the former being inclined to design schemes and to use consistently the deductions from working hypotheses; the latter being more fit for intuitive discoveries of functional relations between phenomena and therefore more able to open up new fields of study. Examples of both character types are Werner and Hutton. Werner was a real classicist. At the end of the eighteenth century he postulated the theory of “neptunism,” according to which all rocks including granites, were deposited in primeval seas. It was an artificial scheme, but, as a classification system, it worked quite satisfactorily at the time. Hutton, his contemporary and opponent, was more a romanticist. His concept of “plutonism” supposed continually recurrent circuits of matter, which like gigantic paddle wheels raise material from various depths of the earth and carry it off again. This is a very flexible system which opens the mind to accept the possible occurrence in the course of time of a great variety of interrelated plutonic and tectonic processes.
In 'The Scientific Character of Geology', The Journal of Geology (Jul 1961), 69, No. 4, 456-7.
He [Lord Bacon] appears to have been utterly ignorant of the discoveries which had just been made by Kepler’s calculations … he does not say a word about Napier’s Logarithms, which had been published only nine years before and reprinted more than once in the interval. He complained that no considerable advance had been made in Geometry beyond Euclid, without taking any notice of what had been done by Archimedes and Apollonius. He saw the importance of determining accurately the specific gravities of different substances, and himself attempted to form a table of them by a rude process of his own, without knowing of the more scientific though still imperfect methods previously employed by Archimedes, Ghetaldus and Porta. He speaks of the εὕρηκα of Archimedes in a manner which implies that he did not clearly appreciate either the problem to be solved or the principles upon which the solution depended. In reviewing the progress of Mechanics, he makes no mention either of Archimedes, or Stevinus, Galileo, Guldinus, or Ghetaldus. He makes no allusion to the theory of Equilibrium. He observes that a ball of one pound weight will fall nearly as fast through the air as a ball of two, without alluding to the theory of acceleration of falling bodies, which had been made known by Galileo more than thirty years before. He proposed an inquiry with regard to the lever,—namely, whether in a balance with arms of different length but equal weight the distance from the fulcrum has any effect upon the inclination—though the theory of the lever was as well understood in his own time as it is now. … He speaks of the poles of the earth as fixed, in a manner which seems to imply that he was not acquainted with the precession of the equinoxes; and in another place, of the north pole being above and the south pole below, as a reason why in our hemisphere the north winds predominate over the south.
From Spedding’s 'Preface' to De Interpretations Naturae Proœmium, in The Works of Francis Bacon (1857), Vol. 3, 511-512. [Note: the Greek word “εὕρηκα” is “Eureka” —Webmaster.]
He [Samuel Johnson] bid me always remember this, that after a system is well settled upon positive evidence, a few objections ought not to shake it. “The human mind is so limited that it cannot take in all parts of a subject; so that there may be objections raised against anything. There are objections against a plenum, and objections against a vacuum. Yet one of them must certainly be true.”
Note: Whereas vacuum means devoid of matter, plenum regards a space with matter throughout.
He who has mastered the Darwinian theory, he who recognizes the slow and subtle process of evolution as the way in which God makes things come to pass, … sees that in the deadly struggle for existence that has raged throughout countless aeons of time, the whole creation has been groaning and travailing together in order to bring forth that last consummate specimen of God’s handiwork, the Human Soul
In The Destiny of Man Viewed in the Light of his Origin (1884), 32. Collected in Studies in Religion (1902), 19–20.
He who loves practice without theory is like a seafarer who boards ship without wheel or compass and knows not wither he travels.
From the original Italian: “Quelli che s’inamorā di pratica sāza sciētia, sō come ’l nochiere che ēstra navilio sanza timone o bussola che mai à certezza dove si uada.” Italian and English in Jean Paul Richter (trans), G. 8a, 'General Introduction to the Book on Painting', The Literary Works of Leonardo da Vinci (1883), Vol. 1, 18. Also seen translated as “He who loves practice without theory is like the sailor who boards ship without a rudder and compass and never knows where he may cast,” in Morris Kline, Mathematical Thought From Ancient to Modern Times (1972), 224.
He who wishes to explain Generation must take for his theme the organic body and its constituent parts, and philosophize about them; he must show how these parts originated, and how they came to be in that relation in which they stand to each other. But he who learns to know a thing not only from its phenomena, but also its reasons and causes; and who, therefore, not by the phenomena merely, but by these also, is compelled to say: “The thing must be so, and it cannot be otherwise; it is necessarily of such a character; it must have such qualities; it is impossible for it to possess others”—understands the thing not only historically but truly philosophically, and he has a philosophic knowledge of it. Our own Theory of Generation is to be such a philosphic comprehension of an organic body, a very different one from one merely historical. (1764)
Quoted as an epigraph to Chap. 2, in Ernst Haeckel, The Evolution of Man, (1886), Vol 1, 25.
Here I shall present, without using Analysis [mathematics], the principles and general results of the Théorie, applying them to the most important questions of life, which are indeed, for the most part, only problems in probability. One may even say, strictly speaking, that almost all our knowledge is only probable; and in the small number of things that we are able to know with certainty, in the mathematical sciences themselves, the principal means of arriving at the truth—induction and analogy—are based on probabilities, so that the whole system of human knowledge is tied up with the theory set out in this essay.
Philosophical Essay on Probabilities (1814), 5th edition (1825), trans. Andrew I. Dale (1995), 1.
His [Henry Cavendish’s] Theory of the Universe seems to have been, that it consisted solely of a multitude of objects which could be weighed, numbered, and measured; and the vocation to which he considered himself called was, to weigh, number and measure as many of those objects as his allotted three-score years and ten would permit. This conviction biased all his doings, alike his great scientific enterprises, and the petty details of his daily life.
In George Wilson, The Life of the Honourable Henry Cavendish: Including the Abstracts of his Important Scientific Papers (1851), 186.
His [Isaac Newton’s] observations of the colours of thin films [were] the origin of the next great theoretical advance, which had to await, over a hundred years, the coming of Thomas Young.
In 'Foreword' to Isaac Newton, Opticks: Or, A Treatise of the Reflections, Refractions, Inflections & Colours of Light. Based on the 4th Ed., London, 1730 (1952), lix-lx.
His [Sherlock Holmes] ignorance was as remarkable as his knowledge. … he was ignorant of the Copernican Theory and of the composition of the Solar System. … “But the Solar System!" I protested. “What the deuce is it to me?” he interrupted impatiently; “you say that we go round the sun. If we went round the moon it would not make a pennyworth of difference to me or to my work.”
In 'The Science Of Deduction', A Study In Scarlet (1887, 1904), 15-16.
His [Thomas Edison] method was inefficient in the extreme, for an immense ground had to be covered to get anything at all unless blind chance intervened and, at first, I was almost a sorry witness of his doings, knowing that just a little theory and calculation would have saved him 90 per cent of the labor. But he had a veritable contempt for book learning and mathematical knowledge, trusting himself entirely to his inventor's instinct and practical American sense. In view of this, the truly prodigious amount of his actual accomplishments is little short of a miracle.
As quoted in 'Tesla Says Edison Was an Empiricist', The New York Times (19 Oct 1931), 25. In 1884, Tesla had moved to America to assist Edison in the designing of motors and generators.
Historical theories are, after all, intellectual apple carts. They are quite likely to be upset. Nor should it be forgotten that they tend to attract, when they gain ascendancy, a fair number of apple-polishers
'Books of the Times'. New York Times (9 Dec 1965), 45.
How strange it would be if the final theory were to be discovered in our lifetimes! The discovery of the final laws of nature will mark a discontinuity in human intellectual history, the sharpest that has occurred since the beginning of modern science in the seventeenth century. Can we now imagine what that would be like?
In Dreams of a Final Theory (1992), 235.
How then did we come to the “standard model”? And how has it supplanted other theories, like the steady state model? It is a tribute to the essential objectivity of modern astrophysics that this consensus has been brought about, not by shifts in philosophical preference or by the influence of astrophysical mandarins, but by the pressure of empirical data.
In The First Three Minutes: A Modern View of the Origin of the Universe (1977), 9.
How would we express in terms of the statistical theory the marvellous faculty of a living organism, by which it delays the decay into thermodynamical equilibrium (death)? … It feeds upon negative entropy … Thus the device by which an organism maintains itself stationary at a fairly high level of orderliness (= fairly low level of entropy) really consists in continually sucking orderliness from its environment.
In 'Organization Maintained by Extracting “Order” from the Environment', What is Life? : The Physical Aspect of the Living Cell (1944), 74.
However far the calculating reason of the mathematician may seem separated from the bold flight of the artist’s phantasy, it must be remembered that these expressions are but momentary images snatched arbitrarily from among the activities of both. In the projection of new theories the mathematician needs as bold and creative a phantasy as the productive artist, and in the execution of the details of a composition the artist too must calculate dispassionately the means which are necessary for the successful consummation of the parts. Common to both is the creation, the generation, of forms out of mind.
From Die Entwickelung der Mathematik im Zusammenhange mit der Ausbreitung der Kultur (1893), 4. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 185. From the original German, “Wie weit auch der rechnende Verstand des Mathematikers von dem kühnen Fluge der Phantasie des Künstlers getrennt zu sein scheint, so bezeichnen diese Ausdrücke doch blosse Augenblicksbilder, die willkürlich aus der Thätigkeit Beider herausgerissen sind. Bei dem Entwurfe neuer Theorieen bedarf der Mathematiker einer ebenso kühnen und schöpferischen Phantasie wie der schaffende Künstler, und bei der Ausführung der Einzelheiten eines Werkes muss auch der Künstler kühl alle Mittel berechnen, welche zum Gelingen der Theile erforderlich sind. Gemeinsam ist Beiden die Hervorbringung, die Erzeugung der Gebilde aus dem Geiste.”
However far the mathematician’s calculating senses seem to be separated from the audacious flight of the artist’s imagination, these manifestations refer to mere instantaneous images, which have been arbitrarily torn from the operation of both. In designing new theories, the mathematician needs an equally bold and inspired imagination as creative as the artist, and in carrying out the details of a work the artist must unemotionally reckon all the resources necessary for the success of the parts. Common to both is the fabrication, the creation of the structure from the intellect.
From Die Entwickelung der Mathematik im Zusammenhange mit der Ausbreitung der Kultur (1893), 4. Translated by Webmaster using online resources. From the original German, “Wie weit auch der rechnende Verstand des Mathematikers von dem kühnen Fluge der Phantasie des Künstlers getrennt zu sein scheint, so bezeichnen diese Ausdrücke doch blosse Augenblicksbilder, die willkürlich aus der Thätigkeit Beider herausgerissen sind. Bei dem Entwurfe neuer Theorieen bedarf der Mathematiker einer ebenso kühnen und schöpferischen Phantasie wie der schaffende Künstler, und bei der Ausführung der Einzelheiten eines Werkes muss auch der Künstler kühl alle Mittel berechnen, welche zum Gelingen der Theile erforderlich sind. Gemeinsam ist Beiden die Hervorbringung, die Erzeugung der Gebilde aus dem Geiste.”
Human consciousness is just about the last surviving mystery. A mystery is a phenomenon that people don’t know how to think about—yet. There have been other great mysteries: the mystery of the origin of the universe, the mystery of life and reproduction, the mystery of the design to be found in nature, the mysteries of time, space, and gravity. These were not just areas of scientific ignorance, but of utter bafflement and wonder. We do not yet have the final answers to any of the questions of cosmology and particle physics, molecular genetics and evolutionary theory, but we do know how to think about them. The mysteries haven't vanished, but they have been tamed. They no longer overwhelm our efforts to think about the phenomena, because now we know how to tell the misbegotten questions from the right questions, and even if we turn out to be dead wrong about some of the currently accepted answers, we know how to go about looking for better answers. With consciousness, however, we are still in a terrible muddle. Consciousness stands alone today as a topic that often leaves even the most sophisticated thinkers tongue-tied and confused. And, as with all the earlier mysteries, there are many who insist—and hope—that there will never be a demystification of consciousness.
Consciousness Explained (1991), 21-22.
Human language is in some ways similar to, but in other ways vastly different from, other kinds of animal communication. We simply have no idea about its evolutionary history, though many people have speculated about its possible origins. There is, for instance, the “bow-bow” theory, that language started from attempts to imitate animal sounds. Or the “ding-dong” theory, that it arose from natural sound-producing responses. Or the “pooh-pooh” theory, that it began with violent outcries and exclamations.
We have no way of knowing whether the kinds of men represented by the earliest fossils could talk or not…
Language does not leave fossils, at least not until it has become written.
We have no way of knowing whether the kinds of men represented by the earliest fossils could talk or not…
Language does not leave fossils, at least not until it has become written.
Man in Nature (1961), 10.
Hygiene is the corruption of medicine by morality. It is impossible to find a
hygienist who does not debase his theory of the healthful with a theory of the virtuous. 3. The aim of medicine is surely not to make men virtuous; it is to safeguard them from the consequences of their vices.
In 'The Physician', Prejudices: third series (1922), 269.
I always love geology. In winter, particularly, it is pleasant to listen to theories about the great mountains one visited in the summer; or about the Flood or volcanoes; about great catastrophes or about blisters; above all about fossils … Everywhere there are hypotheses, but nowhere truths; many workmen, but no experts; priests, but no God. In these circumstances each man can bring his hypothesis like a candle to a burning altar, and on seeing his candle lit declare ‘Smoke for smoke, sir, mine is better than yours’. It is precisely for this reason that I love geology.
In Nouvelles Genevoises (1910), 306. First edition, 1841.
I am a firm believer in the theory that you can do or be anything that you wish in this world, within reason, if you are prepared to make the sacrifices, think and work hard enough and long enough.
From Cameron Prize Lecture (1928), delivered before the University of Edinburgh. As quoted and cited in Editorial Section, 'Sir Frederick Banting', Canadian Public Health Journal (May 1941), 32, No. 5, 266-267.
I am afraid all we can do is to accept the paradox and try to accommodate ourselves to it, as we have done to so many paradoxes lately in modern physical theories. We shall have to get accustomed to the idea that the change of the quantity R, commonly called the 'radius of the universe', and the evolutionary changes of stars and stellar systems are two different processes, going on side by side without any apparent connection between them. After all the 'universe' is an hypothesis, like the atom, and must be allowed the freedom to have properties and to do things which would be contradictory and impossible for a finite material structure.
Kosmos (1932), 133.
I am convinced that it is impossible to expound the methods of induction in a sound manner, without resting them upon the theory of probability. Perfect knowledge alone can give certainty, and in nature perfect knowledge would be infinite knowledge, which is clearly beyond our capacities. We have, therefore, to content ourselves with partial knowledge—knowledge mingled with ignorance, producing doubt.
The Principles of Science: A Treatise on Logic and Scientific Method, 2nd edition (1877), 197.
I am more fond of achieving than striving. My theories must prove to be facts or be discarded as worthless. My efforts must soon be crowned with success, or discontinued.
The Rest of My Life, ch. 4 (1937).Wells wrote, in addition to other works, the popular 'Fleming Stone' detective series.
I am now convinced that we have recently become possessed of experimental evidence of the discrete or grained nature of matter, which the atomic hypothesis sought in vain for hundreds and thousands of years. The isolation and counting of gaseous ions, on the one hand, which have crowned with success the long and brilliant researches of J.J. Thomson, and, on the other, agreement of the Brownian movement with the requirements of the kinetic hypothesis, established by many investigators and most conclusively by J. Perrin, justify the most cautious scientist in now speaking of the experimental proof of the atomic nature of matter, The atomic hypothesis is thus raised to the position of a scientifically well-founded theory, and can claim a place in a text-book intended for use as an introduction to the present state of our knowledge of General Chemistry.
In Grundriss der allgemeinen Chemie (4th ed., 1909), Preface, as cited by Erwin N. Hiebert and Hans-Gunther Korber in article on Ostwald in Charles Coulston Gillespie (ed.), Dictionary of Scientific Biography Supplement 1, Vol 15-16, 464.
I am of the decided opinion, that mathematical instruction must have for its first aim a deep penetration and complete command of abstract mathematical theory together with a clear insight into the structure of the system, and doubt not that the instruction which accomplishes this is valuable and interesting even if it neglects practical applications. If the instruction sharpens the understanding, if it arouses the scientific interest, whether mathematical or philosophical, if finally it calls into life an esthetic feeling for the beauty of a scientific edifice, the instruction will take on an ethical value as well, provided that with the interest it awakens also the impulse toward scientific activity. I contend, therefore, that even without reference to its applications mathematics in the high schools has a value equal to that of the other subjects of instruction.
In 'Ueber das Lehrziel im mathemalischen Unterricht der höheren Realanstalten', Jahresbericht der Deutschen Mathematiker Vereinigung, 2, 192. (The Annual Report of the German Mathematical Association. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 73.
I am particularly concerned to determine the probability of causes and results, as exhibited in events that occur in large numbers, and to investigate the laws according to which that probability approaches a limit in proportion to the repetition of events. That investigation deserves the attention of mathematicians because of the analysis required. It is primarily there that the approximation of formulas that are functions of large numbers has its most important applications. The investigation will benefit observers in identifying the mean to be chosen among the results of their observations and the probability of the errors still to be apprehended. Lastly, the investigation is one that deserves the attention of philosophers in showing how in the final analysis there is a regularity underlying the very things that seem to us to pertain entirely to chance, and in unveiling the hidden but constant causes on which that regularity depends. It is on the regularity of the main outcomes of events taken in large numbers that various institutions depend, such as annuities, tontines, and insurance policies. Questions about those subjects, as well as about inoculation with vaccine and decisions of electoral assemblies, present no further difficulty in the light of my theory. I limit myself here to resolving the most general of them, but the importance of these concerns in civil life, the moral considerations that complicate them, and the voluminous data that they presuppose require a separate work.
Philosophical Essay on Probabilities (1825), trans. Andrew I. Dale (1995), Introduction.
I argued that it was important not to place too much reliance on any single piece of experimental evidence. It might turn out to be misleading, as the 5.1 Å reflection undoubtedly was. Jim was a little more brash, stating that no good model ever accounted for all the facts, since some data was bound to be misleading if not plain wrong. A theory that did fit all the data would have been “carpentered” to do so and would thus be open to suspicion.
In What Mad Pursuit: A Personal View of Scientific Discovery (1988), 59-60.
I asked Fermi whether he was not impressed by the agreement between our calculated numbers and his measured numbers. He replied, “How many arbitrary parameters did you use for your calculations?" I thought for a moment about our cut-off procedures and said, “Four." He said, “I remember my friend Johnny von Neumann used to say, with four parameters I can fit an elephant, and with five I can make him wiggle his trunk.” With that, the conversation was over.
As given in 'A Meeting with Enrico Fermi', Nature (22 Jan 2004), 427, 297. As quoted in Steven A. Frank, Dynamics of Cancer: Incidence, Inheritance, and Evolution (2007), 87. Von Neumann meant nobody need be impressed when a complex model fits a data set well, because if manipulated with enough flexible parameters, any data set can be fitted to an incorrect model, even one that plots a curve on a graph shaped like an elephant!
I beg to present Columbus as a man of science and a man of faith. As a scientist, considering the time in which he lived, he eminently deserves our respect. Both in theory and in practice he was one of the best geographers and cosmographers of the age.
Address, in Chicago (12 Oct 1892). In E.S. Werner (ed.), Werner's Readings and Recitations (1908), 71.
I believe that certain erroneous developments in particle theory ... are caused by a misconception by some physicists that it is possible to avoid philosophical arguments altogether. Starting with poor philosophy, they pose the wrong questions. It is only a slight exaggeration to say that good physics has at times been spoiled by poor philosophy.
In 'The Nature of Elementary Particles', collected in Elena Castellani, Interpreting Bodies: Classical and Quantum Objects in Modern Physics (1998), 211. From Physics Today (1976), 29, No. 3, 32.
I bet it would have been a lot of fun to work with Einstein. What I really respect about Einstein is his desire to throw aside all conventional modes and just concentrate on what seems to be the closest we can get to an accurate theory of nature.
As quoted by Christina Couch, '10 Questions for Alan Guth, Pioneer of the Inflationary Model of the Universe' (7 Jan 2016) on the website for NPR radio program Science Friday.
I can accept the theory of relativity as little as I can accept the existence of atoms and other such dogmas.
Professor of Physics at the University of Vienna, 1913, in The Book of Heroic Failures by Stephen Pile (1979).
I can assure you, reader, that in a very few hours, even during the first day, you will learn more natural philosophy about things contained in this book, than you could learn in fifty years by reading the theories and opinions of the ancient philosophers. Enemies of science will scoff at the astrologers: saying, where is the ladder on which they have climbed to heaven, to know the foundation of the stars? But in this respect I am exempt from such scoffing; for in proving my written reason, I satisfy sight, hearing, and touch: for this reason, defamers will have no power over me: as you will see when you come to see me in my little Academy.
The Admirable Discourses (1580), trans. Aurele La Rocque (1957), 27.
I can see him [Sylvester] now, with his white beard and few locks of gray hair, his forehead wrinkled o’er with thoughts, writing rapidly his figures and formulae on the board, sometimes explaining as he wrote, while we, his listeners, caught the reflected sounds from the board. But stop, something is not right, he pauses, his hand goes to his forehead to help his thought, he goes over the work again, emphasizes the leading points, and finally discovers his difficulty. Perhaps it is some error in his figures, perhaps an oversight in the reasoning. Sometimes, however, the difficulty is not elucidated, and then there is not much to the rest of the lecture. But at the next lecture we would hear of some new discovery that was the outcome of that difficulty, and of some article for the Journal, which he had begun. If a text-book had been taken up at the beginning, with the intention of following it, that text-book was most likely doomed to oblivion for the rest of the term, or until the class had been made listeners to every new thought and principle that had sprung from the laboratory of his mind, in consequence of that first difficulty. Other difficulties would soon appear, so that no text-book could last more than half of the term. In this way his class listened to almost all of the work that subsequently appeared in the Journal. It seemed to be the quality of his mind that he must adhere to one subject. He would think about it, talk about it to his class, and finally write about it for the Journal. The merest accident might start him, but once started, every moment, every thought was given to it, and, as much as possible, he read what others had done in the same direction; but this last seemed to be his real point; he could not read without finding difficulties in the way of understanding the author. Thus, often his own work reproduced what had been done by others, and he did not find it out until too late.
A notable example of this is in his theory of cyclotomic functions, which he had reproduced in several foreign journals, only to find that he had been greatly anticipated by foreign authors. It was manifest, one of the critics said, that the learned professor had not read Rummer’s elementary results in the theory of ideal primes. Yet Professor Smith’s report on the theory of numbers, which contained a full synopsis of Kummer’s theory, was Professor Sylvester’s constant companion.
This weakness of Professor Sylvester, in not being able to read what others had done, is perhaps a concomitant of his peculiar genius. Other minds could pass over little difficulties and not be troubled by them, and so go on to a final understanding of the results of the author. But not so with him. A difficulty, however small, worried him, and he was sure to have difficulties until the subject had been worked over in his own way, to correspond with his own mode of thought. To read the work of others, meant therefore to him an almost independent development of it. Like the man whose pleasure in life is to pioneer the way for society into the forests, his rugged mind could derive satisfaction only in hewing out its own paths; and only when his efforts brought him into the uncleared fields of mathematics did he find his place in the Universe.
A notable example of this is in his theory of cyclotomic functions, which he had reproduced in several foreign journals, only to find that he had been greatly anticipated by foreign authors. It was manifest, one of the critics said, that the learned professor had not read Rummer’s elementary results in the theory of ideal primes. Yet Professor Smith’s report on the theory of numbers, which contained a full synopsis of Kummer’s theory, was Professor Sylvester’s constant companion.
This weakness of Professor Sylvester, in not being able to read what others had done, is perhaps a concomitant of his peculiar genius. Other minds could pass over little difficulties and not be troubled by them, and so go on to a final understanding of the results of the author. But not so with him. A difficulty, however small, worried him, and he was sure to have difficulties until the subject had been worked over in his own way, to correspond with his own mode of thought. To read the work of others, meant therefore to him an almost independent development of it. Like the man whose pleasure in life is to pioneer the way for society into the forests, his rugged mind could derive satisfaction only in hewing out its own paths; and only when his efforts brought him into the uncleared fields of mathematics did he find his place in the Universe.
In Florian Cajori, Teaching and History of Mathematics in the United States (1890), 266-267.
I can still recall vividly how Freud said to me, “My dear Jung, promise me never to abandon the sexual theory. That is the most essential thing of all. You see, we must make a dogma of it, an unshakable bulwark” … In some astonishment I asked him, “A bulwark-against what?” To which he replied, “Against the black tide of mud”—and here he hesitated for a moment, then added—“of occultism.”
Memories, Dreams, Reflections (1963), 147-8.
I can well appreciate, Holy Father, that as soon as certain people realise that in these books which I have written about the Revolutions of the spheres of the universe I attribute certain motions to the globe of the Earth, they will at once clamour for me to be hooted off the stage with such an opinion.
'To His Holiness Pope Paul III', in Copernicus: On the Revolutions of the Heavenly Spheres (1543), trans. A.M. Duncan (1976), 23.
I cannot seriously believe in it [quantum theory] because the theory cannot be reconciled with the idea that physics should represent a reality in time and space, free from spooky actions at a distance [spukhafte Fernwirkungen].
Letter to Max Born (3 Mar 1947). In Born-Einstein Letters (1971), 158.
I conceived and developed a new geometry of nature and implemented its use in a number of diverse fields. It describes many of the irregular and fragmented patterns around us, and leads to full-fledged theories, by identifying a family of shapes I call fractals.
The Fractal Geometry of Nature (1977, 1983), Introduction, xiii.
I confess that Fermat’s Theorem as an isolated proposition has very little interest for me, for a multitude of such theorems can easily be set up, which one could neither prove nor disprove. But I have been stimulated by it to bring our again several old ideas for a great extension of the theory of numbers. Of course, this theory belongs to the things where one cannot predict to what extent one will succeed in reaching obscurely hovering distant goals. A happy star must also rule, and my situation and so manifold distracting affairs of course do not permit me to pursue such meditations as in the happy years 1796-1798 when I created the principal topics of my Disquisitiones arithmeticae. But I am convinced that if good fortune should do more than I expect, and make me successful in some advances in that theory, even the Fermat theorem will appear in it only as one of the least interesting corollaries.
In reply to Olbers' attempt in 1816 to entice him to work on Fermat's Theorem. The hope Gauss expressed for his success was never realised.
In reply to Olbers' attempt in 1816 to entice him to work on Fermat's Theorem. The hope Gauss expressed for his success was never realised.
Letter to Heinrich Olbers (21 Mar 1816). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 413.
I count Maxwell and Einstein, Eddington and Dirac, among “real” mathematicians. The great modern achievements of applied mathematics have been in relativity and quantum mechanics, and these subjects are at present at any rate, almost as “useless” as the theory of numbers.
In A Mathematician's Apology (1940, 2012), 131.
I do not approve of anything that tampers with natural ignorance. Ignorance is like a delicate exotic fruit; touch it and the bloom is gone. The whole theory of modern education is radically unsound. Fortunately in England, at any rate, education produces no effect whatsoever. If it did, it would prove a serious danger to the upper classes.
Lines for fictional Lady Bracknell in play, The Importance of Being Earnest. Collected in Plays (1898), 293-294. [Wilde is speaking his mind through her voice, in characteristic trenchant style, about the mind-boggling stupidity of the British aristocratic leisure class. Wilde is making a serious social and political point. Effective education could threaten the established order. If the English downtrodden poor knew anything about anything they probably would overthrow the ruling class. —condensed from sparknotes.com]
I esteem his understanding and subtlety highly, but I consider that they have been put to ill use in the greater part of his work, where the author studies things of little use or when he builds on the improbable principle of attraction.
Writing about Newton's Principia. Huygens had some time earlier indicated he did not believe the theory of universal gravitation, saying it 'appears to me absurd.'
Writing about Newton's Principia. Huygens had some time earlier indicated he did not believe the theory of universal gravitation, saying it 'appears to me absurd.'
Quoted in Archana Srinivasan, Great Inventors (2007), 37.
I first met J. Robert Oppenheimer on October 8, 1942, at Berkeley, Calif. There we discussed the theoretical research studies he was engaged in with respect to the physics of the bomb. Our discussions confirmed my previous belief that we should bring all of the widely scattered theoretical work together. … He expressed complete agreement, and it was then that the idea of the prompt establishment of a Los Alamos was conceived.”
In 'Some Recollections of July 16, 1945', Bulletin of the Atomic Scientists (Jun 1970), 26, No. 6, 21.
I forget whether you take in the Times; for the chance of your not doing so, I send the enclosed rich letter. It is, I am sure, by Fitz-Roy. … It is a pity he did not add his theory of the extinction of Mastodon, etc., from the door of the Ark being made too small.
Letter (5 Dec 1859) to Charles Lyell. In Francis Darwin and Albert Charles Seward (eds.), More Letters of Charles Darwin: A Record of his Work in a Series of Hitherto Unpublished Letters (103), Vol. 1, 129. The referenced letters in the Times were on 1 Dec and 5 Dec 1859, signed under the pseudonym “Senex”, on the topic of “Works of Art in the Drift.”
I grow daily to honor facts more and more, and theory less and less.
I had made considerable advance ... in calculations on my favourite numerical lunar theory, when I discovered that, under the heavy pressure of unusual matters (two transits of Venus and some eclipses) I had committed a grievous error in the first stage of giving numerical value to my theory. My spirit in the work was broken, and I have never heartily proceeded with it since.
[Concerning his calculations on the orbital motion of the Moon.]
[Concerning his calculations on the orbital motion of the Moon.]
Private note (29 Sep 1890). In George Biddell Airy and Wilfrid Airy (ed.), Autobiography of Sir George Biddell Airy (1896), 350.
I have a peculiar theory about radium, and I believe it is the correct one. I believe that there is some mysterious ray pervading the universe that is fluorescing to it. In other words, that all its energy is not self-constructed but that there is a mysterious something in the atmosphere that scientists have not found that is drawing out those infinitesimal atoms and distributing them forcefully and indestructibly.
Quoted in 'Edison Fears Hidden Perils of the X-Rays', New York World (3 Aug 1903), 1.
I have been scientifically studying the traits and dispositions of the “lower animals” (so-called,) and contrasting them with the traits and dispositions of man. I find the result profoundly humiliating to me. For it obliges me to renounce my allegiance to the Darwinian theory of the Ascent of Man from the Lower Animals; since it now seems plain to me that that theory ought to be vacated in favor of a new and truer one, this new and truer one to be named the Descent of Man from the Higher Animals.
From 'Man's Place in the Animal World' (1896) in What is Man?: and Other Philosophical Writings (1973), 81.
I have had [many letters] asking me,… how to start making a hobby out of astronomy. My answer is always the same. Do some reading, learn the basic facts, and then take a star-map and go outdoors on the first clear night so that you can begin learning the various stars and constellation patterns. The old cliche that ‘an ounce of practice is worth a ton of theory’ is true in astronomy, as it is in everything else.
From 'Introduction', The Amateur Astronomer (11th Ed., 1990), 1.
I have just finished my sketch of my species theory. If as I believe that my theory is true & if it be accepted even by one competent judge, it will be a considerable step in science. I therefore write this, in case of my sudden death, as my most solemn & last request, which I am sure you will consider the same as if legally entered in my will, that you will devote 400£ to its publication & further will yourself, or through Hensleigh [Wedgwood], take trouble in promoting it.
Letter to Emma Darwin, 5 July 1844. In F. Burkhardt and S. Smith (eds.), The Correspondence of Charles Darwin 1844-1846 (1987), Vol. 3, 43.
I have just got a new theory of eternity.
Alleged comment to the secretary of the Netherlands embassy, seated beside him at a National Academy of Sciences annual awards ceremony (1921), after listening to lengthy formal speeches. As quoted in Ronald W. Clark, Einstein: The Life and Times (1971), 389.
I have learnt that all our theories are not Truth itself, but resting places or stages on the way to the conquest of Truth, and that we must be contented to have obtained for the strivers after Truth such a resting place which, if it is on a mountain, permits us to view the provinces already won and those still to be conquered.
Liebig to Gilbert (25 Dec 1870). Rothamsted Archives. Quotation supplied by W. H. Brock.
I have lived myself to see the disciples of Hoffman, Boerhaave, Stalh, Cullen, Brown, succeed one another like the shifting figures of a magic lanthern, and their fancies, like the dresses of the annual doll-babies from Paris, becoming from their novelty, the vogue of the day, and yielding to the next novelty their ephemeral favor. The patient, treated on the fashionable theory, sometimes gets well in spite of the medicine.
In letter to Caspar Wistar (21 Jun 1807), collected in Thomas Jefferson Randolph (ed.), Memoir, Correspondence, And Miscellanies, From The Papers Of Thomas Jefferson (1829), Vol. 4, 93.
I have long recognized the theory and aesthetic of such comprehensive display: show everything and incite wonder by sheer variety. But I had never realized how power fully the decor of a cabinet museum can promote this goal until I saw the Dublin [Natural History Museum] fixtures redone right ... The exuberance is all of one piece–organic and architectural. I write this essay to offer my warmest congratulations to the Dublin Museum for choosing preservation–a decision not only scientifically right, but also ethically sound and decidedly courageous. The avant-garde is not an exclusive locus of courage; a principled stand within a reconstituted rear unit may call down just as much ridicule and demand equal fortitude. Crowds do not always rush off in admirable or defendable directions.
…...
I have no doubt that certain learned men, now that the novelty of the hypotheses in this work has been widely reported—for it establishes that the Earth moves, and indeed that the Sun is motionless in the middle of the universe—are extremely shocked, and think that the scholarly disciplines, rightly established once and for all, should not be upset. But if they are willing to judge the matter thoroughly, they will find that the author of this work has committed nothing which deserves censure. For it is proper for an astronomer to establish a record of the motions of the heavens with diligent and skilful observations, and then to think out and construct laws for them, or rather hypotheses, whatever their nature may be, since the true laws cannot be reached by the use of reason; and from those assumptions the motions can be correctly calculated, both for the future and for the past. Our author has shown himself outstandingly skilful in both these respects. Nor is it necessary that these hypotheses should be true, nor indeed even probable, but it is sufficient if they merely produce calculations which agree with the observations. … For it is clear enough that this subject is completely and simply ignorant of the laws which produce apparently irregular motions. And if it does work out any laws—as certainly it does work out very many—it does not do so in any way with the aim of persuading anyone that they are valid, but only to provide a correct basis for calculation. Since different hypotheses are sometimes available to explain one and the same motion (for instance eccentricity or an epicycle for the motion of the Sun) an astronomer will prefer to seize on the one which is easiest to grasp; a philosopher will perhaps look more for probability; but neither will grasp or convey anything certain, unless it has been divinely revealed to him. Let us therefore allow these new hypotheses also to become known beside the older, which are no more probable, especially since they are remarkable and easy; and let them bring with them the vast treasury of highly learned observations. And let no one expect from astronomy, as far as hypotheses are concerned, anything certain, since it cannot produce any such thing, in case if he seizes on things constructed for another other purpose as true, he departs from this discipline more foolish than he came to it.
Although this preface would have been assumed by contemporary readers to be written by Copernicus, it was unsigned. It is now believed to have been written and added at press time by Andreas Osiander (who was then overseeing the printing of the book). It suggests the earth’s motion as described was merely a mathematical device, and not to be taken as absolute reality. Text as given in 'To the Reader on the Hypotheses in this Work', Copernicus: On the Revolutions of the Heavenly Spheres (1543), translated by Alistair Matheson Duncan (1976), 22-3. By adding this preface, Osiander wished to stave off criticism by theologians. See also the Andreas Osiander Quotes page of this website.
I have no trouble publishing in Soviet astrophysical journals, but my work is unacceptable to the American astrophysical journals.
[Referring to the trouble he had with the peer reviewers of Anglo-American astrophysical journals because his ideas often conflicted with the generally accepted or “standard"” theories.]
[Referring to the trouble he had with the peer reviewers of Anglo-American astrophysical journals because his ideas often conflicted with the generally accepted or “standard"” theories.]
Quoted in Anthony L. Peratt, 'Dean of the Plasma Dissidents', Washington Times, supplement: The World and I (May 1988),197.
I have read various articles on the fourth dimension, the relativity theory of Einstein, and other psychological speculation on the constitution of the universe; and after reading them I feel as Senator Brandegee felt after a celebrated dinner in Washington. “I feel,” he said, “as if I had been wandering with Alice in Wonderland and had tea with the Mad Hatter.”
Quoted in Michio Kaku, Einstein's Cosmos: How Albert Einstein's vision Transformed Our Understanding of Space and Time (2005), 118-119. [Note:Brandegee's original remark was in the context of politics after a White House conference with President Wilson (Feb 1917), and unrelated to Einstein's theory.]
I have tried to read philosophers of all ages and have found many illuminating ideas but no steady progress toward deeper knowledge and understanding. Science, however, gives me the feeling of steady progress: I am convinced that theoretical physics is actual philosophy. It has revolutionized fundamental concepts, e.g., about space and time (relativity), about causality (quantum theory), and about substance and matter (atomistics), and it has taught us new methods of thinking (complementarity) which are applicable far beyond physics.
— Max Born
My Life & My Views (1968), 48.
I know very well the people you mean: they are all mind and theory and haven't the wit to sew on a button. Plenty of head but not hand enough to sew on a button.
Aphorism 75 in Notebook D (1773-1775), as translated by R.J. Hollingdale in Aphorisms (1990). Reprinted as The Waste Books (2000), 55.
I learnt to distrust all physical concepts as the basis for a theory. Instead one should put one's trust in a mathematical scheme, even if the scheme does not appear at first sight to be connected with physics. One should concentrate on getting interesting mathematics.
From a 1977 lecture. Quoted in Pesi Rustom Masani, Norbert Wiener, 1894-1964 (1990), 6.
I noticed affixed to a laboratory door the following words: “Les théories passent. Le Grenouille reste. [The theories pass. The frog remains.] —Jean Rostand, Carnets d’un biologiste.” There is a risk that in the less severe discipline of criticism the result may turn out to be different; the theories will remain but the frog may disappear.
In An Appetite for Poetry (1989), 5.
I once knew an otherwise excellent teacher who compelled his students to perform all their demonstrations with incorrect figures, on the theory that it was the logical connection of the concepts, not the figure, that was essential.
In Ernst Mach and Thomas Joseph McCormack, Space and Geometry (1906), 93.
I pass with relief from the tossing sea of Cause and Theory to the firm ground of Result and Fact.
I remember one occasion when I tried to add a little seasoning to a review, but I wasn’t allowed to. The paper was by Dorothy Maharam, and it was a perfectly sound contribution to abstract measure theory. The domains of the underlying measures were not sets but elements of more general Boolean algebras, and their range consisted not of positive numbers but of certain abstract equivalence classes. My proposed first sentence was: “The author discusses valueless measures in pointless spaces.”
In I Want to be a Mathematician: An Automathography (1985), 120.
I respect Kirkpatrick both for his sponges and for his numinous nummulosphere. It is easy to dismiss a crazy theory with laughter that debars any attempt to understand a man’s motivation–and the nummulosphere is a crazy theory. I find that few men of imagination are not worth my attention. Their ideas may be wrong, even foolish, but their methods often repay a close study ... The different drummer often beats a fruitful tempo.
…...
I shall explain a System of the World differing in many particulars from any yet known, answering in all things to the common Rules of Mechanical Motions: This depends upon three Suppositions. First, That all Cœlestial Bodies whatsoever, have an attraction or gravitating power towards their own Centers, whereby they attract not only their own parts, and keep them from flying from them, as we may observe the Earth to do, but that they do also attract all the other Cœlestial bodies that are within the sphere of their activity; and consequently that not only the Sun and Moon have an influence upon the body and motion the Earth, and the Earth upon them, but that Mercury also Venus, Mars, Saturn and Jupiter by their attractive powers, have a considerable influence upon its motion in the same manner the corresponding attractive power of the Earth hath a considerable influence upon every one of their motions also. The second supposition is this, That all bodies whatsoever that are put into a direct and simple motion, will continue to move forward in a streight line, till they are by some other effectual powers deflected and bent into a Motion, describing a Circle, Ellipse, or some other more compounded Curve Line. The third supposition is, That these attractive powers are so much the more powerful in operating, by how much the nearer the body wrought upon is to their own Centers. Now what these several degrees are I have not yet experimentally verified; but it is a notion, which if fully prosecuted as it ought to be, will mightily assist the Astronomer to reduce all the Cœlestial Motions to a certain rule, which I doubt will never be done true without it. He that understands the nature of the Circular Pendulum and Circular Motion, will easily understand the whole ground of this Principle, and will know where to find direction in Nature for the true stating thereof. This I only hint at present to such as have ability and opportunity of prosecuting this Inquiry, and are not wanting of Industry for observing and calculating, wishing heartily such may be found, having myself many other things in hand which I would first compleat and therefore cannot so well attend it. But this I durst promise the Undertaker, that he will find all the Great Motions of the World to be influenced by this Principle, and that the true understanding thereof will be the true perfection of Astronomy.
An Attempt to Prove the Motion of the Earth from Observations (1674), 27-8. Based on a Cutlerian Lecture delivered by Hooke at the Royal Society four years earlier.
I should like to call the number of atom groups, with which an elementary atom coordinates … to form a complex radical, the coordination number of the atom in question … We must differentiate between valence number and coordination number. The valence number indicates the maximum number of monovalent atoms which can be bound directly to the atom in question without the participation of other elementary atoms … Perhaps this concept [of coordination number] is destined to serve as a basis for the theory of the constitution of inorganic compounds, just as valence theory formed the basis for the constitutional theory of carbon compounds.
In 'Beitrag zur Konstitution anorganischer Verbindungen', Zeitschrift fur anorganische Chemie, (1893), 3, 267-330. Translated in George G. Kauffman (ed.), Classics in Coordination Chemistry: Part I: The Selected Papers of Alfred Werner (1968), 84-87.
I sometimes ask myself how it came about that I was the one to develop the theory of relativity. The reason, I think, is that a normal adult never stops to think about the problem of space and time. These are things which he has thought of as a child. But my intellectual development was retarded, as a result of which I began to wonder about space and time only when I had already grown up.
In Ronald W. Clark, Einstein: The Life and Times (1971), 10.
I suppose that Dr. [Florence] Sabin is the most eminent of living women scientists. The knowledge she has derived from her studies has led to better understanding of the anatomy, physiology, and pathology of the body in health and in disease, and has been not only of theoretical but of practical value. It is of the nature of conspicuous social service to have added to the knowledge of our bodies, well and ill, and thus to have helped make them better instruments for the fulfilment of the purposes of society as a whole.
In Genevieve Parkhurst, 'Dr. Sabin, Scientist: Winner Of Pictorial Review’s Achievement Award', Pictorial Review (Jan 1930), 2.
I therefore took this opportunity and also began to consider the possibility that the Earth moved. Although it seemed an absurd opinion, nevertheless, because I knew that others before me had been granted the liberty of imagining whatever circles they wished to represent the phenomena of the stars, I thought that I likewise would readily be allowed to test whether, by assuming some motion of the Earth's, more dependable representations than theirs could be found for the revolutions of the heavenly spheres.
'To His Holiness Pope Paul III', in Copernicus: On the Revolutions of the Heavenly Spheres (1543), trans. A. M. Duncan (1976), 26.
I think a strong claim can be made that the process of scientific discovery may be regarded as a form of art. This is best seen in the theoretical aspects of Physical Science. The mathematical theorist builds up on certain assumptions and according to well understood logical rules, step by step, a stately edifice, while his imaginative power brings out clearly the hidden relations between its parts. A well constructed theory is in some respects undoubtedly an artistic production. A fine example is the famous Kinetic Theory of Maxwell. ... The theory of relativity by Einstein, quite apart from any question of its validity, cannot but be regarded as a magnificent work of art.
Responding to the toast, 'Science!' at the Royal Academy of the Arts in 1932.)
Responding to the toast, 'Science!' at the Royal Academy of the Arts in 1932.)
Quoted in Lawrence Badash, 'Ernest Rutherford and Theoretical Physics,' in Robert Kargon and Peter Achinstein (eds.) Kelvin's Baltimore Lectures and Modern Theoretical Physics: Historical and Philosophical Perspectives (1987), 352.
I think the name atomic theory was an unfortunate one. We talk fluently about atoms as the smallest particles that exist, and chemists regard them as indivisible … To my mind the infinitely small is as incomprehensible as the infinitely great. … we cannot comprehend it, we cannot take it in. And so with the atom. Therefore I think that it would have been better to have taken a different word—say minim—which would have been a safer term than atom.
Address, in 'Report to the Chemical Society's Jubilee', Nature (26 Mar 1891), 43, 493.
I think the next [21st] century will be the century of complexity. We have already discovered the basic laws that govern matter and understand all the normal situations. We don’t know how the laws fit together, and what happens under extreme conditions. But I expect we will find a complete unified theory sometime this century. The is no limit to the complexity that we can build using those basic laws.
[Answer to question: Some say that while the twentieth century was the century of physics, we are now entering the century of biology. What do you think of this?]
[Answer to question: Some say that while the twentieth century was the century of physics, we are now entering the century of biology. What do you think of this?]
'"Unified Theory" Is Getting Closer, Hawking Predicts', interview in San Jose Mercury News (23 Jan 2000), 29A. Answer quoted in Ashok Sengupta, Chaos, Nonlinearity, Complexity: The Dynamical Paradigm of Nature (2006), vii. Question included in Hans-Joachim Schellnhuber, Nicholas Stern and Mario Molina , Global Sustainability: a Nobel Cause (2010), 13. Cite from Brent Davis and Dennis J. Sumara, Complexity and Education: Inquiries Into Learning, Teaching, and Research (2006), 171.
I want to argue that the ‘sudden’ appearance of species in the fossil record and our failure to note subsequent evolutionary change within them is the proper prediction of evolutionary theory as we understand it ... Evolutionary ‘sequences’ are not rungs on a ladder, but our retrospective reconstruction of a circuitous path running like a labyrinth, branch to branch, from the base of the bush to a lineage now surviving at its top.
…...
I wanted some new names to express my facts in Electrical science without involving more theory than I could help & applied to a friend Dr Nicholl [his doctor], who has given me some that I intend to adopt for instance, a body decomposable by the passage of the Electric current, I call an ‘electrolyte’ and instead of saying that water is electro chemically decomposed I say it is ‘electrolyzed’. The intensity above which a body is decomposed beneath which it conducts without decomposition I call the ‘Electrolyte intensity’ &c &c. What have been called: the poles of the battery I call the electrodes they are not merely surfaces of metal, but even of water & air, to which the term poles could hardly apply without receiving a new sense. Electrolytes must consist of two parts which during the electrolization, are determined the one in the one direction, and the other towards the poles where they are evolved; these evolved substances I call zetodes, which are therefore the direct constituents of electrolites.
Letter to William Whewell (24 Apr 1834). In Frank A. J. L. James (ed.), The Correspondence of Michael Faraday: Volume 2, 1832-1840 (1993), 176.
I was pretty good in science. But again, because of the small budget, in science class we couldn’t do experiments in order to prove theories. We just believed everything. Actually I think that class was call Religion. Religion was always an easy class. All you had to do was suspend the logic and reasoning you were taught in all the other classes.
In autobiography, Brain Droppings (1998), 227.
I was sitting in a chair in the patent office at Bern when all of a sudden a thought occurred to me: “If a person falls freely he will not feel his own weight.” I was startled. This simple thought made a deep impression on me. It impelled me toward a theory of gravitation.
Lecture in Japan (1922). The quote is footnoted in Michael White, John Gribbin, Einstein: a Life in Science (1995), 128, saying the talk is known as the 'Kyoto address', reported in J. Ishiwara, Einstein Koen-Roku (1977).
I was there when Abbe Georges Lemaître first proposed this [Big Bang] theory. ... There is no rational reason to doubt that the universe has existed indefinitely, for an infinite time. .... It is only myth that attempts to say how the universe came to be, either four thousand or twenty billion years ago.
[Expressing his belief that the Big Bang is a myth devised to explain creation. He said he heard Lemaître (who was, at the time both a member of the Catholic hierarchy and an accomplished scientist) say in private that this theory was a way to reconcile science with St. Thomas Aquinas' theological dictum of creatio ex nihilo—creation out of nothing.]
[Expressing his belief that the Big Bang is a myth devised to explain creation. He said he heard Lemaître (who was, at the time both a member of the Catholic hierarchy and an accomplished scientist) say in private that this theory was a way to reconcile science with St. Thomas Aquinas' theological dictum of creatio ex nihilo—creation out of nothing.]
Quoted in Anthony L. Peratt, 'Dean of the Plasma Dissidents', Washington Times, supplement: The World and I (May 1988),196.
I was very impressed that one simple theory could incorporate so much physics
…...
I went to Polynesia to study how animals had reached oceanic islands, carried by winds and currents. I came home with a controversial theory of how man had reached these islands in prehistoric times.
In 'Foreword to the 35th Anniversary Edition', Kon-Tiki: Across the Pacific by Raft (1990), xii.
I will simply express my strong belief, that that point of self-education which consists in teaching the mind to resist its desires and inclinations, until they are proved to be right, is the most important of all, not only in things of natural philosophy, but in every department of dally life.
'Observations On Mental Education', a lecture before the Prince Consort and the Royal Institution, 6 May 1854. Experimental researches in chemistry and physics (1859), 477.
I worked on true Baconian principles, and without any theory collected facts.
cit. Gavin de Beer in Autobiographies: Charles Darwin, Thomas Henry Huxley, 1974
I would trade all my experimental works for the single idea of the benzene theory.
Quoted by B. L. Lepsius in 'Hofmann und die Deutsche Chemische Gesellschaft', Berichte der Deutschen Chemischen Gesellschaft (1918), 51, 51.
I, however, believe that for the ripening of experience the light of an intelligent theory is required. People are amused by the witticism that the man with a theory forces from nature that answer to his question which he wishes to have but nature never answers unless she is questioned, or to speak more accurately, she is always talking to us and with a thousand tongues but we only catch the answer to our own question.
Quoted in Major Greenwood, Epidemiology Historical and Experimental. The Herter Lectures for 1931 (1932), 13.
If a mathematician of the past, an Archimedes or even a Descartes, could view the field of geometry in its present condition, the first feature to impress him would be its lack of concreteness. There are whole classes of geometric theories which proceed not only without models and diagrams, but without the slightest (apparent) use of spatial intuition. In the main this is due, to the power of the analytic instruments of investigations as compared with the purely geometric.
In 'The Present Problems in Geometry', Bulletin American Mathematical Society (1906), 286.
If a scientist uncovers a publishable fact, it will become central to his theory.
'Mann’s Law,' in 'Advanced Researchmanship,' Murphy’s Law Book Two (1980).
If all the individual facts, all the individual phenomena, were directly accessible to us, as we ask for the knowledge of them; no science would ever have arisen.
English translation by Webmaster from the original German, “Wenn uns alle einzelnen Thatsachen, alle einzelnen Erscheinungen unmittelbar zugänglich wären, so wie wir nach der Kenntniss derselben verlangen; so wäre nie eine Wissenschaft entstanden.” In Die Geschichte und die Wurzel des Satzes von der Erhaltung der Arbeit (1872), 30-31. Also found translated as “If all single facts, all separate phenomena, were as directly accessible to us as we demand that knowledge of them to be; science would never have arisen,” in Ernst Cassirer, The Problem of Knowledge: Philosophy, Science, and History since Hegel (1950), 108. Citing from
If all this damned quantum jumping were really here to stay, I should be sorry, I should be sorry I ever got involved with quantum theory.
As reported by Heisenberg describing Schrödinger’s time spent debating with Bohr in Copenhagen (Sep 1926). In Werner Heisenberg, Physics and Beyond: Encounters and Conversations (1971), 75. As cited in John Gribbin, Erwin Schrodinger and the Quantum Revolution.
If an idea presents itself to us, we must not reject it simply because it does not agree with the logical deductions of a reigning theory.
If any human being earnestly desire to push on to new discoveries instead of just retaining and using the old; to win victories over Nature as a worker rather than over hostile critics as a disputant; to attain, in fact, clear and demonstrative knowlegde instead of attractive and probable theory; we invite him as a true son of Science to join our ranks.
Novum Organum (1620), 34, Preface.
If Darwin were alive today the insect world would delight and astound him with its impressive verification of his theories of the survival of the fittest. Under the stress of intensive chemical spraying the weaker members of the insect populations are being weeded out… . Only the strong and fit remain to defy our efforts to control them.
In Silent Spring (1952, 1962), 263.
If Einstein’s theory [of relativity] should prove to be correct, as I expect it will, he will be considered the Copernicus of the twentieth century.
As quoted in Philipp Frank and Shuichi Kusaka, Einstein, His Life and Times (1947), 101.
If even in science there is no a way of judging a theory but by assessing the number, faith and vocal energy of its supporters, then this must be even more so in the social sciences: truth lies in power.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 9.
If faith cannot be reconciled with rational thinking, it has to be eliminated as an anachronistic remnant of earlier stages of culture and replaced by science dealing with facts and theories which are intelligible and can be validated.
In Man for Himself: An Inquiry into the Psychology of Ethics (1947), 197.
If he [Thomas Edison] had a needle to find in a haystack, he would not stop to reason where it was most likely to be, but would proceed at once with the feverish diligence of a bee, to examine straw after straw until he found the object of his search. … [J]ust a little theory and calculation would have saved him ninety percent of his labor.
As quoted in 'Tesla Says Edison Was an Empiricist', The New York Times (19 Oct 1931), 25. In 1884, Tesla had moved to America to assist Edison in the designing of motors and generators.
If history is any guide at all, it seems to me to suggest that there is a final theory. In this century we have seen a convergence of the arrows of explanation, like the convergence of meridians toward the North Pole.
In Dreams of a Final Theory: The Scientist's Search for the Ultimate Laws of Nature (1992), 232.
If I get the impression that Nature itself makes the decisive choice [about] what possibility to realize, where quantum theory says that more than one outcome is possible, then I am ascribing personality to Nature, that is to something that is always everywhere. [An] omnipresent eternal personality which is omnipotent in taking the decisions that are left undetermined by physical law is exactly what in the language of religion is called God.
As quoted by John D. Barrow in The Universe that Discovered Itself (2000), 171.
If in a discussion of many matters … we are not able to give perfectly exact and self-consistent accounts, do not be surprised: rather we would be content if we provide accounts that are second to none in probability.
— Plato
Timaeus. Quoted in Robert J. Scully, The Demon and the Quantum (2007), 20.
If indeed the Earth is, in its own slow way, a very dynamic body and we have regarded it as essentially static, we need to discard most of our old theories and books and start again with a new viewpoint and a new science.
In 'Reply to Beloussov', Geotimes (1968), 13, No. 12, 22. The public disagreement on seafloor spreading between Wilson and V.V. Beloussov is collected in Brainerd Mears, The Nature of Geology: Contemporary Readings (1970).
If it be urged that the action of the potato is chemical and mechanical only, and that it is due to the chemical and mechanical effects of light and heat, the answer would seem to lie in an enquiry whether every sensation is not chemical and mechanical in its operation? Whether those things which we deem most purely spiritual are anything but disturbances of equilibrium in an infinite series of levers, beginning with those that are too small for microscopic detection, and going up to the human arm and the appliances which it makes use of? Whether there be not a molecular action of thought, whence a dynamical theory of the passions shall be deducible?
In Erewhon, Or, Over the Range (1872), 192.
If it could be demonstrated that any complex organ existed which could not possibly have been formed by numerous, successive slight modifications, my theory would absolutely break down.
From On the Origin of Species by Means of Natural Selection; or, The Preservation of Favoured Races in the Struggle for Life (1861), 171.
If my theory of relativity is proven successful, Germany will claim me as a German and France will declare I am a citizen of the world. Should my theory prove untrue, France will say I am a German and Germany will declare I am a Jew.
In Speech (6 Apr 1922) to the French Philosophical Society, Sorbonne. Quoted in Alice Calaprice, The Quotable Einstein (1996), 8.
If the aim of physical theories is to explain experimental laws, theoretical physics is not an autonomous science; it is subordinate to metaphysics.
The Aim and Structure of Physical Theory (1906), 2nd edition (1914), trans. Philip P. Wiener (1954), 10.
If the world has begun with a single quantum, the notions of space and would altogether fail to have any meaning at the beginning; they would only begin to have a sensible meaning when the original quantum had been divided into a sufficient number of quanta. If this suggestion is correct, the beginning of the world happened a little before the beginning of space and time. I think that such a beginning of the world is far enough from the present order of Nature to be not at all repugnant. It may be difficult to follow up the idea in detail as we are not yet able to count the quantum packets in every case. For example, it may be that an atomic nucleus must be counted as a unique quantum, the atomic number acting as a kind of quantum number. If the future development of quantum theory happens to turn in that direction, we could conceive the beginning of the universe in the form of a unique atom, the atomic weight of which is the total mass of the universe. This highly unstable atom would divide in smaller and smaller atoms by a kind of super-radioactive process.
In a seminal short letter (457 words), 'The Beginning of the World from the Point of View of Quantum Theory', Nature (9 May 1931), 127, 706.
If the world may be thought of as a certain definite quantity of force and as a certain definite number of centers of force—and every other representation remains indefinite and therefore useless—it follows that, in the great dice game of existence, it must pass through calculable number of combinations. In infinite time, every possible combination would at some time or another be realized; more: it would be realized an infinite number of times. And since between every combination and its next recurrence all other possible combinations would have to take place, and each of these combination conditions of the entire sequence of combinations in the same series, a circular movement of absolutely identical series is thus demonstrated: the world as a circular movement that has already repeated itself infinitely often and plays its game in infinitum. This conception is not simply a mechanistic conception; for if it were that, it would not condition an infinite recurrence of identical cases, but a final state. Because the world has not reached this, mechanistic theory must be considered an imperfect and merely provisional hypothesis.
The Will to Power (Notes written 1883-1888), book 4, no. 1066. Trans. W. Kaufmann and R. J. Hollingdale and ed. W. Kaufmann (1968), 549.
If there is something very slightly wrong in our definition of the theories, then the full mathematical rigor may convert these errors into ridiculous conclusions.
Feynman Lectures on Gravitation, edited by Brian Hatfield (2002), 21.
If this plane were to crash, we could get a new start on this quasar problem.
Said to colleagues, dramatically cupping his hand over his brow, shortly after the take-off of a propeller plane leaving Austin, Texas, after the Second Texas Symposium for Relativistic Astrophysics in Dec 1964. Various different theories had been presented at the conference. The flight passengers included many of the major scientists in quasar research, including Margaret and Geoffrey Burbridge, Subrahmanyan Chandrasekhar, John Wheeler and Maarten Schmidt.
Said to colleagues, dramatically cupping his hand over his brow, shortly after the take-off of a propeller plane leaving Austin, Texas, after the Second Texas Symposium for Relativistic Astrophysics in Dec 1964. Various different theories had been presented at the conference. The flight passengers included many of the major scientists in quasar research, including Margaret and Geoffrey Burbridge, Subrahmanyan Chandrasekhar, John Wheeler and Maarten Schmidt.
As quoted by Arthur I. Miller, Empire of the Stars (2005), 226.
If we consider that part of the theory of relativity which may nowadays in a sense be regarded as bone fide scientific knowledge, we note two aspects which have a major bearing on this theory. The whole development of the theory turns on the question of whether there are physically preferred states of motion in Nature (physical relativity problem). Also, concepts and distinctions are only admissible to the extent that observable facts can be assigned to them without ambiguity (stipulation that concepts and distinctions should have meaning). This postulate, pertaining to epistemology, proves to be of fundamental importance.
'Fundamental ideas and problems of the theory of relativity', Lecture delivered to the Nordic Assembly of Naturalists at Gothenburg, 11 Jul 1923. In Nobel Physics 1901-1921 (1998), 482.
If we do discover a complete unified theory, it should be in time understandable in broad principle by everyone, not just a few scientists. Then we shall all, philosophers, scientists and just ordinary people, be able to take part in the discussion of why it is that we and the universe exist. If we find the answer to that, it would be the ultimate triumph of human reason—for then we would know the mind of God.
A Brief History of Time (1988), 191.
If we examine the accomplishments of man in his most advanced endeavors, in theory and in practice, we find that the cell has done all this long before him, with greater resourcefulness and much greater efficiency.
Nobel Lecture, The Coming Age of the Cell, 12 Dec 1974
If we look at the problems raised by Aristotle, we are astonished at his gift of observation. What wonderful eyes the Greeks had for many things! Only they committed the mistake of being overhasty, of passing straightway from the phenomenon to the explanation of it, and thereby produced certain theories that are quite inadequate. But this is the mistake of all times, and still made in our own day.
In The Maxims and Reflections of Goethe (1906), 195.
If we take quantum theory seriously as a picture of what’s really going on, each measurement does more than disturb: it profoundly reshapes the very fabric of reality.
…...
If you are out to describe the truth, leave elegance to the tailor.
On being reproached that his formula of gravitation was longer and more cumbersome than Newton’s.
On being reproached that his formula of gravitation was longer and more cumbersome than Newton’s.
Quoted in J. H. Mitchell, Writing for Professional and Technical Journals (1968), Introduction.
In theory there is no difference between theory and practice; but in practice, there is.
Attributed. Although widely seen, a reliable source is never cited. If you know a primary print source, please contact Webmaster. Occasionally also seen attributed to Yogi Berra, Chuck Reid, Grant Gainey or Jerry Percell. The earliest example found by Webmaster is in Walter J. Savitch, An Introduction to the Art and Science of Programming (1986), where it is merely attributed as "Remark overheard at a computer science conference."
In 1900 however, he [Planck] worked out the revolutionary quantum theory, a towering achievement which extended and improved the basic concepts of physics. It was so revolutionary, in fact, that almost no physicist, including Planck himself could bring himself to accept it. (Planck later said that the only way a revolutionary theory could be accepted was to wait until all the old scientists had died.)
(1976). In Isaac Asimov’s Book of Science and Nature Quotations (1988), 324.
In 1975, ... [speaking with Shiing Shen Chern], I told him I had finally learned ... the beauty of fiber-bundle theory and the profound Chern-Weil theorem. I said I found it amazing that gauge fields are exactly connections on fiber bundles, which the mathematicians developed without reference to the physical world. I added, “this is both thrilling and puzzling, since you mathematicians dreamed up these concepts out of nowhere.” He immediately protested: “No, no. These concepts were not dreamed up. They were natural and real.”
In 'Einstein's Impact on Theoretical Physics', collected in Jong-Ping Hsu, Leonard Hsu (eds.), JingShin Theoretical Physics Symposium in Honor of Professor Ta-You Wu (1998), 70. Reprinted from Physics Today (Jun 1980), 49. The article was adapted from a talk given at the Second Marcel Grossman meeting, held in Trieste, Italy (Jul 1979), in honor of the 100th anniversary of the birth of Albert Einstein.
In a sense, of course, probability theory in the form of the simple laws of chance is the key to the analysis of warfare;… My own experience of actual operational research work, has however, shown that its is generally possible to avoid using anything more sophisticated. … In fact the wise operational research worker attempts to concentrate his efforts in finding results which are so obvious as not to need elaborate statistical methods to demonstrate their truth. In this sense advanced probability theory is something one has to know about in order to avoid having to use it.
In 'Operations Research', Physics Today (Nov 1951), 19. As cited by Maurice W. Kirby and Jonathan Rosenhead, 'Patrick Blackett (1897)' in Arjang A. Assad (ed.) and Saul I. Gass (ed.),Profiles in Operations Research: Pioneers and Innovators (2011), 25.
In addition to this it [mathematics] provides its disciples with pleasures similar to painting and music. They admire the delicate harmony of the numbers and the forms; they marvel when a new discovery opens up to them an unexpected vista; and does the joy that they feel not have an aesthetic character even if the senses are not involved at all? … For this reason I do not hesitate to say that mathematics deserves to be cultivated for its own sake, and I mean the theories which cannot be applied to physics just as much as the others.
(1897) From the original French, “Et surtout, leurs adeptes y trouvent des jouissances analogues á celles que donnent la peinture et la musique. Ils admirent la délicate harmonie des nombres et des formes; ils s’émerveillent quand une découverte nouvelle leur ouvre une perspective inattendue; et la joie qu’ils éprouvent ainsi n’a-t-elle pas le caractère esthétique, bien que les sens n’y prennent aucune part?...C’est pourquoi je n’hésite pas à dire que les mathématiques méritent d’être cultivées pour elles-mêmes et que les théories qui ne peuvent être appliquées á la physique doivent l’être comme les autres.” Address read for him at the First International Congress of Mathematicians in Zurich: '‘Sur les rapports de l’analyse pure et de la physique', in Proceedings of that Congress 81-90, (1898). Also published as 'L’Analyse et la Physique', in La Valeur de la Science (1905), 137-151. As translated in Armand Borel, 'On the Place of Mathematics in Culture', in Armand Borel: Œvres: Collected Papers (1983), Vol. 4, 420-421.
In an enterprise such as the building of the atomic bomb the difference between ideas, hopes, suggestions and theoretical calculations, and solid numbers based on measurement, is paramount. All the committees, the politicking and the plans would have come to naught if a few unpredictable nuclear cross sections had been different from what they are by a factor of two.
Epigraph in Richard Rhodes, The Making of the Atomic Bomb (1986), 8.
In chemistry, our theories are crutches; to show that they are valid, they must be used to walk... A theory established with the help of twenty facts must explain thirty, and lead to the discovery of ten more.
Leçons sur la Philosophie Chimique (1837), 60. Trans. S. Kapoor, 'Dumas and Organic Classification', Ambix, 1969, 16, 4.
In consequence of Darwin's reformed Theory of Descent, we are now in a position to establish scientifically the groundwork of a non-miraculous history of the development of the human race. ... If any person feels the necessity of conceiving the coming into existence of this matter as the work of a supernatural creative power, of the creative force of something outside of matter, we have nothing to say against it. But we must remark, that thereby not even the smallest advantage is gained for a scientific knowledge of nature. Such a conception of an immaterial force, which as the first creates matter, is an article of faith which has nothing whatever to do with human science.
In Ernst Haeckel and E. Ray Lankester (trans.), The History of Creation (1880), Vol. 1, 6-9.
In Darwin’s theory, you just have to substitute ‘mutations’ for his ‘slight accidental variations’ (just as quantum theory substitutes ‘quantum jump’ for ‘continuous transfer of energy’). In all other respects little change was necessary in Darwin’s theory.
…...
In degenerating programmes, however, theories are fabricated only in order to accommodate known facts.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 5.
In due time the evolution theory will have to abate its vehemence, cannot be allow’d to dominate everything else, and will have to take its place as a segment of the circle, the cluster—as but one of many theories, many thoughts, of profoundest value—and readjusting the differentiating much, yet leaving the divine secrets just as inexplicable and unreachable as before—maybe more so.
In Specimen days & Collect (1883), 326.
In fact, Gentlemen, no geometry without arithmetic, no mechanics without geometry... you cannot count upon success, if your mind is not sufficiently exercised on the forms and demonstrations of geometry, on the theories and calculations of arithmetic ... In a word, the theory of proportions is for industrial teaching, what algebra is for the most elevated mathematical teaching.
... a l'ouverture du cours de mechanique industrielle á Metz (1827), 2-3, trans. Ivor Grattan-Guinness.
In fact, it is often stated that of all the theories proposed in this century, the silliest is quantum theory. Some say that the only thing that quantum theory has going for it, in fact, is that it is unquestionably correct.
In Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and The Tenth Dimension (1994), 262.
In general, a fact is worth more than theories in the long run. The theory stimulates, but the fact builds. The former in due time is replaced by one better but the fact remains and becomes fertile.
Letter to Dr. E. B. Krumhaar (11 Oct 1933), in Journal of Bacteriology (Jan 1934), 27, No. 1, 20.
In general, art has preceded science. Men have executed great, and curious, and beautiful works before they had a scientific insight into the principles on which the success of their labours was founded. There were good artificers in brass and iron before the principles of the chemistry of metals were known; there was wine among men before there was a philosophy of vinous fermentation; there were mighty masses raised into the air, cyclopean walls and cromlechs, obelisks and pyramids—probably gigantic Doric pillars and entablatures—before there was a theory of the mechanical powers. … Art was the mother of Science.
Lecture (26 Nov 1851), to the London Society of Arts, 'The General Bearing of the Great Exhibition on the Progress of Art and Science', collected in Lectures on the Results of the Great Exhibition of 1851' (1852), 7-8.
In geometry I find certain imperfections which I hold to be the reason why this science, apart from transition into analytics, can as yet make no advance from that state in which it came to us from Euclid.
As belonging to these imperfections, I consider the obscurity in the fundamental concepts of the geometrical magnitudes and in the manner and method of representing the measuring of these magnitudes, and finally the momentous gap in the theory of parallels, to fill which all efforts of mathematicians have so far been in vain.
As belonging to these imperfections, I consider the obscurity in the fundamental concepts of the geometrical magnitudes and in the manner and method of representing the measuring of these magnitudes, and finally the momentous gap in the theory of parallels, to fill which all efforts of mathematicians have so far been in vain.
In Geometric Researches on the Theory of Parallels (1840), as translated by George Bruce Halstead (new ed. 1914) 11.
In geometry, as in most sciences, it is very rare that an isolated proposition is of immediate utility. But the theories most powerful in practice are formed of propositions which curiosity alone brought to light, and which long remained useless without its being able to divine in what way they should one day cease to be so. In this sense it may be said, that in real science, no theory, no research, is in effect useless.
In 'Geometry', A Philosophical Dictionary, (1881), Vol. l, 374.
In its essence, the theory of natural selection is primarily an attempt to give an account of the probable mechanism of the origin of the adaptations of the organisms to their environment, and only secondarily an attempt to explain evolution at large. Some modern biologists seem to believe that the word 'adaptation' has teleological connotations, and should therefore be expunged from the scientific lexicon. With this we must emphatically disagree. That adaptations exist is so evident as to be almost a truism, although this need not mean that ours is the best of all possible worlds. A biologist has no right to close his eyes to the fact that the precarious balance between a living being and its environment must be preserved by some mechanism or mechanisms if life is to endure.
Genetics and Origin of Species (1937), 150.
In less than eight years “The Origin of Species” has produced conviction in the minds of a majority of the most eminent living men of science. New facts, new problems, new difficulties as they arise are accepted, solved, or removed by this theory; and its principles are illustrated by the progress and conclusions of every well established branch of human knowledge.
From a review of four books on the subject 'Mimicry, and Other Protective Resemblances Among Animals', in The Westminster Review (Jul 1867), 88, 1. Wallace is identified as the author in the article as reprinted in William Beebe, The Book of Naturalists: An Anthology of the Best Natural History (1988), 108.
In my intercourse with mankind, I have always found those who would thrust theory into practical matters to be, at bottom, men of no judgement and pure quacks.
Quoted in James Kip Finch, Engineering Classics of James Kip Finch.
In my opinion the English excel in the art of writing text-books for mathematical teaching; as regards the clear exposition of theories and the abundance of excellent examples, carefully selected, very few books exist in other countries which can compete with those of Salmon and many other distinguished English authors that could be named.
In Projective Geometry (1886), Preface.
In my own view, some advice about what should be known, about what technical education should be acquired, about the intense motivation needed to succeed, and about the carelessness and inclination toward bias that must be avoided is far more useful than all the rules and warnings of theoretical logic.
From Reglas y Consejos sobre Investigacíon Cientifica: Los tónicos de la voluntad. (1897), as translated by Neely and Larry W. Swanson, in Advice for a Young Investigator (1999), 6.
In my view all salvation for philosophy may be expected to come from Darwin’s theory.
In Ludwig Boltzmann and B. McGuinness (ed.), Theoretical Physics and Philosophical Problems: Selected Writings (1974), 193.
In October 1838, that is, fifteen months after I had begun my systematic enquiry, I happened to read for amusement Malthus on Population, and being well prepared to appreciate the struggle for existence which everywhere goes on from long-continued observation of the habits of animals and plants, it at once struck me that under these circumstances favourable variations would tend to be, preserved, and unfavourable ones to be destroyed. The result of this would be the formation of new species. Here, then, I had at last got a theory by which to work; but I was so anxious to avoid prejudice, that I determined not for some time to write even the briefest sketch of it.
In Charles Darwin and Francis Darwin (ed.), Charles Darwin: His Life Told in an Autobiographical Chapter, and in a Selected Series of His Published Letters (1892), 40.
In our day grand generalizations have been reached. The theory of the origin of species is but one of them. Another, of still wider grasp and more radical significance, is the doctrine of the Conservation of Energy, the ultimate philosophical issues of which are as yet but dimly seem-that doctrine which “binds nature fast in fate” to an extent not hitherto recognized, exacting from every antecedent its equivalent consequent, and bringing vital as well as physical phenomena under the dominion of that law of causal connexion which, so far as the human understanding has yet pierced, asserts itself everywhere in nature.
'Address Delivered Before The British Association Assembled at Belfast', (19 Aug 1874). Fragments of Science for Unscientific People: A Series of Detached Essays, Lectures, and Reviews (1892), Vol. 2, 1801.
In point of fact, no conclusive disproof of a theory can ever be produced; for it is always possible to say that the experimental results are not reliable or that the discrepancies which are asserted to exist between the experimental results and the theory are only apparent and that they will disappear with the advance of our understanding. If you insist on strict proof (or strict disproof) in the empirical sciences, you will never benefit from experience, and never learn from it how wrong you are.
The Logic of Scientific Discovery: Logik Der Forschung (1959, 2002), 28.
In science “fact” can only mean “confirmed to such a degree that it would be perverse to withhold provisional assent.” I suppose that apples might start to rise tomorrow, but the possibility does not merit equal time in physics classrooms.
'Evolution as Fact and Theory', in Hen’s Teeth and Horse’s Toes: Further Reflections in Natural History (1983), 255.
In scientific study, or, as I prefer to phrase it, in creative scholarship, the truth is the single end sought; all yields to that. The truth is supreme, not only in the vague mystical sense in which that expression has come to be a platitude, but in a special, definite, concrete sense. Facts and the immediate and necessary inductions from facts displace all pre-conceptions, all deductions from general principles, all favourite theories. Previous mental constructions are bowled over as childish play-structures by facts as they come rolling into the mind. The dearest doctrines, the most fascinating hypotheses, the most cherished creations of the reason and of the imagination perish from a mind thoroughly inspired with the scientific spirit in the presence of incompatible facts. Previous intellectual affections are crushed without hesitation and without remorse. Facts are placed before reasonings and before ideals, even though the reasonings and the ideals be more beautiful, be seemingly more lofty, be seemingly better, be seemingly truer. The seemingly absurd and the seemingly impossible are sometimes true. The scientific disposition is to accept facts upon evidence, however absurd they may appear to our pre-conceptions.
The Ethical Functions of Scientific Study: An Address Delivered at the Annual Commencement of the University of Michigan, 28 June 1888, 7-8.
In scientific thought we adopt the simplest theory which will explain all the facts under consideration and enable us to predict new facts of the same kind. The catch in this criterion lies in the world “simplest.” It is really an aesthetic canon such as we find implicit in our criticisms of poetry or painting. The layman finds such a law as dx/dt = κ(d²x/dy²) much less simple than “it oozes,” of which it is the mathematical statement. The physicist reverses this judgment, and his statement is certainly the more fruitful of the two, so far as prediction is concerned. It is, however, a statement about something very unfamiliar to the plain man, namely the rate of change of a rate of change.
In 'Science and Theology as Art-Forms', Possible Worlds (1927), 227.
In the 1860s, Pasteur not only applied his germ theory to create “Pasteurization,” rescuing France’s wine and vinegar industries, but also found both the cause and cure of silkworm disease, saving growers millions of dollars. When Napoleon asked the scientist why he had not legitimately profited by his findings, Pasteur replied: “In France scientists would consider they lowered themselves by doing so.”
In Jacques Cousteau and Susan Schiefelbein, The Human, the Orchid, and the Octopus: Exploring and Conserving Our Natural World (2007), 190.
In the beginning of the year 1665 I found the Method of approximating series & the Rule for reducing any dignity of any Bionomial into such a series. The same year in May I found the method of Tangents of Gregory & Slusius, & in November had the direct method of fluxions & the next year in January had the Theory of Colours & in May following I had entrance into ye inverse method of fluxions. And the same year I began to think of gravity extending to ye orb of the Moon & (having found out how to estimate the force with wch [a] globe revolving within a sphere presses the surface of the sphere) from Keplers rule of the periodic times of the Planets being in sesquialterate proportion of their distances from the center of their Orbs, I deduced that the forces wch keep the Planets in their Orbs must [be] reciprocally as the squares of their distances from the centers about wch they revolve: & thereby compared the force requisite to keep the Moon in her Orb with the force of gravity at the surface of the earth, & found them answer pretty nearly. All this was in the two plague years of 1665-1666. For in those days I was in the prime of my age for invention & minded Mathematicks & Philosophy more then than at any time since.
Quoted in Richard Westfall, Never at Rest: A Biography of Isaac Newton (1980), 143.
In the days when geology was young, now some two hundred years ago, it found a careful foster-mother in theology, who watched over its early growth with anxious solicitude, and stored its receptive mind with the most beautiful stories, which the young science never tired of transforming into curious fancies of its own, which it usually styled “theories of the earth.”
In British Association Address to Workingmen, 'Geology and Deluges', published in Nature (1984), 50, 505-510. Also printed in Popular Science Monthly (Dec 1894), 46 245.
In the expressions we adopt to prescribe physical phenomena we necessarily hover between two extremes. We either have to choose a word which implies more than we can prove, or we have to use vague and general terms which hide the essential point, instead of bringing it out. The history of electrical theories furnishes a good example.
Opening Address to the Annual Meeting of the British Association by Prof. Arthur Schuster, in Nature (4 Aug 1892), 46, 325.
In the field of thinking, the whole history of science from geocentrism to the Copernican revolution, from the false absolutes of Aristotle’s physics to the relativity of Galileo’s principle of inertia and to Einstein’s theory of relativity, shows that it has taken centuries to liberate us from the systematic errors, from the illusions caused by the immediate point of view as opposed to “decentered” systematic thinking.
As quoted in D. E. Berlyne, Structure and Direction in Thinking (1965), 232.
In the history of physics, there have been three great revolutions in thought that first seemed absurd yet proved to be true. The first proposed that the earth, instead of being stationary, was moving around at a great and variable speed in a universe that is much bigger than it appears to our immediate perception. That proposal, I believe, was first made by Aristarchos two millenia ago ... Remarkably enough, the name Aristarchos in Greek means best beginning.
[The next two revolutions occurred ... in the early part of the twentieth century: the theory of relativity and the science of quantum mechanics...]
[The next two revolutions occurred ... in the early part of the twentieth century: the theory of relativity and the science of quantum mechanics...]
Edward Teller with Judith L. Shoolery, Memoirs: A Twentieth-Century Journey in Science and Politics (2001), 562.
In the past we see that periods of great intellectual activity have followed certain events which have acted by freeing the mind from dogma, extending the domain in which knowledge can be sought, and stimulating the imagination. … [For example,] the development of the cell theory and the theory of evolution.
From address, 'A Medical Retrospect'. Published in Yale Medical Journal (Oct 1910), 17, No. 2, 59.
In the present age the human mind must be coerced into theoretical studies; it runs of
its own accord to practical applications.
In Alexis de Tocqueville and Henry Reeve (trans.), Democracy in America (1840, 1899), Vol. 2, 48.
In the vestibule of the Manchester Town Hall are placed two life-sized marble statues facing each other. One of these is that of John Dalton … the other that of James Prescott Joule. … Thus honour is done to Manchester’s two greatest sons—to Dalton, the founder of modern Chemistry and of the Atomic Theory, and the laws of chemical-combining proportions; to Joule, the founder of modern Physics and the discoverer of the Law of Conservation of Energy. The one gave to the world the final and satisfactory proof … that in every kind of chemical change no loss of matter occurs; the other proved that in all the varied modes of physical change, no loss of energy takes place.
In John Dalton and the Rise of Modern Chemistry (1895), 7.
In the world of science different levels of esteem are accorded to different kinds of specialist. Mathematicians have always been eminently respectable, and so are those who deal with hard lifeless theories about what constitutes the physical world: the astronomers, the physicists, the theoretical chemists. But the more closely the scientist interests himself in matters which are of direct human relevance, the lower his social status. The real scum of the scientific world are the engineers and the sociologists and the psychologists. Indeed, if a psychologist wants to rate as a scientist he must study rats, not human beings. In zoology the same rules apply. It is much more respectable to dissect muscle tissues in a laboratory than to observe the behaviour of a living animal in its natural habitat.
From transcript of BBC radio Reith Lecture (12 Nov 1967), 'A Runaway World', on the bbc.co.uk website.
In the year 1666 he retired again from Cambridge... to his mother in Lincolnshire & whilst he was musing in a garden it came into his thought that the power of gravity (wch brought an apple from the tree to the ground) was not limited to a certain distance from the earth but that this power must extend much farther than was usually thought. Why not as high as the moon said he to himself & if so that must influence her motion & perhaps retain her in her orbit, whereupon he fell a calculating what would be the effect of that supposition but being absent from books & taking the common estimate in use among Geographers & our seamen before Norwood had measured the earth, that 60 English miles were contained in one degree of latitude on the surface of the Earth his computation did not agree with his theory & inclined him then to entertain a notion that together with the force of gravity there might be a mixture of that force wch the moon would have if it was carried along in a vortex.
[The earliest account of Newton, gravity and an apple.]
[The earliest account of Newton, gravity and an apple.]
Memorandum of a conversation with Newton in August 1726. Quoted in Richard Westfall, Never at Rest: A Biography of Isaac Newton (1980), 154.
In the year 1902 (while I was attempting to explain to an elementary class in chemistry some of the ideas involved in the periodic law) becoming interested in the new theory of the electron, and combining this idea with those which are implied in the periodic classification, I formed an idea of the inner structure of the atom which, although it contained certain crudities, I have ever since regarded as representing essentially the arrangement of electrons in the atom ... In accordance with the idea of Mendeleef, that hydrogen is the first member of a full period, I erroneously assumed helium to have a shell of eight electrons. Regarding the disposition in the positive charge which balanced the electrons in the neutral atom, my ideas were very vague; I believed I inclined at that time toward the idea that the positive charge was also made up of discrete particles, the localization of which determined the localization of the electrons.
Valence and the Structure of Atoms and Molecules (1923), 29-30.
In theory one is aware that the earth revolves but in practice one does not perceive it, the ground on which one treads seems not to move, and one can live undisturbed. So it is with Time in one's life. (1918)
'À l’ombre des jeunes filles en fleurs', À la recherche du temps perdu (1913-27).
In theory, whole islands of antimatter could be floating in the universe, cut off from matter by the empty void of space. If a large chunk of antimatter fell to Earth, the planet would be vaporized in a blinding flash of energy.
In these researches I followed the principles of the experimental method that we have established, i.e., that, in presence of a well-noted, new fact which contradicts a theory, instead of keeping the theory and abandoning the fact, I should keep and study the fact, and I hastened to give up the theory.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 164.

In-depth studies have an influence on general ideas, whereas theories, in turn, in order to maintain themselves, push their spectators to search for new evidence. The mind’s activity that is maintained by the debates about these works, is probably the source of the greatest joys given to man to experience on Earth.
La théorie des glaciers et ses progrès les plus récents. Bibl. universelle de Geneve, (3), Vol. 41, p. 139. Trans. Karin Verrecchia.
Indeed, nothing more beautifully simplifying has ever happened in the history of science than the whole series of discoveries culminating about 1914 which finally brought practically universal acceptance to the theory that the material world contains but two fundamental entities, namely, positive and negative electrons, exactly alike in charge, but differing widely in mass, the positive electron—now usually called a proton—being 1850 times heavier than the negative, now usually called simply the electron.
Time, Matter and Values (1932), 46. Cited in Karl Raimund Popper and William Warren Bartley (ed.), Quantum Theory and theSchism in Physics (1992), 37.
Indeed, the most important part of engineering work—and also of other scientific work—is the determination of the method of attacking the problem, whatever it may be, whether an experimental investigation, or a theoretical calculation. … It is by the choice of a suitable method of attack, that intricate problems are reduced to simple phenomena, and then easily solved.
In Engineering Mathematics: A Series of Lectures Delivered at Union College (1911, 1917), Vol. 2, 275.
Indeed, this epistemological theory of the relation between theory and experiment differs sharply from the epistemological theory of naive falsificationism.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 35.
Induction and analogy are the special characteristics of modern mathematics, in which theorems have given place to theories and no truth is regarded otherwise than as a link in an infinite chain. “Omne exit in infinitum” is their favorite motto and accepted axiom.
In 'A Plea for the Mathematician', Nature, Vol. 1, 861. [The Latin phrase “Omne exit in infinitum” means “Everything goes to infinity”.
Investigators are commonly said to be engaged in a search for the truth. I think they themselves would usually state their aims less pretentiously. What the experimenter is really trying to do is to learn whether facts can be established which will be recognized as facts by others and which will support some theory that in imagination he has projected. But he must be ingenuously honest. He must face facts as they arise in the course of experimental procedure, whether they are favourable to his idea or not. In doing this he must be ready to surrender his theory at any time if the facts are adverse to it.
The Way of an Investigator: A Scientist's Experiences in Medical Research (1945), 34.
Is evolution a theory, a system or a hypothesis? It is much more: it is a general condition to which all theories, all hypotheses, all systems must bow and which they must satisfy henceforth if they are to be thinkable and true. Evolution is a light illuminating all facts, a curve that all lines must follow. ... The consciousness of each of us is evolution looking at itself and reflecting upon itself....Man is not the center of the universe as once we thought in our simplicity, but something much more wonderful—the arrow pointing the way to the final unification of the world in terms of life. Man alone constitutes the last-born, the freshest, the most complicated, the most subtle of all the successive layers of life. ... The universe has always been in motion and at this moment continues to be in motion. But will it still be in motion tomorrow? ... What makes the world in which we live specifically modern is our discovery in it and around it of evolution. ... Thus in all probability, between our modern earth and the ultimate earth, there stretches an immense period, characterized not by a slowing-down but a speeding up and by the definitive florescence of the forces of evolution along the line of the human shoot.
In The Phenomenon of Man (1975), pp 218, 220, 223, 227, 228, 277.
Is it not true that the doctrine of attraction and gravity has done nothing but astonish our imagination? Is it not true that all chemical discoveries have done only the same?
Letter to Jean le Rond D'Alembert (7 Jan 1768). Collected in Correspondence: Letters Between Frederick II and M. D’Alembert (1789), 79, as translated by Thomas Holcroft.
It appears that anything you say about the way that theory and experiment may interact is likely to be correct, and anything you say about the way that theory and experiment must interact is likely to be wrong.
In Dreams of a Final Theory: The Scientist's Search for the Ultimate Laws of Nature (1992), 128.
It did not cause anxiety that Maxwell’s equations did not apply to gravitation, since nobody expected to find any link between electricity and gravitation at that particular level. But now physics was faced with an entirely new situation. The same entity, light, was at once a wave and a particle. How could one possibly imagine its proper size and shape? To produce interference it must be spread out, but to bounce off electrons it must be minutely localized. This was a fundamental dilemma, and the stalemate in the wave-photon battle meant that it must remain an enigma to trouble the soul of every true physicist. It was intolerable that light should be two such contradictory things. It was against all the ideals and traditions of science to harbor such an unresolved dualism gnawing at its vital parts. Yet the evidence on either side could not be denied, and much water was to flow beneath the bridges before a way out of the quandary was to be found. The way out came as a result of a brilliant counterattack initiated by the wave theory, but to tell of this now would spoil the whole story. It is well that the reader should appreciate through personal experience the agony of the physicists of the period. They could but make the best of it, and went around with woebegone faces sadly complaining that on Mondays, Wednesdays, and Fridays they must look on light as a wave; on Tuesdays, Thursdays, and Saturdays, as a particle. On Sundays they simply prayed.
The Strange Story of the Quantum (1947), 42.
It does appear that on the whole a physicist… tries to reduce his theory at all times to as few parameters as possible and is inclined to feel that a theory is a “respectable” one, though by no means necessarily correct, if in principle it does offer reasonably specific means for its possible refutation. Moreover the physicist will generally arouse the irritation amongst fellow physicists if he is not prepared to abandon his theory when it clashes with subsequent experiments. On the other hand it would appear that the chemist regards theories—or perhaps better his theories (!) —as far less sacrosanct, and perhaps in extreme cases is prepared to modify them continually as each bit of new experimental evidence comes in.
'Discussion: Physics and Chemistry: Comments on Caldin's View of Chemistry', British Journal of the Philosophy of Science, 1960, 11, 222.
It doesn’t matter how beautiful your theory is, it doesn’t matter how smart you are. If it doesn’t agree with experiment, it’s wrong.
…...
It follows from the theory of relativity that mass and energy are both different manifestations of the same thing—a somewhat unfamiliar conception for the average man. Furthermore E=MC2, in which energy is put equal to mass multiplied with the square of the velocity of light, showed that a very small amount of mass may be converted into a very large amount of energy... the mass and energy were in fact equivalent.
As expressed in the Einstein film, produced by Nova Television (1979). Quoted in Alice Calaprice, The Quotable Einstein (1996), 183.
It has been said by a distinguished philosopher that England is “usually the last to enter into the general movement of the European mind.” The author of the remark probably meant to assert that a man or a system may have become famous on the continent, while we are almost ignorant of the name of the man and the claims of his system. Perhaps, however, a wider range might be given to the assertion. An exploded theory or a disadvantageous practice, like a rebel or a patriot in distress, seeks refuge on our shores to spend its last days in comfort if not in splendour.
Opening from essay, 'Elementary Geometry', included in The Conflict of Studies and Other Essays (1873), 136.
It has been the final aim of Lie from the beginning to make progress in the theory of differential equations; as subsidiary to this may be regarded both his geometrical developments and the theory of continuous groups.
In Lectures on Mathematics (1911), 24.
It has often been said that, to make discoveries, one must be ignorant. This opinion, mistaken in itself, nevertheless conceals a truth. It means that it is better to know nothing than to keep in mind fixed ideas based on theories whose confirmation we constantly seek, neglecting meanwhile everything that fails to agree with them.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 37.
It is … a sign of the times—though our brothers of physics and chemistry may smile to hear me say so—that biology is now a science in which theories can be devised: theories which lead to predictions and predictions which sometimes turn out to be correct. These facts confirm me in a belief I hold most passionately—that biology is the heir of all the sciences.
From Nobel Banquet speech (10 Dec 1960).
It is a capital mistake to theorize before one has data. Insensibly one begins to twist facts to suit theories, instead of theories to suit facts.
A Scandal in Bohemia (1891) in Adventures of Sherlock Holmes (1892), 5.
It is a capital mistake to theorize before you have all the evidence. It biases the judgment.
A Study in Scarlet (1887), in Works of Arthur Conan Doyle (1902), Vol. 11, 39.
It is a constant struggle not to let the theory lead the science in the way that is most beneficial to one’s assumptions.
In U.S. News & World Report (1997), 123, 262.
It is a good thing Heaven has not given us the power to change as much of our body as we would like to or as our theory would assert is necessary.
Aphorism 34 in Notebook D (1773-1775), as translated by R.J. Hollingdale in Aphorisms (1990). Reprinted as The Waste Books (2000), 48.
It is a remarkable fact that the second law of thermodynamics has played in the history of science a fundamental role far beyond its original scope. Suffice it to mention Boltzmann’s work on kinetic theory, Planck’s discovery of quantum theory or Einstein’s theory of spontaneous emission, which were all based on the second law of thermodynamics.
From Nobel lecture, 'Time, Structure and Fluctuations', in Tore Frängsmyr and Sture Forsén (eds.), Nobel Lectures, Chemistry 1971-1980, (1993), 263.
It is a serious question whether America, following England’s lead, has not gone into problem-solving too extensively. Certain it is that we are producing no text-books in which the theory is presented in the delightful style which characterizes many of the French works … , or those of the recent Italian school, or, indeed, those of the continental writers in general.
In The Teaching of Elementary Mathematics (1902), 219.
It is a test of true theories not only to account for but to predict phenomena.
Aphorism 12, 'Aphorisms Concerning Science', The Philosophy of the Inductive Sciences (1840), Vol. 1, xxxvi.
It is a vulgar belief that our astronomical knowledge dates only from the recent century when it was rescued from the monks who imprisoned Galileo; but Hipparchus…who among other achievements discovered the precession of the eqinoxes, ranks with the Newtons and the Keplers; and Copernicus, the modern father of our celestial science, avows himself, in his famous work, as only the champion of Pythagoras, whose system he enforces and illustrates. Even the most modish schemes of the day on the origin of things, which captivate as much by their novelty as their truth, may find their precursors in ancient sages, and after a careful analysis of the blended elements of imagination and induction which charaterise the new theories, they will be found mainly to rest on the atom of Epicurus and the monad of Thales. Scientific, like spiritual truth, has ever from the beginning been descending from heaven to man.
Lothair (1879), preface, xvii.
It is also a good rule not to put overmuch confidence in the observational results that are put forward until they are confirmed by theory.
New Pathways in Science (1935), 211.
It is always better to have no ideas than false ones; to believe nothing than to believe what is wrong.
In Letter (19 Jul 1788) to James Madison. Collected in Thomas Jefferson Randolph, Memoirs, Correspondence, and Private Papers of Thomas Jefferson (1829), Vol. 2, 223.
It is always noteworthy that all those who seriously study this science [the theory of numbers] conceive a sort of passion for it.
Letter to Jonos Boyai (2 Sep 1808). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 413.
It is an old saying, abundantly justified, that where sciences meet there growth occurs. It is true moreover to say that in scientific borderlands not only are facts gathered that [are] often new in kind, but it is in these regions that wholly new concepts arise. It is my own faith that just as the older biology from its faithful studies of external forms provided a new concept in the doctrine of evolution, so the new biology is yet fated to furnish entirely new fundamental concepts of science, at which physics and chemistry when concerned with the non-living alone could never arrive.
'Biological Thought and Chemical Thought: A Plea for Unification', Linacre Lecture, Cambridge (6 May 1938), published in Lancet (1938),2, 1204.
It is as if Cleopatra fell off her barge in 40 BC and hasn't hit the water yet.
[Illustrating how strange the behaviour of kaon particles, when first found in cosmic rays, which lived without predicted decay for a surprisingly long time—seemingly postponed a million billion times longer than early theory expected.]
[Illustrating how strange the behaviour of kaon particles, when first found in cosmic rays, which lived without predicted decay for a surprisingly long time—seemingly postponed a million billion times longer than early theory expected.]
In Frank Close, Michael Marten, Christine Sutton, The Particle Odyssey: a Journey to the Heart of the Matter (2004),75.
It is believed that contraction of the Earth due to its emission of lava and volcanic gases provides a tentative theory for the building of mountains and continents which is capable of explaining more of the details of these features than any other theory yet proposed.
In Sigma XI National Lecture (1957-58), published in 'Geophysics and Continental Growth', American Scientist (Mar 1959), 47, No. 1, 23.
It is clear, then, that the idea of a fixed method, or of a fixed theory of rationality, rests on too naive a view of man and his social surroundings. To those who look at the rich material provided by history, and who are not intent on impoverishing it in order to please their lower instincts, their craving for intellectual security in the form of clarity, precision, “objectivity”, “truth”, it will become clear that there is only one principle that can be defended under all circumstances and in all stages of human development. It is the principle: anything goes.
Against Method: Outline of an Anarchistic Theory of Knowledge (1975, 1993), 18-19.
It is curious how often erroneous theories have had a beneficial effect for particular branches of science.
The Growth of Biological Thought: Diversity, Evolution and Inheritance (1982), 847.
It is customary to connect Medicine with Botany, yet scientific treatment demands that we should consider each separately. For the fact is that in every art, theory must be disconnected and separated from practice, and the two must be dealt with singly and individually in their proper order before they are united. And for that reason, in order that Botany, which is, as it were, a special branch of Natural Philosophy [Physica], may form a unit by itself before it can be brought into connection with other sciences, it must be divided and unyoked from Medicine.
Methodi herbariae libri tres (1592), translated in Agnes Arber, Herbals: Their Origin and Evolution, 2nd edition (1938), 144.
It is difficult even to attach a precise meaning to the term “scientific truth.” So different is the meaning of the word “truth” according to whether we are dealing with a fact of experience, a mathematical proposition or a scientific theory. “Religious truth” conveys nothing clear to me at all.
From 'Scientific Truth' in Essays in Science (1934, 2004), 11.
It is easy to make out three areas where scientists will be concentrating their efforts in the coming decades. One is in physics, where leading theorists are striving, with the help of experimentalists, to devise a single mathematical theory that embraces all the basic phenomena of matter and energy. The other two are in biology. Biologists—and the rest of us too—would like to know how the brain works and how a single cell, the fertilized egg cell, develops into an entire organism
Article 'The View From Mars', in Annals of the New York Academy of Sciences: Research Facilities of the Future (1994), 735, 37.
It is easy to obtain confirmations, or verifications, for nearly every theory—if we look for confirmations. Confirmations should count only if they are the result of risky predictions... A theory which is not refutable by any conceivable event is non-scientific. Irrefutability is not a virtue of a theory (as people often think) but a vice. Every genuine test of a theory is an attempt to falsify it, or refute it.
Conjectures and Refutations: The Growth of Scientific Knowledge (1963), 36.
It is going to be necessary that everything that happens in a finite volume of space and time would have to be analyzable with a finite number of logical operations. The present theory of physics is not that way, apparently. It allows space to go down into infinitesimal distances, wavelengths to get infinitely great, terms to be summed in infinite order, and so forth; and therefore, if this proposition [that physics is computer-simulatable] is right, physical law is wrong.
International Journal of Theoretical Physics (1982), 21 Nos. 6-7, 468. Quoted in Brian Rotman, Mathematics as Sign (2000), 82.
It is grindingly, creakingly, crashingly obvious that, if Darwinism were really a theory of chance, it couldn’t work. You don't need to be a mathematician or physicist to calculate that an eye or a haemoglobin molecule would take from here to infinity to self-assemble by sheer higgledy-piggledy luck. Far from being a difficulty peculiar to Darwinism, the astronomic improbability of eyes and knees, enzymes and elbow joints and all the other living wonders is precisely the problem that any theory of life must solve, and that Darwinism uniquely does solve. It solves it by breaking the improbability up into small, manageable parts, smearing out the luck needed, going round the back of Mount Improbable and crawling up the gentle slopes, inch by million-year inch. Only God would essay the mad task of leaping up the precipice in a single bound.
In Climbing Mount Improbable (1996), 67-8.
It is human to search for the theory of everything and it is superhuman to find it.
Quotations: Superultramodern Science and Philosophy (2005).
It is in scientific honesty that I endorse the presentation of alternative theories for the origin of the universe, life and man in the science classroom. It would be an error to overlook the possibility that the universe was planned rather than happening by chance.
In letter to California State board of Education (14 Sep 1972).
It is known that the mathematics prescribed for the high school [Gymnasien] is essentially Euclidean, while it is modern mathematics, the theory of functions and the infinitesimal calculus, which has secured for us an insight into the mechanism and laws of nature. Euclidean mathematics is indeed, a prerequisite for the theory of functions, but just as one, though he has learned the inflections of Latin nouns and verbs, will not thereby be enabled to read a Latin author much less to appreciate the beauties of a Horace, so Euclidean mathematics, that is the mathematics of the high school, is unable to unlock nature and her laws.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 37-38. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 112.
It is more important to have beauty in one's equations than to have them fit experiment... It seems that if one is working from the point of view of getting beauty in one's equations, and if one has really a sound insight, one is on a sure line of progress. If there is not complete agreement between the results of one's work and experiment, one should not allow oneself to be too discouraged, because the discrepancy may well be due to minor features that are not properly taken into account and that will get cleared up with further developments of the theory.
In 'The Evolution of the Physicist’s Picture of Nature', Scientific American, May 1963, 208, 47.
It is not surprising that our language should be incapable of describing the processes occurring within the atoms, for, as has been remarked, it was invented to describe the experiences of daily life, and these consists only of processes involving exceedingly large numbers of atoms. Furthermore, it is very difficult to modify our language so that it will be able to describe these atomic processes, for words can only describe things of which we can form mental pictures, and this ability, too, is a result of daily experience. Fortunately, mathematics is not subject to this limitation, and it has been possible to invent a mathematical scheme—the quantum theory—which seems entirely adequate for the treatment of atomic processes; for visualization, however, we must content ourselves with two incomplete analogies—the wave picture and the corpuscular picture.
The Physical Principles of the Quantum Theory, trans. Carl Eckart and Frank C. Hoyt (1949), 11.
It is not surprising, in view of the polydynamic constitution of the genuinely mathematical mind, that many of the major heros of the science, men like Desargues and Pascal, Descartes and Leibnitz, Newton, Gauss and Bolzano, Helmholtz and Clifford, Riemann and Salmon and Plücker and Poincaré, have attained to high distinction in other fields not only of science but of philosophy and letters too. And when we reflect that the very greatest mathematical achievements have been due, not alone to the peering, microscopic, histologic vision of men like Weierstrass, illuminating the hidden recesses, the minute and intimate structure of logical reality, but to the larger vision also of men like Klein who survey the kingdoms of geometry and analysis for the endless variety of things that flourish there, as the eye of Darwin ranged over the flora and fauna of the world, or as a commercial monarch contemplates its industry, or as a statesman beholds an empire; when we reflect not only that the Calculus of Probability is a creation of mathematics but that the master mathematician is constantly required to exercise judgment—judgment, that is, in matters not admitting of certainty—balancing probabilities not yet reduced nor even reducible perhaps to calculation; when we reflect that he is called upon to exercise a function analogous to that of the comparative anatomist like Cuvier, comparing theories and doctrines of every degree of similarity and dissimilarity of structure; when, finally, we reflect that he seldom deals with a single idea at a tune, but is for the most part engaged in wielding organized hosts of them, as a general wields at once the division of an army or as a great civil administrator directs from his central office diverse and scattered but related groups of interests and operations; then, I say, the current opinion that devotion to mathematics unfits the devotee for practical affairs should be known for false on a priori grounds. And one should be thus prepared to find that as a fact Gaspard Monge, creator of descriptive geometry, author of the classic Applications de l’analyse à la géométrie; Lazare Carnot, author of the celebrated works, Géométrie de position, and Réflections sur la Métaphysique du Calcul infinitesimal; Fourier, immortal creator of the Théorie analytique de la chaleur; Arago, rightful inheritor of Monge’s chair of geometry; Poncelet, creator of pure projective geometry; one should not be surprised, I say, to find that these and other mathematicians in a land sagacious enough to invoke their aid, rendered, alike in peace and in war, eminent public service.
In Lectures on Science, Philosophy and Art (1908), 32-33.
It is not that we propose a theory and Nature may shout NO; rather, we propose a maze of theories, and Nature may shout INCONSISTENT.
In 'Falsification and the Methodology of Scientific Research Programmes', in I. Lakatos and A. Musgrave (eds.), Criticism and the Growth of Knowledge: Proceedings of the International Colloquium in the Philosophy of Science, London 1965 (1970), Vol. 4, 130.
It is often held that scientific hypotheses are constructed, and are to be constructed, only after a detailed weighing of all possible evidence bearing on the matter, and that then and only then may one consider, and still only tentatively, any hypotheses. This traditional view however, is largely incorrect, for not only is it absurdly impossible of application, but it is contradicted by the history of the development of any scientific theory. What happens in practice is that by intuitive insight, or other inexplicable inspiration, the theorist decides that certain features seem to him more important than others and capable of explanation by certain hypotheses. Then basing his study on these hypotheses the attempt is made to deduce their consequences. The successful pioneer of theoretical science is he whose intuitions yield hypotheses on which satisfactory theories can be built, and conversely for the unsuccessful (as judged from a purely scientific standpoint).
Co-author with Raymond Arthur Lyttleton, in 'The Internal Constitution of the Stars', Occasional Notes of the Royal Astronomical Society 1948, 12, 90.
It is scientists, not sceptics, who are most willing to consider explanations that conflict with their own. And far from quashing dissent, it is the scientists, not the sceptics, who do most to acknowledge gaps in their studies and point out the limitations of their data—which is where sceptics get much of the mud they fling at the scientists. By contrast, the [sceptics] are not trying to build a theory of anything. They have set the bar much lower, and are happy muddying the waters.
Editorial, Nature (28 Jul 2011), 475, 423-424.
It is the theory that decides what can be observed.
…...
It is to them [fossils] alone that we owe the commencement of even a Theory of the Earth ... By them we are enabled to ascertain, with the utmost certainty, that our earth has not always been covered over by the same external crust, because we are thoroughly assured that the organized bodies to which these fossil remains belong must have lived upon the surface before they came to be buried, as they now are, at a great depth.
'Preliminary discourse', to Recherches sur les Ossemens Fossiles (1812), trans. R. Kerr Essay on the Theory of the Earth (1813), 54-55.
It is true that Fourier had the opinion that the principal end of mathematics was public utility and the explanation of natural phenomena; but a philosopher as he is should have known that the unique end of science is the honor of the human mind and that from this point of view a question of [the theory of] number is as important as a question of the system of the world.
From letter to Legendre, translation as given in F.R. Moulton, 'The Influence of Astronomy on Mathematics', Science (10 Mar 1911), N.S. Vol. 33, No. 845, 359. A different translation begins, “It is true that M. Fourier believed…” on the Karl Jacobi Quotes web page on this site.
It is well known that the man who first made public the theory of irrationals perished in a shipwreck in order that the inexpressible and unimaginable should ever remain veiled. And so the guilty man, who fortuitously touched on and revealed this aspect of living things, was taken to the place where he began and there is for ever beaten by the waves.
— Proclus
In scholium to Book X of Euclid t. V, 417 as quoted and cited in Ettore Carruccio and Isabel Quigly (trans.), Mathematics And Logic in History And in Contemporary Thought (1964), 27.
It is with theories as with wells: you may see to the bottom of the deepest if there be any water there, while another shall pass for wondrous profound when ‘tis merely shallow, dark, and empty.
As quoted, citing only the author, in 'New Publications: Dr. Wigan', The Edinburgh Review: Or Critical Journal (Oct 1844), 80, 67.
It must be conceded that a theory has an important advantage if its basic concepts and fundamental hypotheses are 'close to experience,' and greater confidence in such a theory is certainly justified. There is less danger of going completely astray, particularly since it takes so much less time and effort to disprove such theories by experience. Yet more and more, as the depth of our knowledge increases, we must give up this advantage in our quest for logical simplicity in the foundations of physical theory...
'On the Generalized Theory of Gravitation', Scientific American (Apr 1950), 13. In David H. Levy (Ed.), The Scientific American Book of the Cosmos (2000), 19.
It must be granted that in every syllogism, considered as an argument to prove the conclusion, there is a petitio principii. When we say, All men are mortal Socrates is a man therefore Socrates is mortal; it is unanswerably urged by the adversaries of the syllogistic theory, that the proposition, Socrates is mortal.
A System of Logic, Ratiocinative and Inductive (1858), 122.
It reveals to me the causes of many natural phenomena that are entirely incomprehensible in the light of the generally accepted hypotheses. To refute the latter I collected many proofs, but I do not publish them ... I would dare to publish my speculations if there were people men like you.
[Declaring his belief in the heliocentric theory of Copernicus.]
[Declaring his belief in the heliocentric theory of Copernicus.]
Letter to Kepler (1596). Quoted in Will Durant, Ariel Duran, The Age of Reason Begins (1961), 603. From Hermann Kesten, Copernicus and His World, translated by E.B. Ashton (pseud.) and Norbert Guterman (1945), 348-349.
It seems sensible to discard all hope of observing hitherto unobservable quantities, such as the position and period of the electron... Instead it seems more reasonable to try to establish a theoretical quantum mechanics, analogous to classical mechanics, but in which only relations between observable quantities occur.
In Helge Kragh, Quantum Generations: A History of Physics in the Twentieth Century (1999), 161.
It seems that the rivers know the theory. It only remains to convince the engineers of the validity of this analysis.
Epigraph, without citation, in Péter Érdi, Complexity Explained (2007), 339. In French, “Il semble que les rivières connaissent déjà la théorie Il ne reste qu'à convaincre les ingénieurs de la validité de cette analyse.”
It seems to me that your Reverence and Signor Galileo act prudently when you content yourselves with speaking hypothetically and not absolutely, as I have always understood that Copernicus spoke. To say that on the supposition of the Earth’s movement and the Sun's quiescence all the celestial appearances are explained better than by the theory of eccentrics and epicycles is to speak with excellent good sense and to run no risk whatsoever. Such a manner of speaking is enough for a mathematician. But to want to affirm that the Sun, in very truth, is at the center of the universe and only rotates on its axis without going from east to west, is a very dangerous attitude and one calculated not only to arouse all Scholastic philosophers and theologians but also to injure our holy faith by contradicting the Scriptures.
Letter to Paolo Antonio Foscarini, 12 April 1615. Quoted in Giorgio De Santillana, The Crime of Galileo (1955), 99.
It seems to me, he says, that the test of “Do we or not understand a particular subject in physics?” is, “Can we make a mechanical model of it?” I have an immense admiration for Maxwell’s model of electromagnetic induction. He makes a model that does all the wonderful things that electricity docs in inducing currents, etc., and there can be no doubt that a mechanical model of that kind is immensely instructive and is a step towards a definite mechanical theory of electromagnetism.
From stenographic report by A.S. Hathaway of the Lecture 20 Kelvin presented at Johns Hopkins University, Baltimore, on 'Molecular Dynamics and the Wave Theory of Light' (1884), 132. (Hathaway was a Mathematics fellow there.) This remark is not included in the first typeset publication—a revised version, printed twenty years later, in 1904, as Lord Kelvin’s Baltimore Lectures on Molecular Dynamics and the Wave Theory of Light. The original notes were reproduced by the “papyrograph” process. They are excerpted in Pierre Maurice Marie Duhem, Essays in the History and Philosophy of Science (1996), 54-55.
It surely can be no offence to state, that the progress of science has led to new views, and that the consequences that can be deduced from the knowledge of a hundred facts may be very different from those deducible from five. It is also possible that the facts first known may be the exceptions to a rule and not the rule itself, and generalisations from these first-known facts, though useful at the time, may be highly mischievous, and impede the progress of the science if retained when it has made some advance.
Sections and Views Illustrative of Geological Phenomena (1830), viii.
It was his [Leibnitz’s] love of method and order, and the conviction that such order and harmony existed in the real world, and that our success in understanding it depended upon the degree and order which we could attain in our own thoughts, that originally was probably nothing more than a habit which by degrees grew into a formal rule. This habit was acquired by early occupation with legal and mathematical questions. We have seen how the theory of combinations and arrangements of elements had a special interest for him. We also saw how mathematical calculations served him as a type and model of clear and orderly reasoning, and how he tried to introduce method and system into logical discussions, by reducing to a small number of terms the multitude of compound notions he had to deal with. This tendency increased in strength, and even in those early years he elaborated the idea of a general arithmetic, with a universal language of symbols, or a characteristic which would be applicable to all reasoning processes, and reduce philosophical investigations to that simplicity and certainty which the use of algebraic symbols had introduced into mathematics.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
In Leibnitz (1884), 44-45. [The first sentence is reworded to better introduce the quotation. —Webmaster]
It would seem at first sight as if the rapid expansion of the region of mathematics must be a source of danger to its future progress. Not only does the area widen but the subjects of study increase rapidly in number, and the work of the mathematician tends to become more and more specialized. It is, of course, merely a brilliant exaggeration to say that no mathematician is able to understand the work of any other mathematician, but it is certainly true that it is daily becoming more and more difficult for a mathematician to keep himself acquainted, even in a general way, with the progress of any of the branches of mathematics except those which form the field of his own labours. I believe, however, that the increasing extent of the territory of mathematics will always be counteracted by increased facilities in the means of communication. Additional knowledge opens to us new principles and methods which may conduct us with the greatest ease to results which previously were most difficult of access; and improvements in notation may exercise the most powerful effects both in the simplification and accessibility of a subject. It rests with the worker in mathematics not only to explore new truths, but to devise the language by which they may be discovered and expressed; and the genius of a great mathematician displays itself no less in the notation he invents for deciphering his subject than in the results attained. … I have great faith in the power of well-chosen notation to simplify complicated theories and to bring remote ones near and I think it is safe to predict that the increased knowledge of principles and the resulting improvements in the symbolic language of mathematics will always enable us to grapple satisfactorily with the difficulties arising from the mere extent of the subject.
In Presidential Address British Association for the Advancement of Science, Section A., (1890), Nature, 42, 466.
It would take a civilization far more advanced than ours, unbelievably advanced, to begin to manipulate negative energy to create gateways to the past. But if you could obtain large quantities of negative energy—and that's a big “IF”—then you could create a time machine that apparently obeys Einstein's equation and perhaps the laws of quantum theory.
Quoted by J.R. Minkel in 'Borrowed Time: Interview with Michio Kaku', Scientific American (23 Nov 2003).
Just now nuclear physicists are writing a great deal about hypothetical particles called neutrinos supposed to account for certain peculiar facts observed in β-ray disintegration. We can perhaps best describe the neutrinos as little bits of spin-energy that have got detached. I am not much impressed by the neutrino theory. In an ordinary way I might say that I do not believe in neutrinos… But I have to reflect that a physicist may be an artist, and you never know where you are with artists. My old-fashioned kind of disbelief in neutrinos is scarcely enough. Dare I say that experimental physicists will not have sufficient ingenuity to make neutrinos? Whatever I may think, I am not going to be lured into a wager against the skill of experimenters under the impression that it is a wager against the truth of a theory. If they succeed in making neutrinos, perhaps even in developing industrial applications of them, I suppose I shall have to believe—though I may feel that they have not been playing quite fair.
From Tarner Lecture, 'Discovery or Manufacture?' (1938), in The Philosophy of Physical Science (1939, 2012), 112.
Langmuir is a regular thinking machine. Put in facts, and you get out a theory.
Quoted in C. Guy Suits (ed.), The Collected Works of Irving Langmuir (1962), Vol. 12, 6.
Later scientific theories are better than earlier ones for solving puzzles in the often quite different environments to which they are applied. That is not a relativist's position, and it displays the sense in which I am a convinced believer in scientific progress.
The Structure of Scientific Revolutions, 2nd edition (1970), 206.
Let him [the author] be permitted also in all humility to add … that in consequence of the large arrears of algebraical and arithmetical speculations waiting in his mind their turn to be called into outward existence, he is driven to the alternative of leaving the fruits of his meditations to perish (as has been the fate of too many foregone theories, the still-born progeny of his brain, now forever resolved back again into the primordial matter of thought), or venturing to produce from time to time such imperfect sketches as the present, calculated to evoke the mental co-operation of his readers, in whom the algebraical instinct has been to some extent developed, rather than to satisfy the strict demands of rigorously systematic exposition.
In Philosophic Magazine (1863), 460.
Let me describe briefly how a black hole might be created. Imagine a star with a mass 10 times that of the sun. During most of its lifetime of about a billion years the star will generate heat at its center by converting hydrogen into helium. The energy released will create sufficient pressure to support the star against its own gravity, giving rise to an object with a radius about five times the radius of the sun. The escape velocity from the surface of such a star would be about 1,000 kilometers per second. That is to say, an object fired vertically upward from the surface of the star with a velocity of less than 1,000 kilometers per second would be dragged back by the gravitational field of the star and would return to the surface, whereas an object with a velocity greater than that would escape to infinity.
When the star had exhausted its nuclear fuel, there would be nothing to maintain the outward pressure, and the star would begin to collapse because of its own gravity. As the star shrank, the gravitational field at the surface would become stronger and the escape velocity would increase. By the time the radius had got down to 10 kilometers the escape velocity would have increased to 100,000 kilometers per second, the velocity of light. After that time any light emitted from the star would not be able to escape to infinity but would be dragged back by the gravitational field. According to the special theory of relativity nothing can travel faster than light, so that if light cannot escape, nothing else can either. The result would be a black hole: a region of space-time from which it is not possible to escape to infinity.
When the star had exhausted its nuclear fuel, there would be nothing to maintain the outward pressure, and the star would begin to collapse because of its own gravity. As the star shrank, the gravitational field at the surface would become stronger and the escape velocity would increase. By the time the radius had got down to 10 kilometers the escape velocity would have increased to 100,000 kilometers per second, the velocity of light. After that time any light emitted from the star would not be able to escape to infinity but would be dragged back by the gravitational field. According to the special theory of relativity nothing can travel faster than light, so that if light cannot escape, nothing else can either. The result would be a black hole: a region of space-time from which it is not possible to escape to infinity.
'The Quantum Mechanics of Black Holes', Scientific American, 1977, 236, 34-40.
Let us award a just, a brilliant homage to those rare men whom nature has endowed with the precious privilege of arranging a thousand isolated facts, of making seductive theories spring from them; but let us not forget to state, that the scythe of the reaper had cut the stalks before one had thought of uniting them into sheaves!
In François Arago, trans. by William Henry Smyth, Baden Powell and Robert Grant, 'Fourier', Biographies of Distinguished Scientific Men (1859), Vol. 1, 409.
Let us now discuss the extent of the mathematical quality in Nature. According to the mechanistic scheme of physics or to its relativistic modification, one needs for the complete description of the universe not merely a complete system of equations of motion, but also a complete set of initial conditions, and it is only to the former of these that mathematical theories apply. The latter are considered to be not amenable to theoretical treatment and to be determinable only from observation.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 125.
Let us now recapitulate all that has been said, and let us conclude that by hermetically sealing the vials, one is not always sure to prevent the birth of the animals in the infusions, boiled or done at room temperature, if the air inside has not felt the ravages of fire. If, on the contrary, this air has been powerfully heated, it will never allow the animals to be born, unless new air penetrates from outside into the vials. This means that it is indispensable for the production of the animals that they be provided with air which has not felt the action of fire. And as it would not be easy to prove that there were no tiny eggs disseminated and floating in the volume of air that the vials contain, it seems to me that suspicion regarding these eggs continues, and that trial by fire has not entirely done away with fears of their existence in the infusions. The partisans of the theory of ovaries will always have these fears and will not easily suffer anyone's undertaking to demolish them.
Nouvelles Recherches sur les Découvertes Microscopiques, et la Génération des Corps Organisés (1769), 134-5. Quoted in Jacques Roger, The Life Sciences in Eighteenth-Century French Thought, ed. Keith R. Benson and trans. Robert Ellrich (1997), 510-1.
Let us sum up the three possible explanations of the decision to drop the bomb and its timing. The first that it was a clever and highly successful move in the field of power politics, is almost certainly correct; the second, that the timing was coincidental, convicts the American government of a hardly credible tactlessness [towards the Soviet Union]; and the third, the Roman
holiday theory [a spectacular event to justify the cost of the Manhattan Project], convicts them of an equally incredible irresponsibility.
In The Political and Military Consequences of Atomic Energy (1948), 126. As cited by Maurice W. Kirby and Jonathan Rosenhead, 'Patrick Blackett (1897)' in Arjang A. Assad (ed.) and Saul I. Gass (ed.),Profiles in Operations Research: Pioneers and Innovators (2011), 17. Blackett regarded the dropping of the atomic bombs on Japan as unnecessary because a Japanese surrender was inevitable.
Like other theorists, I work with just pencil and paper, trying to make simple explanations of complicated phenomena. We leave it to the experimental physicists to decide whether our theories actually describe the real world.
In Facing Up: Science and Its Cultural Adversaries (2001), 185
Looking back … over the long and labyrinthine path which finally led to the discovery [of the quantum theory], I am vividly reminded of Goethe’s saying that men will always be making mistakes as long as they are striving after something.
From Nobel Prize acceptance speech (2 Jun 1920), as quoted and translated by James Murphy in 'Introduction: Max Planck: a Biographical Sketch', in Max Planck and James Murphy (trans.), Where is Science Going (1932), 23. This passage of Planck’s speech is translated very differently for the Nobel Committee. See elsewhere on this web page, beginning, “When I look back…”.
Lord Kelvin had, in a manner hardly and perhaps never equalled before, except by Archimedes, the power of theorizing on the darkest, most obscure, and most intimate secrets of Nature, and at the same time, and almost in the same breath, carrying out effectively and practically some engineering feat, or carrying to a successful issue some engineering invention. He was one of the leaders in the movement which has compelled all modern engineers worthy of the name to be themselves men not merely of practice, but of theory, to carry out engineering undertakings in the spirit of true scientific inquiry and with an eye fixed on the rapidly growing knowledge of the mechanics of Nature, which can only be acquired by the patient work of physicists and mathematicians in their laboratories and studies.
In Speech (May 1921) to the Institute of Civil Engineers, to award the newly created Kelvin Medal. As quoted in Sarah Knowles Bolton, 'Lord Kelvin', Famous Men of Science (1889, Revised Ed. 1926), 316-317.
̈Machines which do not receive their motion from heat, those which have for a motor the force of men or of animal, a waterfall, an air current, etc., can be studied even to their smallest details by the mechanical theory. All cases are foreseen, all imaginable movements are referred to these general principles, firmly established, and applicable under all circumstances. This is the character of a complete theory. A similar theory is evidently needed for heat-engines. A similar theory is evidently needed for heat-engines. We shall have it only when the laws of Physics shall be extended enough, generalized enough, to make known beforehand all of the effects of heat acting in a determined manner on any body.
As translated in Reflections on the Motive Power of Heat and on Machines Fitted to Develop Power (1890, Rev. 1897), 44. Edited by R. H. Thurston, from the original French, Réflexions sur la Puissance Motrice du Feu (1824).
Man has never been a particularly modest or self-deprecatory animal, and physical theory bears witness to this no less than many other important activities. The idea that thought is the measure of all things, that there is such a thing as utter logical rigor, that conclusions can be drawn endowed with an inescapable necessity, that mathematics has an absolute validity and controls experience—these are not the ideas of a modest animal. Not only do our theories betray these somewhat bumptious traits of self-appreciation, but especially obvious through them all is the thread of incorrigible optimism so characteristic of human beings.
In The Nature of Physical Theory (1936), 135-136.
Marxism: The theory that all the important things in history are rooted in an economic motive, that history is a science, a science of the search for food.
From Daily News (31 Jul 1909). In Dale Ahlquist (ed.) The Universe According to G.K. Chesterton: A Dictionary of the Mad, Mundane and Metaphysical (2013), 71.
Mathematical theories have sometimes been used to predict phenomena that were not confirmed until years later. For example, Maxwell’s equations, named after physicist James Clerk Maxwell, predicted radio waves. Einstein’s field equations suggested that gravity would bend light and that the universe is expanding. Physicist Paul Dirac once noted that the abstract mathematics we study now gives us a glimpse of physics in the future. In fact, his equations predicted the existence of antimatter, which was subsequently discovered. Similarly, mathematician Nikolai Lobachevsky said that “there is no branch of mathematics, however abstract, which may not someday be applied to the phenomena of the real world.”
In 'Introduction', The Math Book: From Pythagoras to the 57th Dimension, 250 Milestones in the History of Mathematics (2009), 12.
Mathematicians … believed that prediction was just a function of keeping track of things. If you knew enough, you could predict anything. … Chaos theory throws it right out the window because …
in fact there are great categories of phenomena that are inherently unpredictable.
In novel, Jurassic Park (1990, 1991), 158.
Mathematicians deal with possible worlds, with an infinite number of logically consistent systems. Observers explore the one particular world we inhabit. Between the two stands the theorist. He studies possible worlds but only those which are compatible with the information furnished by observers. In other words, theory attempts to segregate the minimum number of possible worlds which must include the actual world we inhabit. Then the observer, with new factual information, attempts to reduce the list further. And so it goes, observation and theory advancing together toward the common goal of science, knowledge of the structure and observation of the universe.
Lecture to Sigma Xi, 'The Problem of the Expanding Universe' (1941), printed in Sigma Xi Quarterly (1942), 30, 104-105. Reprinted in Smithsonian Institution Report of the Board of Regents (1943), 97, 123. As cited by Norriss S. Hetherington in 'Philosophical Values and Observation in Edwin Hubble's Choice of a Model of the Universe', Historical Studies in the Physical Sciences (1982), 13, No. 1, 63.
Mathematics is not the discoverer of laws, for it is not induction; neither is it the framer of theories, for it is not hypothesis; but it is the judge over both, and it is the arbiter to which each must refer its claims; and neither law can rule nor theory explain without the sanction of mathematics.
In 'Linear Associative Algebra', American Journal of Mathematics (1881), 4, 97.
Mathematics is the queen of the sciences and arithmetic [number theory] is the queen of mathematics. She often condescends to render service to astronomy and other natural sciences, but in all relations, she is entitled to first rank.
I>Sartorius von
Waltershausen: Gauss zum Gedächtniss (1856), 79. Quoted in Robert Edouard Moritz, Memorabilia
Mathematica (1914), 271.
Maxwell's theory is Maxwell's system of equations.
Electric Waves (1893), 21.
Men who believe too firmly in their theories, do not believe enough in the theories of others. So … these despisers of their fellows … make experiments only to destroy a theory, instead of to seek the truth.
From An Introduction to the Study of Experimental Medicine (1927, 1957), as translated by Henry Copley Greene (1957), 38. From the original French by Claude Bernard: “Ceux qui croient trop à leurs théories ne croient pas assez à celles des autres. Alors … ces contempteurs d'autrui … font des expériences que pour détruire une théorie, au lieu de les faire pour chercher la vérité.” (1865), 68. A Google translation gives: “Those who believe too much in their theories do not believe enough in those of others. So … these despisers of others … do experiments only to destroy a theory, instead of doing them to seek the truth.”
Men who have excessive faith in their theories … make poor observations, because they choose among the results of their experiments only what suits their object, neglecting whatever is unrelated to it and carefully setting aside everything which might tend toward the idea they wish to combat.
From An Introduction to the Study of Experimental Medicine (1927, 1957), as translated by Henry Copley Greene (1957), 38. Note: the ellipsis condenses the quote from two paragraphs, beginning with the same clause as another quote on this web page, beginning, “Men who have excessive faith….” From the original French by Claude Bernard: “Les hommes qui ont une foi excessive dans leurs théories … font de mauvaises observations parce qu'ils ne prennent dans les résultats de leurs expériences que ce qui convient à leur but en négligeant ce qui ne s'y rapporte pas, et en écartant bien soigneusement tout ce qui pourrait aller dans le sens de l'idée qu'ils veulent combattre.” (1865), 67-68. A Google translation gives: “Men who have excessive faith in their theories … make bad observations because they only take from the results of their experiments what suits their purpose, neglecting what does not relate to it, and carefully discarding everything that could go in the direction of the idea they want to fight.”
Men who have excessive faith in their theories or ideas are not only ill prepared for making discoveries; they also make very poor observations. Of necessity, they observe with a preconceived idea, and when they devise an experiment, they can see, in its results,only a confirmation of their theory. In this way they distort observation and often neglect very important facts because they do not further their aim.
From An Introduction to the Study of Experimental Medicine (1927, 1957), as translated by Henry Copley Greene (1957), 38. From the original French by Claude Bernard: “Les hommes qui ont une foi excessive dans leurs théories ou dans leurs idées sont non-seulement mal disposés pour faire des découvertes, mais ils font aussi de très-mauvaises observations. Ils observent nécessairement avec une idée préconçue, et quand ils ont institué une expérience, ils ne veulent voir dans ses résultats qu'une confirmation de leur théorie. Ils défigurent ainsi l'observation et négligent souvent des faits très-importants, parce qu’ils ne concourent pas à leur but.” (1865), 68. A Google translation gives: “Men who have excessive faith in their theories or in their ideas are not only ill disposed to make discoveries, but they also make very bad observations. They necessarily observe with a preconceived idea, and when they have instituted an experiment, they only want to see in its results a confirmation of their theory. They thus disfigure observation and often neglect very important facts, because they do not contribute to their end.”
Model-making, the imaginative and logical steps which precede the experiment, may be judged the most valuable part of scientific method because skill and insight in these matters are rare. Without them we do not know what experiment to do. But it is the experiment which provides the raw material for scientific theory. Scientific theory cannot be built directly from the conclusions of conceptual models.
Introduction to the Study of Animal Population (1961), 181.
Modern Science has along with the theory that the Earth dated its beginning with the advent of man, swept utterly away this beautiful imagining. We can, indeed, find no beginning of the world. We trace back events and come to barriers which close our vistabarriers which, for all we know, may for ever close it. They stand like the gates of ivory and of horn; portals from which only dreams proceed; and Science cannot as yet say of this or that dream if it proceeds from the gate of horn or from that of ivory.
In short, of the Earth's origin we have no certain knowledge; nor can we assign any date to it. Possibly its formation was an event so gradual that the beginning was spread over immense periods. We can only trace the history back to certain events which may with considerable certainty be regarded as ushering in our geological era.
In short, of the Earth's origin we have no certain knowledge; nor can we assign any date to it. Possibly its formation was an event so gradual that the beginning was spread over immense periods. We can only trace the history back to certain events which may with considerable certainty be regarded as ushering in our geological era.
Lecture at the Royal Dublin Society, 6 Feb 1914. Published in Science Progress, Vol. 9, 37. Republished in The Birth-Time of the World and Other Scientific Essays, (1915), 2.
Modern theories did not arise from revolutionary ideas which have been, so to speak, introduced into the exact sciences from without. On the contrary they have forced their way into research which was attempting consistently to carry out the programme of classical physics—they arise out of its very nature. It is for this reason that the beginnings of modern physics cannot be compared with the great upheavals of previous periods like the achievements of Copernicus. Copernicus’s idea was much more an import from outside into the concepts of the science of his time, and therefore caused far more telling changes in science than the ideas of modern physics are creating to-day.
In Philosophical Problems of Nuclear Science: Eight Lectures (1952), 13.
MOLECULE, n. The ultimate, indivisible unit of matter. It is distinguished from the corpuscle, also the ultimate, indivisible unit of matter, by a closer resemblance to the atom, also the ultimate, indivisible unit of matter. Three great scientific theories of the structure of the universe are the molecular, the corpuscular and the atomic. A fourth affirms, with Haeckel, the condensation or precipitation of matter from ether—whose existence is proved by the condensation or precipitation. The present trend of scientific thought is toward the theory of ions. The ion differs from the molecule, the corpuscle and the atom in that it is an ion. A fifth theory is held by idiots, but it is doubtful if they know any more about the matter than the others.
The Collected Works of Ambrose Bierce (1911), Vol. 7, The Devil's Dictionary, 220-221.
More about the selection theory: Jerne meant that the Socratic idea of learning was a fitting analogy for 'the logical basis of the selective theories of antibody formation': Can the truth (the capability to synthesize an antibody) be learned? If so, it must be assumed not to pre-exist; to be learned, it must be acquired. We are thus confronted with the difficulty to which Socrates calls attention in Meno [ ... ] namely, that it makes as little sense to search for what one does not know as to search for what one knows; what one knows, one cannot search for, since one knows it already, and what one does not know, one cannot search for, since one does not even know what to search for. Socrates resolves this difficulty by postulating that learning is nothing but recollection. The truth (the capability to synthesize an antibody) cannot be brought in, but was already inherent.
'The Natural Selection Theory', in John Cairns, Gunther S. Stent, and James D. Watson (eds.) Phage and the Origins of Molecular Biology (1966), 301.
Morphological information has provided the greatest single source of data in the formulation and development of the theory of evolution and that even now, when the preponderance of work is experimental, the basis for interpretation in many areas of study remains the form and relationships of structures.
'Morphology, Paleontology, and Evolution', in Sol Tax (ed.), Evolution After Darwin, Vol. 1, The Evolution of Life (1960), 524.
Most of the fundamental ideas of science are essentially simple, and may, as a rule, be expressed in a language comprehensible to everyone.
Co-authored with Leopold Infeld.
Co-authored with Leopold Infeld.
The Evolution of Physics: The Growth of Ideas from the Early Concepts to Relativity and Quanta (1938), 29. Infeld was a Polish physicist (1898-1968).
Most people like to believe something is or is not true. Great scientists tolerate ambiguity very well. They believe the theory enough to go ahead; they doubt it enough to notice the errors and faults so they can step forward and create the new replacement theory. If you believe too much you’ll never notice the flaws; if you doubt too much you won’t get started. It requires a lovely balance.
'You and Your Research', Bell Communications Research Colloquium Seminar, 7 Mar 1986.
Most, if not all, of the great ideas of modern mathematics have had their origin in observation. Take, for instance, the arithmetical theory of forms, of which the foundation was laid in the diophantine theorems of Fermat, left without proof by their author, which resisted all efforts of the myriad-minded Euler to reduce to demonstration, and only yielded up their cause of being when turned over in the blow-pipe flame of Gauss’s transcendent genius; or the doctrine of double periodicity, which resulted from the observation of Jacobi of a purely analytical fact of transformation; or Legendre’s law of reciprocity; or Sturm’s theorem about the roots of equations, which, as he informed me with his own lips, stared him in the face in the midst of some mechanical investigations connected (if my memory serves me right) with the motion of compound pendulums; or Huyghen’s method of continued fractions, characterized by Lagrange as one of the principal discoveries of that great mathematician, and to which he appears to have been led by the construction of his Planetary Automaton; or the new algebra, speaking of which one of my predecessors (Mr. Spottiswoode) has said, not without just reason and authority, from this chair, “that it reaches out and indissolubly connects itself each year with fresh branches of mathematics, that the theory of equations has become almost new through it, algebraic geometry transfigured in its light, that the calculus of variations, molecular physics, and mechanics” (he might, if speaking at the present moment, go on to add the theory of elasticity and the development of the integral calculus) “have all felt its influence”.
In 'A Plea for the Mathematician', Nature, 1, 238 in Collected Mathematical Papers, Vol. 2, 655-56.
Mr Hall's hypothesis has its cause for subsidence, but none for the lifting of the thickened sunken crust into mountains. It is a theory for the origin of mountains, with the origin of mountains left out.
In 'Observations on the Origin of Some of the Earth’s Features', The American Journal of Science (Sep 1866), Second Series, 42, No. 125, 210.
Mr Hooke sent, in his next letter [to Sir Isaac Newton] the whole of his Hypothesis, scil that the gravitation was reciprocall to the square of the distance: ... This is the greatest Discovery in Nature that ever was since the World's Creation. It was never so much as hinted by any man before. I wish he had writt plainer, and afforded a little more paper.
Brief Lives (1680), edited by Oliver Lawson Dick (1949), 166-7.
Mr Justus Liebig is no doubt a very clever gentleman and a most profound chemist, but in our opinion he knows as much of agriculture as the horse that ploughs the ground, and there is not an old man that stands between the stilts of a plough in Virginia, that cannot tell him of facts totally at variance with his finest spun theories.
— Magazine
The Southern Planter (1845), 3, 23.
Much as I admired the elegance of physical theories, which at that time geology wholly lacked, I preferred a life in the woods to one in the laboratory.
From J. Tuzo Wilson, 'Early Days in University Geophysics', Ann. Rev. Earth Planet Sci. (1982), 10, 4.
Much like engineering. Design by theory, then beef it up anyhow.
In Glory Road (1963, 1981), 156.
Much of the geographical work of the past hundred years... has either explicitly or implicitly taken its inspiration from biology, and in particular Darwin. Many of the original Darwinians, such as Hooker, Wallace, Huxley, Bates, and Darwin himself, were actively concerned with geographical exploration, and it was largely facts of geographical distribution in a spatial setting which provided Darwin with the germ of his theory.
'Darwin's Impact on Geography', Annals of the Association of American Geographers (1966), 56, 683.
My colleagues in elementary particle theory in many lands [and I] are driven by the usual insatiable curiosity of the scientist, and our work is a delightful game. I am frequently astonished that it so often results in correct predictions of experimental results. How can it be that writing down a few simple and elegant formulae, like short poems governed by strict rules such as those of the sonnet or the waka, can predict universal regularities of Nature?
Nobel Banquet Speech (10 Dec 1969), in Wilhelm Odelberg (ed.),Les Prix Nobel en 1969 (1970).
My courses in physics and chemistry showed me that science could and indeed should have precise theories, but at that time geology lacked them and all right-minded geologists scoffed at the search for any. They said that this was armchair geology and that more maps were both the aim and the method of geology. So sterile a concept baffled me, but I was too stupid to accept, until I was fifty, the explanation which Frank Taylor and Alfred Wegener had advanced in the year I was born.
In 'Early Days in University Geophysics', Annual Review of Earth and Planetary Sciences (1982), 10, 6.
My decision to begin research in radio astronomy was influenced both by my wartime experience with electronics and antennas and by one of my teachers, Jack Ratcliffe, who had given an excellent course on electromagnetic theory during my final undergraduate year.
From Autobiography in Wilhelm Odelberg (ed.), Les Prix Nobel en 1974/Nobel Lectures (1975)
My Lord said that he who knew men only in this way [from history] was like one who had got the theory of anatomy perfectly, but who in practice would find himself very awkward and liable to mistakes. That he again who knew men by observation was like one who picked up anatomy by practice, but who like all empirics would for a long time be liable to gross errors.
My own thinking (and that of many of my colleagues) is based on two general principles, which I shall call the Sequence Hypothesis and the Central Dogma. The direct evidence for both of them is negligible, but I have found them to be of great help in getting to grips with these very complex problems. I present them here in the hope that others can make similar use of them. Their speculative nature is emphasized by their names. It is an instructive exercise to attempt to build a useful theory without using them. One generally ends in the wilderness.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
'On Protein Synthesis', Symposia of the Society for Experimental Biology: The Biological Replication of Macromolecules, 1958, 12, 152-3.
My theory of electrical forces is that they are called into play in insulating media by slight electric displacements, which put certain small portions of the medium into a state of distortion which, being resisted by the elasticity of the medium, produces an electromotive force ... I suppose the elasticity of the sphere to react on the electrical matter surrounding it, and press it downwards.
From the determination by Kohlrausch and Weber of the numerical relation between the statical and magnetic effects of electricity, I have determined the elasticity of the medium in air, and assuming that it is the same with the luminiferous ether I have determined the velocity of propagation of transverse vibrations.
The result is
193088 miles per second
(deduced from electrical & magnetic experiments).
Fizeau has determined the velocity of light
= 193118 miles per second
by direct experiment.
This coincidence is not merely numerical. I worked out the formulae in the country, before seeing Webers [sic] number, which is in millimetres, and I think we have now strong reason to believe, whether my theory is a fact or not, that the luminiferous and the electromagnetic medium are one.
From the determination by Kohlrausch and Weber of the numerical relation between the statical and magnetic effects of electricity, I have determined the elasticity of the medium in air, and assuming that it is the same with the luminiferous ether I have determined the velocity of propagation of transverse vibrations.
The result is
193088 miles per second
(deduced from electrical & magnetic experiments).
Fizeau has determined the velocity of light
= 193118 miles per second
by direct experiment.
This coincidence is not merely numerical. I worked out the formulae in the country, before seeing Webers [sic] number, which is in millimetres, and I think we have now strong reason to believe, whether my theory is a fact or not, that the luminiferous and the electromagnetic medium are one.
Letter to Michael Faraday (19 Oct 1861). In P. M. Harman (ed.), The Scientific Letters and Papers of James Clerk Maxwell (1990), Vol. 1, 1846-1862, 684-6.
My theory of evolution is that Darwin was adopted.
In The Fourth—and by Far the Most Recent—637 Best Things Anybody Ever Said (1990).
My view, the skeptical one, holds that we may be as far away from an understanding of elementary particles as Newton's successors were from quantum mechanics. Like them, we have two tremendous tasks ahead of us. One is to study and explore the mathematics of the existing theories. The existing quantum field-theories may or may not be correct, but they certainly conceal mathematical depths which will take the genius of an Euler or a Hamilton to plumb. Our second task is to press on with the exploration of the wide range of physical phenomena of which the existing theories take no account. This means pressing on with experiments in the fashionable area of particle physics. Outstanding among the areas of physics which have been left out of recent theories of elementary particles are gravitation and cosmology
In Scientific American (Sep 1958). As cited in '50, 100 & 150 years ago', Scientific American (Sep 2008), 299, No. 3, 14.
Natural selection is a theory of local adaptation to changing environments. It proposes no perfecting principles, no guarantee of general improvement,
Ever Since Darwin: Reflections in Natural History (1977), 45.
Nature is disordered, powerful and chaotic, and through fear of the chaos we impose system on it. We abhor complexity, and seek to simplify things whenever we can by whatever means we have at hand. We need to have an overall explanation of what the universe is and how it functions. In order to achieve this overall view we develop explanatory theories which will give structure to natural phenomena: we classify nature into a coherent system which appears to do what we say it does.
In Day the Universe Changed (1985), 11.
Nazis started the Science of Eugenics. It’s the theory that to them, justified the holocaust. The problem is the Science has been broadly accepted around the world, including the United States. We even went as far as to hire the Scientists that were working on it and brought them over here rather then charging them with war crimes. [Project Paperclip] I think it is a very dangerous Science that contains ideologies that are a grave danger to the entire world.
…...
Nervous messages are invariably associated with an electrical change known as the action potential. This potential is generally believed to arise at a membrane which is situated between the axoplasm and the external medium. If this theory is correct, it should be possible to record the action potential between an electrode inside a nerve fibre and the conducting fluid outside it. Most nerve fibres are too small for this to be tested directly, but we have recently succeeded in inserting micro-electrodes into the giant axons of squids (Loligo forbesi).
Co-author with Andrew Aelding Huxley, British physiologist, (1917-2012), in 'Action Potentials Recorded from Inside a Nerve Fibre', Nature (1939), 144, 710.

Newton lay 'neath a lofty palm tree,
When a coconut smashed on his knee.
“I realize so painfully,
The mystery of gravity.”
A coconut, not an apple, inspired his theory.
When a coconut smashed on his knee.
“I realize so painfully,
The mystery of gravity.”
A coconut, not an apple, inspired his theory.
Original idea by Webmaster, assisted by with Artificial Intelligence: ChatGPT.
Newton’s theory is the circle of generalization which includes all the others [as Kepler’s laws, Ptolemy’s theory, etc.];—the highest point of the inductive ascent;—the catastrophe of the philosophic drama to which Plato had prologized;— the point to which men’s minds had been journeying for two thousand years.
In History of the Inductive Sciences, Bk. 7, chap. 2, sect. 5.
No amount of experimentation can ever prove me right; a single experiment can prove me wrong.
Attributed to Einstein. Quoted in Alice Calaprice, The Quotable Einstein (1996), 224.
No experimental result can ever kill a theory: any theory can be saved from counterinstances either by some auxiliary hypothesis or by a suitable reinterpretation of its terms.
In 'Falsification and the Methodology of Scientific Research Programmes', in I. Lakatos and A. Musgrave (eds.), Criticism and the Growth of Knowledge: Proceedings of the International Colloquium in the Philosophy of Science, London 1965 (1970), Vol. 4, 116.
No generalizing beyond the data, no theory. No theory, no insight. And if no insight, why do research.
'Developing Theory About the Development of Theory,' in Ken G. Smith and Michael A. Hitt, Great Minds in Management: the Theory of Process Development (2005), 361.
No known theory can be distorted so as to provide even an approximate explanation [of wave-particle duality]. There must be some fact of which we are entirely ignorant and whose discovery may revolutionize our views of the relations between waves and ether and matter. For the present we have to work on both theories. On Mondays, Wednesdays, and Fridays we use the wave theory; on Tuesdays, Thursdays, and Saturdays we think in streams of flying energy quanta or corpuscles.
'Electrons and Ether Waves', The Robert Boyle Lecture 1921, Scientific Monthly, 1922, 14, 158.
No matter how we twist and turn we shall always come back to the cell. The eternal merit of Schwann does not lie in his cell theory that has occupied the foreground for so long, and perhaps will soon be given up, but in his description of the development of the various tissues, and in his demonstration that this development (hence all physiological activity) is in the end traceable back to the cell. Now if pathology is nothing but physiology with obstacles, and diseased life nothing but healthy life interfered with by all manner of external and internal influences then pathology too must be referred back to the cell.
In 'Cellular-Pathologie', Archiv für pathologische Anatomie und Physiologie und fur klinische Medizin (1855), 8, 13-14, as translated in LellandJ. Rather, 'Cellular Pathology', Disease, Life, and Man: Selected Essays by Rudolf Virchow (1958), 81.
No medieval schoolman has been singled out as a precursor more often than the French scholastic Nicole Oresme.This brilliant scholar has been credited with … the framing of Gresham’s law before Gresham, the invention of analytic geometry before Descartes, with propounding structural theories of compounds before nineteenth century organic chemists, with discovering the law of free fall before Galileo, and with advocating the rotation of the Earth before Copernicus. None of these claims is, in fact, true, although each is based on discussion by Oresme of some penetration and originality …
In Nicole Oresme and the Medieval Geometry of Quantities and Motions (1968).
No one has yet been found so firm of mind and purpose as resolutely to compel himself to sweep away all theories and common notions, and to apply the understanding, thus made fair and even, to a fresh examination of particulars. Thus it happens that human knowledge, as we have it, is a mere medley and ill-digested mass, made up of much credulity and much accident, and also of the childish notions which we at first imbibed.
In Novum Organum (1620), Book 2, Aphorism 20.
No one must think that Newton’s great creation can be overthrown in any real sense by this [Theory of Relativity] or by any other theory. His clear and wide ideas will for ever retain their significance as the foundation on which our modern conceptions of physics have been built.
In 'Time, Space, and Gravitation', The Times (28 Nov 1919). Excerpted in David E. Rowe and Robert J. Schulmann, Einstein on Politics: His Private Thoughts and Public Stands on Nationalism, Zionism, War, Peace, and the Bomb (2007), 104.
No one shall expel us from the paradise which Cantor has created for us.
Expressing the importance of Cantor's set theory in the development of mathematics.
Expressing the importance of Cantor's set theory in the development of mathematics.
In George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 33.
No other theory known to science [other than superstring theory] uses such powerful mathematics at such a fundamental level. …because any unified field theory first must absorb the Riemannian geometry of Einstein’s theory and the Lie groups coming from quantum field theory… The new mathematics, which is responsible for the merger of these two theories, is topology, and it is responsible for accomplishing the seemingly impossible task of abolishing the infinities of a quantum theory of gravity.
In 'Conclusion', Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth Dimension (1995), 326.
No part of Mathematics suffers more from the triviality of its initial presentation to beginners than the great subject of series. Two minor examples of series, namely arithmetic and geometric series, are considered; these examples are important because they are the simplest examples of an important general theory. But the general ideas are never disclosed; and thus the examples, which exemplify nothing, are reduced to silly trivialities.
In An Introduction to Mathematics (1911), 194.
No theory ever agrees with all the facts in its domain, yet it is not always the theory that is to blame. Facts are constituted by older ideologies, and a clash between facts and theories may be proof of progress. It is also a first step in our attempt to find the principles implicit in familiar observational notions.
Against Method: Outline of an Anarchistic Theory of Knowledge (1975, 1993), 39. This is his summary at the head of Chapter 5.
No theory of physics that deals only with physics will ever explain physics. I believe that as we go on trying to understand the universe, we are at the same time trying to understand man.
In The Intellectual Digest (June 1973), as quoted and cited in Mark Chandos, 'Philosophical Essay: Story Theory", Kosmoautikon: Exodus From Sapiens (2015).
Nothing in our experience suggests the introduction of [complex numbers]. Indeed, if a mathematician is asked to justify his interest in complex numbers, he will point, with some indignation, to the many beautiful theorems in the theory of equations, of power series, and of analytic functions in general, which owe their origin to the introduction of complex numbers. The mathematician is not willing to give up his interest in these most beautiful accomplishments of his genius.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences,' Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1 (February 1960). Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 537.
Nothing in physics seems so hopeful to as the idea that it is possible for a theory to have a high degree of symmetry was hidden from us in everyday life. The physicist's task is to find this deeper symmetry.
In American Scientist (1977) (as cited in The Atlantic (1984), 254, 81.) As an epigraph in Crystal and Dragon: The Cosmic Dance of Symmetry and Chaos in Nature, Art and Consciousness (1993), 139.
Nothing in the whole system of nature is isolated or unimportant. The fall of a leaf and the motion of a planet are governed by the same laws. … It is in the study of objects considered trivial and unworthy of notice by the casual observer that genius finds the most important and interesting phenomena. It was in the investigation of the varying colors of the soap-bubble that Newton detected the remarkable fact of the fits of easy reflection and easy refraction presented by a ray of light in its passage through space, and upon which he established the fundamental principle of the present generalization of the undulatory theory of light. … The microscopic organization of animals and plants is replete with the highest instruction; and, surely, in the language of one of the fathers of modern physical science, “nothing can be unworthy of being investigated by man which was thought worthy of being created by GOD.”
In 'Report of the Secretary', Seventh Annual Report of the Board of Regents of the Smithsonian Institution for 1852 (1853), 15.
Nothing is known in our profession by guess; and I do not believe, that from the first dawn of medical science to the present moment, a single correct idea has ever emanated from conjecture: it is right therefore, that those who are studying their profession should be aware that there is no short road to knowledge; and that observation on the diseased living, examination of the dead, and experiments upon living animals, are the only sources of true knowledge; and that inductions from these are the sole bases of legitimate theory.
Astley Paston Cooper, Astley Cooper, Bransby Blake Cooper, A Treatise on Dislocations and Fractures of the Joints (1851), 155.
Nothing is more interesting to the true theorist than a fact which directly contradicts a theory generally accepted up to that time, for this is his particular work.
A Survey of Physics (1925).
Now Einstein was a very clever man,
with us all his philosophies he shared,
He gave us the theory of relativity,
which is E equals M C squared.
with us all his philosophies he shared,
He gave us the theory of relativity,
which is E equals M C squared.
From lyrics of song Sod’s Law.
Now it came to me: … the independence of the gravitational acceleration from the nature of the falling substance, may be expressed as follows: In a gravitational field (of small spatial extension) things behave as they do in a space free of gravitation. … This happened in 1908. Why were another seven years required for the construction of the general theory of relativity? The main reason lies in the fact that it is not so easy to free oneself from the idea that coordinates must have an immediate metrical meaning.
In Paul Arthur Schilpp, 'Autobiographical Notes', Albert Einstein: Philosopher-Scientist (1949), 65-67.
Now that we locate them [genes] in the chromosomes are we justified in regarding them as material units; as chemical bodies of a higher order than molecules? Frankly, these are questions with which the working geneticist has not much concern himself, except now and then to speculate as to the nature of the postulated elements. There is no consensus of opinion amongst geneticists as to what the genes are—whether they are real or purely fictitious—because at the level at which the genetic experiments lie, it does not make the slightest difference whether the gene is a hypothetical unit, or whether the gene is a material particle. In either case the unit is associated with a specific chromosome, and can be localized there by purely genetic analysis. Hence, if the gene is a material unit, it is a piece of chromosome; if it is a fictitious unit, it must be referred to a definite location in a chromosome—the same place as on the other hypothesis. Therefore, it makes no difference in the actual work in genetics which point of view is taken. Between the characters that are used by the geneticist and the genes that his theory postulates lies the whole field of embryonic development.
'The Relation of Genetics to Physiology and Medicine', Nobel Lecture (4 Jun 1934). In Nobel Lectures, Physiology or Medicine 1922-1941 (1965), 315.
Now, in the development of our knowledge of the workings of Nature out of the tremendously complex assemblage of phenomena presented to the scientific inquirer, mathematics plays in some respects a very limited, in others a very important part. As regards the limitations, it is merely necessary to refer to the sciences connected with living matter, and to the ologies generally, to see that the facts and their connections are too indistinctly known to render mathematical analysis practicable, to say nothing of the complexity.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
Objections … inspired Kronecker and others to attack Weierstrass’ “sequential” definition of irrationals. Nevertheless, right or wrong, Weierstrass and his school made the theory work. The most useful results they obtained have not yet been questioned, at least on the ground of their great utility in mathematical analysis and its implications, by any competent judge in his right mind. This does not mean that objections cannot be well taken: it merely calls attention to the fact that in mathematics, as in everything else, this earth is not yet to be confused with the Kingdom of Heaven, that perfection is a chimaera, and that, in the words of Crelle, we can only hope for closer and closer approximations to mathematical truth—whatever that may be, if anything—precisely as in the Weierstrassian theory of convergent sequences of rationals defining irrationals.
In Men of Mathematics (1937), 431-432.
Observation is so wide awake, and facts are being so rapidly added to the sum of human experience, that it appears as if the theorizer would always be in arrears, and were doomed forever to arrive at imperfect conclusion; but the power to perceive a law is equally rare in all ages of the world, and depends but little on the number of facts observed.
In A Week on the Concord and Merrimack Rivers (1862), 383.
Observations always involve theory.
In The Realm of the Nebulae (1936), 35.
Of all regions of the earth none invites speculation more than that which lies beneath our feet, and in none is speculation more dangerous; yet, apart from speculation, it is little that we can say regarding the constitution of the interior of the earth. We know, with sufficient accuracy for most purposes, its size and shape: we know that its mean density is about 5½ times that of water, that the density must increase towards the centre, and that the temperature must be high, but beyond these facts little can be said to be known. Many theories of the earth have been propounded at different times: the central substance of the earth has been supposed to be fiery, fluid, solid, and gaseous in turn, till geologists have turned in despair from the subject, and become inclined to confine their attention to the outermost crust of the earth, leaving its centre as a playground for mathematicians.
'The Constitution of the Interior of the Earth, as Revealed by Earthquakes', Quarterly Journal of the Geological Society (1906), 62, 456.
Often a liberal antidote of experience supplies a sovereign cure for a paralyzing abstraction built upon a theory.
In The Paradoxes of Legal Science (1928).
Ohm found that the results could be summed up in such a simple law that he who runs may read it, and a schoolboy now can predict what a Faraday then could only guess at roughly. By Ohm's discovery a large part of the domain of electricity became annexed by Coulomb's discovery of the law of inverse squares, and completely annexed by Green's investigations. Poisson attacked the difficult problem of induced magnetisation, and his results, though differently expressed, are still the theory, as a most important first approximation. Ampere brought a multitude of phenomena into theory by his investigations of the mechanical forces between conductors supporting currents and magnets. Then there were the remarkable researches of Faraday, the prince of experimentalists, on electrostatics and electrodynamics and the induction of currents. These were rather long in being brought from the crude experimental state to a compact system, expressing the real essence. Unfortunately, in my opinion, Faraday was not a mathematician. It can scarely be doubted that had he been one, he would have anticipated much later work. He would, for instance, knowing Ampere's theory, by his own results have readily been led to Neumann’s theory, and the connected work of Helmholtz and Thomson. But it is perhaps too much to expect a man to be both the prince of experimentalists and a competent mathematician.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
On one occasion when [William] Smart found him engrossed with his fundamental theory, he asked Eddington how many people he thought would understand what he was writing—after a pause came the reply, 'Perhaps seven.'
A. V. Douglas, The Life of Arthur Stanley Eddington (1956), 110.
On quantum theory I use up more brain grease than on relativity.
…...
On the theory of natural selection we can clearly understand the full meaning of that old canon in natural history, “Natura non facit saltum.” This canon, if we look only to the present inhabitants of the world, is not strictly correct, but if we include all those of past times, it must by my theory be strictly true.
From On the Origin of Species by Means of Natural Selection; or, The Preservation of Favoured Races in the Struggle for Life (1861), 183.
On the whole, I cannot help saying that it appears to me not a little extraordinary, that a theory so new, and of such importance, overturning every thing that was thought to be the best established in chemistry, should rest on so very narrow and precarious a foundation, the experiments adduced in support of it being not only ambiguous or explicable on either hypothesis, but exceedingly few. I think I have recited them all, and that on which the greatest stress is laid, viz. That of the formation of water from the decomposition of the two kinds of air, has not been sufficiently repeated. Indeed it required so difficult and expensive an apparatus, and so many precautions in the use of it, that the frequent repetition of the experiment cannot be expected; and in these circumstances the practised experimenter cannot help suspecting the accuracy of the result and consequently the certainty of the conclusion.
Considerations on the Doctrine of Phlogiston (1796), 57-8.
Once the data are in, the theory has to follow along meekly.
Hardfought (1983). Quoted in Gary Westfahl, Science Fiction Quotations (2005), 322.
One day when the whole family had gone to a circus to see some extraordinary performing apes, I remained alone with my microscope, observing the life in the mobile cells of a transparent star-fish larva, when a new thought suddenly flashed across my brain. It struck me that similar cells might serve in the defence of the organism against intruders. Feeling that there was in this something of surpassing interest, I felt so excited that I began striding up and down the room and even went to the seashore in order to collect my thoughts.
I said to myself that, if my supposition was true, a splinter introduced into the body of a star-fish larva, devoid of blood-vessels or of a nervous system, should soon be surrounded by mobile cells as is to be observed in a man who runs a splinter into his finger. This was no sooner said than done.
There was a small garden to our dwelling, in which we had a few days previously organised a 'Christmas tree' for the children on a little tangerine tree; I fetched from it a few rose thorns and introduced them at once under the skin of some beautiful star-fish larvae as transparent as water.
I was too excited to sleep that night in the expectation of the result of my experiment, and very early the next morning I ascertained that it had fully succeeded.
That experiment formed the basis of the phagocyte theory, to the development of which I devoted the next twenty-five years of my life.
I said to myself that, if my supposition was true, a splinter introduced into the body of a star-fish larva, devoid of blood-vessels or of a nervous system, should soon be surrounded by mobile cells as is to be observed in a man who runs a splinter into his finger. This was no sooner said than done.
There was a small garden to our dwelling, in which we had a few days previously organised a 'Christmas tree' for the children on a little tangerine tree; I fetched from it a few rose thorns and introduced them at once under the skin of some beautiful star-fish larvae as transparent as water.
I was too excited to sleep that night in the expectation of the result of my experiment, and very early the next morning I ascertained that it had fully succeeded.
That experiment formed the basis of the phagocyte theory, to the development of which I devoted the next twenty-five years of my life.
In Olga Metchnikoff, Life of Elie Metchnikoff 1845-1916 (1921), 116-7.
One day while I was teaching at Marburg a man came to me, whose fine features and penetrating, gray-blue eyes I was unable to forget. He had developed an extraordinary theory in regard to the structure of the earth. He asked me whether I, a geologist, was prepared to help him, a physicist, by contributing pertinent geological facts and concepts. I liked the man very much, even though I was skeptical of his ideas. Thus began a loose co-operation on a subject in which the Red Sea rapidly assumed a central position.
The man was Alfred Wegener.
The man was Alfred Wegener.
In Hans Cloos, Ernst Cloos (ed.) and Curt Dietz (ed.), Conversation With the Earth (1953, 1959), 395-396, as translated by E.B. Garside from the original German edition, Gespräch mit der Erde (1947). For a variant translation of this same passage, see elsewhere on this site’s webpage for Hans Cloos quotations, for the one beginning, “The war found me in Marburg.…”
One of Euler’s main recreations was music, and by cultivating it he brought with it all his geometrical spirit; … he rested his serious researches and composed his Essay of a New Theory of Music, published in 1739; a book full of new ideas presented in a new point of view, but that did not have a great success, apparently for the sole reason that it contains too much of geometry for the musician and too much music for the geometer.
From his Eulogy of Leonhard Euler, read at the Imperial Academy of Sciences of Saint Petersburg (23 Oct 1783). Published in 'Éloge de Léonard Euler, Prononcé en Français par Nicolas Fuss'. Collected in Leonard Euler, Oeuvres Complètes en Français de L. Euler (1839), Vol. 1, xii. From the original French, “Un des principaux délassements d'Euler était la musique, et en la cultivant il y apporta tout son esprit géométrique; … il accordait à ses recherches profondes, il composa son Essai d'une nouvelle théorie de la musique, publié en 1739; ouvrage rempli d'idées neuves ou présentées sous un nouveau point de vue, mais qui n’eut pas un grand succès, apparemment par la seule raison qu’il renferme trop de géométrie pour le musicien et trop de musique pour le géomètre.” English version by Webmaster using Google translate.
One of the best examples of a scientific parable that got taken literally at first is the wave-theory of light.
Concluding paragraph of chapter, 'Physics, Astronomy, and Mathematics: Or Beyond Common-Sense', contributed to Naomi Mitchison (ed.), An Outline For Boys And Girls And Their Parents (1932), 357.
One of the endlessly alluring aspects of mathematics is that its thorniest paradoxes have a way of blooming into beautiful theories.
In 'Number', Scientific American (Sep 1964), 211, No. 3, 55.
One of the most beautiful hypotheses ever propounded in physics is ... the Dynamical Theory of Gases
Speaking to the 491st Meeting (30 Jan1861), Proceedings of the American Academy of Arts and Sciences (1862), Vol. 5, 112.
One of the most immediate consequences of the electrochemical theory is the necessity of regarding all chemical compounds as binary substances. It is necessary to discover in each of them the positive and negative constituents... No view was ever more fitted to retard the progress of organic chemistry. Where the theory of substitution and the theory of types assume similar molecules, in which some of the elements can be replaced by others without the edifice becoming modified either in form or outward behaviour, the electrochemical theory divides these same molecules, simply and solely, it may be said, in order to find in them two opposite groups, which it then supposes to be combined with each other in virtue of their mutual electrical activity... I have tried to show that in organic chemistry there exist types which are capable, without destruction, of undergoing the most singular transformations according to the nature of the elements.
Traité de Chemie Appliquée aux Arts, Vol. I (1828), 53. Trans. J. R. Partington, A History of Chemistry, Vol. 4, 366.
Our empirical criterion for a series of theories is that it should produce new facts. The idea of growth and the concept of empirical character are soldered into one.
In 'Falsification and the Methodology of Scientific Research Programmes', in I. Lakatos and A. Musgrave (eds.), Criticism and the Growth of Knowledge: Proceedings of the International Colloquium in the Philosophy of Science, London 1965 (1970), Vol. 4, 119.
Our most successful theories in physics are those that explicitly leave room for the unknown, while confining this room sufficiently to make the theory empirically disprovable. It does not matter whether this room is created by allowing for arbitrary forces as Newtonian dynamics does, or by allowing for arbitrary equations of state for matter, as General Relativity does, or for arbitrary motions of charges and dipoles, as Maxwell's electrodynamics does. To exclude the unknown wholly as a “unified field theory” or a “world equation” purports to do is pointless and of no scientific significance.
Our natural way of thinking about these coarser emotions is that the mental perception of some fact excites the mental affection called the emotion, and that this latter state of mind gives rise to the bodily expression. My theory, on the contrary, is that the bodily changes follow directly the perception of the exciting fact, and that our feeling of the same changes as they occur IS the emotion. Common-sense says, we lose our fortune, are sorry and weep; we meet a bear, are frightened and run; we are insulted by a rival, are angry and strike. The hypothesis here to be defended says that this order of sequence is incorrect, that the one mental state is not immediately induced by the other, that the bodily manifestations must first be interposed between, and that the more rational statement is that we feel sorry because we cry, angry because we strike, afraid because we tremble, and not that we cry, strike, or tremble, because we are sorry, angry, or fearful, as the case may be. Without the bodily states following on the perception, the latter would be purely cognitive in form, pale, colorless, destitute of emotional warmth. We might then see the bear, and judge it best to run, receive the insult and deem it right to strike, but we should not actually feel afraid or angry.
The Principles or Psychology (1890), Vol. 2, 449-50.
Our progress in education has truly been a curious one. We have gone from the hard and arbitrary curriculum, with its primary insistence upon training the memory and the consequent devitalization of valuable and beneficial subjects, to the free elective system, with its wholesale invitations to follow the paths of least resistance, back to a half-hearted compromise somewhere between the two extremes, and we have arrived at what? Certainly at little more than an educational jumble. A maelstrom in which the maximum amount of theory and the minimum amount of practice whirl those who are thrown into it round and round for definitely fixed periods of time, to be cast out as flotsam for another period until corporate business and industrial organizations can accomplish that which could and should have been done by general education.
Co-author with Louis Jay Heath, in A New Basis for Social Progress (1917), 151-152.
Our scientific work in physics consists in asking questions about nature in the language that we possess and trying to get an answer from experiment by the means at our disposal. In this way quantum theory reminds us, as Bohr has put it, of the old wisdom that when searching for harmony in life one must never forget that in the drama of existence we are ourselves both players and spectators. It is understandable that in our scientific relation to nature our own activity becomes very important when we have to deal with parts of nature into which we can penetrate only by using the most elaborate tools.
The Copenhagen Interpretation of Quantum Theory (1958). In Steve Adams, Frontiers (2000), 13.
Owing to his lack of knowledge, the ordinary man cannot attempt to resolve conflicting theories of conflicting advice into a single organized structure. He is likely to assume the information available to him is on the order of what we might think of as a few pieces of an enormous jigsaw puzzle. If a given piece fails to fit, it is not because it is fraudulent; more likely the contradictions and inconsistencies within his information are due to his lack of understanding and to the fact that he possesses only a few pieces of the puzzle. Differing statements about the nature of things, differing medical philosophies, different diagnoses and treatments—all of these are to be collected eagerly and be made a part of the individual's collection of puzzle pieces. Ultimately, after many lifetimes, the pieces will fit together and the individual will attain clear and certain knowledge.
'Strategies of Resort to Curers in South India', contributed in Charles M. Leslie (ed.), Asian Medical Systems: A Comparative Study (1976), 185.
Parkinson's Law is a purely scientific discovery, inapplicable except in theory to the politics of the day. It is not the business of the botanist to eradicate the weeds. Enough for him if he can tell us just how fast they grow.
Parkinson's Law or the Pursuit of Progress (1958), 15.
People declare as much, without, apparently, looking into the matter very closely. They seem able to dispense with the conscientious observer’s scruples, when inflating their bladder of theory.
People have wracked their brains for an explanation of benzene and how the celebrated man [Kekulé] managed to come up with the concept of the benzene theory. With regard to the last point especially, a friend of mine who is a farmer and has a lively interest in chemistry has asked me a question which I would like to share with you. My “agricultural friend” apparently believes he has traced the origins of the benzene theory. “Has Kekulé,” so ran the question, “once been a bee-keeper? You certainly know that bees too build hexagons; they know well that they can store the greatest amount of honey that way with the least amount of wax. I always liked it,” my agricultural friend went on, “When I received a new issue of the Berichte; admittedly, I don't read the articles, but I like the pictures very much. The patterns of benzene, naphthalene and especially anthracene are indeed wonderful. When I look at the pictures I always have to think of the honeycombs of my bee hives.”
A. W. Hofmann, after-dinner speech at Kekulé Benzolfest (Mar 1890). Trans. in W. H. Brock, O. Theodor Benfrey and Susanne Stark, 'Hofmann's Benzene Tree at the Kekulé Festivities', Journal of Chemical Education (1991), 68, 888.
People were pretty well spellbound by what Bohr said… While I was very much impressed by [him], his arguments were mainly of a qualitative nature, and I was not able to really pinpoint the facts behind them. What I wanted was statements which could be expressed in terms of equations, and Bohr's work very seldom provided such statements. I am really not sure how much later my work was influenced by these lectures of Bohr's... He certainly did not have a direct influence because he did not stimulate one to think of new equations.
Recalling the occasion in May 1925 (a year before receiving his Ph.D.) when he met Niels Bohr who was in Cambridge to give a talk on the fundamental difficulties of the quantum theory.
Recalling the occasion in May 1925 (a year before receiving his Ph.D.) when he met Niels Bohr who was in Cambridge to give a talk on the fundamental difficulties of the quantum theory.
In History of Twentieth Century Physics (1977), 109. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 94.
Perfect as the wing of a bird may be, it will never enable the bird to fly if unsupported by the air. Facts are the air of science. Without them a man of science can never rise. Without them your theories are vain surmises. But while you are studying, observing, experimenting, do not remain content with the surface of things. Do not become a mere recorder of facts, but try to penetrate the mystery of their origin. Seek obstinately for the laws that govern them.
Translation of a note, 'Bequest of Pavlov to the Academic Youth of his Country', written a few days before his death for a student magazine, The Generation of the Victors. As published in 'Pavlov and the Spirit of Science', Nature (4 Apr 1936), 137, 572.
Philosophers of science constantly discuss theories and representation of reality, but say almost nothing about experiment, technology, or the use of knowledge to alter the world. This is odd, because ‘experimental method’ used to be just another name for scientific method.... I hope [to] initiate a Back-to-Bacon movement, in which we attend more seriously to experimental science. Experimentation has a life of its own.
Representing and Intervening, p. 149f (1983). Announcing the author's intention to stress 'intervening' as an essential component of science.
Poincaré was a vigorous opponent of the theory that all mathematics can be rewritten in terms of the most elementary notions of classical logic; something more than logic, he believed, makes mathematics what it is.
In Men of Mathematics (1937), 552.
Positivism is a theory of knowledge according to which the only kind of sound knowledge available to human kind is that if science grounded in observation.
(1891). As given as an epigraph in M.J. Vinod and Meena Deshpande, Contemporary Political Theory (2013), 3.
Precise facts alone are worthy of science. They cast premature theories into oblivion.
From the French original, “Les faits précis sont seuls dignes de la science. Ils rejettent dans l’oubli les théories prématurées”, by F. Marguet, 'Les “Souvenirs entomologiques” de J.-H. Fabre', Revue des Deux Mondes (1910), 60, 870. As translated in Augustin Fabre, The Life of Jean Henri Fabre: The Entomologist, 1823-1910 (1921), 315. Marguet gives no source citation for quote. It might be synthesized by Marguet from the writings of Fabre, to represent Fabre’s work ethic. (Webmaster so far has not found a primary source for the French sentences as verbatim. Can you help?)
Professor [Max] Planck, of Berlin, the famous originator of the Quantum Theory, once remarked to me that in early life he had thought of studying economics, but had found it too difficult! Professor Planck could easily master the whole corpus of mathematical economics in a few days. He did not mean that! But the amalgam of logic and intuition and the wide knowledge of facts, most of which are not precise, which is required for economic interpretation in its highest form is, quite truly, overwhelmingly difficult for those whose gift mainly consists in the power to imagine and pursue to their furthest points the implications and prior conditions of comparatively simple facts which are known with a high degree of precision.
'Alfred Marshall: 1842-1924' (1924). In Geoffrey Keynes (ed.), Essays in Biography (1933), 191-2
Professor Bethe … is a man who has this characteristic: If there’s a good experimental number you’ve got to figure it out from theory. So, he forced the quantum electrodynamics of the day to give him an answer [for the experimentally measured Lamb-shift of hydrogen], … and thus, made
the most important discovery in the history of the theory of quantum electrodynamics. He worked this out on the train from Ithaca, New York to Schenectady.
Bethe calculated, what Lamb had experimentally just measured, for the separation of the 2S½ and 2P½ of hydrogen. Both theory and measurement yielded about one thousand megacycles for the Lamb-shift. Feynman was at the time associated with Bethe at Cornell. In Feynman’s Nobel Prize Lecture (11 Dec 1965), 'The Development of the Space-Time View of Quantum Electrodynamics'. Collected in Stig Lundqvist, Nobel Lectures: Physics, 1963-1970 (1998), 170.
Professor, how can you bring yourself to enter this chemical building that has Ionic columns?
[Kahlenberg, a physical chemist, was an opponent of ionic theory.]
[Kahlenberg, a physical chemist, was an opponent of ionic theory.]
Quoted in Ralph Oesper, The Human Side of Scientists (1975), 106.
Professors in every branch of the sciences, prefer their own theories to truth: the reason is that their theories are private property, but truth is common stock.
Reflection 378, in Lacon: or Many things in Few Words; Addressed to Those Who Think (1820), 169.
Progress cannot long be made in the application of the sciences without cultivating the theory of them.
In Alexis de Tocqueville and Henry Reeve (trans.), Democracy in America (1840, 1899), Vol. 2, 37.
Progress is achieved by exchanging our theories for new ones which go further than the old, until we find one based on a larger number of facts. … Theories are only hypotheses, verified by more or less numerous facts. Those verified by the most facts are the best, but even then they are never final, never to be absolutely believed.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 165.
Propose theories which can be criticized. Think about possible decisive falsifying experiments—crucial experiments. But do not give up your theories too easily—not, at any rate, before you have critically examined your criticism.
'The Problem of Demarcation' (1974). Collected in David Miller (ed.) Popper Selections (1985), 126-127.
Psychoanalytic theory is the most stupendous intellectual confidence trick of the twentieth century and a terminal product as well—something akin to a dinosaur or zeppelin in the history of ideas, a vast structure of radically unsound design and with no posterity.
'Victims of Psychiatry', The New York Review of Books (23 Jan 1975), 21. Cited in David E. Stannard, Shrinking History: On Freud and the Failure of Psychohistory (1980), 150.
Psychology appeared to be a jungle of confusing, conflicting, and arbitrary concepts. These pre-scientific theories doubtless contained insights which still surpass in refinement those depended upon by psychiatrists or psychologists today. But who knows, among the many brilliant ideas offered, which are the true ones? Some will claim that the statements of one theorist are correct, but others will favour the views of another. Then there is no objective way of sorting out the truth except through scientific research.
From The Scientific Analysis of Personality (1965), 14.
Psychotherapy–the theory that the patient will probably get well anyhow, and is certainly a damn fool.
In A Mencken Chrestomathy (1949, 1956), 626.
Pure mathematics is a collection of hypothetical, deductive theories, each consisting of a definite system of primitive, undefined, concepts or symbols and primitive, unproved, but self-consistent assumptions (commonly called axioms) together with their logically deducible consequences following by rigidly deductive processes without appeal to intuition.
In 'Non-Euclidian Geometry of the Fourth Dimension', collected in Henry Parker Manning (ed.), The Fourth Dimension Simply Explained (1910), 58.
Pure mathematics is much more than an armoury of tools and techniques for the applied mathematician. On the other hand, the pure mathematician has ever been grateful to applied mathematics for stimulus and inspiration. From the vibrations of the violin string they have drawn enchanting harmonies of Fourier Series, and to study the triode valve they have invented a whole theory of non-linear oscillations.
In 100 Years of Mathematics: a Personal Viewpoint (1981), 3.
Quantum field theory, which was born just fifty years ago from the marriage of quantum mechanics with relativity, is a beautiful but not very robust child.
In Nobel Lecture (8 Dec 1989), 'Conceptual Foundations of the Unified Theory of Weak and Electromagnetic Interactions.'
Quantum mechanics and relativity, taken together, are extraordinarily restrictive, and they therefore provide us with a great logical machine. We can explore with our minds any number of possible universes consisting of all kinds of mythical particles and interactions, but all except a very few can be rejected on a priori grounds because they are not simultaneously consistent with special relativity and quantum mechanics. Hopefully in the end we will find that only one theory is consistent with both and that theory will determine the nature of our particular universe.
As quoted in John D. Barrow, The Universe that Discovered Itself (2000), 360.
Quantum mechanics is certainly imposing. But an inner voice tells me that this is not yet the real thing. The theory says a lot, but does not bring us any closer to the secrets of the “Old One.” I, at any rate, am convinced that He is not playing at dice.
Letter to Max Born (4 Dec 1926). Collected in The Born-Einstein Letters: Correspondence between Albert Einstein and Max and Hedwig Born from 1916-1955 (1971), 91. Also seen as “God does not play dice [with the universe].”
Quantum theory thus reveals a basic oneness of the universe. It shows that we cannot decompose the world into independently existing smallest units. As we penetrate into matter, nature does not show us any isolated “building blocks,” but rather appears as a complicated web of relations between the various parts of the whole. These relations always include the observer in an essential way. The human observer constitute the final link in the chain of observational processes, and the properties of any atomic object can be understood only in terms of the object’s interaction with the observer.
In The Tao of Physics (1975), 68.
Quantum theory—at least in the Heisenberg interpretation—describes the way the world works as a literal moment-to-moment emergence of actual facts out of a background of less factual 'potentia.'
Quoted in article 'Nick Herbert', in Gale Cengage Learning, Contemporary Authors Online (2002).
Quite distinct from the theoretical question of the manner in which mathematics will rescue itself from the perils to which it is exposed by its own prolific nature is the practical problem of finding means of rendering available for the student the results which have been already accumulated, and making it possible for the learner to obtain some idea of the present state of the various departments of mathematics. … The great mass of mathematical literature will be always contained in Journals and Transactions, but there is no reason why it should not be rendered far more useful and accessible than at present by means of treatises or higher text-books. The whole science suffers from want of avenues of approach, and many beautiful branches of mathematics are regarded as difficult and technical merely because they are not easily accessible. … I feel very strongly that any introduction to a new subject written by a competent person confers a real benefit on the whole science. The number of excellent text-books of an elementary kind that are published in this country makes it all the more to be regretted that we have so few that are intended for the advanced student. As an example of the higher kind of text-book, the want of which is so badly felt in many subjects, I may mention the second part of Prof. Chrystal’s Algebra published last year, which in a small compass gives a great mass of valuable and fundamental knowledge that has hitherto been beyond the reach of an ordinary student, though in reality lying so close at hand. I may add that in any treatise or higher text-book it is always desirable that references to the original memoirs should be given, and, if possible, short historic notices also. I am sure that no subject loses more than mathematics by any attempt to dissociate it from its history.
In Presidential Address British Association for the Advancement of Science, Section A (1890), Nature, 42, 466.
Quite recently the human descent theory has been stigmatized as the “gorilla theory of human ancestry.” All this despite the fact that Darwin himself, in the days when not a single bit of evidence regarding the fossil ancestors of man was recognized, distinctly stated that none of the known anthropoid apes, much less any of the known monkeys, should be considered in any way as ancestral to the human stock.
In Henry Fairfield Osborn, 'Osborn States the Case For Evolution', New York Times (12 Jul 1925), XX1. Written at the time of the Scopes Monkey Trial, in rebuttal of the anti-evolution position publicized by William Jennings Bryan.
Reality is what kicks back when you kick it. This is just what physicists do with their particle accelerators. We kick reality and feel it kick back. From the intensity and duration of thousands of those kicks over many years, we have formed a coherent theory of matter and forces, called the standard model, that currently agrees with all observations.
In Has Science Found God?: The Latest Results in the Search for Purpose in the Universe (2003), 41.
Reason may be employed in two ways to establish a point: first for the purpose of furnishing sufficient proof of some principle, as in natural science, where sufficient proof can be brought to show that the movement of the heavens is always of uniform velocity. Reason is employed in another way, not as furnishing a sufficient proof of a principle, but as confirming an already established principle, by showing the congruity of its results, as in astrology the theory of eccentrics and epicycles is considered as established because thereby the sensible appearances of the heavenly movements can be explained; not, however, as if this reason were sufficient, since some other theory might explain them.
Summa Theologica [1266-1273], Part I, question 32, article 2 (reply to objection 2), trans. Fathers of the English Dominican Province (i.e. L. Shapeote), revised D. J. Sullivan (1952), Vol. I, 177.
Relativity was a highly technical new theory that gave new meanings to familiar concepts and even to the nature of the theory itself. The general public looked upon relativity as indicative of the seemingly incomprehensible modern era, educated scientists despaired of ever understanding what Einstein had done, and political ideologues used the new theory to exploit public fears and anxieties—all of which opened a rift between science and the broader culture that continues to expand today.
'The Cultural Legacy of Relativity Theory' in Albert Einstein, Robert W. Lawson, Robert Geroch, Roger Penrose and David C. Cassidy, Relativity (2005), 226.
Religious creeds are a great obstacle to any full sympathy between the outlook of the scientist and the outlook which religion is so often supposed to require … The spirit of seeking which animates us refuses to regard any kind of creed as its goal. It would be a shock to come across a university where it was the practice of the students to recite adherence to Newton's laws of motion, to Maxwell's equations and to the electromagnetic theory of light. We should not deplore it the less if our own pet theory happened to be included, or if the list were brought up to date every few years. We should say that the students cannot possibly realise the intention of scientific training if they are taught to look on these results as things to be recited and subscribed to. Science may fall short of its ideal, and although the peril scarcely takes this extreme form, it is not always easy, particularly in popular science, to maintain our stand against creed and dogma.
Swarthmore Lecture (1929), Science and the Unseen World (1929), 54-56.
Samuel Pierpoint Langley, at that time regarded as one of the most distinguished scientists in the United States … evidently believed that a full sized airplane could be built and flown largely from theory alone. This resulted in two successive disastrous plunges into the Potomac River, the second of which almost drowned his pilot. This experience contrasts with that of two bicycle mechanics Orville and Wilbur Wright who designed, built and flew the first successful airplane. But they did this after hundreds of experiments extending over a number of years.
In article Total Quality: Its Origins and its Future (1995), published at the Center for Quality and Productivity Improvement.
Sarcastic Science, she would like to know,
In her complacent ministry of fear,
How we propose to get away from here
When she has made things so we have to go
Or be wiped out. Will she be asked to show
Us how by rocket we may hope to steer
To some star off there, say, a half light-year
Through temperature of absolute zero?
Why wait for Science to supply the how
When any amateur can tell it now?
The way to go away should be the same
As fifty million years ago we came—
If anyone remembers how that was
I have a theory, but it hardly does.
In her complacent ministry of fear,
How we propose to get away from here
When she has made things so we have to go
Or be wiped out. Will she be asked to show
Us how by rocket we may hope to steer
To some star off there, say, a half light-year
Through temperature of absolute zero?
Why wait for Science to supply the how
When any amateur can tell it now?
The way to go away should be the same
As fifty million years ago we came—
If anyone remembers how that was
I have a theory, but it hardly does.
'Why Wait for Science?' In Edward Connery Latham (ed.), The Poetry of Robert Frost: The Collected Poems, Complete and Unabridged (1979), 395.
Scarcely any attempt is entirely a failure; scarcely any theory, the result of steady thought, is altogether false; no tempting form of Error is without some latent charm derived from Truth.
In 'Lecture 7', Lectures on the History of Moral Philosophy in England (1852), 101.
Scarcely anyone who comprehends this theory can escape its magic.
Quoted, without citation, in Norman K. Glendenning, Our Place in the Universe (2007), 107. Webmaster has not found any other source for this quote, and cautions doubt about its authenticity. If you know a primary source, please contact Webmaster.
Schrodinger’s wave-mechanics is not a physical theory but a dodge—and a very good dodge too.
Gifford Lectures (1927), The Nature of the Physical World (1928), 219.
Science asks no questions about the ontological pedigree or a priori character of a theory, but is content to judge it by its performance; and it is thus that a knowledge of nature, having all the certainty which the senses are competent to inspire, has been attained—a knowledge which maintains a strict neutrality toward all philosophical systems and concerns itself not with the genesis or a priori grounds of ideas.
Originally published in North American Review (1865). 'The Philosophy of Herbert Spencer,' repr. In Philosophical Writings of Chauncey Wright (1963), p. 8.
Science deals with judgments on which it is possible to obtain universal agreement. These judgments do not concern individual facts and events, but the invariable association of facts and events known as the laws of science. Agreement is secured by observation and experiment—impartial courts of appeal to which all men must submit if they wish to survive. The laws are grouped and explained by theories of ever increasing generality. The theories at first are ex post facto—merely plausible interpretations of existing bodies of data. However, they frequently lead to predictions that can be tested by experiments and observations in new fields, and, if the interpretations are verified, the theories are accepted as working hypotheses until they prove untenable. The essential requirements are agreement on the subject matter and the verification of predictions. These features insure a body of positive knowledge that can be transmitted from person to person, and that accumulates from generation to generation.
From manuscript on English Science in the Renaissance (1937), Edwin Hubble collection, Box 2, Huntington Library, San Marino, California. As cited by Norriss S. Hetherington in 'Philosophical Values and Observation in Edwin Hubble's Choice of a Model of the Universe', Historical Studies in the Physical Sciences (1982), 13, No. 1, 41. (Hetherington comments parenthetically that the references to court, judgment and appeal may be attributable to his prior experiences as a Rhodes Scholar reading Roman law at Oxford, and to a year's practice as an attorney in Louisville, Kentucky.)
Science derives its conclusions by the laws of logic from our sense perceptions, Thus it does not deal with the real world, of which we know nothing, but with the world as it appears to our senses. … All our sense perceptions are limited by and attached to the conceptions of time and space. … Modern physics has come to the same conclusion in the relativity theory, that absolute space and absolute time have no existence, but, time and space exist only as far as things or events fill them, that is, are forms of sense perception.
In 'Religion and Modern Science', The Christian Register (16 Nov 1922), 101, 1089. The article is introduced as “the substance of an address to the Laymen’s League in All Soul’s Church (5 Nov 1922).
Science gains from it [the pendulum] more than one can expect. With its huge dimensions, the apparatus presents qualities that one would try in vain to communicate by constructing it on a small [scale], no matter how carefully. Already the regularity of its motion promises the most conclusive results. One collects numbers that, compared with the predictions of theory, permit one to appreciate how far the true pendulum approximates or differs from the abstract system called 'the simple pendulum'.
In 'Demonstration Experimentale du Movement de Rotation de la Terre' (31 May 1851). In C.M. Gariel (ed.), J. Bertrand (ed.) and Harold Burstyn (trans.), Recueil des Travaux Scientifiques de Lion Foucault (1878), Vol. 2, 527.
Science has hitherto been proceeding without the guidance of any rational theory of logic, and has certainly made good progress. It is like a computer who is pursuing some method of arithmetical approximation. Even if he occasionally makes mistakes in his ciphering, yet if the process is a good one they will rectify themselves. But then he would approximate much more rapidly if he did not commit these errors; and in my opinion, the time has come when science ought to be provided with a logic. My theory satisfies me; I can see no flaw in it. According to that theory universality, necessity, exactitude, in the absolute sense of these words, are unattainable by us, and do not exist in nature. There is an ideal law to which nature approximates; but to express it would require an endless series of modifications, like the decimals expressing surd. Only when you have asked a question in so crude a shape that continuity is not involved, is a perfectly true answer attainable.
Letter to G. F. Becker, 11 June 1893. Merrill Collection, Library of Congress. Quoted in Nathan Reingold, Science in Nineteenth-Century America: A Documentary History (1966), 231-2.
Science has so accustomed us to devising and accepting theories to account for the facts we observe, however fantastic, that our minds must begin their manufacture before we are aware of it.
Seven American Nights (1978). In the collection, David G. Hartwell (Ed.), The Dark Descent (1997), 653.
Science in England, in America, is jealous of theory, hates the name of love and moral purpose. There's revenge for this humanity. What manner of man does science make? The boy is not attracted. He says, I do not wish to be such a kind of man as my professor is.
In essay. 'Beauty', collected in The Conduct of Life (1860), 250.
Science is always discovering odd scraps of magical wisdom and making a tremendous fuss about its cleverness.
Referring to Freudian theories.
Referring to Freudian theories.
The Confessions of Aleister Crowley, ch. 14 (1929, rev 1970).
Science is an essentially anarchic enterprise: theoretical anarchism is more humanitarian and more likely to encourage progress than its law-and-order alternatives.
From Against Method: Outline of an Anarchistic Theory of Knowledge (1975, 1993), 9.
Science is in a literal sense constructive of new facts. It has no fixed body of facts passively awaiting explanation, for successful theories allow the construction of new instruments—electron microscopes and deep space probes—and the exploration of phenomena that were beyond description—the behavior of transistors, recombinant DNA, and elementary particles, for example. This is a key point in the progressive nature of science—not only are there more elegant or accurate analyses of phenomena already known, but there is also extension of the range of phenomena that exist to be described and explained.
Co-author with Michael A. Arbib, English-born professor of computer science and biomedical engineering (1940-)
Co-author with Michael A. Arbib, English-born professor of computer science and biomedical engineering (1940-)
Michael A. Arbib and Mary B. Hesse, The Construction of Reality (1986), 8.
Science is not about control. It is about cultivating a perpetual sense of wonder in the face of something that forever grows one step richer and subtler than our latest theory about it. It is about reverence, not mastery.
…...
Science is uncertain. Theories are subject to revision; observations are open to a variety of interpretations, and scientists quarrel amongst themselves. This is disillusioning for those untrained in the scientific method, who thus turn to the rigid certainty of the Bible instead. There is something comfortable about a view that allows for no deviation and that spares you the painful necessity of having to think.
The 'Threat' of Creationism. In Ashley Montagu (ed.), Science and Creationism (1984), 192.
Science preceded the theory of science, and is independent of it. Science preceded naturalism, and will survive it.
The Foundations of Belief: Being Notes Introductory to the Study of Theology (1895), 134.
Science’s biggest mystery is the nature of consciousness. It is not that we possess bad or imperfect theories of human awareness; we simply have no such theories at all.
In Quantum Reality: Beyond the New Physics (1987), 249.
Scientific discovery, or the formulation of scientific theory, starts in with the unvarnished and unembroidered evidence of the senses. It starts with simple observation—simple, unbiased, unprejudiced, naive, or innocent observation—and out of this sensory evidence, embodied in the form of simple propositions or declarations of fact, generalizations will grow up and take shape, almost as if some process of crystallization or condensation were taking place. Out of a disorderly array of facts, an orderly theory, an orderly general statement, will somehow emerge.
In 'Is the Scientific Paper Fraudulent?', The Saturday Review (1 Aug 1964), 42.
Scientific subjects do not progress necessarily on the lines of direct usefulness. Very many applications of the theories of pure mathematics have come many years, sometimes centuries, after the actual discoveries themselves. The weapons were at hand, but the men were not able to use them.
In Perry, Teaching of Mathematics (1902), 35.
Scientific theories can always be improved and are improved. That is one of the glories of science. It is the authoritarian view of the Universe that is frozen in stone and cannot be changed, so that once it is wrong, it is wrong forever.
Essay, 'The Nearest Star', The Magazine of Fantasy and Science Fiction (Oct 1989), collected in The Secret of the Universe (1991), 82.
Scientific theories need reconstruction every now and then. If they didn't need reconstruction they would be facts, not theories. The more facts we know, the less radical become the changes in our theories. Hence they are becoming more and more constant. But take the theory of gravitation; it has not been changed in four hundred years.
As quoted in 'Electricity Will Keep The World From Freezing Up', New York Times (12 Nov 1911), SM4.
Scientific theories tell us what is possible; myths tell us what is desirable. Both are needed to guide proper action.
…...
Scientific theory is a contrived foothold in the chaos of living phenomena.
In Function of the Orgasm: Discovery of the Orgone (1927, 1973), 39-40.
Scientists are not robotic inducing machines that infer structures of explanation only from regularities observed in natural phenomena (assuming, as I doubt, that such a style of reasoning could ever achieve success in principle). Scientists are human beings, immersed in culture, and struggling with all the curious tools of inference that mind permits ... Culture can potentiate as well as constrain–as Darwin’s translation of Adam Smith’s laissez-faire economic models into biology as the theory of natural selection. In any case, objective minds do not exist outside culture, so we must make the best of our ineluctable embedding.
…...
Scientists aren’t one tenth, nor one hundredth of one percent as silly as the asinine theory-hawking befuddlists who attack them.
Letter from London (20 Apr 1937), No. 81, in George Gaylord Simpson and Léo F. LaPorte (ed.), Simple Curiosity: Letters from George Gaylord Simpson to His Family, 1921-1970 (1987), 34.
Scientists can only carry on with their work, addressing legitimate questions as they arise and challenging misinformation. … Scientists work to fill the gaps in human knowledge and to build a theory that can explain observations of the world. Climate sceptics revel in such gaps, sometimes long after they have been filled.
Editorial, Nature (28 Jul 2011), 475, 423-424.
Scientists have odious manners, except when you prop up their theory; then you can borrow money off them.
The Bee (c.1902). In What is Man? And Other Essays (1917), 283. Reprinted in Charles Neider (ed.), Complete Essays (1963). In Mark Twain and Brian Collins (ed.), When in Doubt, Tell the Truth: and Other Quotations from Mark Twain (1996), 118.
Scientists have thick skins. They do not abandon a theory merely because facts contradict it.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 4.
Scientists repeatedly return to established theories to test them in new ways, and tend towards testiness with those priests, religious or secular, who know the answers already—whatever the questions are.
With co-author Jack Cohen. In Terry Pratchett, Ian Stewart and Jack Cohen, Chap. 10, 'The Shape of Things', The Science of Discworld (1999), 78. Pratchett wrote the fantasy story told in the odd-numbered chapters. Following each, relevant real science is provided by his co-authors, Stewart and Cohen, in the even-numbered chapters (such as Chap. 10), but which of the two wrote which lines, is not designated.
Scientists, therefore, are responsible for their research, not only intellectually but also morally. This responsibility has become an important issue in many of today's sciences, but especially so in physics, in which the results of quantum mechanics and relativity theory have opened up two very different paths for physicists to pursue. They may lead us—to put it in extreme terms—to the Buddha or to the Bomb, and it is up to each of us to decide which path to take.
In The Turning Point: Science, Society, and the Rising Culture (1983), 87.
Shortly after electrons were discovered it was thought that atoms were like little solar systems, made up of a … nucleus and electrons, which went around in “orbits,” much like the planets … around the sun. If you think that’s the way atoms are, then you’re back in 1910.
In QED: The Strange Theory of Light and Matter (1985, 2006), 84.
Since religion intrinsically rejects empirical methods, there should never be any attempt to reconcile scientific theories with religion. [An infinitely old universe, always evolving may not be compatible with the Book of Genesis. However, religions such as Buddhism get along without having any explicit creation mythology and are in no way contradicted by a universe without a beginning or end.] Creatio ex nihilo, even as religious doctrine, only dates to around AD 200. The key is not to confuse myth and empirical results, or religion and science.
Quoted in Anthony L. Peratt, 'Dean of the Plasma Dissidents', Washington Times, supplement: The World and I (May 1988),196.
Since the beginning of physics, symmetry considerations have provided us with an extremely powerful and useful tool in our effort to understand nature. Gradually they have become the backbone of our theoretical formulation of physical laws.
Particle Physics and an Introduction to Field Theory (1981), 177.
Since the examination of consistency is a task that cannot be avoided, it appears necessary to axiomatize logic itself and to prove that number theory and set theory are only parts of logic. This method was prepared long ago (not least by Frege’s profound investigations); it has been most successfully explained by the acute mathematician and logician Russell. One could regard the completion of this magnificent Russellian enterprise of the axiomatization of logic as the crowning achievement of the work of axiomatization as a whole.
Address (11 Sep 1917), 'Axiomatisches Denken' delivered before the Swiss Mathematical Society in
Zürich. Translated by Ewald as 'Axiomatic Thought', (1918), in William Bragg Ewald, From Kant to Hilbert (1996), Vol. 2, 1113.
Since the mathematicians have invaded the theory of relativity, I do not understand it myself anymore.
Quoted as “Einstein once joked”, in Carl Seelig, Albert Einstein: A Documentary Biography (1956), 28.
Sir Edward has calculated that quick-growing Indian eucalyptus trees have a yield of nine and one-quarter tons of wood an acre a year. As the wood contains 0.8 per cent of the solar energy reaching the ground in the tropics in the form of heat, Sir Edward has suggested that in theory eucalyptus forests could provide a perpetual source of fuel. He has said that by rotational tree planting and felling, a forest of twenty kilometers square would enable a wood consuming power station to provide 10,000 kilowatts of power.
In 'British Hope to Use Green Trees of Jungles As Source of Power for New Steam Engine,' New York Times (27 Jun 1953), 6.
Sociobiology is not just any statement that biology, genetics, and evolutionary theory have something to do with human behavior. Sociobiology is a specific theory about the nature of genetic and evolutionary input into human behavior. It rests upon the view that natural selection is a virtually omnipotent architect, constructing organisms part by part as best solutions to problems of life in local environments. It fragments organisms into “traits,” explains their existence as a set of best solutions, and argues that each trait is a product of natural selection operating “for” the form or behavior in question. Applied to humans, it must view specific behaviors (not just general potentials) as adaptations built by natural selection and rooted in genetic determinants, for natural selection is a theory of genetic change. Thus, we are presented with unproved and unprovable speculations about the adaptive and genetic basis of specific human behaviors: why some (or all) people are aggressive, xenophobic, religious, acquisitive, or homosexual.
In Hen's Teeth and Horses Toes (1983, 2010), 242-243.
Some of my cousins who had the great advantage of University education used to tease me with arguments to prove that nothing has any existence except what we think of it. … These amusing mental acrobatics are all right to play with. They are perfectly harmless and perfectly useless. ... I always rested on the following argument. … We look up to the sky and see the sun. Our eyes are dazzled and our senses record the fact. So here is this great sun standing apparently on no better foundation than our physical senses. But happily there is a method, apart altogether from our physical senses, of testing the reality of the sun. It is by mathematics. By means of prolonged processes of mathematics, entirely separate from the senses, astronomers are able to calculate when an eclipse will occur. They predict by pure reason that a black spot will pass across the sun on a certain day. You go and look, and your sense of sight immediately tells you that their calculations are vindicated. So here you have the evidence of the senses reinforced by the entirely separate evidence of a vast independent process of mathematical reasoning. We have taken what is called in military map-making “a cross bearing.” When my metaphysical friends tell me that the data on which the astronomers made their calculations, were necessarily obtained originally through the evidence of the senses, I say, “no.” They might, in theory at any rate, be obtained by automatic calculating-machines set in motion by the light falling upon them without admixture of the human senses at any stage. When it is persisted that we should have to be told about the calculations and use our ears for that purpose, I reply that the mathematical process has a reality and virtue in itself, and that onie discovered it constitutes a new and independent factor. I am also at this point accustomed to reaffirm with emphasis my conviction that the sun is real, and also that it is hot— in fact hot as Hell, and that if the metaphysicians doubt it they should go there and see.
In My Early Life (1930).
Something unknown is doing we don’t know what—that is what our theory amounts to.
Expressing the quantum theory description of an electron has no familiar conception of a real form. In The Nature Of The Physical World (1928), 291.
Sometimes a hunch, right or wrong, is sufficient theory to lead to a useful observation.
In On the Management of Statistical Techniques for Quality and Productivity (1981), 86.
Son of a railroad engineer in the western United States, sometimes newspaper man in San Francisco, [Edward] Condon’s scientific career combined the abstract with the practical, theory and observation in a unique way.
From 'Edward Uhler Condon, A Personal Recollection', presented at the Fourth International Conference on Atomic Physics, published in the Proceedings of this conference by G. zu Pulitz et al. (eds.), Atomic Physics (1975), Vol. 4, as collected in Asim O. Barut, Halis Odabasi and Alwyn van der Merwe (eds.), Selected Popular Writings of E.U. Condon (1991), 1.
Sophus Lie, great comparative anatomist of geometric theories.
In Lectures on Science, Philosophy and Art (1908), 31.
Spacetime tells matter how to move; matter tells spacetime how to curve.
With co-author Kenneth William Ford Geons, Black Holes, and Quantum Foam: A Life in Physics (1998, 2010), 235. Adapted from his earlier book, co-authored with Charles W. Misner and Kip S. Thorne, Gravitation (1970, 1973), 5, in which one of the ideas in Einstein’s geometric theory of gravity was summarized as, “Space acts on matter, telling it how to move. In turn, matter reacts back on space, telling it how to curve”.
Statistics: the mathematical theory of ignorance.
From chapter title, 'The Mathematical Theory of Ignorance: The Statistical Approach to the Study of Man', in Mathematics in Western Culture (1953, 1964), 340.
Step by step we cross great eras in the development of thought: there is no sudden gigantic stride; a theory proceeds by slow evolution until it dominates or is destroyed.
In 'Theory of Phlogiston', The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science (Jan 1868), 35, 28-29.
String theory is 21 st century physics that fell accidentally into the 20th century.
In Nina L. Diamond, Voices of Truth (2000), 324.
String Theory is a part of twenty-first century physics that fell by chance into the twentieth century.
…...
String theory is revealing the deepest understanding of the Universe we have ever had.
…...
Students using astrophysical textbooks remain essentially ignorant of even the existence of plasma concepts, despite the fact that some of them have been known for half a century. The conclusion is that astrophysics is too important to be left in the hands of astrophysicists who have gotten their main knowledge from these textbooks. Earthbound and space telescope data must be treated by scientists who are familiar with laboratory and magnetospheric physics and circuit theory, and of course with modern plasma theory.
[Lamenting the traditional neglect of plasma physics]
[Lamenting the traditional neglect of plasma physics]
Quoted in Anthony L. Peratt, 'Dean of the Plasma Dissidents', Washington Times, supplement: The World and I (May 1988),197.
Such an atmosphere is un-American, the most un-American thing we have to contend with today. It is the climate of a totalitarian country in which scientists are expected to change their theories to match changes in the police state's propaganda line.
[Stinging rebuke of J. Parnell Thomas, Chairman, House Committee on Un-American activities, who had attacked Dr. Condon (1 Mar 1948) as a weak link in American atomic security.]
[Stinging rebuke of J. Parnell Thomas, Chairman, House Committee on Un-American activities, who had attacked Dr. Condon (1 Mar 1948) as a weak link in American atomic security.]
Opening address (13 Sep 1953) at the annual meeting of the American Association for the Advancement of Science where Condon would be elected as the new AAAS president. Obituary, New York Times (27 Mar 1974), 46.
Such is professional jealousy; a scientist will never show any kindness for a theory which he did not start himself.
In A Tramp Abroad (1880), 156.
Superstring theories provide a framework in which the force of gravity may be united with the other three forces in nature: the weak, electromagnetic and strong forces. Recent progress has shown that the most promising superstring theories follow from a single theory. For the last generation, physicists have studied five string theories and one close cousin. Recently it has become clear that these five or six theories are different limiting cases of one theory which, though still scarcely understood, is the candidate for superunification of the forces of nature.
His synopsis of lecture, University of Maryland Distinguished Lecture Series (2 Mar 1998), on web page.
Superstrings are totally lacking in empirical support, yet they offer an elegant theory with great explanatory power. I wish I could be around fifty years from now to know whether superstrings turn out to be a fruitful theory or whether they are just another blind alley in the search for a “theory of everything.”
As quoted in Kendrick Frazier, 'A Mind at Play: An Interview with Martin Gardner', Skeptical Inquirer (Mar/Apr 1998), 22, No. 2, 37.
Suppose a number of equal waves of water to move upon the surface of a stagnant lake, with a certain constant velocity, and to enter a narrow channel leading out of the lake. Suppose then another similar cause to have excited another equal series of waves, which arrive at the same time, with the first. Neither series of waves will destroy the other, but their effects will be combined: if they enter the channel in such a manner that the elevations of one series coincide with those of the other, they must together produce a series of greater joint elevations; but if the elevations of one series are so situated as to correspond to the depressions of the other, they must exactly fill up those depressions. And the surface of the water must remain smooth; at least I can discover no alternative, either from theory or from experiment.
A Reply to the Animadversions of the Edinburgh Reviewers on Some Papers Published in the Philosophical Transactions (1804), 17-8.
Surely there’s no problem with having several conflicting theories of evolution? Eventually the fittest will survive.
Comment by Pete Bibby following an article, 'Scientists Are Still Fleshing Out Darwin’s Theory of Evolution', The Guardian (1 Jul 2022).
Sylvester was incapable of reading mathematics in a purely receptive way. Apparently a subject either fired in his brain a train of active and restless thought, or it would not retain his attention at all. To a man of such a temperament, it would have been peculiarly helpful to live in an atmosphere in which his human associations would have supplied the stimulus which he could not find in mere reading. The great modern work in the theory of functions and in allied disciplines, he never became acquainted with …
What would have been the effect if, in the prime of his powers, he had been surrounded by the influences which prevail in Berlin or in Gottingen? It may be confidently taken for granted that he would have done splendid work in those domains of analysis, which have furnished the laurels of the great mathematicians of Germany and France in the second half of the present century.
What would have been the effect if, in the prime of his powers, he had been surrounded by the influences which prevail in Berlin or in Gottingen? It may be confidently taken for granted that he would have done splendid work in those domains of analysis, which have furnished the laurels of the great mathematicians of Germany and France in the second half of the present century.
In Address delivered at a memorial meeting at the Johns Hopkins University (2 May 1897), published in Bulletin of the American Mathematical Society (Jun 1897), 303. Also in Johns Hopkins University Circulars, 16 (1897), 54.
Take the rose—most people think it very beautiful: I don’t care for it at all. I prefer the cactus, for the simple reason that it has a more interesting personality. It has wonderfully adapted itself to its surroundings! It is the best illustration of the theory of evolution in plant life.
From George MacAdam, 'Steinmetz, Electricity’s Mastermind, Enters Politics', New York Times (2 Nov 1913), SM3.
That is the way of the scientist. He will spend thirty years in building up a mountain range of facts with the intent to prove a certain theory; then he is so happy with his achievement that as a rule he overlooks the main chief fact of all—that all his accumulation proves an entirely different thing.
'The Bee'. In What is Man? and Other Essays? (1917), 283.
That John Thomas Scopes, heretofore on the 24th day of April, 1925, in the county aforesaid, then and there unlawfully did wilfully teach in the public schools of Rhea County, Tennessee, which said public schools are supported in part, or in whole by the public school fund of the State, a certain theory or theories that denied the story of the divine creation of man as taught in the Bible, but did teach instead thereof, that man is descended from a lower order of animals, he, the said John Thomas Scopes, being at the time, and prior thereto, a teacher in the public schools of Rhea County, Tennessee, as foresaid, against the peace and dignity of the State.
Text of the indictment of John Scopes for teaching evolution. As given in John Scopes and James Presley, Center of the Storm: Memoirs of John T. Scopes (1967),
That mathematics “do not cultivate the power of generalization,”; … will be admitted by no person of competent knowledge, except in a very qualified sense. The generalizations of mathematics, are, no doubt, a different thing from the generalizations of physical science; but in the difficulty of seizing them, and the mental tension they require, they are no contemptible preparation for the most arduous efforts of the scientific mind. Even the fundamental notions of the higher mathematics, from those of the differential calculus upwards are products of a very high abstraction. … To perceive the mathematical laws common to the results of many mathematical operations, even in so simple a case as that of the binomial theorem, involves a vigorous exercise of the same faculty which gave us Kepler’s laws, and rose through those laws to the theory of universal gravitation. Every process of what has been called Universal Geometry—the great creation of Descartes and his successors, in which a single train of reasoning solves whole classes of problems at once, and others common to large groups of them—is a practical lesson in the management of wide generalizations, and abstraction of the points of agreement from those of difference among objects of great and confusing diversity, to which the purely inductive sciences cannot furnish many superior. Even so elementary an operation as that of abstracting from the particular configuration of the triangles or other figures, and the relative situation of the particular lines or points, in the diagram which aids the apprehension of a common geometrical demonstration, is a very useful, and far from being always an easy, exercise of the faculty of generalization so strangely imagined to have no place or part in the processes of mathematics.
In An Examination of Sir William Hamilton’s Philosophy (1878), 612-13.
That our knowledge only illuminates a small corner of the Universe, that it is incomplete, approximate, tentative and merely probable need not concert us. It is genuine nevertheless. Physical science stands as one of the great achievements of the human spirit.
Scientific Method: An Inquiry into the Character and Validy of Natural Law (1923), 201-202.
That the Anatomy of the Nerves yields more pleasant and profitable Speculations, than the Theory of any parts besides in the animated Body: for from hence the true and genuine Reasons are drawn of very many Actions and Passions that are wont to happen in our Body, which otherwise seem most difficult and unexplicable; and no less from this Fountain the hidden Causes of Diseases and their Symptoms, which commonly are ascribed to the Incantations of Witches, may be found out and clearly laid open. But as to our observations about the Nerves, from our following Discourse it will plainly appear, that I have not trod the paths or footsteps of others, nor repeated what hath been before told.
In Anatomy of the Brain and Nerves (1664), trans. Samuel Pordage (1681), reprinted in William Peindel (ed.), Thomas Willis: Anatomy of the Brain and Nerves (1965), Vol. 2, 125.
That theory is regarded by some enthusiastic opponents, as already on the threshold of the limbo appointed for exploded hypotheses.
In Bonney’s Appendix II, 'Summary of the Principal Contributions to the History of Coral Reefs Since The Year 1874' added to Charles Darwin, The Structure and Distribution of Coral Reefs (3rd Ed., 1889), 323-324. “That theory” is Darwin’s theory of the formation of coral reefs. Bonney summarizes the arguments of the “enthusiastic opponents” and then gives his reasons for disagreeing with those opponents.
That was the beginning, and the idea seemed so obvious to me and so elegant that I fell deeply in love with it. And, like falling in love with a woman, it is only possible if you do not know much about her, so you cannot see her faults. The faults will become apparent later, but after the love is strong enough to hold you to her. So, I was held to this theory, in spite of all difficulties, by my youthful enthusiasm.
Expressing how his work on quantum electrodynamics began with an original idea. In his Nobel Prize Lecture (11 Dec 1965), 'The Development of the Space-Time View of Quantum Electrodynamics'. Collected in Stig Lundqvist, Nobel Lectures: Physics, 1963-1970 (1998), 157.
The ‘mad idea’ which will lie at the basis of a future fundamental physical theory will come from a realization that physical meaning has some mathematical form not previously associated with reality. From this point of view the problem of the ‘mad idea’ is the problem of choosing, not of generating, the right idea. One should not understand that too literally. In the 1960s it was said (in a certain connection) that the most important discovery of recent years in physics was the complex numbers. The author [Yuri Manin] has something like that in mind.
Mathematics and Physics (1981), Foreward. Reprinted in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 90.
The actual evolution of mathematical theories proceeds by a process of induction strictly analogous to the method of induction employed in building up the physical sciences; observation, comparison, classification, trial, and generalisation are essential in both cases. Not only are special results, obtained independently of one another, frequently seen to be really included in some generalisation, but branches of the subject which have been developed quite independently of one another are sometimes found to have connections which enable them to be synthesised in one single body of doctrine. The essential nature of mathematical thought manifests itself in the discernment of fundamental identity in the mathematical aspects of what are superficially very different domains. A striking example of this species of immanent identity of mathematical form was exhibited by the discovery of that distinguished mathematician … Major MacMahon, that all possible Latin squares are capable of enumeration by the consideration of certain differential operators. Here we have a case in which an enumeration, which appears to be not amenable to direct treatment, can actually be carried out in a simple manner when the underlying identity of the operation is recognised with that involved in certain operations due to differential operators, the calculus of which belongs superficially to a wholly different region of thought from that relating to Latin squares.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 290.
The advance of science is not comparable to the changes of a city, where old edifices are pitilessly torn down to give place to new, but to the continuous evolution of zoologic types which develop ceaselessly and end by becoming unrecognisable to the common sight, but where an expert eye finds always traces of the prior work of the centuries past. One must not think then that the old-fashioned theories have been sterile and vain.
The Value of Science (1905), in The Foundations of Science: Science and Hypothesis, The Value of Science, Science and Method(1946), trans. by George Bruce Halsted, 208.
The aether: Invented by Isaac Newton, reinvented by James Clerk Maxwell. This is the stuff that fills up the empty space of the universe. Discredited and discarded by Einstein, the aether is now making a Nixonian comeback. It’s really the vacuum, but burdened by theoretical, ghostly particles.
In Leon Lederman and Dick Teresi, The God Particle: If the Universe is the Answer, What is the
Question (1993, 2006), xiii.
The aim of natural science is to obtain connections among phenomena. Theories, however, are like withered leaves, which drop off after having enabled the organism of science to breathe for a time.
As quoted in Philipp Frank, Modern Science and its Philosophy (1949), 62, which cites Die Geschichte und die Wurzel des Satzes von der Erhaltung der Arbeit (1871) and English translation, History and Root of the Principle of the Conservation of Energy (1911).
The alternative to the Big Bang is not, in my opinion, the steady state; it is instead the more general theory of continuous creation. Continuous creation can occur in bursts and episodes. These mini-bangs can produce all the wonderful element-building that Fred Hoyle discovered and contributed to cosmology. This kind of element and galaxy formation can take place within an unbounded, non-expanding universe. It will also satisfy precisely the Friedmann solutions of general relativity. It can account very well for all the facts the Big Bang explains—and also for those devastating, contradictory observations which the Big Bang must, at all costs, pretend are not there
In 'Letters: Wrangling Over the Bang', Science News (27 Jul 1991), 140, No. 4, 51. Also quoted in Roy C. Martin, Astronomy on Trial: A Devastating and Complete Repudiation of the Big Bang Fiasco (1999), Appendix I, 217.
The ancestors of the higher animals must be regarded as one-celled beings, similar to the Amœbæ which at the present day occur in our rivers, pools, and lakes. The incontrovertible fact that each human individual develops from an egg, which, in common with those of all animals, is a simple cell, most clearly proves that the most remote ancestors of man were primordial animals of this sort, of a form equivalent to a simple cell. When, therefore, the theory of the animal descent of man is condemned as a “horrible, shocking, and immoral” doctrine, tho unalterable fact, which can be proved at any moment under the microscope, that the human egg is a simple cell, which is in no way different to those of other mammals, must equally be pronounced “horrible, shocking, and immoral.”
Translated from his Ueber die Entstehung und den Stammbaum des Menschengeschlechts, (1873), Vol. 2, as an epigraph to Chap. 6, The Evolution of Man, (1879), Vol 1, 120-121.
The architect should be equipped with knowledge of many branches of study and varied kinds of learning, for it is by his judgement that all work done by the other arts is put to test. This knowledge is the child of practice and theory.
In De Architectura, Book 1, Chap 1, Sec. 1. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 3.
The argument advanced by the supporters of the theory of hereditary transmission does not furnish a satisfactory explanation of the cause of the inequalities and diversities of the universe.
Vedanta Philosophy: Five Lectures on Reincarnation (1902), 41.
The average English author [of mathematical texts] leaves one under the impression that he has made a bargain with his reader to put before him the truth, the greater part of the truth, and nothing but the truth; and that if he has put the facts of his subject into his book, however difficult it may be to unearth them, he has fulfilled his contract with his reader. This is a very much mistaken view, because effective teaching requires a great deal more than a bare recitation of facts, even if these are duly set forth in logical order—as in English books they often are not. The probable difficulties which will occur to the student, the objections which the intelligent student will naturally and necessarily raise to some statement of fact or theory—these things our authors seldom or never notice, and yet a recognition and anticipation of them by the author would be often of priceless value to the student. Again, a touch of humour (strange as the contention may seem) in mathematical works is not only possible with perfect propriety, but very helpful; and I could give instances of this even from the pure mathematics of Salmon and the physics of Clerk Maxwell.
In Perry, Teaching of Mathematics (1902), 59-61.
The basic ideas and simplest facts of set-theoretic topology are needed in the most diverse areas of mathematics; the concepts of topological and metric spaces, of compactness, the properties of continuous functions and the like are often indispensable.
As co-author with H. Hopf, Topologie I (1935), 23. As quoted, translated and cited in Reinhold Remmert, Theory of Complex Functions (1991), 9-10. From the original German, “Die Grundbegriffe und die einfachsten Tatsachen aus der mengentheoretischen Topologie braucht man in sehr verschiedenen Gebieten der Mathematik; die Begriffe des topologischen und des metrischen Raumes, der Kompaktheit, die Eigenschaften stetiger Abbildungen u. dgl. sind oft unentbehrlich.”
The basic thesis of gestalt theory might be formulated thus: there are contexts in which what is happening in the whole cannot be deduced from the characteristics of the separate pieces, but conversely; what happens to a part of the whole is, in clearcut cases, determined by the laws of the inner structure of its whole.
Lecture at the Kantgesellschaft (Kant Society), Berlin (17 Dec 1924), 'Über Gestalttheorie', as taken down in shorthand. Translated by N. Nairn-Allison in Social Research (1944), 11, 84.
The best class of scientific mind is the same as the best class of business mind. The great desideratum in either case is to know how much evidence is enough to warrant action. It is as unbusiness-like to want too much evidence before buying or selling as to be content with too little. The same kind of qualities are wanted in either case. The difference is that if the business man makes a mistake, he commonly has to suffer for it, whereas it is rarely that scientific blundering, so long as it is confined to theory, entails loss on the blunderer. On the contrary it very often brings him fame, money and a pension. Hence the business man, if he is a good one, will take greater care not to overdo or underdo things than the scientific man can reasonably be expected to take.
Samuel Butler, Henry Festing Jones (ed.), The Note-Books of Samuel Butler (1917), 217.
The Big Bang theory says nothing about what banged, why it banged, or what happened before it banged.
As quoted in Neil Swidey, 'Alan Guth: What Made the Big Bang Bang', Boston Globe (2 May 2014).
The Big Idea that had been developed in the seventeenth century ... is now known as the scientific method. It says that the way to proceed when investigating how the world works is to first carry out experiments and/or make observations of the natural world. Then, develop hypotheses to explain these observations, and (crucially) use the hypothesis to make predictions about the future outcome of future experiments and/or observations. After comparing the results of those new observations with the predictions of the hypotheses, discard those hypotheses which make false predictions, and retain (at least, for the time being) any hypothesis that makes accurate predictions, elevating it to the status of a theory. Note that a theory can never be proved right. The best that can be said is that it has passed all the tests applied so far.
In The Fellowship: the Story of a Revolution (2005), 275.
The catastrophist constructs theories, the uniformitarian demolishes them. The former adduces evidence of an Origin, the latter explains the evidence away.
Aphorism 110, 'Aphorisms Concerning Ideas', The Philosophy of the Inductive Sciences (1840), Vol. 1, xxxvi.
The chemist in America has in general been content with what I have called a loafer electron theory. He has imagined the electrons sitting around on dry goods boxes at every corner [viz. the cubic atom], ready to shake hands with, or hold on to similar loafer electrons in other atoms.
'Atomism in Modern Physics', Journal of the Chemical Society (1924), 1411.
The Chinese, who aspire to be thought an enlightened nation, to this day are ignorant of the circulation of the blood; and even in England the man who made that noble discovery lost all his practice in the consequence of his ingenuity; and Hume informs us that no physician in the United Kingdom who had attained the age of forty ever submitted to become a convert to Harvey’s theory, but went on preferring numpsimus to sumpsimus to the day of his death.
Reflection 352, in Lacon: or Many things in Few Words; Addressed to Those Who Think (1820), 164-165.
The Christians who engaged in infamous persecutions and shameful inquisitions were not evil men but misguided men. The churchmen who felt they had an edict from God to withstand the progress of science, whether in the form of a Copernican revolution or a Darwinian theory of natural selection, were not mischievous men but misinformed men. And so Christ’s words from the cross are written in sharp-edged terms across some of the most inexpressible tragedies of history: 'They know not what they do'.
'Love in Action', Strength To Love (1963, 1981), 43.
The classical example of a successful research programme is Newton’s gravitational theory: possibly the most successful research programme ever.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 48.
The complacent manner in which geologists have produced their theories has been extremely amusing; for often with knowledge (and that frequently inaccurate) not extending beyond a given province, they have described the formation of a world with all the detail and air of eye-witnesses. That much good ensues, and that the science is greatly advanced, by the collision of various theories, cannot be doubted. Each party is anxious to support opinions by facts. Thus, new countries are explored, and old districts re-examined; facts come to light that do not suit either party; new theories spring up; and, in the end, a greater insight into the real structure of the earth's surface is obtained.
Sections and Views Illustrative of Geological Phenomena (1830), iii.
The confirmation of theories relies on the compact adaption of their parts, by which, like those of an arch or dome, they mutually sustain each other, and form a coherent whole.
As quoted in J.F.W. Herschel, A Treatise on Astronomy (1833), 4. With the original Latin, “Theoriarum vires, arcta et quasi se mutuo sustinente partium adaptatione, quâ, quasi in orbem cohaerent, firmantur.”
The conflict of theories, leading, as it eventually must, to the survival of the fittest, is advantageous.
In Address (11 Dec 1895) as President of the Geological Society, 'The Origin of Hypotheses, illustrated by the Discussion of a Topographical Problem', printed as Presidential Address of Grove Karl Gilbert (1896), 4. Also collected in Science (1896), 3, 2.
The contents of this section will furnish a very striking illustration of the truth of a remark, which I have more than once made in my philosophical writings, and which can hardly be too often repeated, as it tends greatly to encourage philosophical investigations viz. That more is owing to what we call chance, that is, philosophically speaking, to the observation of events arising from unknown causes, than to any proper design, or pre-conceived theory in this business. This does not appear in the works of those who write synthetically upon these subjects; but would, I doubt not, appear very strikingly in those who are the most celebrated for their philosophical acumen, did they write analytically and ingenuously.
'On Dephlogisticated Air, and the Constitution of the Atmosphere', in The Discovery of Oxygen, Part I, Experiments by Joseph Priestley 1775 (Alembic Club Reprint, 1894), 5.
The contest [between the wave and particle theories of light] is something like one between a shark and a tiger, each is supreme in its own element but helpless in that of the other.
In Fison Memorial Lecture (7 May 1925) at Guy’s Hospital Medical School, London, published as The Structure of Light: The Fison Memorial Lecture 1925 (1925), 15.
The description of some of the experiments, which are communicated here, was completely worked out at my writing-table, before I had seen anything of the phenomena in question. After making the experiments on the following day, it was found that nothing in the description required to be altered. I do not mention this from feelings of pride, but in order to make clear the extraordinary ease and security with which the relations in question can be considered on the principles of Arrhenius' theory of free ions. Such facts speak more forcibly then any polemics for the value of this theory .
Philosophical Magazine (1891), 32, 156.
The development of mathematics is largely a natural, not a purely logical one: mathematicians are continually answering questions suggested by astronomers or physicists; many essential mathematical theories are but the reflex outgrowth from physical puzzles.
In 'The Teaching of the History of Science', The Scientific Monthly (Sep 1918), 194.
The development of mathematics toward greater precision has led, as is well known, to the formalization of large tracts of it, so that one can prove any theorem using nothing but a few mechanical rules... One might therefore conjecture that these axioms and rules of inference are sufficient to decide any mathematical question that can at all be formally expressed in these systems. It will be shown below that this is not the case, that on the contrary there are in the two systems mentioned relatively simple problems in the theory of integers that cannot be decided on the basis of the axioms.
'On Formally Undecidable Propositions of Principia Mathematica and Related Systems I' (193 1), in S. Feferman (ed.), Kurt Gödel Collected Works: Publications 1929-1936 (1986), Vol. I, 145.
The difference between myth and science is the difference between divine inspiration of “unaided reason” (as Bertrand Russell put it) on the one hand and theories developed in observational contact with the real world on the other. It is the difference between the belief in prophets and critical thinking, between Credo quia absurdum (I believe because it is absurd–Tertullian) and De omnibus est dubitandum (Everything should be questioned–Descartes). To try to write a grand cosmical drama leads necessarily to myth. To try to let knowledge substitute ignorance in increasingly large regions of space and time is science.
In 'Cosmology: Myth or Science?' Journal of Astrophysics and Astronomy (1984), 5, 79-98.
The difficulties connected with my criterion of demarcation (D) are important, but must not be exaggerated. It is vague, since it is a methodological rule, and since the demarcation between science and nonscience is vague. But it is more than sharp enough to make a distinction between many physical theories on the one hand, and metaphysical theories, such as psychoanalysis, or Marxism (in its present form), on the other. This is, of course, one of my main theses; and nobody who has not understood it can be said to have understood my theory.
The situation with Marxism is, incidentally, very different from that with psychoanalysis. Marxism was once a scientific theory: it predicted that capitalism would lead to increasing misery and, through a more or less mild revolution, to socialism; it predicted that this would happen first in the technically highest developed countries; and it predicted that the technical evolution of the 'means of production' would lead to social, political, and ideological developments, rather than the other way round.
But the (so-called) socialist revolution came first in one of the technically backward countries. And instead of the means of production producing a new ideology, it was Lenin's and Stalin's ideology that Russia must push forward with its industrialization ('Socialism is dictatorship of the proletariat plus electrification') which promoted the new development of the means of production.
Thus one might say that Marxism was once a science, but one which was refuted by some of the facts which happened to clash with its predictions (I have here mentioned just a few of these facts).
However, Marxism is no longer a science; for it broke the methodological rule that we must accept falsification, and it immunized itself against the most blatant refutations of its predictions. Ever since then, it can be described only as nonscience—as a metaphysical dream, if you like, married to a cruel reality.
Psychoanalysis is a very different case. It is an interesting psychological metaphysics (and no doubt there is some truth in it, as there is so often in metaphysical ideas), but it never was a science. There may be lots of people who are Freudian or Adlerian cases: Freud himself was clearly a Freudian case, and Adler an Adlerian case. But what prevents their theories from being scientific in the sense here described is, very simply, that they do not exclude any physically possible human behaviour. Whatever anybody may do is, in principle, explicable in Freudian or Adlerian terms. (Adler's break with Freud was more Adlerian than Freudian, but Freud never looked on it as a refutation of his theory.)
The point is very clear. Neither Freud nor Adler excludes any particular person's acting in any particular way, whatever the outward circumstances. Whether a man sacrificed his life to rescue a drowning, child (a case of sublimation) or whether he murdered the child by drowning him (a case of repression) could not possibly be predicted or excluded by Freud's theory; the theory was compatible with everything that could happen—even without any special immunization treatment.
Thus while Marxism became non-scientific by its adoption of an immunizing strategy, psychoanalysis was immune to start with, and remained so. In contrast, most physical theories are pretty free of immunizing tactics and highly falsifiable to start with. As a rule, they exclude an infinity of conceivable possibilities.
The situation with Marxism is, incidentally, very different from that with psychoanalysis. Marxism was once a scientific theory: it predicted that capitalism would lead to increasing misery and, through a more or less mild revolution, to socialism; it predicted that this would happen first in the technically highest developed countries; and it predicted that the technical evolution of the 'means of production' would lead to social, political, and ideological developments, rather than the other way round.
But the (so-called) socialist revolution came first in one of the technically backward countries. And instead of the means of production producing a new ideology, it was Lenin's and Stalin's ideology that Russia must push forward with its industrialization ('Socialism is dictatorship of the proletariat plus electrification') which promoted the new development of the means of production.
Thus one might say that Marxism was once a science, but one which was refuted by some of the facts which happened to clash with its predictions (I have here mentioned just a few of these facts).
However, Marxism is no longer a science; for it broke the methodological rule that we must accept falsification, and it immunized itself against the most blatant refutations of its predictions. Ever since then, it can be described only as nonscience—as a metaphysical dream, if you like, married to a cruel reality.
Psychoanalysis is a very different case. It is an interesting psychological metaphysics (and no doubt there is some truth in it, as there is so often in metaphysical ideas), but it never was a science. There may be lots of people who are Freudian or Adlerian cases: Freud himself was clearly a Freudian case, and Adler an Adlerian case. But what prevents their theories from being scientific in the sense here described is, very simply, that they do not exclude any physically possible human behaviour. Whatever anybody may do is, in principle, explicable in Freudian or Adlerian terms. (Adler's break with Freud was more Adlerian than Freudian, but Freud never looked on it as a refutation of his theory.)
The point is very clear. Neither Freud nor Adler excludes any particular person's acting in any particular way, whatever the outward circumstances. Whether a man sacrificed his life to rescue a drowning, child (a case of sublimation) or whether he murdered the child by drowning him (a case of repression) could not possibly be predicted or excluded by Freud's theory; the theory was compatible with everything that could happen—even without any special immunization treatment.
Thus while Marxism became non-scientific by its adoption of an immunizing strategy, psychoanalysis was immune to start with, and remained so. In contrast, most physical theories are pretty free of immunizing tactics and highly falsifiable to start with. As a rule, they exclude an infinity of conceivable possibilities.
'The Problem of Demarcation' (1974). Collected in David Miller (ed.) Popper Selections (1985), 127-128.
The dimmed outlines of phenomenal things all merge into one another unless we put on the focusing-glass of theory, and screw it up sometimes to one pitch of definition and sometimes to another, so as to see down into different depths through the great millstone of the world.
Are There Real Analogies in Nature?' (Feb 1856). Quoted in Lewis Campbell and William GarnettThe Life of James Clerk Maxwell (1882), 237.
The discovery of the conic sections, attributed to Plato, first threw open the higher species of form to the contemplation of geometers. But for this discovery, which was probably regarded in Plato’s tune and long after him, as the unprofitable amusement of a speculative brain, the whole course of practical philosophy of the present day, of the science of astronomy, of the theory of projectiles, of the art of navigation, might have run in a different channel; and the greatest discovery that has ever been made in the history of the world, the law of universal gravitation, with its innumerable direct and indirect consequences and applications to every department of human research and industry, might never to this hour have been elicited.
In 'A Probationary Lecture on Geometry, Collected Mathematical Papers, Vol. 2 (1908), 7.
The discovery of the telephone has made us acquainted with many strange phenomena. It has enabled us, amongst other things, to establish beyond a doubt the fact that electric currents actually traverse the earth’s crust. The theory that the earth acts as a great reservoir for electricity may be placed in the physicist's waste-paper basket, with phlogiston, the materiality of light, and other old-time hypotheses.
From Recent Progress in Telephony: British Association Report (1882). Excerpted in John Joseph Fahie, A History of Wireless Telegraphy (1902), 136.
The discovery which has been pointed to by theory is always one of profound interest and importance, but it is usually the close and crown of a long and fruitful period, whereas the discovery which comes as a puzzle and surprise usually marks a fresh epoch and opens a new chapter in science.
Becquerel Memorial Lecture, Journal of the Chemical Society, Transactions (1912), 101(2), 2005. Quoted by Simon Flexnor in 'The Scientific Career for Women', a commencement address at Bryn Mawr College (2 Jun 1921), The Scientific Monthly (Aug 1921), 13, 98.
The dispute between evolutionists and creation scientists offers textbook writers and teachers a wonderful opportunity to provide students with insights into the philosophy and methods of science. … What students really need to know is … how scientists judge the merit of a theory. Suppose students were taught the criteria of scientific theory evaluation and then were asked to apply these criteria … to the two theories in question. Wouldn’t such a task qualify as authentic science education? … I suspect that when these two theories are put side by side, and students are given the freedom to judge their merit as science, creation theory will fail ignominiously (although natural selection is far from faultless). … It is not only bad science to allow disputes over theory to go unexamined, but also bad education.
In Building a Bridge to the 18th Century: How the Past Can Improve Our Future (1999), 168.
The empirical basis of objective science has nothing “absolute” about it. Science does not rest upon solid bedrock. The bold structure of its theories rises, as it were, above a swamp. It is like a building erected on piles. The piles are driven down from above into the swamp, but not down to any natural or “given” base; and when we cease our attempts to drive our piles into a deeper layer, it is not because we have reached firm ground. We simply stop when we are satisfied that they are firm enough to carry the structure, at least for the time being.
The Logic of Scientific Discovery: Logik Der Forschung (1959, 2002), 94.
The engineer is concerned to travel from the abstract to the concrete. He begins with an idea and ends with an object. He journeys from theory to practice. The scientist’s job is the precise opposite. He explores nature with his telescopes or microscopes, or much more sophisticated techniques, and feeds into a computer what he finds or sees in an attempt to define mathematically its significance and relationships. He travels from the real to the symbolic, from the concrete to the abstract. The scientist and the engineer are the mirror image of each other.
In The Development of Design (1981), 19-20.
The entire annals of Observation probably do not elsewhere exhibit so extraordinary a verification of any theoretical conjecture adventured on by the human spirit!
[On the mathematical work by Urbain Le Verrier predicting the planet Neptune.]
[On the mathematical work by Urbain Le Verrier predicting the planet Neptune.]
In The Planet Neptune: An Exposition and History (1848), 90. The verification of the existence of the planet Neptune was made when Johan Galle found a star in an evening observation at the position predicted in the letter he received from Le Verrier earlier that same day.
The eventual goal of science is to provide a single theory that describes the whole universe.
…...
The evolution of higher and of lower forms of life is as well and as soundly established as the eternal hills. It has long since ceased to be a theory; it is a law of Nature as universal in living things as is the law of gravitation in material things and in the motions of the heavenly spheres.
Evolution and Religion in Education (1926), 118.
The existence of these patterns [fractals] challenges us to study forms that Euclid leaves aside as being formless, to investigate the morphology of the amorphous. Mathematicians have disdained this challenge, however, and have increasingly chosen to flee from nature by devising theories unrelated to anything we can see or feel.
The Fractal Geometry of Nature (1977, 1983), Introduction, xiii.
The experimental investigation by which Ampere established the law of the mechanical action between electric currents is one of the most brilliant achievements in science. The whole theory and experiment, seems as if it had leaped, full grown and full armed, from the brain of the 'Newton of Electricity'. It is perfect in form, and unassailable in accuracy, and it is summed up in a formula from which all the phenomena may be deduced, and which must always remain the cardinal formula of electro-dynamics.
A Treatise on Electricity and Magnetism (1873), Vol. 2, 162.
The experimental investigation by which Ampère established the law of the mechanical action between electric currents is one of the most brilliant achievements in science. The whole, theory and experiment, seems as if it had leaped, full grown and full armed, from the brain of the “Newton of Electricity”. It is perfect in form, and unassailable in accuracy, and it is summed up in a formula from which all the phenomena may be deduced, and which must always remain the cardinal formula of electro-dynamics.
In James Clerk Maxwell, Electricity and Magnetism (1881), Vol. 2, 163
The experimental verification of a theory concerning any natural phenomenon generally rests on the result of an integration.
Epigraph in Higher Mathematics for Students of Chemistry and Physics: With Special Reference to Practical Work (1902), Chap 4, 150.
The experimental verifications are not the basis of the theory, but its culmination.
As quoted in Philipp Frank, Modern Science and its Philosophy (1949), 16, which cites Théorie Physique; Son Objet—Son Structure (1906), 285. [Note: the experimentum crucis is a “crucial experiment” to be a conclusive test to decide between different hypotheses.]
The extracellular genesis of cells in animals seemed to me, ever since the publication of the cell theory [of Schwann], just as unlikely as the spontaneous generation of organisms. These doubts produced my observations on the multiplication of blood cells by division in bird and mammalian embryos and on the division of muscle bundles in frog larvae. Since then I have continued these observations in frog larvae, where it is possible to follow the history of tissues back to segmentation.
'Ueber extracellulare Eutstehung thierischer Zelleu und üüber Vermehrung derselben durch Theilung', Archiv für Anatomie, Physiologie und Wissenschaftliche Medicin (1852), 1, 49-50. Quoted in Erwin H. Ackerknecht, Rudolf Virchow: Doctor Statesman Anthropologist (1953), 83-4.
The fact that Science walks forward on two feet, namely theory and experiment, is nowhere better illustrated than in the two fields for slight contributions to which you have done me the great honour of awarding the the Nobel Prize in Physics for the year 1923. Sometimes it is one foot that is put forward first, sometimes the other, but continuous progress is only made by the use of both—by theorizing and then testing, or by finding new relations in the process of experimenting and then bringing the theoretical foot up and pushing it on beyond, and so on in unending alterations.
'The Electron and the Light-quant from the Experimental Point of View', Nobel Lecture (23 May 1924). In Nobel Lectures: Physics 1922-1941 (1998), 54.
The farther a mathematical theory is developed, the more harmoniously and uniformly does its construction proceed, and unsuspected relations are disclosed between hitherto separated branches of the science.
In 'Mathematical Problems', Lecture at the International Congress of Mathematics, Paris, (8 Aug 1900). Translated by Dr. Maby Winton Newson in Bulletin of the American Mathematical Society (1902), 8, 479.
The farther an experiment is from theory, the closer it is to the Nobel Prize.
Also French chemist, Irène Joliot-Curie (1897-1956)
Also French chemist, Irène Joliot-Curie (1897-1956)
Attributed to either.
The final results [of work on the theory of relativity] appear almost simple; any intelligent undergraduate can understand them without much trouble. But the years of searching in the dark for a truth that one feels, but cannot express; the intense effort and the alternations of confidence and misgiving, until one breaks through to clarity and understanding, are only known to him who has himself experienced them.
Concluding remark of George Gibson lecture at the University of Glasgow, 'The Origins of the General Theory of Relativity', (20 Jun 1933). Published by Glasgow University as The Origins of the General Theory of Relativity: Being the First Lecture on the George A. Gibson Foundation in the University of Glasgow, Delivered on June 20th, 1933 (1933), 11. Also quoted in 'No Hitching Posts' The Atlantic (1936), 157, 251.
The fundamental biological variant is DNA. That is why Mendel's definition of the gene as the unvarying bearer of hereditary traits, its chemical identification by Avery (confirmed by Hershey), and the elucidation by Watson and Crick of the structural basis of its replicative invariance, are without any doubt the most important discoveries ever made in biology. To this must be added the theory of natural selection, whose certainty and full significance were established only by those later theories.
In Jacques Monod and Austryn Wainhouse (trans.), Chance and Necessity: An Essay on the Natural Philosophy of Modern Biology (1971), 104.
The fundamental laws of the universe which correspond to the two fundamental theorems of the mechanical theory of heat.
1. The energy of the universe is constant.
2. The entropy of the universe tends to a maximum.
1. The energy of the universe is constant.
2. The entropy of the universe tends to a maximum.
The Mechanical Theory of Heat (1867), 365.
The future science of government should be called “la cybernétique”.
In Essai sur la philosophie des sciences, ou Exposition analytique d'une classification naturelle de toutes les connaissances humaines (1834). Coining the French word to mean “the art of governing,” from the Greek (Kybernetes = navigator or steersman), subsequently adopted as cybernetics by Norbert Weiner for the field of control and communication theory (see Oxford English Dictionary).
The generalized theory of relativity has furnished still more remarkable results. This considers not only uniform but also accelerated motion. In particular, it is based on the impossibility of distinguishing an acceleration from the gravitation or other force which produces it. Three consequences of the theory may be mentioned of which two have been confirmed while the third is still on trial: (1) It gives a correct explanation of the residual motion of forty-three seconds of arc per century of the perihelion of Mercury. (2) It predicts the deviation which a ray of light from a star should experience on passing near a large gravitating body, the sun, namely, 1".7. On Newton's corpuscular theory this should be only half as great. As a result of the measurements of the photographs of the eclipse of 1921 the number found was much nearer to the prediction of Einstein, and was inversely proportional to the distance from the center of the sun, in further confirmation of the theory. (3) The theory predicts a displacement of the solar spectral lines, and it seems that this prediction is also verified.
Studies in Optics (1927), 160-1.
The great object is to find the theory of the matter [of X-rays] before anyone else, for nearly every professor in Europe is now on the warpath.
In Letter to Mary Newton (28 Jan 1896), collected in Arthur Stewart Eve, Rutherford: Being the Life and Letters of the Rt. Hon. Lord Rutherford, O.M. (1939), 25.
The great revelation of the quantum theory was that features of discreteness were discovered in the Book of Nature, in a context in which anything other than continuity seemed to be absurd according to the views held until then.
What is Life? (1944), 48.

The greatest mathematicians, as Archimedes, Newton, and Gauss, always united theory and applications in equal measure.
In Elementarmathematik von höheren Standpunkte aus (1909), Bd. 2, 392.
The hallmark of scientific behaviour is a certain scepticism even towards one’s most cherished theories.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 1.
The history of acceptance of new theories frequently shows the following steps: At first the new idea is treated as pure nonsense, not worth looking at. Then comes a time when a multitude of contradictory objections are raised, such as: the new theory is too fancy, or merely a new terminology; it is not fruitful, or simply wrong. Finally a state is reached when everyone seems to claim that he had always followed this theory. This usually marks the last state before general acceptance.
In 'Field Theory and the Phase Space', collected in Melvin Herman Marx, Psychological Theory: Contemporary Readings (1951), 299.
The history of science should not be an instrument to defend any kind of social or philosophic theory; it should be used only for its own purpose, to illustrate impartially the working of reason against unreason, the gradual unfolding of truth, in all its forms, whether pleasant or unpleasant, useful of useless, welcome or unwelcome.
In A History of Science: Volume 1: Ancient Science Through the Golden Age of Greece (1952), xiv.
The history of science shows so many examples of the 'irrational' notions and theories of to-day becoming the 'rational' notions and theories of to-morrow, that it seems largely a matter of being accustomed to them whether they are considered rational or not, natural or not.
Natural Law and Divine Miracle: The Principle of Uniformity in Geology, Biology and Theology (1963),167.
The history of thought should warn us against concluding that because the scientific theory of the world is the best that has yet been formulated, it is necessarily complete and final. We must remember that at bottom the generalizations of science or, in common parlance, the laws of nature are merely hypotheses devised to explain that ever-shifting phantasmagoria of thought which we dignify with the high-sounding names of the world and the universe. In the last analysis magic, religion, and science are nothing but theories of thought.
In The Golden Bough: A Study in Magic and Religion (1890, 1900), Vol. 3, 460.
The idea that our natural resources were inexhaustible still obtained, and there was as yet no real knowledge of their extent and condition. The relation of the conservation of natural resources to the problems of National welfare and National efficiency had not yet dawned on the public mind. The reclamation of arid public lands in the West was still a matter for private enterprise alone; and our magnificent river system, with its superb possibilities for public usefulness, was dealt with by the National Government not as a unit, but as a disconnected series of pork-barrel problems, whose only real interest was in their effect on the re-election or defeat of a Congressman here and there —a theory which, I regret to say, still obtains.
The Works of Theodore Roosevelt. Vol. 20: Theodore Roosevelt, An Autobiography (1926), 386.
The idealistic tinge in my conception of the physical world arose out of mathematical researches on the relativity theory. In so far as I had any earlier philosophical views, they were of an entirely different complexion.
From the beginning I have been doubtful whether it was desirable for a scientist to venture so far into extra-scientific territory. The primary justification for such an expedition is that it may afford a better view of his own scientific domain.
From the beginning I have been doubtful whether it was desirable for a scientist to venture so far into extra-scientific territory. The primary justification for such an expedition is that it may afford a better view of his own scientific domain.
From 'Introduction', The Nature of the Physical World (1928), vi.
The importance of C.F. Gauss for the development of modern physical theory and especially for the mathematical fundament of the theory of relativity is overwhelming indeed; also his achievement of the system of absolute measurement in the field of electromagnetism. In my opinion it is impossible to achieve a coherent objective picture of the world on the basis of concepts which are taken more or less from inner psychological experience.
Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 350.
The importance of group theory was emphasized very recently when some physicists using group theory predicted the existence of a particle that had never been observed before, and described the properties it should have. Later experiments proved that this particle really exists and has those properties.
Groups in the New Mathematics (1967), 7. Quoted in Rosemary Schmalz, Out of the Mouths of Mathematicians: A Quotation Book for Philomaths (1993), 42.
The incomplete knowledge of a system must be an essential part of every formulation in quantum theory. Quantum theoretical laws must be of a statistical kind. To give an example: we know that the radium atom emits alpha-radiation. Quantum theory can give us an indication of the probability that the alpha-particle will leave the nucleus in unit time, but it cannot predict at what precise point in time the emission will occur, for this is uncertain in principle.
The Physicist's Conception of Nature (1958), 41.
The initial stage, the act of conceiving or inventing a theory, seems to me neither to call for logical analysis nor to be susceptible of it. (1959)
In The Logic of Scientific Discovery: Logik Der Forschung (1959, 2002), 7.
The intensity and quantity of polemical literature on scientific problems frequently varies inversely as the number of direct observations on which the discussions are based: the number and variety of theories concerning a subject thus often form a coefficient of our ignorance. Beyond the superficial observations, direct and indirect, made by geologists, not extending below about one two-hundredth of the Earth's radius, we have to trust to the deductions of mathematicians for our ideas regarding the interior of the Earth; and they have provided us successively with every permutation and combination possible of the three physical states of matter—solid, liquid, and gaseous.
'Address delivered by the President of Section [Geology] at Sydney (Friday, Aug 21), Report of the Eighty-Fourth Meeting of the British Association for the Advancement of Science: Australia 1914, 1915, 345.
The intricate edifice of verifiable fact and tested theory that has been patiently created in just a brief few hundred years is man’s most solid achievement on earth.
In Francis Bello, Lawrence Lessing and George A.W. Boehm, Great American Scientists (1960, 1961), 117.
The job of theorists, especially in biology, is to suggest new experiments. A good theory makes not only predictions, but surprising predictions that then turn out to be true. (If its predictions appear obvious to experimentalists, why would they need a theory?)
In What Mad Pursuit: A Personal View of Scientific Discovery (1988), 142.
The kinetic concept of motion in classical theory will have to undergo profound modifications. (That is why I also avoided the term “orbit” in my paper throughout.) … We must not bind the atoms in the chains of our prejudices—to which, in my opinion, also belongs the assumption that electron orbits exist in the sense of ordinary mechanics—but we must, on the contrary, adapt our concepts to experience.
Letter to Niels Bohr (12 Dec 1924), in K. von Meyenn (ed.), Wolfgang Pauli - Wissenschaftliche Korrespondenz (1979), Vol. 1, 188. Quoted and cited in Daniel Greenberger, Klaus Hentschel and Friedel Weinert, Compendium of Quantum Physics: Concepts, Experiments, History and Philosophy (2009), 615.
The law that entropy always increases—the Second Law of Thermodynamics—holds, I think, the supreme position among the laws of Nature. If someone points out to you that your pet theory of the universe is in disagreement with Maxwell’s equations—then so much the worse for Maxwell’s equations. If it is found to be contradicted by observation—well these experimentalists do bungle things sometimes. But if your theory is found to be against the second law of thermodynamics I can give you no hope; there is nothing for it but to collapse in deepest humiliation.
Gifford Lectures (1927), The Nature of the Physical World (1928), 74.
The laws of science are the permanent contributions to knowledge—the individual pieces that are fitted together in an attempt to form a picture of the physical universe in action. As the pieces
fall into place, we often catch glimpses of emerging patterns, called theories; they set us searching for the missing pieces that will fill in the gaps and complete the patterns. These theories, these provisional interpretations of the data in hand, are mere working hypotheses, and they are treated with scant respect until they can be tested by new pieces of the puzzle.
In Commencement Address, California Institute of Technology (10 Jun 1938), 'Experiment and Experience'. Collected in abridged form in The Huntington Library Quarterly (Apr 1939), 2, No. 3, 244.
The layman, taught to revere scientists for their absolute respect for the observed facts, and for the judiciously detached and purely provisional manner in which they hold scientific theories (always ready to abandon a theory at the sight of any contradictory evidence) might well have thought that, at [Dayton C.] Miller's announcement of this overwhelming evidence of a “positive effect” [indicating that the speed of light is not independent from the motion of the observer, as Einstein's theory of relativity demands] in his presidential address to the American Physical Society on December 29th, 1925, his audience would have instantly abandoned the theory of relativity. Or, at the very least, that scientists—wont to look down from the pinnacle of their intellectual humility upon the rest of dogmatic mankind—might suspend judgment in this matter until Miller's results could be accounted for without impairing the theory of relativity. But no: by that time they had so well closed their minds to any suggestion which threatened the new rationality achieved by Einstein's world-picture, that it was almost impossible for them to think again in different terms. Little attention was paid to the experiments, the evidence being set aside in the hope that it would one day turn out to be wrong.
Personal Knowledge: Towards a Post-Critical Philosophy (1958, 1998), 13. Miller had earlier presented his evidence against the validity of the relativity theory at the annual meeting, 28 Apr 1925, of the National Academy of Sciences. Miller believed he had, by a much-refined and improved repetition of the so-called Michelson-Morley experiment, shown that there is a definite and measurable motion of the earth through the ether. In 1955, a paper by R.S. Shankland, et al., in Rev. Modern Phys. (1955), 27, 167, concluded that statistical fluctuations and temperature effects in the data had simulated what Miller had taken to be he apparent ether drift.
The lessons of science should be experimental also. The sight of a planet through a telescope is worth all the course on astronomy; the shock of the electric spark in the elbow outvalues all theories; the taste of the nitrous oxide, the firing of an artificial volcano, are better than volumes of chemistry.
The Prose Works of Ralph Waldo Emerson (1870), 552.
The longing to behold this pre-established harmony [of phenomena and theoretical principles] is the source of the inexhaustible patience and perseverance with which Planck has devoted himself ... The state of mind which enables a man to do work of this kind is akin to that of the religious worshiper or the lover; the daily effort comes from no deliberate intention or program, but straight from the heart.
Address (1918) for Max Planck's 60th birthday, at Physical Society, Berlin, 'Principles of Research' in Essays in Science (1934), 4-5.
The loveliest theories are being overthrown by these damned experiments; it's no fun being a chemist anymore.
Liebig to Berzelius, 22 Jul 1834. Quoted in J. Carriere (ed.), Berzelius und Liebig: ihre Briefe (1898), 94. Trans. W. H. Brock.
The majority of mathematical truths now possessed by us presuppose the intellectual toil of many centuries. A mathematician, therefore, who wishes today to acquire a thorough understanding of modern research in this department, must think over again in quickened tempo the mathematical labors of several centuries. This constant dependence of new truths on old ones stamps mathematics as a science of uncommon exclusiveness and renders it generally impossible to lay open to uninitiated readers a speedy path to the apprehension of the higher mathematical truths. For this reason, too, the theories and results of mathematics are rarely adapted for popular presentation … This same inaccessibility of mathematics, although it secures for it a lofty and aristocratic place among the sciences, also renders it odious to those who have never learned it, and who dread the great labor involved in acquiring an understanding of the questions of modern mathematics. Neither in the languages nor in the natural sciences are the investigations and results so closely interdependent as to make it impossible to acquaint the uninitiated student with single branches or with particular results of these sciences, without causing him to go through a long course of preliminary study.
In Mathematical Essays and Recreations (1898), 32.
The mass starts into a million suns;
Earths round each sun with quick explosions burst,
And second planets issue from the first.
[The first concept of a 'big bang' theory of the universe.]
Earths round each sun with quick explosions burst,
And second planets issue from the first.
[The first concept of a 'big bang' theory of the universe.]
The Botanic Garden (1789-1791, 1805), 12.
The mathematical framework of quantum theory has passed countless successful tests and is now universally accepted as a consistent and accurate description of all atomic phenomena. The verbal interpretation, on the other hand – i.e., the metaphysics of quantum theory – is on far less solid ground. In fact, in more than forty years physicists have not been able to provide a clear metaphysical model.
In The Tao of Physics (1975), 132.
The mathematical framework of quantum theory has passed countless successful tests and is now universally accepted as a consistent and accurate description of all atomic phenomena. The verbal interpretation, on the other hand, i.e. the metaphysics of quantum physics, is on far less solid ground. In fact, in more than forty years physicists have not been able to provide a clear metaphysical model.
In The Tao of Physics: An Exploration of the Parallels Between Modern Physics (1975), 132.
The mathematically formulated laws of quantum theory show clearly that our ordinary intuitive concepts cannot be unambiguously applied to the smallest particles. All the words or concepts we use to describe ordinary physical objects, such as position, velocity, color, size, and so on, become indefinite and problematic if we try to use them of elementary particles.
In Across the Frontiers (1974), 114.
The mathematicians have been very much absorbed with finding the general solution of algebraic equations, and several of them have tried to prove the impossibility of it. However, if I am not mistaken, they have not as yet succeeded. I therefore dare hope that the mathematicians will receive this memoir with good will, for its purpose is to fill this gap in the theory of algebraic equations.
Opening of Memoir on Algebraic Equations, Proving the Impossibility of a Solution of the General Equation of the Fifth Degree. The paper was originally published (1824) in French, as a pamphlet, in Oslo. Collected in Œuvres Complètes (1881), Vol. 1, 28. Translation by W.H. Langdon collected in David Eugene Smith, A Source Book in Mathematics (2012), 261. In this work, he showed why—despite two centuries of efforts by mathematicians—solving equations of the fifth degree would remain futile. The insights from this paper led to the modern theory of equations.
The mathematics involved in string theory … in subtlety and sophistication vastly exceeds previous uses of mathematics in physical theories. … String theory has led to a whole host of amazing results in mathematics in areas that seem far removed from physics. To many this indicates that string theory must be on the right track.
In Book Review 'Pulling the Strings,' of Lawrence Krauss's Hiding in the Mirror: The Mysterious Lure of Extra Dimensions, from Plato to String Theory and Beyond in Nature (22 Dec 2005), 438, 1082.
The mathematics of the twenty-first century may be very different from our own; perhaps the schoolboy will begin algebra with the theory of substitution groups, as he might now but for inherited habits.
From Address before the New York Mathematical Society, Bulletin of the New York Mathematical Society (1893), 3, 107. As cited in G.A. Miller, 'Appreciative Remarks on the Theory of Groups', The American Mathematical Monthly (1903), 10, No. 4, 89.
https://books.google.com/books?id=hkM0AQAAMAAJ
1903
The maxim is, that whatever can be affirmed (or denied) of a class, may be affirmed (or denied) of everything included in the class. This axiom, supposed to be the basis of the syllogistic theory, is termed by logicians the dictum de omni et nullo.
A System of Logic, Ratiocinative and Inductive (1858), 117.
The method of inquiry which all our ingenious Theorists of the Earth have pursued is certainly erroneous. They first form an hypothesis to solve the phenomena, but in fact the Phenomena are always used as a prop to the hypothesis.
Instead therefore of attempting to cut the gordian knot by Hypothetical analysis, we shall follow the synthetic method of inquiry and content ourselves with endeavouring to establish facts rather than attempt solutions and try by experiments how far that method may leave us thro' the mazes of this subject
Instead therefore of attempting to cut the gordian knot by Hypothetical analysis, we shall follow the synthetic method of inquiry and content ourselves with endeavouring to establish facts rather than attempt solutions and try by experiments how far that method may leave us thro' the mazes of this subject
Introduction to his lecture course. In Robert Jameson, edited by H. W. Scott, Lectures on Geology, (1966), 27. In Patrick Wyse Jackson, Four Centuries of Geological Travel (2007), 33.
The method of science depends on our attempts to describe the world with simple theories: theories that are complex may become untestable, even if they happen to be true. Science may be described as the art of systematic over-simplification—the art of discerning what we may with advantage omit.
Karl Raimund Popper and William Warren Bartley (ed.), The Open Universe: an Argument for Indeterminism (1991), 44.
by Karl Raimund Popper, William Warren Bartley - Science - 1991
The methods of science may be described as the discovery of laws, the explanation of laws by theories, and the testing of theories by new observations. A good analogy is that of the jigsaw puzzle, for which the laws are the individual pieces, the theories local patterns suggested by a few pieces, and the tests the completion of these patterns with pieces previously unconsidered. … The scientist likes to fancy … that sufficient pieces may be assembled to indicate eventually the entire pattern of the puzzle, and thus to reveal the structure and behavior of the physical universe as it appears to man.
The Nature of Science and Other Lectures (1954), 11.
The mind may, as it appears to me, divide science into three parts. The first comprises the most theoretical principles, and those more abstract notions whose application is either unknown or very remote. The second is composed of those general truths which still belong to pure theory, but lead, nevertheless, by a straight and short road to practical results. Methods of application and means of execution make up the third. Each of these different portions of science may be separately cultivated, although reason and experience show that none of them can prosper long, if it be absolutely cut off from the two others.
In Alexis de Tocqueville and Henry Reeve (trans.), Democracy in America (1840, 1899), Vol. 2, 42-43.
The modern physicist is a quantum theorist on Monday, Wednesday, and Friday and a student of gravitational relativity theory on Tuesday, Thursday, and Saturday. On Sunday he is neither, but is praying to his God that someone, preferably himself, will find the reconciliation between the two views.
In I Am a Mathematician, the Later Life of a Prodigy (1956), 109.
The Modern Theory of Functions—that stateliest of all the pure creations of the human intellect.
In Lectures on Science, Philosophy and Art (1908), 16.
The modern, and to my mind true, theory is that mathematics is the abstract form of the natural sciences; and that it is valuable as a training of the reasoning powers not because it is abstract, but because it is a representation of actual things.
From 'Introduction', Mathematical Teaching and its Modern Methods (1886), 9-10.
The moment a person forms a theory, his imagination sees, in every object, only the traits which favor that theory.
In Letter (20 Sep 1787) to Charles Thompson. Collected in Thomas Jefferson Randolph, Memoirs, Correspondence, and Private Papers of Thomas Jefferson (1829), Vol. 2, 223.
The moment one has offered an original explanation for a phenomenon which seems satisfactory, that moment affection for his intellectual child springs into existence, and as the explanation grows into a definite theory his parental affections cluster about his offspring and it grows more and more dear to him. ... There springs up also unwittingly a pressing of the theory to make it fit the facts and a pressing of the facts to make them fit the theory... To avoid this grave danger, the method of multiple working hypotheses is urged. It differs from the simple working hypothesis in that it distributes the effort and divides the affections... In developing the multiple hypotheses, the effort is to bring up into view every rational exploration of the phenomenon in hand and to develop every tenable hypothesis relative to its nature, cause or origin, and to give to all of these as impartially as possible a working form and a due place in the investigation. The investigator thus becomes the parent of a family of hypotheses; and by his parental relations to all is morally forbidden to fasten his affections unduly upon anyone. ... Each hypothesis suggests its own criteria, its own method of proof, its own method of developing the truth, and if a group of hypotheses encompass the subject on all sides, the total outcome of means and of methods is full and rich.
'Studies for Students. The Method of Multiple Working Hypotheses', Journal of Geology (1897), 5, 840-6.
The moment you encounter string theory and realise that almost all of the major developments in physics over the last hundred years emerge—and emerge with such elegance—from such a simple starting point, you realise that this incredibly compelling theory is in a class of its own.
…...
The more experiences and experiments accumulate in the exploration of nature, the more precarious the theories become. But it is not always good to discard them immediately on this account. For every hypothesis which once was sound was useful for thinking of previous phenomena in the proper interrelations and for keeping them in context. We ought to set down contradictory experiences separately, until enough have accumulated to make building a new structure worthwhile.
Lichtenberg: Aphorisms & Letters (1969), 61.
The more I meditate on the principles of the theory of functions—and I do this unremittingly the stronger becomes my conviction that the foundations upon which these must be built are the truths of Algebra…
From Letter (3 Oct 1875), to Professor Schwartz. As quoted and translated in Ernst Hairer and Gerhard Wanner, Analysis by Its History (2008), 177. From the original German in Mathematische Werke (1875, 1895), Vol. 2, 235, “Je mehr ich über die Principien der Functionentheorie nachdenke—und ich thue dies unablässig—, um so fester wird meine Überzeugung, dass diese auf dem Fundamente algebraischer Wahrheiten aufgebaut werden muss…”
The more I think about the physical portion of Schrödinger’s theory, the more repulsive I find it…. What Schrödinger writes about the visualizability of his theory “is probably not quite right”; in other words it’s crap.
Letter to Wolfgang Pauli (8 Jun 1926). 17. In a subsequent letter to Pauli, he referred to Dirac’s theory as “learned crap.”
The more success the quantum theory has, the sillier it looks.
The more success the quantum theory has, the sillier it looks.
The most dangerous tendency of the modern world is the way in which bogus theories are given the force of dogma.
The Lord of History (1958), 103.
The most practical solution is a good theory.
Epigraph, without citation, in Eberhard Zeidler, Applied Functional Analysis: main principles and their applications (1995), 1.
The new chemistry indeed has given us a new principle of the generation of rain, by proving water to be a composition of different gases, and has aided our theory of meteoric lights.
Letter (5 Sep 1822) to George F. Hopkins. Collected in The Writings of Thomas Jefferson (1854), Vol. 7, 260.
The Newton of drift theory has not yet appeared. His absence need cause no anxiety; the theory is still young and still often treated with suspicion. In the long run, one cannot blame a theoretician for hesitating to spend time and trouble on explaining a law about whose validity no unanimity prevails.
In The Origins of Continents and Oceans (4th ed. 1929), trans. John Biram (1966), 167.
The night before Easter Sunday of that year (1920) I awoke, turned on the light, and jotted down a few notes on a tiny slip of thin paper. Then I fell asleep again. It occurred to me at six o’clock in the morning that during the night I had written down something most important, but I was unable to decipher the scrawl. The next night, at three o’clock, the idea returned. It was the design of an experiment to determine whether the hypothesis of chemical transmission that I had uttered seventeen years ago was correct. I got up immediately, went to the laboratory, and performed a simple experiment on a frog heart according to the nocturnal design. I have to describe this experiment briefly since its results became the foundation of the theory of chemical transmission of the nervous impulse. The hearts of two frogs were isolated, the first with its nerves, the second without. Both hearts were attached to Straub cannulas filled with a little Ringer solution. The vagus nerve of the first heart was stimulated for a few minutes. Then the Ringer solution that had been in the first heart during the stimulation of the vagus was transferred to the second heart. It slowed and its beats diminished just as if its vagus had been stimulated. Similarly, when the accelerator nerve was stimulated and the Ringer from this period transferred, the second heart speeded up and its beats increased. These results unequivocally proved that the nerves do not influence the heart directly but liberate from their terminals specific chemical substances which, in their turn, cause the well-known modifications of the function of the heart characteristic of the stimulation of its nerves.
'An Autobiographic Sketch', Perspectives in Biology and Medicine (1960), 4, 17.
The old scientific ideal of episteme — of absolutely certain, demonstrable knowledge — has proved to be an idol. The demand for scientific objectivity makes it inevitable that every scientific statement must remain tentative for ever. (1959)
The Logic of Scientific Discovery: Logik Der Forschung (1959, 2002), 280.
The only sure foundations of medicine are, an intimate knowledge of the human body, and observation on the effects of medicinal substances on that. The anatomical and clinical schools, therefore, are those in which the young physician should be formed. If he enters with innocence that of the theory of medicine, it is scarcely possible he should come out untainted with error. His mind must be strong indeed, if, rising above juvenile credulity, it can maintain a wise infidelity against the authority of his instructors, and the bewitching delusions of their theories.
In letter to Caspar Wistar (21 Jun 1807), collected in Thomas Jefferson Randolph (ed.), Memoir, Correspondence, And Miscellanies, From The Papers Of Thomas Jefferson (1829), Vol. 4, 93.
The owner of the means of production is in a position to purchase the labor power of the worker. By using the means of production, the worker produces new goods which become the property of the capitalist. The essential point about this process is the relation between what the worker produces and what he is paid, both measured in terms of real value. In so far as the labor contract is free what the worker receives is determined not by the real value of the goods he produces, but by his minimum needs and by the capitalists’ requirements for labor power in relation to the number of workers competing for jobs. It is important to understand that even in theory the payment of the worker is not determined by the value of his product.
…...
The phenomena in these exhausted tubes reveal to physical science a new world—a world where matter may exist in a fourth state, where the corpuscular theory of light may be true, and where light does not always move in straight lines, but where we can never enter, and with which we must be content to observe and experiment from the outside.
'On the Illumination of Lines of Molecular Pressure and the Trajectory of Molecules', Philosophical Transactions 1879, 170, 164.
The philosopher of science is not much interested in the thought processes which lead to scientific discoveries; he looks for a logical analysis of the completed theory, including the establishing its validity. That is, he is not interested in the context of discovery, but in the context of justification.
'The Philosophical Significance of the Theory of Relativity' (1938). Collected in P.A. Schillp (ed.). Albert Einstein: Philosopher-Scientist (1949, 1970), 292. Cited in G. Holton, Thematic Origins of Scientific Thought (1973), 7.
The philosopher of science is not much interested in the thought processes which lead to scientific discoveries; he looks for a logical analysis of the completed theory, including the relationships establishing its validity. That is, he is not interested in the context of discovery, but in the context of justification.
In'The Philosophical Significance of the Theory of Relativity' (1949), collected in P.A. Schilpp (ed), Albert Einstein: Philosopher-Scientist (1969), 292. As quoted and cited in Stanley Goldberg, Understanding Relativity: Origin and Impact of a Scientific Revolution (1984, 2013), 306.
The physicist cannot simply surrender to the philosopher the critical contemplation of the theoretical foundations for he himself knows best and feels most surely where the shoe pinches. … he must try to make clear in his own mind just how far the concepts which he uses are justified … The whole of science is nothing more than a refinement of everyday thinking. It is for this reason that the critical thinking of the physicist cannot possibly be restricted by the examination of the concepts of his own specific field. He cannot proceed without considering critically a much more difficult problem, the problem of analyzing the nature of everyday thinking.
‘Physics and Reality’, Franklin Institute Journal (Mar 1936). Collected in Out of My Later Years (1950), 59.
The physiological combustion theory takes as its starting point the fundamental principle that the amount of heat that arises from the combustion of a given substance is an invariable quantity–i.e., one independent of the circumstances accompanying the combustion–from which it is more specifically concluded that the chemical effect of the combustible materials undergoes no quantitative change even as a result of the vital process, or that the living organism, with all its mysteries and marvels, is not capable of generating heat out of nothing.
Bemerkungen über das mechanische Aequivalent der Wärme [Remarks on the Mechanical Equivalent of Heat] (1851), 17-9. Trans. Kenneth L. Caneva, Robert Mayer and the Conservation of Energy (1993), 240.
The powerful notion of entropy, which comes from a very special branch of physics … is certainly useful in the study of communication and quite helpful when applied in the theory of language.
From 'The Growth of Science and the Structure of Culture', Daedalus (Winter 1958), 87, No. 1, 67.
The practice of that which is ethically best—what we call goodness or virtue—involves a course of conduct which, in all respects, is opposed to that which leads to success in the cosmic struggle for existence. In place of ruthless self-assertion it demands self-restraint; in place of thrusting aside, or treading down, all competitors, it requires that the individual shall not merely respect, but shall help his fellows… It repudiates the gladiatorial theory of existence… Laws and moral precepts are directed to the end of curbing the cosmic process.
'Evolution and Ethics' (1893). In Collected Essays (1894), Vol. 9, 81-2.
The pre-Darwinian age had come to be regarded as a Dark Age in which men still believed that the book of Genesis was a standard scientific treatise, and that the only additions to it were Galileo’s demonstration of Leonardo da Vinci’s simple remark that the earth is a moon of the sun, Newton’s theory of gravitation, Sir Humphry Davy's invention of the safety-lamp, the discovery of electricity, the application of steam to industrial purposes, and the penny post.
Back to Methuselah: a Metabiological Pentateuch (1921), viii.
The present gigantic development of the mathematical faculty is wholly unexplained by the theory of natural selection, and must be due to some altogether distinct cause.
In Darwinism, chap. 15.
The present knowledge of the biochemical constitution of the cell was achieved largely by the use of destructive methods. Trained in the tradition of the theory of solutions, many a biochemist tends, even today, to regard the cell as a “bag of enzymes”. However, everyone realizes now that the biochemical processes studied in vitro may have only a remote resemblance to the events actually occurring in the living cell.
Nucleo-cytoplasmic Relations in Micro-Organisms: Their Bearing on Cell Heredity and Differentiation (1953), 108.
The present state of atomic theory is characterized by the fact that we not only believe the existence of atoms to be proved beyond a doubt, but also we even believe that we have an intimate knowledge of the constituents of the individual atoms.
'The structure of the atom', Nobel Lecture, 11 December 1922. In Nobel Lectures: Physics 1922-1941 (1998), 5.
The present state of the system of nature is evidently a consequence of what it was in the preceding moment, and if we conceive of an intelligence that at a given instant comprehends all the relations of the entities of this universe, it could state the respective position, motions, and general affects of all these entities at any time in the past or future. Physical astronomy, the branch of knowledge that does the greatest honor to the human mind, gives us an idea, albeit imperfect, of what such an intelligence would be. The simplicity of the law by which the celestial bodies move, and the relations of their masses and distances, permit analysis to follow their motions up to a certain point; and in order to determine the state of the system of these great bodies in past or future centuries, it suffices for the mathematician that their position and their velocity be given by observation for any moment in time. Man owes that advantage to the power of the instrument he employs, and to the small number of relations that it embraces in its calculations. But ignorance of the different causes involved in the production of events, as well as their complexity, taken together with the imperfection of analysis, prevents our reaching the same certainty about the vast majority of phenomena. Thus there are things that are uncertain for us, things more or less probable, and we seek to compensate for the impossibility of knowing them by determining their different degrees of likelihood. So it was that we owe to the weakness of the human mind one of the most delicate and ingenious of mathematical theories, the science of chance or probability.
'Recherches, 1º, sur l'Intégration des Équations Différentielles aux Différences Finies, et sur leur Usage dans la Théorie des Hasards' (1773, published 1776). In Oeuvres complètes de Laplace, 14 Vols. (1843-1912), Vol. 8, 144-5, trans. Charles Coulston Gillispie, Pierre-Simon Laplace 1749-1827: A Life in Exact Science (1997), 26.
The present theory of relativity is based on a division of physical reality into a metric field (gravitation) on the one hand and into an electromagnetic field and matter on the other hand. In reality space will probably be of a uniform character and the present theory will be valid only as a limiting case. For large densities of field and of matter, the field equations and even the field variables which enter into them will have no real significance. One may not therefore assume the validity of the equations for very high density of field and matter, and one may not conclude that the 'beginning of the expansion' must mean a singularity in the mathematical sense. All we have to realise is that the equations may not be continued over such regions.
In O. Nathan and H. Norden (eds.), Einstein on Peace (1960), 640.
The prevailing trend in modern physics is thus much against any sort of view giving primacy to ... undivided wholeness of flowing movement. Indeed, those aspects of relativity theory and quantum theory which do suggest the need for such a view tend to be de-emphasized and in fact hardly noticed by most physicists, because they are regarded largely as features of the mathematical calculus and not as indications of the real nature of things.
Wholeness and the Implicate Order? (1981), 14.
The problem with linear theory is that it is not nonlinear.
Epigraph in Mathematics in Nature: Modeling Patterns in the Natural World (2003), 173. Quoting from his own first professional presentation as a first-year graduate student.
The progress of science is strewn, like an ancient desert trail, with the bleached skeletons of discarded theories which once seemed to possess eternal life.
In The Ghost in the Machine (1967), 178.
The purpose of the present course is the deepening and development of difficulties underlying contemporary theory...
…...
The quantum entered physics with a jolt. It didn’t fit anywhere; it made no sense; it contradicted everything we thought we knew about nature. Yet the data seemed to demand it. ... The story of Werner Heisenberg and his science is the story of the desperate failures and ultimate triumphs of the small band of brilliant physicists who—during an incredibly intense period of struggle with the data, the theories, and each other during the 1920s—brought about a revolutionary new understanding of the atomic world known as quantum mechanics.
Beyond Uncertainty: Heisenberg, Quantum Physics, and the Bomb (2009), 90. Selected and contributed to this website by the author.
The quantum theory of gravity has opened up a new possibility, in which there would be no boundary to space-time and so there would be no need to specify the behaviour at the boundary. There would be no singularities at which the laws of science broke down and no edge of space-time at which one would have to appeal to God or some new law to set the boundary conditions for space-time. One could say: 'The boundary condition of the universe is that it has no boundary.' The universe would be completely self-contained and not affected by anything outside itself. It would neither be created nor destroyed. It would just BE.
A Brief History of Time: From the Big Bang to Black Holes (1988), 136.
The question, What is cholera? is left unsolved. Concerning this, the fundamental point, all is darkness and confusion, vague theory, and a vain speculation. Is it a fungus, an insect, a miasm,
an electrical disturbance, a deficiency of ozone, a morbid offscouring from the intestinal canal? We know nothing; we are at sea, in a whirlpool of conjecture.
Editorial in Lancet 1 (1853), 393. As quoted in Peter Vinten-Johansen, et al., Cholera, Chloroform, and the Science of Medicine: A Life of John Snow (2003), 116.
The really profound changes in human life all have their ultimate origin in knowledge pursued for its own sake. The use of the compass was not introduced into Europe till the end of the twelfth century A.D., more than three thousand years after its first use in China. The importance which the science of electromagnetism has since assumed in every department of human life is due not to the superior practical bias of Europeans, but to the fact that in the West electrical and magnetic phenomena were studied by men who were dominated by abstract theoretic interests.
In Introduction to Mathematics (1911), 32-33.
The Reason of making Experiments is, for the Discovery of the Method of Nature, in its Progress and Operations. Whosoever, therefore doth rightly make Experiments, doth design to enquire into some of these Operations; and, in order thereunto, doth consider what Circumstances and Effects, in the Experiment, will be material and instructive in that Enquiry, whether for the confirming or destroying of any preconceived Notion, or for the Limitation and Bounding thereof, either to this or that Part of the Hypothesis, by allowing a greater Latitude and Extent to one Part, and by diminishing or restraining another Part within narrower Bounds than were at first imagin'd, or hypothetically supposed. The Method therefore of making Experiments by the Royal Society I conceive should be this.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
'Dr Hooke's Method of Making Experiments' (1664-5). In W. Derham (ed.), Philosophical Experiments and Observations Of the Late Eminent Dr. Robert Hooke, F.R.S. And Geom. Prof. Gresh. and Other Eminent Virtuoso's in his Time (1726), 26-8.
The recurrence of a phenomenon like Edison is not very likely. The profound change of conditions and the ever increasing necessity of theoretical training would seem to make it impossible. He will occupy a unique and exalted position in the history of his native land, which might well be proud of his great genius and undying achievements in the interest of humanity.
As quoted in 'Tesla Says Edison Was an Empiricist', The New York Times (19 Oct 1931), 25. In 1884, Tesla had moved to America to assist Edison in the designing of motors and generators.
The relationships of free and latent heat set forth in the language of the materialistic theory remain the same if in place of the quantity of matter we put the constant quantity of motion in accordance with the laws of mechanics. The only difference enters where it concerns the generations of heat through other motive forces and where it concerns the equivalent of heat that can be produced by a particular quantity of a mechanical or electrical force.
'Wärme, physiologisch', Handwörterbuch der medicinischen Wissenschaften (1845). In Timothy Lenoir, The Strategy of Life (1982), 203.
The result of all these experiments has given place to a new division of the parts of the human body, which I shall follow in this short essay, by distinguishing those which are susceptible of Irritability and Sensibility, from those which are not. But the theory, why some parts of the human body are endowed with these properties, while others are not, I shall not at all meddle with. For I am persuaded that the source of both lies concealed beyond the reach of the knife and microscope, beyond which I do not chuse to hazard many conjectures, as I have no desire of teaching what I am ignorant of myself. For the vanity of attempting to guide others in paths where we find ourselves in the dark, shews, in my humble opinion, the last degree of arrogance and ignorance.
'A Treatise on the Sensible and Irritable Parts of Animals' (Read 1752). Trans. 1755 and reprinted in Bulletin of the Institute of the History of Medicine, 1936, 4(2), 657-8.
The results have exhibited one striking feature which has been frequently emphasized, namely that at high pressures all twelve liquids become more nearly like each other. This suggests that it might be useful in developing a theory of liquids to arbitrarily construct a 'perfect liquid' and to discuss its properties. Certainly the conception of a 'perfect gas' has been of great service in the kinetic theory of gases; and the reason is that all actual gases approximate closely to the 'perfect gas.' In the same way, at high pressures all liquids approximate to one and the same thing, which may be called by analogy the 'perfect liquid.' It seems to offer at least a promising line of attack to discuss the properties of this 'perfect liquid,' and then to invent the simplest possible mechanism to explain them.
'Thermodynamic Properties of Twelve Liquids Between 200 and 800 and up to 1200 KGM. Per Sq. Cm.', Memoirs of the American Academy of Arts and Sciences, 1913, 49, 113.
The scientific theorist is not to be envied. For Nature, or more precisely experiment, is an inexorable and not very friendly judge of his work. It never says “Yes” to a theory. In the most favorable cases it says “Maybe,” and in the great majority of cases simply “No.” If an experiment agrees with a theory it means for the latter “Maybe,” and if it does not agree it means “No.” Probably every theory will someday experience its “No”—most theories, soon after conception.
In Albert Einstein: The Human Side by Helen Dukas and Banesh Hoffmann (1979).
The scientific theory I like best is that the rings of Saturn are composed entirely of lost airline luggage
In Eileen Mason, Great Book of Funny Quotes: Witty Words for Every Day of the Year (1993). Quoted in Lilly Walters, What to Say When … You're Dying on the Platform (1995), 173.
The scientist explores the world of phenomena by successive approximations. He knows that his data are not precise and that his theories must always be tested. It is quite natural that he tends to develop healthy skepticism, suspended judgment, and disciplined imagination.
In Commencement Address, California Institute of Technology (10 Jun 1938), 'Experiment and Experience'. Collected in abridged form in The Huntington Library Quarterly (Apr 1939), 2, No. 3, 245
The scientist knows that the ultimate of everything is unknowable. No matter What subject you take, the current theory of it if carried to the ultimate becomes ridiculous. Time and space are excellent examples of this.
As quoted in 'Electricity Will Keep The World From Freezing Up', New York Times (12 Nov 1911), SM4.
The so-called science of psychology is now in chaos, with no sign that order is soon to be restored. It is hard to find two of its professors who agree, and when the phenomenon is encountered it usually turns out that one of them is not a psychologist at all, but simply a teacher of psychology. … Not even anthropology offers a larger assortment of conflicting theories, or a more gaudy band of steaming and blood-sweating professors.
From book review (of Psychology: A Simplification) in American Mercury (Jul 1927), 582-583. Collected in A Mencken Chrestomathy (1949, 1956), 317.
The starting point of Darwin’s theory of evolution is precisely the existence of those differences between individual members of a race or species which morphologists for the most part rightly neglect. The first condition necessary, in order that any process of Natural Selection may begin among a race, or species, is the existence of differences among its members; and the first step in an enquiry into the possible effect of a selective process upon any character of a race must be an estimate of the frequency with which individuals, exhibiting any given degree of abnormality with respect to that, character, occur. The unit, with which such an enquiry must deal, is not an individual but a race, or a statistically representative sample of a race; and the result must take the form of a numerical statement, showing the relative frequency with which the various kinds of individuals composing the race occur.
Biometrika: A Joumal for the Statistical Study of Biological Problems (1901), 1, 1-2.
The story of a theory’s failure often strikes readers as sad and unsatisfying. Since science thrives on self-correction, we who practice this most challenging of human arts do not share such a feeling. We may be unhappy if a favored hypothesis loses or chagrined if theories that we proposed prove inadequate. But refutation almost always contains positive lessons that overwhelm disappointment, even when no new and comprehensive theory has yet filled the void.
…...
The structural theory of Kekulé has been the growth hormone of organic chemistry.
Co-author with Melville Calvin (1911-1997)
Co-author with Melville Calvin (1911-1997)
The Theory of Organic Chemistry (1941), Preface, v.
The struggle between the unitary and dualistic theories of chemical affinity, which raged for nearly a century, was a form of civil war between inorganic and organic chemists.
Comment made on a postcard sent to Robin O. Gandy. Reproduced in Andrew Hodges, Alan Turing: The Enigma (1983), 513.
The struggle for existence holds as much in the intellectual as in the physical world. A theory is a species of thinking, and its right to exist is coextensive with its power of resisting extinction by its rivals.
Science and Culture, and Other Essays (1890), 335.
The study of mathematics is apt to commence in disappointment. The important applications of the science, the theoretical interest of its ideas, and the logical rigour of its methods all generate the expectation of a speedy introduction to processes of interest. We are told that by its aid the stars are weighed and the billions of molecules in a drop of water are counted. Yet, like the ghost of Hamlet's father, this great science eludes the efforts of our mental weapons to grasp it.
Opening to An Introduction to Mathematics (1911), 7.
The study of the radio-active substances and of the discharge of electricity through gases has supplied very strong experimental evidence in support of the fundamental ideas of the existing atomic theory. It has also indicated that the atom itself is not the smallest unit of matter, but is a complicated structure made up of a number of smaller bodies.
In Radio-activity (1905), 1.
The study of the theory of a physical science should be preceded by some general experimental acquaintance therewith, in order to secure the inimitable advantage of a personal acquaintance with something real and living.
Opening sentence of Electromagnetic Theory (1892), Vol. 2, 1.
The sublime discoveries of Newton, and, together with these, his not less fruitful than wonderful application, of the higher mathesis to the movement of the celestial bodies, and to the laws of light, gave almost religious sanction to the corpuscular system and mechanical theory. It became synonymous with philosophy itself. It was the sole portal at which truth was permitted to enter. The human body was treated an hydraulic machine... In short, from the time of Kepler to that of Newton, and from Newton to Hartley, not only all things in external nature, but the subtlest mysteries of life, organization, and even of the intellect and moral being, were conjured within the magic circle of mathematical formulae.
Hints Towards the Formation of a more Comprehensive Theory of Life (1848). In The Collected Works of Samuel Taylor Coleridge: Shorter Works and Fragments (1995), H. J. Jackson and J. R. de J. Jackson (eds.), Vol. 11, 1, 498.
The success of the paradigm... is at the start largely a promise of success ... Normal science consists in the actualization of that promise... Mopping up operations are what engage most scientists throughout their careers. They constitute what I am here calling normal science... That enterprise seems an attempt to force nature into the preformed and relatively inflexible box that the paradigm supplies. No part of the aim of normal science is to call forth new sorts of phenomena; indeed those that will not fit the box are often not seen at all. Nor do scientists normally aim to invent new theories, and they are often intolerant of those invented by others.
The Structure of Scientific Revolutions (1962), 23-4.
The supposed astronomical proofs of the theory [of relativity], as cited and claimed by Einstein, do not exist. He is a confusionist. The Einstein theory is a fallacy. The theory that ether does not exist, and that gravity is not a force but a property of space can only be described as a
crazy vagary, a disgrace to our age.
Quoted in Elizabeth Dilling, A "Who's Who" and Handbook of Radicalism for Patriots (1934), 49.
The supreme achievement would be to see that stating a fact is starting a theory.
In The Maxims and Reflections of Goethe (1906), 194.
The supreme goal of all theory is to make the irreducible basic elements as simple and as few as possible without having to surrender the adequate representation of a single datum of experience.
…...
The synthetic theory of evolution has always seemed to me to be one of the most impressive achievements of the human intellect, a collective scientific product of indubitable validity.
In Internal Factors in Evolution (1965), 23.
The technologies which have had the most profound effects on human life are usually simple. A good example of a simple technology with profound historical consequences is hay. Nobody knows who invented hay, the idea of cutting grass in the autumn and storing it in large enough quantities to keep horses and cows alive through the winter. All we know is that the technology of hay was unknown to the Roman Empire but was known to every village of medieval Europe. Like many other crucially important technologies, hay emerged anonymously during the so-called Dark Ages. According to the Hay Theory of History, the invention of hay was the decisive event which moved the center of gravity of urban civilization from the Mediterranean basin to Northern and Western Europe. The Roman Empire did not need hay because in a Mediterranean climate the grass grows well enough in winter for animals to graze. North of the Alps, great cities dependent on horses and oxen for motive power could not exist without hay. So it was hay that allowed populations to grow and civilizations to flourish among the forests of Northern Europe. Hay moved the greatness of Rome to Paris and London, and later to Berlin and Moscow and New York. ... Great inventions like hay and printing, whatever their immediate social costs may be, result in a permanent expansion of our horizons, a lasting acquisition of new territory for human bodies and minds to cultivate.
Infinite In All Directions (1988, 2004), 135. The book is a revised version of a series of the Gifford Lectures under the title 'In Praise of Diversity', given at Aberdeen, Scotland.
The test of a theory is its ability to cope with all the relevant phenomena, not its a priori 'reasonableness'. The latter would have proved a poor guide in the development of science, which often makes progress by its encounter with the totally unexpected and initially extremely puzzling.
'From DAMTP [Department of Applied Mathematics and Theoretical Physics] to Westcott House', Cambridge Review (1981), 103, 61.
The test of science is not whether you are reasonable—there would not be much of physics if that was the case—the test is whether it works. And the great point about Newton’s theory of gravitation was that it worked, that you could actually say something about the motion of the moon without knowing very much about the constitution of the Earth.
From Assumption and Myth in Physical Theory (1967), 10.
The theoretical broadening which comes from having many humanities subjects on the campus is offset by the general dopiness of the people who study these things and by the Department of Home Economics.
Letter to Robert Bacher (6 Apr 1950), as quoted in James Gleick, Genius: The Life and Science of Richard Feynman (1992), 278.
The theoretical idea … does not arise apart from and independent of experience; nor can it be derived from experience by a purely logical procedure. It is produced by a creative act. Once a theoretical idea has been acquired, one does well to hold fast to it until it leads to an untenable conclusion.
'On the Generalized Theory of Gravitation', Scientific American (Apr 1950). Collected in David H. Levy (ed.), The Scientific American Book of the Cosmos (2000), 14.
The theoretical side of physical chemistry is and will probably remain the dominant one; it is by this peculiarity that it has exerted such a great influence upon the neighboring sciences, pure and applied, and on this ground physical chemistry may be regarded as an excellent school of exact reasoning for all students of the natural sciences.
In Theories of Solutions (1912), xx.
The theory here developed is that mega-evolution normally occurs among small populations that become preadaptive and evolve continuously (without saltation, but at exceptionally rapid rates) to radically different ecological positions. The typical pattern involved is probably this: A large population is fragmented into numerous small isolated lines of descent. Within these, inadaptive differentiation and random fixation of mutations occur. Among many such inadaptive lines one or a few are preadaptive, i.e., some of their characters tend to fit them for available ecological stations quite different from those occupied by their immediate ancestors. Such groups are subjected to strong selection pressure and evolve rapidly in the further direction of adaptation to the new status. The very few lines that successfully achieve this perfected adaptation then become abundant and expand widely, at the same time becoming differentiated and specialized on lower levels within the broad new ecological zone.
Tempo and Mode in Evolution (1944), 123.
The theory I propose may therefore be called a theory of the Electromagnetic Field because it has to do with the space in the neighbourhood of the electric or magnetic bodies, and it may be called a Dynamical Theory, because it assumes that in the space there is matter in motion, by which the observed electromagnetic phenomena are produced.
'A Dynamical Theory of the Electromagnetic Field' (1865). In W. D. Niven (ed.), The Scientific Papers of James Clerk Maxwell(1890), Vol. 2, 527.
The theory is confirmed that pea hybrids form egg and pollen cells, which, in their constitution, represent in equal numbers all constant forms which result for the combination of the characters united in fertilization.
As collected in Forest Ray Moulton (ed.), The Autobiography of Science (1945), 586.
The theory of evolution by natural selection is an ecological theory—founded on ecological observation by perhaps the greatest of all ecologists. It has been adopted by and brought up by the science of genetics, and ecologists, being modest people, are apt to forget their distinguished parenthood.
'A Darwinian Approach to Plant Ecology', Journal of Ecology, 1967, 55, 247.
The theory of evolution has often been perverted so as to indicate that what is merely animal and brutal must gain the ascendancy. The contrary seems to me to be the case, for in man it is the spirit, and not the body, which is the deciding factor.
In Proceedings of Linnean Society of London (1913), 36.
The Theory of Groups is a branch of mathematics in which one does something to something and then compares the result with the result obtained from doing the same thing to something else, or something else to the same thing.
In J.R. Newman (ed.) The World of Mathematics (1956), Vol. 3, 1534.
The theory of medicine, therefore, presents what is useful in thought, but does not indicate how it is to be applied in practice—the mode of operation of these principles. The theory, when mastered, gives us a certain kind of knowledge. Thus we say, for example, there are three forms of fevers and nine constitutions. The practice of medicine is not the work which the physician carries out, but is that branch of medical knowledge which, when acquired, enables one to form an opinion upon which to base the proper plan of treatment.
— Avicenna
'The Definition of Medicine', in The Canon of Medicine, adapted by L. Bakhtiar (1999), 10.
The theory of numbers is particularly liable to the accusation that some of its problems are the wrong sort of questions to ask. I do not myself think the danger is serious; either a reasonable amount of concentration leads to new ideas or methods of obvious interest, or else one just leaves the problem alone. “Perfect numbers” certainly never did any good, but then they never did any particular harm.
In A Mathematician’s Miscellany (1953). Reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 74.
The theory of probabilities is at bottom nothing but common sense reduced to calculus; it enables us to appreciate with exactness that which accurate minds feel with a sort of instinct for which of times they are unable to account.
Introduction to Théorie Analytique des Probabilitiés
The theory of probabilities is at bottom only common sense reduced to calculation; it makes us appreciate with exactitude what reasonable minds feel by a sort of instinct, often without being able to account for it. … It is remarkable that [this] science, which originated in the consideration of games of chance, should have become the most important object of human knowledge.
From A Philosophical Essay on Probabilities. As given in epigraph, E.T. Bell, Men of Mathematics (2014), 71.
The theory of probabilities is basically only common sense reduced to a calculus. It makes one estimate accurately what right-minded people feel by a sort of instinct, often without being able to give a reason for it.
Philosophical Essay on Probabilities (1814), 5th edition (1825), trans. Andrew I. Dale (1995), 124.
The theory of probability is the only mathematical tool available to help map the unknown and the uncontrollable. It is fortunate that this tool, while tricky, is extraordinarily powerful and convenient.
The Fractal Geometry of Nature (1977, 1983), 201.
The theory of punctuated equilibrium, proposed by Niles Eldredge and myself, is not, as so often misunderstood, a radical claim for truly sudden change, but a recognition that ordinary processes of speciation, properly conceived as glacially slow by the standard of our own life-span, do not resolve into geological time as long sequences of insensibly graded intermediates (the traditional, or gradualistic, view), but as geologically ‘sudden’ origins at single bedding planes.
…...
The theory of quantum mechanics also explained all kinds of details, such as why an oxygen atom combines with two hydrogen atoms to make water, and so on. Quantum mechanics thus supplied the theory behind chemistry. So, fundamental theoretical chemistry is really physics.
In 'Introduction', QED: The Strange Theory of Light and Matter (1985), 5.
The theory of ramification is one of pure colligation, for it takes no account of magnitude or position; geometrical lines are used, but these have no more real bearing on the matter than those employed in genealogical tables have in explaining the laws of procreation.
From 'On Recent Discoveries in Mechanical Conversion of Motion', Proceedings of the Royal Institution of Great Britain (1873-75), 7, 179-198, reprinted in The Collected Mathematical Papers of James Joseph Sylvester: (1870-1883) (1909), Vol. 3, 23.
The Theory of Relativity confers an absolute meaning on a magnitude which in classical theory has only a relative significance: the velocity of light. The velocity of light is to the Theory of Relativity as the elementary quantum of action is to the Quantum Theory: it is its absolute core.
'A Scientific Autobiography' (1948), in Scientific Autobiography and Other Papers, trans. Frank Gaynor (1950), 47.
The theory of the earth is the science which describes and explains changes that the terrestrial globe has undergone from its beginning until today, and which allows the prediction of those it shall undergo in the future. The only way to understand these changes and their causes is to study the present-day state of the globe in order to gradually reconstruct its earlier stages, and to develop probable hypotheses on its future state. Therefore, the present state of the earth is the only solid base on which the theory can rely.
In Albert V. Carozzi, 'Forty Years of Thinking in Front of the Alps: Saussure's (1796) Unpublished Theory of the Earth', Earth Sciences History (1989), 8 136.
The theory of the lung as a gland has justified its existence and done excellent service in bringing forward facts, which shall survive any theoretical construction that has been or may hereafter be put upon them.
From The Mechanism of Gas Exchange (1910), 257, as cited by E. Snorrason, 'Krogh, Schack August Steenberg', in Charles Coulton Gillispie (ed.), Dictionary of Scientific Biography (1973), Vol 7, 502.
The theory of the method of knowing which is advanced in these pages may be termed pragmatic. ... Only that which has been organized into our disposition so as to enable us to adapt the environment to our needs and adapt our aims and desires to the situation in which we live is really knowledge.
Democracy and Education: an Introduction to the Philosophy of Education (1916), 400.
The theory that gravitational attraction is inversely proportional to the square of the distance leads by remorseless logic to the conclusion that the path of a planet should be an ellipse, … It is this logical thinking that is the real meat of the physical sciences. The social scientist keeps the skin and throws away the meat. … His theorems no more follow from his postulates than the hunches of a horse player follow logically from the latest racing news. The result is guesswork clad in long flowing robes of gobbledygook.
In Science is a Sacred Cow (1950), 149-150.
The theory which I would offer, is simply, that as the land with the attached reefs subsides very gradually from the action of subterranean causes, the coral-building polypi soon raise again their solid masses to the level of the water: but not so with the land; each inch lost is irreclaimably gone; as the whole gradually sinks, the water gains foot by foot on the shore, till the last and highest peak is finally submerged.
Journal of Researches: into the Natural History and Geology of the Countries Visited During the Voyage of H.M.S. Beagle Round the World (1839), ch. XXll, 557.
The title affixed to it is “The Chemical Theory of Electrolytes,” but it is a bigger thing than this: it really is an attempt at an electrolytic theory of chemistry.
On Svante Arrhenius’ Theorie Chemique des Electrolytes, abstract and report by Oliver Lodge.
On Svante Arrhenius’ Theorie Chemique des Electrolytes, abstract and report by Oliver Lodge.
56th Report of The British Association for the Advancement of Science, 1886, 362.
The tool which serves as intermediary between theory and practice, between thought and observation, is mathematics; it is mathematics which builds the linking bridges and gives the ever more reliable forms. From this it has come about that our entire contemporary culture, inasmuch as it is based on the intellectual penetration and the exploitation of nature, has its foundations in mathematics. Already Galileo said: one can understand nature only when one has learned the language and the signs in which it speaks to us; but this language is mathematics and these signs are mathematical figures.
Radio broadcast (8 Sep 1930). As quoted in Michael Fitzgerald and Ioan James, The Mind of the Mathematician (2007), 6-7.
The transition from a paradigm in crisis to a new one from which a new tradition of normal science can emerge is far from a cumulative process, one achieved by an articulation or extension of the old paradigm. Rather it is a reconstruction of the field from new fundamentals, a reconstruction that changes some of the field's most elementary theoretical generalizations as well as many of its paradigm methods and applications. During the transition period there will be a large but never complete overlap between the problems that can be solved by the old and by the new paradigm. But there will also be a decisive difference in the modes of solution. When the transition is complete, the profession will have changed its view of the field, its methods, and its goals.
The Structure of Scientific Revolutions (1962), 84-5.
The trend of mathematics and physics towards unification provides the physicist with a powerful new method of research into the foundations of his subject. … The method is to begin by choosing that branch of mathematics which one thinks will form the basis of the new theory. One should be influenced very much in this choice by considerations of mathematical beauty. It would probably be a good thing also to give a preference to those branches of mathematics that have an interesting group of transformations underlying them, since transformations play an important role in modern physical theory, both relativity and quantum theory seeming to show that transformations are of more fundamental importance than equations.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 122.
The universe came into being in a big bang, before which, Einstein’s theory instructs us, there was no before. Not only particles and fields of force had to come into being at the big bang, but the laws of physics themselves, and this by a process as higgledy-piggledy as genetic mutation or the second law of thermodynamics.
In 'The Computer and the Universe', International Journal of Theoretical Physics (1982), 21, 565.
The universe is governed by science. But science tells us that we can’t solve the equations, directly in the abstract. We need to use the effective theory of Darwinian natural selection of those societies most likely to survive. We assign them higher value.
[Answer to question: What is the value in knowing “Why are we here?”]
[Answer to question: What is the value in knowing “Why are we here?”]
'Stephen Hawking: "There is no heaven; it’s a fairy story"', interview in newspaper The Guardian (15 May 2011).
The velocity of light is one of the most important of the fundamental constants of Nature. Its measurement by Foucault and Fizeau gave as the result a speed greater in air than in water, thus deciding in favor of the undulatory and against the corpuscular theory. Again, the comparison of the electrostatic and the electromagnetic units gives as an experimental result a value remarkably close to the velocity of light–a result which justified Maxwell in concluding that light is the propagation of an electromagnetic disturbance. Finally, the principle of relativity gives the velocity of light a still greater importance, since one of its fundamental postulates is the constancy of this velocity under all possible conditions.
Studies in Optics (1927), 120.
The very closest stars would require many years to visit, even traveling at the speed of light, which is impossible according to Einstein's theory of relativity. Today's fastest spaceships would require 200,000 years to travel to Alpha Centauri, our closest bright star. The energy required to send a hundred colonists to another star, as Frank Drake has pointed out, would be enough to meet the energy needs of the entire United States over a human lifetime. And these estimates are regarding nearby stars. When we consider the distances across the entire galaxy, and between galaxies, interstellar travel seems absolutely untenable.
As co-author with his son, Marshall Fisher, in Strangers in the Night: a Brief History of Life on Other Worlds (1998).
The vital act is the act of participation. “Participator” is the incontrovertible new concept given by quantum mechanics. It strikes down the term “observer” of classical theory, the man who stands safely behind the thick glass wall and watches what goes on without taking part. It can’t be done, quantum mechanics says.
In Charles W. Misner, Kip S. Thorne, John Archibald Wheeler, 'Beyond the Edge of Time', Gravitation (1973), Part 3, 1217.
The vortex theory [of the atom] is only a dream. Itself unproven, it can prove nothing, and any speculations founded upon it are mere dreams about dreams.
Quoted in Henry Smith Williams, 'Some Unsolved Scientific Problems', Harper's New Monthly Magazine (1899-1900), Vol. 100, 779.
The Wegener hypothesis has been so stimulating and has such fundamental implications in geology as to merit respectful and sympathetic interest from every geologist. Some striking arguments in his favor have been advanced, and it would be foolhardy indeed to reject any concept that offers a possible key to the solution of profound problems in the Earth’s history.
Published while geologists remained sceptical of Alfred Wegener’s idea of Continental Drift, Though unconvinced, he published these thoughts suggesting that critics should be at least be open-minded. His patience was proven justified when two decades later, the theory of plate tectonics provided a mechanism for the motion of the continents.
Published while geologists remained sceptical of Alfred Wegener’s idea of Continental Drift, Though unconvinced, he published these thoughts suggesting that critics should be at least be open-minded. His patience was proven justified when two decades later, the theory of plate tectonics provided a mechanism for the motion of the continents.
Some Thoughts on the Evidence for Continental Drift (1944).
The whole theory of the motive power of heat is founded on the two following propositions, due respectively to Joule, and to Carnot and Clausius.
PROP. I. Joule).—When equal quantities of mechanical effect are produced by any means whatever from purely thermal sources, or lost in purely thermal effects, equal quantities of heat are put out of existence or are generated.
PROP. II. (Carnot and Clausius).—If an engine be such that, when it is worked backwards, the physical and mechanical agencies in every part of its motions are all reversed, it produces as much mechanical effect as can be produced by any thermo-dynamic engine, with the same temperatures of source and refrigerator, from a given quantity of heat.
PROP. I. Joule).—When equal quantities of mechanical effect are produced by any means whatever from purely thermal sources, or lost in purely thermal effects, equal quantities of heat are put out of existence or are generated.
PROP. II. (Carnot and Clausius).—If an engine be such that, when it is worked backwards, the physical and mechanical agencies in every part of its motions are all reversed, it produces as much mechanical effect as can be produced by any thermo-dynamic engine, with the same temperatures of source and refrigerator, from a given quantity of heat.
In 'On the Dynamical Theory of Heat, with Numerical Results Deduced from Mr Joule's Equivalent of a Thermal Unit, and M. Regnault's Observations on Steam' (1851). In Mathematical and Physical Papers (1882), Vol. 1, 178.
The words are strung together, with their own special grammar—the laws of quantum theory—to form sentences, which are molecules. Soon we have books, entire libraries, made out of molecular “sentences.” The universe is like a library in which the words are atoms. Just look at what has been written with these hundred words! Our own bodies are books in that library, specified by the organization of molecules—but the universe and literature are organizations of identical, interchangeable objects; they are information systems.
In The Cosmic Code: Quantum Physics as the Language of Nature (1983), 255.
The world has arisen in some way or another. How it originated is the great question, and Darwin's theory, like all other attempts, to explain the origin of life, is thus far merely conjectural. I believe he has not even made the best conjecture possible in the present state of our knowledge.
In Evolution and Permanence of Type (1874), 12.
The world hath been much abused by the opinion of making gold; the work itself I judge to be possible; but the means, hitherto propounded, to effect it are, in the practice, full of error and imposture; and in the theory, full of unfound imaginations.
From 'Experiment Solitary Touching the Making of Gold', Sylva Sylvarum: Or, a Natural History (1627), published posthumously by William Rawley. As collected and translated in The Works of Francis Bacon (1765), Vol. 1, 204. As quoted in Samuel Austin Allibone, Prose Quotations From Socrates to Macaulay (1876), 28.
The world little knows how many of the thoughts and theories which have passed through the mind of a scientific investigator, have been crushed in silence and secrecy by his own severe criticism and adverse examination; that in the most successful instances not a tenth of the suggestions, the hopes, the wishes, the preliminary conclusions have been realized.
In 'Observations On Mental Education', a lecture before the Prince Consort and the Royal Institution (6 May 1854). Experimental Researches in Chemistry and Physics (1859), 486.
The young specialist in English Lit, having quoted me, went on to lecture me severely on the fact that in every century people have thought they understood the Universe at last, and in every century they were proved to be wrong. It follows that the one thing we can say about our modern “knowledge” is that it is wrong.
The young man then quoted with approval what Socrates had said on learning that the Delphic oracle had proclaimed him the wisest man in Greece. “If I am the wisest man,” said Socrates, “it is because I alone know that I know nothing.” The implication was that I was very foolish because I was under the impression I knew a great deal.
Alas, none of this was new to me. (There is very little that is new to me; I wish my correspondents would realize this.) This particular theme was addressed to me a quarter of a century ago by John Campbell, who specialized in irritating me. He also told me that all theories are proven wrong in time.
My answer to him was, “John, when people thought the Earth was flat, they were wrong. When people thought the Earth was spherical, they were wrong. But if you think that thinking the Earth is spherical is just as wrong as thinking the Earth is flat, then your view is wronger than both of them put together.”
The young man then quoted with approval what Socrates had said on learning that the Delphic oracle had proclaimed him the wisest man in Greece. “If I am the wisest man,” said Socrates, “it is because I alone know that I know nothing.” The implication was that I was very foolish because I was under the impression I knew a great deal.
Alas, none of this was new to me. (There is very little that is new to me; I wish my correspondents would realize this.) This particular theme was addressed to me a quarter of a century ago by John Campbell, who specialized in irritating me. He also told me that all theories are proven wrong in time.
My answer to him was, “John, when people thought the Earth was flat, they were wrong. When people thought the Earth was spherical, they were wrong. But if you think that thinking the Earth is spherical is just as wrong as thinking the Earth is flat, then your view is wronger than both of them put together.”
In The Relativity of Wrong (1989), 214.
Their minds sang with the ecstatic knowledge that either what they were doing was completely and utterly and totally impossible or that physics had a lot of catching up to do.
So Long, and Thanks for All the Fish (1985). Quoted in Gary Westfahl, Science Fiction Quotations (2005), 322.
Their specific effect on the glucosides might thus be explained by assuming that the intimate contact between the molecules necessary for the release of the chemical reaction is possible only with similar geometrical configurations. To give an illustration I will say that enzyme and glucoside must fit together like lock and key in order to be able to exercise a chemical action on each other. This concept has undoubtedly gained in probability and value for stereochemical research, after the phenomenon itself was transferred from the biological to the purely chemical field. It is an extension of the theory of asymmetry without being a direct consequence of it: for the conviction that the geometrical structure of the molecule even for optical isomers exercises such a great influence on the chemical affinities, in my opinion could only be gained by new actual observations.
'Einfluss der Configuration auf die wirkung der Enzyme', Berichte der deutschen Chemischen Gesellschaft, 1894, 27, 2985-93. Trans. B. Holmstedt and G. Liljestrand (eds.) Readings in Pharmacology (1963), 251.
Their theories should be carefully examined and their arguments fairly weighed, but the scientist cannot compel acceptance of any argument he advances, except as, judged upon its merits, it is convincing.
In chapter, 'The Origin of Man', In His Image (1922), 93.
Then one day Lagrange took out of his pocket a paper which he read at the Académe, and which contained a demonstration of the famous Postulatum of Euclid, relative to the theory of parallels. This demonstration rested on an obvious paralogism, which appeared as such to everybody; and probably Lagrange also recognised it such during his lecture. For, when he had finished, he put the paper back in his pocket, and spoke no more of it. A moment of universal silence followed, and one passed immediately to other concerns.
Quoting Lagrange at a meeting of the class of mathematical and physical sciences at the Institut de France (3 Feb 1806) in Journal des Savants (1837), 84, trans. Ivor Grattan-Guinness.
Theoretical and experimental physicists are now studying nothing at all—the vacuum. But that nothingness contains all of being.
The Cosmic Code: Quantum Physics as the Language of Nature (1982), 279.
Theoretical physicists accept the need for mathematical beauty as an act of faith... For example, the main reason why the theory of relativity is so universally accepted is its mathematical beauty.
'Methods in Theoretical Physics', From A Life of Physics: Evening Lectures at the International Centre for Theoretical Physics, Trieste, Italy. A Special Supplement of the IAEA Bulletin (1968), 22.
Theories are like a stairway; by climbing, science widens its horizon more and more, because theories embody and necessarily include proportionately more facts as they advance.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 165.
Theories cannot claim to be indestructible. They are only the plough which the ploughman uses to draw his furrow and which he has every right to discard for another one, of improved design, after the harvest. To be this ploughman, to see my labours result in the furtherance of scientific progress, was the height of my ambition, and now the Swedish Academy of Sciences has come, at this harvest, to add the most brilliant of crowns.
'The Method of Direct Hydrogenation by Catalysis', Nobel Lecture (11 Dec 1912). Noble Lectures in Chemistry 1901-1921 (1966), 230-1.
Theories rarely arise as patient inferences forced by accumulated facts. Theories are mental constructs potentiated by complex external prods (including, in idealized cases, a commanding push from empirical reality) . But the prods often in clude dreams, quirks, and errors–just as we may obtain crucial bursts of energy from foodstuffs or pharmaceuticals of no objective or enduring value. Great truth can emerge from small error. Evolution is thrilling, liberating, and correct. And Macrauchenia is a litoptern.
…...
Theories should not be used to select observations; on the contrary, it is observations which should be used to select the theories.
From webpage 'The Selection Effect' on laserstars.org website. The article does not specify an author, but the homepage attributes the site to Y.P. Varshni and J. Talbot.
Theory always tends to become abstract as it emerges successfully from the chaos of facts by the processes of differentiation and elimination, whereby the essentials and their connections become recognised, whilst minor effects are seen to be secondary or unessential, and are ignored temporarily, to be explained by additional means.
In Electromagnetic Theory (1892), Vol. 2, 1.
Theory and fact are equally strong and utterly interdependent; one has no meaning without the other. We need theory to organize and interpret facts, even to know what we can or might observe. And we need facts to validate theories and give them substance.
Leonardo's Mountain of Clams and the Diet of Worms: Essays on Natural History (1998), 155.
Theory attracts practice as the magnet attracts iron.
Attributed. Quoted in Speaking of Science: Notable Quotes on Science, Engineering, and the Environment (2000), 53.
Theory helps us to bear our ignorance of fact.
In The Sense of Beauty: Being the Outlines of Aesthetic Theory (1896), 125.
Theory is a window into the world. Theory leads to prediction. Without prediction, experience and examples teach nothing.
In The New Economics for Industry, Government, Education (1993), 103.
Theory is the essence of facts. Without theory scientific knowledge would be only worthy of the madhouse.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
Theory provides the maps that turn an uncoordinated set of experiments or computer simulations into a cumulative exploration.
…...
There are 60 sub-atomic particles they’ve discovered that can explain the thousands of other sub-atomic particles, and the model is too ugly. This is my analogy: it’s like taking Scotch tape and taping a giraffe to a mule to a whale to a tiger and saying this is the ultimate theory of particles. … We have so many particles that Oppenheimer once said you could give a Nobel Prize to the physicist that did not discover a particle that year. We were drowning in sub-atomic particles.
Now we realize that this whole zoo of sub-atomic particles, thousands of them coming out of our accelerators, can be explained by little vibrating strings.
Now we realize that this whole zoo of sub-atomic particles, thousands of them coming out of our accelerators, can be explained by little vibrating strings.
Quoted in Nina L. Diamond, Voices of Truth (2000), 334.
There are many examples of old, incorrect theories that stubbornly persisted, sustained only by the prestige of foolish but well-connected scientists. ... Many of these theories have been killed off only when some decisive experiment exposed their incorrectness.
In Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and The Tenth Dimension (1994), 314.
There are now three types of scientists: experimental, theoretical, and computational.
Quoted by Victor F. Weisskopf, 'One Hundred Years of the Physical Review', in H. Henry Stroke, Physical Review: The First Hundred Years: a Selection of Seminal Papers and Commentaries, Vol. 1, 13.
There are some modern practitioners, who declaim against medical theory in general, not considering that to think is to theorize; and that no one can direct a method of cure to a person labouring under disease, without thinking, that is, without theorizing; and happy therefore is the patient, whose physician possesses the best theory.
Zoonomia, or, The Laws Of Organic Life (1801), Vol. 2, ix.
There are something like ten million million million million million million million million million million million million million million (1 with eighty zeroes after it) particles in the region of the universe that we can observe. Where did they all come from? The answer is that, in quantum theory, particles can be created out of energy in the form of particle/antiparticle pairs. But that just raises the question of where the energy came from. The answer is that the total energy of the universe is exactly zero. The matter in the universe is made out of positive energy. However, the matter is all attracting itself by gravity. Two pieces of matter that are close to each other have less energy than the same two pieces a long way apart, because you have to expend energy to separate them against the gravitational force that is pulling them together. Thus, in a sense, the gravitational field has negative energy. In the case of a universe that is approximately uniform in space, one can show that this negative gravitational energy exactly cancels the positive energy represented by the matter. So the total energy of the universe is zero.
A Brief History of Time: From the Big Bang to Black Holes (1988), 129.
There are still psychologists who, in a basic misunderstanding, think that gestalt theory tends to underestimate the role of past experience. Gestalt theory tries to differentiate between and-summative aggregates, on the one hand, and gestalten, structures, on the other, both in sub-wholes and in the total field, and to develop appropriate scientific tools for investigating the latter. It opposes the dogmatic application to all cases of what is adequate only for piecemeal aggregates. The question is whether an approach in piecemeal terms, through blind connections, is or is not adequate to interpret actual thought processes and the role of the past experience as well. Past experience has to be considered thoroughly, but it is ambiguous in itself; so long as it is taken in piecemeal, blind terms it is not the magic key to solve all problems.
In Productive Thinking (1959), 65.
There is a great deal of emotional satisfaction in the elegant demonstration, in the elegant ordering of facts into theories, and in the still more satisfactory, still more emotionally exciting discovery that the theory is not quite right and has to be worked over again, very much as any other work of art—a painting, a sculpture has to be worked over in the interests of aesthetic perfection. So there is no scientist who is not to some extent worthy of being described as artist or poet.
'Scientist and Citizen', Speech to the Empire Club of Canada (29 Jan 1948), The Empire Club of Canada Speeches (29 Jan 1948), 209-221.
There is a mask of theory over the whole face of nature, if it be theory to infer more than we see.
The Philosophy of the Inductive Sciences: Founded Upon Their History (1840), Vol. 1, 24.
There is a theory that creativity arises when individuals are out of sync with their environment. To put it simply, people who fit in with their communities have insufficient motivation to risk their psyches in creating something truly new, while those who are out of sync are driven by the constant need to prove their worth.
In 'Beyond the Soapsuds Universe', Discover Magazine (1997). The author explains (in a blog found online) that this “idea comes from a theory by Mihaly Csikszentmihalyi, who wrote a book called Flow: the Psychology of Optimal Experience. You do need someone to come in from outside. One of the theories about why mathematicians do their best work when they’re young is because they’re not yet educated enough to know what seems obviously wrong. So they try new things.”
There is a theory which states that if ever anyone discovers exactly what the Universe is for and why it is here, it will instantly disappear and be replaced by something even more bizarre and inexplicable. There is another theory which states that this has already happened.
In The Restaurant at the End of the Universe (1980), facing p. 1.
There is an attraction and a charm inherent in the colossal that is not subject to ordinary theories of art … The tower will be the tallest edifice ever raised by man. Will it therefore be imposing in its own way?
Quoted in J. Harriss, The Tallest Tower: Eiffel and the Belle Epoque (1975), 25. Cited by David P. Billington, 'Bridges and the New Art of Structural Engineering,' in National Research Council (U.S.). Transportation Research Board Subcommittee on Bridge Aesthetics, Bridge Aesthetics Around the World (1991), 67. From the original French in interview of Eiffel by Paul Bourde, in the newspaper Le Temps (14 Feb 1887). Reprinted in 'Au Jour le Jour: Les Artistes Contre la Tour Eiffel', Gazette Anecdotique, Littéraire, Artistique et Bibliographique (Feb 1887), 126, and in Gustave Eiffel, Travaux Scientifiques Exécutés à la Tour de 300 Mètres de 1889 à 1900 (1900), 14. “Il y a du reste dans le colossal une attraction, un charme propre auxquels les théories d’art ordinaires ne sont guère applicables. … Ma tour sera le plus haut édifice qu'aient jamais élevé les hommes. Ne serat-elle donc pas grandiose aussi a sa façon?
There is no falsification before the emergence of a better theory.
In 'Falsification and the Methodology of Scientific Research Programmes', in I. Lakatos and A. Musgrave (eds.), Criticism and the Growth of Knowledge: Proceedings of the International Colloquium in the Philosophy of Science, London 1965 (1970), Vol. 4, 119.
There is no foundation in geological facts, for the popular theory of the successive development of the animal and vegetable world, from the simplest to the most perfect forms.
Principles of Geology (1830-3), Vol. 1, 153.
There is no great harm in the theorist who makes up a new theory to fit a new event. But the theorist who starts with a false theory and then sees everything as making it come true is the most dangerous enemy of human reason.
In The Flying Inn (1914), 103.
There is no more convincing proof of the truth of a comprehensive theory than its power of absorbing and finding a place for new facts, and its capability of interpreting phenomena which had been previously looked upon as unaccountable anomalies. It is thus that the law of universal gravitation and the undulatory theory of light have become established and universally accepted by men of science. Fact after fact has been brought forward as being apparently inconsistent with them, and one alter another these very facts have been shown to be the consequences of the laws they were at first supposed to disprove. A false theory will never stand this test. Advancing knowledge brings to light whole groups of facts which it cannot deal with, and its advocates steadily decrease in numbers, notwithstanding the ability and scientific skill with which it may have been supported.
From a review of four books on the subject 'Mimicry, and Other Protective Resemblances Among Animals', in The Westminster Review (Jul 1867), 88, 1. Wallace is identified as the author in the article as reprinted in William Beebe, The Book of Naturalists: An Anthology of the Best Natural History (1988), 108.
There is no such thing as absolute truth and absolute falsehood. The scientific mind should never recognise the perfect truth or the perfect falsehood of any supposed theory or observation. It should carefully weigh the chances of truth and error and grade each in its proper position along the line joining absolute truth and absolute error.
In 'The Highest Aim of the Physicist: Presidential Address Delivered at the 2nd Meeting of the Society, October 28th, 1899', Bulletin of the American Physical Society (1899), 1, 13.
There is now a feeling that the pieces of physics are falling into place, not because of any single revolutionary idea or because of the efforts of any one physicist, but because of a flowering of many seeds of theory, most of them planted long ago.
In 'The Forces of Nature', Bulletin of the American Academy of Arts and Sciences (Jan 1976), 29:4, 14.
There is thus a possibility that the ancient dream of philosophers to connect all Nature with the properties of whole numbers will some day be realized. To do so physics will have to develop a long way to establish the details of how the correspondence is to be made. One hint for this development seems pretty obvious, namely, the study of whole numbers in modern mathematics is inextricably bound up with the theory of functions of a complex variable, which theory we have already seen has a good chance of forming the basis of the physics of the future. The working out of this idea would lead to a connection between atomic theory and cosmology.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 129.
There may only be a small number of laws, which are self-consistent and which lead to complicated beings like ourselves. … And even if there is only one unique set of possible laws, it is only a set of equations. What is it that breathes fire into the equations and makes a universe for them to govern? Is the ultimate unified theory so compelling that it brings about its own existence?
Lecture (1987), 'The Origin of the Universe', collected in Black Holes And Baby Universes And Other Essays (1993), 99.
There should be no mystery in our use of the word science; it means knowledge, not theory nor speculation nor hypothesis, but hard facts, and the framework of laws to which they belong.
In 'The Meteorological Work of the U.S. Signal Service, 1870 to 1891', U.S. Department of Agriculture, Weather Bureau, Bulletin No. 11, Report of the International Meteorological Congress, Chicago, Ill., August 21-24, 1893 (1894), 242.
Therefore on long pondering this uncertainty of mathematical traditions on the deduction of the motions of the system of the spheres, I began to feel disgusted that no more certain theory of the motions of the mechanisms of the universe, which has been established for us by the best and most systematic craftsman of all, was agreed by the philosophers, who otherwise theorised so minutely with most careful attention to the details of this system. I therefore set myself the task of reading again the books of all philosophers which were available to me, to search out whether anyone had ever believed that the motions of the spheres of the, universe were other than was supposed by those who professed mathematics in the schools.
'To His Holiness Pope Paul III', in Copernicus: On the Revolutions of the Heavenly Spheres (1543), trans. A. M. Duncan (1976), 25.
These results demonstrate that there is a new polymerase inside the virions of RNA tumour viruses. It is not present in supernatents of normal cells but is present in virions of avian sarcoma and leukemia RNA tumour viruses. The polymerase seems to catalyse the incorporation of deoxyrinonucleotide triphosphates into DNA from an RNA template. Work is being performed to characterize further the reaction and the product. If the present results and Baltimore's results with Rauscher leukemia virus are upheld, they will constitute strong evidence that the DNA proviruses have a DNA genome when they are in virions. This result would have strong implications for theories of viral carcinogenesis and, possibly, for theories of information transfer in other biological systems. [Co-author with American virologist Satoshi Mizutani]
'RNA-dependent DNA Polymerase in Virions of Rous Sarcoma Virus', Nature (1970), 226, 1213.
These were errors of thought which cost me two years of excessively hard work, until I finally recognized them as such at the end of 1915, and after having ruefully returned to the Riemannian curvature, succeeded in linking the theory with the facts of astronomical experience.
In the light of knowledge attained, the happy achievement seems almost a matter of course, and any intelligent student can grasp it without too much trouble. But the years of anxious searching in the dark, with their intense longing, their alternations of confidence and exhaustion and the final emergence into the light—only those who have experienced it can understand it.
In the light of knowledge attained, the happy achievement seems almost a matter of course, and any intelligent student can grasp it without too much trouble. But the years of anxious searching in the dark, with their intense longing, their alternations of confidence and exhaustion and the final emergence into the light—only those who have experienced it can understand it.
From address, 'Notes on the Origin of the General Theory of Relativity', part of the collection of essays in Mein Weltbild (1934). Translation from the original German by Sonja Bargmann, in Ideas And Opinions (1954), 289-290.
They thought I was crazy, absolutely mad.
The response (1944) of the National Academy of Sciences, to her (later Nobel prize-winning) theory that proposed that genes could transition—'jumping'—to new locations on a chromosome.
The response (1944) of the National Academy of Sciences, to her (later Nobel prize-winning) theory that proposed that genes could transition—'jumping'—to new locations on a chromosome.
Quoted in Claudia Wallis, 'Honoring a Modern Mendel', Time (24 Oct 1983), 43.
This [the fact that the pursuit of mathematics brings into harmonious action all the faculties of the human mind] accounts for the extraordinary longevity of all the greatest masters of the Analytic art, the Dii Majores of the mathematical Pantheon. Leibnitz lived to the age of 70; Euler to 76; Lagrange to 77; Laplace to 78; Gauss to 78; Plato, the supposed inventor of the conic sections, who made mathematics his study and delight, who called them the handles or aids to philosophy, the medicine of the soul, and is said never to have let a day go by without inventing some new theorems, lived to 82; Newton, the crown and glory of his race, to 85; Archimedes, the nearest akin, probably, to Newton in genius, was 75, and might have lived on to be 100, for aught we can guess to the contrary, when he was slain by the impatient and ill mannered sergeant, sent to bring him before the Roman general, in the full vigour of his faculties, and in the very act of working out a problem; Pythagoras, in whose school, I believe, the word mathematician (used, however, in a somewhat wider than its present sense) originated, the second founder of geometry, the inventor of the matchless theorem which goes by his name, the pre-cognizer of the undoubtedly mis-called Copernican theory, the discoverer of the regular solids and the musical canon who stands at the very apex of this pyramid of fame, (if we may credit the tradition) after spending 22 years studying in Egypt, and 12 in Babylon, opened school when 56 or 57 years old in Magna Græcia, married a young wife when past 60, and died, carrying on his work with energy unspent to the last, at the age of 99. The mathematician lives long and lives young; the wings of his soul do not early drop off, nor do its pores become clogged with the earthy particles blown from the dusty highways of vulgar life.
In Presidential Address to the British Association, Collected Mathematical Papers, Vol. 2 (1908), 658.
This incomparable Author having at length been prevailed upon to appear in public, has in this Treatise given a most notable instance of the extent of the powers of the Mind; and has at once shown what are the Principles of Natural Philosophy, and so far derived from them their consequences, that he seems to have exhausted his Argument, and left little to be done by those that shall succeed him.a
Attributed.
This irrelevance of molecular arrangements for macroscopic results has given rise to the tendency to confine physics and chemistry to the study of homogeneous systems as well as homogeneous classes. In statistical mechanics a great deal of labor is in fact spent on showing that homogeneous systems and homogeneous classes are closely related and to a considerable extent interchangeable concepts of theoretical analysis (Gibbs theory). Naturally, this is not an accident. The methods of physics and chemistry are ideally suited for dealing with homogeneous classes with their interchangeable components. But experience shows that the objects of biology are radically inhomogeneous both as systems (structurally) and as classes (generically). Therefore, the method of biology and, consequently, its results will differ widely from the method and results of physical science.
Atom and Organism: A New Approach to Theoretical Biology (1966), 34.
This is often the way it is in physics—our mistake is not that we take our theories too seriously, but that we do not take them seriously enough. It is always hard to realize that these numbers and equations we play with at our desks have something to do with the real world.
In The First Three Minutes: A Modern View of the Origin of the Universe (1977, Rev. ed. 1993), 131-132.
This leads us to ask for the reasons which call for this new theory of transmutation. The beginning of things must needs lie in obscurity, beyond the bounds of proof, though within those of conjecture or of analogical inference. Why not hold fast to the customary view, that all species were directly, instead of indirectly, created after their respective kinds, as we now behold them,--and that in a manner which, passing our comprehension, we intuitively refer to the supernatural? Why this continual striving after “the unattained and dim,”—these anxious endeavors, especially of late years, by naturalists and philosophers of various schools and different tendencies, to penetrate what one of them calls “the mystery of mysteries,” the origin of species? To this, in general, sufficient answer may be found in the activity of the human intellect, “the delirious yet divine desire to know,” stimulated as it has been by its own success in unveiling the laws and processes of inorganic Nature,—in the fact that the principal triumphs of our age in physical science have consisted in tracing connections where none were known before, in reducing heterogeneous phenomena to a common cause or origin, in a manner quite analogous to that of the reduction of supposed independently originated species to a common ultimate origin,—thus, and in various other ways, largely and legitimately extending the domain of secondary causes. Surely the scientific mind of an age which contemplates the solar system as evolved from a common, revolving, fluid mass,— which, through experimental research, has come to regard light, heat, electricity, magnetism, chemical affinity, and mechanical power as varieties or derivative and convertible forms of one force, instead of independent species,—which has brought the so-called elementary kinds of matter, such as the metals, into kindred groups, and raised the question, whether the members of each group may not be mere varieties of one species,—and which speculates steadily in the direction of the ultimate unity of matter, of a sort of prototype or simple element which may be to the ordinary species of matter what the protozoa or component cells of an organism are to the higher sorts of animals and plants,—the mind of such an age cannot be expected to let the old belief about species pass unquestioned.
— Asa Gray
'Darwin on the Origin of Species', The Atlantic Monthly (Jul 1860), 112-3. Also in 'Natural Selection Not Inconsistent With Natural Theology', Darwiniana: Essays and Reviews Pertaining to Darwinism (1876), 94-95.
This morning’s messages announce the death oF Thomas Alva Edison, the great American inventor who for two generations has been an almost legendary figure. … He seemed to belong to a past which was already becoming remote, a past, without the incandescent electric light, and without the phonograph, and without other gifts with which his ingenuity and persistence endowed the world. … In the philosophical, theoretical sense he [Thomas Edison] was not a scientific man. To the understanding of electricity, with the use of which most of his inventions are concerned, he contributed little or nothing. Yet no man discovered more, or more ingenious, ways of using electricity for practical purposes.
In 'Thomas Edison', The Times (19 Oct 1931), 13.
This success permits us to hope that after thirty or forty years of observation on the new Planet [Neptune], we may employ it, in its turn, for the discovery of the one following it in its order of distances from the Sun. Thus, at least, we should unhappily soon fall among bodies invisible by reason of their immense distance, but whose orbits might yet be traced in a succession of ages, with the greatest exactness, by the theory of Secular Inequalities.
[Following the success of the confirmation of the existence of the planet Neptune, he considered the possibility of the discovery of a yet further planet.]
[Following the success of the confirmation of the existence of the planet Neptune, he considered the possibility of the discovery of a yet further planet.]
In John Pringle Nichol, The Planet Neptune: An Exposition and History (1848), 90.
This theory [the oxygen theory] is not as I have heard it described, that of the French chemists, it is mine (elle est la mienne); it is a property which I claim from my contemporaries and from posterity.
Memoires de Chimie (1805), Vol. 2, 87, trans. M. P. Crosland.
This very important property of rods, and indeed also of each kind of cone, this limitation of output to a single dimension of change, may be called the Principle of Univariance and stated thus: “The output of a receptor depends upon its quantum catch, but not upon what quanta are caught.” … Young's theory of colour vision may now be stated in terms of cone pigments. “There are three classes of cone each containing a different visual pigment. The output of each cone is univariant, depending simply upon the quantum catch of its pigment. Our sensation of colour depends upon the ratios of these three cone outputs.”
Principle of Univariance, concerning color vision, as stated in Lecture to a meeting of the Physiological Society at Chelsea College, London (17 Apr 1970), and reported in 'Pigments and Signals in Colour Vision', The Journal of Physiology (1972), 220 No. 3, 4P.
This whole theory of electrostatics constitutes a group of abstract ideas and general propositions, formulated in the clear and precise language of geometry and algebra, and connected with one another by the rules of strict logic. This whole fully satisfies the reason of a French physicist and his taste for clarity, simplicity and order. The same does not hold for the Englishman. These abstract notions of material points, force, line of force, and equipotential surface do not satisfy his need to imagine concrete, material, visible, and tangible things. 'So long as we cling to this mode of representation,' says an English physicist, 'we cannot form a mental representation of the phenomena which are really happening.' It is to satisfy the need that he goes and creates a model.
The French or German physicist conceives, in the space separating two conductors, abstract lines of force having no thickness or real existence; the English physicist materializes these lines and thickens them to the dimensions of a tube which he will fill with vulcanised rubber. In place of a family of lines of ideal forces, conceivable only by reason, he will have a bundle of elastic strings, visible and tangible, firmly glued at both ends to the surfaces of the two conductors, and, when stretched, trying both to contact and to expand. When the two conductors approach each other, he sees the elastic strings drawing closer together; then he sees each of them bunch up and grow large. Such is the famous model of electrostatic action imagined by Faraday and admired as a work of genius by Maxwell and the whole English school.
The employment of similar mechanical models, recalling by certain more or less rough analogies the particular features of the theory being expounded, is a regular feature of the English treatises on physics. Here is a book* [by Oliver Lodge] intended to expound the modern theories of electricity and to expound a new theory. In it are nothing but strings which move around pulleys, which roll around drums, which go through pearl beads, which carry weights; and tubes which pump water while others swell and contract; toothed wheels which are geared to one another and engage hooks. We thought we were entering the tranquil and neatly ordered abode of reason, but we find ourselves in a factory.
*Footnote: O. Lodge, Les Théories Modernes (Modern Views on Electricity) (1889), 16.
The French or German physicist conceives, in the space separating two conductors, abstract lines of force having no thickness or real existence; the English physicist materializes these lines and thickens them to the dimensions of a tube which he will fill with vulcanised rubber. In place of a family of lines of ideal forces, conceivable only by reason, he will have a bundle of elastic strings, visible and tangible, firmly glued at both ends to the surfaces of the two conductors, and, when stretched, trying both to contact and to expand. When the two conductors approach each other, he sees the elastic strings drawing closer together; then he sees each of them bunch up and grow large. Such is the famous model of electrostatic action imagined by Faraday and admired as a work of genius by Maxwell and the whole English school.
The employment of similar mechanical models, recalling by certain more or less rough analogies the particular features of the theory being expounded, is a regular feature of the English treatises on physics. Here is a book* [by Oliver Lodge] intended to expound the modern theories of electricity and to expound a new theory. In it are nothing but strings which move around pulleys, which roll around drums, which go through pearl beads, which carry weights; and tubes which pump water while others swell and contract; toothed wheels which are geared to one another and engage hooks. We thought we were entering the tranquil and neatly ordered abode of reason, but we find ourselves in a factory.
*Footnote: O. Lodge, Les Théories Modernes (Modern Views on Electricity) (1889), 16.
The Aim and Structure of Physical Theory (1906), 2nd edition (1914), trans. Philip P. Wiener (1954), 70-1.
Those who knew that the judgements of many centuries had reinforced the opinion that the Earth is placed motionless in the middle of heaven, as though at its centre, if I on the contrary asserted that the Earth moves, I hesitated for a long time whether to bring my treatise, written to demonstrate its motion, into the light of day, or whether it would not be better to follow the example of the Pythagoreans and certain others, who used to pass on the mysteries of their philosophy merely to their relatives and friends, not in writing but by personal contact, as the letter of Lysis to Hipparchus bears witness. And indeed they seem to me to have done so, not as some think from a certain jealousy of communicating their doctrines, but so that their greatest splendours, discovered by the devoted research of great men, should not be exposed to the contempt of those who either find it irksome to waste effort on anything learned, unless it is profitable, or if they are stirred by the exhortations and examples of others to a high-minded enthusiasm for philosophy, are nevertheless so dull-witted that among philosophers they are like drones among bees.
'To His Holiness Pope Paul III', in Copernicus: On the Revolutions of the Heavenly Spheres (1543), trans. A. M. Duncan (1976), 24.
Though the theories of plate tectonics now provide us with a modus operandi, they still seem to me to be a periodic phenomenon. Nothing is world-wide, but everything is episodic. In other words, the history of any one part of the earth, like the life of a soldier, consists of long periods of boredom and short periods of terror.
In The Nature of the Stratigraphical Record (1973), 100.
Throughout his last half-dozen books, for example, Arthur Koestler has been conducting a campaign against his own misunderstanding of Darwinism. He hopes to find some ordering force, constraining evolution to certain directions and overriding the influence of natural selection ... Darwinism is not the theory of capricious change that Koestler imagines. Random variation may be the raw material of change, but natural selection builds good design by rejecting most variants while accepting and accumulating the few that improve adaptation to local environments.
In The Panda’s Thumb: More Reflections in Natural History (1990, 2010), 38.
Throughout the last four hundred years, during which the growth of science had gradually shown men how to acquire knowledge of the ways of nature and mastery over natural forces, the clergy have fought a losing battle against science, in astronomy and geology, in anatomy and physiology, in biology and psychology and sociology. Ousted from one position, they have taken up another. After being worsted in astronomy, they did their best to prevent the rise of geology; they fought against Darwin in biology, and at the present time they fight against scientific theories of psychology and education. At each stage, they try to make the public forget their earlier obscurantism, in order that their present obscurantism may not be recognized for what it is.
From An Outline of Intellectual Rubbish (1937, 1943), 6. Collected in The Basic Writings of Bertrand Russell (2009), 47.
Thus a statement may be pseudoscientific even if it is eminently ‘plausible’ and everybody believes in it, and it may he scientifically valuable even if it is unbelievable and nobody believes in it. A theory may even be of supreme scientific value even if no one understands it, let alone believes it.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 1.
Thus with every advance in our scientific knowledge new elements come up, often forcing us to recast our entire picture of physical reality. No doubt, theorists would much prefer to perfect and amend their theories rather than be obliged to scrap them continually. But this obligation is the condition and price of all scientific progress.
New Perspectives in Physics (1962), 31.
To a sound judgment, the most abstract truth is the most practical. Whenever a true theory appears, it will be its own evidence. Its test is, that it will explain all phenomena.
In 'Introduction', Nature: Addresses, and Lectures (1849), 2.
To Avogadro and Cannizzaro, as to Couper and Kekulé, the molecules and atoms considered in this great theory were real objects: they were thought of the same way as one thinks of tables and chairs.
On the Operational Interpretation of Classical Chemistry', British Journal for the Philosophy of Science (1955), 6, 32. In Mary Jo Nye, From Chemical Philosophy to Theoretical Chemistry (1993), 58
To come very near to a true theory, and to grasp its precise application, are two different things, as the history of science teaches us. Everything of importance has been said before by someone who did not discover it.
In The Organisation of Thought (1917), 127. Collected in The Interpretation of Science: Selected Essays (1961), 33.
To connect the dinosaurs, creatures of interest to everyone but the veriest dullard, with a spectacular extraterrestrial event like the deluge of meteors … seems a little like one of those plots that a clever publisher might concoct to guarantee enormous sales. All the Alvarez-Raup theories lack is some sex and the involvement of the Royal family and the whole world would be paying attention to them.
In The Canberra Times (20 May 1984).
To emphasize this opinion that mathematicians would be unwise to accept practical issues as the sole guide or the chief guide in the current of their investigations, ... let me take one more instance, by choosing a subject in which the purely mathematical interest is deemed supreme, the theory of functions of a complex variable. That at least is a theory in pure mathematics, initiated in that region, and developed in that region; it is built up in scores of papers, and its plan certainly has not been, and is not now, dominated or guided by considerations of applicability to natural phenomena. Yet what has turned out to be its relation to practical issues? The investigations of Lagrange and others upon the construction of maps appear as a portion of the general property of conformal representation; which is merely the general geometrical method of regarding functional relations in that theory. Again, the interesting and important investigations upon discontinuous two-dimensional fluid motion in hydrodynamics, made in the last twenty years, can all be, and now are all, I believe, deduced from similar considerations by interpreting functional relations between complex variables. In the dynamics of a rotating heavy body, the only substantial extension of our knowledge since the time of Lagrange has accrued from associating the general properties of functions with the discussion of the equations of motion. Further, under the title of conjugate functions, the theory has been applied to various questions in electrostatics, particularly in connection with condensers and electrometers. And, lastly, in the domain of physical astronomy, some of the most conspicuous advances made in the last few years have been achieved by introducing into the discussion the ideas, the principles, the methods, and the results of the theory of functions. … the refined and extremely difficult work of Poincare and others in physical astronomy has been possible only by the use of the most elaborate developments of some purely mathematical subjects, developments which were made without a thought of such applications.
In Presidential Address British Association for the Advancement of Science, Section A, (1897), Nature, 56, 377.
To produce a really good biological theory one must try to see through the clutter produced by evolution to the basic mechanisms lying beneath them, realizing that they are likely to be overlaid by other, secondary mechanisms. What seems to physicists to be a hopelessly complicated process may have been what nature found simplest, because nature could only build on what was already there.
In What Mad Pursuit (1990), 139.
To say that, a scientific man puts forth a theory and, supports it and adheres to it, not because he thinks it true, but because he wishes it to be true, is the same thing as saying that he is not a seeker after truth at all, and is therefore a traitor to his profession.
In Reason and Belief (1910), 136.
To sum up:
1. The cosmos is a gigantic fly-wheel making 10,000 revolutions a minute.
2. Man is a sick fly taking a dizzy ride on it.
3. Religion is the theory that the wheel was designed and set spinning to give him the ride.
1. The cosmos is a gigantic fly-wheel making 10,000 revolutions a minute.
2. Man is a sick fly taking a dizzy ride on it.
3. Religion is the theory that the wheel was designed and set spinning to give him the ride.
From The Smart Set (Dec 1920), 45. Collected in 'Coda', A Mencken Chrestomathy (1949, 1956), 9.
To test a perfect theory with imperfect instruments did not impress the Greek philosophers as a valid way to gain knowledge.
The New Intelligent Man's Guide to Science (1965), Vol. 1, 12.
To the average mathematician who merely wants to know his work is securely based, the most appealing choice is to avoid difficulties by means of Hilbert's program. Here one regards mathematics as a formal game and one is only concerned with the question of consistency ... . The Realist position is probably the one which most mathematicians would prefer to take. It is not until he becomes aware of some of the difficulties in set theory that he would even begin to question it. If these difficulties particularly upset him, he will rush to the shelter of Formalism, while his normal position will be somewhere between the two, trying to enjoy the best of two worlds.
In Axiomatic Set Theory (1971), 9-15. In Thomas Tymoczko, New Directions in the Philosophy of Mathematics: an Anthology (), 11-12.
To trace the series of these revolutions, to explain their causes, and thus to connect together all the indications of change that are found in the mineral kingdom, is the proper object of a THEORY OF THE EARTH.
Illustrations of the Huttonian Theory of the Earth (1802), 2.
To turn Karl [Popper]'s view on its head, it is precisely the abandonment of critical discourse that marks the transition of science. Once a field has made the transition, critical discourse recurs only at moments of crisis when the bases of the field are again in jeopardy. Only when they must choose between competing theories do scientists behave like philosophers.
'Logic of Discovery or Psychology of Research', in I. Lakatos and A. Musgrave (eds.), Criticism and the Growth of Knowledge (1970), 6-7.
To what purpose should People become fond of the Mathematicks and Natural Philosophy? … People very readily call Useless what they do not understand. It is a sort of Revenge… One would think at first that if the Mathematicks were to be confin’d to what is useful in them, they ought only to be improv'd in those things which have an immediate and sensible Affinity with Arts, and the rest ought to be neglected as a Vain Theory. But this would be a very wrong Notion. As for Instance, the Art of Navigation hath a necessary Connection with Astronomy, and Astronomy can never be too much improv'd for the Benefit of Navigation. Astronomy cannot be without Optics by reason of Perspective Glasses: and both, as all parts of the Mathematicks are grounded upon Geometry … .
Of the Usefulness of Mathematical Learning (1699)
Today it is no longer questioned that the principles of the analysts are the more far-reaching. Indeed, the synthesists lack two things in order to engage in a general theory of algebraic configurations: these are on the one hand a definition of imaginary elements, on the other an interpretation of general algebraic concepts. Both of these have subsequently been developed in synthetic form, but to do this the essential principle of synthetic geometry had to be set aside. This principle which manifests itself so brilliantly in the theory of linear forms and the forms of the second degree, is the possibility of immediate proof by means of visualized constructions.
In Riemannsche Flächen (1906), Bd. 1, 234.
Today scientists describe the universe in terms of two basic partial theories—the general theory of relativity and quantum mechanics. They are the great intellectual achievements of the first half of this century. The general theory of relativity describes the force of gravity and the large-scale structure of the universe, that is, the structure on scales from only a few miles to as large as a million million million million (1 with twenty-four zeros after it) miles, the size of the observable universe. Quantum mechanics, on the other hand, deals with phenomena on extremely small scales, such as a millionth of a millionth of an inch. Unfortunately, however, these two theories are known to be inconsistent with each other—they cannot both be correct.
A Brief History of Time: From the Big Bang to Black Holes (1988), 11-2.
Today, the theory of evolution is an accepted fact for everyone but a fundamentalist minority, whose objections are based not on reasoning but on doctrinaire adherence to religious principles.
Molecular Biology of the Gene (1970), 2
Traditional dinosaur theory is full of short circuits. Like the antiquated wiring in an old house, the details sputter and burn out when specific parts are tested.
The Dinosaur Heresies (1986), 27.
Truly grand and powerful theories … do not and cannot rest upon single observations. Evolution is an inference from thousands of independent sources, the only conceptual structure that can make unified sense of all this disparate information. The failure of a particular claim usually records a local error, not the bankruptcy of a central theory … If I mistakenly identify your father’s brother as your own dad, you don’t become genealogically rootless and created de novo. You still have a father; we just haven’t located him properly.
Leonardo’s Mountain of Clams and the Diet of Worms: Essays on Natural History (1998), 155.
Truth travels down from the heights of philosophy to the humblest walks of life, and up from the simplest perceptions of an awakened intellect to the discoveries which almost change the face of the world. At every stage of its progress it is genial, luminous, creative. When first struck out by some distinguished and fortunate genius, it may address itself only to a few minds of kindred power. It exists then only in the highest forms of science; it corrects former systems, and authorizes new generalizations. Discussion, controversy begins; more truth is elicited, more errors exploded, more doubts cleared up, more phenomena drawn into the circle, unexpected connexions of kindred sciences are traced, and in each step of the progress, the number rapidly grows of those who are prepared to comprehend and carry on some branches of the investigation,— till, in the lapse of time, every order of intellect has been kindled, from that of the sublime discoverer to the practical machinist; and every department of knowledge been enlarged, from the most abstruse and transcendental theory to the daily arts of life.
In An Address Delivered Before the Literary Societies of Amherst College (25 Aug 1835), 16-17.
Twenty years ago many chemists would have defended the theory of bond arms as a satisfactory explanation because they had become accustomed to thinking of it as unique and as ultimate.
The Nature of Physical Reality: A Philosophy of Modern Physics (1950), 99, n.1.
Two extreme views have always been held as to the use of mathematics. To some, mathematics is only measuring and calculating instruments, and their interest ceases as soon as discussions arise which cannot benefit those who use the instruments for the purposes of application in mechanics, astronomy, physics, statistics, and other sciences. At the other extreme we have those who are animated exclusively by the love of pure science. To them pure mathematics, with the theory of numbers at the head, is the only real and genuine science, and the applications have only an interest in so far as they contain or suggest problems in pure mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
In History of European Thought in the Nineteenth Century (1903), 630.
Underneath all the various theories which are only created to be destroyed; underneath all the hypotheses which one century regards as disclosing the secret mechanism and hidden spring of the universe—and which the following century breaks to pieces as children’s toys—may be recognized the slow progress, slow but incessant, of mathematical physics.
Quote translated from 'Les Théories de l’Optique', Revue des Deux Mondes (1 May 1894), Vol. 123, 125; in 'What is Science', compiled by Mrs. H.O. Ward, in J. M. Stoddart (ed.), The New Science Review: A Miscellany of Modern Thought and Discovery (Oct 1894), Vol. 1, No. 2, 173. From the original French, “Sous les théories qui ne s’élèvent que pour être abuttues, sous les hypothèses qu’un siècle contemple comme le mécanisme secret et le ressort caché de l’Univers, et que le siècle suivant brise comme des jouets d’enfant, se poursuit le progrès lent, mais incessant, de la physique mathématique.” Note: in the New Science Review, the author is incorrectly identified as “Duhene.”
Understanding a theory has, indeed, much in common with understanding a human personality. We may know or understand a man's system of dispositions pretty well; that is to say, we may be able to predict how he would act in a number of different situations. But since there are infinitely many possible situations, of infinite variety, a full understanding of a man's dispositions does not seem to be possible.
Objective Knowledge: an Evolutionary Approach (1972), 299.
Undeterred by poverty, failure, domestic tragedy, and persecution, but sustained by his mystical belief in an attainable mathematical harmony and perfection of nature, Kepler persisted for fifteen years before finding the simple regularity [of planetary orbits] he sought… . What stimulated Kepler to keep slaving all those fifteen years? An utter absurdity. In addition to his faith in the mathematical perfectibility of astronomy, Kepler also believed wholeheartedly in astrology. This was nothing against him. For a scientist of Kepler’s generation astrology was as respectable scientifically and mathematically as the quantum theory or relativity is to theoretical physicists today. Nonsense now, astrology was not nonsense in the sixteenth century.
In The Handmaiden of the Sciences (1937), 30.

Undoubtedly, the capstone of every mathematical theory is a convincing proof of all of its assertions. Undoubtedly, mathematics inculpates itself when it foregoes convincing proofs. But the mystery of brilliant productivity will always be the posing of new questions, the anticipation of new theorems that make accessible valuable results and connections. Without the creation of new viewpoints, without the statement of new aims, mathematics would soon exhaust itself in the rigor of its logical proofs and begin to stagnate as its substance vanishes. Thus, in a sense, mathematics has been most advanced by those who distinguished themselves by intuition rather than by rigorous proofs.
As quoted in Hermann Weyl, Unterrichtsblätter für Mathematik und Naturwissenschaften (1932), 38, 177-188. As translated by Abe Shenitzer, in 'Part I. Topology and Abstract Algebra as Two Roads of Mathematical Comprehension', The American Mathematical Monthly (May 1995), 102, No. 7, 453.
Until now the theory of infinite series in general has been very badly grounded. One applies all the operations to infinite series as if they were finite; but is that permissible? I think not. Where is it demonstrated that one obtains the differential of an infinite series by taking the differential of each term? Nothing is easier than to give instances where this is not so.
As quoted and translated in Reinhold Remmert and Robert B. Burckel, Theory of Complex Functions: Readings in Mathematics (1991), 125. From the original French, “La théorie des séries infinies en général est justqu’à présent très mal fondée. On applique aux séries infinies toutes les opérations, come si elles aient finies; mais cela est-il bien permis? Je crois que non. Où est-il démonstré qu/on ontient la différentielle dune série infinie en prenant la différentiaella de chaque terme. Rien n’est plus facile que de donner des exemples où cela n’est pas juste.” In Oeuvres Complètes (1881), Vol. 2, 258.
Until now, physical theories have been regarded as merely models with approximately describe the reality of nature. As the models improve, so the fit between theory and reality gets closer. Some physicists are now claiming that supergravity is the reality, that the model and the real world are in mathematically perfect accord.
Superforce (1984, 1985), 149.
Vagueness is very much more important in the theory of knowledge than you would judge it to be from the writings of most people. Everything is vague to a degree you do not realize till you have tried to make it precise, and everything precise is so remote from everything that we normally think, that you cannot for a moment suppose that is what we really mean when we say what we think.
In The Philosophy of Logical Atomism (1918, 1919), 2.
Very simple was my explanation, and plausible enough—as most wrong theories are!
In The Time Machine (1898), 77.
Vous avez trouve par de long ennuis
Ce que Newton trouva sans sortir de chez lui.
Ce que Newton trouva sans sortir de chez lui.
In Letter to La Condamine, whose surveying expedition (after Newton’s death) had measured the arc at the equator. As Newton had calculated, the Earth was a flattened sphere, due to the effects of rotation and gravitational pull. Roughly translates as, “You have found by prolonged difficulty / What Newton found without leaving home.” In The Royal Society Newton Tercentenary Celebrations: 15-19 July 1946 (1946), 15. [Newton was born in 1642; the tercentenary celebration was delayed by WW II —Webmaster.]
Wallace’s error on human intellect arose from the in adequacy of his rigid selectionism, not from a failure to apply it. And his argument repays our study today, since its flaw persists as the weak link in many of the most ‘modern’ evolutionary speculations of our current literature. For Wallace’s rigid selectionism is much closer than Darwin’s pluralism to the attitude embodied in our favored theory today, which, ironically in this context, goes by the name of ‘Neo-Darwinism.’
…...
We all agreed that your theory is crazy. The question which divides us is whether it is crazy enough to have a chance of being correct.
In The Ambidextrous Universe by Martin Gardner (1964).
We believe in the possibility of a theory which is able to give a complete description of reality, the laws of which establish relations between the things themselves and not merely between their probabilities ... God does not play dice.
…...
We called the new [fourth] quark the “charmed quark” because we were pleased, and fascinated by the symmetry it brought to the subnuclear world. “Charm” also means a “a magical device to avert evil,” and in 1970 it was realized that the old three quark theory ran into very serious problems. ... As if by magic the existence of the charmed quark would [solve those problems].
From asppearance in the BBC-TV program written by Nigel Calder, 'The Key to the Universe,' (27 Jan 1977). As cited in Arthur Lewis Caso, 'The Production of New Scientific Terms', American Speech (Summer 1980), 55, No. 2, 102.
We can invent as many theories we like, and any one of them can be made to fit the facts. But that theory is always preferred which makes the fewest number of assumptions.
From interview by S.J. Woolf, 'Einstein’s Own Corner of Space’, New York Times (18 Aug 1929), Sunday Magazine, 2.
We cannot but think there is something like a fallacy in Mr. Buckle’s theory that the advance of mankind is necessarily in the direction of science, and not in that of morals.
In 'Dante', The Writings of James Russell Lowell: Literary Essays (1890), 254. [The reclusive Henry Thomas Buckle (1821-1862) began writing his History of Civilization, trying to construct a science of society on the basis of inductions from history. —Webmaster]
We cannot see how the evidence afforded by the unquestioned progressive development of organised existence—crowned as it has been by the recent creation of the earth's greatest wonder, MAN, can be set aside, or its seemingly necessary result withheld for a moment. When Mr. Lyell finds, as a witty friend lately reported that there had been found, a silver-spoon in grauwacke, or a locomotive engine in mica-schist, then, but not sooner, shall we enrol ourselves disciples of the Cyclical Theory of Geological formations.
Review of Murchison's Silurian System, Quarterly Review (1839), 64, 112-3.
We come finally, however, to the relation of the ideal theory to real world, or “real” probability. If he is consistent a man of the mathematical school washes his hands of applications. To someone who wants them he would say that the ideal system runs parallel to the usual theory: “If this is what you want, try it: it is not my business to justify application of the system; that can only be done by philosophizing; I am a mathematician”. In practice he is apt to say: “try this; if it works that will justify it”. But now he is not merely philosophizing; he is committing the characteristic fallacy. Inductive experience that the system works is not evidence.
In A Mathematician’s Miscellany (1953). Reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 73.
We debase the richness of both nature and our own minds if we view the great pageant of our intellectual history as a compendium of new in formation leading from primal superstition to final exactitude. We know that the sun is hub of our little corner of the universe, and that ties of genealogy connect all living things on our planet, because these theories assemble and explain so much otherwise disparate and unrelated information–not because Galileo trained his telescope on the moons of Jupiter or because Darwin took a ride on a Galápagos tortoise.
…...
We don't know what we are talking about. Many of us believed that string theory was a very dramatic break with our previous notions of quantum theory. But now we learn that string theory, well, is not that much of a break. The state of physics today is like it was when we were mystified by radioactivity. They were missing something absolutely fundamental. We are missing perhaps something as profound as they were back then.
Closing address to the 23rd Solvay Conference in Physics, Brussels, Belgium (Dec 2005). Quoted in Ashok Sengupta, Chaos, Nonlinearity, Complexity: The Dynamical Paradigm of Nature (2006), vii. Cite in Alfred B. Bortz, Physics: Decade by Decade (2007), 206.
We have decided to call the entire field of control and communication theory, whether in the machine or in the animal, by the name Cybernetics, which we form from the Greek … for steersman. In choosing this term, we wish to recognize that the first significant paper on feedback mechanisms is an article on governors, which was published by Clerk Maxwell in 1868, and that governor is derived from a Latin corruption … We also wish to refer to the fact that the steering engines of a ship are indeed one of the earliest and best-developed forms of feedback mechanisms.
In Cybernetics (1948), 19.
We have found that where science has progressed the farthest, the mind has but regained from nature that which the mind has put into nature.
We have found a strange foot-print on the shores of the unknown. We have devised profound theories, one after another, to account for its origin. At last, we have succeeded in reconstructing the creature that made the foot-print. And Lo! it is our own.
We have found a strange foot-print on the shores of the unknown. We have devised profound theories, one after another, to account for its origin. At last, we have succeeded in reconstructing the creature that made the foot-print. And Lo! it is our own.
Concluding sentences in Space, Time and Gravitation: An Outline of the General Relativity Theory (1921), 200-201
We have here no esoteric theory of the ultimate nature of concepts, nor a philosophical championing of the primacy of the 'operation'. We have merely a pragmatic matter, namely that we have observed after much experience that if we want to do certain kinds of things with our concepts, our concepts had better be constructed in certain ways. In fact one can see that the situation here is no different from what we always find when we push our analysis to the limit; operations are not ultimately sharp or irreducible any more than any other sort of creature. We always run into a haze eventually, and all our concepts are describable only in spiralling approximation.
In Reflections of a Physicist (1950), 9.
We have long been seeking a different kind of evolutionary process and have now found one; namely, the change within the pattern of the chromosomes. ... The neo-Darwinian theory of the geneticists is no longer tenable.
The Material Basis of Evolution (1940), 397.
We have the satisfaction to find, that in nature there is wisdom, system and consistency. For having, in the natural history of this earth, seen a succession of worlds, we may from this conclude that, there is a system in nature; in like manner as, from seeing revolutions of the planets, it is concluded, that there is a system by which they are intended to continue those revolutions. But if the succession of worlds is established in the system of nature, it is vain to look for anything higher in the origin of the earth. The result, therefore, of our present enquiry is, that we find no vestige of a beginning,-no prospect of an end.
'Theory of the Earth', Transactions of the Royal Society of Edinburgh, 1788, 1, 304.
We have theories of races and of functions, but scarcely yet a remote approach to an idea of creation. We are now so far from the road to truth, that religious teachers dispute and hate each other, and speculative men are esteemed unsound and frivolous.
In 'Introduction', Nature: Addresses, and Lectures (1849), 2.
We have, through our play schools, attempted to fit our children to enjoy life by feeding them upon the pap manufactured by theoretical educators possessing little knowledge of the vital sciences of life.
Co-author with Louis Jay Heath, in A New Basis for Social Progress (1917), 152.
We hold these truths to be self-evident.
Franklin's edit to the assertion of religion in Thomas Jefferson's original wording, “We hold these truths to be sacred and undeniable” in a draft of the Declaration of Independence changes it instead into an assertion of rationality. The scientific mind of Franklin drew on the scientific determinism of Isaac Newton and the analytic empiricism of David Hume and Gottfried Leibniz. In what became known as “Hume's Fork” the latters' theory distinguished between synthetic truths that describe matters of fact, and analytic truths that are self-evident by virtue of reason and definition.
Franklin's edit to the assertion of religion in Thomas Jefferson's original wording, “We hold these truths to be sacred and undeniable” in a draft of the Declaration of Independence changes it instead into an assertion of rationality. The scientific mind of Franklin drew on the scientific determinism of Isaac Newton and the analytic empiricism of David Hume and Gottfried Leibniz. In what became known as “Hume's Fork” the latters' theory distinguished between synthetic truths that describe matters of fact, and analytic truths that are self-evident by virtue of reason and definition.
As explained by Walter Isaacson in Benjamin Franklin: An American Life (2004), 312.
We lay down a fundamental principle of generalization by abstraction: The existence of analogies between central features of various theories implies the existence of a general theory which underlies the particular theories and unifies them with respect to those central features.
Introduction to a Form of General Analysis (1910), Preface, 1.
We may affirm of Mr. Buffon, that which has been said of the chemists of old; though he may have failed in attaining his principal aim, of establishing a theory, yet he has brought together such a multitude of facts relative to the history of the earth, and the nature of its fossil productions, that curiosity finds ample compensation, even while it feels the want of conviction.
In History of the Earth and Animated Nature (1774, 1847), Vol. 1, 73.
We may as well cut out group theory. That is a subject that will never be of any use in physics.
Discussing mathematics curriculum reform at Princeton University (1910), as quoted in Abraham P. Hillman, Gerald L. Alexanderson, Abstract Algebra: A First Undergraduate Course (1994), 94.
We may be sure, that if Lyell were now living he would frankly recognize new facts, as soon as they were established, and would not shrink from any modification of his theory which these might demand. Great as were his services to geology, this, perhaps, is even greater—for the lesson applies to all sciences and to all seekers alter knowledge—that his career, from first to lost, was the manifestation of a judicial mind, of a noble spirit, raised far above all party passions and petty considerations, of an intellect great in itself, but greater still in its grand humility; that he was a man to whom truth was as the “pearl of price,” worthy of the devotion and, if need be, the sacrifice of a life.
Conclusion in Charles Lyell and Modern Geology (1895), 213.
We may be well be justified in saying that quantum theory is of greater importance to chemistry than physics. For where there are large fields of physics that can be discussed in a completely penetrating way without reference to Planck's constant and to quantum theory at all, there is no part of chemistry that does not depend, in its fundamental theory, upon quantum principles.
As quoted in Leonard W. Fine, Chemistry (1972), 537. Please contact Webmaster if you know the primary source.
We may see how unexpectedly recondite parts of pure mathematics may bear upon physical science, by calling to mind the circumstance that Fresnel obtained one of the most curious confirmations of the theory (the laws of Circular Polarization by reflection) through an interpretation of an algebraical expression, which, according to the original conventional meaning of the symbols, involved an impossible quantity.
In History of Scientific Ideas, Bk. 2, chap. 14, sect. 8.
We must alter theory to adapt it to nature, but not nature to adapt it to theory.
An Introduction to the Study of Experimental Medicine (1865, translation 1927, 1957), 39.
We must keep our freedom of mind, … and must believe that in nature what is absurd, according to our theories, is not always impossible.
In An Introduction to the Study of Experimental Medicine (1927, 1957), 38, as translated by Henry Copley Greene. From the original French by Claude Bernard: “Il faut garder sa liberté d'esprit, et croire que dans la nature l'absurde suivant nos théories n'est pas toujours impossible.” (1865), 67. A Google translation gives: “You have to keep your freedom of mind, … and believe that in nature the absurd according to our theories is not always impossible.”
We must make the following remark: a proof, that after a certain time t1, the spheres must necessarily be mixed uniformly, whatever may be the initial distribution of states, cannot be given. This is in fact a consequence of probability theory, for any non-uniform distribution of states, no matter how improbable it may be, is still not absolutely impossible. Indeed it is clear that any individual uniform distribution, which might arise after a certain time from some particular initial state, is just as improbable as an individual non-uniform distribution; just as in the game of Lotto, any individual set of five numbers is as improbable as the set 1, 2, 3, 4, 5. It is only because there are many more uniform distributions than non-uniform ones that the distribution of states will become uniform in the course of time. One therefore cannot prove that, whatever may be the positions and velocities of the spheres at the beginning, the distributions must become uniform after a long time; rather one can only prove that infinitely many more initial states will lead to a uniform one after a definite length of time than to a non-uniform one. Loschmidt's theorem tells us only about initial states which actually lead to a very non-uniform distribution of states after a certain time t1; but it does not prove that there are not infinitely many more initial conditions that will lead to a uniform distribution after the same time. On the contrary, it follows from the theorem itself that, since there are infinitely many more uniform distributions, the number of states which lead to uniform distributions after a certain time t1, is much greater than the number that leads to non-uniform ones, and the latter are the ones that must be chosen, according to Loschmidt, in order to obtain a non-uniform distribution at t1.
From 'On the Relation of a General Mechanical Theorem to the Second Law of Thermodynamics' (1877), in Stephen G. Brush (ed.), Selected Readings in Physics (1966), Vol. 2, Irreversible Processes, 191-2.
We must trust our observations or our theories only after experimental verification. If we trust too much, the mind becomes bound and cramped by the results of its own reasoning; it no longer has freedom of action, and so lacks the power to break away from that blind faith in theories which is only scientific superstition.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 37.
We need nothing to explain behavior but the ordinary laws of physics and chemistry. There are many things we cannot explain in behavior just as there are many things we cannot explain in physics and chemistry, but where objectively verifiable experimentation ends, hypothesis, and later theory, begin. But even theories and hypotheses must be couched in terms of what is already known about physical and chemical processes. … The Behaviorist cannot find consciousness in the test-tube of his science.
In The Battle Of Behaviorism: An Exposition and an Exposure (1927), 26.
We now realize with special clarity, how much in error are those theorists who believe that theory comes inductively from experience.
In section 3, 'The Field Concept', Physics and Reality (1936), collected in Essays in Physics (1950), 28.
We regard as 'scientific' a method based on deep analysis of facts, theories, and views, presupposing unprejudiced, unfearing open discussion and conclusions. The complexity and diversity of all the phenomena of modern life, the great possibilities and dangers linked with the scientific-technical revolution and with a number of social tendencies demand precisely such an approach, as has been acknowledged in a number of official statements.
Progress, Coexistence and Intellectual Freedom (1968), 25.
We see that each surface is really a pair of surfaces, so that, where they appear to merge, there are really four surfaces. Continuing this process for another circuit, we see that there are really eight surfaces etc and we finally conclude that there is an infinite complex of surfaces, each extremely close to one or the other of two merging surfaces.
An intermediate thought about some surface in state space, while evolving his Prototype Model of Chaos. In 'Deterministic Nonperiodic Flow', Journal of Atmospheric Science (1963), 20, 130–141. As quoted and cited in: T.N. Palmer, 'Edward Norton Lorenz: 23 May 1917—16 April 2008', Biographical Memoirs of Fellows of the Royal Society (2009) 55, 144.
We see, then, that the elements of the scientific method are interrelated. Facts are necessary materials; but their working up by experimental reasoning, i.e., by theory, is what establishes and really builds up science. Ideas, given form by facts, embody science. A scientific hypothesis is merely a scientific idea, preconceived or previsioned. A theory is merely a scientific idea controlled by experiment. Reasoning merely gives a form to our ideas, so that everything, first and last, leads back to an idea. The idea is what establishes, as we shall see, the starting point or the primum movens of all scientific reasoning, and it is also the goal in the mind's aspiration toward the unknown.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 26.
We shall not have a complete theory until we can do more than merely say that “Things are as they are because they were as they were.”
From Inaugural Lecture (29 Apr 1980) as Lucasian Professor at Cambridge University, 'Is the End in Sight for Theoretical Physics?', collected in Black Holes and Baby Universes and Other Essays (1993), 51.
We should admit in theory what is already very largely a case in practice, that the main currency of scientific information is the secondary sources in the forms of abstracts, reports, tables, &c., and that the primary sources are only for detailed reference by very few people. It is possible that the fate of most scientific papers will be not to be read by anyone who uses them, but with luck they will furnish an item, a number, some facts or data to such reports which may, but usually will not, lead to the original paper being consulted. This is very sad but it is the inevitable consequence of the growth of science. The number of papers that can be consulted is absolutely limited, no more time can be spent in looking up papers, by and large, than in the past. As the number of papers increase the chance of any one paper being looked at is correspondingly diminished. This of course is only an average, some papers may be looked at by thousands of people and may become a regular and fixed part of science but most will perish unseen.
'The Supply of Information to the Scientist: Some Problems of the Present Day', The Journal of Documentation, 1957, 13, 195.
Weierstrass related … that he followed Sylvester’s papers on the theory of algebraic forms very attentively until Sylvester began to employ Hebrew characters. That was more than he could stand and after that he quit him.
From Naturwissenschaftliche Rundschau (1897), 12 (1897), 361. As cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 180.
Well-established theories collapse under the weight of new facts and observations which cannot be explained, and then accumulate to the point where the once useful theory is clearly obsolete.
[Using Thomas S. Kuhn's theories to frame his argument about the relationship beween science and technology: as new facts continue to accumulate, a new, more accurate paradigm must replace the old one.]
[Using Thomas S. Kuhn's theories to frame his argument about the relationship beween science and technology: as new facts continue to accumulate, a new, more accurate paradigm must replace the old one.]
— Al Gore
Commencement address at M.I.T. (7 Jun 1996). In obituary, 'Thomas S. Kuhn', The Tech (26 Jun 1996), 9.
Well-observed facts, though brought to light by passing theories, will never die; they are the material on which alone the house of science will at last be built.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 165.
Well, evolution is a theory. It is also a fact. And facts and theories are different things, not rungs in a hierarchy of increasing certainty. Facts are the world’s data. Theories are structures of ideas that explain and interpret facts. Facts do not go away while scientists debate rival theories for explaining them. Einstein’s theory of gravitation replaced Newton’s, but apples did not suspend themselves in mid-air pending the outcome. And human beings evolved from apelike ancestors whether they did so by Darwin’s proposed mechanism or by some other, yet to be discovered … Evolutionists make no claim for perpetual truth, though creationists often do (and then attack us for a style of argument that they themselves favor).
'Evolution as Fact and Theory', in Hen’s Teeth and Horse’s Toes: Further Reflections in Natural History (1983), 254-255.
Well, it [evolution] is a theory, it is a scientific theory only, and it has in recent years been challenged in the world of science and is not yet believed in the scientific community to be as infallible as it once was believed. But if it was going to be taught in the schools, then I think that also the biblical theory of creation, which is not a theory but the biblical story of creation, should also be taught.
Responding to a question, during his 1980 Presidential Campaign, whether he agreed with the teaching of evolution in schools. Quoted in New Scientist (9 Oct 1980), 136.
Were I disposed to consider the comparative merit of each of them [facts or theories in medical practice], I should derive most of the evils of medicine from supposed facts, and ascribe all the remedies which have been uniformly and extensively useful, to such theories as are true. Facts are combined and rendered useful only by means of theories, and the more disposed men are to reason, the more minute and extensive they become in their observations.
Quoted in John Edmonds Stock, Memoirs of the life of Thomas Beddoes (1810), 401.
What has been learned in physics stays learned. People talk about scientific revolutions. The social and political connotations of revolution evoke a picture of a body of doctrine being rejected, to be replaced by another equally vulnerable to refutation. It is not like that at all. The history of physics has seen profound changes indeed in the way that physicists have thought about fundamental questions. But each change was a widening of vision, an accession of insight and understanding. The introduction, one might say the recognition, by man (led by Einstein) of relativity in the first decade of this century and the formulation of quantum mechanics in the third decade are such landmarks. The only intellectual casualty attending the discovery of quantum mechanics was the unmourned demise of the patchwork quantum theory with which certain experimental facts had been stubbornly refusing to agree. As a scientist, or as any thinking person with curiosity about the basic workings of nature, the reaction to quantum mechanics would have to be: “Ah! So that’s the way it really is!” There is no good analogy to the advent of quantum mechanics, but if a political-social analogy is to be made, it is not a revolution but the discovery of the New World.
From Physics Survey Committee, U.S. National Academy of Sciences, National
Research Council, 'The Nature of Physics', in report Physics in Perspective (1973), 61-62. As cited in I. Bernard Cohen, Revolution in Science (1985), 554-555.
What I know is, not that such a thing [scientific theory] is true, but that the best course for me is to act as if it were true.
Expressing the merit of pragmatism, in La Science et l’Hypothèse (1901, 1908), 218, as translated in Henri Poincaré and William John Greenstreet (trans.), Science and Hypothesis (1902, 1905), 187. From the original French, “Ce que je sais, ce n’est pas que telle chose est vraie, mais que le mieux pour moi est encore d’agir comme si elle était vraie.” [In the article on Poincaré in Encyclopedia of World Biography: Orozco-Radisson, (2nd ed., 1998), 366, a loose translation of Poincaré’s views is given as, “One does not ask whether a scientific theory is true, but only whether it is convenient.” Although that was enclosed in quotation marks in the encyclopedia article, Webmaster has so far not found a verbatim match in the original French edition, and suspects it is merely the article author’s summary of Poincaré’s views—a valid interpretation, but not verbatim. —Webmaster]
What I remember most clearly was that when I put down a suggestion that seemed to me cogent and reasonable, Einstein did not in the least contest this, but he only said, 'Oh, how ugly.' As soon as an equation seemed to him to be ugly, he really rather lost interest in it and could not understand why somebody else was willing to spend much time on it. He was quite convinced that beauty was a guiding principle in the search for important results in theoretical physics.
quoted in Fearful Symmetry: The Search for Beauty in Modern Physics (1987)
What is important is the gradual development of a theory, based on a careful analysis of the ... facts. ... Its first applications are necessarily to elementary problems where the result has never been in doubt and no theory is actually required. At this early stage the application serves to corroborate the theory. The next stage develops when the theory is applied to somewhat more complicated situations in which it may already lead to a certain extent beyond the obvious and familiar. Here theory and application corroborate each other mutually. Beyond lies the field of real success: genuine prediction by theory. It is well known that all mathematized sciences have gone through these successive stages of evolution.
'Formulation of the Economic Problem' in Theory of Games and Economic Behavior (1964), 8. Reprinted in John Von Neumann, F. Bródy (ed.) and Tibor Vámos (ed.), The Neumann Compendium (2000), 416.
What makes the theory of relativity so acceptable to physicists in spite of its going against the principle of simplicity is its great mathematical beauty. This is a quality which cannot be defined, any more than beauty in art can be defined, but which people who study mathematics usually have no difficulty in appreciating. … The restricted theory changed our ideas of space and time in a way that may be summarised by stating that the group of transformations to which the space-time continuum is subject must be changed from the Galilean group to the Lorentz group.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 123.
What nature demands of us is not a quantum theory or a wave theory, instead nature demands of us a synthesis both conceptions, which, to be sure, until now still exceeds the powers of thought of the physicists.
Concluding remark in Lecture (23 Feb 1927) to Mathematisch-physikalische Arbeitsgemeinschaft, University of Berlin, reported in 'Theoretisches und Experimentelles zur Frage der Lichtentstehung', Zeitschr. f. ang. Chem., 40, 546. As translated and cited in Arthur I. Miller, Sixty-Two Years of Uncertainty: Historical, Philosophical, and Physical Inquiries into the Foundations of Quantum Mechanics (2012), 89 & 108.
What renders a problem definite, and what leaves it indefinite, may best be understood from mathematics. The very important idea of solving a problem within limits of error is an element of rational culture, coming from the same source. The art of totalizing fluctuations by curves is capable of being carried, in conception, far beyond the mathematical domain, where it is first learnt. The distinction between laws and co-efficients applies in every department of causation. The theory of Probable Evidence is the mathematical contribution to Logic, and is of paramount importance.
In Education as a Science (1879), 151-152.
What the founders of modern science, among them Galileo, had to do, was not to criticize and to combat certain faulty theories, and to correct or to replace them by better ones. They had to do something quite different. They had to destroy one world and to replace it by another. They had to reshape the framework of our intellect itself, to restate and to reform its concepts, to evolve a new approach to Being, a new concept of knowledge, a new concept of science—and even to replace a pretty natural approach, that of common sense, by another which is not natural at all.
In 'Galileo and Plato', Journal of the History of Ideas (1943), 405.
What, then, is light according to the electromagnetic theory? It consists of alternate and opposite rapidly recurring transverse magnetic disturbances, accompanied with electric displacements, the direction of the electric displacement being at the right angles to the magnetic disturbance, and both at right angles to the direction of the ray.
'A Dynamical Theory of the Electromagnetic Field' (1864). In W. D. Niven (ed.), The Scientific Letters and Papers of James Clerk Maxwell(1890), Vol. 2, 1862-1973, 195.
Whatever may happen to the latest theory of Dr. Einstein, his treatise represents a mathematical effort of overwhelming proportions. It is the more remarkable since Einstein is primarily a physicist and only incidentally a mathematician. He came to mathematics rather of necessity than by predilection, and yet he has here developed mathematical formulae and calculations springing from a colossal knowledge.
In 'Marvels at Einstein For His Mathematics', New York Times (4 Feb 1929), 3.
Wheeler hopes that we can discover, within the context of physics, a principle that will enable the universe to come into existence “of its own accord.” In his search for such a theory, he remarks: “No guiding principle would seem more powerful than the requirement that it should provide the universe with a way to come into being.” Wheeler likened this 'self-causing' universe to a self-excited circuit in electronics.
In God and the New Physics (1984), 39. Wheeler quotation footnoted 'From the Black Hole', in H. Woolf (Ed.),Some Strangeness in the Proportion (1980).
When an investigator has developed a formula which gives a complete representation of the phenomena within a certain range, he may be prone to satisfaction. Would it not be wiser if he should say “Foiled again! I can find out no more about Nature along this line.”
Quoted in Astro-Physical Journal, 1945, 101, 133.
When an observation is made on any atomic system that has been prepared in a given way and is thus in a given state, the result will not in general be determinate, i.e. if the experiment is repeated several times under identical conditions several different results may be obtained. If the experiment is repeated a large number of times it will be found that each particular result will be obtained a definite fraction of the total number of times, so that one can say there is a definite probability of its being obtained any time that the experiment is performed. This probability the theory enables one to calculate. (1930)
The Principles of Quantum Mechanics 4th ed. (1981), 13-14
When Big Bang proponents make assertions such as … “the evidence taken together … hangs together beautifully,” they overlook observational facts that have been piling up for 25 years and that have now become overwhelming. Of course, if one ignores contradictory observations, one can claim to have an “elegant” or “robust” theory. But it isn’t science.
In 'Letters: Wrangling Over the Bang', Science News (27 Jul 1991), 140, No. 4, 51. Also quoted in Roy C. Martin, Astronomy on Trial: A Devastating and Complete Repudiation of the Big Bang Fiasco (1999), Appendix I, 217.
When Einstein has criticized quantum theory he has done so from the basis of dogmatic realism.
In Physics and Philosophy: The Revolution in Modern Science (1958), 82.
When facts are insufficient, theorizing is ridiculous at best, misleading at worst.
The Queen of Air and Darkness (1971). Quoted in Gary Westfahl, Science Fiction Quotations (2005), 322.
When Faraday filled space with quivering lines of force, he was bringing mathematics into electricity. When Maxwell stated his famous laws about the electromagnetic field it was mathematics. The relativity theory of Einstein which makes gravity a fiction, and reduces the mechanics of the universe to geometry, is mathematical research.
In 'The Spirit of Research', III, 'Mathematical Research', in The Monist (Oct 1922), 32, No. 4, 542-543.
When I worked on the polio vaccine, I had a theory. Experiments were done to determine what might or might not occur. I guided each one by imagining myself in the phenomenon in which I was interested. The intuitive realm is constantly active—the realm of imagination guides my thinking.
From interview with James Reston, Jr., in Pamela Weintraub (ed.), The Omni Interviews (1984), 98. Previously published in magazine, Omni (May 1982).
When physicists speak of “beauty” in their theories, they really mean that their theory possesses at least two essential features: 1. A unifying symmetry 2. The ability to explain vast amounts of experimental data with the most economical mathematical expressions.
In 'Quantum Heresy', Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth Dimension (1995), 127.
When Ramanujan was sixteen, he happened upon a copy of Carr’s Synopsis of Mathematics. This chance encounter secured immortality for the book, for it was this book that suddenly woke Ramanujan into full mathematical activity and supplied him essentially with his complete mathematical equipment in analysis and number theory. The book also gave Ramanujan his general direction as a dealer in formulas, and it furnished Ramanujan the germs of many of his deepest developments.
In Mathematical Circles Squared (1972), 158. George Shoobridge Carr (1837-1914) wrote his Synopsis of Elementary Results in Mathematics in 1886.
When silhouetted against historical background Maxwell’s electromagnetic theory and its remarkable experimental confirmation by Hertz loomed up as large to the physicist of 1895 as the de Broglie-Schrödinger wave theory of matter and its experimental confirmation by Davison and Germer does to the physicist of to-day. [1931]
In 'The Romance of the Next Decimal Place', Science (1 Jan 1932), 75, No. 1931, 2.
When someone admits one and rejects another which is equally in accordance with the appearances, it is clear that he has quitted all physical explanation and descended into myth.
— Epicurus
Letter to Pythocles, 87. Trans. R. W. Sharples.
When the 1880s began. Maxwell’s theory was virtually a trackless jungle. By the second half of the decade, guided by the principle of energy flow. Poynting, FitzGerald, and above all Heaviside had succeeded in taming and pruning that jungle and in rendering it almost civilized.
In The Maxwellians (2008), 128.
When we meet a fact which contradicts a prevailing theory, we must accept the fact and abandon the theory, even when the theory is supported by great names and generally accepted.
From An Introduction to the Study of Experimental Medicine (1927, 1957), 164, as translated by Henry Copley Greene. From the original French by Claude Bernard: “Quand le fait qu’on rencontre est en opposition avec une théorie régnante, il faut accepter le fait et abandonner la théorie, lors même que celle-ci, soutenue par de grands noms, est généralement adoptée.” (1865), 287-288. A Google translation gives: “When the fact one encounters is in opposition to a reigning theory, one must accept the fact and abandon the theory, even when the latter, supported by great names, is generally adopted.”
When you are famous it is hard to work on small problems. This is what did [Claude Elwood] Shannon in. After information theory, what do you do for an encore? The great scientists often make this error. They fail to continue to plant the little acorns from which the mighty oak trees grow. They try to get the big thing right off. And that isn’t the way things go. So that is another reason why you find that when you get early recognition it seems to sterilize you.
'You and Your Research', Bell Communications Research Colloquium Seminar, 7 Mar 1986.
When... the biologist is confronted with the fact that in the organism the parts are so adapted to each other as to give rise to a harmonious whole; and that the organisms are endowed with structures and instincts calculated to prolong their life and perpetuate their race, doubts as to the adequacy of a purely physiochemical viewpoint in biology may arise. The difficulties besetting the biologist in this problem have been rather increased than diminished by the discovery of Mendelian heredity, according to which each character is transmitted independently of any other character. Since the number of Mendelian characters in each organism is large, the possibility must be faced that the organism is merely a mosaic of independent hereditary characters. If this be the case the question arises: What moulds these independent characters into a harmonious whole? The vitalist settles this question by assuming the existence of a pre-established design for each organism and of a guiding 'force' or 'principle' which directs the working out of this design. Such assumptions remove the problem of accounting for the harmonious character of the organism from the field of physics or chemistry. The theory of natural selection invokes neither design nor purpose, but it is incomplete since it disregards the physiochemical constitution of living matter about which little was known until recently.
The Organism as a Whole: From a Physiochemical Viewpoint (1916), v-vi.
Where theory lags behind the facts, we are dealing with miserable degenerating research programmes.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 6.
Wherever possible, scientists experiment. Which experiments suggest themselves often depends on which theories currently prevail. Scientists are intent of testing those theories to the breaking point. They do not trust what is intuitively obvious. That the Earth is flat was once obvious. That heavy bodies fall faster than light ones was once obvious. That bloodsucking leeches cure most diseases was once obvious. That some people are naturally and by divine decree slaves was once obvious. That there is such a place as the center of the Universe, and that the Earth sits in that exalted spot was once obvious. That there is an absolute standard of rest was once obvious. The truth may be puzzling or counterintuitive. It may contradict deeply held beliefs. Experiment is how we get a handle on it.
In The Demon-Haunted World: Science As A Candle in the Dark (1995), 36.
Whether statistics be an art or a science... or a scientific art, we concern ourselves little. It is the basis of social and political dynamics, and affords the only secure ground on which the truth or falsehood of the theories and hypotheses of that complicated science can be brought to the test.
Letters on the Theory of Probabilities (1846), trans. O. G. Downes (1849).
Whether you can observe a thing or not depends on the theory which you use. It is the theory which decides what can be observed.
Quoted in Werner Heisenberg, Physics and Beyond: Encounters and Conversations (1971), 77.
Who … is not familiar with Maxwell’s memoirs on his dynamical theory of gases? … from one side enter the equations of state; from the other side, the equations of motion in a central field. Ever higher soars the chaos of formulae. Suddenly we hear, as from kettle drums, the four beats “put n=5.” The evil spirit v vanishes; and … that which had seemed insuperable has been overcome as if by a stroke of magic … One result after another follows in quick succession till at last … we arrive at the conditions for thermal equilibrium together with expressions for the transport coefficients.
In Ceremonial Speech (15 Nov 1887) celebrating the 301st anniversary of the Karl-Franzens-University Graz. Published as Gustav Robert Kirchhoff: Festrede zur Feier des 301. Gründungstages der Karl-Franzens-Universität zu Graz (1888), 29, as translated in Michael Dudley Sturge, Statistical and Thermal Physics (2003), 343. A more complete alternate translation also appears on the Ludwig Boltzmann Quotes page of this website.
Who could believe an ant in theory?
A giraffe in blueprint?
Ten thousand doctors of what’s possible
Could reason half the jungle out of being.
A giraffe in blueprint?
Ten thousand doctors of what’s possible
Could reason half the jungle out of being.
In The Achievement of John Ciardi: A Comprehensive Selection of His Poems (1969), 73.
Who does not know Maxwell’s dynamic theory of gases? At first there is the majestic development of the variations of velocities, then enter from one side the equations of condition and from the other the equations of central motions, higher and higher surges the chaos of formulas, suddenly four words burst forth: “Put n = 5.” The evil demon V disappears like the sudden ceasing of the basso parts in music, which hitherto wildly permeated the piece; what before seemed beyond control is now ordered as by magic. There is no time to state why this or that substitution was made, he who cannot feel the reason may as well lay the book aside; Maxwell is no program-musician who explains the notes of his composition. Forthwith the formulas yield obediently result after result, until the temperature-equilibrium of a heavy gas is reached as a surprising final climax and the curtain drops.
In Ceremonial Speech (15 Nov 1887) celebrating the 301st anniversary of the Karl-Franzens-University Graz. Published as Gustav Robert Kirchhoff: Festrede zur Feier des 301. Gründungstages der Karl-Franzens-Universität zu Graz (1888), 29-30, as translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 187. From the original German, “Wer kennt nicht seine dynamische Gastheorie? – Zuerst entwickeln sich majestätisch die Variationen der Geschwindigkeiten, dann setzen von der einen Seite die Zustands-Gleichungen, von der anderen die Gleichungen der Centralbewegung ein, immer höher wogt das Chaos der Formeln; plötzlich ertönen die vier Worte: „Put n=5.“Der böse Dämon V verschwindet, wie in der Musik eine wilde, bisher alles unterwühlende Figur der Bässe plötzlich verstummt; wie mit einem Zauberschlage ordnet sich, was früher unbezwingbar schien. Da ist keine Zeit zu sagen, warum diese oder jene Substitution gemacht wird; wer das nicht fühlt, lege das Buch weg; Maxwell ist kein Programmmusiker, der über die Noten deren Erklärung setzen muss. Gefügig speien nun die Formeln Resultat auf Resultat aus, bis überraschend als Schlusseffect noch das Wärme-Gleichgewicht eines schweren Gases gewonnen wird und der Vorhang sinkt.” A condensed alternate translation also appears on the Ludwig Boltzmann Quotes page of this website.
Why has not anyone seen that fossils alone gave birth to a theory about the formation of the earth, that without them, no one would have ever dreamed that there were successive epochs in the formation of the globe.
Discours sur les révolutions du globe, (Discourse on the Revolutions of the Surface of the Globe), originally the introduction to Recherches sur les ossemens fossiles des quadrupèdes (1812). Translated by Ian Johnston from the 1825 edition. Online at Vancouver island University website.
With accurate experiment and observation to work upon, imagination becomes the architect of physical theory.
In discourse delivered before the British Association at Liverpool (16 Sep 1870), 'Scientific Use of the Imagination', collected in Fragments of Science: a Series of Detached Essays, Addresses and Reviews (1892), Vol. 2, 104.
With all reserve we advance the view that a supernova represents the transition of an ordinary star into a neutron star consisting mainly of neutrons. Such a star may possess a very small radius and an extremely high density. As neutrons can be packed much more closely than ordinary nuclei and electrons, the gravitational packing energy in a cold neutron star may become very large, and under certain conditions may far exceed the ordinary nuclear packing fractions...
[Co-author with Walter Baade]
[Co-author with Walter Baade]
Paper presented to American Physical Society meeting at Stanford (15-16 Dec 1933). Published in Physical Review (15 Jan 1934). Cited in P. Haensel, Paweł Haensel and A. Y. Potekhin, D. G. Yakovlev, Neutron Stars: Equation of State and Structure (2007), 2-3. Longer version of quote from Freeman Dyson, From Eros to Gaia (1992), 34. The theoretical prediction of neutron stars was made after analyzing observations of supernovae and proposed as an explanation of the enormous energy released in such explosions. It was written just two years after Chadwick discovered the neutron.
With the extension of mathematical knowledge will it not finally become impossible for the single investigator to embrace all departments of this knowledge? In answer let me point out how thoroughly it is ingrained in mathematical science that every real advance goes hand in hand with the invention of sharper tools and simpler methods which, at the same time, assist in understanding earlier theories and in casting aside some more complicated developments.
In 'Mathematical Problems', Lecture at the International Congress of Mathematics, Paris, (8 Aug 1900). Translated by Dr. Maby Winton Newson in Bulletin of the American Mathematical Society (1902), 8, 479. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 94-95. It is reprinted in Jeremy Gray, The Hilbert Challenge (2000), 282.
With thermodynamics, one can calculate almost everything crudely; with kinetic theory, one can calculate fewer things, but more accurately; and with statistical mechanics, one can calculate almost nothing exactly.
Edward B. Stuart, Alan J. Brainard and Benjamin Gal-Or (eds.), A Critical Review of Thermodynamics (1970), 205.
With whom [do] the adherents of historicism actually empathize[?] The answer is inevitable: with the victor. And all rulers are the heirs of those who conquered before them. Hence, empathy with the victor invariably benefits the rulers. Historical materialists know what that means. Whoever has emerged victorious participates to this day in the triumphal procession in which the present rulers step over those who are lying prostrate. According to traditional practice, the spoils are carried along in the procession. They are called cultural treasures, and a historical materialist views them with cautious detachment. For without exception the cultural treasures he surveys have an origin which he cannot contemplate without horror. They owe their existence not only to the efforts of the great minds and talents who have created them, but also to the anonymous toil of their contemporaries. There is no document of civilization which is not at the same time a document of barbarism.
'Theses on the Philosophy of History' (completed 1940, first published 1950). In Illuminations, ed. Hannah Arendt and trans. Harry Zohn (1970), 258.
Without doubt one of the most characteristic features of mathematics in the last century is the systematic and universal use of the complex variable. Most of its great theories received invaluable aid from it, and many owe their very existence to it.
In 'History of Mathematics in the Nineteenth Century', Congress of Arts and Sciences (1905), Vol. 1, 474. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 115.
Without preparing fluorine, without being able to separate it from the substances with which it is united, chemistry has been able to study and to analyze a great number of its compounds. The body was not isolated, and yet its place was marked in our classifications. This well demonstrates the usefulness of a scientific theory, a theory which is regarded as true during a certain time, which correlates facts and leads the mind to new hypotheses, the first causes of experimentation; which, little by little, destroy the theory itself, in order to replace it by another more in harmony with the progress of science.
[Describing the known history of fluorine compounds before his isolation of the element.]
[Describing the known history of fluorine compounds before his isolation of the element.]
'Fluorine', lecture at the Royal Institution (28 May 1897), translated from the French, in Proceedings of the Royal Institution (1897). In Annual Report of the Board of Regents of the Smithsonian Institution to July 1897 (1898), 262.
Without theory, experience has no meaning. Without theory, one has no questions to ask. Hence without theory there is no learning.
In The New Economics for Industry, Government, Education (1993), 103.
Without theory, practice is but routine born of habit. Theory alone can bring forth and develop the spirit of invention. ... [Do not] share the opinion of those narrow minds who disdain everything in science which has not an immediate application. ... A theoretical discovery has but the merit of its existence: it awakens hope, and that is all. But let it be cultivated, let it grow, and you will see what it will become.
Inaugural Address as newly appointed Professor and Dean (Sep 1854) at the opening of the new Faculté des Sciences at Lille (7 Dec 1854). In René Vallery-Radot, The Life of Pasteur, translated by Mrs. R. L. Devonshire (1919), 76.
Yet the widespread [planetary theories], advanced by Ptolemy and most other [astronomers], although consistent with the numerical [data], seemed likewise to present no small difficulty. For these theories were not adequate unless they also conceived certain equalizing circles, which made the planet appear to move at all times with uniform velocity neither on its deferent sphere nor about its own [epicycle's] center … Therefore, having become aware of these [defects], I often considered whether there could perhaps be found a more reasonable arrangement of circles, from which every apparent irregularity would be derived while everything in itself would move uniformly, as is required by the rule of perfect motion.
From Nicholaus Copernicus, Edward Rosen (trans.), Pawel Czartoryski (ed.) 'Commentariolus', in Nicholas Copernicus: Minor Works (1985), 81-83. Excerpted in Lisa M. Dolling, Arthur F. Gianelli and Glenn N. Statile (eds.) The Tests of Time: Readings in the Development of Physical Theory (2003), 40.
You believe in the God who plays dice, and I in complete law and order in a world that objectively exists, and which I, in a wildly speculative way, am trying to capture. … Even the great initial success of the quantum theory does not make me believe in the fundamental dice-game, although I am well aware that our younger colleagues interpret this as a consequence of senility. No doubt the day will come when we will see whose instinctive attitude was the correct one.
Letter to Max Born (7 Sep 1944). In Born-Einstein Letters, 146. Einstein Archives 8-207. In Albert Einstein, Alice Calaprice, Freeman Dyson, The Ultimate Quotable Einstein (2011), 393-394. Often seen paraphrased as “I cannot believe that God plays dice with the cosmos.” Also see a related quote about God playing dice on the Stephen W. Hawking Quotes page of this website.
You know very well that unless you are a scientist, it’s much more important for a theory to be shapely, than for it to be true.
From the play, The Philanthropist: A Bourgeois Comedy (1970), Scene 2, 17.
You make experiments and I make theories. Do you know the difference? A theory is something nobody believes, except the person who made it. An experiment is something everybody believes, except the person who made it.
Remark to Hermann F. Mark.
Remark to Hermann F. Mark.
As related by Herman F. Mark to the author. Quoted in Gerald Holton, The Advancement of Science, and Its Burdens, (1986), 13.
Your remarks upon chemical notation with the variety of systems which have arisen, &c., &c., had almost stirred me up to regret publicly that such hindrances to the progress of science should exist. I cannot help thinking it a most unfortunate thing that men who as experimentalists & philosophers are the most fitted to advance the general cause of science & knowledge should by promulgation of their own theoretical views under the form of nomenclature, notation, or scale, actually retard its progress.
Letter to William Whewell (21 Feb 1831). In Isaac Todhunter, William Whewell, An Account of his Writings (1876), Vol. 1., 307. Faraday may have been referring to a paper by Whewell published in the Journal of the Royal Institution of England (1831), 437-453.

Your theories are those which you and many other people find easiest and pleasantest to believe, but, so far as I can see, they have no foundation other than they lead to a pleasant view of life … I agree that faith is essential to success in life … but I do not accept your definition of faith, i.e. belief in life after death. In my view, all that is necessary for faith is the belief that by doing our best we shall come nearer to success and that success in our aims (the improvement of the lot of mankind, present and future) is worth attaining … I maintain that faith in this world is perfectly possible without faith in another world.
Letter to her father, Ellis Franklin (undated, summer 1940? while she was an undergraduate at Cambridge). Excerpted in Brenda Maddox, The Dark Lady of DNA (2002), 61.
Your theory is crazy...but it’s not crazy enough to be true.
Restatement of the longer quote: “We all agreed that your theory is crazy. The question which divides us is whether it is crazy enough to have a chance of being correct.”







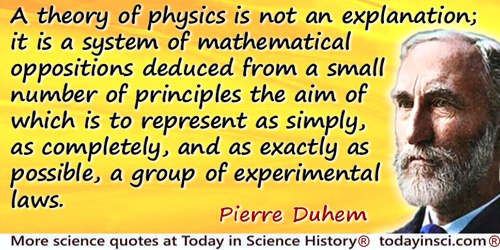









![Nikola Tesla quote: If he [Thomas Edison] had a needle to find in a haystack, he would not stop to reason where it was most like](https://todayinsci.com/T/Tesla_Nikola/TeslaNikola-Haystack500x250px.jpg)











![Carl Friedrich Gauss quote: Mathematics is the queen of the sciences and arithmetic [number theory] is the queen of mathematics.](https://todayinsci.com/G/Gauss_Carl/GaussCarl-QueenThm.jpg)























 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


