Problem Quotes (731 quotes)
Problems Quotes
Problems Quotes
… our “Physick” and “Anatomy” have embraced such infinite varieties of being, have laid open such new worlds in time and space, have grappled, not unsuccessfully, with such complex problems, that the eyes of Vesalius and of Harvey might be dazzled by the sight of the tree that has grown out of their grain of mustard seed.
A Lay Sermon, delivered at St. Martin's Hall (7 Jan 1866), 'On the Advisableness of Improving Natural Knowledge', published in The Fortnightly Review (1866), Vol. 3, 629.
...after my first feeling of revulsion had passed, I spent three of the most entertaining and instructive weeks of my life studying the fascinating molds which appeared one by one on the slowly disintegrating mass of horse-dung. Microscopic molds are both very beautiful and absorbingly interesting. The rapid growth of their spores, the way they live on each other, the manner in which the different forms come and go, is so amazing and varied that I believe a man could spend his life and not exhaust the forms or problems contained in one plate of manure.
The World Was My Garden (1938, 1941), 55.
…forcing automakers to sell smaller cars to improve fuel economy [is like]… fighting the nation’s obesity problem by forcing clothing manufacturers to sell garments in only small sizes.
— Bob Lutz
…...
...it would be a simple way of solving the goiter problem. And in addition to that it would be the biggest thing in a medical proposition to be carried out in the state of Michigan, and Michigan is a large place. And as I thought of the thing the more convinced I became that this oughtn't to be a personal thing, This ought to be something done by the Michigan State Medical Society as a body.
Recommending the addition of a trace of iodine to table salt.
Recommending the addition of a trace of iodine to table salt.
Opening address to the Medical Department of the University of Michigan, Sep 1914. Quoted by Howard Markel in 'When it Rains it Pours' : Endemic Goiter, Iodized Salt, and David Murray Cowie, M.D. American Journal of Public Health, Feb 1987, vol.77, No.2, page 222.
...Outer space, once a region of spirited international competition, is also a region of international cooperation. I realized this as early as 1959, when I attended an international conference on cosmic radiation in Moscow. At this conference, there were many differing views and differing methods of attack, but the problems were common ones to all of us and a unity of basic purpose was everywhere evident. Many of the papers presented there depended in an essential way upon others which had appeared originally in as many as three or four different languages. Surely science is one of the universal human activities.
…resort to science has rendered modern war so destructive of life and property that it presents a new problem to mankind, such, that unless our civilization shall find some means of making an end to war, war will make an end to our civilization.
America and World Peace (1925), 37. In Edward C. Luck, Mixed Messages: American Politics and International Organization, 1919-1999 (1999), 143.
“Yes,” he said. “But these things (the solutions to problems in solid geometry such as the duplication of the cube) do not seem to have been discovered yet.” “There are two reasons for this,” I said. “Because no city holds these things in honour, they are investigated in a feeble way, since they are difficult; and the investigators need an overseer, since they will not find the solutions without one. First, it is hard to get such an overseer, and second, even if one did, as things are now those who investigate these things would not obey him, because of their arrogance. If however a whole city, which did hold these things in honour, were to oversee them communally, the investigators would be obedient, and when these problems were investigated continually and with eagerness, their solutions would become apparent.”
— Plato
In The Republic 7 528bc, trans. R.W. Sharples.
[An appealing problem is] a combination of being fairly concrete—so one can understand concretely examples—and also connecting with a lot of other ideas. For example, you see the analysis in a minimal surface equation, but then you also realize it has connections with other geometric questions that are not just analysis. I am definitely very attracted to the idea that there are a lot of different facets in mathematics and seeing the connections.
From Allyn Jackson, 'Interview with Karen Uhlenbeck', part of Celebratio Mathematica on the celebratio.org website.
[Because a nation’s level of prosperity depends directly on the amount of energy used,] it is an illusion to think that we can solve our problem by energy conservation alone. For the next few years, conservation must play a very important role, but at the same time, we must use and develop all our alternative energy sources. With the demand for energy increasing constantly, there seems to me no prospect that oil will be plentiful. The hope for a lower oil price is paralyzing long-range action on energy in Washington and elsewhere. Only if we achieve virtual energy independence can there be any hope for a drop in the oil price.
In address at City College, reported in Victor K. McElheny, 'Hans Bethe Urges U.S. Drive for Atom Power and Coal', The New York Times (14 Dec 1974), 58.
[Blackett] came one morning, deep in thought, into the G (technical) Office at Stanmore. It was a bitterly cold day, and the staff were shivering in a garret warmed over only with an oil-stove. Without a word of greeting, Blackett stepped silently up on to the table and stood there pondering with his feet among the plans. After ten minutes somebody coughed uneasily and said, diffidently: “Wouldn’t you like a chair, sir … or something?” “No, thank you,” said Professor Blackett, “it is necessary to apply scientific methods. Hot air rises. The warmest spot in this room, therefore, will be near the ceiling.” At this, Colonel Krohn, my technical G.S.O., stepped up on the table beside the Professor, and for the next half-hour, the two stayed there in silence. At the end of this period Professor Blackett stepped down from the table saying: “Well! That’s that problem solved.” And so it was.
Anecdote as told by General Sir Frederick Pile, in Frederick Pile, Ack-Ack: Britain’s Defence Against Air Attack During Second World War (1949), 161. As cited by Maurice W. Kirby and Jonathan Rosenhead, 'Patrick Blackett (1897)' in Arjang A. Assad (ed.) and Saul I. Gass (ed.),Profiles in Operations Research: Pioneers and Innovators (2011), 7.
[Certain students] suppose that because science has penetrated the structure of the atom it can solve all the problems of the universe. ... They are known in every ... college as the most insufferable, cocksure know-it-alls. If you want to talk to them about poetry, they are likely to reply that the "emotive response" to poetry is only a conditioned reflex .... If they go on to be professional scientists, their sharp corners are rubbed down, but they undergo no fundamental change. They most decidedly are not set apart from the others by their intellectual integrity and faith, and their patient humility in front of the facts of nature.... They are uneducated, in the fullest sense of the word, and they certainly are no advertisement for the claims of science teachers.
In Science is a Sacred Cow (1950), 18-19.
[Dubious attribution] We are all continually faced with great opportunities which are brilliantly disguised as unsolvable problems.
Attributed. (?) Note: So far, Webmaster has been unable to find a primary source for this quote. It can be found seen quoted in several books, but always without citation. The earliest found with attribution to Mead is in Brian E. Walsh, Unleashing Your Brilliance (2005). However, earlier books attribute differently, for example to Lee Iacocca (2000), and to John Gardner (1986). Also found without any attribution (“it has been said”), without any citation, in Christopher H. Lovelock and Charles B. Weinberg, Readings in Public and Nonprofit Marketing (1978), 152. If you know the primary source, please contact Webmaster.
[It] is not the nature of things for any one man to make a sudden, violent discovery; science goes step by step and every man depends on the work of his predecessors. When you hear of a sudden unexpected discovery—a bolt from the blue—you can always be sure that it has grown up by the influence of one man or another, and it is the mutual influence which makes the enormous possibility of scientific advance. Scientists are not dependent on the ideas of a single man, but on the combined wisdom of thousands of men, all thinking of the same problem and each doing his little bit to add to the great structure of knowledge which is gradually being erected.
Concluding remark in Lecture ii (1936) on 'Forty Years of Physics', revised and prepared for publication by J.A. Ratcliffe, collected in Needham and Pagel (eds.), Background to Modern Science: Ten Lectures at Cambridge Arranged by the History of Science Committee, (1938), 73-74. Note that the words as prepared for publication may not be verbatim as spoken in the original lecture by the then late Lord Rutherford.
[King Hiero II] requested Archimedes to consider [whether a crown was pure gold or alloyed with silver]. The latter, while the case was still on his mind, happened to go to the bath, and on getting into a tub observed that the more his body sank into it the more water ran out over the tub. As this pointed out the way to explain the case in question, without a moment’s delay, and transported with joy, he jumped out of the tub and rushed home naked, crying with a loud voice that he had found what he was seeking; for as he ran he shouted repeatedly in Greek, “Eὕρηκα, εὕρηκα.”
This famous anecdote, being written about two centuries after Archimedes, is of questionable authenticity, but Vitruvius provided the origin of the story as we know it. In De Architectura, Book 9, Introduction, Sec. 10. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 254. Also seen translated as “While Archimedes was turning the problem over, he chanced to come to the place of bathing, and there, as he was sitting down in the tub, he noticed that the amount of water which flowed over the tub was equal to the amount by which his body was immersed. This showed him a means of solving the problem. … In his joy, he leapt out of the tub and, rushing naked towards his home, he cried out with a loud voice that he had found what he sought.” In Ivor Bulmer-Thomas, Selections Illustrating the History of Greek Mathematics (1939), 37.
[Mathematics is] the study of ideal constructions (often applicable to real problems), and the discovery thereby of relations between the parts of these constructions, before unknown.
In 'Mathematics', Century Dictionary.
[My dream dinner guest is] Charles Darwin. It’s an obvious answer, but it’s the truth. Think of any problem and before you start theorising, just check up whether Charles Darwin mentioned it in one of those green books sitting on your shelf. Whether it’s earthworms, human gestures or the origin of species, the observations that man made are unbelievable. He touched on so many subjects. Then, Alexander von Humboldt, the last polymath. There was no aspect of the natural world that he wasn’t curious about or didn’t write about in Kosmos, an extraordinary book.
From interview with Alice Roberts, 'Attenborough: My Life on Earth', The Biologist (Aug 2015), 62, No. 4, 16.
[O]ur long-term security is threatened by a problem at least as dangerous as chemical, nuclear or biological weapons, or indeed international terrorism: human-induced climate change. … The impacts of global warming are such that I have no hesitation in describing it as a “weapon of mass destruction.” Like terrorism, this weapon knows no boundaries. It can strike anywhere, in any form…
London Guardian (28 Jul 2003)
[O]ur own existence once presented the greatest of all mysteries, but … it is a mystery no longer because it is solved. Darwin and Wallace solved it … I was surprised that so many people seemed not only unaware of the elegant and beautiful solution to this deepest of problems but, incredibly, in many cases actually unaware that there was a problem in the first place!
The Blind Watchmaker (1996), front matter.
[On research] It’s got to be fun. I don't think anybody should tell you that he’s slogged his way through 25 years on a problem and there's only one reward at the end, and that's the value of the Hubble constant. That’s a bunch of hooey. The reward is learning all the wonderful properties of the things that don’t work.
As quoted in Obituary, 'Allan Sandage, 84, Astronomer, Dies; Charted Cosmos’s Age and Expansion', New York Times (17 Nov 2010), B19.
[On solving problems:] The first thing you do is scream.
As quoted in interview with Frances Glennon, 'Student and Teacher of Human Ways', Life (14 Sep 1959), 147.
[T]he explosive development of our intellect, … I personally think was at least partly triggered by the fact we developed this way of talking with words. … We can bring people from different disciplines together to discuss a problem. That’s because of words. We now have developed a moral code with our words. And we know perfectly well what we should and shouldn’t do.
From huffpost.com webpage interview by Alexander C. Kaufman, 'Jane Goodall: If We Don’t Make Peace With Nature, Expect More Deadly Pandemics' (28 May 2021).
[The problem I hope scientists will have solved by the end of the 21st century is:] The production of energy without any deleterious effects. The problem is then we’d be so powerful, there’d be no restraint and we’d continue wrecking everything. Solar energy would be preferable to nuclear. If you could harness it to produce desalination, you could make the Sahara bloom.
From 'Interview: Of Mind and Matter: David Attenborough Meets Richard Dawkins', The Guardian (11 Sep 2010).
[The problem I hope scientists will have solved by the end of the 21st century] thinking more academically: the problem of human consciousness.
From 'Interview: Of Mind and Matter: David Attenborough Meets Richard Dawkins', The Guardian (11 Sep 2010).
[The purpose of flight research] is to separate the real from the imagined problems and to make known the overlooked and the unexpected.
Description of the purpose of the X-15 program given in a meeting at the Langley Research Center (Oct 1956). Quoted in Michael H. Gorn, Expanding the Envelope (2001), 3.
[The religion of science was] an implicit faith that by the methods of physical science, and by these methods alone, could be solved all the problems arising out of the relation of man to man and of man towards the universe.
In My Apprenticeship (1926), 89.
[Using a hand calculator and writing things down longhand] I was able to solve this problem because I don’t have a computer. I know what I am doing every step, and the steps go slowly enough that I can think.
As quoted in Charles Petit, 'The Curious Quester', The San Francisco Chronicle. Reprinted in The Courier-Journal (3 Mar 1991), Magazine, 33.
[We] can easily distinguish what relates to Mathematics in any question from that which belongs to the other sciences. But as I considered the matter carefully it gradually came to light that all those matters only were referred to Mathematics in which order and measurements are investigated, and that it makes no difference whether it be in numbers, figures, stars, sounds or any other object that the question of measurement arises. I saw consequently that there must be some general science to explain that element as a whole which gives rise to problems about order and measurement, restricted as these are to no special subject matter. This, I perceived was called “Universal Mathematics,” not a far-fetched asignation, but one of long standing which has passed into current use, because in this science is contained everything on account of which the others are called parts of Mathematics.
Rules for the Direction of the Mind (written 1628). As translated by Elizabeth Sanderson Haldane and George Robert Thomson Ross in The Philosophical Works of Descartes (1911, 1931), 13.
Les mathématiciens parviennent à la solution d’un problême par le simple arrangement des données, & en réduisant le raisonnement à des opérations si simples, à des jugemens si courts, qu’ils ne perdent jamais de vue l’évidence qui leur sert de guide.
Mathematicians come to the solution of a problem by the simple arrangement of the data, and reducing the reasoning to such simple operations, to judgments so brief, that they never lose sight of the evidence that serves as their guide.
Mathematicians come to the solution of a problem by the simple arrangement of the data, and reducing the reasoning to such simple operations, to judgments so brief, that they never lose sight of the evidence that serves as their guide.
From a paper read to the Académie Royales des Sciences (18 Apr 1787), printed in Méthode de Nomenclature Chimique (1787), 12. Translation from the French by Webmaster.
Neumann, to a physicist seeking help with a difficult problem: Simple. This can be solved by using the method of characteristics.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Physicist: I'm afraid I don’t understand the method of characteristics.
Neumann: In mathematics you don't understand things. You just get used to them.
Attributed, as related by Dr. Felix Smith (Head of Molecular Physics, Stanford Research Institute) to author Gary Zukav, who quoted it in The Dancing Wu Li Masters: An Overview of the New Physics (1979, 2001), 208, footnote. The physicist (a friend of Dr. Smith) worked at Los Alamos after WW II. It should be noted that although the author uses quotation marks around the spoken remarks, that they represent the author's memory of Dr. Smith's recollection, who heard it from the physicist. Therefore the fourth-hand wording is very likely not verbatim. Webmaster finds Zukav's book seems to be the only source for this quote.
Quand les physiciens nous demandent la solution d'un problème, ce n'est pas une corvée qu'ils nous impsent, c'est nous au contraire qui leur doivent des remercîments.
When the physicists ask us for the solution of a problem, it is not drudgery that they impose on us, on the contrary, it is us who owe them thanks.
When the physicists ask us for the solution of a problem, it is not drudgery that they impose on us, on the contrary, it is us who owe them thanks.
La valeur de la science. In Anton Bovier, Statistical Mechanics of Disordered Systems (2006), 111.
Quod est, Nullum non problema solvere.
There is no problem that cannot be solved.
There is no problem that cannot be solved.
In The New Algebra.
Sir Robert Chiltern: You think science cannot grapple with the problem of women?
Mrs. Cheveley: Science can never grapple with the irrational. That is why it has no future before it in this world.
Mrs. Cheveley: Science can never grapple with the irrational. That is why it has no future before it in this world.
In play, An Ideal Husband (1912, 2001), Act 1, 6.
Une même expression, dont les géomètres avaient considéré les propriétés abstraites, … représente'aussi le mouvement de la lumière dans l’atmosphère, quelle détermine les lois de la diffusion de la chaleur dans la matière solide, et quelle entre dans toutes les questions principales de la théorie des probabilités.
The same expression whose abstract properties geometers had considered … represents as well the motion of light in the atmosphere, as it determines the laws of diffusion of heat in solid matter, and enters into all the chief problems of the theory of probability.
The same expression whose abstract properties geometers had considered … represents as well the motion of light in the atmosphere, as it determines the laws of diffusion of heat in solid matter, and enters into all the chief problems of the theory of probability.
From Théorie Analytique de la Chaleur (1822), translated by Alexander Freeman in The Analytical Theory of Heat (1878), 7.
~~[Reinterpretation]~~ The significant problems we face cannot be solved at the same level of thinking we were at when we created them.
Yet another of the Einstein-like quotes in common circulation for which there appears to be no known source in the given wording. There are also a number of variations on the the theme. It resembles an authentic quote, “A new type of thinking is essential if mankind is to survive and move toward higher levels,” from a longer discussion, in 'Atomic Education Urged by Einstein', New York Times (25 May 1946), 13. Other reinterpretations, not in exactly Einstein’s wording, include: “No problem can be solved from the same level of consciousness that created it.” “The world will not evolve past its current state of crisis by using the same thinking that created the situation.” “The significant problems we have cannot be solved at the same level of thinking with which we created them.” “The world we have made, as a result of the level of thinking we have done thus far, creates problems we cannot solve at the same level of thinking at which we created them.” For more context, see the authentic quote that begins, “Our world faces a crisis as yet unperceived…,” on the Albert Einstein Quotes page on this website.
A cat finds it easy to be a cat, as nearly as we can tell. It isn’t afraid to be a cat. But being a full human being is difficult, frightening, and problematical. While human beings love knowledge and seek it—they are curious—they also fear it. The closer to the personal it is, the more they fear
In The Psychology of Science: A Reconnaissance (1966), 16.
A central lesson of science is that to understand complex issues (or even simple ones), we must try to free our minds of dogma and to guarantee the freedom to publish, to contradict, and to experiment. Arguments from authority are unacceptable.
Billions and Billions: Thoughts on Life and Death at the Brink of the Millenium (1998), 190.
A chess problem is genuine mathematics, but it is in some way “trivial” mathematics. However, ingenious and intricate, however original and surprising the moves, there is something essential lacking. Chess problems are unimportant. The best mathematics is serious as well as beautiful—“important” if you like, but the word is very ambiguous, and “serious” expresses what I mean much better.
'A Mathematician's Apology', in James Roy Newman, The World of Mathematics (2000), 2029.
A clever person solves a problem. A wise person avoids it.
Widely found on the web as an Einstein quote, but Webmaster has not yet found a primary source. Can you help? It is probably yet another example of a “wise” quote to which Einstein’s name has been falsely attributed. For authentic quotes see Albert Einstein Quotes on Problem.
A designer must always think about the unfortunate production engineer who will have to manufacture what you have designed; try to understand his problems.
On the official Raymond Loewry website.
A good deal of my research in physics has consisted in not setting out to solve some particular problem, but simply examining mathematical quantities of a kind that physicists use and trying to fit them together in an interesting way, regardless of any application that the work may have. It is simply a search for pretty mathematics. It may turn out later to have an application. Then one has good luck. At age 78.
International Journal of Theoretical Physics (1982), 21, 603. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 110.
A good psychologist has to be able to distinguish strongly between problems of process, which are causal, and problems of structure, which are analytic and descriptive. In particular the statistics adequate for the latter are not sufficient for the former.
From archive recording (3 Jun 1959) with to John C. Kenna, giving his recollection of his farewell speech to Cambridge Psychological Society (4 Mar 1952), in which he gave a summary of points he considered to be basic requirements for a good experimental psychologist. Point 5 of 7, from transcription of recording held at British Psychological Society History of Psychology Centre, London, as abridged on thepsychologist.bps.org.uk website.
A great department of thought must have its own inner life, however transcendent may be the importance of its relations to the outside. No department of science, least of all one requiring so high a degree of mental concentration as Mathematics, can be developed entirely, or even mainly, with a view to applications outside its own range. The increased complexity and specialisation of all branches of knowledge makes it true in the present, however it may have been in former times, that important advances in such a department as Mathematics can be expected only from men who are interested in the subject for its own sake, and who, whilst keeping an open mind for suggestions from outside, allow their thought to range freely in those lines of advance which are indicated by the present state of their subject, untrammelled by any preoccupation as to applications to other departments of science. Even with a view to applications, if Mathematics is to be adequately equipped for the purpose of coping with the intricate problems which will be presented to it in the future by Physics, Chemistry and other branches of physical science, many of these problems probably of a character which we cannot at present forecast, it is essential that Mathematics should be allowed to develop freely on its own lines.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 286.
A great discovery solves a great problem, but there is a grain of discovery in the solution of any problem. Your problem may be modest, but if it challenges your curiosity and brings into play your inventive faculties, and if you solve it by your own means, you may experience the tension and enjoy the triumph of discovery.
From Preface to the first printing, reprinted in How to Solve It: A New Aspect of Mathematical Method (2004), v.
A human being should be able to change a diaper, plan an invasion, butcher a hog, conn a ship, design a building, write a sonnet, balance accounts, build a wall, set a bone, comfort the dying, take orders, give orders, cooperate, act alone, solve equations, analyze a new problem, pitch manure, program a computer, cook a tasty meal, fight efficiently, die gallantly. Specialization is for insects.
In Time Enough for Love: The Lives of Lazarus Long (1973), 265.
A man is flying in a hot air balloon and realizes he is lost. He reduces height, spots a man down below and asks,“Excuse me, can you help me? I promised to return the balloon to its owner, but I don’t know where I am.”
The man below says: “You are in a hot air balloon, hovering approximately 350 feet above mean sea level and 30 feet above this field. You are between 40 and 42 degrees north latitude, and between 58 and 60 degrees west longitude.”
“You must be an engineer,” says the balloonist.
“I am,” replies the man.“How did you know?”
“Well,” says the balloonist, “everything you have told me is technically correct, but I have no idea what to make of your information, and the fact is I am still lost.”
The man below says, “You must be a manager.”
“I am,” replies the balloonist,“but how did you know?”
“Well,” says the engineer,“you don’t know where you are, or where you are going. You have made a promise which you have no idea how to keep, and you expect me to solve your problem.The fact is you are in the exact same position you were in before we met, but now it is somehow my fault.”
The man below says: “You are in a hot air balloon, hovering approximately 350 feet above mean sea level and 30 feet above this field. You are between 40 and 42 degrees north latitude, and between 58 and 60 degrees west longitude.”
“You must be an engineer,” says the balloonist.
“I am,” replies the man.“How did you know?”
“Well,” says the balloonist, “everything you have told me is technically correct, but I have no idea what to make of your information, and the fact is I am still lost.”
The man below says, “You must be a manager.”
“I am,” replies the balloonist,“but how did you know?”
“Well,” says the engineer,“you don’t know where you are, or where you are going. You have made a promise which you have no idea how to keep, and you expect me to solve your problem.The fact is you are in the exact same position you were in before we met, but now it is somehow my fault.”
In Jon Fripp, Michael Fripp and Deborah Fripp, Speaking of Science (2000), 199.
A mathematical problem should be difficult in order to entice us, yet not completely inaccessible, lest it mock at our efforts. It should be to us a guide post on the mazy paths to hidden truths, and ultimately a reminder of our pleasure in the successful solution.
In Mathematical Problems', Bulletin American Mathematical Society, 8, 438.
A mathematician who can only generalise is like a monkey who can only climb UP a tree. ... And a mathematician who can only specialise is like a monkey who can only climb DOWN a tree. In fact neither the up monkey nor the down monkey is a viable creature. A real monkey must find food and escape his enemies and so must be able to incessantly climb up and down. A real mathematician must be able to generalise and specialise. ... There is, I think, a moral for the teacher. A teacher of traditional mathematics is in danger of becoming a down monkey, and a teacher of modern mathematics an up monkey. The down teacher dishing out one routine problem after another may never get off the ground, never attain any general idea. and the up teacher dishing out one definition after the other may never climb down from his verbiage, may never get down to solid ground, to something of tangible interest for his pupils.
From 'A Story With A Moral', Mathematical Gazette (Jun 1973), 57, No. 400, 86-87
A million years is a short time—the shortest worth messing with for most problems. You begin tuning your mind to a time scale that is the planet’s time scale. For me, it is almost unconscious now and is a kind of companionship with the earth.
In Basin and Range (1981), 134.
A modern branch of mathematics, having achieved the art of dealing with the infinitely small, can now yield solutions in other more complex problems of motion, which used to appear insoluble. This modern branch of mathematics, unknown to the ancients, when dealing with problems of motion, admits the conception of the infinitely small, and so conforms to the chief condition of motion (absolute continuity) and thereby corrects the inevitable error which the human mind cannot avoid when dealing with separate elements of motion instead of examining continuous motion. In seeking the laws of historical movement just the same thing happens. The movement of humanity, arising as it does from innumerable human wills, is continuous. To understand the laws of this continuous movement is the aim of history. … Only by taking an infinitesimally small unit for observation (the differential of history, that is, the individual tendencies of man) and attaining to the art of integrating them (that is, finding the sum of these infinitesimals) can we hope to arrive at the laws of history.
War and Peace (1869), Book 11, Chap. 1.
A multidisciplinary study group ... estimated that it would be 1980 before developments in artificial intelligence make it possible for machines alone to do much thinking or problem solving of military significance. That would leave, say, five years to develop man-computer symbiosis and 15 years to use it. The 15 may be 10 or 500, but those years should be intellectually the most creative and exciting in the history of mankind.
From article 'Man-Computer Symbiosis', in IRE Transactions on Human Factors in Electronics (Mar 1960), Vol. HFE-1, 4-11.
A principle of induction would be a statement with the help of which we could put inductive inferences into a logically acceptable form. In the eyes of the upholders of inductive logic, a principle of induction is of supreme importance for scientific method: “... this principle”, says Reichenbach, “determines the truth of scientific theories. To eliminate it from science would mean nothing less than to deprive science of the power to decide the truth or falsity of its theories. Without it, clearly, science would no longer have the right to distinguish its theories from the fanciful and arbitrary creations of the poet’s mind.” Now this principle of induction cannot be a purely logical truth like a tautology or an analytic statement. Indeed, if there were such a thing as a purely logical principle of induction, there would be no problem of induction; for in this case, all inductive inferences would have to be regarded as purely logical or tautological transformations, just like inferences in inductive logic. Thus the principle of induction must be a synthetic statement; that is, a statement whose negation is not self-contradictory but logically possible. So the question arises why such a principle should be accepted at all, and how we can justify its acceptance on rational grounds.
…...
A problem is a chance for you to do your best.
…...
A problem is really a springboard for a leap into the unknown.
In 'The Arts and the Sciences', American Scientist (Jul 1953). Epigraph in Meta Riley Emberger and Marian Ross Hall, Scientific Writing (1955),
A problem well stated is a problem half-solved.
A reasonable content for general education today, then, seems to me to be as follows: First, a command of the principal linguistic tools essential to the pursuit of either science or art. Second, a familiarity with the scientific method and with its principal applications to both physical and social problems. And third, appreciation and practice of the arts, including literature. Furthermore, these three fields should be so integrated toward a common purpose that the question of their relative importance would not even arise. One does not ask which is the most important leg of a tripod.
In 'Education in a Scientific Age', Can Science Save Us? (1947, 2nd ed. 1961), 74-75.
A research problem is not solved by apparatus; it is solved in a man's head.
A scientist works largely by intuition. Given enough experience, a scientist examining a problem can leap to an intuition as to what the solution ‘should look like.’ ... Science is ultimately based on insight, not logic.
…...
A strong feeling of adventure is animating those who are working on bacterial viruses, a feeling that they have a small part in the great drive towards a fundamental problem in biology.
From 'Experiments with Bacterial Viruses (Bacteriophages)', Harvey Lecture (1946), 41, 187. As cited in Robert Olby, The Path of the Double Helix: The Discovery of DNA (1974, 1994), 238.
A study of Dr. [Florence] Sabin’s work shows the greatness of her achievement and the character of her mind. She has dealt with the primary and fundamental problem of the cell—the unit of plant and animal life. All through her investigations she has followed the cell, seeking the secret of differentiations by newer and finer methods, both physical and chemical. Always through her work runs the great strong, continuous cord of cell differentiations. This is one of the great concepts of man, for all life begins as a single cell. I have known and followed Dr. Sabin’s work since her student days, and have lately been more closely associated with her in her tuberculosis studies. She is all in mind and spirit and ideals that man or woman ever accomplishes. She belongs to the great students of both sexes, for when these have the brains and the will to work I see little difference.
In Genevieve Parkhurst, 'Dr. Sabin, Scientist: Winner Of Pictorial Review’s Achievement Award', Pictorial Review (Jan 1930), 2.
A survey of the literature published during the last ten years dealing with education and the educational problems in America,… cannot fail to impress even the most casual that antagonists and protagonists fall into three roughly classified camps: at one extreme the culturalists, at the other the vocationalists, and between and exposed to the ceaseless fire of both the bewildered parents, who are concerned with the problem primarily as it touches the education of their own children, and who, confused by the amount of ammunition expended by the opposing forces, have been compelled to draw the small solace possible from an ancient stalemate, that “Much may be said for both sides,” and have blindly trusted precedent with an historical faith in the traditional good lying somewhere in the thing called “education.” The tide of battle has ebbed and flowed, the advantage of ammunition and popular support being now with one, now with the other; and the plight of the bewildered yet vitally concerned non-combatant has remained virtually the same.
Co-author with Louis Jay Heath, in 'Preface', A New Basis for Social Progress (1917), ix
A teacher of mathematics has a great opportunity. If he fills his allotted time with drilling his students in routine operations he kills their interest, hampers their intellectual development, and misuses his opportunity. But if he challenges the curiosity of his students by setting them problems proportionate to their knowledge, and helps them to solve their problems with stimulating questions, he may give them a taste for, and some means of, independent thinking.
In How to Solve It (1948), Preface.
A troubling question for those of us committed to the widest application of intelligence in the study and solution of the problems of men is whether a general understanding of the social sciences will be possible much longer. Many significant areas of these disciplines have already been removed by the advances of the past two decades beyond the reach of anyone who does not know mathematics; and the man of letters is increasingly finding, to his dismay, that the study of mankind proper is passing from his hands to those of technicians and specialists. The aesthetic effect is admittedly bad: we have given up the belletristic “essay on man” for the barbarisms of a technical vocabulary, or at best the forbidding elegance of mathematical syntax.
Opening paragraph of 'The Study of Man: Sociology Learns the Language of Mathematics' in Commentary (1 Sep 1952). Reprinted in James Roy Newman, The World of Mathematics (1956), Vol. 2, 1294.
A wonderful exhilaration comes from holding in the mind the deepest questions we can ask. Such questions animate all scientists. Many students of science were first attracted to the field as children by popular accounts of important unsolved problems. They have been waiting ever since to begin working on a mystery. [With co-author Arthur Zajonc]
In George Greenstein and Arthur Zajonc, The Quantum Challenge: Modern Research on the Foundations of Quantum Mechanics (2006), xii.
Accordingly, we find Euler and D'Alembert devoting their talent and their patience to the establishment of the laws of rotation of the solid bodies. Lagrange has incorporated his own analysis of the problem with his general treatment of mechanics, and since his time M. Poinsôt has brought the subject under the power of a more searching analysis than that of the calculus, in which ideas take the place of symbols, and intelligent propositions supersede equations.
J. C. Maxwell on Louis Poinsôt (1777-1859) in 'On a Dynamical Top' (1857). In W. D. Niven (ed.), The Scientific Papers of James Clerk Maxwell (1890), Vol. 1, 248.
After Gibbs, one the most distinguished [American scientists] was Langley, of the Smithsonian. … He had the physicist’s heinous fault of professing to know nothing between flashes of intense perception. … Rigidly denying himself the amusement of philosophy, which consists chiefly in suggesting unintelligible answers to insoluble problems, and liked to wander past them in a courteous temper, even bowing to them distantly as though recognizing their existence, while doubting their respectability.
The Education of Henry Adams: An Autobiography? (1918), 377.
After I had addressed myself to this very difficult and almost insoluble problem, the suggestion at length came to me how it could be solved with fewer and much simpler constructions than were formerly used, if some assumptions (which are called axioms) were granted me. They follow in this order.
There is no one center of all the celestial circles or spheres.
The center of the earth is not the center of the universe, but only of gravity and of the lunar sphere.
All the spheres revolve about the sun as their mid-point, and therefore the sun is the center of the universe.
The ratio of the earth’s distance from the sun to the height of the firmament is so much smaller than the ratio of the earth’s radius to its distance from the sun that the distance from the earth to the sun is imperceptible in comparison with the height of the firmament.
Whatever motion appears in the firmament arises not from any motion of the firmament, but from the earth’s motion. The earth together with its circumjacent elements performs a complete rotation on its fixed poles in a daily motion, while the firmament and highest heaven abide unchanged.
What appears to us as motions of the sun arise not from its motion but from the motion of the earth and our sphere, with which we revolve about the sun like any other planet. The earth has, then, more than one motion.
The apparent retrograde and direct motion of the planets arises not from their motion but from the earth’s. The motion of the earth alone, therefore, suffices to explain so many apparent inequalities in the heavens.
There is no one center of all the celestial circles or spheres.
The center of the earth is not the center of the universe, but only of gravity and of the lunar sphere.
All the spheres revolve about the sun as their mid-point, and therefore the sun is the center of the universe.
The ratio of the earth’s distance from the sun to the height of the firmament is so much smaller than the ratio of the earth’s radius to its distance from the sun that the distance from the earth to the sun is imperceptible in comparison with the height of the firmament.
Whatever motion appears in the firmament arises not from any motion of the firmament, but from the earth’s motion. The earth together with its circumjacent elements performs a complete rotation on its fixed poles in a daily motion, while the firmament and highest heaven abide unchanged.
What appears to us as motions of the sun arise not from its motion but from the motion of the earth and our sphere, with which we revolve about the sun like any other planet. The earth has, then, more than one motion.
The apparent retrograde and direct motion of the planets arises not from their motion but from the earth’s. The motion of the earth alone, therefore, suffices to explain so many apparent inequalities in the heavens.
'The Commentariolus', in Three Copernican Treatises (c.1510), trans. E. Rosen (1939), 58-9.
After the discovery of spectral analysis no one trained in physics could doubt the problem of the atom would be solved when physicists had learned to understand the language of spectra. So manifold was the enormous amount of material that has been accumulated in sixty years of spectroscopic research that it seemed at first beyond the possibility of disentanglement. An almost greater enlightenment has resulted from the seven years of Röntgen spectroscopy, inasmuch as it has attacked the problem of the atom at its very root, and illuminates the interior. What we are nowadays hearing of the language of spectra is a true 'music of the spheres' in order and harmony that becomes ever more perfect in spite of the manifold variety. The theory of spectral lines will bear the name of Bohr for all time. But yet another name will be permanently associated with it, that of Planck. All integral laws of spectral lines and of atomic theory spring originally from the quantum theory. It is the mysterious organon on which Nature plays her music of the spectra, and according to the rhythm of which she regulates the structure of the atoms and nuclei.
Atombau und Spektrallinien (1919), viii, Atomic Structure and Spectral Lines, trans. Henry L. Brose (1923), viii.
All anybody has to say to Edward [Teller] is, ‘We’ve got a problem here, we need you,’ and— zip! he’s into it. It’s helpfulness, plus maybe vanity, but mostly just curiosity.
As described by an unidentified friend, quoted in Robert Coughlan, 'Dr. Edward Teller’s Magnificent Obsession', Life (6 Sep 1954), 62.
All interpretations made by a scientist are hypotheses, and all hypotheses are tentative. They must forever be tested and they must be revised if found to be unsatisfactory. Hence, a change of mind in a scientist, and particularly in a great scientist, is not only not a sign of weakness but rather evidence for continuing attention to the respective problem and an ability to test the hypothesis again and again.
The Growth of Biological Thought: Diversity, Evolution and Inheritance (1982), 831.
All Nature bristles with the marks of interrogation—among the grass and the petals of flowers, amidst the feathers of birds and the hairs of mammals, on mountain and moorland, in sea and sky-everywhere. It is one of the joys of life to discover those marks of interrogation, these unsolved and half-solved problems and try to answer their questions.
In Riddles of Science (1932), 5.
All of modern physics is governed by that magnificent and thoroughly confusing discipline called quantum mechanics ... It has survived all tests and there is no reason to believe that there is any flaw in it.... We all know how to use it and how to apply it to problems; and so we have learned to live with the fact that nobody can understand it.
…...
All problems are finally scientific problems.
Preface, The Doctor’s Dilemma (1911).
All stable processes we shall predict. All unstable processes we shall control.
Describing John von Neumann's aspiration for the application of computers sufficiently large to solve the problems of meteorology, despite the sensitivity of the weather to small perturbations.
Describing John von Neumann's aspiration for the application of computers sufficiently large to solve the problems of meteorology, despite the sensitivity of the weather to small perturbations.
Infinite in All Directions (2004), 182. Dyson wrote his recollection of a talk given by Neumann at Princeton around 1950. The words are not a direct quotation, merely Dyson's description of Neumann's idea.
All that can be said upon the number and nature of elements is, in my opinion, confined to discussions entirely of a metaphysical nature. The subject only furnishes us with indefinite problems, which may be solved in a thousand different ways, not one of which, in all probability, is consistent with nature. I shall therefore only add upon this subject, that if, by the term elements, we mean to express those simple and indivisible atoms of which matter is composed, it is extremely probable we know nothing at all about them; but, if we apply the term elements, or principles of bodies, to express our idea of the last point which analysis is capable of reaching, we must admit, as elements, all the substances into which we are capable, by any means, to reduce bodies by decomposition.
Elements of Chemistry (1790), trans. R. Kerr, Preface, xxiv.
All the events which occur upon the earth result from Law: even those actions which are entirely dependent on the caprices of the memory, or the impulse of the passions, are shown by statistics to be, when taken in the gross, entirely independent of the human will. As a single atom, man is an enigma; as a whole, he is a mathematical problem. As an individual, he is a free agent; as a species, the offspring of necessity.
In The Martyrdom of Man (1876), 185-186.
All the mathematical sciences are founded on relations between physical laws and laws of numbers, so that the aim of exact science is to reduce the problems of nature to the determination of quantities by operations with numbers.
from Faraday's Lines of Force (1856)
All the more recent work on alkaptonuria has... strengthened the belief that the homogentisic acid excreted is derived from tyrosin, but why alkaptonuric individuals pass the benzene ring of their tyrosin unbroken and how and where the peculiar chemical change from tyrosin to homogentisic acid is brought about, remain unsolved problems.
'The Incidence of Alkaptonuria: A Study in Chemical Individuality', The Lancet, 1902, 2, 1616.
Almost every major systematic error which has deluded men for thousands of years relied on practical experience. Horoscopes, incantations, oracles, magic, witchcraft, the cures of witch doctors and of medical practitioners before the advent of modern medicine, were all firmly established through the centuries in the eyes of the public by their supposed practical successes. The scientific method was devised precisely for the purpose of elucidating the nature of things under more carefully controlled conditions and by more rigorous criteria than are present in the situations created by practical problems.
Personal Knowledge (1958), 183.
Almost everyone... seems to be quite sure that the differences between the methodologies of history and of the natural sciences are vast. For, we are assured, it is well known that in the natural sciences we start from observation and proceed by induction to theory. And is it not obvious that in history we proceed very differently? Yes, I agree that we proceed very differently. But we do so in the natural sciences as well.
In both we start from myths—from traditional prejudices, beset with error—and from these we proceed by criticism: by the critical elimination of errors. In both the role of evidence is, in the main, to correct our mistakes, our prejudices, our tentative theories—that is, to play a part in the critical discussion, in the elimination of error. By correcting our mistakes, we raise new problems. And in order to solve these problems, we invent conjectures, that is, tentative theories, which we submit to critical discussion, directed towards the elimination of error.
In both we start from myths—from traditional prejudices, beset with error—and from these we proceed by criticism: by the critical elimination of errors. In both the role of evidence is, in the main, to correct our mistakes, our prejudices, our tentative theories—that is, to play a part in the critical discussion, in the elimination of error. By correcting our mistakes, we raise new problems. And in order to solve these problems, we invent conjectures, that is, tentative theories, which we submit to critical discussion, directed towards the elimination of error.
The Myth of the Framework: In Defence of Science and Rationality (1993), 140.
Altering a gene in the gene line to produce improved offspring is likely to be very difficult because of the danger of unwanted side effects. It would also raise obvious ethical problems.
Although I must say that research problems I worked on were frequently the result of serendipity and often grew out of my interest in some species or some environment which I found to be particularly appealing—marine birds and tropical islands for example.
Bartholomew, April 1993, unpublished remarks when receiving the Miller Award from the Cooper Ornithological Society.
Although Rick [Richard Smalley] made enormous contributions to science, I believe his worldwide contributions in making so many of us aware of the huge energy problem is even greater and longer-lasting than the beautiful science that he discovered.
As quoted in Eric Berger, Houston Chronicle (28 Oct 2005).
Although the cooking of food presents some unsolved problems, the quick warming of cooked food and the thawing of frozen food both open up some attractive uses. ... There is no important reason why the the housewife of the future should not purchase completely frozen meals at the grocery store just as she buys quick frozen vegetables. With a quick heating, high-frequency unit in her kitchen, food preparation from a pre-cooked, frozen meal becomes a simple matter.
[Predicting home kitchen appliances could be developed from the radionic tube employed to jam enemy radar in World War II.]
[Predicting home kitchen appliances could be developed from the radionic tube employed to jam enemy radar in World War II.]
In 'Physics of Today Become the Engineering of Tomorrow', Proceedings of the National Electronics Conference (1947), Vols. 1-2, 24-25. Note: by 1947 Ratheon was able to demonstrate a refrigerator-sized commercial microwave oven.
Among the current discussions, the impact of new and sophisticated methods in the study of the past occupies an important place. The new 'scientific' or 'cliometric' history—born of the marriage contracted between historical problems and advanced statistical analysis, with economic theory as bridesmaid and the computer as best man—has made tremendous advances in the last generation.
Co-author with Geoffrey Rudolph Elton (1921-94), British historian. Which Road to the Past? Two Views of History (1983), 2.
An expert problem solver must be endowed with two incompatible qualities, a restless imagination and a patient pertinacity.
From In Mathematical Circles (1969).
An undefined problem has an infinite number of solutions.
…...
Any problem can be solved using the materials in the room.
In Peter C. Wensberg, Land's Polaroid: A Company and the Man Who Invented It (1987).
Any scientist of any age who wants to make important discoveries must study important problems. Dull or piffling problems yield dull or piffling answers. It is not not enough that a problem should be “interesting.” … The problem must be such that it matters what the answer is—whether to science generally or to mankind.
From 'What Shall I Do Research On?', Advice to a Young Scientist (1979), 13.
Apart from its healthful mental training as a branch of ordinary education, geology as an open-air pursuit affords an admirable training in habits of observation, furnishes a delightful relief from the cares and routine of everyday life, takes us into the open fields and the free fresh face of nature, leads us into all manner of sequestered nooks, whither hardly any other occupation or interest would be likely to send us, sets before us problems of the highest interest regarding the history of the ground beneath our feet, and thus gives a new charm to scenery which may be already replete with attractions.
Outlines of Field-Geology (1900), 251-2.
Archimedes … had stated that given the force, any given weight might be moved, and even boasted, we are told, relying on the strength of demonstration, that if there were another earth, by going into it he could remove this. Hiero being struck with amazement at this, and entreating him to make good this problem by actual experiment, and show some great weight moved by a small engine, he fixed accordingly upon a ship of burden out of the king’s arsenal, which could not be drawn out of the dock without great labor and many men; and, loading her with many passengers and a full freight, sitting himself the while far off with no great endeavor, but only holding the head of the pulley in his hand and drawing the cords by degrees, he drew the ship in a straight line, as smoothly and evenly, as if she had been in the sea. The king, astonished at this, and convinced of the power of the art, prevailed upon Archimedes to make him engines accommodated to all the purposes, offensive and defensive, of a siege. … the apparatus was, in most opportune time, ready at hand for the Syracusans, and with it also the engineer himself.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
Archimedes had discovered the truth about several important natural laws, but more significant—at least from Galileo’s standpoint—was Archimedes’s discovery of a way for a scientist to solve problems: first separating what he truly wants to solve from irrelevant externals and then attacking the core of the problem with boldness and imagination. Galileo realized that this approach was suitable for his own studies.
In Galileo and Newton (1964), 23.
As I look back over my efforts, I would characterize my contributions as being largely in the realm of model building. ... I perceive myself as rather uninhibited, with a certain mathematical facility and more interest in the broad aspect of a problem than the delicate nuances. I am more interested in discovering what is over the next rise than in assiduously cultivating the beautiful garden close at hand.
'Men, Mines and Molecules', Annual Review of Physical Chemistry, 1977, 28, 13.
As long as a branch of science offers an abundance of problems, so long it is alive; a lack of problems foreshadows extinction or the cessation of independent development.
In 'Mathematical Problems', Bulletin American Mathematical Society, 8, 438.
As long as museums and universities send out expeditions to bring to light new forms of living and extinct animals and new data illustrating the interrelations of organisms and their environments, as long as anatomists desire a broad comparative basis human for anatomy, as long as even a few students feel a strong curiosity to learn about the course of evolution and relationships of animals, the old problems of taxonomy, phylogeny and evolution will gradually reassert themselves even in competition with brilliant and highly fruitful laboratory studies in cytology, genetics and physiological chemistry.
'Genetics Versus Paleontology', The American Naturalist, 1917, 51, 623.
As soon as we got rid of the backroom attitude and brought our apparatus fully into the Department with an inexhaustible supply of living patients with fascinating clinical problems, we were able to get ahead really fast. Any new technique becomes more attractive if its clinical usefulness can be demonstrated without harm, indignity or discomfort to the patient... Anyone who is satisfied with his diagnostic ability and with his surgical results is unlikely to contribute much to the launching of a new medical science. He should first be consumed with a divine discontent with things as they are. It greatly helps, of course, to have the right idea at the right time, and quite good ideas may come, Archimedes fashion, in one's bath..
As soon as we touch the complex processes that go on in a living thing, be it plant or animal, we are at once forced to use the methods of this science [chemistry]. No longer will the microscope, the kymograph, the scalpel avail for the complete solution of the problem. For the further analysis of these phenomena which are in flux and flow, the investigator must associate himself with those who have labored in fields where molecules and atoms, rather than multicellular tissues or even unicellular organisms, are the units of study.
'Experimental and Chemical Studies of the Blood with an Appeal for More Extended Chemical Training for the Biological and Medical Investigator', Science (6 Aug 1915), 42, 176.
As the sun eclipses the stars by his brilliancy, so the man of knowledge will eclipse the fame of others in assemblies of the people if he proposes algebraic problems, and still more if he solves them.
In Florian Cajori, History of Mathematics (1893), 92.
As the world of science has grown in size and in power, its deepest problems have changed from the epistemological to the social.
Scientific Knowledge and its Social Problems (1971), 10.
As was predicted at the beginning of the Human Genome Project, getting the sequence will be the easy part as only technical issues are involved. The hard part will be finding out what it means, because this poses intellectual problems of how to understand the participation of the genes in the functions of living cells.
Loose Ends from Current Biology (1997), 71.
At a given instant everything the surgeon knows suddenly becomes important to the solution of the problem. You can't do it an hour later, or tomorrow. Nor can you go to the library and look it up.
Quoted in 'The Best Hope of All', Time (3 May 1963)
At night I would return home, set out a lamp before me, and devote myself to reading and writing. Whenever sleep overcame me or I became conscious of weakening, I would turn aside to drink a cup of wine, so that my strength would return to me. Then I would return to reading. And whenever sleep seized me I would see those very problems in my dream; and many questions became clear to me in my sleep. I continued in this until all of the sciences were deeply rooted within me and I understood them as is humanly possible. Everything which I knew at the time is just as I know it now; I have not added anything to it to this day. Thus I mastered the logical, natural, and mathematical sciences, and I had now reached the science.
— Avicenna
W. E. Gohhnan, The Life of Ibn Sina: A Critical Edition and Annotated Translation (1974), 29-31.
At the present time there exist problems beyond our ability to solve, not because of theoretical difficulties, but because of insufficient means of mechanical computation.
In 'Proposed Automatic Calculating Machine' (1937). As quoted in I. Bernard Cohen, Gregory W. Welch (eds.), Makin' Numbers: Howard Aiken and the Computer (1999), 13.
Atoms for peace. Man is still the greatest miracle and the greatest problem on earth. [Message tapped out by Sarnoff using a telegraph key in a tabletop circuit demonstrating an RCA atomic battery as a power source.]
The Wisdom of Sarnoff and the World of RCA (1967), 251.
Because we are urban dwellers we are obsessed with human problems. … We are so alienated from the world of nature that few of us can name the wild flowers and insects of our locality or notice the rapidity of their extinction.
In 'The Earth as a Living Organism', Essay collected in E. O. Wilson and F. M. Peter (eds.), Biodiversity (1988), Chap. 56, 489. The ellipsis is for a sentence that Webmaster has extracted as a standalone quote on this webpage, beginning: “Even environmentalists seem more concerned”.
Behold the mighty dinosaur,
Famous in prehistoric lore,
Not only for his power and strength
But for his intellectual length.
You will observe by these remains
The creature had two sets of brains—
One in his head (the usual place),
The other at his spinal base.
Thus he could reason 'A priori'
As well as 'A posteriori'.
No problem bothered him a bit
He made both head and tail of it.
So wise was he, so wise and solemn,
Each thought filled just a spinal column.
If one brain found the pressure strong
It passed a few ideas along.
If something slipped his forward mind
'Twas rescued by the one behind.
And if in error he was caught
He had a saving afterthought.
As he thought twice before he spoke
He had no judgment to revoke.
Thus he could think without congestion
Upon both sides of every question.
Oh, gaze upon this model beast
Defunct ten million years at least.
Famous in prehistoric lore,
Not only for his power and strength
But for his intellectual length.
You will observe by these remains
The creature had two sets of brains—
One in his head (the usual place),
The other at his spinal base.
Thus he could reason 'A priori'
As well as 'A posteriori'.
No problem bothered him a bit
He made both head and tail of it.
So wise was he, so wise and solemn,
Each thought filled just a spinal column.
If one brain found the pressure strong
It passed a few ideas along.
If something slipped his forward mind
'Twas rescued by the one behind.
And if in error he was caught
He had a saving afterthought.
As he thought twice before he spoke
He had no judgment to revoke.
Thus he could think without congestion
Upon both sides of every question.
Oh, gaze upon this model beast
Defunct ten million years at least.
'The Dinosaur: A Poem' (1912). In E. H. Colbert (ed.), The Dinosaur Book (1951), 78.
Beware of the problem of testing too many hypotheses; the more you torture the data, the more likely they are to confess, but confessions obtained under duress may not be admissible in the court of scientific opinion.
In Matthew H. Nitecki and Antoni Hoffman (eds.), 'Testing Hypotheses or Fitting Models? Another Look at Mass Extinctions', Neutral Models in Biology (1987), 148.
Biology occupies a position among the sciences both marginal and central. Marginal because, the living world, constituting only a tiny and very “special” part of the universe, it does not seem likely that the study of living beings will ever uncover general laws applicable outside the biosphere. But if the ultimate aim of the whole of science is indeed, as I believe, to clarify man's relationship to the universe, then biology must be accorded a central position, since of all the disciplines it is the one that endeavours to go most directly to the heart of the problems that must be resolved before that of “human nature” can even be framed in other than metaphysical terms.
In Jacques Monod and Austryn Wainhouse (trans.), Chance and Necessity: An Essay on the Natural Philosophy of Modern Biology (1971), xi.
But in its [the corpuscular theory of radiation] relation to the wave theory there is one extraordinary and, at present, insoluble problem. It is not known how the energy of the electron in the X-ray bulb is transferred by a wave motion to an electron in the photographic plate or in any other substance on which the X-rays fall. It is as if one dropped a plank into the sea from the height of 100 ft. and found that the spreading ripple was able, after travelling 1000 miles and becoming infinitesimal in comparison with its original amount, to act upon a wooden ship in such a way that a plank of that ship flew out of its place to a height of 100 ft. How does the energy get from one place to the other?
'Aether Waves and Electrons' (Summary of the Robert Boyle Lecture), Nature, 1921, 107, 374.
By relieving the brain of all unnecessary work, a good notation sets it free to concentrate on more advanced problems, and in effect increases the mental power of the race.
In An Introduction to Mathematics (1911), 59.
Can any thoughtful person admit for a moment that, in a society so constituted that these overwhelming contrasts of luxury and privation are looked upon as necessities, and are treated by the Legislature as matters with which it has practically nothing do, there is the smallest probability that we can deal successfully with such tremendous social problems as those which involve the marriage tie and the family relation as a means of promoting the physical and moral advancement of the race? What a mockery to still further whiten the sepulchre of society, in which is hidden ‘all manner of corruption,’ with schemes for the moral and physical advancement of the race!
In 'Human Selection', Fortnightly Review (1890),48, 330.
Cancer is a biological, not a statistical problem.
'Shoot Out in Marlboro Country', Mother Jones Magazine (Jan 1979), 36.
Cell and tissue, shell and bone, leaf and flower, are so many portions of matter, and it is in obedience to the laws of physics that their particles have been moved, moulded and confirmed. They are no exception to the rule that God always geometrizes. Their problems of form are in the first instance mathematical problems, their problems of growth are essentially physical problems, and the morphologist is, ipso facto, a student of physical science.
On Growth and Form (1917), 7-8.
Change requires experimentation. But no problem can be solved by the same consciousness that created it. Our job is to dream—and to make those dreams happen.
In interview article, 'Designing For The Future', Newsweek (15 May 2005).
Charles Kettering ... said that from studying conventional text-books we fall into a rut and to escape from this takes as much effort as to solve the problem.
In W.I.B. Beveridge, The Art of Scientific Investigation (1957), 2.
Chess problems are the hymn-tunes of mathematics.
'A Mathematician's Apology', in James Roy Newman, The World of Mathematics (2000), 2028.
Child psychology and animal psychology are of relatively slight importance, as compared with the sciences which deal with the corresponding physiological problems of ontogeny and phylogeny.
City wisdom became almost entirely centered on the problems of human relationships, in contrast to the wisdom of any natural tribal group, where relationships with the rest of the animate and inanimate world are still given due place.
In Gaia, a New Look at Life on Earth (1979), 135.
Clarity about the aims and problems of socialism is of greatest significance in our age of transition. Since, under present circumstances, free and unhindered discussion of these problems has come under a powerful taboo, I consider the foundation of this magazine to be an important public service.
…...
Clean water is a great example of something that depends on energy. And if you solve the water problem, you solve the food problem.
In Lecture (2003) at the National Renewable Energy Laboratories in Golden, Colorado, as quoted in obituary, Barnaby J. Feder, 'Richard E. Smalley, 62, Dies; Chemistry Nobel Winner:', New York Times (29 Oct 2005), Late Edition (East Coast), C16.
Committees are dangerous things that need most careful watching. I believe that a research committee can do one useful thing and one only. It can find the workers best fitted to attack a particular problem, bring them together, give them the facilities they need, and leave them to get on with the work. It can review progress from time to time, and make adjustments; but if it tries to do more, it will do harm.
Attributed.
Consciously and systematically Klein sought to enthrall me with the problems of mathematical physics, and to win me over to his conception of these problems as developed it in lecture courses in previous years. I have always regarded Klein as my real teacher only in things mathematical, but also in mathematical physics and in my conception of mechanics.
As quoted in Paul Forman and Armin Hermann, 'Sommerfeld, Arnold (Johannes Wilhelm)', Biography in Dictionary of Scientific Biography (1975), Vol. 12, 526. Cited from 'Autobiographische Skizze', Gesammelte Schriften, Vol 4, 673–682.
Consider a cow. A cow doesn’t have the problem-solving skill of a chimpanzee, which has discovered how to get termites out of the ground by putting a stick into a hole. Evolution has developed the brain’s ability to solve puzzles, and at the same time has produced in our brain a pleasure of solving problems.
In John Tierney, 'For Decades, Puzzling People With Mathematics', New York Times (20 Oct 2009), D2.
Crowds are somewhat like the sphinx of ancient fable: It is necessary to arrive at a solution of the problems offered by their psychology or to resign ourselves to being devoured by them.
From Psychologie des Foules (1895), 90. English text in The Crowd: A Study of the Popular Mind (1897), Book 2, Chap. 2, 95. Original French text: “Les foules sont un peu comme le sphinx de la fable antique: il faut savoir résoudre les problèmes que leur psychologie nous pose, ou se résigner à être dévoré par elles.”
Dad [Walter C. Alvarez] … advised me to sit every few months in my reading chair for an entire evening, close my eyes and try to think of new problems to solve. I took his advice very seriously and have been glad ever since that he did.
In Alvarez: Adventures of a Physicist (1987), 58.
Dad, how do soldiers killing each other solve the world’s problems?
Dialog by Calvin (fictional character) in syndicated newspaper comic strip Calvin and Hobbes (18 Feb 1991).
Daniel Bernoulli used to tell two little adventures, which he said had given him more pleasure than all the other honours he had received. Travelling with a learned stranger, who, being pleased with his conversation, asked his name; “I am Daniel Bernoulli,” answered he with great modesty; “and I,” said the stranger (who thought he meant to laugh at him) “am Isaac Newton.” Another time, having to dine with the celebrated Koenig, the mathematician, who boasted, with some degree of self-complacency, of a difficult problem he had solved with much trouble, Bernoulli went on doing the honours of his table, and when they went to drink coffee he presented Koenig with a solution of the problem more elegant than his own.
In A Philosophical and Mathematical Dictionary (1815), 1, 226.
Despite the recurrence of events in which the debris-basin system fails in its struggle to contain the falling mountains, people who live on the front line are for the most part calm and complacent. It appears that no amount of front-page or prime-time attention will ever prevent such people from masking out the problem.
The Control of Nature
Do not worry about your problems in mathematics. I assure you, my problems with mathematics are much greater than yours.
…...
Does it mean, if you don’t understand something, and the community of physicists don’t understand it, that means God did it? Is that how you want to play this game? Because if it is, here’s a list of the things in the past that the physicists—at the time—didn’t understand … [but now we do understand.] If that’s how you want to invoke your evidence for God, then God is an ever-receding pocket of scientific ignorance, that’s getting smaller and smaller and smaller, as time moves on. So just be ready for that to happen, if that’s how you want to come at the problem. That’s simply the “God of the Gaps” argument that’s been around for ever.
From interview, The Science Studio video series of The Science Network website, episode 'The Moon, the Tides and why Neil DeGrasse Tyson is Colbert’s God' (20 Jan 2011), time 26:58-27:55.
During a conversation with the writer in the last weeks of his life, Sylvester remarked as curious that notwithstanding he had always considered the bent of his mind to be rather analytical than geometrical, he found in nearly every case that the solution of an analytical problem turned upon some quite simple geometrical notion, and that he was never satisfied until he could present the argument in geometrical language.
In Proceedings London Royal Society, 63, 17.
During the half-century that has elapsed since the enunciation of the cell-theory by Schleiden and Schwann, in 1838-39, it has became ever more clearly apparent that the key to all ultimate biological problems must, in the last analysis, be sought in the cell. It was the cell-theory that first brought the structure of plants and animals under one point of view by revealing their common plan of organization. It was through the cell-theory that Kolliker and Remak opened the way to an understanding of the nature of embryological development, and the law of genetic continuity lying at the basis of inheritance. It was the cell-theory again which, in the hands of Virchaw and Max Schultze, inaugurated a new era in the history of physiology and pathology, by showing that all the various functions of the body, in health and in disease, are but the outward expression of cell-activities. And at a still later day it was through the cell-theory that Hertwig, Fol, Van Beneden, and Strasburger solved the long-standing riddle of the fertilization of the egg, and the mechanism of hereditary transmission. No other biological generalization, save only the theory of organic evolution, has brought so many apparently diverse phenomena under a common point of view or has accomplished more far the unification of knowledge. The cell-theory must therefore be placed beside the evolution-theory as one of the foundation stones of modern biology.
In The Cell in Development and Inheritance (1896), 1.
During the time that [Karl] Landsteiner gave me an education in the field of imununology, I discovered that he and I were thinking about the serologic problem in very different ways. He would ask, What do these experiments force us to believe about the nature of the world? I would ask, What is the most. simple and general picture of the world that we can formulate that is not ruled by these experiments? I realized that medical and biological investigators were not attacking their problems the same way that theoretical physicists do, the way I had been in the habit of doing.
‘Molecular Disease’, Pfizer Spectrum (1958), 6:9, 234.
Dust consisting of fine fibers of asbestos, which are insoluble and virtually indestructible, may become a public health problem in the near future. At a recent international conference on the biological effects of asbestos sponsored by the New York Academy of Sciences, participants pointed out on the one hand that workers exposed to asbestos dust are prone in later life to develop lung cancer, and on the other hand that the use of this family of fibrous silicate compounds has expanded enormously during the past few decades. A laboratory curiosity 100 years ago, asbestos today is a major component of building materials.
— Magazine
In Scientific American (Sep 1964). As cited in '50, 100 & 150 Years Ago', Scientific American (Dec 2014), 311, No. 6, 98.
Each new scientific development is due to the pressure of some social need. Of course … insatiable curiosity … is still nothing but a response either to an old problem of nature, or to one arising from new social circumstances.
In 'The Teaching of the History of Science', The Scientific Monthly (Sep 1918), 194.
Each problem that I solved became a rule which served afterwards to solve other problems.
In Discours de la Méthode (1637), collected in Œuvres, vol. VI, 20-21. As translated and cited in epigraph, George Polya, Mathematical Discovery (1981), 1.
Each species has evolved a special set of solutions to the general problems that all organisms must face. By the fact of its existence, a species demonstrates that its members are able to carry out adequately a series of general functions. … These general functions offer a framework within which one can integrate one’s view of biology and focus one’s research. Such a view helps one to avoid becoming lost in a morass of unstructured detail—even though the ways in which different species perform these functions may differ widely. A few obvious examples will suffice. Organisms must remain functionally integrated. They must obtain materials from their environments, and process and release energy from these materials. … They must differentiate and grow, and they must reproduce. By focusing one’s questions on one or another of these obligatory and universal capacities, one can ensure that one’s research will not be trivial and that it will have some chance of achieving broad general applicability.
In 'Integrative Biology: An Organismic Biologist’s Point of View', Integrative and Comparative Biology (2005), 45, 331.
Early in my school career, I turned out to be an incorrigible disciplinary problem. I could understand what the teacher was saying as fast as she could say it, I found time hanging heavy, so I would occasionally talk to my neighbor. That was my great crime, I talked in school.
In In Memory Yet Green: the Autobiography of Isaac Asimov, 1920-1954 (1979), 73.
Education has, thus, become the chief problem of the world, its one holy cause. The nations that see this will survive, and those that fail to do so will slowly perish. There must be re-education of the will and of the heart as well as of the intellect, and the ideals of service must supplant those of selfishness and greed. ... Never so much as now is education the one and chief hope of the world.
Confessions of a Psychologist (1923). Quoted in Bruce A. Kimball, The True Professional Ideal in America: A History (1996), 198.
Electronic calculators can solve problems which the man who made them cannot solve but no government-subsidized commission of engineers and physicists could create a worm.
In 'March', The Twelve Seasons: A Perpetual Calendar for the Country (1949), 184.
Engineering is not merely knowing and being knowledgeable, like a walking encyclopedia; engineering is not merely analysis; engineering is not merely the possession of the capacity to get elegant solutions to non-existent engineering problems; engineering is practicing the art of the organizing forces of technological change ... Engineers operate at the interface between science and society.
In Bert Scalzo, et al., Database Benchmarking: Practical Methods for Oracle & SQL Server (2007), 37.
Engineering is quite different from science. Scientists try to understand nature. Engineers try to make things that do not exist in nature. Engineers stress invention. To embody an invention the engineer must put his idea in concrete terms, and design something that people can use. That something can be a device, a gadget, a material, a method, a computing program, an innovative experiment, a new solution to a problem, or an improvement on what is existing. Since a design has to be concrete, it must have its geometry, dimensions, and characteristic numbers. Almost all engineers working on new designs find that they do not have all the needed information. Most often, they are limited by insufficient scientific knowledge. Thus they study mathematics, physics, chemistry, biology and mechanics. Often they have to add to the sciences relevant to their profession. Thus engineering sciences are born.
Y.C. Fung and P. Tong, Classical and Computational Solid Mechanics (2001), 1.
Engineering is the conscious application of science to the problems of economic production.
1910
Engineers apply the theories and principles of science and mathematics to research and develop economical solutions to practical technical problems. Their work is the link between scientific discoveries and commercial applications. Engineers design products, the machinery to build those products, the factories in which those products are made, and the systems that ensure the quality of the product and efficiency of the workforce and manufacturing process. They design, plan, and supervise the construction of buildings, highways, and transit systems. They develop and implement improved ways to extract, process, and use raw materials, such as petroleum and natural gas. They develop new materials that both improve the performance of products, and make implementing advances in technology possible. They harness the power of the sun, the earth, atoms, and electricity for use in supplying the Nation’s power needs, and create millions of products using power. Their knowledge is applied to improving many things, including the
quality of health care, the safety of food products, and the efficient operation of financial systems.
Bureau of Labor Statistics, Occupational Outlook Handbook (2000) as quoted in Charles R. Lord. Guide to Information Sources in Engineering (2000), 5. This definition has been revised and expanded over time in different issues of the Handbook.
Enlist a great mathematician and a distinguished Grecian; your problem will be solved. Such men can teach in a dwelling-house as well as in a palace. Part of the apparatus they will bring; part we will furnish.
Advice given to the Trustees of Johns Hopkins University on the choice of a professorial staff. In Report of the President of Johns Hopkins University (1888), 29. As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 122.
Equations are Expressions of Arithmetical Computation, and properly have no place in Geometry, except as far as Quantities truly Geometrical (that is, Lines, Surfaces, Solids, and Proportions) may be said to be some equal to others. Multiplications, Divisions, and such sort of Computations, are newly received into Geometry, and that unwarily, and contrary to the first Design of this Science. For whosoever considers the Construction of a Problem by a right Line and a Circle, found out by the first Geometricians, will easily perceive that Geometry was invented that we might expeditiously avoid, by drawing Lines, the Tediousness of Computation. Therefore these two Sciences ought not to be confounded. The Ancients did so industriously distinguish them from one another, that they never introduced Arithmetical Terms into Geometry. And the Moderns, by confounding both, have lost the Simplicity in which all the Elegance of Geometry consists. Wherefore that is Arithmetically more simple which is determined by the more simple Equation, but that is Geometrically more simple which is determined by the more simple drawing of Lines; and in Geometry, that ought to be reckoned best which is geometrically most simple.
In 'On the Linear Construction of Equations', Universal Arithmetic (1769), Vol. 2, 470.
Even fairly good students, when they have obtained the solution of the problem and written down neatly the argument, shut their books and look for something else. Doing so, they miss an important and instructive phase of the work. ... A good teacher should understand and impress on his students the view that no problem whatever is completely exhausted.
In How to Solve It: A New Aspect of Mathematical Method (2004), 14.
Even mistaken hypotheses and theories are of use in leading to discoveries. This remark is true in all the sciences. The alchemists founded chemistry by pursuing chimerical problems and theories which are false. In physical science, which is more advanced than biology, we might still cite men of science who make great discoveries by relying on false theories. It seems, indeed, a necessary weakness of our mind to be able to reach truth only across a multitude of errors and obstacles.
An Introduction to the Study of Experimental Medicine (1865, translation 1927, 1957), 170.
Every new theory as it arises believes in the flush of youth that it has the long sought goal; it sees no limits to its applicability, and believes that at last it is the fortunate theory to achieve the 'right' answer. This was true of electron theory—perhaps some readers will remember a book called The Electrical Theory of the Universe by de Tunzelman. It is true of general relativity theory with its belief that we can formulate a mathematical scheme that will extrapolate to all past and future time and the unfathomed depths of space. It has been true of wave mechanics, with its first enthusiastic claim a brief ten years ago that no problem had successfully resisted its attack provided the attack was properly made, and now the disillusionment of age when confronted by the problems of the proton and the neutron. When will we learn that logic, mathematics, physical theory, are all only inventions for formulating in compact and manageable form what we already know, like all inventions do not achieve complete success in accomplishing what they were designed to do, much less complete success in fields beyond the scope of the original design, and that our only justification for hoping to penetrate at all into the unknown with these inventions is our past experience that sometimes we have been fortunate enough to be able to push on a short distance by acquired momentum.
The Nature of Physical Theory (1936), 136.
Every thoughtful man who hopes for the creation of a contemporary culture knows that this hinges on one central problem: to find a coherent relation between science and the humanities.
With co-author Bruce Mazlish, in The Western Intellectual Tradition (1960).
Everybody’s a mad scientist, and life is their lab. We’re all trying to experiment to find a way to live, to solve problems, to fend off madness and chaos.
In David Chronenberg and Chris Rodley (ed.), Chronenberg on Chronenberg (1992), 7. As cited in Carl Royer, B Lee Cooper, The Spectacle of Isolation in Horror Films: Dark Parades (2013), 55.
Everyone admits that the male is the primary efficient cause in generation, as being that in whom the species or form resides, and they further assert that his genitures emitted in coitus causes the egg both to exist and to be fertile. But how the semen of the cock produces the chick from the egg, neither the philosophers nor the physicians of yesterday or today have satisfactorily explained, or solved the problem formulated by Aristotle.
Disputations Touching the Generation of Animals (1651), trans. Gweneth Whitteridge (1981), Chapter 47, 214.
Experience hobbles progress and leads to abandonment of difficult problems; it encourages the initiated to walk on the shady side of the street in the direction of experiences that have been pleasant. Youth without experience attacks the unsolved problems which maturer age with experience avoids, and from the labors of youth comes progress. Youth has dreams and visions, and will not be denied.
From speech 'In the Time of Henry Jacob Bigelow', given to the Boston Surgical Society, Medalist Meeting (6 Jun 1921). Printed in Journal of the Medical Association (1921), 77, 599.
Experimental psychology itself has, it is true, now and again suffered relapse into a metaphysical treatment of its problems.
Few problems are less recognized, but more important than, the accelerating disappearance of the earth’s biological resources. In pushing other species to extinction, humanity is busy sawing off the limb on which it is perched.
In Ashton Applewhite, William R. Evans and Andrew Frothingham, And I Quote (2003)
Fine, fine; don't do anything to patch it up. The way things are going, gangrene will set in. Then we can amputate and clean up the problem once and for all.
Ray Boundy and J. Laurence Amos (eds.), A History of the Dow Chemical Physics Lab, The Freedom to Be Creative (1990), 180.
For me, the first challenge for computing science is to discover how to maintain order in a finite, but very large, discrete universe that is intricately intertwined. And a second, but not less important challenge is how to mould what you have achieved in solving the first problem, into a teachable discipline: it does not suffice to hone your own intellect (that will join you in your grave), you must teach others how to hone theirs. The more you concentrate on these two challenges, the clearer you will see that they are only two sides of the same coin: teaching yourself is discovering what is teachable.
…...
For some months the astronomer Halley and other friends of Newton had been discussing the problem in the following precise form: what is the path of a body attracted by a force directed toward a fixed point, the force varying in intensity as the inverse of the distance? Newton answered instantly, “An ellipse.” “How do you know?” he was asked. “Why, I have calculated it.” Thus originated the imperishable Principia, which Newton later wrote out for Halley. It contained a complete treatise on motion.
In The Handmaiden of the Sciences (1937), 37.
For the same energy output as from coal or oil, methane combustion releases only half as much carbon dioxide. This implies that powering a nation entirely by gas reduces emissions of carbon dioxide by half. … The problem with [production leaks and other escapes of] … methane is that this substance is twenty-four times more potent a greenhouse gas than carbon dioxide.
In The Revenge of Gaia: Earth’s Climate Crisis & The Fate of Humanity (2006, 2007), 95.
For the saving the long progression of the thoughts to remote and first principles in every case, the mind should provide itself several stages; that is to say, intermediate principles, which it might have recourse to in the examining those positions that come in its way. These, though they are not self-evident principles, yet, if they have been made out from them by a wary and unquestionable deduction, may be depended on as certain and infallible truths, and serve as unquestionable truths to prove other points depending upon them, by a nearer and shorter view than remote and general maxims. … And thus mathematicians do, who do not in every new problem run it back to the first axioms through all the whole train of intermediate propositions. Certain theorems that they have settled to themselves upon sure demonstration, serve to resolve to them multitudes of propositions which depend on them, and are as firmly made out from thence as if the mind went afresh over every link of the whole chain that tie them to first self-evident principles.
In The Conduct of the Understanding, Sect. 21.
FORTRAN —’the infantile disorder’—, by now nearly 20 years old, is hopelessly inadequate for whatever computer application you have in mind today: it is now too clumsy, too risky, and too expensive to use. PL/I —’the fatal disease’— belongs more to the problem set than to the solution set. It is practically impossible to teach good programming to students that have had a prior exposure to BASIC: as potential programmers they are mentally mutilated beyond hope of regeneration. The use of COBOL cripples the mind; its teaching should, therefore, be regarded as a criminal offence. APL is a mistake, carried through to perfection. It is the language of the future for the programming techniques of the past: it creates a new generation of coding bums.
…...
Four college students taking a class together, had done so well through the semester, and each had an “A”. They were so confident, the weekend before finals, they went out partying with friends. Consequently, on Monday, they overslept and missed the final. They explained to the professor that they had gone to a remote mountain cabin for the weekend to study, but, unfortunately, they had a flat tire on the way back, didn’t have a spare, and couldn’t get help for a long time. As a result, they missed the final. The professor kindly agreed they could make up the final the following day. When they arrived the next morning, he placed them each in separate rooms, handed each one a test booklet, and told them to begin. The the first problem was simple, worth 5 points. Turning the page they found the next question, written: “(For 95 points): Which tire?”
Free men are aware of the imperfection inherent in human affairs, and they are willing to fight and die for that which is not perfect. They know that basic human problems can have no final solutions, that our freedom, justice, equality, etc. are far from absolute, and that the good life is compounded of half measures, compromises, lesser evils, and gropings toward the perfect. The rejection of approximations and the insistence on absolutes are the manifestation of a nihilism that loathes freedom, tolerance, and equity.
In The Temper of Our Time (1967), 103.
From somewhere, back in my youth, heard Prof say, “Manuel, when faced with a problem you do not understand, do any part of it you do understand, then look at it again.” He had been teaching me something he himself did not understand very well—something in math—but had taught me something far more important, a basic principle.
In The Moon Is a Harsh Mistress (1996), 365. The sentence in quote marks is also listed on this webpage as a quote in its own right.
From the point of view of the pure morphologist the recapitulation theory is an instrument of research enabling him to reconstruct probable lines of descent; from the standpoint of the student of development and heredity the fact of recapitulation is a difficult problem whose solution would perhaps give the key to a true understanding of the real nature of heredity.
Form and Function: A Contribution to the History of Animal Morphology (1916), 312-3.
Geometric writings are not rare in which one would seek in vain for an idea at all novel, for a result which sooner or later might be of service, for anything in fact which might be destined to survive in the science; and one finds instead treatises on trivial problems or investigations on special forms which have absolutely no use, no importance, which have their origin not in the science itself but in the caprice of the author; or one finds applications of known methods which have already been made thousands of times; or generalizations from known results which are so easily made that the knowledge of the latter suffices to give at once the former. Now such work is not merely useless; it is actually harmful because it produces a real incumbrance in the science and an embarrassment for the more serious investigators; and because often it crowds out certain lines of thought which might well have deserved to be studied.
From 'On Some Recent Tendencies in Geometric Investigations', Rivista di Matematica (1891), 43. In Bulletin American Mathematical Society (1904), 443.
Good people are seldom fully recognised during their lifetimes, and here, there are serious problems of corruption. One day it will be realised that my findings should have been acknowledged.
It was difficult, but she always smiled when asked why she went on when recognition eluded her in her own country.
It was difficult, but she always smiled when asked why she went on when recognition eluded her in her own country.
Quoted in obituary by Anthony Tucker, 'Alice Stewart', The Guardian newspaper (28 Jun 2002).
He [General Nathan Bedford Forrest] possessed a remarkable genius for mathematics, a subject in which he had absolutely no training. He could with surprising facility solve the most difficult problems in algebra, geometry, and trigonometry, only requiring that the theorem or rule be carefully read aloud to him.
In Life of General Nathan Bedford Forrest (1899), 627.
He [Lord Bacon] appears to have been utterly ignorant of the discoveries which had just been made by Kepler’s calculations … he does not say a word about Napier’s Logarithms, which had been published only nine years before and reprinted more than once in the interval. He complained that no considerable advance had been made in Geometry beyond Euclid, without taking any notice of what had been done by Archimedes and Apollonius. He saw the importance of determining accurately the specific gravities of different substances, and himself attempted to form a table of them by a rude process of his own, without knowing of the more scientific though still imperfect methods previously employed by Archimedes, Ghetaldus and Porta. He speaks of the εὕρηκα of Archimedes in a manner which implies that he did not clearly appreciate either the problem to be solved or the principles upon which the solution depended. In reviewing the progress of Mechanics, he makes no mention either of Archimedes, or Stevinus, Galileo, Guldinus, or Ghetaldus. He makes no allusion to the theory of Equilibrium. He observes that a ball of one pound weight will fall nearly as fast through the air as a ball of two, without alluding to the theory of acceleration of falling bodies, which had been made known by Galileo more than thirty years before. He proposed an inquiry with regard to the lever,—namely, whether in a balance with arms of different length but equal weight the distance from the fulcrum has any effect upon the inclination—though the theory of the lever was as well understood in his own time as it is now. … He speaks of the poles of the earth as fixed, in a manner which seems to imply that he was not acquainted with the precession of the equinoxes; and in another place, of the north pole being above and the south pole below, as a reason why in our hemisphere the north winds predominate over the south.
From Spedding’s 'Preface' to De Interpretations Naturae Proœmium, in The Works of Francis Bacon (1857), Vol. 3, 511-512. [Note: the Greek word “εὕρηκα” is “Eureka” —Webmaster.]
He who seeks for methods without having a definite problem in mind seeks for the most part in vain.
'Mathematical Problems', Bulletin of the American Mathematical Society (Jul 1902), 8, 444.
Hence, even in the domain of natural science the aid of the experimental method becomes indispensable whenever the problem set is the analysis of transient and impermanent phenomena, and not merely the observation of persistent and relatively constant objects.
Here I shall present, without using Analysis [mathematics], the principles and general results of the Théorie, applying them to the most important questions of life, which are indeed, for the most part, only problems in probability. One may even say, strictly speaking, that almost all our knowledge is only probable; and in the small number of things that we are able to know with certainty, in the mathematical sciences themselves, the principal means of arriving at the truth—induction and analogy—are based on probabilities, so that the whole system of human knowledge is tied up with the theory set out in this essay.
Philosophical Essay on Probabilities (1814), 5th edition (1825), trans. Andrew I. Dale (1995), 1.

Heredity is to-day the central problem of biology. This problem may be approached from many sides—that of the breeder, the experimenter, the statistician, the physiologist, the embryologist, the cytologist—but the mechanism of heredity can be studied best by the investigation of the germ cells and their development.
From Address of the vice-president and chairman of Section F, Zoology, American Association for the Advancement of Science, Chicago Meeting (1907-8). Published in 'The Mechanism of Heredity', Science (17 Jan 1908), 27, No. 691, 89-90.
Hieron asked Archimedes to discover, without damaging it, whether a certain crown or wreath was made of pure gold, or if the goldsmith had fraudulently alloyed it with some baser metal. While Archimedes was turning the problem over in his mind, he chanced to be in the bath house. There, as he was sitting in the bath, he noticed that the amount of water that was flowing over the top of it was equal in volume to that part of his body that was immersed. He saw at once a way of solving the problem. He did not delay, but in his joy leaped out of the bath. Rushing naked through the streets towards his home, he cried out in a loud voice that he had found what he sought. For, as he ran, he repeatedly shouted in Greek; “Eureka! Eurekal I’ve found it! I’ve found it!”
Vitrivius Pollio, De Architectura, ix, prologue, section 10.
How is it that there are so many minds that are incapable of understanding mathematics? ... the skeleton of our understanding, ... and actually they are the majority. ... We have here a problem that is not easy of solution, but yet must engage the attention of all who wish to devote themselves to education.
Science and Method (1914, 2003), 117-118.
How many and how curious problems concern the commonest of the sea-snails creeping over the wet sea-weed! In how many points of view may its history be considered! There are its origin and development, the mystery of its generation, the phenomena of its growth, all concerning each apparently insignificant individual; there is the history of the species, the value of its distinctive marks, the features which link it with the higher and lower creatures, the reason why it takes its stand where we place it in the scale of creation, the course of its distribution, the causes of its diffusion, its antiquity or novelty, the mystery (deepest of mysteries) of its first appearance, the changes of the outline of continents and of oceans which have taken place since its advent, and their influence on its own wanderings.
On the Natural History of European Seas. In George Wilson and Archibald Geikie, Memoir of Edward Forbes F.R.S. (1861), 547-8.
Humanity stands ... before a great problem of finding new raw materials and new sources of energy that shall never become exhausted. In the meantime we must not waste what we have, but must leave as much as possible for coming generations.
Chemistry in Modern Life (1925), trans. Clifford Shattuck-Leonard, vii.
Hypotheses are cradle-songs by which the teacher lulls his scholars to sleep. The thoughtful and honest observer is always learning more and more of his limitations; he sees that the further knowledge spreads, the more numerous are the problems that make their appearance.
In The Maxims and Reflections of Goethe (1906), 195.
I believe that a scientist looking at nonscientific problems is just as dumb as the next guy—and when he talks about a nonscientific matter, he will sound as naive as anyone untrained in the matter.
In 'The Value of Science' (Dec 1955), collected in The Pleasure of Finding Things Out: The Best Short Works of Richard P. Feynman (1999, 2005), 142.
I believe that nursing is the compassionate, effective, and humane care given by one who is educated and trained in the art and science of nursing to someone who is in need of help because of problems in health or in activities of his daily life.
As quoted in American Nurses’ Association, Contemporary Minority Leaders in Nursing: Afro-American, Hispanic, Native American Perspectives (1983), 92.
I believe that, as men occupied with the study and treatment of disease, we cannot have too strong a conviction that the problems presented to us are physical problems, which perhaps we may never solve, but still admitting of solution only in one way, namely, by regarding them as part of an unbroken series, running up from the lowest elementary conditions of matter to the highest composition of organic structure.
From Address (7 Aug 1868), the Hunterian Oration, 'Clinical Observation in Relation to medicine in Modern Times' delivered to a meeting of the British Medical Association, Oxford. Collected in Sir William Withey Gull and Theodore Dyke Acland (ed.), A Collection of the Published Writings of William Withey Gull (1896), 4.
I believed that, instead of the multiplicity of rules that comprise logic, I would have enough in the following four, as long as I made a firm and steadfast resolution never to fail to observe them.
The first was never to accept anything as true if I did not know clearly that it was so; that is, carefully to avoid prejudice and jumping to conclusions, and to include nothing in my judgments apart from whatever appeared so clearly and distinctly to my mind that I had no opportunity to cast doubt upon it.
The second was to subdivide each on the problems I was about to examine: into as many parts as would be possible and necessary to resolve them better.
The third was to guide my thoughts in an orderly way by beginning, as if by steps, to knowledge of the most complex, and even by assuming an order of the most complex, and even by assuming an order among objects in! cases where there is no natural order among them.
And the final rule was: in all cases, to make such comprehensive enumerations and such general review that I was certain not to omit anything.
The long chains of inferences, all of them simple and easy, that geometers normally use to construct their most difficult demonstrations had given me an opportunity to think that all the things that can fall within the scope of human knowledge follow from each other in a similar way, and as long as one avoids accepting something as true which is not so, and as long as one always observes the order required to deduce them from each other, there cannot be anything so remote that it cannot be reached nor anything so hidden that it cannot be uncovered.
The first was never to accept anything as true if I did not know clearly that it was so; that is, carefully to avoid prejudice and jumping to conclusions, and to include nothing in my judgments apart from whatever appeared so clearly and distinctly to my mind that I had no opportunity to cast doubt upon it.
The second was to subdivide each on the problems I was about to examine: into as many parts as would be possible and necessary to resolve them better.
The third was to guide my thoughts in an orderly way by beginning, as if by steps, to knowledge of the most complex, and even by assuming an order of the most complex, and even by assuming an order among objects in! cases where there is no natural order among them.
And the final rule was: in all cases, to make such comprehensive enumerations and such general review that I was certain not to omit anything.
The long chains of inferences, all of them simple and easy, that geometers normally use to construct their most difficult demonstrations had given me an opportunity to think that all the things that can fall within the scope of human knowledge follow from each other in a similar way, and as long as one avoids accepting something as true which is not so, and as long as one always observes the order required to deduce them from each other, there cannot be anything so remote that it cannot be reached nor anything so hidden that it cannot be uncovered.
Discourse on Method in Discourse on Method and Related Writings (1637), trans. Desmond M. Clarke, Penguin edition (1999), Part 2, 16.
I came to realize that exaggerated concern about what others are doing can be foolish. It can paralyze effort, and stifle a good idea. One finds that in the history of science almost every problem has been worked out by someone else. This should not discourage anyone from pursuing his own path.
From Theodore von Karman and Lee Edson (ed.), The Wind and Beyond: Theodore von Karman, Pioneer in Aviation and Pathfinder in Science (1967).
I can’t recall a single problem in my life, of any sort, that I ever started on that I didn't solve, or prove that I couldn’t solve it. I never let up, until I had done everything that I could think of, no matter how absurd it might seem as a means to the end I was after.
As quoted in French Strother, 'The Modern Profession of Inventing', World's Work and Play (Jul 1905), 6, No. 32, 186.
I can’t say I’m particularly happy about all the spam and the viruses and the equivalent that we see on the Net, but I think technology can deal with many of the problems that we’re now seeing, whether it’s filtering or whatever, and laws may help a lot.
From transcript of interview by Steve Inskeep, 'Computing Pioneers Discuss the State of the Net', Morning Edition (22 Aug 2005) on npr.org website.
I can’t work well under the conditions at Bell Labs. Walter [Brattain] and I are looking at a few questions relating to point-contact transistors, but [William] Shockley keeps all the interesting problems for himself.
From conversation with Frederick Seitz as quoted in Lillian Hoddeson, 'John Bardeen: A Place to Win Two Nobel Prizes and Make a Hole in One', collected in Lillian Hoddeson (ed.), No Boundaries: University of Illinois Vignettes (2004), Chap. 16, 242.
I carried this problem around in my head basically the whole time. I would wake up with it first thing in the morning, I would be thinking about it all day, and I would be thinking about it when I went to sleep. Without distraction I would have the same thing going round and round in my mind.
Recalling the degree of focus and determination that eventually yielded the proof of Fermat's Last Theorem.
Recalling the degree of focus and determination that eventually yielded the proof of Fermat's Last Theorem.
Quoted in interview for PBS TV program Nova. In William Byers, How Mathematicians Think (2007), 1.
I contend that the continued racial classification of Homo sapiens represents an outmoded approach to the general problem of differentiation within a species. In other words, I reject a racial classification of humans for the same reasons that I prefer not to divide into subspecies the prodigiously variable West Indian land snails that form the subject of my own research.
…...
I decided to study science and, on arrival at Cambridge, became extremely excited and interested in biochemistry when I first heard about it…. It seemed to me that here was a way to really understand living matter and to develop a more scientific basis to many medical problems.
From biographical sketch in Wilhelm Odelberg (ed.) Les Prix Nobel. The Nobel Prizes 1980, (1981).
I did some very technical work in partial differential equations, made an unsuccessful pass at shock waves, worked in scale invariant variational problems, made a poor stab at three manifold topology, learned gauge field theory and then some about applications to four manifolds, and have recently been working in equations with algebraic infinite symmetries. I find that I am bored with anything I understand. My excuse is that I am too poor an expositor to want to spend time on formal matters.
In 'A Personal Profile of Karen K. Uhlenbeck', collected in Susan Ambrose et al., Journeys of Women in Science and Engineering, No Universal Constants (1999).
I distinguish two kinds of "applied" research: problem-solving research — government or commercially initiated, centrally managed and institutionally coupled to a plan for application of the results, useful science—investigator-initiated, competitively evaluated and widely communicated. Then we have basic science—useful also, also investigator-initiated, competitively evaluated and widely communicated.
In Confessions of a Technophile (1994), 31.
I do not think words alone will solve humanity’s present problems. The sound of bombs drowns out
men’s voices. In times of peace I have great faith in the communication of ideas among thinking men, but today, with brute force dominating so many millions of lives, I fear that the appeal to
man’s intellect is fast becoming virtually meaningless.
In 'I Am an American' (22 Jun 1940), Einstein Archives 29-092. Excerpted in David E. Rowe and Robert J. Schulmann, Einstein on Politics: His Private Thoughts and Public Stands on Nationalism, Zionism, War, Peace, and the Bomb (2007), 470. It was during a radio broadcast for the Immigration and Naturalization Service, interviewed by a State Department Official. Einstein spoke following an examination on his application for American citizenship in Trenton, New Jersey. The attack on Pearl Harbor and America’s declaration of war on Japan was still over a year in the future.
I don’t like rats but there’s not much else I don’t like. The problem with rats is they have no fear of human beings, they’re loaded with foul diseases, they would run the place given half the chance…
Interview by Simon Gage in 'David Attenborough: I’m not an animal lover', Metro newspaper (29 Jan 2013, London).
I feel that I have at last struck the solution of a great problem—and the day is coming when telegraph wires will be laid on to houses just like water or gas—and friends converse with each other without leaving home.
Letter (10 Mar 1876) to his father on the day his first words were sent by wire to Mr. Watson. As quoted in Robert V. Bruce, Bell: Alexander Graham Bell and the Conquest of Solitude (1973, 1990), 181.
I found out that the main ability to have was a visual, and also an almost tactile, way to imagine the physical situations, rather than a merely logical picture of the problems. … Very soon I discovered that if one gets a feeling for no more than a dozen … radiation and nuclear constants, one can imagine the subatomic world almost tangibly, and manipulate the picture dimensionally and qualitatively, before calculating more precise relationships.
In Adventures of a Mathematician (1976), 147.
I had an immense advantage over many others dealing with the problem inasmuch as I had no fixed ideas derived from long-established practice to control and bias my mind, and did not suffer from the general belief that whatever is, is right.
In Sir Henry Bessemer, F.R.S.: An Autobiography (1905), 93.
I had made up my mind to find that for which I was searching even if it required the remainder of my life. After innumerable failures I finally uncovered the principle for which I was searching, and I was astounded at its simplicity. I was still more astounded to discover the principle I had revealed not only beneficial in the construction of a mechanical hearing aid but it served as well as means of sending the sound of the voice over a wire. Another discovery which came out of my investigation was the fact that when a man gives his order to produce a definite result and stands by that order it seems to have the effect of giving him what might be termed a second sight which enables him to see right through ordinary problems. What this power is I cannot say; all I know is that it exists and it becomes available only when a man is in that state of mind in which he knows exactly what he wants and is fully determined not to quit until he finds it.
As quoted, without citation, in Mack R. Douglas, Making a Habit of Success: How to Make a Habit of Succeeding, How to Win With High Self-Esteem (1966, 1994), 38. Note: Webmaster is dubious of a quote which seems to appear in only one source, without a citation, decades after Bell’s death. If you know a primary source, please contact Webmaster.
I have been able to solve a few problems of mathematical physics on which the greatest mathematicians since Euler have struggled in vain … But the pride I might have held in my conclusions was perceptibly lessened by the fact that I knew that the solution of these problems had almost always come to me as the gradual generalization of favorable examples, by a series of fortunate conjectures, after many errors. I am fain to compare myself with a wanderer on the mountains who, not knowing the path, climbs slowly and painfully upwards and often has to retrace his steps because he can go no further—then, whether by taking thought or from luck, discovers a new track that leads him on a little till at length when he reaches the summit he finds to his shame that there is a royal road by which he might have ascended, had he only the wits to find the right approach to it. In my works, I naturally said nothing about my mistake to the reader, but only described the made track by which he may now reach the same heights without difficulty.
(1891) As quoted in translation in Leo Koenigsberger and Frances A. Welby (trans.), Hermann von Helmholtz (1906), 180-181.
I have been speculating last night what makes a man a discoverer of undiscovered things; and a most perplexing problem it is. Many men who are very clever - much cleverer than the discoverers - never originate anything.
A Century of Family Letters, 1792-1896
I have no doubt that the fundamental problem the planet faces is the enormous increase in the human population. You see it overrunning everywhere. Places that were very remote when I went there 50 years ago are now overrun.
From interview with Michael Bond, 'It’s a Wonderful Life', New Scientist (14 Dec 2002), 176, No. 2373, 48.
I have paid special attention to those Properties of the Positive Rays which seem to throw light on the problems of the structure of molecules and atoms and the question of chemical combination … I am convinced that as yet we are only at the beginning of the harvest of results which will elucidate the process of chemical combination, and thus bridge over the most serious gap which now exists between Physics and Chemistry.
Rays of Positive Electricity and their Application to Chemical Analyses (1921), v.
I have yet to see any problem, however complicated, which, when you looked at it in the right way, did not become still more complicated.
Quoted in William Thorpe, 'Reduction v. Organicism,' New Scientist, 25 Sep 1969, 43, No 66, 638. As cited in Carl C. Gaither, Statistically Speaking: A Dictionary of Quotations (1996), 187.
I have, also, a good deal of respect for the job they [physicists] did in the first months after Hiroshima. The world desperately needed information on this new problem in the daily life of the planet, and the physicists, after a slow start, did a good job of giving it to them. It hasn’t come out with a fraction of the efficiency that the teachers might have wished, but it was infinitely more effective than anyone would have dared expect.
In 'A Newsman Looks at Physicists', Physics Today (May 1948), 1, No. 1, 15.
I imagined in the beginning, that a few experiments would determine the problem; but experience soon convinced me, that a very great number indeed were necessary before such an art could be brought to any tolerable degree of perfection.
Upon pursuing the ''
Upon pursuing the ''
Preface to An Essay on Combustion with a View to a New Art of Dyeing and Painting (1794), iii. In Marilyn Bailey Ogilvie and Joy Dorothy Harvey, The Biographical Dictionary of Women in Science (2000), 478.
I keep looking for some … problem where someone has made an observation that doesn’t fit into my picture of the universe. If it doesn't fit in, then I find some way of fitting it in.
Interview with George B. Kauffman and Laurie M. Kauffman, in 'Linus Pauling: Reflections', American Scientist (Nov-Dec 1994), 82, No. 6, 522.
I know of no department of natural science more likely to reward a man who goes into it thoroughly than anthropology. There is an immense deal to be done in the science pure and simple, and it is one of those branches of inquiry which brings one into contact with the great problems of humanity in every direction.
…...
I know that most men, including those at ease with problems of the greatest complexity, can seldom accept even the simplest and most obvious truth if it be such as would oblige them to admit the falsity of conclusions which they have delighted in explaining to colleagues, which they have proudly taught to others, and which they have woven, thread by thread, into the fabric of their lives.
Attributed. Quoted in James GleickChaos (1988), 38. Contact webmaster if you know a primary print source.
I never allow myself to become discouraged under any circumstances. … After we had conducted
thousands of experiments on a certain project without solving the problem, … we had learned something. For we had learned for a certainty that the thing couldn’t be done that way, and that we would have to try some other way. We sometimes learn a lot from our failures if we have put into the effort the best thought and work we are capable of.
As quoted from an interview by B.C. Forbes in The American Magazine (Jan 1921), 89.
I once had the honour of hearing the great molecular biologist Jacques Monod talking about creativity in science. I have forgotten his exact words, but he said approximately that, when trying to think through a chemical problem, he would ask himself what he would do if he were an electron.
In 'Introduction to the 30th Anniversary Edition', The Selfish Gene: 30th Anniversary Edition (1976, 2006), xi.
I regard sex as the central problem of life. And now that the problem of religion has practically been settled, and that the problem of labor has at least been placed on a practical foundation, the question of sex—with the racial questions that rest on it—stands before the coming generations as the chief problem for solution. Sex lies at the root of life, and we can never learn to reverence life until we know how to understand sex.
Studies in the Psychology of Sex (1897), Vol. 1, xxx.
I should like to draw attention to the inexhaustible variety of the problems and exercises which it [mathematics] furnishes; these may be graduated to precisely the amount of attainment which may be possessed, while yet retaining an interest and value. It seems to me that no other branch of study at all compares with mathematics in this. When we propose a deduction to a beginner we give him an exercise in many cases that would have been admired in the vigorous days of Greek geometry. Although grammatical exercises are well suited to insure the great benefits connected with the study of languages, yet these exercises seem to me stiff and artificial in comparison with the problems of mathematics. It is not absurd to maintain that Euclid and Apollonius would have regarded with interest many of the elegant deductions which are invented for the use of our students in geometry; but it seems scarcely conceivable that the great masters in any other line of study could condescend to give a moment’s attention to the elementary books of the beginner.
In Conflict of Studies (1873), 10-11.
I should not like to leave an impression that all structural problems can be settled by X-ray analysis or that all crystal structures are easy to solve. I seem to have spent much more of my life not solving structures than solving them.
In 'X-ray Analysis of Complicated Molecules', Nobel Lecture (11 Dec 1964). In Nobel Lectures: Chemistry 1942-1962 (1964), 88.
I sometimes ask myself how it came about that I was the one to develop the theory of relativity. The reason, I think, is that a normal adult never stops to think about the problem of space and time. These are things which he has thought of as a child. But my intellectual development was retarded, as a result of which I began to wonder about space and time only when I had already grown up.
In Ronald W. Clark, Einstein: The Life and Times (1971), 10.
I think it’s a very valuable thing for a doctor to learn how to do research, to learn how to approach research, something there isn't time to teach them in medical school. They don't really learn how to approach a problem, and yet diagnosis is a problem; and I think that year spent in research is extremely valuable to them.
On mentoring a medical student.
On mentoring a medical student.
Quoted in interview by Mary Ellen Avery (1997)
I think that our cooperative conservation approaches get people to sit down and grapple with problem solving.
…...
I think that the difference between pure and applied mathematics is social rather than scientific. A pure mathematician is paid for making mathematical discoveries. An applied mathematician is paid for the solution of given problems.
When Columbus set sail, he was like an applied mathematician, paid for the search of the solution of a concrete problem: find a way to India. His discovery of the New World was similar to the work of a pure mathematician.
When Columbus set sail, he was like an applied mathematician, paid for the search of the solution of a concrete problem: find a way to India. His discovery of the New World was similar to the work of a pure mathematician.
In S.H. Lui, 'An Interview with Vladimir Arnol’d', Notices of the AMS (Apr 1997) 44, No. 4, 438. Reprinted from the Hong Kong Mathematics Society (Feb 1996).
I took him [Lawrence Bragg] to a young zoologist working on pattern formation in insect cuticles. The zoologist explained how disturbances introduced into these regular patterns pointed to their formation being governed by some kind of gradient. Bragg listened attentively and then exclaimed: “Your disturbed gradient behaves like a stream of sand running downhill and encountering an obstacle.” “Good heavens,” replied the zoologist, “I had been working on this problem for years before this simple analogy occurred to me and you think of it after twenty minutes.”
As quoted in David Phillips, Biographical Memoirs of Fellows of the Royal Society (Nov 1979), 25, 132, citing: Perutz, M.F. 1971 New Sci. & Sci. J. 8 July 1967.
I was fascinated by fractional distillation as a method while still a school-boy, and built in the cellar of my home, which was my combined workshop and laboratory, distillation columns, packed with coke of graded size, some five feet in height. They were made from coffee tins (obtained from the kitchen), with the bottoms removed and soldered together! Experience with them served me in good stead and by the time I graduated I had a good understanding of the problems of fractional distillation.
Nobel Lectures in Chemistry (1999), Vol. 3, 359-360.
I would like it if everyone could make the prejudice vanish as I have that there is really a problem whether ants are machines, whether my brother is a machine, whether we are in the world, or the world is in us, if perhaps behind the word there is matter, power pushes or not, or if Locke is right that the intellect is between us and things. Or whether we are free or not free…
Given as “fashioned from Boltzmann’s notes for his lecture on natural philosophy on October 26, 1904” and translated in John Blackmore (ed.), Ludwig Boltzmann: His Later Life and Philosophy, 1900-1906 (1995), 136. Blackmore indicates (p.133) that since Boltzmann spoke freely, this may not be verbatim for what he actually said, because he did not read his lectures from his notes. However, it does “rather accurately represent his thinking” at the time he wrote his lecture. His Lectures on Natural Philosophy (1903-1906) were reconstructed from Boltzmann’s shorthand notes by Ilse M. Fasol-Boltzmann (ed.), in Ludwig Boltzmann Principien der Naturfolosofti (1990). This quote is translated from p.109.
I would picture myself as a virus, or as a cancer cell, for example, and try to sense what it would be like to be either. I would also imagine myself as the immune system, and I would try to reconstruct what I would do as an immune system engaged in combating a virus or cancer cell. When I had played through a series of such scenarios on a particular problem and had acquired new insights, I would design laboratory experiments accordingly… Based upon the results of the experiment, I would then know what question to ask next… When I observed phenomena in the laboratory that I did not understand, I would also ask questions as if interrogating myself: “Why would I do that if I were a virus or a cancer cell, or the immune system?” Before long, this internal dialogue became second nature to me; I found that my mind worked this way all the time.
In Anatomy of Reality: Merging of Intuition and Reason (1983), 7, footnote b, as quoted and cited in Roger Frantz, Two Minds: Intuition and Analysis in the History of Economic Thought (2006), 7.
I'm not a wizard or a Frankenstein tampering with Nature. We are not creating life. We have merely done what many people try to do in all kinds of medicine—to help nature. We found nature could not put an egg and sperm together, so we did it. We do not see anything immoral in doing that in the interests of the mother. I cannot see anything immoral in trying to help the patient’s problem.
As quoted by thr Associated Press after the birth of Louise Brown, the first baby born by in vitro fertilization. Reprinted in, for example,'First test-tube baby born in England', Toledo Blade (27 Jul 1978), 1. As reported, the first sentence was given in its own quote marks, followed by “Dr. Steptoe said,” so the quote may not have been delivered as a single statement.
I’ll deal with the most difficult problem first. Creation ex nihilo. The adipose argument is that “God did it.” That of course is the lazy man’s elixir. Sort of a cocktail made up of a swig of credulity and a teaspoon full of unwillingness to think. In short, it’s an explanation that avoids explanation.
From transcript of debate (Apr 1998) with William Lane Craig at the Carter Presidential Center, Atlanta, Georgia, 'What is the evidence for/against the existence of God?' on reasonablefaith.org website.
I’m not sure what solutions we’ll find to deal with all our environmental problems, but I’m sure of this: They will be provided by industry; they will be products of technology. Where else can they come from?
Nation's Business (12 Jun 1988).
I’m very intense in my work. At any given moment, I think I know the answer to some problem, and that I’m right. Since science is the only self-correcting human institution I know of, you should not be frightened to take an extreme stand, if that causes the stand to be examined more thoroughly than it might be if you are circumspect. I’ve always been positive about the value of the Hubble constant, knowing full well that it probably isn’t solved.
As quoted in John Noble Wilford, 'Sizing up the Cosmos: An Astronomers Quest', New York Times (12 Mar 1991), C10.
I’ve met a lot of people in important positions, and he [Wernher von Braun] was one that I never had any reluctance to give him whatever kind of credit they deserve. He owned his spot, he knew what he was doing, and he was very impressive when you met with him. He understood the problems. He could come back and straighten things out. He moved with sureness whenever he came up with a decision. Of all the people, as I think back on it now, all of the top management that I met at NASA, many of them are very, very good. But Wernher, relative to the position he had and what he had to do, I think was the best of the bunch.
From interview with Ron Stone (24 May 1999) for NASA Johnson Space Center Oral History Project on NASA website.
I’ve tried to make the men around me feel as I do, that we are embarked as pioneers upon a new science and industry in which our problems are so new and unusual that it behooves no one to dismiss any novel idea with the statement, “It can’t be done.”
Start of Boeing’s quote, inscribed on his memorial at the Boeing Developmental Center, Tukwila, WA, as given in Mike Lombardi, 'Historical Perspective: 50 years at the Leading Edge', Boeing Frontiers (Aug 2009), 8.
If a photographic plate under the center of a lens focused on the heavens is exposed for hours, it comes to reveal stars so far away that even the most powerful telescopes fail to reveal them to the naked eye. In a similar way, time and concentration allow the intellect to perceive a ray of light in the darkness of the most complex problem.
From Reglas y Consejos sobre Investigacíon Cientifica: Los tónicos de la voluntad. (1897), as translated by Neely and Larry W. Swanson, in Advice for a Young Investigator (1999), 34.
If a problem is clearly stated, it has no further interest to the physicist.
In Richard Hamming, Numerical Methods for Scientists and Engineers (1973), 704, footnote, without citation.
If arithmetical skill is the measure of intelligence, then computers have been more intelligent than all human beings all along. If the ability to play chess is the measure, then there are computers now in existence that are more intelligent than any but a very few human beings. However, if insight, intuition, creativity, the ability to view a problem as a whole and guess the answer by the “feel” of the situation, is a measure of intelligence, computers are very unintelligent indeed. Nor can we see right now how this deficiency in computers can be easily remedied, since human beings cannot program a computer to be intuitive or creative for the very good reason that we do not know what we ourselves do when we exercise these qualities.
In Machines That Think (1983).
If I have succeeded in discovering any truths in the sciences…, I can declare that they are but the consequences and results of five or six principal difficulties which I have surmounted, and my encounters with which I reckoned as battles in which victory declared for me.
In Discours de la Méthode (1637), as translated by J. Veitch, A Discourse on Method (1912), 53. Also seen translated as, “If I found any new truths in the sciences…, I can say that they follow from, or depend on, five or six principal problems which I succeeded in solving and which I regard as so many battles where the fortunes of war were on my side.”
If I’m concerned about what an electron does in an amorphous mass then I become an electron. I try to have that picture in my mind and to behave like an electron, looking at the problem in all its dimensions and scales.
Quoted in Timothy L. O’Brien, 'Not Invented here: Are U.S. Innovators Losing Their Competitive Edge?', New York Times (13 Nov 2005), B6.
If the man of science chose to follow the example of historians and pulpit-orators, and to obscure strange and peculiar phenomena by employing a hollow pomp of big and sounding words, this would be his opportunity; for we have approached one of the greatest mysteries which surround the problem of animated nature and distinguish it above all other problems of science. To discover the relations of man and woman to the egg-cell would be almost equivalent of the egg-cell in the body of the mother, the transfer to it by means of the seed, of the physical and mental characteristics of the father, affect all the questions which the human mind has ever raised in regard to existence.
Quoted in Ernst Heinrich Philipp August Haeckel, The Evolution of Man (1897), vol 1, 148.
If the observation of the amount of heat the sun sends the earth is among the most important and difficult in astronomical physics, it may also be termed the fundamental problem of meteorology, nearly all whose phenomena would become predictable, if we knew both the original quantity and kind of this heat.
In Report of the Mount Whitney Expedition, quoted in Charles Greeley Abbot, Adventures in the World of Science (1958), 17. Also quoted and cited in David H. Devorkin, 'Charles Greeley Abbot', Biographical Memoirs (1998), 4.
If the only tool you have is a hammer, then every problem looks like a nail.
If there is a problem you can’t solve, then there is an easier problem you can solve: find it.
Quoted in Preface, How to Solve It: A New Aspect of Mathematical Method (2004), xxi.
If these d'Hérelle bodies were really genes, fundamentally like our chromosome genes, they would give us an utterly new angle from which to attack the gene problem. They are filterable, to some extent isolable, can be handled in test-tubes, and their properties, as shown by their effects on the bacteria, can then be studied after treatment. It would be very rash to call these bodies genes, and yet at present we must confess that there is no distinction known between the genes and them. Hence we can not categorically deny that perhaps we may be able to grind genes in a mortar and cook them in a beaker after all. Must we geneticists become bacteriologists, physiological chemists and physicists, simultaneously with being zoologists and botanists? Let us hope so.
'Variation Due to Change in the Individual Gene', The American Naturalist (1922), 56, 48-9.
If this plane were to crash, we could get a new start on this quasar problem.
Said to colleagues, dramatically cupping his hand over his brow, shortly after the take-off of a propeller plane leaving Austin, Texas, after the Second Texas Symposium for Relativistic Astrophysics in Dec 1964. Various different theories had been presented at the conference. The flight passengers included many of the major scientists in quasar research, including Margaret and Geoffrey Burbridge, Subrahmanyan Chandrasekhar, John Wheeler and Maarten Schmidt.
Said to colleagues, dramatically cupping his hand over his brow, shortly after the take-off of a propeller plane leaving Austin, Texas, after the Second Texas Symposium for Relativistic Astrophysics in Dec 1964. Various different theories had been presented at the conference. The flight passengers included many of the major scientists in quasar research, including Margaret and Geoffrey Burbridge, Subrahmanyan Chandrasekhar, John Wheeler and Maarten Schmidt.
As quoted by Arthur I. Miller, Empire of the Stars (2005), 226.
If thou art able, O stranger, to find out all these things and gather them together in your mind, giving all the relations, thou shalt depart crowned with glory and knowing that thou hast been adjudged perfect in this species of wisdom.
From a letter to Eratosthenes, the chief librarian at Alexandria, containing the Cattle Problem, an exceedingly difficult calculation involving huge numbers (which was not solved exactly until the use of a supercomputer in 1981). In David J. Darling, The Universal Book of Mathematics (2004), 23. The debate by scholars regarding whether Archimedes is the true author is in T. L. Heath (ed.), The Works of Archimedes (1897), xxxiv.
If two masters of the same art differ in their statement of it, in all likelihood the insoluble problem lies midway between them.
In The Maxims and Reflections of Goethe (1906), 186.
If we assume that there is only one enzyme present to act as an oxidizing agent, we must assume for it as many different degrees of activity as are required to explain the occurrence of the various colors known to mendelize (three in mice, yellow, brown, and black). If we assume that a different enzyme or group of enzymes is responsible for the production of each pigment we must suppose that in mice at least three such enzymes or groups of enzymes exist. To determine which of these conditions occurs in mice is not a problem for the biologist, but for the chemist. The biologist must confine his attention to determining the number of distinct agencies at work in pigment formation irrespective of their chemical nature. These agencies, because of their physiological behavior, the biologist chooses to call 'factors,' and attempts to learn what he can about their functions in the evolution of color varieties.
Experimental Studies of the Inheritance of Color in Mice (1913), 17-18.
If we compare a mathematical problem with an immense rock, whose interior we wish to penetrate, then the work of the Greek mathematicians appears to us like that of a robust stonecutter, who, with indefatigable perseverance, attempts to demolish the rock gradually from the outside by means of hammer and chisel; but the modern mathematician resembles an expert miner, who first constructs a few passages through the rock and then explodes it with a single blast, bringing to light its inner treasures.
In Die Entwickelung der Mathematik in den letzten Jahrhunderten (1869), 9. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 114. From the original German, “Vergleichen wir ein mathematisches Problem mit einem gewaltigen Felsen, in dessen Inneres wir eindringen wollen, so erscheint die Arbeit der griechischen Mathematiker uns als die eines rüstigen Steinhauers, der mit Hammer und Meissel in unermüdlicher Ausdauer den Felsen langsam von aussen her zu zerbröckeln beginnt; der moderne Mathematiker aber als ein trefflicher Minirer, der diesen Felsen zunächst mit wenigen Gängen durchzieht, von denen aus er dann den Felsblock mit einem gewaltigem Schlage zersprengt und die Schätze des Inneren zu Tage fördert.”
If we consider that part of the theory of relativity which may nowadays in a sense be regarded as bone fide scientific knowledge, we note two aspects which have a major bearing on this theory. The whole development of the theory turns on the question of whether there are physically preferred states of motion in Nature (physical relativity problem). Also, concepts and distinctions are only admissible to the extent that observable facts can be assigned to them without ambiguity (stipulation that concepts and distinctions should have meaning). This postulate, pertaining to epistemology, proves to be of fundamental importance.
'Fundamental ideas and problems of the theory of relativity', Lecture delivered to the Nordic Assembly of Naturalists at Gothenburg, 11 Jul 1923. In Nobel Physics 1901-1921 (1998), 482.
If we look at the problems raised by Aristotle, we are astonished at his gift of observation. What wonderful eyes the Greeks had for many things! Only they committed the mistake of being overhasty, of passing straightway from the phenomenon to the explanation of it, and thereby produced certain theories that are quite inadequate. But this is the mistake of all times, and still made in our own day.
In The Maxims and Reflections of Goethe (1906), 195.
If we turn to the problems to which the calculus owes its origin, we find that not merely, not even primarily, geometry, but every other branch of mathematical physics—astronomy, mechanics, hydrodynamics, elasticity, gravitation, and later electricity and magnetism—in its fundamental concepts and basal laws contributed to its development and that the new science became the direct product of these influences.
Opening of Presidential Address (27 Apr 1907) to the American Mathematical Society, 'The Calculus in Colleges and Technical Schools', published in Bulletin of the American Mathematical Society (Jun 1907), 13, 449.
If we want to solve a problem that we have never solved before, we must leave the door to the unknown ajar.
In 'The Value of Science,' What Do You Care What Other People Think? (1988, 2001), 247. Collected in The Pleasure of Finding Things Out (2000), 149.
If you ask mathematicians what they do, you always get the same answer. They think. They think about difficult and unusual problems. (They never think about ordinary problems—they just write down the answers.)
As translated from Russian in 'A byl li brak?', Literaturnaya Gazeta (5 Dec 1979), 49, 12, as quoted and cited in The American Mathematical Monthly (Nov 1980), 87, No. 97, 696.
If you ask me whether science has solved, or is likely to solve, the problem of this universe, I must shake my head in doubt. We have been talking of matter and force; but whence came matter, and whence came force? You remember the first Napoleon’s question, when the savans who accompanied him to Egypt discussed in his presence the problem of the universe, and solved it to their apparent satisfaction. He looked aloft to the starry heavens, and said—“It is all very well, gentlemen, but who made all these!” That question still remains unanswered, and science makes no attempt to answer it.
Lecture 'On Matter and Force', to nearly 3,000 working men, at the Dundee Meeting of the British Association for the Advancement of Science (Sep 1867), reported in 'Dundee Meeting, 1867', Chemical News and Journal of Physical Science (Nov 1867)
If you don’t work on important problems, it’s not likely that you'll do important work.
As quoted in obituary for Richard Hamming, by Herschel H. Loomis and David S. Potter, in National Academy of Engineering, Memorial Tributes (2002), 123.
If you walk along the street you will encounter a number of scientific problems. Of these, about 80 per cent are insoluble, while 19½ per cent are trivial. There is then perhaps half a per cent where skill, persistence, courage, creativity and originality can make a difference. It is always the task of the academic to swim in that half a per cent, asking the questions through which some progress can be made.
'The Making of a Scientist', Journal of the Royal Society of Arts, June 1983, 406.
Ignorance more frequently begets confidence than does knowledge: it is those who know little, and not those who know much, who so positively assert that this or that problem will never be solved by science.
The Descent of Man (1871), Vol. 1, 3.
In 1735 the solving of an astronomical problem, proposed by the Academy, for which several eminent mathematicians had demanded several months’ time, was achieved in three days by Euler with aid of improved methods of his own. … With still superior methods this same problem was solved by the illustrious Gauss in one hour.
In History of Mathematics (1897), 248.
In 1905, a physicist measuring the thermal conductivity of copper would have faced, unknowingly, a very small systematic error due to the heating of his equipment and sample by the absorption of cosmic rays, then unknown to physics. In early 1946, an opinion poller, studying Japanese opinion as to who won the war, would have faced a very small systematic error due to the neglect of the 17 Japanese holdouts, who were discovered later north of Saipan. These cases are entirely parallel. Social, biological and physical scientists all need to remember that they have the same problems, the main difference being the decimal place in which they appear.
In William G. Cochran, Frederick Mosteller and John W. Tukey, 'Principles of Sampling', Journal of the American Statistical Society, 1954, 49, 31. Collected in Selected Papers of Frederick Mosteller (2006), 290.
In a sense Shapley’s telling me that space was transparent, which I shouldn’t have believed, illustrates a fundamental problem in science, believing what people tell you. Go and find it out for yourself. That same error has persisted in my life and in many other people’s. Authorities are not always authorities on everything; they often cling to their own mistakes.
Oral History Transcript of interview with Dr. Jesse Greenstein by Paul Wright (31 Jul 1974), on website of American Institute of Physics.
In early times, when the knowledge of nature was small, little attempt was made to divide science into parts, and men of science did not specialize. Aristotle was a master of all science known in his day, and wrote indifferently treatises on physics or animals. As increasing knowledge made it impossible for any one man to grasp all scientific subjects, lines of division were drawn for convenience of study and of teaching. Besides the broad distinction into physical and biological science, minute subdivisions arose, and, at a certain stage of development, much attention was, given to methods of classification, and much emphasis laid on the results, which were thought to have a significance beyond that of the mere convenience of mankind.
But we have reached the stage when the different streams of knowledge, followed by the different sciences, are coalescing, and the artificial barriers raised by calling those sciences by different names are breaking down. Geology uses the methods and data of physics, chemistry and biology; no one can say whether the science of radioactivity is to be classed as chemistry or physics, or whether sociology is properly grouped with biology or economics. Indeed, it is often just where this coalescence of two subjects occurs, when some connecting channel between them is opened suddenly, that the most striking advances in knowledge take place. The accumulated experience of one department of science, and the special methods which have been developed to deal with its problems, become suddenly available in the domain of another department, and many questions insoluble before may find answers in the new light cast upon them. Such considerations show us that science is in reality one, though we may agree to look on it now from one side and now from another as we approach it from the standpoint of physics, physiology or psychology.
But we have reached the stage when the different streams of knowledge, followed by the different sciences, are coalescing, and the artificial barriers raised by calling those sciences by different names are breaking down. Geology uses the methods and data of physics, chemistry and biology; no one can say whether the science of radioactivity is to be classed as chemistry or physics, or whether sociology is properly grouped with biology or economics. Indeed, it is often just where this coalescence of two subjects occurs, when some connecting channel between them is opened suddenly, that the most striking advances in knowledge take place. The accumulated experience of one department of science, and the special methods which have been developed to deal with its problems, become suddenly available in the domain of another department, and many questions insoluble before may find answers in the new light cast upon them. Such considerations show us that science is in reality one, though we may agree to look on it now from one side and now from another as we approach it from the standpoint of physics, physiology or psychology.
In article 'Science', Encyclopedia Britannica (1911), 402.
In every case the awakening touch has been the mathematical spirit, the attempt to count, to measure, or to calculate. What to the poet or the seer may appear to be the very death of all his poetry and all his visions—the cold touch of the calculating mind,—this has proved to be the spell by which knowledge has been born, by which new sciences have been created, and hundreds of definite problems put before the minds and into the hands of diligent students. It is the geometrical figure, the dry algebraical formula, which transforms the vague reasoning of the philosopher into a tangible and manageable conception; which represents, though it does not fully describe, which corresponds to, though it does not explain, the things and processes of nature: this clothes the fruitful, but otherwise indefinite, ideas in such a form that the strict logical methods of thought can be applied, that the human mind can in its inner chamber evolve a train of reasoning the result of which corresponds to the phenomena of the outer world.
In A History of European Thought in the Nineteenth Century (1896), Vol. 1, 314.
In fact a favourite problem of [Tyndall] is—Given the molecular forces in a mutton chop, deduce Hamlet or Faust therefrom. He is confident that the Physics of the Future will solve this easily.
Letter to Herbert Spencer (3 Aug 1861). In L. Huxley, The Life and Letters of Thomas Henry Huxley (1900), Vol. 1, 249.
In general the position as regards all such new calculi is this That one cannot accomplish by them anything that could not be accomplished without them. However, the advantage is, that, provided such a calculus corresponds to the inmost nature of frequent needs, anyone who masters it thoroughly is able—without the unconscious inspiration of genius which no one can command—to solve the respective problems, yea, to solve them mechanically in complicated cases in which, without such aid, even genius becomes powerless. Such is the case with the invention of general algebra, with the differential calculus, and in a more limited region with Lagrange’s calculus of variations, with my calculus of congruences, and with Möbius’s calculus. Such conceptions unite, as it were, into an organic whole countless problems which otherwise would remain isolated and require for their separate solution more or less application of inventive genius.
Letter (15 May 1843) to Schumacher, collected in Carl Friedrich Gauss Werke (1866), Vol. 8, 298, as translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath's Quotation-book (1914), 197-198. From the original German, “Überhaupt verhält es sich mit allen solchen neuen Calculs so, dass man durch sie nichts leisten kann, was nicht auch ohne sie zu leisten wäre; der Vortheil ist aber der, dass, wenn ein solcher Calcul dem innersten Wesen vielfach vorkommender Bedürfnisse correspondirt, jeder, der sich ihn ganz angeeignet hat, auch ohne die gleichsam unbewussten Inspirationen des Genies, die niemand erzwingen kann, die dahin gehörigen Aufgaben lösen, ja selbst in so verwickelten Fällen gleichsam mechanisch lösen kann, wo ohne eine solche Hülfe auch das Genie ohnmächtig wird. So ist es mit der Erfindung der Buchstabenrechnung überhaupt; so mit der Differentialrechnung gewesen; so ist es auch (wenn auch in partielleren Sphären) mit Lagranges Variationsrechnung, mit meiner Congruenzenrechnung und mit Möbius' Calcul. Es werden durch solche Conceptionen unzählige Aufgaben, die sonst vereinzelt stehen, und jedesmal neue Efforts (kleinere oder grössere) des Erfindungsgeistes erfordern, gleichsam zu einem organischen Reiche.”
In geologists’ own lives, the least effect of time is that they think in two languages, function on two different scales. … “A million years is a short time—the shortest worth messing with for most problems.”
In Basin and Range (1981), 134.
In less than eight years “The Origin of Species” has produced conviction in the minds of a majority of the most eminent living men of science. New facts, new problems, new difficulties as they arise are accepted, solved, or removed by this theory; and its principles are illustrated by the progress and conclusions of every well established branch of human knowledge.
From a review of four books on the subject 'Mimicry, and Other Protective Resemblances Among Animals', in The Westminster Review (Jul 1867), 88, 1. Wallace is identified as the author in the article as reprinted in William Beebe, The Book of Naturalists: An Anthology of the Best Natural History (1988), 108.
In my first publication I might have claimed that I had come to the conclusion, as a result of serious study of the literature and deep thought, that valuable antibacterial substances were made by moulds and that I set out to investigate the problem. That would have been untrue and I preferred to tell the truth that penicillin started as a chance observation. My only merit is that I did not neglect the observation and that I pursued the subject as a bacteriologist. My publication in 1929 was the starting-point of the work of others who developed penicillin especially in the chemical field.
'Penicillin', Nobel Lecture, 11 Dec 1945. In Nobel Lectures: Physiology or Medicine 1942-1962 (1964), 83.
In presenting a mathematical argument the great thing is to give the educated reader the chance to catch on at once to the momentary point and take details for granted: his successive mouthfuls should be such as can be swallowed at sight; in case of accidents, or in case he wishes for once to check in detail, he should have only a clearly circumscribed little problem to solve (e.g. to check an identity: two trivialities omitted can add up to an impasse). The unpractised writer, even after the dawn of a conscience, gives him no such chance; before he can spot the point he has to tease his way through a maze of symbols of which not the tiniest suffix can be skipped.
In A Mathematician's Miscellany (1953). Reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 49.
In scientific matters there was a common language and one standard of values; in moral and political problems there were many. … Furthermore, in science there is a court of last resort, experiment, which is unavailable in human affairs.
In Enrico Fermi: Physicist (1970), 149. Segrè refers to the issues regarding the consequences of mastering the release of atomic energy.
In short, the greatest contribution to real security that science can make is through the extension of the scientific method to the social sciences and a solution of the problem of complete avoidance of war.
In "Science and Security", Science (25 Jun 1948), 107, 665. Written while Director of the U.S. National Bureau of Standards.
In so far as such developments utilise the natural energy running to waste, as in water power, they may be accounted as pure gain. But in so far as they consume the fuel resources of the globe they are very different. The one is like spending the interest on a legacy, and the other is like spending the legacy itself. ... [There is] a still hardly recognised coming energy problem.
Matter and Energy (1911), 139.
In the end, poverty, putridity and pestilence; work, wealth and worry; health, happiness and hell, all simmer down into village problems.
In the medical field [scientific ignorance] could lead to horrendous results. People who don’t understand the difference between a controlled experiment and claims by some quack may die as a result of not taking medical science seriously. One of the most damaging examples of pseudoscience is false memory syndrome. I’m on the board of a foundation exposing this problem.
As quoted by Lawrence Toppman, 'Mastermind', The Charlotte Observer (20 Jun 1993), 6E. As quoted and cited in Dana Richards, 'Martin Gardner: A “Documentary”', collected in Elwyn R. Berlekamp and Tom Rodgers (ed.) The Mathemagician and Pied Puzzler: A Collection in Tribute to Martin Gardner (1999), 11.
In the modern world, science and society often interact in a perverse way. We live in a technological society, and technology causes political problems. The politicians and the public expect science to provide answers to the problems. Scientific experts are paid and encouraged to provide answers. The public does not have much use for a scientist who says, “Sorry, but we don’t know.” The public prefers to listen to scientists who give confident answers to questions and make confident predictions of what will happen as a result of human activities. So it happens that the experts who talk publicly about politically contentious questions tend to speak more clearly than they think. They make confident predictions about the future, and end up believing their own predictions. Their predictions become dogmas which they do not question. The public is led to believe that the fashionable scientific dogmas are true, and it may sometimes happen that they are wrong. That is why heretics who question the dogmas are needed.
Frederick S. Pardee Distinguished Lecture (Oct 2005), Boston University. Collected in 'Heretical Thoughts About Science and Society', A Many-Colored Glass: Reflections on the Place of Life in the Universe (2007), 43-44.
In the next twenty centuries … humanity may begin to understand its most baffling mystery—where are we going? The earth is, in fact, traveling many thousands of miles per hour in the direction of the constellation Hercules—to some unknown destination in the cosmos. Man must understand his universe in order to understand his destiny. Mystery, however, is a very necessary ingredient in our lives. Mystery creates wonder and wonder is the basis for man’s desire to understand. Who knows what mysteries will be solved in our lifetime, and what new riddles will become the challenge of the new generation? Science has not mastered prophesy. We predict too much for the next year yet far too little for the next ten. Responding to challenges is one of democracy’s great strengths. Our successes in space can be used in the next decade in the solution of many of our planet’s problems.
In a speech to a Joint Meeting of the Two Houses of Congress to Receive the Apollo 11 Astronauts (16 Sep 1969), in the Congressional Record.
In the past, you wouldn’t have had any problem in getting a countryman to explain the difference between a blackbird and a song thrush, but you might have that difficulty with a kid now. Equally, if you asked a chap about gorillas in the 19th-century, he wouldn’t have heard of the creatures, but today an urban boy knows all about them.
Explaining how the success of nature documentaries may result in children who know more about gorillas than the wildlife in their own gardens. As reported by Adam Lusher in 'Sir David Attenborough', Daily Mail (28 Feb 2014).
In the sphere of natural science let us remember that we have always to deal with an insoluble problem. Let us prove keen and honest in attending to anything which is in any way brought to our notice, most of all when it does not fit in with our previous ideas. For it is only thereby that we perceive the problem, which does indeed lie in nature, but still more in man.
In The Maxims and Reflections of Goethe (1906), 183.
In the training and in the exercise of medicine a remoteness abides between the field of neurology and that of mental health, psychiatry. It is sometimes blamed to prejudice on the part of the one side or the other. It is both more grave and less grave than that. It has a reasonable basis. It is rooted in the energy-mind problem. Physiology has not enough to offer about the brain in relation to the mind to lend the psychiatrist much help.
In 'The Brain Collaborates With Psyche', Man On His Nature: The Gifford Lectures, Edinburgh 1937-8 (1940), 283.
In this age of specialization men who thoroughly know one field are often incompetent to discuss another. … The old problems, such as the relation of science and religion, are still with us, and I believe present as difficult dilemmas as ever, but they are not often publicly discussed because of the limitations of specialization.
Opening statement, in transcript of talk to the Caltech Lunch Forum (2 May 1956), 'The Relation of Science and Religion', collected in Richard Phillips Feynman and Jeffrey Robbins (ed.), The Pleasure of Finding Things Out: The Best Short Works of Richard P. Feynman (1999, 2005), 245-246.
In working out physical problems there should be, in the first place, no pretence of rigorous formalism. The physics will guide the physicist along somehow to useful and important results, by the constant union of physical and geometrical or analytical ideas. The practice of eliminating the physics by reducing a problem to a purely mathematical exercise should be avoided as much as possible. The physics should be carried on right through, to give life and reality to the problem, and to obtain the great assistance which the physics gives to the mathematics.
In Electromagnetic Theory (1892), Vol. 2, 5.
Indeed, the aim of teaching [mathematics] should be rather to strengthen his [the pupil’s] faculties, and to supply a method of reasoning applicable to other subjects, than to furnish him with an instrument for solving practical problems.
In John Perry (ed.), Discussion on the Teaching of Mathematics (1901), 84. The discussion took place on 14 Sep 1901 at the British Association at Glasgow, during a joint meeting of the mathematics and physics sections with the education section. The proceedings began with an address by John Perry. Magnus spoke in the Discussion that followed.
Indeed, the most important part of engineering work—and also of other scientific work—is the determination of the method of attacking the problem, whatever it may be, whether an experimental investigation, or a theoretical calculation. … It is by the choice of a suitable method of attack, that intricate problems are reduced to simple phenomena, and then easily solved.
In Engineering Mathematics: A Series of Lectures Delivered at Union College (1911, 1917), Vol. 2, 275.
Inspiration in the field of science by no means plays any greater role, as academic conceit fancies, than it does in the field of mastering problems of practical life by a modern entrepreneur. On the other hand, and this also is often misconstrued, inspiration plays no less a role in science than it does in the realm of art.
From a Speech (1918) presented at Munich University, published in 1919, and collected in 'Wissenschaft als Beruf', Gessammelte Aufsätze zur Wissenschaftslehre (1922), 524-525. As given in H.H. Gerth and C. Wright-Mills (translators and eds.), 'Science as a Vocation', Max Weber: Essays in Sociology (1946), 136.
Insurance is the biggest single problem with this industry [commercial space flight].
— Pat Bahn
As quoted by Braddock Gaskill, 'TGV Rockets ‘Walking before they can run’', on web page of nasaspaceflight.com (Sep 2005). Gaskill wrote comment, “One barrier to space flight today that 1920’s aviation pioneers were not overly concerned with is a highly litigious society.”
Intellectuals solve problems, geniuses prevent them.
Widely found on the web as an Einstein quote, but Webmaster has not yet found a primary source. Can you help? It is probably yet another example of a “wise” quote to which Einstein’s name has been falsely attributed. For authentic quotes see Albert Einstein Quotes on Problem.
Intelligence is an extremely subtle concept. It’s a kind of understanding that flourishes if it’s combined with a good memory, but exists anyway even in the absence of good memory. It’s the ability to draw consequences from causes, to make correct inferences, to foresee what might be the result, to work out logical problems, to be reasonable, rational, to have the ability to understand the solution from perhaps insufficient information. You know when a person is intelligent, but you can be easily fooled if you are not yourself intelligent.
In Irv Broughton (ed.), The Writer's Mind: Interviews with American Authors (1990), Vol. 2, 57.
Intelligence is important in psychology for two reasons. First, it is one of the most scientifically developed corners of the subject, giving the student as complete a view as is possible anywhere of the way scientific method can be applied to psychological problems. Secondly, it is of immense practical importance, educationally, socially, and in regard to physiology and genetics.
From Intelligence: Its Structure, Growth and Action: Its Structure, Growth and Action (1987), 1.
Investigation may be likened to the long months of pregnancy, and solving a problem to the day of birth. To investigate a problem is, indeed, to solve it.
In Winberg Chai, The Foreign Relations of the People's Republic of China (1972), 46.
Is pure science to be considered as something potentially harmful? Answer: Most certainly! Every child knows that it is potentially exceedingly harmful. … The menace of blowing ourselves up by atom bombs, doing ourselves in by chemical or biological warfare, or by population explosion, is certainly with us. I consider the environment thing a trivial question, by comparison—like housekeeping. In any home, the dishes have to be washed, the floors swept, the beds made, and there must be rules as to who is allowed to produce how much stink and noise, and where in the house: When the garbage piles up, these questions become pressing. But they are momentary problems. Once the house is in order, you still want to live in it, not just sit around enjoying its orderliness. I would be sorry to see Caltech move heavily into this type of applied research. … SCIENCE POTENTIALLY HARMFUL? DEFINITELY.
In 'Homo Scientificus According to Beckett," collected in William Beranek, Jr. (ed.)Science, Scientists, and Society, (1972), 135. Excerpted in Ann E. Kammer, Science, Sex, and Society (1979), 277.
It [an ethical problem with in vitro fertilization] depends on whether you're talking ethics from the standpoint of some religious denomination or from just truly religious people. The Jewish or Catholic faiths, for example, have their own rules. But just religious people, who will make very devoted parents, have no problem with in vitro fertilization.
From address to the annual meeeting of the American Fertility Society in San Francisco (5 Feb 1979), as quoted in a UPI news article, reprinted in, for example, 'Steptoe Discusses Test Tube Ethics', The Milwaukee Journal (6 Feb 1979), 5. As reported, each sentence was separated in its own quote marks, separated by “Dr. Patrick Steptoe said” and “he said,” so the quote may not have been delivered as a single statement.
It appears that the solution of the problem of time and space is reserved to philosophers who, like Leibniz, are mathematicians, or to mathematicians who, like Einstein, are philosophers.
Collected in Paul Arthur Schilpp (ed.), Albert Einstein: Philosopher-Scientist (1959), Vol. 1, 307. Also, in James Louis Jarrett and Sterling M. McMurrin (eds.), Contemporary Philosophy: A Book of Readings (1954), 71.
It appears, nevertheless, that all such simple solutions of the problem of vertebrate ancestry are without warrant. They arise from a very common tendency of the mind, against which the naturalist has to guard himself,—a tendency which finds expression in the very widespread notion that the existing anthropoid apes, and more especially the gorilla, must be looked upon as the ancestors of mankind, if once the doctrine of the descent of man from ape-like forefathers is admitted. A little reflexion suffices to show that any given living form, such as the gorilla, cannot possibly be the ancestral form from which man was derived, since ex-hypothesi that ancestral form underwent modification and development, and in so doing, ceased to exist.
'Vertebrata', entry in Encyclopaedia Britannica, 9th edition (1899), Vol. 24, 180.
It has been asserted … that the power of observation is not developed by mathematical studies; while the truth is, that; from the most elementary mathematical notion that arises in the mind of a child to the farthest verge to which mathematical investigation has been pushed and applied, this power is in constant exercise. By observation, as here used, can only be meant the fixing of the attention upon objects (physical or mental) so as to note distinctive peculiarities—to recognize resemblances, differences, and other relations. Now the first mental act of the child recognizing the distinction between one and more than one, between one and two, two and three, etc., is exactly this. So, again, the first geometrical notions are as pure an exercise of this power as can be given. To know a straight line, to distinguish it from a curve; to recognize a triangle and distinguish the several forms—what are these, and all perception of form, but a series of observations? Nor is it alone in securing these fundamental conceptions of number and form that observation plays so important a part. The very genius of the common geometry as a method of reasoning—a system of investigation—is, that it is but a series of observations. The figure being before the eye in actual representation, or before the mind in conception, is so closely scrutinized, that all its distinctive features are perceived; auxiliary lines are drawn (the imagination leading in this), and a new series of inspections is made; and thus, by means of direct, simple observations, the investigation proceeds. So characteristic of common geometry is this method of investigation, that Comte, perhaps the ablest of all writers upon the philosophy of mathematics, is disposed to class geometry, as to its method, with the natural sciences, being based upon observation. Moreover, when we consider applied mathematics, we need only to notice that the exercise of this faculty is so essential, that the basis of all such reasoning, the very material with which we build, have received the name observations. Thus we might proceed to consider the whole range of the human faculties, and find for the most of them ample scope for exercise in mathematical studies. Certainly, the memory will not be found to be neglected. The very first steps in number—counting, the multiplication table, etc., make heavy demands on this power; while the higher branches require the memorizing of formulas which are simply appalling to the uninitiated. So the imagination, the creative faculty of the mind, has constant exercise in all original mathematical investigations, from the solution of the simplest problems to the discovery of the most recondite principle; for it is not by sure, consecutive steps, as many suppose, that we advance from the known to the unknown. The imagination, not the logical faculty, leads in this advance. In fact, practical observation is often in advance of logical exposition. Thus, in the discovery of truth, the imagination habitually presents hypotheses, and observation supplies facts, which it may require ages for the tardy reason to connect logically with the known. Of this truth, mathematics, as well as all other sciences, affords abundant illustrations. So remarkably true is this, that today it is seriously questioned by the majority of thinkers, whether the sublimest branch of mathematics,—the infinitesimal calculus—has anything more than an empirical foundation, mathematicians themselves not being agreed as to its logical basis. That the imagination, and not the logical faculty, leads in all original investigation, no one who has ever succeeded in producing an original demonstration of one of the simpler propositions of geometry, can have any doubt. Nor are induction, analogy, the scrutinization of premises or the search for them, or the balancing of probabilities, spheres of mental operations foreign to mathematics. No one, indeed, can claim preeminence for mathematical studies in all these departments of intellectual culture, but it may, perhaps, be claimed that scarcely any department of science affords discipline to so great a number of faculties, and that none presents so complete a gradation in the exercise of these faculties, from the first principles of the science to the farthest extent of its applications, as mathematics.
In 'Mathematics', in Henry Kiddle and Alexander J. Schem, The Cyclopedia of Education, (1877.) As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 27-29.
It has been proposed (in despair) to define mathematics as “what mathematicians do.” Only such a broad definition, it was felt, would cover all the things that might become embodied in mathematics; for mathematicians today attack many problems not regarded as mathematics in the past, and what they will do in the future there is no saying.
In 'The Extent of Mathematics', Prelude to Mathematics (1955), 11.
It has been recognized that hydrogen bonds restrain protein molecules to their native configurations, and I believe that as the methods of structural chemistry are further applied to physiological problems it will be found that the significance of the hydrogen bond for physiology is greater than that of any other single structural feature.
Nature of the Chemical Bond and the Structure of Molecules and Crystals (1939), 265.
It is a commonplace of modern technology that problems have solutions before there is knowledge of how they are to be solved.
In The New Industrial State (1977).
It is a curious property of research activity that after the problem has been solved the solution seems obvious. This is true not only for those who have not previously been acquainted with the problem, but also for those who have worked over it for years.
Address at the Franklin Institute (1937). Journal of the Franklin Institute (1937), 224, 277. Also see Paul C. Wensberg, Land's Polaroid: A Company and the Man who Invented It (1987), 31.
It is a serious question whether America, following England’s lead, has not gone into problem-solving too extensively. Certain it is that we are producing no text-books in which the theory is presented in the delightful style which characterizes many of the French works … , or those of the recent Italian school, or, indeed, those of the continental writers in general.
In The Teaching of Elementary Mathematics (1902), 219.
It is a strange fact, characteristic of the incomplete state of our present knowledge, that totally opposing conclusions are drawn about prehistoric conditions on our planet, depending on whether the problem is approached from the biological or the geophysical viewpoint.
In The Origins of Continents and Oceans (4th ed. 1929), trans. John Biram (1966), 5.
It is an occupational risk of biologists to claim, towards the end of their careers, that the problems which they have not solved are insoluble.
'Popper's World', The London Review of Books (18-31 August 1983), 12.
It is apparent to me that the possibilities of the aeroplane, which two or three years ago were thought to hold the solution to the [flying machine] problem, have been exhausted, and that we must turn elsewhere.
…...
It is better to do the right problem the wrong way than the wrong problem the right way.
Quoted in Julie K. Petersen, Fiber Optics Illustrated Dictionary (2003), 435.
It is by no means hopeless to expect to make a machine for really very difficult mathematical problems. But you would have to proceed step-by-step. I think electricity would be the best thing to rely on.
In Charles Sanders Peirce, Max Harold Fisch, Christian J. W. Kloesel Writings of Charles S. Peirce: 1884-1886 (1993), 422.
It is evident, therefore, that one of the most fundamental problems of psychology is that of investigating the laws of mental growth. When these laws are known, the door of the future will in a measure be opened; determination of the child's present status will enable us to forecast what manner of adult he will become.
In The Intelligence of School Children: How Children Differ in Ability, the Use of Mental Tests in School Grading and the Proper Education of Exceptional Children (1919), 136
It is grindingly, creakingly, crashingly obvious that, if Darwinism were really a theory of chance, it couldn’t work. You don't need to be a mathematician or physicist to calculate that an eye or a haemoglobin molecule would take from here to infinity to self-assemble by sheer higgledy-piggledy luck. Far from being a difficulty peculiar to Darwinism, the astronomic improbability of eyes and knees, enzymes and elbow joints and all the other living wonders is precisely the problem that any theory of life must solve, and that Darwinism uniquely does solve. It solves it by breaking the improbability up into small, manageable parts, smearing out the luck needed, going round the back of Mount Improbable and crawling up the gentle slopes, inch by million-year inch. Only God would essay the mad task of leaping up the precipice in a single bound.
In Climbing Mount Improbable (1996), 67-8.
It is no valid objection that science as yet throws no light on the far higher problem of the essence or origin of life. Who can explain gravity? No one now objects to following out the results consequent on this unknown element of attraction...
The Origin of Species (1909), 519-520.
It is not failure but success that is forcing man off this earth. It is not sickness but the triumph of health... Our capacity to survive has expanded beyond the capacity of Earth to support us. The pains we are feeling are growing pains. We can solve growth problems in direct proportion to our capacity to find new worlds... If man stays on Earth, his extinction is sure even if he lasts till the sun expands and destroys him... It is no longer reasonable to assume that the meaning of life lies on this earth alone. If Earth is all there is for man, we are reaching the foreseeable end of man.
…...
It is not possession of the solution, but the recognition of the problem itself that provides a resource and the answers.
In Take Today: The Executive as Dropout (1972), 92.
It is now widely realized that nearly all the “classical” problems of molecular biology have either been solved or will be solved in the next decade. The entry of large numbers of American and other biochemists into the field will ensure that all the chemical details of replication and transcription will be elucidated. Because of this, I have long felt that the future of molecular biology lies in the extension of research to other fields of biology, notably development and the nervous system.
Letter to Max Perua, 5 June 1963. Quoted in William B. Wood (ed.), The Nematode Caenorhabditis Elegans (1988), x-xi.
It is only necessary to check the comic books and Reader’s Digest to see the extent of the influence of applied science on the popular imagination. How much it is used to provide an atmosphere of endless thrill and excitement, quite apart from its accidental menace or utility, one can decide from such typical daily headlines as these:
London, March 10, 1947, Reuters: ROCKET TO MOON SEEN POSSIBLE BUT THOUSANDS TO DIE IN ATTEMPT
Cleveland, January 5, 1948.: LIFE SPAN OF 100, BE YOUNG AT 80, ATOM PREDICTION
Washington, June 11, 1947: SCIENTISTS AWAIT COW’S DEATH TO SOLVE MATHEMATICS PROBLEM
Needham Market, Suffolk, England. (U.P.): VICAR PROPOSES BABIES FOR YEARNING SPINSTERS, TEST-TUBE BABIES WILL PRODUCE ROBOTS
Washington, D.C., January 3, 1948. U.S. FLYER PASSING SONIC BARRIER OPENS NEW VISTAS OF DESTRUCTION ONE OF BRAVEST ACTS IN HISTORY
Those headlines represent “human interest” attempts to gear science to the human nervous system.
London, March 10, 1947, Reuters: ROCKET TO MOON SEEN POSSIBLE BUT THOUSANDS TO DIE IN ATTEMPT
Cleveland, January 5, 1948.: LIFE SPAN OF 100, BE YOUNG AT 80, ATOM PREDICTION
Washington, June 11, 1947: SCIENTISTS AWAIT COW’S DEATH TO SOLVE MATHEMATICS PROBLEM
Needham Market, Suffolk, England. (U.P.): VICAR PROPOSES BABIES FOR YEARNING SPINSTERS, TEST-TUBE BABIES WILL PRODUCE ROBOTS
Washington, D.C., January 3, 1948. U.S. FLYER PASSING SONIC BARRIER OPENS NEW VISTAS OF DESTRUCTION ONE OF BRAVEST ACTS IN HISTORY
Those headlines represent “human interest” attempts to gear science to the human nervous system.
In The Mechanical Bride: Folklore of Industrial Man (1967), 93.
It is sages and grey-haired philosophers who ought to sit up all night reading Alice in Wonderland in order to study that darkest problem of metaphysics, the borderland between reason and unreason, and the nature of the most erratic of spiritual forces, humour, which eternally dances between the two. That we do find a pleasure in certain long and elaborate stories, in certain complicated and curious forms of diction, which have no intelligible meaning whatever, is not a subject for children to play with; it is a subject for psychologists to go mad over.
In 'The Library of the Nursery', in Lunacy and Letters (1958), 26.
It is science alone that can solve the problems of hunger and poverty, of insanitation and literacy, of superstition and tradition, of vast resources running to waste, of a rich country inhabited by starving people. ... The future belongs to science and to those who make friends with science.
Address to the Indian Institute of Science, Proceedings of the National Institute of Science of India (1960), 27, 564, cited in Mary Midgley, The myths We live By (2004), 14., x. In Vinoth Ramachandra, Subverting Global Myths: Theology and the Public Issues Shaping our World (2008), 172.
It is science alone that can solve the problems of hunger and poverty, of insanitation and illiteracy, of superstition and deadening custom and tradition, of vast resources running to waste, of a rich country inhabited by starving people… Who indeed could afford to ignore science today? At every turn we have to seek its aid … the future belongs to science and those who make friends with science.
From address to the Indian Science Congress (26 Dec 1937). As cited in M.J. Vinod and Meena Deshpande, Contemporary Political Theory (2013), 507. An earlier, longer version of the quote is in Atma Ram, 'The Making of Optical Glass in India: Its Lessons for Industrial Development', Proceedings of the National Institute of Sciences of India (1961), 27, 564-5.
It is the man not the method that solves the problem.
In 'Present Problems of Algebra and Analysis', Congress of Arts and Sciences: Universal Exposition, St. Louis, 1904 (1905), Vol. 1, 530.
It is the business of science to offer rational explanations for all the events in the real world, and any scientist who calls on God to explain something is falling down on his job. This applies as much to the start of the expansion as to any other event. If the explanation is not forthcoming at once, the scientist must suspend judgment: but if he is worth his salt he will always maintain that a rational explanation will eventually be found. This is the one piece of dogmatism that a scientist can allow himself—and without it science would be in danger of giving way to superstition every time that a problem defied solution for a few years.
The Mystery of the Expanding Universe (1964), 122.
It is time, therefore, to abandon the superstition that natural science cannot be regarded as logically respectable until philosophers have solved the problem of induction. The problem of induction is, roughly speaking, the problem of finding a way to prove that certain empirical generalizations which are derived from past experience will hold good also in the future.
Language, Truth and Logic (1960), 49.
It is true that M. Fourier believed that the main aim of mathematics was public utility and the explanation of natural phenomena; but a philosopher of his ability ought to have known that the sole aim of science is the honour of the human intellect, and that on this ground a problem in numbers is as important as a problem on the system of the world.
In Letter to Legendre, as quoted in an Address by Emile Picard to the Congress of Science and Art, St. Louis (22 Sep 1904), translated in 'Development of Mathematical Analysis', The Mathematical Gazette (Jul 1905), 3, No. 52, 200. A different translation begins, “It is true that Fourier had the opinion…” on the Karl Jacobi Quotes web page on this site.
It is, so to speak, a scientific tact, which must guide mathematicians in their investigations, and guard them from spending their forces on scientifically worthless problems and abstruse realms, a tact which is closely related to esthetic tact and which is the only thing in our science which cannot be taught or acquired, and is yet the indispensable endowment of every mathematician.
In Die Entwickelung der Mathematik in den letzten Jahrhunderten (1869), 28. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 92. From the original German, “Es ist, so zu sagen, ein wissenschaftlicher Tact, welcher die Mathematiker bei ihren Untersuchungen leiten, und sie davor bewahren muss, ihre Kräfte auf wissenschaftlich werthlose Probleme und abstruse Gebiete zu wenden, ein Tact, der dem ästhetischen nahe verwandt, das einzige ist, was in unserer Wissenschaft nicht gelehrt und gelernt werden kann, aber eine unentbehrliche Mitgift eines Mathematikers sein sollte.”
It isn't that they can't see the solution. It is that they can't see the problem.
'The Point of a Pin', in The Scandal of Father Brown (1935,2000), 142.
It isn’t that they can’t see the solution. It is that they can’t see the problem.
In 'The Point of a Pin', The Scandal of Father Brown (1935), Chap. 7, 209.
It may very properly be asked whether the attempt to define distinct species, of a more or less permanent nature, such as we are accustomed to deal with amongst the higher plants and animals, is not altogether illusory amongst such lowly organised forms of life as the bacteria. No biologist nowadays believes in the absolute fixity of species … but there are two circumstances which here render the problem of specificity even more difficult of solution. The bacteriologist is deprived of the test of mutual fertility or sterility, so valuable in determining specific limits amongst organisms in which sexual reproduction prevails. Further, the extreme rapidity with which generation succeeds generation amongst bacteria offers to the forces of variation and natural selection a field for their operation wholly unparalleled amongst higher forms of life.
'The Evolution of the Streptococci', The Lancet, 1906, 2, 1415-6.
It seems to me that you are solving a problem which goes beyond the limits of physiology in too simple a way. Physiology has realized its problem with fortitude, breaking man down into endless actions and counteractions and reducing him to a crossing, a vortex of reflex acts. Let it now permit sociology to restore him as a whole. Sociology will wrest man from the anatomical theatre and return him to history.
Letter to his son, Alexander, July-Aug 1868. Trans. Roger Smith, Inhibition: History and Meaning in the Sciences of Mind and Brain (1992), 223.
It sometimes seems necessary to suspend one's normal critical faculties not to find the problems of fusion overwhelming.
Science (1976). In Ervan G. Garrison, A History of Engineering and Technology
It was Plato, according to Sosigenes, who set this as a problem for those concerned with these things, through what suppositions of uniform and ordered movements the appearances concerning the movements of the wandering heavenly bodies could be preserved.
— Plato
Simplicius, On Aristotle's On the Heavens, 488.21. Trans. R. W. Sharples.
It was through living among these groups and much more I think, through moving regularly from one to the other and back again that I got occupied with the problem of what, long before I put it on paper, I christened to myself as the ‘two cultures’. For constantly I felt I was moving among two groups [scientists and literary intellectuals] comparable in intelligence, identical in race, not grossly different in social origin, earning about the same incomes, who had almost ceased to communicate at all, who in intellectual, moral and psychological climate had so little in common that instead of going from Burlington House or South Kensington to Chelsea, one might have crossed an ocean.
The Two Cultures: The Rede Lecture (1959), 2. The places mentioned are all in London. Burlington House is the home of the Royal Society and South Kensington is the site of the Natural History Museum, whereas Chelsea represents an affluent centre of artistic life.
It’s important for students to be put in touch with real-world problems. The curriculum should include computer science. Mathematics should include statistics. The curriculums should really adjust.
From address at a conference on Google campus, co-hosted with Common Sense Media and the Joan Ganz Cooney Center at Sesame Workshop 'Breakthrough Learning in the Digital Age'. As quoted in Technology blog report by Dan Fost, 'Google co-founder Sergey Brin wants more computers in schools', Los Angeles Times (28 Oct 2009). On latimesblogs.latimes.com website.
As quoted, without citation, in Can Akdeniz, Fast MBA (2014), 280.
It’s much more effective to allow solutions to problems to emerge from the people close to the problem rather than to impose them from higher up.
Interviewed in 'Simple, Yet Complex', CIO (15 Apr 1998), 64.
It’s not that I’m so smart, it’s just that I stay with problems longer.
…...
Knowledge is a sacred cow, and my problem will be how we can milk her while keeping clear of her horns.
In 'Teaching and Expanding Knowledge,' Science, December 4, 1964.
Language is a guide to 'social reality.' Though language is not ordinarily thought of as essential interest to the students of social science, it powerfully conditions all our thinking about social problems and processes. Human beings do not live in the objective world alone, nor alone in the world of social activity as ordinarily understood, but are very much at the mercy of the particular language which has become the medium of expression for their society. It is quite an illusion to imagine that one adjusts to reality essentially without the use of language and that language is merely an incidental means of solving specific problems of communication or reflection. The fact of the matter is that the 'real world' is to a large extent unconsciously built up on the language habits of the group. No two languages are ever sufficiently similar to be considered as representing the same social reality. The worlds in which different societies live are distinct worlds, not merely the same world with different labels attached.
'The Status of Linguistics as a Science', Language (1929), 5, 207-14. In David Mandelbaum (ed.), Selected Writings of Edward Sapir in Language, Culture, and Personality (1949), 162.
Leibnitz’s discoveries lay in the direction in which all modern progress in science lies, in establishing order, symmetry, and harmony, i.e., comprehensiveness and perspicuity,—rather than in dealing with single problems, in the solution of which followers soon attained greater dexterity than himself.
In Leibnitz (1884), 112.
Liebig taught the world two great lessons. The first was that in order to teach chemistry it was necessary that students should be taken into a laboratory. The second lesson was that he who is to apply scientific thought and method to industrial problems must have a thorough knowledge of the sciences. The world learned the first lesson more readily than it learned the second.
Ira Remsen, Address to the Industrial Chemistry Society, Glasgow (1910). Quoted in Frederick Hutton Getman, The Life of Ira Remsen (1980), 121-122.
Liebig was not a teacher in the ordinary sense of the word. Scientifically productive himself in an unusual degree, and rich in chemical ideas, he imparted the latter to his advanced pupils, to be put by them to experimental proof; he thus brought his pupils gradually to think for themselves, besides showing and explaining to them the methods by which chemical problems might be solved experimentally.
As quoted in G. H. Getman, The Life of Ira Remsen (1980), 18-19.
Life arose as a living molecule or protogene, the progression from this stage to that of the ameba is at least as great as from ameba to man. All the essential problems of living organisms are already solved in the one-celled (or, as many now prefer to say, noncellular) protozoan and these are only elaborated in man or the other multicellular animals. The step from nonlife to life may not have been so complex, after all, and that from cell to multicellular organism is readily comprehensible. The change from protogene to protozoan was probably the most complex that has occurred in evolution, and it may well have taken as long as the change from protozoan to man.
The Meaning of Evolution: A Study of the History of Life and of its Significance for Man (1949), 16
Life is too complicated to permit a complete understanding through the study of whole organisms. Only by simplifying a biological problem—breaking it down into a multitude of individual problems—can you get the answers.
From interview with Neil A. Campbell, in 'Crossing the Boundaries of Science', BioScience (Dec 1986), 36, No. 11, 738.
Listen to the community: it’s defining its own problems, and may well know what to do about them.
As quoted in 'Aphorism of the Month', Journal of Epidemiology and Community Health (Nov 2007), 61, No. 11, 932.
Littlewood, on Hardy’s own estimate, is the finest mathematician he has ever known. He was the man most likely to storm and smash a really deep and formidable problem; there was no one else who could command such a combination of insight, technique and power.
(1943). In Béla Bollobás, Littlewood's Miscellany (1986), Foreward, 22.
Logic is the last scientific ingredient of Philosophy; its extraction leaves behind only a confusion of non-scientific, pseudo problems.
The Unity of Science, trans. Max Black (1934), 22.
Man is born, not to solve the problems of the universe, but to find out where the problem applies, and then to restrain himself within the limits of the comprehensible.
Wed. 12 Oct 1825. Johann Peter Eckermann, Conversations with Goethe, ed. J. K. Moorhead and trans. J. Oxenford (1971), 120.
Man is not a machine, ... although man most certainly processes information, he does not necessarily process it in the way computers do. Computers and men are not species of the same genus. .... No other organism, and certainly no computer, can be made to confront genuine human problems in human terms. ... However much intelligence computers may attain, now or in the future, theirs must always be an intelligence alien to genuine human problems and concerns.
Computer Power and Human Reason: From Judgment to Calculation, (1976) 203 and 223. Also excerpted in Ronald Chrisley (ed.), Artificial Intelligence: Critical Concepts (2000), Vol. 3, 313 and 321. Note that the second ellipsis spans 8 pages.
Man is not born to solve the problem of the universe, but to find out where the problem begins and then restrain himself within the limits of the comprehensible.
The Homiletic Review, Vol. 83-84 (1922), Vol. 84, 290.
Mankind always takes up only such problems as it can solve; since, looking at the matter more closely, we will always find that the problem itself arises only when the material conditions necessary for its solution already exist or are at least in the process of formation.
In Karl Marx and N.I. Stone (trans.), A Contribution to the Critique of Political Economy (1904), 12.
Many people are shrinking from the future and from participation in the movement toward a new, expanded reality. And, like homesick travelers abroad, they are focusing their anxieties on home. The reasons are not far to seek. We are at a turning point in human history. … We could turn our attention to the problems that going to the moon certainly will not solve … But I think this would be fatal to our future. … A society that no longer moves forward does not merely stagnate; it begins to die.
In 'Man On the Moon' (1969) collected in Margaret Mead and Robert B. Textor (ed.), The World Ahead: An Anthropologist Anticipates the Future (2005), 248. The original magazine article was written shortly before the first Moon landing for the lay public, in Redbook (Jun 1969). It was later reprinted in the Congressional Record—Senate (30 Jun 1969), 17725-17726.
Mars is the next frontier, what the Old West was, what America was 500 years ago. It’s been 500 years since Columbus. It’s time to strike out anew. There’s a big argument at the moment. The moon is closer, and we’ve got to go back there sometime. But whether it will ever be settled on a large scale is a question. But Mars—there’s no doubt about it. … Everything you need is on Mars.
The characteristic of human nature, and perhaps our simian family group, is curiosity and exploration. When we stop doing that, we won't be human anymore. You say there's been a decline, well, I’ve seen far more happen in my lifetime than I ever dreamed. And the momentary plateau now, well, many of our problems on Earth can only be solved by space technology. … When we get out of the present sort of slump and confusion, well, I mean the next step is space. It's inevitable.
The characteristic of human nature, and perhaps our simian family group, is curiosity and exploration. When we stop doing that, we won't be human anymore. You say there's been a decline, well, I’ve seen far more happen in my lifetime than I ever dreamed. And the momentary plateau now, well, many of our problems on Earth can only be solved by space technology. … When we get out of the present sort of slump and confusion, well, I mean the next step is space. It's inevitable.
Interview in Sri Lanka by Steve Coll for The Washington Post (9 Mar 1992), B1.
Marxist philosophy holds that the most important problem does not lie in understanding the laws of the objective world and thus being able to explain it, but in applying the knowledge of these laws actively to change the world.
From 'On Practice,' (Jul 1937), in Quotations from Chairman Mao Tse-Tung (2017), 106.
Marxists are more right than wrong when they argue that the problems scientists take up,. the way they go about solving them, and even the solutions they arc inclined to accept, arc conditioned by the intellectual, social, and economic environments in which they live and work.
In Mankind Evolving: The Evolution of the Human Species, 128. As cited in Ted Woods & Alan Grant, Reason in Revolt - Dialectical Philosophy and Modern Science (2003), Vol. 2, 183.
Mathematicians attach great importance to the elegance of their methods and their results. This is not pure dilettantism. What is it indeed that gives us the feeling of elegance in a solution, in a demonstration? It is the harmony of the diverse parts, their symmetry, their happy balance; in a word it is all that introduces order, all that gives unity, that permits us to see clearly and to comprehend at once both the ensemble and the details. But this is exactly what yields great results, in fact the more we see this aggregate clearly and at a single glance, the better we perceive its analogies with other neighboring objects, consequently the more chances we have of divining the possible generalizations. Elegance may produce the feeling of the unforeseen by the unexpected meeting of objects we are not accustomed to bring together; there again it is fruitful, since it thus unveils for us kinships before unrecognized. It is fruitful even when it results only from the contrast between the simplicity of the means and the complexity of the problem set; it makes us then think of the reason for this contrast and very often makes us see that chance is not the reason; that it is to be found in some unexpected law. In a word, the feeling of mathematical elegance is only the satisfaction due to any adaptation of the solution to the needs of our mind, and it is because of this very adaptation that this solution can be for us an instrument. Consequently this esthetic satisfaction is bound up with the economy of thought.
In 'The Future of Mathematics', Monist, 20, 80. Translated from the French by George Bruce Halsted.
Mathematicians have long since regarded it as demeaning to work on problems related to elementary geometry in two or three dimensions, in spite of the fact that it it precisely this sort of mathematics which is of practical value.
As coauthor with and G.C. Shephard, in Handbook of Applicable Mathematics, Volume V, Combinatorics and Geometry (1985), v.
Mathematics … above all other subjects, makes the student lust after knowledge, fills him, as it were, with a longing to fathom the cause of things and to employ his own powers independently; it collects his mental forces and concentrates them on a single point and thus awakens the spirit of individual inquiry, self-confidence and the joy of doing; it fascinates because of the view-points which it offers and creates certainty and assurance, owing to the universal validity of its methods. Thus, both what he receives and what he himself contributes toward the proper conception and solution of a problem, combine to mature the student and to make him skillful, to lead him away from the surface of things and to exercise him in the perception of their essence. A student thus prepared thirsts after knowledge and is ready for the university and its sciences. Thus it appears, that higher mathematics is the best guide to philosophy and to the philosophic conception of the world (considered as a self-contained whole) and of one’s own being.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 40. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 49.
Mathematics is a public activity. It occurs in a social context and has social consequences. Posing a problem, formulating a definition, proving a theorem are none of them private acts. They are all part of that larger social process we call science.
In 'Mathematics as an Objective Science', The American Mathematical Monthly (Aug-Sep 1979), 86, No. 7, 542. Reprinted in The Mathematical Intelligencer (1983), 5, No. 3.
Mathematics is not yet ready for such problems.
Given, without citation, as the comment by Paul Erdös on the intractability of the 3x + 1 problem, by Jeffrey C. Lagarias in 'The 3x + 1 Problem and Its Generalizations', The American Mathematical Monthly, (Jan 1985), 92, No. 1, 3. Collected in Jeffrey C. Lagarias, The Ultimate Challenge: The 3x+1 Problem (2010), 31.
May every young scientist remember … and not fail to keep his eyes open for the possibility that an irritating failure of his apparatus to give consistent results may once or twice in a lifetime conceal an important discovery.
Commenting on the discovery of thoron gas because one of Rutherford’s students had found his measurements of the ionizing property of thorium were variable. His results even seemed to relate to whether the laboratory door was closed or open. After considering the problem, Rutherford realized a radioactive gas was emitted by thorium, which hovered close to the metal sample, adding to its radioactivity—unless it was dissipated by air drafts from an open door. (Thoron was later found to be argon.)
Commenting on the discovery of thoron gas because one of Rutherford’s students had found his measurements of the ionizing property of thorium were variable. His results even seemed to relate to whether the laboratory door was closed or open. After considering the problem, Rutherford realized a radioactive gas was emitted by thorium, which hovered close to the metal sample, adding to its radioactivity—unless it was dissipated by air drafts from an open door. (Thoron was later found to be argon.)
In Barbara Lovett Cline, Men Who Made a New Physics (1987), 21.
Models so constructed, though of no practical value, serve a useful academic function. The oldest problem in economic education is how to exclude the incompetent. The requirement that there be an ability to master difficult models, including ones for which mathematical competence is required, is a highly useful screening device.
In Economics, Peace, and Laughter (1981), 40-41.
Modern Physics impresses us particularly with the truth of the old doctrine which teaches that there are realities existing apart from our sense-perceptions, and that there are problems and conflicts where these realities are of greater value for us than the richest treasures of the world of experience.
In The Universe in the Light of Modern Physics (1931), 107.
More and more of out colleagues fail to understand our work because of the high specialization of research problems. We must not be discouraged if the products of our labor are not read or even known to exist. The joy of research must be found in doing since every other harvest is uncertain.
Letter to Dr. E. B. Krumhaar (11 Oct 1933), in Journal of Bacteriology (Jan 1934), 27, No. 1, 20.
Most of the scientists in their twenties and thirties who went in 1939 to work on wartime problems were profoundly affected by their experience. The belief that Rutherford's boys were the best boys, that we could do anything that was do-able and could master any subject in a few days was of enormous value.
'The Effect of World War II on the Development of Knowledge in the Physical Sciences', Proceedings of the Royal Society of London, 1975, Series A, 342, 531.
Much later, when I discussed the problem with Einstein, he remarked that the introduction of the cosmological term was the biggest blunder he ever made in his life. But this “blunder,” rejected by Einstein, is still sometimes used by cosmologists even today, and the cosmological constant denoted by the Greek letter Λ rears its ugly head again and again and again.
My World Line (1970). Cited in Edward Robert Harrison, Cosmology: the Science of the Universe (2000), 379, which adds: “The Λ force is referred to by various names, such as the cosmological constant, cosmological term, cosmical constant or cosmical term.”
My conviction based upon extensive experiences of village life is that in India at any rate for generations to come, we shall not be able to make much use of mechanical power for solving the problem of the ever growing poverty of the masses.
Letter (1934) to Mokshagundam Visvesvaraya expressing his different vision for the future of India. Visvesvaraya believed in, and achieved, heavy industry manufacturing in India. See the Visvesvaraya Quotations page for his reply.
My life as a surgeon-scientist, combining humanity and science, has been fantastically rewarding. In our daily patients we witness human nature in the raw–fear, despair, courage, understanding, hope, resignation, heroism. If alert, we can detect new problems to solve, new paths to investigate.
In Tore Frängsmyr and Jan E. Lindsten (eds.), Nobel Lectures: Physiology Or Medicine: 1981-1990 (1993), 565.
My own thinking (and that of many of my colleagues) is based on two general principles, which I shall call the Sequence Hypothesis and the Central Dogma. The direct evidence for both of them is negligible, but I have found them to be of great help in getting to grips with these very complex problems. I present them here in the hope that others can make similar use of them. Their speculative nature is emphasized by their names. It is an instructive exercise to attempt to build a useful theory without using them. One generally ends in the wilderness.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
'On Protein Synthesis', Symposia of the Society for Experimental Biology: The Biological Replication of Macromolecules, 1958, 12, 152-3.
Mythology is wondrous, a balm for the soul. But its problems cannot be ignored. At worst, it buys inspiration at the price of physical impossibility ... At best, it purveys the same myopic view of history that made this most fascinating subject so boring and misleading in grade school as a sequential take of monarchs and battles.
…...
Natural selection produces systems that function no better than necessary. It results in ad hoc adaptive solutions to immediate problems. Whatever enhances fitness is selected. The product of natural selection is not perfection but adequacy, not final answers but limited, short-term solutions.
In 'The role of natural history in contemporary biology', BioScience (1986), 36, 325.
Nature does not consist entirely, or even largely, of problems designed by a Grand Examiner to come out neatly in finite terms, and whatever subject we tackle the first need is to overcome timidity about approximating.
As co-author with Bertha Swirles Jeffreys, in Methods of Mathematical Physics (1946, 1999), 8.
Nature has put itself the problem how to catch in flight light streaming to the earth and to store the most elusive of all powers in rigid form. To achieve this aim, it has covered the crust of earth with organisms which in their life processes absorb the light of the sun and use this power to produce a continuously accumulating chemical difference. ... The plants take in one form of power, light; and produce another power, chemical difference.
In pamphlet, The Organic Motion in its Relation to Metabolism (1845), as translated in Eugene Rabinowitch, Govindjee, Photosynthesis (1969), 9.
Nazis started the Science of Eugenics. It’s the theory that to them, justified the holocaust. The problem is the Science has been broadly accepted around the world, including the United States. We even went as far as to hire the Scientists that were working on it and brought them over here rather then charging them with war crimes. [Project Paperclip] I think it is a very dangerous Science that contains ideologies that are a grave danger to the entire world.
…...
Never depend upon institutions or government to solve any problem. All social movements are founded by, guided by, motivated and seen through by the passion of individuals.
As quoted, without citation, in David Suzuki and Holly Dressel , From Naked Ape to Superspecies: Humanity and the Global Eco-Crisis (1999, 2009), 347.
Never mind what two tons refers to. What is it? How has it entered in so definite a way into our exprerience? Two tons is the reading of the pointer when the elephant was placed on a weighing machine. Let us pass on. … And so we see that the poetry fades out of the problem, and by the time the serious application of exact science begins we are left only with pointer readings.
From Gifford Lecture, Edinburgh, (1927), 'Pointer Readings', collected in The Nature of the Physical World (1928), 252.
Nevertheless, it is necessary to remember that a planned economy is not yet socialism. A planned economy as such may be accompanied by the complete enslavement of the individual. The achievement of socialism requires the solution of some extremely difficult socio-political problems: how is it possible, in view of the far-reaching centralisation of political and economic power, to prevent bureaucracy from becoming all-powerful and overweening? How can the rights of the individual be protected and therewith a democratic counterweight to the power of bureaucracy be assured?
…...
New scientific ideas never spring from a communal body, however organized, but rather from the head of an individually inspired researcher who struggles with his problems in lonely thought and unites all his thought on one single point which is his whole world for the moment.
Address on the 25th anniversary of the Kaiser-Wilhelm Gesellschaft (Jan 1936). Quoted in Surviving the Swastika: Scientific Research in Nazi Germany (1993), 97.
No one has ever found a problem for which Hans [Bethe] did not have an unfair advantage. He could just calculate better than other people.
As quoted in 'Hans Bethe Still Spends Time on His Passion', The New York Times (16 Feb 1997), E9.
No problem can be solved until it is reduced to some simple form. The changing of a vague difficulty into a specific, concrete form is a very essential element in thinking.
Seen, for example, in The Grain and Feed Review (1931), 21, 34.
No research will answer all queries that the future may raise. It is wiser to praise the work for what it has accomplished and then to formulate the problems still to be solved.
Letter to Dr. E. B. Krumhaar (11 Oct 1933), in Journal of Bacteriology (Jan 1934), 27, No. 1, 19.
No scientist is admired for failing in the attempt to solve problems that lie beyond his competence. … Good scientists study the most important problems they think they can solve. It is, after all, their professional business to solve problems, not merely to grapple with them.
The Art of the Soluble: Creativity and Originality in Science (1967), 7.
None of us deny evolution. We know it and study it with pleasure. Catholic universities do not see anything in evolution to prevent a Christian accepting it, but with the reservation that the great problem of the origin of the world and of the creation of man is the secret of God. The Catholic church accepts what science gives it on condition that science reports facts which can be proved, for it is a fact that there is no scientific truth which can contradict eternal truth.
The position of the Catholic Church on the theory of evolution, as stated by Monseigneur Piette, as he introduced the next speaker Dr. Thomas Hunt Morgan, professor of experimental zoology at Columbia University. As given in The School of Education Record of the University of North Dakota (Jun 1926), Vol.11, No. 9, 72.
Nor do I know any study which can compete with mathematics in general in furnishing matter for severe and continued thought. Metaphysical problems may be even more difficult; but then they are far less definite, and, as they rarely lead to any precise conclusion, we miss the power of checking our own operations, and of discovering whether we are thinking and reasoning or merely fancying and dreaming.
In Conflict of Studies (1873), 13.
Not only are there meaningless questions, but many of the problems with which the human intellect has tortured itself turn out to be only 'pseudo problems,' because they can be formulated only in terms of questions which are meaningless. Many of the traditional problems of philosophy, of religion, or of ethics, are of this character. Consider, for example, the problem of the freedom of the will. You maintain that you are free to take either the right- or the left-hand fork in the road. I defy you to set up a single objective criterion by which you can prove after you have made the turn that you might have made the other. The problem has no meaning in the sphere of objective activity; it only relates to my personal subjective feelings while making the decision.
The Nature of Physical Theory (1936), 12.
Nothing afflicted Marcellus so much as the death of Archimedes, who was then, as fate would have it, intent upon working out some problem by a diagram, and having fixed his mind alike and his eyes upon the subject of his speculation, he never noticed the incursion of the Romans, nor that the city was taken. In this transport of study and contemplation, a soldier, unexpectedly coming up to him, commanded him to follow to Marcellus, which he declined to do before he had worked out his problem to a demonstration; the soldier, enraged, drew his sword and ran him through. Others write, that a Roman soldier, running upon him with a drawn sword, offered to kill him; and that Archimedes, looking back, earnestly besought him to hold his hand a little while, that he might not leave what he was at work upon inconclusive and imperfect; but the soldier, nothing moved by his entreaty, instantly killed him. Others again relate, that as Archimedes was carrying to Marcellus mathematical instruments, dials, spheres, and angles, by which the magnitude of the sun might be measured to the sight, some soldiers seeing him, and thinking that he carried gold in a vessel, slew him. Certain it is, that his death was very afflicting to Marcellus; and that Marcellus ever after regarded him that killed him as a murderer; and that he sought for his kindred and honoured them with signal favours.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
Nothing afflicted Marcellus so much as the death of Archimedes, who was then, as fate would have it, intent upon working out some problem by a diagram, and having fixed his mind alike and his eyes upon the subject of his speculation, he never noticed the incursion of the Romans, nor that the city was taken. In this transport of study and contemplation, a soldier, unexpectedly coming up to him, commanded him to follow to Marcellus, which he declined to do before he had worked out his problem to a demonstration; the soldier, enraged, drew his sword and ran him through. Others write, that a Roman soldier, running upon him with a drawn sword, offered to kill him; and that Archimedes, looking back, earnestly besought him to hold his hand a little while, that he might not leave what he was at work upon inconclusive and imperfect; but the soldier, nothing moved by his entreaty, instantly killed him. Others again relate, that as Archimedes was carrying to Marcellus mathematical instruments, dials, spheres, and angles, by which the magnitude of the sun might be measured to the sight, some soldiers seeing him, and thinking that he carried gold in a vessel, slew him. Certain it is, that his death was very afflicting to Marcellus; and that Marcellus ever after regarded him that killed him as a murderer; and that he sought for his kindred and honoured them with signal favours.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
Now, it may be stretching an analogy to compare epidemics of cholera—caused by a known agent—with that epidemic of violent crime which is destroying our cities. It is unlikely that our social problems can be traced to a single, clearly defined cause in the sense that a bacterial disease is ‘caused’ by a microbe. But, I daresay, social science is about as advanced in the late twentieth century as bacteriological science was in the mid nineteenth century. Our forerunners knew something about cholera; they sensed that its spread was associated with misdirected sewage, filth, and the influx of alien poor into crowded, urban tenements. And we know something about street crime; nowhere has it been reported that a member of the New York Stock Exchange has robbed ... at the point of a gun. Indeed, I am naively confident that an enlightened social scientist of the next century will be able to point out that we had available to us at least some of the clues to the cause of urban crime.
'Cholera at the Harvey,' Woods Hole Cantata: Essays on Science and Society (1985).
Now, the causes being four, it is the business of the student of nature to know about them all, and if he refers his problems back to all of them, he will assign the “why” in the way proper to his science—the matter, the form, the mover, that for the sake of which.
Physics, 198a, 22-4. In Jonathan Barnes (ed.), The Complete Works of Aristotle (1984), Vol. I, 338.
Nowadays everyone knows that the US is the world’s biggest polluter, and that with only one 20th of the world’s population it produces a quarter of its greenhouse gas emissions. But the US government, in an abdication of leadership of epic proportions, is refusing to take the problem seriously. … Emissions from the US are up 14% on those in 1990 and are projected to rise by a further 12% over the next decade.
In 'Global Warming is Now a Weapon of Mass Destruction', The Guardian (28 Jul 2003).
Of all the Prizes endowed by Alfred Nobel, only one has an ambiguous name—the Prize for Physiology or Medicine. Nobel believed that physiology was an experimental science like physics and chemistry. On the other hand, medicine was an empirical art that would rarely merit a scientific prize. To the contrary, however, many of the advances in biology during the subsequent 85 years were made by people trained in medicine who were attempting to solve medical problems.
In Banquet Speech, 'The Nobel Prize in Physiology or Medicine 1985', on website nobelprize.org. Published in Les Prix Nobel, 1985: Nobel Prizes, Presentations, Biographies and Lectures (1986).
Often the great scientists, by turning the problem around a bit, changed a defect to an asset. For example, many scientists when they found they couldn't do a problem finally began to study why not. They then turned it around the other way and said, “But of course, this is what it is” and got an important result.
'You and Your Research', Bell Communications Research Colloquium Seminar, 7 Mar 1986.
Ohm found that the results could be summed up in such a simple law that he who runs may read it, and a schoolboy now can predict what a Faraday then could only guess at roughly. By Ohm's discovery a large part of the domain of electricity became annexed by Coulomb's discovery of the law of inverse squares, and completely annexed by Green's investigations. Poisson attacked the difficult problem of induced magnetisation, and his results, though differently expressed, are still the theory, as a most important first approximation. Ampere brought a multitude of phenomena into theory by his investigations of the mechanical forces between conductors supporting currents and magnets. Then there were the remarkable researches of Faraday, the prince of experimentalists, on electrostatics and electrodynamics and the induction of currents. These were rather long in being brought from the crude experimental state to a compact system, expressing the real essence. Unfortunately, in my opinion, Faraday was not a mathematician. It can scarely be doubted that had he been one, he would have anticipated much later work. He would, for instance, knowing Ampere's theory, by his own results have readily been led to Neumann’s theory, and the connected work of Helmholtz and Thomson. But it is perhaps too much to expect a man to be both the prince of experimentalists and a competent mathematician.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
Once we have contemplated a set of data, the mind tends to follow the same line of thought each time and therefore unprofitable lines of thought tend to be repeated. There are two aids to freeing our thought from this conditioning; to abandon the problem temporarily and to discuss it with another person, preferably someone not familiar with our work.
In The Art of Scientific Investigation (1950), 67.
One feature which will probably most impress the mathematician accustomed to the rapidity and directness secured by the generality of modern methods is the deliberation with which Archimedes approaches the solution of any one of his main problems. Yet this very characteristic, with its incidental effects, is calculated to excite the more admiration because the method suggests the tactics of some great strategist who foresees everything, eliminates everything not immediately conducive to the execution of his plan, masters every position in its order, and then suddenly (when the very elaboration of the scheme has almost obscured, in the mind of the spectator, its ultimate object) strikes the final blow. Thus we read in Archimedes proposition after proposition the bearing of which is not immediately obvious but which we find infallibly used later on; and we are led by such easy stages that the difficulties of the original problem, as presented at the outset, are scarcely appreciated. As Plutarch says: “It is not possible to find in geometry more difficult and troublesome questions, or more simple and lucid explanations.” But it is decidedly a rhetorical exaggeration when Plutarch goes on to say that we are deceived by the easiness of the successive steps into the belief that anyone could have discovered them for himself. On the contrary, the studied simplicity and the perfect finish of the treatises involve at the same time an element of mystery. Though each step depends on the preceding ones, we are left in the dark as to how they were suggested to Archimedes. There is, in fact, much truth in a remark by Wallis to the effect that he seems “as it were of set purpose to have covered up the traces of his investigation as if he had grudged posterity the secret of his method of inquiry while he wished to extort from them assent to his results.” Wallis adds with equal reason that not only Archimedes but nearly all the ancients so hid away from posterity their method of Analysis (though it is certain that they had one) that more modern mathematicians found it easier to invent a new Analysis than to seek out the old.
In The Works of Archimedes (1897), Preface, vi.
One is always a long way from solving a problem until one actually has the answer.
…...
One major problem with any science is that people who don't know the conceptual history of their field go round re-inventing the elliptical wheel.
Telephone conversation with Susan Abrams 1983.
One might be led to question whether the scientists acted wisely in presenting the statesmen of the world with this appalling problem. Actually there was no choice. Once basic knowledge is acquired, any attempt at preventing its fruition would be as futile as hoping to stop the earth from revolving around the sun.
'Atomic Energy for Power', Collected Papers (Note e Memorie): The United States 1939-1945 (1962), Vol. 2, 556.
One never knows how hard a problem is until it has been solved. You don’t necessarily know that you will succeed if you work harder or longer.
From interview with Neil A. Campbell, in 'Crossing the Boundaries of Science', BioScience (Dec 1986), 36, No. 11, 739.
One of the big misapprehensions about mathematics that we perpetrate in our classrooms is that the teacher always seems to know the answer to any problem that is discussed. This gives students the idea that there is a book somewhere with all the right answers to all of the interesting questions, and that teachers know those answers. And if one could get hold of the book, one would have everything settled. That’s so unlike the true nature of mathematics.
As quoted in L.A. Steen and D.J. Albers (eds.), Teaching Teachers, Teaching Students (1981), 89.
One of the characteristics of successful scientists is having courage. Once you get your courage up and believe that you can do important problems, then you can. If you think you can't, almost surely you are not going to.
'You and Your Research', Bell Communications Research Colloquium Seminar, 7 Mar 1986.
One of the commonest dietary superstitions of the day is a belief in instinct as a guide to dietary excellence ... with a corollary that the diets of primitive people are superior to diets approved by science ... [and even] that light might be thrown on the problems of human nutrition by study of what chimpanzees eat in their native forests. ... Such notions are derivative of the eighteenth-century fiction of the happy and noble savage.
Nutrition and Public Health', League of Nations Health Organization Quarterly Bulletin (1935) 4, 323–474. In Kenneth J. Carpenter, 'The Work of Wallace Aykroyd: International Nutritionist and Author', The Journal of Nutrition (2007), 137, 873-878.
One of the first and foremost duties of the teacher is not to give his students the impression that mathematical problems have little connection with each other, and no connection at all with anything else. We have a natural opportunity to investigate the connections of a problem when looking back at its solution.
In How to Solve It: A New Aspect of Mathematical Method (2004), 15.
One of the great problems of philosophy, is the relationship between the realm of knowledge and the realm of values. Knowledge is what is; values are what ought to be. I would say that all traditional philosophies up to and including Marxism have tried to derive the “ought” from the “is.” My point of view is that this is impossible, this is a farce.
Quoted in John C. Hess, 'French Nobel Biologist Says World Based On Chance', New York Times (15 Mar 1971), 6. Cited in Barbara Bennett, Linda Amster, Who Said what (and When, and Where, and How) in 1971 (1972, 168.
One of the great problems of the world today is undoubtedly this problem of not being able to talk to scientists, because we don’t understand science; they can’t talk to us because they don’t understand anything else, poor dears.
From dialogue in At the Drop of a Hat, revue performed by Michael Flanders and Donald Swann (1959).
One of the most important choices any researcher makes is picking a significant topic to study. If you choose the right problem, you get important results that transform our perception of the underlying structure of the universe. If you don’t choose the right problem, you may work very hard but only get an interesting result.
Unverified - source citation needed. Can you help?
One reason which has led the organic chemist to avert his mind from the problems of Biochemistry is the obsession that the really significant happenings in the animal body are concerned in the main with substances of such high molecular weight and consequent vagueness of molecular structure as to make their reactions impossible of study by his available and accurate methods. There remains, I find, pretty widely spread, the feeling—due to earlier biological teaching—that, apart from substances which are obviously excreta, all the simpler products which can be found in cells or tissues are as a class mere objects, already too remote from the fundamental biochemical events to have much significance. So far from this being the case, recent progress points in the clearest way to the fact that the molecules with which a most important and significant part of the chemical dynamics of living tissues is concerned are of a comparatively simple character.
In 'The Dynamic Side of Biochemistry', Address (11 Sep 1913) in Report on the 83rd Meeting of the British Association for the Advancement of Science (1914), 657-8.
One striking peculiarity of mathematics is its unlimited power of evolving examples and problems. A student may read a book of Euclid, or a few chapters of Algebra, and within that limited range of knowledge it is possible to set him exercises as real and as interesting as the propositions themselves which he has studied; deductions which might have pleased the Greek geometers, and algebraic propositions which Pascal and Fermat would not have disdained to investigate.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 82.
One wonders whether a generation that demands instant satisfaction of all its needs and instant solution of the world’s problems will produce anything of lasting value. Such a generation, even when equipped with the most modern technology, will be essentially primitive - it will stand in awe of nature, and submit to the tutelage of medicine men.
In Reflections on the Human Condition (1973), 38.
Organic chemistry has literally placed a new nature beside the old. And not only for the delectation and information of its devotees; the whole face and manner of society has been altered by its products. We are clothed, ornamented and protected by forms of matter foreign to Nature; we travel and are propelled, in, on and by them. Their conquest of our powerful insect enemies, their capacity to modify the soil and control its microscopic flora, their ability to purify and protect our water, have increased the habitable surface of the earth and multiplied our food supply; and the dramatic advances in synthetic medicinal chemistry comfort and maintain us, and create unparalleled social opportunities (and problems).
In 'Synthesis', in A. Todd (ed.), Perspectives in Organic Chemistry (1956), 180.
Our atom of carbon enters the leaf, colliding with other innumerable (but here useless) molecules of nitrogen and oxygen. It adheres to a large and complicated molecule that activates it, and simultaneously receives the decisive message from the sky, in the flashing form of a packet of solar light; in an instant, like an insect caught by a spider, it is separated from its oxygen, combined with hydrogen and (one thinks) phosphorus, and finally inserted in a chain, whether long or short does not matter, but it is the chain of life. All this happens swiftly, in silence, at the temperature and pressure of the atmosphere, and gratis: dear colleagues, when we learn to do likewise we will be sicut Deus [like God], and we will have also solved the problem of hunger in the world.
Levi Primo and Raymond Rosenthal (trans.), The Periodic Table (1975, 1984), 227-228. In this final section of his book, Levi imagines the life of a carbon atom. He calls this his first “literary dream”. It came to him at Auschwitz.
Our contemporary culture, primed by population growth and driven by technology, has created problems of environmental degradation that directly affect all of our senses: noise, odors and toxins which bring physical pain and suffering, and ugliness, barrenness, and homogeneity of experience which bring emotional and psychological suffering and emptiness. In short, we are jeopardizing our human qualities by pursuing technology as an end rather than a means. Too often we have failed to ask two necessary questions: First, what human purpose will a given technology or development serve? Second, what human and environmental effects will it have?
Report of the Subcommittee on Air and Water Pollution (7 Aug 1969). 'Environmental Quality: Summary and Discussion of Major Provisions', U.S. Environmental Protection Agency, Legal Compilation, (Jan 1973), Water, Vol. 3, 1365. EPA website.
Our present work sets forth mathematical principles of philosophy. For the basic problem of philosophy seems to be to discover the forces of nature from the phenomena of motions and then to demonstrate the other phenomena from these forces. It is to these ends that the general propositions in books 1 and 2 are directed, while in book 3 our explanation of the system of the world illustrates these propositions.
The Principia: Mathematical Principles of Natural Philosophy (1687), 3rd edition (1726), trans. I. Bernard Cohen and Anne Whitman (1999), Preface to the first edition, 382.
Our problem is that the climate crisis hatched in our laps at a moment in history when political and social conditions were uniquely hostile to a problem of this nature and magnitude—that moment being the tail end of the go-go ’80s, the blastoff point for the crusade to spread deregulated capitalism around the world. Climate change is a collective problem demanding collective action the likes of which humanity has never actually accomplished. Yet it entered mainstream consciousness in the midst of an ideological war being waged on the very idea of the collective sphere.
In 'The Change Within: The Obstacles We Face Are Not Just External', The Nation (12 May 2014).
Our problem is, in fact, to lit the world to our perceptions, and not our perceptions to the world.
In The Organisation of Thought: Educational and Scientific (1917), 228.
Our problems lie not in the genes of the common man but in the ambitions of those with power.
In An Introduction to Anthropology: Volume 2, Ethnology (1971).

Our science, in contrast with others, is not founded on a single period of human history, but has accompanied the development of culture through all its stages. Mathematics is as much interwoven with Greek culture as with the most modern problems in Engineering. She not only lends a hand to the progressive natural sciences but participates at the same time in the abstract investigations of logicians and philosophers.
In Klein und Riecke: Ueber angewandte Mathematik und Physik (1900), 228.
Our two greatest problems are gravity and paperwork. We can lick gravity, but sometimes the paperwork is overwhelming.
In the Chicago Sun Times (10 Jul 1958)
Particular and contingent inventions in the solution of problems, which, though many times more concise than a general method would allow, yet, in my judgment, are less proper to instruct a learner, as acrostics, and such kind of artificial poetry, though never so excellent, would be but improper examples to instruct one that aims at Ovidean poetry.
In Letter to Collins (Macclesfield, 1670), Correspondence of Scientific Men (1841), Vol. 2, 307.
People are the quintessential element in all technology... Once we recognize the inescapable human nexus of all technology our attitude toward the reliability problem is fundamentally changed.
Skeptic (Jul-Aug 1976).
People ask, “Is the science going to run ahead of the ethics?” I don't think that’s always the problem. I think it’s that the science runs ahead of the politics. Bioethics can alert people to something coming down the road, but it doesn't mean policy and politicians are going to pay attention. They tend to respond when there’s an immediate crisis. The job of the ethicist, in some ways, is to warn or be prophetic. You can yell loudly, but you can’t necessarily get everybody to leave the cinema, so to speak.
Interview by Karen Pallarito in The Scientist (Jan 2008), supplement, 74.
Perhaps the central problem we face in all of computer science is how we are to get to the situation where we build on top of the work of others rather than redoing so much of it in a trivially different way.
From Turing Award lecture (1968), 'One Man's View of Computer Science', collected in ACM Turing Award Lectures: The First Twenty Years, 1966 to 1985 (1987), 216. ACM is the Association for Computing Machinery. The lecture is also published in Journal of the ACM (Jan 1969), 16, No. 1, 10.
Perhaps the problem is the seeming need that people have of making black-and-white cutoffs when it comes to certain mysterious phenomena, such as life and consciousness. People seem to want there to be an absolute threshold between the living and the nonliving, and between the thinking and the “merely mechanical,” ... But the onward march of science seems to force us ever more clearly into accepting intermediate levels of such properties.
‘Shades of Gray Along the Consciousness Continuum’, Fluid Concepts & Creative Analogies: Computer Models of the Fundamental Mechanisms of Thought (1995), 310.
Philosophers no longer write for the intelligent, only for their fellow professionals. The few thousand academic philosophers in the world do not stint themselves: they maintain more than seventy learned journals. But in the handful that cover more than one subdivision of philosophy, any given philosopher can hardly follow more than one or two articles in each issue. This hermetic condition is attributed to “technical problems” in the subject. Since William James, Russell, and Whitehead, philosophy, like history, has been confiscated by scholarship and locked away from the contamination of general use.
In The Culture We Deserve (1989), 9.
Philosophy … consists chiefly in suggesting unintelligible answers to insoluble problems..
In The Education of Henry Adams: An Autobiography (1918), 377.
Philosophy is that part of science which at present people chose to have opinions about, but which they have no knowledge about. Therefore every advance in knowledge robs philosophy of some problems which formerly it had …and will belong to science.
'The Philosophy of Logical Atomism' (1918). In Betrand Russell and Robert Charles Marsh (Ed.), Logic and Knowledge: Essays, 1901-1950 (1988), 281.
Physics is becoming so unbelievably complex that it is taking longer and longer to train a physicist. It is taking so long, in fact, to train a physicist to the place where he understands the nature of physical problems that he is already too old to solve them.
As quoted by Colin Pittendrigh (1971). In George C. Beakley, Ernest G. Chilton, Introduction to Engineering Design and Graphics (1973), 40
Plants have all the same kinds of problems of survival that animals have. … They must find food, a place to live, a mate and they must get on with their neighbors and fight to survive.
In Nadine Brozan, 'Chronicle: Bringing the Secret Life of Plants to Light', New York Times (9 Oct 1995), Sec. A, 13. Attenborough was on a lecture tour about The Private Life of Plants, the title of his book and a six-part BBC TV series.
Possible ideas and thoughts are vast in number. A distinct word for every distinct idea and thought would require a vast vocabulary. The problem in language is to express many ideas and thoughts with comparatively few words.
In Introduction to the Study of Indian Languages: With Words, Phrases and Sentences to be Collected (1880), 55.
Probably the most important skill that children learn is how to learn. … Too often we give children answers to remember rather than problems to solve. This is a mistake.
In 'Observing the Brain Through a Cat's Eyes', Saturday Review World (1974), 2, 132.
Problems are the price of progress. Don’t bring me anything but trouble. Good news weakens me.
Problems in human engineering will receive during the coming years the same genius and attention which the nineteenth century gave to the more material forms of engineering.
We have laid good foundations for industrial prosperity, now we want to assure the happiness and growth of the workers through vocational education, vocational guidance, and wisely managed employment departments. A great field for industrial experimentation and statemanship is opening up.
We have laid good foundations for industrial prosperity, now we want to assure the happiness and growth of the workers through vocational education, vocational guidance, and wisely managed employment departments. A great field for industrial experimentation and statemanship is opening up.
Letter printed in Engineering Magazine (Jan 1917), cover. Quoted in an article by Meyer Bloomfield, 'Relation of Foremen to the Working Force', reproduced in Daniel Bloomfield, Selected Articles on Employment Management (1919), 301.
Protein synthesis is a central problem for the whole of biology, and that it is in all probability closely related to gene action.
'On Protein Synthesis', Symposia of the Society for Experimental Biology: The Biological Replication of Macromolecules, 1958, 12, 160.
Questions that pertain to the foundations of mathematics, although treated by many in recent times, still lack a satisfactory solution. Ambiguity of language is philosophy's main source of problems. That is why it is of the utmost importance to examine attentively the very words we use.
Arithmetices Principia, (1889)
Quite distinct from the theoretical question of the manner in which mathematics will rescue itself from the perils to which it is exposed by its own prolific nature is the practical problem of finding means of rendering available for the student the results which have been already accumulated, and making it possible for the learner to obtain some idea of the present state of the various departments of mathematics. … The great mass of mathematical literature will be always contained in Journals and Transactions, but there is no reason why it should not be rendered far more useful and accessible than at present by means of treatises or higher text-books. The whole science suffers from want of avenues of approach, and many beautiful branches of mathematics are regarded as difficult and technical merely because they are not easily accessible. … I feel very strongly that any introduction to a new subject written by a competent person confers a real benefit on the whole science. The number of excellent text-books of an elementary kind that are published in this country makes it all the more to be regretted that we have so few that are intended for the advanced student. As an example of the higher kind of text-book, the want of which is so badly felt in many subjects, I may mention the second part of Prof. Chrystal’s Algebra published last year, which in a small compass gives a great mass of valuable and fundamental knowledge that has hitherto been beyond the reach of an ordinary student, though in reality lying so close at hand. I may add that in any treatise or higher text-book it is always desirable that references to the original memoirs should be given, and, if possible, short historic notices also. I am sure that no subject loses more than mathematics by any attempt to dissociate it from its history.
In Presidential Address British Association for the Advancement of Science, Section A (1890), Nature, 42, 466.
Reflexion is careful and laborious thought, and watchful attention directed to the agreeable effect of one’s plan. Invention, on the other hand, is the solving of intricate problems and the discovery of new principles by means of brilliancy and versatility.
In De Architectura, Book 1, Chap 2, Sec. 2. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 14.
Research has deserted the individual and entered the group. The individual worker find the problem too large, not too difficult. He must learn to work with others.
Letter to Dr. E. B. Krumhaar (11 Oct 1933), in Journal of Bacteriology (Jan 1934), 27, No. 1, 20.
Research may start from definite problems whose importance it recognizes and whose solution is sought more or less directly by all forces. But equally legitimate is the other method of research which only selects the field of its activity and, contrary to the first method, freely reconnoitres in the search for problems which are capable of solution. Different individuals will hold different views as to the relative value of these two methods. If the first method leads to greater penetration it is also easily exposed to the danger of unproductivity. To the second method we owe the acquisition of large and new fields, in which the details of many things remain to be determined and explored by the first method.
In Zum Gedächtniss an Julius Plucker', Göttinger Abhandlungen (1871), 16, Mathematische Classe, 6.
Richard Feynman was fond of giving the following advice on how to be a genius. You have to keep a dozen of your favorite problems constantly present in your mind, although by and large they will lay in a dormant state. Every time you hear or read a new trick or a new result, test it against each of your twelve problems to see whether it helps. Every once in a while there will be a hit, and people will say, “How did he do it? He must be a genius!”
In 'Ten Lessons I Wish I Had Been Taught', Indiscrete Thoughts (2008), 202.
Rutherford was as straightforward and unpretentious as a physicist as he was elsewhere in life, and that no doubt was one of the secrets of his success. “I was always a believer in simplicity, being a simple man myself,” he said. If a principle of physics could not be explained to a barmaid, he insisted, the problem was with the principle, not the barmaid.
In Great Physicists (2001), 328.
Science by itself produces a very badly deformed man who becomes rounded out into a useful creative being only with great difficulty and large expenditure of time. … It is a much smaller matter to both teach and learn pure science than it is to intelligently apply this science to the solution of problems as they arise in daily life.
As quoted in Gary W. Matkin, Technology Transfer and the University (1990), 24.
Science fiction writers foresee the inevitable, and although problems and catastrophes may be inevitable, solutions are not.
'How Easy to See the Future'. In Asimov on Science Fiction (1981), 86.
Science has not solved problems, only shifted the points of problems.
…...
Science has taught us how to put the atom to work. But to make it work for good instead of for evil lies in the domain dealing with the principles of human duty. We are now facing a problem more of ethics than physics.
Speech to the United Nations Atomic Energy Commission (14 Jun 1946). In Alfred J. Kolatch, Great Jewish Quotations (1996), 39.
Science is a dynamic undertaking directed to lowering the degree of the empiricism involved in solving problems; or, if you prefer, science is a process of fabricating a web of interconnected concepts and conceptual schemes arising from experiments and ob
Modern Science and Modern Man, p. 62, New York (1952).
Science is a game—but a game with reality, a game with sharpened knives … If a man cuts a picture carefully into 1000 pieces, you solve the puzzle when you reassemble the pieces into a picture; in the success or failure, both your intelligences compete. In the presentation of a scientific problem, the other player is the good Lord. He has not only set the problem but also has devised the rules of the game—but they are not completely known, half of them are left for you to discover or to deduce. The experiment is the tempered blade which you wield with success against the spirits of darkness—or which defeats you shamefully. The uncertainty is how many of the rules God himself has permanently ordained, and how many apparently are caused by your own mental inertia, while the solution generally becomes possible only through freedom from its limitations.
Quoted in Walter Moore, Schrödinger: Life and Thought (1989), 348.
Science is always wrong; … Science can never solve one problem without creating ten more problems.
Speech at the Einstein Dinner, Savoy Hotel, London (28 Oct 1930). Reproduced in George Bernard Shaw and Warren Sylvester Smith (ed.), The Religious Speeches of George Bernard Shaw (1963), 83. This is part of a longer quote, comparing science and religion, which begins, “We call the one side…,” which can be found elsewhere on the page of George Bernard Shaw Quotations on this website.
Science is bound by the everlasting law of honour, to face fearlessly every problem which can fairly be presented to it. If a probable solution, consistent with the ordinary course of nature, can be found, we must not invoke an abnormal act of Creative Power.
From Inaugural Address of the President (2 Aug 1871) to the Edinburgh meeting of the British Association. Published in Chemical News and Journal of Industrial Science (4 Aug 1871), 24, 55.
Science is measurement. If I cannot make measurements, I cannot study a problem scientifically.
In 'Musical Acoustics Today', New Scientist (1 Nov 1962), 16 No. 311, 257.
Science is something we depend on all the time. If I develop a pain in the chest I must take an X-ray. But what if the radiation from the X-ray causes me deeper problems? Before I know it. I’m going in for surgery. Naturally, while they’re giving me oxygen an intern decides to light up a cigarette. The next thing you know I’m rocketing over the World Trade Center in bed clothes. Is this science?
In 'My Speech to the Graduates', Side Effects (1986), 82.
Science is the search for truth. It is not a game in which one tries to beat his opponent, to do harm to others. We need to have the spirit of science in international affairs, to make the conduct of international affairs the effort to find the right solution, the just solution of international problems, not the effort by each nation to get the better of other nations, to do harm to them when it is possible.
In No More War! (1958).
Science itself, therefore, may be regarded as a minimal problem, consisting of the completest possible presentment of facts with the least possible expenditure of thought.
Ernst Mach and Thomas Joseph McCormick (trans.), The Science of Mechanics: a Critical and Historical Account of its Development (1919), 490.
Science tells us how very far we are from attaining our industrial aims with anything approaching the theoretical expenditure of force. Science also tells us in what directions we may look forward to arriving at improvements. I might say that we are on the eve of creating a science of invention, that is, of developing scientific methods for solving industrial problems.
From paper at inaugural meeting of the Society of the Chemical Industry, London (1881), 'Patent Laws as Applied to Chemical Invention', quoted in obituary by Charles Baskerville, 'Ludwig Mond', The Engineering and Mining Journal (1 Jan 1910), 89, No. 1, 3.
Science, in the very act of solving problems, creates more of them.
In Universities: American, English, German (1930), 19.
Scientists are the easiest to fool. ... They think in straight, predictable, directable, and therefore misdirectable, lines. The only world they know is the one where everything has a logical explanation and things are what they appear to be. Children and conjurors—they terrify me. Scientists are no problem; against them I feel quite confident.
Code of the Lifemaker (1983, 2000),Chapter 1.
Scientists come in two varieties, hedgehogs and foxes. I borrow this terminology from Isaiah Berlin (1953), who borrowed it from the ancient Greek poet Archilochus. Archilochus told us that foxes know many tricks, hedgehogs only one. Foxes are broad, hedgehogs are deep. Foxes are interested in everything and move easily from one problem to another. Hedgehogs are only interested in a few problems that they consider fundamental, and stick with the same problems for years or decades. Most of the great discoveries are made by hedgehogs, most of the little discoveries by foxes. Science needs both hedgehogs and foxes for its healthy growth, hedgehogs to dig deep into the nature of things, foxes to explore the complicated details of our marvelous universe. Albert Einstein and Edwin Hubble were hedgehogs. Charley Townes, who invented the laser, and Enrico Fermi, who built the first nuclear reactor in Chicago, were foxes.
In 'The Future of Biotechnology', A Many-Colored Glass: Reflections on the Place of Life in the Universe (2007), 1.
Scientists like ripping problems apart, collecting as much data as possible and then assembling the parts back together to make a decision. [Reflecting on being president of Princeton University.]
As quoted by Diane Cole in 'Shirley Tilghman, Educator: From Lab Table to President's Chair', U.S. News & World Reports (12 Nov 2007)
Scientists often have a naive faith that if only they could discover enough facts about a problem, these facts would somehow arrange themselves in a compelling and true solution.
In Mankind Evolving: The Evolution of the Human Species, 128. As cited in Ted Woods & Alan Grant, Reason in Revolt - Dialectical Philosophy and Modern Science (2003), Vol. 2, 183.
Search the scriptures of human achievement and you cannot find any to equal in beneficence the introduction of Anæsthesia, Sanitation, with ail that it includes, and Asepsis—a short half century’s contribution towards the practical solution of the problems of human suffering, regarded as eternal and insoluble.
Address to the Canadian Medical association, Montreal (1902). Collected in 'Chavinism in Medicine', Aequanimitas (1904), 283.
Sexuality is the key to the problem of the psychoneuroses and of the neuroses in general. No one who disdains the key will ever be able to unlock the door.
Fragment of an Analysis of a Case of Hysteria (1905), In James Strachey (ed.), The Standard Edition of the Complete Psychological Works of Sigmund Freud (1953), Vol. 7, 115.
Shoe leather epidemiology.
[Langmuir stressed that investigators go into the field to collect their own data and directly view the locale of a public health problem. His graduates wore lapel pins of a shoe with a hole in the sole.]
[Langmuir stressed that investigators go into the field to collect their own data and directly view the locale of a public health problem. His graduates wore lapel pins of a shoe with a hole in the sole.]
As stated in 'Alexander Langmuir Dies at 83', New York Times (24 Nov 1993), D19.
Since 1957, influenza epidemics have continued to be a major, serious, and intractable health problem, as frustrating to an action and control orientated epidemiologist as poliomyelitis has been gratifying.
(1971). As quoted in Nancy J. Cox, 'Prevention and Control of Influenza', The Lancet 2000 (Dec 1999), 354.
Since my first discussions of ecological problems with Professor John Day around 1950 and since reading Konrad Lorenz's “King Solomon's Ring,” I have become increasingly interested in the study of animals for what they might teach us about man, and the study of man as an animal. I have become increasingly disenchanted with what the thinkers of the so-called Age of Enlightenment tell us about the nature of man, and with what the formal religions and doctrinaire political theorists tell us about the same subject.
'Autobiography of Allan M. Cormack,' Les Prix Nobel/Nobel Lectures 1979, editted by Wilhelm Odelberg.
Since the seventeenth century, physical intuition has served as a vital source for mathematical porblems and methods. Recent trends and fashions have, however, weakened the connection between mathematics and physics; mathematicians, turning away from their roots of mathematics in intuition, have concentrated on refinement and emphasized the postulated side of mathematics, and at other times have overlooked the unity of their science with physics and other fields. In many cases, physicists have ceased to appreciate the attitudes of mathematicians. This rift is unquestionably a serious threat to science as a whole; the broad stream of scientific development may split into smaller and smaller rivulets and dry out. It seems therefore important to direct our efforts towards reuniting divergent trends by classifying the common features and interconnections of many distinct and diverse scientific facts.
As co-author with David Hilbert, in Methods of Mathematical Physics (1937, 1989), Preface, v.
Since you are now studying geometry and trigonometry, I will give you a problem. A ship sails the ocean. It left Boston with a cargo of wool. It grosses 200 tons. It is bound for Le Havre. The mainmast is broken, the cabin boy is on deck, there are 12 passengers aboard, the wind is blowing East-North-East, the clock points to a quarter past three in the afternoon. It is the month of May. How old is the captain?
Letter (14 Aug 1853) to Louise Colet. As quote and cited in Robert A. Nowlan, Masters of Mathematics: The Problems They Solved, Why These Are Important, and What You Should Know about Them (2017), 271.
Sociobiology is not just any statement that biology, genetics, and evolutionary theory have something to do with human behavior. Sociobiology is a specific theory about the nature of genetic and evolutionary input into human behavior. It rests upon the view that natural selection is a virtually omnipotent architect, constructing organisms part by part as best solutions to problems of life in local environments. It fragments organisms into “traits,” explains their existence as a set of best solutions, and argues that each trait is a product of natural selection operating “for” the form or behavior in question. Applied to humans, it must view specific behaviors (not just general potentials) as adaptations built by natural selection and rooted in genetic determinants, for natural selection is a theory of genetic change. Thus, we are presented with unproved and unprovable speculations about the adaptive and genetic basis of specific human behaviors: why some (or all) people are aggressive, xenophobic, religious, acquisitive, or homosexual.
In Hen's Teeth and Horses Toes (1983, 2010), 242-243.
Solving big problems is easier than solving little problems.
Quoted as “Mr Page likes to say” in 'Enlightenment Man', Technology Quarterly (4 Dec 2008).
Some mathematics problems look simple, and you try them for a year or so, and then you try them for a hundred years, and it turns out that they're extremely hard to solve. There's no reason why these problems shouldn't be easy, and yet they turn out to be extremely intricate. [Fermat's] Last Theorem is the most beautiful example of this.
From interview for PBS website on the NOVA program, 'The Proof'.
Some of Feynman’s ideas about cosmology have a modern ring. A good example is his attitude toward the origin of matter. The idea of continuous matter creation in the steady state cosmology does not seriously offend him (and he notes … that the big bang cosmology has a problem just as bad, to explain where all the matter came from in the beginning). … He emphasizes that the total energy of the universe could really be zero, and that matter creation is possible because the rest energy of the matter is actually canceled by its gravitational potential energy. “It is exciting to think that it costs nothing to create a new particle, …”
In John Preskill and Kip S. Thorne, 'Foreword to Feynman Lectures on Gravitation' (15 May 1995). Feynman delivered his lectures in 1962–63.
Some problems are just too complicated for rational logical solutions. They admit of insights, not answers.
In 'Profiles: A Scientist’s Advice II' by D. Lang, The New Yorker (26 Jan 1963).
Some see a clear line between genetic enhancement and other ways that people seek improvement in their children and themselves. Genetic manipulation seems somehow worse—more intrusive, more sinister—than other ways of enhancing performance and seeking success. But, morally speaking, the difference is less significant than it seems. Bioengineering gives us reason to question the low-tech, high-pressure child-rearing practices we commonly accept. The hyperparenting familiar in our time represents an anxious excess of mastery and dominion that misses the sense of life as a gift. This draws it disturbingly close to eugenics... Was the old eugenics objectionable only insofar as it was coercive? Or is there something inherently wrong with the resolve to deliberately design our progeny’s traits... But removing coercion does not vindicate eugenics. The problem with eugenics and genetic engineering is that they represent a one-sided triumph of willfulness over giftedness, of dominion over reverence, of molding over beholding.
Michael J. Sandel, 'The Case Against Perfection', The Atlantic Monthly (Apr 2004).
Sometime between 1740 and 1780, electricians were for the first time enabled to take the foundations for their field for granted. From that point they pushed on to more concrete and recondite problems.
From The Structure of Scientific Revolutions (1970, 2012), 21-22.
Subtle as the mind is it can effect little without knowledge. It cannot construct a bridge, or a building, or make a canal, or work a problem in algebra, unless it is provided with information.
In Chap. 7, The Story of My Heart: My Autobiography (1883), 176.
Success in the solution of a problem generally depends in a great measure on the selection of the most appropriate method of approaching it; many properties of conic sections (for instance) being demonstrable by a few steps of pure geometry which would involve the most laborious operations with trilinear co-ordinates, while other properties are almost self-evident under the method of trilinear co-ordinates, which it would perhaps be actually impossible to prove by the old geometry.
In Trilinear Coordinates and Other Methods of Modern Analytical Geometry of Two Dimensions (1866), 154.
Suppose [an] imaginary physicist, the student of Niels Bohr, is shown an experiment in which a virus particle enters a bacterial cell and 20 minutes later the bacterial cell is lysed and 100 virus particles are liberated. He will say: “How come, one particle has become 100 particles of the same kind in 20 minutes? That is very interesting. Let us find out how it happens! How does the particle get in to the bacterium? How does it multiply? Does it multiply like a bacterium, growing and dividing, or does it multiply by an entirely different mechanism ? Does it have to be inside the bacterium to do this multiplying, or can we squash the bacterium and have the multiplication go on as before? Is this multiplying a trick of organic chemistry which the organic chemists have not yet discovered ? Let us find out. This is so simple a phenomenon that the answers cannot be hard to find. In a few months we will know. All we have to do is to study how conditions will influence the multiplication. We will do a few experiments at different temperatures, in different media, with different viruses, and we will know. Perhaps we may have to break into the bacteria at intermediate stages between infection and lysis. Anyhow, the experiments only take a few hours each, so the whole problem can not take long to solve.”
[Eight years later] he has not got anywhere in solving the problem he set out to solve. But [he may say to you] “Well, I made a slight mistake. I could not do it in a few months. Perhaps it will take a few decades, and perhaps it will take the help of a few dozen other people. But listen to what I have found, perhaps you will be interested to join me.”
[Eight years later] he has not got anywhere in solving the problem he set out to solve. But [he may say to you] “Well, I made a slight mistake. I could not do it in a few months. Perhaps it will take a few decades, and perhaps it will take the help of a few dozen other people. But listen to what I have found, perhaps you will be interested to join me.”
From 'Experiments with Bacterial Viruses (Bacteriophages)', Harvey Lecture (1946), 41, 161-162. As cited in Robert Olby, The Path of the Double Helix: The Discovery of DNA (1974, 1994), 237.
Suppose then I want to give myself a little training in the art of reasoning; suppose I want to get out of the region of conjecture and probability, free myself from the difficult task of weighing evidence, and putting instances together to arrive at general propositions, and simply desire to know how to deal with my general propositions when I get them, and how to deduce right inferences from them; it is clear that I shall obtain this sort of discipline best in those departments of thought in which the first principles are unquestionably true. For in all our thinking, if we come to erroneous conclusions, we come to them either by accepting false premises to start with—in which case our reasoning, however good, will not save us from error; or by reasoning badly, in which case the data we start from may be perfectly sound, and yet our conclusions may be false. But in the mathematical or pure sciences,—geometry, arithmetic, algebra, trigonometry, the calculus of variations or of curves,— we know at least that there is not, and cannot be, error in our first principles, and we may therefore fasten our whole attention upon the processes. As mere exercises in logic, therefore, these sciences, based as they all are on primary truths relating to space and number, have always been supposed to furnish the most exact discipline. When Plato wrote over the portal of his school. “Let no one ignorant of geometry enter here,” he did not mean that questions relating to lines and surfaces would be discussed by his disciples. On the contrary, the topics to which he directed their attention were some of the deepest problems,— social, political, moral,—on which the mind could exercise itself. Plato and his followers tried to think out together conclusions respecting the being, the duty, and the destiny of man, and the relation in which he stood to the gods and to the unseen world. What had geometry to do with these things? Simply this: That a man whose mind has not undergone a rigorous training in systematic thinking, and in the art of drawing legitimate inferences from premises, was unfitted to enter on the discussion of these high topics; and that the sort of logical discipline which he needed was most likely to be obtained from geometry—the only mathematical science which in Plato’s time had been formulated and reduced to a system. And we in this country [England] have long acted on the same principle. Our future lawyers, clergy, and statesmen are expected at the University to learn a good deal about curves, and angles, and numbers and proportions; not because these subjects have the smallest relation to the needs of their lives, but because in the very act of learning them they are likely to acquire that habit of steadfast and accurate thinking, which is indispensable to success in all the pursuits of life.
In Lectures on Teaching (1906), 891-92.
Suppose you had a small electrical fire and... a structural engineer [looked] at your home’s wiring [and] reports that the wiring is “shot” and there is a 50% chance that your house would burn down in the next few years unless you replace all the wiring. The job will cost $20,000... so you get an independent assessment. The next engineer agrees with the first warning. You can either continue to shop for additional evaluations until you find the one engineer in 1,000 that is willing to give you the answer you want, “Your family is not in danger” or you can change the wiring.
[Comparing the urgency of action on climate change to a problem with electrical wiring in a house.]
[Comparing the urgency of action on climate change to a problem with electrical wiring in a house.]
From press conference at National Press Club (17 Sep 2008), 'Basic Research: Fueling America's Future'. Quoted on the Science Coalition website.
Surely there’s no problem with having several conflicting theories of evolution? Eventually the fittest will survive.
Comment by Pete Bibby following an article, 'Scientists Are Still Fleshing Out Darwin’s Theory of Evolution', The Guardian (1 Jul 2022).
Teach to the the problems, not to the text.
As quoted, without citation, in Howard W. Eves Return to Mathematical Circles, (1988), 159. [Note the E. Kim Nebeuts is probably a pen name since reversed it reads Mike Stueben —Webmaster]
Technology can relieve the symptoms of a problem without affecting the underlying causes. Faith in technology as the ultimate solution to all problems can thus divert our attention from the most fundamental problem—the problem of growth in a finite system
et al., The Limits to Growth (1972).
That man can interrogate as well as observe nature was a lesson slowly learned in his evolution. Of the two methods by which he can do this, the mathematical and the experimental, both have been equally fruitful—by the one he has gauged the starry heights and harnessed the cosmic forces to his will; by the other he has solved many of the problems of life and lightened many of the burdens of humanity.
In 'The Evolution of the Idea of Experiment in Medicine', in C.G. Roland, Sir William Osler, 1849-1919: A Selection for Medical Students (1982), 103. As cited in William Osler and Mark E. Silverman (ed.), The Quotable Osler (2002), 249
That mathematics “do not cultivate the power of generalization,”; … will be admitted by no person of competent knowledge, except in a very qualified sense. The generalizations of mathematics, are, no doubt, a different thing from the generalizations of physical science; but in the difficulty of seizing them, and the mental tension they require, they are no contemptible preparation for the most arduous efforts of the scientific mind. Even the fundamental notions of the higher mathematics, from those of the differential calculus upwards are products of a very high abstraction. … To perceive the mathematical laws common to the results of many mathematical operations, even in so simple a case as that of the binomial theorem, involves a vigorous exercise of the same faculty which gave us Kepler’s laws, and rose through those laws to the theory of universal gravitation. Every process of what has been called Universal Geometry—the great creation of Descartes and his successors, in which a single train of reasoning solves whole classes of problems at once, and others common to large groups of them—is a practical lesson in the management of wide generalizations, and abstraction of the points of agreement from those of difference among objects of great and confusing diversity, to which the purely inductive sciences cannot furnish many superior. Even so elementary an operation as that of abstracting from the particular configuration of the triangles or other figures, and the relative situation of the particular lines or points, in the diagram which aids the apprehension of a common geometrical demonstration, is a very useful, and far from being always an easy, exercise of the faculty of generalization so strangely imagined to have no place or part in the processes of mathematics.
In An Examination of Sir William Hamilton’s Philosophy (1878), 612-13.
That’s the whole problem with science. You’ve got a bunch of empiricists trying to describe things of unimaginable wonder.
Dialog by Calvin (fictional character) in syndicated newspaper comic strip Calvin and Hobbes (21 Jun 1992).
The ‘mad idea’ which will lie at the basis of a future fundamental physical theory will come from a realization that physical meaning has some mathematical form not previously associated with reality. From this point of view the problem of the ‘mad idea’ is the problem of choosing, not of generating, the right idea. One should not understand that too literally. In the 1960s it was said (in a certain connection) that the most important discovery of recent years in physics was the complex numbers. The author [Yuri Manin] has something like that in mind.
Mathematics and Physics (1981), Foreward. Reprinted in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 90.
The advancement of science is slow; it is effected only by virtue of hard work and perseverance. And when a result is attained, should we not in recognition connect it with the efforts of those who have preceded us, who have struggled and suffered in advance? Is it not truly a duty to recall the difficulties which they vanquished, the thoughts which guided them; and how men of different nations, ideas, positions, and characters, moved solely by the love of science, have bequeathed to us the unsolved problem? Should not the last comer recall the researches of his predecessors while adding in his turn his contribution of intelligence and of labor? Here is an intellectual collaboration consecrated entirely to the search for truth, and which continues from century to century.
[Respecting how the work of prior researchers had enabled his isolation of fluorine.]
[Respecting how the work of prior researchers had enabled his isolation of fluorine.]
Proceedings of the Royal Institution (1897). In Annual Report of the Board of Regents of the Smithsonian Institution to July 1897 (1898), 262.
The advances of biology during the past 20 years have been breathtaking, particularly in cracking the mystery of heredity. Nevertheless, the greatest and most difficult problems still lie ahead. The discoveries of the 1970‘s about the chemical roots of memory in nerve cells or the basis of learning, about the complex behavior of man and animals, the nature of growth, development, disease and aging will be at least as fundamental and spectacular as those of the recent past.
As quoted in 'H. Bentley Glass', New York Times (12 Jan 1970), 96.
The advantage is that mathematics is a field in which one’s blunders tend to show very clearly and can be corrected or erased with a stroke of the pencil. It is a field which has often been compared with chess, but differs from the latter in that it is only one’s best moments that count and not one’s worst. A single inattention may lose a chess game, whereas a single successful approach to a problem, among many which have been relegated to the wastebasket, will make a mathematician’s reputation.
In Ex-Prodigy: My Childhood and Youth (1953), 21.
The American Businessman has a problem: if he comes up with something new, the Russians invent it six months later and the Japanese make it cheaper.
In E.C. McKenzie, 14,000 Quips and Quotes for Speakers, Writers, Editors, Preachers, and Teachers (1990), 58.
The answers are always inside the problem, not outside.
(Attributed ??) This quote is often seen, but without a citation, even on the official Marshall McLuhan website. If you known a primary print source, please contact Webmaster.
The architect does not demand things which cannot be found or made ready without great expense. For example: it is not everywhere that there is plenty of pitsand, rubble, fir, clear fir, and marble… Where there is no pitsand, we must use the kinds washed up by rivers or by the sea… and other problems we must solve in similar ways.
In De Architectura, Book 1, Chap 2, Sec. 8. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 16.
The art of research [is] the art of making difficult problems soluble by devising means of getting at them.
Pluto's Republic (1982), 2.
The atom could not be split until it was regarded as a problem.
In Take Today: The Executive as Dropout (1972), 92.
The belief that mathematics, because it is abstract, because it is static and cold and gray, is detached from life, is a mistaken belief. Mathematics, even in its purest and most abstract estate, is not detached from life. It is just the ideal handling of the problems of life, as sculpture may idealize a human figure or as poetry or painting may idealize a figure or a scene. Mathematics is precisely the ideal handling of the problems of life, and the central ideas of the science, the great concepts about which its stately doctrines have been built up, are precisely the chief ideas with which life must always deal and which, as it tumbles and rolls about them through time and space, give it its interests and problems, and its order and rationality. That such is the case a few indications will suffice to show. The mathematical concepts of constant and variable are represented familiarly in life by the notions of fixedness and change. The concept of equation or that of an equational system, imposing restriction upon variability, is matched in life by the concept of natural and spiritual law, giving order to what were else chaotic change and providing partial freedom in lieu of none at all. What is known in mathematics under the name of limit is everywhere present in life in the guise of some ideal, some excellence high-dwelling among the rocks, an “ever flying perfect” as Emerson calls it, unto which we may approximate nearer and nearer, but which we can never quite attain, save in aspiration. The supreme concept of functionality finds its correlate in life in the all-pervasive sense of interdependence and mutual determination among the elements of the world. What is known in mathematics as transformation—that is, lawful transfer of attention, serving to match in orderly fashion the things of one system with those of another—is conceived in life as a process of transmutation by which, in the flux of the world, the content of the present has come out of the past and in its turn, in ceasing to be, gives birth to its successor, as the boy is father to the man and as things, in general, become what they are not. The mathematical concept of invariance and that of infinitude, especially the imposing doctrines that explain their meanings and bear their names—What are they but mathematicizations of that which has ever been the chief of life’s hopes and dreams, of that which has ever been the object of its deepest passion and of its dominant enterprise, I mean the finding of the worth that abides, the finding of permanence in the midst of change, and the discovery of a presence, in what has seemed to be a finite world, of being that is infinite? It is needless further to multiply examples of a correlation that is so abounding and complete as indeed to suggest a doubt whether it be juster to view mathematics as the abstract idealization of life than to regard life as the concrete realization of mathematics.
In 'The Humanization of Teaching of Mathematics', Science, New Series, 35, 645-46.
The bottom line for mathematicians is that the architecture has to be right. In all the mathematics that I did, the essential point was to find the right architecture. It’s like building a bridge. Once the main lines of the structure are right, then the details miraculously fit. The problem is the overall design.
In interview by Donald J. Albers, in 'Freeman Dyson: Mathematician, Physicist, and Writer', The College Mathematics Journal (Jan 1994), 25, No. 1, 20.
The carbon output that melts the ice in the Arctic also causes ocean acidification, which results from the ocean absorbing excess carbon dioxide from the atmosphere (the same carbon dioxide that is the primary cause of global warming, hence the nickname “the other carbon problem”).
In 'What do the Arctic, a Thermostat and COP15 Have in Common?', Huffington Post (18 Mar 2010).
The Catholic Church excommunicated Copernicans, the Communist Party persecuted Mendelians on the ground that their doctrines were pseudoscientific. The demarcation between science and pseudoscience is not merely a problem of armchair philosophy: it is of vital social and political relevance.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 1.
The central problem of biological evolution is the nature of mutation, but hitherto the occurrence of this has been wholly refractory and impossible to influence by artificial means, although a control of it might obviously place the process of evolution in our hands.
'The Recent Findings in Heredity' (unpublished lecture, 1916, Lilly Library), 3. Quoted in Elof Axel Carlson, Genes, Radiation, and Society: The Life and Work of H. J. Muller (1981), 104.
The century of biology upon which we are now well embarked is no matter of trivialities. It is a movement of really heroic dimensions, one of the great episodes in man’s intellectual history. The scientists who are carrying the movement forward talk in terms of nucleo-proteins, of ultracentrifuges, of biochemical genetics, of electrophoresis, of the electron microscope, of molecular morphology, of radioactive isotopes. But do not be misled by these horrendous terms, and above all do not be fooled into thinking this is mere gadgetry. This is the dependable way to seek a solution of the cancer and polio problems, the problems of rheumatism and of the heart. This is the knowledge on which we must base our solution of the population and food problems. This is the understanding of life.
Letter to H. M. H. Carsan (17 Jun 1949). Quoted in Raymond B. Fosdick, The Story of the Rockefeller Foundation (1952), 166.
The certainties of one age are the problems of the next.
Religion and the Rise of Capitalism (1926, 2008), 282.
The chief problem of the commercial farmers is overproduction. The chief problem of the low-income farmers is poverty.
In Public Papers of Nelson A. Rockefeller: Fifty-Third Governor of the State of New York (1959), Vol. 1, 1206.
The complexity of contemporary biology has led to an extreme specialization, which has inevitably been followed by a breakdown in communication between disciplines. Partly as a result of this, the members of each specialty tend to feel that their own work is fundamental and that the work of other groups, although sometimes technically ingenious, is trivial or at best only peripheral to an understanding of truly basic problems and issues. There is a familiar resolution to this problem but it is sometimes difficulty to accept emotionally. This is the idea that there are a number of levels of biological integration and that each level offers problems and insights that are unique to it; further, that each level finds its explanations of mechanism in the levels below, and its significances in the levels above it.
From 'Interaction of physiology and behavior under natural conditions', collected in R.I. Bowman (ed.), The Galapagos (1966), 39.
The conservation of natural resources is the fundamental problem. Unless we solve that problem it will avail us little to solve all others.
'Our National Inland Waterways Policy', Address to the Deep Waterway Convention, Memphis, Tennessee, 4 Oct 1907. In American Waterways (1908), 9.
The definition of a good mathematical problem is the mathematics it generates rather than the problem itself.
From interview for PBS website on the NOVA program, 'The Proof'.
The development of mathematics toward greater precision has led, as is well known, to the formalization of large tracts of it, so that one can prove any theorem using nothing but a few mechanical rules... One might therefore conjecture that these axioms and rules of inference are sufficient to decide any mathematical question that can at all be formally expressed in these systems. It will be shown below that this is not the case, that on the contrary there are in the two systems mentioned relatively simple problems in the theory of integers that cannot be decided on the basis of the axioms.
'On Formally Undecidable Propositions of Principia Mathematica and Related Systems I' (193 1), in S. Feferman (ed.), Kurt Gödel Collected Works: Publications 1929-1936 (1986), Vol. I, 145.
The difficulty lies not in solving problems but expressing them.
From 'The Evolution of Chastity' (Feb 1934), as translated by René Hague in Toward the Future (1975), 86.
The distributed architecture and its technique of packet switching were built around the problem of getting messages delivered despite blockages, holes and malfunctions. Imagine the poor censor faced with such a system. There is no central exchange to seize and hold; messages actively “seek out” alternative routes so that even if one path is blocked another may open up. Here is the civil libertarian’s dream.
As quoted in Richard Rogers, 'The Internet Treats Censorship as a Malfunction and Routes Around It? : A New Media Approach to the Study of State Internet Censorship', collected in Jussi Parikka and Tony D. Sampson (eds.), The Spam Book: On Viruses, Porn, and Other Anomalies from the Dark Side of Digital Culture (2009), 243.
The diversity of life is extraordinary. There is said to be a million or so different kinds of living animals, and hundreds of thousands of kinds of plants. But we don’t need to think of the world at large. It is amazing enough to stop and look at a forest or at a meadow—at the grass and trees and caterpillars and hawks and deer. How did all these different kinds of things come about; what forces governed their evolution; what forces maintain their numbers and determine their survival or extinction; what are their relations to each other and to the physical environment in which they live? These are the problems of natural history.
In The Nature of Natural History (1950), 8.
The dropping of the Atomic Bomb is a very deep problem… Instead of commemorating Hiroshima we should celebrate… man’s triumph over the problem [of transmutation], and not its first misuse by politicians and military authorities.
Address to New Europe Group meeting on the third anniversary of the Hiroshima bomb. Quoted in New Europe Group, In Commemoration of Professor Frederick Soddy (1956), 6-7.
The economic and technological triumphs of the past few years have not solved as many problems as we thought they would, and, in fact, have brought us new problems we did not foresee.
In 'Henry Ford on What’s Wrong With the U.S.', U.S. News & World Report (1966), 60, 24.
The empirical domain of objective contemplation, and the delineation of our planet in its present condition, do not include a consideration of the mysterious and insoluble problems of origin and
existence.
In lecture, 'Organic Life', collected in Cosmos, the Elements of the Physical World (1849), 348, as translated by E.C. Otté. Also seen translated as “The mysterious and unsolved problem of how things came to be does not enter the empirical province of objective research, which is confined to a description of things as they are.”
The employment of mathematical symbols is perfectly natural when the relations between magnitudes are under discussion; and even if they are not rigorously necessary, it would hardly be reasonable to reject them, because they are not equally familiar to all readers and because they have sometimes been wrongly used, if they are able to facilitate the exposition of problems, to render it more concise, to open the way to more extended developments, and to avoid the digressions of vague argumentation.
From Recherches sur les Principes Mathématiques de la Théorie des Richesses (1838), as translated by Nathaniel T. Bacon in 'Preface', Researches Into Mathematical Principles of the Theory of Wealth (1897), 3-4. From the original French, “L’emploi des signes mathématiques est chose naturelle toutes les fois qu'il s'agit de discuter des relations entre des grandeurs ; et lors même qu’ils ne seraient pas rigoureusement nécessaires, s’ils peuvent faciliter l’exposition, la rendre plus concise, mettre sur la voie de développements plus étendus, prévenir les écarts d’une vague argumentation, il serait peu philosophique de les rebuter, parce qu'ils ne sont pas également familiers à tous les lecteurs et qu'on s'en est quelquefois servi à faux.”
The enchanting charms of this sublime science reveal only to those who have the courage to go deeply into it. But when a woman, who because of her sex and our prejudices encounters infinitely more obstacles that an man in familiarizing herself with complicated problems, succeeds nevertheless in surmounting these obstacles and penetrating the most obscure parts of them, without doubt she must have the noblest courage, quite extraordinary talents and superior genius.
in a letter to Sophie Germain (c.April 1807)
The ends to be attained [in Teaching of Mathematics in the secondary schools] are the knowledge of a body of geometrical truths, the power to draw correct inferences from given premises, the power to use algebraic processes as a means of finding results in practical problems, and the awakening of interest in the science of mathematics.
In 'Aim of the Mathematical Instruction', International Commission on Teaching of Mathematics, American Report: United States Bureau of Education: Bulletin 1912, No. 4, 7.
The equation of animal and vegetable life is too complicated a problem for human intelligence to solve, and we can never know how wide a circle of disturbance we produce in the harmonies of nature when we throw the smallest pebble into the ocean of organic life.
Man and Nature, (1864), 103.
The essence of engineering consists not so much in the mere construction of the spectacular layouts or developments, but in the invention required—the analysis of the problem, the design, the solution by the mind which directs it all.
As quoted, “he said to the writer in effect,” Robert Fletcher, 'William Hood '67, Chief Engineer of the Southern Pacific Railroad Lines, Dartmouth Alumni Magazine (1919), Vol. 11, 223.
The Excellence of Modern Geometry is in nothing more evident, than in those full and adequate Solutions it gives to Problems; representing all possible Cases in one view, and in one general Theorem many times comprehending whole Sciences; which deduced at length into Propositions, and demonstrated after the manner of the Ancients, might well become the subjects of large Treatises: For whatsoever Theorem solves the most complicated Problem of the kind, does with a due Reduction reach all the subordinate Cases.
In 'An Instance of the Excellence of Modern Algebra, etc', Philosophical Transactions, 1694, 960.
The fact remains that, if the supply of energy failed, modern civilization would come to an end as abruptly as does the music of an organ deprived of wind.
Matter and Energy (1911), 251.
The fact that death from cancer is on the increase is not only a problem of medicine, but its at the same time testifies to the wonderful efficiency of medical science... [as it] enables more persons top live long enough to develop some kind of cancer in old and less resistant tissues.
Charles H. Mayo and William A. Hendricks, 'Carcinoma of the Right Segment of the Colon', presented to Southern Surgical Assoc. (15 Dec 1925). In Annals of Surgery (Mar 1926), 83, 357.
The fact that human life can be prolonged with fewer physical problems requires that we give increasing attention to improving the quality of life. As the poet Edwin Markham stated: “We are all fools until we know that in the common plan, nothing is worth the building if it does not build the man; why build these temples glorious, if man unbuilded goes?”
In 'Millenial Musings', Chemical & Engineering News (6 Dec 1999), 77, No. 49, 48.
The first nonabsolute number is the number of people for whom the table is reserved. This will vary during the course of the first three telephone calls to the restaurant, and then bear no apparent relation to the number of people who actually turn up, or to the number of people who subsequently join them after the show/match/party/gig, or to the number of people who leave when they see who else has turned up.
The second nonabsolute number is the given time of arrival, which is now known to be one of the most bizarre of mathematical concepts, a recipriversexcluson, a number whose existence can only be defined as being anything other than itself. In other words, the given time of arrival is the one moment of time at which it is impossible that any member of the party will arrive. Recipriversexclusons now play a vital part in many branches of math, including statistics and accountancy and also form the basic equations used to engineer the Somebody Else’s Problem field.
The third and most mysterious piece of nonabsoluteness of all lies in the relationship between the number of items on the check [bill], the cost of each item, the number of people at the table and what they are each prepared to pay for. (The number of people who have actually brought any money is only a subphenomenon of this field.)
The second nonabsolute number is the given time of arrival, which is now known to be one of the most bizarre of mathematical concepts, a recipriversexcluson, a number whose existence can only be defined as being anything other than itself. In other words, the given time of arrival is the one moment of time at which it is impossible that any member of the party will arrive. Recipriversexclusons now play a vital part in many branches of math, including statistics and accountancy and also form the basic equations used to engineer the Somebody Else’s Problem field.
The third and most mysterious piece of nonabsoluteness of all lies in the relationship between the number of items on the check [bill], the cost of each item, the number of people at the table and what they are each prepared to pay for. (The number of people who have actually brought any money is only a subphenomenon of this field.)
Life, the Universe and Everything (1982, 1995), 47-48.
The first step in finding the solution to a problem often involves discovering a problem with the existing solution.
The flight of most members of a profession to the high empyrean, where they can work peacefully on purely scientific problems, isolated from the turmoil of real life, was perhaps quite appropriate at an earlier stage of science; but in today's world it is a luxury we cannot afford.
The Scientific Imagination: Case Studies (1978), 250.
The formulation of a problem is often more essential than its solution, which may be merely a matter of mathematical or experimental skill. To raise new questions, new possibilities, to regard old problems from a new angle requires creative imagination and marks real advances in science.
In Albert Einstein and Léopold Infeld, The Evolution of Physics: The Growth of Ideas from Early Concepts to Relativity and Quanta (1938, 1966), 92.
The fundamental problem in the origin of species is not the origin of differences in appearance, since these arise at the level of the geographical race, but the origin of genetic segregation. The test of species-formation is whether, when two forms meet, they interbreed and merge, or whether they keep distinct.
Darwin's Finches (1947), 129.
The future does not belong to those who are content with today, apathetic toward common problems and their fellow man alike, timid and fearful in the face of bold projects and new ideas. Rather, it will belong to those who can blend passion, reason and courage in a personal commitment to the great enterprises and ideals of American society.
…...
The future mathematician ... should solve problems, choose the problems which are in his line, meditate upon their solution, and invent new problems. By this means, and by all other means, he should endeavor to make his first important discovery: he should discover his likes and dislikes, his taste, his own line.
How to Solve it: A New Aspect of Mathematical Method (1957), 206.
The genesis of mathematical creation is a problem which should intensely interest the psychologist.
In 'Mathematical Creation', The Value of Science, collected in Henri Poincaré and George bruce Halsted (trans.), The Foundations of Science (1913), 383.
The genesis of mathematical invention is a problem that must inspire the psychologist with the keenest interest. For this is the process in which the human mind seems to borrow least from the exterior world, in which it acts, or appears to act, only by itself and on itself, so that by studying the process of geometric thought, we may hope to arrive at what is most essential in the human mind
As translated in Arthur I. Miller, Imagery in Scientific Thought Creating 20th-Century Physics (1984, 2013), 307. Opening of Paper delivered at Conference at the Institut Général Psychologique, Paris, 'L’Invention Mathématique', published in Enseignment Mathématique (1908), 10, 357. From the original French, “La genèse do l’Invention mathématique est un problème qui doit inspirer le plus vif intérêt au psychologue. C’est l’acte dans lequel l’esprit humain semble le moins emprunter au monde extérieur, où il n’agit ou ne paraît agir que par lui-même et sur lui-même, de sorte, qu’en étudiant le processus de la pensée géométrique, c’est ce qu’il y a de plus essentiel dans l’esprit humain que nous pouvons espérer atteindre.”
The geometrical problems and theorems of the Greeks always refer to definite, oftentimes to rather complicated figures. Now frequently the points and lines of such a figure may assume very many different relative positions; each of these possible cases is then considered separately. On the contrary, present day mathematicians generate their figures one from another, and are accustomed to consider them subject to variation; in this manner they unite the various cases and combine them as much as possible by employing negative and imaginary magnitudes. For example, the problems which Apollonius treats in his two books De sectione rationis, are solved today by means of a single, universally applicable construction; Apollonius, on the contrary, separates it into more than eighty different cases varying only in position. Thus, as Hermann Hankel has fittingly remarked, the ancient geometry sacrifices to a seeming simplicity the true simplicity which consists in the unity of principles; it attained a trivial sensual presentability at the cost of the recognition of the relations of geometric forms in all their changes and in all the variations of their sensually presentable positions.
In 'Die Synthetische Geometrie im Altertum und in der Neuzeit', Jahresbericht der Deutschen Mathematiker Vereinigung (1902), 2, 346-347. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 112. The spelling of the first “Apollonius” has been corrected from “Appolonius” in the original English text. From the original German, “Die geometrischen Probleme und Sätze der Griechen beziehen sich allemal auf bestimmte, oft recht komplizierte Figuren. Nun können aber die Punkte und Linien einer solchen Figur häufig sehr verschiedene Lagen zu einander annehmen; jeder dieser möglichen Fälle wird alsdann für sich besonders erörtert. Dagegen lassen die heutigen Mathematiker ihre Figuren aus einander entstehen und sind gewohnt, sie als veränderlich zu betrachten; sie vereinigen so die speziellen Fälle und fassen sie möglichst zusammen unter Benutzung auch negativer und imaginärer Gröfsen. Das Problem z. B., welches Apollonius in seinen zwei Büchern de sectione rationis behandelt, löst man heutzutage durch eine einzige, allgemein anwendbare Konstruktion; Apollonius selber dagegen zerlegt es in mehr als 80 nur durch die Lage verschiedene Fälle. So opfert, wie Hermann Hankel treffend bemerkt, die antike Geometrie einer scheinbaren Einfachheit die wahre, in der Einheit der Prinzipien bestehende; sie erreicht eine triviale sinnliche Anschaulichkeit auf Kosten der Erkenntnis vom Zusammenhang geometrischer Gestalten in aller Wechsel und in aller Veränderlichkeit ihrer sinnlich vorstellbaren Lage.”
The Golden Gate Bridge is a giant moving math problem.
Quoted on web site for PBS American Experience episode for 'Golden Gate Bridge.'
The great problem of today is, how to subject all physical phenomena to dynamical laws. With all the experimental devices, and all the mathematical appliances of this generation, the human mind has been baffled in its attempts to construct a universal science of physics.
'President's Address', Proceedings of the American Association for the Advancement of Science (1874), 23, 34-5.
The greatest challenge facing mankind is the challenge of distinguishing reality from fantasy, truth from propaganda. We must daily decide whether the threats we face are real, whether the solutions we are offered will do any good, whether the problems we’re told exist are in fact real problems, or non-problems.
…...
The greatest enemy, however, to true arithmetic work is found in so-called practical or illustrative problems, which are freely given to our pupils, of a degree of difficulty and complexity altogether unsuited to their age and mental development. … I am, myself, no bad mathematician, and all the reasoning powers with which nature endowed me have long been as fully developed as they are ever likely to be; but I have, not infrequently, been puzzled, and at times foiled, by the subtle logical difficulty running through one of these problems, given to my own children. The head-master of one of our Boston high schools confessed to me that he had sometimes been unable to unravel one of these tangled skeins, in trying to help his own daughter through her evening’s work. During this summer, Dr. Fairbairn, the distinguished head of one of the colleges of Oxford, England, told me that not only had he himself encountered a similar difficulty, in the case of his own children, but that, on one occasion, having as his guest one of the first mathematicians of England, the two together had been completely puzzled by one of these arithmetical conundrums.
Address before the Grammar-School Section of the Massachusetts Teachers’ Association (25 Nov 1887), 'The Teaching of Arithmetic in the Boston Schools', printed The Academy (Jan 1888).
Collected in Francis Amasa Walker, Discussions in Education (1899), 253.
The greatest problem of communication is the illusion that it has been achieved.
…...
The history of mathematics may be instructive as well as agreeable; it may not only remind us of what we have, but may also teach us to increase our store. Says De Morgan, “The early history of the mind of men with regards to mathematics leads us to point out our own errors; and in this respect it is well to pay attention to the history of mathematics.” It warns us against hasty conclusions; it points out the importance of a good notation upon the progress of the science; it discourages excessive specialization on the part of the investigator, by showing how apparently distinct branches have been found to possess unexpected connecting links; it saves the student from wasting time and energy upon problems which were, perhaps, solved long since; it discourages him from attacking an unsolved problem by the same method which has led other mathematicians to failure; it teaches that fortifications can be taken by other ways than by direct attack, that when repulsed from a direct assault it is well to reconnoiter and occupy the surrounding ground and to discover the secret paths by which the apparently unconquerable position can be taken.
In History of Mathematics (1897), 1-2.
The human race believes in not taking its problems seriously enough to solve them.
In The Decline and Fall of Science (1976), 170.
The idea that our natural resources were inexhaustible still obtained, and there was as yet no real knowledge of their extent and condition. The relation of the conservation of natural resources to the problems of National welfare and National efficiency had not yet dawned on the public mind. The reclamation of arid public lands in the West was still a matter for private enterprise alone; and our magnificent river system, with its superb possibilities for public usefulness, was dealt with by the National Government not as a unit, but as a disconnected series of pork-barrel problems, whose only real interest was in their effect on the re-election or defeat of a Congressman here and there —a theory which, I regret to say, still obtains.
The Works of Theodore Roosevelt. Vol. 20: Theodore Roosevelt, An Autobiography (1926), 386.
The ideal engineer is a composite ... He is not a scientist, he is not a mathematician, he is not a sociologist or a writer; but he may use the knowledge and techniques of any or all of these disciplines in solving engineering problems.
…...
The ideal engineer is a composite. … He is not a scientist, he is not a mathematician, he is not a sociologist or a writer. But he may use the knowledge and techniques of any or all of these disciplines in solving problems.
Student, Teacher, and Engineer: Selected Speeches and Articles of Nathan W Dougherty (1972), 33.
The imaginary expression √(-a) and the negative expression -b, have this resemblance, that either of them occurring as the solution of a problem indicates some inconsistency or absurdity. As far as real meaning is concerned, both are imaginary, since 0 - a is as inconceivable as √(-a).
The importance of a result is largely relative, is judged differently by different men, and changes with the times and circumstances. It has often happened that great importance has been attached to a problem merely on account of the difficulties which it presented; and indeed if for its solution it has been necessary to invent new methods, noteworthy artifices, etc., the science has gained more perhaps through these than through the final result. In general we may call important all investigations relating to things which in themselves are important; all those which have a large degree of generality, or which unite under a single point of view subjects apparently distinct, simplifying and elucidating them; all those which lead to results that promise to be the source of numerous consequences; etc.
From 'On Some Recent Tendencies in Geometric Investigations', Rivista di Matematica (1891), 44. In Bulletin American Mathematical Society (1904), 444.
The inherent unpredictability of future scientific developments—the fact that no secure inference can be drawn from one state of science to another—has important implications for the issue of the limits of science. It means that present-day science cannot speak for future science: it is in principle impossible to make any secure inferences from the substance of science at one time about its substance at a significantly different time. The prospect of future scientific revolutions can never be precluded. We cannot say with unblinking confidence what sorts of resources and conceptions the science of the future will or will not use. Given that it is effectively impossible to predict the details of what future science will accomplish, it is no less impossible to predict in detail what future science will not accomplish. We can never confidently put this or that range of issues outside “the limits of science”, because we cannot discern the shape and substance of future science with sufficient clarity to be able to say with any assurance what it can and cannot do. Any attempt to set “limits” to science—any advance specification of what science can and cannot do by way of handling problems and solving questions—is destined to come to grief.
The Limits of Science (1984), 102-3.
The intellect has little to do on the road to discovery. There comes a leap in consciousness, call it intuition or what you will, and the solution comes to you and you don’t know why or how.
Quoted in Forbes (15 Sep 1974). In Larry Chang, Wisdom for the Soul (2006), 179.
The intensity and quantity of polemical literature on scientific problems frequently varies inversely as the number of direct observations on which the discussions are based: the number and variety of theories concerning a subject thus often form a coefficient of our ignorance. Beyond the superficial observations, direct and indirect, made by geologists, not extending below about one two-hundredth of the Earth's radius, we have to trust to the deductions of mathematicians for our ideas regarding the interior of the Earth; and they have provided us successively with every permutation and combination possible of the three physical states of matter—solid, liquid, and gaseous.
'Address delivered by the President of Section [Geology] at Sydney (Friday, Aug 21), Report of the Eighty-Fourth Meeting of the British Association for the Advancement of Science: Australia 1914, 1915, 345.
The interactions of man with his environment are so complex that only an ecological approach to nutrition permits an understanding of the whole spectrum of factors determining the nutritional problems that exist in human societies.
World Health Organization, Nutrition in Preventive Medicine, 13. From http://whqlibdoc.who.int/monograph/WHO_MONO_62_(chp1).pdf
The investigation of causal relations between economic phenomena presents many problems of peculiar difficulty, and offers many opportunities for fallacious conclusions. Since the statistician can seldom or never make experiments for himself, he has to accept the data of daily experience, and discuss as best he can the relations of a whole group of changes; he cannot, like the physicist, narrow down the issue to the effect of one variation at a time. The problems of statistics are in this sense far more complex than the problems of physics.
In 'On the Theory of Correlation', Journal of the Royal Statistical Society (Dec 1897), 60, 812, as cited in Stephen M. Stigler, The History of Statistics: The Measurement of Uncertainty Before 1900 (1986), 348.
The large collection of problems which our modern Cambridge books supply will be found to be almost an exclusive peculiarity of these books; such collections scarcely exist in foreign treatises on mathematics, nor even in English treatises of an earlier date. This fact shows, I think, that a knowledge of mathematics may be gained without the perpetual working of examples. … Do not trouble yourselves with the examples, make it your main business, I might almost say your exclusive business, to understand the text of your author.
In 'Private Study of Mathematics', Conflict of Studies and other Essays (1873), 74.
The life and soul of science is its practical application, and just as the great advances in mathematics have been made through the desire of discovering the solution of problems which were of a highly practical kind in mathematical science, so in physical science many of the greatest advances that have been made from the beginning of the world to the present time have been made in the earnest desire to turn the knowledge of the properties of matter to some purpose useful to mankind.
From 'Electrical Units of Measurement', a lecture delivered at the Institution of Civil Engineers, London (3 May 1883), Popular Lectures and Addresses Vol. 1 (1891), 86-87.
The line separating investment and speculation, which is never bright and clear, becomes blurred still further when most market participants have recently enjoyed triumphs. Nothing sedates rationality like large doses of effortless money. After a heady experience of that kind, normally sensible people drift into behavior akin to that of Cinderella at the ball. They know that overstaying the festivities—that is, continuing to speculate in companies that have gigantic valuations relative to the cash they are likely to generate in the future—will eventually bring on pumpkins and mice. But they nevertheless hate to miss a single minute of what is one helluva party. Therefore, the giddy participants all plan to leave just seconds before midnight. There’s a problem, though: They are dancing in a room in which the clocks have no hands.
…...
The logic of the subject [algebra], which, both educationally and scientifically speaking, is the most important part of it, is wholly neglected. The whole training consists in example grinding. What should have been merely the help to attain the end has become the end itself. The result is that algebra, as we teach it, is neither an art nor a science, but an ill-digested farrago of rules, whose object is the solution of examination problems. … The result, so far as problems worked in examinations go, is, after all, very miserable, as the reiterated complaints of examiners show; the effect on the examinee is a well-known enervation of mind, an almost incurable superficiality, which might be called Problematic Paralysis—a disease which unfits a man to follow an argument extending beyond the length of a printed octavo page.
In Presidential Address British Association for the Advancement of Science (1885), Nature, 32, 447-448.
The machine does not isolate man from the great problems of nature but plunges him more deeply into them.
Wind, Sand, and Stars (1939).
The major credit I think Jim and I deserve … is for selecting the right problem and sticking to it. It’s true that by blundering about we stumbled on gold, but the fact remains that we were looking for gold. Both of us had decided, quite independently of each other, that the central problem in molecular biology was the chemical structure of the gene. … We could not see what the answer was, but we considered it so important that we were determined to think about it long and hard, from any relevant point of view.
In What Mad Pursuit (1990), 74-75.
The man in the street will, therefore, twist the statement that the scientist has come to the end of meaning into the statement that the scientist has penetrated as far as he can with the tools at his command, and that there is something beyond the ken of the scientist. This imagined beyond, which the scientist has proved he cannot penetrate, will become the playground of the imagination of every mystic and dreamer. The existence of such a domain will be made the basis of an orgy of rationalizing. It will be made the substance of the soul; the spirits of the dead will populate it; God will lurk in its shadows; the principle of vital processes will have its seat here; and it will be the medium of telepathic communication. One group will find in the failure of the physical law of cause and effect the solution of the age-long problem of the freedom of the will; and on the other hand the atheist will find the justification of his contention that chance rules the universe.
Reflections of a Physicist (1950),102-3.
The mathematician can afford to leave to his clients, the engineers, or perhaps the popular philosophers, the emotion of belief: for himself he keeps the lyrical pleasure of metre and of evolving equations: and it is a pleasant surprise to him and an added problem if he finds that the arts can use his calculations, or that the senses can verify them, much as if a composer found that sailors could heave better when singing his songs.
In 'Revolution in Science', Some Turns of Thought in Modern Philosophy (1933), 81.
The mathematician of to-day admits that he can neither square the circle, duplicate the cube or trisect the angle. May not our mechanicians, in like manner, be ultimately forced to admit that aerial flight is one of that great class of problems with which men can never cope… I do not claim that this is a necessary conclusion from any past experience. But I do think that success must await progress of a different kind from that of invention.
[Written following Samuel Pierpoint Langley's failed attempt to launch his flying machine from a catapult device mounted on a barge in Oct 1903. The Wright Brother's success came on 17 Dec 1903.]
[Written following Samuel Pierpoint Langley's failed attempt to launch his flying machine from a catapult device mounted on a barge in Oct 1903. The Wright Brother's success came on 17 Dec 1903.]
'The Outlook for the Flying Machine'. The Independent: A Weekly Magazine (22 Oct 1903), 2509.
The meaning of time has become terribly problematic in contemporary physics. The situation is so uncomfortable that by far the best thing to do is declare oneself an agnostic.
Quoted by Tim Folger in 'Newsflash: Time May Not Exist', Discover Magazine (Jun 2007).
The mechanist is intimately convinced that a precise knowledge of the chemical constitution, structure, and properties of the various organelles of a cell will solve biological problems. This will come in a few centuries. For the time being, the biologist has to face such concepts as orienting forces or morphogenetic fields. Owing to the scarcity of chemical data and to the complexity of life, and despite the progresses of biochemistry, the biologist is still threatened with vertigo.
Problems of Morphogenesis in Ciliates: The Kinetosomes in Development, Reproduction and Evolution (1950), 92-3.
The message from the Moon which we have flashed to the far corners of this planet is that no problem need any longer be considered insoluble.
…...
The most difficult problem in mathematics is to make the date of a woman's birth agree with her present age.
In Evan Esar, 20,000 Quips and Quotes, 22.
The most direct, and in a sense the most important, problem which our conscious knowledge of Nature should enable us to solve is the anticipation of future events, so that we may arrange our present affairs in accordance with such anticipation. As a basis for the solution of this problem we always make use of our knowledge of events which have already occurred, obtained by chance observation or by prearranged experiment.
In Heinrich Hertz, D.E. Jones (trans.) and J.T. Walley (trans.), 'Introduction', The Principles of Mechanics (1899), 1.
The most important and urgent problems of the technology of today are no longer the satisfactions of the primary needs or of archetypal wishes, but the reparation of the evils and damages by technology of yesterday.
Innovations: Scientific Technological and Social (1970), 9.
The mystery of life is certainly the most persistent problem ever placed before the thought of man. There is no doubt that from the time humanity began to think it has occupied itself with the problem of its origin and its future which undoubtedly is the problem of life. The inability of science to solve it is absolute. This would be truly frightening were it not for faith.
Address (10 Sep 1934) to the International Congress of Electro-Radio Biology, Venice. In Associated Press, 'Life a Closed Book, Declares Marconi', New York Times (11 Sep 1934), 15.
The new mathematics is a sort of supplement to language, affording a means of thought about form and quantity and a means of expression, more exact, compact, and ready than ordinary language. The great body of physical science, a great deal of the essential facts of financial science, and endless social and political problems are only accessible and only thinkable to those who have had a sound training in mathematical analysis, and the time may not be very remote when it will be understood that for complete initiation as an efficient citizen of the great complex world-wide States that are now developing, it is as necessary to be able to compute, to think in averages and maxima and minima, as it is now to be able to read and write.
Mankind in the Making (1903), 204. This is seen in a shorter form, somewhat misquoted in a paraphrase as: “Statistical thinking will one day be as necessary for efficient citizenship as the ability to read and write.” However, note that in fact, Wells refers only to “mathematical analysis” such as “averages and maxima and minima” — and did not specify (more complex) “statistics” at all! For citation of the paraphrase, see Samuel Wilks Quotations on this site.
The next decade will perhaps raise us a step above despair to a cleaner, clearer wisdom and biology cannot fail to help in this. As we become increasingly aware of the ethical problems raised by science and technology, the frontiers between the biological and social sciences are clearly of critical importance—in population density and problems of hunger, psychological stress, pollution of the air and water and exhaustion of irreplaceable resources.
As quoted in 'H. Bentley Glass', New York Times (12 Jan 1970), 96.
The one who stays in my mind as the ideal man of science is, not Huxley or Tyndall, Hooker or Lubbock, still less my friend, philosopher and guide Herbert Spencer, but Francis Galton, whom I used to observe and listen to—I regret to add, without the least reciprocity—with rapt attention. Even to-day. I can conjure up, from memory’s misty deep, that tall figure with its attitude of perfect physical and mental poise; the clean-shaven face, the thin, compressed mouth with its enigmatical smile; the long upper lip and firm chin, and, as if presiding over the whole personality of the man, the prominent dark eyebrows from beneath which gleamed, with penetrating humour, contemplative grey eyes. Fascinating to me was Francis Galton’s all-embracing but apparently impersonal beneficence. But, to a recent and enthusiastic convert to the scientific method, the most relevant of Galton’s many gifts was the unique contribution of three separate and distinct processes of the intellect; a continuous curiosity about, and rapid apprehension of individual facts, whether common or uncommon; the faculty for ingenious trains of reasoning; and, more admirable than either of these, because the talent was wholly beyond my reach, the capacity for correcting and verifying his own hypotheses, by the statistical handling of masses of data, whether collected by himself or supplied by other students of the problem.
In My Apprenticeship (1926), 134-135.
The only difference between a problem and a solution is that people understand the solution.
The only place where a dollar is still worth one hundred cents today is in the problems in an arithmetic book.
In Evan Esar, 20,000 Quips and Quotes, 509.
The open secret of real success is to throw your whole personality into your problem.
How to Solve it: A New Aspect of Mathematical Method (1957), 207.
The ordinary naturalist is not sufficiently aware that when dogmatizing on what species are, he is grappling with the whole question of the organic world & its connection with the time past & with Man; that it involves the question of Man & his relation to the brutes, of instinct, intelligence & reason, of Creation, transmutation & progressive improvement or development. Each set of geological questions & of ethnological & zool. & botan. are parts of the great problem which is always assuming a new aspect.
Leonard G. Wilson (ed.), Sir Charles Lyell's Scientific Journals on the Species Question (1970), 164.
The origin of a science is usually to be sought for not in any systematic treatise, but in the investigation and solution of some particular problem. This is especially the case in the ordinary history of the great improvements in any department of mathematical science. Some problem, mathematical or physical, is proposed, which is found to be insoluble by known methods. This condition of insolubility may arise from one of two causes: Either there exists no machinery powerful enough to effect the required reduction, or the workmen are not sufficiently expert to employ their tools in the performance of an entirely new piece of work. The problem proposed is, however, finally solved, and in its solution some new principle, or new application of old principles, is necessarily introduced. If a principle is brought to light it is soon found that in its application it is not necessarily limited to the particular question which occasioned its discovery, and it is then stated in an abstract form and applied to problems of gradually increasing generality.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
Other principles, similar in their nature, are added, and the original principle itself receives such modifications and extensions as are from time to time deemed necessary. The same is true of new applications of old principles; the application is first thought to be merely confined to a particular problem, but it is soon recognized that this problem is but one, and generally a very simple one, out of a large class, to which the same process of investigation and solution are applicable. The result in both of these cases is the same. A time comes when these several problems, solutions, and principles are grouped together and found to produce an entirely new and consistent method; a nomenclature and uniform system of notation is adopted, and the principles of the new method become entitled to rank as a distinct science.
In A Treatise on Projections (1880), Introduction, xi. Published as United States Coast and Geodetic Survey, Treasury Department Document, No. 61.
The pace of science forces the pace of technique. Theoretical physics forces atomic energy on us; the successful production of the fission bomb forces upon us the manufacture of the hydrogen bomb. We do not choose our problems, we do not choose our products; we are pushed, we are forced—by what? By a system which has no purpose and goal transcending it, and which makes man its appendix.
…...
The persons who have been employed on these problems of applying the properties of matter and the laws of motion to the explanation of the phenomena of the world, and who have brought to them the high and admirable qualities which such an office requires, have justly excited in a very eminent degree the admiration which mankind feels for great intellectual powers. Their names occupy a distinguished place in literary history; and probably there are no scientific reputations of the last century higher, and none more merited, than those earned by great mathematicians who have laboured with such wonderful success in unfolding the mechanism of the heavens; such for instance as D ’Alembert, Clairaut, Euler, Lagrange, Laplace.
In Astronomy and General Physics (1833), Bk. 3, chap. 4, 327.
The physicians surely are the natural advocates of the poor and the social problem largely falls within their scope.
Introductory article, 'The Aims of the Journal “Medical Reform”', in the first edition of Die medizinische Reform (10 Jul 1848). From the original in German, “Die Ärzte sind die natürlichen Anwälte der Armen und die sociale Frage fallt zu einem erheblichen Theil in ihre Jurisdiction.” As translated in Rudolf Virchow and L.J. Rather (ed.), Collected Essays on Public Health and Epidemiology (1985), Vol. 1, 4. Elsewhere seen translated as “Physicians are the natural attorneys of the poor, and the social problems should largely be solved by them,” or “The physicians are the natural attorneys of the poor, and social problems fall to a large extent within their jurisdiction.”
The physicist cannot simply surrender to the philosopher the critical contemplation of the theoretical foundations for he himself knows best and feels most surely where the shoe pinches. … he must try to make clear in his own mind just how far the concepts which he uses are justified … The whole of science is nothing more than a refinement of everyday thinking. It is for this reason that the critical thinking of the physicist cannot possibly be restricted by the examination of the concepts of his own specific field. He cannot proceed without considering critically a much more difficult problem, the problem of analyzing the nature of everyday thinking.
‘Physics and Reality’, Franklin Institute Journal (Mar 1936). Collected in Out of My Later Years (1950), 59.
The physicist’s problem is the problem of ultimate origins and ultimate natural laws. The biologist's problem is the problem of complexity.
In The Blind Watchmaker (1996), 15.
The politician … is sometimes tempted to encroach on the normal territory of the scientific estate. Sometimes he interferes directly with the scientist’s pursuit of basic science; but he is more likely to interfere when the scientist proposes to publish findings that upset the established political or economic order, or when he joins with the engineering or medical profession in proposing to translate the findings of science into new policies. … Who decides when the apparent consensus of scientific opinion on the relation of cigarettes to lung cancer is great enough to justify governmental regulatory action, and of what kind? In such issues the problem is less often whether politics will presume to dictate to science than it is how much politics is to be influenced by the new findings of science.
In The Scientific Estate (1965), 201.
The prediction of nuclear winter is drawn not, of course, from any direct experience with the consequences of global nuclear war, but rather from an investigation of the governing physics. (The problem does not lend itself to full experimental verification—at least not more than once.)[co-author with American atmospheric chemist Richard P. Turco (1943- )]
A Path Where No Man Thought: Nuclear Winter and the End of the Arms Race (1990), 26.
The present rate of progress [in X-ray crystallography] is determined, not so much by the lack of problems to investigate or the limited power of X-ray analysis, as by the restricted number of investigators who have had a training in the technique of the new science, and by the time it naturally takes for its scientific and technical importance to become widely appreciated.
Concluding remark in Lecture (1936) on 'Forty Years of Crystal Physics', collected in Needham and Pagel (eds.) in Background to Modern Science: Ten Lectures at Cambridge Arranged by the History of Science Committee, (1938), 89.
The principles of medical management are essentially the same for individuals of all ages, albeit the same problem is handled differently in different patients. ... [just as] the principles of driving an automobile are uniform,
but one drives in one manner on the New Jersey Turnpike and in another manner on a narrow, winding road in the Rocky Mountains.
Quoted in Joseph Earle Moore, The Neurologic and Psychiatric Aspects of the Disorders of Aging (1956), 247.
The prize is such an extraordinary honor. It might seem unfair, however, to reward a person for having so much pleasure over the years, asking the maize plant to solve specific problems and then watching its responses.
Quoted in the New York Times, 11 Oct 1983.
The problem [evolution] presented itself to me, and something led me to think of the positive checks described by Malthus in his Essay on Population, a work I had read several years before, and which had made a deep and permanent impression on my mind. These checks—war, disease, famine, and the like—must, it occurred to me, act on animals as well as man. Then I thought of the enormously rapid multiplication of animals, causing these checks to be much more effective in them than in the case of man; and while pondering vaguely on this fact, there suddenly flashed upon me the idea of the survival of the fittest—that the individuals removed by these checks must be on the whole inferior to those that survived. I sketched the draft of my paper … and sent it by the next post to Mr. Darwin.
In 'Introductory Note to Chapter II in Present Edition', Natural Selection and Tropical Nature Essays on Descriptive and Theoretical Biology (1891, New ed. 1895), 20.
The problem [with genetic research] is, we're just starting down this path, feeling our way in the dark. We have a small lantern in the form of a gene, but the lantern doesn't penetrate more than a couple of hundred feet. We don't know whether we're going to encounter chasms, rock walls or mountain ranges along the way. We don't even know how long the path is.
Quoted in J. Madeleine Nash, et al., 'Tracking Down Killer Genes', Time magazine (17 Sep 1990).
The problem for a writer of a text-book has come now, in fact, to be this—to write a book so neatly trimmed and compacted that no coach, on looking through it, can mark a single passage which the candidate for a minimum pass can safely omit. Some of these text-books I have seen, where the scientific matter has been, like the lady’s waist in the nursery song, compressed “so gent and sma’,” that the thickness barely, if at all, surpasses what is devoted to the publisher’s advertisements. We shall return, I verily believe, to the Compendium of Martianus Capella. The result of all this is that science, in the hands of specialists, soars higher and higher into the light of day, while educators and the educated are left more and more to wander in primeval darkness.
In Presidential Address British Association for the Advancement of Science (1885), Nature, 32, 448. [Martianus Capella, who flourished c.410-320, wrote a compendium of the seven liberal arts. —Webmaster]
The problem in the world is that there are too many rich people.
In Associated Press, 'Population Expert Faults Wealthy', Sarasota Herald-Tribune (6 Apr 1990), 15A.
The problem is not to find the best or most efficient method to proceed to a discovery, but to find any method at all.
In his Nobel Prize Lecture (11 Dec 1965), 'The Development of the Space-Time View of Quantum Electrodynamics'. Collected in Stig Lundqvist, Nobel Lectures: Physics, 1963-1970 (1998), 177.
The problem is that people think faith is something to be admired. In fact, faith means you believe in something for which you have no evidence.
In God and the Folly of Faith: The Incompatibility of Science (2012), 18.
The problem of distinguishing prime numbers from composite numbers and of resolving the latter into their prime factors is known to be one of the most important and useful in arithmetic. It has engaged the industry and wisdom of ancient and modern geometers to such an extent that it would be superfluous to discuss the problem at length... Further, the dignity of the science itself seems to require that every possible means be explored for the solution of a problem so elegant and so celebrated.
Disquisitiones Arithmeticae (1801), Article 329
The problem of experiences is not limited to the interpretation of sense-impressions.
Swarthmore Lecture (1929) at Friends’ House, London, printed in Science and the Unseen World (1929), 40.
The problem of modern democracy is not that the people have lost their power, but that they have lost their appreciation for the extraordinary power they wield. Consider one astonishing truth: Famine has never struck a democracy.
In Jacques Cousteau and Susan Schiefelbein, The Human, the Orchid, and the Octopus: Exploring and Conserving Our Natural World (2007), 102.
The problem of values arises only when men try to fit together their need to be social animals with their need to be free men. There is no problem, and there are no values, until men want to do both. If an anarchist wants only freedom, whatever the cost, he will prefer the jungle of man at war with man. And if a tyrant wants only social order, he will create the totalitarian state.
Science and Human Values (1961), 63.
The problem with linear theory is that it is not nonlinear.
Epigraph in Mathematics in Nature: Modeling Patterns in the Natural World (2003), 173. Quoting from his own first professional presentation as a first-year graduate student.
The problem with quotes on the Internet is that it is hard to verify their authenticity.
…...
The problem with the gene pool is that there is no lifeguard.
The problem, then, is how to bring about a striving for harmony with land among a people many of whom have forgotten there is any such thing as land, among whom education and culture have become almost synonymous with landlessness. This is the problem of conservation education.
In 'Conservation' (1938), collected in Luna B. Leopold (ed.), Round River: From the Journals of Aldo Leopold (1966, 1972), 155.
The problems of analyzing war operations are … rather nearer, in general, to many problems, say of biology or of economics, than to most problems of physics, where usually a great deal of numerical data are ascertainable about relatively simple phenomena.
In report at the British Association Annual Meeting, Dundee (30 Aug 1947), published in 'Operational Research in War and Peace', The Advancement of Science (1948), 17, 320-332. Collected in P.M.S. Blackett, Studies of War: Nuclear and Conventional (1962), 177.
The problems of the infinite have challenged man’s mind and have fired his imagination as no other single problem in the history of thought. The infinite appears both strange and familiar, at times beyond our grasp, at times easy and natural to understand. In conquering it, man broke the fetters that bound him to earth. All his faculties were required for this conquest—his reasoning powers, his poetic fancy, his desire to know.
With co-author James R Newman, in 'Beyond the Google', Mathematics and the Imagination (1940), 35.
The problems of the world cannot possibly be solved by skeptics or cynics whose horizons are limited by the obvious realities. We need men who can dream of things that never were.
From Address (Jun 1963) to the Irish Parliament, Dublin, as collected in Public Papers of the Presidents of the United States: John F. Kennedy (1964), 537.
The professor may choose familiar topics as a starting point. The students collect material, work problems, observe regularities, frame hypotheses, discover and prove theorems for themselves. … the student knows what he is doing and where he is going; he is secure in his mastery of the subject, strengthened in confidence of himself. He has had the experience of discovering mathematics. He no longer thinks of mathematics as static dogma learned by rote. He sees mathematics as something growing and developing, mathematical concepts as something continually revised and enriched in the light of new knowledge. The course may have covered a very limited region, but it should leave the student ready to explore further on his own.
In A Concrete Approach to Abstract Algebra (1959), 1-2.
The psyche is distinctly more complicated and inaccessible than the body. It is, so to speak, the half of the world which comes into existence only when we become conscious of it. For that reason the psyche is not only a personal but a world problem, and the psychiatrist has to deal with an entire world.
From Erinnerungen, Träume, Gedanken, as translated in Memories, Dreams, Reflections (1963), 132.
The question of questions for mankind—the problem which underlies all others, and is more deeply interesting than any other—is the ascertainment of the place which Man occupies in nature and of his relations to the universe of things.
'On the Relations of Man to the Lower Animals' (1863). In Collected Essays (1894). Vol. 7, 77.
The real achievement in discoveries … is seeing an analogy where no one saw one before. … The essence of discovery is that unlikely marriage of … previously unrelated forms of reference or universes of discourse, whose union will solve the previously insoluble problem.
In Act of Creation (1964), 201.
The real problem in speech is not precise language. The problem is clear language. The desire is to have the idea clearly communicated to the other person. [But] precise language is not precise in any sense if you deal with the real objects of the world, and is overly pedantic and quite confusing to use it unless there are some special subtleties which have to be carefully distinguished.
Criticizing “overly pedantic” language in proposed textbooks for a modified arithmetic course for grades 1-8 in California schools. In article, 'New Textbooks for the ‘New’ Mathematics', Engineering and Science (Mar 1965), 28, No. 6. Collected in Perfectly Reasonable Deviations from the Beaten Track: The Letters of Richard Feynman (2008), 454. He was writing as a member of the California State Curriculum Committee
The real problem is not the loss of a particular species but the loss of particular kinds of environments. … When you lose a big, dramatic species like the whooping crane, you don’t notice that you are also losing other plants and animals. … We are only putting Band-Aids on until we recognize we need to be protecting environments, not just endangered species.
In Philip Shabecoff, 'Further Safeguards Urged For Endangered Species', New York Times (14 Mar 1985), B9. Attenborough was in America to testify before a Congressional subcommittee considering the reauthorization of the Endangered Species Act (originally passed in 1973).
The real value of science is in the getting, and those who have tasted the pleasure of discovery alone know what science is. A problem solved is dead. A world without problems to be solved would be devoid of science.
In Matter and Energy (1912), 18.
The release of atomic energy has not created a new problem. It has merely made more urgent the necessity of solving an existing one … I do not believe that civilization will be wiped out in a war fought with the atomic bomb. Perhaps two thirds of the people of the Earth would be killed.
In interview with Raymond Swing, 'Einstein on the Atomic Bomb' Atlantic Monthly, (Nov 1945), 176, No. 5, 43.

The reproaches against science for not having yet solved the problems of the universe are exaggerated in an unjust and malicious manner; it has truly not had time enough yet for these great achievements. Science is very young—a human activity which developed late.
The Question of a Weltanschauung? (1932), in James Strachey (ed.), The Standard Edition of the Complete Psychological Works of Sigmund Freud (1964), Vol. 22, 173.
The rigid career path of a professor at a modern university is that One Must Build the Big Research Group, recruit doctoral students more vigorously than the head football coach, bombard the federal agencies with grant applications more numerous than the pollen falling from the heavens in spring, and leave the paper writing and the research to the postdocs, research associates, and students who do all the bench work and all the computer programming. A professor is chained to his previous topics by his Big Group, his network of contacts built up laboriously over decades, and the impossibility of large funding except in areas where the grantee has grown the group from a corner of the building to an entire floor. The senior tenure-track faculty at a research university–the “silverbacks” in anthropological jargon–are bound by invisible chains stronger than the strongest steel to a narrow range of what the Prevailing Consensus agrees are Very Important Problems. The aspiring scientist is confronted with the reality that his mentors are all business managers.
In his Foreword to Cornelius Lanczos, Discourse on Fourier Series, ix-x.
The same society which receives the rewards of technology must, as a cooperating whole, take responsibility for control. To deal with these new problems will require a new conservation. We must not only protect the countryside and save it from destruction, we must restore what has been destroyed and salvage the beauty and charm of our cities. Our conservation must be not just the classic conservation of protection and development, but a creative conservation of restoration and innovation. Its concern is not with nature alone, but with the total relation between man and the world around him. Its object is not just man's welfare, but the dignity of man's spirit.
In his 'Message to Congress on Conservation and Restoration of Natural Beauty' written to Congress (8 Feb 1965). It was a broad initiative aimed at beautifying America, guaranteeing water and air quality, and preserving natural areas. In Lyndon B. Johnson: Containing the Public Messages, Speeches, and Statements of the President (1965), Vol.1, 156.
United States. President (1963-1969 : Johnson), Lyndon Baines Johnson, United States. Office of the Federal Register - 1970
The scientific method of examining facts is not peculiar to one class of phenomena and to one class of workers; it is applicable to social as well as to physical problems, and we must carefully guard ourselves against supposing that the scientific frame of mind is a peculiarity of the professional scientist.
From The Grammar of Science (1892), 8.
The scientist has marched in and taken the place of the poet. But one day somebody will find the solution to the problems of the world and remember, it will be a poet, not a scientist.
As quoted in The Star (1959). Collected in Jonathon Green, Morrow's International Dictionary of Contemporary Quotations (1982).
The scientist, by the very nature of his commitment, creates more and more questions, never fewer. Indeed the measure of our intellectual maturity, one philosopher suggests, is our capacity to feel less and less satisfied with our answers to better problems.
Becoming: Basic Considerations for a Psychology of Personality (1955), 67.
The second law of thermodynamics is, without a doubt, one of the most perfect laws in physics. Any reproducible violation of it, however small, would bring the discoverer great riches as well as a trip to Stockholm. The world’s energy problems would be solved at one stroke… . Not even Maxwell’s laws of electricity or Newton’s law of gravitation are so sacrosanct, for each has measurable corrections coming from quantum effects or general relativity. The law has caught the attention of poets and philosophers and has been called the greatest scientific achievement of the nineteenth century.
In Thermodynamics (1964). As cited in The Mathematics Devotional: Celebrating the Wisdom and Beauty of Physics (2015), 82.
The secret of science is to ask the right question, and it is the choice of problem more than anything else that marks the man of genius in the scientific world.
As quoted in the Inaugural Sir Henry Tizard Memorial Lecture at Westminster School (21 Feb 1963) by Sir George Thomson 'Research in Theory and Practice'. As cited Ray Corrigan, Digital Decision Making: Back to the Future (2007), 142.
The situation with regard to insulin is particularly clear. In many parts of the world diabetic children still die from lack of this hormone. ... [T]hose of us who search for new biological facts and for new and better therapeutic weapons should appreciate that one of the central problems of the world is the more equitable distribution and use of the medical and nutritional advances which have already been established. The observations which I have recently made in parts of Africa and South America have brought this fact very forcible to my attention.
'Studies on Diabetes and Cirrhosis', Proceedings, American Philosophical Society (1952) 96, No. 1, 29.
The skeptic does not mean him who doubts, but him who investigates or researches, as opposed to him who asserts and thinks that he has found. The one is the man who studies the problem and the other is the man who gives us a formula, correct or incorrect, as the solution of it.
'My Religion', Essays and Soliloquies, translated by John Ernest Crawford Flitch (1925), 56. In Robert Andrews, The Columbia Dictionary of Quotations (1993), 844:9.
The solution of problems is one of the lowest forms of mathematical research, … yet its educational value cannot be overestimated. It is the ladder by which the mind ascends into higher fields of original research and investigation. Many dormant minds have been aroused into activity through the mastery of a single problem.
With co-editor J. M. Colaw, Editorial introducing the first issue of The American Mathematical Monthly (Jan 1894), 1, No. 1, 2.
The solutions put forth by imperialism are the quintessence of simplicity...When they speak of the problems of population and birth, they are in no way moved by concepts related to the interests of the family or of society...Just when science and technology are making incredible advances in all fields, they resort to technology to suppress revolutions and ask the help of science to prevent population growth. In short, the peoples are not to make revolutions, and women are not to give birth. This sums up the philosophy of imperialism.
From Fidel Castro (1968).
The stakes are immense, the task colossal, the time is short. But we may hope–we must hope–that man’s own creation, man’s own genius, will not destroy him. Scholars, indeed all men, must move forward in the faith of that philosopher who held that there is no problem the human reason can propound which the human reason cannot reason out.
From 'Is Einstein Right?', in William Allison Shimer (ed.), The American Scholar (1946), 15, 476. Reprinted in American Thought 1947 (1947), 196. Gauss is commenting on an article by Einstein about the challenges following the creation of the atomic bomb, 'The Real Problem Is in the Hearts of Men', New York Times Magazine (23 Jun 1946), SM4.
The successes of the differential equation paradigm were impressive and extensive. Many problems, including basic and important ones, led to equations that could be solved. A process of self-selection set in, whereby equations that could not be solved were automatically of less interest than those that could.
In Does God Play Dice? The Mathematics of Chaos (1989, 1997), 33.
The suppression of crime is not entirely a legal question. It is a problem for the physician, the economist and the lawyer. We, as physicians, should encourage the criminologist by lending to him the surgeon, the internist and all of the rest of the resources of medicine, just as we have done in the case of the flea man, the fly man, the mosquito man, the bed-bug man and all the other ologists.
From paper read at the Section on State Medicine and Public Hygiene of the State Medical Association of Texas at El Paso (11 May 1922), 'The Use Of Scopolamine In Criminology', published in Texas State Journal of Medicine (Sep 1922). Reprinted in The American Journal of Police Science (Jul-Aug 1931), 2, No. 4, 328.
The teacher can seldom afford to miss the questions: What is the unknown? What are the data? What is the condition? The student should consider the principal parts of the problem attentively, repeatedly, and from various sides.
In How to Solve It: A New Aspect of Mathematical Method (2004), 77
The theory of numbers is particularly liable to the accusation that some of its problems are the wrong sort of questions to ask. I do not myself think the danger is serious; either a reasonable amount of concentration leads to new ideas or methods of obvious interest, or else one just leaves the problem alone. “Perfect numbers” certainly never did any good, but then they never did any particular harm.
In A Mathematician’s Miscellany (1953). Reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 74.
The traditional method of confronting the student not with the problem but with the finished solution means depriving him of all excitement, to shut off the creative impulse, to reduce the adventure of mankind to a dusty heap of theorems.
In The Act of Creation (1964), 266.
The transition from a paradigm in crisis to a new one from which a new tradition of normal science can emerge is far from a cumulative process, one achieved by an articulation or extension of the old paradigm. Rather it is a reconstruction of the field from new fundamentals, a reconstruction that changes some of the field's most elementary theoretical generalizations as well as many of its paradigm methods and applications. During the transition period there will be a large but never complete overlap between the problems that can be solved by the old and by the new paradigm. But there will also be a decisive difference in the modes of solution. When the transition is complete, the profession will have changed its view of the field, its methods, and its goals.
The Structure of Scientific Revolutions (1962), 84-5.
The transition from sea-floor spreading to plate tectonics is largely a change of emphasis. Sea-floor spreading is a view about the method of production of new oceans floor on the ridge axis. The magnetic lineations give the history of this production back into the late Mesozoic and illuminate the history of the new aseismic parts of the ocean floor. This naturally directed attention to the relation of the sea-floor to the continents. There are two approaches: in the first, one looks back in time to earlier arrangements of the continents; in the second, one considers the current problem of the disposal of the rapidly growing sea floor.
'The Emergence of Plate Tectonics: A Personal View', Annual Review of Earth and Planetary Sciences, 1975, 3, 20.
The understanding of a complex problem such as atherosclerosis requires the tools of basic science. We are fortunate to live at a time when the methods of basic science are so powerful that they can be applied directly to clinical problems. … [T]he two attributes that are required – basic training and technical courage.
In Banquet Speech, 'The Nobel Prize in Physiology or Medicine 1985', on website nobelprize.org. Published in Les Prix Nobel, 1985: Nobel Prizes, Presentations, Biographies and Lectures (1986).
The Unexpected stalks a farm in big boots like a vagrant bent on havoc. Not every farmer is an inventor, but the good ones have the seeds of invention within them. Economy and efficiency move their relentless tinkering and yet the real motive often seems to be aesthetic. The mind that first designed a cutter bar is not far different from a mind that can take the intractable steel of an outsized sickle blade and make it hum in the end. The question is how to reduce the simplicity that constitutes a problem (“It's simple; it’s broke.”) to the greater simplicity that constitutes a solution.
In Making Hay (2003), 33-34.
The United States is the most powerful technically advanced country in the world to-day. Its influence on the shaping of international relations is absolutely incalculable. But America is a large country and its people have so far not shown much interest in great international problems, among which the problem of disarmament occupies first place today. This must be changed, if only in the essential interests of the Americans. The last war has shown that there are no longer any barriers between the continents and that the destinies of all countries are closely interwoven. The people of this country must realize that they have a great responsibility in the sphere of international politics. The part of passive spectator is unworthy of this country and is bound in the end to lead to disaster all round.
…...
The universality of parasitism as an offshoot of the predatory habit negatives the position taken by man that it is a pathological phenomenon or a deviation from the normal processes of nature. The pathological manifestations are only incidents in a developing parasitism. As human beings intent on maintaining man's domination over nature we may regard parasitism as pathological insofar as it becomes a drain upon human resources. In our efforts to protect ourselves we may make every kind of sacrifice to limit, reduce, and even eliminate parasitism as a factor in human life. Science attempts to define the terms on which this policy of elimination may or may not succeed. We must first of all thoroughly understand the problem, put ourselves in possession of all the facts in order to estimate the cost. Too often it has been assumed that parasitism was abnormal and that it needed only a slight force to reestablish what was believed to be a normal equilibrium without parasitism. On the contrary, biology teaches us that parasitism is a normal phenomenon and if we accept this view we shall be more ready to pay the price of freedom as a permanent and ever recurring levy of nature for immunity from a condition to which all life is subject. The greatest victory of man over nature in the physical realm would undoubtedly be his own delivery from the heavy encumbrance of parasitism with which all life is burdened.
Parasitism and Disease (1934), 4.
The value of fundamental research does not lie only in the ideas it produces. There is more to it. It affects the whole intellectual life of a nation by determining its way of thinking and the standards by which actions and intellectual production are judged. If science is highly regarded and if the importance of being concerned with the most up-to-date problems of fundamental research is recognized, then a spiritual climate is created which influences the other activities. An atmosphere of creativity is established which penetrates every cultural frontier. Applied sciences and technology are forced to adjust themselves to the highest intellectual standards which are developed in the basic sciences. This influence works in many ways: some fundamental students go into industry; the techniques which are applied to meet the stringent requirements of fundamental research serve to create new technological methods. The style, the scale, and the level of scientific and technical work are determined in pure research; that is what attracts productive people and what brings scientists to those countries where science is at the highest level. Fundamental research sets the standards of modern scientific thought; it creates the intellectual climate in which our modern civilization flourishes. It pumps the lifeblood of idea and inventiveness not only into the technological laboratories and factories, but into every cultural activity of our time. The case for generous support for pure and fundamental science is as simple as that.
In 'Why Pure Science?' in Bulletin of the Atomic Scientists, 1965.
The virgin fertility of our soils, and the vast amount of unskilled labor, have been more of a curse than a blessing to agriculture. This exhaustive system for cultivation, the destruction of forests, the rapid and almost constant decomposition of organic matter, together with the problems of nitrification and denitrification, the multitudinous insects and fungus diseases which are ever increasing with marvelous rapidity year by year, make our agricultural problem one requiring more brains than of the North, East or West.
In Farmer’s Leaflet 7: The Need of Scientific Agriculture in the South (1902). Reprinted in The Review of Reviews (1902), 25, 322.
The way of pure research is opposed to all the copy-book maxims concerning the virtues of industry and a fixed purpose, and the evils of guessing, but it is damned useful when it comes off. It is the diametrical opposite of Edison’s reputed method of trying every conceivable expedient until he hit the right one. It requires, not diligence, but experience, information, and a good nose for the essence of a problem.
Letter to Paul de Kruif (3 Aug 1933), as quoted in Nathan Reingold, Science in America: A Documentary History 1900-1939 (1981), 409.
The Wegener hypothesis has been so stimulating and has such fundamental implications in geology as to merit respectful and sympathetic interest from every geologist. Some striking arguments in his favor have been advanced, and it would be foolhardy indeed to reject any concept that offers a possible key to the solution of profound problems in the Earth’s history.
Published while geologists remained sceptical of Alfred Wegener’s idea of Continental Drift, Though unconvinced, he published these thoughts suggesting that critics should be at least be open-minded. His patience was proven justified when two decades later, the theory of plate tectonics provided a mechanism for the motion of the continents.
Published while geologists remained sceptical of Alfred Wegener’s idea of Continental Drift, Though unconvinced, he published these thoughts suggesting that critics should be at least be open-minded. His patience was proven justified when two decades later, the theory of plate tectonics provided a mechanism for the motion of the continents.
Some Thoughts on the Evidence for Continental Drift (1944).
The whole problem with the world is that fools and fanatics are always so certain of themselves, but wiser people so full of doubts.
Apparently apocryphal. Although found widely quoted, Webmaster has as yet not found when or where he is purported to have uttered these exact words. Webmaster believes the idea may have existed as an aphorism that predates Russell. (If you know the primary source, please make contact.)
The whole question of imagination in science is often misunderstood by people in other disciplines. They try to test our imagination in the following way. They say, “Here is a picture of some people in a situation. What do you imagine will happen next?” When we say, “I can’t imagine,” they may think we have a weak imagination. They overlook the fact that whatever we are allowed to imagine in science must be consistent with everything else we know; that the electric fields and the waves we talk about are not just some happy thoughts which we are free to make as we wish, but ideas which must be consistent with all the laws of physics we know. We can’t allow ourselves to seriously imagine things which are obviously in contradiction to the laws of nature. And so our kind of imagination is quite a difficult game. One has to have the imagination to think of something that has never been seen before, never been heard of before. At the same time the thoughts are restricted in a strait jacket, so to speak, limited by the conditions that come from our knowledge of the way nature really is. The problem of creating something which is new, but which is consistent with everything which has been seen before, is one of extreme difficulty
In The Feynman Lectures in Physics (1964), Vol. 2, Lecture 20, p.20-10 to p.20-11.
The world won’t come to an end, but the incidence of disasters will have a very big impact, and in ways we can't predict. … Rises in seas levels will displace millions of people. It’s estimated there will be 150 million refugees by 2050, homeless as a result of global warming. It’s how we deal with these problems that is as much the challenge as tackling the causes of global warming.
In The Independent (10 Aug 2003).
There are children playing in the street who could solve some of my top problems in physics, because they have modes of sensory perception that I lost long ago.
…...
There are no small problems. Problems that appear small are large problems that are not understood
From Reglas y Consejos sobre Investigacíon Cientifica: Los tónicos de la voluntad. (1897), as translated by Neely and Larry W. Swanson, in Advice for a Young Investigator (1999), 17.
There are problems to whose solution I would attach an infinitely greater importance than to those of mathematics, for example touching ethics, or our relation to God, or concerning our destiny and our future; but their solution lies wholly beyond us and completely outside the province of science.
Quoted in J.R. Newman, The World of Mathematics (1956), 314.
There are some four million different kinds of animals and plants in the world. Four million different solutions to the problems of staying alive.
As quoted in Jack Shepherd, "David Attenborough: 15 of the naturalist’s best quotes: In celebration of his 94th birthday", Independent (8 May 2017), on independent.co.uk website.
There are still many unsolved problems about bird life, among which are the age that birds attain, the exact time at which some birds acquire their adult dress, and the changes which occur in this with years. Little, too, is known about the laws and routes of bird migration, and much less about the final disposition of the untold thousands which are annually produced.
There are still psychologists who, in a basic misunderstanding, think that gestalt theory tends to underestimate the role of past experience. Gestalt theory tries to differentiate between and-summative aggregates, on the one hand, and gestalten, structures, on the other, both in sub-wholes and in the total field, and to develop appropriate scientific tools for investigating the latter. It opposes the dogmatic application to all cases of what is adequate only for piecemeal aggregates. The question is whether an approach in piecemeal terms, through blind connections, is or is not adequate to interpret actual thought processes and the role of the past experience as well. Past experience has to be considered thoroughly, but it is ambiguous in itself; so long as it is taken in piecemeal, blind terms it is not the magic key to solve all problems.
In Productive Thinking (1959), 65.
There are, at present, fundamental problems in theoretical physics … the solution of which … will presumably require a more drastic revision of our fundmental concepts than any that have gone before. Quite likely, these changes will be so great that it will be beyond the power of human intelligence to get the necessary new ideas by direct attempts to formulate the experimental data in mathematical terms. The theoretical worker in the future will, therefore, have to proceed in a more direct way. The most powerful method of advance that can be suggested at present is to employ all the resources of pure mathematics in attempts to perfect and generalize the mathematical formalism that forms the existing basis of theoretical physics, and after each success in this direction, to try to interpret the new mathematical features in terms of physical entities.
At age 28.
At age 28.
Proceedings of the Royal Society (1931), A133, 60. In A. Pais, 'Playing With Equations, the Dirac Way'. Behram N. Kursunoglu (Ed.) and Eugene Paul Wigner (Ed.), Paul Adrien Maurice Dirac: Reminiscences about a Great Physicist (1990), 109.
There is always a well-known solution to every human problem—neat, plausible, and wrong.
As given in 'The Divine Afflatus', Prejudices: Second Series (1920), 157. Various paraphrases changing Mencken’s original words, are also found. For example, “…an easy solution…” or “Every complex problem has a solution that is simple, neat, and wrong.” Also sometimes seen both paraphrased and misattributed, for example, to Thomas Huxley. [See further discussion on quoteinvestigator.com website.]
There is no area in our minds reserved for superstition, such as the Greeks had in their mythology; and superstition, under cover of an abstract vocabulary, has revenged itself by invading the entire realm of thought. Our science is like a store filled with the most subtle intellectual devices for solving the most complex problems, and yet we are almost incapable of applying the elementary principles of rational thought. In every sphere, we seem to have lost the very elements of intelligence: the ideas of limit, measure, degree, proportion, relation, comparison, contingency, interdependence, interrelation of means and ends. To keep to the social level, our political universe is peopled exclusively by myths and monsters; all it contains is absolutes and abstract entities. This is illustrated by all the words of our political and social vocabulary: nation, security, capitalism, communism, fascism, order, authority, property, democracy. We never use them in phrases such as: There is democracy to the extent that… or: There is capitalism in so far as… The use of expressions like “to the extent that” is beyond our intellectual capacity. Each of these words seems to represent for us an absolute reality, unaffected by conditions, or an absolute objective, independent of methods of action, or an absolute evil; and at the same time we make all these words mean, successively or simultaneously, anything whatsoever. Our lives are lived, in actual fact, among changing, varying realities, subject to the casual play of external necessities, and modifying themselves according to specific conditions within specific limits; and yet we act and strive and sacrifice ourselves and others by reference to fixed and isolated abstractions which cannot possibly be related either to one another or to any concrete facts. In this so-called age of technicians, the only battles we know how to fight are battles against windmills.
From 'The Power of Words', collected in Siân Miles (ed.), Simone Weil: An Anthology (2000), 222-223.
There is no counting the unsolved problems of Natural History.
In Riddles of Science (1932), 102.
There is no one central problem in philosophy, but countless little problems. Philosophy is like trying to open a safe with a combination lock: each little adjustment of the dials seems to achieve nothing, only when everything is in place does the door open.
From conversation with Rush Rhees (1930) as given by Rush Rhees in Ludwig Wittgenstein: Personal Recollections (1981), 96.
There was yet another disadvantage attaching to the whole of Newton’s physical inquiries, ... the want of an appropriate notation for expressing the conditions of a dynamical problem, and the general principles by which its solution must be obtained. By the labours of LaGrange, the motions of a disturbed planet are reduced with all their complication and variety to a purely mathematical question. It then ceases to be a physical problem; the disturbed and disturbing planet are alike vanished: the ideas of time and force are at an end; the very elements of the orbit have disappeared, or only exist as arbitrary characters in a mathematical formula
Address to the Mechanics Institute, 'An Address on the Genius and Discoveries of Sir Isaac Newton' (1835), excerpted in paper by Luis M. Laita, Luis de Ledesma, Eugenio Roanes-Lozano and
Alberto Brunori, 'George Boole, a Forerunner of Symbolic Computation', collected in John A. Campbell and Eugenio Roanes-Lozano (eds.), Artificial Intelligence and Symbolic Computation: International Conference AISC 2000 (2001), 3.
There will always be a psychological problem in the peasant’s soul: no one is born a Communist. In the Soviet Union farmers look in the barn for “their” horses even after they have given them to the collective.
As quoted in Editorial, 'The High Cost of Marx on the Farm', Life (23 Nov 1962), 38.
These machines [used in the defense of the Syracusans against the Romans under Marcellus] he [Archimedes] had designed and contrived, not as matters of any importance, but as mere amusements in geometry; in compliance with king Hiero’s desire and request, some time before, that he should reduce to practice some part of his admirable speculation in science, and by accommodating the theoretic truth to sensation and ordinary use, bring it more within the appreciation of people in general. Eudoxus and Archytas had been the first originators of this far-famed and highly-prized art of mechanics, which they employed as an elegant illustration of geometrical truths, and as means of sustaining experimentally, to the satisfaction of the senses, conclusions too intricate for proof by words and diagrams. As, for example, to solve the problem, so often required in constructing geometrical figures, given the two extremes, to find the two mean lines of a proportion, both these mathematicians had recourse to the aid of instruments, adapting to their purpose certain curves and sections of lines. But what with Plato’s indignation at it, and his invectives against it as the mere corruption and annihilation of the one good of geometry,—which was thus shamefully turning its back upon the unembodied objects of pure intelligence to recur to sensation, and to ask help (not to be obtained without base supervisions and depravation) from matter; so it was that mechanics came to be separated from geometry, and, repudiated and neglected by philosophers, took its place as a military art.
— Plutarch
In John Dryden (trans.), Life of Marcellus.
They think that differential equations are not reality. Hearing some colleagues speak, it’s as though theoretical physics was just playing house with plastic building blocks. This absurd idea has gained currency, and now people seem to feel that theoretical physicists are little more than dreamers locked away ivory towers. They think our games, our little houses, bear no relation to their everyday worries, their interests, their problems, or their welfare. But I’m going to tell you something, and I want you to take it as a ground rule for this course. From now on I will be filling this board with equations. … And when I'm done, I want you to do the following: look at those numbers, all those little numbers and Greek letters on the board, and repeat to yourselves, “This is reality,” repeat it over and over.
Zig Zag, trans. Lisa Dillman (2008), 63.
Think of a single problem confronting the world today. Disease, poverty, global warming… If the problem is going to be solved, it is science that is going to solve it. Scientists tend to be unappreciated in the world at large, but you can hardly overstate the importance of the work they do. If anyone ever cures cancer, it will be a guy with a science degree. Or a woman with a science degree.
Quoted in Max Davidson, 'Bill Bryson: Have faith, science can solve our problems', Daily Telegraph (26 Sep 2010)
This [the fact that the pursuit of mathematics brings into harmonious action all the faculties of the human mind] accounts for the extraordinary longevity of all the greatest masters of the Analytic art, the Dii Majores of the mathematical Pantheon. Leibnitz lived to the age of 70; Euler to 76; Lagrange to 77; Laplace to 78; Gauss to 78; Plato, the supposed inventor of the conic sections, who made mathematics his study and delight, who called them the handles or aids to philosophy, the medicine of the soul, and is said never to have let a day go by without inventing some new theorems, lived to 82; Newton, the crown and glory of his race, to 85; Archimedes, the nearest akin, probably, to Newton in genius, was 75, and might have lived on to be 100, for aught we can guess to the contrary, when he was slain by the impatient and ill mannered sergeant, sent to bring him before the Roman general, in the full vigour of his faculties, and in the very act of working out a problem; Pythagoras, in whose school, I believe, the word mathematician (used, however, in a somewhat wider than its present sense) originated, the second founder of geometry, the inventor of the matchless theorem which goes by his name, the pre-cognizer of the undoubtedly mis-called Copernican theory, the discoverer of the regular solids and the musical canon who stands at the very apex of this pyramid of fame, (if we may credit the tradition) after spending 22 years studying in Egypt, and 12 in Babylon, opened school when 56 or 57 years old in Magna Græcia, married a young wife when past 60, and died, carrying on his work with energy unspent to the last, at the age of 99. The mathematician lives long and lives young; the wings of his soul do not early drop off, nor do its pores become clogged with the earthy particles blown from the dusty highways of vulgar life.
In Presidential Address to the British Association, Collected Mathematical Papers, Vol. 2 (1908), 658.
This conviction of the solvability of every mathematical problem is a powerful incentive to the worker. We hear within us the perpetual call: There is the problem. Seek its solution. You can find it by pure reason, for in mathematics there is no ignorabimus!
Ignorabimus as used here, means “we will not know” (which is slightly different from ignoramus meaning present ignorance, “we do not know”). In Lecture (1900), 'Mathematische Probleme' (Mathematical Problems), to the International Congress of Mathematicians, Paris. From the original German reprinted in David Hilbert: Gesammelte Abhandlungen (Collected Treatises, 1970), Vol. 3, 298, “Diese Überzeugung von der Lösbarkeit eines jeden mathematischer Problems ist uns ein kräftiger Ansporn während der Arbeit ; wir hören in uns den steten Zuruf: Da ist das Problem, suche die Lösung. Du kannst sie durch reines Denken finden; denn in der Mathematik gibt es kein Ignorabimus. English version as translated by Dr. Maby Winton Newson for Bulletin of the American Mathematical Society (1902), 8, 437-479. The address was first published in Göttinger Nachrichten is Nachrichten von der Königl. Gesellschaft der Wiss. zu Göttingen (1900), 253-297; and Archiv der Mathematik und Physik (1901), 3, No. 1, 44-63.
This integrative action in virtue of which the nervous system unifies from separate organs an animal possessing solidarity, an individual, is the problem before us.
The Integrative Action of the Nervous System (1906), 2.
This is one of the greatest advantages of modern geometry over the ancient, to be able, through the consideration of positive and negative quantities, to include in a single enunciation the several cases which the same theorem may present by a change in the relative position of the different parts of a figure. Thus in our day the nine principal problems and the numerous particular cases, which form the object of eighty-three theorems in the two books De sectione determinata of Appolonius constitute only one problem which is resolved by a single equation.
In Histoire de la Géométrie, chap. 1, sect. 35.
This is the element that distinguishes applied science from basic. Surprise is what makes the difference. When you are organized to apply knowledge, set up targets, produce a usable product, you require a high degree of certainty from the outset. All the facts on which you base protocols must be reasonably hard facts with unambiguous meaning. The challenge is to plan the work and organize the workers so that it will come out precisely as predicted. For this, you need centralized authority, elaborately detailed time schedules, and some sort of reward system based on speed and perfection. But most of all you need the intelligible basic facts to begin with, and these must come from basic research. There is no other source. In basic research, everything is just the opposite. What you need at the outset is a high degree of uncertainty; otherwise it isn’t likely to be an important problem. You start with an incomplete roster of facts, characterized by their ambiguity; often the problem consists of discovering the connections between unrelated pieces of information. You must plan experiments on the basis of probability, even bare possibility, rather than certainty.
The Planning of Science, The Lives of a Cell: Notes of a Biology Watcher, (1974) .
This paper gives wrong solutions to trivial problems. The basic error, however, is not new.
In Mathematical Reviews 12, 561. As quoted and cited in P.R. Halmos, I Want to be a Mathematician: An Automathography (2013), 120
This statistical regularity in moral affairs fully establishes their being under the presidency of law. Man is seen to be an enigma only as an individual: in the mass he is a mathematical problem.
Vestiges of the Natural History of Creation (1844), 331.
This was what the universities were turning out nowadays. The science-is-a-sacred-cow boys. People who believe you could pour mankind into a test-tube and titrate it, and come up with all the answers to the problems of the human race.
The Day the World Ended (1953). Quoted in Gary Westfahl, Science Fiction Quotations (2005), 320-321.
This whole period was a golden age of immunology, an age abounding in important synthetic discoveries all over the world, a time we all thought it was good to be alive. We, who were working on these problems, all knew each other and met as often as we could to exchange ideas and hot news from the laboratory.
In Memoir of a Thinking Radish: An Autobiography (1986), 135.
Those of us who were familiar with the state of inorganic chemistry in universities twenty to thirty years ago will recall that at that time it was widely regarded as a dull and uninteresting part of the undergraduate course. Usually, it was taught almost entirely in the early years of the course and then chiefly as a collection of largely unconnected facts. On the whole, students concluded that, apart from some relationships dependent upon the Periodic table, there was no system in inorganic chemistry comparable with that to be found in organic chemistry, and none of the rigour and logic which characterised physical chemistry. It was widely believed that the opportunities for research in inorganic chemistry were few, and that in any case the problems were dull and uninspiring; as a result, relatively few people specialized in the subject... So long as inorganic chemistry is regarded as, in years gone by, as consisting simply of the preparations and analysis of elements and compounds, its lack of appeal is only to be expected. The stage is now past and for the purpose of our discussion we shall define inorganic chemistry today as the integrated study of the formation, composition, structure and reactions of the chemical elements and compounds, excepting most of those of carbon.
Inaugural Lecture delivered at University College, London (1 Mar 1956). In The Renaissance of Inorganic Chemistry (1956), 4-5.
Those that can readily master the difficulties of Mathematics find a considerable charm in the study, sometimes amounting to fascination. This is far from universal; but the subject contains elements of strong interest of a kind that constitutes the pleasures of knowledge. The marvellous devices for solving problems elate the mind with the feeling of intellectual power; and the innumerable constructions of the science leave us lost in wonder.
In Education as a Science (1879), 153.
Those who nod sagely and quote the tragedy of the commons in relation to environmental problems from pollution of the atmosphere to poaching of national parks tend to forget that Garrett Hardin revised his conclusions many times…. He recognized, most importantly, that anarchy did not prevail on the common pastures of medieval England in the way he had described…. “A managed commons, though it may have other defects, is not automatically subject to the tragic fate of the unmanaged commons,” wrote Hardin…. At sea, where a common exists in most waters… None of Hardin’s requirements for a successfully managed common is fulfilled by high-seas fishery regimes.
In The End of the Line: How Overfishing is Changing the World and what We Eat (2004), 153-155.
Though science can cause problems, it is not by ignorance that we will solve them.
…...
Though we must not without further consideration condemn a body of reasoning merely because it is easy, nevertheless we must not allow ourselves to be lured on merely by easiness; and we should take care that every problem which we choose for attack, whether it be easy or difficult, shall have a useful purpose, that it shall contribute in some measure to the up-building of the great edifice.
From 'On Some Recent Tendencies in Geometric Investigation', Rivista di Matematica (1891), 63. In Bulletin American Mathematical Society (1904), 465.
Through the discovery of Buchner, Biology was relieved of another fragment of mysticism. The splitting up of sugar into CO2 and alcohol is no more the effect of a 'vital principle' than the splitting up of cane sugar by invertase. The history of this problem is instructive, as it warns us against considering problems as beyond our reach because they have not yet found their solution.
The Dynamics of Living Matter (1906), 22.
Thus, we have three principles for increasing adequacy of data: if you must work with a single object, look for imperfections that record historical descent; if several objects are available, try to render them as stages of a single historical process; if processes can be directly observed, sum up their effects through time. One may discuss these principles directly or recognize the ‘little problems’ that Darwin used to exemplify them: orchids, coral reefs, and worms–the middle book, the first, and the last.
…...
Time is a fixed income and, as with any income, the real problem facing most of us is how to live successfully within our daily allotment.
…...
To my knowledge there are no written accounts of Fermi’s contributions to the [first atomic bomb] testing problems, nor would it be easy to reconstruct them in detail. This, however, was one of those occasions in which Fermi’s dominion over all physics, one of his most startling characteristics, came into its own. The problems involved in the Trinity test ranged from hydrodynamics to nuclear physics, from optics to thermodynamics, from geophysics to nuclear chemistry. Often they were closely interrelated, and to solve one’it was necessary to understand all the others. Even though the purpose was grim and terrifying, it was one of the greatest physics experiments of all time. Fermi completely immersed himself in the task. At the time of the test he was one of the very few persons (or perhaps the only one) who understood all the technical ramifications.
In Enrico Fermi: Physicist (1970), 145
To say that mind is a product or function of protoplasm, or of its molecular changes, is to use words to which we can attach no clear conception. You cannot have, in the whole, what does not exist in any of the parts; and those who argue thus should put forth a definite conception of matter, with clearly enunciated properties, and show, that the necessary result of a certain complex arrangement of the elements or atoms of that matter, will be the production of self-consciousness. There is no escape from this dilemma—either all matter is conscious, or consciousness is something distinct from matter, and in the latter case, its presence in material forms is a proof of the existence of conscious beings, outside of, and independent of, what we term matter. The foregoing considerations lead us to the very important conclusion, that matter is essentially force, and nothing but force; that matter, as popularly understood, does not exist, and is, in fact, philosophically inconceivable. When we touch matter, we only really experience sensations of resistance, implying repulsive force; and no other sense can give us such apparently solid proofs of the reality of matter, as touch does. This conclusion, if kept constantly present in the mind, will be found to have a most important bearing on almost every high scientific and philosophical problem, and especially on such as relate to our own conscious existence.
In 'The Limits of Natural Selection as Applied to Man', last chapter of Contributions to the Theory of Natural Selection (1870), 365-366.
To solve a problem is to create new problems, new knowledge immediately reveals new areas of ignorance, and the need for new experiments. At least, in the field of fast reactions, the experiments do not take very long to perform.
From Nobel Lecture (11 Dec 1967), 'Flash Photolysis and Some of its Applications.' In Nobel Lectures: Chemistry 1963-1970 (1972), 261.
To solve a problem means to reduce it to something simpler than itself.
In 'On Groups', Prelude to Mathematics (1955), 203.
To this day, we see all around us the Promethean drive to omnipotence through technology and to omniscience through science. The effecting of all things possible and the knowledge of all causes are the respective primary imperatives of technology and of science. But the motivating imperative of society continues to be the very different one of its physical and spiritual survival. It is now far less obvious than it was in Francis Bacon's world how to bring the three imperatives into harmony, and how to bring all three together to bear on problems where they superpose.
In 'Science, Technology and the Fourth Discontinuity' (1982). Reprinted in The Advancement of Science, and its Burdens (1986), 183.
Today when the public thinks of the products of science it is likely to think about environmental problems, an unproductive armament industry, careless or dishonest 'scientific' reports, Livermore cheers for 'nukes forever' and a huge amount of self-serving noise on every subject from global warming to 'the face of God'.
'Hard Times', Physics Today (Oct 1992), 45, 9.
Today's water institutions—the policies and laws, government agencies and planning and engineering practices that shape patterns of water use—are steeped in a supply-side management philosophy no longer appropriate to solving today's water problems.
From a study Postel wrote for Worldwatch Institute, quoted in New York Times (22 Sep 1985), 19.
Train yourselves. Don’t wait to be fed knowledge out of a book. Get out and seek it. Make explorations. Do your own research work. Train your hands and your mind. Become curious. Invent your own problems and solve them. You can see things going on all about you. Inquire into them. Seek out answers to your own questions. There are many phenomena going on in nature the explanation of which cannot be found in books. Find out why these phenomena take place. Information a boy gets by himself is enormously more valuable than that which is taught to him in school.
In 'Dr. Irving Langmuir', Boys' Life (Jul 1941), 12.
True rigor is productive, being distinguished in this from another rigor which is purely formal and tiresome, casting a shadow over the problems it touches.
From address to the section of Algebra and Analysis, International Congress of Arts and Sciences, St. Louis (22 Sep 1904), 'On the Development of Mathematical Analysis and its Relation to Certain Other Sciences,' as translated by M.W. Haskell in Bulletin of the American Mathematical Society (May 1905), 11, 417.
Truth and falsity, indeed understanding, is not necessarily something purely intellectual, remote from feelings and attitudes. ... It is in the total conduct of men rather than in their statements that truth or falsehood lives, more in what a man does, in his real reaction to other men and to things, in his will to do them justice, to live at one with them. Here lies the inner connection between truth and justice. In the realm of behavior and action, the problem recurs as to the difference between piece and part.
From 'On Truth', collected in Mary Henle (ed.), Documents of Gestalt Psychology (1961), 28.
Turbulence is the most important unsolved problem of classical physics.
In The Feynman Lectures on Physics (1964).
Two extreme views have always been held as to the use of mathematics. To some, mathematics is only measuring and calculating instruments, and their interest ceases as soon as discussions arise which cannot benefit those who use the instruments for the purposes of application in mechanics, astronomy, physics, statistics, and other sciences. At the other extreme we have those who are animated exclusively by the love of pure science. To them pure mathematics, with the theory of numbers at the head, is the only real and genuine science, and the applications have only an interest in so far as they contain or suggest problems in pure mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
In History of European Thought in the Nineteenth Century (1903), 630.
Typical of the fundamental scientific problems whose solution should lead to important industrial consequences are, for example, the release of atomic energy, which experiment has shown to exist in quantities millions of times greater than is liberated by combustion.
An early speculation on using the amount of energy that could be released from uranium atoms. In a letter to Henry Ford (18 May 1931). He recorded earlier thoughts on the subject in his Research Notebook, entry for 23 Jul 1930, in Arthur H. Compton Notebooks, Washington University, St. Louis, and AIP. Cited by Stanley Coben, in 'The Scientific Establishment and the Transmission of Quantum Mechanics to the United States, 1919-32', The American Historical Review (Apr 1971), 76, No. 2, 466.
Very old and wide-spread is the opinion that forests have an important impact on rainfall. ... If forests enhance the amount and frequency of precipitation simply by being there, deforestation as part of agricultural expansion everywhere, must necessarily result in less rainfall and more frequent droughts. This view is most poignantly expressed by the saying: Man walks the earth and desert follows his steps! ... It is not surprising that under such circumstances the issue of a link between forests and climate has ... been addressed by governments. Lately, the Italian government has been paying special attention to reforestation in Italy and its expected improvement of the climate. ... It must be prevented that periods of heavy rainfall alternate with droughts. ...In the Unites States deforestation plays an important role as well and is seen as the cause for a reduction in rainfall. ... committee chairman of the American Association for Advancement of Science demands decisive steps to extend woodland in order to counteract the increasing drought. ... some serious concerns. In 1873, in Vienna, the congress for agriculture and forestry discussed the problem in detail; and when the Prussian house of representatives ordered a special commission to examine a proposed law pertaining to the preservation and implementation of forests for safeguarding, it pointed out that the steady decrease in the water levels of Prussian rivers was one of the most serious consequences of deforestation only to be rectified by reforestation programs. It is worth mentioning that ... the same concerns were raised in Russia as well and governmental circles reconsidered the issue of deforestation.
as quoted in Eduard Brückner - The Sources and Consequences of Climate Change and Climate Variability in Historical Times editted by N. Stehr and H. von Storch (2000)
Von Helmholtz, the great German physicist said that after previous investigation of a problem “in all directions … happy ideas came unexpectedly without effort like an inspiration.” He found that ideas did not come to him when his mind was fatigued or when at the working table, but often in the morning after a night’s rest or during the slow ascent of wooded hills on a sunny day.
In The Art of Scientific Investigation (1950), 69.
Watson and I had been often discussing the problem, the ways you could go wrong solving problems of this sort, the techniques you have to use, and in particular, such rather curious things as you mustn’t pay too much attention to the all the experimental evidence, some of it may be wrong, for example.
From Transcript of BBC TV program, The Prizewinners (1962).
We academic scientists move within a certain sphere, we can go on being useless up to a point, in the confidence that sooner or later some use will be found for our studies. The mathematician, of course, prides himself on being totally useless, but usually turns out to be the most useful of the lot. He finds the solution but he is not interested in what the problem is: sooner or later, someone will find the problem to which his solution is the answer.
'Concluding Remarks', Proceedings of the Royal Society of London, Series A, A Discussion of New Materials, 1964, 282, 152-3.
We are at the very beginning of time for the human race. It is not unreasonable that we grapple with problems. But there are tens of thousands of years in the future. Our responsibility is to do what we can, learn what we can, improve the solutions, and pass them on.
…...
We are concerned to understand the motivation for the development of pure mathematics, and it will not do simply to point to aesthetic qualities in the subject and leave it at that. It must be remembered that there is far more excitement to be had from creating something than from appreciating it after it has been created. Let there be no mistake about it, the fact that the mathematician is bound down by the rules of logic can no more prevent him from being creative than the properties of paint can prevent the artist. … We must remember that the mathematician not only finds the solutions to his problems, he creates the problems themselves.
In A Signpost to Mathematics (1951), 19. As quoted and cited in William L. Schaaf, 'Memorabilia Mathematica', The Mathematics Teacher (Mar 1957), 50, No. 3, 230. Note that this paper incorrectly attributes “A.H. Head”.
We are fishing out the top of the food chain, and it’s pretty crucial because about 200 million people depend on fish and fishing for their livelihood, and about a billion people, mostly in poorer countries, depend on fish for their protein. So this is a big problem. Good news is, it’s fixable.
From transcript of PBS TV interview by Tavis Smiley (28 Mar 2011).
We are going to have full success for the reason that we have attacked the problem in an entirely different way than did those who have failed.
Referring to his own typesetting machine development. From short Speech at the Chamberlain Hotel, Washington, D.C. (Feb 1885), concluding the exhibition of his own Linotype invention. As given in Carl Schlesinger (ed.), 'Mr. Mergenthaler’s Speech', The Biography of Ottmar Merganthaler: Inventor of the Linotype (1989), 19.
We are Marxists, and Marxism teaches that in our approach to a problem we should start from objective facts, not from abstract definitions, and that we should derive our guiding principles, policies, and measures from an analysis of these facts.
As quoted in William Theodore De Bary, Sources of Chinese Tradition (1960), 929.
We call the one side [of humanity] religion, and we call the other science. Religion is always right. ... Science is always wrong; it is the very artifice of men. Science can never solve one problem without raising ten more problems.
Speech at the Einstein Dinner, Savoy Hotel, London (28 Oct 1930). Reproduced in George Bernard Shaw and Warren Sylvester Smith (ed.), The Religious Speeches of George Bernard Shaw (1963), 83.
We called the new [fourth] quark the “charmed quark” because we were pleased, and fascinated by the symmetry it brought to the subnuclear world. “Charm” also means a “a magical device to avert evil,” and in 1970 it was realized that the old three quark theory ran into very serious problems. ... As if by magic the existence of the charmed quark would [solve those problems].
From asppearance in the BBC-TV program written by Nigel Calder, 'The Key to the Universe,' (27 Jan 1977). As cited in Arthur Lewis Caso, 'The Production of New Scientific Terms', American Speech (Summer 1980), 55, No. 2, 102.
We can’t solve problems by using the same kind of thinking we used when we created them.
…...
We do not listen with the best regard to the verses of a man who is only a poet, nor to his problems if he is only an algebraist; but if a man is at once acquainted with the geometric foundation of things and with their festal splendor, his poetry is exact and his arithmetic musical.
In 'Works and Days', Society and Solitude (1883), Chap. 7, 171.
We feel that even if all possible scientific questions be answered, the problems of life have still not been touched at all. Of course there is then no question left, and just this is the answer.
From the German, “Wir fühlen, dass selbst, wenn alle möglichen wissenschaftlichen Fragen beantwortet sind, unsere Lebensprobleme noch gar nicht berührt sind. Freilich bleibt dann eben keine Frage mehr; und eben dies ist die Antwort,” in Logisch-Philosophische Abhandlung (1921). German text with English translation in Tractatus Logico-Philosophicus (1922), 186-187.
We have elves here, and they help me. … While I’m digging in the tunnel, the elves will often come to me with solutions to my problem.
Revealing the secrets of his success, in a legend told by colleague, John Rollwagen, about Cray showing his house to a visiting French scientist, and a tunnel that Cray was building under it. Cray explained that when he reached an impasse in his computer design, he would retire to the tunnel to dig. As quoted in Toby Howard, 'Seymour Cray: An Appreciation', Computer World (Feb 1997).
We have gone a long way towards solving a problem when we are able to formulate it.
In Le Phénomène Humain (1955) as translated by Bernard Wall in 'The Expansion of Life',The Phenomenon of Man (1959, 2008), 115.
We live in a capitalist economy, and I have no particular objection to honorable self-interest. We cannot hope to make the needed, drastic improvement in primary and secondary education without a dramatic restructuring of salaries. In my opinion, you cannot pay a good teacher enough money to recompense the value of talent applied to the education of young children. I teach an hour or two a day to tolerably well-behaved near-adults–and I come home exhausted. By what possible argument are my services worth more in salary than those of a secondary-school teacher with six classes a day, little prestige, less support, massive problems of discipline, and a fundamental role in shaping minds. (In comparison, I only tinker with intellects already largely formed.)
…...
We may, I think, draw a yet higher and deeper teaching from the phenomena of degeneration. We seem to learn from it the absolute necessity of labour and effort, of struggle and difficulty, of discomfort and pain, as the condition of all progress, whether physical or mental, and that the lower the organism the more need there is of these ever-present stimuli, not only to effect progress, but to avoid retrogression. And if so, does not this afford us the nearest attainable solution of the great problem of the origin of evil? What we call evil is the essential condition of progress in the lower stages of the development of conscious organisms, and will only cease when the mind has become so thoroughly healthy, so well balanced, and so highly organised, that the happiness derived from mental activity, moral harmony, and the social affections, will itself be a sufficient stimulus to higher progress and to the attainment of a more perfect life.
In 'Two Darwinian Essays', Nature (1880), 22, 142.
We need people who can see straight ahead and deep into the problems. Those are the experts. But we also need peripheral vision and experts are generally not very good at providing peripheral vision.
…...
We often think, naïvely, that missing data are the primary impediments to intellectual progress–just find the right facts and all problems will dissipate. But barriers are often deeper and more abstract in thought. We must have access to the right metaphor, not only to the requisite information. Revolutionary thinkers are not, primarily, gatherers of fact s, but weavers of new intellectual structures.
…...
We took on things which people might think would take a year or two. They weren't particularly hard. What was hard was believing they weren't hard.
[Recalling high-pressure, short-deadline problem solving leading up to planned release date of Polaroid instant color film.]
[Recalling high-pressure, short-deadline problem solving leading up to planned release date of Polaroid instant color film.]
Quoted in Alix Kerr, 'What It Took: Intuition, Goo,' Life (25 Jan 1963), 54, No. 4, 86.
We wanted to fly. We also had such big egos that we felt that we could fly the crates they shipped these things in. We honestly felt that, with things that were wrong, we always had a mental workaround on them.
Rejecting concern about Apollo spacecraft safety. From interview with Ron Stone (24 May 1999) for NASA Johnson Space Center Oral History Project.
We woke periodically throughout the night to peel off leeches. In the light of the head torch, the ground was a sea of leeches - black, slithering, standing up on one end to sniff the air and heading inexorably our way to feed. Our exposed faces were the main problem, with leeches feeding off our cheeks and becoming entangled in our hair. I developed a fear of finding one feeding in my ear, and that it would become too large to slither out, causing permanent damage.
Kinabalu Escape: The Soldiers’ Story
What can I say of the perpetual motion machine that is my husband? What makes Francis run? It is a mysterious and propelling force which, injected into all mankind, would solve all the problems that plague this day and age.
Describing her husband, opthalmologist Francis Heed Adler.
Describing her husband, opthalmologist Francis Heed Adler.
Investigative Ophthalmology (Feb 1968), 7 No. 1, 4.
What good your beautiful proof on [the transcendence of] π? Why investigate such problems, given that irrational numbers do not even exist?
What I then got hold of, something frightful and dangerous, a problem with horns but not necessarily a bull, in any case a new problem—today I should say that it was the problem of science itself, science considered for the first time as problematic, as questionable. But the book in which my youthful courage and suspicion found an outlet—what an impossible book had to result from a task so uncongenial to youth! Constructed from a lot of immature, overgreen personal experiences, all of them close to the limits of communication, presented in the context of art—for the problem of science cannot be recognized in the context of science—a book perhaps for artists who also have an analytic and retrospective penchant (in other words, an exceptional type of artist for whom one might have to look far and wide and really would not care to look) …
In The Birth of Tragedy (1872). Collected in Friedrich Nietzsche and Walter Kaufmann (trans.), The Birth of Tragedy and The Case of Wagner (1967), 18.
What is important is the gradual development of a theory, based on a careful analysis of the ... facts. ... Its first applications are necessarily to elementary problems where the result has never been in doubt and no theory is actually required. At this early stage the application serves to corroborate the theory. The next stage develops when the theory is applied to somewhat more complicated situations in which it may already lead to a certain extent beyond the obvious and familiar. Here theory and application corroborate each other mutually. Beyond lies the field of real success: genuine prediction by theory. It is well known that all mathematized sciences have gone through these successive stages of evolution.
'Formulation of the Economic Problem' in Theory of Games and Economic Behavior (1964), 8. Reprinted in John Von Neumann, F. Bródy (ed.) and Tibor Vámos (ed.), The Neumann Compendium (2000), 416.
What makes planets go around the sun? At the time of Kepler, some people answered this problem by saying that there were angels behind them beating their wings and pushing the planets around an orbit. As you will see, the answer is not very far from the truth. The only difference is that the angels sit in a different direction and their wings push inward.
In The Character of Physical Law (1965), 18.
What merely annoys and discourages a person not accustomed to thinking … is a stimulus and guide to the trained enquirer. … It either brings to light a new problem or helps to define and clarify the problem.
In How We Think (1933), 114.
What renders a problem definite, and what leaves it indefinite, may best be understood from mathematics. The very important idea of solving a problem within limits of error is an element of rational culture, coming from the same source. The art of totalizing fluctuations by curves is capable of being carried, in conception, far beyond the mathematical domain, where it is first learnt. The distinction between laws and co-efficients applies in every department of causation. The theory of Probable Evidence is the mathematical contribution to Logic, and is of paramount importance.
In Education as a Science (1879), 151-152.
What we usually consider as impossible are simply engineering problems … there’s no law of physics preventing them.
As quoted in Alok Ajh, 'Science Weekly with Michio Kaku: Impossibility is Relative' (15 Jun 2009), on website of The Guardian.
Whatever terrain the environmental historian chooses to investigate, he has to address the age-old predicament of how humankind can feed itself without degrading the primal source of life. Today as ever, that problem is the fundamental challenge in human ecology, and meeting it will require knowing the earth well—knowing its history and knowing its limits.
In 'Transformations of the Earth: toward an Agroecological Perspective in History', Journal of American History (Mar 1990), 76, No. 4, 1106.
When a man of science speaks of his “data,” he knows very well in practice what he means. Certain experiments have been conducted, and have yielded certain observed results, which have been recorded. But when we try to define a “datum” theoretically, the task is not altogether easy. A datum, obviously, must be a fact known by perception. But it is very difficult to arrive at a fact in which there is no element of inference, and yet it would seem improper to call something a “datum” if it involved inferences as well as observation. This constitutes a problem. …
In The Analysis of Matter (1954).
When a problem begins to clear, so that the conclusions are evident and so that all the paths to the end are clear, then I lose interest in it and want to try something else.
As quoted in Harold Walker, 'Academy of Sciences Opens to a Woman: Dr. Florence Sabin Describes Her Successful Blood Investigations as Romantic Adventure', New York Times (17 May 1925), Sunday Magazine, 6. The reporter noted that Sabin described her work with words such as “romantic”, “an adventure” and often used the word “fun” to explain its fascination to her. Also, “She sees it in prospect, rather than in retrospect.”
When a thing is said to be not worth refuting you may be sure that either it is flagrantly stupid—in which case all comment is superfluous—or it is something formidable, the very crux of the problem.
In Tragic Sense of Life (1913), translated by John Ernest Crawford Flitch (1954), 99.
When an inquiry becomes so convoluted, we must suspect that we are proceeding in the wrong way. We must return to go, change gears, and reformulate the problem, not pursue every new iota of information or nuance of argument jn the old style, hoping all the time that our elusive solution simply awaits a crucial item, yet undiscovered.
In The Flamingo’s Smile (1985).
When faced with a problem you do not understand, do any part of it you do understand and then look at it again.
In The Moon Is a Harsh Mistress (1996), 365. The paragraph containing this quote is also on this webpage to give it in context, beginning: “From somewhere, back in my youth…”.
When he [Wilhelm His] set a problem it was concisely stated; he outlined the general plan by which it was to be solved. All of the details were left to the pupil and it annoyed him to be consulted regarding them. He desired that the pupil should have full freedom to work out his own solution and aided him mainly through severe criticism.
As quoted, without citation, in Florence R. Sabin, Franklin Paine Mall: The Story of a Mind. (1934), 39.
When I am working on a problem, I never think about beauty … but when I have finished, if the solution is not beautiful, I know it is wrong.
Quoted in David J. Darling, The Universal Book of Mathematics (2004). 34.
When I started on this problem I surveyed the field and selected the best road, regardless of the roads which others have taken. I knew the direction in which others had attempted to solve the problem, and was careful not to fall into the same rut which had led every previous effort into failure and ruin.
From short Speech at the Chamberlain Hotel, Washington, D.C. (Feb 1885), concluding the exhibition of his own Linotype invention. As given in Carl Schlesinger (ed.), 'Mr. Mergenthaler’s Speech', The Biography of Ottmar Merganthaler: Inventor of the Linotype (1989), 20.
When I was an undergraduate, I went to the professor of geology and said, “Would you talk to us about the way that continents are drifting?” And he said, “The moment we can demonstrate that continents are moving by a millimetre, I will consider it, but until then it’s sheer moonshine, dear boy.” And within five years of me leaving Cambridge, it was confirmed, and all the problems disappeared—why Australian animals were different—that one thing changed our understanding and made sense of everything.
From 'Interview: Of Mind and Matter: David Attenborough Meets Richard Dawkins', The Guardian (11 Sep 2010).
When I was research head of General Motors and wanted a problem solved, I’d place a table outside the meeting room with a sign: “Leave slide rules here.” If I didn’t do that, I'd find someone reaching for his slide rule. Then he’d be on his feet saying, “Boss, you can’t do it.”
In Jacob Morton Braude, Speaker's Desk Book of Quips, Quotes, & Anecdotes (1966), 323.
When the difficulty of a problem lies only in finding out what follows from certain fixed premises, mathematical methods furnish invaluable wings for flying over intermediate obstructions.
From The Economic Theory of the Location of Railways (1887, 1914), viii.
When we had no computers, we had no programming problem either. When we had a few computers, we had a mild programming problem. Confronted with machines a million times as powerful, we are faced with a gigantic programming problem.
…...
When you are famous it is hard to work on small problems. This is what did [Claude Elwood] Shannon in. After information theory, what do you do for an encore? The great scientists often make this error. They fail to continue to plant the little acorns from which the mighty oak trees grow. They try to get the big thing right off. And that isn’t the way things go. So that is another reason why you find that when you get early recognition it seems to sterilize you.
'You and Your Research', Bell Communications Research Colloquium Seminar, 7 Mar 1986.
When... the biologist is confronted with the fact that in the organism the parts are so adapted to each other as to give rise to a harmonious whole; and that the organisms are endowed with structures and instincts calculated to prolong their life and perpetuate their race, doubts as to the adequacy of a purely physiochemical viewpoint in biology may arise. The difficulties besetting the biologist in this problem have been rather increased than diminished by the discovery of Mendelian heredity, according to which each character is transmitted independently of any other character. Since the number of Mendelian characters in each organism is large, the possibility must be faced that the organism is merely a mosaic of independent hereditary characters. If this be the case the question arises: What moulds these independent characters into a harmonious whole? The vitalist settles this question by assuming the existence of a pre-established design for each organism and of a guiding 'force' or 'principle' which directs the working out of this design. Such assumptions remove the problem of accounting for the harmonious character of the organism from the field of physics or chemistry. The theory of natural selection invokes neither design nor purpose, but it is incomplete since it disregards the physiochemical constitution of living matter about which little was known until recently.
The Organism as a Whole: From a Physiochemical Viewpoint (1916), v-vi.
Whenever the essential nature of things is analysed by the intellect, it must seem absurd or paradoxical. This has always been recognized by the mystics, but has become a problem in science only very recently.
In The Tao of Physics (1975), 50.
Whenever there is a simple error that most laymen fall for, there is always a slightly more sophisticated version of the same problem that experts fall for.
As quoted in Brooks Jackson and Kathleen Hall Jamieson, unSpun: Finding Facts in a World of Disinformation (2007), 70-71.
Where should I start? Start from the statement of the problem. ... What can I do? Visualize the problem as a whole as clearly and as vividly as you can. ... What can I gain by doing so? You should understand the problem, familiarize yourself with it, impress its purpose on your mind.
How to Solve It: a New Aspect of Mathematical Method (1957), 33.
Whereas at the outset geometry is reported to have concerned herself with the measurement of muddy land, she now handles celestial as well as terrestrial problems: she has extended her domain to the furthest bounds of space.
In The Story of Euclid. (1902) 14-15.
Wherever there is the slightest possibility for the human mind to know, there is a legitimate problem of science.
In The Grammar of Science (1892), 25.
While knowledge can create problems, it is not through ignorance that we can solve them.
In Asimov's New Guide to Science (1984), 15.
While the method of the natural sciences is... analytic, the method of the social sciences is better described as compositive or synthetic. It is the so-called wholes, the groups of elements which are structurally connected, which we learn to single out from the totality of observed phenomena... Insofar as we analyze individual thought in the social sciences the purpose is not to explain that thought, but merely to distinguish the possible types of elements with which we shall have to reckon in the construction of different patterns of social relationships. It is a mistake... to believe that their aim is to explain conscious action ... The problems which they try to answer arise only insofar as the conscious action of many men produce undesigned results... If social phenomena showed no order except insofar as they were consciously designed, there would indeed be no room for theoretical sciences of society and there would be, as is often argued, only problems of psychology. It is only insofar as some sort of order arises as a result of individual action but without being designed by any individual that a problem is raised which demands a theoretical explanation... people dominated by the scientistic prejudice are often inclined to deny the existence of any such order... it can be shown briefly and without any technical apparatus how the independent actions of individuals will produce an order which is no part of their intentions... The way in which footpaths are formed in a wild broken country is such an instance. At first everyone will seek for himself what seems to him the best path. But the fact that such a path has been used once is likely to make it easier to traverse and therefore more likely to be used again; and thus gradually more and more clearly defined tracks arise and come to be used to the exclusion of other possible ways. Human movements through the region come to conform to a definite pattern which, although the result of deliberate decision of many people, has yet not be consciously designed by anyone.
…...
While we keep an open mind on this question of vitalism, or while we lean, as so many of us now do, or even cling with a great yearning, to the belief that something other than the physical forces animates the dust of which we are made, it is rather the business of the philosopher than of the biologist, or of the biologist only when he has served his humble and severe apprenticeship to philosophy, to deal with the ultimate problem. It is the plain bounden duty of the biologist to pursue his course unprejudiced by vitalistic hypotheses, along the road of observation and experiment, according to the accepted discipline of the natural and physical sciences. … It is an elementary scientific duty, it is a rule that Kant himself laid down, that we should explain, just as far as we possibly can, all that is capable of such explanation, in the light of the properties of matter and of the forms of energy with which we are already acquainted.
From Presidential Address to Zoological Section of the British Association for the Advancement of Science. As quoted in H.V. Neal, 'The Basis of Individuality in Organisms: A Defense of Vitalism', Science (21 Jul 1916), 44 N.S., No. 1125, 82.
Why does man behave like perfect idiot? This is the problem I wish to deal with.
The Crazy Ape (1970), 11.
Why had we come to the moon?
The thing presented itself to me as a perplexing problem. What is this spirit in man that urges him for ever to depart from happiness and security, to toil, to place himself in danger, to risk an even a reasonable certainty of death? It dawned upon me that there in the moon as a thing I ought always to have known, that man is not made to go about safe and comfortable and well fed and amused. ... against his interest, against his happiness, he is constantly being driven to do unreasonable things. Some force not himself impels him, and he must go.
The thing presented itself to me as a perplexing problem. What is this spirit in man that urges him for ever to depart from happiness and security, to toil, to place himself in danger, to risk an even a reasonable certainty of death? It dawned upon me that there in the moon as a thing I ought always to have known, that man is not made to go about safe and comfortable and well fed and amused. ... against his interest, against his happiness, he is constantly being driven to do unreasonable things. Some force not himself impels him, and he must go.
The First Men in the Moon (1901)
Why is it so easy to acquire the solutions of past problems and so difficult to solve current ones
(Attributed ??) This quote is often seen, but without a citation, even on the official Marshall McLuhan website. If you known a primary print source, please contact Webmaster.
Why we love science. It’s more than a school subject, or the periodic table, or the properties of waves. It is an approach to the world, a critical way to understand and explore and engage with the world, and then have the capacity to change that world, and to share this accumulated knowledge. It’s a mindset that says we that can use reason and logic and honest inquiry to reach new conclusions and solve big problems.
From remarks at the fifth White House Science Fair, in Press Release (23 Mar 2015).
Will it be possible to solve these problems? It is certain that nobody has thus far observed the transformation of dead into living matter, and for this reason we cannot form a definite plan for the solution of this problem of transformation. But we see that plants and animals during their growth continually transform dead into living matter, and that the chemical processes in living matter do not differ in principle from those in dead matter. There is, therefore, no reason to predict that abiogenesis is impossible, and I believe that it can only help science if the younger investigators realize that experimental abiogenesis is the goal of biology.
The Dynamics of Living Matter (1906), 223.
Willis Rodney Whitney ... once compared scientific research to a bridge being constructed by a builder who was fascinated by the construction problems involved. Basic research, he suggested, is such a bridge built wherever it strikes the builder's fancy—wherever the construction problems seem to him to be most challenging. Applied research, on the other hand, is a
bridge built where people are waiting to get across the river. The challenge to the builder's ingenuity and skill, Whitney pointed out, can be as great in one case as the other.
'Willis Rodney Whitney', National Academy of Sciences, Biographical Memoirs (1960), 351.
With reference to … dyspepsia, it is saddening to see the perpetuation of the term “functional” as shorthand for “I don’t know the nature of the problem.”
In Letters, British Medical Journal (9 Feb 2002), 324, No. 7333, 364.
With terminal illness, your fate is sealed. Morally, we're more comfortable with a situation where you don't cause death, but you hasten it. We think that's a bright line.
Comparing the U.S. with Switzerland, where assisted suicide is legal for patients suffering 'intolerable health problems.'
Comparing the U.S. with Switzerland, where assisted suicide is legal for patients suffering 'intolerable health problems.'
Quoted in Amanda Ripley, 'True Freedom', Time magazine (20 Apr 2003).
Without a commitment to science and rationality in its proper domain, there can be no solution to the problems that engulf us. Still, the Yahoos never rest.
Ever Since Darwin (1980),146.
Without consciousness the mind-body problem would be much less interesting. With consciousness it seems hopeless.
Moral Questions (1979), 166.
You can't really discover the most interesting conflicts and problems in a subject until you've tried to write about them. At that point, one discovers discontinuities in the data, perhaps, or in one's own thinking; then the act of writing forces you to work harder to resolve these contradictions.
From Robert S. Grumet, 'An Interview with Anthony F. C. Wallace', Ethnohistory (Winter 1998), 45, No. 1, 109.
You propound a complicated arithmetical problem: say cubing a number containing four digits. Give me a slate and half an hour’s time, and I can produce a wrong answer.
Cashel Byron's Profession (1886, 1901), xxiii.

![John Houghton quote: [O]ur long-term security is threatened by a problem at least as dangerous as chemical, nuclear or biologica](https://todayinsci.com/H/Houghton_John/HoughtonJohn-GlobalWarming500x250px.jpg)
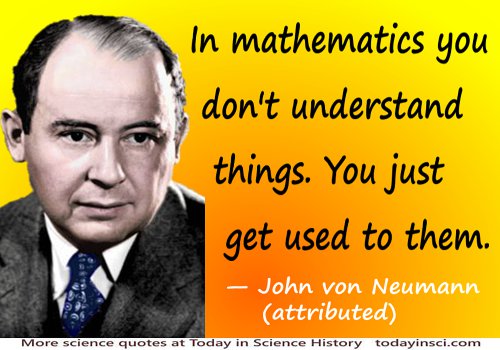





![Luis W. Alvarez quote: Dad [Walter C. Alvarez] … advised me to sit every few months in my reading chair for an entire evening, c](https://todayinsci.com/A/Alvarez_Luis/AlvarezLuis-Problems500x250px.jpg)
























 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


