... he could have gone around among his students with his penis hanging out, and everyone would have been charmed by his eccentricity.

Neumann, to a physicist seeking help with a difficult problem: Simple. This can be solved by using the method of characteristics.
Physicist: I'm afraid I don’t understand the method of characteristics.
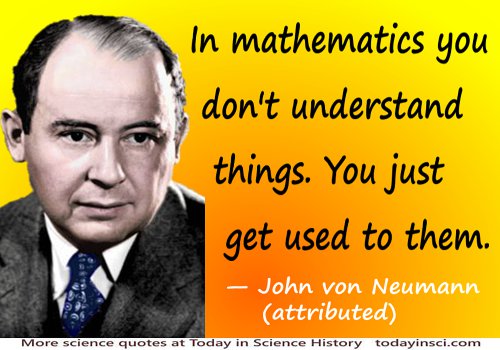
Neumann: In mathematics you don't understand things. You just get used to them.

~~[No source found]~~ There’s no sense in being precise when you don't even know what you're talking about.

A large part of mathematics which becomes useful developed with absolutely no desire to be useful, and in a situation where nobody could possibly know in what area it would become useful; and there were no general indications that it ever would be so.

Anybody who looks at living organisms knows perfectly well that they can produce other organisms like themselves. This is their normal function, they wouldn’t exist if they didn’t do this, and it’s not plausible that this is the reason why they abound in the world. In other words, living organisms are very complicated aggregations of elementary parts, and by any reasonable theory of probability or thermodynamics highly improbable. That they should occur in the world at all is a miracle of the first magnitude; the only thing which removes, or mitigates, this miracle is that they reproduce themselves. Therefore, if by any peculiar accident there should ever be one of them, from there on the rules of probability do not apply, and there will be many of them, at least if the milieu is reasonable. But a reasonable milieu is already a thermodynamically much less improbable thing. So, the operations of probability somehow leave a loophole at this point, and it is by the process of self-reproduction that they are pierced.

Anyone who considers arithmetical methods of producing random digits is, of course, in the state of sin. For, as has been pointed out several times, there is no such thing as a random number—there are only methods to produce random numbers, and a strict arithmetic procedure of course is not such a method.

By and large it is uniformly true in mathematics that there is a time lapse between a mathematical discovery and the moment when it is useful; and that this lapse of time can be anything from 30 to 100 years, in some cases even more.

Chess is not a game. Chess is a well-defined form of computation. You may not be able to work out the answers, but in theory there must be a solution, a right procedure in any position. Now real games are not like that at all. Real life is not like that. Real life consists of bluffing, of little tactics of deception, of asking yourself what is the other man going to think I mean to do.

Furthermore, it’s equally evident that what goes on is actually one degree better than self-reproduction, for organisms appear to have gotten more elaborate in the course of time. Today's organisms are phylogenetically descended from others which were vastly simpler than they are, so much simpler, in fact, that it’s inconceivable, how any kind of description of the latter, complex organism could have existed in the earlier one. It’s not easy to imagine in what sense a gene, which is probably a low order affair, can contain a description of the human being which will come from it. But in this case you can say that since the gene has its effect only within another human organism, it probably need not contain a complete description of what is to happen, but only a few cues for a few alternatives. However, this is not so in phylogenetic evolution. That starts from simple entities, surrounded by an unliving amorphous milieu, and produce, something more complicated. Evidently, these organisms have the ability to produce something more complicated than themselves.

I think that it is a relatively good approximation to truth—which is much too complicated to allow anything but approximations—that mathematical ideas originate in empirics.

If people do not believe that mathematics is simple, it is only because they do not realize how complicated life is.

In any conceivable method ever invented by man, an automaton which produces an object by copying a pattern, will go first from the pattern to a description to the object. It first abstracts what the thing is like, and then carries it out. It’s therefore simpler not to extract from a real object its definition, but to start from the definition.

It is exceptional that one should be able to acquire the understanding of a process without having previously acquired a deep familiarity with running it, with using it, before one has assimilated it in an instinctive and empirical way. Thus any discussion of the nature of intellectual effort in any field is difficult, unless it presupposes an easy, routine familiarity with that field. In mathematics this limitation becomes very severe.

It is just as foolish to complain that people are selfish and treacherous as it is to complain that the magnetic field does not increase unless the electric field has a curl. Both are laws of nature.

Kurt Gödel’s achievement in modern logic is singular and monumental—indeed it is more than a monument, it is a landmark which will remain visible far in space and time. … The subject of logic has certainly completely changed its nature and possibilities with Gödel's achievement.

The calculus was the first achievement of modern mathematics and it is difficult to overestimate its importance. I think it defines more unequivocally than anything else the inception of modern mathematics; and the system of mathematical analysis, which is its logical development, still constitutes the greatest technical advance in exact thinking.

The other line of argument, which leads to the opposite conclusion, arises from looking at artificial automata. Everyone knows that a machine tool is more complicated than the elements which can be made with it, and that, generally speaking, an automaton A, which can make an automaton B, must contain a complete description of B, and also rules on how to behave while effecting the synthesis. So, one gets a very strong impression that complication, or productive potentiality in an organization, is degenerative, that an organization which synthesizes something is necessarily more complicated, of a higher order, than the organization it synthesizes. This conclusion, arrived at by considering artificial automaton, is clearly opposite to our early conclusion, arrived at by considering living organisms.

The sciences do not try to explain, they hardly even try to interpret, they mainly make models. By a model is meant a mathematical construct which, with the addition of certain verbal interpretations, describes observed phenomena. The justification of such a mathematical construct is solely and precisely that it is expected to work—that is, correctly to describe phenomena from a reasonably wide area. Furthermore, it must satisfy certain esthetic criteria—that is, in relation to how much it describes, it must be rather simple.

This is true of all science. Successes were largely due to forgetting completely about what one ultimately wanted, or whether one wanted anything ultimately; in refusing to investigate things which profit, and in relying solely on guidance by criteria of intellectual elegance. … And I think it extremely instructive to watch the role of science in everyday life, and to note how in this area the principle of laissez faire has led to strange and wonderful results.

What is important is the gradual development of a theory, based on a careful analysis of the ... facts. ... Its first applications are necessarily to elementary problems where the result has never been in doubt and no theory is actually required. At this early stage the application serves to corroborate the theory. The next stage develops when the theory is applied to somewhat more complicated situations in which it may already lead to a certain extent beyond the obvious and familiar. Here theory and application corroborate each other mutually. Beyond lies the field of real success: genuine prediction by theory. It is well known that all mathematized sciences have gone through these successive stages of evolution.

When we talk mathematics, we may be discussing a secondary language built on the primary language of the nervous system.

You should call it entropy, for two reasons. In the first place your uncertainty function has been used in statistical mechanics under that name, so it already has a name. In the second place, and more important, no one really knows what entropy really is, so in a debate you will always have the advantage.

The spectacular thing about Johnny [von Neumann] was not his power as a mathematician, which was great, or his insight and his clarity, but his rapidity; he was very, very fast. And like the modern computer, which no longer bothers to retrieve the logarithm of 11 from its memory (but, instead, computes the logarithm of 11 each time it is needed), Johnny didn’t bother to remember things. He computed them. You asked him a question, and if he didn’t know the answer, he thought for three seconds and would produce and answer.

There was a seminar for advanced students in Zürich that I was teaching and von Neumann was in the class. I came to a certain theorem, and I said it is not proved and it may be difficult. Von Neumann didn’t say anything but after five minutes he raised his hand. When I called on him he went to the blackboard and proceeded to write down the proof. After that I was afraid of von Neumann.

Running overtime is the one unforgivable error a lecturer can make. After fifty minutes (one microcentury as von Neumann used to say) everybody's attention will turn elsewhere.

Von Neumann gave me an interesting idea: that you don’t have to be responsible for the world that you’re in. So I have developed a very powerful sense of social irresponsibility as a result of Von Neumann’s advice. It’s made me a very happy man ever since.

In the summer of 1937, … I told Banach about an expression Johnny [von Neumann] had once used in conversation with me in Princeton before stating some non-Jewish mathematician’s result, “Die Goim haben den folgendenSatzbewiesen” (The goys have proved the following theorem). Banach, who was pure goy, thought it was one of the funniest sayings he had ever heard. He was enchanted by its implication that if the goys could do it, Johnny and I ought to be able to do it better. Johnny did not invent this joke, but he liked it and we started using it.

I asked Fermi whether he was not impressed by the agreement between our calculated numbers and his measured numbers. He replied, “How many arbitrary parameters did you use for your calculations?" I thought for a moment about our cut-off procedures and said, “Four." He said, “I remember my friend Johnny von Neumann used to say, with four parameters I can fit an elephant, and with five I can make him wiggle his trunk.” With that, the conversation was over.

[Pure mathematics is] good to give chills in the spine to a certain number of people, me included. I don’t know what else it is good for, and I don’t care. But … like von Neumann said, one never knows whether someone is going to find another use for it.