Number Quotes (717 quotes)
... every chemical combination is wholly and solely dependent on two opposing forces, positive and negative electricity, and every chemical compound must be composed of two parts combined by the agency of their electrochemical reaction, since there is no third force. Hence it follows that every compound body, whatever the number of its constituents, can be divided into two parts, one of which is positively and the other negatively electrical.
Essai sur la théorie des proportions chemiques (1819), 98. Quoted by Henry M. Leicester in article on Bessel in Charles Coulston Gillespie (editor), Dictionary of Scientific Biography (1981), Vol. 2, 94.
… the definition of irrational numbers, on which geometric representations have often had a confusing influence. … I take in my definition a purely formal point of view, calling some given symbols numbers, so that the existence of these numbers is beyond doubt.
(1872). As quoted in Ernst Hairer and Gerhard Wanner, Analysis by Its History (2008), 177.
...That day in the account of creation, or those days that are numbers according to its recurrence, are beyond the experience and knowledge of us mortal earthbound men. And if we are able to make any effort towards an understanding of those days, we ought not to rush forward with an ill considered opinion, as if no other reasonable and plausible interpretation could be offered.
iv.44
…this discussion would be unprofitable if it did not lead us to appreciate the wisdom of our Creator, and the wondrous knowledge of the Author of the world, Who in the beginning created the world out of nothing, and set everything in number, measure and weight, and then, in time and the age of man, formulated a science which reveals fresh wonders the more we study it.
— Hrosvita
From her play Sapientia, as quoted and cited in Philip Davis with Reuben Hersh, in The Mathematical Experience (1981), 110-111. Davis and Hersh introduce the quote saying it comes “after a rather long and sophisticated discussion of certain facts in the theory of numbers” by the character Sapientia.
“Bitzer,” said Thomas Gradgrind. “Your definition of a horse.”
“Quadruped. Graminivorous. Forty teeth; namely, twenty-four grinders, four eye-teeth, and twelve incisive. Sheds coat in the Spring; in marshy countries, sheds hoofs, too. Hoofs hard, but requiring to be shod with iron. Age known by marks in mouth.” Thus (and much more) Bitzer.
“Now girl number twenty,” said Mr. Gradgrind. “You know what a horse is.”
“Quadruped. Graminivorous. Forty teeth; namely, twenty-four grinders, four eye-teeth, and twelve incisive. Sheds coat in the Spring; in marshy countries, sheds hoofs, too. Hoofs hard, but requiring to be shod with iron. Age known by marks in mouth.” Thus (and much more) Bitzer.
“Now girl number twenty,” said Mr. Gradgrind. “You know what a horse is.”
Spoken by fictional character Thomas Gringrind in his schoolroom with pupil Bitzer, Hard Times, published in Household Words (1 Apr 1854), Vol. 36, 3.
“Conservation” (the conservation law) means this … that there is a number, which you can calculate, at one moment—and as nature undergoes its multitude of changes, this number doesn't change. That is, if you calculate again, this quantity, it'll be the same as it was before. An example is the conservation of energy: there's a quantity that you can calculate according to a certain rule, and it comes out the same answer after, no matter what happens, happens.
'The Great Conservation Principles', The Messenger Series of Lectures, No. 3, Cornell University, 1964. From transcript of BBC programme (11 Dec 1964).
[1665-06-20] ...This day I informed myself that there died four or five at Westminster of the Plague, in one alley in several houses upon Sunday last - Bell Alley, over against the Palace gate. yet people do think that the number will be fewer in the town then it was last week. ...
Diary of Samuel Pepys (20 Jun 1665)
[1665-08-31] Up, and after putting several things in order to my removal to Woolwich, the plague having a great increase this week beyond all expectation, of almost 2000 - making the general Bill 7000, odd 100 and the plague above 6000 .... Thus this month ends, with great sadness upon the public through the greateness of the plague, everywhere through the Kingdom almost. Every day sadder and sadder news of its increase. In the City died this week 7496; and all of them, 6102 of the plague. But it is feared that the true number of the dead this week is near 10000 - partly from the poor that cannot be taken notice of through the greatness of the number, and partly from the Quakers and others that will not have any bell ring for them. As to myself, I am very well; only, in fear of the plague, and as much of an Ague, by being forced to go early and late to Woolwich, and my family to lie there continually.
Diary of Samuel Pepys (31 August 1665)
[After the flash of the atomic bomb test explosion] Fermi got up and dropped small pieces of paper … a simple experiment to measure the energy liberated by the explosion … [W]hen the front of the shock wave arrived (some seconds after the flash) the pieces of paper were displaced a few centimeters in the direction of propagation of the shock wave. From the distance of the source and from the displacement of the air due to the shock wave, he could calculate the energy of the explosion. This Fermi had done in advance having prepared himself a table of numbers, so that he could tell immediately the energy liberated from this crude but simple measurement. … It is also typical that his answer closely approximated that of the elaborate official measurements. The latter, however, were available only after several days’ study of the records, whereas Fermi had his within seconds.
In Enrico Fermi: Physicist (1970), 147-148.
[All phenomena] are equally susceptible of being calculated, and all that is necessary, to reduce the whole of nature to laws similar to those which Newton discovered with the aid of the calculus, is to have a sufficient number of observations and a mathematics that is complex enough.
Unpublished Manuscript. Quoted In Frank Edward Manuel and Fritzie Prigohzy Manuel, Utopian Thought in the Western World (1979, 2009), 493.
[At my secondary school] if you were very bright, you did classics; if you were pretty thick, you did woodwork; and if you were neither of those poles, you did science. The number of kids in my school who did science because they were excited by the notion of science was pretty small. You were allocated to those things, you weren’t asked. This was in the late 1930s/early 1940s … Science was seen as something more remote and less to do with everyday life.
From interview with Brian Cox and Robert Ince, in 'A Life Measured in Heartbeats', New Statesman (21 Dec 2012), 141, No. 5138, 32.
[Boswell]: Sir Alexander Dick tells me, that he remembers having a thousand people in a year to dine at his house: that is, reckoning each person as one, each time that he dined there.
[Johnson]: That, Sir, is about three a day.
[Boswell]: How your statement lessens the idea.
[Johnson]: That, Sir, is the good of counting. It brings every thing to a certainty, which before floated in the mind indefinitely.
[Johnson]: That, Sir, is about three a day.
[Boswell]: How your statement lessens the idea.
[Johnson]: That, Sir, is the good of counting. It brings every thing to a certainty, which before floated in the mind indefinitely.
Entry for Fri 18 Apr 1783. In George Birkbeck-Hill (ed.), Boswell's Life of Johnson (1934-50), Vol. 4, 204.
[Decimal currency is desirable because] by that means all calculations of interest, exchange, insurance, and the like are rendered much more simple and accurate, and, of course, more within the power of the great mass of people. Whenever such things require much labor, time, and reflection, the greater number who do not know, are made the dupes of the lesser number who do.
Letter to Congress (15 Jan 1782). 'Coinage Scheme Proposed by Robert Morris, Superintendent of Finance', from MS. letters and reports of the Superintendent of Finance, No, 137, Vol. 1, 289-300. Reprinted as Appendix, in Executive Documents, Senate of the U.S., Third Session of the Forty-Fifth Congress, 1878-79 (1879), 430.
[For imaginary numbers,] their success … has been what the French term a succès de
scandale.
In An Introduction to Mathematics (1911), 87. The French phrase (success from scandal), is applied to notoriety attributed to public controversy.
[In the Royal Society, there] has been, a constant Resolution, to reject all the amplifications, digressions, and swellings of style: to return back to the primitive purity, and shortness, when men deliver'd so many things, almost in an equal number of words. They have exacted from all their members, a close, naked, natural way of speaking; positive expressions; clear senses; a native easiness: bringing all things as near the Mathematical plainness, as they can: and preferring the language of Artizans, Countrymen, and Merchants, before that, of Wits, or Scholars.
The History of the Royal Society (1667), 113.
[It has been ascertained by statistical observation that in engineering enterprises one man is killed for every million francs that is spent on the works.] Supposing you have to build a bridge at an expense of one hundred million francs, you must be prepared for the death of one hundred men. In building the Eiffel Tower, which was a construction costing six million and a half, we only lost four men, thus remaining below the average. In the construction of the Forth Bridge, 55 men were lost in over 45,000,000 francs’ worth of work. That would appear to be a large number according to the general rule, but when the special risks are remembered, this number shows as a very small one.
As quoted in 'M. Eiffel and the Forth Bridge', The Tablet (15 Mar 1890), 75, 400. Similarly quoted in Robert Harborough Sherard, Twenty Years in Paris: Being Some Recollections of a Literary Life (1905), 169, which adds to the end “, and reflects very great credit on the engineers for the precautions which they took on behalf of their men.” Sherard gave the context that Eiffel was at the inauguration [4 Mar 1890] of the Forth Bridge, and gave this compliment when conversing there with the Prince of Wales.
[Louis Rendu, Bishop of Annecy] collects observations, makes experiments, and tries to obtain numerical results; always taking care, however, so to state his premises and qualify his conclusions that nobody shall be led to ascribe to his numbers a greater accuracy than they merit. It is impossible to read his work, and not feel that he was a man of essentially truthful mind and that science missed an ornament when he was appropriated by the Church.
In The Glaciers of the Alps (1860), 299.
[Mathematics is] the study of the measurement, properties, and relationships of quantities and sets, using numbers and symbols.
Definition of Mathematics in William morris (ed.), American Heritage Dictionary (2000).
[Mitscherlich Law of Isomerism] An equal number of atoms, combined in the same way produce the same crystal forms, and the same crystal form does not depend on the nature of the atoms, but only on their number and mode of combination.
Originally published in 'Om Förhållandet emellan chemiska sammansättningen och krystallformen hos Arseniksyrade och Phosphorsyrade Salter', (On the Relation between the Chemical Composition and Crystal Form of Salts of Arsenic and Phosphoric Acids), Kungliga Svenska vetenskapsakademiens handlingar (1821), 4. This alternate translation of the law appears is given by F. Szabadváry article on 'Eilhard Mitscherlich' in Charles Coulston Gillispie (ed.), Dictionary of Scientific Biography (1974), Vol. 9, 424; perhaps from J.R. Partington, A History of Chemistry, Vol. 4 (1964), 210.
[My brother] Neville always got things to add to his Meccano set for Christmas. Nowadays there are such wonderful things for kids, but in those days Meccano was a real novelty, because you could make something that really worked. There were little screws with square bits that went on the end, and tiny screwdrivers. They were beautiful things. Every lad had Meccano in those days. Your father bought you the number one, number two, number three—whichever you hadn’t got. One year Neville got one that made a steam engine, so that when the wheel went round it moved along. And my father would help him to build it—never mind breakfast, they just wanted to get down to building the Meccano.
In Thora Hird’s Book of Bygones (1998), 35-36.
[On why are numbers beautiful?] It’s like asking why is Beethoven’s Ninth Symphony beautiful. If you don’t see why, someone can’t tell you. I know numbers are beautiful. If they aren’t beautiful, nothing is.
As quoted in Paul Hoffman, The Man who Loves Only Numbers (1998), 44.
[Professor W.L. Bragg asserts that] In sodium chloride there appear to be no molecules represented by NaCl. The equality in number of sodium and chlorine atoms is arrived at by a chess-board pattern of these atoms; it is a result of geometry and not of a pairing-off of the atoms.
In Henry E. Armstrong, 'Poor Common Salt!', Nature (1927), 120, 478.
[Pure mathematics is] good to give chills in the spine to a certain number of people, me included. I don’t know what else it is good for, and I don’t care. But … like von Neumann said, one never knows whether someone is going to find another use for it.
In The Beauty of Doing Mathematics: Three Public Dialogues (1985), 49.
[Reading a cartoon story,] the boy favored reading over reality. Adults might have characterized him in any number of negative ways—as uninquisitive, uninvolved, apathetic about the world around him and his place in it. I’ve often wondered: Are many adults much different when they read the scriptures of their respective faiths?
In Jacques Cousteau and Susan Schiefelbein, The Human, the Orchid, and the Octopus: Exploring and Conserving Our Natural World (2007), 117.
[The famous attack of Sir William Hamilton on the tendency of mathematical studies] affords the most express evidence of those fatal lacunae in the circle of his knowledge, which unfitted him for taking a comprehensive or even an accurate view of the processes of the human mind in the establishment of truth. If there is any pre-requisite which all must see to be indispensable in one who attempts to give laws to the human intellect, it is a thorough acquaintance with the modes by which human intellect has proceeded, in the case where, by universal acknowledgment, grounded on subsequent direct verification, it has succeeded in ascertaining the greatest number of important and recondite truths. This requisite Sir W. Hamilton had not, in any tolerable degree, fulfilled. Even of pure mathematics he apparently knew little but the rudiments. Of mathematics as applied to investigating the laws of physical nature; of the mode in which the properties of number, extension, and figure, are made instrumental to the ascertainment of truths other than arithmetical or geometrical—it is too much to say that he had even a superficial knowledge: there is not a line in his works which shows him to have had any knowledge at all.
In Examination of Sir William Hamilton's Philosophy (1878), 607.
[The screw machine] was on the principle of the guage or sliding lathe now in every workshop throughout the world; the perfection of which consists in that most faithful agent gravity, making the joint, and that almighty perfect number three, which is in harmony itself. I was young when I learned that principle. I had never seen my grandmother putting a chip under a three-legged milking-stool; but she always had to put a chip under a four-legged table, to keep it steady. I cut screws of all dimensions by this machine, and did them perfectly. (1846)
Quoted in ASME International and Heritage Committee, Landmarks in Mechanical Engineering.
[The steamboat] will answer for sea voyages as well as for inland navigation, in particular for packets, where there may be a great number of passengers. He is also of opinion, that fuel for a short voyage would not exceed the weight of water for a long one, and it would produce a constant supply of fresh water. ... [T]he boat would make head against the most violent tempests, and thereby escape the danger of a lee shore; and that the same force may be applied to a pump to free a leaky ship of her water. ... [T]he good effects of the machine, is the almost omnipotent force by which it is actuated, and the very simple, easy, and natural way by which the screws or paddles are turned to answer the purpose of oars.
[This letter was written in 1785, before the first steamboat carried a man (Fitch) on 27 Aug 1787.]
[This letter was written in 1785, before the first steamboat carried a man (Fitch) on 27 Aug 1787.]
Letter to Benjamin Franklin (12 Oct 1785), in The Works of Benjamin Franklin (1882), Vol. 10, 232.
[The] structural theory is of extreme simplicity. It assumes that the molecule is held together by links between one atom and the next: that every kind of atom can form a definite small number of such links: that these can be single, double or triple: that the groups may take up any position possible by rotation round the line of a single but not round that of a double link: finally that with all the elements of the first short period [of the periodic table], and with many others as well, the angles between the valencies are approximately those formed by joining the centre of a regular tetrahedron to its angular points. No assumption whatever is made as to the mechanism of the linkage. Through the whole development of organic chemistry this theory has always proved capable of providing a different structure for every different compound that can be isolated. Among the hundreds of thousands of known substances, there are never more isomeric forms than the theory permits.
Presidential Address to the Chemical Society (16 Apr 1936), Journal of the Chemical Society (1936), 533.
[This] may prove to be the beginning of some embracing generalization, which will throw light, not only on radioactive processes, but on elements in general and the Periodic Law.... Chemical homogeneity is no longer a guarantee that any supposed element is not a mixture of several of different atomic weights, or that any atomic weight is not merely a mean number.
From Chemical Society's Annual Reports (1910), Vol. 7, 285. As quoted in Francis Aston in Lecture (1936) on 'Forty Years of Atomic Theory', collected in Needham and Pagel (eds.) in Background to Modern Science: Ten Lectures at Cambridge Arranged by the History of Science Committee, (1938), 100. Cited in Alfred Walter Stewart, Recent Advances in Physical and Inorganic Chemistry (1920), 198.
[To elucidate using models] the different combining powers in elementary atoms, I … select my illustrations from that most delightful of games, croquet. Let the croquet balls represent our atoms, and let us distinguish the atoms of different elements by different colours. The white balls are hydrogen, the green ones chlorine atoms; the atoms of fiery oxygen are red, those of nitrogen, blue; the carbon atoms, lastly, are naturally represented by black balls. But we have, in addition, exhibit the different combining powers of these atoms … by screwing into the balls a number of metallic arms (tubes and pins), which correspond respectively to the combining powers of the atoms represented … to join the balls … in imitation of the atomic edifices represented.
Paper presented at the Friday Discourse of the the Royal Institution (7 Apr 1865). 'On the Combining Power of Atoms', Proceedings of the Royal Institution (1865), 4, No. 42, 416.
[We] can easily distinguish what relates to Mathematics in any question from that which belongs to the other sciences. But as I considered the matter carefully it gradually came to light that all those matters only were referred to Mathematics in which order and measurements are investigated, and that it makes no difference whether it be in numbers, figures, stars, sounds or any other object that the question of measurement arises. I saw consequently that there must be some general science to explain that element as a whole which gives rise to problems about order and measurement, restricted as these are to no special subject matter. This, I perceived was called “Universal Mathematics,” not a far-fetched asignation, but one of long standing which has passed into current use, because in this science is contained everything on account of which the others are called parts of Mathematics.
Rules for the Direction of the Mind (written 1628). As translated by Elizabeth Sanderson Haldane and George Robert Thomson Ross in The Philosophical Works of Descartes (1911, 1931), 13.
Den förslags-mening: att olika element förenade med ett lika antal atomer af ett eller flere andra gemensamma element … och att likheten i krystallformen bestämmes helt och hållet af antalet af atomer, och icke af elementens.
[Mitscherlich Law of Isomerism] The same number of atoms combined in the same way produces the same crystalline form, and the same crystalline form is independent of the chemical nature of the atoms, and is determined only by their number and relative position.
[Mitscherlich Law of Isomerism] The same number of atoms combined in the same way produces the same crystalline form, and the same crystalline form is independent of the chemical nature of the atoms, and is determined only by their number and relative position.
Original Swedish from 'Om Förhållandet emellan chemiska sammansättningen och krystallformen hos Arseniksyrade och Phosphorsyrade Salter', Kungl. Svenska vetenskapsakademiens handlingar (1821), 4. In English as expressed later by James F.W. Johnston, 'Report on the Recent Progress and present State of Chemical Science', to Annual Meeting at Oxford (1832), collected in Report of the First and Second Meetings of the British Association for the Advancement of Science (1833), 422. A Google raw translation of the Swedish is: “The present proposal-sense: that various elements associated with an equal number of atoms of one or several other common elements … and that the similarity in: crystal shape is determined entirely by the number of atoms, and not by the elements.”
La théorie est l’hypothèse vérifiée, après qu’elle a été soumise au contrôle du raisonnement et de la critique expérimentale. La meilleure théorie est celle qui a été vérifiée par le plus grand nombre de faits. Mais une théorie, pour rester bonne, doit toujours se modifier avec les progrès de la science et demeurer constamment soumise à la vérification et à la critique des faits nouveaux qui apparaissent.
A theory is a verified hypothesis, after it has been submitted to the control of reason and experimental criticism. The soundest theory is one that has been verified by the greatest number of facts. But to remain valid, a theory must be continually altered to keep pace with the progress of science and must be constantly resubmitted to verification and criticism as new facts appear.
A theory is a verified hypothesis, after it has been submitted to the control of reason and experimental criticism. The soundest theory is one that has been verified by the greatest number of facts. But to remain valid, a theory must be continually altered to keep pace with the progress of science and must be constantly resubmitted to verification and criticism as new facts appear.
Original work in French, Introduction à l'Étude de la Médecine Expérimentale (1865), 385. English translation by Henry Copley Green in An Introduction to the Study of Experimental Medicine (1927, 1957), 220.
Numero pondere et mensura Deus omnia condidit.
God created everything by number, weight and measure.
God created everything by number, weight and measure.
On more than one occasion, Newton wrote these Latin words as his autograph, with his signature below. A photo of one example, dated “London 11 Sep 1722,” can be seen in I. Hargitta (ed.), Symmetry 2: Unifying Human Understanding (2014), 837. Reprinted from M. and B. Rozsondai, 'Symmetry Aspects of Bookbindings', Computers Math. Applic. (1989), 17, No. 4-6, 837. On this piece of paper, Newton dedicated these words to a Hungarian student, Ferenc Páriz Pápai Jr., whose album is now held by the Department of Manuscripts and Rare Books of the Library of the Hungarian Academy of Sciences (Shelf-number Tort. naplók, kis 8⁰ 6). The sentiment existed long before Newton used it. In the Bible, Wisdom of Solomon, 11:20, it appears as “Pondere, mensura, numero Deus omnia fecit,” (Vulgate) which the King James Version translates as “thou hast ordered all things in measure and number and weight.” Another Newton signed autograph with his Latin quote, dated 13 Jul 1716, sold (4 Apr 1991) for DM 9500 (about $31,000). See Dept of English, Temple University, The Scriblerian and the Kit-Cats (1991), 24-25, 230. A few years later, the sale (31 Mar 1998) of Newton's autograph cost the buyer $46,000. See Frank Ryan, Darwin's Blind Spot: Evolution Beyond Natural Selection (2002), 11-12. Other authors, including mathematicians, used the same Biblical passage. In Gilles Personne de Roberval's Aristarchi Samii de Mundi Systemate (1644), he frequently uses the abbreviation “P.N.E.M.” standing for “Pondere, mensura et mensura.” It was adopted as the motto of the Smeatonian Society of Engineers as “Omnia in Numero Pondere et Mensura.”
Quinquies exscriptus, maneat tot millibus annis.
(I wrote it out five times, may it last the same number of millennia.)
(I wrote it out five times, may it last the same number of millennia.)
final line of Ars magna

Replying to G. H. Hardy’s suggestion that the number of a taxi (1729) was “dull”: No, it is a very interesting number; it is the smallest number expressible as a sum of two cubes in two different ways, the two ways being 1³ + 12³ and 9³ + 10³.
Proceedings of the London Mathematical Society (26 May 1921).
Science for the Citizen is ... also written for the large and growing number of adolescents, who realize that they will be the first victims of the new destructive powers of science misapplied.
Science for the Citizen: A Self-Educator based on the Social Background of Scientific Discovery (1938), Author's Confessions, 9.
Surtout l’astronomie et l’anatomie sont les deux sciences qui nous offrent le plus sensiblement deux grands caractères du Créateur; l’une, son immensité, par les distances, la grandeur, et le nombre des corps célestes; l’autre, son intelligence infinie, par la méchanique des animaux.
Above all, astronomy and anatomy are the two sciences which present to our minds most significantly the two grand characteristics of the Creator; the one, His immensity, by the distances, size, and number of the heavenly bodies; the other, His infinite intelligence, by the mechanism of animate beings.
Above all, astronomy and anatomy are the two sciences which present to our minds most significantly the two grand characteristics of the Creator; the one, His immensity, by the distances, size, and number of the heavenly bodies; the other, His infinite intelligence, by the mechanism of animate beings.
Original French and translation in Craufurd Tait Ramage (ed.) Beautiful Thoughts from French and Italian Authors (1866), 119-120.
Thomasina: Every week I plot your equations dot for dot, x’s against y’s in all manner of algebraical relation, and every week they draw themselves as commonplace geometry, as if the world of forms were nothing but arcs and angles. God’s truth, Septimus, if there is an equation for a curve like a bell, there must be an equation for one like a bluebell, and if a bluebell, why not a rose? Do we believe nature is written in numbers?
Septimus: We do.
Thomasina: Then why do your shapes describe only the shapes of manufacture?
Septimus: I do not know.
Thomasina: Armed thus, God could only make a cabinet.
Septimus: We do.
Thomasina: Then why do your shapes describe only the shapes of manufacture?
Septimus: I do not know.
Thomasina: Armed thus, God could only make a cabinet.
In the play, Acadia (1993), Scene 3, 37.
Tolle numerum omnibus rebus et omnia pereunt.
Take from all things their number and all shall perish.
Take from all things their number and all shall perish.
Etymologies [c.600], Book III, chapter 4, quoted in E. Grant (ed.), A Source Book in Medieval Science (1974), trans. E. Brehaut (1912), revised by E. Grant, 5.
~~[Attributed]~~ It is not once nor twice but times without number that the same ideas make their appearance in the world.
As quoted in Thomas L. Heath Manual of Greek Mathematics (1931, 2003), 205. Webmaster has so far found no primary source, and is dubious about authenticity. Can you help?
~~[need primary source]~~ One of the most frightening things in the Western world and in this country in particular is the number of people who believe in things that are scientifically false. If someone tells me that the earth is less than 10000 years old in my opinion he should see a psychiatrist.
As quoted, without citation, in Joan Konner, The Atheist’s Bible: An Illustrious Collection of Irreverent Thought (2007), 46. Webmaster is dubious about authenticity, and as yet, has been unable to find a reliable primary source - can you help?
~~[Orphan]~~ Perfect numbers like perfect men are very rare.
Appears without citation in Howard W. Eves, Mathematical Circles Squared (1972), 7, and it is noted with the fact that only 12 perfect numbers were known in Descartes’ time. Webmaster regards this as an orphan (better regarded as anonymous), and has so far been unable to trace a primary source in the work of Descartes. Can you help?
1095 … Then after Easter on the eve of St. Ambrose, which is on 4 April [recte 3 April], almost everywhere in this country and almost the whole night, stars in very large numbers were seen to fall from heaven, not by ones or twos, but in such quick succession that they could not be counted.
In The Anglo-Saxon Chronicle, as translated in The Anglo-Saxon Chronicle, Issue 1624 (1975), 230. The Chronicle is the work of many successive hands at several monasteries across England. For the date recorded, This meteor shower could have been a display of the Lyrids (according to The Starseeker, reprinted in O. Vrazell, 'Astronomical Observations in the Anglo Saxon Chronicle', Journal of the Royal Astronomical Society of Canada Newsletter (Aug 1984), 78, 57.
230(231-1) ... is the greatest perfect number known at present, and probably the greatest that ever will be discovered; for; as they are merely curious without being useful, it is not likely that any person will attempt to find a number beyond it.
In An Elementary Investigation of the Theory of Numbers (1811), 43. The stated number, which evaluates as 2305843008139952128 was discovered by Euler in 1772 as the eighth known perfect number. It has 19 digits. By 2013, the 48th perfect number found had 34850340 digits.
A … difference between most system-building in the social sciences and systems of thought and classification of the natural sciences is to be seen in their evolution. In the natural sciences both theories and descriptive systems grow by adaptation to the increasing knowledge and experience of the scientists. In the social sciences, systems often issue fully formed from the mind of one man. Then they may be much discussed if they attract attention, but progressive adaptive modification as a result of the concerted efforts of great numbers of men is rare.
The Study of Man (1941), 19-20.
A biologist, if he wishes to know how many toes a cat has, does not "frame the hypothesis that the number of feline digital extremities is 4, or 5, or 6," he simply looks at a cat and counts. A social scientist prefers the more long-winded expression every time, because it gives an entirely spurious impression of scientificness to what he is doing.
In Science is a Sacred Cow (1950), 151.
A commonsense interpretation of the facts suggests that a superintellect has monkeyed with physics, as well as with chemistry and biology, and that there are no blind forces worth speaking about in nature. The numbers one calculates from the facts seem to me so overwhelming as to put this conclusion almost beyond question.
In 'The Universe: Past and Present Reflections', Astrophysics and Relativity (1981), 70, 26.
A comparatively small variety of species is found in the older rocks, although of some particular ones the remains are very abundant; ... Ascending to the next group of rocks, we find the traces of life become more abundant, the number of species extended.
Vestiges of the Natural History of Creation (1844), 60-1.
A considerable number of persons are able to protect themselves against the outbreak of serious neurotic phenomena only through intense work.
From Observations on Ferenczi's paper on 'Sunday Neuroses' (1918). Quoted in Peter Bryan Warr, Work, Happiness, and Unhappiness (2007), 161.
A depressing number of people seem to process everything literally. They are to wit as a blind man is to a forest, able to find every tree, but each one coming as a surprise.
Quoted in Kim Lim (ed.), 1,001 Pearls of Spiritual Wisdom: Words to Enrich, Inspire, and Guide Your Life (2014), 32
A distinguished writer [Siméon Denis Poisson] has thus stated the fundamental definitions of the science:
“The probability of an event is the reason we have to believe that it has taken place, or that it will take place.”
“The measure of the probability of an event is the ratio of the number of cases favourable to that event, to the total number of cases favourable or contrary, and all equally possible” (equally like to happen).
From these definitions it follows that the word probability, in its mathematical acceptation, has reference to the state of our knowledge of the circumstances under which an event may happen or fail. With the degree of information which we possess concerning the circumstances of an event, the reason we have to think that it will occur, or, to use a single term, our expectation of it, will vary. Probability is expectation founded upon partial knowledge. A perfect acquaintance with all the circumstances affecting the occurrence of an event would change expectation into certainty, and leave neither room nor demand for a theory of probabilities.
“The probability of an event is the reason we have to believe that it has taken place, or that it will take place.”
“The measure of the probability of an event is the ratio of the number of cases favourable to that event, to the total number of cases favourable or contrary, and all equally possible” (equally like to happen).
From these definitions it follows that the word probability, in its mathematical acceptation, has reference to the state of our knowledge of the circumstances under which an event may happen or fail. With the degree of information which we possess concerning the circumstances of an event, the reason we have to think that it will occur, or, to use a single term, our expectation of it, will vary. Probability is expectation founded upon partial knowledge. A perfect acquaintance with all the circumstances affecting the occurrence of an event would change expectation into certainty, and leave neither room nor demand for a theory of probabilities.
An Investigation of the Laws of Thought (1854), 243-244. The Poisson quote is footnoted as from Recherches sur la Probabilité des Jugemens.
A doctor’s reputation is made by the number of eminent men who die under his care.
Statement (14 Sep 1950) at age 94 to his doctor. As quoted in Michael Holroyd, Bernard Shaw: The Lure of Fantasy: Vol. 3: 1918-1951 (1991).
A fair number of people who go on to major in astronomy have decided on it certainly by the time they leave junior high, if not during junior high. I think it’s somewhat unusual that way. I think most children pick their field quite a bit later, but astronomy seems to catch early, and if it does, it sticks.
From interview by Rebecca Wright, 'Oral History Transcript' (15 Sep 2000), on NASA website.
A first step in the study of civilization is to dissect it into details, and to classify these in their proper groups. Thus, in examining weapons, they are to be classed under spear, club, sling, bow and arrow, and so forth; among textile arts are to be ranged matting, netting, and several grades of making and weaving threads; myths are divided under such headings as myths of sunrise and sunset, eclipse-myths, earthquake-myths, local myths which account for the names of places by some fanciful tale, eponymic myths which account for the parentage of a tribe by turning its name into the name of an imaginary ancestor; under rites and ceremonies occur such practices as the various kinds of sacrifice to the ghosts of the dead and to other spiritual beings, the turning to the east in worship, the purification of ceremonial or moral uncleanness by means of water or fire. Such are a few miscellaneous examples from a list of hundreds … To the ethnographer, the bow and arrow is the species, the habit of flattening children’s skulls is a species, the practice of reckoning numbers by tens is a species. The geographical distribution of these things, and their transmission from region to region, have to be studied as the naturalist studies the geography of his botanical and zoological species.
In Primitive Culture (1871), Vol. 1, 7.
A fossil hunter needs sharp eyes and a keen search image, a mental template that subconsciously evaluates everything he sees in his search for telltale clues. A kind of mental radar works even if he isn’t concentrating hard. A fossil mollusk expert has a mollusk search image. A fossil antelope expert has an antelope search image. … Yet even when one has a good internal radar, the search is incredibly more difficult than it sounds. Not only are fossils often the same color as the rocks among which they are found, so they blend in with the background; they are also usually broken into odd-shaped fragments. … In our business, we don’t expect to find a whole skull lying on the surface staring up at us. The typical find is a small piece of petrified bone. The fossil hunter’s search therefore has to have an infinite number of dimensions, matching every conceivable angle of every shape of fragment of every bone on the human body.
Describing the skill of his co-worker, Kamoya Kimeu, who discovered the Turkana Boy, the most complete specimen of Homo erectus, on a slope covered with black lava pebbles.
Describing the skill of his co-worker, Kamoya Kimeu, who discovered the Turkana Boy, the most complete specimen of Homo erectus, on a slope covered with black lava pebbles.
Richard Leakey and Roger Lewin, Origins Reconsidered: In Search of What Makes Us Human (1992), 26.
A googleplex is precisely as far from infinity as is the number 1 ... No matter what number you have in mind, infinity is larger.
In Cosmos (1980, 2011), 181.
A hundred years ago … an engineer, Herbert Spencer, was willing to expound every aspect of life, with an effect on his admiring readers which has not worn off today.
Things do not happen quite in this way nowadays. This, we are told, is an age of specialists. The pursuit of knowledge has become a profession. The time when a man could master several sciences is past. He must now, they say, put all his efforts into one subject. And presumably, he must get all his ideas from this one subject. The world, to be sure, needs men who will follow such a rule with enthusiasm. It needs the greatest numbers of the ablest technicians. But apart from them it also needs men who will converse and think and even work in more than one science and know how to combine or connect them. Such men, I believe, are still to be found today. They are still as glad to exchange ideas as they have been in the past. But we cannot say that our way of life is well-fitted to help them. Why is this?
Things do not happen quite in this way nowadays. This, we are told, is an age of specialists. The pursuit of knowledge has become a profession. The time when a man could master several sciences is past. He must now, they say, put all his efforts into one subject. And presumably, he must get all his ideas from this one subject. The world, to be sure, needs men who will follow such a rule with enthusiasm. It needs the greatest numbers of the ablest technicians. But apart from them it also needs men who will converse and think and even work in more than one science and know how to combine or connect them. Such men, I believe, are still to be found today. They are still as glad to exchange ideas as they have been in the past. But we cannot say that our way of life is well-fitted to help them. Why is this?
In 'The Unification of Biology', New Scientist (11 Jan 1962), 13, No. 269, 72.
A large number of areas of the brain are involved when viewing equations, but when one looks at a formula rated as beautiful it activates the emotional brain—the medial orbito-frontal cortex—like looking at a great painting or listening to a piece of music. … Neuroscience can’t tell you what beauty is, but if you find it beautiful the medial orbito-frontal cortex is likely to be involved; you can find beauty in anything.
As quoted in James Gallagher, 'Mathematics: Why The Brain Sees Maths As Beauty,' BBC News (13 Feb 2014), on bbc.co.uk web site.
A mathematician’s reputation rests on the number of bad proofs he has given.
As quoted in John E. Littlewood, A Mathematician’s Miscellany (1953), 41. [His meaning was that an important result is built upon earlier clumsy work. —Webmaster]
A number of years ago, when I was a freshly-appointed instructor, I met, for the first time, a certain eminent historian of science. At the time I could only regard him with tolerant condescension.
I was sorry of the man who, it seemed to me, was forced to hover about the edges of science. He was compelled to shiver endlessly in the outskirts, getting only feeble warmth from the distant sun of science-in-progress; while I, just beginning my research, was bathed in the heady liquid heat up at the very center of the glow.
In a lifetime of being wrong at many a point, I was never more wrong. It was I, not he, who was wandering in the periphery. It was he, not I, who lived in the blaze.
I had fallen victim to the fallacy of the “growing edge;” the belief that only the very frontier of scientific advance counted; that everything that had been left behind by that advance was faded and dead.
But is that true? Because a tree in spring buds and comes greenly into leaf, are those leaves therefore the tree? If the newborn twigs and their leaves were all that existed, they would form a vague halo of green suspended in mid-air, but surely that is not the tree. The leaves, by themselves, are no more than trivial fluttering decoration. It is the trunk and limbs that give the tree its grandeur and the leaves themselves their meaning.
There is not a discovery in science, however revolutionary, however sparkling with insight, that does not arise out of what went before. “If I have seen further than other men,” said Isaac Newton, “it is because I have stood on the shoulders of giants.”
I was sorry of the man who, it seemed to me, was forced to hover about the edges of science. He was compelled to shiver endlessly in the outskirts, getting only feeble warmth from the distant sun of science-in-progress; while I, just beginning my research, was bathed in the heady liquid heat up at the very center of the glow.
In a lifetime of being wrong at many a point, I was never more wrong. It was I, not he, who was wandering in the periphery. It was he, not I, who lived in the blaze.
I had fallen victim to the fallacy of the “growing edge;” the belief that only the very frontier of scientific advance counted; that everything that had been left behind by that advance was faded and dead.
But is that true? Because a tree in spring buds and comes greenly into leaf, are those leaves therefore the tree? If the newborn twigs and their leaves were all that existed, they would form a vague halo of green suspended in mid-air, but surely that is not the tree. The leaves, by themselves, are no more than trivial fluttering decoration. It is the trunk and limbs that give the tree its grandeur and the leaves themselves their meaning.
There is not a discovery in science, however revolutionary, however sparkling with insight, that does not arise out of what went before. “If I have seen further than other men,” said Isaac Newton, “it is because I have stood on the shoulders of giants.”
Adding A Dimension: Seventeen Essays on the History of Science (1964), Introduction.
A poet is, after all, a sort of scientist, but engaged in a qualitative science in which nothing is measurable. He lives with data that cannot be numbered, and his experiments can be done only once. The information in a poem is, by definition, not reproducible. ... He becomes an equivalent of scientist, in the act of examining and sorting the things popping in [to his head], finding the marks of remote similarity, points of distant relationship, tiny irregularities that indicate that this one is really the same as that one over there only more important. Gauging the fit, he can meticulously place pieces of the universe together, in geometric configurations that are as beautiful and balanced as crystals.
In The Medusa and the Snail: More Notes of a Biology Watcher (1974, 1995), 107.
A quarter-horse jockey learns to think of a twenty-second race as if it were occurring across twenty minutes—in distinct parts, spaced in his consciousness. Each nuance of the ride comes to him as he builds his race. If you can do the opposite with deep time, living in it and thinking in it until the large numbers settle into place, you can sense how swiftly the initial earth packed itself together, how swiftly continents have assembled and come apart, how far and rapidly continents travel, how quickly mountains rise and how quickly they disintegrate and disappear.
Annals of the Former World
A right understanding of the words which are names of names, is of great importance in philosophy. The tendency was always strong to believe that whatever receives a name must be an entity or being, having an independent existence of its own; and if no real entity answering to the name could be found, men did not for that reason suppose that none existed, but imagined that it was something peculiarly abstruse and mysterious, too high to be an object of sense. The meaning of all general, and especially of all abstract terms, became in this way enveloped in a mystical haze; and none of these have been more generally misunderstood, or have been a more copious source of futile and bewildering speculation, than some of the words which are names of names. Genus, Species, Universal, were long supposed to be designations of sublime hyperphysical realities; Number, instead of a general name of all numerals, was supposed to be the name, if not of a concrete thing, at least of a single property or attribute.
A footnote by John Stuart Mill, which he added as editor of a new edition of a work by his father, James Mill, Analysis of the Phenomena of the Human Mind (1869), Vol. 2, 5.
A scientific invention consists of six (or some number) ideas, five of which are absurd but which, with the addition of the sixth and enough rearrangement of the combinations, results in something no one has thought of before.
As quoted in Robert Coughlan, 'Dr. Edward Teller’s Magnificent Obsession', Life (6 Sep 1954), 66.
A single kind of red cell is supposed to have an enormous number of different substances on it, and in the same way there are substances in the serum to react with many different animal cells. In addition, the substances which match each kind of cell are different in each kind of serum. The number of hypothetical different substances postulated makes this conception so uneconomical that the question must be asked whether it is the only one possible. ... We ourselves hold that another, simpler, explanation is possible.
Landsteiner and Adriano Sturli, 'Hamagglutinine normaler Sera', Wiener klinische Wochenschrift (1902), 15, 38-40. Trans. Pauline M. H. Mazumdar.
A statistician is someone who is good with numbers but lacks the personality to be an accountant.
[Or economist]
[Or economist]
Found, for example, in A Prairie Home Companion Pretty Good Joke Book (4th ed., 2005), 216. Also seen as defining an Economist in Eighteen Annual Institute on Securities Regulation (1987), 131, which quotes it as “Tim Wirth used to say.”
A superficial knowledge of mathematics may lead to the belief that this subject can be taught incidentally, and that exercises akin to counting the petals of flowers or the legs of a grasshopper are mathematical. Such work ignores the fundamental idea out of which quantitative reasoning grows—the equality of magnitudes. It leaves the pupil unaware of that relativity which is the essence of mathematical science. Numerical statements are frequently required in the study of natural history, but to repeat these as a drill upon numbers will scarcely lend charm to these studies, and certainly will not result in mathematical knowledge.
In Primary Arithmetic: First Year, for the Use of Teachers (1897), 26-27.
A superficial knowledge of mathematics may lead to the belief that this subject can be taught incidentally, and that exercises akin to counting the petals of flowers or the legs of a grasshopper are mathematical. Such work ignores the fundamental idea out of which quantitative reasoning grows—the equality of magnitudes. It leaves the pupil unaware of that relativity which is the essence of mathematical science. Numerical statements are frequently required in the study of natural history, but to repeat these as a drill upon numbers will scarcely lend charm to these studies, and certainly will not result in mathematical knowledge.
In Primary Arithmetic: First Year, for the Use of Teachers (1897), 26-27.
A surprising number [of novels] have been read aloud to me, and I like all if moderately good, and if they do not end unhappily—against which a law ought to be passed.
Francis Darwin (ed.), The Life and Letters of Charles Darwin, Including an Autobiographical Chapter (1888), Vol. 1, 101.
A theory of physics is not an explanation; it is a system of mathematical oppositions deduced from a small number of principles the aim of which is to represent as simply, as completely, and as exactly as possible, a group of experimental laws.
As quoted in Philipp Frank, Modern Science and its Philosophy (1949), 15, which cites Théorie Physique; Son Objet—Son Structure (1906), 24.
A vast number, perhaps the numerical majority, of animal forms cannot be shown unequivocally to possess mind.
In 'The Brain Collaborates With Psyche', Man On His Nature: The Gifford Lectures, Edinburgh 1937-8 (1940), 284.
A young person who reads a science book is confronted with a number of facts, x = ma … ma - me² … You never see in the scientific books what lies behind the discovery—the struggle and the passion of the person, who made that discovery.
From 'Asking Nature', collected in Lewis Wolpert and Alison Richards (eds.), Passionate Minds: The Inner World of Scientists (1997), 197.
According to our ancient Buddhist texts, a thousand million solar systems make up a galaxy. … A thousand million of such galaxies form a supergalaxy. … A thousand million supergalaxies is collectively known as supergalaxy Number One. Again, a thousand million supergalaxy Number
Ones form a Supergalaxy Number Two. A thousand million supergalaxy Number Twos make up a supergalaxy Number Three, and of these, it is stated in the texts that there are a countless number in the universe.
In 'Reactions to Man’s Landing on the Moon Show Broad Variations in Opinions', The New York Times (21 Jul 1969), 6.
According to the older view, for every single effect of a serum, there was a separate substance, or at least a particular chemical group... A normal serum contained as many different haemagglutinins as it agglutinated different cells. The situation was undoubtedly made much simpler if, to use the Ehrlich terminology... the separate haptophore groups can combine with an extremely large number of receptors in stepwise differing quantities as a stain does with different animal tissues, though not always with the same intensity. A normal serum would therefore visibly affect such a large number of different blood cells... not because it contained countless special substances, but because of the colloids of the serum, and therefore of the agglutinins by reason of their chemical constitution and the electrochemical properties resulting from it. That this manner of representation is a considerable simplification is clear; it also opens the way to direct experimental testing by the methods of structural chemistry.
'Die Theorien der Antikorperbildung ... ', Wiener klinische Wöchenschrift (1909), 22, 1623-1631. Trans. Pauline M. H. Mazumdar.
Accurate and minute measurement seems to the non-scientific imagination, a less lofty and dignified work than looking for something new. But nearly all the grandest discoveries of science have been but the rewards of accurate measurement and patient long-continued labour in the minute sifting of numerical results.
Presidential inaugural address, to the General Meeting of the British Association, Edinburgh (2 Aug 1871). In Report of the Forty-First Meeting of the British Association for the Advancement of Science (1872), xci.
After the birth of printing books became widespread. Hence everyone throughout Europe devoted himself to the study of literature... Every year, especially since 1563, the number of writings published in every field is greater than all those produced in the past thousand years. Through them there has today been created a new theology and a new jurisprudence; the Paracelsians have created medicine anew and the Copernicans have created astronomy anew. I really believe that at last the world is alive, indeed seething, and that the stimuli of these remarkable conjunctions did not act in vain.
De Stella Nova, On the New Star (1606), Johannes Kepler Gesammelte Werke (1937- ), Vol. 1, 330-2. Quoted in N. Jardine, The Birth of History and Philosophy of Science: Kepler's A Defence of Tycho Against Ursus With Essays on its Provenance and Significance (1984), 277-8.
Again, it [the Analytical Engine] might act upon other things besides number, were objects found whose mutual fundamental relations could be expressed by those of the abstract science of operations, and which should be also susceptible of adaptations to the action of the operating notation and mechanism of the engine. Supposing for instance, that the fundamental relations of pitched sounds in the science of harmony and of musical composition were susceptible of such expression and adaptations, the engine might compose elaborate and scientific pieces of music of any degree of complexity or extent.
In Richard Taylor (ed.), 'Translator’s Notes to M. Menabrea’s Memoir', Scientific Memoirs, Selected from the Transactions of Foreign Academies and Learned Societies and from Foreign Journals (1843), 3, Note A, 694. Her notes were appended to L.F. Menabrea, of Turin, Officer of the Military Engineers, 'Article XXIX: Sketch of the Analytical Engine invented by Charles Babbage Esq.', Bibliothèque Universelle de Gnve (Oct 1842), No. 82.
All change is relative. The universe is expanding relatively to our common material standards; our material standards are shrinking relatively to the size of the universe. The theory of the “expanding universe” might also be called the theory of the “shrinking atom”. …
:Let us then take the whole universe as our standard of constancy, and adopt the view of a cosmic being whose body is composed of intergalactic spaces and swells as they swell. Or rather we must now say it keeps the same size, for he will not admit that it is he who has changed. Watching us for a few thousand million years, he sees us shrinking; atoms, animals, planets, even the galaxies, all shrink alike; only the intergalactic spaces remain the same. The earth spirals round the sun in an ever-decreasing orbit. It would be absurd to treat its changing revolution as a constant unit of time. The cosmic being will naturally relate his units of length and time so that the velocity of light remains constant. Our years will then decrease in geometrical progression in the cosmic scale of time. On that scale man’s life is becoming briefer; his threescore years and ten are an ever-decreasing allowance. Owing to the property of geometrical progressions an infinite number of our years will add up to a finite cosmic time; so that what we should call the end of eternity is an ordinary finite date in the cosmic calendar. But on that date the universe has expanded to infinity in our reckoning, and we have shrunk to nothing in the reckoning of the cosmic being.
We walk the stage of life, performers of a drama for the benefit of the cosmic spectator. As the scenes proceed he notices that the actors are growing smaller and the action quicker. When the last act opens the curtain rises on midget actors rushing through their parts at frantic speed. Smaller and smaller. Faster and faster. One last microscopic blurr of intense agitation. And then nothing.
:Let us then take the whole universe as our standard of constancy, and adopt the view of a cosmic being whose body is composed of intergalactic spaces and swells as they swell. Or rather we must now say it keeps the same size, for he will not admit that it is he who has changed. Watching us for a few thousand million years, he sees us shrinking; atoms, animals, planets, even the galaxies, all shrink alike; only the intergalactic spaces remain the same. The earth spirals round the sun in an ever-decreasing orbit. It would be absurd to treat its changing revolution as a constant unit of time. The cosmic being will naturally relate his units of length and time so that the velocity of light remains constant. Our years will then decrease in geometrical progression in the cosmic scale of time. On that scale man’s life is becoming briefer; his threescore years and ten are an ever-decreasing allowance. Owing to the property of geometrical progressions an infinite number of our years will add up to a finite cosmic time; so that what we should call the end of eternity is an ordinary finite date in the cosmic calendar. But on that date the universe has expanded to infinity in our reckoning, and we have shrunk to nothing in the reckoning of the cosmic being.
We walk the stage of life, performers of a drama for the benefit of the cosmic spectator. As the scenes proceed he notices that the actors are growing smaller and the action quicker. When the last act opens the curtain rises on midget actors rushing through their parts at frantic speed. Smaller and smaller. Faster and faster. One last microscopic blurr of intense agitation. And then nothing.
In The Expanding Universe (1933) , 90-92.
All is number
Quoted in Robert J. Scully, The Demon and the Quantum (2007), 7.
All sorts of computer errors are now turning up. You'd be surprised to know the number of doctors who claim they are treating pregnant men.
Official of the Quebec Health Insurance Board, on Use of Computers in Quebec Province's Comprehensive Medical-care system. F. 19, 4:5. In Barbara Bennett and Linda Amster, Who Said What (and When, and Where, and How) in 1971: December-June, 1971 (1972), Vol. 1, 38. (Later sources cite Isaac Asimov.)
All that can be said upon the number and nature of elements is, in my opinion, confined to discussions entirely of a metaphysical nature. The subject only furnishes us with indefinite problems, which may be solved in a thousand different ways, not one of which, in all probability, is consistent with nature. I shall therefore only add upon this subject, that if, by the term elements, we mean to express those simple and indivisible atoms of which matter is composed, it is extremely probable we know nothing at all about them; but, if we apply the term elements, or principles of bodies, to express our idea of the last point which analysis is capable of reaching, we must admit, as elements, all the substances into which we are capable, by any means, to reduce bodies by decomposition.
Elements of Chemistry (1790), trans. R. Kerr, Preface, xxiv.
All the effects of Nature are only the mathematical consequences of a small number of immutable laws.
From the original French, “Tous les effets de la nature ne sont que résultats mathématiques d'un petit noinbre de lois immuables.”, in Oeuvres de Laplace, Vol. VII: Théorie des probabilités (1847), Introduction, cliv.
All the mathematical sciences are founded on relations between physical laws and laws of numbers, so that the aim of exact science is to reduce the problems of nature to the determination of quantities by operations with numbers.
from Faraday's Lines of Force (1856)
All the modern higher mathematics is based on a calculus of operations, on laws of thought. All mathematics, from the first, was so in reality; but the evolvers of the modern higher calculus have known that it is so. Therefore elementary teachers who, at the present day, persist in thinking about algebra and arithmetic as dealing with laws of number, and about geometry as dealing with laws of surface and solid content, are doing the best that in them lies to put their pupils on the wrong track for reaching in the future any true understanding of the higher algebras. Algebras deal not with laws of number, but with such laws of the human thinking machinery as have been discovered in the course of investigations on numbers. Plane geometry deals with such laws of thought as were discovered by men intent on finding out how to measure surface; and solid geometry with such additional laws of thought as were discovered when men began to extend geometry into three dimensions.
In Lectures on the Logic of Arithmetic (1903), Preface, 18-19.
All the species recognized by Botanists came forth from the Almighty Creator’s hand, and the number of these is now and always will be exactly the same, while every day new and different florists’ species arise from the true species so-called by Botanists, and when they have arisen they finally revert to the original forms. Accordingly to the former have been assigned by Nature fixed limits, beyond which they cannot go: while the latter display without end the infinite sport of Nature.
In Philosophia Botanica (1751), aphorism 310. Trans. Frans A. Stafleu, Linnaeus and the Linnaeans: The Spreading of their Ideas in Systematic Botany, 1735-1789 (1971), 90.
Although [Charles Darwin] would patiently go on repeating experiments where there was any good to be gained, he could not endure having to repeat an experiment which ought, if complete care had been taken, to have told its story at first—and this gave him a continual anxiety that the experiment should not be wasted; he felt the experiment to be sacred, however slight a one it was. He wished to learn as much as possible from an experiment, so that he did not confine himself to observing the single point to which the experiment was directed, and his power of seeing a number of other things was wonderful. ... Any experiment done was to be of some use, and ... strongly he urged the necessity of keeping the notes of experiments which failed, and to this rule he always adhered.
In Charles Darwin: His Life Told in an Autobiographical Chapter, and in a Selected Series of his Published Letters (1908), 92.
Although we are mere sojourners on the surface of the planet, chained to a mere point in space, enduring but for a moment of time, the human mind is not only enabled to number worlds beyond the unassisted ken of mortal eye, but to trace the events of indefinite ages before the creation of our race, and is not even withheld from penetrating into the dark secrets of the ocean, or the interior of the solid globe; free, like the spirit which the poet described as animating the universe.
In Principles of Geology (1830).
Always preoccupied with his profound researches, the great Newton showed in the ordinary-affairs of life an absence of mind which has become proverbial. It is related that one day, wishing to find the number of seconds necessary for the boiling of an egg, he perceived, after waiting a minute, that he held the egg in his hand, and had placed his seconds watch (an instrument of great value on account of its mathematical precision) to boil!
This absence of mind reminds one of the mathematician Ampere, who one day, as he was going to his course of lectures, noticed a little pebble on the road; he picked it up, and examined with admiration the mottled veins. All at once the lecture which he ought to be attending to returned to his mind; he drew out his watch; perceiving that the hour approached, he hastily doubled his pace, carefully placed the pebble in his pocket, and threw his watch over the parapet of the Pont des Arts.
This absence of mind reminds one of the mathematician Ampere, who one day, as he was going to his course of lectures, noticed a little pebble on the road; he picked it up, and examined with admiration the mottled veins. All at once the lecture which he ought to be attending to returned to his mind; he drew out his watch; perceiving that the hour approached, he hastily doubled his pace, carefully placed the pebble in his pocket, and threw his watch over the parapet of the Pont des Arts.
Popular Astronomy: a General Description of the Heavens (1884), translated by J. Ellard Gore, (1907), 93.
Among innumerable footsteps of divine providence to be found in the works of nature, there is a very remarkable one to be observed in the exact balance that is maintained, between the numbers of men and women; for by this means is provided, that the species never may fail, nor perish, since every male may have its female, and of proportionable age. This equality of males and females is not the effect of chance but divine providence, working for a good end, which I thus demonstrate.
'An Argument for Divine Providence, taken from the Constant Regularity observ’d in the Births of both Sexes', Philosophical Transactions of the Royal Society, 1710-12, 27, 186. This has been regarded as the origin of mathematical statistics
Among the social sciences, economists are the snobs. Economics, with its numbers and graphs and curves, at least has the coloration and paraphernalia of a hard science. It's not just putting on sandals and trekking out to take notes on some tribe.
'A Cuba Policy That's Stuck On Plan A', opinion column in Washington Post (17 April 2009).
An astronomer must be the wisest of men; his mind must be duly disciplined in youth; especially is mathematical study necessary; both an acquaintance with the doctrine of number, and also with that other branch of mathematics, which, closely connected as it is with the science of the heavens, we very absurdly call geometry, the measurement of the earth.
— Plato
From the 'Epilogue to the Laws' (Epinomis), 988-990. As quoted in William Whewell, History of the Inductive Sciences from the Earliest to the Present Time (1837), Vol. 1, 161. (Although referenced to Plato’s Laws, the Epinomis is regarded as a later addition, not by Plato himself.)
An author has always great difficulty in avoiding unnecessary and tedious detail on the one hand; while, on the other, he must notice such a number of facts as may convince a student, that he is not wandering in a wilderness of crude hypotheses or unsupported assumptions.
In A Geological Manual (1832), Preface, iii.
An event experienced is an event perceived, digested, and assimilated into the substance of our being, and the ratio between the number of cases seen and the number of cases assimilated is the measure of experience.
Address, opening of 1932-3 session of U.C.H. Medical School (4 Oct 1932), 'Art and Science in medicine', The Collected Papers of Wilfred Trotter, FRS (1941), 98.
An important fact, an ingenious aperçu, occupies a very great number of men, at first only to make acquaintance with it; then to understand it; and afterwards to work it out and carry it further.
In The Maxims and Reflections of Goethe (1906), 189.
An Individual, whatever species it might be, is nothing in the Universe. A hundred, a thousand individuals are still nothing. The species are the only creatures of Nature, perpetual creatures, as old and as permanent as it. In order to judge it better, we no longer consider the species as a collection or as a series of similar individuals, but as a whole independent of number, independent of time, a whole always living, always the same, a whole which has been counted as one in the works of creation, and which, as a consequence, makes only a unity in Nature.
'De la Nature: Seconde Vue', Histoire Naturelle, Générale et Particulière, Avec la Description du Cabinet du Roi (1765), Vol. 13, i. Trans. Phillip R. Sloan.
An undefined problem has an infinite number of solutions.
…...
And since the portions of the great and the small are equal in number, so too all things would be in everything. Nor is it possible that they should exist apart, but all things have a portion of everything.
Simplicius, Commentary on Aristotle’s Physics, 164, 26-8. In G. S. Kirk, J. E. Raven and M. Schofield (eds.), The Presocratic Philosophers: A Critical History with a Selection of Texts (1983) , p. 365-6.
And therefore, sir, as you desire to live,
A day or two before your laxative,
Take just three worms, nor under nor above,
Because the gods unequal numbers love.
These digestives prepare you for your purge,
Of fumetery, centaury, and spurge;
And of ground-ivy add a leaf or two.
All which within our yard or garden grow.
Eat these, and be, my lord, of better cheer:
Your father’s son was never born to fear.
A day or two before your laxative,
Take just three worms, nor under nor above,
Because the gods unequal numbers love.
These digestives prepare you for your purge,
Of fumetery, centaury, and spurge;
And of ground-ivy add a leaf or two.
All which within our yard or garden grow.
Eat these, and be, my lord, of better cheer:
Your father’s son was never born to fear.
And yet surely to alchemy this right is due, that it may be compared to the husbandman whereof Æsop makes the fable, that when he died he told his sons that he had left unto them gold buried under the ground in his vineyard: and they digged over the ground, gold they found none, but by reason of their stirring and digging the mould about the roots of their vines, they had a great vintage the year following: so assuredly the search and stir to make gold hath brought to light a great number of good and fruitful inventions and experiments, as well for the disclosing of nature as for the use of man's life.
The Advancement of Learning (1605, 1712), Vol. 1, 15.
Any conception which is definitely and completely determined by means of a finite number of specifications, say by assigning a finite number of elements, is a mathematical conception. Mathematics has for its function to develop the consequences involved in the definition of a group of mathematical conceptions. Interdependence and mutual logical consistency among the members of the group are postulated, otherwise the group would either have to be treated as several distinct groups, or would lie beyond the sphere of mathematics.
In 'Mathematics', Encyclopedia Britannica (9th ed.).
Any experiment may be regarded as forming an individual of a 'population' of experiments which might be performed under the same conditions. A series of experiments is a sample drawn from this population.
Now any series of experiments is only of value in so far as it enables us to form a judgment as to the statistical constants of the population to which the experiments belong. In a great number of cases the question finally turns on the value of a mean, either directly, or as the mean difference between the two qualities.
If the number of experiments be very large, we may have precise information as to the value of the mean, but if our sample be small, we have two sources of uncertainty:— (I) owing to the 'error of random sampling' the mean of our series of experiments deviates more or less widely from the mean of the population, and (2) the sample is not sufficiently large to determine what is the law of distribution of individuals.
Now any series of experiments is only of value in so far as it enables us to form a judgment as to the statistical constants of the population to which the experiments belong. In a great number of cases the question finally turns on the value of a mean, either directly, or as the mean difference between the two qualities.
If the number of experiments be very large, we may have precise information as to the value of the mean, but if our sample be small, we have two sources of uncertainty:— (I) owing to the 'error of random sampling' the mean of our series of experiments deviates more or less widely from the mean of the population, and (2) the sample is not sufficiently large to determine what is the law of distribution of individuals.
'The Probable Error of a Mean', Biometrika, 1908, 6, 1.
Any time you wish to demonstrate something, the number of faults is proportional to the number of viewers.
Bye's First Law of Model Railroading. In Paul Dickson, The Official Rules, (1978), 23.
Anyone of common mental and physical health can practice scientific research. … Anyone can try by patient experiment what happens if this or that substance be mixed in this or that proportion with some other under this or that condition. Anyone can vary the experiment in any number of ways. He that hits in this fashion on something novel and of use will have fame. … The fame will be the product of luck and industry. It will not be the product of special talent.
In Essays of a Catholic Layman in England (1931).
Anyone who considers arithmetical methods of producing random digits is, of course, in the state of sin. For, as has been pointed out several times, there is no such thing as a random number—there are only methods to produce random numbers, and a strict arithmetic procedure of course is not such a method.
In paper delivered at a symposium on the Monte Carlo method. 'Various Techniques Used in Connection with Random Digits', Journal of Research of the National Bureau of Standards, Appl. Math. Series, Vol. 3 (1951), 3, 36. Reprinted in John von Neumann: Collected Works (1963), Vol. 5, 700. Also often seen misquoted (?) as “Anyone who attempts to generate random numbers by deterministic means is, of course, living in a state of sin.”
Architecture is geometry made visible in the same sense that music is number made audible.
In The Beautiful Necessity: Seven Essays on Theosophy and Architecture (1910),
Arithmetic is numbers you squeeze from your head to your hand to your pencil to your paper till you get the answer.
From 'Arithmetic', Harvest Poems, 1910-1960 (1960), 115.
Arithmetic is where numbers fly like pigeons in and out of your head.
From 'Arithmetic', Harvest Poems, 1910-1960 (1960), 115.
Arithmetic must be discovered in just the same sense in which Columbus discovered the West Indies, and we no more create numbers than he created the Indians.
The Principles of Mathematics (1903), 451.
As a little boy, I showed an abnormal aptitude for mathematics this gift played a horrible part in tussles with quinsy or scarlet fever, when I felt enormous spheres and huge numbers swell relentlessly in my aching brain.
In Speak, Memory: An Autobiography Revisited (1999), 2
As an individual opinion of mine, perhaps not as yet shared by many, I may be permitted to state, by the way, that I consider pure Mathematics to be only one branch of general Logic, the branch originating from the creation of Number, to the economical virtues of which is due the enormous development that particular branch has been favored with in comparison with the other branches of Logic that until of late almost remained stationary.
In Lecture (10 Aug 1898) present in German to the First International Congress of Mathematicians in Zürich, 'On Pasigraphy: Its Present State and the Pasigraphic Movement in Italy'. As translated and published in The Monist (1899), 9, No. 1, 46.
As I strayed into the study of an eminent physicist, I observed hanging against the wall, framed like a choice engraving, several dingy, ribbon-like strips of, I knew not what... My curiosity was at once aroused. What were they? ... They might be shreds of mummy-wraps or bits of friable bark-cloth from the Pacific, ... [or] remnants from a grandmother’s wedding dress... They were none of these... He explained that they were carefully-prepared photographs of portions of the Solar Spectrum. I stood and mused, absorbed in the varying yet significant intensities of light and shade, bordered by mystic letters and symbolic numbers. As I mused, the pale legend began to glow with life. Every line became luminous with meaning. Every shadow was suffused with light shining from behind, suggesting some mighty achievement of knowledge; of knowledge growing more daring in proportion to the remoteness of the object known; of knowledge becoming more positive in its answers, as the questions which were asked seemed unanswerable. No Runic legend, no Babylonish arrowhead, no Egyptian hieroglyph, no Moabite stone, could present a history like this, or suggest thoughts of such weighty import or so stimulate and exalt the imagination.
The Sciences of Nature Versus the Science of Man: A Plea for the Science of Man (1871), 7-9.
As immoral and unethical as this may be [to clone a human], there is a real chance that could have had some success. This is a pure numbers game. If they have devoted enough resources and they had access to enough eggs, there is a distinct possibility. But, again, without any scientific data, one has to be extremely skeptical.
Commenting on the announcement of the purported birth of the first cloned human.
Commenting on the announcement of the purported birth of the first cloned human.
Transcript of TV interview by Sanjay Gupta aired on CNN (27 Dec 2002).
As new areas of the world came into view through exploration, the number of identified species of animals and plants grew astronomically. By 1800 it had reached 70,000. Today more than 1.25 million different species, two-thirds animal and one-third plant, are known, and no biologist supposes that the count is complete.
In The Intelligent Man's Guide to Science: The Biological Sciences (1960), 654. Also in Isaac Asimov’s Book of Science and Nature Quotations (1988), 320.
As regards the co-ordination of all ordinary properties of matter, Rutherford’s model of the atom puts before us a task reminiscent of the old dream of philosophers: to reduce the interpretation of the laws of nature to the consideration of pure numbers.
In Faraday Lecture (1930), Journal of the Chemical Society (Feb 1932), 349. As quoted and cited in Chen Ning Yang, Elementary Particles (1961), 7.
As there is no study which may be so advantageously entered upon with a less stock of preparatory knowledge than mathematics, so there is none in which a greater number of uneducated men have raised themselves, by their own exertions, to distinction and eminence. … Many of the intellectual defects which, in such cases, are commonly placed to the account of mathematical studies, ought to be ascribed to the want of a liberal education in early youth.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 3, 183.
As to the Christian religion, Sir, … there is a balance in its favor from the number of great men who have been convinced of its truth after a serious consideration of the question. Grotius was an acute man, a lawyer, a man accustomed to examine evidence, and he was convinced. Grotius was not a recluse, but a man of the world, who surely had no bias on the side of religion. Sir Isaac Newton set out an infidel, and came to be a very firm believer.
(1763). In George Birkbeck Hill (ed.), Boswell’s Life of Johnson (1799), Vol. 1, 524.
Astronomers tell us that there are about 1023 stars in the universe. That’s a meaningful number to chemists—an Avogadro number of potential solar systems of which between 1 and 50 percent are estimated to have planets. … Planets are plentiful—and from this fact we can begin our exploration of how life might have evolved on any one of them.
In 'Cosmochemistry The Earliest Evolution', The Science Teacher (Oct 1983), 50, No. 7, 34.
Astronomy affords the most extensive example of the connection of physical sciences. In it are combined the sciences of number and quantity, or rest and motion. In it we perceive the operation of a force which is mixed up with everything that exists in the heavens or on earth; which pervades every atom, rules the motion of animate and inanimate beings, and is a sensible in the descent of the rain-drop as in the falls of Niagara; in the weight of the air, as in the periods of the moon.
On the Connexion of the Physical Sciences (1858), 1.
At present we begin to feel impatient, and to wish for a new state of chemical elements. For a time the desire was to add to the metals, now we wish to diminish their number. They increase upon us continually, and threaten to enclose within their ranks the bounds of our fair fields of chemical science. The rocks of the mountain and the soil of the plain, the sands of the sea and the salts that are in it, have given way to the powers we have been able to apply to them, but only to be replaced by metals.
In his 16th Lecture of 1818, in Bence Jones, The Life and Letters of Faraday (1870), Vol. 1, 256-257.
Atoms have a nucleus, made of protons and neutrons bound together. Around this nucleus shells of electrons spin, and each shell is either full or trying to get full, to balance with the number of protons—to balance the number of positive and negative charges. An atom is like a human heart, you see.
The Lunatics (1988). In Gary Westfahl, Science Fiction Quotations: From the Inner Mind to the Outer Limits (2006), 323.
Before the introduction of the Arabic notation, multiplication was difficult, and the division even of integers called into play the highest mathematical faculties. Probably nothing in the modern world could have more astonished a Greek mathematician than to learn that, under the influence of compulsory education, the whole population of Western Europe, from the highest to the lowest, could perform the operation of division for the largest numbers. This fact would have seemed to him a sheer impossibility. … Our modern power of easy reckoning with decimal fractions is the most miraculous result of a perfect notation.
In Introduction to Mathematics (1911), 59.
Before the promulgation of the periodic law the chemical elements were mere fragmentary incidental facts in nature; there was no special reason to expect the discovery of new elements, and the new ones which were discovered from time to time appeared to be possessed of quite novel properties. The law of periodicity first enabled us to perceive undiscovered elements at a distance which formerly were inaccessible to chemical vision, and long ere they were discovered new elements appeared before our eyes possessed of a number of well-defined properties.
In Faraday Lecture, delivered before the Fellows of the Chemical Society in the Theatre of the Royal Institution (4 Jun 1889), printed in Professor Mendeléeff, 'The Periodic Law of the Chemical Elements', Transactions of the Chemical Society (1889), 55, 648.
Being also in accord with Goethe that discoveries are made by the age and not by the individual, I should consider the instances to be exceedingly rare of men who can be said to be living before their age, and to be the repository of knowledge quite foreign to the thought of the time. The rule is that a number of persons are employed at a particular piece of work, but one being a few steps in advance of the others is able to crown the edifice with his name, or, having the ability to generalise already known facts, may become in time to be regarded as their originator. Therefore it is that one name is remembered whilst those of coequals have long been buried in obscurity.
In Historical Notes on Bright's Disease, Addison's Disease, and Hodgkin's Disease', Guy's Hospital Reports (1877), 22, 259-260.
Between the lowest and the highest degree of spiritual and corporal perfection, there is an almost infinite number of intermediate degrees. The succession of degrees comprises the Universal Chain. It unites all beings, ties together all worlds, embraces all the spheres. One SINGLE BEING is outside this chain, and this is HE who made it.
Contemplation de la nature (1764), Vol. I, 27. Trans. Stephen Jay Gould, Ontogeny and Phylogeny (1977), 23.
Beyond a critical point within a finite space, freedom diminishes as numbers increase. ...The human question is not how many can possibly survive within the system, but what kind of existence is possible for those who do survive.
Dune
Bistromathics itself is simply a revolutionary new way of understanding the behavior of numbers. Just as Einstein observed that space was not an absolute but depended on the observer's movement in space, and that time was not an absolute, but depended on the observer's movement in time, so it is now realized that numbers are not absolute, but depend on the observer's movement in restaurants.
Life, the Universe and Everything (1982, 1995), 47.
Borel makes the amusing supposition of a million monkeys allowed to play upon the keys of a million typewriters. What is the chance that this wanton activity should reproduce exactly all of the volumes which are contained in the library of the British Museum? It certainly is not a large chance, but it may be roughly calculated, and proves in fact to be considerably larger than the chance that a mixture of oxygen and nitrogen will separate into the two pure constituents. After we have learned to estimate such minute chances, and after we have overcome our fear of numbers which are very much larger or very much smaller than those ordinarily employed, we might proceed to calculate the chance of still more extraordinary occurrences, and even have the boldness to regard the living cell as a result of random arrangement and rearrangement of its atoms. However, we cannot but feel that this would be carrying extrapolation too far. This feeling is due not merely to a recognition of the enormous complexity of living tissue but to the conviction that the whole trend of life, the whole process of building up more and more diverse and complex structures, which we call evolution, is the very opposite of that which we might expect from the laws of chance.
The Anatomy of Science (1926), 158-9.
Bridges would not be safer if only people who knew the proper definition of a real number were allowed to design them.
In 'Topological Theory of Defects', Review of Modern Physics (Jul 1979), 51, No. 3. 591–648. This is seen widely on the web (? mis-)attributed to H.L. Mencken, for which Webmaster has so far been unable to find any validation whatsoever.
Bring out number, weight, and measure in a year of dearth.
In 'Proverbs', The Poems: With Specimens of the Prose Writings of William Blake (1885), 279.
But as no two (theoreticians) agree on this (skin friction) or any other subject, some not agreeing today with what they wrote a year ago, I think we might put down all their results, add them together, and then divide by the number of mathematicians, and thus find the average coefficient of error. (1908)
In Artificial and Natural Flight (1908), 3. Quoted in John David Anderson, Jr., Hypersonic and High Temperature Gas Dynamics (2000), 335.
But if anyone, well seen in the knowledge, not onely of Sacred and exotick History, but of Astronomical Calculation, and the old Hebrew Kalendar, shall apply himself to these studies, I judge it indeed difficult, but not impossible for such a one to attain, not onely the number of years, but even, of dayes from the Creation of the World.
In 'Epistle to the Reader', The Annals of the World (1658). As excerpted in Wallen Yep, Man Before Adam: A Correction to Doctrinal Theology, "The Missing Link Found" (2002), 18.
But many of our imaginations and investigations of nature are futile, especially when we see little living animals and see their legs and must judge the same to be ten thousand times thinner than a hair of my beard, and when I see animals living that are more than a hundred times smaller and am unable to observe any legs at all, I still conclude from their structure and the movements of their bodies that they do have legs... and therefore legs in proportion to their bodies, just as is the case with the larger animals upon which I can see legs... Taking this number to be about a hundred times smaller, we therefore find a million legs, all these together being as thick as a hair from my beard, and these legs, besides having the instruments for movement, must be provided with vessels to carry food.
Letter to N. Grew, 27 Sep 1678. In The Collected Letters of Antoni van Leeuwenhoek (1957), Vol. 2, 391.
But the nature of our civilized minds is so detached from the senses, even in the vulgar, by abstractions corresponding to all the abstract terms our languages abound in, and so refined by the art of writing, and as it were spiritualized by the use of numbers, because even the vulgar know how to count and reckon, that it is naturally beyond our power to form the vast image of this mistress called ‘Sympathetic Nature.’
The New Science, bk. 2, para. 378 (1744, trans. 1984).
But, as we consider the totality of similarly broad and fundamental aspects of life, we cannot defend division by two as a natural principle of objective order. Indeed, the ‘stuff’ of the universe often strikes our senses as complex and shaded continua, admittedly with faster and slower moments, and bigger and smaller steps, along the way. Nature does not dictate dualities, trinities, quarterings, or any ‘objective’ basis for human taxonomies; most of our chosen schemes, and our designated numbers of categories, record human choices from a cornucopia of possibilities offered by natural variation from place to place, and permitted by the flexibility of our mental capacities. How many seasons (if we wish to divide by seasons at all) does a year contain? How many stages shall we recognize in a human life?
…...
By destroying the biological character of phenomena, the use of averages in physiology and medicine usually gives only apparent accuracy to the results. From our point of view, we may distinguish between several kinds of averages: physical averages, chemical averages and physiological and pathological averages. If, for instance, we observe the number of pulsations and the degree of blood pressure by means of the oscillations of a manometer throughout one day, and if we take the average of all our figures to get the true or average blood pressure and to learn the true or average number of pulsations, we shall simply have wrong numbers. In fact, the pulse decreases in number and intensity when we are fasting and increases during digestion or under different influences of movement and rest; all the biological characteristics of the phenomenon disappear in the average. Chemical averages are also often used. If we collect a man's urine during twenty-four hours and mix all this urine to analyze the average, we get an analysis of a urine which simply does not exist; for urine, when fasting, is different from urine during digestion. A startling instance of this kind was invented by a physiologist who took urine from a railroad station urinal where people of all nations passed, and who believed he could thus present an analysis of average European urine! Aside from physical and chemical, there are physiological averages, or what we might call average descriptions of phenomena, which are even more false. Let me assume that a physician collects a great many individual observations of a disease and that he makes an average description of symptoms observed in the individual cases; he will thus have a description that will never be matched in nature. So in physiology, we must never make average descriptions of experiments, because the true relations of phenomena disappear in the average; when dealing with complex and variable experiments, we must study their various circumstances, and then present our most perfect experiment as a type, which, however, still stands for true facts. In the cases just considered, averages must therefore be rejected, because they confuse, while aiming to unify, and distort while aiming to simplify. Averages are applicable only to reducing very slightly varying numerical data about clearly defined and absolutely simple cases.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 134-135.
By science, then, I understand the consideration of all subjects, whether of a pure or mixed nature, capable of being reduced to measurement and calculation. All things comprehended under the categories of space, time and number properly belong to our investigations; and all phenomena capable of being brought under the semblance of a law are legitimate objects of our inquiries.
In Report of the British Association for the Advancement of Science (1833), xxviii.
Cayley was singularly learned in the work of other men, and catholic in his range of knowledge. Yet he did not read a memoir completely through: his custom was to read only so much as would enable him to grasp the meaning of the symbols and understand its scope. The main result would then become to him a subject of investigation: he would establish it (or test it) by algebraic analysis and, not infrequently, develop it so to obtain other results. This faculty of grasping and testing rapidly the work of others, together with his great knowledge, made him an invaluable referee; his services in this capacity were used through a long series of years by a number of societies to which he was almost in the position of standing mathematical advisor.
In Proceedings of London Royal Society (1895), 58, 11-12.
Certain elements have the property of producing the same crystal form when in combination with an equal number of atoms of one or more common elements, and the elements, from his point of view, can be arranged in certain groups. For convenience I have called the elements belonging to the same group … isomorphous.
Originally published in 'Om Förhållandet emellan chemiska sammansättningen och krystallformen hos Arseniksyrade och Phosphorsyrade Salter', (On the Relation between the Chemical Composition and Crystal Form of Salts of Arsenic and Phosphoric Acids), Kungliga Svenska vetenskapsakademiens handlingar (1821), 4. In F. Szabadváry article on 'Eilhard Mitscherlich' in Charles Coulston Gillispie (ed.), Dictionary of Scientific Biography (1974), Vol. 9, 424; perhaps from J.R. Partington, A History of Chemistry, Vol. 4 (1964), 210.
Charles Babbage proposed to make an automaton chess-player which should register mechanically the number of games lost and gained in consequence of every sort of move. Thus, the longer the automaton went on playing game, the more experienced it would become by the accumulation of experimental results. Such a machine precisely represents the acquirement of experience by our nervous organization.
In ‘Experimental Legislation’, Popular Science (Apr 1880), 16, 754-5.
Chemistry is an art that has furnished the world with a great number of useful facts, and has thereby contributed to the improvement of many arts; but these facts lie scattered in many different books, involved in obscure terms, mixed with many falsehoods, and joined to a great deal of false philosophy; so that it is not great wonder that chemistry has not been so much studied as might have been expected with regard to so useful a branch of knowledge, and that many professors are themselves but very superficially acquainted with it. But it was particularly to be expected, that, since it has been taught in universities, the difficulties in this study should have been in some measure removed, that the art should have been put into form, and a system of it attempted—the scattered facts collected and arranged in a proper order. But this has not yet been done; chemistry has not yet been taught but upon a very narrow plan. The teachers of it have still confined themselves to the purposes of pharmacy and medicine, and that comprehends a small branch of chemistry; and even that, by being a single branch, could not by itself be tolerably explained.
John Thomson, An Account of the Life, Lectures and Writings of William Cullen, M.D. (1832), Vol. 1, 40.
Chemistry is one of those branches of human knowledge which has built itself upon methods and instruments by which truth can presumably be determined. It has survived and grown because all its precepts and principles can be re-tested at any time and anywhere. So long as it remained the mysterious alchemy by which a few devotees, by devious and dubious means, presumed to change baser metals into gold, it did not flourish, but when it dealt with the fact that 56 g. of fine iron, when heated with 32 g. of flowers of sulfur, generated extra heat and gave exactly 88 g. of an entirely new substance, then additional steps could be taken by anyone. Scientific research in chemistry, since the birth of the balance and the thermometer, has been a steady growth of test and observation. It has disclosed a finite number of elementary reagents composing an infinite universe, and it is devoted to their inter-reaction for the benefit of mankind.
Address upon receiving the Perkin Medal Award, 'The Big Things in Chemistry', The Journal of Industrial and Engineering Chemistry (Feb 1921), 13, No. 2, 163.
Chemistry works with an enormous number of substances, but cares only for some few of their properties; it is an extensive science. Physics on the other hand works with rather few substances, such as mercury, water, alcohol, glass, air, but analyses the experimental results very thoroughly; it is an intensive science. Physical chemistry is the child of these two sciences; it has inherited the extensive character from chemistry. Upon this depends its all-embracing feature, which has attracted so great admiration. But on the other hand it has its profound quantitative character from the science of physics.
In Theories of Solutions (1912), xix.
Come, see the north-wind’s masonry,
Out of an unseen quarry evermore
Furnished with tile, the fierce artificer
Curves his white bastions with projected roof
Round every windward stake, or tree, or door.
Speeding, the myriad-handed, his wild work
So fanciful, so savage, naught cares he
For number or proportion.
Out of an unseen quarry evermore
Furnished with tile, the fierce artificer
Curves his white bastions with projected roof
Round every windward stake, or tree, or door.
Speeding, the myriad-handed, his wild work
So fanciful, so savage, naught cares he
For number or proportion.
From 'The Snow-Storm', Poems of R. W. Emerson (1847), 65-66.
Compare the length of a moment with the period of ten thousand years; the first, however minuscule, does exist as a fraction of a second. But that number of years, or any multiple of it that you may name, cannot even be compared with a limitless extent of time, the reason being that comparisons can be drawn between finite things, but not between finite and infinite.
The Consolation of Philosophy [before 524], Book II, trans. P. G. Walsh (1999), 36.
Concerning the alchemist, Mamugnano, no one harbors doubts any longer about his daily experiments in changing quicksilver into gold. It was realized that his craft did not go beyond one pound of quicksilver… . Thus the belief is now held that his allegations to produce a number of millions have been a great fraud.
'Further Successes by Bragadini. From Vienna on the 26th day of January 1590'. As quoted in George Tennyson Matthews (ed.) News and Rumor in Renaissance Europe: The Fugger Newsletters (1959), 179. A handwritten collection of news reports (1568-1604) by the powerful banking and merchant house of Fugger in Ausburg.
Conservation is the foresighted utilization, preservation. And/or renewal of forest, waters, lands and minerals, for the greatest good of the greatest number for the longest time.
In Breaking New Ground (1947, 1998), 505.
De Morgan was explaining to an actuary what was the chance that a certain proportion of some group of people would at the end of a given time be alive; and quoted the actuarial formula, involving p [pi], which, in answer to a question, he explained stood for the ratio of the circumference of a circle to its diameter. His acquaintance, who had so far listened to the explanation with interest, interrupted him and exclaimed, “My dear friend, that must be a delusion, what can a circle have to do with the number of people alive at a given time?”
In Mathematical Recreations and Problems (1896), 180; See also De Morgan’s Budget of Paradoxes (1872), 172.
Defenders of the short-sighted men who in their greed and selfishness will, if permitted, rob our country of half its charm by their reckless extermination of all useful and beautiful wild things sometimes seek to champion them by saying the “the game belongs to the people.” So it does; and not merely to the people now alive, but to the unborn people. The “greatest good for the greatest number” applies to the number within the womb of time, compared to which those now alive form but an insignificant fraction. Our duty to the whole, including the unborn generations, bids us restrain an unprincipled present-day minority from wasting the heritage of these unborn generations. The movement for the conservation of wild life and the larger movement for the conservation of all our natural resources are essentially democratic in spirit, purpose, and method.
'Bird Reserves at the Mouth of the Mississippi', A Book-Lover's Holidays in the Open (1920), 300-301.
Defendit numerus: There is safety in numbers.
Latin proverb, first recorded in English about 1550. In James Roy Newman (ed.) The World of Mathematics (1956), Vol. 3, 1452.
Definition of Mathematics.—It has now become apparent that the traditional field of mathematics in the province of discrete and continuous number can only be separated from the general abstract theory of classes and relations by a wavering and indeterminate line. Of course a discussion as to the mere application of a word easily degenerates into the most fruitless logomachy. It is open to any one to use any word in any sense. But on the assumption that “mathematics” is to denote a science well marked out by its subject matter and its methods from other topics of thought, and that at least it is to include all topics habitually assigned to it, there is now no option but to employ “mathematics” in the general sense of the “science concerned with the logical deduction of consequences from the general premisses of all reasoning.”
In article 'Mathematics', Encyclopedia Britannica (1911, 11th ed.), Vol. 17, 880. In the 2006 DVD edition of the encyclopedia, the definition of mathematics is given as “The science of structure, order, and relation that has evolved from elemental practices of counting, measuring, and describing the shapes of objects.” [Premiss is a variant form of “premise”. —Webmaster]
Dissection … teaches us that the body of man is made up of certain kinds of material, so differing from each other in optical and other physical characters and so built up together as to give the body certain structural features. Chemical examination further teaches us that these kinds of material are composed of various chemical substances, a large number of which have this characteristic that they possess a considerable amount of potential energy capable of being set free, rendered actual, by oxidation or some other chemical change. Thus the body as a whole may, from a chemical point of view, be considered as a mass of various chemical substances, representing altogether a considerable capital of potential energy.
From Introduction to A Text Book of Physiology (1876, 1891), Book 1, 1.
Each nerve cell receives connections from other nerve cells at six sites called synapses. But here is an astonishing fact—there are about one million billion connections in the cortical sheet. If you were to count them, one connection (or synapse) per second, you would finish counting some thirty-two million years after you began. Another way of getting a feeling for the numbers of connections in this extraordinary structure is to consider that a large match-head’s worth of your brain contains about a billion connections. Notice that I only mention counting connections. If we consider how connections might be variously combined, the number would be hyperastronomical—on the order of ten followed by millions of zeros. (There are about ten followed by eighty zero’s worth of positively charged particles in the whole known universe!)
Bright and Brilliant Fire, On the Matters of the Mind (1992), 17.
Each new machine or technique, in a sense, changes all existing machines and techniques, by permitting us to put them together into new combinations. The number of possible combinations rises exponentially as the number of new machines or techniques rises
Future Shock (1970).
Each thing in the world has names or unnamed relations to everything else. Relations are infinite in number and kind. To be is to be related. It is evident that the understanding of relations is a major concern of all men and women. Are relations a concern of mathematics? They are so much its concern that mathematics is sometimes defined to be the science of relations.
In Mole Philosophy and Other Essays (1927), 94-95.
Ecology has not yet explicitly developed the kind of cohesive, simplifying generalizations exemplified by, say, the laws of physics. Nevertheless there are a number of generalizations that are already evident in what we now know about the ecosphere and that can be organized into a kind of informal set of laws of ecology.
In The Closing Circle: Nature, Man, and Technology (2014).
Edmund Wilson attacked the pedantry of scholarly editions of literary classics, which (he claimed) took the pleasure out of reading. Extensive footnotes … spoiled the reader’s pleasure in the text. Wilson’s friend Lewis Mumford had compared footnote numbers … to “barbed wire” keeping the reader at arm’s length.
In Academic Instincts (2001), 39.
Education is like a diamond with many facets: It includes the basic mastery of numbers and letters that give us access to the treasury of human knowledge, accumulated and refined through the ages; it includes technical and vocational training as well as instruction in science, higher mathematics, and humane letters.
In Proclamation 5463, for Education Day (19 Apr 1986). Collected in Public Papers of the Presidents of the United States: Ronald Reagan, 1986 (1988), 490.
ENGINEER, in the military art, an able expert man, who, by a perfect knowledge in mathematics, delineates upon paper, or marks upon the ground, all sorts of forts, and other works proper for offence and defence. He should understand the art of fortification, so as to be able, not only to discover the defects of a place, but to find a remedy proper for them; as also how to make an attack upon, as well as to defend, the place. Engineers are extremely necessary for these purposes: wherefore it is requisite that, besides being ingenious, they should be brave in proportion. When at a siege the engineers have narrowly surveyed the place, they are to make their report to the general, by acquainting him which part they judge the weakest, and where approaches may be made with most success. Their business is also to delineate the lines of circumvallation and contravallation, taking all the advantages of the ground; to mark out the trenches, places of arms, batteries, and lodgments, taking care that none of their works be flanked or discovered from the place. After making a faithful report to the general of what is a-doing, the engineers are to demand a sufficient number of workmen and utensils, and whatever else is necessary.
In Encyclopaedia Britannica or a Dictionary of Arts and Sciences (1771), Vol. 2, 497.
Engineering is quite different from science. Scientists try to understand nature. Engineers try to make things that do not exist in nature. Engineers stress invention. To embody an invention the engineer must put his idea in concrete terms, and design something that people can use. That something can be a device, a gadget, a material, a method, a computing program, an innovative experiment, a new solution to a problem, or an improvement on what is existing. Since a design has to be concrete, it must have its geometry, dimensions, and characteristic numbers. Almost all engineers working on new designs find that they do not have all the needed information. Most often, they are limited by insufficient scientific knowledge. Thus they study mathematics, physics, chemistry, biology and mechanics. Often they have to add to the sciences relevant to their profession. Thus engineering sciences are born.
Y.C. Fung and P. Tong, Classical and Computational Solid Mechanics (2001), 1.
England was nothing, compared to continental nations until she had become commercial … until about the middle of the last century, when a number of ingenious and inventive men, without apparent relation to each other, arose in various parts of the kingdom, succeeded in giving an immense impulse to all the branches of the national industry; the result of which has been a harvest of wealth and prosperity, perhaps without a parallel in the history of the world.
In Lives of the Engineers (1862, 1874), xvii.
Enormous numbers of people are taken in, or at least beguiled and fascinated, by what seems to me to be unbelievable hocum, and relatively few are concerned with or thrilled by the astounding—yet true—facts of science, as put forth in the pages of, say, Scientific American.
Metamagical Themas (1985), 93.
Euclidean mathematics assumes the completeness and invariability of mathematical forms; these forms it describes with appropriate accuracy and enumerates their inherent and related properties with perfect clearness, order, and completeness, that is, Euclidean mathematics operates on forms after the manner that anatomy operates on the dead body and its members. On the other hand, the mathematics of variable magnitudes—function theory or analysis—considers mathematical forms in their genesis. By writing the equation of the parabola, we express its law of generation, the law according to which the variable point moves. The path, produced before the eyes of the student by a point moving in accordance to this law, is the parabola.
If, then, Euclidean mathematics treats space and number forms after the manner in which anatomy treats the dead body, modern mathematics deals, as it were, with the living body, with growing and changing forms, and thus furnishes an insight, not only into nature as she is and appears, but also into nature as she generates and creates,—reveals her transition steps and in so doing creates a mind for and understanding of the laws of becoming. Thus modern mathematics bears the same relation to Euclidean mathematics that physiology or biology … bears to anatomy.
If, then, Euclidean mathematics treats space and number forms after the manner in which anatomy treats the dead body, modern mathematics deals, as it were, with the living body, with growing and changing forms, and thus furnishes an insight, not only into nature as she is and appears, but also into nature as she generates and creates,—reveals her transition steps and in so doing creates a mind for and understanding of the laws of becoming. Thus modern mathematics bears the same relation to Euclidean mathematics that physiology or biology … bears to anatomy.
In Die Mathematik die Fackelträgerin einer neuen Zeit (1889), 38. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 112-113.
Everything material which is the subject of knowledge has number, order, or position; and these are her first outlines for a sketch of the universe. If our feeble hands cannot follow out the details, still her part has been drawn with an unerring pen, and her work cannot be gainsaid. So wide is the range of mathematical sciences, so indefinitely may it extend beyond our actual powers of manipulation that at some moments we are inclined to fall down with even more than reverence before her majestic presence. But so strictly limited are her promises and powers, about so much that we might wish to know does she offer no information whatever, that at other moments we are fain to call her results but a vain thing, and to reject them as a stone where we had asked for bread. If one aspect of the subject encourages our hopes, so does the other tend to chasten our desires, and he is perhaps the wisest, and in the long run the happiest, among his fellows, who has learned not only this science, but also the larger lesson which it directly teaches, namely, to temper our aspirations to that which is possible, to moderate our desires to that which is attainable, to restrict our hopes to that of which accomplishment, if not immediately practicable, is at least distinctly within the range of conception.
From Presidential Address (Aug 1878) to the British Association, Dublin, published in the Report of the 48th Meeting of the British Association for the Advancement of Science (1878), 31.
Exercising the right of occasional suppression and slight modification, it is truly absurd to see how plastic a limited number of observations become, in the hands of men with preconceived ideas.
Meteorographica (1863), 5.
Exper. I. I made a small hole in a window-shutter, and covered it with a piece of thick paper, which I perforated with a fine needle. For greater convenience of observation I placed a small looking-glass without the window-shutter, in such a position as to reflect the sun's light, in a direction nearly horizontal, upon the opposite wall, and to cause the cone of diverging light to pass over a table on which were several little screens of card-paper. I brought into the sunbeam a slip of card, about one-thirtieth of an inch in breadth, and observed its shadow, either on the wall or on other cards held at different distances. Besides the fringes of colour on each side of the shadow, the shadow itself was divided by similar parallel fringes, of smaller dimensions, differing in number, according to the distance at which the shadow was observed, but leaving the middle of the shadow always white. Now these fringes were the joint effects of the portions of light passing on each side of the slip of card and inflected, or rather diffracted, into the shadow. For, a little screen being placed a few inches from the card, so as to receive either edge of the shadow on its margin, all the fringes which had before been observed in the shadow on the wall, immediately disappeared, although the light inflected on the other side was allowed to retain its course, and although this light must have undergone any modification that the proximity of the other edge of the slip of card might have been capable of occasioning... Nor was it for want of a sufficient intensity of light that one of the two portions was incapable of producing the fringes alone; for when they were both uninterrupted, the lines appeared, even if the intensity was reduced to one-tenth or one-twentieth.
'Experiments and Calculations Relative to Physical Optics' (read in 1803), Philosophical Transactions (1804), 94, 2-3.
Facts are of not much use, considered as facts. They bewilder by their number and their apparent incoherency. Let them be digested into theory, however, and brought into mutual harmony, and it is another matter.
From article 'Electro-magnetic Theory II', in The Electrician (16 Jan 1891), 26, No. 661, 331.
Facts are to the mind the same thing as food to the body. On the due digestion of facts depends the strength and wisdom of the one, just as vigor and health depend on the other. The wisest in council, the ablest in debate, and the most agreeable in the commerce of life is that man who has assimilated to his understanding the greatest number of facts.
Far from becoming discouraged, the philosopher should applaud nature, even when she appears miserly of herself or overly mysterious, and should feel pleased that as he lifts one part of her veil, she allows him to glimpse an immense number of other objects, all worthy of investigation. For what we already know should allow us to judge of what we will be able to know; the human mind has no frontiers, it extends proportionately as the universe displays itself; man, then, can and must attempt all, and he needs only time in order to know all. By multiplying his observations, he could even see and foresee all phenomena, all of nature's occurrences, with as much truth and certainty as if he were deducing them directly from causes. And what more excusable or even more noble enthusiasm could there be than that of believing man capable of recognizing all the powers, and discovering through his investigations all the secrets, of nature!
'Des Mulets', Oeuvres Philosophiques, ed. Jean Piveteau (1954), 414. Quoted in Jacques Roger, The Life Sciences in Eighteenth-Century French Thought, ed. Keith R. Benson and trans. Robert Ellrich (1997), 458.
Finally, from what we now know about the cosmos, to think that all this was created for just one species among the tens of millions of species who live on one planet circling one of a couple of hundred billion stars that are located in one galaxy among hundreds of billions of galaxies, all of which are in one universe among perhaps an infinite number of universes all nestled within a grand cosmic multiverse, is provincially insular and anthropocentrically blinkered. Which is more likely? That the universe was designed just for us, or that we see the universe as having been designed just for us?
…...
For chemistry is no science form’d à priori; ’tis no production of the human mind, framed by reasoning and deduction: it took its rise from a number of experiments casually made, without any expectation of what follow’d; and was only reduced into an art or system, by collecting and comparing the effects of such unpremeditated experiments, and observing the uniform tendency thereof. So far, then, as a number of experimenters agree to establish any undoubted truth; so far they may be consider'd as constituting the theory of chemistry.
From 'The Author's Preface', in A New Method of Chemistry (1727), vi.
For example, there are numbers of chemists who occupy themselves exclusively with the study of dyestuffs. They discover facts that are useful to scientific chemistry; but they do not rank as genuine scientific men. The genuine scientific chemist cares just as much to learn about erbium—the extreme rarity of which renders it commercially unimportant—as he does about iron. He is more eager to learn about erbium if the knowledge of it would do more to complete his conception of the Periodic Law, which expresses the mutual relations of the elements.
From 'Lessons from the History of Science: The Scientific Attitude' (c.1896), in Collected Papers (1931), Vol. 1, 20.
For it is not number of Experiments, but weight to be regarded; & where one will do, what need many?
In 'Mr. Newton's Answer to the Precedent Letter, Sent to the Publisher', Philosophical Transactions (1665-1678), Vol. 11 (25 Sep 1676), No. 128, 703.
For the harmony of the world is made manifest in Form and Number, and the heart and soul and all the poetry of Natural Philosophy are embodied in the concept of mathematical beauty.
In 'Epilogue', On Growth and Form (1917), 778-9.
For us, an atom shall be a small, spherical, homogeneous body or an essentially indivisible, material point, whereas a molecule shall be a separate group of atoms in any number and of any nature.
Annales de Chimie 1833, 52, 133. Trans. W. H. Brock.
From a mathematical standpoint it is possible to have infinite space. In a mathematical sense space is manifoldness, or combinations of numbers. Physical space is known as the 3-dimension system. There is the 4-dimension system, the 10-dimension system.
As quoted in 'Electricity Will Keep The World From Freezing Up', New York Times (12 Nov 1911), SM4.
Gauss once said, “Mathematics is the queen of the sciences and number theory the queen of mathematics.” If this is true we may add that the Disquisitions is the Magna Charter of number theory.
In Allgemeine Deutsche Biographie (1878, 8, 435. As cited and translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 158.
Geologists have not been slow to admit that they were in error in assuming that they had an eternity of past time for the evolution of the earth’s history. They have frankly acknowledged the validity of the physical arguments which go to place more or less definite limits to the antiquity of the earth. They were, on the whole, disposed to acquiesce in the allowance of 100 millions of years granted to them by Lord Kelvin, for the transaction of the whole of the long cycles of geological history. But the physicists have been insatiable and inexorable. As remorseless as Lear’s daughters, they have cut down their grant of years by successive slices, until some of them have brought the number to something less than ten millions. In vain have the geologists protested that there must somewhere be a flaw in a line of argument which tends to results so entirely at variance with the strong evidence for a higher antiquity, furnished not only by the geological record, but by the existing races of plants and animals. They have insisted that this evidence is not mere theory or imagination, but is drawn from a multitude of facts which become hopelessly unintelligible unless sufficient time is admitted for the evolution of geological history. They have not been able to disapprove the arguments of the physicists, but they have contended that the physicists have simply ignored the geological arguments as of no account in the discussion.
'Twenty-five years of Geological Progress in Britain', Nature, 1895, 51, 369.
Having discovered … by observation and comparison that certain objects agree in certain respects, we generalise the qualities in which they coincide,—that is, from a certain number of individual instances we infer a general law; we perform an act of Induction. This induction is erroneously viewed as analytic; it is purely a synthetic process.
In Lecture VI of his Biennial Course, by William Hamilton and Henry L. Mansel (ed.) and John Veitch (ed.), Metaphysics (1860), Vol. 1, 101.
He telleth the number of stars; he calleth them all by their names.
— Bible
Psalms 147:4 in The Holy Bible (1746), 549.
Hence, a generative grammar must be a system of rules that can iterate to generate an indefinitely large number of structures. This system of rules can be analyzed into the three major components of a generative grammar: the syntactic, phonological, and semantic components... the syntactic component of a grammar must specify, for each sentence, a deep structure that determines its semantic interpretation and a surface structure that determines its phonetic interpretation. The first of these is interpreted by the semantic component; the second, by the phonological component.
Aspects of the Theory of Syntax (1965), 15-6.
Here I am at the limit which God and nature has assigned to my individuality. I am compelled to depend upon word, language and image in the most precise sense, and am wholly unable to operate in any manner whatever with symbols and numbers which are easily intelligible to the most highly gifted minds.
In Letter to Naumann (1826), in Vogel, Goethe's Selbstzeugnisse (1903), 56.
Here I shall present, without using Analysis [mathematics], the principles and general results of the Théorie, applying them to the most important questions of life, which are indeed, for the most part, only problems in probability. One may even say, strictly speaking, that almost all our knowledge is only probable; and in the small number of things that we are able to know with certainty, in the mathematical sciences themselves, the principal means of arriving at the truth—induction and analogy—are based on probabilities, so that the whole system of human knowledge is tied up with the theory set out in this essay.
Philosophical Essay on Probabilities (1814), 5th edition (1825), trans. Andrew I. Dale (1995), 1.
His [Henry Cavendish’s] Theory of the Universe seems to have been, that it consisted solely of a multitude of objects which could be weighed, numbered, and measured; and the vocation to which he considered himself called was, to weigh, number and measure as many of those objects as his allotted three-score years and ten would permit. This conviction biased all his doings, alike his great scientific enterprises, and the petty details of his daily life.
In George Wilson, The Life of the Honourable Henry Cavendish: Including the Abstracts of his Important Scientific Papers (1851), 186.
Historical theories are, after all, intellectual apple carts. They are quite likely to be upset. Nor should it be forgotten that they tend to attract, when they gain ascendancy, a fair number of apple-polishers
'Books of the Times'. New York Times (9 Dec 1965), 45.
Homo sapiens is a compulsive communicator. Look at the number of people you see walking around talking on mobile phones. We seem to have an infinite capacity for communicating and being communicated with. I’m not sure how admirable it is, but it certainly demonstrates that we are social organisms.
From interview with Michael Bond, 'It’s a Wonderful Life', New Scientist (14 Dec 2002), 176, No. 2373, 48.
Houston, that may have seemed like a very long final phase. The autotargeting was taking us right into a... crater, with a large number of big boulders and rocks ... and it required... flying manually over the rock field to find a reasonably good area.
…...
How does it arise that, while the statements of geologists that other organic bodies existed millions of years ago are tacitly accepted, their conclusions as to man having existed many thousands of years ago should be received with hesitation by some geologists, and be altogether repudiated by a not inconsiderable number among the other educated classes of society?
'Anniversary Address of the Geological Society of London', Proceedings of the Geological Society of London (1861), 17, lxvii.
I accepted the Copernican position several years ago and discovered from thence the causes of many natural effects which are doubtless inexplicable by the current theories. I have written up many reasons and refutations on the subject, but I have not dared until now to bring them into the open, being warned by the fortunes of Copernicus himself, our master, who procured for himself immortal fame among a few but stepped down among the great crowd (for this is how foolish people are to be numbered), only to be derided and dishonoured. I would dare publish my thoughts if there were many like you; but since there are not, I shall forbear.
Letter to Johannes Kepler, 4 Aug 1597. Quoted in G. de Santillana, Crime of Galileo (1955), 11.
I am ill at these numbers.
In Hamlet (1603), Act 2, Scene 2. [Note: Not for arithmetic. The numbers relate to the number of syllables in each line to write poetry; he is a bad poet. —Webmaster]
I am more and more convinced that the ant colony is not so much composed of separate individuals as that the colony is a sort of individual, and each ant like a loose cell in it. Our own blood stream, for instance, contains hosts of white corpuscles which differ little from free-swimming amoebae. When bacteria invade the blood stream, the white corpuscles, like the ants defending the nest, are drawn mechanically to the infected spot, and will die defending the human cell colony. I admit that the comparison is imperfect, but the attempt to liken the individual human warrior to the individual ant in battle is even more inaccurate and misleading. The colony of ants with its component numbers stands half way, as a mechanical, intuitive, and psychical phenomenon, between our bodies as a collection of cells with separate functions and our armies made up of obedient privates. Until one learns both to deny real individual initiative to the single ant, and at the same time to divorce one's mind from the persuasion that the colony has a headquarters which directs activity … one can make nothing but pretty fallacies out of the polity of the ant heap.
In An Almanac for Moderns (1935), 121
I am of opinion, then, ... that, if there is any circumstance thoroughly established in geology, it is, that the crust of our globe has been subjected to a great and sudden revolution, the epoch of which cannot be dated much farther back than five or six thousand years ago; that this revolution had buried all the countries which were before inhabited by men and by the other animals that are now best known; that the same revolution had laid dry the bed of the last ocean, which now forms all the countries at present inhabited; that the small number of individuals of men and other animals that escaped from the effects of that great revolution, have since propagated and spread over the lands then newly laid dry; and consequently, that the human race has only resumed a progressive state of improvement since that epoch, by forming established societies, raising monuments, collecting natural facts, and constructing systems of science and of learning.
'Preliminary discourse', to Recherches sur les Ossemens Fossiles (1812), trans. R. Kerr Essay on the Theory of the Earth (1813), 171-2.
I am one of the unpraised, unrewarded millions without whom Statistics would be a bankrupt science. It is we who are born, who marry, who die, in constant ratios. It is we who are born, who marry, who die,
in constant ratios; who regularly lose so many umbrellas, post just so many unaddressed letters every year. And there are enthusiasts among us who, without the least thought of their own convenience, allow omnibuses to run over them; or throw themselves month by month, in fixed numbers, from the London bridges.
In 'Where Do I Come In', Trivia (1917,1921), 106.
I am particularly concerned to determine the probability of causes and results, as exhibited in events that occur in large numbers, and to investigate the laws according to which that probability approaches a limit in proportion to the repetition of events. That investigation deserves the attention of mathematicians because of the analysis required. It is primarily there that the approximation of formulas that are functions of large numbers has its most important applications. The investigation will benefit observers in identifying the mean to be chosen among the results of their observations and the probability of the errors still to be apprehended. Lastly, the investigation is one that deserves the attention of philosophers in showing how in the final analysis there is a regularity underlying the very things that seem to us to pertain entirely to chance, and in unveiling the hidden but constant causes on which that regularity depends. It is on the regularity of the main outcomes of events taken in large numbers that various institutions depend, such as annuities, tontines, and insurance policies. Questions about those subjects, as well as about inoculation with vaccine and decisions of electoral assemblies, present no further difficulty in the light of my theory. I limit myself here to resolving the most general of them, but the importance of these concerns in civil life, the moral considerations that complicate them, and the voluminous data that they presuppose require a separate work.
Philosophical Essay on Probabilities (1825), trans. Andrew I. Dale (1995), Introduction.
I asked Fermi whether he was not impressed by the agreement between our calculated numbers and his measured numbers. He replied, “How many arbitrary parameters did you use for your calculations?" I thought for a moment about our cut-off procedures and said, “Four." He said, “I remember my friend Johnny von Neumann used to say, with four parameters I can fit an elephant, and with five I can make him wiggle his trunk.” With that, the conversation was over.
As given in 'A Meeting with Enrico Fermi', Nature (22 Jan 2004), 427, 297. As quoted in Steven A. Frank, Dynamics of Cancer: Incidence, Inheritance, and Evolution (2007), 87. Von Neumann meant nobody need be impressed when a complex model fits a data set well, because if manipulated with enough flexible parameters, any data set can be fitted to an incorrect model, even one that plots a curve on a graph shaped like an elephant!
I believe that the useful methods of mathematics are easily to be learned by quite young persons, just as languages are easily learned in youth. What a wondrous philosophy and history underlie the use of almost every word in every language—yet the child learns to use the word unconsciously. No doubt when such a word was first invented it was studied over and lectured upon, just as one might lecture now upon the idea of a rate, or the use of Cartesian co-ordinates, and we may depend upon it that children of the future will use the idea of the calculus, and use squared paper as readily as they now cipher. … When Egyptian and Chaldean philosophers spent years in difficult calculations, which would now be thought easy by young children, doubtless they had the same notions of the depth of their knowledge that Sir William Thomson might now have of his. How is it, then, that Thomson gained his immense knowledge in the time taken by a Chaldean philosopher to acquire a simple knowledge of arithmetic? The reason is plain. Thomson, when a child, was taught in a few years more than all that was known three thousand years ago of the properties of numbers. When it is found essential to a boy’s future that machinery should be given to his brain, it is given to him; he is taught to use it, and his bright memory makes the use of it a second nature to him; but it is not till after-life that he makes a close investigation of what there actually is in his brain which has enabled him to do so much. It is taken because the child has much faith. In after years he will accept nothing without careful consideration. The machinery given to the brain of children is getting more and more complicated as time goes on; but there is really no reason why it should not be taken in as early, and used as readily, as were the axioms of childish education in ancient Chaldea.
In Teaching of Mathematics (1902), 14.
I believe there are
15,747,724,136,275,002,577,605,653,961,181,555,468,044,717,
914,527,116,709,366,231,025,076,185,631,031,296
protons in the universe, and the same number of electrons.
15,747,724,136,275,002,577,605,653,961,181,555,468,044,717,
914,527,116,709,366,231,025,076,185,631,031,296
protons in the universe, and the same number of electrons.
From Tarner Lecture (1938), 'The Physical Universe', in The Philosophy of Physical Science (1939, 2012), 170. Note: the number is 136 x 2256.
I can see him [Sylvester] now, with his white beard and few locks of gray hair, his forehead wrinkled o’er with thoughts, writing rapidly his figures and formulae on the board, sometimes explaining as he wrote, while we, his listeners, caught the reflected sounds from the board. But stop, something is not right, he pauses, his hand goes to his forehead to help his thought, he goes over the work again, emphasizes the leading points, and finally discovers his difficulty. Perhaps it is some error in his figures, perhaps an oversight in the reasoning. Sometimes, however, the difficulty is not elucidated, and then there is not much to the rest of the lecture. But at the next lecture we would hear of some new discovery that was the outcome of that difficulty, and of some article for the Journal, which he had begun. If a text-book had been taken up at the beginning, with the intention of following it, that text-book was most likely doomed to oblivion for the rest of the term, or until the class had been made listeners to every new thought and principle that had sprung from the laboratory of his mind, in consequence of that first difficulty. Other difficulties would soon appear, so that no text-book could last more than half of the term. In this way his class listened to almost all of the work that subsequently appeared in the Journal. It seemed to be the quality of his mind that he must adhere to one subject. He would think about it, talk about it to his class, and finally write about it for the Journal. The merest accident might start him, but once started, every moment, every thought was given to it, and, as much as possible, he read what others had done in the same direction; but this last seemed to be his real point; he could not read without finding difficulties in the way of understanding the author. Thus, often his own work reproduced what had been done by others, and he did not find it out until too late.
A notable example of this is in his theory of cyclotomic functions, which he had reproduced in several foreign journals, only to find that he had been greatly anticipated by foreign authors. It was manifest, one of the critics said, that the learned professor had not read Rummer’s elementary results in the theory of ideal primes. Yet Professor Smith’s report on the theory of numbers, which contained a full synopsis of Kummer’s theory, was Professor Sylvester’s constant companion.
This weakness of Professor Sylvester, in not being able to read what others had done, is perhaps a concomitant of his peculiar genius. Other minds could pass over little difficulties and not be troubled by them, and so go on to a final understanding of the results of the author. But not so with him. A difficulty, however small, worried him, and he was sure to have difficulties until the subject had been worked over in his own way, to correspond with his own mode of thought. To read the work of others, meant therefore to him an almost independent development of it. Like the man whose pleasure in life is to pioneer the way for society into the forests, his rugged mind could derive satisfaction only in hewing out its own paths; and only when his efforts brought him into the uncleared fields of mathematics did he find his place in the Universe.
A notable example of this is in his theory of cyclotomic functions, which he had reproduced in several foreign journals, only to find that he had been greatly anticipated by foreign authors. It was manifest, one of the critics said, that the learned professor had not read Rummer’s elementary results in the theory of ideal primes. Yet Professor Smith’s report on the theory of numbers, which contained a full synopsis of Kummer’s theory, was Professor Sylvester’s constant companion.
This weakness of Professor Sylvester, in not being able to read what others had done, is perhaps a concomitant of his peculiar genius. Other minds could pass over little difficulties and not be troubled by them, and so go on to a final understanding of the results of the author. But not so with him. A difficulty, however small, worried him, and he was sure to have difficulties until the subject had been worked over in his own way, to correspond with his own mode of thought. To read the work of others, meant therefore to him an almost independent development of it. Like the man whose pleasure in life is to pioneer the way for society into the forests, his rugged mind could derive satisfaction only in hewing out its own paths; and only when his efforts brought him into the uncleared fields of mathematics did he find his place in the Universe.
In Florian Cajori, Teaching and History of Mathematics in the United States (1890), 266-267.
I conceived and developed a new geometry of nature and implemented its use in a number of diverse fields. It describes many of the irregular and fragmented patterns around us, and leads to full-fledged theories, by identifying a family of shapes I call fractals.
The Fractal Geometry of Nature (1977, 1983), Introduction, xiii.
I confess that Fermat’s Theorem as an isolated proposition has very little interest for me, for a multitude of such theorems can easily be set up, which one could neither prove nor disprove. But I have been stimulated by it to bring our again several old ideas for a great extension of the theory of numbers. Of course, this theory belongs to the things where one cannot predict to what extent one will succeed in reaching obscurely hovering distant goals. A happy star must also rule, and my situation and so manifold distracting affairs of course do not permit me to pursue such meditations as in the happy years 1796-1798 when I created the principal topics of my Disquisitiones arithmeticae. But I am convinced that if good fortune should do more than I expect, and make me successful in some advances in that theory, even the Fermat theorem will appear in it only as one of the least interesting corollaries.
In reply to Olbers' attempt in 1816 to entice him to work on Fermat's Theorem. The hope Gauss expressed for his success was never realised.
In reply to Olbers' attempt in 1816 to entice him to work on Fermat's Theorem. The hope Gauss expressed for his success was never realised.
Letter to Heinrich Olbers (21 Mar 1816). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 413.
I count Maxwell and Einstein, Eddington and Dirac, among “real” mathematicians. The great modern achievements of applied mathematics have been in relativity and quantum mechanics, and these subjects are at present at any rate, almost as “useless” as the theory of numbers.
In A Mathematician's Apology (1940, 2012), 131.
I do hate sums. There is no greater mistake than to call arithmetic an exact science. There are permutations and aberrations discernible to minds entirely noble like mine; subtle variations which ordinary accountants fail to discover; hidden laws of number which it requires a mind like mine to perceive. For instance, if you add a sum from the bottom up, and then from the top down, the result is always different. Again if you multiply a number by another number before you have had your tea, and then again after, the product will be different. It is also remarkable that the Post-tea product is more likely to agree with other people’s calculations than the Pre-tea result.
Letter to Mrs Arthur Severn (Jul 1878), collected in The Letters of a Noble Woman (Mrs. La Touche of Harristown) (1908), 50. Also in 'Gleanings Far and Near', Mathematical Gazette (May 1924), 12, 95.
I had formally advanced, in my first Memoirs upon the formation of water, that it was decomposed in a great number of chemical experiments, and particularly during the vinous fermentation. I then supposed that water exists ready formed in sugar, though I am now convinced that sugar only contains the elements proper for composing it. It may be readily conceived, that it must have cost me a good deal to abandon my first notions, but by several years reflection, and after a great number of experiments and observations upon vegetable substances, I have fixed my ideas as above.
In The Elements of Chemistry (1790), Pt. 1, Chap. 13, 139-140.
I had made considerable advance ... in calculations on my favourite numerical lunar theory, when I discovered that, under the heavy pressure of unusual matters (two transits of Venus and some eclipses) I had committed a grievous error in the first stage of giving numerical value to my theory. My spirit in the work was broken, and I have never heartily proceeded with it since.
[Concerning his calculations on the orbital motion of the Moon.]
[Concerning his calculations on the orbital motion of the Moon.]
Private note (29 Sep 1890). In George Biddell Airy and Wilfrid Airy (ed.), Autobiography of Sir George Biddell Airy (1896), 350.
I have divers times examined the same matter (human semen) from a healthy man... not from a sick man... nor spoiled by keeping... for a long time and not liquefied after the lapse of some time... but immediately after ejaculation before six beats of the pulse had intervened; and I have seen so great a number of living animalcules... in it, that sometimes more than a thousand were moving about in an amount of material the size of a grain of sand... I saw this vast number of animalcules not all through the semen, but only in the liquid matter adhering to the thicker part.
Letter to W. Brouncker, President of the Royal Society, undated, Nov 1677. In The Collected Letters of Antoni van Leeuwenhoek (1957), Vol. 2, 283-4.
I have little patience with scientists who take a board of wood, look for its thinnest part and drill a great number of holes where drilling is easy.
P. Frank in 'Einstein's Philosophy of Science', Reviews of Modern Physics (1949).
I have often admired the mystical way of Pythagoras, and the secret magick of numbers.
In Religio Medici (1642, 1754), pt. 1, sec. 12, 28.
I have often thought that an interesting essay might be written on the influence of race on the selection of mathematical methods. methods. The Semitic races had a special genius for arithmetic
and algebra, but as far as I know have never produced a single geometrician of any eminence. The Greeks on the other hand adopted a geometrical procedure wherever it was possible, and they even treated arithmetic as a branch of geometry by means of the device of representing numbers by lines.
In A History of the Study of Mathematics at Cambridge (1889), 123
I have said that mathematics is the oldest of the sciences; a glance at its more recent history will show that it has the energy of perpetual youth. The output of contributions to the advance of the science during the last century and more has been so enormous that it is difficult to say whether pride in the greatness of achievement in this subject, or despair at his inability to cope with the multiplicity of its detailed developments, should be the dominant feeling of the mathematician. Few people outside of the small circle of mathematical specialists have any idea of the vast growth of mathematical literature. The Royal Society Catalogue contains a list of nearly thirty- nine thousand papers on subjects of Pure Mathematics alone, which have appeared in seven hundred serials during the nineteenth century. This represents only a portion of the total output, the very large number of treatises, dissertations, and monographs published during the century being omitted.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 285.
I have tried to avoid long numerical computations, thereby following Riemann’s postulate that proofs should be given through ideas and not voluminous computations.
In Report on Number Theory (1897). As given in epigraph, without citation, in Eberhard Zeidler and Juergen Quandt (trans.), Nonlinear Functional Analysis and its Applications: IV: Applications to Mathematical Physics (2013), 448.
I have written many direct and indirect arguments for the Copernican view, but until now I have not dared to publish them, alarmed by the fate of Copernicus himself, our master. He has won for himself undying fame in the eyes of a few, but he has been mocked and hooted at by an infinite multitude (for so large is the number of fools). I would dare to come forward publicly with my ideas if there were more people of your [Johannes Kepler’s] way of thinking. As this is not the case, I shall refrain.
Letter to Kepler (4 Aug 1597). In James Bruce Ross (ed.) and Mary Martin (ed., trans.), 'Comrades in the Pursuit of Truth', The Portable Renaissance Reader (1953, 1981), 597-599. As quoted and cited in Merry E. Wiesner, Early Modern Europe, 1450-1789 (2013), 377.
I hope that in 50 years we will know the answer to this challenging question: are the laws of physics unique and was our big bang the only one? … According to some speculations the number of distinct varieties of space—each the arena for a universe with its own laws—could exceed the total number of atoms in all the galaxies we see. … So do we live in the aftermath of one big bang among many, just as our solar system is merely one of many planetary systems in our galaxy? (2006)
In 'Martin Rees Forecasts the Future', New Scientist (18 Nov 2006), No. 2578.
I imagined in the beginning, that a few experiments would determine the problem; but experience soon convinced me, that a very great number indeed were necessary before such an art could be brought to any tolerable degree of perfection.
Upon pursuing the ''
Upon pursuing the ''
Preface to An Essay on Combustion with a View to a New Art of Dyeing and Painting (1794), iii. In Marilyn Bailey Ogilvie and Joy Dorothy Harvey, The Biographical Dictionary of Women in Science (2000), 478.
I must … explain how I was led to concern myself with the pathogenic protozoa. … I was sent to Algeria and put in charge of a department of the hospital at Bone. A large number of my patients had malarial fevers and I was naturally led to study these fevers of which I had only seen rare and benign forms in France.
From Nobel Lecture (11 Dec 1907), 'Protozoa as Causes of Diseases', collected in Nobel Lectures, Physiology or Medicine 1901-1921 (1967, 1999), 264.
I never could do anything with figures, never had any talent for mathematics, never accomplished anything in my efforts at that rugged study, and to-day the only mathematics I know is multiplication, and the minute I get away up in that, as soon as I reach nine times seven— [He lapsed into deep thought, trying to figure nine times seven. Mr. McKelway whispered the answer to him.] I’ve got it now. It’s eighty-four. Well, I can get that far all right with a little hesitation. After that I am uncertain, and I can’t manage a statistic.
Speech at the New York Association for Promoting the Interests of the Blind (29 Mar 1906). In Mark Twain and William Dean Howells (ed.), Mark Twain’s Speeches? (1910), 323.
I observed on most collected stones the imprints of innumerable plant fragments which were so different from those which are growing in the Lyonnais, in the nearby provinces, and even in the rest of France, that I felt like collecting plants in a new world… The number of these leaves, the way they separated easily, and the great variety of plants whose imprints I saw, appeared to me just as many volumes of botany representing in the same quarry the oldest library of the world.
In 'Examen des causes des Impressions des Plantes marquees sur certaines Pierres des environs de Saint-Chaumont dans le Lionnais', Memoires de l’ Academie Royale des Sciences (1718), 364, as trans. by Albert V. and Marguerite Carozzi.
I picture the vast realm of the sciences as an immense landscape scattered with patches of dark and light. The goal towards which we must work is either to extend the boundaries of the patches of light, or to increase their number. One of these tasks falls to the creative genius; the other requires a sort of sagacity combined with perfectionism.
Thoughts on the Interpretation of Nature and Other Philosophical Works (1753/4), ed. D. Adams (1999), Section XIV, 42.
I recall my own emotions: I had just been initiated into the mysteries of the complex number. I remember my bewilderment: here were magnitudes patently impossible and yet susceptible of manipulations which lead to concrete results. It was a feeling of dissatisfaction, of restlessness, a desire to fill these illusory creatures, these empty symbols, with substance. Then I was taught to interpret these beings in a concrete geometrical way. There came then an immediate feeling of relief, as though I had solved an enigma, as though a ghost which had been causing me apprehension turned out to be no ghost at all, but a familiar part of my environment.
In Tobias Dantzig and Joseph Mazur (ed.), 'The Two Realities', Number: The Language of Science (1930, ed. by Joseph Mazur 2007), 254.
I regarded as quite useless the reading of large treatises of pure analysis: too large a number of methods pass at once before the eyes. It is in the works of application that one must study them; one judges their utility there and appraises the manner of making use of them.
As reported by J. F. Maurice in Moniteur Universel (1814), 228.
I remember once going to see him when he was lying ill at Putney. I had ridden in taxi cab number 1729 and remarked that the number seemed to me rather a dull one, and that I hoped it was not an unfavorable omen. “No,” he replied, “it is a very interesting number; it is the smallest number expressible as the sum of two cubes in two different ways.”
Quoted in G.H. Hardy, Ramanujan; Twelve Lectures on Subjects Suggested by his Life and Work (1940, reprint 1999), 12.
I remember one occasion when I tried to add a little seasoning to a review, but I wasn’t allowed to. The paper was by Dorothy Maharam, and it was a perfectly sound contribution to abstract measure theory. The domains of the underlying measures were not sets but elements of more general Boolean algebras, and their range consisted not of positive numbers but of certain abstract equivalence classes. My proposed first sentence was: “The author discusses valueless measures in pointless spaces.”
In I Want to be a Mathematician: An Automathography (1985), 120.
I should like to call the number of atom groups, with which an elementary atom coordinates … to form a complex radical, the coordination number of the atom in question … We must differentiate between valence number and coordination number. The valence number indicates the maximum number of monovalent atoms which can be bound directly to the atom in question without the participation of other elementary atoms … Perhaps this concept [of coordination number] is destined to serve as a basis for the theory of the constitution of inorganic compounds, just as valence theory formed the basis for the constitutional theory of carbon compounds.
In 'Beitrag zur Konstitution anorganischer Verbindungen', Zeitschrift fur anorganische Chemie, (1893), 3, 267-330. Translated in George G. Kauffman (ed.), Classics in Coordination Chemistry: Part I: The Selected Papers of Alfred Werner (1968), 84-87.
I should like to compare this rearrangement which the proteins undergo in the animal or vegetable organism to the making up of a railroad train. In their passage through the body parts of the whole may be left behind, and here and there new parts added on. In order to understand fully the change we must remember that the proteins are composed of Bausteine united in very different ways. Some of them contain Bausteine of many kinds. The multiplicity of the proteins is determined by many causes, first through the differences in the nature of the constituent Bausteine; and secondly, through differences in the arrangement of them. The number of Bausteine which may take part in the formation of the proteins is about as large as the number of letters in the alphabet. When we consider that through the combination of letters an infinitely large number of thoughts may be expressed, we can understand how vast a number of the properties of the organism may be recorded in the small space which is occupied by the protein molecules. It enables us to understand how it is possible for the proteins of the sex-cells to contain, to a certain extent, a complete description of the species and even of the individual. We may also comprehend how great and important the task is to determine the structure of the proteins, and why the biochemist has devoted himself with so much industry to their analysis.
'The Chemical Composition of the Cell', The Harvey Lectures (1911), 7, 45.
I think if we had not repaired the telescope, it would have been the end of the space station, because space station requires a huge number of space walks. I think it was fair to use the Hubble space telescope as a test case for space walks, to say, “Can NASA really do what they say they can do up there?”
Interview (22 May 1997). On Academy of Achievement website.
I think it’s going to be great if people can buy a ticket to fly up and see black sky and the stars. I’d like to do it myself - but probably after it has flown a serious number of times first!
…...
I think, and I am not the only one who does, that it is important never to introduce any conception which may not be completely defined by a finite number of words. Whatever may be the remedy adopted, we can promise ourselves the joy of the physician called in to follow a beautiful pathological case [beau cas pathologique].
From address read at the general session of the Fourth International Congress of Mathematicians in Rome (10 Apr 1908). As translated in 'The Future of Mathematics: by Henri Poincaré', General Appendix, Annual Report of the Boars of Regents of The Smithsonian Institution: For the Year Ending June 1909 (1910), 140.
I trust ... I have succeeded in convincing you that modern chemistry is not, as it has so long appeared, an ever-growing accumulation of isolated facts, as impossible for a single intellect to co-ordinate as for a single memory to grasp.
The intricate formulae that hang upon these walls, and the boundless variety of phenomena they illustrate, are beginning to be for us as a labyrinth once impassable, but to which we have at length discovered the clue. A sense of mastery and power succeeds in our minds to the sort of weary despair with which we at first contemplated their formidable array. For now, by the aid of a few general principles, we find ourselves able to unravel the complexities of these formulae, to marshal the compounds which they represent in orderly series; nay, even to multiply their numbers at our will, and in a great measure to forecast their nature ere we have called them into existence. It is the great movement of modern chemistry that we have thus, for an hour, seen passing before us. It is a movement as of light spreading itself over a waste of obscurity, as of law diffusing order throughout a wilderness of confusion, and there is surely in its contemplation something of the pleasure which attends the spectacle of a beautiful daybreak, something of the grandeur belonging to the conception of a world created out of chaos.
The intricate formulae that hang upon these walls, and the boundless variety of phenomena they illustrate, are beginning to be for us as a labyrinth once impassable, but to which we have at length discovered the clue. A sense of mastery and power succeeds in our minds to the sort of weary despair with which we at first contemplated their formidable array. For now, by the aid of a few general principles, we find ourselves able to unravel the complexities of these formulae, to marshal the compounds which they represent in orderly series; nay, even to multiply their numbers at our will, and in a great measure to forecast their nature ere we have called them into existence. It is the great movement of modern chemistry that we have thus, for an hour, seen passing before us. It is a movement as of light spreading itself over a waste of obscurity, as of law diffusing order throughout a wilderness of confusion, and there is surely in its contemplation something of the pleasure which attends the spectacle of a beautiful daybreak, something of the grandeur belonging to the conception of a world created out of chaos.
Concluding remark for paper presented at the Friday Discourse of the the Royal Institution (7 Apr 1865). 'On the Combining Power of Atoms', Proceedings of the Royal Institution (1865), 4, No. 42, 416.
I want to put in something about Bernoulli’s numbers, in one of my Notes, as an example of how the implicit function may be worked out by the engine, without having been worked out by human head & hands first. Give me the necessary data & formulae.
Lovelace Papers, Bodleian Library, Oxford University, 42, folio 12 (6 Feb 1841). As quoted and cited in Dorothy Stein (ed.), 'This First Child of Mine', Ada: A Life and a Legacy (1985), 106-107.
I was suffering from a sharp attack of intermittent fever, and every day during the cold and succeeding hot fits had to lie down for several hours, during which time I had nothing to do but to think over any subjects then particularly interesting me. One day something brought to my recollection Malthus's 'Principles of Population', which I had read about twelve years before. I thought of his clear exposition of 'the positive checks to increase'—disease, accidents, war, and famine—which keep down the population of savage races to so much lower an average than that of more civilized peoples. It then occurred to me that these causes or their equivalents are continually acting in the case of animals also; and as animals usually breed much more rapidly than does mankind, the destruction every year from these causes must be enormous in order to keep down the numbers of each species, since they evidently do not increase regularly from year to year, as otherwise the world would long ago have been densely crowded with those that breed most quickly. Vaguely thinking over the enormous and constant destruction which this implied, it occurred to me to ask the question, Why do some die and some live? The answer was clearly, that on the whole the best fitted live. From the effects of disease the most healthy escaped; from enemies, the strongest, swiftest, or the most cunning; from famine, the best hunters or those with the best digestion; and so on. Then it suddenly flashed upon me that this self-acting process would necessarily improve the race, because in every generation the inferior would inevitably be killed off and the superior would remain—that is, the fittest would survive.
[The phrase 'survival of the fittest,' suggested by the writings of Thomas Robert Malthus, was expressed in those words by Herbert Spencer in 1865. Wallace saw the term in correspondence from Charles Darwin the following year, 1866. However, Wallace did not publish anything on his use of the expression until very much later, and his recollection is likely flawed.]
[The phrase 'survival of the fittest,' suggested by the writings of Thomas Robert Malthus, was expressed in those words by Herbert Spencer in 1865. Wallace saw the term in correspondence from Charles Darwin the following year, 1866. However, Wallace did not publish anything on his use of the expression until very much later, and his recollection is likely flawed.]
My Life: A Record of Events and Opinions (1905), Vol. 1, 361-362, or in reprint (2004), 190.
I was unable to devote myself to the learning of this al-jabr [algebra] and the continued concentration upon it, because of obstacles in the vagaries of Time which hindered me; for we have been deprived of all the people of knowledge save for a group, small in number, with many troubles, whose concern in life is to snatch the opportunity, when Time is asleep, to devote themselves meanwhile to the investigation and perfection of a science; for the majority of people who imitate philosophers confuse the true with the false, and they do nothing but deceive and pretend knowledge, and they do not use what they know of the sciences except for base and material purposes; and if they see a certain person seeking for the right and preferring the truth, doing his best to refute the false and untrue and leaving aside hypocrisy and deceit, they make a fool of him and mock him.
A. P. Youschkevitch and B. A. Rosenfeld, 'Al-Khayyami', in C. C. Gillispie (ed.), Dictionary of Scientific Biography (1973), Vol. 7, 324.
I wish that one would be persuaded that psychological experiments, especially those on the complex functions, are not improved [by large studies]; the statistical method gives only mediocre results; some recent examples demonstrate that. The American authors, who love to do things big, often publish experiments that have been conducted on hundreds and thousands of people; they instinctively obey the prejudice that the persuasiveness of a work is proportional to the number of observations. This is only an illusion.
L' Études expérimentale de l'intelligence (1903), 299.
Iamblichus in his treatise On the Arithmetic of Nicomachus observes p. 47- “that certain numbers were called amicable by those who assimilated the virtues and elegant habits to numbers.” He adds, “that 284 and 220 are numbers of this kind; for the parts of each are generative of each other according to the nature of friendship, as was shown by Pythagoras. For some one asking him what a friend was, he answered, another I (ετεϑος εγω) which is demonstrated to take place in these numbers.” [“Friendly” thus: Each number is equal to the sum of the factors of the other.]
In Theoretic Arithmetic (1816), 122. (Factors of 284 are 1, 2, 4 ,71 and 142, which give the sum 220. Reciprocally, factors of 220 are 1, 2, 4, 5, 10, 11 ,22, 44, 55 and 110, which give the sum 284.) Note: the expression “alter ego” is Latin for “the other I.”
If “Number rules the universe” as Pythagoras asserted, Number is merely our delegate to the throne, for we rule Number.
In Men of Mathematics (1937), 16.
If a mixture of different kinds of electrified atoms is moving along in one stream, then when electric and magnetic forces are applied to the stream simultaneously, the different kinds of atoms are sorted out, and the original stream is divided up into a number of smaller streams separated from each other. The particles in any one of the smaller streams are all of the same kind.
From the Romanes Lecture (10 Jun 1914) delivered in the Sheldonian Theatre, published as The Atomic Theory (1914), 9.
If all sentient beings in the universe disappeared, there would remain a sense in which mathematical objects and theorems would continue to exist even though there would be no one around to write or talk about them. Huge prime numbers would continue to be prime, even if no one had proved them prime.
In When You Were a Tadpole and I Was a Fish: And Other Speculations About This and That (), 124.
If an event can be produced by a number n of different causes, the probabilities of the existence of these causes, given the event (prises de l'événement), are to each other as the probabilities of the event, given the causes: and the probability of each cause is equal to the probability of the event, given that cause, divided by the sum of all the probabilities of the event, given each of the causes.
'Mémoire sur la Probabilité des Causes par les Événements' (1774). In Oeuvres complètes de Laplace, 14 Vols. (1843-1912), Vol. 8, 29, trans. Charles Coulston Gillispie, Pierre-Simon Laplace 1749-1827: A Life in Exact Science (1997), 16.
If any layman were to ask a number of archaeologists to give, on the spur of the moment, a definition of archaeology, I suspect that such a person might find the answers rather confusing. He would, perhaps, sympathize with Socrates who, when he hoped to learn from the poets and artisans something about the arts they practised, was forced to go away with the conviction that, though they might themselves be able to accomplish something, they certainly could give no clear account to others of what they were trying to do.
Opening statement in lecture at Columbia University (8 Jan 1908), 'Archaeology'. Published by the Columbia University Press (1908).
If E is considered to be a continuously divisible quantity, this distribution is possible in infinitely many ways. We consider, however—this is the most essential point of the whole calculation—E to be composed of a well-defined number of equal parts and use thereto the constant of nature h = 6.55 ×10-27 erg sec. This constant multiplied by the common frequency ν of the resonators gives us the energy element ε in erg, and dividing E by ε we get the number P of energy elements which must be divided over the N resonators.
[Planck’s constant, as introduced in 1900; subsequently written e = hν.]
[Planck’s constant, as introduced in 1900; subsequently written e = hν.]
In 'On the theory of the energy distribution law of the normal spectrum', in D. ter Haar and Stephen G. Brush, trans., Planck’s Original Papers in Quantum Physics (1972), 40.
If equations are trains threading the landscape of numbers, then no train stops at pi.
In Panic in Level 4: Cannibals, Killer Viruses, and Other Journeys to the Edge (2009), 33-34.
If even in science there is no a way of judging a theory but by assessing the number, faith and vocal energy of its supporters, then this must be even more so in the social sciences: truth lies in power.
In Radio Lecture (30 Jun 1973) broadcast by the Open University, collected in Imre Lakatos, John Worrall (ed.) and Gregory Currie (ed.), 'Introduction: Science and Pseudoscience', The Methodology of Scientific Research Programmes (1978, 1980), Vol. 1, 9.
If it is true as Whewell says, that the essence of the triumphs of Science and its progress consists in that it enables us to consider evident and necessary, views which our ancestors held to be unintelligible and were unable to comprehend, then the extension of the number concept to include the irrational, and we will at once add, the imaginary, is the greatest forward step which pure mathematics has ever taken.
In Theorie der Complexen Zahlensysteme (1867), 60. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 281. From the original German, “Wenn es wahr ist, dass, wie Whewell meint, das Wesen der Triumphe der Wissenschaft und ihres Fortschrittes darin besteht, dass wir veranlasst werden, Ansichten, welche unsere Vorfahren für unbegreiflich hielten und unfähig waren zu begreifen, für evident und nothwendig zu halten, so war die Erweiterung des Zahlenbegriffes auf das Irrationale, und wollen wir sogleich hinzufügen, das Imaginäre, der grösste Fortschritt, den die reine Mathematik jemals gemacht hat.”
If one proves the equality of two numbers a and b by showing first that “a is less than or equal to b” and then “a is greater than or equal to b”, it is unfair, one should instead show that they are really equal by disclosing the inner ground for their equality.
As quoted, without citation, in biography by Hermann Wehl, Emmy Noether (1935), 18.
If the arrangement of society is bad (as ours is), and a small number of people have power over the majority and oppress it, every victory over Nature will inevitably serve only to increase that power and that oppression.
In Science, Liberty and Peace by Aldous Huxley (1947).
If the views we have ventured to advance be correct, we may almost consider {greek words} of the ancients to be realised in hydrogen, an opinion, by the by, not altogether new. If we actually consider the specific gravities of bodies in their gaseous state to represent the number of volumes condensed into one; or in other words, the number of the absolute weight of a single volume of the first matter ({greek words}) which they contain, which is extremely probable, multiples in weight must always indicate multiples in volume, and vice versa; and the specific gravities, or absolute weights of all bodies in a gaseous state, must be multiples of the specific gravity or absolute weight of the first matter, ({Greek words}), because all bodies in the gaseous state which unite with one another unite with reference to their volume.
'Correction of a Mistake in the Essay on the Relation between the Specific Gravities of Bodies in their Gaseous State and the Weights of their Atoms', Annals of Philosophy (1816), 7, 113.
If the world has begun with a single quantum, the notions of space and would altogether fail to have any meaning at the beginning; they would only begin to have a sensible meaning when the original quantum had been divided into a sufficient number of quanta. If this suggestion is correct, the beginning of the world happened a little before the beginning of space and time. I think that such a beginning of the world is far enough from the present order of Nature to be not at all repugnant. It may be difficult to follow up the idea in detail as we are not yet able to count the quantum packets in every case. For example, it may be that an atomic nucleus must be counted as a unique quantum, the atomic number acting as a kind of quantum number. If the future development of quantum theory happens to turn in that direction, we could conceive the beginning of the universe in the form of a unique atom, the atomic weight of which is the total mass of the universe. This highly unstable atom would divide in smaller and smaller atoms by a kind of super-radioactive process.
In a seminal short letter (457 words), 'The Beginning of the World from the Point of View of Quantum Theory', Nature (9 May 1931), 127, 706.
If the world may be thought of as a certain definite quantity of force and as a certain definite number of centers of force—and every other representation remains indefinite and therefore useless—it follows that, in the great dice game of existence, it must pass through calculable number of combinations. In infinite time, every possible combination would at some time or another be realized; more: it would be realized an infinite number of times. And since between every combination and its next recurrence all other possible combinations would have to take place, and each of these combination conditions of the entire sequence of combinations in the same series, a circular movement of absolutely identical series is thus demonstrated: the world as a circular movement that has already repeated itself infinitely often and plays its game in infinitum. This conception is not simply a mechanistic conception; for if it were that, it would not condition an infinite recurrence of identical cases, but a final state. Because the world has not reached this, mechanistic theory must be considered an imperfect and merely provisional hypothesis.
The Will to Power (Notes written 1883-1888), book 4, no. 1066. Trans. W. Kaufmann and R. J. Hollingdale and ed. W. Kaufmann (1968), 549.
If there is such a thing as luck, then I must be the most unlucky fellow in the world. I’ve never once made a lucky strike in all my life. When I get after something that I need, I start finding everything in the world that I don’t need—one damn thing after another. I find ninety-nine things that I don’t need, and then comes number one hundred, and that—at the very last—turns out to be just what I had been looking for.
In Martin André Rosanoff, 'Edison in His Laboratory', Harper’s Magazine (Sep 1932), 406.
If to-day you ask a physicist what he has finally made out the æther or the electron to be, the answer will not be a description in terms of billiard balls or fly-wheels or anything concrete; he will point instead to a number of symbols and a set of mathematical equations which they satisfy. What do the symbols stand for? The mysterious reply is given that physics is indifferent to that; it has no means of probing beneath the symbolism. To understand the phenomena of the physical world it is necessary to know the equations which the symbols obey but not the nature of that which is being symbolised. …this newer outlook has modified the challenge from the material to the spiritual world.
Swarthmore Lecture (1929) at Friends’ House, London, printed in Science and the Unseen World (1929), 30.
If we assume that there is only one enzyme present to act as an oxidizing agent, we must assume for it as many different degrees of activity as are required to explain the occurrence of the various colors known to mendelize (three in mice, yellow, brown, and black). If we assume that a different enzyme or group of enzymes is responsible for the production of each pigment we must suppose that in mice at least three such enzymes or groups of enzymes exist. To determine which of these conditions occurs in mice is not a problem for the biologist, but for the chemist. The biologist must confine his attention to determining the number of distinct agencies at work in pigment formation irrespective of their chemical nature. These agencies, because of their physiological behavior, the biologist chooses to call 'factors,' and attempts to learn what he can about their functions in the evolution of color varieties.
Experimental Studies of the Inheritance of Color in Mice (1913), 17-18.
If we consider what science already has enabled men to know—the immensity of space, the fantastic philosophy of the stars, the infinite smallness of the composition of atoms, the macrocosm whereby we succeed only in creating outlines and translating a measure into numbers without our minds being able to form any concrete idea of it—we remain astounded by the enormous machinery of the universe.
Address (10 Sep 1934) to the International Congress of Electro-Radio Biology, Venice. In Associated Press, 'Life a Closed Book, Declares Marconi', New York Times (11 Sep 1934), 15.
If we knew all the laws of Nature, we should need only one fact or the description of one actual phenomenon to infer all the particular results at that point. Now we know only a few laws, and our result is vitiated, not, of course, by any confusion or irregularity in Nature, but by our ignorance of essential elements in the calculation. Our notions of law and harmony are commonly confined to those instances which we detect, but the harmony which results from a far greater number of seemingly conflicting, but really concurring, laws which we have not detected, is still more wonderful. The particular laws are as our points of view, as to the traveler, a mountain outline varies with every step, and it has an infinite number of profiles, though absolutely but one form. Even when cleft or bored through, it is not comprehended in its entireness.
In Walden (1878), 311.
If we take in our hand any Volume; of Divinity or School Metaphysics, for Instance; let us ask, Does it contain any abstract Reasoning concerning Quantity or Number? No. Does it contain any experimental Reasoning concerning Matter of Fact and Existence? No. Commit it then to the Flames: For it can contain nothing but Sophistry and Illusion.
An Enquiry Concerning Human Understanding (1748), 256.
If we were capable of following the progress of increase of the number of the parts of the most perfect animal, as they first formed in succession, from the very first to its state of full perfection, we should probably be able to compare it with some one of the incomplete animals themselves, of every order of animals in the Creation, being at no stage different from some of the inferior orders; or, in other words, if we were to take a series of animals, from the more imperfect to the perfect, we should probably find an imperfect animal, corresponding with some stage of the most perfect.
R. Owen (ed.), John Hunter's Observations on Animal Development (1841), 14.
If we wish to give an account of the atomic constitution of the aromatic compounds, we are bound to explain the following facts:
1) All aromatic compounds, even the most simple, are relatively richer in carbon than the corresponding compounds in the class of fatty bodies.
2) Among the aromatic compounds, as well as among the fatty bodies, a large number of homologous substances exist.
3) The most simple aromatic compounds contain at least six atoms of carbon.
4) All the derivatives of aromatic substances exhibit a certain family likeness; they all belong to the group of 'Aromatic compounds'. In cases where more vigorous reactions take place, a portion of the carbon is often eliminated, but the chief product contains at least six atoms of carbon These facts justify the supposition that all aromatic compounds contain a common group, or, we may say, a common nucleus consisting of six atoms of carbon. Within this nucleus a more intimate combination of the carbon atoms takes place; they are more compactly placed together, and this is the cause of the aromatic bodies being relatively rich in carbon. Other carbon atoms can be joined to this nucleus in the same way, and according to the same law, as in the case of the group of fatty bodies, and in this way the existence of homologous compounds is explained.
1) All aromatic compounds, even the most simple, are relatively richer in carbon than the corresponding compounds in the class of fatty bodies.
2) Among the aromatic compounds, as well as among the fatty bodies, a large number of homologous substances exist.
3) The most simple aromatic compounds contain at least six atoms of carbon.
4) All the derivatives of aromatic substances exhibit a certain family likeness; they all belong to the group of 'Aromatic compounds'. In cases where more vigorous reactions take place, a portion of the carbon is often eliminated, but the chief product contains at least six atoms of carbon These facts justify the supposition that all aromatic compounds contain a common group, or, we may say, a common nucleus consisting of six atoms of carbon. Within this nucleus a more intimate combination of the carbon atoms takes place; they are more compactly placed together, and this is the cause of the aromatic bodies being relatively rich in carbon. Other carbon atoms can be joined to this nucleus in the same way, and according to the same law, as in the case of the group of fatty bodies, and in this way the existence of homologous compounds is explained.
Bulletin de la Societé Chimique de France (1865), 1, 98. Trans. W. H. Brock.
If you are surprised at the number of our maladies, count our cooks.
In Noble Words and Noble Deeds (1877), 239.
If you take a number and double it and double it again and then double it a few more times, the number gets bigger and bigger and goes higher and higher and only arithmetic can tell you what the number is when you decide to quit doubling.
From 'Arithmetic', Harvest Poems, 1910-1960 (1960), 115-116.
If you walk along the street you will encounter a number of scientific problems. Of these, about 80 per cent are insoluble, while 19½ per cent are trivial. There is then perhaps half a per cent where skill, persistence, courage, creativity and originality can make a difference. It is always the task of the academic to swim in that half a per cent, asking the questions through which some progress can be made.
'The Making of a Scientist', Journal of the Royal Society of Arts, June 1983, 406.
Imaginary numbers are a fine and wonderful refuge of the divine spirit almost an amphibian between being and non-being. (1702)
[Alternate translation:] The Divine Spirit found a sublime outlet in that wonder of analysis, that portent of the ideal world, that amphibian between being and not-being, which we call the imaginary root of negative unity.
[Alternate translation:] The Divine Spirit found a sublime outlet in that wonder of analysis, that portent of the ideal world, that amphibian between being and not-being, which we call the imaginary root of negative unity.
Quoted in Félix Klein, Elementary Mathematics From an Advanced Standpoint: Arithmetic, Algebra, Analysis (1924), 56. Alternate translation as quoted in Tobias Dantzig, Number, the Language of Science: a Critical Survey Written for the Cultured Non-Mathematician (1930), 204
In 1963, when I assigned the name “quark” to the fundamental constituents of the nucleon, I had the sound first, without the spelling, which could have been “kwork.” Then, in one of my occasional perusals of Finnegans Wake, by James Joyce, I came across the word “quark” in the phrase “Three quarks for Muster Mark.” Since “quark” (meaning, for one thing, the cry of a gull) was clearly intended to rhyme with “Mark,” as well as “bark” and other such words, I had to find an excuse to pronounce it as “kwork.” But the book represents the dreams of a publican named Humphrey Chimpden Earwicker. Words in the text are typically drawn from several sources at once, like the “portmanteau words” in Through the Looking Glass. From time to time, phrases occur in the book that are partially determined by calls for drinks at the bar. I argued, therefore, that perhaps one of the multiple sources of the cry “Three quarks for Muster Mark” might be pronunciation for “Three quarts for Mister Mark,” in which case the pronunciation “kwork” would not be totally unjustified. In any case, the number three fitted perfectly the way quarks occur in nature.
The Quark and the Jaguar (1994), 180.
In a class I was taking there was one boy who was much older than the rest. He clearly had no motive to work. I told him that, if he could produce for me, accurately to scale, drawings of the pieces of wood required to make a desk like the one he was sitting at, I would try to persuade the Headmaster to let him do woodwork during the mathematics hours—in the course of which, no doubt, he would learn something about measurement and numbers. Next day, he turned up with this task completed to perfection. This I have often found with pupils; it is not so much that they cannot do the work, as that they see no purpose in it.
In Mathematician's Delight (1943), 52.
In a dispassionate comparison of the relative values of human and robotic spaceflight, the only surviving motivation for continuing human spaceflight is the ideology of adventure. But only a tiny number of Earth’s six billion inhabitants are direct participants. For the rest of us, the adventure is vicarious and akin to that of watching a science fiction movie.
In 'Is Human Spaceflight Obsolete?', Issues in Science and Technology (Summer 2004). [Note: published one year after the loss of seven lives in the Space Shuttle Columbia disaster. —Webmaster]
In a great number of programmes I’m not a scientist—I’m simply a commentator. So I should claim no virtue for the fact that [people] seem to trust me, if that is indeed the case. It’s simply that I very seldom talk about something they can’t see. If I say a lion is attacking a wildebeest, they can see it is; if I were to say something about a proton, it might be different.
As quoted in Bill Parry, 'Sir David Attenborough in Conversation', The Biologist (Jun 2010), 57, No. 2, 93.
In a great number of the cosmogonic myths the world is said to have developed from a great water, which was the prime matter. In many cases, as for instance in an Indian myth, this prime matter is indicated as a solution, out of which the solid earth crystallized out.
In Theories of Solutions (1912), 1.
In a randomly infinite Universe, any event occurring here and now with finite probability must be occurring simultaneously at an infinite number of other sites in the Universe. It is hard to evaluate this idea any further, but one thing is certain: if it is true then it is certainly not original!
With co-author Frank Tipler, The Anthropic Cosmological Principle (1986).
In a sense cosmology contains all subjects because it is the story of everything, including biology, psychology and human history. In that single sense it can be said to contain an explanation also of time's arrow. But this is not what is meant by those who advocate the cosmological explanation of irreversibility. They imply that in some way the time arrow of cosmology imposes its sense on the thermodynamic arrow. I wish to disagree with this view. The explanation assumes that the universe is expanding. While this is current orthodoxy, there is no certainty about it. The red-shifts might be due to quite different causes. For example, when light passes through the expanding clouds of gas it will be red-shifted. A large number of such clouds might one day be invoked to explain these red shifts. It seems an odd procedure to attempt to 'explain' everyday occurrences, such as the diffusion of milk into coffee, by means of theories of the universe which are themselves less firmly established than the phenomena to be explained. Most people believe in explaining one set of things in terms of others about which they are more certain, and the explanation of normal irreversible phenomena in terms of the cosmological expansion is not in this category.
'Thermodynamics, Cosmology) and the Physical Constants', in J. T. Fraser (ed.), The Study of Time III (1973), 117-8.
In addition to this it [mathematics] provides its disciples with pleasures similar to painting and music. They admire the delicate harmony of the numbers and the forms; they marvel when a new discovery opens up to them an unexpected vista; and does the joy that they feel not have an aesthetic character even if the senses are not involved at all? … For this reason I do not hesitate to say that mathematics deserves to be cultivated for its own sake, and I mean the theories which cannot be applied to physics just as much as the others.
(1897) From the original French, “Et surtout, leurs adeptes y trouvent des jouissances analogues á celles que donnent la peinture et la musique. Ils admirent la délicate harmonie des nombres et des formes; ils s’émerveillent quand une découverte nouvelle leur ouvre une perspective inattendue; et la joie qu’ils éprouvent ainsi n’a-t-elle pas le caractère esthétique, bien que les sens n’y prennent aucune part?...C’est pourquoi je n’hésite pas à dire que les mathématiques méritent d’être cultivées pour elles-mêmes et que les théories qui ne peuvent être appliquées á la physique doivent l’être comme les autres.” Address read for him at the First International Congress of Mathematicians in Zurich: '‘Sur les rapports de l’analyse pure et de la physique', in Proceedings of that Congress 81-90, (1898). Also published as 'L’Analyse et la Physique', in La Valeur de la Science (1905), 137-151. As translated in Armand Borel, 'On the Place of Mathematics in Culture', in Armand Borel: Œvres: Collected Papers (1983), Vol. 4, 420-421.
In all chemical investigations, it has justly been considered an important object to ascertain the relative weights of the simples which constitute a compound. But unfortunately the enquiry has terminated here; whereas from the relative weights in the mass, the relative weights of the ultimate particles or atoms of the bodies might have been inferred, from which their number and weight in various other compounds would appear, in order to assist and to guide future investigations, and to correct their results. Now it is one great object of this work, to shew the importance and advantage of ascertaining the relative weights of the ultimate particles, both of simple and compound bodies, the number of simple elementary particles which constitute one compound particle, and the number of less compound particles which enter into the formation of one more compound particle.
If there are two bodies, A and B, which are disposed to combine, the following is the order in which the combinations may take place, beginning with the most simple: namely,
1 atom of A + 1 atom of B = 1 atom of C, binary
1 atom of A + 2 atoms of B = 1 atom of D, ternary
2 atoms of A + 1 atom of B = 1 atom of E, ternary
1 atom of A + 3 atoms of B = 1 atom of F, quaternary
3 atoms of A and 1 atom of B = 1 atom of G, quaternary
If there are two bodies, A and B, which are disposed to combine, the following is the order in which the combinations may take place, beginning with the most simple: namely,
1 atom of A + 1 atom of B = 1 atom of C, binary
1 atom of A + 2 atoms of B = 1 atom of D, ternary
2 atoms of A + 1 atom of B = 1 atom of E, ternary
1 atom of A + 3 atoms of B = 1 atom of F, quaternary
3 atoms of A and 1 atom of B = 1 atom of G, quaternary
A New System of Chemical Philosophy (1808), Vol. 1, 212-3.
In all works on Natural History, we constantly find details of the marvellous adaptation of animals to their food, their habits, and the localities in which they are found. But naturalists are now beginning to look beyond this, and to see that there must be some other principle regulating the infinitely varied forms of animal life. It must strike every one, that the numbers of birds and insects of different groups having scarcely any resemblance to each other, which yet feed on the same food and inhabit the same localities, cannot have been so differently constructed and adorned for that purpose alone. Thus the goat-suckers, the swallows, the tyrant fly-catchers, and the jacamars, all use the same kind ‘Of food, and procure it in the same manner: they all capture insects on the wing, yet how entirely different is the structure and the whole appearance of these birds!
In A Narrative of Travels on the Amazon and Rio Negro (1853), 83-84.
In an enterprise such as the building of the atomic bomb the difference between ideas, hopes, suggestions and theoretical calculations, and solid numbers based on measurement, is paramount. All the committees, the politicking and the plans would have come to naught if a few unpredictable nuclear cross sections had been different from what they are by a factor of two.
Epigraph in Richard Rhodes, The Making of the Atomic Bomb (1986), 8.
In describing a protein it is now common to distinguish the primary, secondary and tertiary structures. The primary structure is simply the order, or sequence, of the amino-acid residues along the polypeptide chains. This was first determined by Sanger using chemical techniques for the protein insulin, and has since been elucidated for a number of peptides and, in part, for one or two other small proteins. The secondary structure is the type of folding, coiling or puckering adopted by the polypeptide chain: the a-helix structure and the pleated sheet are examples. Secondary structure has been assigned in broad outline to a number of librous proteins such as silk, keratin and collagen; but we are ignorant of the nature of the secondary structure of any globular protein. True, there is suggestive evidence, though as yet no proof, that a-helices occur in globular proteins, to an extent which is difficult to gauge quantitatively in any particular case. The tertiary structure is the way in which the folded or coiled polypeptide chains are disposed to form the protein molecule as a three-dimensional object, in space. The chemical and physical properties of a protein cannot be fully interpreted until all three levels of structure are understood, for these properties depend on the spatial relationships between the amino-acids, and these in turn depend on the tertiary and secondary structures as much as on the primary. Only X-ray diffraction methods seem capable, even in principle, of unravelling the tertiary and secondary structures.
Co-author with G. Bodo, H. M. Dintzis, R. G. Parrish, H. Wyckoff, and D. C. Phillips
Co-author with G. Bodo, H. M. Dintzis, R. G. Parrish, H. Wyckoff, and D. C. Phillips
'A Three-Dimensional Model of the Myoglobin Molecule Obtained by X-ray Analysis', Nature (1958) 181, 662.
In England, more than in any other country, science is felt rather than thought. … A defect of the English is their almost complete lack of systematic thinking. Science to them consists of a number of successful raids into the unknown.
The Social Function of Science (1939), 197.
In every section of the entire area where the word science may properly be applied, the limiting factor is a human one. We shall have rapid or slow advance in this direction or in that depending on the number of really first-class men who are engaged in the work in question. ... So in the last analysis, the future of science in this country will be determined by our basic educational policy.
Quoted in Vannevar Bush, Science, the Endless Frontier: A Report to the President, July 1945. In Transactions of the Kansas Academy of Science: Volumes 48-49, 246.
In experimenting on the arc, my aim was not so much to add to the large number of isolated facts that had already been discovered, as to form some idea of the bearing of these upon one another, and thus to arrive at a clear conception of what takes place in each part of the arc and carbons at every moment. The attempt to correlate all the known phenomena, and to bind them together into one consistent whole, led to the deduction of new facts, which, when duly tested by experiment, became parts of the growing body, and, themselves, opened up fresh questions, to be answered in their turn by experiment.
In The Electric Arc (1902), Preface, iii. Ayrton described the growth of her published work on the electric arc, from a series of articles in The Electrician in 1895-6, to the full book, which “has attained to its present proportions almost with the growth of an organic body.”
In France, where an attempt has been made to deprive me of the originality of these discoveries, experiments without number and without mercy have been made on living animals; not under the direction of anatomical knowledge, or the guidance of just induction, but conducted with cruelty and indifference, in hope to catch at some of the accidental facts of a system, which, is evident, the experimenters did not fully comprehend.
An Exposition of the Natural System of the Nerves of the Human Body (1824), 2-3.
In general, the more one augments the number of divisions of the productions of nature, the more one approaches the truth, since in nature only individuals exist, while genera, orders, and classes only exist in our imagination.
Histoire Naturelle (1749), trans. by John Lyon, The 'Initial Discourse' to Buffon’s Histoire Naturelle: The First Complete English Translation, Journal of the History of Biology, 9(1), 1976, 164.
In India we have clear evidence that administrative statistics had reached a high state of organization before 300 B.C. In the Arthasastra of Kautilya … the duties of the Gopa, the village accountant, [include] “by setting up boundaries to villages, by numbering plots of grounds as cultivated, uncultivated, plains, wet lands, gardens, vegetable gardens, fences (váta), forests altars, temples of gods, irrigation works, cremation grounds, feeding houses (sattra), places where water is freely supplied to travellers (prapá), places of pilgrimage, pasture grounds and roads, and thereby fixing the boundaries of various villages, of fields, of forests, and of roads, he shall register gifts, sales, charities, and remission of taxes regarding fields.”
Editorial, introducing the new statistics journal of the Indian Statistical Institute, Sankhayā (1933), 1, No. 1. Also reprinted in Sankhyā: The Indian Journal of Statistics (Feb 2003), 65, No. 1, viii.
In Man the brain presents an ascensive step in development, higher and more strongly marked than that by which the preceding subclass was distinguished from the one below it. Not only do the cerebral hemispheres overlap the olfactory lobes and cerebellum, but they extend in advance of the one, and further back than the other. Their posterior development is so marked, that anatomists have assigned to that part the character of a third lobe; it is peculiar to the genus Homo, and equally peculiar is the 'posterior horn of the lateral ventricle,' and the 'hippocampus minor,' which characterize the hind lobe of each hemisphere. The superficial grey matter of the cerebrum, through the number and depth of the convolutions, attains its maximum of extent in Man. Peculiar mental powers are associated with this highest form of brain, and their consequences wonderfully illustrate the value of the cerebral character; according to my estimate of which, I am led to regard the genus Homo, as not merely a representative of a distinct order, but of a distinct subclass of the Mammalia, for which I propose a name of 'ARCHENCEPHALA.'
'On the Characters, Principles of Division, and Primary Groups of the Class MAMMALIA' (1857), Journal of the Proceedings of the Linnean Society of London (1858), 2, 19-20.
In man, then, let us take the amount that is extruded by the individual beats, and that cannot return into the heart because of the barrier set in its way by the valves, as half an ounce, or three drachms, or at least one drachm. In half an hour the heart makes over a thousand beats; indeed, in some individuals, and on occasion, two, three, or four thousand. If you multiply the drachms per beat by the number of beats you will see that in half an hour either a thousand times three drachms or times two drachms, or five hundred ounces, or other such proportionate quantity of blood has been passed through the heart into the arteries, that is, in all cases blood in greater amount than can be found in the whole of the body. Similarly in the sheep or the dog. Let us take it that one scruple passes in a single contraction of the heart; then in half an hour a thousand scruples, or three and a half pounds of blood, do so. In a body of this size, as I have found in the sheep, there is often not more than four pounds of blood.
In the above sort of way, by calculating the amount of blood transmitted [at each heart beat] and by making a count of the beats, let us convince ourselves that the whole amount of the blood mass goes through the heart from the veins to the arteries and similarly makes the pulmonary transit.
Even if this may take more than half an hour or an hour or a day for its accomplishment, it does nevertheless show that the beat of the heart is continuously driving through that organ more blood than the ingested food can supply, or all the veins together at any time contain.
In the above sort of way, by calculating the amount of blood transmitted [at each heart beat] and by making a count of the beats, let us convince ourselves that the whole amount of the blood mass goes through the heart from the veins to the arteries and similarly makes the pulmonary transit.
Even if this may take more than half an hour or an hour or a day for its accomplishment, it does nevertheless show that the beat of the heart is continuously driving through that organ more blood than the ingested food can supply, or all the veins together at any time contain.
De Motu Cordis (1628), The Circulation of the Blood and Other Writings, trans. Kenneth J. Franklin (1957), Chapter 9, 62-3.
In mathematics, which is but a mirror of the society in which it thrives or suffers, the pre-Athenian period is one of colorful men and important discoveries. Sparta, like most militaristic states before and after it, produced nothing. Athens, and the allied Ionians, produced a number of works by philosophers and mathematicians; some good, some controversial, some grossly overrated.
In A History of Pi (1970), 34.
In modern Europe, the Middle Ages were called the Dark Ages. Who dares to call them so now? … Their Dante and Alfred and Wickliffe and Abelard and Bacon; their Magna Charta, decimal numbers, mariner’s compass, gunpowder, glass, paper, and clocks; chemistry, algebra, astronomy; their Gothic architecture, their painting,—are the delight and tuition of ours. Six hundred years ago Roger Bacon explained the precession of the equinoxes, and the necessity of reform in the calendar; looking over how many horizons as far as into Liverpool and New York, he announced that machines can be constructed to drive ships more rapidly than a whole galley of rowers could do, nor would they need anything but a pilot to steer; carriages, to move with incredible speed, without aid of animals; and machines to fly into the air like birds.
In 'Progress of Culture', an address read to the Phi Beta Kappa Society at Cambridge, 18 July 1867. Collected in Works of Ralph Waldo Emerson (1883), 475.
In my view, the proper attitude of a public-service broadcaster is that it should attempt to cover as broad as possible a spectrum of human interest and should measure success by the width of those views. There shouldn’t be all that large a number of gaps in the spectrum; and a major element in the spectrum is scientific understanding. The fact that it doesn’t necessarily get as big an audience as cookery is of no consequence.
From interview with Brian Cox and Robert Ince, in 'A Life Measured in Heartbeats', New Statesman (21 Dec 2012), 141, No. 5138, 33.
In physical science a first essential step in the direction of learning any subject is to find principles of numerical reckoning and practicable methods for measuring some quality connected with it. I often say that when you can measure what you are speaking about, and express it in numbers, you know something about it; but when you cannot measure it, when you cannot express it in numbers, your knowledge is of a meagre and unsatisfactory kind; it may be the beginning of knowledge, but you have scarcely in your thoughts advanced to the stage of science, whatever the matter may be.
Often seen quoted in a condensed form: If you cannot measure it, then it is not science.
Often seen quoted in a condensed form: If you cannot measure it, then it is not science.
From lecture to the Institution of Civil Engineers, London (3 May 1883), 'Electrical Units of Measurement', Popular Lectures and Addresses (1889), Vol. 1, 80-81.
In place of infinity we usually put some really big number, like 15.
Perhaps referring to the programmer’s hexadecimal counting scheme which has 16 digits (0-9 followed by digits A-F), useful in binary context as a power of 2.
Perhaps referring to the programmer’s hexadecimal counting scheme which has 16 digits (0-9 followed by digits A-F), useful in binary context as a power of 2.
Attributed to a Computer Science Professor on various web pages. Webmaster has found no print source for this wording and comments, but its originality makes it worthy of inclusion here. Webmaster comments: perhaps one of those infinite number of monkeys typed it! Please make contact if you know a primary print source.
In Pure Mathematics, where all the various truths are necessarily connected with each other, (being all necessarily connected with those hypotheses which are the principles of the science), an arrangement is beautiful in proportion as the principles are few; and what we admire perhaps chiefly in the science, is the astonishing variety of consequences which may be demonstrably deduced from so small a number of premises.
In Elements of the Philosophy of the Human Mind (1827), Vol. 3, Chap. 1, Sec. 8, 186.
In recent years several new particles have been discovered which are currently assumed to be “elementary,” that is, essentially structureless. The probability that all such particles should be really elementary becomes less and less as their number increases. It is by no means certain that nucleons, mesons, electrons, neutrinos are all elementary particles.
Opening statement, Enrico Fermi and C.N. Yang, 'Are Mesons Elementary Particles?', Physical Review (1949), 76, 1739. As cited in James Gleick, Genius: The Life and Science of Richard Feynman (1992), 283.
In that same year [1932], the number of [known] particles was suddenly doubled. In two beautiful experiments, Chadwick showed that the neutron existed, and Anderson photographed the first unmistakable positron track.
In Nobel Lecture (11 Dec 1968), 'Recent Developments in Particle Physics', collected in Nobel Lectures: Physics 1963-1970 (1972), 241.
In the benzene nucleus we have been given a soil out of which we can see with surprise the already-known realm of organic chemistry multiply, not once or twice but three, four, five or six times just like an equivalent number of trees. What an amount of work had suddenly become necessary, and how quickly were busy hands found to carry it out! First the eye moves up the six stems opening out from the tremendous benzene trunk. But already the branches of the neighbouring stems have become intertwined, and a canopy of leaves has developed which becomes more spacious as the giant soars upwards into the air. The top of the tree rises into the clouds where the eye cannot yet follow it. And to what an extent is this wonderful benzene tree thronged with blossoms! Everywhere in the sea of leaves one can spy the slender hydroxyl bud: hardly rarer is the forked blossom [Gabelblüte] which we call the amine group, the most frequent is the beautiful cross-shaped blossom we call the methyl group. And inside this embellishment of blossoms, what a richness of fruit, some of them shining in a wonderful blaze of color, others giving off an overwhelming fragrance.
A. W. Hofmann, after-dinner speech at Kekulé Benzolfest (Mar 1890). Trans. in W. H. Brock, O. Theodor Benfrey and Susanne Stark, 'Hofmann's Benzene Tree at the Kekulé Festivities', Journal of Chemical Education (1991), 68, 887-8.
In the conception of a machine or the product of a machine there is a point where one may leave off for parsimonious reasons, without having reached aesthetic perfection; at this point perhaps every mechanical factor is accounted for, and the sense of incompleteness is due to the failure to recognize the claims of the human agent. Aesthetics carries with it the implications of alternatives between a number of mechanical solutions of equal validity; and unless this awareness is present at every stage of the process … it is not likely to come out with any success in the final stage of design.
From 'The Esthetic Assimilation of the Machine', Technics and Civilization (1934), 349.
In the discussion of the. energies involved in the deformation of nuclei, the concept of surface tension of nuclear matter has been used and its value had been estimated from simple considerations regarding nuclear forces. It must be remembered, however, that the surface tension of a charged droplet is diminished by its charge, and a rough estimate shows that the surface tension of nuclei, decreasing with increasing nuclear charge, may become zero for atomic numbers of the order of 100. It seems therefore possible that the uranium nucleus has only small stability of form, and may, after neutron capture, divide itself into two nuclei of roughly equal size (the precise ratio of sizes depending on liner structural features and perhaps partly on chance). These two nuclei will repel each other and should gain a total kinetic energy of c. 200 Mev., as calculated from nuclear radius and charge. This amount of energy may actually be expected to be available from the difference in packing fraction between uranium and the elements in the middle of the periodic system. The whole 'fission' process can thus be described in an essentially classical way, without having to consider quantum-mechanical 'tunnel effects', which would actually be extremely small, on account of the large masses involved.
[Co-author with Otto Robert Frisch]
[Co-author with Otto Robert Frisch]
Lise Meitner and O. R. Frisch, 'Disintegration of Uranium by Neutrons: a New Type of Nuclear Reaction', Nature (1939), 143, 239.
In the mountains of Parma and Piacenza, multitudes of shells and corals filled with worm-holes may be seen still adhering to the rocks, and when I was making the great horse at Milan a large sack of those which had been found in these parts was brought to my workshop by some peasants... The red stone of the mountains of Verona is found with shells all intermingled, which have become part of this stone... And if you should say that these shells have been and still constantly are being created in such places as these by the nature of the locality or by potency of the heavens in these spots, such an opinion cannot exist in brains possessed of any extensive powers of reasoning because the years of their growth are numbered upon the outer coverings of their shells; and both small and large ones may be seen; and these would not have grown without feeding, or fed without movement, and here [embedded in rock] they would not have been able to move... The peaks of the Apennines once stood up in a sea, in the form of islands surrounded by salt water... and above the plains of Italy where flocks of birds are flying today, fishes were once moving in large shoals.
'Physical Geography', in The Notebooks of Leonardo da Vinci, trans. E. MacCurdy (1938), Vol. 1, 355-6, 359.
In the summer after kindergarten, a friend introduced me to the joys of building plastic model airplanes and warships. By the fourth grade, I graduated to an erector set and spent many happy hours constructing devices of unknown purpose where the main design criterion was to maximize the number of moving parts and overall size. The living room rug was frequently littered with hundreds of metal “girders” and tiny nuts and bolts surrounding half-finished structures. An understanding mother allowed me to keep the projects going for days on end.
Autobiography in Gösta Ekspong (ed.), Nobel Lectures: Physics 1996-2000 (2002), 116.
In this communication I wish first to show in the simplest case of the hydrogen atom (nonrelativistic and undistorted) that the usual rates for quantization can be replaced by another requirement, in which mention of “whole numbers” no longer occurs. Instead the integers occur in the same natural way as the integers specifying the number of nodes in a vibrating string. The new conception can be generalized, and I believe it touches the deepest meaning of the quantum rules.
'Quantisierung als Eigenwertproblem', Annalen der Physik (1926), 79, 361. Trans. Walter Moore, Schrödinger: Life and Thought (1989), 200-2.
Indeed, if one understands by algebra the application of arithmetic operations to composite magnitudes of all kinds, whether they be rational or irrational number or space magnitudes, then the learned Brahmins of Hindostan are the true inventors of algebra.
In Geschichte der Mathematik im Altertum und im Mittelalter (1874), 195. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 284. From the original German, “Ja, wenn man unter Algebra die Anwendung arithmetischer Operationen auf zusammengesetzte Grössen aller Art, mögen sie rationale oder irrationale Zahl- oder Raumgrössen sein, versteht, so sind die gelehrten Brahmanen Hindustans die wahren Erfinder der Algebra.”
Induction. The mental operation by which from a number of individual instances, we arrive at a general law. The process, according to Hamilton, is only logically valid when all the instances included in the law are enumerated. This being seldom, if ever, possible, the conclusion of an Induction is usually liable to more or less uncertainty, and Induction is therefore incapable of giving us necessary (general) truths.
Stated as narrative, not a direct quote, by his biographer W.H.S. Monck in 'Glossary of Philosophical Terms', appended in Sir William Hamilton (1881), 181.
Isolated facts and experiments have in themselves no value, however great their number may be. They only become valuable in a theoretical or practical point of view when they make us acquainted with the law of a series of uniformly recurring phenomena, or, it may be, only give a negative result showing an incompleteness in our knowledge of such a law, till then held to be perfect.
'The Aim and Progress of Physical Science' (1869). Trans. E. Atkinson, Popular Lectures on Scientific Subjects (1873), 369.
It always bothers me that according to the laws as we understand them today, it takes a computing machine an infinite number of logical operations to figure out what goes on in no matter how tiny a region of space and no matter how tiny a region of time … I have often made the hypothesis that ultimately physics will not require a mathematical statement, that in the end the machinery will be revealed and the laws will turn out to be simple, like the chequer board with all its apparent complexities. But this speculation is of the same nature as those other people make—“I like it”,“I don't like it”—and it is not good to be too prejudiced about these things.
In The Character of Physical Law (1965, 2001), 57.
It appears that all that can be, is. The Creator’s hand does not appear to have been opened in order to give existence to a certain determinate number of species, but it seems that it has thrown out all at once a world of relative and non-relative creatures, an infinity of harmonic and contrary combinations and a perpetuity of destructions and replacements. What idea of power is not given us by this spectacle! What feeling of respect for its Author is not inspired in us by this view of the universe!
In 'Premier Discours: De la Manière d'Étudier et de Traiter l'Histoire naturelle', Histoire Naturelle, Generale et Particulière, Avec la Description du Cabinet du Roi (1749), Vol. I, 11. Trans. Phillip R. Sloan.
It appears that the extremely important papers that trigger a revolution may not receive a proportionately large number of citations. The normal procedures of referencing are not used for folklore. A real scientific revolution, like any other revolution, is news. The Origin of Species sold out as fast as it could be printed and was denounced from the pulpit almost immediately. Sea-floor spreading has been explained, perhaps not well, in leading newspapers, magazines, books, and most recently in a color motion picture. When your elementary school children talk about something at dinner, you rarely continue to cite it.
'Citations in a Scientific Revolution', in R. Shagam et al., Studies in Earth and Space Sciences: A Memoir in Honor of Harry Hammond Hess (1972), 4.
It can happen to but few philosophers, and but at distant intervals, to snatch a science, like Dalton, from the chaos of indefinite combination, and binding it in the chains of number, to exalt it to rank amongst the exact. Triumphs like these are necessarily 'few and far between.'
Reflections on the Decline of Science in England (1830), 22.
It has been asserted … that the power of observation is not developed by mathematical studies; while the truth is, that; from the most elementary mathematical notion that arises in the mind of a child to the farthest verge to which mathematical investigation has been pushed and applied, this power is in constant exercise. By observation, as here used, can only be meant the fixing of the attention upon objects (physical or mental) so as to note distinctive peculiarities—to recognize resemblances, differences, and other relations. Now the first mental act of the child recognizing the distinction between one and more than one, between one and two, two and three, etc., is exactly this. So, again, the first geometrical notions are as pure an exercise of this power as can be given. To know a straight line, to distinguish it from a curve; to recognize a triangle and distinguish the several forms—what are these, and all perception of form, but a series of observations? Nor is it alone in securing these fundamental conceptions of number and form that observation plays so important a part. The very genius of the common geometry as a method of reasoning—a system of investigation—is, that it is but a series of observations. The figure being before the eye in actual representation, or before the mind in conception, is so closely scrutinized, that all its distinctive features are perceived; auxiliary lines are drawn (the imagination leading in this), and a new series of inspections is made; and thus, by means of direct, simple observations, the investigation proceeds. So characteristic of common geometry is this method of investigation, that Comte, perhaps the ablest of all writers upon the philosophy of mathematics, is disposed to class geometry, as to its method, with the natural sciences, being based upon observation. Moreover, when we consider applied mathematics, we need only to notice that the exercise of this faculty is so essential, that the basis of all such reasoning, the very material with which we build, have received the name observations. Thus we might proceed to consider the whole range of the human faculties, and find for the most of them ample scope for exercise in mathematical studies. Certainly, the memory will not be found to be neglected. The very first steps in number—counting, the multiplication table, etc., make heavy demands on this power; while the higher branches require the memorizing of formulas which are simply appalling to the uninitiated. So the imagination, the creative faculty of the mind, has constant exercise in all original mathematical investigations, from the solution of the simplest problems to the discovery of the most recondite principle; for it is not by sure, consecutive steps, as many suppose, that we advance from the known to the unknown. The imagination, not the logical faculty, leads in this advance. In fact, practical observation is often in advance of logical exposition. Thus, in the discovery of truth, the imagination habitually presents hypotheses, and observation supplies facts, which it may require ages for the tardy reason to connect logically with the known. Of this truth, mathematics, as well as all other sciences, affords abundant illustrations. So remarkably true is this, that today it is seriously questioned by the majority of thinkers, whether the sublimest branch of mathematics,—the infinitesimal calculus—has anything more than an empirical foundation, mathematicians themselves not being agreed as to its logical basis. That the imagination, and not the logical faculty, leads in all original investigation, no one who has ever succeeded in producing an original demonstration of one of the simpler propositions of geometry, can have any doubt. Nor are induction, analogy, the scrutinization of premises or the search for them, or the balancing of probabilities, spheres of mental operations foreign to mathematics. No one, indeed, can claim preeminence for mathematical studies in all these departments of intellectual culture, but it may, perhaps, be claimed that scarcely any department of science affords discipline to so great a number of faculties, and that none presents so complete a gradation in the exercise of these faculties, from the first principles of the science to the farthest extent of its applications, as mathematics.
In 'Mathematics', in Henry Kiddle and Alexander J. Schem, The Cyclopedia of Education, (1877.) As quoted and cited in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 27-29.
It has been said that no science is established on a firm basis unless its generalisations can be expressed in terms of number, and it is the special province of mathematics to assist the investigator in finding numerical relations between phenomena. After experiment, then mathematics. While a science is in the experimental or observational stage, there is little scope for discerning numerical relations. It is only after the different workers have “collected data” that the mathematician is able to deduce the required generalisation. Thus a Maxwell followed Faraday and a Newton completed Kepler.
In Higher Mathematics for Students of Chemistry and Physics (1902), 3.
It has been said that numbers rule the world; but I know that the numbers teach us, whether it is governed well or badly.
English using Google translate from the original German, “Man hat behauptet, die Welt werde durch Zahlen regiert; das aber weiss ich, dass die Zahlen uns belehren, ob sie gut oder schlecht regiert werde,” in J.P. Eckermann, entry for Sonntag den 31 Januar 1830', Gespräche mit Goethe (1836), 180. However, notice that in the original context, the sense of Zahen is “pay.” In the translation by S.M. Fuller, the full context is thus: “Goethe read in the French periodical, the ‘Times,’ an article on the enormous salaries of the English clergy, who receive more than all other ecclesiastics in Christendom put together. ‘It has been maintained,’ said Goethe, ‘that the world is governed by pay; this I know, by examining the distribution of pay, we can find out whether it is well or ill governed.’” In J.P. Eckermann and S.M. Fuller (trans.), 'Sunday, 31st January', Conversations with Goethe in the Last Years of His Life (1839), 336. Yet commonly seen on the web as “It has been said that figures rule the world. Maybe. But I am sure that figures show us whether it is being ruled well or badly.”
It has just occurred to me to ask if you are familiar with Lissajous’ experiments. I know nothing about them except what I found in Flammarion’s great “Astronomie Populaire.” One extraordinary chapter on numbers gives diagrams of the vibrations of harmonics—showing their singular relation to the geometrical designs of crystal-formation;—and the chapter is aptly closed by the Pythagorian quotation: Ἀεὶ ὁ θεὸς ὁ μέγας γεωμετρεῖ—“God geometrizes everywhere.” … I should imagine that the geometry of a fine opera would—were the vibrations outlined in similar fashion—offer a network of designs which for intricate beauty would double discount the arabesque of the Alhambra.
In letter to H.E. Krehbiel (1887), collected in Elizabeth Bisland The Writings of Lafcadio Hearn (1922), Vol. 14, 8.
It is a great thing to start life with a small number of really good books which are your very own.
In Through the Magic Door (1908), Chap. 2, 24.
It is a profoundly erroneous truism, repeated by all copy-books and eminent people when they are making speeches, that we should cultivate habit of thinking of what we are doing. The precise opposite is the case. Civilization advances by extending the number of important operations which we can perform without thinking about them. Operations of thought are like cavalry charges in a battle—they are strictly limited in number, they require fresh horses, and must only be made at decisive moments.
In An Introduction to Mathematics (1911), 61.
It is a right, yes a duty, to search in cautious manner for the numbers, sizes, and weights, the norms for everything [God] has created. For He himself has let man take part in the knowledge of these things ... For these secrets are not of the kind whose research should be forbidden; rather they are set before our eyes like a mirror so that by examining them we observe to some extent the goodness and wisdom of the Creator.
Epitome of Copernican Astronomy. In Michael B. Foster, Mystery and Philosophy, 61. Cited by Max Casper and Doris Hellman, trans., ed. Kepler (1954), 381. Cited by Gerald J. Galgan, Interpreting the Present: Six Philosophical Essays (1993), 105.
Gerald J. Galgan
It is agreed that all sound which is the material of music is of three sorts. First is harmonica, which consists of vocal music; second is organica, which is formed from the breath; third is rhythmica, which receives its numbers from the beat of the fingers. For sound is produced either by the voice, coming through the throat; or by the breath, coming through the trumpet or tibia, for example; or by touch, as in the case of the cithara or anything else that gives a tuneful sound on being struck.
Etymologies [c.600], Book III, chapter 19, quoted in E. Grant (ed.), A Source Book in Medieval Science (1974), trans. E. Brehaut (1912), revised by E. Grant, 10.
It is always noteworthy that all those who seriously study this science [the theory of numbers] conceive a sort of passion for it.
Letter to Jonos Boyai (2 Sep 1808). Quoted in G. Waldo Dunnington, Carl Friedrich Gauss: Titan of Science (2004), 413.
It is arguable whether the human race have been gainers by the march of science beyond the steam engine. Electricity opens a field of infinite conveniences to ever greater numbers, but they may well have to pay dearly for them. But anyhow in my thought I stop short of the internal combustion engine which has made the world so much smaller. Still more must we fear the consequences of entrusting a human race so little different from their predecessors of the so-called barbarous ages such awful agencies as the atomic bomb. Give me the horse.
Address to the Royal College of Surgeons (10 Jul 1951). Collected in Stemming the Tide: Speeches 1951 and 1952 (1953), 91.
It is clear that in maize, seemingly blending is really segregating inheritance, but with entire absence of dominance, and it seems probably that the same will be found to be true among rabbits and other mammals; failure to observe it hitherto is probably due to the fact that the factors concerned are numerous. For the greater the number of factors concerned, the more nearly will the result obtained approximate a complete and permanent blend. As the number of factors approaches infinity, the result will become identical with a permanent blend.
Heredity: In Relation to Evolution and Animal Breeding (1911), 138-9.
It is contrary to the usual order of things, that events so harmonious as those of the system of the world, should depend on such diversified agents as are supposed to exist in our artificial arrangements; and there is reason to anticipate a great reduction in the number of undecompounded bodies, and to expect that the analogies of nature will be found conformable to the refined operations of art. The more the phenomena of the universe are studied, the more distinct their connection appears, and the more simple their causes, the more magnificent their design, and the more wonderful the wisdom and power of their Author.
Elements of Chemical Philosophy (1812), in J. Davy (ed.), The Collected Works of Sir Humphry Davy(1839-40), Vol. 4, 42.
It is curious to observe how differently these great men [Plato and Bacon] estimated the value of every kind of knowledge. Take Arithmetic for example. Plato, after speaking slightly of the convenience of being able to reckon and compute in the ordinary transactions of life, passes to what he considers as a far more important advantage. The study of the properties of numbers, he tells us, habituates the mind to the contemplation of pure truth, and raises us above the material universe. He would have his disciples apply themselves to this study, not that they may be able to buy or sell, not that they may qualify themselves to be shop-keepers or travelling merchants, but that they may learn to withdraw their minds from the ever-shifting spectacle of this visible and tangible world, and to fix them on the immutable essences of things.
Bacon, on the other hand, valued this branch of knowledge only on account of its uses with reference to that visible and tangible world which Plato so much despised. He speaks with scorn of the mystical arithmetic of the later Platonists, and laments the propensity of mankind to employ, on mere matters of curiosity, powers the whole exertion of which is required for purposes of solid advantage. He advises arithmeticians to leave these trifles, and employ themselves in framing convenient expressions which may be of use in physical researches.
Bacon, on the other hand, valued this branch of knowledge only on account of its uses with reference to that visible and tangible world which Plato so much despised. He speaks with scorn of the mystical arithmetic of the later Platonists, and laments the propensity of mankind to employ, on mere matters of curiosity, powers the whole exertion of which is required for purposes of solid advantage. He advises arithmeticians to leave these trifles, and employ themselves in framing convenient expressions which may be of use in physical researches.
In 'Lord Bacon', Edinburgh Review (Jul 1837). Collected in Critical and Miscellaneous Essays: Contributed to the Edinburgh Review (1857), Vol. 1, 394.
It is easy to create an interstellar radio message which can be recognized as emanating unambiguously from intelligent beings. A modulated signal (‘beep,’ ‘beep-beep,’…) comprising the numbers 1, 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, for example, consists exclusively of the first 12 prime numbers…. A signal of this kind, based on a simple mathematical concept, could only have a biological origin. … But by far the most promising method is to send pictures.
From 'The Quest for Extraterrestrial Intelligence', in the magazine Smithsonian (May 1978), 43-44. Reprinted in Cosmic Search (Mar 1979), 1, No. 2, 5.
It is going to be necessary that everything that happens in a finite volume of space and time would have to be analyzable with a finite number of logical operations. The present theory of physics is not that way, apparently. It allows space to go down into infinitesimal distances, wavelengths to get infinitely great, terms to be summed in infinite order, and so forth; and therefore, if this proposition [that physics is computer-simulatable] is right, physical law is wrong.
International Journal of Theoretical Physics (1982), 21 Nos. 6-7, 468. Quoted in Brian Rotman, Mathematics as Sign (2000), 82.
It is India that gave us the ingenious method of expressing all numbers by means of ten symbols, each symbol receiving a value of position as well as an absolute value; a profound and important idea which appears so simple to us now that we ignore its true merit. But its very simplicity and the great ease which it has lent to computations put our arithmetic in the first rank of useful inventions; and we shall appreciate the grandeur of the achievement the more when we remember that it escaped the genius of Archimedes and Apollonius, two of the greatest men produced by antiquity.
Quoted in Return to Mathematical Circles H. Eves (Boston 1988).
It is known that there are an infinite number of worlds, simply because there is an infinite amount of space for them to be in. However, not every one of them is inhabited. Therefore, there must be a finite number of inhabited worlds. Any finite number divided by infinity is as near to nothing as makes no odds, so the average population of all the planets in the Universe can be said to be zero. From this it follows that the population of the whole Universe is also zero, and that any people you may meet from time to time are merely the products of a deranged imagination.
In The Restaurant at the End of the Universe (1980, 2005), 142-143. Slightly revised from 'Fit the Fifth', The Original Hitchhiker Radio Scripts (1985), 102. The show was recorded for the BBC on 21 Feb 1978.
It is not necessary to probe into the nature of things, as was done by those whom the Greeks call physici; nor need we be in alarm lest the Christian should be ignorant of the force and number of the elements—the motion, and order, and eclipses of the heavenly bodies; the form of the heavens; the species and the natures of animals, plants, stones, fountains, rivers, mountains; about chronology and distances; the signs of coming storms; and a thousand other things which those philosophers either have found out, or think they have found out. … It is enough for the Christian to believe that the only cause of all created things, whether heavenly or earthly … is the goodness of the Creator, the one true God.
In Marcus Dods (ed.), J.F. Shaw (trans.), The Enchiridion of Augustine, Chap. 9, collected in The Works of Aurelius Augustine, Bishop of Hippo: A new translation (1873), Vol. 9, 180-181. The physici are natural philosophers.
It is not of the essence of mathematics to be conversant with the ideas of number and quantity. Whether as a general habit of mind it would be desirable to apply symbolic processes to moral argument, is another question.
An Investigation of the Laws of Thought (1854), 12.
It is not surprising that our language should be incapable of describing the processes occurring within the atoms, for, as has been remarked, it was invented to describe the experiences of daily life, and these consists only of processes involving exceedingly large numbers of atoms. Furthermore, it is very difficult to modify our language so that it will be able to describe these atomic processes, for words can only describe things of which we can form mental pictures, and this ability, too, is a result of daily experience. Fortunately, mathematics is not subject to this limitation, and it has been possible to invent a mathematical scheme—the quantum theory—which seems entirely adequate for the treatment of atomic processes; for visualization, however, we must content ourselves with two incomplete analogies—the wave picture and the corpuscular picture.
The Physical Principles of the Quantum Theory, trans. Carl Eckart and Frank C. Hoyt (1949), 11.
It is now widely realized that nearly all the “classical” problems of molecular biology have either been solved or will be solved in the next decade. The entry of large numbers of American and other biochemists into the field will ensure that all the chemical details of replication and transcription will be elucidated. Because of this, I have long felt that the future of molecular biology lies in the extension of research to other fields of biology, notably development and the nervous system.
Letter to Max Perua, 5 June 1963. Quoted in William B. Wood (ed.), The Nematode Caenorhabditis Elegans (1988), x-xi.
It is of priceless value to the human race to know that the sun will supply the needs of the earth, as to light and heat, for millions of years; that the stars are not lanterns hung out at night, but are suns like our own; and that numbers of them probably have planets revolving around them, perhaps in many cases with inhabitants adapted to the conditions existing there. In a sentence, the main purpose of the science is to learn the truth about the stellar universe; to increase human knowledge concerning our surroundings, and to widen the limits of intellectual life.
In 'The Nature of the Astronomer’s Work', North American Review (Jun 1908), 187, No. 631, 915.
It is often assumed that because the young child is not competent to study geometry systematically he need be taught nothing geometrical; that because it would be foolish to present to him physics and mechanics as sciences it is useless to present to him any physical or mechanical principles.
An error of like origin, which has wrought incalculable mischief, denies to the scholar the use of the symbols and methods of algebra in connection with his early essays in numbers because, forsooth, he is not as yet capable of mastering quadratics! … The whole infant generation, wrestling with arithmetic, seek for a sign and groan and travail together in pain for the want of it; but no sign is given them save the sign of the prophet Jonah, the withered gourd, fruitless endeavor, wasted strength.
An error of like origin, which has wrought incalculable mischief, denies to the scholar the use of the symbols and methods of algebra in connection with his early essays in numbers because, forsooth, he is not as yet capable of mastering quadratics! … The whole infant generation, wrestling with arithmetic, seek for a sign and groan and travail together in pain for the want of it; but no sign is given them save the sign of the prophet Jonah, the withered gourd, fruitless endeavor, wasted strength.
From presidential address (9 Sep 1884) to the General Meeting of the American Social Science Association, 'Industrial Education', printed in Journal of Social Science (1885), 19, 121. Collected in Francis Amasa Walker, Discussions in Education (1899), 132.
It is popular to believe that the age of the individual and, above all, of the free individual, is past in science. There are many administrators of science and a large component of the general population who believe that mass attacks can do anything, and even that ideas are obsolete. Behind this drive to the mass attack there are a number of strong psychological motives. Neither the public or the big administrator has too good an understanding of the inner continuity of science, but they both have seen its world-shaking consequences, and they are afraid of it. Both of them wish to decerebrate the scientist, even as the Byzantine State emasculated its civil servants. Moreover, the great administrator who is not sure of his own intellectual level can aggrandize himself only by cutting his scientific employees down to size.
In I am a Mathematician (1956), Epilogue, 363-364.
It is possible that in ten years’ time penicillin itself will be a back number and will be replaced by something better. It is quite certain though that to displace penicillin any newcomer will have to be very, very good.
In 'Truman Hails Fleming For Penicillin Drug', New York Times (26 Jul 1945), 17.
It is profitable nevertheless to permit ourselves to talk about 'meaningless' terms in the narrow sense if the preconditions to which all profitable operations are subject are so intuitive and so universally accepted as to form an almost unconscious part of the background of the public using the term. Physicists of the present day do constitute a homogenous public of this character; it is in the air that certain sorts of operation are valueless for achieving certain sorts of result. If one wants to know how many planets there are one counts them but does not ask a philosopher what is the perfect number.
Reflections of a Physicist (1950), 6.
It is strange that the immense variety in nature can be resolved into a series of numbers.
Lecture (Christmas 1923), 'The Atoms of Which Things Are Made'. Collected in Concerning the Nature of Things: Six Lectures Delivered at the Royal Institution (1925, 1954), 37.
It is strange that we know so little about the properties of numbers. They are our handiwork, yet they baffle us; we can fathom only a few of their intricacies. Having defined their attributes and prescribed their behavior, we are hard pressed to perceive the implications of our formulas.
In James R. Newman (ed.), 'Commentary on The Mysteries of Arithmetic', The World of Mathematics (1956), Vol. 1, 497.
It is true that Fourier had the opinion that the principal end of mathematics was public utility and the explanation of natural phenomena; but a philosopher as he is should have known that the unique end of science is the honor of the human mind and that from this point of view a question of [the theory of] number is as important as a question of the system of the world.
From letter to Legendre, translation as given in F.R. Moulton, 'The Influence of Astronomy on Mathematics', Science (10 Mar 1911), N.S. Vol. 33, No. 845, 359. A different translation begins, “It is true that M. Fourier believed…” on the Karl Jacobi Quotes web page on this site.
It is true that M. Fourier believed that the main aim of mathematics was public utility and the explanation of natural phenomena; but a philosopher of his ability ought to have known that the sole aim of science is the honour of the human intellect, and that on this ground a problem in numbers is as important as a problem on the system of the world.
In Letter to Legendre, as quoted in an Address by Emile Picard to the Congress of Science and Art, St. Louis (22 Sep 1904), translated in 'Development of Mathematical Analysis', The Mathematical Gazette (Jul 1905), 3, No. 52, 200. A different translation begins, “It is true that Fourier had the opinion…” on the Karl Jacobi Quotes web page on this site.
It is true that mathematics, owing to the fact that its whole content is built up by means of purely logical deduction from a small number of universally comprehended principles, has not unfittingly been designated as the science of the self-evident [Selbstverständlichen]. Experience however, shows that for the majority of the cultured, even of scientists, mathematics remains the science of the incomprehensible [Unverständlichen].
In Ueber Wert und angeblichen Unwert der Mathematik, Jahresbericht der Deutschen Maihemaliker Vereinigung (1904), 357.
It is we, we alone, who have dreamed up the causes, the one-thing-after-another, the one-thing-reciprocating-another, the relativity, the constraint, the numbers, the laws, the freedom, the ‘reason why,’ the purpose. ... We are creating myths.
…...
It is well known, that on the Ohio, and in many parts of America further north, tusks, grinders, and skeletons of unparalleled magnitude are found in great numbers, some lying on the surface of the earth, and some a little below it ... But to whatever animal we ascribe these remains, it is certain that such a one has existed in America, and that it has been the largest of all terrestrial beings.
Notes on the State of Virginia (1782), 71, 77.
It must … be admitted that very simple relations … exist between the volumes of gaseous substances and the numbers of simple or compound molecules which form them. The first hypothesis to present itself in this connection, and apparently even the only admissible one, is the supposition that the number of integral molecules in any gases is always the same for equal volumes, or always proportional to the volumes. Indeed, if we were to suppose that the number of molecules contained in a given volume were different for different gases, it would scarcely be possible to conceive that the law regulating the distance of molecules could give in all cases relations so simple as those which the facts just detailed compel us to acknowledge between the volume and the number of molecules.
In 'Essay on a Manner of Determining the Relative Masses of the Elementary Molecules of Bodies, and the Proportions in which they enter into these Compounds', Journal de Physique, 1811, 73, 58-76. In Foundations of the Molecular Theory; Alembic Club Reprints, Number 4 (1923), 28-9.
It often happens that men, even of the best understandings and greatest circumspection, are guilty of that fault in reasoning which the writers on logick call the insufficient, or imperfect enumeration of parts, or cases: insomuch that I will venture to assert, that this is the chief, and almost the only, source of the vast number of erroneous opinions, and those too very often in matters of great importance, which we are apt to form on all the subjects we reflect upon, whether they relate to the knowledge of nature, or the merits and motives of human actions. It must therefore be acknowledged, that the art which affords a cure to this weakness, or defect, of our understandings, and teaches us to enumerate all the possible ways in which a given number of things may be mixed and combined together, that we may be certain that we have not omitted anyone arrangement of them that can lead to the object of our inquiry, deserves to be considered as most eminently useful and worthy of our highest esteem and attention. And this is the business of the art, or doctrine of combinations ... It proceeds indeed upon mathematical principles in calculating the number of the combinations of the things proposed: but by the conclusions that are obtained by it, the sagacity of the natural philosopher, the exactness of the historian, the skill and judgement of the physician, and the prudence and foresight of the politician, may be assisted; because the business of all these important professions is but to form reasonable conjectures concerning the several objects which engage their attention, and all wise conjectures are the results of a just and careful examination of the several different effects that may possibly arise from the causes that are capable of producing them.
Ars conjectandi (1713). In F. Maseres, The Doctrine of Permutations and Combinations (1795), 36.
It seems that the increased number of scientific workers, their being split up into groups whose studies are limited to a small subject, and over-specialization have brought about a shrinking of intelligence. There is no doubt that the quality of any human group decreases when the number of the individuals composing this group increases beyond certain limits… The best way to increase the intelligence of scientists would be to decrease their number.
Man the Unknown (1935), 48-9.
It seems to me, that the only Objects of the abstract Sciences or of Demonstration is Quantity and Number, and that all Attempts to extend this more perfect Species of Knowledge beyond these Bounds are mere Sophistry and Illusion.
An Enquiry Concerning Human Understanding (1748), 252.
It was his [Leibnitz’s] love of method and order, and the conviction that such order and harmony existed in the real world, and that our success in understanding it depended upon the degree and order which we could attain in our own thoughts, that originally was probably nothing more than a habit which by degrees grew into a formal rule. This habit was acquired by early occupation with legal and mathematical questions. We have seen how the theory of combinations and arrangements of elements had a special interest for him. We also saw how mathematical calculations served him as a type and model of clear and orderly reasoning, and how he tried to introduce method and system into logical discussions, by reducing to a small number of terms the multitude of compound notions he had to deal with. This tendency increased in strength, and even in those early years he elaborated the idea of a general arithmetic, with a universal language of symbols, or a characteristic which would be applicable to all reasoning processes, and reduce philosophical investigations to that simplicity and certainty which the use of algebraic symbols had introduced into mathematics.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
A mental attitude such as this is always highly favorable for mathematical as well as for philosophical investigations. Wherever progress depends upon precision and clearness of thought, and wherever such can be gained by reducing a variety of investigations to a general method, by bringing a multitude of notions under a common term or symbol, it proves inestimable. It necessarily imports the special qualities of number—viz., their continuity, infinity and infinite divisibility—like mathematical quantities—and destroys the notion that irreconcilable contrasts exist in nature, or gaps which cannot be bridged over. Thus, in his letter to Arnaud, Leibnitz expresses it as his opinion that geometry, or the philosophy of space, forms a step to the philosophy of motion—i.e., of corporeal things—and the philosophy of motion a step to the philosophy of mind.
In Leibnitz (1884), 44-45. [The first sentence is reworded to better introduce the quotation. —Webmaster]
It would appear... that moral phenomena, when observed on a great scale, are found to resemble physical phenomena; and we thus arrive, in inquiries of this kind, at the fundamental principle, that the greater the number of individuals observed, the more do individual peculiarities, whether physical or moral, become effaced, and leave in a prominent point of view the general facts, by virtue of which society exists and is preserved.
A Treatise on Man and the Development of his Faculties (1842). Reprinted with an introduction by Solomon Diamond (1969), 6.
It would seem at first sight as if the rapid expansion of the region of mathematics must be a source of danger to its future progress. Not only does the area widen but the subjects of study increase rapidly in number, and the work of the mathematician tends to become more and more specialized. It is, of course, merely a brilliant exaggeration to say that no mathematician is able to understand the work of any other mathematician, but it is certainly true that it is daily becoming more and more difficult for a mathematician to keep himself acquainted, even in a general way, with the progress of any of the branches of mathematics except those which form the field of his own labours. I believe, however, that the increasing extent of the territory of mathematics will always be counteracted by increased facilities in the means of communication. Additional knowledge opens to us new principles and methods which may conduct us with the greatest ease to results which previously were most difficult of access; and improvements in notation may exercise the most powerful effects both in the simplification and accessibility of a subject. It rests with the worker in mathematics not only to explore new truths, but to devise the language by which they may be discovered and expressed; and the genius of a great mathematician displays itself no less in the notation he invents for deciphering his subject than in the results attained. … I have great faith in the power of well-chosen notation to simplify complicated theories and to bring remote ones near and I think it is safe to predict that the increased knowledge of principles and the resulting improvements in the symbolic language of mathematics will always enable us to grapple satisfactorily with the difficulties arising from the mere extent of the subject.
In Presidential Address British Association for the Advancement of Science, Section A., (1890), Nature, 42, 466.
It’s a numbers game—in a dense urban area there are so many of us that even unintentional pollution would cause all the crap we see in the water.
Posted by 'Lisa' (6 Mar 2008), Reply in blog 'Emerald City' to item 'The Plague that is the plastic bag, in photos', Los Angeles Times website (1 Mar 2008).
Just as a tree constitutes a mass arranged in a definite manner, in which, in every single part, in the leaves as in the root, in the trunk as in the blossom, cells are discovered to be the ultimate elements, so is it also with the forms of animal life. Every animal presents itself as a sum of vital unities, every one of which manifests all the characteristics of life. The characteristics and unity of life cannot be limited to anyone particular spot in a highly developed organism (for example, to the brain of man), but are to be found only in the definite, constantly recurring structure, which every individual element displays. Hence it follows that the structural composition of a body of considerable size, a so-called individual, always represents a kind of social arrangement of parts, an arrangement of a social kind, in which a number of individual existences are mutually dependent, but in such a way, that every element has its own special action, and, even though it derive its stimulus to activity from other parts, yet alone effects the actual performance of its duties.
In Lecture I, 'Cells and the Cellular Theory' (1858), Rudolf Virchow and Frank Chance (trans.) ,Cellular Pathology (1860), 13-14.
Just as it will never be successfully challenged that the French language, progressively developing and growing more perfect day by day, has the better claim to serve as a developed court and world language, so no one will venture to estimate lightly the debt which the world owes to mathematicians, in that they treat in their own language matters of the utmost importance, and govern, determine and decide whatever is subject, using the word in the highest sense, to number and measurement.
In 'Sprüche in Prosa', Natur, III, 868.
Just as mathematics aims to study such entities as numbers, functions, spaces, etc., the subject matter of metamathematics is mathematics itself.
In 'Mathematics: A Non-Technical Exposition', American Scientist (3 Jul 1954), 42, No. 3, 490.
Just as the introduction of the irrational numbers … is a convenient myth [which] simplifies the laws of arithmetic … so physical objects are postulated entities which round out and simplify our account of the flux of existence… The conceptional scheme of physical objects is [likewise] a convenient myth, simpler than the literal truth and yet containing that literal truth as a scattered part.
In J. Koenderink Solid Shape (1990.), 16.
Know, oh Brother (May God assist thee and us by the Spirit from Him) that God, Exalted Be His Praise, when He created all creatures and brought all things into being, arranged them and brought them into existence by a process similar to the process of generation of numbers from one, so that the multiplicity [of numbers] should be a witness to his Oneness, and their classification and order an indication of the perfection of His wisdom in creation. And this would be a witness to the fact, too, that they [creatures] are related to Him who created them, in the same way as the numbers are related to the One which is prior to two, and which is the principle, origin and source of numbers, as we have shown in our treatise on arithmetic.
Rasa'il. In Seyyed Hossein Nasr, Science and Civilisation in Islam (1968), 155-6.
Leibnitz believed he saw the image of creation in his binary arithmetic in which he employed only two characters, unity and zero. Since God may be represented by unity, and nothing by zero, he imagined that the Supreme Being might have drawn all things from nothing, just as in the binary arithmetic all numbers are expressed by unity with zero. This idea was so pleasing to Leibnitz, that he communicated it to the Jesuit Grimaldi, President of the Mathematical Board of China, with the hope that this emblem of the creation might convert to Christianity the reigning emperor who was particularly attached to the sciences.
In 'Essai Philosophique sur les Probabiliés', Oeuvres (1896), t. 7, 119.
Let us ... consider the ovum [egg] as a physical system. Its potentialities are prodigious and one's first impulse is to expect that such vast potentialities would find expression in complexity of
structure. But what do we find? The substance is clouded with particles, but these can be
centrifuged away leaving it optically structureless but still capable of development.... On the
surface of the egg there is a fine membrane, below it fluid of high viscosity, next fluid of
relatively low viscosity, and within this the nucleus, which in the resting stage is simply a bag
of fluid enclosed in a delicate membrane.... The egg's simplicity is not that of a machine or a
crystal, but that of a nebula. Gathered into it are units relatively simple but capable by their
combinations of forming a vast number of dynamical systems...
As guest of honour, closing day address (Jun 1928), Sixth Colloid Symposium, Toronto, Canada, 'Living Matter', printed in Harry Boyer Weiser (ed.), Colloid Symposium Monograph (1928), Vol. 6, 15. Quoted in Joseph Needham, Chemical Embryology (1931), Vol. 1, 612-613.
Look round the world, contemplate the whole and every part of it: you will find it to be nothing but one great machine, subdivided into an infinite number of lesser machines, which again admit of subdivisions to a degree beyond what human senses and faculties can trace and explain. All these various machines, and even their most minute parts, are adjusted to each other with an accuracy which ravishes into admiration all men who have ever contemplated them. The curious adapting of means to ends, throughout all nature, resembles exactly, though it much exceeds, the productions of human contrivance-of human design, thought, wisdom, and intelligence.
Dialogues Concerning Natural Religion (1779), 47-48.
Look somewhere else for someone who can follow you in your researches about numbers. For my part, I confess that they are far beyond me, and I am competent only to admire them.
In Letter (27 Oct 1654), the third from Pascal to Fermat. As translated in George F. Simmons, Calculus Gems: Brief Lives and Memorable Mathematics (2007), 103. From the original French, “Cherchez ailleurs qui vous suive dans vos inventions numériques,… pour moi je vous confesse que cela me passé de bien loin: je ne fuis capable que de les admirer,” in Francis Maseres,Scriptores Logarithmici: Or, a Collection of Several Curious Tracts on the Nature and Construction of Logarithms (1801), Vol. 4, 495.
Lord Kelvin was so satisfied with this triumph of science that he declared himself to be as certain of the existence of the ether as a man can be about anything.... “When you can measure what you are speaking about, and express it in numbers, you know something about it....” Thus did Lord Kelvin lay down the law. And though quite wrong, this time he has the support of official modern Science. It is NOT true that when you can measure what you are speaking about, you know something about it. The fact that you can measure something doesn't even prove that that something exists.... Take the ether, for example: didn't they measure the ratio of its elasticity to its density?
In Science is a Sacred Cow (1950), 69-70; 85.
Man is still by instinct a predatory animal given to devilish aggression.
The discoveries of science have immensely increased productivity of material things. They have increased the standards of living and comfort. They have eliminated infinite drudgery. They have increased leisure. But that gives more time for devilment.
The work of science has eliminated much disease and suffering. It has increased the length of life. That, together with increase in productivity, has resulted in vastly increased populations. Also it increased the number of people engaged in devilment.
The discoveries of science have immensely increased productivity of material things. They have increased the standards of living and comfort. They have eliminated infinite drudgery. They have increased leisure. But that gives more time for devilment.
The work of science has eliminated much disease and suffering. It has increased the length of life. That, together with increase in productivity, has resulted in vastly increased populations. Also it increased the number of people engaged in devilment.
Address delivered to Annual Meeting of the York Bible Class, Toronto, Canada (22 Nov 1938), 'The Imperative Need for Moral Re-armament', collected in America's Way Forward (1939), 50.
Marx founded a new science: the science of history. … The sciences we are familiar with have been installed in a number of great “continents”. Before Marx, two such continents had been opened up to scientific knowledge: the continent of Mathematics and the continent of Physics. The first by the Greeks (Thales), the second by Galileo. Marx opened up a third continent to scientific knowledge: the continent of History.
In Lenin and Philosophy, and Other Writings (1971), 4.
Mathematical knowledge is not—as all Cambridge men are surely aware—the result of any special gift. It is merely the development of those conceptions of form and number which every human being possesses; and any person of average intellect can make himself a fair mathematician if he will only pay continuous attention; in plain English, think enough about the subject.
'Science', a lecture delivered at the Royal Institution. The Works of Charles Kingsley (1880), 241.
Mathematicians deal with possible worlds, with an infinite number of logically consistent systems. Observers explore the one particular world we inhabit. Between the two stands the theorist. He studies possible worlds but only those which are compatible with the information furnished by observers. In other words, theory attempts to segregate the minimum number of possible worlds which must include the actual world we inhabit. Then the observer, with new factual information, attempts to reduce the list further. And so it goes, observation and theory advancing together toward the common goal of science, knowledge of the structure and observation of the universe.
Lecture to Sigma Xi, 'The Problem of the Expanding Universe' (1941), printed in Sigma Xi Quarterly (1942), 30, 104-105. Reprinted in Smithsonian Institution Report of the Board of Regents (1943), 97, 123. As cited by Norriss S. Hetherington in 'Philosophical Values and Observation in Edwin Hubble's Choice of a Model of the Universe', Historical Studies in the Physical Sciences (1982), 13, No. 1, 63.
Mathematicians have tried in vain to this day to discover some order in the sequence of prime numbers, and we have reason to believe that it is a mystery into which the human mind will never penetrate.
As quoted in G. Simmons Calculus Gems (1992).
MATHEMATICS … the general term for the various applications of mathematical thought, the traditional field of which is number and quantity. It has been usual to define mathematics as “the science of discrete and continuous magnitude.”
Opening statement in article 'Mathematics', Encyclopedia Britannica (1911, 11th ed.), Vol. 17, 878. Whitehead then indicated this was an inadequate definition, which he then discussed at length and tried to give an improved definition later in the article. See the quote beginning “Definition of Mathematics…” on the Alfred North Whitehead Quotes page on this website.
Mathematics contain a great number of premises, and there is perhaps a kind of intellect that can search with ease a few premises to the bottom, and cannot in the least penetrate those matters in which there are many premises.
In Pascal’s Pensées (1958), 3.
Mathematics is not a book confined within a cover and bound between brazen clasps, whose contents it needs only patience to ransack; it is not a mine, whose treasures may take long to reduce into possession, but which fill only a limited number of veins and lodes; it is not a soil, whose fertility can be exhausted by the yield of successive harvests; it is not a continent or an ocean, whose area can be mapped out and its contour defined: it is limitless as that space which it finds too narrow for its aspirations; its possibilities are as infinite as the worlds which are forever crowding in and multiplying upon the astronomer’s gaze; it is as incapable of being restricted within assigned boundaries or being reduced to definitions of permanent validity, as the consciousness of life, which seems to slumber in each monad, in every atom of matter, in each leaf and bud cell, and is forever ready to burst forth into new forms of vegetable and animal existence.
From Commemoration Day Address (22 Feb 1877) at Johns Hopkins University, Baltimore, collected in The Collected Mathematical Papers: (1870-1883) (1909), 77-78.
Mathematics is that peculiar science in which the importance of a work can be measured by the number of earlier publications rendered superfluous by it.
As stated in narrative, without quotation marks, in Joong Fang, Bourbaki (1970), 18, citing “as Hilbert declared at the end of his famous paper on the twenty-three unsolved problems.” Webmaster has not identified this in that paper, however. Also quoted, without citation, in Harold Eves, Mathematical Circles Revisited (1971), as “One can measure the importance of a scientific work by the number of earlier publications rendered superfluous by it.”
Mathematics is the queen of the sciences and arithmetic [number theory] is the queen of mathematics. She often condescends to render service to astronomy and other natural sciences, but in all relations, she is entitled to first rank.
I>Sartorius von
Waltershausen: Gauss zum Gedächtniss (1856), 79. Quoted in Robert Edouard Moritz, Memorabilia
Mathematica (1914), 271.
Mathematics is the science of the functional laws and transformations which enable us to convert figured extension and rated motion into number.
In 'The Departments of Mathematics, and their Mutual Relations', Journal of Speculative Philosophy (Apr 1871), 5, No. 2, 170.
Mathematics, including not merely Arithmetic, Algebra, Geometry, and the higher Calculus, but also the applied Mathematics of Natural Philosophy, has a marked and peculiar method or character; it is by preeminence deductive or demonstrative, and exhibits in a
nearly perfect form all the machinery belonging to this mode of obtaining truth. Laying down a very small number of first principles, either self-evident or requiring very little effort to prove them, it evolves a vast number of deductive truths and applications, by a procedure in the highest degree mathematical and systematic.
In Education as a Science (1879), 148.
Measure, time and number are nothing but modes of thought or rather of imagination.
Letter to Ludvicus Meyer (20 Apr 1663), in Correspondence of Spinoza (2003), 118.
Mere numbers cannot bring out … the intimate essence of the experiment. This conviction comes naturally when one watches a subject at work. … What things can happen! What reflections, what remarks, what feelings, or, on the other hand, what blind automatism, what absence of ideas! … The experimenter judges what may be going on in [the subject’s] mind, and certainly feels difficulty in expressing all the oscillations of a thought in a simple, brutal number, which can have only a deceptive precision. How, in fact, could it sum up what would need several pages of description!
In La Suggestibilité (1900), 119-20.
Might one not say that in the chance combination of nature's production, since only those endowed with certain relations of suitability could survive, it is no cause for wonder that this suitability is found in all species that exist today? Chance, one might say, produced an innumerable multitude of individuals; a small number turned out to be constructed in such fashion that the parts of the animal could satisfy its needs; in another, infinitely greater number, there was neither suitability nor order: all of the later have perished; animals without a mouth could not live, others lacking organs for reproduction could not perpetuate themselves: the only ones to have remained are those in which were found order and suitability; and these species, which we see today, are only the smallest part of what blind fate produced.
'Essai de Cosmologie' in Oeuvres de Mr. De Maupertuis (1756), Vol. 1, 11-12. Quoted in Jacques Roger, The Life Sciences in Eighteenth-Century French Thought, ed. Keith R. Benson and trans. Robert Ellrich (1997), 381.
Money. It has such an inherent power to run itself clear of taint that human ingenuity cannot devise the means of making it work permanent mischief, any more than means can be found of torturing people beyond what they can bear. Even if a man founds a College of Technical Instruction, the chances are ten to one that no one will be taught anything and that it will have been practically left to a number of excellent professors who will know very well what to do with it.
Samuel Butler, Henry Festing Jones (ed.), The Note-Books of Samuel Butler (1917), 221.
Most discussions of the population crisis lead logically to zero population growth as the ultimate goal, because any growth rate, if continued, will eventually use up the earth... Turning to the actual measures taken we see that the very use of family planning as the means for implementing population policy poses serious but unacknowledged limits the intended reduction in fertility. The family-planning movement, clearly devoted to the improvement and dissemination of contraceptive devices, states again and again that its purpose is that of enabling couples to have the number of children they want.
With the publication of this article 'zero population growth' and the acronym 'ZPG' came into general use.
With the publication of this article 'zero population growth' and the acronym 'ZPG' came into general use.
'Population Policy: Will Current Programs Succeed?', Science, 1967, 158, 732.
Most of his [Euler’s] memoirs are contained in the transactions of the Academy of Sciences at St. Petersburg, and in those of the Academy at Berlin. From 1728 to 1783 a large portion of the Petropolitan transactions were filled by his writings. He had engaged to furnish the Petersburg Academy with memoirs in sufficient number to enrich its acts for twenty years—a promise more than fulfilled, for down to 1818 [Euler died in 1793] the volumes usually contained one or more papers of his. It has been said that an edition of Euler’s complete works would fill 16,000 quarto pages.
In History of Mathematics (1897), 263-264.
Most people today still believe, perhaps unconsciously, in the heliocentric universe. ... Every newspaper in the land has a section on astrology, yet few have anything at all on astronomy.
[Realizing that his plasma universe may take a long time to penetrate the popular consciousness. When addressing a number of physicists with the first half of the quote, the groups was at first incredulous, but nodded agreement upon hearing the remainder of the quote.]
[Realizing that his plasma universe may take a long time to penetrate the popular consciousness. When addressing a number of physicists with the first half of the quote, the groups was at first incredulous, but nodded agreement upon hearing the remainder of the quote.]
Quoted in Anthony L. Peratt, 'Dean of the Plasma Dissidents', Washington Times, supplement: The World and I (May 1988),196.
My own thinking (and that of many of my colleagues) is based on two general principles, which I shall call the Sequence Hypothesis and the Central Dogma. The direct evidence for both of them is negligible, but I have found them to be of great help in getting to grips with these very complex problems. I present them here in the hope that others can make similar use of them. Their speculative nature is emphasized by their names. It is an instructive exercise to attempt to build a useful theory without using them. One generally ends in the wilderness.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
The Sequence Hypothesis
This has already been referred to a number of times. In its simplest form it assumes that the specificity of a piece of nucleic acid is expressed solely by the sequence of its bases, and that this sequence is a (simple) code for the amino acid sequence of a particular protein...
The Central Dogma
This states that once 'information' has passed into protein it cannot get out again. In more detail, the transfer of information from nucleic acid to nucleic acid, or from nucleic acid to protein may be possible, but transfer from protein to protein, or from protein to nucleic acid is impossible. Information means here the precise determination of sequence, either of bases in the nucleic acid or of amino acid residues in the protein. This is by no means universally held—Sir Macfarlane Burnet, for example, does not subscribe to it—but many workers now think along these lines. As far as I know it has not been explicitly stated before.
'On Protein Synthesis', Symposia of the Society for Experimental Biology: The Biological Replication of Macromolecules, 1958, 12, 152-3.
My theory of electrical forces is that they are called into play in insulating media by slight electric displacements, which put certain small portions of the medium into a state of distortion which, being resisted by the elasticity of the medium, produces an electromotive force ... I suppose the elasticity of the sphere to react on the electrical matter surrounding it, and press it downwards.
From the determination by Kohlrausch and Weber of the numerical relation between the statical and magnetic effects of electricity, I have determined the elasticity of the medium in air, and assuming that it is the same with the luminiferous ether I have determined the velocity of propagation of transverse vibrations.
The result is
193088 miles per second
(deduced from electrical & magnetic experiments).
Fizeau has determined the velocity of light
= 193118 miles per second
by direct experiment.
This coincidence is not merely numerical. I worked out the formulae in the country, before seeing Webers [sic] number, which is in millimetres, and I think we have now strong reason to believe, whether my theory is a fact or not, that the luminiferous and the electromagnetic medium are one.
From the determination by Kohlrausch and Weber of the numerical relation between the statical and magnetic effects of electricity, I have determined the elasticity of the medium in air, and assuming that it is the same with the luminiferous ether I have determined the velocity of propagation of transverse vibrations.
The result is
193088 miles per second
(deduced from electrical & magnetic experiments).
Fizeau has determined the velocity of light
= 193118 miles per second
by direct experiment.
This coincidence is not merely numerical. I worked out the formulae in the country, before seeing Webers [sic] number, which is in millimetres, and I think we have now strong reason to believe, whether my theory is a fact or not, that the luminiferous and the electromagnetic medium are one.
Letter to Michael Faraday (19 Oct 1861). In P. M. Harman (ed.), The Scientific Letters and Papers of James Clerk Maxwell (1990), Vol. 1, 1846-1862, 684-6.
Natural causes, as we know, are at work, which tend to modify, if they do not at length destroy, all the arrangements and dimensions of the earth and the whole solar system. But though in the course of ages catastrophes have occurred and may yet occur in the heavens, though ancient systems may be dissolved and new systems evolved out of their ruins, the molecules [i.e. atoms] out of which these systems are built—the foundation stones of the material universe—remain unbroken and unworn. They continue to this day as they were created—perfect in number and measure and weight.
Lecture to the British Association at Bradford, 'Molecules', Nature (1873), 8, 437-441. Reprinted in James Clerk Maxwell and W. D. Niven, editor, The Scientific Papers of James Clerk Maxwell (2003), 377.
By
Naturally, some intriguing thoughts arise from the discovery that the three chief particles making up matter—the proton, the neutron, and the electron—all have antiparticles. Were particles and antiparticles created in equal numbers at the beginning of the universe? If so, does the universe contain worlds, remote from ours, which are made up of antiparticles?
In The Intelligent Man's Guide to the Physical Sciences (1960, 1968), 222. Also in Isaac Asimov’s Book of Science and Nature Quotations (1988), 138.
Nature ... tends to repeat the same organs in the same number and in the same relations, and varies to infinity only their form. In accordance with this principle I shall have to draw my conclusions, in the determining the bones of the fish's skull, not from a consideration of their form, but from a consideration of their connections.
'Considérations sur les pieces de la tête osseuse des animaux vertebras, et particulièrement sur celle du crane des oiseaux', Annales du Museum d'Histoire Naturelle, 1807, 10, 344. Trans. E. S. Russell, Form and Function (1916), 71.
Nature becomes fertile only by virtue of laws that oblige matter to organize itself into one of a number of necessarily very simple primitive forms. Because of their very simplicity, these are capable of constituting the basis for increasingly complex bodies, by the addition of organs calculated according to identical laws of possibility.
'Matiere', Dictionnaire Classique d' Histoire Naturelle (1822-31), Vol. 10, 277, trans. J. Mandelbaum. Quoted in Pietro Corsi, The Age of Lamarck (1988), 225.
Nature progresses by unknown gradations and consequently does not submit to our absolute division when passing by imperceptible nuances, from one species to another and often from one genus to another. Inevitably there are a great number of equivocal species and in-between specimens that one does not know where to place and which throw our general systems into turmoil.
Jean Piveteau (ed.), Oeuvres Philosophiques de Buffon (1965), 10. Trans. in Paul Farber, 'Buffon and the Concept of Species', in Journal of the History of Biology, 1972, 5, 260.
Nature, … in order to carry out the marvelous operations [that occur] in animals and plants has been pleased to construct their organized bodies with a very large number of machines, which are of necessity made up of extremely minute parts so shaped and situated as to form a marvelous organ, the structure and composition of which are usually invisible to the naked eye without the aid of a microscope. … Just as Nature deserves praise and admiration for making machines so small, so too the physician who observes them to the best of his ability is worthy of praise, not blame, for he must also correct and repair these machines as well as he can every time they get out of order.
'Reply to Doctor Sbaraglia' in Opera Posthuma (1697), in H. B. Adelmann (ed.), Marcello Malpighi and the Evolution of Embryology (1966), Vol. 1, 568.
Neither in the subjective nor in the objective world can we find a criterion for the reality of the number concept, because the first contains no such concept, and the second contains nothing that is free from the concept. How then can we arrive at a criterion? Not by evidence, for the dice of evidence are loaded. Not by logic, for logic has no existence independent of mathematics: it is only one phase of this multiplied necessity that we call mathematics.
How then shall mathematical concepts be judged? They shall not be judged. Mathematics is the supreme arbiter. From its decisions there is no appeal. We cannot change the rules of the game, we cannot ascertain whether the game is fair. We can only study the player at his game; not, however, with the detached attitude of a bystander, for we are watching our own minds at play.
How then shall mathematical concepts be judged? They shall not be judged. Mathematics is the supreme arbiter. From its decisions there is no appeal. We cannot change the rules of the game, we cannot ascertain whether the game is fair. We can only study the player at his game; not, however, with the detached attitude of a bystander, for we are watching our own minds at play.
In Number: The Language of Science; a Critical Survey Written for the Cultured Non-Mathematician (1937), 244-245.
Newton was probably responsible for the concept that there are seven primary colours in the spectrum—he had a strong interest in musical harmonies and, since there are seven distinct notes in the musical scale, he divided up the spectrum into spectral bands with widths corresponding to the ratios of the small whole numbers found in the just scale.
In 'Light and Colour', Trevor Lamb and Janine Bourriau, Colour: Art & Science (1995), 72.
No Geologist worth anything is permanently bound to a desk or laboratory, but the charming notion that true science can only be based on unbiased observation of nature in the raw is mythology. Creative work, in geology and anywhere else, is interaction and synthesis: half-baked ideas from a bar room, rocks in the field, chains of thought from lonely walks, numbers squeezed from rocks in a laboratory, numbers from a calculator riveted to a desk, fancy equipment usually malfunctioning on expensive ships, cheap equipment in the human cranium, arguments before a road cut.
An Urchin in the Storm (1988), 98.
No history of civilization can be tolerably complete which does not give considerable space to the explanation of scientific progress. If we had any doubts about this, it would suffice to ask ourselves what constitutes the essential difference between our and earlier civilizations. Throughout the course of history, in every period, and in almost every country, we find a small number of saints, of great artists, of men of science. The saints of to-day are not necessarily more saintly than those of a thousand years ago; our artists are not necessarily greater than those of early Greece; they are more likely to be inferior; and of course, our men of science are not necessarily more intelligent than those of old; yet one thing is certain, their knowledge is at once more extensive and more accurate. The acquisition and systematization of positive knowledge is the only human activity which is truly cumulative and progressive. Our civilization is essentially different from earlier ones, because our knowledge of the world and of ourselves is deeper, more precise, and more certain, because we have gradually learned to disentangle the forces of nature, and because we have contrived, by strict obedience to their laws, to capture them and to divert them to the gratification of our own needs.
Introduction to the History of Science (1927), Vol. 1, 3-4.
No place affords a more striking conviction of the vanity of human hopes than a publick library; for who can see the wall crouded on every side by mighty volumes, the works of laborious meditation, and accurate inquiry, now scarcely known but by the catalogue, and preserved only to encrease the pomp of learning, without considering how many hours have been wasted in vain endeavours, how often imagination has anticipated the praises of futurity, how many statues have risen to the eye of vanity, how many ideal converts have elevated zeal, how often wit has exulted in the eternal infamy of his antagonists, and dogmatism has delighted in the gradual advances of his authority, the immutability of his decrees, and the perpetuity of his power.
Non unquam dedit
Documenta fors majora, quam fragili loco
Starent superbi.
Seneca, Troades, II, 4-6
Insulting chance ne'er call'd with louder voice,
On swelling mortals to be proud no more.
Of the innumerable authors whose performances are thus treasured up in magnificent obscurity, most are forgotten, because they never deserved to be remembered, and owed the honours which they have once obtained, not to judgment or to genius, to labour or to art, but to the prejudice of faction, the stratagem of intrigue, or the servility of adulation.
Nothing is more common than to find men whose works are now totally neglected, mentioned with praises by their contemporaries, as the oracles of their age, and the legislators of science. Curiosity is naturally excited, their volumes after long enquiry are found, but seldom reward the labour of the search. Every period of time has produced these bubbles of artificial fame, which are kept up a while by the breath of fashion and then break at once and are annihilated. The learned often bewail the loss of ancient writers whose characters have survived their works; but perhaps if we could now retrieve them we should find them only the Granvilles, Montagus, Stepneys, and Sheffields of their time, and wonder by what infatuation or caprice they could be raised to notice.
It cannot, however, be denied, that many have sunk into oblivion, whom it were unjust to number with this despicable class. Various kinds of literary fame seem destined to various measures of duration. Some spread into exuberance with a very speedy growth, but soon wither and decay; some rise more slowly, but last long. Parnassus has its flowers of transient fragrance as well as its oaks of towering height, and its laurels of eternal verdure.
Non unquam dedit
Documenta fors majora, quam fragili loco
Starent superbi.
Seneca, Troades, II, 4-6
Insulting chance ne'er call'd with louder voice,
On swelling mortals to be proud no more.
Of the innumerable authors whose performances are thus treasured up in magnificent obscurity, most are forgotten, because they never deserved to be remembered, and owed the honours which they have once obtained, not to judgment or to genius, to labour or to art, but to the prejudice of faction, the stratagem of intrigue, or the servility of adulation.
Nothing is more common than to find men whose works are now totally neglected, mentioned with praises by their contemporaries, as the oracles of their age, and the legislators of science. Curiosity is naturally excited, their volumes after long enquiry are found, but seldom reward the labour of the search. Every period of time has produced these bubbles of artificial fame, which are kept up a while by the breath of fashion and then break at once and are annihilated. The learned often bewail the loss of ancient writers whose characters have survived their works; but perhaps if we could now retrieve them we should find them only the Granvilles, Montagus, Stepneys, and Sheffields of their time, and wonder by what infatuation or caprice they could be raised to notice.
It cannot, however, be denied, that many have sunk into oblivion, whom it were unjust to number with this despicable class. Various kinds of literary fame seem destined to various measures of duration. Some spread into exuberance with a very speedy growth, but soon wither and decay; some rise more slowly, but last long. Parnassus has its flowers of transient fragrance as well as its oaks of towering height, and its laurels of eternal verdure.
The Rambler, Number 106, 23 Mar 1751. In W. J. Bate and Albrecht B. Strauss (eds.), The Rambler (1969), Vol. 2, 200-1.
No shreds of dignity encumber
The undistinguished Random Number
He has, so sad a lot is his,
No reason to be what he is.
The undistinguished Random Number
He has, so sad a lot is his,
No reason to be what he is.
In Kenneth Ewart Boulding and Richard P. Beilock (Ed.), Illustrating Economics: Beasts, Ballads and Aphorisms (1980, 2009), 153.
No! What we need are not prohibitory marriage laws, but a reformed society, an educated public opinion which will teach individual duty in these matters. And it is to the women of the future that I look for the needed reformation. Educate and train women so that they are rendered independent of marriage as a means of gaining a home and a living, and you will bring about natural selection in marriage, which will operate most beneficially upon humanity. When all women are placed in a position that they are independent of marriage, I am inclined to think that large numbers will elect to remain unmarried—in some cases, for life, in others, until they encounter the man of their ideal. I want to see women the selective agents in marriage; as things are, they have practically little choice. The only basis for marriage should be a disinterested love. I believe that the unfit will be gradually eliminated from the race, and human progress secured, by giving to the pure instincts of women the selective power in marriage. You can never have that so long as women are driven to marry for a livelihood.
In 'Heredity and Pre-Natal Influences. An Interview With Dr. Alfred Russel Wallace', Humanitarian (1894), 4, 87.
Nobody before the Pythagoreans had thought that mathematical relations held the secret of the universe. Twenty-five centuries later, Europe is still blessed and cursed with their heritage. To non-European civilizations, the idea that numbers are the key to both wisdom and power, seems never to have occurred.
In The Sleepwalkers: A History of Man's Changing Vision of the Universe (1959), Preface, 40.
Nobody grows old merely by living a number of years. We grow old by deserting our ideals. Years may wrinkle the skin, but to give up enthusiasm wrinkles the soul.
…...
Nobody knows more than a tiny fragment of science well enough to judge its validity and value at first hand. For the rest he has to rely on views accepted at second hand on the authority of a community of people accredited as scientists. But this accrediting depends in its turn on a complex organization. For each member of the community can judge at first hand only a small number of his fellow members, and yet eventually each is accredited by all. What happens is that each recognizes as scientists a number of others by whom he is recognized as such in return, and these relations form chains which transmit these mutual recognitions at second hand through the whole community. This is how each member becomes directly or indirectly accredited by all. The system extends into the past. Its members recognize the same set of persons as their masters and derive from this allegiance a common tradition, of which each carries on a particular strand.
Personal Knowledge (1958), 163.
Nonmathematical people sometimes ask me, “You know math, huh? Tell me something I’ve always wondered, What is infinity divided by infinity?” I can only reply, “The words you just uttered do not make sense. That was not a mathematical sentence. You spoke of ‘infinity’ as if it were a number. It’s not. You may as well ask, 'What is truth divided by beauty?’ I have no clue. I only know how to divide numbers. ‘Infinity,’ ‘truth,’ ‘beauty’—those are not numbers.”
From Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics (2003), 16.
Nor can it be supposed that the diversity of chemical structure and process stops at the boundary of the species, and that within that boundary, which has no real finality, rigid uniformity reigns. Such a conception is at variance with any evolutionary conception of the nature and origin of species. The existence of chemical individuality follows of necessity from that of chemical specificity, but we should expect the differences between individuals to be still more subtle and difficult of detection. Indications of their existence are seen, even in man, in the various tints of skin, hair, and eyes, and in the quantitative differences in those portions of the end-products of metabolism which are endogenous and are not affected by diet, such as recent researches have revealed in increasing numbers. Even those idiosyncrasies with regard to drugs and articles of food which are summed up in the proverbial saying that what is one man's meat is another man's poison presumably have a chemical basis.
Inborn Errors of Metabolism: The Croonian Lectures delivered before the Royal College of Physicians of London, in June, 1908 (1909), 2-3.
Not only did he teach by accomplishment, but he taught by the inspiration of a marvelous imagination that refused to accept the permanence of what appeared to others to be insuperable difficulties: an imagination of the goals of which, in a number of instances, are still in the realms of speculation.
Testimonial on Tesla’s 75th birthday, Tesla Museum, Belgrade, Serbia. In Margaret Cheney, Tesla: Man Out of Time (2001), 86.
Nothing in our experience suggests the introduction of [complex numbers]. Indeed, if a mathematician is asked to justify his interest in complex numbers, he will point, with some indignation, to the many beautiful theorems in the theory of equations, of power series, and of analytic functions in general, which owe their origin to the introduction of complex numbers. The mathematician is not willing to give up his interest in these most beautiful accomplishments of his genius.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences,' Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1 (February 1960). Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 537.
Now it is a well-known principle of zoological evolution that an isolated region, if large and sufficiently varied in its topography, soil, climate and vegetation, will give rise to a diversified fauna according to the law of adaptive radiation from primitive and central types. Branches will spring off in all directions to take advantage of every possible opportunity of securing food. The modifications which animals undergo in this adaptive radiation are largely of mechanical nature, they are limited in number and kind by hereditary, stirp or germinal influences, and thus result in the independent evolution of similar types in widely-separated regions under the law of parallelism or homoplasy. This law causes the independent origin not only of similar genera but of similar families and even of our similar orders. Nature thus repeats herself upon a vast scale, but the similarity is never complete and exact.
'The Geological and Faunal Relations of Europe and America during the Tertiary Period and the Theory of the Successive Invasions of an African Fauna', Science (1900), 11, 563-64.
Now when you cut a forest, an ancient forest in particular, you are not just removing a lot of big trees and a few birds fluttering around in the canopy. You are drastically imperiling a vast array of species within a few square miles of you. The number of these species may go to tens of thousands. … Many of them are still unknown to science, and science has not yet discovered the key role undoubtedly played in the maintenance of that ecosystem, as in the case of fungi, microorganisms, and many of the insects.
From On Human Nature (2000). As quoted in John H. Morgan, Naturally Good: A Behavioral History of Moral Development (2005), 251-252.
Now, there are a very large number of bodily movements, having their source in our nervous system, that do not possess the character of conscious actions.
Number is divided into even and odd. Even number is divided into the following: evenly even, evenly uneven, and unevenly uneven. Odd number is divided into the following: prime and incomposite, composite, and a third intermediate class (mediocris) which in a certain way is prime and incomposite but in another way secondary and composite.
Etymologies [c.600], Book III, chapter 5, quoted in E. Grant (ed.), A Source Book in Medieval Science (1974), trans. E. Brehaut (1912), revised by E. Grant, 5.
Number is the within of all things.
Attributed as a concept rather than actual words (none of the original writings of Pythagoras have survived). In L. A. Michael, The Principles of Existence & Beyond (2007), 16, but webmaster is unable to validate or find in quote dictionaries.
Number is therefore the most primitive instrument of bringing an unconscious awareness of order into consciousness.
In Creation Myths (1995), 326.
Number rules the universe
Motto of the Pythagoreans as given in George Edward Martin, The Foundations of Geometry and the Non-Euclidean Plane (1982), 19.
Number theorists are like lotus-eaters—having tasted this food they can never give it up.
As quoted in Howard Eves, Mathematical Circles Squared (1972), 149. In Homer’s The Odyssey, lotus-eaters live in a state of dreamy forgetfulness and idleness from eating lotus fruit. Thus a lotus-eater pursues pleasure and luxury rather than dealing with practical concerns.
Number, place, and combination … the three intersecting but distinct spheres of thought to which all mathematical ideas admit of being referred.
In Philosophical Magazine (1844), 84, 285; Collected Mathematical Papers, Vol. 1, 91.
Number, the most excellent of all inventions.
Spoken by the character Prometheus in play, 'Prometheus Bound', as translated by G.M. Cookson, in Four Plays of Aeschylus (1922), 182.
Numbers … were his friends. In the simplest array of digits [Ramanujan] detected wonderful properties: congruences, symmetries and relationships which had escaped the notice of even the outstandingly gifted theoreticians.
In James R. Newman (ed.), 'Commentary on Srinivasa Ramanujan', The World of Mathematics (1956), Vol. 1, 367.
Numbers are a fearful thing.
Spoken by the character Hecuba in the play, 'Hecuba', as translated by Edward P. Coleridge, in The Plays of Euripides (1907), Vol. 2, 157.
Numbers are intellectual witnesses that belong only to mankind.
From the French original, “Le nombre est un témoin intellectuel qui n’appartient qu’à l’homme”, in Oeuvres illustrées de Balzac (1852), Vol. 3, Louis Lambert, 28. As translated in Robert Collison (ed.), Dictionary of Foreign Quotations (1980), 223.
Numbers have neither substance, nor meaning, nor qualities. They are nothing but marks, and all that is in them we have put into them by the simple rule of straight succession.
In 'Mathematics and the Laws of Nature', The Armchair Science Reader (1959), 301.
Numbers written on restaurant checks [bills] within the confines of restaurants do not follow the same mathematical laws as numbers written on any other pieces of paper in any other parts of the Universe.
This single statement took the scientific world by storm. It completely revolutionized it. So many mathematical conferences got held in such good restaurants that many of the finest minds of a generation died of obesity and heart failure and the science of math was put back by years.
This single statement took the scientific world by storm. It completely revolutionized it. So many mathematical conferences got held in such good restaurants that many of the finest minds of a generation died of obesity and heart failure and the science of math was put back by years.
Life, the Universe and Everything (1982, 1995), 49.
Numerical logistic is that which employs numbers; symbolic logistic that which uses symbols, as, say, the letters of the alphabet.
In Introduction to the Analytic Art (1591).
O comfortable allurement, O ravishing perswasion, to deal with a Science, whose subject is so Auncient, so pure, so excellent, so surmounting all creatures... By Numbers propertie ... we may... arise, clime, ascend, and mount up (with Speculative winges) in spirit, to behold in the Glas of creation, the Forme of Formes, the Exemplar Number of all things Numerable... Who can remaine, therefore, unpersuaded, to love, allow, and honor the excellent sciehce of Arithmatike?
— John Dee
'Mathematicall Preface', in H. Billingsley, trans. The Elements of Geometry of the most Aunceint Philosopher Euclide of Megara (1570), in J. L. Hellbron, Weighing Imponderables and Other Quantitative Science around 1800 (1993), 2.
Observation is so wide awake, and facts are being so rapidly added to the sum of human experience, that it appears as if the theorizer would always be in arrears, and were doomed forever to arrive at imperfect conclusion; but the power to perceive a law is equally rare in all ages of the world, and depends but little on the number of facts observed.
In A Week on the Concord and Merrimack Rivers (1862), 383.
Occurrences that other men would have noted only with the most casual interest became for Whitney exciting opportunities to experiment. Once he became disturbed by a scientist's seemingly endless pursuit of irrelevant details in the course of an experiment, and criticized this as being as pointless as grabbing beans out of a pot, recording the numbers, and then analyzing the results. Later that day, after he had gone home, his simile began to intrigue him, and he asked himself whether it would really be pointless to count beans gathered in such a random manner. Another man might well have dismissed this as an idle fancy, but to Whitney an opportunity to conduct an experiment was not to be overlooked. Accordingly, he set a pot of beans beside his bed, and for several days each night before retiring he would take as many beans as he could grasp in one hand and make a note of how many were in the handful. After several days had passed he was intrigued to find that the results were not as unrewarding as he had expected. He found that each handful
contained more beans than the one before, indicating that with practice he was learning to grasp more and more beans. “This might be called research in morphology, the science of animal structure,” he mused. “My hand was becoming webbed … so I said to myself: never label a real experiment useless, it may reveal something unthought of but worth knowing.”
'Willis Rodney Whitney', National Academy of Sciences, Biographical Memoirs (1960), 358-359.
Of all many-sided subjects, [education] is the one which has the greatest number of sides.
Inaugural Address Delivered to the University of St. Andrews, Feb. 1st, 1867 (1867), 3.
Of what use are the great number of petrifactions, of different species, shape and form which are dug up by naturalists? Perhaps the collection of such specimens is sheer vanity and inquisitiveness. I do not presume to say; but we find in our mountains the rarest animals, shells, mussels, and corals embalmed in stone, as it were, living specimens of which are now being sought in vain throughout Europe. These stones alone whisper in the midst of general silence.
Philosophia Botanica (1751), aphorism 132. Trans. Frans A. Stafleu, Linnaeus and the Linnaeans: The Spreading of their Ideas in Systematic Botany, 1735-1789 (1971), 56.
On the basis of the results recorded in this review, it can be claimed that the average sand grain has taken many hundreds of millions of years to lose 10 per cent. of its weight by abrasion and become subangular. It is a platitude to point to the slowness of geological processes. But much depends on the way things are put. For it can also be said that a sand grain travelling on the bottom of a river loses 10 million molecules each time it rolls over on its side and that representation impresses us with the high rate of this loss. The properties of quartz have led to the concentration of its grains on the continents, where they could now form a layer averaging several hundred metres thick. But to my mind the most astounding numerical estimate that follows from the present evaluations, is that during each and every second of the incredibly long geological past the number of quartz grains on earth has increased by 1,000 million.
'Sand-its Origin, Transportation, Abrasion and Accumulation', The Geological Society of South Africa (1959), Annexure to Volume 62, 31.
On the most usual assumption, the universe is homogeneous on the large scale, i.e. down to regions containing each an appreciable number of nebulae. The homogeneity assumption may then be put in the form: An observer situated in a nebula and moving with the nebula will observe the same properties of the universe as any other similarly situated observer at any time.
From 'Review of Cosmology,', Monthly Notices of the Royal Astronomical Society (1948), 107-8; as quoted and cited in Hermann Friedmann, Wissenschaft und Symbol, Biederstein (1949), 472.
One could not be a successful scientist without realizing that, in contrast to the popular conception supported by newspapers and mothers of scientists, a goodly number of scientists are not only narrow-minded and dull, but also just stupid.
The Double Helix: A Personal Account of the Discovery of the Structure of DNA (1968, 1998), 14.
One is hard pressed to think of universal customs that man has successfully established on earth. There is one, however, of which he can boast the universal adoption of the Hindu-Arabic numerals to record numbers. In this we perhaps have man’s unique worldwide victory of an idea.
In Mathematical Circles Squared (1972), 13.
Our brains have evolved to get us out of the rain, find where the berries are, and keep us from getting killed. Our brains did not evolve to help us grasp really large numbers or to look a things in a hundred thousand dimensions.
As quoted by Paul Hoffman, 'The Man Who Loves Only Numbers', The Atlantic (Nov 1987), 260, No. 5, 74. Also quoted in Clifford Pickover, 'Preface', Wonders of Numbers: Adventures in Mathematics, Mind and Meaning (2000), x.
Our confused wish finds expression in the confused question as to the nature of force and electricity. But the answer which we want is not really an answer to this question. It is not by finding out more and fresh relations and connections that it can be answered; but by removing the contradictions existing between those already known, and thus perhaps by reducing their number. When these painful contradictions are removed, the question as to the nature of force will not have been answered; but our minds, no longer vexed, will cease to ask illegitimate questions.
Principles of Mechanics (1899), 7-8.
Our knowledge of the external world must always consist of numbers, and our picture of the universe—the synthesis of our knowledge—must necessarily be mathematical in form. All the concrete details of the picture, the apples, the pears and bananas, the ether and atoms and electrons, are mere clothing that we ourselves drape over our mathematical symbols— they do not belong to Nature, but to the parables by which we try to make Nature comprehensible. It was, I think, Kronecker who said that in arithmetic God made the integers and man made the rest; in the same spirit, we may add that in physics God made the mathematics and man made the rest.
From Address (1934) to the British Association for the Advancement of Science, Aberdeen, 'The New World—Picture of Modern Physics'. Printed in Nature (Sep 1934) 134, No. 3384, 356. As quoted and cited in Wilbur Marshall Urban, Language and Reality: The Philosophy of Language and the Principles of Symbolism (2004), Vol. 15, 542.
Perhaps five or even ten per cent of men can do something rather well. It is a tiny minority who can do anything really well, and the number of men who can do two things well is negligible. If a man has any genuine talent, he should be ready to make almost any sacrifice in order to cultivate it to the full.
In A Mathematician's Apology (1940, 2012), 53.
Perhaps the least inadequate description of the general scope of modern Pure Mathematics—I will not call it a definition—would be to say that it deals with form, in a very general sense of the term; this would include algebraic form, functional relationship, the relations of order in any ordered set of entities such as numbers, and the analysis of the peculiarities of form of groups of operations.
In Presidential Address British Association for the Advancement of Science, Sheffield, Section A,
Nature (1 Sep 1910), 84, 287.
Physical changes take place continuously, while chemical changes take place discontinuously. Physics deals chiefly with continuous varying quantities, while chemistry deals chiefly with whole numbers.
Treatise on Thermodynamics (1897), trans. Alexander Ogg (1903), 22, footnote.
Physics is NOT a body of indisputable and immutable Truth; it is a body of well-supported probable opinion only .... Physics can never prove things the way things are proved in mathematics, by eliminating ALL of the alternative possibilities. It is not possible to say what the alternative possibilities are.... Write down a number of 20 figures; if you multiply this by a number of, say, 30 figures, you would arrive at some enormous number (of either 49 or 50 figures). If you were to multiply the 30-figure number by the 20-figure number you would arrive at the same enormous 49- or 50-figure number, and you know this to be true without having to do the multiplying. This is the step you can never take in physics.
In Science is a Sacred Cow (1950), 68, 88, 179.
Population, when unchecked, increases in a geometrical ratio. Subsistence increases only in an arithmetical ratio. A slight acquaintance with numbers will show the immensity of the first power in comparison of the second.
An Essay on the Principle of Population (1798), 1st edition, 14. As cited in James Bonar, Parson Malthus (1881), 18.
Portable communication instruments will be developed that will enable an individual to communicate directly and promptly with anyone, anywhere in the world. As we learn more about the secrets of space, we shall increase immeasurably the number of usable frequencies until we are able to assign a separate frequency to an individual as a separate telephone number is assigned to each instrument.
In address (Fall 1946) at a dinner in New York to commemorate the 40 years of Sarnoff’s service in the radio field, 'Institute News and Radio Notes: The Past and Future of Radio', Proceedings of the Institute of Radio Engineers (I.R.E.), (May 1947), 35, No. 5, 498. [In 1946, foretelling the cell phone and a hint of the communications satellite? —Webmaster]
Prayers for the condemned man will be offered on an adding machine. Numbers … constitute the only universal language.
In Miss Lonelyhearts (1933), 15.
Probably our atomic weights merely represent a mean value around which the actual atomic weights of the atoms vary within certain narrow limits... when we say, the atomic weight of, for instance, calcium is 40, we really express the fact that, while the majority of calcium atoms have an actual atomic weight of 40, there are not but a few which are represented by 39 or 41, a less number by 38 or 42, and so on.
Presidential Address, 2 September 1886, Section B, Chemical Science. Reports of the British Association for the Advancement of Science (1886), 569.
Professor Bethe … is a man who has this characteristic: If there’s a good experimental number you’ve got to figure it out from theory. So, he forced the quantum electrodynamics of the day to give him an answer [for the experimentally measured Lamb-shift of hydrogen], … and thus, made
the most important discovery in the history of the theory of quantum electrodynamics. He worked this out on the train from Ithaca, New York to Schenectady.
Bethe calculated, what Lamb had experimentally just measured, for the separation of the 2S½ and 2P½ of hydrogen. Both theory and measurement yielded about one thousand megacycles for the Lamb-shift. Feynman was at the time associated with Bethe at Cornell. In Feynman’s Nobel Prize Lecture (11 Dec 1965), 'The Development of the Space-Time View of Quantum Electrodynamics'. Collected in Stig Lundqvist, Nobel Lectures: Physics, 1963-1970 (1998), 170.
Progress is achieved by exchanging our theories for new ones which go further than the old, until we find one based on a larger number of facts. … Theories are only hypotheses, verified by more or less numerous facts. Those verified by the most facts are the best, but even then they are never final, never to be absolutely believed.
From An Introduction to the Study of Experimental Medicine (1865), as translated by Henry Copley Greene (1957), 165.
Quantum mechanics and relativity, taken together, are extraordinarily restrictive, and they therefore provide us with a great logical machine. We can explore with our minds any number of possible universes consisting of all kinds of mythical particles and interactions, but all except a very few can be rejected on a priori grounds because they are not simultaneously consistent with special relativity and quantum mechanics. Hopefully in the end we will find that only one theory is consistent with both and that theory will determine the nature of our particular universe.
As quoted in John D. Barrow, The Universe that Discovered Itself (2000), 360.
Quite distinct from the theoretical question of the manner in which mathematics will rescue itself from the perils to which it is exposed by its own prolific nature is the practical problem of finding means of rendering available for the student the results which have been already accumulated, and making it possible for the learner to obtain some idea of the present state of the various departments of mathematics. … The great mass of mathematical literature will be always contained in Journals and Transactions, but there is no reason why it should not be rendered far more useful and accessible than at present by means of treatises or higher text-books. The whole science suffers from want of avenues of approach, and many beautiful branches of mathematics are regarded as difficult and technical merely because they are not easily accessible. … I feel very strongly that any introduction to a new subject written by a competent person confers a real benefit on the whole science. The number of excellent text-books of an elementary kind that are published in this country makes it all the more to be regretted that we have so few that are intended for the advanced student. As an example of the higher kind of text-book, the want of which is so badly felt in many subjects, I may mention the second part of Prof. Chrystal’s Algebra published last year, which in a small compass gives a great mass of valuable and fundamental knowledge that has hitherto been beyond the reach of an ordinary student, though in reality lying so close at hand. I may add that in any treatise or higher text-book it is always desirable that references to the original memoirs should be given, and, if possible, short historic notices also. I am sure that no subject loses more than mathematics by any attempt to dissociate it from its history.
In Presidential Address British Association for the Advancement of Science, Section A (1890), Nature, 42, 466.
Research is industrial prospecting. The oil prospectors use every scientific means to find new paying wells. Oil is found by each one of a number of methods. My own group of men are prospecting in a different field, using every possible scientific means. We believe there are still things left to be discovered. We have only stumbled upon a few barrels of physical laws from the great pool of knowledge. Some day we are going to hit a gusher.
'Industrial Prospecting', an address to the Founder Societies of Engineers (20 May 1935). In National Research Council, Reprint and Circular Series of the National Research Council (1933), No. 107, 1.
Samuel Pierpoint Langley, at that time regarded as one of the most distinguished scientists in the United States … evidently believed that a full sized airplane could be built and flown largely from theory alone. This resulted in two successive disastrous plunges into the Potomac River, the second of which almost drowned his pilot. This experience contrasts with that of two bicycle mechanics Orville and Wilbur Wright who designed, built and flew the first successful airplane. But they did this after hundreds of experiments extending over a number of years.
In article Total Quality: Its Origins and its Future (1995), published at the Center for Quality and Productivity Improvement.
Saturated with that speculative spirit then pervading the Greek mind, he [Pythagoras] endeavoured to discover some principle of homogeneity in the universe. Before him, the philosophers of the Ionic school had sought it in the matter of things; Pythagoras looked for it in the structure of things. He observed the various numerical relations or analogies between numbers and the phenomena of the universe. Being convinced that it was in numbers and their relations that he was to find the foundation to true philosophy, he proceeded to trace the origin of all things to numbers. Thus he observed that musical strings of equal lengths stretched by weights having the proportion of 1/2, 2/3, 3/4, produced intervals which were an octave, a fifth and a fourth. Harmony, therefore, depends on musical proportion; it is nothing but a mysterious numerical relation. Where harmony is, there are numbers. Hence the order and beauty of the universe have their origin in numbers. There are seven intervals in the musical scale, and also seven planets crossing the heavens. The same numerical relations which underlie the former must underlie the latter. But where number is, there is harmony. Hence his spiritual ear discerned in the planetary motions a wonderful “Harmony of spheres.”
In History of Mathematics (1893), 67.
Science … is perpetually advancing. It is like a torch in the sombre forest of mystery. Man enlarges every day the circle of light which spreads round him, but at the same time, and in virtue of his very advance, he finds himself confronting, at an increasing number of points, the darkness of the Unknown.
In Einstein and the Universe; A Popular Exposition of the Famous Theory (1922), xvi.
Science gains from it [the pendulum] more than one can expect. With its huge dimensions, the apparatus presents qualities that one would try in vain to communicate by constructing it on a small [scale], no matter how carefully. Already the regularity of its motion promises the most conclusive results. One collects numbers that, compared with the predictions of theory, permit one to appreciate how far the true pendulum approximates or differs from the abstract system called 'the simple pendulum'.
In 'Demonstration Experimentale du Movement de Rotation de la Terre' (31 May 1851). In C.M. Gariel (ed.), J. Bertrand (ed.) and Harold Burstyn (trans.), Recueil des Travaux Scientifiques de Lion Foucault (1878), Vol. 2, 527.
Science has been arranging, classifying, methodizing, simplifying, everything except itself. It has made possible the tremendous modern development of power of organization which has so multiplied the effective power of human effort as to make the differences from the past seem to be of kind rather than of degree. It has organized itself very imperfectly. Scientific men are only recently realizing that the principles which apply to success on a large scale in transportation and manufacture and general staff work to apply them; that the difference between a mob and an army does not depend upon occupation or purpose but upon human nature; that the effective power of a great number of scientific men may be increased by organization just as the effective power of a great number of laborers may be increased by military discipline.
'The Need for Organization in Scientific Research', in Bulletin of the National Research Council: The National Importance of Scientific and Industrial Research (Oct 1919), Col 1, Part 1, No. 1, 8.
Science is not a sacred cow—but there are a large number of would-be sacred cowherds busily devoting quantities of time, energy and effort to the task of making it one, so they can be sacred cowherds.
From 'Introduction', to Prologue to Analog (1962).
Science is concerned with what is possible while engineering is concerned with choosing, from among the many possible ways, one that meets a number of often poorly stated economic and practical objectives.
From Turing Award lecture (1968), 'One Man's View of Computer Science', collected in ACM Turing Award Lectures: The First Twenty Years, 1966 to 1985 (1987), 209. ACM is the Association for Computing Machinery. Also in Journal of the ACM (Jan 1969), 16, No. 1, 5.
Science is the reduction of the bewildering diversity of unique events to manageable uniformity within one of a number of symbol systems, and technology is the art of using these symbol systems so as to control and organize unique events. Scientific observation is always a viewing of things through the refracting medium of a symbol system, and technological praxis is always handling of things in ways that some symbol system has dictated. Education in science and technology is essentially education on the symbol level.
Essay in Daedalus (Spring1962), 279.
Science itself is badly in need of integration and unification. The tendency is more and more the other way ... Only the graduate student, poor beast of burden that he is, can be expected to know a little of each. As the number of physicists increases, each specialty becomes more self-sustaining and self-contained. Such Balkanization carries physics, and indeed, every science further away, from natural philosophy, which, intellectually, is the meaning and goal of science.
Science, The Center of Culture (1970), 92. Quoted by Victor F. Weisskopf, 'One Hundred Years of the Physical Review', in H. Henry Stroke, Physical Review: The First Hundred Years: a Selection of Seminal Papers and Commentaries, Vol. 1, 15.
Science, being human enquiry, can hear no answer except an answer couched somehow in human tones. Primitive man stood in the mountains and shouted against a cliff; the echo brought back his own voice, and he believed in a disembodied spirit. The scientist of today stands counting out loud in the face of the unknown. Numbers come back to him—and he believes in the Great Mathematician.
Concluding paragraph of chapter, 'Physics, Astronomy, and Mathematics: Or Beyond Common-Sense', contributed to Naomi Mitchison (ed.), An Outline For Boys And Girls And Their Parents (1932), 357.
Scientific studies on marine reserves around the world show that if you close a place to fishing, the number of species increases 20 percent, the average size of a fish increases by a third, and the total weight of fish per hectare increases almost five times—in less than a decade.
From interview with Terry Waghorn, 'Can We Eat Our Fish and Protect Them Too?', Forbes (21 Feb 2012)
Scientists are convinced that they, as scientists, possess a number of very admirable human qualities, such as accuracy, observation, reasoning power, intellectual curiosity, tolerance, and even humility.
In Science is a Sacred Cow (1950), 15-16.
Seeing there is nothing that is so troublesome to mathematical practice … than the multiplications, divisions, square and cubical extractions of great numbers, which besides the tedious expense of time are … subject to many slippery errors, I began therefore to consider [how] I might remove those hindrances.
From Mirifici Logarithmorum Canonis Descriptio (1614), as translated by William Rae Macdonald in A Description of the Wonderful Canon of Logarithms (1889),
Since the examination of consistency is a task that cannot be avoided, it appears necessary to axiomatize logic itself and to prove that number theory and set theory are only parts of logic. This method was prepared long ago (not least by Frege’s profound investigations); it has been most successfully explained by the acute mathematician and logician Russell. One could regard the completion of this magnificent Russellian enterprise of the axiomatization of logic as the crowning achievement of the work of axiomatization as a whole.
Address (11 Sep 1917), 'Axiomatisches Denken' delivered before the Swiss Mathematical Society in
Zürich. Translated by Ewald as 'Axiomatic Thought', (1918), in William Bragg Ewald, From Kant to Hilbert (1996), Vol. 2, 1113.
So highly did the ancients esteem the power of figures and numbers, that Democritus ascribed to the figures of atoms the first principles of the variety of things; and Pythagoras asserted that the nature of things consisted of numbers.
In De Augmentis, Bk. 3; Advancement of Learning, Bk. 2.
Sociology is the science with the greatest number of methods and the least results.
…...
Some people say they cannot understand a million million. Those people cannot understand that twice two makes four. That is the way I put it to people who talk to me about the incomprehensibility of such large numbers. I say finitude is incomprehensible, the infinite in the universe is comprehensible. Now apply a little logic to this. Is the negation of infinitude incomprehensible? What would you think of a universe in which you could travel one, ten, or a thousand miles, or even to California, and then find it comes to an end? Can you suppose an end of matter or an end of space? The idea is incomprehensible. Even if you were to go millions and millions of miles the idea of coming to an end is incomprehensible. You can understand one thousand per second as easily as you can understand one per second. You can go from one to ten, and then times ten and then to a thousand without taxing your understanding, and then you can go on to a thousand million and a million million. You can all understand it.
In 'The Wave Theory of Light' (1884), Popular Lectures and Addresses (1891), Vol. 1, 322.
Sometimes progress is slow. But then there does come a time when a lot of people accept a new idea and see ways in which it can be exploited. And because of the larger number of workers in the field, progress becomes rapid. That is what happened with the study of protein structure.
From interview with Neil A. Campbell, in 'Crossing the Boundaries of Science', BioScience (Dec 1986), 36, No. 11, 739.
Somewhere in the arrangement of this world there seems to be a great concern about giving us delight, which shows that, in the universe, over and above the meaning of matter and forces, there is a message conveyed through the magic touch of personality. ...
Is it merely because the rose is round and pink that it gives me more satisfaction than the gold which could buy me the necessities of life, or any number of slaves. ... Somehow we feel that through a rose the language of love reached our hearts.
Is it merely because the rose is round and pink that it gives me more satisfaction than the gold which could buy me the necessities of life, or any number of slaves. ... Somehow we feel that through a rose the language of love reached our hearts.
The Religion of Man (1931), 102. Quoted in H. E. Hunter, The Divine Proportion (1970), 6.
Standard mathematics has recently been rendered obsolete by the discovery that for years we have been writing the numeral five backward. This has led to reevaluation of counting as a method of getting from one to ten. Students are taught advanced concepts of Boolean algebra, and formerly unsolvable equations are dealt with by threats of reprisals.
Getting Even (1978), 44.
Statistical analysis in cases involving small numbers can be particularly helpful because on many occasions intuition can be highly misleading.
In essay 'Statistical Proof of Employment Discrimination', collected in Judith M. Tanur et al. (eds.), Statistics, a Guide to the Unknown (3rd ed., 1989), 83.
Statistician: A man who believes figures don’t lie but admits that, under analysis some of them won’t stand up either.
In Esar’s Comic Dictionary (1943, 4th ed. 1983), 568.
Statistician: One who knows which numbers to use in any eventuality.
Statisticians tell us that for many years the death-rate from cancer has been slowly but steadily rising: and not unnaturally, many people conclude from this that for some reason or other we are becoming more susceptible to cancer. Actually, that conclusion does not follow at all. The rise in the cancer death-rate is probably due entirely to the fact that other causes of death have been reduced. Numbers of people who, if they had been born a century earlier, would have died in their twenties of typhoid or smallpox, say, are now living on into their seventies and dying of cancer.
From 'Figures Can Lie', Science Digest (Sep 1951), 30, No. 3, 53. (As condensed from The Listener). Excerpted in Meta Riley Emberger and Marian Ross Hall, Scientific Writing (1955), 407.
Statistics: The only science that enables different experts using the same figures to draw different conclusions.
In Esar’s Comic Dictionary (1943, 4th ed. 1983), 569.
Strictly speaking, it is really scandalous that science has not yet clarified the nature of number. It might be excusable that there is still no generally accepted definition of number, if at least there were general agreement on the matter itself. However, science has not even decided on whether number is an assemblage of things, or a figure drawn on the blackboard by the hand of man; whether it is something psychical, about whose generation psychology must give information, or whether it is a logical structure; whether it is created and can vanish, or whether it is eternal. It is not known whether the propositions of arithmetic deal with those structures composed of calcium carbonate [chalk] or with non-physical entities. There is as little agreement in this matter as there is regarding the meaning of the word “equal” and the equality sign. Therefore, science does not know the thought content which is attached to its propositions; it does not know what it deals with; it is completely in the dark regarding their proper nature. Isn’t this scandalous?
From opening paragraph of 'Vorwort', Über die Zahlen des Herrn H. Schubert (1899), iii. ('Foreword', On the Numbers of Mr. H. Schubert). Translated by Theodore J. Benac in Friedrich Waismann, Introduction to Mathematical Thinking: The Formation of Concepts in Modern Mathematics (1959, 2003), 107. Webmaster added “[chalk]”.
Suicide is merely the product of the general condition of society, and that the individual felon only carries into effect what is a necessary consequence of preceding circumstances. In a given state of society, a certain number of persons must put an end to their own life. This is the general law; and the special question as to who shall commit the crime depends of course upon special laws; which, however, in their total action, must obey the large social law to which they are all subordinate. And the power of the larger law is so irresistible, that neither the love of life nor the fear of another world can avail any thing towards even checking its operation.
In History of Civilization in England (1857, 1904), 15-16.
Suppose a number of equal waves of water to move upon the surface of a stagnant lake, with a certain constant velocity, and to enter a narrow channel leading out of the lake. Suppose then another similar cause to have excited another equal series of waves, which arrive at the same time, with the first. Neither series of waves will destroy the other, but their effects will be combined: if they enter the channel in such a manner that the elevations of one series coincide with those of the other, they must together produce a series of greater joint elevations; but if the elevations of one series are so situated as to correspond to the depressions of the other, they must exactly fill up those depressions. And the surface of the water must remain smooth; at least I can discover no alternative, either from theory or from experiment.
A Reply to the Animadversions of the Edinburgh Reviewers on Some Papers Published in the Philosophical Transactions (1804), 17-8.
Suppose then I want to give myself a little training in the art of reasoning; suppose I want to get out of the region of conjecture and probability, free myself from the difficult task of weighing evidence, and putting instances together to arrive at general propositions, and simply desire to know how to deal with my general propositions when I get them, and how to deduce right inferences from them; it is clear that I shall obtain this sort of discipline best in those departments of thought in which the first principles are unquestionably true. For in all our thinking, if we come to erroneous conclusions, we come to them either by accepting false premises to start with—in which case our reasoning, however good, will not save us from error; or by reasoning badly, in which case the data we start from may be perfectly sound, and yet our conclusions may be false. But in the mathematical or pure sciences,—geometry, arithmetic, algebra, trigonometry, the calculus of variations or of curves,— we know at least that there is not, and cannot be, error in our first principles, and we may therefore fasten our whole attention upon the processes. As mere exercises in logic, therefore, these sciences, based as they all are on primary truths relating to space and number, have always been supposed to furnish the most exact discipline. When Plato wrote over the portal of his school. “Let no one ignorant of geometry enter here,” he did not mean that questions relating to lines and surfaces would be discussed by his disciples. On the contrary, the topics to which he directed their attention were some of the deepest problems,— social, political, moral,—on which the mind could exercise itself. Plato and his followers tried to think out together conclusions respecting the being, the duty, and the destiny of man, and the relation in which he stood to the gods and to the unseen world. What had geometry to do with these things? Simply this: That a man whose mind has not undergone a rigorous training in systematic thinking, and in the art of drawing legitimate inferences from premises, was unfitted to enter on the discussion of these high topics; and that the sort of logical discipline which he needed was most likely to be obtained from geometry—the only mathematical science which in Plato’s time had been formulated and reduced to a system. And we in this country [England] have long acted on the same principle. Our future lawyers, clergy, and statesmen are expected at the University to learn a good deal about curves, and angles, and numbers and proportions; not because these subjects have the smallest relation to the needs of their lives, but because in the very act of learning them they are likely to acquire that habit of steadfast and accurate thinking, which is indispensable to success in all the pursuits of life.
In Lectures on Teaching (1906), 891-92.
Suppose we divide the space into little volume elements. If we have black and white molecules, how many ways could we distribute them among the volume elements so that white is on one side and black is on the other? On the other hand, how many ways could we distribute them with no restriction on which goes where? Clearly, there are many more ways to arrange them in the latter case. We measure “disorder” by the number of ways that the insides can be arranged, so that from the outside it looks the same. The logarithm of that number of ways is the entropy. The number of ways in the separated case is less, so the entropy is less, or the “disorder” is less.
In 'Order And Entropy', The Feynman Lectures on Physics (1964), 46-7.
Tactics used by many practitioners of pseudoscience: make a large number of vaguely scientific arguments in the hope of making the desired conclusion seem inevitable. It is essential to recognize that a disconnected assemblage of weak arguments does not create a single, strong scientific argument.
Co-author with Matt Ford, Chris Lee and Jonathan Gitlin, in 'Diluting the Scientific Method: Ars Looks at Homeopathy' (11 Sep 2007) on arstechnica.com web site.
That many very remarkable change and involuntary motions are sudden produced in the body by various affections of the mind, is undeniably evinced from a number of facts. Thus fear often causes a sudden and uncommon flow of pale urine. Looking much at one troubled with sore eyes, has sometimes affected the spectator with the same disease.—Certain sounds cause a shivering over the whole body.—The noise of a bagpipe has raised in some persons an inclination to make urine.—The sudden appearance of any frightful object, will, in delicate people, cause an uncommon palpitation of the heart.—The sight of an epileptic person agitated with convulsions, has brought on an epilepsy; and yawning is so very catching, as frequently to be propagated through whole companies.
In An Essay on the Vital and Other Involuntary Motions of Animals (1751), 253-254.
That this subject [of imaginary magnitudes] has hitherto been considered from the wrong point of view and surrounded by a mysterious obscurity, is to be attributed largely to an ill-adapted notation. If, for example, +1, -1, and the square root of -1 had been called direct, inverse and lateral units, instead of positive, negative and imaginary (or even impossible), such an obscurity would have been out of the question.
Theoria Residiorum Biquadraticorum, Commentario secunda', Werke (1863), Vol. 2. Quoted in Robert Edouard Moritz, Memorabilia Mathematica (1914), 282.
The ‘mad idea’ which will lie at the basis of a future fundamental physical theory will come from a realization that physical meaning has some mathematical form not previously associated with reality. From this point of view the problem of the ‘mad idea’ is the problem of choosing, not of generating, the right idea. One should not understand that too literally. In the 1960s it was said (in a certain connection) that the most important discovery of recent years in physics was the complex numbers. The author [Yuri Manin] has something like that in mind.
Mathematics and Physics (1981), Foreward. Reprinted in Mathematics as Metaphor: Selected Essays of Yuri I. Manin (2007), 90.
The arithmetization of mathematics … which began with Weierstrass … had for its object the separation of purely mathematical concepts, such as number and correspondence and aggregate, from intuitional ideas, which mathematics had acquired from long association with geometry and mechanics. These latter, in the opinion of the formalists, are so firmly entrenched in mathematical thought that in spite of the most careful circumspection in the choice of words, the meaning concealed behind these words, may influence our reasoning. For the trouble with human words is that they possess content, whereas the purpose of mathematics is to construct pure thought. But how can we avoid the use of human language? The … symbol. Only by using a symbolic language not yet usurped by those vague ideas of space, time, continuity which have their origin in intuition and tend to obscure pure reason—only thus may we hope to build mathematics on the solid foundation of logic.
In Tobias Dantzig and Joseph Mazur (ed.), Number: The Language of Science (1930, ed. by Joseph Mazur 2007), 99.
The Qualities then that are in Bodies rightly considered, are of Three sorts.
First, the Bulk, Figure, Number, Situation, and Motion, or Rest of their solid Parts; those are in them, whether we perceive them or no; and when they are of that size, that we can discover them, we have by these an Idea of the thing, as it is in it self, as is plain in artificial things. These I call primary Qualities.
Secondly, The Power that is in any Body, by Reason of its insensible primary Qualities, to operate after a peculiar manner on any of our Senses, and thereby produce in us the different Ideas of several Colours, Sounds, Smells, Tastes, etc. These are usually called sensible Qualities.
Thirdly, The Power that is in any Body, by Reason of the particular Constitution of its primary Qualities, to make such a change in the Bulk, Figure, Texture, and Motion of another Body, as to make it operate on our Senses, differently from what it did before. Thus the Sun has a Power to make Wax white, and Fire to make Lead fluid. These are usually called Powers.
First, the Bulk, Figure, Number, Situation, and Motion, or Rest of their solid Parts; those are in them, whether we perceive them or no; and when they are of that size, that we can discover them, we have by these an Idea of the thing, as it is in it self, as is plain in artificial things. These I call primary Qualities.
Secondly, The Power that is in any Body, by Reason of its insensible primary Qualities, to operate after a peculiar manner on any of our Senses, and thereby produce in us the different Ideas of several Colours, Sounds, Smells, Tastes, etc. These are usually called sensible Qualities.
Thirdly, The Power that is in any Body, by Reason of the particular Constitution of its primary Qualities, to make such a change in the Bulk, Figure, Texture, and Motion of another Body, as to make it operate on our Senses, differently from what it did before. Thus the Sun has a Power to make Wax white, and Fire to make Lead fluid. These are usually called Powers.
An Essay Concerning Human Understanding (1690). Edited by Peter Nidditch (1975), Book 2, Chapter 8, Section 23, 140-1.
The ancients devoted a lifetime to the study of arithmetic; it required days to extract a square root or to multiply two numbers together. Is there any harm in skipping all that, in letting the school boy learn multiplication sums, and in starting his more abstract reasoning at a more advanced point? Where would be the harm in letting the boy assume the truth of many propositions of the first four books of Euclid, letting him assume their truth partly by faith, partly by trial? Giving him the whole fifth book of Euclid by simple algebra? Letting him assume the sixth as axiomatic? Letting him, in fact, begin his severer studies where he is now in the habit of leaving off? We do much less orthodox things. Every here and there in one’s mathematical studies one makes exceedingly large assumptions, because the methodical study would be ridiculous even in the eyes of the most pedantic of teachers. I can imagine a whole year devoted to the philosophical study of many things that a student now takes in his stride without trouble. The present method of training the mind of a mathematical teacher causes it to strain at gnats and to swallow camels. Such gnats are most of the propositions of the sixth book of Euclid; propositions generally about incommensurables; the use of arithmetic in geometry; the parallelogram of forces, etc., decimals.
In Teaching of Mathematics (1904), 12.
The ancients had a taste, let us say rather a passion, for the marvellous, which caused … grouping together the lofty deeds of a great number of heroes, whose names they have not even deigned to preserve, and investing the single personage of Hercules with them. … In our own time the public delight in blending fable with history. In every career of life, in the pursuit of science especially, they enjoy a pleasure in creating Herculeses.
In François Arago, trans. by William Henry Smyth, Baden Powell and Robert Grant, 'Fourier', Biographies of Distinguished Scientific Men (1859), Vol. 1, 408. This comment indicates that a single scientist or inventor may be held as the exemplar, such as James Watt and the steam-engine, although groundwork was laid by predecessors.
The ancients knew a few … numerical laws…. But prior to the Scientific Revolution, the goal of science (or the study of nature) was not to seek laws of nature expressed in terms of numbers or number relations. Those who created the new science of the seventeenth century not only found laws based on numbers but they were also willing to express these laws in terms of higher powers of numbers—squares and cubes.
From The Triumph of Numbers: How Counting Shaped Modern Life (2005), 37. The second ellipsis is for examples of those laws: “the law of reflection of light, the law of the lever, and the law of buoyancy.”
The answer to the Great Question of … Life, the Universe and Everything … is Forty-two
The Hitch Hiker’s Guide to the Galaxy (1979), Chapter 27.
The astronomer may speak to you of his understanding of space, but he cannot give you his understanding. … And he who is versed in the science of numbers can tell of the regions of weight and measure, but he cannot conduct you thither.
In Kahlil Gibran: The Collected Works (2007), 134.
The automatic computing engine now being designed at N.P.L. [National Physics Laboratory] is atypical large scale electronic digital computing machine. In a single lecture it will not be possible to give much technical detail of this machine, and most of what I shall say will apply equally to any other machine of this type now being planned. From the point of view of the mathematician the property of being digital should be of greater interest than that of being electronic. That it is electronic is certainly important because these machines owe their high speed to this, and without the speed it is doubtful if financial support for their construction would be forthcoming. But this is virtually all that there is to be said on that subject. That the machine is digital however has more subtle significance. It means firstly that numbers are represented by sequences of digits which can be as long as one wishes. One can therefore work to any desired degree of accuracy. This accuracy is not obtained by more careful machining of parts, control of temperature variations, and such means, but by a slight increase in the amount of equipment in the machine.
Lecture to the London Mathematical Society, 20 February 1947. Quoted in B. E. Carpenter and R. W. Doran (eds.), A. M. Turing's Ace Report of 1946 and Other Papers (1986), 106.
The believer has the whole world of wealth (Prov. 17: 6 LXX) and “possesses all things as if he had nothing” (2 Cor. 6: 10) by virtue of his attachment to you whom all things serve; yet he may know nothing about the circuits of the Great Bear. It is stupid to doubt that he is better than the person who measures the heaven and counts the stars and weighs the elements, but neglects you who have disposed everything “by measure and number and weight” (Wisd. 11: 21).
Confessions [c.397], Book V, chapter 4 (7), trans. H. Chadwick (1991), 76.
The best way to increase the intelligence of scientists would be to decrease their number.
In Man the Unknown (1935), 49.
The best way to look out for Number One is to care for Numbers Two, Three and Four first.
Quoted in 'Obituaries: Archibald Malloch, M.D., 1887-1953', Bulletin of the Medical Library Association (Jan 1954), 42(1), 153.
The bushels of rings taken from the fingers of the slain at the battle of Cannæ, above two thousand years ago, are recorded; … but the bushels of corn produced in England at this day, or the number of the inhabitants of the country, are unknown, at the very time that we are debating that most important question, whether or not there is sufficient substance for those who live in the kingdom.
In The Statistical Breviary: Shewing, on a Principle Entirely New, the Resources of Every State and Kingdom in Europe (1801), 7-8.
The collective IQ of the group is the lowest IQ of any member of the group, divided by the numbers of members in the group.
In Lily Splane, Quantum Consciousness (2004), 309
The competition of the sellers would cause a momentary fall in the price of the lands, and would enable small farmers to become land-owners. The number of vast estates often badly managed would then be diminished, and considerable fortunes, changing hands more easily, would naturally pass into those which would be most likely to increase their value.
From Appendix A, 'Extracts From the Unpublished Writings of Carnot', Reflections on the Motive Power of Heat (1890, 2nd ed. 1897), 213.
The complexity of contemporary biology has led to an extreme specialization, which has inevitably been followed by a breakdown in communication between disciplines. Partly as a result of this, the members of each specialty tend to feel that their own work is fundamental and that the work of other groups, although sometimes technically ingenious, is trivial or at best only peripheral to an understanding of truly basic problems and issues. There is a familiar resolution to this problem but it is sometimes difficulty to accept emotionally. This is the idea that there are a number of levels of biological integration and that each level offers problems and insights that are unique to it; further, that each level finds its explanations of mechanism in the levels below, and its significances in the levels above it.
From 'Interaction of physiology and behavior under natural conditions', collected in R.I. Bowman (ed.), The Galapagos (1966), 39.
The complexity of the world is the outcome of huge numbers of sometimes conflicting simple events.
In 'Religion - The Antithesis to Science', Chemistry & Industry (Feb 1997).
The concept of number is the obvious distinction between the beast and man. Thanks to number, the cry becomes a song, noise acquires rhythm, the spring is transformed into a dance, force becomes dynamic, and outlines figures.
Epigraph, without citation, in Corrective and Social Psychiatry and Journal of Behavior Technology Methods and Therapy (1966), Vol. 12, 409.
The diversity of life is extraordinary. There is said to be a million or so different kinds of living animals, and hundreds of thousands of kinds of plants. But we don’t need to think of the world at large. It is amazing enough to stop and look at a forest or at a meadow—at the grass and trees and caterpillars and hawks and deer. How did all these different kinds of things come about; what forces governed their evolution; what forces maintain their numbers and determine their survival or extinction; what are their relations to each other and to the physical environment in which they live? These are the problems of natural history.
In The Nature of Natural History (1950), 8.
The education explosion is producing a vast number of people who want to live significant, important lives but lack the ability to satisfy this craving for importance by individual achievement. The country is being swamped with nobodies who want to be somebodies.
From address to employees of the Phillips Petroleum Co. In Bartlesville, Oklahoma, excerpted in the Franklin, Indiana, The Daily Journal (23 Jan 1978), 2.
The elegance of a mathematical theorem is directly proportional to the number of independent ideas one can see in the theorem and inversely proportional to the effort it takes to see them.
In Mathematical Discovery: On Understanding, Learning, and Teaching Problem Solving (1981). As cited, with no more details, in Yi Ma, An Invitation to 3-D Vision (2004), 228.
The ends of scientific classification are best answered, when the objects are formed into groups respecting which a greater number of general propositions can be made, and those propositions more important, than could be made respecting any other groups into which the same things could be distributed. ... A classification thus formed is properly scientific or philosophical, and is commonly called a Natural, in contradistinction to a Technical or Artificial, classification or arrangement.
A System of Logic, Ratiocinative and Inductive (1843), Vol. 2, Book 4, Chapter 7, 302-3.
The equation eπi = -1 has been called the eutectic point of mathematics, for no matter how you boil down and explain this equation, which relates four of the most remarkable numbers of mathematics, it still has a certain mystery about it that cannot be explained away.
The essential character of a species in biology is, that it is a group of living organisms, separated from all other such groups by a set of distinctive characters, having relations to the environment not identical with those of any other group of organisms, and having the power of continuously reproducing its like. Genera are merely assemblages of a number of these species which have a closer resemblance to each other in certain important and often prominent characters than they have to any other species.
In 'The Method of Organic Evolution', Fortnightly Review (1895), 57, 441.
The evidence at present available points strongly to the conclusion that the spirals are individual galaxies, or island universes, comparable with our own galaxy in dimension and in number of component units.
[Stating his conviction on the nature of nebulae during the Shapley-Curtis debate on 26 Apr 1920 to the National Academy of Sciences.]
[Stating his conviction on the nature of nebulae during the Shapley-Curtis debate on 26 Apr 1920 to the National Academy of Sciences.]
In Aleksandr Sergeevich Sharov and Igor Dmitrievich Novikov, Edwin Hubble: The Discoverer of the Big Bang Universe (1993), 27.
The examples which a beginner should choose for practice should be simple and should not contain very large numbers. The powers of the mind cannot be directed to two things at once; if the complexity of the numbers used requires all the student’s attention, he cannot observe the principle of the rule which he is following.
In Study and Difficulties of Mathematics (1902), chap. 3.
The explosive component in the contemporary scene is not the clamor of the masses but the self-righteous claims of a multitude of graduates from schools and universities. This army of scribes is clamoring for a society in which planning, regulation, and supervision are paramount and the prerogative of the educated. They hanker for the scribe’s golden age, for a return to something like the scribe-dominated societies of ancient Egypt, China, and Europe of the Middle Ages. There is little doubt that the present trend in the new and renovated countries toward social regimentation stems partly from the need to create adequate employment for a large number of scribes. And since the tempo of the production of the literate is continually increasing, the prospect is of ever-swelling bureaucracies.
In 'Scribe, Writer, and Rebel', The Ordeal of Change (1963), 109.
The faculty for remembering is not diminished in proportion to what one has learnt, just as little as the number of moulds in which you cast sand lessens its capacity for being cast in new moulds.
Religion: a Dialogue, and Other Essays (1890), 99.
The farther researches we make into this admirable scene of things, the more beauty and harmony we see in them: And the stronger and clearer convictions they give us, of the being, power and wisdom of the divine Architect, who has made all things to concur with a wonderful conformity, in carrying on, by various and innumerable combinations of matter, such a circulation of causes, and effects, as was necessary to the great ends of nature. And since we are assured that the all-wise Creator has observed the most exact proportions, of number, weight and measure, in the make of all things; the most likely way therefore, to get any insight into the nature of those parts of the creation, which come within our observation, must in all reason be to number, weigh and measure. And we have much encouragement to pursue this method, of searching into the nature of things, from the great success that has attended any attempts of this kind.
Vegetable Staticks (1727), xxxi.
The feeling of understanding is as private as the feeling of pain. The act of understanding is at the heart of all scientific activity; without it any ostensibly scientific activity is as sterile as that of a high school student substituting numbers into a formula. For this reason, science, when I push the analysis back as far as I can, must be private.
Reflections of a Physicist (1950), 72.
The figure of 2.2 children per adult female was felt to be in some respects absurd, and a Royal Commission suggested that the middle classes be paid money to increase the average to a rounder and more convenient number.
— Magazine
Quoted from Punch in epigraph, M.J. Moroney, 'On the Average', Facts From Figures (1951), Chap. 4, 34.
The first nonabsolute number is the number of people for whom the table is reserved. This will vary during the course of the first three telephone calls to the restaurant, and then bear no apparent relation to the number of people who actually turn up, or to the number of people who subsequently join them after the show/match/party/gig, or to the number of people who leave when they see who else has turned up.
The second nonabsolute number is the given time of arrival, which is now known to be one of the most bizarre of mathematical concepts, a recipriversexcluson, a number whose existence can only be defined as being anything other than itself. In other words, the given time of arrival is the one moment of time at which it is impossible that any member of the party will arrive. Recipriversexclusons now play a vital part in many branches of math, including statistics and accountancy and also form the basic equations used to engineer the Somebody Else’s Problem field.
The third and most mysterious piece of nonabsoluteness of all lies in the relationship between the number of items on the check [bill], the cost of each item, the number of people at the table and what they are each prepared to pay for. (The number of people who have actually brought any money is only a subphenomenon of this field.)
The second nonabsolute number is the given time of arrival, which is now known to be one of the most bizarre of mathematical concepts, a recipriversexcluson, a number whose existence can only be defined as being anything other than itself. In other words, the given time of arrival is the one moment of time at which it is impossible that any member of the party will arrive. Recipriversexclusons now play a vital part in many branches of math, including statistics and accountancy and also form the basic equations used to engineer the Somebody Else’s Problem field.
The third and most mysterious piece of nonabsoluteness of all lies in the relationship between the number of items on the check [bill], the cost of each item, the number of people at the table and what they are each prepared to pay for. (The number of people who have actually brought any money is only a subphenomenon of this field.)
Life, the Universe and Everything (1982, 1995), 47-48.
The first principles of the universe are atoms and empty space. Everything else is merely thought to exist. The worlds are unlimited. They come into being and perish. Nothing can come into being from that which is not nor pass away into that which is not. Further, the atoms are unlimited in size and number, and they are borne along in the whole universe in a vortex, and thereby generate all composite things—-fire, water, air, earth. For even these are conglomerations of given atoms. And it is because of their solidarity that these atoms are impassive and unalterable. The sun and the moon have been composed of such smooth and spherical masses [i.e. atoms], and so also the soul, which is identical with reason.
Diogenes Laertius IX, 44. Trans. R. D. Hicks (1925), Vol. 2, 453-5. An alternate translation of the opening is "Nothing exists except atoms and empty space; everything else is opinion."
The game of chess has always fascinated mathematicians, and there is reason to suppose that the possession of great powers of playing that game is in many features very much like the possession of great mathematical ability. There are the different pieces to learn, the pawns, the knights, the bishops, the castles, and the queen and king. The board possesses certain possible combinations of squares, as in rows, diagonals, etc. The pieces are subject to certain rules by which their motions are governed, and there are other rules governing the players. … One has only to increase the number of pieces, to enlarge the field of the board, and to produce new rules which are to govern either the pieces or the player, to have a pretty good idea of what mathematics consists.
In Book review, 'What is Mathematics?', Bulletin American Mathematical Society (May 1912), 18, 386-387.
The generalized theory of relativity has furnished still more remarkable results. This considers not only uniform but also accelerated motion. In particular, it is based on the impossibility of distinguishing an acceleration from the gravitation or other force which produces it. Three consequences of the theory may be mentioned of which two have been confirmed while the third is still on trial: (1) It gives a correct explanation of the residual motion of forty-three seconds of arc per century of the perihelion of Mercury. (2) It predicts the deviation which a ray of light from a star should experience on passing near a large gravitating body, the sun, namely, 1".7. On Newton's corpuscular theory this should be only half as great. As a result of the measurements of the photographs of the eclipse of 1921 the number found was much nearer to the prediction of Einstein, and was inversely proportional to the distance from the center of the sun, in further confirmation of the theory. (3) The theory predicts a displacement of the solar spectral lines, and it seems that this prediction is also verified.
Studies in Optics (1927), 160-1.
The generation of random numbers is too important to be left to chance.
Title of an article (1970). Quoted by M. Gardner (1977), 169. Cited in Gideon Keren and Charles Lewis, A Handbook for data analysis in the behavioral sciences (), 390.
The golden age of mathematics—that was not the age of Euclid, it is ours. Ours is the age when no less than six international congresses have been held in the course of nine years. It is in our day that more than a dozen mathematical societies contain a growing membership of more than two thousand men representing the centers of scientific light throughout the great culture nations of the world. It is in our time that over five hundred scientific journals are each devoted in part, while more than two score others are devoted exclusively, to the publication of mathematics. It is in our time that the Jahrbuch über die Fortschritte der Mathematik, though admitting only condensed abstracts with titles, and not reporting on all the journals, has, nevertheless, grown to nearly forty huge volumes in as many years. It is in our time that as many as two thousand books and memoirs drop from the mathematical press of the world in a single year, the estimated number mounting up to fifty thousand in the last generation. Finally, to adduce yet another evidence of a similar kind, it requires not less than seven ponderous tomes of the forthcoming Encyclopaedie der Mathematischen Wissenschaften to contain, not expositions, not demonstrations, but merely compact reports and bibliographic notices sketching developments that have taken place since the beginning of the nineteenth century.
In Lectures on Science, Philosophy and Art (1908), 8.
The good news is that Americans will, in increasing numbers, begin to value and protect the vast American Landscape. The bad news is that they may love it to death.
The American Land
The grand aim of all science is to cover the greatest number of empirical facts by logical deduction from the smallest possible number of hypotheses or axioms.
(1923). As quoted in Lincoln Barnett, The Universe and Dr. Einstein (1950), 110.
The great object of all knowledge is to enlarge and purify the soul, to fill the mind with noble contemplations, to furnish a refined pleasure, and to lead our feeble reason from the works of nature up to its great Author and Sustainer. Considering this as the ultimate end of science, no branch of it can surely claim precedence of Astronomy. No other science furnishes such a palpable embodiment of the abstractions which lie at the foundation of our intellectual system; the great ideas of time, and space, and extension, and magnitude, and number, and motion, and power. How grand the conception of the ages on ages required for several of the secular equations of the solar system; of distances from which the light of a fixed star would not reach us in twenty millions of years, of magnitudes compared with which the earth is but a foot-ball; of starry hosts—suns like our own—numberless as the sands on the shore; of worlds and systems shooting through the infinite spaces.
Oration at Inauguration of the Dudley Astronomical Observatory, Albany (28 Jul 1856). Text published as The Uses of Astronomy (1856), 36.
The harmony of the universe knows only one musical form - the legato; while the symphony of number knows only its opposite - the staccato. All attempts to reconcile this discrepancy are based on the hope that an accelerated staccato may appear to our senses as a legato.
…...
The history of the word sankhyā shows the intimate connection which has existed for more than 3000 years in the Indian mind between ‘adequate knowledge’ and ‘number.’ As we interpret it, the fundamental aim of statistics is to give determinate and adequate knowledge of reality with the help of numbers and numerical analysis. The ancient Indian word Sankhyā embodies the same idea, and this is why we have chosen this name for the Indian Journal of Statistics.
Editorial, Vol. 1, Part 1, in the new statistics journal of the Indian Statistical Institute, Sankhayā (1933). Also reprinted in Sankhyā: The Indian Journal of Statistics (Feb 2003), 65, No. 1, xii.
The human understanding when it has once adopted an opinion (either as being the received opinion or as being agreeable to itself) draws all things else to support and agree with it. And though there be a greater number and weight of instances to be found on the other side, yet these it either neglects and despises, or else by some distinction sets aside and rejects, in order that by this great and pernicious predetermination the authority of its former conclusions may remain inviolate.
From Aphorism 46, Novum Organum, Book I (1620). Collected in James Spedding (ed.), The Works of Francis Bacon (1858), Vol. 4, 56.
The ideas which these sciences, Geometry, Theoretical Arithmetic and Algebra involve extend to all objects and changes which we observe in the external world; and hence the consideration of mathematical relations forms a large portion of many of the sciences which treat of the phenomena and laws of external nature, as Astronomy, Optics, and Mechanics. Such sciences are hence often termed Mixed Mathematics, the relations of space and number being, in these branches of knowledge, combined with principles collected from special observation; while Geometry, Algebra, and the like subjects, which involve no result of experience, are called Pure Mathematics.
In The Philosophy of the Inductive Sciences (1868), Part 1, Bk. 2, chap. 1, sect. 4.
The ingenuity and effective logic that enabled chemists to determine complex molecular structures from the number of isomers, the reactivity of the molecule and of its fragments, the freezing point, the empirical formula, the molecular weight, etc., is one of the outstanding triumphs of the human mind.
'Trends in Chemistry', Chemical Engineering News, 7 Jan 1963, 5.
The intensity and quantity of polemical literature on scientific problems frequently varies inversely as the number of direct observations on which the discussions are based: the number and variety of theories concerning a subject thus often form a coefficient of our ignorance. Beyond the superficial observations, direct and indirect, made by geologists, not extending below about one two-hundredth of the Earth's radius, we have to trust to the deductions of mathematicians for our ideas regarding the interior of the Earth; and they have provided us successively with every permutation and combination possible of the three physical states of matter—solid, liquid, and gaseous.
'Address delivered by the President of Section [Geology] at Sydney (Friday, Aug 21), Report of the Eighty-Fourth Meeting of the British Association for the Advancement of Science: Australia 1914, 1915, 345.
The judicial mind is too commonly characterized by a regard for a fourth decimal as the equal of a whole number.
The key to understanding overpopulation is not population density but the numbers of people in an area relative to its resources and the capacity of the environment to sustain human activities; that is, to the area’s carrying capacity. When is an area overpopulated? When its population can’t be maintained without rapidly depleting nonrenewable resources…. By this standard, the entire planet and virtually every nation is already vastly overpopulated.
In The Population Explosion (1990), 39.
The last proceeding of reason is to recognize that there an infinity of things which are beyond it.
Pensées (1670), Section 5, 1. As translated in Blaise Pascal and W.F. Trotter (trans.), 'Thoughts', No. 267, collected in Charles W. Eliot (ed.), The Harvard Classics (1910), Vol. 48, 97. Also seen translated as, “Reason’s last step is the recognition that…”. From the original French, “La dernière démarche de la raison est de reconnaître qu’il y a une infinité de choses qui la surpassent; elle n’est que faible, si elle ne va jusqu’à connaître cela,” in Pensées de Blaise Pascal (1847), 110.
The late Alan Gregg pointed out that human population growth within the ecosystem was closely analogous to the growth of malignant tumor cells within an organism: that man was acting like a cancer on the biosphere. The multiplication of human numbers certainly seems wild and uncontrolled… Four million a month—the equivalent of the population of Chicago… We seem to be doing all right at the moment; but if you could ask cancer cells, I suspect they would think they were doing fine. But when the organism dies, so do they; and for our own, selfish, practical, utilitarian reasons, I think we should be careful about how we influence the rest of the ecosystem.
From Horace M. Albright Conservation Lectureship Berkeley, California (23 Apr 1962), 'The Human Environment', collected in Conservators of Hope: the Horace M. Albright Conservation Lectures (1988), 44.
The law of conservation rigidly excludes both creation and annihilation. Waves may change to ripples, and ripples to waves,—magnitude may be substituted for number, and number for magnitude,—asteroids may aggregate to suns, suns may resolve themselves into florae and faunae, and florae and faunae melt in air,—the flux of power is eternally the same. It rolls in music through the ages, and all terrestrial energy,—the manifestations of life, as well as the display of phenomena, are but the modulations of its rhythm.
Conclusion to lecture 12 (10 Apr 1862) at the Royal Institution, collected in Heat Considered as a Mode of Motion: Being a Course of Twelve Lectures (1863), 449.
The laws of thermodynamics, as empirically determined, express the approximate and probable behavior of systems of a great number of particles, or, more precisely, they express the laws of mechanics for such systems as they appear to beings who have not the fineness of perception to enable them to appreciate quantities of the order of magnitude of those which relate to single particles, and who cannot repeat their experiments often enough to obtain any but the most probable results.
Elementary Principles in Statististical Mechanics (1902), Preface, viii.
The limitations of archaeology are galling. It collects phenomena, but hardly ever can isolate them so as to interpret scientifically; it can frame any number of hypotheses, but rarely, if ever, scientifically prove.
In Times Literary Supplement (29 Dec 1921), 869, as cited in J.A. Macgillivray, Minotaur: Sir Arthur Evans and the Archaeology of the Minoan Myth (2000), 271.
The long-range trend toward federal regulation, which found its beginnings in the Interstate Commerce Act of 1887 and the Sherman Act of 1890, which was quickened by a large number of measures in the Progressive era, and which has found its consummation in our time, was thus at first the response of a predominantly individualistic public to the uncontrolled and starkly original collectivism of big business. In America the growth of the national state and its regulative power has never been accepted with complacency by any large part of the middle-class public, which has not relaxed its suspicion of authority, and which even now gives repeated evidence of its intense dislike of statism. In our time this growth has been possible only under the stress of great national emergencies, domestic or military, and even then only in the face of continuous resistance from a substantial part of the public. In the Progressive era it was possible only because of widespread and urgent fear of business consolidation and private business authority. Since it has become common in recent years for ideologists of the extreme right to portray the growth of statism as the result of a sinister conspiracy of collectivists inspired by foreign ideologies, it is perhaps worth emphasizing that the first important steps toward the modern organization of society were taken by arch-individualists—the tycoons of the Gilded Age—and that the primitive beginning of modern statism was largely the work of men who were trying to save what they could of the eminently native Yankee values of individualism and enterprise.
In The Age of Reform: From Bryan to F.D.R. (1955, 2011), 233-234.
The maintenance of biological diversity requires special measures that extend far beyond the establishment of nature reserves. Several reasons for this stand out. Existing reserves have been selected according to a number of criteria, including the desire to protect nature, scenery, and watersheds, and to promote cultural values and recreational opportunities. The actual requirements of individual species, populations, and communities have seldom been known, nor has the available information always been employed in site selection and planning for nature reserves. The use of lands surrounding nature reserves has typically been inimical to conservation, since it has usually involved heavy use of pesticides, industrial development, and the presence of human settlements in which fire, hunting, and firewood gathering feature as elements of the local economy.
The Fragmented Forest: Island Biogeography Theory and the Preservation of Biotic Diversity (1984), xii.
The material universe must consist ... of bodies ... such that each of them exercises its own separate, independent, and invariable effect, a change of the total state being compounded of a number of separate changes each of which is solely due to a separate portion of the preceding state.
A Treatise on Probability (), 249. In Antony Flew, A Dictionary of Philosophy (1984), 30.
The mathematical formulation of the physicist’s often crude experience leads in an uncanny number of cases to an amazingly accurate description of a large class of phenomena. This shows that the mathematical language has more to commend it than being the only language which we can speak; it shows that it is, in a very real sense, the correct language.
In 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences,' Communications in Pure and Applied Mathematics (Feb 1960), 13, No. 1 (February 1960). Collected in Eugene Paul Wigner, A.S. Wightman (ed.), Jagdish Mehra (ed.), The Collected Works of Eugene Paul Wigner (1955), Vol. 6, 542.
The mathematical intellectualism is henceforth a positive doctrine, but one that inverts the usual doctrines of positivism: in place of originating progress in order, dynamics in statics, its goal is to make logical order the product of intellectual progress. The science of the future is not enwombed, as Comte would have had it, as Kant had wished it, in the forms of the science already existing; the structure of these forms reveals an original dynamism whose onward sweep is prolonged by the synthetic generation of more and more complicated forms. No speculation on number considered as a category a priori enables one to account for the questions set by modern mathematics … space affirms only the possibility of applying to a multiplicity of any elements whatever, relations whose type the intellect does not undertake to determine in advance, but, on the contrary, it asserts their existence and nourishes their unlimited development.
As translated in James Byrnie Shaw, Lectures on the Philosophy of Mathematics (1918), 193. From Léon Brunschvicg, Les Étapes de La Philosophie Mathématique (1912), 567-568, “L’intellectualisme mathématique est désormais une doctrine positive, mais qui intervertira les formules habituelles du positivisme: au lieu de faire sortir le progrès de l’ordre, ou le dynamique du statique, il tend à faire de l'ordre logique le produit du progrès intellectuel. La science à venir n'est pas enfermée, comme l’aurait voulu Comte, comme le voulait déjà Kant, dans les formes de la science déjà faite; la constitution de ces formes révèle un dynamisme originel dont l’élan se prolonge par la génération synthétique de notions de plus en plus compliquées. Aucune spéculation sur le nombre, considéré comme catégorie a priori, ne permet de rendre compte des questions qui se sont posées pour la mathématique moderne … … l’espace ne fait qu'affirmer la possibilité d'appliquer sur une multiplicité d’éléments quelconques des relations dont l’intelligence ne cherche pas à déterminer d’avance le type, dont elle constate, au contraire, dont elle suscite le développement illimité.”
The Mathematician deals with two properties of objects only, number and extension, and all the inductions he wants have been formed and finished ages ago. He is now occupied with nothing but deduction and verification.
In 'On the Educational Value of the Natural History Sciences', Lay Sermons, Addresses and Reviews (1872), 87.
The mathematics of cooperation of men and tools is interesting. Separated men trying their individual experiments contribute in proportion to their numbers and their work may be called mathematically additive. The effect of a single piece of apparatus given to one man is also additive only, but when a group of men are cooperating, as distinct from merely operating, their work raises with some higher power of the number than the first power. It approaches the square for two men and the cube for three. Two men cooperating with two different pieces of apparatus, say a special furnace and a pyrometer or a hydraulic press and new chemical substances, are more powerful than their arithmetical sum. These facts doubtless assist as assets of a research laboratory.
Quoted from a speech delivered at the fiftieth anniversary of granting of M.I.T's charter, in Guy Suits, 'Willis Rodney Whitney', National Academy of Sciences, Biographical Memoirs (1960), 352.
The measure of the probability of an event is the ratio of the number of cases favourable to that event, to the total number of cases favourable or contrary, and all equally possible, or all of which have the same chance.
In 'Règles générales des probabilités', Recherches sur la Probabilités des Jugemens (1837), Chap. 1, 31, as translated in George Boole, An Investigation of the Laws of Thought (1854), 244. From the original French, “La mesure de la probabilité d'un événement, est le rapport du nombre de cas favorables à cet événement, au nombre total de cas favorables ou contraires, et tous également possibles, ou qui ont tous une même chance.”
The method I take to do this is not yet very usual; for instead of using only comparative and superlative Words, and intellectual Arguments, I have taken the course (as a Specimen of the Political Arithmetic I have long aimed at) to express myself in Terms of Number, Weight, or Measure; to use only Arguments of Sense, and to consider only such Causes, as have visible Foundations in Nature.
From Essays in Political Arithmetic (1679, 1755), 98.
The method of producing these numbers is called a sieve by Eratosthenes, since we take the odd numbers mingled and indiscriminate and we separate out of them by this method of production, as if by some instrument or sieve, the prime and incomposite numbers by themselves, and the secondary and composite numbers by themselves, and we find separately those that are mixed.
Nicomachus, Introduction to Arithmetic, 1.13.2. Quoted in Morris R. Cohen and I. E. Drabkin, A Sourcebook in Greek Science (1948), 19-20.
The methods of theoretical physics should be applicable to all those branches of thought in which the essential features are expressible with numbers.
Nobel Prize Banquet Speech (10 Dec1933). In Carl Gustaf Santesson (Ed.), Les Prix Nobel en 1933 (1935), 78
The more man inquires into the laws which regulate the material universe, the more he is convinced that all its varied forms arise from the action of a few simple principles. These principles themselves converge, with accelerating force, towards some still more comprehensive law to which all matter seems to be submitted. Simple as that law may possibly be, it must be remembered that it is only one amongst an infinite number of simple laws: that each of these laws has consequences at least as extensive as the existing one, and therefore that the Creator who selected the present law must have foreseen the consequences of all other laws.
In Passages from the Life of a Philosopher (1864), 402.
The most abstract statements or propositions in science are to be regarded as bundles of hypothetical maxims packed into a portable shape and size. Every scientific fact is a short-hand expression for a vast number of practical directions: if you want so-and-so, do so-and-so.
In 'On The Scientific Basis of Morals', Contemporary Review (Sep 1875), collected in Leslie Stephen and Frederick Pollock (eds.), Lectures and Essays: By the Late William Kingdon Clifford, F.R.S. (1886), 289.
The natural history of these islands is eminently curious, and well deserves attention. Most of the organic productions are aboriginal creations, found nowhere else; there is even a difference between the inhabitants of the different islands; yet all show a marked relationship with those of America, though separated from that continent by an open space of ocean, between 500 and 600 miles in width. The archipelago is a little world within itself, or rather a satellite attached to America, whence it has derived a few stray colonists, and has received the general character of its indigenous productions. Considering the small size of these islands, we feel the more astonished at the number of their aboriginal beings, and at their confined range. Seeing every height crowned with its crater, and the boundaries of most of the lava-streams still distinct, we are led to believe that within a period, geologically recent, the unbroken ocean was here spread out. Hence, both in space and time, we seem to be brought somewhere near to that great fact—that mystery of mysteries—the first appearance of new beings on this earth.
Journal of Researches into the Natural History and Geology of the Countries Visited During the Voyage of H.M.S. Beagle Round the World, 2nd edn. (1845), 377-8.
The next care to be taken, in respect of the Senses, is a supplying of their infirmities with Instruments, and, as it were, the adding of artificial Organs to the natural; this in one of them has been of late years accomplisht with prodigious benefit to all sorts of useful knowledge, by the invention of Optical Glasses. By the means of Telescopes, there is nothing so far distant but may be represented to our view; and by the help of Microscopes, there is nothing so small, as to escape our inquiry; hence there is a new visible World discovered to the understanding. By this means the Heavens are open'd, and a vast number of new Stars, and new Motions, and new Productions appear in them, to which all the ancient Astronomers were utterly Strangers. By this the Earth it self, which lyes so neer us, under our feet, shews quite a new thing to us, and in every little particle of its matter, we now behold almost as great a variety of creatures as we were able before to reckon up on the whole Universe it self.
Micrographia, or some Physiological Descriptions of Minute Bodies made by Magnifying Glasses with Observations and Inquiries thereupon (1665), preface, sig. A2V.
The northern ocean is beautiful, ... and beautiful the delicate intricacy of the snowflake before it melts and perishes, but such beauties are as nothing to him who delights in numbers, spurning alike the wild irrationality of life and baffling complexity of nature’s laws.
In Kandelman's Krim: A Realistic Fantasy (1957), 101.
The number of fixed stars which observers have been able to see without artificial powers of sight up to this day can be counted. It is therefore decidedly a great feat to add to their number, and to set distinctly before the eyes other stars in myriads, which have never been seen before, and which surpass the old, previously known stars in number more than ten times.
In pamphlet, The Sidereal Messenger (1610), reprinted in The Sidereal Messenger of Galileo Galilei: And a Part of the Preface to the Preface to Kepler's Dioptrics Containing the Original Account of Galileo's Astronomical Discoveries (1880), 7-8.
The number of humble-bees in any district depends in a great degree on the number of field-mice, which destroy their combs and nests; and Mr. H. Newman, who has long attended to the habits of humble-bees, ... says “Near villages and small towns I have found the nests of humble-bees more numerous than elsewhere, which I attribute to the number of cats that destroy the mice.” Hence it is quite credible that the presence of a feline animal in large numbers in a district might determine, through the intervention first of mice and then of bees, the frequency of certain flowers in that district!
From On the Origin of Species by Means of Natural Selection; or, The Preservation of Favoured Races in the Struggle for Life (1861), 72.
The number of hypotheses and theories about climate change are numerous. Quite naturally they have caught the public attention, as any proof of past climactic change points to the possibility of future climate change, which inevitably will have significant implications for global economics.
'Klimaschwankungen seit 1700 nebst Bemerkungen fiber die Klimaschwankungen der Diluvialzeit' Pencks Geographische Abhandlingen (1890) 4,2. In Eduard Brückner, Nico Stehr (Ed.), Hans von Storch (Ed.) and Barbara Stehr, Eduard Brückner (2000), 11.
The number of mathematical students … would be much augmented if those who hold the highest rank in science would condescend to give more effective assistance in clearing the elements of the difficulties which they present.
In Study and Difficulties of Mathematics (1902), Preface.
The number of rational hypotheses that can explain any given phenomenon is infinite.
In Zen and the Art of Motorcycle Maintenance: An inquiry into Values (1974), 107.
The number of stars making up the Milky Way is about 10¹¹ or something like the number of raindrops falling in Hyde Park in a day’s heavy rain.
From review of Harlow Shapley, Of Stars and Men in 'Man and his Universe', New Scientist (12 Mar 1959), 594,
The number of travellers by gigs, the outside of coaches, and on horseback, have, since the introduction of railways, been prodigiously diminished; and as, in addition, the members of the medical faculty having lent their aid to run down the use of water-proof (apparently having found it decided enemy against their best friends colds and catarrhs), the use of the article [the Macintosh] in the form of cloaks, etc., has of late become comparatively extinct.
A Biographical Memoir of the late Charles Macintosh Esq FRS (1847), 89.
The numbers are a catalyst that can help turn raving madmen into polite humans.
Excerpt from address by Davis to a scientific society, 'The Philadelphia Story' collected in Philip Davis and William G. Chinn, 3.146 and All That (1985), 64. The context is the result of a "take a number" ticket used to organize a train station crowd competing for available taxis. Instead of chaos from queue jumping, the dispatcher used the ticket numbers for the loading order as each taxi pulled up.
The opinion I formed from attentive observation of the facts and phenomena, is as follows. When ice, for example, or any other solid substance, is changing into a fluid by heat, I am of opinion that it receives a much greater quantity of heat than that what is perceptible in it immediately after by the thermometer. A great quantity of heat enters into it, on this occasion, without making it apparently warmer, when tried by that instrument. This heat, however, must be thrown into it, in order to give it the form of a fluid; and I affirm, that this great addition of heat is the principal, and most immediate cause of the fluidity induced. And, on the other hand, when we deprive such a body of its fluidity again, by a diminution of its heat, a very great quantity of heat comes out of it, while it is assuming a solid form, the loss of which heat is not to be perceived by the common manner of using the thermometer. The apparent heat of the body, as measured by that instrument, is not diminished, or not in proportion to the loss of heat which the body actually gives out on this occasion; and it appears from a number of facts, that the state of solidity cannot be induced without the abstraction of this great quantity of heat. And this confirms the opinion, that this quantity of heat, absorbed, and, as it were, concealed in the composition of fluids, is the most necessary and immediate cause of their fluidity.
Lectures on the Elements of Chemistry, delivered in the University of Edinburgh (1803), Vol. I, 116-7.
The owner of the means of production is in a position to purchase the labor power of the worker. By using the means of production, the worker produces new goods which become the property of the capitalist. The essential point about this process is the relation between what the worker produces and what he is paid, both measured in terms of real value. In so far as the labor contract is free what the worker receives is determined not by the real value of the goods he produces, but by his minimum needs and by the capitalists’ requirements for labor power in relation to the number of workers competing for jobs. It is important to understand that even in theory the payment of the worker is not determined by the value of his product.
…...
The past is a bank where an unlimited number of ideas have been deposited to our credit.
Life and History (1922), 19.
The present rate of progress [in X-ray crystallography] is determined, not so much by the lack of problems to investigate or the limited power of X-ray analysis, as by the restricted number of investigators who have had a training in the technique of the new science, and by the time it naturally takes for its scientific and technical importance to become widely appreciated.
Concluding remark in Lecture (1936) on 'Forty Years of Crystal Physics', collected in Needham and Pagel (eds.) in Background to Modern Science: Ten Lectures at Cambridge Arranged by the History of Science Committee, (1938), 89.
The present state of the system of nature is evidently a consequence of what it was in the preceding moment, and if we conceive of an intelligence that at a given instant comprehends all the relations of the entities of this universe, it could state the respective position, motions, and general affects of all these entities at any time in the past or future. Physical astronomy, the branch of knowledge that does the greatest honor to the human mind, gives us an idea, albeit imperfect, of what such an intelligence would be. The simplicity of the law by which the celestial bodies move, and the relations of their masses and distances, permit analysis to follow their motions up to a certain point; and in order to determine the state of the system of these great bodies in past or future centuries, it suffices for the mathematician that their position and their velocity be given by observation for any moment in time. Man owes that advantage to the power of the instrument he employs, and to the small number of relations that it embraces in its calculations. But ignorance of the different causes involved in the production of events, as well as their complexity, taken together with the imperfection of analysis, prevents our reaching the same certainty about the vast majority of phenomena. Thus there are things that are uncertain for us, things more or less probable, and we seek to compensate for the impossibility of knowing them by determining their different degrees of likelihood. So it was that we owe to the weakness of the human mind one of the most delicate and ingenious of mathematical theories, the science of chance or probability.
'Recherches, 1º, sur l'Intégration des Équations Différentielles aux Différences Finies, et sur leur Usage dans la Théorie des Hasards' (1773, published 1776). In Oeuvres complètes de Laplace, 14 Vols. (1843-1912), Vol. 8, 144-5, trans. Charles Coulston Gillispie, Pierre-Simon Laplace 1749-1827: A Life in Exact Science (1997), 26.
The Principle of Uncertainty is a bad name. In science or outside of it we are not uncertain; our knowledge is merely confined, within a certain tolerance. We should call it the Principle of Tolerance. And I propose that name in two senses: First, in the engineering sense, science has progressed, step by step, the most successful enterprise in the ascent of man, because it has understood that the exchange of information between man and nature, and man and man, can only take place with a certain tolerance. But second, I also use the word, passionately, about the real world. All knowledge, all information between human beings, can only be exchanged within a play of tolerance. And that is true whether the exchange is in science, or in literature, or in religion, or in politics, or in any form of thought that aspires to dogma. It’s a major tragedy of my lifetime and yours that scientists were refining, to the most exquisite precision, the Principle of Tolerance, and turning their backs on the fact that all around them, tolerance was crashing to the ground beyond repair. The Principle of Uncertainty or, in my phrase, the Principle of Tolerance, fixed once for all the realization that all knowledge is limited. It is an irony of history that at the very time when this was being worked out there should rise, under Hitler in Germany and other tyrants elsewhere, a counter-conception: a principle of monstrous certainty. When the future looks back on the 1930s it will think of them as a crucial confrontation of culture as I have been expounding it, the ascent of man, against the throwback to the despots’ belief that they have absolute certainty. It is said that science will dehumanize people and turn them into numbers. That is false: tragically false. Look for yourself. This is the concentration camp and crematorium at Auschwitz. This is where people were turned into numbers. Into this pond were flushed the ashes of four million people. And that was not done by gas. It was done by arrogance. It was done by dogma. It was done by ignorance. When people believe that they have absolute knowledge, with no test in reality this is how they behave. This is what men do when they aspire to the knowledge of gods. Science is a very human form of knowledge. We are always at the brink of the known; we always feel forward for what is to be hoped. Every judgment in science stands on the edge of error, and is personal. Science is a tribute to what we can know although we are fallible. In the end, the words were said by Oliver Cromwell: “I beseech you, in the bowels of Christ: Think it possible you may be mistaken.” We have to cure ourselves of the itch for absolute knowledge and power. We have to close the distance between the push-button order and the human act. We have to touch people. [Referring to Heisenberg’s Uncertainty Principle.]
'Knowledge or Certainty,' episode 11, The Ascent of Man (1972), BBC TV series.
The problem of distinguishing prime numbers from composite numbers and of resolving the latter into their prime factors is known to be one of the most important and useful in arithmetic. It has engaged the industry and wisdom of ancient and modern geometers to such an extent that it would be superfluous to discuss the problem at length... Further, the dignity of the science itself seems to require that every possible means be explored for the solution of a problem so elegant and so celebrated.
Disquisitiones Arithmeticae (1801), Article 329
The processes concerned in simple descent are those of Family Variability and Reversion. It is well to define these words clearly. By family variability is meant the departure of the children of the same or similarly descended families from the ideal mean type of all of them. Reversion is the tendency of that ideal mean type to depart from the parent type, 'reverting' towards what may be roughly and perhaps fairly described as the average ancestral type. If family variability had been the only process in simple descent, the dispersion of the race would indefinitely increase with the number of the generations, but reversion checks this increase, and brings it to a standstill.
Typical Laws of Heredity (1877), 513.
The progress of the art of rational discovery depends in a great part upon the art of characteristic (ars characteristica). The reason why people usually seek demonstrations only in numbers and lines and things represented by these is none other than that there are not, outside of numbers, convenient characters corresponding to the notions.
Translated by Gerhard from Philosophische Schriften, 8, 198. As quoted in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-Book (1914), 205.
The purely formal sciences, logic and mathematics, deal with such relations which are independent of the definite content, or the substance of the objects, or at least can be. In particular, mathematics involves those relations of objects to each other that involve the concept of size, measure, number.
In Theorie der Complexen Zahlensysteme, (1867), 1. Translated by Webmaster using Google Translate from the original German, “Die rein formalen Wissenschaften, Logik und Mathematik, haben solche Relationen zu behandeln, welche unabhängig von dem bestimmten Inhalte, der Substanz der Objecte sind oder es wenigstens sein können.”
The purely formal Sciences, logic and mathematics, deal with those relations which are, or can be, independent of the particular content or the substance of objects. To mathematics in particular fall those relations between objects which involve the concepts of magnitude, of measure and of number.
In Theorie der Complexen Zahlensysteme (1867), 1. As translated in Robert Édouard Moritz, Memorabilia Mathematica; Or, The Philomath’s Quotation-book (1914), 4. From the original German, “Die rein formalen Wissenschaften, Logik und Mathematik, haben solche Relationen zu behandeln, welche unabhängig von dem bestimmten Inhalte, der Substanz der Objecte sind oder es wenigstens sein können. Der Mathematik fallen ins Besondere diejenigen Beziehungen der Objecte zu einander zu, die den Begriff der Grösse, des Maasses, der Zahl involviren.”
The purpose of computation is insight, not numbers.
Motto of the book, Numerical Analysis for Scientists and Engineers (1973), v.
The purpose of computing is insight, not numbers. … [But] sometimes … the purpose of computing numbers is not yet in sight.
Motto for the book, Numerical Methods for Scientists and Engineers (1962, 1973), 3. The restatement of the motto (merged above as second sentence) is suggested on p.504, footnote.
The pursuit of mathematical science makes its votary appear singularly indifferent to the ordinary interests and cares of men. Seeking eternal truths, and finding his pleasures in the realities of form and number, he has little interest in the disputes and contentions of the passing hour. His views on social and political questions partake of the grandeur of his favorite contemplations, and, while careful to throw his mite of influence on the side of right and truth, he is content to abide the workings of those general laws by which he doubts not that the fluctuations of human history are as unerringly guided as are the perturbations of the planetary hosts.
In 'Imagination in Mathematics', North American Review, 85, 227.
The qualities of number appear to lead to the apprehension of truth.
— Plato
The Republic 7 525b, trans. P. Shorey (1935), Vol. 2, Book 7, 161.
The reactions follow a pattern, which is valid for the blood of all humans... Basically, in fact, there are four different types of human blood, the so-called blood groups. The number of the groups follows from the fact that the erythrocytes evidently contain substances (iso-agglutinogens) with two different structures, of which both may be absent, or one or both present, in the erythrocytes of a person. This alone would still not explain the reactions; the active substances of the sera, the iso-agglutinins, must also be present in a specific distribution. This is actually the case, since every serum contains those agglutinins which react with the agglutinogens not present in the cells—a remarkable phenomenon, the cause of which is not yet known for certain.
'On Individual Differences in Human Blood', Nobel Lecture (11 Dec 1930). In Nobel Lectures: Physiology or Medicine 1922-1941 (1965), 235.
The Reader may here observe the Force of Numbers, which can be successfully applied, even to those things, which one would imagine are subject to no Rules. There are very few things which we know, which are not capable of being reduc’d to a Mathematical Reasoning, and when they cannot, it’s a sign our Knowledge of them is very small and confus’d; and where a mathematical reasoning can be had, it’s as great folly to make use of any other, as to grope for a thing in the dark when you have a Candle standing by you.
From 'Preface' to Of the Laws of Chance, or, a Method of the Hazards of Game (1692), The book was Arbuthnot’s translation of Christiaan Huygen, Tractatus de Rationciniis in Aleae Ludo, in which Huygen expanded on the work of Blaise Pascal in probability and statistics.
The Reason of making Experiments is, for the Discovery of the Method of Nature, in its Progress and Operations. Whosoever, therefore doth rightly make Experiments, doth design to enquire into some of these Operations; and, in order thereunto, doth consider what Circumstances and Effects, in the Experiment, will be material and instructive in that Enquiry, whether for the confirming or destroying of any preconceived Notion, or for the Limitation and Bounding thereof, either to this or that Part of the Hypothesis, by allowing a greater Latitude and Extent to one Part, and by diminishing or restraining another Part within narrower Bounds than were at first imagin'd, or hypothetically supposed. The Method therefore of making Experiments by the Royal Society I conceive should be this.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
First, To propound the Design and Aim of the Curator in his present Enquiry.
Secondly, To make the Experiment, or Experiments, leisurely, and with Care and Exactness.
Thirdly, To be diligent, accurate, and curious, in taking Notice of, and shewing to the Assembly of Spectators, such Circumstances and Effects therein occurring, as are material, or at least, as he conceives such, in order to his Theory .
Fourthly, After finishing the Experiment, to discourse, argue, defend, and further explain, such Circumstances and Effects in the preceding Experiments, as may seem dubious or difficult: And to propound what new Difficulties and Queries do occur, that require other Trials and Experiments to be made, in order to their clearing and answering: And farther, to raise such Axioms and Propositions, as are thereby plainly demonstrated and proved.
Fifthly, To register the whole Process of the Proposal, Design, Experiment, Success, or Failure; the Objections and Objectors, the Explanation and Explainers, the Proposals and Propounders of new and farther Trials; the Theories and Axioms, and their Authors; and, in a Word the history of every Thing and Person, that is material and circumstantial in the whole Entertainment of the said Society; which shall be prepared and made ready, fairly written in a bound Book, to be read at the Beginning of the Sitting of the Society: The next Day of their Meeting, then to be read over and further discoursed, augmented or diminished, as the Matter shall require, and then to be sign'd by a certain Number of the Persons present, who have been present, and Witnesses of all the said Proceedings, who, by Subscribing their names, will prove undoubted testimony to Posterity of the whole History.
'Dr Hooke's Method of Making Experiments' (1664-5). In W. Derham (ed.), Philosophical Experiments and Observations Of the Late Eminent Dr. Robert Hooke, F.R.S. And Geom. Prof. Gresh. and Other Eminent Virtuoso's in his Time (1726), 26-8.
The result of teaching small parts of a large number of subjects is the passive reception of disconnected ideas, not illuminated with any spark of vitality. Let the main ideas which are introduced into a child’s education be few and important, and let them be thrown into every combination possible.
In The Organisation of Thought: Educational and Scientific (1917), 5.
The risk of developing carcinoma of the lung increases steadily as the amount smoked increases. If the risk among non-smokers is taken as unity and the resulting ratios in the three age groups in which a large number of patients were interviewed (ages 45 to 74) are averaged, the relative risks become 6, 19, 26, 49, and 65 when the number of cigarettes smoked a day are 3, 10, 20, 35, and, say, 60—that is, the mid-points of each smoking group. In other words, on the admittedly speculative assumptions we have made, the risk seems to vary in approximately simple proportion with the amount smoked.
William Richard Shaboe Doll and Austin Bradford Hill (1897-1991 British medical statistician) 'Smoking and Carcinoma of the Lung', British Medical Journal, 1950, ii, 746.
The role of inhibition in the working of the central nervous system has proved to be more and more extensive and more and more fundamental as experiment has advanced in examining it. Reflex inhibition can no longer be regarded merely as a factor specially developed for dealing with the antagonism of opponent muscles acting at various hinge-joints. Its role as a coordinative factor comprises that, and goes beyond that. In the working of the central nervous machinery inhibition seems as ubiquitous and as frequent as is excitation itself. The whole quantitative grading of the operations of the spinal cord and brain appears to rest upon mutual interaction between the two central processes 'excitation' and 'inhibition', the one no less important than the other. For example, no operation can be more important as a basis of coordination for a motor act than adjustment of the quantity of contraction, e.g. of the number of motor units employed and the intensity of their individual tetanic activity. This now appears as the outcome of nice co-adjustment of excitation and inhibition upon each of all the individual units which cooperate in the act.
Inhibition as a Coordinative Factor', Nobel Lecture (12 Dec 1932). Nobel Lectures: Physiology or Medicine 1922-1941 (1965), 288.
The rudest numerical scales, such as that by which the mineralogists distinguish different degrees of hardness, are found useful. The mere counting of pistils and stamens sufficed to bring botany out of total chaos into some kind of form. It is not, however, so much from counting as from measuring, not so much from the conception of number as from that of continuous quantity, that the advantage of mathematical treatment comes. Number, after all, only serves to pin us down to a precision in our thoughts which, however beneficial, can seldom lead to lofty conceptions, and frequently descend to pettiness.
On the Doctrine of Chances, with Later Reflections (1878), 61-2.
The rules of scientific investigation always require us, when we enter the domains of conjecture, to adopt that hypothesis by which the greatest number of known facts and phenomena may be reconciled.
In The Physical Geography of the Sea (1855), 123.
The same algebraic sum of positive and negative charges in the nucleus, when the arithmetical sum is different, gives what I call “isotopes” or “isotopic elements,” because they occupy the same place in the periodic table. They are chemically identical, and save only as regards the relatively few physical properties which depend upon atomic mass directly, physically identical also. Unit changes of this nuclear charge, so reckoned algebraically, give the successive places in the periodic table. For any one “place” or any one nuclear charge, more than one number of electrons in the outer-ring system may exist, and in such a case the element exhibits variable valency. But such changes of number, or of valency, concern only the ring and its external environment. There is no in- and out-going of electrons between ring and nucleus.
Concluding paragraph of 'Intra-atomic Charge', Nature (1913), 92, 400. Collected in Alfred Romer, Radiochemistry and the Discovery of Isotopes (1970), 251-252.
The scientist, if he is to be more than a plodding gatherer of bits of information, needs to exercise an active imagination. The scientists of the past whom we now recognize as great are those who were gifted with transcendental imaginative powers, and the part played by the imaginative faculty of his daily life is as least as important for the scientist as it is for the worker in any other field—much more important than for most. A good scientist thinks logically and accurately when conditions call for logical and accurate thinking—but so does any other good worker when he has a sufficient number of well-founded facts to serve as the basis for the accurate, logical induction of generalizations and the subsequent deduction of consequences.
‘Imagination in Science’, Tomorrow (Dec 1943), 38-9. Quoted In Barbara Marinacci (ed.), Linus Pauling In His Own Words: Selected Writings, Speeches, and Interviews (1995), 82.
The search for extraterrestrial intelligence (SETI, to us insiders) has so far only proved that no matter what you beam up—the Pythagorean theorem, pictures of attractive nude people, etc.—the big 800 number in the sky does not return calls.
From essay 'First Person Secular: Blocking the Gates to Heaven', Mother Jones Magazine (Jun 1986), 48. Collected in The Worst Years of our Lives: Irreverent Notes from a Decade of Greed (1995), 267.
The secrets of evolution are death and time—the deaths of enormous numbers of lifeforms that were imperfectly adapted to the environment; and time for a long succession of small mutations.
Cosmos (1980, 1985), 20.
The seventeenth century witnessed the birth of modern science as we know it today. This science was something new, based on a direct confrontation of nature by experiment and observation. But there was another feature of the new science—a dependence on numbers, on real numbers of actual experience.
From The Triumph of Numbers: How Counting Shaped Modern Life (2005), 37.
The sight of day and night, and the months and the revolutions of the years, have created number and have given us conception of time, and the power of inquiring about the nature of the Universe.
— Plato
In Timaeus section 47a, as translated by Benjamin Jowett (1871, 1959), 29. The translation by W.R.M. Lamb gives this passage as “The vision of day and night and of months and circling years has created the art of number and has given us not only the notion of Time but also means of research into the nature of the Universe.”
The signs of liver inflammation [hepatitis] are eight in number, as follows: high fever, thirst, complete anorexia, a tongue which is initially red and then turns black, biliary vomitus initially yellow egg yolk in color, which later turns dark green, pain on the right side which ascends up to the clavicle. … Occasionally a mild cough may occur and a sensation of heaviness which is first felt on the right side and then spreads widely.
As quoted in Fred Rosner, The Medical Legacy of Moses Maimonides (1998), 53-54.
The simplicity of nature is not to be measured by that of our conceptions. Infinitely varied in its effects, nature is simple only in its causes, and its economy consists in producing a great number of phenomena, often very complicated, by means of a small number of general laws.
Philosophical Essay on Probabilities (1825), trans. Andrew I. Dale (1995), book 1, chap. 14.
The species does not grow in perfection: the weak again and again get the upper hand of the strong,—and their large number and their greater cunning are the cause of it. Darwin forgot the intellect (that was English!); the weak have more intellect. ... One must need intellect in order to acquire it; one loses it when it is no longer necessary.
[Criticism of Darwin’s Origin of Species.]
[Criticism of Darwin’s Origin of Species.]
In The Twilight of the Idols (1886) collected in Thomas Common (trans.), The Works of Nietzsche (1896), Vol. 11, 177. Also see alternate translations.
The starting point of Darwin’s theory of evolution is precisely the existence of those differences between individual members of a race or species which morphologists for the most part rightly neglect. The first condition necessary, in order that any process of Natural Selection may begin among a race, or species, is the existence of differences among its members; and the first step in an enquiry into the possible effect of a selective process upon any character of a race must be an estimate of the frequency with which individuals, exhibiting any given degree of abnormality with respect to that, character, occur. The unit, with which such an enquiry must deal, is not an individual but a race, or a statistically representative sample of a race; and the result must take the form of a numerical statement, showing the relative frequency with which the various kinds of individuals composing the race occur.
Biometrika: A Joumal for the Statistical Study of Biological Problems (1901), 1, 1-2.
The strata of the earth are frequently very much bent, being raised in some places, and depressed in others, and this sometimes with a very quick ascent or descent; but as these ascents and descents, in a great measure, compensate one another, if we take a large extent of country together, we may look upon the whole set of strata, as lying nearly horizontally. What is very remarkable, however, in their situation, is, that from most, if not all, large tracts of high and mountainous countries, the strata lie in a situation more inclined to the horizon, than the country itself, the mountainous countries being generally, if not always, formed out of the lower strata of earth. This situation of the strata may be not unaptly represented in the following manner. Let a number of leaves of paper, of several different sorts or colours, be pasted upon one another; then bending them up together into a ridge in the middle, conceive them to be reduced again to a level surface, by a plane so passing through them, as to cut off all the part that had been raised; let the middle now be again raised a little, and this will be a good general representation of most, if not of all, large tracts of mountainous countries, together with the parts adjacent, throughout the whole world.
'Conjectures Concerning the Cause, and Observations upon the Phenomena of Earthquakes', Philosophical Transactions of the Royal Society of London (1760), 51, 584-5.
The study of the radio-active substances and of the discharge of electricity through gases has supplied very strong experimental evidence in support of the fundamental ideas of the existing atomic theory. It has also indicated that the atom itself is not the smallest unit of matter, but is a complicated structure made up of a number of smaller bodies.
In Radio-activity (1905), 1.
The sun’s rays proceed from the sun along straight lines and are reflected from every polished object at equal angles, i.e. the reflected ray subtends, together with the line tangential to the polished object which is in the plane of the reflected ray, two equal angles. Hence it follows that the ray reflected from the spherical surface, together with the circumference of the circle which is in the plane of the ray, subtends two equal angles. From this it also follows that the reflected ray, together with the diameter of the circle, subtends two equal angles. And every ray which is reflected from a polished object to a point produces a certain heating at that point, so that if numerous rays are collected at one point, the heating at that point is multiplied: and if the number of rays increases, the effect of the heat increases accordingly.
— Alhazan
In H.J.J. Winter, 'A Discourse of the Concave Spherical Mirror by Ibn Al-Haitham', Journal of the Royal Asiatic Society of Bengal, 1950, 16, 2.
The supreme task of the physicist is to arrive at those universal elementary laws from which the cosmos can be built up by pure deduction. There is no logical path to these laws; only intuition, resting on sympathetic understanding of experience, can reach them. In this methodological uncertainty, one might suppose that there were any number of possible systems of theoretical physics all equally well justified; and this opinion is no doubt correct, theoretically. But the development of physics has shown that at any given moment, out of all conceivable constructions, a single one has always proved itself decidedly superior to all the rest.
Address (1918) for Max Planck's 60th birthday, at Physical Society, Berlin, 'Principles of Research' in Essays in Science (1934), 4.
The technologists claim that if everything works [in a nuclear fission reactor] according to their blueprints, fission energy will be a safe and very attractive solution to the energy needs of the world. ... The real issue is whether their blueprints will work in the real world and not only in a “technological paradise.”...
Opponents of fission energy point out a number of differences between the real world and the “technological paradise.” ... No acts of God can be permitted.
Opponents of fission energy point out a number of differences between the real world and the “technological paradise.” ... No acts of God can be permitted.
'Energy and Environment', Bulletin of the Atomic Scientists (May 1972), 6.
The term ‘community’ implies a diversity but at the same time a certain organized uniformity in the units. The units are the many individual plants that occur in every community, whether this be a beech-forest, a meadow, or a heath. Uniformity is established when certain atmospheric, terrestrial, and any of the other factors discussed in Section I are co-operating, and appears either because a certain, defined economy makes its impress on the community as a whole, or because a number of different growth-forms are combined to form a single aggregate which has a definite and constant guise.
Oecology of Plants: An Introduction to the Study of Plant Communities (1909), 91-92.
The theory is confirmed that pea hybrids form egg and pollen cells, which, in their constitution, represent in equal numbers all constant forms which result for the combination of the characters united in fertilization.
As collected in Forest Ray Moulton (ed.), The Autobiography of Science (1945), 586.
The theory of numbers is particularly liable to the accusation that some of its problems are the wrong sort of questions to ask. I do not myself think the danger is serious; either a reasonable amount of concentration leads to new ideas or methods of obvious interest, or else one just leaves the problem alone. “Perfect numbers” certainly never did any good, but then they never did any particular harm.
In A Mathematician’s Miscellany (1953). Reissued as Béla Bollobás (ed.), Littlewood’s Miscellany (1986), 74.
The total number of people who understand relativistic time, even after eighty years since the advent of special relativity, is still much smaller than the number of people who believe in horoscopes.
'Classical to Quantum: A Generalized Phase Transition', in A. van der Merwe et al. (eds.) Microphysical Reality and Quantum Formalism (1987), 145.
The transfinite numbers are in a sense the new irrationalities [ ... they] stand or fall with the finite irrational numbers.
Gesammelte Abhandlungen (1932),395, trans. Ivor Grattan-Guinness.
The tropical rain forests of the world harbor the majority of the planet’s species, yet this wealth of species is being quickly spent. While the exact numbers of species involved and the rate of forest clearing are still under debate, the trend is unmistakable—the richest terrestrial biome is being altered at a scale unparalleled in geologic history.
In The Fragmented Forest: Island Biogeography Theory and the Preservation of Biotic Diversity (1984),
The trouble with the integers is that we have examined only the small ones. Maybe all the exciting stuff happens at really big numbers, ones we can’t get our hands on or even begin to think about in any very definite way. So maybe all the action is really inaccessible and we’re just fiddling around.
As quoted by Paul Hoffman, 'The Man Who Loves Only Numbers', The Atlantic (Nov 1987), 260, No. 5, 74. Also quoted in Clifford Pickover, 'Preface', Wonders of Numbers: Adventures in Mathematics, Mind and Meaning (2000), x.
The varieties of chemical substances actually found in living things are vastly more restricted than the possible varieties. A striking illustration is that if one molecule each of all the possible types of proteins were made, they would together weigh more than the observable universe. Obviously there are a fantastically large number of protein types that are not made by living cells.
In The Closing Circle: Nature, Man, and Technology (2014).
The various elements had different places before they were arranged so as to form the universe. At first, they were all without reason and measure. But when the world began to get into order, fire and water and earth and air had only certain faint traces of themselves, and were altogether such as everything might be expected in the absence of God; this, I say, was their nature at that time, and God fashioned them by form and number.
— Plato
In Plato and B. Jowett (trans.), The Dialogues of Plato: Republic (3rd ed., 1892), Vol. 3, 473.
The weakness of a soul is proportionate to the number of truths that must be kept from it.
In The Passionate State of Mind (1955), 40.
The whole numbers have been made by dear God, everything else is the work of man.
Also often seen as “God made the integers, all else is the work of man.” From the original German, “Die ganzen Zahlen hat der liebe Gott gemacht, alles andere ist Menschenwerk,” in Speech (1886) to the Berliner Naturforscher-Versammlung (Berlin Conference of Natural Scientists). As quoted by H. Weber, in obituary, 'Leopold Kronecker', Jahresbericht der Deutschen Mathematiker-Vereinigung (1893), 2, 19. (Yearbook of the German Mathematics Association.) In a message (26 May 1999) to the 'Historia Mathematica' mailing list, German mathematician Walter Felscher explained that “liebe Gott” (Dear God) is “a colloquial phrase usually used only when speaking to children.” When spoken to an adult audience, it signals that the statement is merely a witticism. Equally, it could have been expressed as “About the integers let us not ask, but all the rest came about by men.” Thus, the statement does not mean that Kronecker believed integers had a divine, pre-human origin. Therefore it should not be regarded as challenging “Dedekind’s viewpoint that also the integers are man-made (i.e. man-invented).”
The world is so full of a number of things,
I’m sure we should all be as happy as kings.
I’m sure we should all be as happy as kings.
In 'Happy Thought', in A Child's Garden of Verses (1885), 28.
Then I had shown, in the same place, what the structure of the nerves and muscles of the human body would have to be in order for the animal spirits in the body to have the power to move its members, as one sees when heads, soon after they have been cut off, still move and bite the ground even though they are no longer alive; what changes must be made in the brain to cause waking, sleep and dreams; how light, sounds, odours, tastes, warmth and all the other qualities of external objects can impress different ideas on it through the senses; how hunger, thirst, and the other internal passions can also send their ideas there; what part of the brain should be taken as “the common sense”, where these ideas are received; what should be taken as the memory, which stores the ideas, and as the imagination, which can vary them in different ways and compose new ones and, by the same means, distribute the animal spirits to the muscles, cause the limbs of the body to move in as many different ways as our own bodies can move without the will directing them, depending on the objects that are present to the senses and the internal passions in the body. This will not seem strange to those who know how many different automata or moving machines can be devised by human ingenuity, by using only very few pieces in comparison with the larger number of bones, muscles, nerves, arteries, veins and all the other parts in the body of every animal. They will think of this body like a machine which, having been made by the hand of God, is incomparably better structured than any machine that could be invented by human beings, and contains many more admirable movements.
Discourse on Method in Discourse on Method and Related Writings (1637), trans. Desmond M. Clarke, Penguin edition (1999), Part 5, 39-40.
There are infinite worlds both like and unlike this world of ours. For the atoms being infinite in number... are borne on far out into space.
— Epicurus
Letter to Herodotus, in Epicurus: The Extant Remains (1926), trans. C. Bailey, 25.
There are many occasions when the muscles that form the lips of the mouth move the lateral muscles that are joined to them, and there are an equal number of occasions when these lateral muscles move the lips of this mouth, replacing it where it cannot return of itself, because the function of muscle is to pull and not to push except in the case of the genitals and the tongue.
'Anatomy', in The Notebooks of Leonardoda Vinci, trans. E. MacCurdy (1938), Vol. 1, 152.
There are then two kinds of intellect: the one able to penetrate acutely and deeply into the conclusions of given premises, and this is the precise intellect; the other able to comprehend a great number of premises without confusing them, and this is the mathematical intellect. The one has force and exactness, the other comprehension. Now the one quality can exist without the other; the intellect can be strong and narrow, and can also be comprehensive and weak.
In Pascal’s Pensées (1958), 3.
There are three distinctions in the kinds of bodies, or three states, which have more especially claimed the attention of philosophical chemists; namely, those which are marked by the terms elastic fluids, liquids, and solids. A very familiar instance is exhibited to us in water, of a body, which, in certain circumstances, is capable of assuming all the three states. In steam we recognise a perfectly elastic fluid, in water, a perfect liquid, and in ice of a complete solid. These observations have tacitly led to the conclusion which seems universally adopted, that all bodies of sensible magnitude, whether liquid or solid, are constituted of a vast number of extremely small particles, or atoms of matter bound together by a force of attraction.
A New System of Chemical Philosophy (1808), Vol. 1, 141.
There are three ruling ideas, three so to say, spheres of thought, which pervade the whole body of mathematical science, to some one or other of which, or to two or all three of them combined, every mathematical truth admits of being referred; these are the three cardinal notions, of Number, Space and Order.
Arithmetic has for its object the properties of number in the abstract. In algebra, viewed as a science of operations, order is the predominating idea. The business of geometry is with the evolution of the properties of space, or of bodies viewed as existing in space.
Arithmetic has for its object the properties of number in the abstract. In algebra, viewed as a science of operations, order is the predominating idea. The business of geometry is with the evolution of the properties of space, or of bodies viewed as existing in space.
In 'A Probationary Lecture on Geometry, York British Association Report (1844), Part 2; Collected Mathematical Papers, Vol. 2, 5.
There are, as we have seen, a number of different modes of technological innovation. Before the seventeenth century inventions (empirical or scientific) were diffused by imitation and adaption while improvement was established by the survival of the fittest. Now, technology has become a complex but consciously directed group of social activities involving a wide range of skills, exemplified by scientific research, managerial expertise, and practical and inventive abilities. The powers of technology appear to be unlimited. If some of the dangers may be great, the potential rewards are greater still. This is not simply a matter of material benefits for, as we have seen, major changes in thought have, in the past, occurred as consequences of technological advances.
Concluding paragraph of "Technology," in Dictionary of the History of Ideas (1973), Vol. 4, 364.
There can never be two or more equivalent electrons in an atom, for which in a strong field the values of all the quantum numbers n, k1, k2 and m are the same. If an electron is present, for which these quantum numbers (in an external field) have definite values, then this state is ‘occupied.’
Quoted by M. Fierz, in article ‘Wolfgang Pauli’, in C. C. Gillispie (ed.), Dictionary of Scientific Biography (1974), Vol. 10, 423.
There is a finite number of species of plants and animals—even of insects—upon the earth. … Moreover, the universality of the genetic code, the common character of proteins in different species, the generality of cellular structure and cellular reproduction, the basic similarity of energy metabolism in all species and of photosynthesis in green plants and bacteria, and the universal evolution of living forms through mutation and natural selection all lead inescapably to a conclusion that, although diversity may be great, the laws of life, based on similarities, are finite in number and comprehensible to us in the main even now.
Presidential Address (28 Dec 1970) to the American Association for the Advancement of Science. 'Science: Endless Horizons or Golden Age?', Science (8 Jan 1971), 171, No. 3866, 24.
There is another approach to the extraterrestrial hypothesis of UFO origins. This assessment depends on a large number of factors about which we know little, and a few about which we know literally nothing. I want to make some crude numerical estimate of the probability that we are frequently visited by extraterrestrial beings.
Now, there is a range of hypotheses that can be examined in such a way. Let me give a simple example: Consider the Santa Claus hypothesis, which maintains that, in a period of eight hours or so on December 24-25 of each year, an outsized elf visits one hundred million homes in the United States. This is an interesting and widely discussed hypothesis. Some strong emotions ride on it, and it is argued that at least it does no harm.
We can do some calculations. Suppose that the elf in question spends one second per house. This isn't quite the usual picture—“Ho, Ho, Ho,” and so on—but imagine that he is terribly efficient and very speedy; that would explain why nobody ever sees him very much-only one second per house, after all. With a hundred million houses he has to spend three years just filling stockings. I have assumed he spends no time at all in going from house to house. Even with relativistic reindeer, the time spent in a hundred million houses is three years and not eight hours. This is an example of hypothesis-testing independent of reindeer propulsion mechanisms or debates on the origins of elves. We examine the hypothesis itself, making very straightforward assumptions, and derive a result inconsistent with the hypothesis by many orders of magnitude. We would then suggest that the hypothesis is untenable.
We can make a similar examination, but with greater uncertainty, of the extraterrestrial hypothesis that holds that a wide range of UFOs viewed on the planet Earth are space vehicles from planets of other stars.
Now, there is a range of hypotheses that can be examined in such a way. Let me give a simple example: Consider the Santa Claus hypothesis, which maintains that, in a period of eight hours or so on December 24-25 of each year, an outsized elf visits one hundred million homes in the United States. This is an interesting and widely discussed hypothesis. Some strong emotions ride on it, and it is argued that at least it does no harm.
We can do some calculations. Suppose that the elf in question spends one second per house. This isn't quite the usual picture—“Ho, Ho, Ho,” and so on—but imagine that he is terribly efficient and very speedy; that would explain why nobody ever sees him very much-only one second per house, after all. With a hundred million houses he has to spend three years just filling stockings. I have assumed he spends no time at all in going from house to house. Even with relativistic reindeer, the time spent in a hundred million houses is three years and not eight hours. This is an example of hypothesis-testing independent of reindeer propulsion mechanisms or debates on the origins of elves. We examine the hypothesis itself, making very straightforward assumptions, and derive a result inconsistent with the hypothesis by many orders of magnitude. We would then suggest that the hypothesis is untenable.
We can make a similar examination, but with greater uncertainty, of the extraterrestrial hypothesis that holds that a wide range of UFOs viewed on the planet Earth are space vehicles from planets of other stars.
The Cosmic Connection: An Extraterrestrial Perspective (1973), 200.
There is more danger of numerical sequences continued indefinitely than of trees growing up to heaven. Each will some time reach its greatest height.
Grundgesetz der Arithmetik(1893), Vol. 2, Section 60, In P. Greach and M. Black (eds., Translations from the Philosophical Writings of Gottlob Frege (1952), 204.
There is no arithmetician like him who hath learned to number his days, and to apply his heart unto wisdom.
In 'The Epistle Dedicatory, The Works of George Swinnock (1868), Vol. 1, 370.
There is no more convincing proof of the truth of a comprehensive theory than its power of absorbing and finding a place for new facts, and its capability of interpreting phenomena which had been previously looked upon as unaccountable anomalies. It is thus that the law of universal gravitation and the undulatory theory of light have become established and universally accepted by men of science. Fact after fact has been brought forward as being apparently inconsistent with them, and one alter another these very facts have been shown to be the consequences of the laws they were at first supposed to disprove. A false theory will never stand this test. Advancing knowledge brings to light whole groups of facts which it cannot deal with, and its advocates steadily decrease in numbers, notwithstanding the ability and scientific skill with which it may have been supported.
From a review of four books on the subject 'Mimicry, and Other Protective Resemblances Among Animals', in The Westminster Review (Jul 1867), 88, 1. Wallace is identified as the author in the article as reprinted in William Beebe, The Book of Naturalists: An Anthology of the Best Natural History (1988), 108.
There is no profession so incompatible with original enquiry as is a Scotch Professorship, where one’s income depends on the numbers of pupils. Is there one Professor in Edinburgh pursuing science with zeal? Are they not all occupied as showmen whose principal object is to attract pupils and make money?
Brewster to J. D. Forbes, 11 February 1830 (St. Andrew's University Library). Quoted in William Cochlan, 'Sir David Brewster: An Outline Biography', in J.R.R. Christie (ed.), Martyr of Science: Sir David Brewster, 1781-1868 (1984), 13.
There is not, we believe, a single example of a medicine having been received permanently into the Materia Medica upon the sole ground of its physical, chemical, or physiological properties. Nearly every one has become a popular remedy before being adopted or even tried by physicians; by far the greater number were first employed in countries which were and are now in a state of scientific ignorance....
Therapeutics and Materia Medica (2006), 31
There is thus a possibility that the ancient dream of philosophers to connect all Nature with the properties of whole numbers will some day be realized. To do so physics will have to develop a long way to establish the details of how the correspondence is to be made. One hint for this development seems pretty obvious, namely, the study of whole numbers in modern mathematics is inextricably bound up with the theory of functions of a complex variable, which theory we have already seen has a good chance of forming the basis of the physics of the future. The working out of this idea would lead to a connection between atomic theory and cosmology.
From Lecture delivered on presentation of the James Scott prize, (6 Feb 1939), 'The Relation Between Mathematics And Physics', printed in Proceedings of the Royal Society of Edinburgh (1938-1939), 59, Part 2, 129.
There may be fairies at the bottom of the garden. There is no evidence of it, but you can’t prove that there aren’t any so shouldn’t we be agnostic with respect to fairies? The trouble with the agnostic argument is that it can be applied to anything. There is an infinite number of hypothetical beliefs we could hold which we can’t positively disprove. On the whole, people don’t believe in most of them, such as fairies, unicorns, dragons, Father Christmas, and so on. But on the whole they do believe in a creator God, together with whatever particular baggage goes with the religion of their parents.
From speech at the Edinburgh International Science Festival (15 Apr 1992), published in the Independent newspaper. Included in excerpt in Alec Fisher, The Logic of Real Arguments (2004), 84. The full speech was reprinted in The Nullifidian, (Dec 1994). Transcribed online in the Richard Dawkins archive, article 89, titled: Lecture from 'The Nullifidian' (Dec 94).
There may only be a small number of laws, which are self-consistent and which lead to complicated beings like ourselves. … And even if there is only one unique set of possible laws, it is only a set of equations. What is it that breathes fire into the equations and makes a universe for them to govern? Is the ultimate unified theory so compelling that it brings about its own existence?
Lecture (1987), 'The Origin of the Universe', collected in Black Holes And Baby Universes And Other Essays (1993), 99.
There was a young fellow from Trinity,
Who took the square root of infinity.
But the number of digits,
Gave him the fidgets;
He dropped Math and took up Divinity.
Who took the square root of infinity.
But the number of digits,
Gave him the fidgets;
He dropped Math and took up Divinity.
Epigraph on title page of One, Two, Three… Infinity: Facts and Speculations of Science (1947, 1988), i. The original text shows symbols instead of the words which appear above as “square root of infinity.”
These duplicates in those parts of the body, without which a man might have very well subsisted, though not so well as with them, are a plain demonstration of an all-wise Contriver, as those more numerous copyings which are found among the vessels of the same body are evident demonstrations that they could not be the work of chance. This argument receives additional strength if we apply it to every animal and insect within our knowledge, as well as to those numberless living creatures that are objects too minute for a human eye: and if we consider how the several species in this whole world of life resemble one another in very many particulars, so far as is convenient for their respective states of existence, it is much more probable that a hundred millions of dice should be casually thrown a hundred millions of times in the same number than that the body of any single animal should be produced by the fortuitous concourse of matter.
In The Spectator (22 Nov 1712), No. 543, as collected in Vol. 4 (1721, 10th ed.), 48.
These works [the creation of the world] are recorded to have been completed in six days … because six is a perfect number … [and] the perfection of the works was signified by the number six.
From De Civitate Dei (early 400s), as translated by Marcus Dods, collected in The Works of Aurelius Augustine (1871), Vol. 1: 'Of the Perfection of the Number Six, which is the First of the Numbers Which is Composed of its Aliquot Parts', The City of God, Book 11, sect. 30, 474. Also seen translated as “Six is a number perfect in itself, and not because God created the world in six days; rather the contrary is true. God created the world in six days because this number is perfect, and it would remain perfect, even if the work of the six days did not exist.” [Note: 6 can be exactly divided (excluding itself) by 1, 2 or 3. It is a perfect number because those possible divisors also add up to a sum of 6.]
They think that differential equations are not reality. Hearing some colleagues speak, it’s as though theoretical physics was just playing house with plastic building blocks. This absurd idea has gained currency, and now people seem to feel that theoretical physicists are little more than dreamers locked away ivory towers. They think our games, our little houses, bear no relation to their everyday worries, their interests, their problems, or their welfare. But I’m going to tell you something, and I want you to take it as a ground rule for this course. From now on I will be filling this board with equations. … And when I'm done, I want you to do the following: look at those numbers, all those little numbers and Greek letters on the board, and repeat to yourselves, “This is reality,” repeat it over and over.
Zig Zag, trans. Lisa Dillman (2008), 63.
Things of all kinds are subject to a universal law which may be called the law of large numbers. It consists in the fact that, if one observes very considerable numbers of events of the same nature, dependent on constant causes and causes which vary irregularly, sometimes in one direction, sometimes in the other, it is to say without their variation being progressive in any definite direction, one shall find, between these numbers, relations which are almost constant.
Poisson’s Law of Large Numbers (16 Nov 1837), in Recherches sur la Probabilités (1837), 7. English version by Webmaster using Google Translate, from the original French, “Les choses de toutes natures sont soumises à une loi universelle qu’on) peut appeler la loi des grands nombres. Elle consiste en ce que, si l’on observe des nombres très considérables d’événements d’une même nature, dépendants de causes constantes et de causes qui varient irrégulièrement, tantôt dans un sens, tantôt daus l’autre, c’est-à-dire sans que leur variation soit progressive dans aucun sens déterminé, on trouvera, entre ces nombres, des rapports a très peu près constants.”
Think of Adam and Eve like an imaginary number, like the square root of minus one: you can never see any concrete proof that it exists, but if you include it in your equations, you can calculate all manner of things that couldn't be imagined without it.
In The Golden Compass (1995, 2001), 372-373.
This formula [for computing Bernoulli’s numbers] was first given by James Bernoulli…. He gave no general demonstration; but was quite aware of the importance of his theorem, for he boasts that by means of it he calculated intra semi-quadrantem horæ! the sum of the 10th powers of the first thousand integers, and found it to be
91,409,924,241,424,243,424,241,924,242,500.
In 'Bernoulli’s Expression for ΣNr', Algebra, Vol. 2 (1879, 1889), 209. The ellipsis is for the reference (Ars Conjectandi (1713), 97). [The Latin phrase, “intra semi-quadrantem horæ!” refers to within a fraction of an hour. —Webmaster]
This interpretation of the atomic number [as the number of orbital electrons] may be said to signify an important step toward the solution of the boldest dreams of natural science, namely to build up an understanding of the regularities of nature upon the consideration of pure number.
Atomic Theory and the Description of Nature (1934), 103-104Cited in Gerald James Holton, Thematic Origins of Scientific Thought: Kepler to Einstein (1985), 74.
This is often the way it is in physics—our mistake is not that we take our theories too seriously, but that we do not take them seriously enough. It is always hard to realize that these numbers and equations we play with at our desks have something to do with the real world.
In The First Three Minutes: A Modern View of the Origin of the Universe (1977, Rev. ed. 1993), 131-132.
This method is, to define as the number of a class the class of all classes similar to the given class. Membership of this class of classes (considered as a predicate) is a common property of all the similar classes and of no others; moreover every class of the set of similar classes has to the set of a relation which it has to nothing else, and which every class has to its own set. Thus the conditions are completely fulfilled by this class of classes, and it has the merit of being determinate when a class is given, and of being different for two classes which are not similar. This, then, is an irreproachable definition of the number of a class in purely logical terms.
The Principles of Mathematics (1903), 115.
This notion that “science” is something that belongs in a separate compartment of its own, apart from everyday life, is one that I should like to challenge. We live in a scientific age; yet we assume that knowledge of science is the prerogative of only a small number of human beings, isolated and priest-like in their laboratories. This is not true. It cannot be true. The materials of science are the materials of life itself. Science is part of the reality of living; it is the what, the how, and the why of everything in our experience. It is impossible to understand man without understanding his environment and the forces that have molded him physically and mentally.
Address upon receiving National Book Award at reception, Hotel Commodore, New York (27 Jan 1952). As cited in Linda Lear, Rachel Carson: Witness for Nature (1997), 218-219.
This relation logical implication is probably the most rigorous and powerful of all the intellectual enterprises of man. From a properly selected set of the vast number of prepositional functions a set can be selected from which an infinitude of prepositional functions can be implied. In this sense all postulational thinking is mathematics. It can be shown that doctrines in the sciences, natural and social, in history, in jurisprudence and in ethics are constructed on the postulational thinking scheme and to that extent are mathematical. Together the proper enterprise of Science and the enterprise of Mathematics embrace the whole knowledge-seeking activity of mankind, whereby “knowledge” is meant the kind of knowledge that admits of being made articulate in the form of propositions.
In Mathematics as a Culture Clue: And Other Essays (1947), 127.
This science, Geometry, is one of indispensable use and constant reference, for every student of the laws of nature; for the relations of space and number are the alphabet in which those laws are written. But besides the interest and importance of this kind which geometry possesses, it has a great and peculiar value for all who wish to understand the foundations of human knowledge, and the methods by which it is acquired. For the student of geometry acquires, with a degree of insight and clearness which the unmathematical reader can but feebly imagine, a conviction that there are necessary truths, many of them of a very complex and striking character; and that a few of the most simple and self-evident truths which it is possible for the mind of man to apprehend, may, by systematic deduction, lead to the most remote and unexpected results.
In The Philosophy of the Inductive Sciences Part 1, Bk. 2, chap. 4, sect. 8 (1868).
This tomb holds Diophantus Ah, what a marvel! And the tomb tells scientifically the measure of his life. God vouchsafed that he should be a boy for the sixth part of his life; when a twelfth was added, his cheeks acquired a beard; He kindled for him the light of marriage after a seventh, and in the fifth year after his marriage He granted him a son. Alas! late-begotten and miserable child, when he had reached the measure of half his father’s life, the chill grave took him. After consoling his grief by this science of numbers for four years, he reached the end of his life.
Epigram-problem for age at his death (84). Original Greek with English translation in The Greek Anthology (1918), Vol. 5, 92-93.
Those skilled in mathematical analysis know that its object is not simply to calculate numbers, but that it is also employed to find the relations between magnitudes which cannot be expressed in numbers and between functions whose law is not capable of algebraic expression.
In Antoine-Augustin Cournot and Nathaniel T. Bacon (trans.), Mathematical Theory of the Principles of Wealth (1897), Preface, 3.
Those who are unacquainted with the details of scientific investigation have no idea of the amount of labour expended in the determination of those numbers on which important calculations or inferences depend. They have no idea of the patience shown by a Berzelius in determining atomic weights; by a Regnault in determining coefficients of expansion; or by a Joule in determining the mechanical equivalent of heat.
In Sound: A Course of Eight Lectures Delivered at the Royal Institution of Great Britain (1867), 26.
Those who think “Science is Measurement” should search Darwin’s works for numbers and equations.
From his autobiographical chapter, 'David H. Hubel', collected in Larry R. Squire (ed.), The History of Neuroscience in Autobiography (1996), Vol. 1, 313.
Though he may not always recognise his bondage, modern man lives under a tyranny of numbers.
The Tyranny of Numbers; Mismeasurement and Misrule (1995), 1.
Though the parallel is not complete, it is safe to say that science will never touch them unaided by its practical applications. Its wonders may be catalogued for purposes of education, they may be illustrated by arresting experiments, by numbers and magnitudes which startle or fatigue the imagination but they will form no familiar portion of the intellectual furniture of ordinary men unless they be connected, however remotely, with the conduct of ordinary life.
Decadence (1908), 53.
Thought-economy is most highly developed in mathematics, that science which has reached the highest formal development, and on which natural science so frequently calls for assistance. Strange as it may seem, the strength of mathematics lies in the avoidance of all unnecessary thoughts, in the utmost economy of thought-operations. The symbols of order, which we call numbers, form already a system of wonderful simplicity and economy. When in the multiplication of a number with several digits we employ the multiplication table and thus make use of previously accomplished results rather than to repeat them each time, when by the use of tables of logarithms we avoid new numerical calculations by replacing them by others long since performed, when we employ determinants instead of carrying through from the beginning the solution of a system of equations, when we decompose new integral expressions into others that are familiar,—we see in all this but a faint reflection of the intellectual activity of a Lagrange or Cauchy, who with the keen discernment of a military commander marshalls a whole troop of completed operations in the execution of a new one.
In Populär-wissenschafliche Vorlesungen (1903), 224-225.
Through [the growing organism's] power of assimilation there is a constant encroachment of the organic upon the inorganic, a constant attempt to convert all available material into living substance, and to indefinitely multiply the total number of individual organisms.
In History of the Human Body (1919), 2.
Through and through the world is infected with quantity: To talk sense is to talk quantities. It is not use saying the nation is large—How large? It is no use saying the radium is scarce—How scarce? You cannot evade quantity. You may fly to poetry and music, and quantity and number will face you in your rhythms and your octaves.
In 'The Aims of Education', The Aims of Education: & Other Essays (1917), 11.
Through the Middle Ages and down to the late eighteenth century, many philosophers, most men of science, and, indeed, most educated men, were to accept without question—the conception of the universe as a Great Chain of Being, composed of an immense, or—by the strict but seldom rigorously applied logic of the principle of continuity—of an infinite number of links ranging in hierarchical order from the meagerest kind of existents, which barely escape non-existence, through 'every possible' grade up to the ens perfectissimum—or, in a somewhat more orthodox version, to the highest possible kind of creature, between which and the Absolute Being the disparity was assumed to be infinite—every one of them differing from that immediately above and that immediately below it by the 'least possible' degree of difference.
The Great Chain of Being (1936), 59.
Thus, remarkably, we do not know the true number of species on earth even to the nearest order of magnitude. My own guess, based on the described fauna and flora and many discussions with entomologists and other specialists, is that the absolute number falls somewhere between five and thirty million.
Conservation for the 21st Century
To a mathematician the eleventh means only a single unit: to the bushman who cannot count further than his ten fingers it is an incalculable myriad.
In 'Maxims for Revolutionists: Greatness', in Man and Superman (1903), 236.
To him who devotes his life to science, nothing can give more happiness than increasing the number of discoveries, but his cup of joy is full when the results of his studies immediately find practical applications.
As quoted in René J. Dubos, Louis Pasteur, Free Lance of Science (1960, 1986), 85.
To illustrate the apparent contrast between statistics and truth … may I quote a remark I once overheard: “There are three kinds of lies: white lies, which are justifiable; common lies—these have no justification; and statistics.” Our meaning is similar when we say: “Anything can be proved by figures”; or, modifying a well-known quotation from Goethe, with numbers “all men may contend their charming systems to defend.”
In Probability, Statistics, and Truth (1939), 1.
To mean understandings, it is sufficient honour to be numbered amongst the lowest labourers of learning; but different abilities must find different tasks. To hew stone, would have been unworthy of Palladio; and to have rambled in search of shells and flowers, had but ill suited with the capacity of Newton.
From 'Numb. 83, Tuesday, January 1, 1750', The Rambler (1756), Vol. 2, 154. (Italian architect Palladio, 1509-80, is widely considered the most influential in the history of Western architecture.)
To my mathematical brain, the numbers alone make thinking about aliens perfectly rational. The real challenge is to work out what aliens might actually be like.
From Discovery TV series, Into the Universe With Stephen Hawking (2010). As quoted on nbcnews.com webpage, 'Hawking: Aliens may pose risks to Earth' (25 Apr 2010). He warned that rather than actively trying to communicate with extra-terrestrials, humans should do everything possible to avoid contact.
To Nature nothing can be added; from Nature nothing can be taken away; the sum of her energies is constant, and the utmost man can do in the pursuit of physical truth, or in the applications of physical knowledge, is to shift the constituents of the never-varying total. The law of conservation rigidly excludes both creation and annihilation. Waves may change to ripples, and ripples to waves; magnitude may be substituted for number, and number for magnitude; asteroids may aggregate to suns, suns may resolve themselves into florae and faunae, and floras and faunas melt in air: the flux of power is eternally the same. It rolls in music through the ages, and all terrestrial energy—the manifestations of life as well as the display of phenomena—are but the modulations of its rhythm.
Conclusion of Heat Considered as a Mode of Motion: Being a Course of Twelve Lectures Delivered at the Royal Institution of Great Britain in the Season of 1862 (1863), 449.
To pick a hole–say in the 2nd law of Ωcs, that if two things are in contact the hotter cannot take heat from the colder without external agency.
Now let A & B be two vessels divided by a diaphragm and let them contain elastic molecules in a state of agitation which strike each other and the sides. Let the number of particles be equal in A & B but let those in A have equal velocities, if oblique collisions occur between them their velocities will become unequal & I have shown that there will be velocities of all magnitudes in A and the same in B only the sum of the squares of the velocities is greater in A than in B.
When a molecule is reflected from the fixed diaphragm CD no work is lost or gained.
If the molecule instead of being reflected were allowed to go through a hole in CD no work would be lost or gained, only its energy would be transferred from the one vessel to the other.
Now conceive a finite being who knows the paths and velocities of all the molecules by simple inspection but who can do no work, except to open and close a hole in the diaphragm, by means of a slide without mass.
Let him first observe the molecules in A and when lie sees one coming the square of whose velocity is less than the mean sq. vel. of the molecules in B let him open a hole & let it go into B. Next let him watch for a molecule in B the square of whose velocity is greater than the mean sq. vel. in A and when it comes to the hole let him draw and slide & let it go into A, keeping the slide shut for all other molecules.
Then the number of molecules in A & B are the same as at first but the energy in A is increased and that in B diminished that is the hot system has got hotter and the cold colder & yet no work has been done, only the intelligence of a very observant and neat fingered being has been employed. Or in short if heat is the motion of finite portions of matter and if we can apply tools to such portions of matter so as to deal with them separately then we can take advantage of the different motion of different portions to restore a uniformly hot system to unequal temperatures or to motions of large masses. Only we can't, not being clever enough.
Now let A & B be two vessels divided by a diaphragm and let them contain elastic molecules in a state of agitation which strike each other and the sides. Let the number of particles be equal in A & B but let those in A have equal velocities, if oblique collisions occur between them their velocities will become unequal & I have shown that there will be velocities of all magnitudes in A and the same in B only the sum of the squares of the velocities is greater in A than in B.
When a molecule is reflected from the fixed diaphragm CD no work is lost or gained.
If the molecule instead of being reflected were allowed to go through a hole in CD no work would be lost or gained, only its energy would be transferred from the one vessel to the other.
Now conceive a finite being who knows the paths and velocities of all the molecules by simple inspection but who can do no work, except to open and close a hole in the diaphragm, by means of a slide without mass.
Let him first observe the molecules in A and when lie sees one coming the square of whose velocity is less than the mean sq. vel. of the molecules in B let him open a hole & let it go into B. Next let him watch for a molecule in B the square of whose velocity is greater than the mean sq. vel. in A and when it comes to the hole let him draw and slide & let it go into A, keeping the slide shut for all other molecules.
Then the number of molecules in A & B are the same as at first but the energy in A is increased and that in B diminished that is the hot system has got hotter and the cold colder & yet no work has been done, only the intelligence of a very observant and neat fingered being has been employed. Or in short if heat is the motion of finite portions of matter and if we can apply tools to such portions of matter so as to deal with them separately then we can take advantage of the different motion of different portions to restore a uniformly hot system to unequal temperatures or to motions of large masses. Only we can't, not being clever enough.
Letter to Peter Guthrie Tait (11 Dec 1867). In P. M. Harman (ed.), The Scientific Letters and Papers of James Clerk Maxwell (1995), Vol. 2, 331-2.
To the Victorian scientist, science was the pursuit of truth about Nature. In imagination, each new truth discovered could be ticked off on a list kept perhaps in a celestial planning office, so reducing by one the total number of truths to be discovered. But the practising scientist now knows that he is dealing with a living, growing thing. His task is never done.
Opening remark in article 'Musical Acoustics Today', New Scientist (1 Nov 1962), 16 No. 311, 256.
To what heights would science now be raised if Archimedes had made that discovery [of decimal number notation]!
As quoted, without citation, in Eric Temple Bell, Men of Mathematics (1937), 256.
To work our railways, even to their present extent, there must be at least 5,000 locomotive engines; and supposing an engine with its tender to measure only 35 feet, it will be seen, that the whole number required to work our railway system would extend, in one straight line, over 30 miles, or the whole distance from London to Chatham.
From 'Railway System and its Results' (Jan 1856) read to the Institution of Civil Engineers, reprinted in Samuel Smiles, Life of George Stephenson (1857), 512.
Tolstoi explains somewhere in his writings why, in his opinion, “Science for Science's sake” is an absurd conception. We cannot know all the facts since they are infinite in number. We must make a selection ... guided by utility ... Have we not some better occupation than counting the number of lady-birds in existence on this planet?
In Science and Method (1914, 2003), 15
Torture numbers, and they will confess to anything.
'Our Warming World', New Republic, 11 November 1999, Vol. 221, 42.
Truly I say to you, a single number has more genuine and permanent value than an expensive library full of hypotheses.
Letter to Griesinger (20 Jul 1844). In Jacob J. Weyrauch (ed.), Kleinere Schriften und Briefe von Robert Milyer, nebst Mittheilungen aus seinem Leben (1893), 226. Trans. Kenneth L. Caneva, Robert Mayer and the Conservation of Energy (1993), 37.
Truth travels down from the heights of philosophy to the humblest walks of life, and up from the simplest perceptions of an awakened intellect to the discoveries which almost change the face of the world. At every stage of its progress it is genial, luminous, creative. When first struck out by some distinguished and fortunate genius, it may address itself only to a few minds of kindred power. It exists then only in the highest forms of science; it corrects former systems, and authorizes new generalizations. Discussion, controversy begins; more truth is elicited, more errors exploded, more doubts cleared up, more phenomena drawn into the circle, unexpected connexions of kindred sciences are traced, and in each step of the progress, the number rapidly grows of those who are prepared to comprehend and carry on some branches of the investigation,— till, in the lapse of time, every order of intellect has been kindled, from that of the sublime discoverer to the practical machinist; and every department of knowledge been enlarged, from the most abstruse and transcendental theory to the daily arts of life.
In An Address Delivered Before the Literary Societies of Amherst College (25 Aug 1835), 16-17.
Truths are immortal, my dear friend; they are immortal like God! What we call a falsity is like a fruit; it has a certain number of days; it is bound to decay. Whereas, what we call truth is like gold; days, months, even centuries can hide gold, can overlook it but they can never make it decay.
From the play Galileo Galilei (2001) .
Two extreme views have always been held as to the use of mathematics. To some, mathematics is only measuring and calculating instruments, and their interest ceases as soon as discussions arise which cannot benefit those who use the instruments for the purposes of application in mechanics, astronomy, physics, statistics, and other sciences. At the other extreme we have those who are animated exclusively by the love of pure science. To them pure mathematics, with the theory of numbers at the head, is the only real and genuine science, and the applications have only an interest in so far as they contain or suggest problems in pure mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
Of the two greatest mathematicians of modern tunes, Newton and Gauss, the former can be considered as a representative of the first, the latter of the second class; neither of them was exclusively so, and Newton’s inventions in the science of pure mathematics were probably equal to Gauss’s work in applied mathematics. Newton’s reluctance to publish the method of fluxions invented and used by him may perhaps be attributed to the fact that he was not satisfied with the logical foundations of the Calculus; and Gauss is known to have abandoned his electro-dynamic speculations, as he could not find a satisfying physical basis. …
Newton’s greatest work, the Principia, laid the foundation of mathematical physics; Gauss’s greatest work, the Disquisitiones Arithmeticae, that of higher arithmetic as distinguished from algebra. Both works, written in the synthetic style of the ancients, are difficult, if not deterrent, in their form, neither of them leading the reader by easy steps to the results. It took twenty or more years before either of these works received due recognition; neither found favour at once before that great tribunal of mathematical thought, the Paris Academy of Sciences. …
The country of Newton is still pre-eminent for its culture of mathematical physics, that of Gauss for the most abstract work in mathematics.
In History of European Thought in the Nineteenth Century (1903), 630.
Tyndall declared that he saw in Matter the promise and potency of all forms of life, and with his Irish graphic lucidity made a picture of a world of magnetic atoms, each atom with a positive and a negative pole, arranging itself by attraction and repulsion in orderly crystalline structure. Such a picture is dangerously fascinating to thinkers oppressed by the bloody disorders of the living world. Craving for purer subjects of thought, they find in the contemplation of crystals and magnets a happiness more dramatic and less childish than the happiness found by mathematicians in abstract numbers, because they see in the crystals beauty and movement without the corrupting appetites of fleshly vitality.
In Back to Methuselah: A Metabiological Pentateuch (1921), lxi-lxii.
Understanding a theory has, indeed, much in common with understanding a human personality. We may know or understand a man's system of dispositions pretty well; that is to say, we may be able to predict how he would act in a number of different situations. But since there are infinitely many possible situations, of infinite variety, a full understanding of a man's dispositions does not seem to be possible.
Objective Knowledge: an Evolutionary Approach (1972), 299.
Undeveloped though the science [of chemistry] is, it already has great power to bring benefits. Those accruing to physical welfare are readily recognized, as in providing cures, improving the materials needed for everyday living, moving to ameliorate the harm which mankind by its sheer numbers does to the environment, to say nothing of that which even today attends industrial development. And as we continue to improve our understanding of the basic science on which applications increasingly depend, material benefits of this and other kinds are secured for the future.
Speech at the Nobel Banquet (10 Dec 1983) for his Nobel Prize in Chemistry. In Wilhelm Odelberg (ed.), Les Prix Nobel: The Nobel Prizes (1984), 43.
Unfortunately, only a small number of patients with peptic ulcer are financially able to make a pet of an ulcer.
Journal of the American Medical Association (1922).
Unless we choose to decentralize and to use applied science, not as the end to which human beings are to be made the means, but as the means to producing a race of free individuals, we have only two alternatives to choose from: either a number of national
Brave New World (1932, 1998), Preface, xvii.
Upon viewing the milt or semen Masculinum of a living Codfish with a Microscope, such Numbers of Animalcules with long Tails were found therein, that at least ten thousand of them were supposed to exist in the quantity of a Grain of Sand.
Verily God is an odd number and loves the odd numbers.
Islamic saying, as quoted in Clifford A. Pickover, The Loom of God: Tapestries of Mathematics and Mysticism (1997, 2009), 42.
Vision, in my view, is the cause of the greatest benefit to us, inasmuch as none of the accounts now given concerning the Universe would ever have been given if men had not seen the stars or the sun or the heavens. But as it is, the vision of day and night and of months and circling years has created the art of number and has given us not only the notion of Time but also means of research into the nature of the Universe. From these we have procured Philosophy in all its range, than which no greater boon ever has come or will come, by divine bestowal, unto the race of mortals.
— Plato
…...
Voice is a flowing breath of air, perceptible to the hearing by contact. It moves in an endless number of circular rounds, like the innumerably increasing circular waves which appear when a stone is thrown into smooth water, and which keep on spreading indefinitely from the centre.
In De Architectura, Book 5, Chap 1, Sec. 6. As translated in Morris Hicky Morgan (trans.), Vitruvius: The Ten Books on Architecture (1914), 138.
We … find a number of quotations illustrating the use of the word [probability], most of them taken from philosophical works. I shall only refer to a few examples: “The probable is something which lies midway between truth and error” (Thomasius, 1688); “An assertion, of which the contrary is not completely self-contradictory or impossible, is called probable” (Reimarus). Kant says: “That which, if it were held as truth, would be more than half certain, is called probable.”
In Probability, Statistics, and Truth (1939), 3.
We are fast approaching a situation in which nobody will believe anything we [physicists] say in any matter that touches upon our self-interest. Nothing we do is likely to arrest our decline in numbers, support or social value.
'Hard Times', Physics Today (Oct 1992), 45, 9.
We can invent as many theories we like, and any one of them can be made to fit the facts. But that theory is always preferred which makes the fewest number of assumptions.
From interview by S.J. Woolf, 'Einstein’s Own Corner of Space’, New York Times (18 Aug 1929), Sunday Magazine, 2.
We have seen so many, and those of his [Leeuwenhoek] most surprising discoveries, so perfectly confirmed by great numbers of the most curious and judicious Observers, that there can surely be no reason to distrust his accuracy in those others which have not yet been so frequently or carefully examined.
In 'Account of Mr. Leeuwenhoek’s Microscopes', Philosophical Transactions (Nov-Dec 1723), No. 380, Sec. 6, 453. At the time of writing, Folkes was the Royal Society vice-president.
We know that nature invariably uses the same materials in its operations. Its ingeniousness is displayed only in the variation of form. Indeed, as if nature had voluntarily confined itself to using only a few basic units, we observe that it generally causes the same elements to reappear, in the same number, in the same circumstances, and in the same relationships to one another. If an organ happens to grow in an unusual manner, it exerts a considerable influence on adjacent parts, which as a result fail to reach their standard degree of development.
'Considérations sur les pieces de la tête osseuse des animaux vertebras, et particulièrement sur celle du crane des oiseaux', Annales du Museum d'Histoire Naturelle, 1807, 10, 343. Trans. J. Mandelbaum. Quoted in Pietro Corsi, The Age of Lamarck (1988), 232.
We know that there is an infinite, and we know not its nature. As we know it to be false that numbers are finite, it is therefore true that there is a numerical infinity. But we know not of what kind; it is untrue that it is even, untrue that it is odd; for the addition of a unit does not change its nature; yet it is a number, and every number is odd or even (this certainly holds of every finite number). Thus we may quite well know that there is a God without knowing what He is.
Pensées (1670), Section 1, aphorism 223. In H. F. Stewart (ed.), Pascal's Pensées (1950), 117.
We know the laws of trial and error, of large numbers and probabilities. We know that these laws are part of the mathematical and mechanical fabric of the universe, and that they are also at play in biological processes. But, in the name of the experimental method and out of our poor knowledge, are we really entitled to claim that everything happens by chance, to the exclusion of all other possibilities?
From Nobel Prize Lecture (Dec 1974), 'The Coming Age of the Cell'. Collected in Jan Lindsten (ed.) Nobel Lectures, Physiology or Medicine 1971-1980 (1992).
We live on an obscure hunk of rock and metal circling a humdrum sun, which is on the outskirts of a perfectly ordinary galaxy comprised of 400 billion other suns, which, in turn, is one of some hundred billion galaxies that make up the universe, which, current thinking suggests, is one of a huge number—perhaps an infinite number—of other closed-off universes. From that perspective, the idea that we’re at the center, that we have some cosmic importance, is ludicrous.
From interview with Linda Obst in her article 'Valentine to Science', in Interview (Feb 1996). Quoted and cited in Tom Head (ed.), Conversations with Carl Sagan (2006), ix, and cited on p.xix.
We must admit with humility that, while number is purely a product of our minds, space has a reality outside our minds, so that we cannot completely prescribe its properties a priori.
Letter to Friedrich Bessel (1830).
We must make the following remark: a proof, that after a certain time t1, the spheres must necessarily be mixed uniformly, whatever may be the initial distribution of states, cannot be given. This is in fact a consequence of probability theory, for any non-uniform distribution of states, no matter how improbable it may be, is still not absolutely impossible. Indeed it is clear that any individual uniform distribution, which might arise after a certain time from some particular initial state, is just as improbable as an individual non-uniform distribution; just as in the game of Lotto, any individual set of five numbers is as improbable as the set 1, 2, 3, 4, 5. It is only because there are many more uniform distributions than non-uniform ones that the distribution of states will become uniform in the course of time. One therefore cannot prove that, whatever may be the positions and velocities of the spheres at the beginning, the distributions must become uniform after a long time; rather one can only prove that infinitely many more initial states will lead to a uniform one after a definite length of time than to a non-uniform one. Loschmidt's theorem tells us only about initial states which actually lead to a very non-uniform distribution of states after a certain time t1; but it does not prove that there are not infinitely many more initial conditions that will lead to a uniform distribution after the same time. On the contrary, it follows from the theorem itself that, since there are infinitely many more uniform distributions, the number of states which lead to uniform distributions after a certain time t1, is much greater than the number that leads to non-uniform ones, and the latter are the ones that must be chosen, according to Loschmidt, in order to obtain a non-uniform distribution at t1.
From 'On the Relation of a General Mechanical Theorem to the Second Law of Thermodynamics' (1877), in Stephen G. Brush (ed.), Selected Readings in Physics (1966), Vol. 2, Irreversible Processes, 191-2.
We need a number of solutions - we need more efficiency and conservation. Efficiency is a big one. I think car companies need to do a lot better in producing more efficient cars. They have the technology, we just need to demand them as consumers.
…...
We regard as 'scientific' a method based on deep analysis of facts, theories, and views, presupposing unprejudiced, unfearing open discussion and conclusions. The complexity and diversity of all the phenomena of modern life, the great possibilities and dangers linked with the scientific-technical revolution and with a number of social tendencies demand precisely such an approach, as has been acknowledged in a number of official statements.
Progress, Coexistence and Intellectual Freedom (1968), 25.
We should admit in theory what is already very largely a case in practice, that the main currency of scientific information is the secondary sources in the forms of abstracts, reports, tables, &c., and that the primary sources are only for detailed reference by very few people. It is possible that the fate of most scientific papers will be not to be read by anyone who uses them, but with luck they will furnish an item, a number, some facts or data to such reports which may, but usually will not, lead to the original paper being consulted. This is very sad but it is the inevitable consequence of the growth of science. The number of papers that can be consulted is absolutely limited, no more time can be spent in looking up papers, by and large, than in the past. As the number of papers increase the chance of any one paper being looked at is correspondingly diminished. This of course is only an average, some papers may be looked at by thousands of people and may become a regular and fixed part of science but most will perish unseen.
'The Supply of Information to the Scientist: Some Problems of the Present Day', The Journal of Documentation, 1957, 13, 195.
We suppose ... that the constituent molecules of any simple gas whatever (i.e., the molecules which are at such a distance from each other that they cannot exercise their mutual action) are not formed of a solitary elementary molecule, but are made up of a certain number of these molecules united by attraction to form a single one.
'Essay on a Manner of Determining the Relative Masses of the Elementary Molecules of Bodies, and the Proportions in which they enter into these Compounds', Journal de Physique, 1811, 73, 58-76. In Foundations of the Molecular Theory: Alembic Club Reprints, Number 4 (1923), 31.
What about the magical number seven? What about the seven wonders of the world, the seven seas, the seven deadly sins, the seven daughters of Atlas in the Pleiades, the seven ages of man, the seven levels of hell, the seven primary colors, the seven notes of the musical scale, and the seven days of the week? What about the seven-point rating scale, the seven categories for absolute judgment, the seven objects in the span of attention, and the seven digits in the span of immediate memory? For the present I propose to withhold judgment. Perhaps there is something deep and profound behind all these sevens, something just calling out for us to discover it. But I suspect that it is only a pernicious, Pythagorean coincidence.
The Magical Number Seven, Plus or Minus Two (1956), 42-3.
What else can the human mind hold besides numbers and magnitudes? These alone we apprehend correctly, and if piety permits to say so, our comprehension is in this case of the same kind as God’s, at least insofar as we are able to understand it in this mortal life.
As quoted in Epilogue, The Sleepwalkers: A History of Man’s Changing Vision of the Universe (1959), 524.
What good your beautiful proof on [the transcendence of] π? Why investigate such problems, given that irrational numbers do not even exist?
What I especially admire about you [Arnold Sommerfeld] is the way, at a stamp of your foot, a great number of talented young theorists spring up out of the ground.
As quoted in Paul Forman and Armin Hermann, 'Sommerfeld, Arnold (Johannes Wilhelm)', Biography in Dictionary of Scientific Biography (1975), Vol. 12, 529. Cited from Armin Herman (ed.), Albert Einstein/Arnold Sommerfeld. Briefwechsel: Sechzig Briefe aus dem goldenen Zeitalter der modernen Physik (1968, German), 98.
What is mathematics? What is it for? What are mathematicians doing nowadays? Wasn't it all finished long ago? How many new numbers can you invent anyway? Is today’s mathematics just a matter of huge calculations, with the mathematician as a kind of zookeeper, making sure the precious computers are fed and watered? If it’s not, what is it other than the incomprehensible outpourings of superpowered brainboxes with their heads in the clouds and their feet dangling from the lofty balconies of their ivory towers?
Mathematics is all of these, and none. Mostly, it’s just different. It’s not what you expect it to be, you turn your back for a moment and it's changed. It's certainly not just a fixed body of knowledge, its growth is not confined to inventing new numbers, and its hidden tendrils pervade every aspect of modern life.
Mathematics is all of these, and none. Mostly, it’s just different. It’s not what you expect it to be, you turn your back for a moment and it's changed. It's certainly not just a fixed body of knowledge, its growth is not confined to inventing new numbers, and its hidden tendrils pervade every aspect of modern life.
Opening paragraphs of 'Preface', From Here to Infinity (1996), vii.
What we call man is a mechanism made up of … uncrystallized matter … all the colloid matter of his mechanism is concentrated in a countless number of small cells. … [T]hese cells [are] dwelling places, communes, a walled town within which are many citizens. ... [T]hese are the units of life and when they pass out into space man as we think we know him is dead, a mere machine from which the crew have left,so to speak. ... [T]hese units are endowed with great intelligence. They have memories, they must be divided into countless thousands of groups, most are workers, there are directing groups. Some are chemists, they manufacture the most complicated chemicals that are secreted by the glands.
Diary and Sundry Observations of Thomas Alva Edison (1948), 203-44. In Mark Seltzer, Serial Killers (1998), 215-6.
What, in fact, is mathematical discovery? It does not consist in making new combinations with mathematical entities that are already known. That can be done by anyone, and the combinations that could be so formed would be infinite in number, and the greater part of them would be absolutely devoid of interest. Discovery consists precisely in not constructing useless combinations, but in constructing those that are useful, which are an infinitely small minority. Discovery is discernment, selection.
In Science et Méthode (1920), 48, as translated by Francis Maitland, in Science and Method (1908, 1952), 50-51. Also seen elsewhere translated with “invention” in place of “discovery”.
Whatever opinions we may adopt as to the physical constitution of comets, we must admit that they serve some grand and important purpose in the economy of the universe; for we cannot suppose that the Almighty has created such an immense number of bodies, and set them in rapid motion according to established laws, without an end worthy of his perfections, and, on the whole, beneficial to the inhabitants of the system through which they move.
In The Sidereal Heavens and Other Subjects Connected with Astronomy: As Illustrative of the Character of the Deity, and of an Infinity of Worlds (1871), 353.
When … a large number of renegade specialists and amateurs believe contrary to the most prestigious experts, the latter say, well science is not democratic, it is what the people who know the most say—that is what counts!
In Seeing Red: Redshifts, Cosmology and Academic Science (1998), 273.
When an element A has an affinity for another substance B, I see no mechanical reason why it should not take as many atoms of B as are presented to it, and can possibly come into contact with it (which may probably be 12 in general), except so far as the repulsion of the atoms of B among themselves are more than a match for the attraction of an atom of A. Now this repulsion begins with 2 atoms of B to 1 atom of A, in which case the 2 atoms of B are diametrically opposed; it increases with 3 atoms of B to 1 of A, in which case the atoms are only 120° asunder; with 4 atoms of B it is still greater as the distance is then only 90; and so on in proportion to the number of atoms. It is evident from these positions, that, as far as powers of attraction and repulsion are concerned (and we know of no other in chemistry), binary compounds must first be formed in the ordinary course of things, then ternary and so on, till the repulsion of the atoms of B (or A, whichever happens to be on the surface of the other), refuse to admit any more.
Observations on Dr. Bostock's Review of the Atomic Principles of Chemistry', Nicholson's Journal, 1811, 29, 147.
When an observation is made on any atomic system that has been prepared in a given way and is thus in a given state, the result will not in general be determinate, i.e. if the experiment is repeated several times under identical conditions several different results may be obtained. If the experiment is repeated a large number of times it will be found that each particular result will be obtained a definite fraction of the total number of times, so that one can say there is a definite probability of its being obtained any time that the experiment is performed. This probability the theory enables one to calculate. (1930)
The Principles of Quantum Mechanics 4th ed. (1981), 13-14
When cowardice becomes a fashion its adherents are without number, and it masquerades as forbearance, reasonableness and whatnot.
In 'Thoughts on the Present', First Things, Last Things (1971), 109.
When first I applied my mind to Mathematics I read straight away most of what is usually given by the mathematical writers, and I paid special attention to Arithmetic and Geometry because they were said to be the simplest and so to speak the way to all the rest. But in neither case did I then meet with authors who fully satisfied me. I did indeed learn in their works many propositions about numbers which I found on calculation to be true. As to figures, they in a sense exhibited to my eyes a great number of truths and drew conclusions from certain consequences. But they did not seem to make it sufficiently plain to the mind itself why these things are so, and how they discovered them. Consequently I was not surprised that many people, even of talent and scholarship, should, after glancing at these sciences, have either given them up as being empty and childish or, taking them to be very difficult and intricate, been deterred at the very outset from learning them. … But when I afterwards bethought myself how it could be that the earliest pioneers of Philosophy in bygone ages refused to admit to the study of wisdom any one who was not versed in Mathematics … I was confirmed in my suspicion that they had knowledge of a species of Mathematics very different from that which passes current in our time.
In Elizabeth S. Haldane (trans.) and G.R.T. Ross (trans.), 'Rules for the Direction of the Mind', The Philosophical Works of Descartes (1911, 1973), Vol. 1, Rule 4, 11.
When I was younger, Statistics was the science of large numbers. Now, it seems to me rapidly to be becoming the science of no numbers at all.
In Facts From Figures (1951), Chap. 7, 82. Webmaster has not, as yet, identified any earlier primary source. Can you help?
When one ponders on the tremendous journey of evolution over the past three billion years or so, the prodigious wealth of structures it has engendered, and the extraordinarily effective teleonomic performances of living beings from bacteria to man, one may well find oneself beginning to doubt again whether all this could conceiveably be the product of an enormous lottery presided over by natural selection, blindly picking the rare winners from among numbers drawn at random. [Nevertheless,] a detailed review of the accumulated modern evidence [shows] that this conception alone is compatible with the facts.
In Jacques Monod and Austryn Wainhouse (trans.), Chance and Necessity: An Essay on the Natural Philosophy of Modern Biology (1971), 138.
When Ramanujan was sixteen, he happened upon a copy of Carr’s Synopsis of Mathematics. This chance encounter secured immortality for the book, for it was this book that suddenly woke Ramanujan into full mathematical activity and supplied him essentially with his complete mathematical equipment in analysis and number theory. The book also gave Ramanujan his general direction as a dealer in formulas, and it furnished Ramanujan the germs of many of his deepest developments.
In Mathematical Circles Squared (1972), 158. George Shoobridge Carr (1837-1914) wrote his Synopsis of Elementary Results in Mathematics in 1886.
When the aggregate amount of solid matter transported by rivers in a given number of centuries from a large continent, shall be reduced to arithmetical computation, the result will appear most astonishing to those...not in the habit of reflecting how many of the mightiest of operations in nature are effected insensibly, without noise or disorder.
Principles of Geology (1837), Vol. 1, 230.
When the formulae of inorganic chemical compounds are considered, even a superficial observer is struck with the general symmetry of their construction; the compounds of nitrogen, phosphorus, antimony and arsenic especially exhibit the tendency of the elements to form compounds containing 3 or 5 equivs. of other elements, and it is in these proportions that their affinities are best satisfied; thus in the ternal group we have NO3, NH3, NI3, NS3, PO3, PH3, PCl3, SbO3, SbH3, SbCl3, AsO3, AsH3, AsCl3 &c; and in the five-atom group NO4, NH4O, NH4I, PO5, PH4I, &c. Without offering any hypothesis regarding the cause of this symmetrical grouping of atoms, it is sufficiently evident, from the examples just given, that such a tendency or law prevails, and that, no matter what the character of the uniting atoms may be, the combining power of the attracting element, if I may be allowed the term, is always satisfied by the same number of these atoms.
'On a New Series of Organic Bodies Containing Metals', Philosophical Transactions of the Royal Society of London, 1852, 14:2, 440.
When the number of factors coming into play in a phenomenological complex is too large, scientific method in most cases fails us. One need only think of the weather, in which case prediction even for a few days ahead is impossible. Nevertheless no one doubts that we are confronted with a causal connection whose causal components are in the main known to us.
Out of My Later Years (1995), 28.
When the state is shaken to its foundations by internal or external events, when commerce, industry and all trades shall be at a stand, and perhaps on the brink of ruin; when the property and fortune of all are shaken or changed, and the inhabitants of towns look forward with dread and apprehension to the future, then the agriculturalist holds in his hand the key to the money chest of the rich, and the savings-box of the poor; for political events have not the slightest influence on the natural law, which forces man to take into his system, daily, a certain number of ounces of carbon and nitrogen.
Reflecting on events of 1848.
Reflecting on events of 1848.
Familiar Letters on Chemistry (1851), 3rd edn., 483.
When... the biologist is confronted with the fact that in the organism the parts are so adapted to each other as to give rise to a harmonious whole; and that the organisms are endowed with structures and instincts calculated to prolong their life and perpetuate their race, doubts as to the adequacy of a purely physiochemical viewpoint in biology may arise. The difficulties besetting the biologist in this problem have been rather increased than diminished by the discovery of Mendelian heredity, according to which each character is transmitted independently of any other character. Since the number of Mendelian characters in each organism is large, the possibility must be faced that the organism is merely a mosaic of independent hereditary characters. If this be the case the question arises: What moulds these independent characters into a harmonious whole? The vitalist settles this question by assuming the existence of a pre-established design for each organism and of a guiding 'force' or 'principle' which directs the working out of this design. Such assumptions remove the problem of accounting for the harmonious character of the organism from the field of physics or chemistry. The theory of natural selection invokes neither design nor purpose, but it is incomplete since it disregards the physiochemical constitution of living matter about which little was known until recently.
The Organism as a Whole: From a Physiochemical Viewpoint (1916), v-vi.
Whenever a man can get hold of numbers, they are invaluable: if correct, they assist in informing his own mind, but they are still more useful in deluding the minds of others. Numbers are the masters of the weak, but the slaves of the strong.
Passages From the Life of a Philosopher (1864), 410.
Where we reach the sphere of mathematics we are among processes which seem to some the most inhuman of all human activities and the most remote from poetry. Yet it is just here that the artist has the fullest scope for his imagination. … We are in the imaginative sphere of art, and the mathematician is engaged in a work of creation which resembles music in its orderliness, … It is not surprising that the greatest mathematicians have again and again appealed to the arts in order to find some analogy to their own work. They have indeed found it in the most varied arts, in poetry, in painting, and in sculpture, although it would certainly seem that it is in music, the most abstract of all the arts, the art of number and time, that we find the closest analogy.
In The Dance of Life (1923), 138-139.
Wherefore also these Kinds [elements] occupied different places even before the universe was organised and generated out of them. Before that time, in truth, all these were in a state devoid of reason or measure, but when the work of setting in order this Universe was being undertaken, fire and water and earth and air, although possessing some traces of their known nature, were yet disposed as everything is likely to be in the absence of God; and inasmuch as this was then their natural condition, God began by first marking them out into shapes by means of forms and numbers.
— Plato
Timaeus 53ab, trans. R. G. Bury, in Plato: Timaeus, Critias, Cleitophon, Menexenus, Epistles (1929), 125-7.
Wherever it was, I did not come to know it through the bodily senses; the only things we know through the bodily senses are material objects, which we have found are not truly and simply one. Moreover, if we do not perceive one by the bodily sense, then we do not perceive any number by that sense, at least of those numbers that we grasp by understanding.
De Ubero Arbitrio (On Free Choice of the Will) [386], trans. T. Williams (1993), 45.
Wherever there is number, there is beauty.
— Proclus
Quoted in Morris Kline, Mathematical Thought from Ancient to Modern Times (1990), Vol. 1, 131.
Whether moral and social phenomena are really exceptions to the general certainty and uniformity of the course of nature; and how far the methods, by which so many of the laws of the physical world have been numbered among truths irrevocably acquired and universally assented to, can be made instrumental to the gradual formation of a similar body of received doctrine in moral and political science.
A System of Logic, Ratiocinative and Inductive (1858), v.
Whether we like it or not, quantification in history is here to stay for reasons which the quantifiers themselves might not actively approve. We are becoming a numerate society: almost instinctively there seems now to be a greater degree of truth in evidence expressed numerically than in any literary evidence, no matter how shaky the statistical evidence, or acute the observing eye.
Is History Sick? (1973), 64.
While seeing any number of black crows does not prove all the crows are black, seeing one white crow disproves it. Thus science proceeds not by proving models correct but by discarding false ones or improving incomplete ones.
In 'On the Nature of Science', Physics in Canada (Jan/Feb 2007), 63, No. 1, 7.
While working with staphylococcus variants a number of culture-plates were set aside on the laboratory bench and examined from time to time. In the examinations these plates were necessarily exposed to the air and they became contaminated with various micro-organisms. It was noticed that around a large colony of a contaminating mould the staphylococcus colonies became transparent and were obviously undergoing lysis. Subcultures of this mould were made and experiments conducted with a view to ascertaining something of the properties of the bacteriolytic substance which had evidently been formed in the mould culture and which had diffused into the surrounding medium. It was found that broth in which the mould had been grown at room temperature for one or two weeks had acquired marked inhibitory, bacteriocidal and bacteriolytic properties to many of the more common pathogenic bacteria.
'On the Antibacterial Action of Cultures of a Penicillium, with Special Reference to their Use in the Isolation of B. Influenzae', British Journal of Experimental Pathology, 1929, 10, 226.
While, on the one hand, the end of scientific investigation is the discovery of laws, on the other, science will have reached its highest goal when it shall have reduced ultimate laws to one or two, the necessity of which lies outside the sphere of our cognition. These ultimate laws—in the domain of physical science at least—will be the dynamical laws of the relations of matter to number, space, and time. The ultimate data will be number, matter, space, and time themselves. When these relations shall be known, all physical phenomena will be a branch of pure mathematics.
'Address to the section of Mathematical and Physical Science', Reports of the British Association for the Advancement of Science (1895), 595.
Who thinks all Science, as all Virtue, vain;
Who counts Geometry and numbers Toys…
Who counts Geometry and numbers Toys…
In 'The First Satire of Persius', The Satires of D. J. Juvenalis, Translated Into English Verse, By Mr. Dryden. (1702), 355.
Why can the chemist not take the requisite numbers of atoms and simply put them together? The answer is that the chemist never has atoms at his disposal, and if he had, the direct combination of the appropriate numbers of atoms would lead only to a Brobdingnagian potpourri of different kinds of molecules, having a vast array of different structures. What the chemist has at hand always consists of substances, themselves made up of molecules, containing defined numbers of atoms in ordered arrangements. Consequently, in order to synthesize anyone substance, his task is that of combining, modifying, transforming, and tailoring known substances, until the total effect of his manipulations is the conversion of one or more forms of matter into another.
In 'Art and Science in the Synthesis of Organic Compounds: Retrospect and Prospect', in Maeve O'Connor (ed.), Pointers and Pathways in Research (1963), 28.
Winwood Reade … remarks that while a man is an insoluble puzzle, in the aggregate he becomes a mathematical certainty. You can, for example, never foretell what any one man will do, but you can say with precision what an average number will be up to. Individuals vary, but percentages remain constant. So says the statistician.
Character Sherlock Holmes recommends Winwood Reade’s book The Martyrdom of Man to Dr. Watson in The Sign of the Four (1890), 196. Earlier in the novel, Holmes calls Reade’s book “one of the most remarkable ever penned.” Reade is a real person and his book was published in 1872. The actual statement in it reads: “As a single atom man is an enigma: as a whole he is a mathematical problem.”
With equal passion I have sought knowledge. I have wished to understand the hearts of men. I have wished to know why the stars shine. And I have tried to apprehend the Pythagorean power by which numbers holds sway above the flux. A little of this, but not much, I have achieved.
In 'Prologue: What I Have Lived For', The Autobiography of Bertrand Russell (1969). 3-4.
Within the nucleus [of a cell] is a network of fibers, a sap fills the interstices of the network. The network resolves itself into a definite number of threads at each division of the cell. These threads we call chromosomes. Each species of animals and plants possesses a characteristic number of these threads which have definite size and sometimes a specific shape and even characteristic granules at different levels. Beyond this point our strongest microscopes fail to penetrate.
In A Critique of the Theory of Evolution (1916), 91.
Without preparing fluorine, without being able to separate it from the substances with which it is united, chemistry has been able to study and to analyze a great number of its compounds. The body was not isolated, and yet its place was marked in our classifications. This well demonstrates the usefulness of a scientific theory, a theory which is regarded as true during a certain time, which correlates facts and leads the mind to new hypotheses, the first causes of experimentation; which, little by little, destroy the theory itself, in order to replace it by another more in harmony with the progress of science.
[Describing the known history of fluorine compounds before his isolation of the element.]
[Describing the known history of fluorine compounds before his isolation of the element.]
'Fluorine', lecture at the Royal Institution (28 May 1897), translated from the French, in Proceedings of the Royal Institution (1897). In Annual Report of the Board of Regents of the Smithsonian Institution to July 1897 (1898), 262.
Xenophanes of Kolophon ... says that ... [t]he sun is formed each day from small fiery particles which are gathered together: the earth is infinite, and is not surrounded by air or by sky; an infinite number of suns and moons exist, and all things come from earth. The sea, he said, is salt because so many things flow together and become mixed in it...
Doxographists, Epiph. adv. Haer. iii. 9; Dox. 590. Quoted in Arthur Fairbanks (ed. And trans.), The First Philosophers of Greece (1898), 83.
You can’t see oxygen being generated by trees, carbon dioxide being taken up by trees, but we get that. We’re beginning to understand the importance of forests. But the ocean has its forests, too. They just happen to be very small. They’re very small in size but they’re very large in numbers.
In interview with Pierce Nahigyan, 'Dr. Sylvia Earle: “We’re Literally Destroying The Systems That Keep Us Alive”', Huffington Post (6 Jan 2016).
You cannot become a nuclear physicist capable of real work in the field merely by studying alone in a library, any more than you can become a Jesuit without a certain number of years spent in company with Jesuit scholars. This, and the fact that scientists are among the most international-minded of men, may well be the most important factor in our survival.
As quoted in Michael Amrine, 'I’m A Frightened Man', Collier’s (1946), 117, 51.
You know the formula m over naught equals infinity, m being any positive number? [m/0 = ∞]. Well, why not reduce the equation to a simpler form by multiplying both sides by naught? In which case you have m equals infinity times naught [m = ∞ × 0]. That is to say, a positive number is the product of zero and infinity. Doesn't that demonstrate the creation of the Universe by an infinite power out of nothing? Doesn't it?
In Point Counter Point (1928), 162.
You must remember that nothing happens quite by chance. It’s a question of accretion of information and experience … it’s just chance that I happened to be here at this particular time when there was available and at my disposal the great experience of all the investigators who plodded along for a number of years.
on his discovery of the polio vaccine, in Breakthrough: The Saga of Jonas Salk by Richard Carter (1965).
You propound a complicated arithmetical problem: say cubing a number containing four digits. Give me a slate and half an hour’s time, and I can produce a wrong answer.
Cashel Byron's Profession (1886, 1901), xxiii.
You would be surprised at the number of academics who say things like ‘I didn’t realise what a sponge was until I saw a programme of yours’.
Interview with David Barrett, 'Attenborough: Children Don’t Know Enough About Nature', Daily Telegraph (17 Apr 2011).
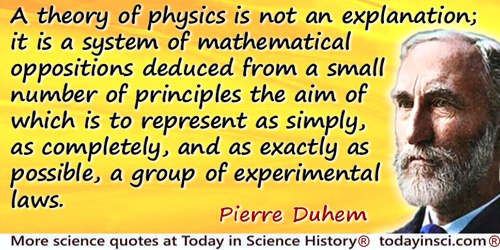












![Carl Friedrich Gauss quote: Mathematics is the queen of the sciences and arithmetic [number theory] is the queen of mathematics.](https://todayinsci.com/G/Gauss_Carl/GaussCarl-Queen500x250px.jpg)












 In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) --
In science it often happens that scientists say, 'You know that's a really good argument; my position is mistaken,' and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn't happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.
(1987) -- 


